Geodetic Model of the March 2021 Thessaly Seismic Sequence Inferred from Seismological and InSAR Data
Abstract
:1. Introduction
2. Exploited Data
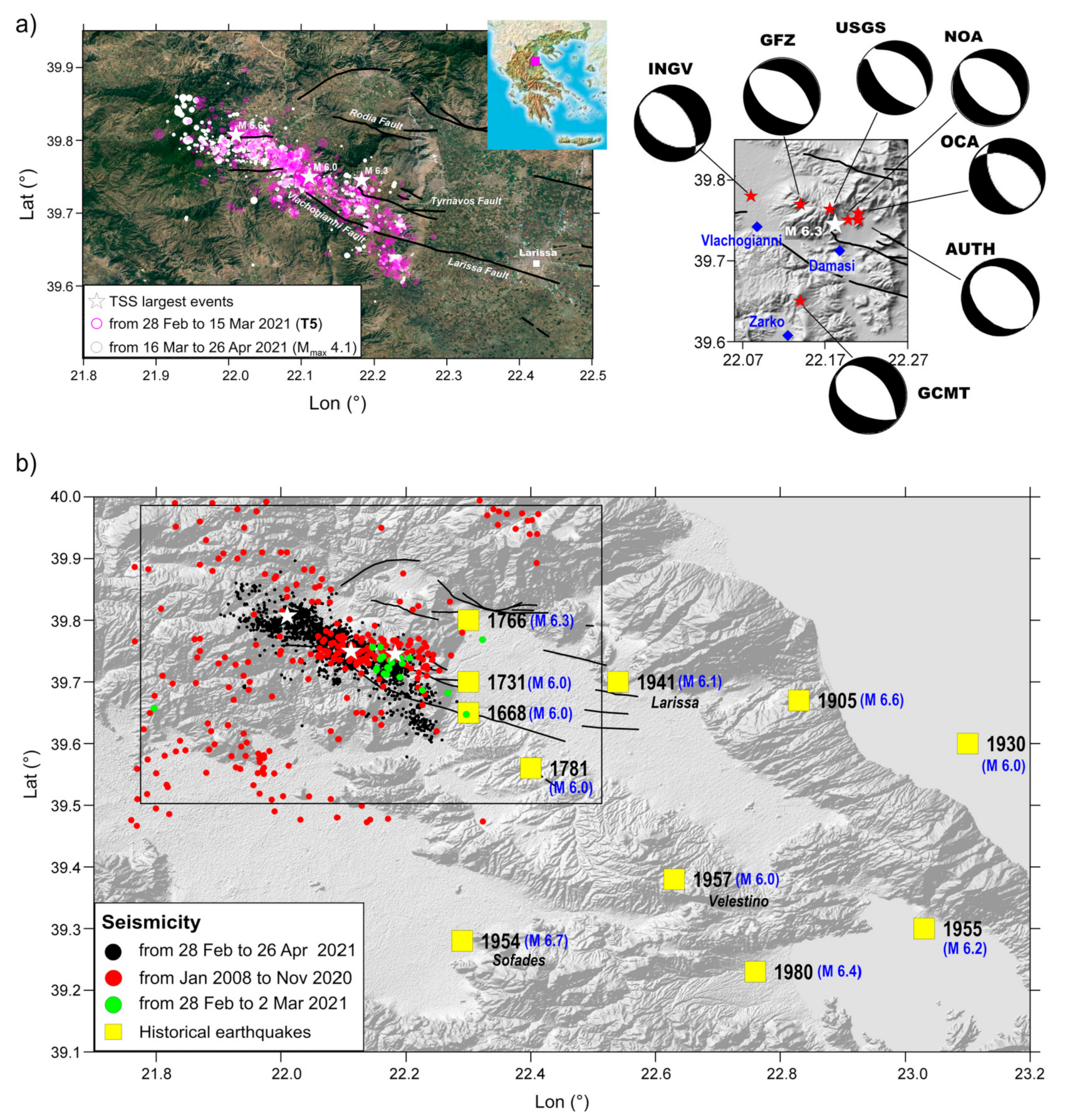
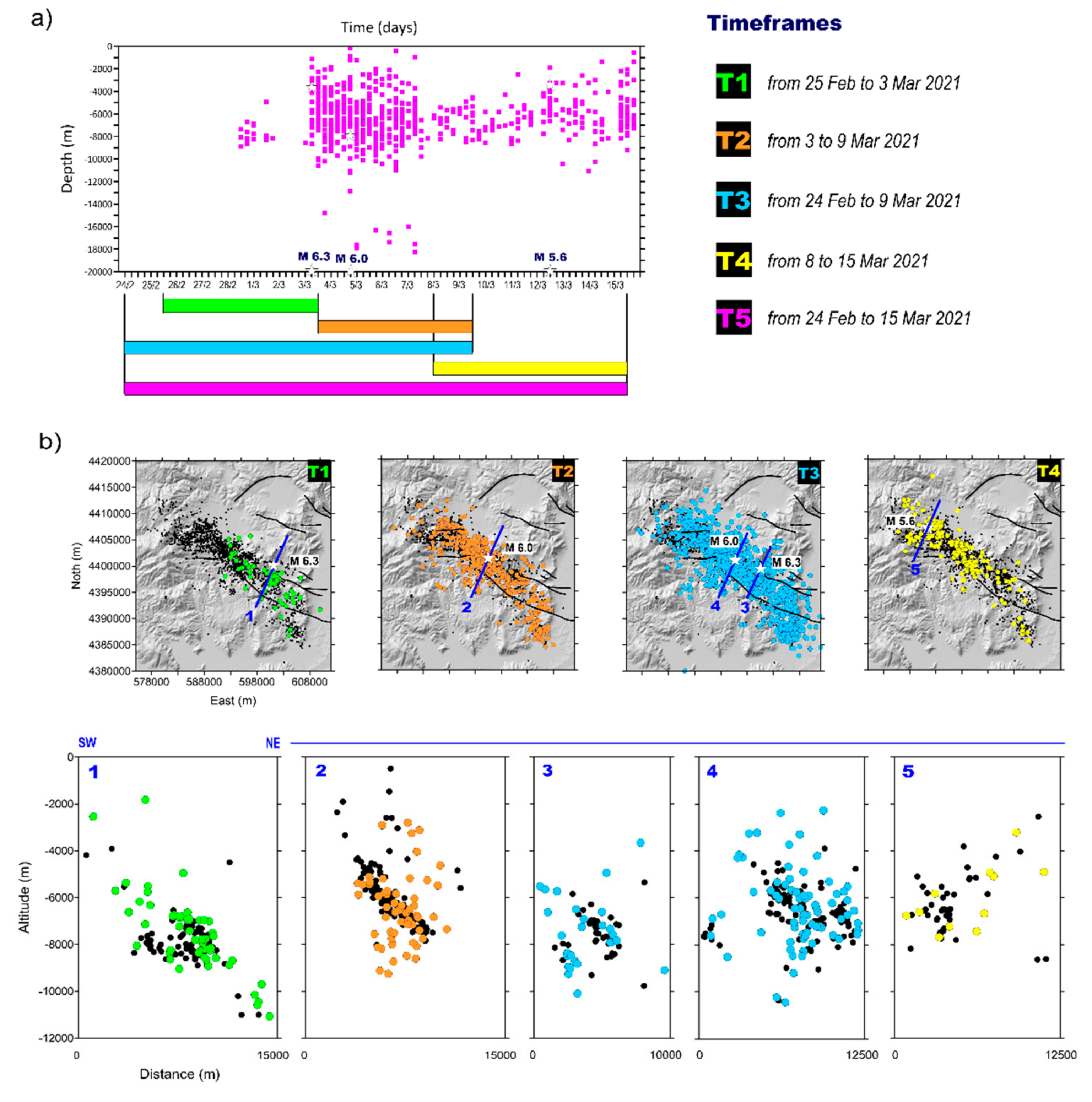
2.1. Seismological Data
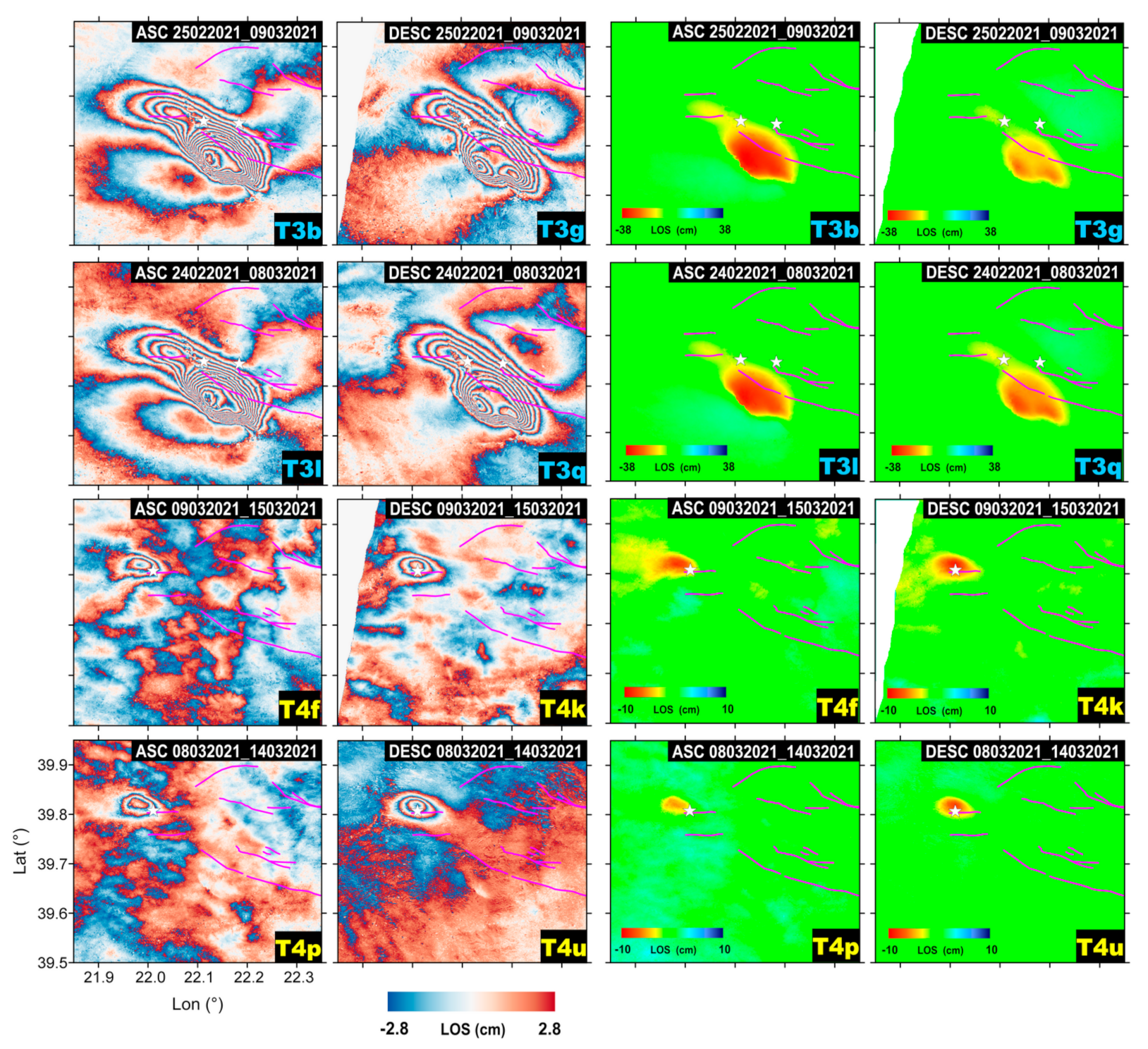
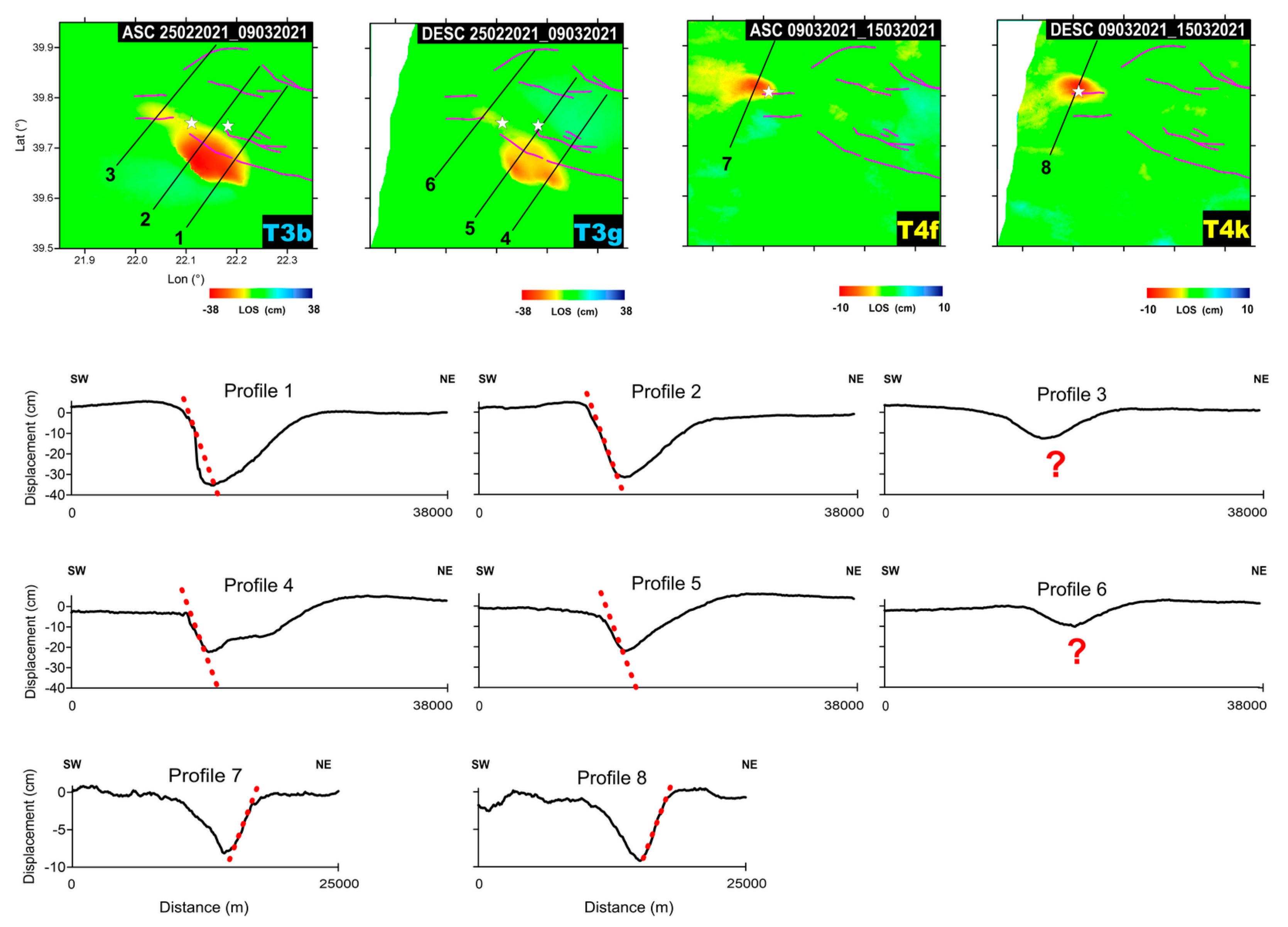
2.2. InSAR Measurements
3. Modeling and Results
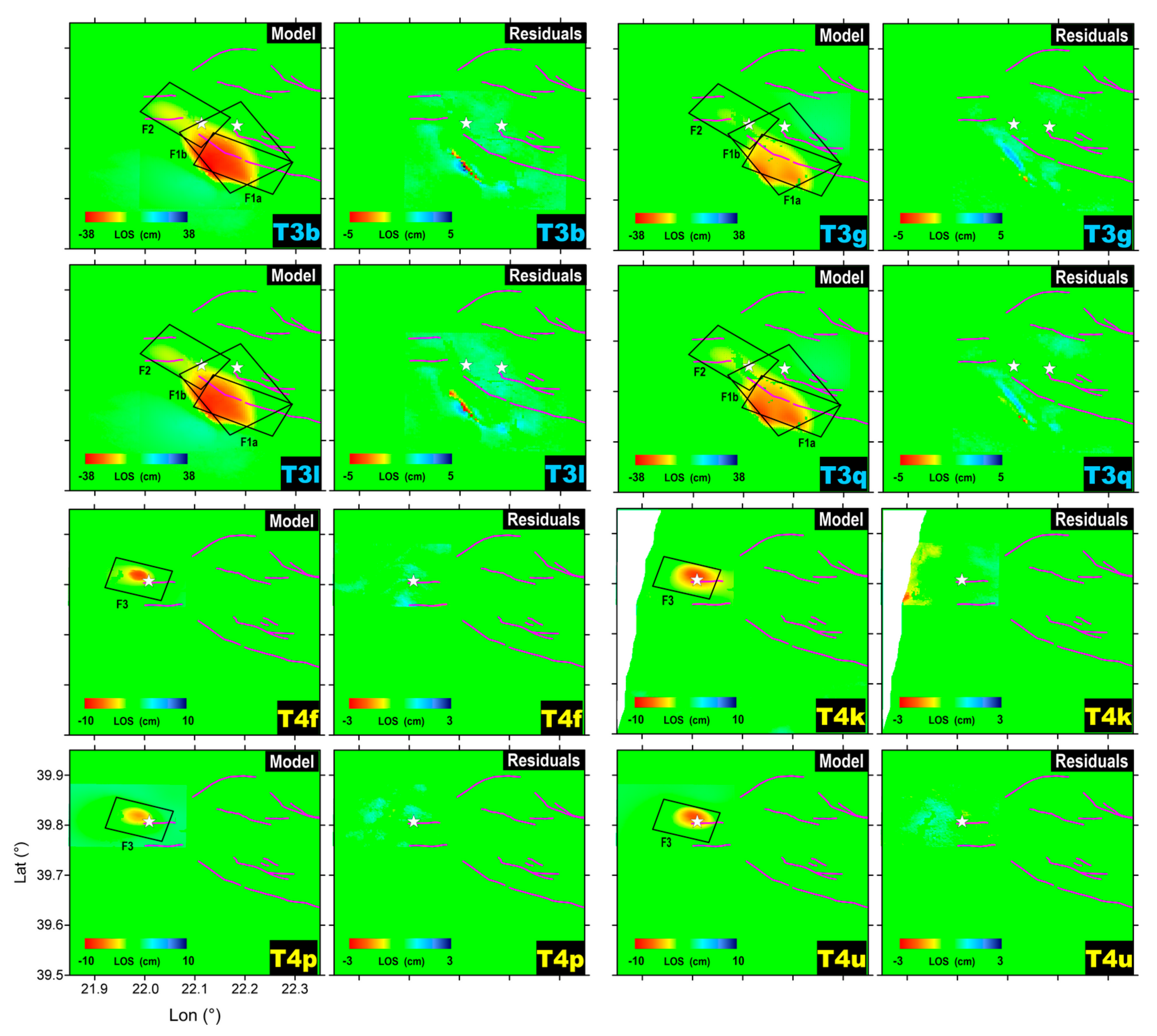
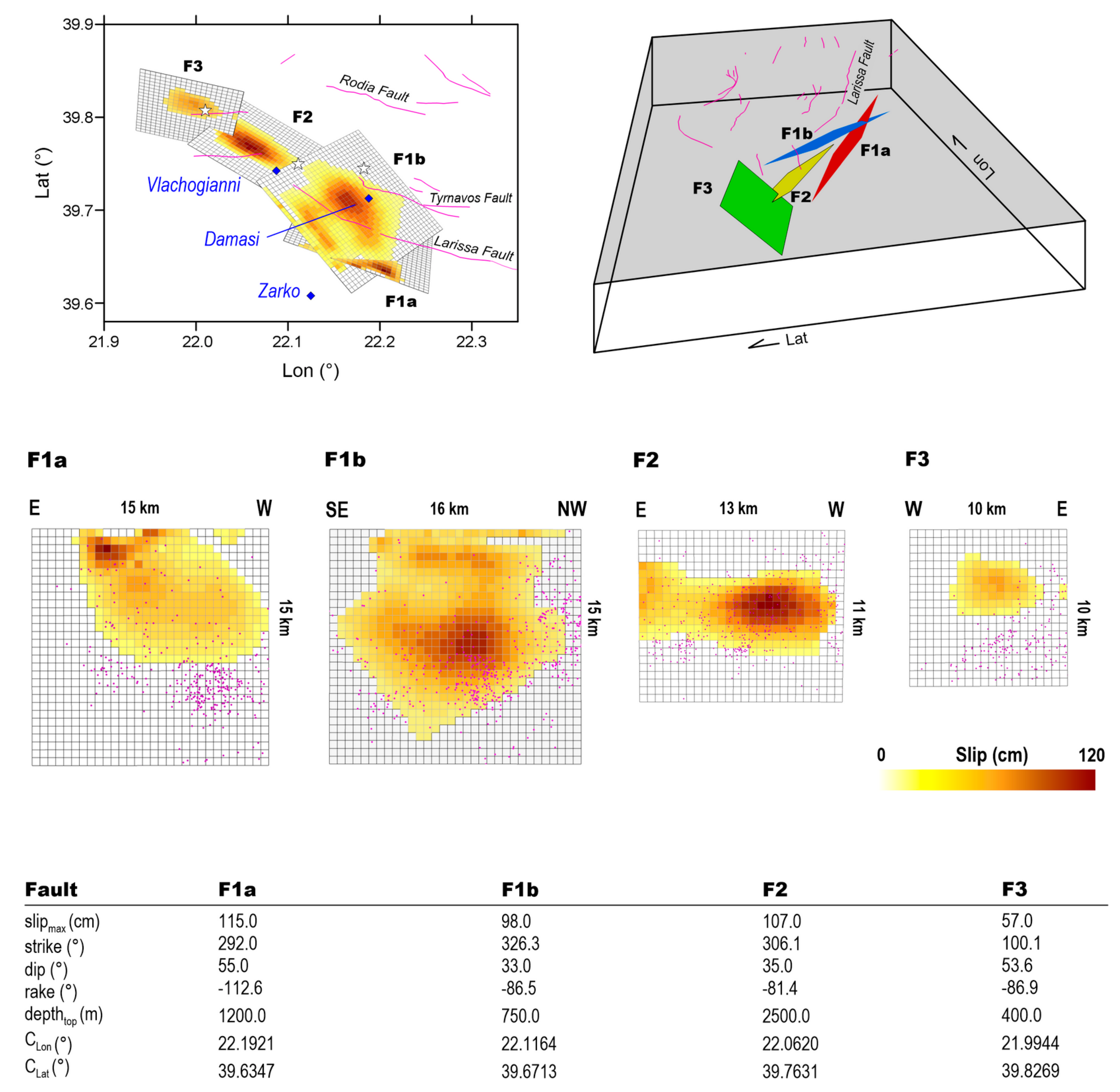
3.1. Analytical Modeling
3.2. Coulomb Stress Transfer Analysis
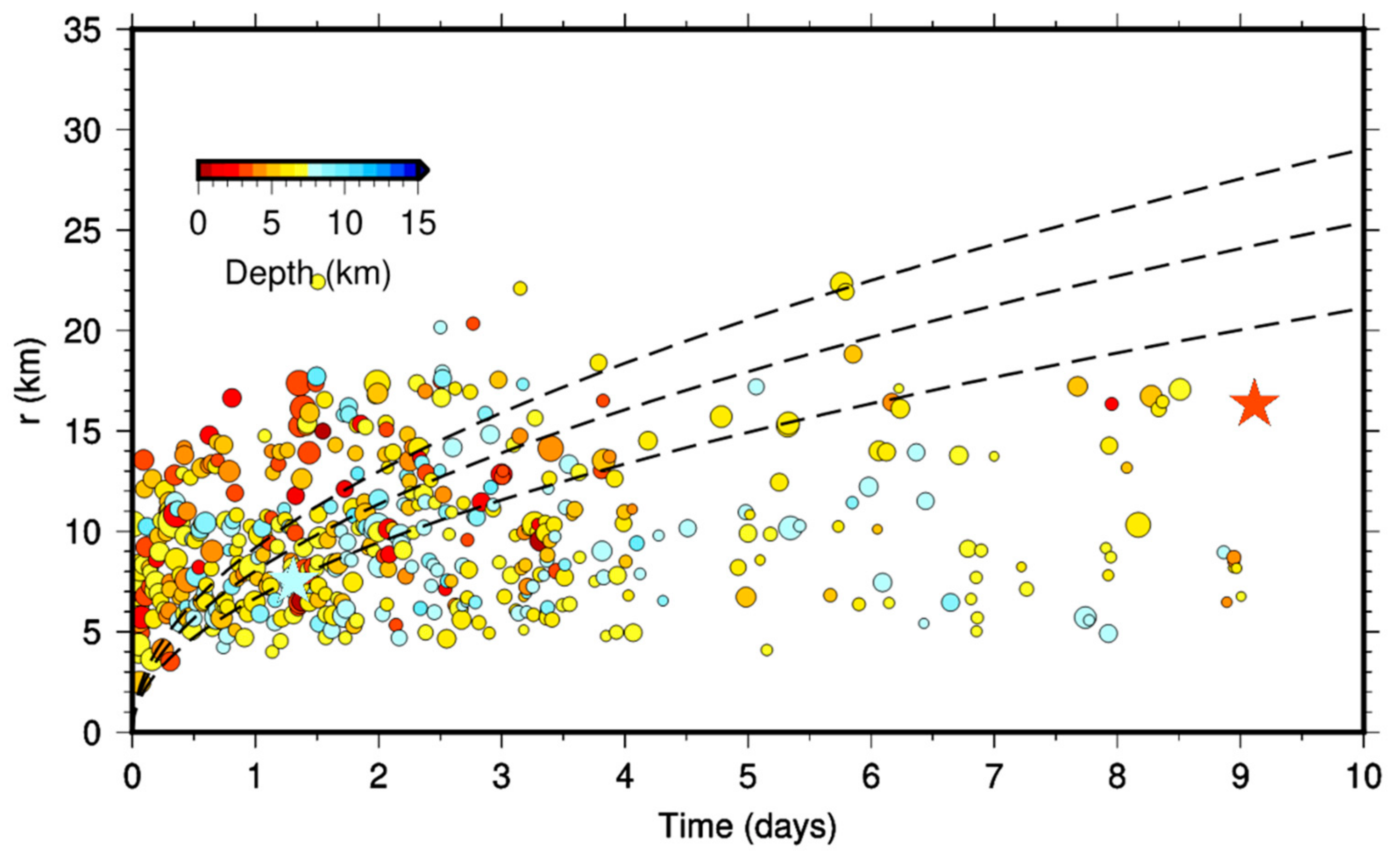
3.3. Diffusivity Analysis
4. Discussion
5. Conclusions
- the Thessaly seismic sequence nucleated at shallow depths (<12 km) and is related to the activation of several blind, previously unknown faults;
- the seismic sequence developed in a sort of domino effect involving a complex interaction among the normal faults within the activated crustal volume;
- InSAR data and modeling are also extremely useful to constrain the rupture characteristics in the case of blind faults; and
- the used approach can help improve our knowledge of the seismic potential of the Thessaly region and refine the associated seismic hazard.
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Lekkas, E.; Agorastos, K.; Mavroulis, S.; Kranis, C.; Skourtsos, E.; Carydis, P.; Gogou, M.; Katsetsiadou, K.-N.; Papadopoulos, G. The early March 2021 Thessaly earthquake sequence. In Newsletter of Environmental, Disaster and Crises Management Strategies; National and Kapodistrian University of Athens: Athens, Greece, 2021; p. 195. [Google Scholar] [CrossRef]
- Caputo, R.; Pavlides, S. Late Cainozoic geodynamic evolution of Thessaly and surroundings (central-northern Greece). Tectonophys 1993, 223, 339–362. [Google Scholar] [CrossRef]
- Caputo, R.; Helly, B.; Pavlides, S.; Papadopoulos, G. Archaeo- and palaeoseismological investigationsin Northern Thessaly (Greece): Insights for the seismic potential of the region. Nat. Haz. 2006, 39, 195–212. [Google Scholar] [CrossRef]
- Caputo, R.; Bravard, J.-P.; Helly, B. The Pliocene-quaternary tecto-sedimentary evolution of the Larissa Plain (Eastern Thessaly, Greece). Geodin. Acta 1994, 7, 57–85. [Google Scholar] [CrossRef]
- Caputo, R.; Helly, B. The Holocene activity of the Rodià Fault, Central Greece. J. Geodyn. 2005, 40, 153–169. [Google Scholar] [CrossRef]
- Caputo, R.; Pavlides, S. The Greek Database of Seismogenic Sources (GreDaSS), Version 2.0.0: A Compilation of Potential Seismogenic Sources (Mw > 5.5) in the Aegean Region; CINECA IRIS: Bologna, Italy, 2013. [Google Scholar] [CrossRef]
- Caputo, R.; Piscitelli, S.; Oliveto, A.; Rizzo, E.; Lapenna, V. The use of electrical resistivity tomography in active tectonics. Examples from the Tyrnavos Basin, Greece. J. Geodyn. 2003, 36, 19–35. [Google Scholar] [CrossRef]
- Caputo, R. Inference of a seismic gap from geological data: Thessaly (Central Greece) as a case study. Ann. Geofis. 1995, 38. [Google Scholar] [CrossRef]
- Lomax, A.; Virieux, J.; Volant, P.; Berge-Thierry, C. Probabilistic earthquake location in 3D and layered models. In Advances in Seismic Event Location; Springer: Dordrecht, The Netherlands, 2000; pp. 101–134. [Google Scholar]
- Papadimitriou, P.; Kaviris, G.; Makropoulos, K. The Cornet seismological network: 10 years of operation, recorded seismicity and significant applications. Hell. J. Geosci. 2010, 45, 193–208. [Google Scholar]
- Waldhauser, F.; Ellsworth, W.L. A double-difference earthquake location algorithm: Method and application to the northern Hayward fault, California. Bull. Seismol. Soc. Am. 2000, 90, 1353–1368. [Google Scholar] [CrossRef]
- Chiaraluce, L.; Valoroso, L.; Piccinini, D.; Di Stefano, R.; De Gori, P. The anatomy of the 2009 L’Aquila normal fault system (central Italy) imaged by high resolution foreshock and aftershock locations. J. Geophys. Res. Solid Earth 2011, 116. [Google Scholar] [CrossRef]
- Chiaraluce, L.; Di Stefano, R.; Tinti, E.; Scognamiglio, L.; Michele, M.; Casarotti, E.; Marzorati, S. The 2016 central Italy seismic sequence: A first look at the mainshocks, aftershocks, and source models. Seismol. Res. Lett. 2017, 88, 757–771. [Google Scholar] [CrossRef]
- Sansosti, E.; Berardino, P.; Manunta, M.; Serafino, F.; Fornaro, G. Geometrical SAR image registration. IEEE Trans. Geosci. Remote Sens. 2006, 44, 2861–2870. [Google Scholar] [CrossRef]
- Fornaro, G.; Sansosti, E. A two-dimensional region growing least squares phase unwrapping algorithm for interferometric SAR processing. IEEE Trans. Geosci. Remote Sens. 1999, 37, 2215–2226. [Google Scholar] [CrossRef]
- Fornaro, G.; Pauciullo, A.; Reale, D. A Null-Space Method for the Phase Unwrapping of Multitemporal SAR Interferometric Stacks. IEEE Trans. Geosci. Remote Sens. 2011, 49, 2323–2334. [Google Scholar] [CrossRef]
- Wright, T.J.; Parsons, B.E.; Lu, Z. Toward mapping surface deformation in three dimensions using InSAR. Geophys. Res. Lett. 2004, 31, L01607. [Google Scholar] [CrossRef] [Green Version]
- Okada, Y. Surface deformation due to shear and tensile faults in a half-space. Bull. Seismol. Soc. Am. 1985, 75, 1135–1154. [Google Scholar] [CrossRef]
- Okada, Y. Internal deformation due to shear and tensile faults in a half-space. Bull. Seismol. Soc. Am. 1992, 82, 1018–1040. [Google Scholar]
- Levenberg, K. A method for the solution of certain problems in least squares. Q. Appl. Math. 1944, 2, 164–168. [Google Scholar] [CrossRef] [Green Version]
- Marquardt, D. An algorithm for least-squares estimation of nonlinear parameters. SIAM J. Soc. Ind. Appl. Math. 1963, 11, 431–441. [Google Scholar] [CrossRef]
- Williams, C.A.; Wadge, G. An accurate and efficient method for including the effects of topography in three-dimensional elastic models of ground deformation with applications to radar interferometry. J. Geophys. Res. 2000, 105, 8103–8120. [Google Scholar] [CrossRef]
- Pepe, A.; Berardino, P.; Bonano, M.; Euillades, L.D.; Lanari, R.; Sansosti, E. SBAS-based satellite orbit correction for the generation of DInSAR time-series: Application to RADARSAT-1 data. IEEE Trans. Geosci. Remote Sens. 2011, 49, 5150–5165. [Google Scholar] [CrossRef]
- Atzori, S.; Hunstad, I.; Chini, M.; Salvi, S.; Tolomei, C.; Bignami, C.; Stramondo, S.; Trasatti, E.; Antonioli, A.; Boschi, E. Finite fault inversionof DInSAR coseismic displacement of the 2009 L’Aquila earthquake (central Italy). Geophys. Res. Lett. 2009, 36, L15305. [Google Scholar] [CrossRef]
- De Novellis, V.; Atzori, S.; De Luca, C.; Manzo, M.; Valerio, E.; Bonano, M.; Cardaci, C.; Castaldo, R.; Di Bucci, D.; Manunta, M.; et al. DInSAR analysis and analytical modeling of Mount Etna displacements: The December 2018 volcano-tectonic crisis. Geophys. Res. Lett. 2019, 46, 5817–5827. [Google Scholar] [CrossRef] [Green Version]
- Menke, W. Geophysical Data Analysis: Discrete Inverse Theory; Academic Press: Cambridge, MA, USA, 1989. [Google Scholar]
- Pino, N.A.; Convertito, V.; Madariaga, R. Clock advance and magnitude limitation through fault interaction: The case of the 2016 central Italy earthquake sequence. Sci. Rep. 2019, 9, 5005. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Wang, R.; Lorenzo-Martin, F.; Roth, F. PSGRN/PSCMP—A new code for calculating co- and post-seismic deformation, geoid and gravity changes based on the viscoelastic-gravitational dislocation theory. Comput. Geosci. 2006, 32, 527–541. [Google Scholar] [CrossRef] [Green Version]
- Noir, J.; Jacques, E.; Bekri, S.; Adler, P.M.; Tapponier, P.; King, G.C.P. Fluid flow triggered migration of events in the 1989 Dobi earthquake sequence of central Afar. Geophys. Res. Lett. 1997, 24, 2335–2338. [Google Scholar] [CrossRef]
- Shapiro, S.; Patzig, R.; Rothert, E.; Rindschwentner, J. Triggering of Seismicity by Pore-pressure Perturbations: Permeability-related Signatures of the Phenomenon. Pure Appl. Geophys. 2003, 456, 1051–1066. [Google Scholar] [CrossRef]
- Wiemer, S.; Wyss, M. Minimum magnitude of complete reporting in earthquake catalogs: Examples from Alaska, the western United States, and Japan. Bull. Seismol. Soc. Am. 2000, 90, 859–869. [Google Scholar] [CrossRef]
- Malagnini, L.; Lucente, F.P.; De Gori, P.; Akinci, A.; Munafo’, I. Control of pore fluid pressure diffusion on fault failure mode: Insights from the 2009 L’Aquila seismic sequence. J. Geophys. Res. 2012, 117, B05302. [Google Scholar] [CrossRef]
- Das, S.; Kostrov, B.V. Breaking of a single asperity: Rupture process and seismic radiation. J. Geophys. Res. 1983, 88, 4277–4288. [Google Scholar] [CrossRef]
- Gomberg, J. Unsettled earthquake nucleation. Nat. Geosci. 2018, 11, 463–464. [Google Scholar] [CrossRef]
- Abercrombie, R.E. Similar starts for small and large earthquakes. Nature 2019, 573, 42–43. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kato, A.; Ben-Zion, Y. The generation of large earthquakes. Nat. Rev. Earth Environ. 2021, 2, 26–39. [Google Scholar] [CrossRef]
- Gavrilenko, P. Hydromechanical coupling in response to earthquakes: On the possible consequences for aftershocks. Geophys. J. Int. 2005, 161, 113–129. [Google Scholar] [CrossRef] [Green Version]
- Tung, S.; Masterlark, T. Delayed poroelastic triggering of the 2016 October Visso earthquake by the August Amatrice earthquake, Italy. Geophys. Res. Lett. 2018, 45, 2221–2229. [Google Scholar] [CrossRef]
- Convertito, V.; De Matteis, R.; Improta, L.; Pino, N.A. Fluid-Triggered Aftershocks in an Anisotropic Hydraulic Conductivity Geological Complex: The Case of the 2016 Amatrice Sequence, Italy. Front. Earth Sci. 2020, 8, 541323. [Google Scholar] [CrossRef]
- Scholz, C.H. The Mechanics of Earthquakes and Faulting, 2nd ed.; Cambridge University Press: Cambridge, UK, 2002. [Google Scholar]
- Wessel, P.; Smith, W.H.F. Free software helps map and display data. EOS Trans. AGU 1991, 72, 445–446. [Google Scholar] [CrossRef]

| Depth (km) | VP (km/s) | VS (km/s) | ρ (kg/m3) |
|---|---|---|---|
| 0.0–4.0 | 4.8 | 2.7 | 4437 |
| 4.0–7.0 | 5.7 | 3.3 | 3146 |
| 7.0–11.50 | 6.1 | 4.5 | 2747 |
| 11.50–16.50 | 6.3 | 3.6 | 2575 |
| 16.50–35.0 | 6.5 | 3.7 | 2419 |
| 35.0 ∞ | 7.8 | 4.4 | 1680 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
De Novellis, V.; Reale, D.; Adinolfi, G.M.; Sansosti, E.; Convertito, V. Geodetic Model of the March 2021 Thessaly Seismic Sequence Inferred from Seismological and InSAR Data. Remote Sens. 2021, 13, 3410. https://doi.org/10.3390/rs13173410
De Novellis V, Reale D, Adinolfi GM, Sansosti E, Convertito V. Geodetic Model of the March 2021 Thessaly Seismic Sequence Inferred from Seismological and InSAR Data. Remote Sensing. 2021; 13(17):3410. https://doi.org/10.3390/rs13173410
Chicago/Turabian StyleDe Novellis, Vincenzo, Diego Reale, Guido Maria Adinolfi, Eugenio Sansosti, and Vincenzo Convertito. 2021. "Geodetic Model of the March 2021 Thessaly Seismic Sequence Inferred from Seismological and InSAR Data" Remote Sensing 13, no. 17: 3410. https://doi.org/10.3390/rs13173410
APA StyleDe Novellis, V., Reale, D., Adinolfi, G. M., Sansosti, E., & Convertito, V. (2021). Geodetic Model of the March 2021 Thessaly Seismic Sequence Inferred from Seismological and InSAR Data. Remote Sensing, 13(17), 3410. https://doi.org/10.3390/rs13173410









