The Dynamical Structure of a Warm Core Ring as Inferred from Glider Observations and Along-Track Altimetry
Abstract
:1. Introduction
2. Data
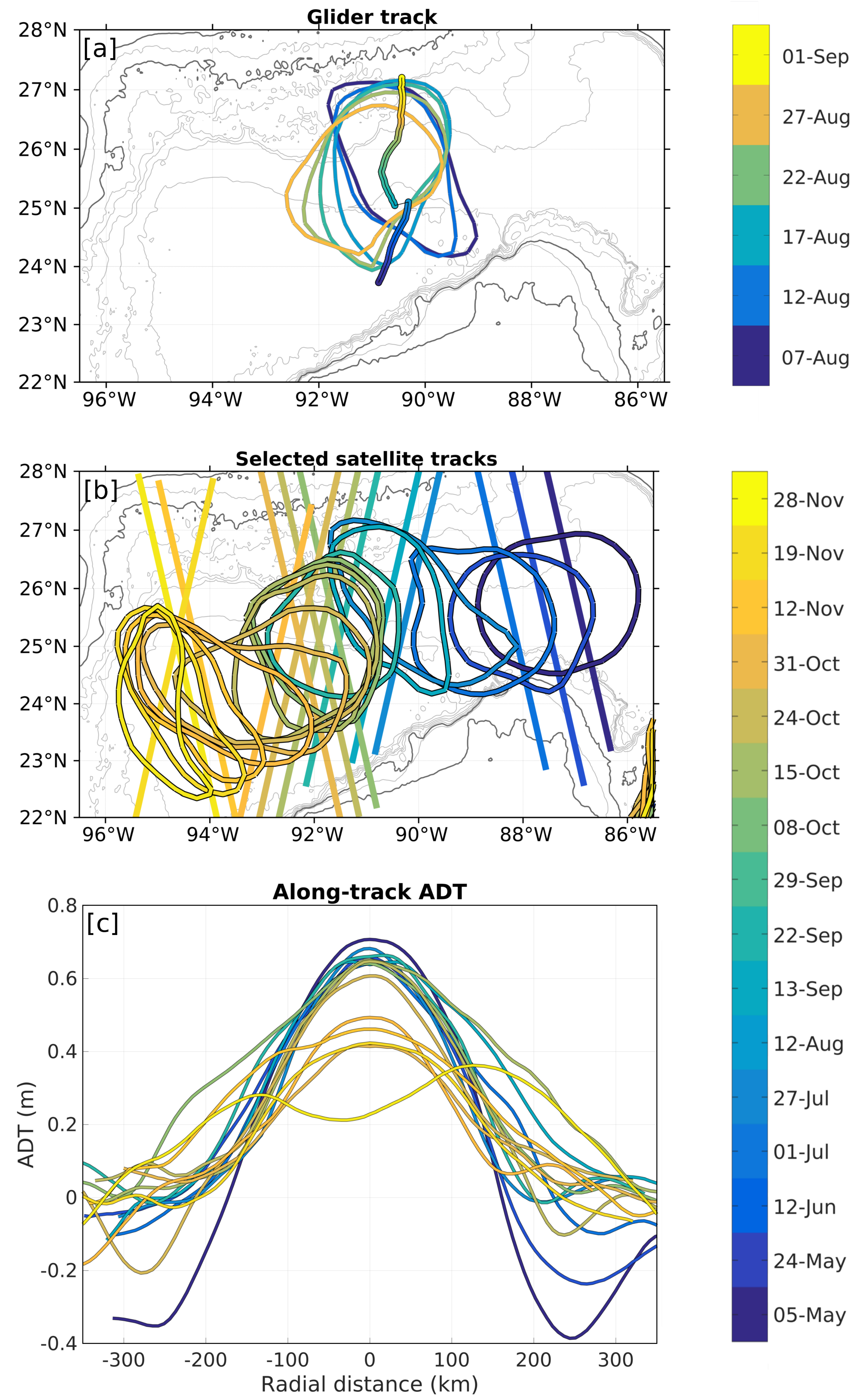
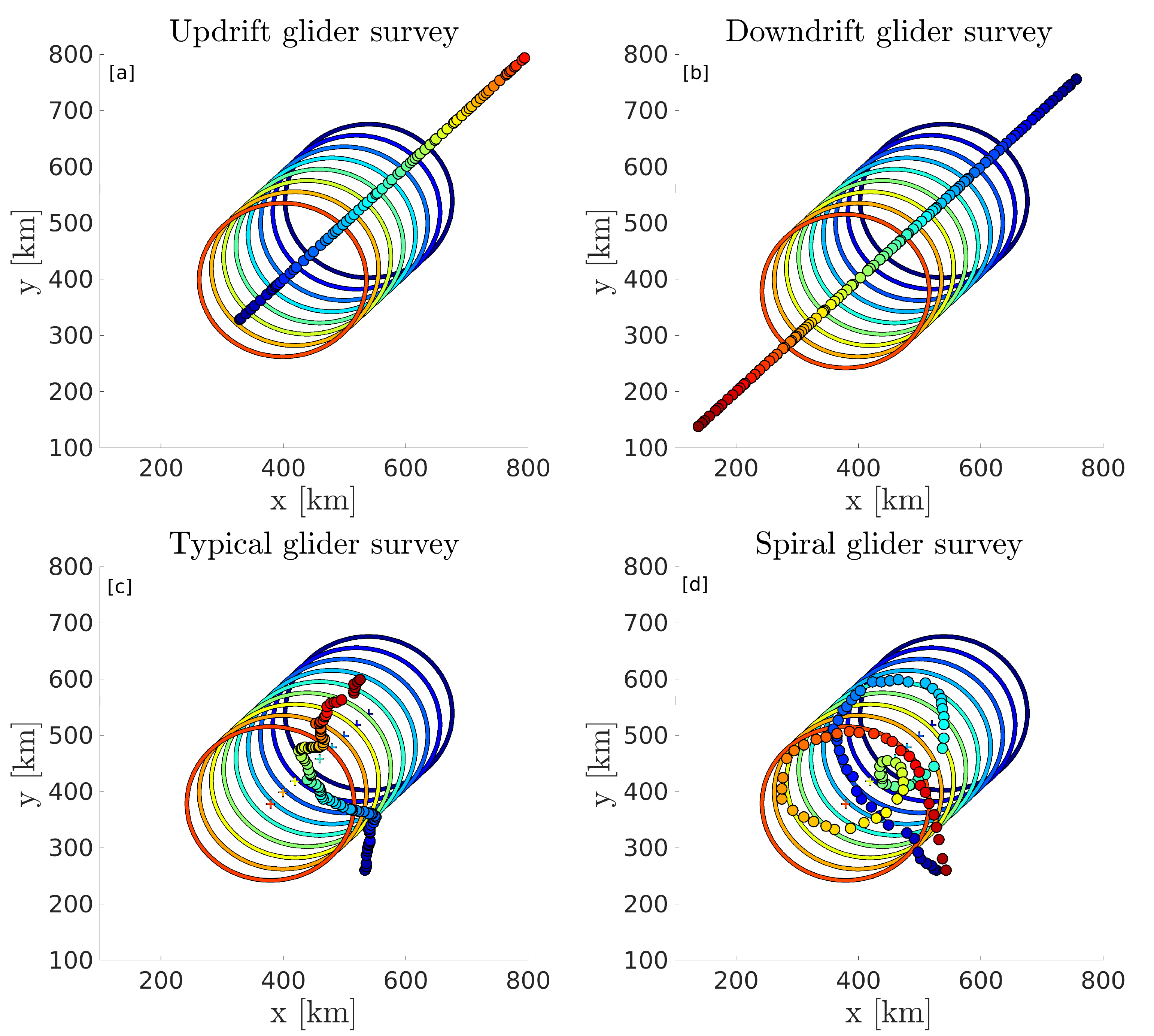
2.1. The Glider Survey
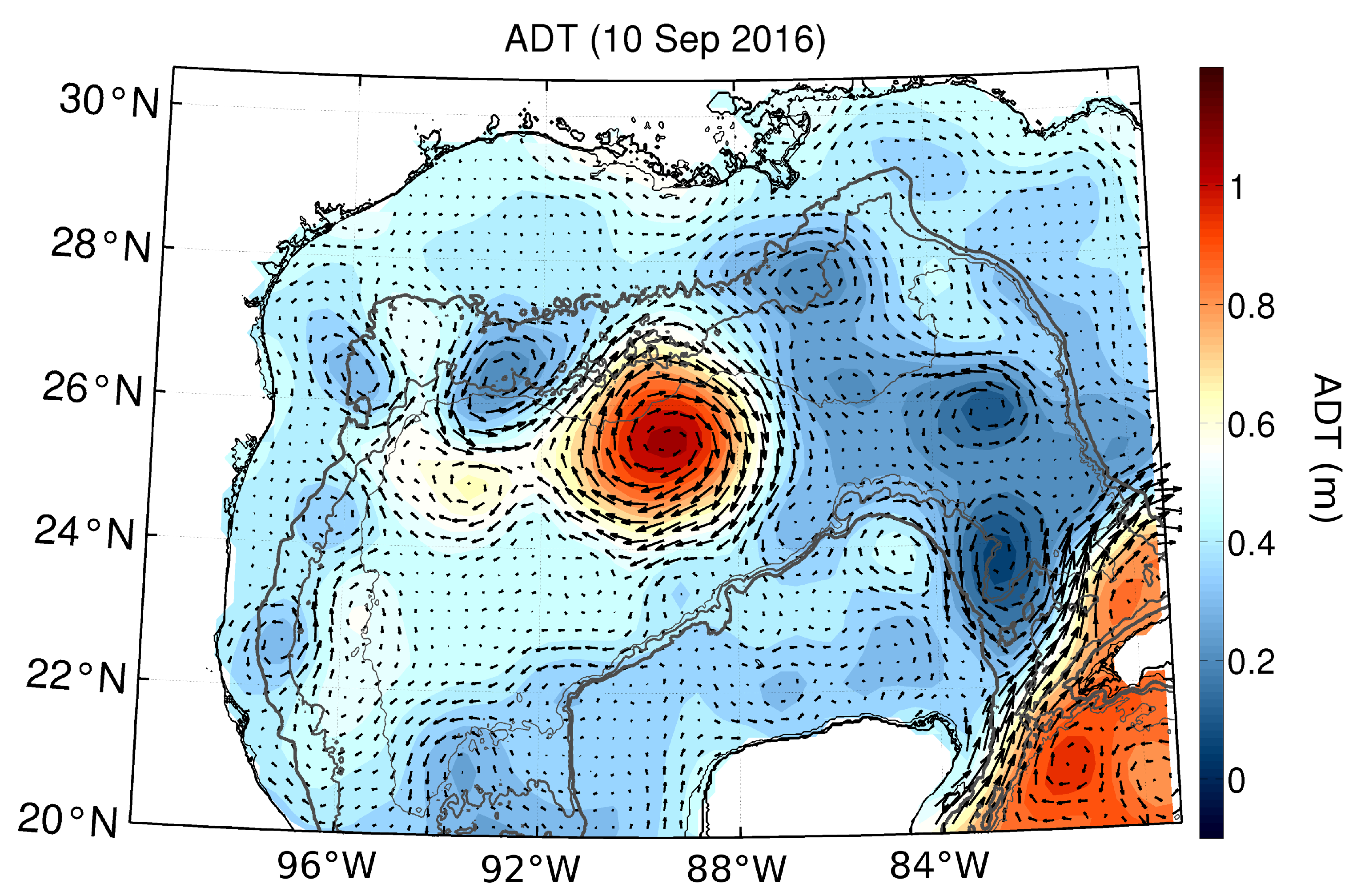
2.2. Satellite Altimetry
3. Methods
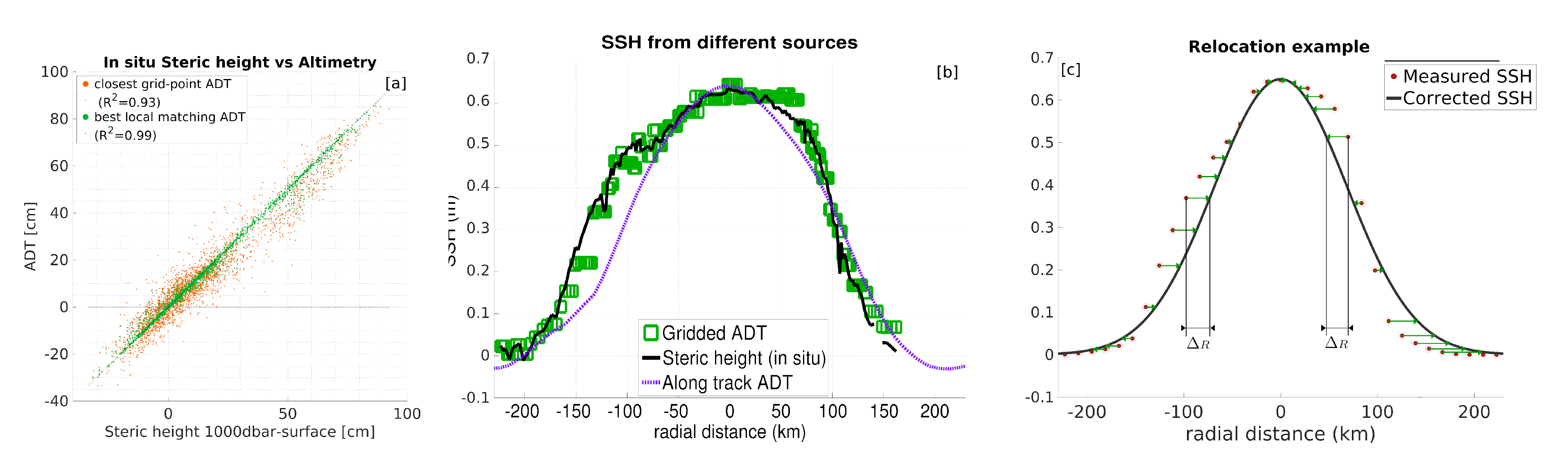
3.1. The Relocation Method
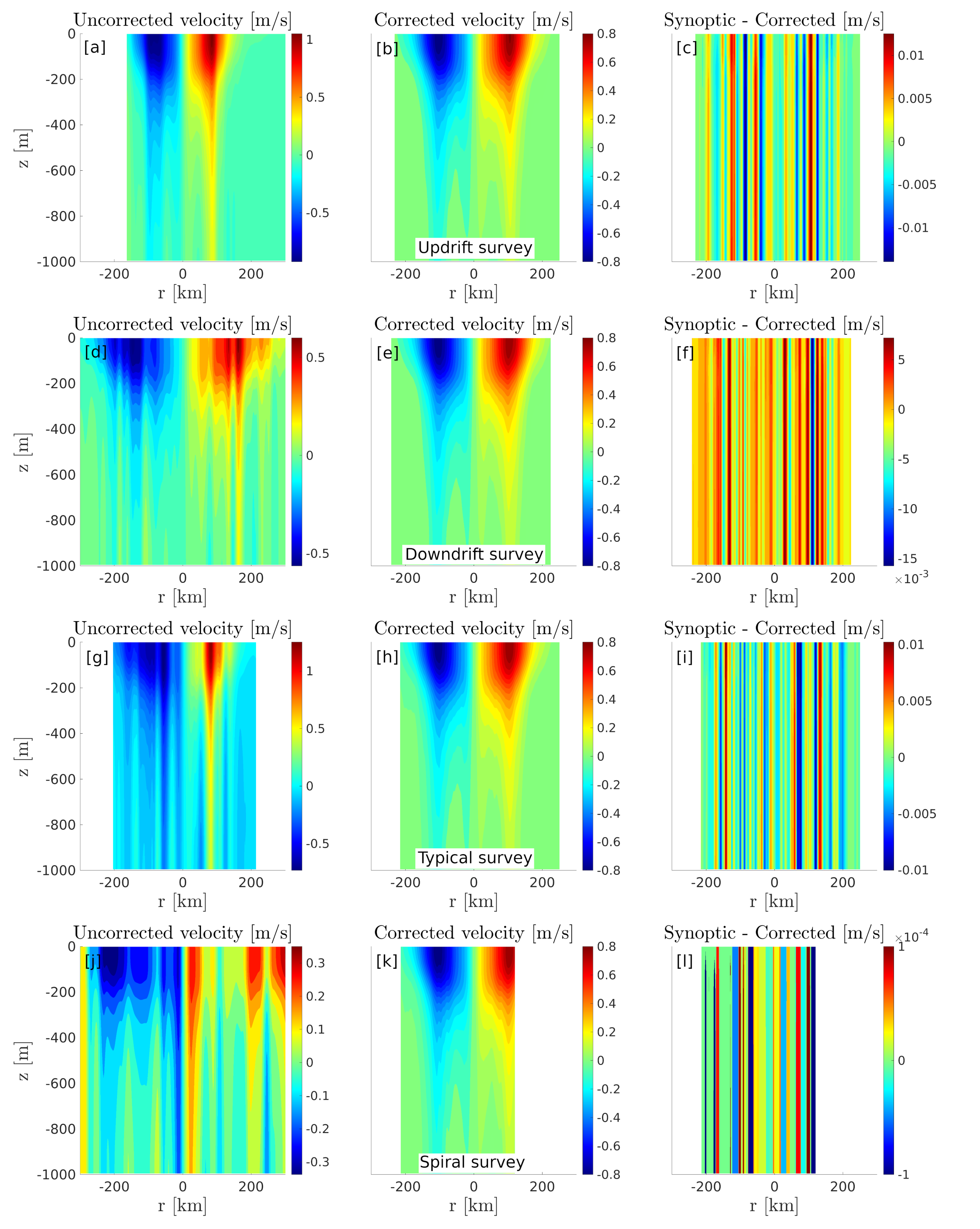
3.2. Validation
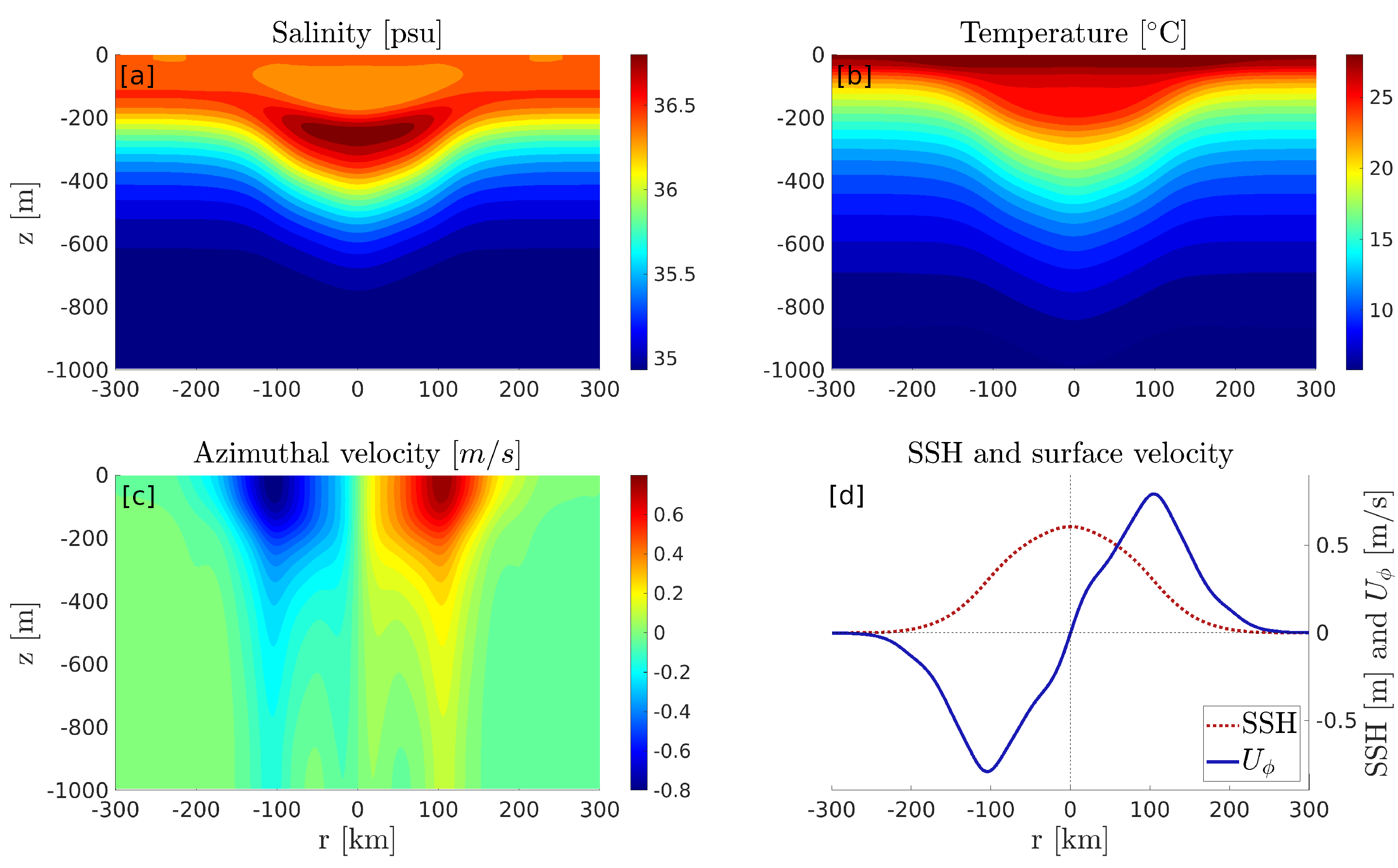
3.3. Theoretical Framework
4. Results
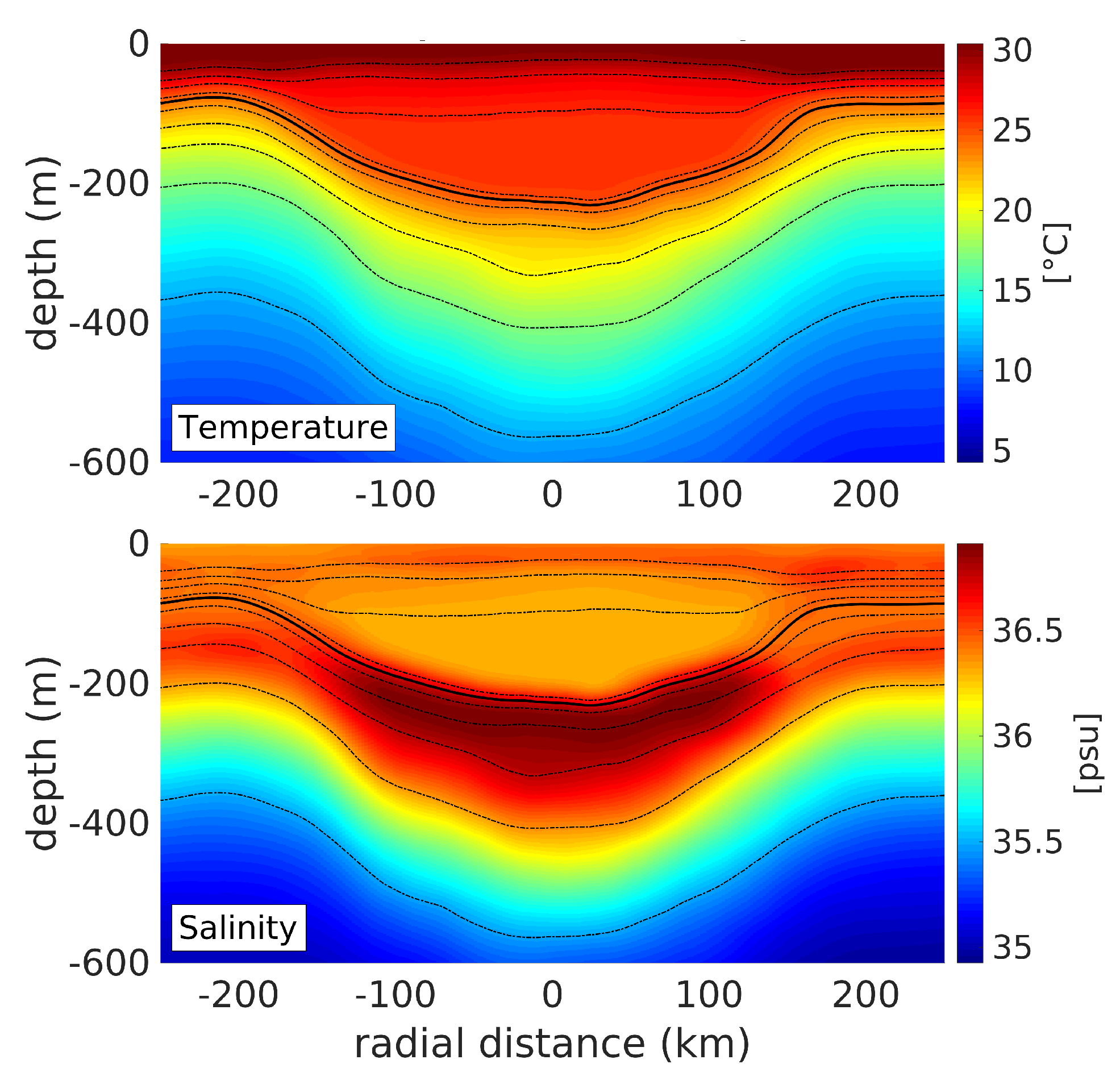
4.1. Thermohaline Structure
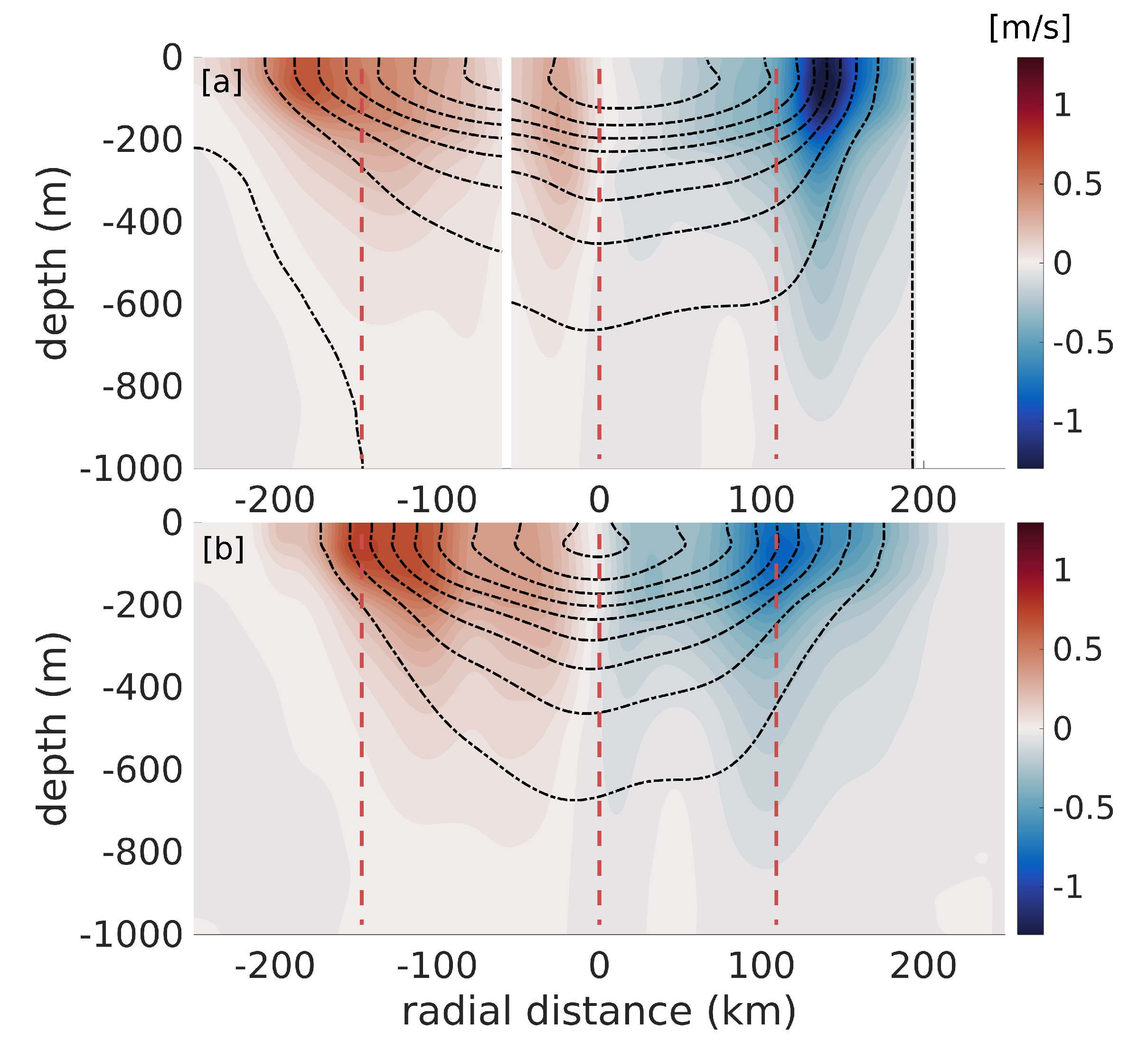
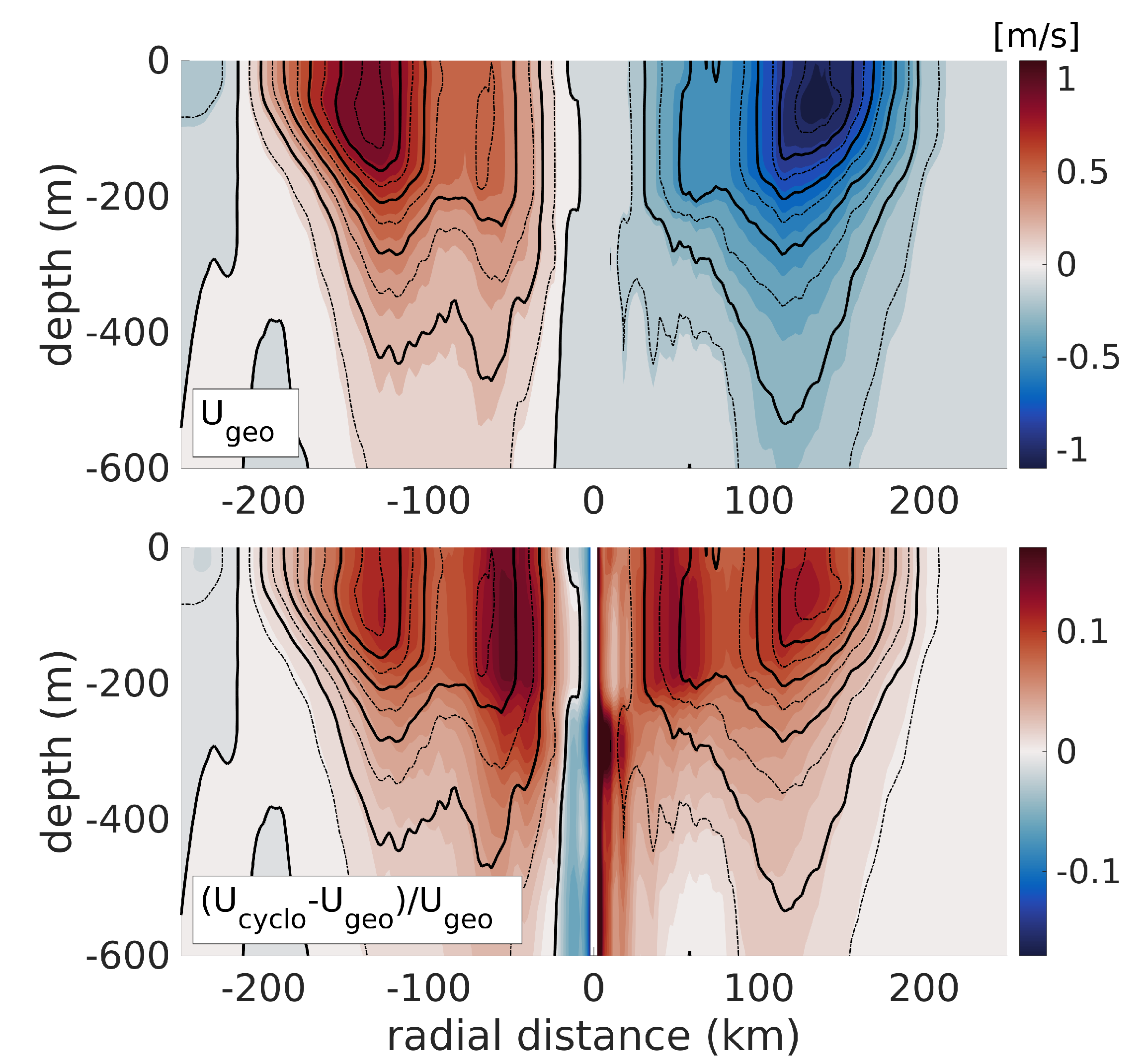
4.2. Velocity
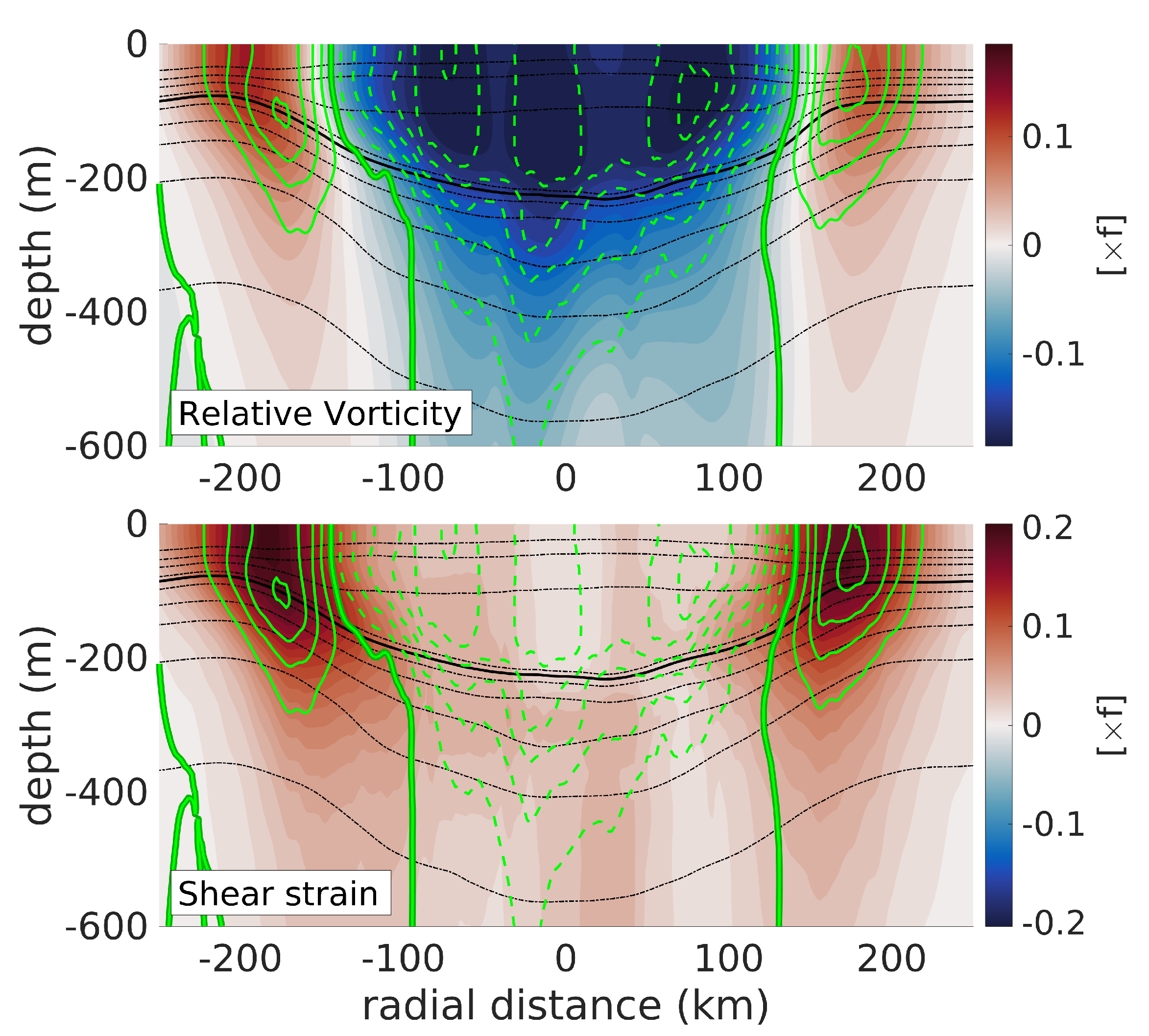
4.3. Relative Vorticity and Strain
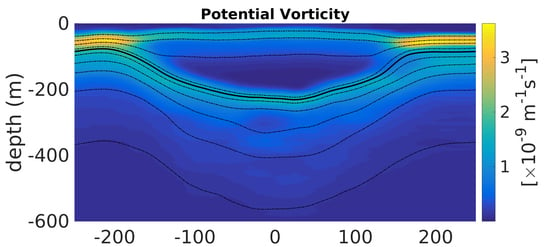
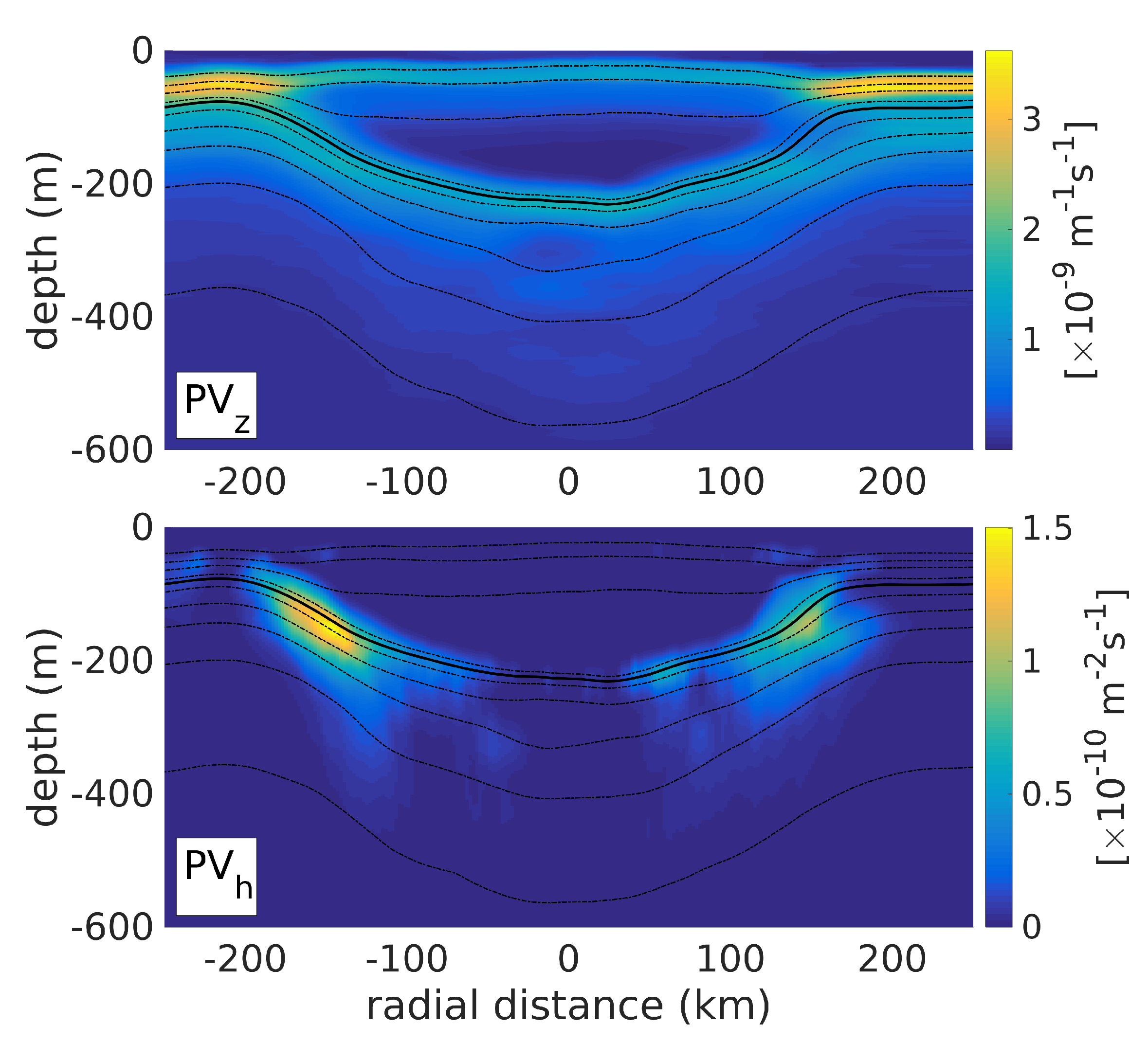
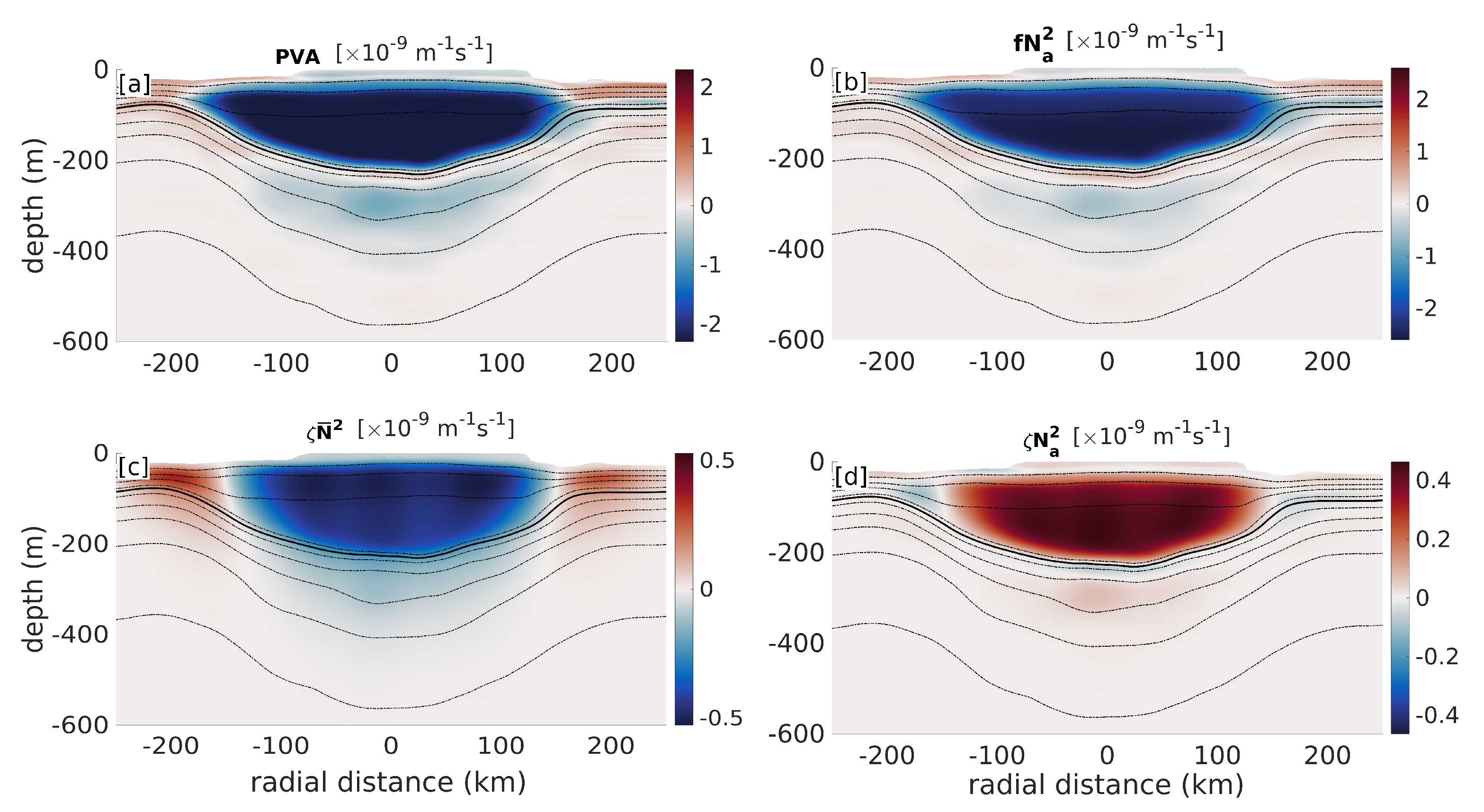
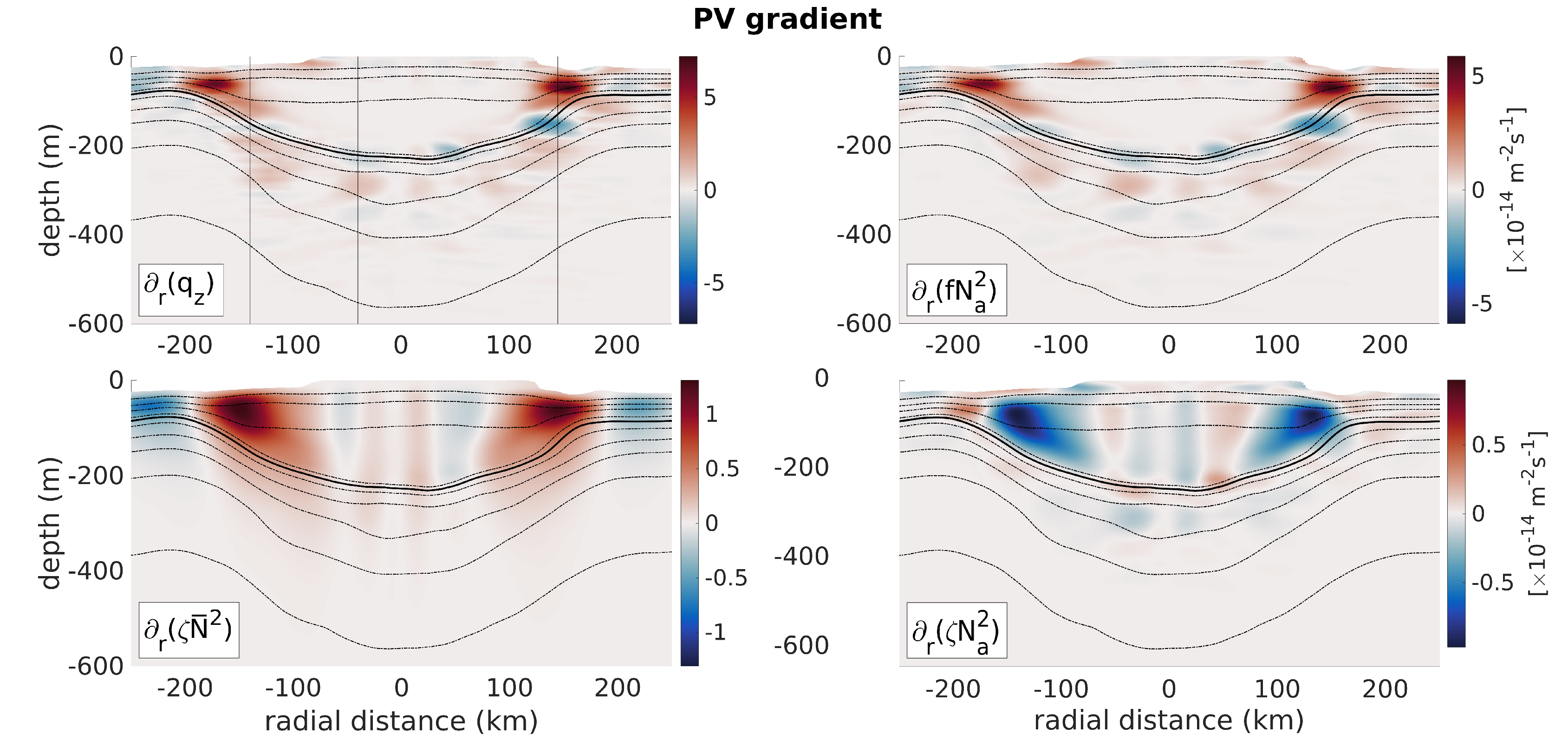
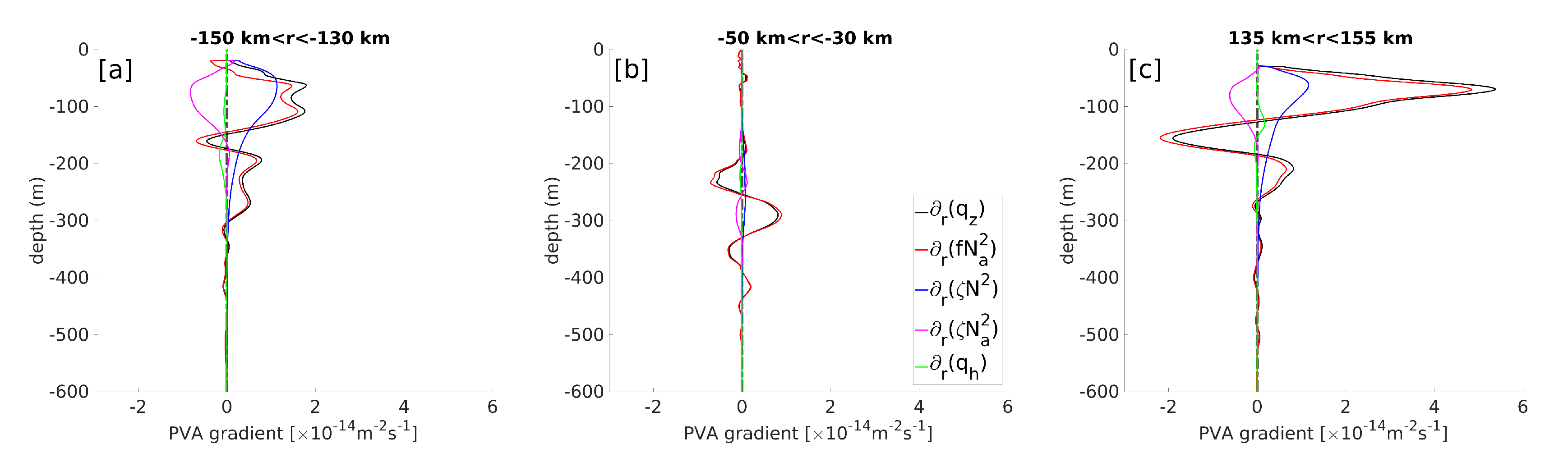
4.4. Potential Vorticity Structure
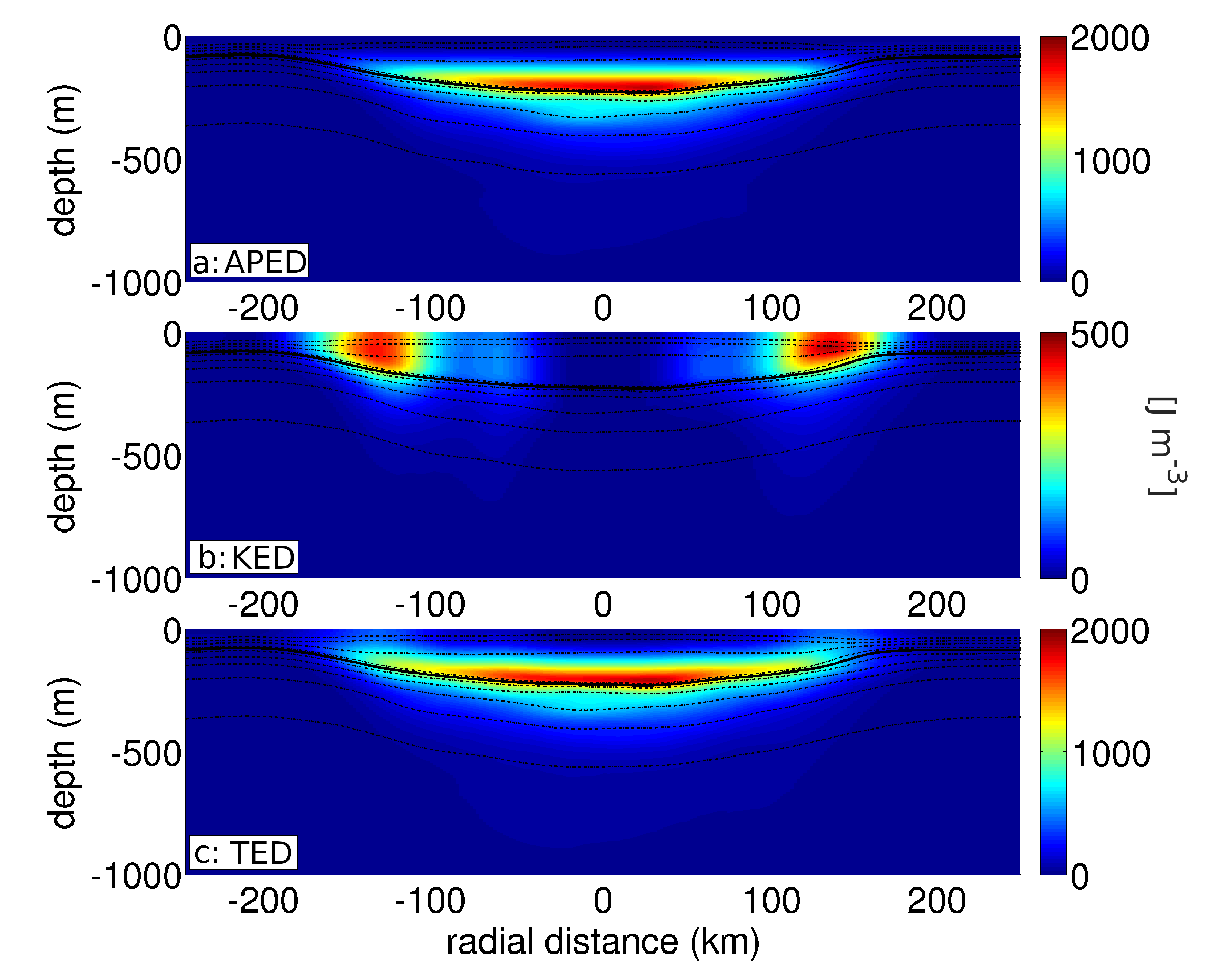
4.5. Energetics
5. Discussion
5.1. The Relocation Method
5.2. The LCR’s Vertical Structure
6. Summary
- A new altimetry-based method to relocate gliders observations in a synoptic frame of reference was designed and applied to recent observations of a Loop Current ring in the Gulf of Mexico.
- The method was tested using an analytical anticyclonic eddy drifting on the -plane, and shown to recover the exact vertical structure of the eddy, whatever the sampling strategy, in the ideal case of a stable and circular eddy.
- The method was successful in correcting the errors in horizontal thermohaline gradients, related to the lack of synopticity of glider surveys.
- The relocation method also allows to precisely locate the eddy’s rotation axis, yielding more reliable estimates of cyclo-geostrophic velocity, relative vorticity, and shear strain.
- The warm core ring consisted of a bowl of homogeneous negative relative vorticity, surrounded by a crown of positive shear strain, resulting in a negative Okubo–Weiss parameter in the core and positive at the periphery.
- The PV structure of the warm-core ring is largely dominated by vortex stretching.
- The along-isopycnal radial PV gradient is also dominated by gradients of the vortex stretching term.
- Sign-changes of the PV-gradient suggests that the warm core ring might be baroclinically unstable.
- The energy density partition revealed a clear dominance of available potential energy over kinetic energy.
- Available potential energy density is mostly contained within the vorticity-dominated core of the warm-core ring (negative Okubo–Weiss), where properties are expected to be well conserved. This might possibly contribute to its longevity.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Testor, P.; de Young, B.; Rudnick, D.L.; Glenn, S.; Hayes, D. OceanGliders: A component of the integrated GOOS. Front. Mar. Sci. 2019, 6, 422. [Google Scholar] [CrossRef] [Green Version]
- Rudnick, D.L.; Davis, R.E.; Eriksen, C.C.; Fratantoni, D.M.; Perry, M.J. Underwater gliders for ocean research. Mar. Technol. Soc. J. 2004, 38, 73–84. [Google Scholar] [CrossRef]
- Rudnick, D.L.; Cole, S.T. On sampling the ocean using underwater gliders. J. Geophys. Res. 2011, 116, C08010. [Google Scholar] [CrossRef] [Green Version]
- Rudnick, D.L. Ocean Research Enabled by Underwater Gliders. Annu. Rev. Mar. Sci. 2016, 8, 519–541. [Google Scholar] [CrossRef] [PubMed]
- Rudnick, D.L.; Johnston, T.M.S.; Sherman, J.T. High-frequency internal waves near the Luzon Strait observed by underwater gliders. J. Geophys. Res. 2013, 118, 774–784. [Google Scholar] [CrossRef]
- Todd, R.E. Gulf Stream Mean and Eddy Kinetic Energy: Three-Dimensional Estimates From Underwater Glider Observations. Geophys. Res. Lett. 2021, 48, 2020GL090281. [Google Scholar] [CrossRef]
- Kolodziejczyk, N.; Testor, P.; Lazar, A.; Echevin, V.; Krahmann, G.; Chaigneau, A.; Gourcuff, C.; Wade, M.; Faye, S.; Estrade, P.; et al. Subsurface Fine-Scale Patterns in an Anticyclonic Eddy Off Cap-Vert Peninsula Observed From Glider Measurements. J. Geophys. Res. Ocean. 2018, 123, 6312–6329. [Google Scholar] [CrossRef]
- Rudnick, D.L.; Gopalakrishnan, G.; Cornuelle, B.D. Cyclonic Eddies in the Gulf of Mexico: Observations by Underwater Gliders and Simulations by Numerical Model. J. Phys. Oceanogr. 2015, 45, 313–326. [Google Scholar] [CrossRef]
- Meunier, T.; Pallás-Sanz, E.; Tenreiro, M.; Portela, E.; Ochoa, J.; Ruiz-Angulo, A.; Cusí, S. The vertical structure of a Loop Current Eddy. J. Geophys. Res. Ocean. 2018, 123, 6070–6090. [Google Scholar] [CrossRef]
- Meunier, T.; Tenreiro, M.; Pallàs-Sanz, E.; Ochoa, J.; Ruiz-Angulo, A.; Portela, E.; Cusí, S.; Damien, P.; Carton, X. Intrathermocline Eddies Embedded within an Anticyclonic Vortex Ring. Geophys. Res. Lett. 2018, 45, 7624–7633. [Google Scholar] [CrossRef] [Green Version]
- Meunier, T.; Pallàs Sanz, E.; Tenreiro, M.; Ochoa, J.; Ruiz Angulo, A.; Buckingham, C. Observations of Layering under a Warm-Core Ring in the Gulf of Mexico. J. Phys. Oceanogr. 2019, 49, 3145–3162. [Google Scholar] [CrossRef]
- Molodtsov, S.; Anis, A.; Amon, R.M.W.; Perez-Brunius, P. Turbulent Mixing in a Loop Current Eddy from Glider-Based Microstructure Observations. Geophys. Res. Lett. 2020, 47, e88033. [Google Scholar] [CrossRef]
- Ichiye, T. Circulation and water-mass distribution in the Gulf of Mexico. J. Geophys. Res. 1959, 64, 1109–1110. [Google Scholar]
- Elliott, B.A. Anticyclonic Rings in the Gulf of Mexico. J. Phys. Oceanogr. 1982, 12, 1292–1309. [Google Scholar] [CrossRef] [Green Version]
- Vukovich, F.M. An updated evaluation of the Loop Current’s eddy-shedding frequency. J. Geophys. Res. 1995, 100, 8655–8659. [Google Scholar] [CrossRef]
- Liu, Y.; Wilson, C.; Green, M.A.; Hughes, C.W. Gulf Stream Transport and Mixing Processes via Coherent Structure Dynamics. J. Geophys. Res. 2018, 123, 3014–3037. [Google Scholar] [CrossRef]
- Richardson, P. Gulf stream rings. In Eddies in Marine Science; Springer: Berlin/Heidelberg, Germany, 1983; pp. 19–45. [Google Scholar] [CrossRef]
- Li, L.; Nowlin, W.D.; Jilan, S. Anticyclonic rings from the Kuroshio in the South China Sea. Deep Sea Res. Part I Oceanogr. Res. 1998, 45, 1469–1482. [Google Scholar] [CrossRef]
- Sasaki, Y.N.; Minobe, S. Climatological Mean Features and Interannual to Decadal Variability of Ring Formations in the Kuroshio Extension Region. In AGU Fall Meeting Abstracts; Springer: Tokyo, Japan, 2014; Volume 2014, p. OS41F-07. [Google Scholar] [CrossRef] [Green Version]
- Fratantoni, D.M.; Johns, W.E.; Townsend, T.L. Rings of the North Brazil Current: Their structure and behavior inferred from observations and a numerical simulation. J. Geophys. Res. 1995, 100, 10633–10654. [Google Scholar] [CrossRef]
- Olson, D.B.; Evans, R.H. Rings of the Agulhas current. Deep Sea Res. A 1986, 33, 27–42. [Google Scholar] [CrossRef]
- Wang, Y.; Beron-Vera, F.J.; Olascoaga, M.J. The life cycle of a coherent Lagrangian Agulhas ring. J. Geophys. Res. 2016, 121, 3944–3954. [Google Scholar] [CrossRef] [Green Version]
- Olson, D.B.; Schmitt, R.W.; Kennelly, M.; Joyce, T.M. A two-layer diagnostic model of the long-term physical evolution of warm-core ring 82B. J. Geophys. Res. 1985, 90, 8813–8822. [Google Scholar] [CrossRef]
- Meunier, T.; Sheinbaum, J.; Pallàs-Sanz, E.; Tenreiro, M.; Ochoa, J.; Ruiz-Angulo, A.; Carton, X.; de Marez, C. Heat Content Anomaly and Decay of Warm-Core Rings: The Case of the Gulf of Mexico. Geophys. Res. Lett. 2020, 47, e85600. [Google Scholar] [CrossRef]
- Cooper, C.; Forristall, G.Z.; Joyce, T.M. Velocity and hydrographic structure of two Gulf of Mexico warm-core rings. J. Geophys. Res. 1990, 95, 1663–1679. [Google Scholar] [CrossRef]
- Hamilton, P.; Leben, R.; Bower, A.; Furey, H.; Pérez-Brunius, P. Hydrography of the Gulf of Mexico Using Autonomous Floats. J. Phys. Oceanogr. 2018, 48, 773–794. [Google Scholar] [CrossRef]
- Hamilton, P.; Fargion, G.S.; Biggs, D.C. Loop Current Eddy Paths in the Western Gulf of Mexico. J. Phys. Oceanogr. 1999, 29, 1180–1207. [Google Scholar] [CrossRef]
- Hoskins, B.J.; McIntyre, M.E.; Robertson, A.W. On the use and significance of isentropic potential vorticity maps. Q. J. R. Meteorol. Soc. 1985, 111, 877–946. [Google Scholar] [CrossRef]
- Penven, P.; Halo, I.; Pous, S.; Marié, L. Cyclogeostrophic balance in the Mozambique Channel. J. Geophys. Res. 2014, 119, 1054–1067. [Google Scholar] [CrossRef] [Green Version]
- Weiss, J. The dynamics of enstrophy transfer in two-dimensional hydrodynamics. Phys. D Nonlinear Phenom. 1991, 48, 273–294. [Google Scholar] [CrossRef]
- Isern-Fontanet, J.; Font, J.; García-Ladona, E.; Emelianov, M.; Millot, C.; Taupier-Letage, I. Spatial structure of anticyclonic eddies in the Algerian basin (Mediterranean Sea) analyzed using the Okubo Weiss parameter. Deep Sea Res. Part II Top. Stud. Oceanogr. 2004, 51, 3009–3028. [Google Scholar] [CrossRef]
- Provenzale, A. Transport by Coherent Barotropic VORTICES. Annu. Rev. Fluid Mech. 1999, 31, 55–93. [Google Scholar] [CrossRef] [Green Version]
- Lorenz, E.N. Available Potential Energy and the Maintenance of the General Circulation. Tellus Ser. A 1955, 7, 157–167. [Google Scholar] [CrossRef]
- Holliday, D.; McIntyre, M.E. On potential energy density in an incompressible, stratified fluid. J. Fluid Mech. 1981, 107, 221–225. [Google Scholar] [CrossRef]
- Huang, R.X. Available potential energy in the world’s oceans. J. Mar. Res. 2005, 63, 141–158. [Google Scholar] [CrossRef]
- D’Asaro, E.A. Observations of small eddies in the Beaufort Sea. J. Geophys. Res. 1988, 93, 6669–6684. [Google Scholar] [CrossRef]
- Armi, L.; Hebert, D.; Oakey, N.; Price, J.F.; Richardson, P.L.; Thomas Rossby, H.; Ruddick, B. Two Years in the Life of a Mediterranean Salt Lens. J. Phys. Oceanogr. 1989, 19, 354–370. [Google Scholar] [CrossRef]
- Saunders, P.M. The Instability of a Baroclinic Vortex. J. Phys. Oceanogr. 1973, 3, 61–65. [Google Scholar] [CrossRef] [Green Version]
- Flierl, G.R. On the instability of geostrophic vortices. J. Fluid Mech. 1988, 197, 349–388. [Google Scholar] [CrossRef]
- Carton, X.; McWilliams, J. Barotropic and baroclinic instabilities of axisymmetric vortices in a quasigeostrophic model. In Elsevier Oceanography Series; Elsevier: Amsterdam, The Netherlands, 1989; Volume 50, pp. 225–244. [Google Scholar] [CrossRef]
- Carton, X. Hydrodynamical Modeling Of Oceanic Vortices. Surv. Geophys. 2001, 22, 179–263. [Google Scholar] [CrossRef]
- Chaigneau, A.; Le Texier, M.; Eldin, G.; Grados, C.; Pizarro, O. Vertical structure of mesoscale eddies in the eastern South Pacific Ocean: A composite analysis from altimetry and Argo profiling floats. J. Geophys. Res. 2011, 116, C11025. [Google Scholar] [CrossRef]
- Sosa-Gutiérrez, R.; Pallàs-Sanz, E.; Jouanno, J.; Chaigneau, A.; Candela, J.; Tenreiro, M. Erosion of the Subsurface Salinity Maximum of the Loop Current Eddies From Glider Observations and a Numerical Model. J. Geophys. Res. Ocean. 2020, 125, e2019JC015397. [Google Scholar] [CrossRef]
- de Marez, C.; L’Hégaret, P.; Morvan, M.; Carton, X. On the 3D structure of eddies in the Arabian Sea. Deep Sea Res. Part I Oceanogr. Res. 2019, 150, 103057. [Google Scholar] [CrossRef]
- Elliott, B.A. Anticyclonic Rings and the Energetics of the Circulation of the Gulf of Mexico; Texas A&M University: College Station, TX, USA, 1979. [Google Scholar]
- Joyce, T.M. Velocity and Hydrographic Structure of a Gulf Stream Warm-Core Ring. J. Phys. Oceanogr. 1984, 14, 936–947. [Google Scholar] [CrossRef] [Green Version]
- Dewar, W.K.; Killworth, P.D. On the Stability of Oceanic Rings. J. Phys. Oceanogr. 1995, 25, 1467–1487. [Google Scholar] [CrossRef] [Green Version]
- Dewar, W.K.; Killworth, P.D.; Blundell, J.R. Primitive-Equation Instability of Wide Oceanic Rings. Part II: Numerical Studies of Ring Stability. J. Phys. Oceanogr. 1999, 29, 1744–1758. [Google Scholar] [CrossRef]
- Killworth, P.D.; Blundell, J.R.; Dewar, W.K. Primitive Equation Instability of Wide Oceanic Rings. Part I: Linear Theory. J. Phys. Oceanogr. 1997, 27, 941–962. [Google Scholar] [CrossRef]
- Lipphardt, B.; Poje, A.; Kirwan, A.; Kantha, L.; Zweng, M. Death of three Loop Current rings. J. Mar. Res. 2008, 66, 25–60. [Google Scholar] [CrossRef]
- Thomas, L.N.; Taylor, J.R.; Ferrari, R.; Joyce, T.M. Symmetric instability in the Gulf Stream. Deep Sea Res. Part II Top. Stud. Oceanogr. 2013, 91, 96–110. [Google Scholar] [CrossRef]
- de Marez, C.; Meunier, T.; Morvan, M.; L’Hégaret, P.; Carton, X. Study of the stability of a large realistic cyclonic eddy. Ocean Model. 2020, 146, 101540. [Google Scholar] [CrossRef]
- Brannigan, L. Intense submesoscale upwelling in anticyclonic eddies. Geophys. Res. Lett. 2016, 43, 3360–3369. [Google Scholar] [CrossRef] [Green Version]
- Buckingham, C.E.; Gula, J.; Carton, X. The Role of Curvature in Modifying Frontal Instabilities. Part I: Review of Theory and Presentation of a Nondimensional Instability Criterion. J. Phys. Oceanogr. 2021, 51, 299–315. [Google Scholar] [CrossRef]
- Buckingham, C.E.; Gula, J.; Carton, X. The role of curvature in modifying frontal instabilities. Part II: Application of the criterion to curved density fronts at low Richardson numbers. J. Phys. Oceanogr. 2021, 51, 317–341. [Google Scholar] [CrossRef]



Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Meunier, T.; Pallás Sanz, E.; de Marez, C.; Pérez, J.; Tenreiro, M.; Ruiz Angulo, A.; Bower, A. The Dynamical Structure of a Warm Core Ring as Inferred from Glider Observations and Along-Track Altimetry. Remote Sens. 2021, 13, 2456. https://doi.org/10.3390/rs13132456
Meunier T, Pallás Sanz E, de Marez C, Pérez J, Tenreiro M, Ruiz Angulo A, Bower A. The Dynamical Structure of a Warm Core Ring as Inferred from Glider Observations and Along-Track Altimetry. Remote Sensing. 2021; 13(13):2456. https://doi.org/10.3390/rs13132456
Chicago/Turabian StyleMeunier, Thomas, Enric Pallás Sanz, Charly de Marez, Juan Pérez, Miguel Tenreiro, Angel Ruiz Angulo, and Amy Bower. 2021. "The Dynamical Structure of a Warm Core Ring as Inferred from Glider Observations and Along-Track Altimetry" Remote Sensing 13, no. 13: 2456. https://doi.org/10.3390/rs13132456
APA StyleMeunier, T., Pallás Sanz, E., de Marez, C., Pérez, J., Tenreiro, M., Ruiz Angulo, A., & Bower, A. (2021). The Dynamical Structure of a Warm Core Ring as Inferred from Glider Observations and Along-Track Altimetry. Remote Sensing, 13(13), 2456. https://doi.org/10.3390/rs13132456