Photometric Correction of Chang’E-1 Interference Imaging Spectrometer’s (IIM) Limited Observing Geometries Data with Hapke Model
Abstract
1. Introduction
2. Methods
3. Data Set
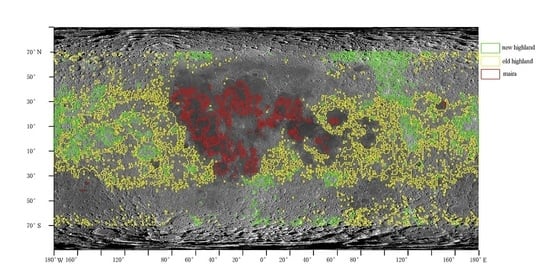
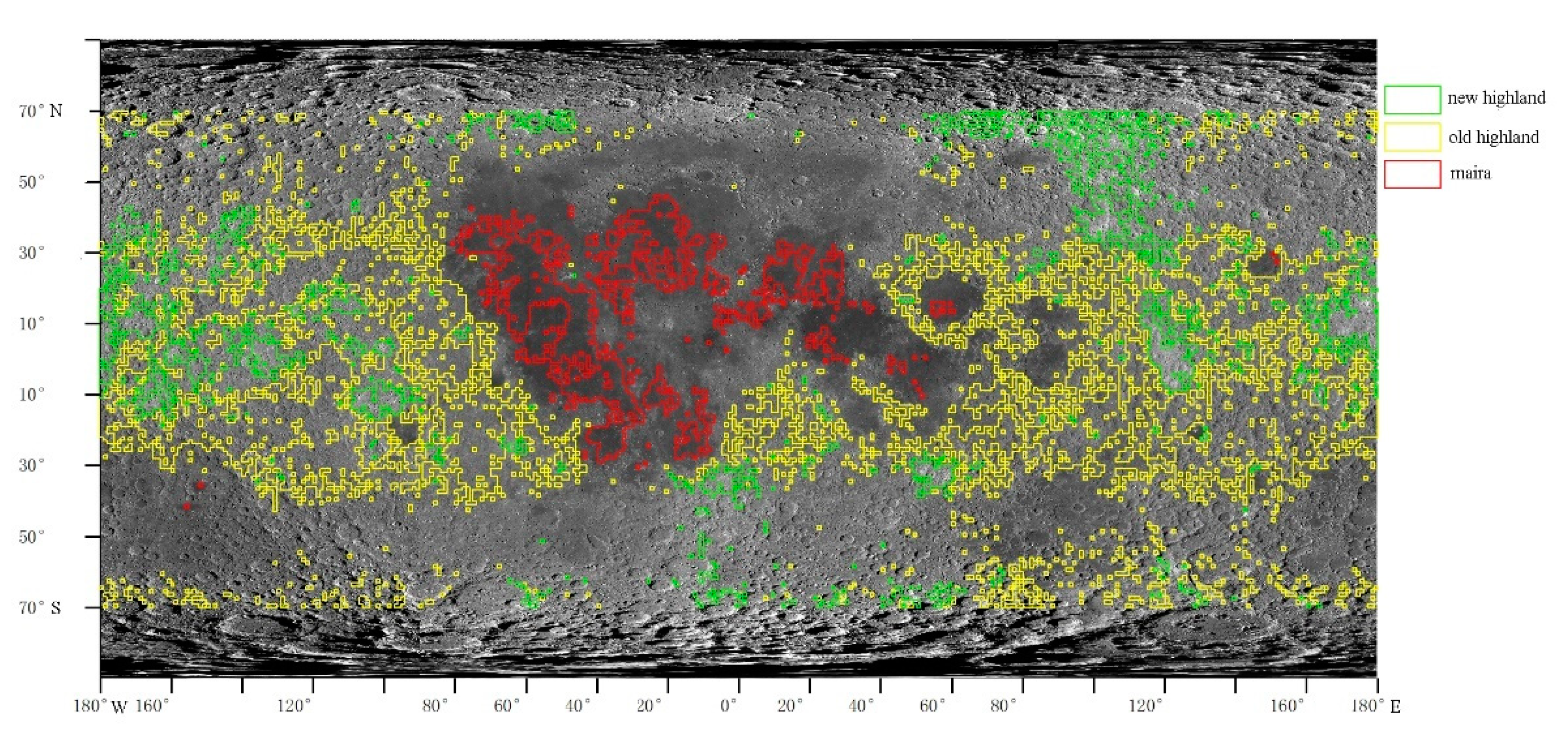
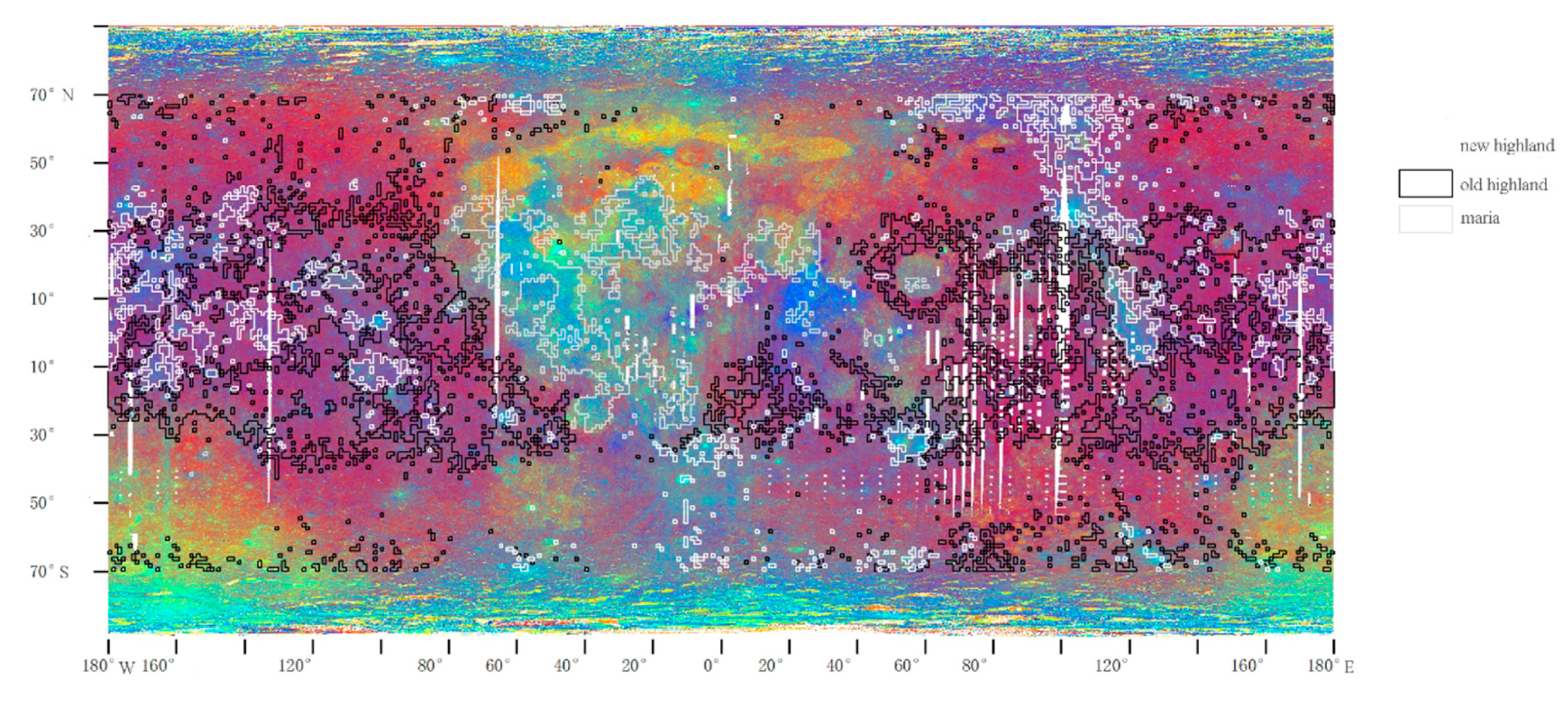
3.1. Sample Data
3.2. Preprocessing of the Sample Data
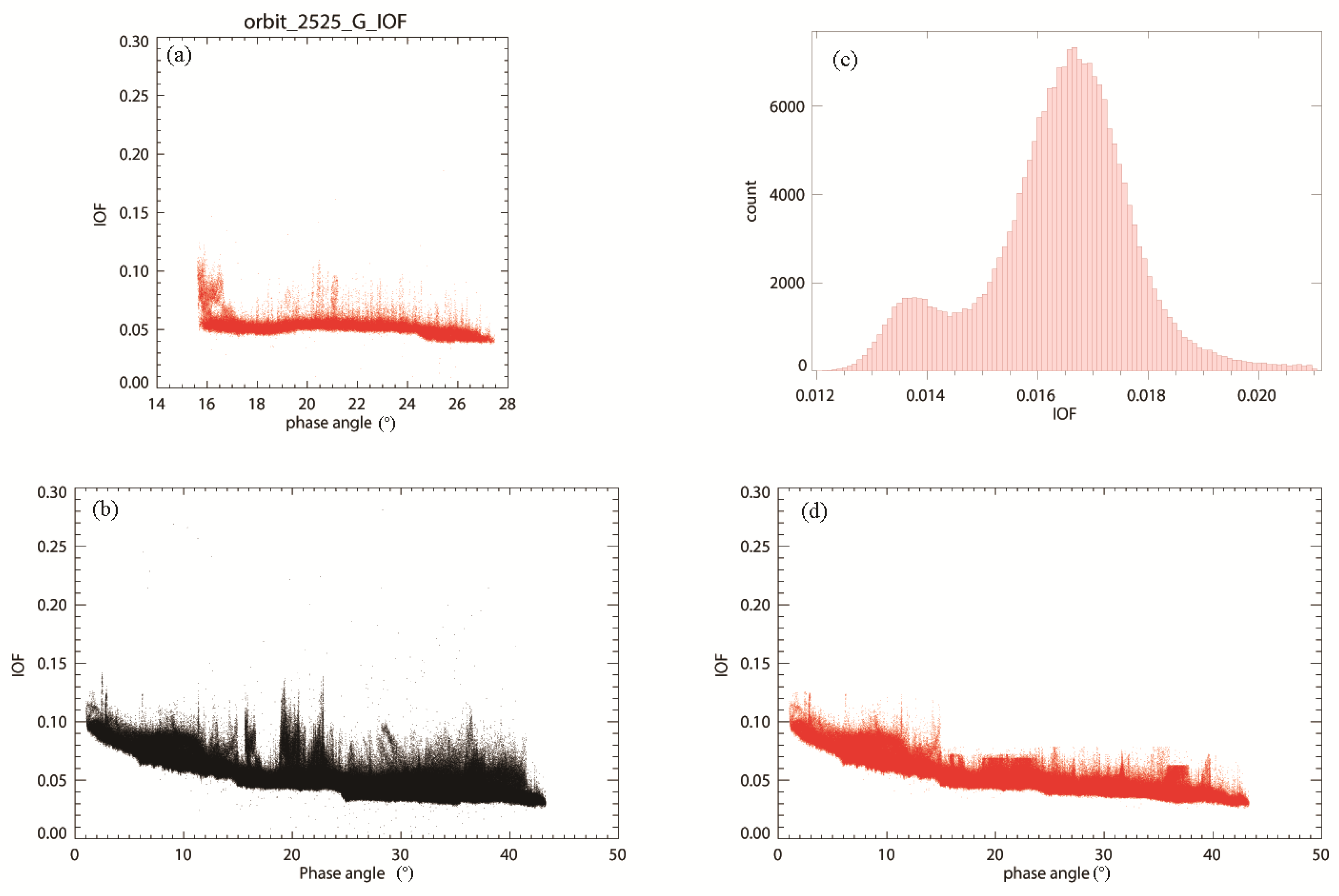
4. Results and Discussion
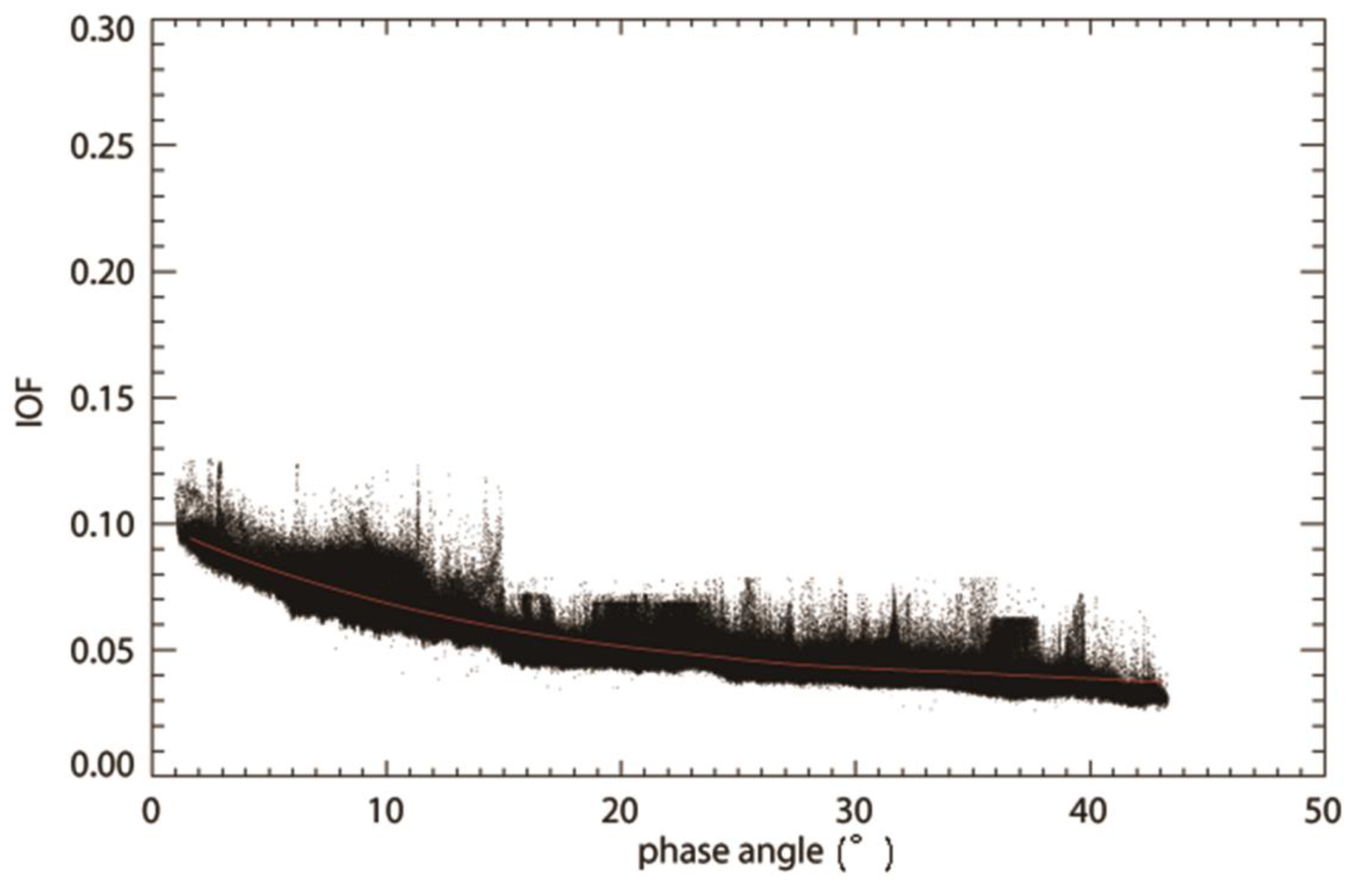
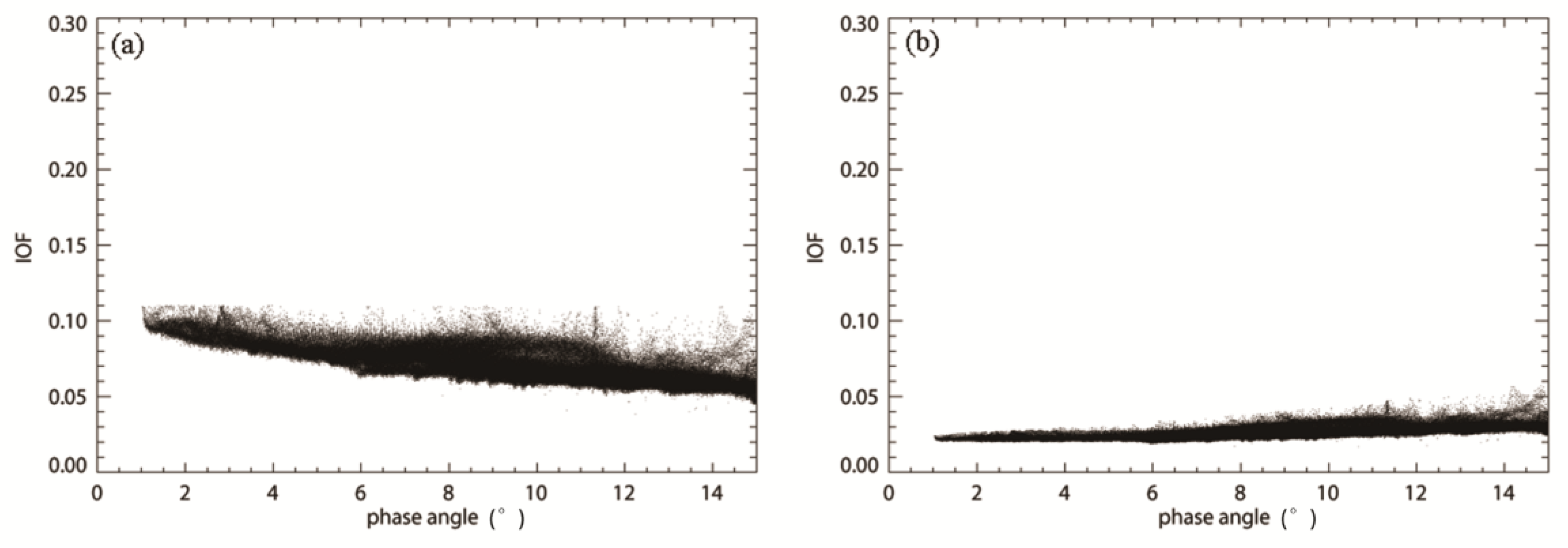
4.1. Photometric Characteristics of the Three Areas
4.2. Hapke Model Parameters Calculation
4.3. Photometric Correction Results of the Reflectance Data
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Lucey, P.G. Model near-infrared optical constants of olivine and pyroxene as a function of iron content. J. Geophys. Res. Space Phys. 1998, 103, 1703–1713. [Google Scholar] [CrossRef]
- Tompkins, S.; Pieters, C.M. Mineralogy of the lunar crust: Results from Clementine. Meteorit. Planet. Sci. 1999, 34, 25–41. [Google Scholar] [CrossRef]
- Garrick-Bethell, I.; Zuber, M.T. Elliptical structure of the lunar South Pole-Aitken basin. Icarus 2009, 204, 399–408. [Google Scholar] [CrossRef]
- McEwen, A.; Eliason, E.; Lucey, P. Summary of Radiometric Calibration and Photometric Normalization Steps for the Clementine UVVIS images. Lunar Planet. Sci. Conf. 1998, XXIX, 1466. [Google Scholar]
- Yokota, Y.; Matsunaga, T.; Ohtake, M. Lunar Photometric Properties at Wavelengths 0.5–1.6 μm Acquired by SELENE Spectral Profiler and Their Dependency on Local Albedo and Latitudinal Zones. Icarus 2011, 215, 639–660. [Google Scholar] [CrossRef]
- Besse, S.; Boardman, J.; Nettles, J.; Staid, M.; Sunshine, J.M.; Li, J.Y.; Yokota, Y.; Buratti, B.; Hicks, M.; Pieters, C. Deriving a photometric model for the Moon Mineralogy Mapper data (M3). Lunar Planet. Sci. Conf. 2011, 42, 1773. [Google Scholar]
- Sato, H.; Robinson, M.; Hapke, B.; Denevi, B.W.; Boyd, A.K. Resolved Hapke parameter maps of the Moon. J. Geophys. Res. Planets 2014, 119, 1775–1805. [Google Scholar] [CrossRef]
- Wu, Y.; Besse, S.; Li, J.-Y.; Combe, J.-P.; Wang, Z.; Zhou, X.; Wang, C. Photometric correction and in-flight calibration of Chang’E-1 Interference Imaging Spectrometer (IIM) data. Icarus 2013, 222, 283–295. [Google Scholar] [CrossRef]
- Xu, X.-S.; Liu, J.-J. Hapke’s parameters inverse method of Lunar analog sample. J. Infrared Millim. Waves 2018, 37, 227–234. [Google Scholar]
- Hapke, B. Bidirectional reflectance spectroscopy: 1. Theory. J. Geophys. Res. Space Phys. 1981, 86, 3039–3054. [Google Scholar] [CrossRef]
- Hapke, B.; Wells, E. Bidirectional reflectance spectroscopy: 2. Experiments and observations. J. Geophys. Res. Space Phys. 1981, 86, 3055–3060. [Google Scholar] [CrossRef]
- Hapke, B. Bidirectional reflectance spectroscopy: 3. Correction for macroscopic roughness. Icarus 1984, 59, 41–59. [Google Scholar] [CrossRef]
- Hapke, B. Bidirectional reflectance spectroscopy: 4. The extinction coefficient and the opposition effect. Icarus 1986, 67, 264–280. [Google Scholar] [CrossRef]
- Hapkea, B.; Nelson, R.M.; Smythe, W.D. The Opposition Effect of the Moon: Coherent Backscatter and Shadow Hiding. Icarus 1998, 133, 89–97. [Google Scholar] [CrossRef]
- Hapke, B. Bidirectional reflectance spectroscopy: 5. The coherent backscatter opposition effect and anisotropic scattering. Icarus 2002, 157, 523–534. [Google Scholar] [CrossRef]
- Hapke, B. Bidirectional reflectance spectroscopy: 6. Effects of porosity. Icarus 2008, 195, 918–926. [Google Scholar] [CrossRef]
- Hapke, B. Bidirectional reflectance spectroscopy: 7. The single particle phase function hockey stick relation. Icarus 2012, 221, 1079–1083. [Google Scholar] [CrossRef]
- Hapke, B. Theory of Reflectance and Emittance Spectroscopy; Cambridge University Press: Cambridge, UK, 2012. [Google Scholar]
- Sun, L.; Ling, Z.; Zhang, J.; Li, B.; Chen, J.; Wu, Z.; Liu, J. Lunar iron and optical maturity mapping: Results from partial least squares modeling of Chang’E-1 IIM data. Icarus 2016, 280, 183–198. [Google Scholar] [CrossRef]
- Li, C.-L.; Mu, L.-L.; Zou, X.-D.; Liu, J.-J.; Ren, X.; Zeng, X.-G.; Yang, Y.; Zhang, Z.-B.; Liu, Y.-X.; Zuo, W.; et al. Analysis of the geomorphology surrounding the Chang’e-3 landing site. Res. Astron. Astrophys. 2014, 14, 1514–1529. [Google Scholar] [CrossRef]
- Helfenstein, P. Photometrically determined surface physical properties of terrains on Ganymede. Lunar Planet. Sci. 1986, 28, 333–334. [Google Scholar]
- Mustard, J.F.; Pieters, C.M. Photometric phase functions of common geologic minerals and applications to quantitative analysis of mineral mixture reflectance spectra. J. Geophys. Res. Space Phys. 1989, 94, 13619–13634. [Google Scholar] [CrossRef]
- Hapke, B.; Denevi, B.W.; Sato, H.; Braden, S.E.; Robinson, M.S. The wavelength dependence of the lunar phase curve as seen by the Lunar Reconnaissance Orbiter wide-angle camera. J. Geophys. Res. Space Phys. 2012, 117. [Google Scholar] [CrossRef]
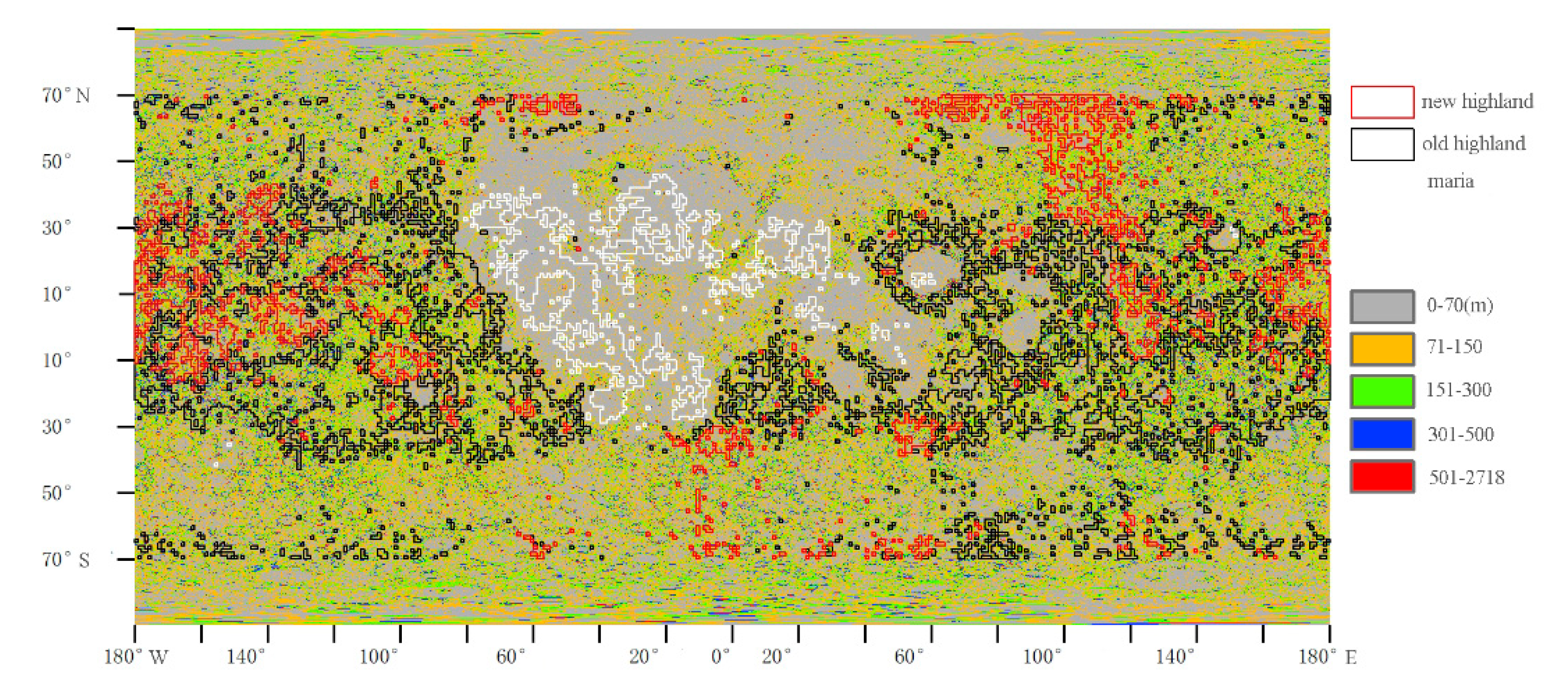
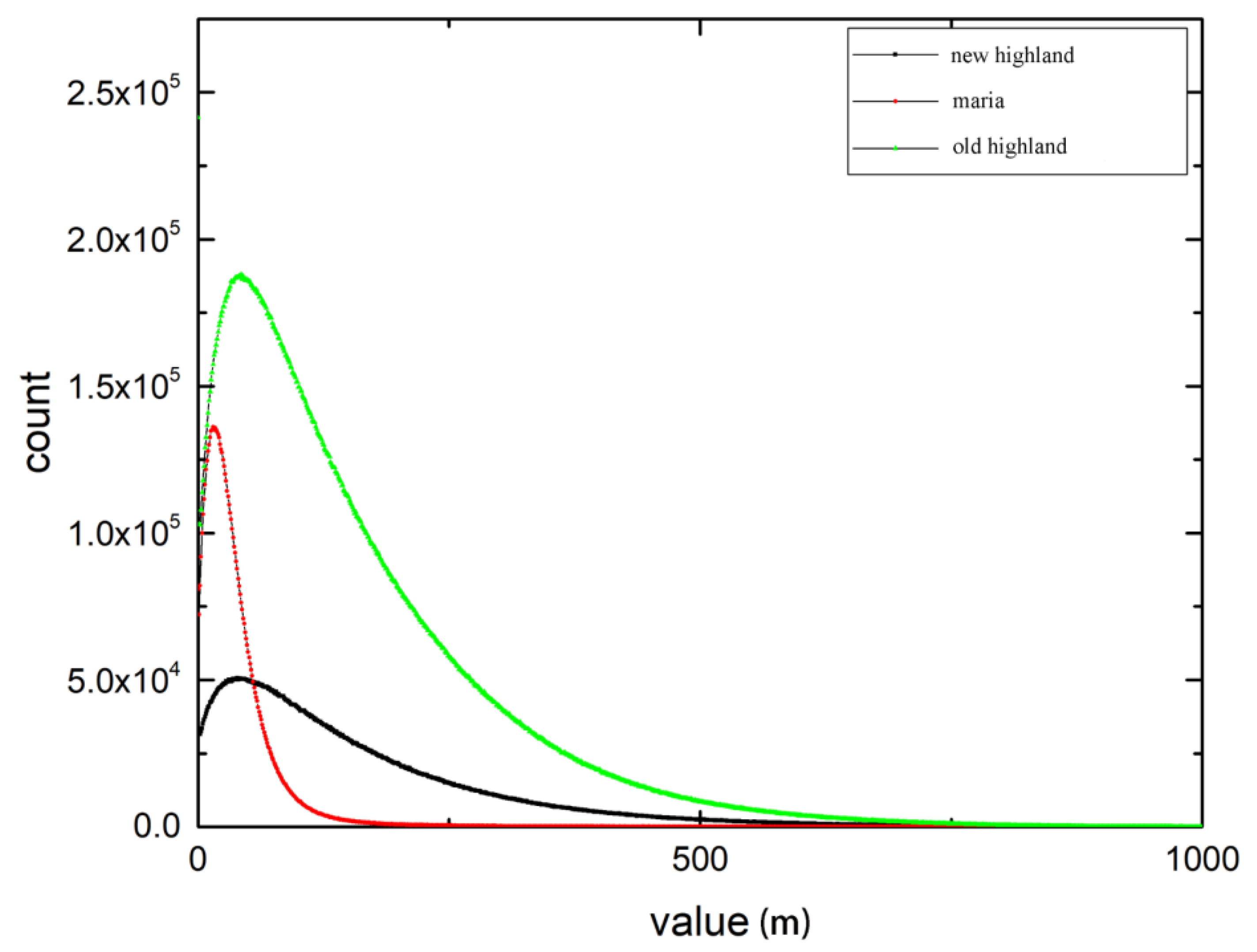

| Area | Parameter | |||
|---|---|---|---|---|
| Maria | <0.29 | >0.259 | >1.9 | >0.0558 |
| New highland | (0.38, 0.475) | (0.232, 0.255) | (1.5867, 1.72235) | >0.0626 |
| Old highland | >0.48 | <0.232 | <1.5867 | >0.0626 |
| Orbit | Start Latitude | End Latitude | Start Line | End Line |
|---|---|---|---|---|
| 2525 | 28 | 16 | 7300 | 9000 |
| 2568 | 44 | 29 | 5300 | 7590 |
| 2576 | 0 | −11 | 11,860 | 13,580 |
| 2576 | −17 | −26 | 14,540 | 15,870 |
| 2600 | 33 | 7 | 6624 | 10,610 |
| 2611 | 38 | 30 | 6147 | 7374 |
| 2845 | 30 | 17 | 6700 | 8600 |
| 2894 | 19 | −11 | 8340 | 12,960 |
| 2914 | 25 | 19 | 7392 | 8320 |
| Symbols | Name | Value |
|---|---|---|
| Single scattering albedo | Fitting | |
| Henyey-Greenstein double-lobed single particle phase function parameter | Fitting | |
| Henyey-Greenstein double-lobed single particle phase function parameter | Equation (8) | |
| Amplitude of Coherent Backscatter Opposition Effect (CBOE) | 0 | |
| Angular width of CBOE | 1.0 | |
| Amplitude of Shadow Hiding Opposition Effect (SHOE) | Fitting | |
| Angular width of SHOE | Fitting | |
| Effective value of the photometric roughness | 0 | |
| Filling factor | 0 |
| original parameter ranges | <0.29 | >0.259 | >1.9 | >0.0558 |
| results | 0.275988 | 0.700692 | 1.38499 | 0.0754915 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xu, X.; Liu, J.; Liu, D.; Liu, B.; Shu, R. Photometric Correction of Chang’E-1 Interference Imaging Spectrometer’s (IIM) Limited Observing Geometries Data with Hapke Model. Remote Sens. 2020, 12, 3676. https://doi.org/10.3390/rs12223676
Xu X, Liu J, Liu D, Liu B, Shu R. Photometric Correction of Chang’E-1 Interference Imaging Spectrometer’s (IIM) Limited Observing Geometries Data with Hapke Model. Remote Sensing. 2020; 12(22):3676. https://doi.org/10.3390/rs12223676
Chicago/Turabian StyleXu, Xuesen, Jianjun Liu, Dawei Liu, Bin Liu, and Rong Shu. 2020. "Photometric Correction of Chang’E-1 Interference Imaging Spectrometer’s (IIM) Limited Observing Geometries Data with Hapke Model" Remote Sensing 12, no. 22: 3676. https://doi.org/10.3390/rs12223676
APA StyleXu, X., Liu, J., Liu, D., Liu, B., & Shu, R. (2020). Photometric Correction of Chang’E-1 Interference Imaging Spectrometer’s (IIM) Limited Observing Geometries Data with Hapke Model. Remote Sensing, 12(22), 3676. https://doi.org/10.3390/rs12223676