Abstract
The construction of bridges has been necessary for societies since ancient times, when the communication between and within towns, cities or communities was established. Until recently, the economic factor has been the only one considered in the decision-making of any type of construction process for bridges. However, nowadays, the objective should not be just the construction of bridges, but of sustainable bridges. Economic, social and environmental factors, which form the three pillars of sustainability, have been recently added. These three factors usually have conflicting perspectives. The decision-making process allows the conversion of a judgment into a rational procedure to reach a compromise solution. The aim of this paper is to review different methods and sustainable criteria used for decision-making at each life-cycle phase of a bridge, from design to recycling or demolition. This paper examines 77 journal articles for which different methods have been used. The most used methods are briefly described. In addition, a statistical study was carried out on the Multiple Attribute Decision-making papers reviewed.
1. Introduction
Bridges are major components of territorial communication in a society. This is why guaranteeing a sustainable structure is essential to provide safety and service to users. The sustainable development of bridges is mainly based on meeting the three pillars of sustainability—economic, social and environmental factors—which each have different goals. The economic and environmental factors have been widely studied, and even, in some cases, a specific software has been developed to assess the environmental impacts of some structures []. Despite the fact that some authors have carried out studies to evaluate social sustainability throughout the life-cycle of an infrastructure [,], there is still limited knowledge on social factors affecting structures’ sustainability, including bridges. Thus, to achieve a consensus among these three pillars, one must resort to a process, such as decision-making. This process facilitates the rational selection of a bridge solution based on certain information and judgment about the criteria chosen for a life-cycle phase. Wass et al. [] stated that the sustainable development must be considered as a decision-making strategy.
Balali et al. [] pointed out that decision-supporting systems applied to different steps of bridge life-cycles can be categorized as (a) planning and design; (b) construction; and (c) operation and maintenance. These stages are also considered by other authors [,]. Our paper reviews these phases and incorporates the last life-cycle phase of a bridge. This phase can be either the demolition or the recycling of the structure. Decision-making on the last phase is necessary since the corresponding transport of materials has an impact in terms of the three pillars of sustainability.
The decision-making process can be done by applying different methods and tools, as well as using different objectives. Hwang and Yoon [] divided the Multi-Criteria Decision-Making (MCDM) processes into Multiple Attribute Decision-making (MADM) and Multiple Objective Decision-making (MODM). MADM is used to evaluate discrete variables. In addition, this is an a priori process. Experts take part in the initial stage of the process, giving the weightings of the criteria or assessing any attribute of the bridge. Finally, the best solution or a solution ranking is obtained. MODM allows for the obtainment of a continuous set of solutions regarding two or more criteria, called Pareto front. These solutions are characterized by each being considered equally good. The experts also take part in the end stage of the process, choosing one among the many solutions. Therefore, this process is a posteriori. Most of these traditional methods have limitations, and they are not enough to solve real problems []. Achieving a sustainable bridge is a very complicated problem that involves a lot of information and different points of view. Thus, decision-making should take into account the complexity of the real world. New trends and concepts of hybrid MCDM models can solve these limitations [,].
The main aim of this review is to classify and analyze the MADM and sustainable criteria applied to bridges and show a sample of MODM studies to point out the differences between these two processes. The review is carried out for each phase of the bridge’s life-cycle. Also, this review includes studies that do not carry out direct decision-making, but conduct a breakdown of criteria and a subsequent evaluation of the condition, risk or state of the bridge or any part of it. This is important because these results can be used for assessing the sustainable maintenance and rehabilitation of the bridge. In addition, the limitations of traditional MCDM methods and new trends in decision-making are described.
First, traditional MADM methods are classified into different groups and an outline of the properties of these groups is carried out. The most used methods in sustainable bridge works are briefly described. The least used methods are grouped into “Others”. A brief description of MODM is made. Then, the limitations of traditional MCDM methods are explained. The studies are ordered according to life-cycle phases. In each phase, a description of the most outstanding MADM is conducted. Then, all MADM investigations are summarized in a table, which shows the methods and criteria in chronological order. In this way, it is possible to see the most used criteria to assess the sustainability at each phase of the bridge life-cycle. After, a statistical study is carried out with the aim of showing the interactions between the multi-attribute methods and the bridge life-cycle phases. Finally, new trends in MCDM are exposed.
To conduct the review, a total of 77 studies were collected that focused solely on bridge MADM. The observation period ranged from 1991 to 2016. The planning and design phase includes 15 (19.48%) studies, construction included seven studies (9.09%), operation and maintenance contained 53 studies (68.83%) and recycle or demolition had two studies (2.6%). The final phase has only previously been studied by two different authors, indicating a gap in research.
2. Multi-Criteria Decision-Making
2.1. Multi-Attribute Decision-Making
There are many methods and tools that can be used for MADM. Despite the large number of traditional MADM methods [], none is perfect. Most of them make unrealistic assumptions hardly applicable to the real world. However, the traditional MADM methods can be classified into different groups according to similar characteristics [,]. Table 1 shows an outline of traditional MADM.

Table 1.
Multi-Attribute Decision-Making (MADM) methods description.
The scoring methods are the simplest MADM methods. Their basis consists of assessing the alternatives using basic arithmetical operations. The Simple Additive Weighting (SAW) and the Complex Proportional Assessment (COPRAS) methods obtain the sum of the weighted normalized values of all the criteria. SAW is the oldest MADM method and allows the consideration of maximizing criteria. COPRAS is an evolution of SAW. The difference between both is that COPRAS allows the consideration of maximizing and minimizing criteria.
The basic principle of the distance-based method is obtaining the distance among each alternative and a specific point. Within this group, there are two different philosophies. The objective of the Goal Programming (GP) method is to obtain the alternative that satisfies a set of goals. The objective of the Compromise Programming (CP) method is to get the closer alternative to the hypothetical best alternative. While the Data Envelopment Analysis (DEA) method comes from GP, CP is the basis for the Multicriteria Optimization and Compromise Solution (VIKOR) and the Technique for Order of Preference by Similarity to Ideal Solution (TOPSIS) methods.
The pairwise comparison methods are very useful to obtain the weight of the different criteria and compare alternatives with respect to a subjective criterion. The problem of these methods is that they are only based on the knowledge of the decision makers. Furthermore, it is possible that different decision makers have different points of view to the same problem. The Analytic Hierarchy Process (AHP) was the first pairwise method presented and one of the most used in decision-making problems. The Analytic Network Process (ANP) is a method that tries to solve the problem of the independence of the criteria of the AHP. The Measuring Attractiveness by a Categorical Based Evaluation Technique (MACBETH) is an alternative to AHP.
The outranking methods consist of establishing a preference relation on a set of alternatives that indicates the degree of dominance among them. These methods can deal with unclear and incomplete information and their application results in the partial preference ranking of alternatives, instead of a cardinal measure of their preference relation.
The utility/value methods define expressions that determine the degree of satisfaction of the criteria. These functions convert the ratings that define the behavior of the alternatives in relation to the criteria into their degree of satisfaction according to the method (MAUT or MAVT). The expression of the function can model different shapes to relate the ratings and the degree of satisfaction.
This paper reviews the most important methods (Table 1) and tools, and the MADM methods and tools used for decision-making at each life-cycle phase of a bridge are: Complex Proportional Assessment (COPRAS), Technique for Order of Preference by Similarity to Ideal Solution (TOPSIS), Data Envelopment Analysis (DEA), Analytical Hierarchy Process (AHP), Analytical Network Process (ANP), Preference Ranking Organization Method for Enrichment Evaluations (PROMETHEE), Quality Function Deployment (QFD), Monte Carlo Simulation (MCS), Delphi, Fuzzy logic and Grey numbers (G).
2.1.1. Methods
Complex Proportional Assessment
The Complex Proportional Assessment (COPRAS) method was developed by Zavadskas and Kaklauskas in 1996 []. It expresses the criteria values in intervals based on real conditions and allows for the evaluation of the uncertainty value of criteria. The method uses a stepwise ranking and assessing procedure of the different alternatives in terms of significance and utility degree []. Recently, Liou et al. [] proposed a new hybrid COPRAS model for improving and selecting suppliers in green supply chain management.
Technique for Order of Preference by Similarity to Ideal Solution
The Technique for Order of Preference by Similarity to Ideal Solution (TOPSIS) is a method proposed by Hwang and Yoon in 1981 []. It is based on the concept that the best alternative is the one that simultaneously has the shortest geometric distance from the positive ideal solution and the longest geometric distance to the negative ideal solution. The ideal alternative takes the best score of each criterion. It is a method of compensatory aggregation that compares a set of alternatives by identifying weights for each criterion, normalizing their scores and calculating the geometric distance between each alternative and the ideal alternative.
TOPSIS assesses situations where the criteria values and the criteria weights are expressed in exact and non-exact numerical values. The positive ideal solution maximizes the benefit criteria and minimizes the disadvantage criteria, whereas the negative ideal solution maximizes the disadvantage criteria and minimizes the benefit criteria [,,].
Data Envelopment Analysis
The Data Envelopment Analysis (DEA) is a tool developed by Charnes et al. in 1978 [], built to measure the relative performance of different units when multiple inputs and outputs make comparisons difficult. DEA allows for the comparison of the relative management of a group of inputs to produce the same group of outputs.
The methodology identifies boundaries and allows for the location of relative management indicators for each unit in relation to those that are on the efficient boundary. In addition, this method allows for the identification of and assessment of inefficiencies in relation to input and output, providing guidelines for improving the units analyzed.
Analytic Hierarchy Process
The Analytic Hierarchy Process (AHP) is a method proposed by Saaty in 1980 [] to support multi-criteria decision-making. In the first step, the method decomposes the problem into a hierarchical structure. Based on this structure, experts assess the relative importance of decision criteria and use pairwise comparisons that are assigned from a pre-determined scale of relative importance. The scale uses values varying from 1 to 9. The weight of each criterion at the same level can be evaluated by calculating the eigenvector of the matrix [].
The comparison of criteria is performed for each level, obtaining the weight of each criterion. Assessing the bottom level criteria and multiplying by each weight allows for the assessment of the next criterion level. The process is repeated until the overall decision goal, which is at the top level, is reached [,,,,,]. The AHP assumes the independence of all criteria (outer and inner dimensions), which can be an unrealistic assumption for solving actual real-world problems [].
Analytic Network Process
Saaty and Takizawa introduced the Analytic Network Process (ANP) in 1986 [] to solve dependence and feedback problems between dimensions and criteria in diagonal matrices under the hypothesis that they are independent or show self-relation. The ANP is a general form of the AHP which can release the restriction of hierarchical structure, and is recommended for decision-making problems where there are cross relations in outer dimensions. In fact, the greatest difference between the two methodologies is that ANP is applied to decision-making problems for interrelationships in outer dimensions, whereas the AHP assumes the independence of outer and inner dimensions. As Lu et al. [] claim, the outcome of the decisions may be influenced if there exists a significant interrelationship between dimensions and criteria that is not considered. On the other hand, ANP allows for a representation of decision-making in a complex environment with a network structure rather than with a hierarchical structure [,].
Preference Ranking Organization Method for Enrichment Evaluations
The Preference Ranking Organization Method for Enrichment Evaluations (PROMETHEE) is a method developed by Brans et al. [] in 1984. It is based on the pairwise comparison between alternatives for creating an outranked relationship to display the degree of domination of one alternative over the other and to select among conflicting criteria. This method uses positive and negative assessments for each alternative to create a ranking in relation to decision weights. This method allows for direct operation on the criteria included in the decision matrix without normalization and it obtains a suitable solution even when information is missing [,].
Quality Function Deployment
The Quality Function Deployment (QFD) was first introduced in 1983 by Kogure and Akao []. Although initially conceived as a tool for design and product development, today it can be considered as an important tool in the field of multi-criteria decision problems [,].
2.1.2. Tools
Monte Carlo Simulation
The Monte Carlo Simulation (MCS) is a statistical method that allows for the application of solutions to hard mathematical problems. This method generates random values according to a probability function and is used to address the uncertainty and vagueness assessment of qualitative criteria.
Delphi
Delphi, first introduced in 1963 by Dalkey and Helmer [], is a tool that approaches expert opinions by minimizing the existing uncertainty of the qualitative criteria assessment. This tool consists of making questionnaires and interviews at different phases. First, each expert assesses the criteria; then, if the experts’ opinions are different, a second round of questionnaires and interviews is performed showing the first rounds’ results. In this way, the experts can assess the criteria another time, but with an aiding orientation. This process is performed until a consensus is reached.
Fuzzy
Fuzzy was first formalized by Zadeh in 1965 [] as a way of representing uncertainty or vagueness in real life. Fuzzy starts with a set of user-supplied human language rules. The fuzzy systems convert these rules into their mathematical equivalents. This simplifies the job of the system designer and the computer and results in much more accurate representations of the way in which systems behave in the real world.
Grey Numbers
Deng presented grey system theory in 1989 [], and Lin et al. [] introduced the concept of the grey number as a number whose exact value is unknown, but a range within which the value lies is known. There are several types of grey number []: a grey number with only lower limits or upper limits, an interval of grey number with lower and upper limits, a continuous grey number and discrete grey number, and black and white numbers.
2.2. Multi-Objective Decision-Making
The Multi-Objective Decision-making (MODM) methods or tools are used to find multiple trade-off solutions. Zavala et al. [] reviewed the most used multi-objective metaheuristics applied to structural optimization. The heuristic algorithms used most frequently are Genetic Algorithm (GA), Particle Swarm Optimization (PSO), Ant Colony Optimization (ACO), Simulated Annealing (SA) and Harmony Search (HS). These are stochastic methods characterized by combining central rules and randomness.
GA was developed by Holland in the early 1970s [] based on natural selection. In GA, each variable is represented by a gene, a set of variables describing one individual and a set of individuals describing a population. Each individual is reproduced by combining genes of different parents, which is called crossover. Then, the children are introduced into the population and the process is repeated.
PSO and ACO present swarm behavior. The PSO method was proposed by Kennedy and Eberhart []. PSO imitates the social behavior of flocks of birds and insects (particles). The aim is to find the optimal solution from the population of moving particles based on a fitness function. Dorigo et al. [] developed the ACO, which imitates the behavior of ant groups looking for food. Usually, the first group of ants chooses a random trajectory covered by a pheromone trace. The second group follows an individual path, which is adapted from the previous trajectory and their individual behavior.
Kirkpatrick et al. [] presented SA based on the natural phenomenon of crystal formation from masses, which are molten and cooled down under controlled conditions. The SA algorithm generates a new configuration from a previous solution and then accepts or rejects the solution according to a probability function. Geem et al. [] developed the HS. This method tries to imitate the activity of jazz musicians when they are looking for the best harmony. A similar principle is followed when optimizing a specific objective function instead of harmonies.
2.3. Limitations of Traditional MCDM Methods
The traditional MCDM methods have limitations, and they are not enough to resolve real problems. While MADM methods mainly rank a limited set of alternatives and select the best one, the MODM methods obtain a set of efficient solutions in a fixed feasible region that can be represented on a Pareto front. Liou and Tzeng [] stated that normally, MCDM methods consider unrealistic assumptions in the real problems, such as the independence of the criteria, the linear aggregation, or the provision of the best alternative among different alternatives instead of the alternative that reaches the aspiration levels.
Some authors stated that the TOPSIS method cannot be used for ranking and selection purposes. Opricovic and Tzeng [] compared VIKOR and TOPSIS in an illustrative example, and showed that the best alternative is not always the closet to the ideal according to TOPSIS. Furthermore, Wang et al. [] stated that TOPSIS cannot be used for ranking purposes after analyzing the fuzzy TOPSIS proposed by Kuo et al. [].
3. Life-Cycle Steps for Bridge
3.1. Planning and Design
Multi-attribute Decision-making in the planning and design phases of bridges is studied according to the topic of the study: In selecting (1) the bridge site; (2) the type of material; (3) the type of foundation and (4) the type of superstructure.
(1) Ardeshir et al. [] stated that the selection of bridge construction sites over rivers is one of the most important tasks in construction feasibility studies. Ardeshir et al. [] and Aghdaie et al. [] used a modified AHP with an uncertainty tool. The first authors used Fuzzy and the second authors used COPRAS-G to select the best bridge construction site. The AHP method was used to assess the weight of the criteria. Both studies applied economic and area characteristic criteria to select the bridge construction site. However, Aghdaie et al. [] considered social criteria, as the bridge was located in an urban area.
(2) Utomo and Idrus [] and Balali et al. [] applied MADM to select the appropriate material for the bridges. Utomo and Idrus [] presented a model to achieve the most suitable material for a bridge based on the following value analysis function:
They suggested the FAST (Function Analysis System Technique) method to perform the function analysis. In addition, they used AHP to weight each criterion and compare three different types of material. Balali et al. [] applied PROMETHEE to carry out the comparison using a degree of dominance of one alternative over another. This method requires less information compared to others that create a single score. Economic and durability criteria were used by Utomo and Idrus [], and Balali et al. [], with the difference being that Utomo and Idrus [] divided durability criteria in several sub-criteria. Most authors are concerned with sustainability in the design and planning phases of a bridge and focus their efforts on the foundation and superstructure.
(3) Joshi et al. [] studied foundations and employed a Fuzzy tool to evaluate a set of criteria and obtain the most suitable foundation among three different types. Two years later, Ugwu et al. [] used a method called SUSAIP (Sustainability appraisal) to compare two different foundations. They employed several criteria to finally obtain a sustainability index.
(4) On the other hand, one of the first investigations of superstructure type decision-making was carried out by Moore et al. in 1996 [], using only expert opinion to make a decision supporting bridge design. In 1998, Ohkubo et al. [], applied a fuzzy system to approach an optimization of pre-stressed concrete bridges, considering cost and aesthetic feelings. In 2000, Itoh et al. [], included CO2 and energy as environmental criteria for selecting the type of bridge. While Moore et al. [], Ohkubo et al. [], and Itoh et al. [] studied the three pillars of sustainability separately, the following papers encompass multiple criteria to approach the sustainable goal.
Several researches suggested a hybrid AHP, where AHP was used for evaluating the criterion weight and another method or tool (Fuzzy or PROMETHEE) to deal with the potential uncertainty. For example, Wang et al. [] and Jakiet et al. [] proposed a Fuzzy AHP; Gervasio and Simoes Da Silva [] employed a hybrid method between AHP and PROMETHEE; and Farkas [] used AHP supported by seven different experts having varying priorities. Alternatively, other authors used different methods. Malekly et al. [] stated that choosing the most suitable superstructure is vital for the success of a bridge design. To achieve this goal, Malekly et al. [] proposed a hybrid method between QFD and TOPSIS and Balali et al. [] applied the PROMETHEE method.
Table 2 shows the methods used and criteria applied in different studies in chronological order. To differentiate the sub-criteria from the main criteria, the sub-criteria are written between brackets and italics.

Table 2.
Method and criteria of design and planning.
Some authors applied MODM in the planning and design phase because they observed that knowing the trade-off relationships between the economic, environmental and social indicators is the best way to take sustainable decisions. Optimization methods allow for carrying-out of the decision-making of continuous criteria, where a set of valid solutions are obtained and represented on a Pareto front. Some authors carried out a single-objective optimization, in which the objectives are analyzed separately and then compared [,,,,,]. However, others prefer to apply a multi-objective optimization to get a Pareto set of solutions. These solutions are characterized by improving all objectives simultaneously [,,,].
3.2. Construction
El-Diraby and O’Connor [] evaluated bridge construction plans using a method they designed. This investigation focused on urban bridges. Chou et al. [] applied a hierarchical structure Fuzzy AHP. Pan [] suggested three different types of construction methods: full-span, pre-cast and launching, and applied Fuzzy AHP. Then, Mousavi et al. [] proposed a modified type of Fuzzy AHP with the same criteria and type of construction used by Pan. Mousavi et al. [] made a comparison between both methods and stated that the comparison analysis shows that the ranking of the proposed HF-AHP method is similar to the ranking of Pan’s [] method. AHP was used to evaluate factor weights and Fuzzy was used to take into account the uncertainty.
Gu et al. [] used the same criteria as Pan [] and Mousavi et al. []. These were quality, cost, safety, duration and shape. Balali et al. [], in the same paper that applied the PROMETHEE method to make the selections on material and the type of bridge structures, assessed different types of construction methods also using PROMETHEE. Chen et al. [] used PROMETHEE with Fuzzy to rank different types of construction method. Table 3 shows the methods and criteria used in the different investigations on the construction phase.

Table 3.
Method and criteria of construction.
3.3. Operation and Maintenance
Multi-attribute Decision-Making in the operation and maintenance phase must be differentiated into two types. In the first one, the decision-making process is carried out for the purpose of choosing the most suitable and final type of maintenance and/or maintenance time. On the other hand, in the second one, the decision-making process is carried out indirectly. The assessment of the condition, damage, or risk of a structure or a part of it, provides a basis on which decisions on maintenance can be made.
Regarding the direct process, Dabous and Alkass [] evaluated and ranked different bridge rehabilitation strategies using a proposed modified type of AHP with fuzzy logic. Each comparison was carried out with linguistic terms that are delimited between the pessimistic and optimistic values. The most likely value is inside the range. Afterwards, the Monte Carlo tool is used to select random values and generate a comparison matrix. If the matrix is consistent, it is used to obtain the weights, which follow a probability distribution.
Bitarafan et al. [], El-Mikawi et al. [], Sobanjo et al. [], and Dabous and Alkass [] applied an AHP, while the last two studies also considered the existing uncertainty. Sobanejo et al. [] and Dabous and Alkass [] used these hybrid methods to create a ranking method for bridge rehabilitation where more subjective criteria are considered. This is the reason for using an uncertainty tool. Bitafaran et al. [] and El-Mikawi et al. [] used only AHP because their criteria were more objective.
Yehia et al. [] developed a decision support for bridge maintenance through interviews with bridge maintenance experts and national surveys. The method suggests repair and rehabilitation strategies for problems in the concrete bridge deck. Chassiakos et al. [] presented a knowledge-based system used for maintenance planning of highway concrete bridges. To demonstrate the usefulness of the system, the method was applied to assess the different scenarios and choose the most suitable type of maintenance planning.
Sabatino et al. [] used multi attribute utility theory to provide a framework for decision makers to get the optimal life-cycle maintenance actions. The framework is applied to an existing highway bridge to evaluate the consequences of structural failure to the economy, society and environment of different maintenance plans. Table 4 shows the methods and criteria applied in the operation and maintenance phase.

Table 4.
Method and criteria of operation and maintenance.
Within the indirect decision-making process, there are more approaches available. This type of investigation assesses the condition, damage or deterioration, or the risk (wherein seismic risk is the most important) of a bridge or a part of it. Once the time, the state or the risk have been assessed, a decision could be made. Several studies have compiled a rating system for conditions, damages or deteriorations [,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,,] of the bridge, which is the step before decision-making.
Qiao et al. [] evaluated the condition of a cable-stayed bridge. He proposed an uncertain type of AHP, which uses an interval number judgment matrix to reflect the uncertainty in the bridge assessment. The hierarchy process involves different parts of the bridge. First, the weight of each layer is calculated through a pairwise comparison matrix. Then, the parts of the lower layer are assessed and in turn, the general assessment of the bridge can be obtained. Dan et al. [] also assessed a cable-stayed bridge, but used a hybrid Fuzzy AHP method supported by a Health Monitoring System. They developed a model that combines the inspection factors and the data received from sensors. Sasmal and Ramanjaneyulu [] and Dabous and Alkass [] applied the hybrid method Fuzzy AHP to make the condition assessment of the bridge. The process is similar to the previous one. The structure is divided into parts analyzed by experts to obtain a final bridge assessment.
Regarding the other study types, it is worth highlighting risk assessment. If the probability of any kind of failure is known, the expert has the possibility of deciding whether to prevent or correct this type of risk. Some authors define risks as the products of the likelihood and consequences of an occurring event [,,] or as a multiplication function of three parameters: hazard, the probability of disaster occurrence or impact vulnerability of the disaster and the consequences of the disaster []. Wang and Elhag [,], and Wang et al. [] evaluated consequences using four main criteria: safety, functionality, sustainability and environment, which are assessed separately. The methods that Wang et al. applied were Fuzzy [], a hybrid method between AHP and DEA [] and a hybrid method Fuzzy TOPSIS []. Finally, the author achieved a risk propriety ranking for bridge structures. Andric and Lu [] proposed a hybrid method Fuzzy AHP to carry out a risk analysis and assessment of different disaster risks to which the bridge is exposed. He stated that an appropriate bridge safety management plan is required to reduce or prevent the effects of the disasters. Lu et al. [] used the ANP method to provide a model that allows for assessing different risks. He expounded that a comprehensive risk analysis can provide a project contractor with a more rational basis on which to make a decision. The main risk to bridges is seismic activity [,,,]. The authors evaluated this type of risk to provide a tool for assisting with making decisions.
Multi-objective decision-making in the operation and maintenance phase is studied considering serviceability and safety criteria. Liu and Frangopol [,] proposed a multi-objective genetic algorithm to find different maintenance options that present tradeoffs among the lifetime condition, safety level and life-cycle maintenance costs. In addition, Monte Carlo simulations are used to identify the uncertainties of the parameters. Then, Neves et al. [] also incorporated a probabilistic lifetime-oriented multi-objective optimization framework in which the criteria are fully probabilistically described. In this phase, probabilistic frameworks are commonly used (Kim et al. []; Dong et al. []). Frangopol and Soliman [] described the achievements and challenges of each, the life-cycle performance, maintenance, management and optimization of structural systems, taking into account uncertainties.
3.4. Demolition or Recycle
The use of decision-making within the last bridge life-cycle phase—demolition or recycle—has only been investigated by very few authors. However, decision-making in this phase is important too, because this stage has an impact on each of the three pillars of sustainability.
Chen et al. [] used ANP to make a demolition plan where three different options are compared. Itoh [] suggested the recycling of construction materials in order to reduce environmental impacts. Using recycled steel instead of virgin iron extracted from mines results in approximately 60% energy savings and consequently, in a reduction in the environmental impact. Recycling concrete as an aggregate for the production of new concrete requires the use of machines for crushing the concrete, which accounts for around 86% of the environmental impact compared with conventional concrete. Table 5 shows the methods and criteria, which were studied in the final phase.

Table 5.
Method and criteria of the destruction or recycle phase.
4. Discussion
4.1. Overview
The 77 analyzed papers that use MADM were divided into four different phases of the bridge life-cycle (Figure 1). The planning and design phase and the construction phase have 19.48% and 9.09%, respectively. The operation and maintenance phase has 68.83%. This percentage is as high as it is mainly due to two reasons. First, this phase involves more qualitative criteria because there are a lot of visual inspection factors for assessing the condition of a bridge that are difficult to quantify. Therefore, this is a phase that lends itself to being analyzed using MADM. Second, this is the longest phase in a bridge’s life-cycle. While the operation and maintenance duration is commonly defined as 100 years or more, the other phases last less than 5% of the total lifetime. The demolition or recycle phase has only been studied in 2.60% of all papers. This phase was studied less as it is viewed as having a lower overall impact. Still, this phase should be taken into account in the decision-making process because decisions regarding demolition or recycling have to be made. Figure 2 shows the percentage of methods used in the different studies, considering both single and hybrid methods. Note that the methods most used are Fuzzy and AHP. These methods are typically joined together to form the most used hybrid method: Fuzzy AHP.
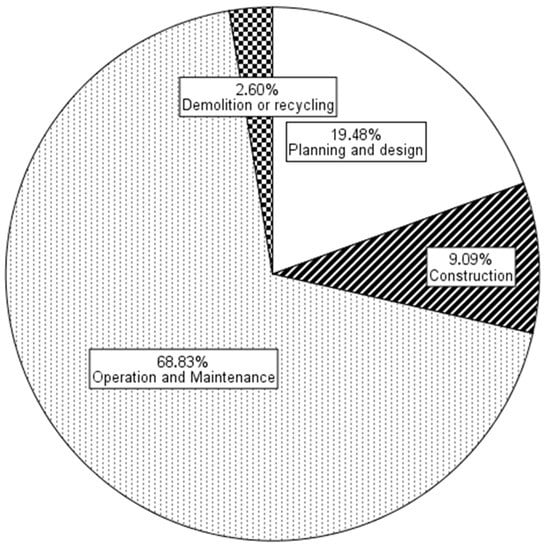
Figure 1.
Percentage frequency for phases.
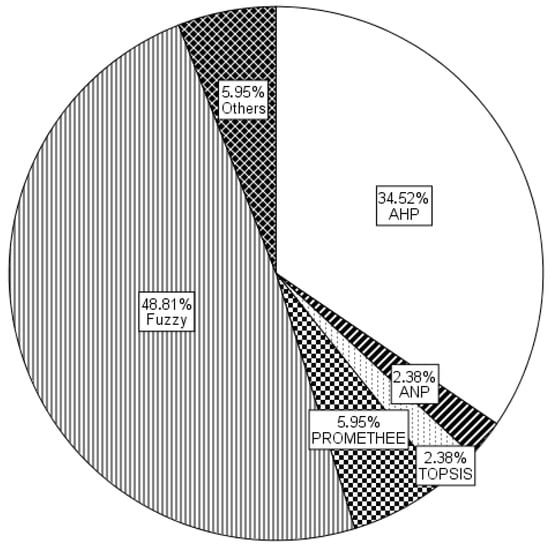
Figure 2.
Percentage frequency for methods.
4.2. Statistical Analysis
A statistical analysis was carried out in order to determine any existing patterns. A simple correspondence analysis is used to analyze inertia and association relations between two variables. The results summarize the information in the rows and columns so that it can be projected on a reduced space and simultaneously represent the row points and the column points. In this way, it can obtain conclusions about the relationships between two variables. To this end, the data was ordered in a table where the rows are the different life-cycle phases, the columns are the different methods used and the cells correspond to the frequencies of use of each method within each life-cycle phase. Once this information was ordered, a simple correspondence analysis was carried out using the IBM SPSS Statistics 22.0 (IBM Corp., Armonk, NY, USA) [] software with the aim of showing the interaction between the methods and the life-cycle phases of a bridge.
Figure 3 shows the interaction between the methods and the life-cycle phases of a bridge. This interaction represents the tendency of a method to be used in a phase; this tendency is greater when the method and life-cycle phase points are closer. Furthermore, a greater distance between the center and the related points implies a greater exclusivity.
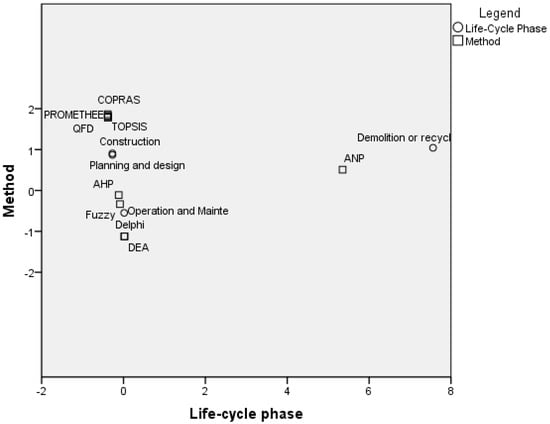
Figure 3.
Row and column point.
A clear relationship between the Fuzzy method and the operation and maintenance phase is indicated in Figure 3. This is because most of the criteria used in this phase are visual and are related to the condition of one part of the bridge or one material; therefore, these are subjective criteria. Thus, Fuzzy is used to include uncertainties and provide greater validity to the final decision. In addition, the AHP method is centered and located in an intermediate position between the design and planning phase, the construction phase and the operation and maintenance phase. Consequently, this method is widely used in these three life-cycle phases. Other methods, such as TOPSIS, PROMETHEE, QFD and COPRAS, are more likely to be used in the design, planning, and operation phases. The ANP method is far from the center and near to the demolition and recycle phase. This shows that in this review, ANP is the only method used in the last bridge life-cycle phase.
5. New Concepts and Trends of MCDM
Section 2, introduces the traditional MCDM methods, and their limitations. Section 3 and Section 4, show that most of the decision-making problems studied in bridges use these traditional methods. Furthermore, these studies usually consider only one stage of the bridge life-cycle. These two points should be considered to define a better process to obtain sustainable bridges. To avoid the unrealistic assumptions of traditional MCDM and resolve complicated problems, Tzeng and Shen [] suggested a new hybrid MCDM comprised of two hybrid MCDM categorized by Hwang and Yoon [] (hybrid MADM and hybrid MODM), and hybrid Multiple Rule/Rough-based Decision-Making (MRDM). Tzeng and Shen [] stated that in hybrid MADM methods (a) the traditional MADM methods normally assume that the criteria are independent, but in real-world problems, there is interdependence and sometimes feedback between the criteria. The DEMATEL (Decision-Making Trial and Evaluation Laboratory) [,,,,,,] technique can build the influential relation matrix to construct the influential network relations map (INRM) and determine the influential weights of DANP (DEMATEL-based ANP) using the basic concepts of ANP. Therefore, the DEMATEL method can be used to solve the inter-dependence and feedback of criteria in the real world; (b) The traditional MCDM methods provide the relative best solution from the fixed set (“max–min” as target) of alternatives to use for ranking and selection, but generally, the real-world problems are assessed by achieving the aspiration levels (“aspiration-worst” as target). The modified MCDM have replaced the traditional MCDM methods as modified VIKOR [,,,], modified GRA (grey relation analysis) [], modified PROMETHEE [] and so on, to obtain the desirable value (aspiration level); (c) MADM methods and tools should be used to compare alternatives and goals or aspiration levels of the criteria, but also, as an improvement, MADM methods can only conduct a single alternative evaluation for building the best improvement strategy plan towards continuous improvement and sustainability to achieve the aspiration level [,] and not only in ranking and selection; (d) The traditional aggregation function model in MADM methods should be changed, including a non-additive (super-additive) approach for solving real-world problems [,]; even fuzzy logic [,] can also be used to aggregate the values of multiple attributes in a non-additive approach.
Based on the above points, new hybrid MODM methods with changeable spaces are proposed to improve the solving of MADM problems. The traditional MODM are used to obtain the Pareto front in a fixed set of conditions. These new MODM models can help decision-makers to reach win–win planning or design, and can achieve the aspiration level [].
MRDM is a new trend that has emerged recently in MCDM. The essential ideas are considered as critical factors or criteria retrieved from historical data, and can induct understandable decision rules in the form of “if…, then…,” logics [].
Achieving a sustainable bridge in the real world is a complicated problem that involves a large number of criteria in different stages of the bridge life-cycle. Most of these criteria have interdependent characteristics or provide feedback, and the feature of the criteria can change throughout bridge life-cycle (e.g., the economy of a country or, the construction of a new bridge near to the old bridge that allows the communication between two sites). Another point to consider is that the decision-making regarding bridges should satisfy decision makers with different points of view. Therefore, while the optimal solution to a problem is very difficult to find out, getting a solution for aspiration levels or goals is a better option. These points make it difficult to solve the problem of obtaining a sustainable bridge using traditional MCDM methods. Thus, decision-making should take into account the complexity of the real world. Some authors [,,,,] use a new hybrid MCDM method (DEMATEL-based ANP with VIKOR) to try to solve the limitations of the traditional MCDM methods and achieve sustainable development strategies in other fields.
6. Conclusions
This paper studied the application of multi-attribute decision analyses on bridges. A total of 77 studies published since 1991 were examined. This investigation showed the use of different methods in the decision-making phases of sustainable bridges. In addition, the differences between multi-attribute and multi-objective decision-making were explained, showing examples of multi-objective decision-making. The criteria and methods applied to each life-cycle phase, as described by the authors, are indicated. Finally, a statistical study was carried out to show trends between the methods and the life-cycle phases.
Authors should select both the correct MCDM method and criteria to achieve a sustainable bridge. On the one hand, MCDM is a powerful process for selecting the most sustainable solution from a wide range of bridge problems. This is done to reach a consensus among economic, social and environmental impacts, which are the three basic pillars of sustainability. Traditional MADM methods often consider unrealistic hypothesis, such as the independence of the criteria, the linear aggregation, or the provision of the best alternative among themselves instead of the alternative of reaching the aspiration levels. New hybrid MCDM methods can solve these limitations. On the other hand, most ancient decision-making works in bridges only consider criteria that involve one or two pillars of sustainability, but some current works already consider criteria of the three pillars of sustainability. This paper reviews the criteria used in each life-cycle bridge phase for each main pillar of sustainability. These criteria are used in each life-cycle bridge phase for each main pillar of sustainability. Therefore, these criteria are a great basis to achieve future sustainable bridge works.
In addition, the statistical study showed the existence of trends between methods and life-cycle phases of a bridge. The most obvious relationship was identified between the Fuzzy method and the operation and maintenance phase. AHP was used in all of the life-cycle phases except for the demolition or recycle phase. The last stage was only used by two authors. Only one of them used ANP, which is the complementary method to AHP.
A future direction of this research field could be the unification of the criteria for decision-making at each life-cycle phase of a bridge. Thus, a comparison of the results of the different studies could be made. In addition, this could provide a systematic process based on factors that impact the designers’ decisions.
Acknowledgments
The authors acknowledge the financial support of the Spanish Ministry of Economy and Competitiveness, along with FEDER funding (BRIDLIFE Project: BIA2014-56574-R). In addition, the authors wish to thanks the comments of Dan M. Frangopol from Center for Advanced Technology for Large Structural Systems.
Author Contributions
This paper represents a result of teamwork. Vicent Penadés-Plà and Víctor Yepes jointly designed the research. Vicent Penadés-Plà drafted the manuscript, Tatiana García-Segura and José V. Martí revised the manuscript. Tatiana García-Segura, José V. Martí and Víctor Yepes edited and improved the manuscript until all authors are satisfied with the final version.
Conflicts of Interest
The authors declare no conflict of interest.
References
- Zastrow, P.; Molina-Moreno, F.; García-Segura, T.; Martí, J.V.; Yepes, V. Life cycle assessment of cost-optimized buttress earth-retaining walls: A parametric study. J. Clean. Prod. 2017, 140, 1037–1048. [Google Scholar] [CrossRef]
- Sierra, L.A.; Pellicer, E.; Yepes, V. Social sustainability in the lifecycle of chilean public infrastructure. J. Constr. Eng. Manag. 2016, 142, 05015020. [Google Scholar] [CrossRef]
- Pellicer, E.; Sierra, L.A.; Yepes, V. Appraisal of infrastructure sustainability by graduate students using an active-learning method. J. Clean. Prod. 2016, 113, 884–896. [Google Scholar] [CrossRef]
- Waas, T.; Hugé, J.; Block, T.; Wright, T.; Benitez-Capistros, F.; Verbruggen, A. Sustainability Assessment and Indicators: Tools in a Decision-Making Strategy for Sustainable Development. Sustainability 2014, 6, 5512–5534. [Google Scholar] [CrossRef]
- Balali, V.; Mottaghi, A.; Shoghli, O.; Golabchi, M. Selection of appropriate material, construction technique, and structural system of bridges by use of multicriteria decision-making method. Transp. Res. Rec. J. Transp. Res. Board 2014, 2431, 79–87. [Google Scholar] [CrossRef]
- Dutil, Y.; Rousse, D.; Quesada, G. Sustainable buildings: An ever evolving target. Sustainability 2011, 3, 443–464. [Google Scholar] [CrossRef]
- Malekly, H.; Meysam Mousavi, S.; Hashemi, H. A fuzzy integrated methodology for evaluating conceptual bridge design. Expert Syst. Appl. 2010, 37, 4910–4920. [Google Scholar] [CrossRef]
- Hwang, C.L.; Yoon, K. Multiple attributes Decision Making: Methods and Applications; Springer: Berlin, Germany, 1981. [Google Scholar]
- Liou, J.J.H.; Tzeng, G.-H. Comments on “Multiple criteria decision making (MCDM) methods in economics: An overview”. Technol. Econ. Dev. Econ. 2012, 18, 672–695. [Google Scholar] [CrossRef]
- Liou, J.J.H. New concepts and trends of MCDM for tomorrow—In honor of Professor Gwo-Hshiung Tzeng on the occasion of his 70th birthday. Technol. Econ. Dev. Econ. 2013, 19, 367–375. [Google Scholar] [CrossRef]
- Tzeng, G.-H.; Shen, K.-Y. New Concepts and Trends of Hybrid Multiple Criteria Decision Making; CRC Press; Taylor and Francis Group: Boca Raton, FL, USA, 2017. [Google Scholar]
- Tzeng, G.-H.; Huang, J.-J. Multiple Attribute Decision Making: Methods and Applications; CRC Press; Taylor and Francis Group: Boca Raton, FL, USA, 2011. [Google Scholar]
- Hajkowicz, S.; Collins, K. A review of multiple criteria analysis for water resource planning and management. Water Resour. Manag. 2007, 21, 1553–1566. [Google Scholar] [CrossRef]
- De Brito, M.M.; Evers, M. Multi-criteria decision-making for flood risk management: A survey of the current state of the art. Nat. Hazards Earth Syst. Sci. 2016, 16, 1019–1033. [Google Scholar] [CrossRef]
- Podvezko, V. The Comparative Analysis of MCDA Methods SAW and COPRAS. Eng. Econ. 2011, 22, 134–146. [Google Scholar] [CrossRef]
- Tamiz, M.; Jones, D.; Romero, C. Goal programming for decision making: An overview of the current state-of-the-art. Eur. J. Oper. Res. 1998, 111, 569–581. [Google Scholar] [CrossRef]
- Ballestero, E. Compromise programming: A utility-based linear-quadratic composite metric from the trade-off between achievement and balanced (non-corner) solutions. Eur. J. Oper. Res. 2007, 182, 1369–1382. [Google Scholar] [CrossRef]
- Opricovic, S.; Tzeng, G.H. Compromise solution by MCDM methods: A comparative analysis of VIKOR and TOPSIS. Eur. J. Oper. Res. 2004, 156, 445–455. [Google Scholar] [CrossRef]
- Podinovski, V.V. Optimal weights in DEA models with weight restrictions. Eur. J. Oper. Res. 2016, 254, 916–924. [Google Scholar] [CrossRef]
- Görener, A. Comparing AHP and ANP: An application of strategic decisions making in a manufacturing company. Int. J. Bus. Soc. Sci. 2012, 3, 194–208. [Google Scholar]
- Bana e Costa, C.A.; Chagas, M.P. A career choice problem: An example of how to use MACBETH to build a quantitative value model based on qualitative value judgments. Eur. J. Oper. Res. 2004, 153, 323–331. [Google Scholar] [CrossRef]
- Behzadian, M.; Kazemzadeh, R.B.; Albadvi, A.; Aghdasi, M. PROMETHEE: A comprehensive literature review on methodologies and applications. Eur. J. Oper. Res. 2010, 200, 198–215. [Google Scholar] [CrossRef]
- Govindan, K.; Jepsen, M.B. ELECTRE: A comprehensive literature review on methodologies and applications. Eur. J. Oper. Res. 2016, 250, 1–29. [Google Scholar] [CrossRef]
- Sarabando, P.; Dias, L.C. Simple procedures of choice in multicriteria problems without precise information about the alternatives’ values. Comput. Oper. Res. 2010, 37, 2239–2247. [Google Scholar] [CrossRef]
- Shahin, A. Quality Function Deployment: A Comprehensive Review; Department of Management, University of Isfahan: Isfahan, Iran, 2005; pp. 1–25. [Google Scholar]
- Zavadskas, E.K.; Kaklauskas, A. Determination of an Efficient Contractor by Using the New Method of Multicriteria Assessment, International Symposium for “The Organization and Management of Construction”. In Shaping Theory and Practice, Vol. 2: Managing the Construction Project and Managing Risk, CIB W 65; Langford, D.A., Retik, A., Eds.; E and FN SPON: London, UK; Weinheim, Germany; New York, NY, USA; Tokyo, Japan; Melbourne, Australia; Madras, India, 1996; pp. 94–104. [Google Scholar]
- Aghdaie, M.H.; Zolfani, S.H.; Zavadskas, E.K. Prioritizing constructing projects of municipalities based on AHP and COPRAS-G: A case study about footbridges in Iran. Balt. J. Road Bridge Eng. 2012, 7, 145–153. [Google Scholar] [CrossRef]
- Liou, J.J.H.; Tamosaitiene, J.; Zavadskas, E.K.; Tzeng, G.-H. New hybrid COPRAS-G MADM Model for improving and selecting suppliers in green supply chain management. Int. J. Prod. Res. 2016, 54, 114–134. [Google Scholar] [CrossRef]
- Wang, Y.-M.; Elhag, T.M.S. Fuzzy TOPSIS method based on alpha level sets with an application to bridge risk assessment. Expert Syst. Appl. 2006, 31, 309–319. [Google Scholar] [CrossRef]
- Gu, X.; Wang, Y.; Yang, B. Method for selecting the suitable bridge construction projects with interval-valued intuitionistic Fuzzy information. Int. J. Digit. Content Technol. Appl. 2011, 5, 201–206. [Google Scholar]
- Charnes, A.; Cooper, W.W.; Rhodes, E. Measuring the efficiency of decision making units. Eur. J. Oper. Res. 1978, 2, 429–444. [Google Scholar] [CrossRef]
- Saaty, T.L. The Analytic Hierarchy Process; McGraw-Hill: New York, NY, USA, 1980. [Google Scholar]
- Saaty, R.W. The analytic hierarchy process—What it is and how it is used. Math. Model. 1987, 9, 161–176. [Google Scholar] [CrossRef]
- Wang, Y.-M.; Liu, J.; Elhag, T.M.S. An integrated AHP-DEA methodology for bridge risk assessment. Comput. Ind. Eng. 2008, 54, 513–525. [Google Scholar] [CrossRef]
- Gervásio, H.; Da Silva, L.S. A probabilistic decision-making approach for the sustainable assessment of infrastructures. Expert Syst. Appl. 2012, 39, 7121–7131. [Google Scholar] [CrossRef]
- Farkas, A. Multi-criteria comparison of bridge designs. Acta Polytech. Hung. 2011, 8, 173–191. [Google Scholar]
- Abu Dabous, S.; Alkass, S. Decision support method for multi-criteria selection of bridge rehabilitation strategy. Constr. Manag. Econ. 2008, 26, 883–893. [Google Scholar] [CrossRef]
- Huang, Q.; Ren, Y.; Lin, Y.-Z. Application of uncertain type of AHP to the condition assessment of cable-stayed bridges 1. J. Southeast Univ. 2007, 23, 599–603. [Google Scholar]
- Torres-Machí, C.; Chamorro, A.; Pellicer, E.; Yepes, V.; Videla, C. Sustainable pavement management: Integrating economic, technical, and environmental aspects in decision making. Transp. Res. Rec. 2015, 2523, 56–63. [Google Scholar] [CrossRef]
- Lu, I.-Y.; Kuo, T.; Lin, T.-S.; Tzeng, G.-H.; Huang, S.-L. Multicriteria decision analysis to develop effective sustainable development strategies for enhancing competitive advantages: Case of the TFT-LCD industry in Taiwan. Sustainability 2016, 8, 646. [Google Scholar] [CrossRef]
- Saaty, T.L.; Takizawa, M. Dependence and independence: From linear hierarchies to nonlinear networks. Eur. J. Oper. Res. 1986, 26, 229–237. [Google Scholar] [CrossRef]
- Lu, S.T.; Lin, C.W.; Ko, P.H. Application of Analytic Network Process (ANP) in assessing construction risk of urban bridge project. In Proceedings of the Second International Conference on Innovative Computing, Information and Control (ICICIC), Kumamoto, Japan, 5–7 September 2007; pp. 1–4.
- Brans, J.P.; Mareschal, B.; Vincke, P. PROMETHEE: A new family of outranking methods in multicriteria analysis. Oper. Res. 1984, 408–421. [Google Scholar]
- Kogure, M.; Akao, Y. Quality function deployment and CWQC in Japan. Qual. Prog. 1983, 16, 25–29. [Google Scholar]
- Osorio Gómez, J.C. Fuzzy QFD for multicriteria decision making—Application example. Prospectiva 2011, 9, 22–29. [Google Scholar]
- Dalkey, N.; Helmer, O. An experimental application of the Delphi method to the use of experts. Manag. Sci. 1963, 9, 458–467. [Google Scholar] [CrossRef]
- Zadeh, L.A. Fuzzy sets. Inf. Control 1965, 8, 338–353. [Google Scholar] [CrossRef]
- Deng, J.L. Introduction to grey system theory. J. Grey Theory 1989, 1, 1–24. [Google Scholar]
- Lin, Y.; Chen, M.; Liu, S. Theory of grey systems: Capturing uncertainties of grey information. Kybernetes 2004, 33, 196–218. [Google Scholar] [CrossRef]
- Zavadskas, E.; Kaklauskas, A.; Turskis, Z.; Tamošaitien, J. Multi-attribute decision-making model by applying grey numbers. Inst. Math. Inform. Vilnius 2009, 20, 305–320. [Google Scholar]
- Zavala, G.R.; Nebro, A.J.; Luna, F.; Coello Coello, C.A. A survey of multi-objective metaheuristics applied to structural optimization. Struct. Multidiscip. Optim. 2013, 49, 537–558. [Google Scholar] [CrossRef]
- Holland, J.H. Adaptation in Natural and Artificial Systems; The MIT Press: Cambridge, MA, USA, 1975. [Google Scholar]
- Kennedy, J.; Eberhart, R. Particle swarm optimization. In Proceedings of ICNN’95—International Conference on Neural Networks, Perth, Australia, 27 November–1 December 1995; Volume 4, pp. 1942–1948.
- Dorigo, M.; Maniezzo, V.; Colorni, A. Ant system: Optimization by a colony of cooperating agents. IEEE Trans. Syst. Man. Cybern. B 1996, 26, 29–41. [Google Scholar] [CrossRef] [PubMed]
- Kirkpatrick, S.; Gelatt, C.D.; Vecchi, M.P. Optimization by simulated annealing. Science 1983, 220, 671–680. [Google Scholar] [CrossRef] [PubMed]
- Geem, Z.; Kim, J.H.; Loganathan, G.V. A new heuristic optimization algorithm: Harmony search. Simulation 2001, 76, 60–68. [Google Scholar] [CrossRef]
- Wang, Y.-M.; Luo, Y.; Hua, Z.-S. A note on group decision-making based on concepts of ideal and anti-ideal points in a fuzzy environment. Math. Comput. Model. 2007, 46, 1256–1264. [Google Scholar] [CrossRef]
- Kuo, M.-S.; Tzeng, G.-H.; Huang, W.-C. Group decision-making based on concepts of ideal and anti-ideal points in a fuzzy environment. Math. Comput. Model. 2007, 45, 324–339. [Google Scholar] [CrossRef]
- Ardeshir, A.; Mohseni, N.; Behzadian, K.; Errington, M. Selection of a bridge construction site using fuzzy analytical hierarchy process in geographic information system. Arab. J. Sci. Eng. 2014, 39, 4405–4420. [Google Scholar] [CrossRef]
- Utomo, C.; Idrus, A. Value—Based Group Decision on Support Bridge Selection. World Acad. Sci. Eng. Technol. 2010, 4, 188–193. [Google Scholar]
- Joshi, P.K.; Sharma, P.C.; Upadhyay, S.; Sharma, S. Multi objective Fuzzy decision making approach for selection of type of caisson for bridge foundation. Indian J. Pure Appl. Math. 2004, 35, 783–791. [Google Scholar]
- Ugwu, O.O.; Kumaraswamy, M.M.; Wong, A.; Ng, S.T. Sustainability appraisal in infrastructure projects (SUSAIP): Part 2: A case study in bridge design. Autom. Constr. 2006, 15, 229–238. [Google Scholar] [CrossRef]
- Moore, C.J.; Miles, J.C.; Rees, D.W.G. Decision support for conceptual bridge design. Artif. Intell. Eng. 1996, 11, 259–272. [Google Scholar] [CrossRef]
- Ohkubo, S.; Dissanayake, P.B.R.; Taniwaki, K. An approach to multicriteria fuzzy optimization of a prestressed concrete bridge system considering cost and aesthetic feeling. Struct. Optim. 1998, 15, 132–140. [Google Scholar] [CrossRef]
- Itoh, Y.; Sunuwar, L.; Hirano, T.; Hammad, A.; Nishido, T. Bridge type selection system incorporating environmental impacts. J. Glob. Environ. Eng. 2000, 6, 81–101. [Google Scholar]
- Wang, H.-L.; Zhang, Z.; Qin, S.-F.; Huang, C.-L. Fuzzy optimum model of semi-structural decision for lectotype. China Ocean Eng. 2001, 15, 453–466. [Google Scholar]
- Jakiel, P.; Fabianowski, D. FAHP model used for assessment of highway RC bridge structural and technological arrangements. Expert Syst. Appl. 2015, 42, 4054–4061. [Google Scholar] [CrossRef]
- Yepes, V.; Martí, J.V.; García-Segura, T. Cost and CO2 emission optimization of precast-prestressed concrete U-beam road bridges by a hybrid glowworm swarm algorithm. Autom. Constr. 2015, 49, 123–134. [Google Scholar] [CrossRef]
- Camp, C.V.; Assadollahi, A. CO2 and cost optimization of reinforced concrete footings using a hybrid big bang-big crunch algorithm. Struct. Multidiscip. Optim. 2013, 48, 411–426. [Google Scholar] [CrossRef]
- García-Segura, T.; Yepes, V.; Martí, J.V.; Alcalá, J. Optimization of concrete I-beams using a new hybrid glowworm swarm algorithm. Latin Am. J. Solids Struct. 2014, 11, 1190–1205. [Google Scholar] [CrossRef]
- García-Segura, T.; Yepes, V.; Alcalá, J.; Pérez-López, E. Hybrid harmony search for sustainable design of post-tensioned concrete box-girder pedestrian bridges. Eng. Struct. 2015, 92, 112–122. [Google Scholar] [CrossRef]
- Martí, J.V.; García-Segura, T.; Yepes, V. Structural design of precast-prestressed concrete U-beam road bridges based on embodied energy. J. Clean. Prod. 2016, 120, 231–240. [Google Scholar] [CrossRef]
- De Medeiros, G.F.; Kripka, M. Optimization of reinforced concrete columns according to different environmental impact assessment parameters. Eng. Struct. 2014, 59, 185–194. [Google Scholar] [CrossRef]
- Martinez-Martin, F.J.; Gonzalez-Vidosa, F.; Hospitaler, A.; Yepes, V. Multi-objective optimization design of bridge piers with hybrid heuristic algorithms. J. Zhejiang Univ. Sci. A 2012, 13, 420–432. [Google Scholar] [CrossRef]
- García-Segura, T.; Yepes, V.; Alcalá, J. Sustainable design using multiobjective optimization of high-strength concrete I-beams. In Proceedings of the 2014 International Conference on High Performance and Optimum Design of Structures and Materials HPSM/OPTI, Ostend, Belgium, 9–11 June 2014; pp. 347–358.
- Yepes, V.; García-Segura, T.; Moreno-Jiménez, J.M. A cognitive approach for the multi-objective optimization of RC structural problems. Arch. Civ. Mech. Eng. 2015, 15, 1024–1036. [Google Scholar] [CrossRef]
- García-Segura, T.; Yepes, V. Multiobjective optimization of post-tensioned concrete box-girder road bridges considering cost, CO2 emissions, and safety. Eng. Struct. 2016, 125, 325–336. [Google Scholar] [CrossRef]
- El-Diraby, T.E.; O’Connor, J.T. Model for evaluationg bridge construction plans. J. Constr. Eng. Manag. 2001, 127, 399–405. [Google Scholar] [CrossRef]
- Chou, J.-S.; Pham, A.-D.; Wang, H. Bidding strategy to support decision-making by integrating Fuzzy AHP and regression-based simulation. Autom. Constr. 2013, 35, 517–527. [Google Scholar] [CrossRef]
- Pan, N.-F. Fuzzy AHP approach for selecting the suitable bridge construction method. Autom. Constr. 2008, 17, 958–965. [Google Scholar] [CrossRef]
- Mousavi, S.M.; Gitinavard, H.; Siadat, A. A new hesitant fuzzy Analytical Hierarchy Process method for decision-making problems under uncertainty. In Proceedings of the IEEE International Conference on Industrial Engineering and Engineering Management, Piscataway, NJ, USA, 9–12 December 2014; pp. 622–626.
- Chen, Y.; Okudan, G.E.; Riley, D.R. Decision support for construction method selection in concrete buildings: Prefabrication adoption and optimization. Autom. Constr. 2010, 19, 665–675. [Google Scholar] [CrossRef]
- Chen, T.-Y. The extended linear assignment method for multiple criteria decision analysis based on interval-valued intuitionistic fuzzy sets. Appl. Math. Model. 2014, 38, 2101–2117. [Google Scholar] [CrossRef]
- Bitarafan, M.; Arefi, S.L.; Zolfani, S.H.; Mahmoudzadeh, A. Selecting the best design scenario of the smart structure of bridges for probably future earthquakes. Procedia Eng. 2013, 57, 193–199. [Google Scholar] [CrossRef]
- Ei-Mikawi, M.; Mosallam, A.S. A methodology for evaluation of the use of advanced composites in structural civil engineering applications. Compos. Part B 1996, 27, 203–215. [Google Scholar] [CrossRef]
- Sobanjo, J.O.; Stukhart, G.; James, R.W. Evaluation of projects for rehabilitation of highway bridges. J. Struct. Eng. 1994, 120, 81–99. [Google Scholar] [CrossRef]
- Abu Dabous, S.; Alkass, S. A multi-attribute ranking method for bridge management. Eng. Constr. Archit. Manag. 2010, 17, 282–291. [Google Scholar] [CrossRef]
- Yehia, S.; Abudayyeh, O.; Fazal, I.; Randolph, D. A decision support system for concrete bridge deck maintenance. Adv. Eng. Softw. 2008, 39, 202–210. [Google Scholar] [CrossRef]
- Chassiakos, A.P.; Vagiotas, P.; Theodorakopoulos, D.D. A knowledge-based system for maintenance planning of highway concrete bridges. Adv. Eng. Softw. 2005, 36, 740–749. [Google Scholar] [CrossRef]
- Sabatino, S.; Frangopol, D.M.; Dong, Y. Sustainability-informed maintenance optimization of highway bridges considering multi-attribute utility and risk attitude. Eng. Struct. 2015, 102, 310–321. [Google Scholar] [CrossRef]
- Adey, B.; Hajdin, R.; Brühwiler, E. Risk-based approach to the determination of optimal interventions for bridges affected by multiple hazards. Eng. Struct. 2003, 25, 903–912. [Google Scholar] [CrossRef]
- Aktan, A.E.; David, N.F.; Vikram, L.B.; Helmicki, A.J.; Hunt, V.J.; Shelley, S.J. Condition assessment for bridge managment. J. Infrastruct. Syst. 1996, 2, 108–117. [Google Scholar] [CrossRef]
- Al-Wazeer, A.; Harris, B.; Dekelbab, W. Applying Fuzzy concept to bridge managment. Publics Roads 2016, 72, 28–37. [Google Scholar]
- Andrić, J.M.; Lu, D.G. Risk assessment of bridges under multiple hazards in operation period. Saf. Sci. 2016, 83, 80–92. [Google Scholar] [CrossRef]
- Anoop, M.B.; Balaji Rao, K. Application of Fuzzy sets for remaining life assessment of corrosion affected reinforced concrete bridge girders. J. Perform. Constr. Facil. 2007, 21, 166–171. [Google Scholar] [CrossRef]
- Caterino, N.; Iervolino, I.; Manfredi, G.; Cosenza, E. Comparative analysis of multi-criteria decision-making methods for seismic structural retrofitting. Comput. Civ. Infrastruct. Eng. 2009, 24, 432–445. [Google Scholar] [CrossRef]
- Cheng, M.-Y.; Hoang, N.-D. Risk score inference for bridge maintenance project using evolutionary Fuzzy least squares support vector machine. J. Comput. Civ. Eng. 2012, 28, 1–9. [Google Scholar] [CrossRef]
- Cheng, J.; Xiao, R. An efficient method for identification of risk factors. Sci. China Ser. E 2009, 52, 3626–3631. [Google Scholar] [CrossRef]
- Dan, D.; Sun, L.; Yang, Z.; Xie, D. The application of a fuzzy inference system and analytical hierarchy process based online evaluation framework to the donghai bridge health monitoring system. Smart Struct. Syst. 2014, 14, 129–144. [Google Scholar] [CrossRef]
- De Brito, J.; Branco, F.A.; Thoft-Christensen, P.; Sørensen, J.D. An expert system for concrete bridge management. Eng. Struct. 1997, 19, 519–526. [Google Scholar] [CrossRef]
- Deng, J.; Li, J.; Fang, X. Condition evaluation of existing long-span bridges using fuzzy based analytic hierarchy process. Adv. Mater. Res. 2011, 163–167, 3328–3331. [Google Scholar] [CrossRef]
- Duchaczek, A.; Skorupka, D. Evaluation of probability of bridge damage as a result of terrorist attack. Arch. Civ. Eng. 2013, 59, 215–227. [Google Scholar] [CrossRef]
- Furuta, H.; Shiraishi, N.; Umano, M.; Kawakami, K. An expert system for damage assessment of a reinforced concrete bridge deck. Fuzzy Sets Syst. 1991, 44, 449–457. [Google Scholar]
- Kawamura, K.; Miyamoto, A. Condition state evaluation of existing reinforced concrete bridges using neuro-Fuzzy hybrid system. Comput. Struct. 2003, 81, 1931–1940. [Google Scholar] [CrossRef]
- Kushida, M.; Miyamoto, A. Modal Logic to evaluate a knowledge-based bridge rating system. Comput. Civ. Infrastruct. Eng. 1998, 13, 227–236. [Google Scholar] [CrossRef]
- Lee, J.; Liu, K.F.R.; Chiang, W. A Fuzzy Petri net-based expert system and its application to damage assessment of bridges. In Proceedings of the IEEE International Conference on Industrial Engineering and Engineering Management, Piscataway, NJ, USA, 10–12 November 1998; Volume 29, pp. 350–369.
- Li, Q.; Xu, Y.-L.; Zheng, Y.; Guo, A.-X.; Wong, K.-Y.; Xia, Y. SHM-based F-AHP bridge rating system with application to Tsing Ma Bridge. Front. Archit. Civ. Eng. China 2011, 5, 465–478. [Google Scholar] [CrossRef]
- Li, Y.; Wang, T.; Song, X.; Li, G. Optimal resource allocation for anti-terrorism in protecting overpass bridge based on AHP risk assessment model. KSCE J. Civ. Eng. 2015, 20, 309–322. [Google Scholar] [CrossRef]
- Liang, M.-T.; Wu, J.-H.; Liang, C.-H. Applying Fuzzy mathematics to evaluating the membership of existing reinforced concrete bridges in Taipei. J. Mar. Sci. Technol. 2000, 8, 16–29. [Google Scholar]
- Liang, M.-T.; Chu, T.-B.; Tsao, W.-H.; Yeh, C.-J. Determining the repair ranking of existing RC bridges using multi-pole fuzzy pattern recognition evaluation method. J. Mar. Sci. Technol. 2006, 29, 159–173. [Google Scholar]
- Liang, M.-T.; Lin, C.-M.; Yeh, C.-J. Comparison matrix method and its applications to damage evaluation for existing reinforced concrete bridges. J. Mar. Sci. Technol. 2003, 11, 70–82. [Google Scholar]
- Liang, M.-T.; Wu, J.-H.; Liang, C.-H. Multiple layer Fuzzy evaluation for existing reinforced concrete bridges. J. Infrastruct. Syst. 2001, 7, 144–159. [Google Scholar] [CrossRef]
- Lounis, Z. Risk-based maintenance optimization of aging highway bridge decks. Adv. Eng. Struct. Mech. Constr. 2006, 140, 723–734. [Google Scholar]
- Min, Z.; Jingshan, B.; Xiaolei, Z. Quick analysis method for bridge seismic risk based on AHP. In Proceedings of the IEEE International Conference on Electric Technology and Civil Engineering, Piscataway, NJ, USA, 22–24 April 2011; pp. 575–577.
- Moufti, S.A.; Zayed, T.; Dabous, S.A. Fuzzy defect based condition assessment of concrete bridges. In Proceedings of the IEEE International Conference on Industrial Engineering and Engineering Management, Piscataway, NJ, USA, 24–28 June 2013; pp. 1489–1494.
- Ning, X.-L.; Tan, P.; Huang, D.-Y.; Zhou, F.-L. Application of adaptative fuzzy sliding mode control to a seismically excited highway bridge. Struct. Control Heal. Monit. 2009, 16, 639–656. [Google Scholar] [CrossRef]
- Ozbek, M.E.; De la Garza, J.M.; Triantis, K. Efficiency measurement of bridge maintenance using data envelopment analysis. J. Infrastruct. Syst. 2010, 342, 31–39. [Google Scholar] [CrossRef]
- Park, K.-S.; Koh, H.-M.; Ok, S.-Y.; Seo, C.-W. Fuzzy supervisory control of earthquake-excited cable-stayed bridges. Eng. Struct. 2005, 27, 1086–1100. [Google Scholar] [CrossRef]
- Rashidi, M.; Gibson, P. Proposal of a methodology for bridge condition assessment. In Proceedings of the 2011 Australasian Transport Research Forum, Adelaide, Australia, 28–30 September 2011; pp. 1–15.
- Rashidi, M.; Gibson, P. A methodology for bridge condition evaluation. J. Civ. Eng. Archit. 2012, 6, 1149–1157. [Google Scholar]
- Saito, M.; Sinha, K.C. Delphi study on bridge condition rating and effects of improvements. J. Transp. Eng. 1991, 117, 320–334. [Google Scholar] [CrossRef]
- Sasmal, S.; Ramanjaneyulu, K. Condition evaluation of existing reinforced concrete bridges using Fuzzy based analytic hierarchy approach. Expert Syst. Appl. 2008, 35, 1430–1443. [Google Scholar] [CrossRef]
- Sasmal, S.; Ramanjaneyulu, K.; Gopalakrishan, S.; Lakshmanan, N. Fuzzy logic based condition rating of existing reinforced concrete bridges. J. Perform. Constr. Facil. 2006, 20, 261–273. [Google Scholar] [CrossRef]
- Stewart, M.G. Reliability-based assessment of ageing bridges using risk ranking and life cycle cost decision analyses. Reliab. Eng. Syst. Saf. 2001, 74, 263–273. [Google Scholar] [CrossRef]
- Tarighat, A. Model based damage detection of concrete bridge deck using adaptive neuro-Fuzzy inference system. Int. J. Civ. Eng. 2013, 11, 170–181. [Google Scholar]
- Tarighat, A.; Miyamoto, A. Fuzzy concrete bridge deck condition rating method for practical bridge management system. Expert Syst. Appl. 2009, 36, 12077–12085. [Google Scholar] [CrossRef]
- Chiang, W.; Liu, K.R.R.; Lee, J. Bridge damage assessment through Fuzzy petri net based expert system. 2000, 14, 141–149. [Google Scholar] [CrossRef]
- Ugwu, O.O.; Kumaraswamy, M.M.; Kung, F.; Ng, S.T. Object-oriented framework for durability assessment and life cycle costing of highway bridges. Autom. Constr. 2005, 14, 611–632. [Google Scholar] [CrossRef]
- Zhao, Z.; Chen, C. A Fuzzy system for concrete bridge damage diagnosis. Comput. Struct. 2002, 80, 629–641. [Google Scholar] [CrossRef]
- Zhao, Z.Y.; Chen, C.Y. Concrete bridge deterioration diagnosis using Fuzzy inference system. J. Adv. Eng. Softw. 2001, 32, 317–325. [Google Scholar] [CrossRef]
- Wang, Y.-M.; Elhag, T.M.S. A Fuzzy group decision making approach for bridge risk assessment. Comput. Ind. Eng. 2007, 53, 137–148. [Google Scholar] [CrossRef]
- Liu, M.; Frangopol, D.M. Optimal bridge maintenance planning based on probabilistic performance prediction. Eng. Struct. 2004, 26, 991–1002. [Google Scholar] [CrossRef]
- Liu, M.; Frangopol, D.M. Multiobjective maintenance planning optimization for deteriorating bridges considering condition, safety, and life-cycle cost. J. Struct. Eng. 2003, 131, 833–842. [Google Scholar] [CrossRef]
- Neves, L.A.C.; Frangopol, D.M.; Cruz, P.J.S. Probabilistic lifetime-oriented multiobjective optimization of bridge maintenance: Single maintenance type. J. Struct. Eng. 2006, 132, 991–1005. [Google Scholar] [CrossRef]
- Kim, S.; Frangopol, D.M.; Zhu, B. Probabilistic optimum inspection/repair planning to extend lifetime of deteriorating structures. J. Perform. Constr. Facil. 2011, 25, 534–545. [Google Scholar] [CrossRef]
- Dong, Y.; Frangopol, D.M.; Saydam, D. Time-variant sustainability assessment of seismically vulnerable bridges subjected to multiple hazards. Earthq. Eng. Struct. Dyn. 2013, 42, 1451–1467. [Google Scholar] [CrossRef]
- Frangopol, D.M.; Soliman, M. Life-cycle of structural systems: Recent achievements and future directions. Struct. Infrastruct. Eng. 2015, 12, 1–20. [Google Scholar] [CrossRef]
- Chen, Z.; Abdullah, A.B.; Anumba, C.J.; Li, H. ANP experiment for demolition plan evaluation. J. Constr. Eng. Manag. 2013, 138, 51–60. [Google Scholar] [CrossRef]
- IBM Corp. IBM SSPS Statics for Windows, version 22.00; IBM Corp.: Armonk, NY, USA, 2013.
- Gölcük, I.; Baykasoglu, A. An analysis of DEMATEL approaches for criteria interaction handling within ANP. Expert Syst. Appl. 2016, 46, 346–366. [Google Scholar] [CrossRef]
- Huang, K.-W.; Huang, J.-H.; Tzeng, G.-H. New hybrid multiple attribute decision-making model for improving competence sets: Enhancing a company’s core competitiveness. Sustainability 2016, 8, 175. [Google Scholar] [CrossRef]
- Yang, Y.-P.O.; Leu, J.-D.; Tzeng, G.-H. A novel hybrid MCDM model combined with DEMATEL and ANP with applications. Int. J. Oper. Res. 2008, 5, 160–168. [Google Scholar]
- Ou Yang, Y.-P.; Shieh, H.-M.; Tzeng, G.-H. A VIKOR technique based on DEMATEL and ANP for information security risk control assessment. Inf. Sci. 2013, 232, 482–500. [Google Scholar] [CrossRef]
- Shen, K.-Y.; Hu, S.-K.; Tzeng, G.-H. Financial modeling and improvement planning for the life insurance industry by using a rough knowledge based hybrid MCDM model. Inf. Sci. 2017, 375, 296–313. [Google Scholar] [CrossRef]
- Liou, J.J.H.; Tsai, C.; Lin, R.; Tzeng, G.-H. A modified VIKOR multiple-criteria decision method for improving domestic airlines service quality. J. Air Transp. Manag. 2011, 17, 57–61. [Google Scholar] [CrossRef]
- Shen, K.-Y.; Yan, M.-R.; Tzeng, G.-H. Combining VIKOR-DANP model for glamor stock selection and stock performance improvement. Knowl.-Based Syst. 2014, 58, 86–97. [Google Scholar] [CrossRef]
- Tsui, C.-W.; Tzeng, G.-H.; Wen, U.-P. A hybrid MCDM approach for improving the performance of green suppliers in the TFT-LCD industry. Int. J. Prod. Res. 2015, 53, 6436–6454. [Google Scholar] [CrossRef]
- Liou, J.J.H.; Chuang, Y.-C.; Tzeng, G.-H. A fuzzy integral-based model for supplier evaluation and improvement. Inf. Sci. 2014, 266, 199–217. [Google Scholar] [CrossRef]
- Canto-Perello, J.; Martinez-Leon, J.; Curiel-Esparza, J.; Martin-Utrillas, M. Consensus in prioritizing river rehabilitation projects through the integration of social, economic and landscape indicators. Ecol. Indic. 2017, 72, 659–666. [Google Scholar] [CrossRef]
- Hu, K.-H.; Chen, F.-H.; Tzeng, G.-H. Evaluating the improvement of sustainability of sports industry policy based on MADM. Sustainability 2016, 8, 606. [Google Scholar] [CrossRef]
- Chang, D.-S.; Chen, S.-H.; Hsu, C.-W.; Hu, A.; Tzeng, G.-H. Evaluation framework for a alternative fuel vehicles: Sustainable development perspective. Sustainability 2015, 7, 11570–11594. [Google Scholar] [CrossRef]
© 2016 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC-BY) license (http://creativecommons.org/licenses/by/4.0/).