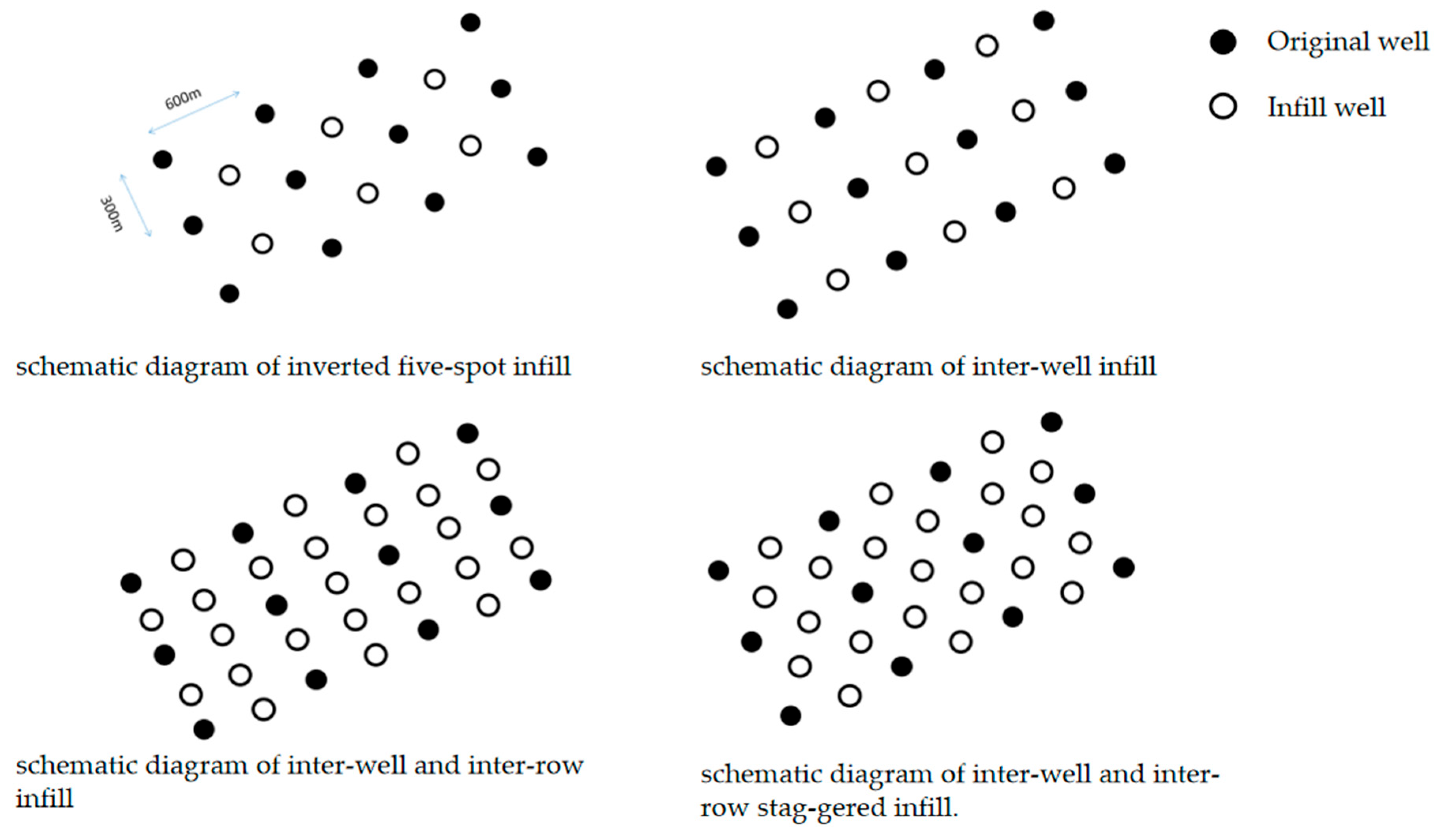
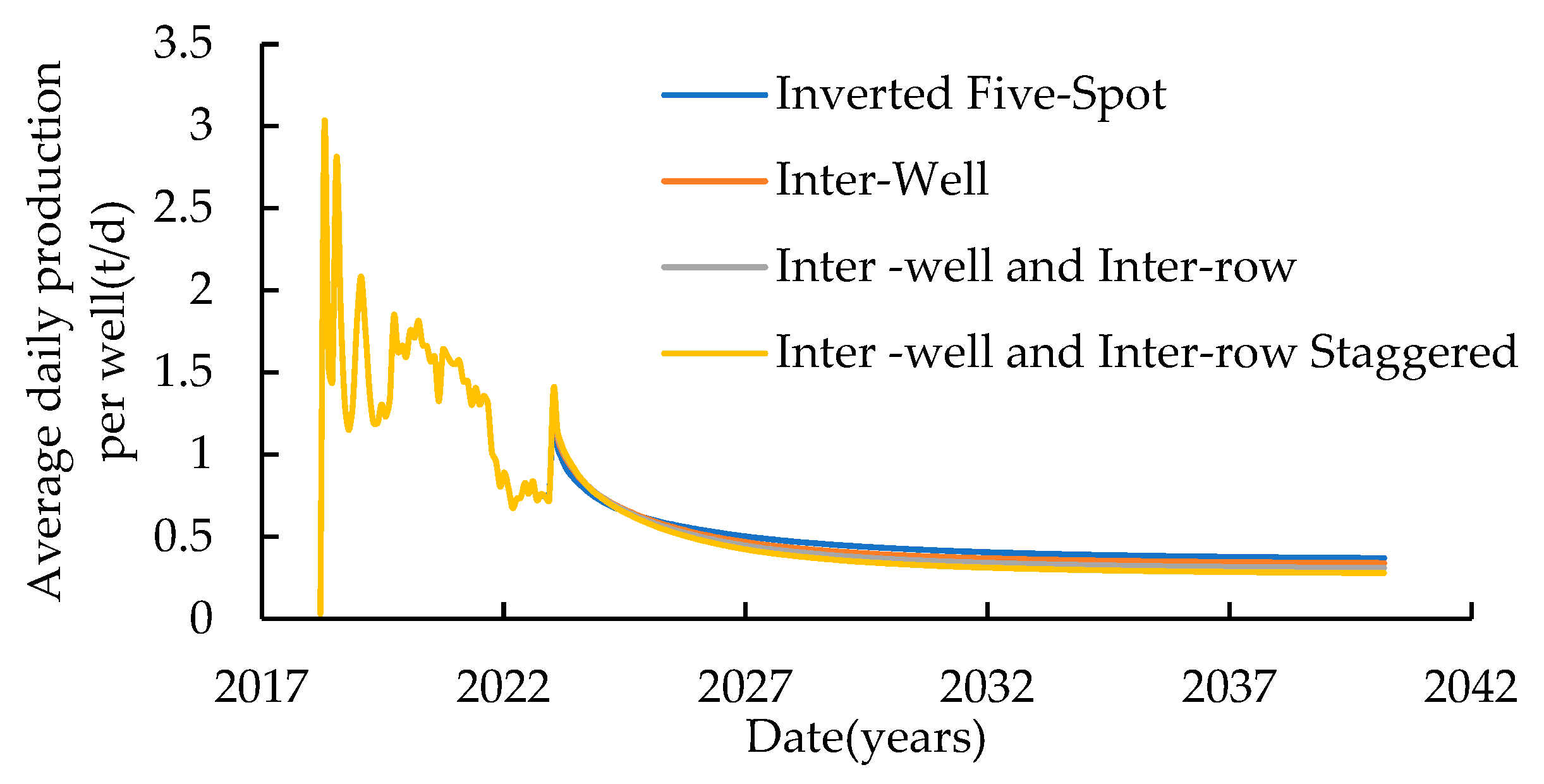
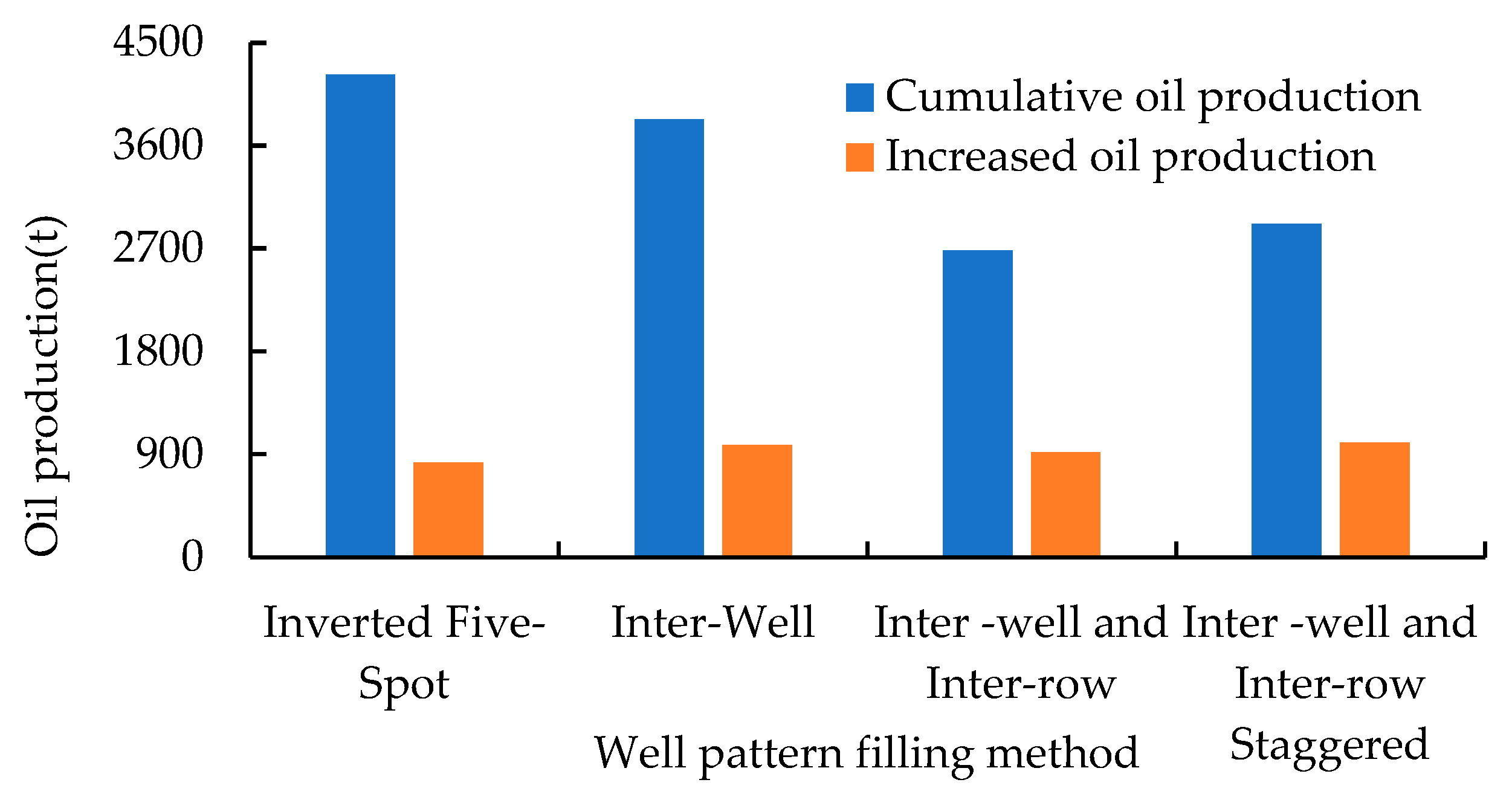
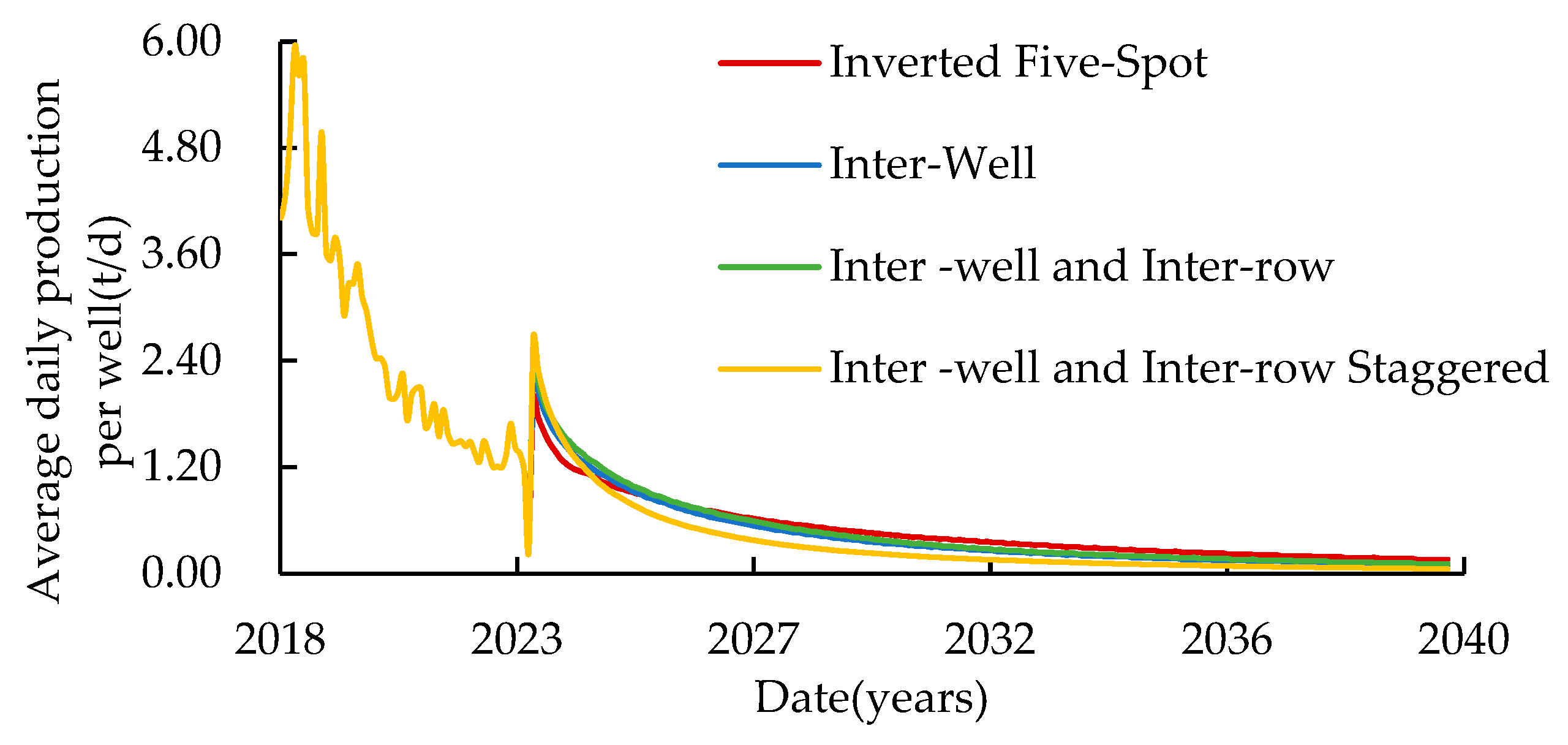
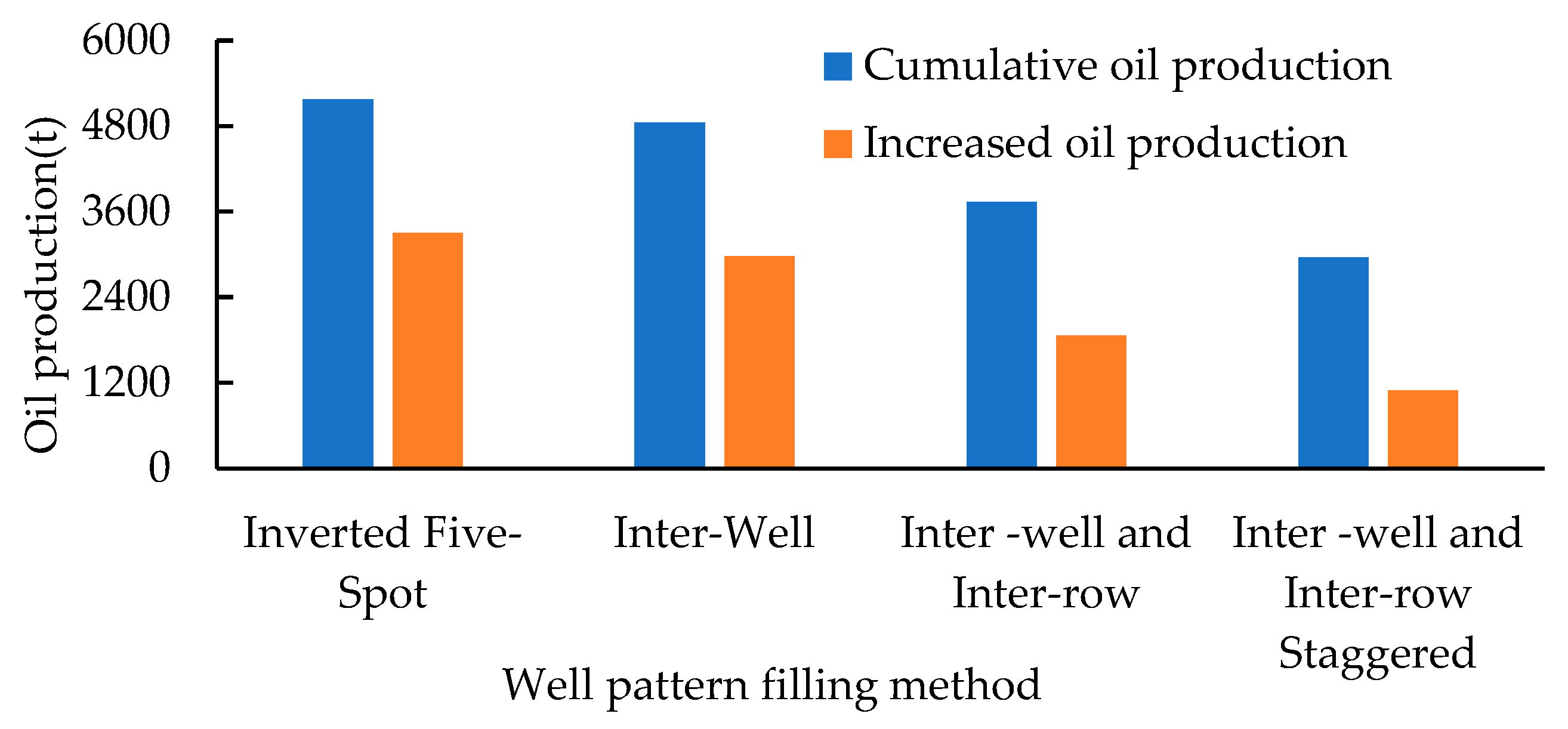
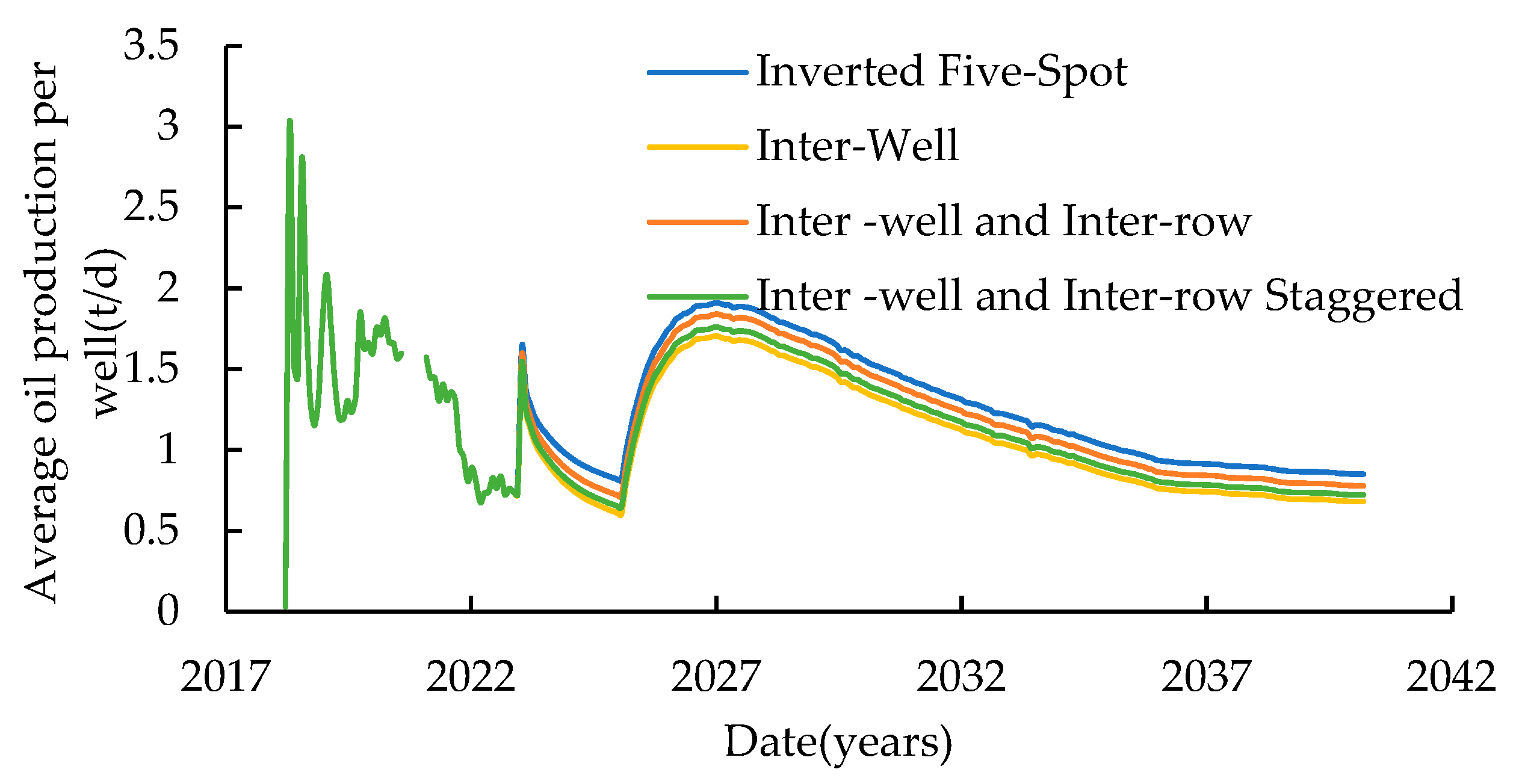
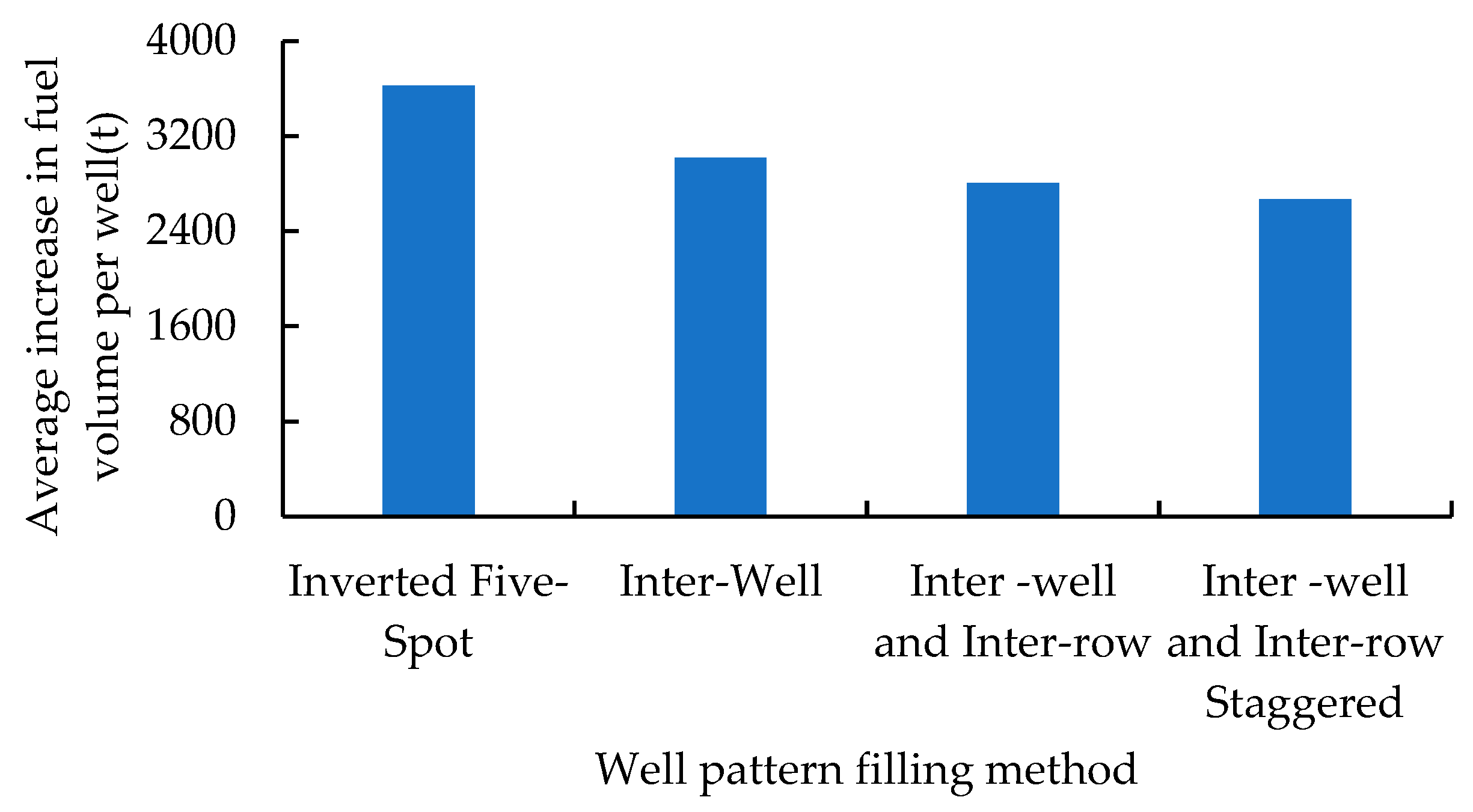
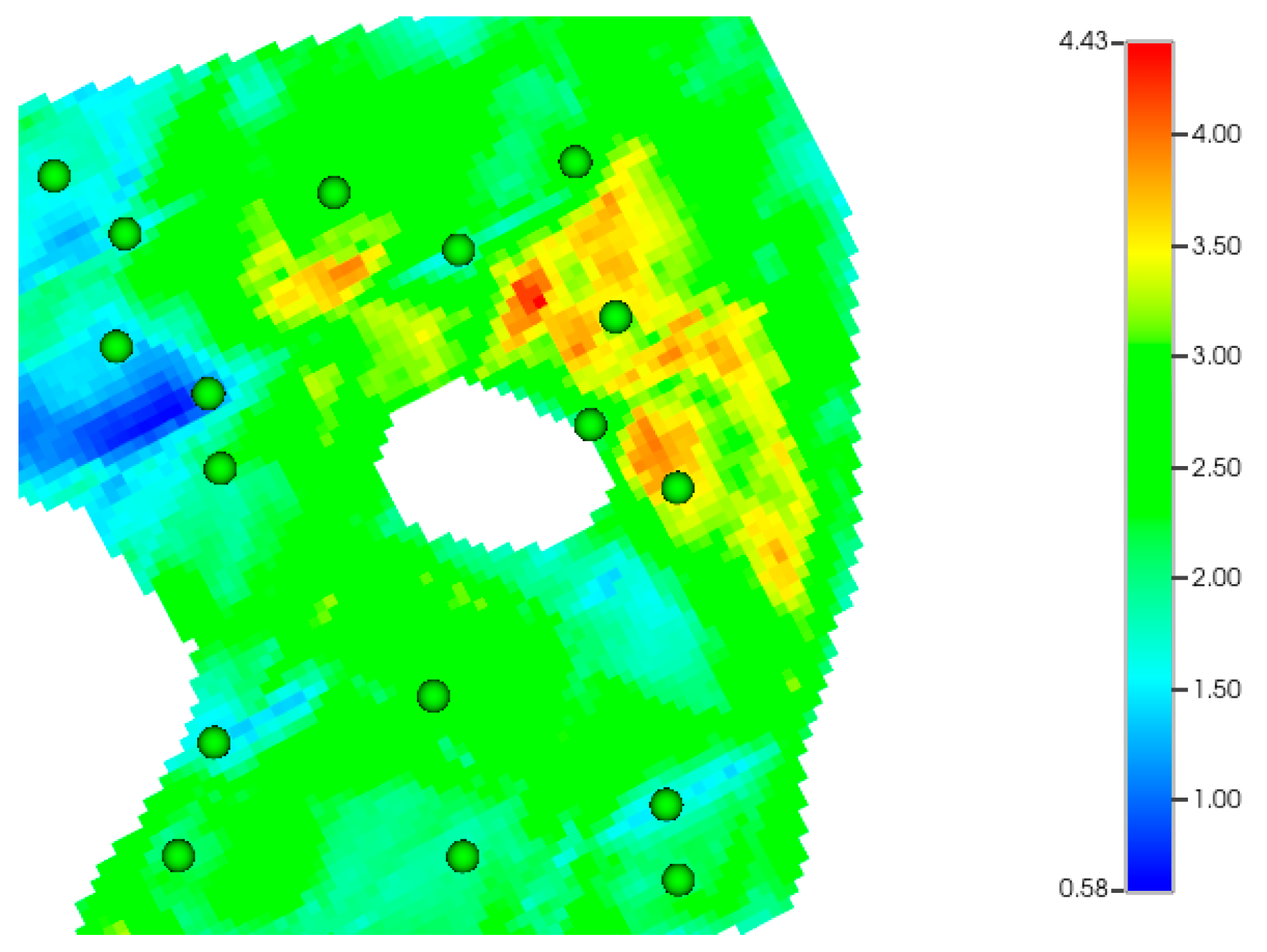
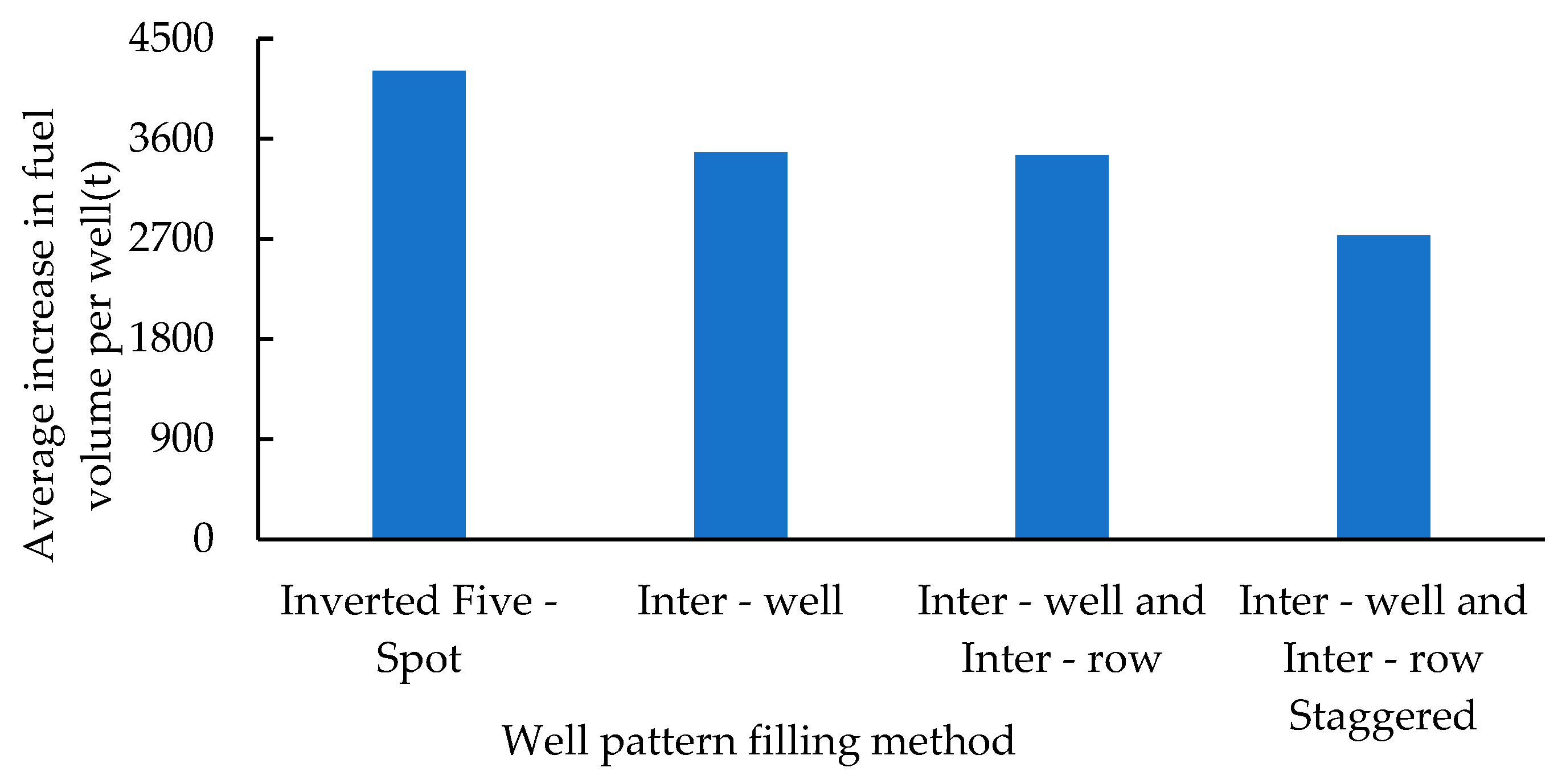
Well pattern type is a critical consideration in CO
2 flooding reservoir engineering design. Based on the stress-sensitive-seepage coupling development concept proposed by Li et al. [
35], well pattern optimization requires an integrated analysis of fracture characteristics and in situ stress field distribution to achieve efficient displacement through optimized well row orientation and injection-production parameters. Studies demonstrate that aligning injector rows with the maximum principal stress direction creates preferential linear flow paths, enabling uniform CO
2 diffusion within fractured networks and effectively mitigating gas channeling risks [
36]. This deployment strategy controls the gas–liquid interface propagation velocity within 0.3–0.5 m/d through injection-production pressure differential management, increasing sweep volume coefficients to 68–72% [
37].
2.1. Mathematical Model of CO2-Flooding in Tight Oil Reservoir Fracturing Development
Considering that the study focused on the influence of well pattern parameters on CO2 storage area (quantified by effective drive coefficient) and displacement efficiency, complex injection processes such as WAG were not included in the model to ensure a clear description of the core percolation mechanisms (such as starting pressure gradient and phase permeability curve).
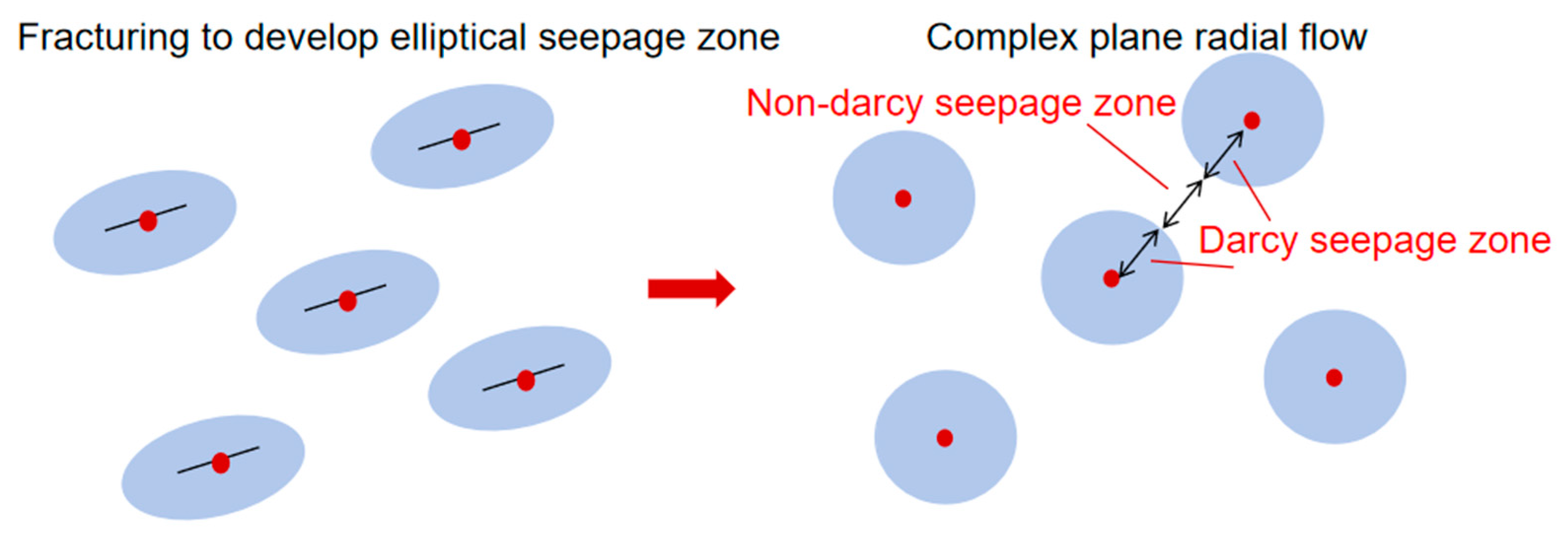
By setting model assumptions, fully applying the radial flow theory, and using the conformal transformation method, the elliptical seepage region is transformed into a circular one. Compared with direct production, fracturing development will form an unutilized region between the oil well reconstruction area, the injection well reconstruction area, and the controlled region of oil and water wells. The transformation of the seepage region in the anti-five-point well pattern fracturing CO
2-flooding is shown in
Figure 1. Considering the miscible effect of CO
2 and crude oil, the injection well reconstruction area follows the binomial seepage law, the production well reconstruction area conforms to the Darcy seepage law, and the corresponding formulas are used to calculate the pressure distribution; the original reservoir between the oil and water well reconstruction area follows the nonlinear seepage law. Therefore, the overall model derivation is divided into three regions, each region satisfies the law of conservation of mass and has its own boundary conditions, and each region is coupled by the boundary pressure and mass transfer.
For the production well reconstruction area:
where
,
are the densities of crude oil and liquid CO
2, respectively, in kg/m
3;
,
are the relative permeabilities of the oil phase and liquid CO
2 phase;
,
are the viscosities of the oil phase and liquid CO
2 phase, respectively, in mPa·s;
is the velocity at the junction between the control area of the injection well and the unmodified area, respectively, in m
3/d/m;
is the intensity of fluid production, respectively, in m
3/d/m;
is the average porosity of the producing well area, respectively, in %;
is the comprehensive compression coefficient of oil well control area, respectively, in MPa
−1;
is the permeability of the reconstructed area, respectively, in 10
−3 μm
2;
is the static pressure of the reservoir, respectively, in MPa;
is the pressure in the untransformed area, respectively, in MPa;
is the oil saturation in the control zone of the production well.
where
is the width of the crack;
is the effective thickness of the oil reservoir;
is the pressure at the junction between the unmodified area and the production well control area, respectively, in MPa;
is the bottomhole flow pressure, respectively, in MPa;
is the drainage radius.
For the matrix unmodified area:
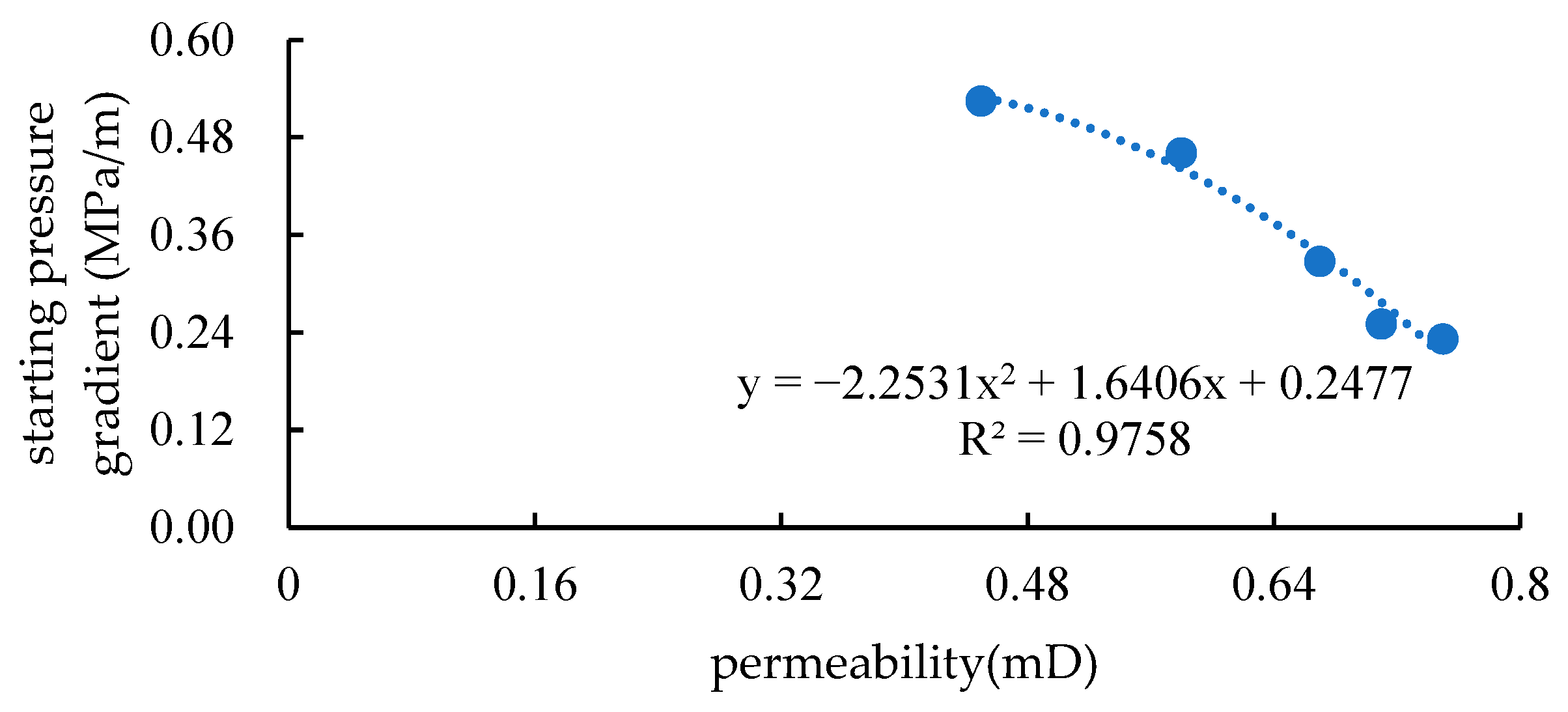
is the average porosity of the unmodified area, respectively, in %; is the integrated compression coefficient in the control area of the injection well, respectively, in MPa−1; is the permeability of the unmodified area, respectively, in 10−3 μm2; α is the sensitive factor in the exponential decay law of the pressure sensitivity effect, in MPa−1; is the pressure at the junction between the control area and the unreconstructed area of the injection well, respectively, in MPa; Gm is the starting pressure gradient of the matrix in the unmodified area, in MPa/m; d is the range of the unmodified area, in m.
For the injection well reconstruction area:
is the average porosity of the injection well area, respectively, in %; is the oil saturation of the injection well control area.
The initial conditions are:
pi is the original formation pressure, in MPa; is the injection strength, respectively, in m3/d/m; C1, C2, C3 are the initial condition constants.
During the CO2 injection process, there are three-phase seepage of crude oil, supercritical CO2, and free water underground. Considering that only part of the free water enters the reservoir when the fracturing fluid is injected in the initial stage and is almost completely produced during the flowback period, to ensure the solvability of the model, the multiphase seepage is simplified to two-phase seepage of oil–liquid CO2.
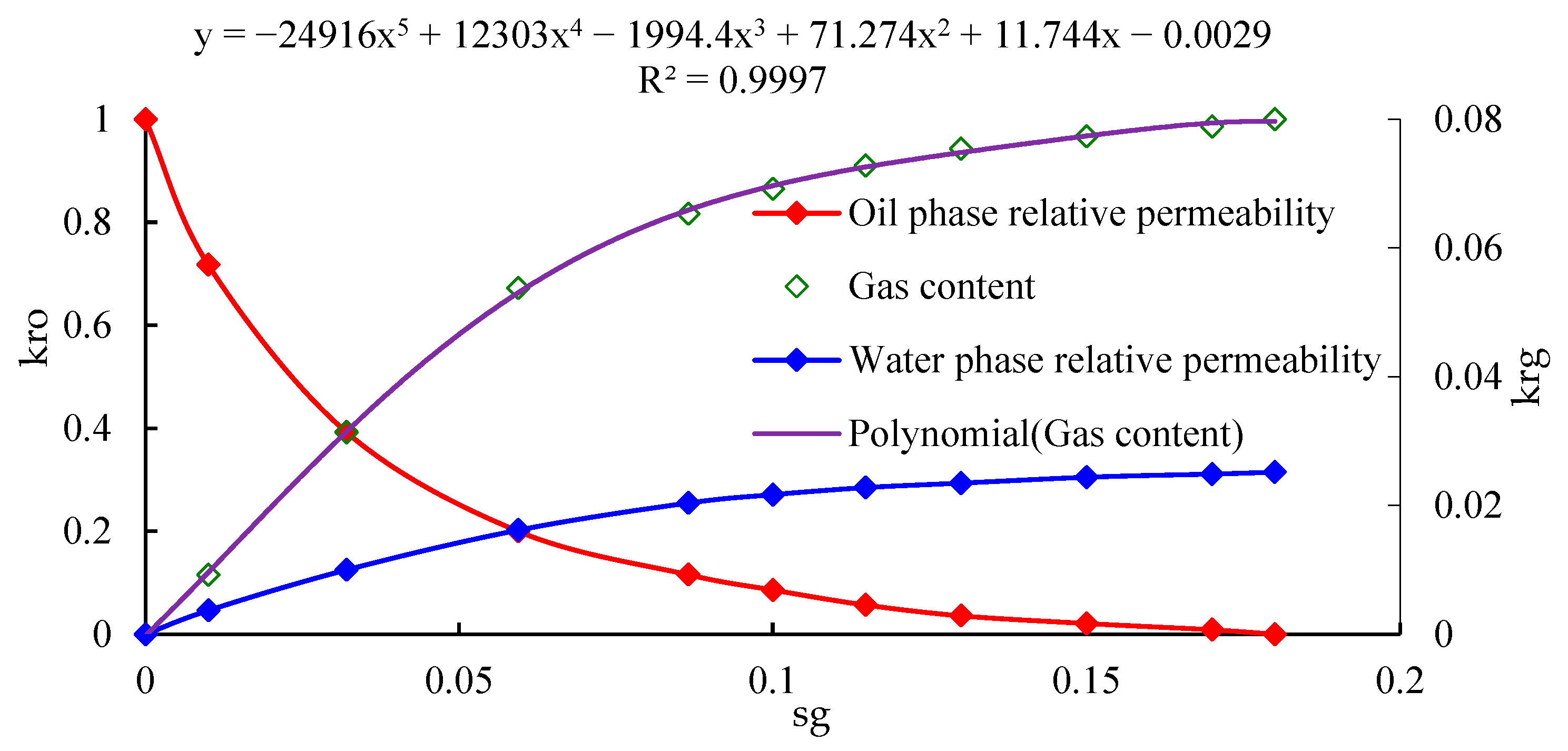
Based on the physical simulation results of the gas–oil two-phase relative permeability test, and by referring to the derivation process of the oil–water fractional flow equation, a gas–oil ratio calculation model is constructed. The processed phase permeability given by the physical simulation and the relationship graph between the derivative of the gas content and the gas saturation is shown in
Figure 2.
By using the gas–oil two-phase gas content fractional flow equation and combining it with the phase permeability, the gas content under any gas saturation can be determined. Therefore, the key to determining the gas–oil ratio is how to determine the gas saturation. The calculation equation for the gas saturation at any time after the gas breakthrough is as follows:
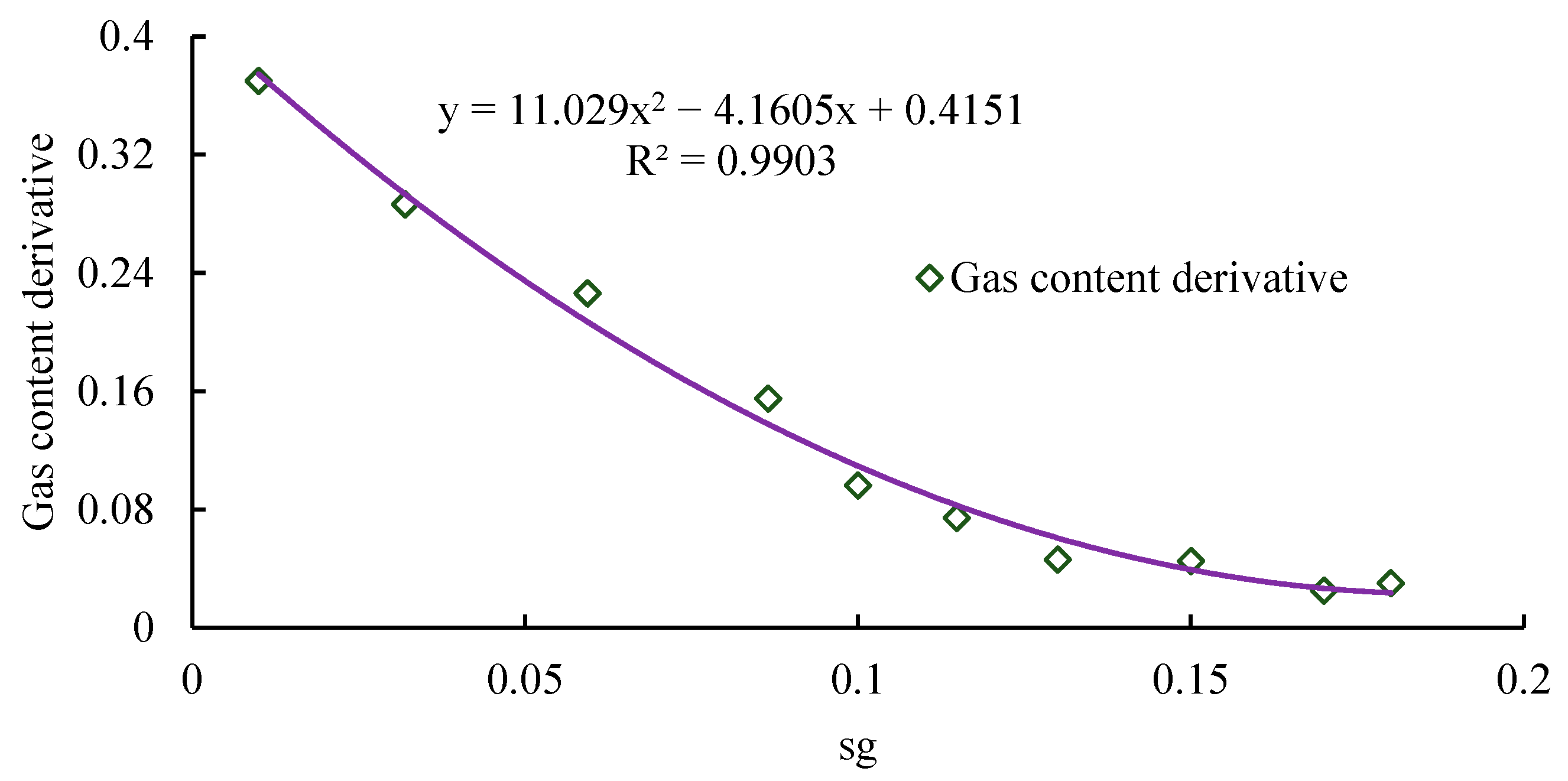
According to the cumulative injection volume at any time, the derivative of the gas content is determined. Then, based on the relationship curve between
fCO2 and
, the relationship graph between
and
is established, as shown in
Figure 3. By fitting, the functional relationship between
and
is determined, and the gas saturation at any time is determined according to the root formula:
By fitting the fractional flow equation, the relationship function between the gas content and the gas saturation is determined. Substituting the gas saturation into the fractional flow equation to determine the gas content, and then converting the gas content into the gas–oil ratio, the gas–oil ratio calculation model after gas breakthrough in CO
2 flooding of tight oil reservoirs is obtained:
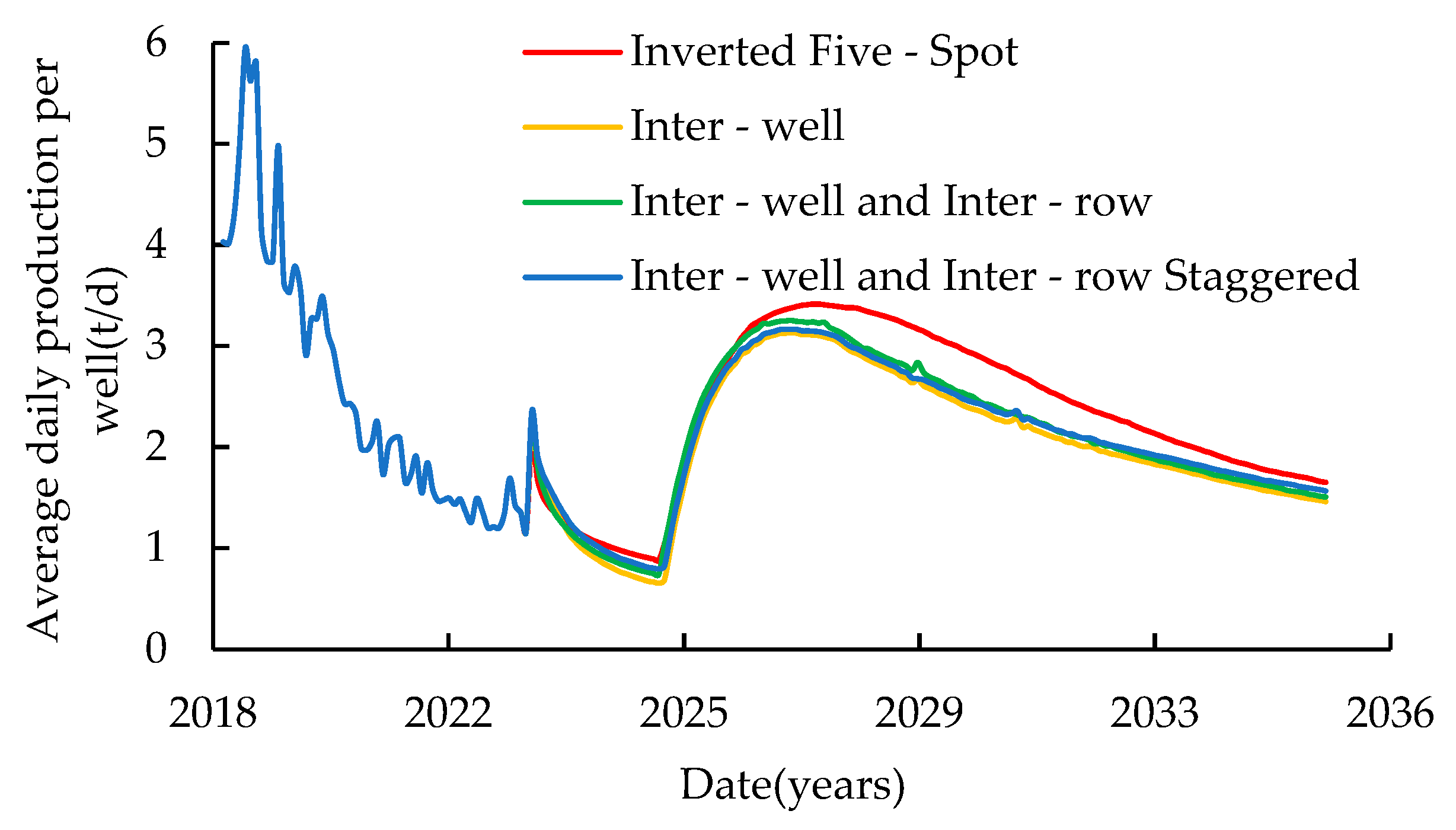
According to the CO2 flooding reservoir engineering model, the oil production and gas–oil ratio at any time can be calculated. Combining with their definitions, the development indicators such as the recovery degree and the oil production rate can be determined.
2.2. Semi-Analytic Solution of Mathematical Model
The solution of the above mathematical model mainly includes two parts: one is the change in formation energy caused by the injection and production volumes of the source and sink, and the produced liquid volume continuously changes; the other is to decompose the produced liquid volume into the produced oil volume and the produced gas volume, that is, to determine the gas–oil ratio. The specific semi-analytical solution method is as follows.
Based on the bottom hole flowing pressure of the production well zone and the original formation pressure of the unmodified area, determine the produced liquid volume at the start-up time; according to the mass conservation equation when combined with the unsteady-state pressure distribution formula, determine the pressure change value at the junction of the unmodified area and the oil well zone in the first time step, which is used as the boundary pressure in the next time step. Calculate the injection volume according to the injection intensity of the injection well zone, and combine with the material balance equation. Since the initial pressure at the junction of the injection well zone and the unmodified area is the original formation pressure, the injection volume is all used to increase the pressure in the injection well zone (the specific pressure increase range and the material conservation equation need to be judged whether they are miscible), thereby determining the pressure change value at the bottom hole of the injection well in the first time step. Based on the bottom hole flowing pressure of the injection well in the first time step and the boundary pressure between the injection well zone and the unmodified area, determine the seepage intensity of the injection well zone flowing into the unmodified area (the injection volume does not all flow into the unmodified area, and part is used to restore the formation pressure in the injection well zone). Judge whether there is a flow into the production well zone to supplement energy in the unmodified area according to the pressure difference at both ends of the unmodified area and the starting pressure. If Δpm > G, there is energy entering the production well zone and the supplement is completed; if Δpm < G, the injection volume in the injection well zone is insufficient to increase the pressure in the unmodified area to overcome the starting pressure. At this time, the average pressure in the injection well zone continues to rise, and the formation pressure in the production well zone continues to decline until the pressure difference is greater than the starting pressure. Based on the key position index calculation in the first time step, the index in the next time step can be iteratively calculated. Overall, it is manifested as: the pressure in the production well zone decreases; the pressure in the injection well zone rises; the driving system is gradually established in the unmodified area; and the continuous change and solution of the injection volume-produced liquid volume are realized.
Qualitatively judge the miscible driving situation in the injection well zone. The pressure in the injection well zone is high, and the formation pressure conduction is fast after fracturing, so it is easier to form miscible or near-miscible driving. The specific situation is judged by the calculated formation pressure; in the unmodified area, it is partially miscible or immiscible. In the part close to the injection well zone, when the energy propagates, there may be partial miscible driving, while in the part close to the production well zone, the slow pressure propagation leads to immiscible gas–liquid two-phase flow; although the energy in the oil well zone can be supplemented in the later stage, it is difficult to reach the miscible driving of about 28 MPa, and it is in the state of immiscible gas–liquid two-phase flow. Based on this, the multiphase flow state in each region is divided. According to the above method, determine the flow rate in each region at any time, that is, the cumulative injection volume. Judge whether the injected CO2 phase reaches the production well (that is, whether gas breakthrough occurs) according to the cumulative injection volume. Before gas breakthrough, the production well is in pure oil flow, and the formation pressure declines; after gas breakthrough, calculate the rising derivative of the gas content according to the flow rate, combined with the fitted relationship to determine the gas saturation at the production well position, and then calculate the gas content in reverse, and finally realize the quantitative calculation of the gas–oil ratio. Combine the dynamic calculation results of the gas–oil ratio and the flow rate calculation results to determine the change laws of the oil production and the gas–oil ratio before and after gas breakthrough.
2.5. Geological Model
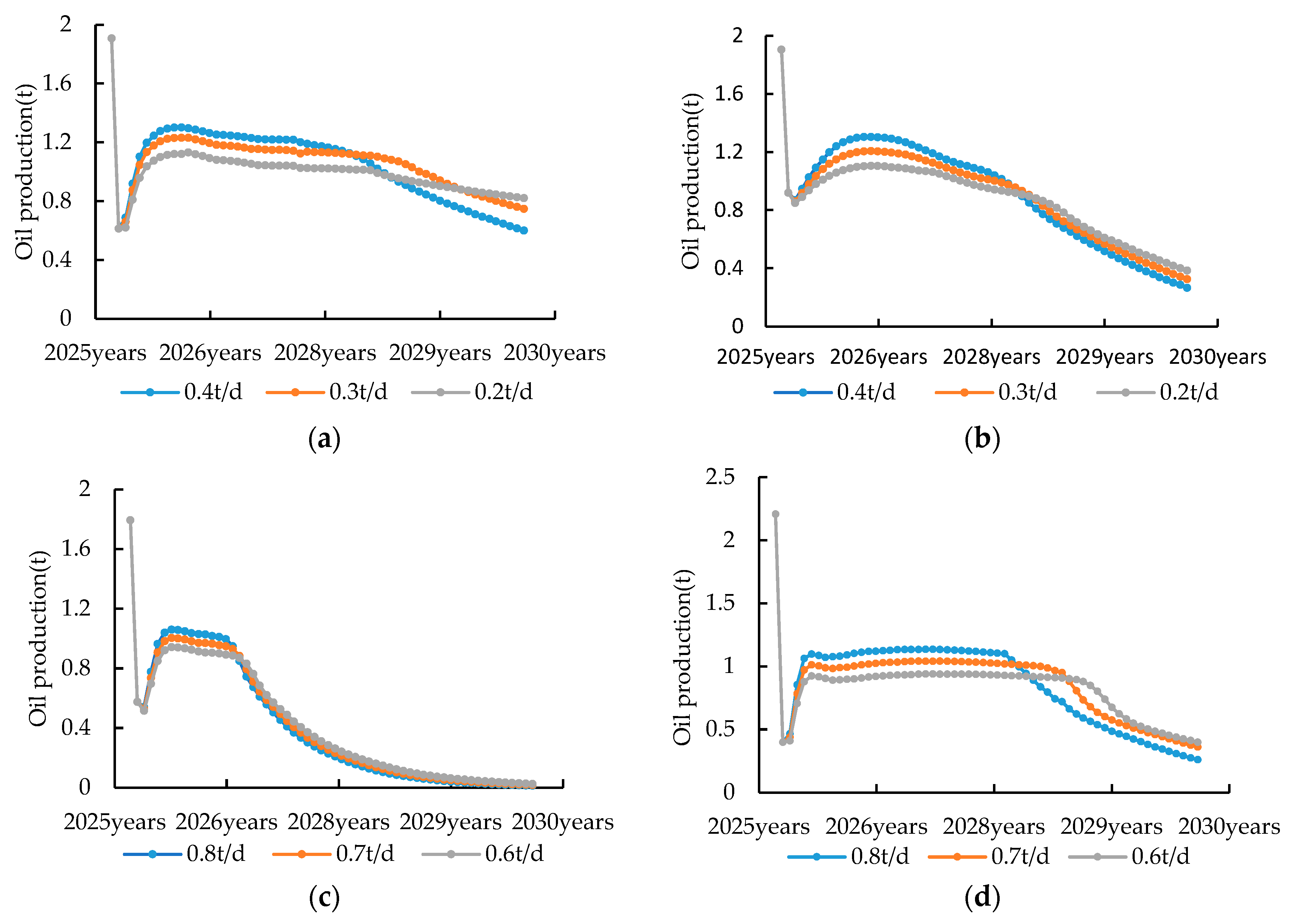
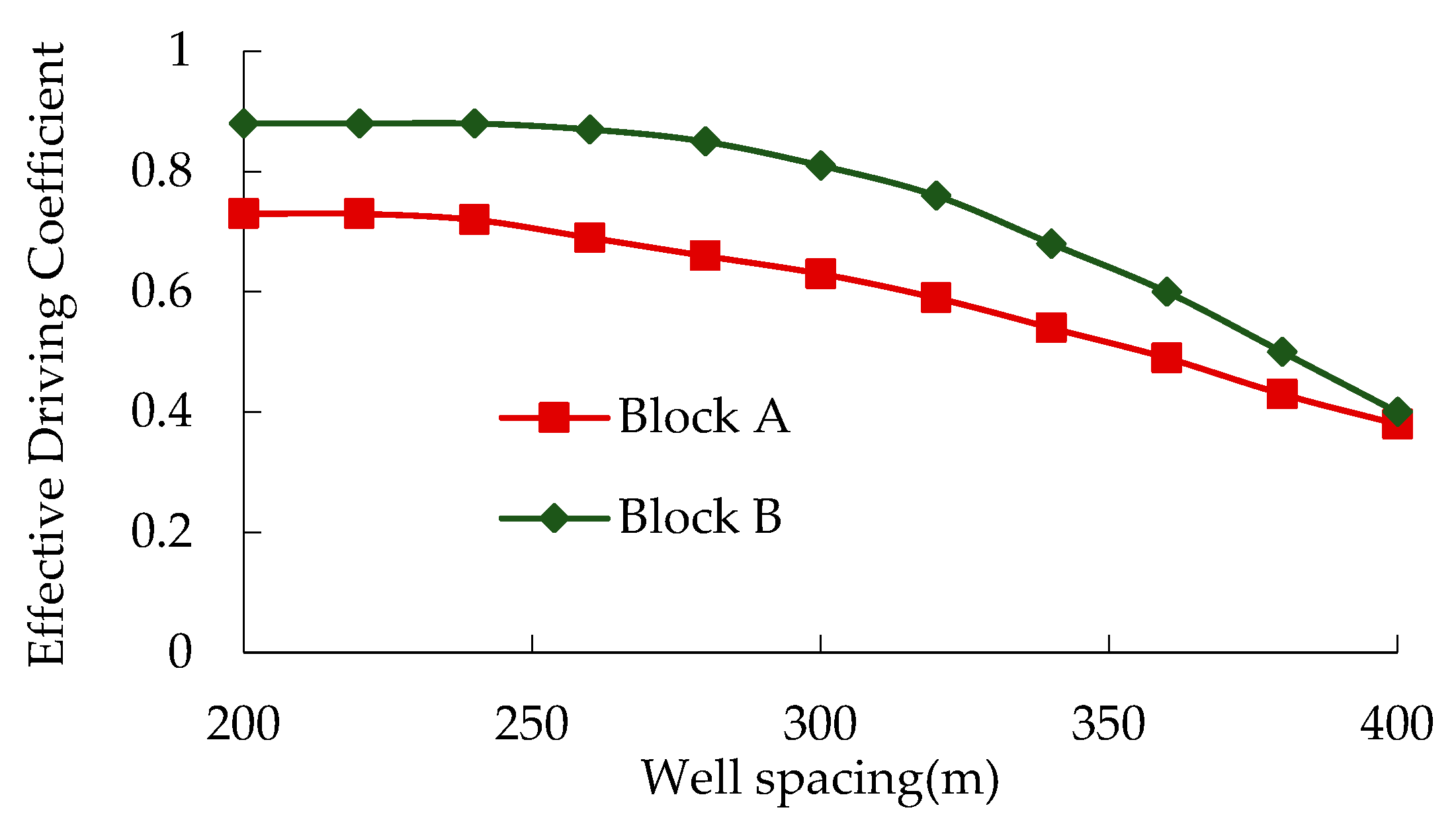
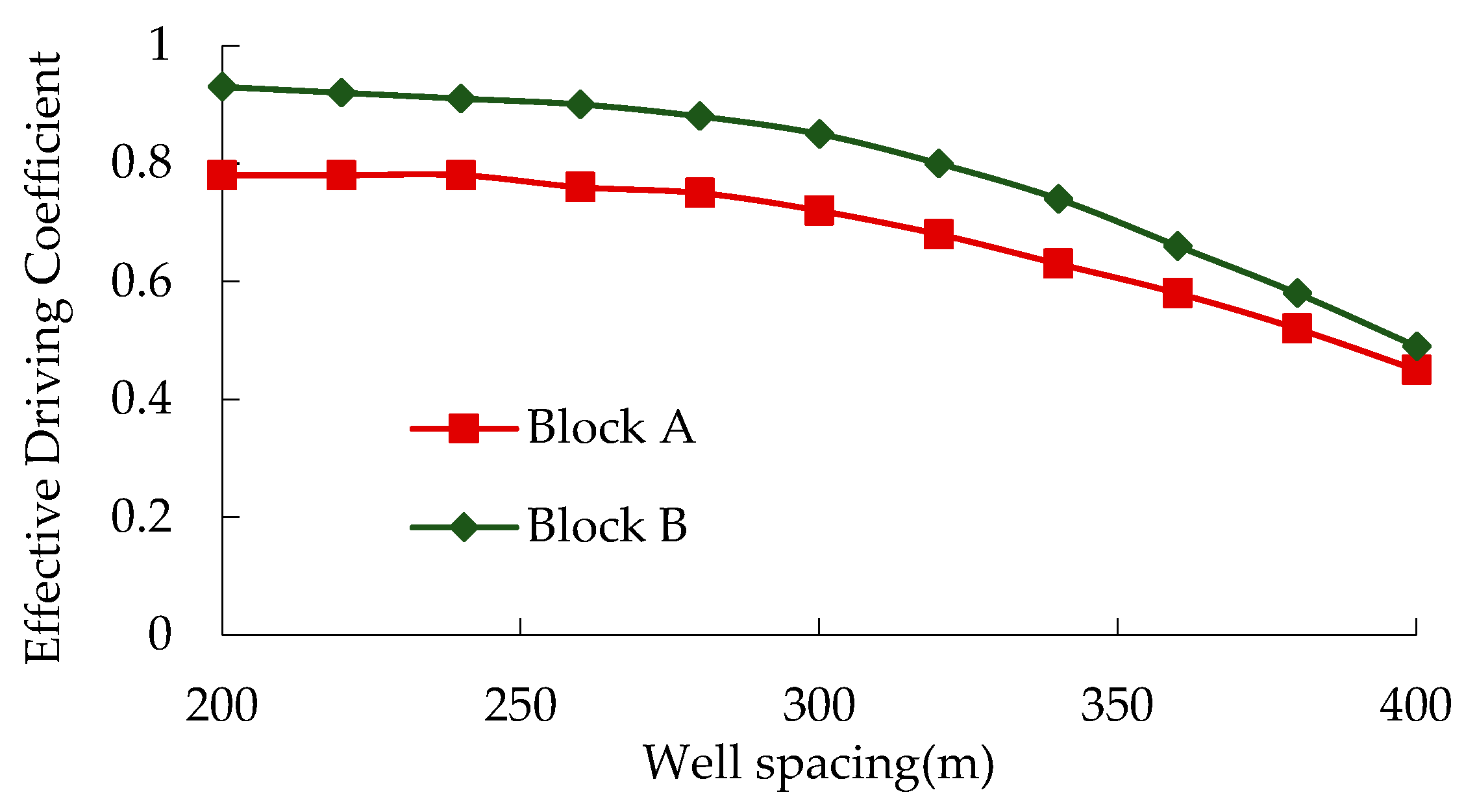
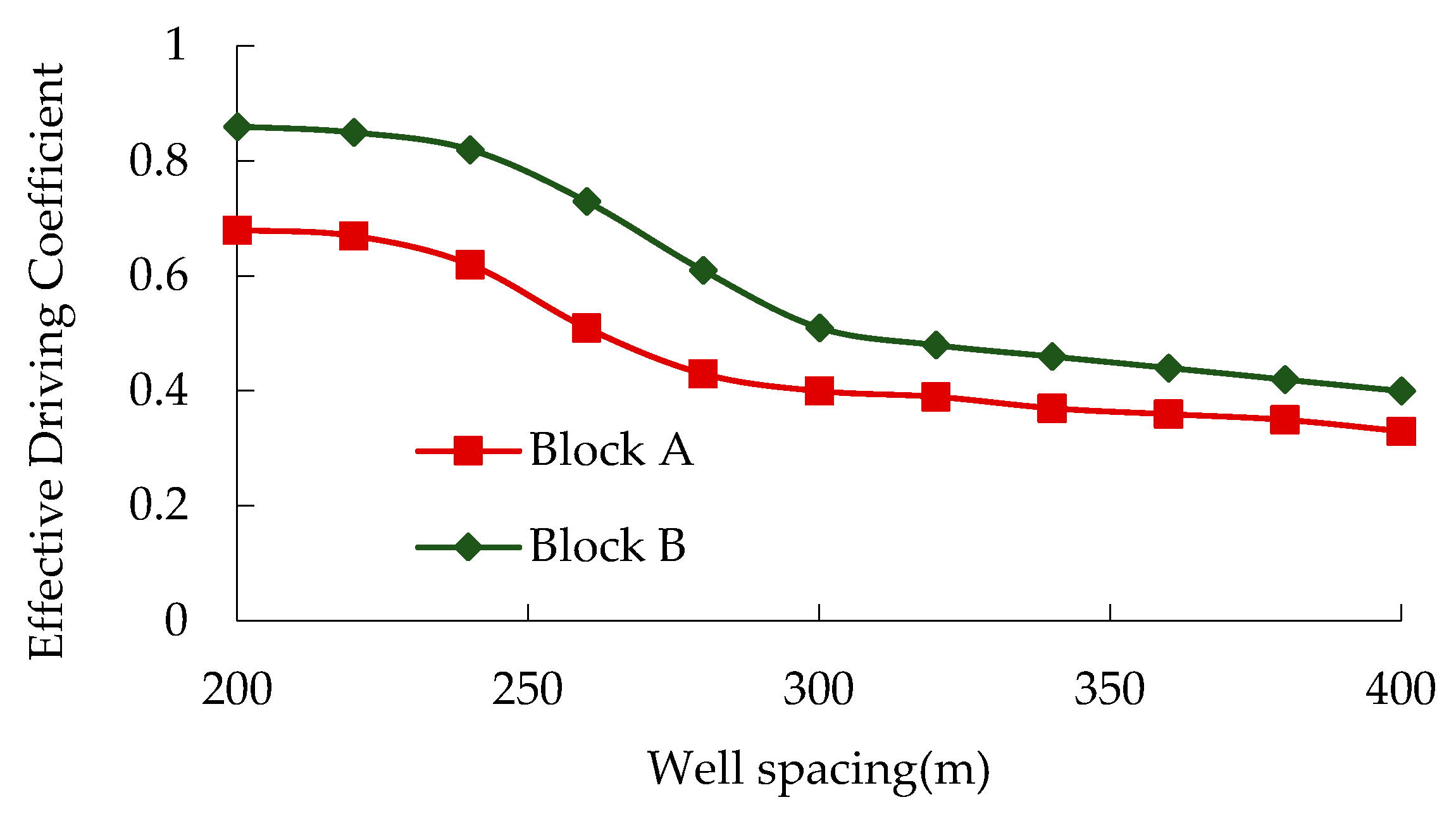
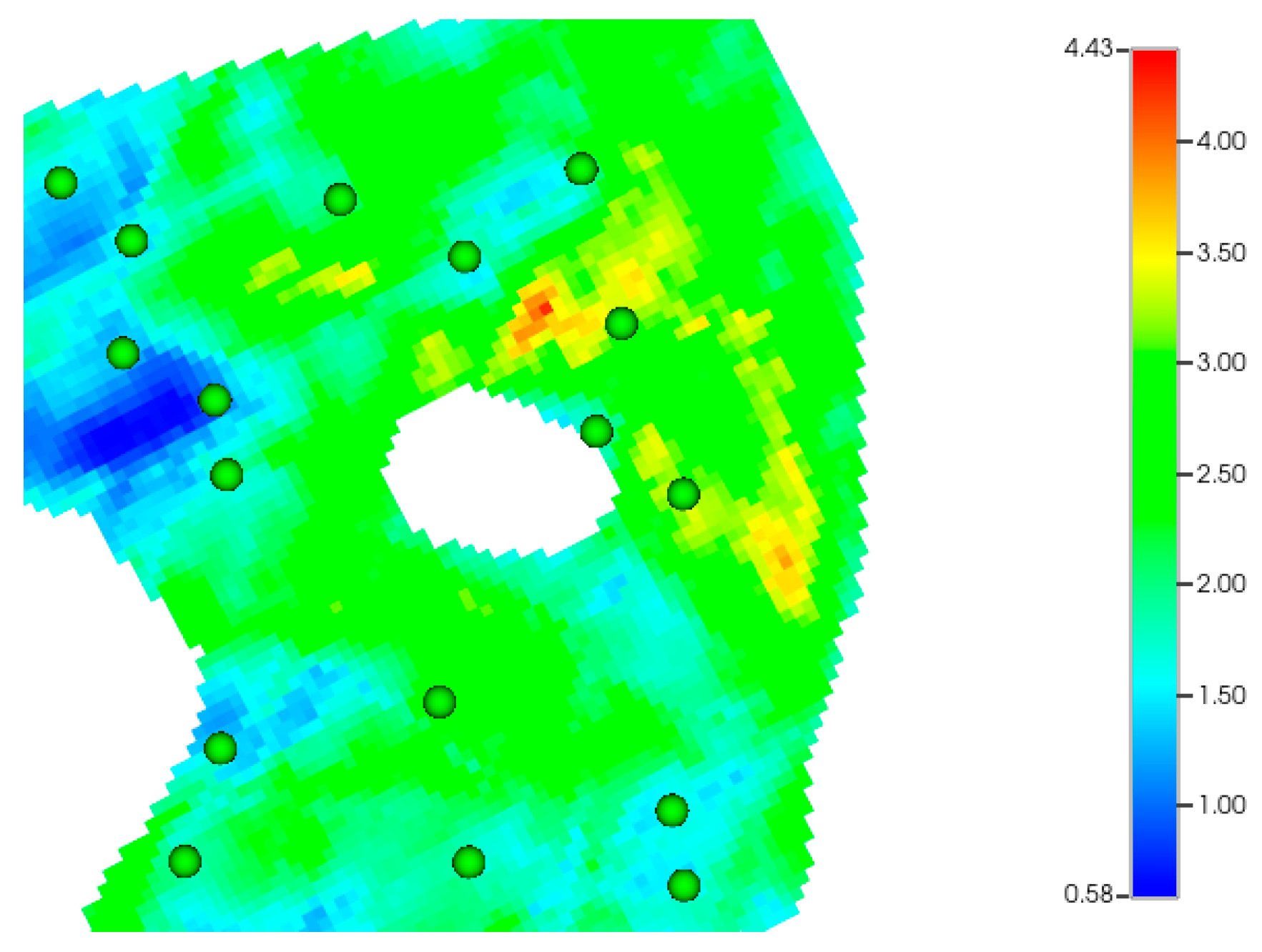
The study area includes the target Block A and the target Block B, which are developed with natural energy. The formation energy declines rapidly, with a decline rate of more than 30%, and the predicted recovery factor is less than 10%. Block A belongs to ultra-low permeability, with underdeveloped fractures and small-scale sand body development, making it difficult to establish an effective driving system. An excessive injection rate will lead to gas channeling, while too slow injection rate will result in slow pressure recovery in the oil well area, inability to supplement the formation energy, and inability to achieve miscibility in the oil well area.
Based on the static data of the target blocks, the parameters required for the model are selected, and the geological models of Block A and Block B are established using the Petrel2021 (Schlumberger, Houston, TX, USA) software. The plane grid step size is 20 m × 20 m, and the three-dimensional grid is generated using the pillar-griding module with the edge of the sedimentary facies as the boundary. The grid processing results of the modeling area are shown in
Table 4.
The CMG2021 (for Canada) software is used to perform history matching on the model. The calculation error of the oil production in the whole area is less than 5%, and the error of the formation pressure in the whole area is not more than 4%. The history-matching accuracy meets the prediction requirements.
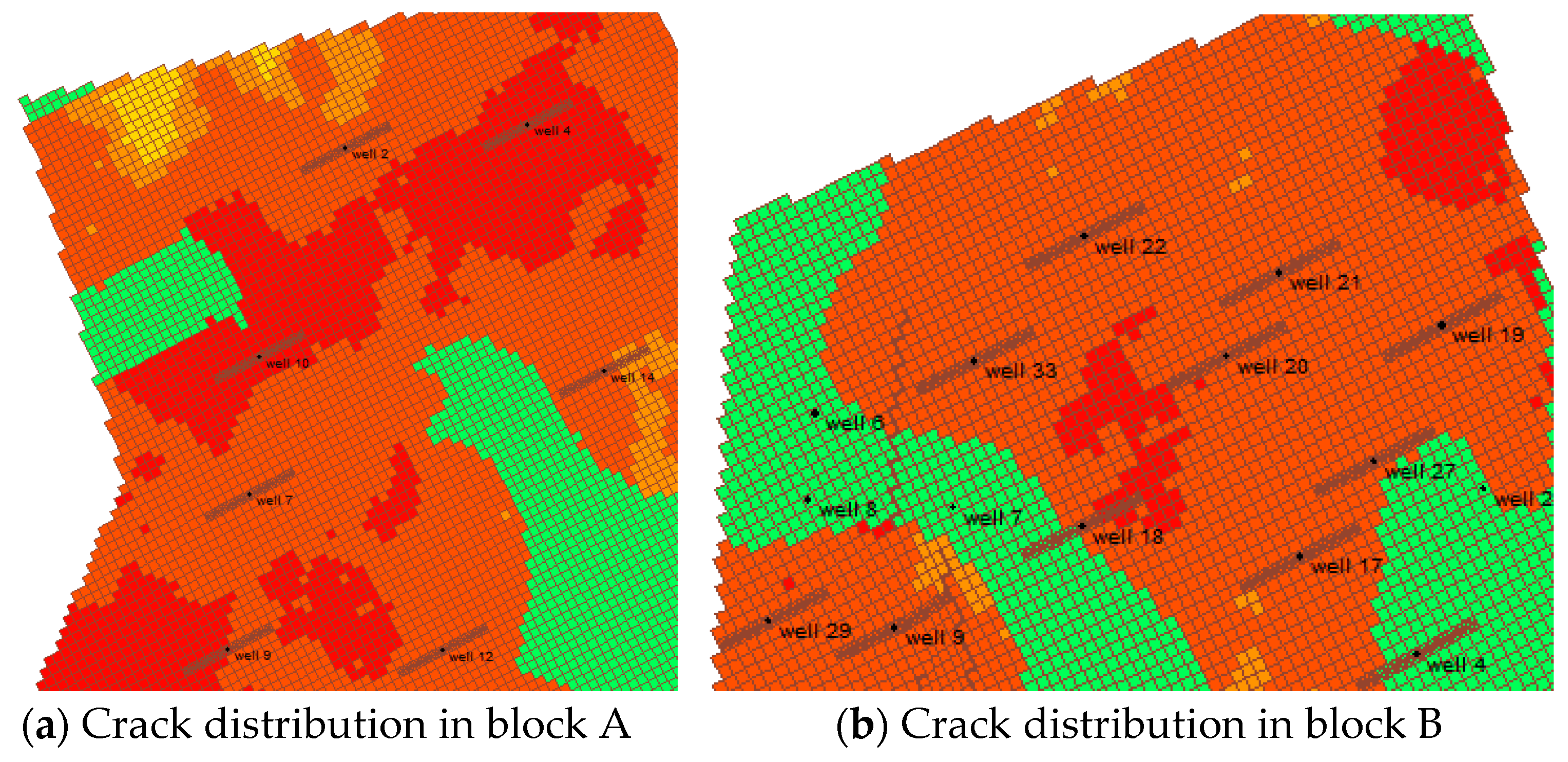
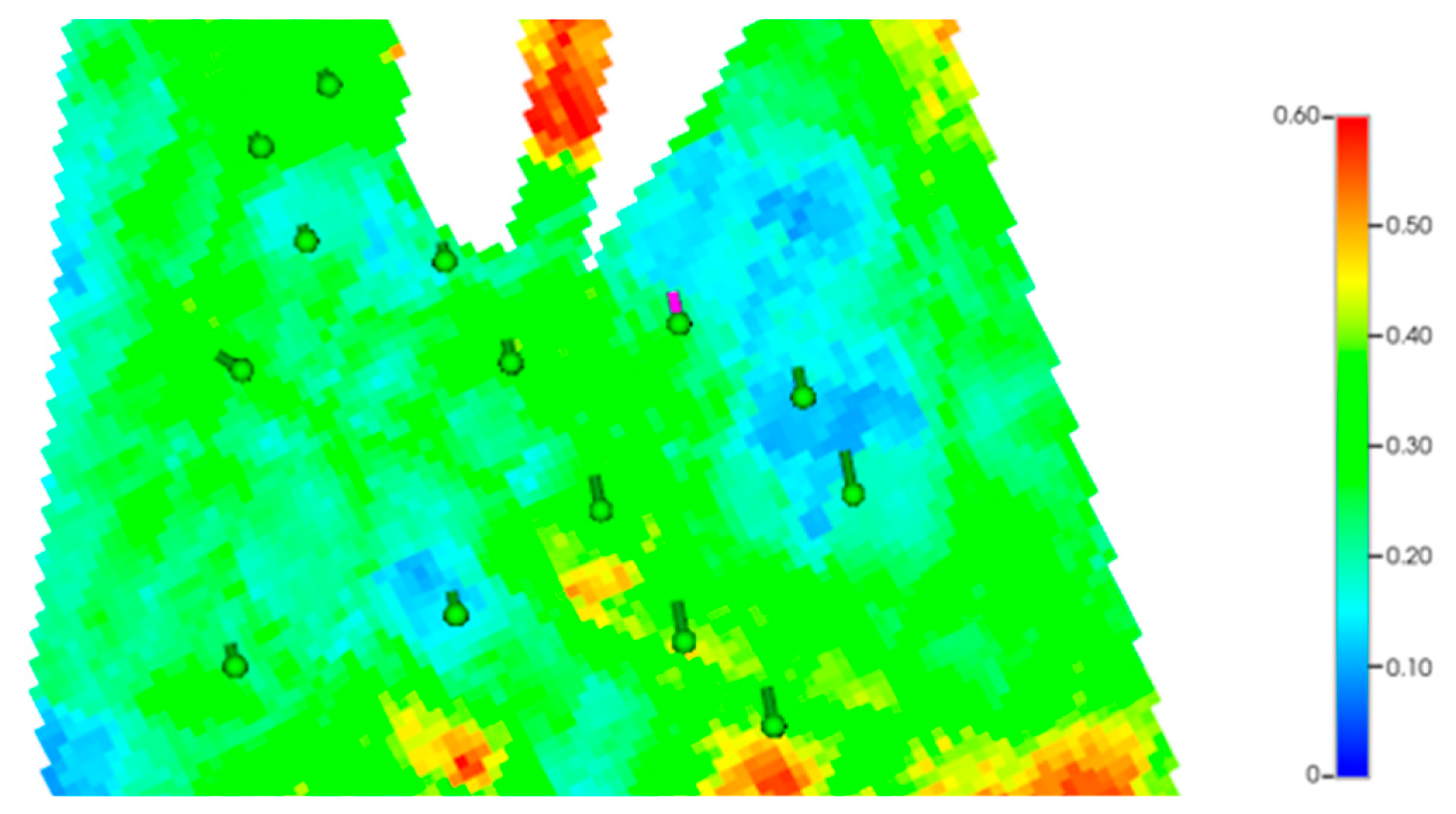
In the fractured development and stimulation zone of tight oil reservoirs, there are main fractures and induced fractures. Based on the fracturing construction summary data provided by the site, the main fractures and the induced fractures in the stimulation zone are simulated using the hydraulic fracturing module in the CMG numerical simulation software. The fracture parameters and setting interfaces of each block are shown in
Table 5, and the fracture distribution is shown in
Figure 6.
2.6. Determination of Effective Driving Coefficient of Different Well Patterns
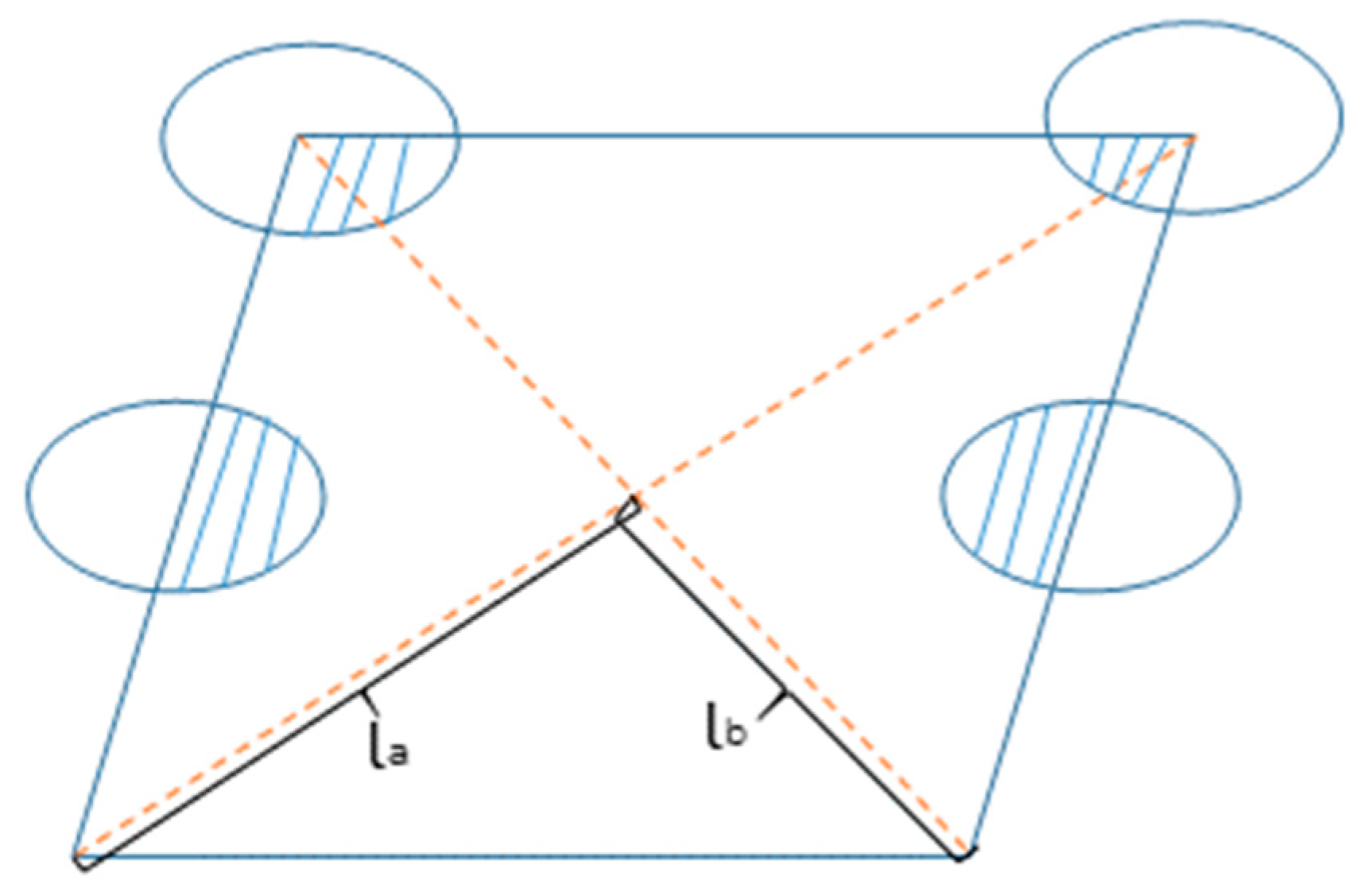
The effective driving coefficient of the well pattern (also known as the utilization coefficient) is the ratio of the producible area to the well pattern area when considering the starting pressure gradient. Based on the possible infill methods and the diamond-shaped anti-nine-point well pattern, the calculation formulas for the effective driving coefficient when the well pattern is consistent with or at an angle to the fracture direction are determined. The results of the anti-five-point method, as a special case of the diamond-shaped anti-nine-point method, are directly given.
For the well pattern with the well row direction consistent with the fracture direction, the long axis direction of the elliptical seepage field after the oil well fracturing is consistent with the well row direction. Taking the diamond-shaped anti-nine-point well pattern as an example, with the fracture length as the focal length and the radial flow driving radius as the short axis of the ellipse, the sum of the elliptical driving radius and the shaded area formed by the well pattern is the producible area of this well pattern, as shown in
Figure 7.
The calculation formula for the effective driving coefficient is:
where
is the movable area, in m
2; S is the control area of the diamond-shaped anti-nine-point well pattern, in m
2;
is the effective driving coefficient.
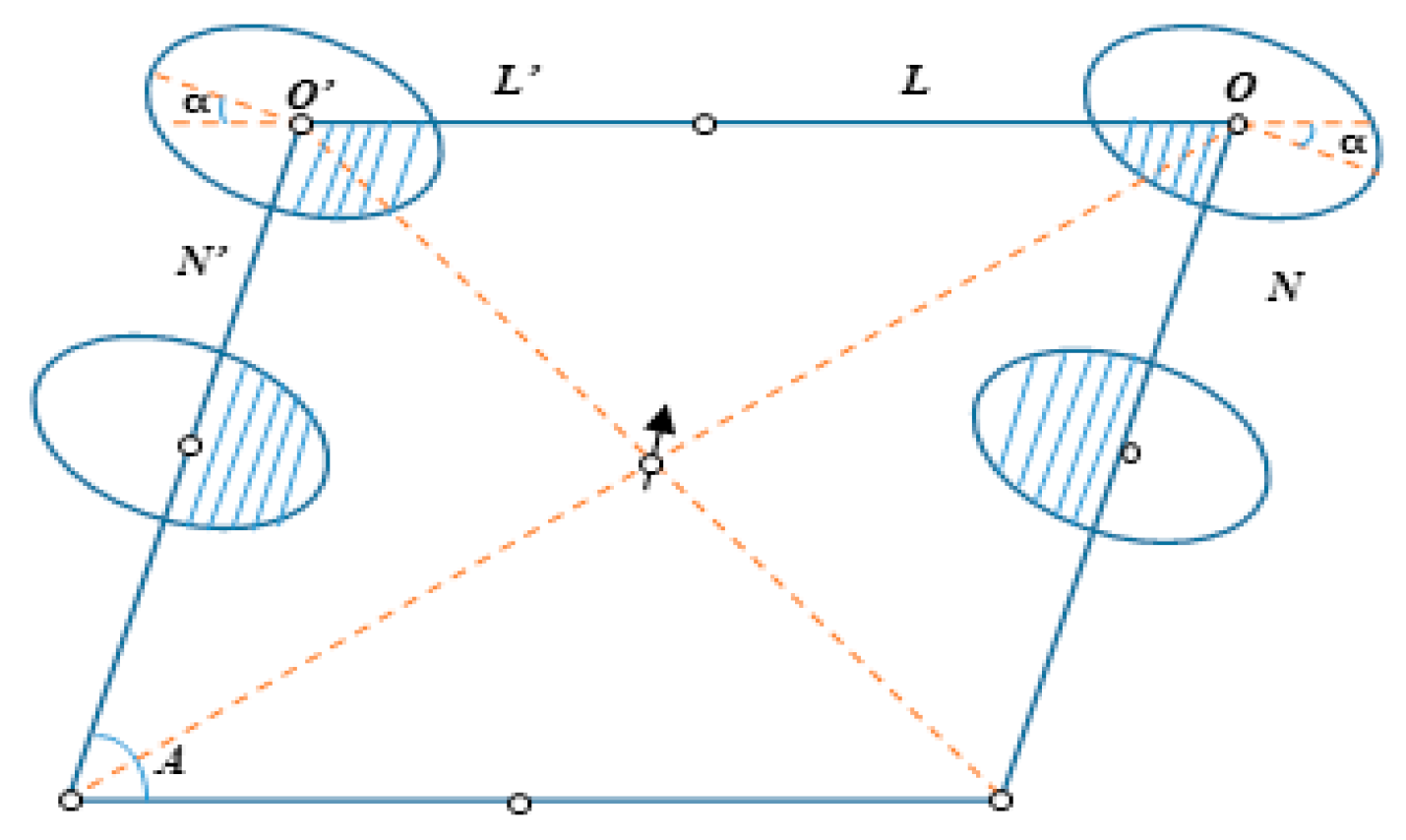
For the well pattern with the well row direction at an angle to the fracture, take the diamond-shaped anti-nine-point well pattern as an example, as shown in
Figure 8.
The calculation formula for the effective driving coefficient is:
The five-point well pattern, the diamond-shaped well pattern, and the square anti-nine-point well pattern are all special forms of the above formula. When applied to the calculation of different well patterns, only the angle between the fracture and the well pattern needs to be changed. This formula can be extended to different well patterns in other oilfield blocks.