Theoretical Modeling and Analysis of Energy Recovery Efficiency and Influencing Factors of Energy Recovery Ventilators Under Dynamic Thermal Environments
Abstract
1. Introduction
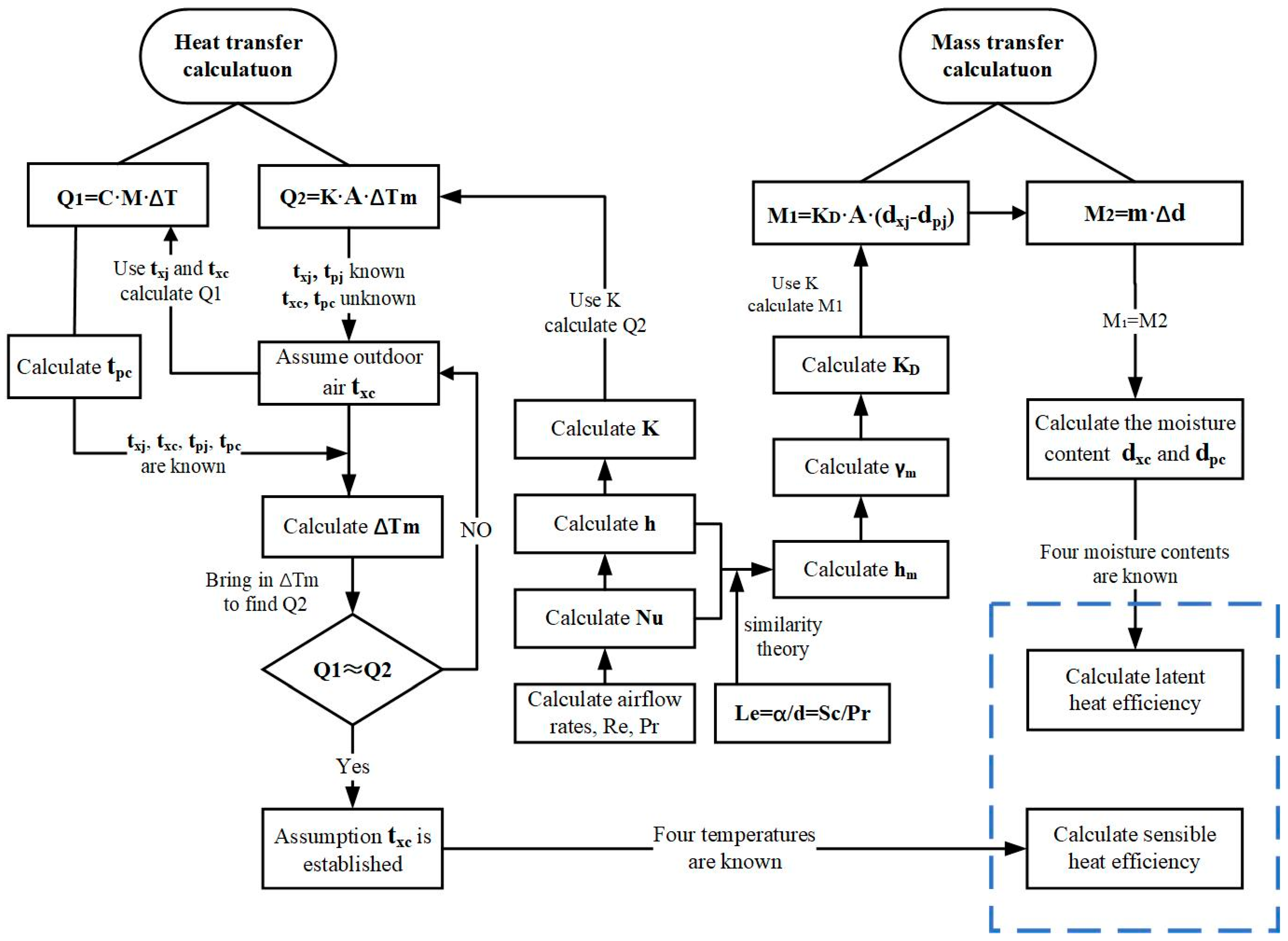
2. Mathematical Model of Heat and Mass Transfer
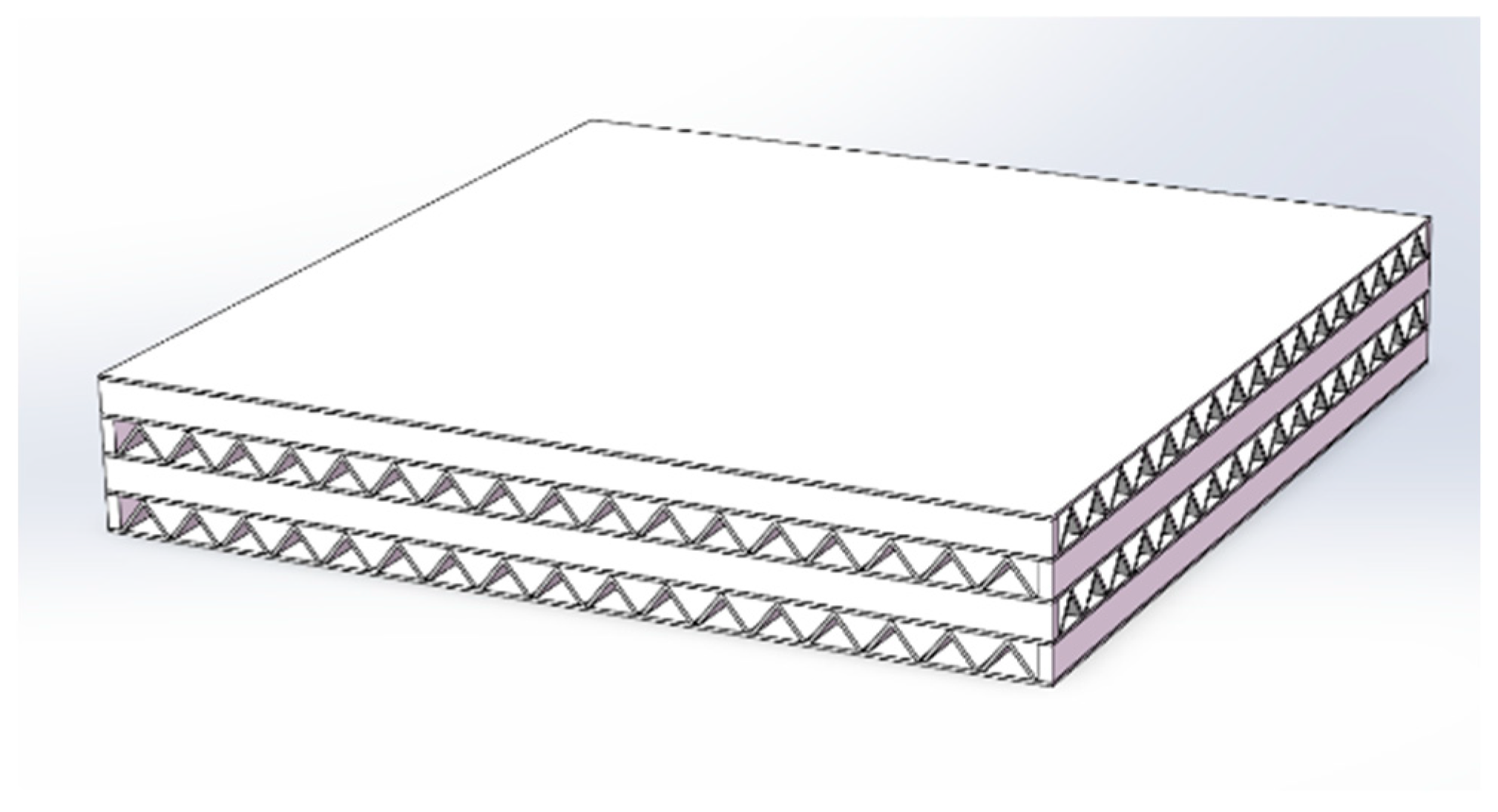
2.1. Physical Model
2.2. Ventilator Core Thermal Performance Analysis
2.3. Heat Transfer Model
2.4. Mass Transfer Model
3. Mathematical Model Verification
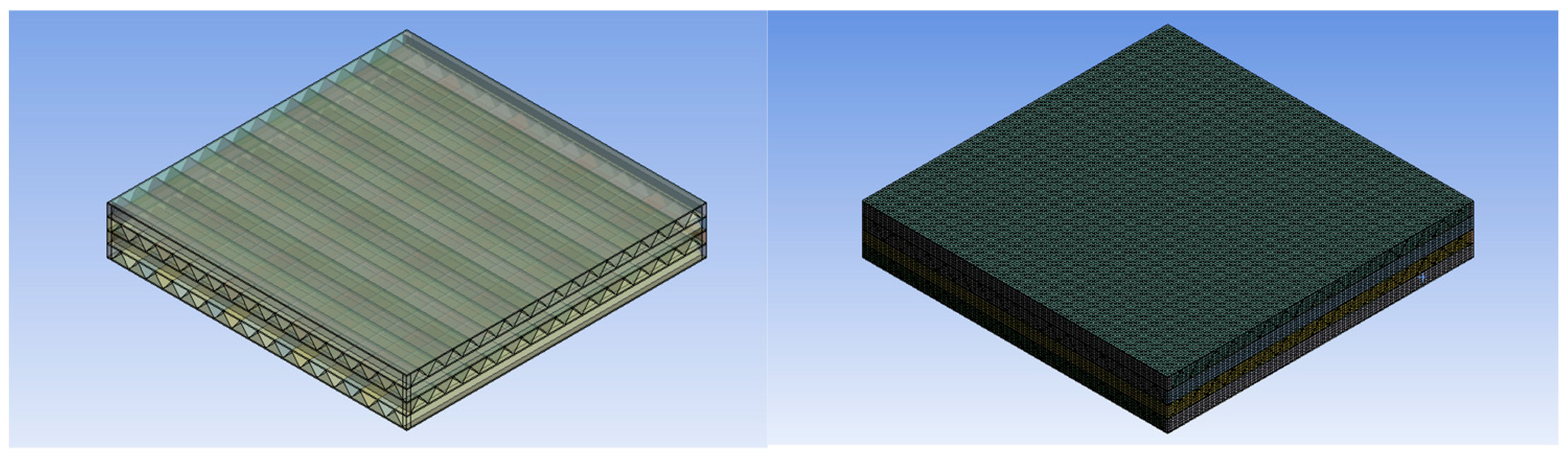
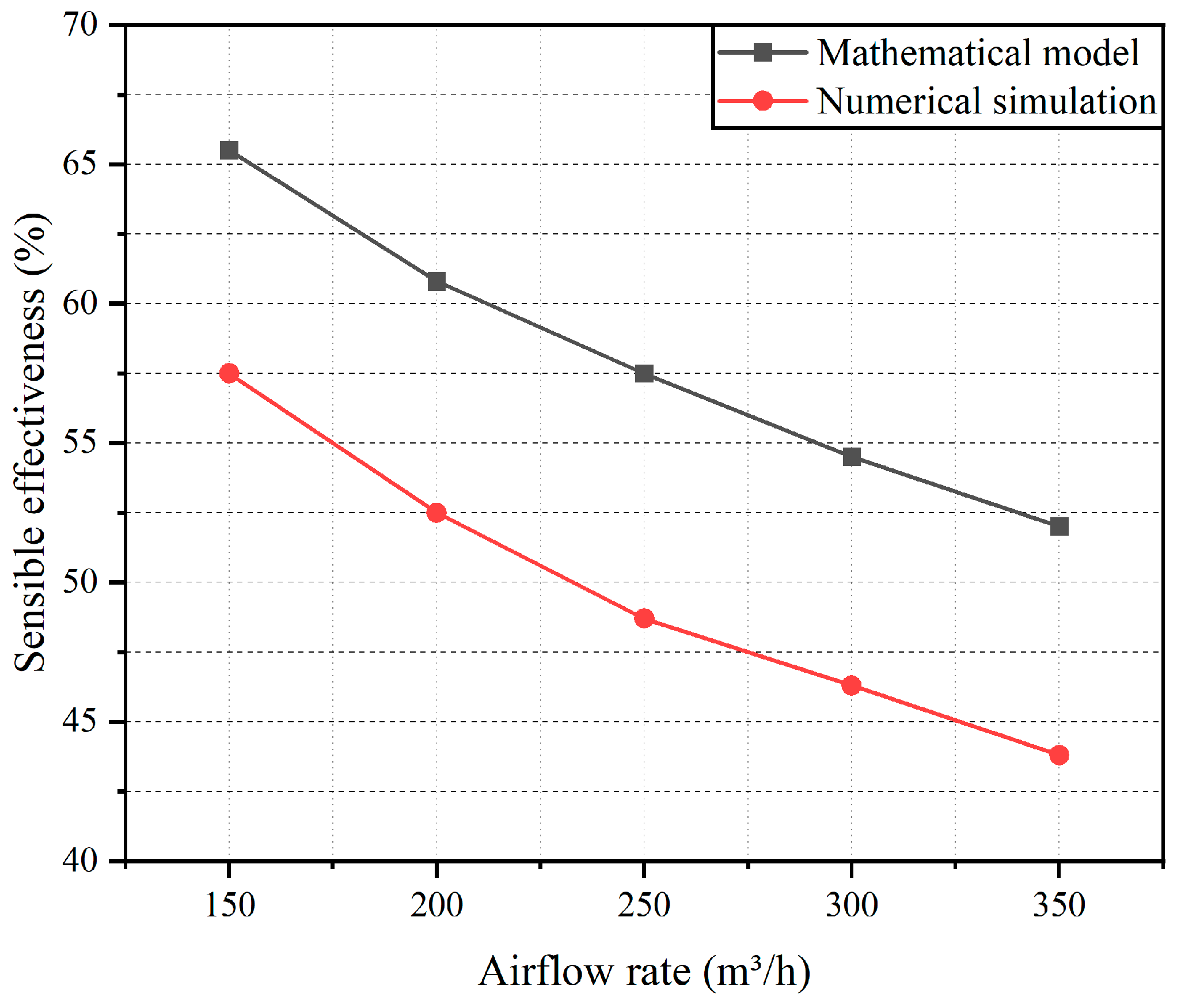
3.1. CFD Simulation
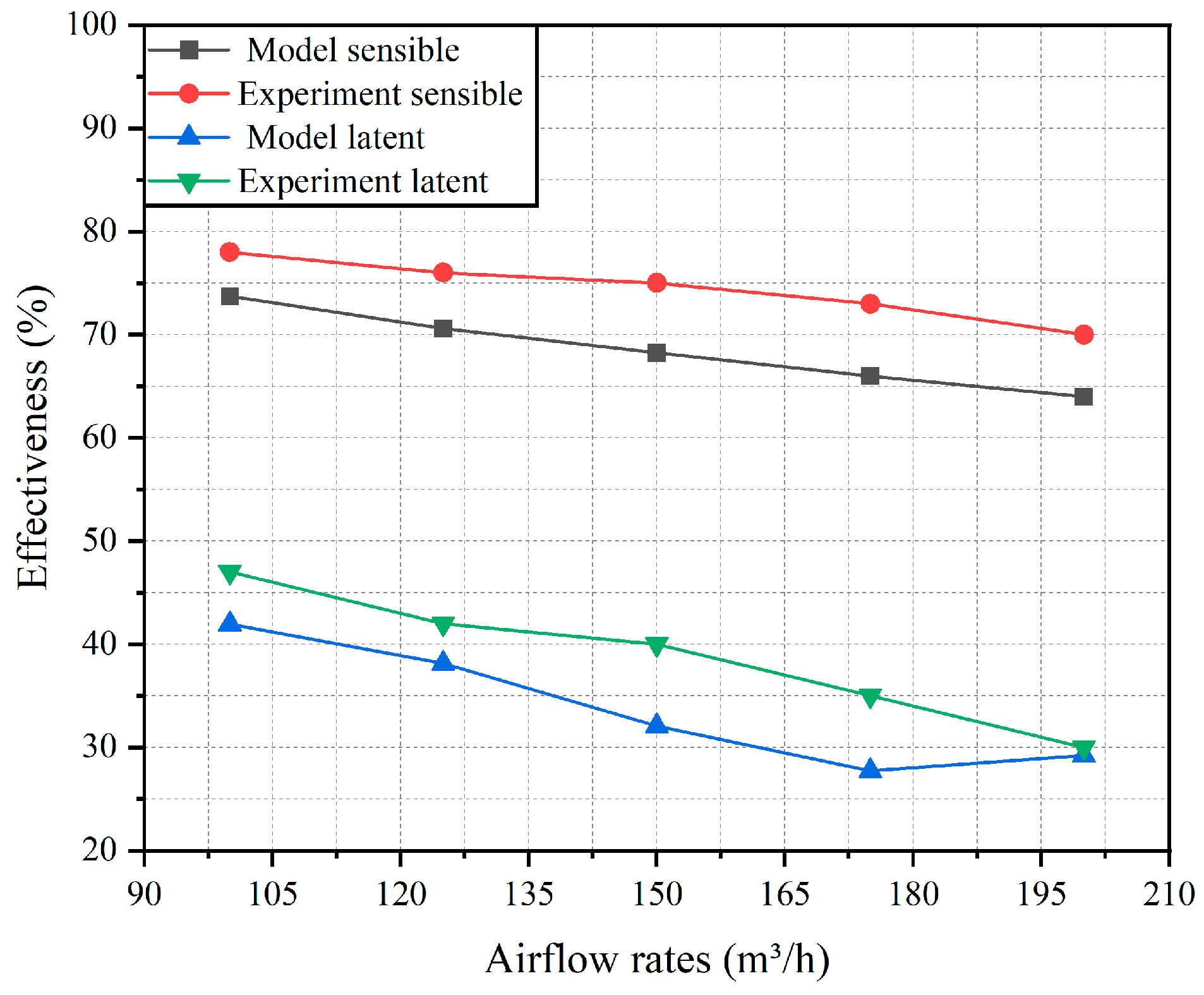
3.2. Experimental Research
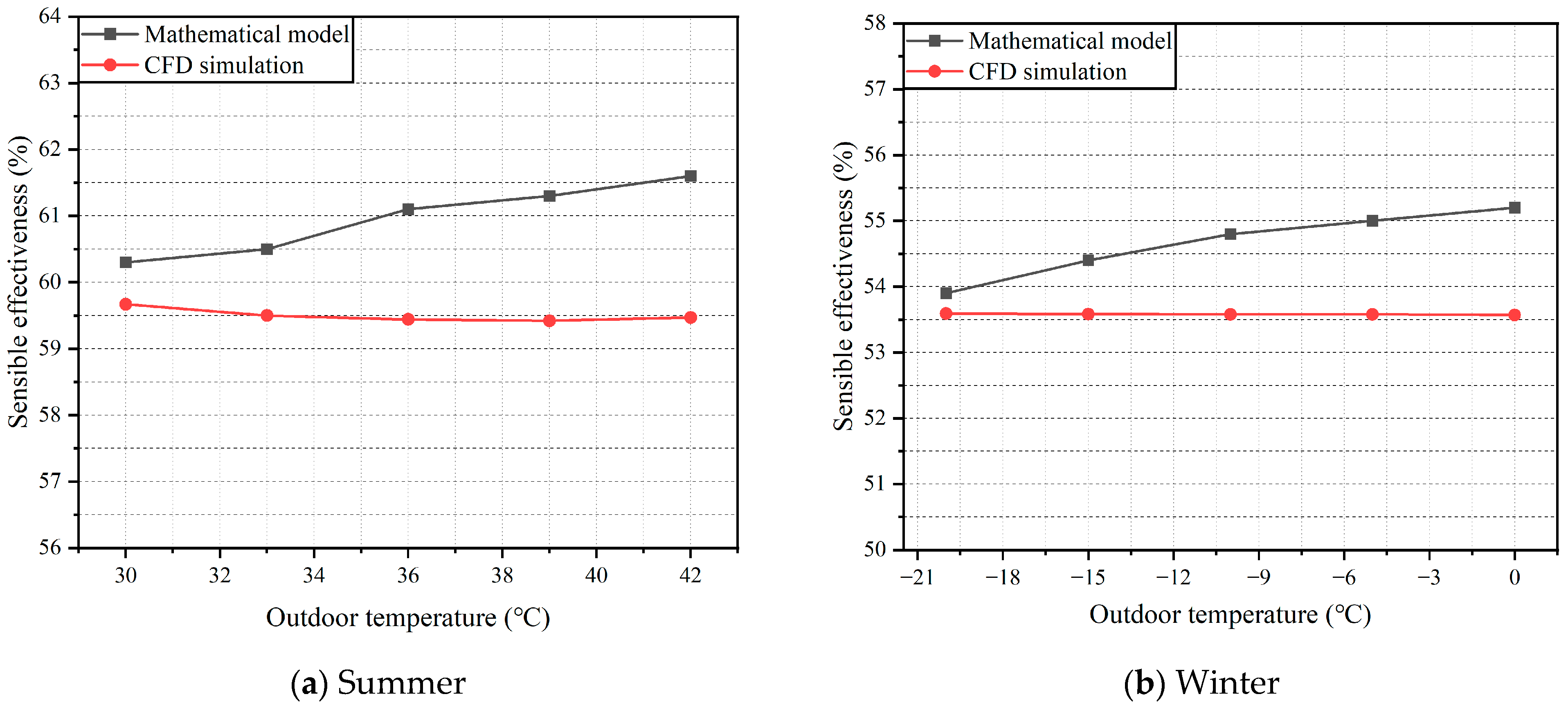
3.3. Validation Results
4. Influencing Factors Analysis
4.1. Material Thermal Conductivity and Mass Diffusion Coefficient
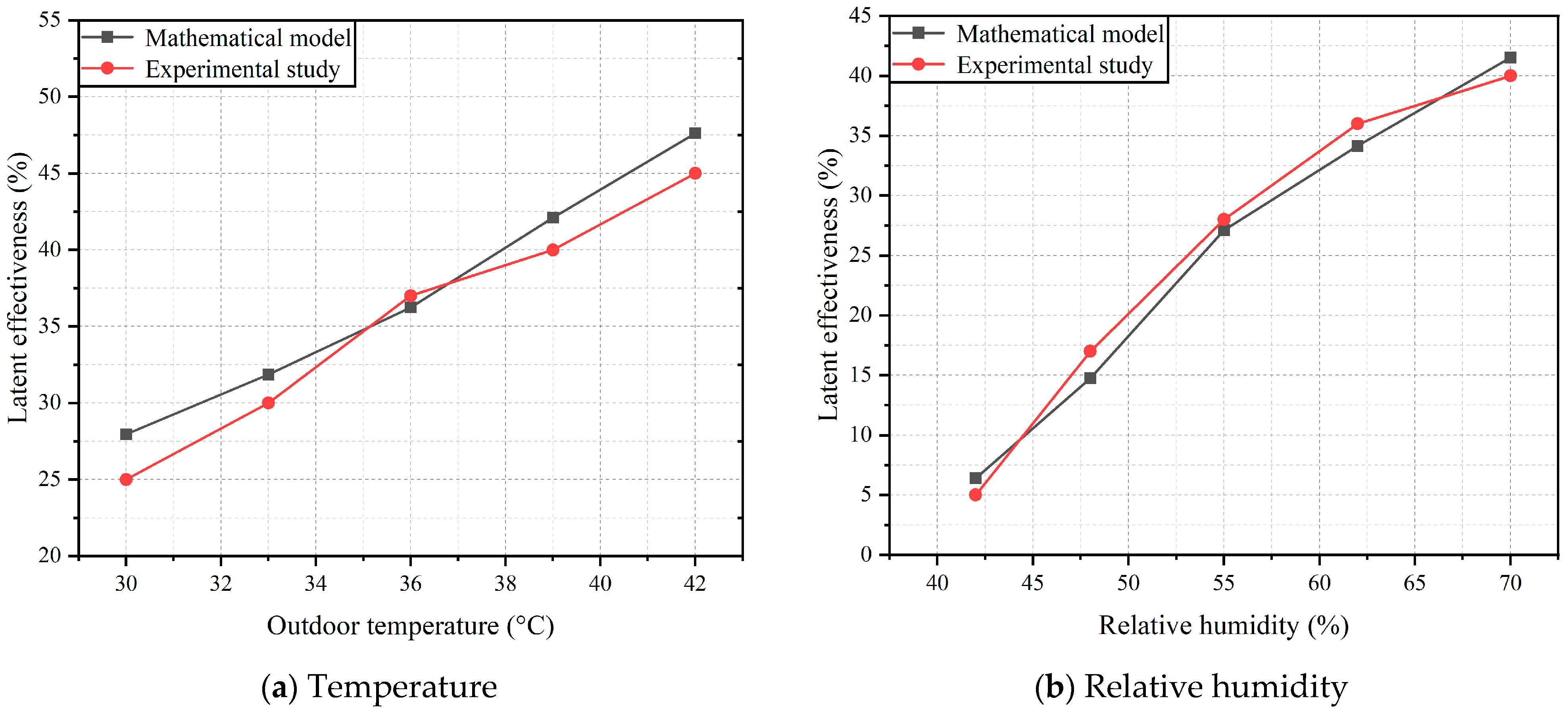
4.2. Effect of Outdoor Air Temperature on Efficiency
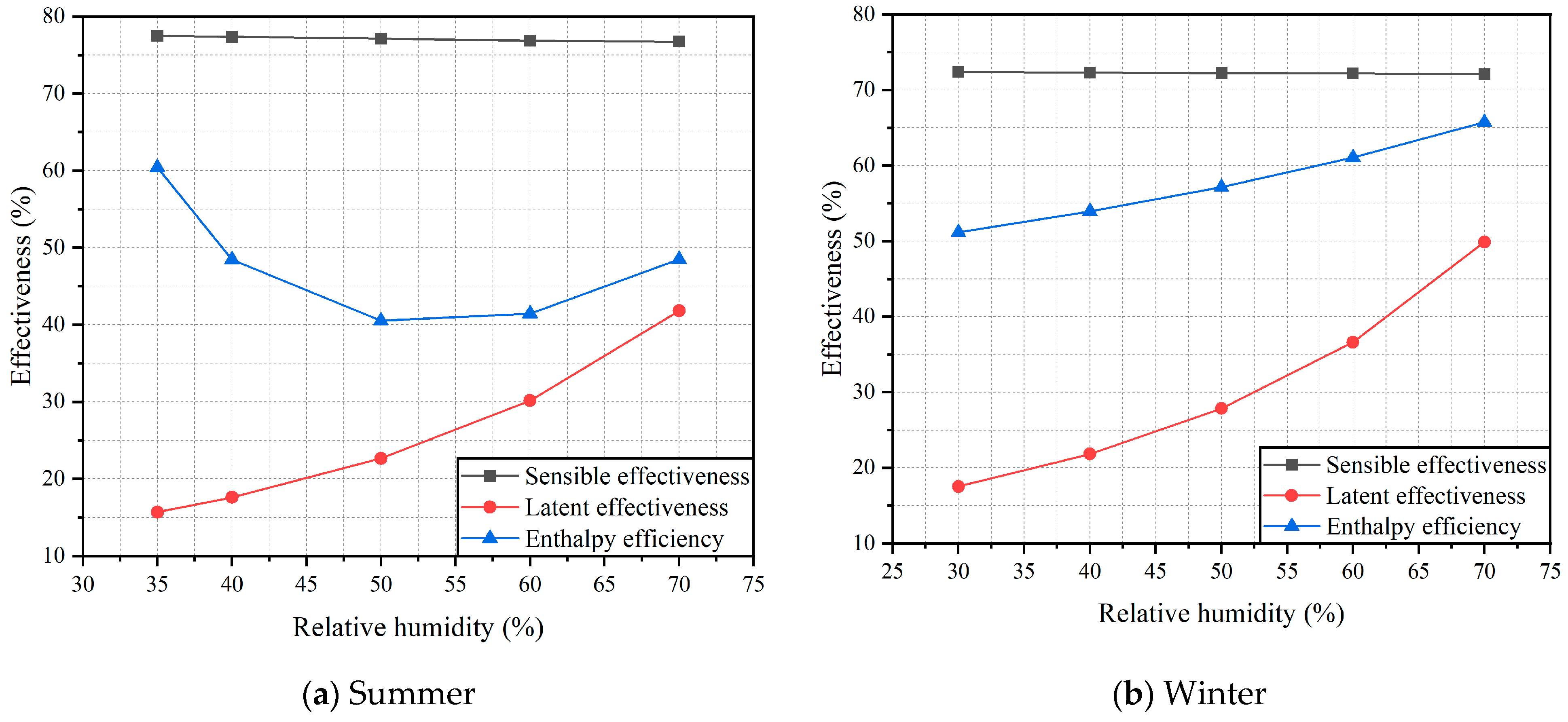
4.3. Effect of Relative Humidity on Efficiency
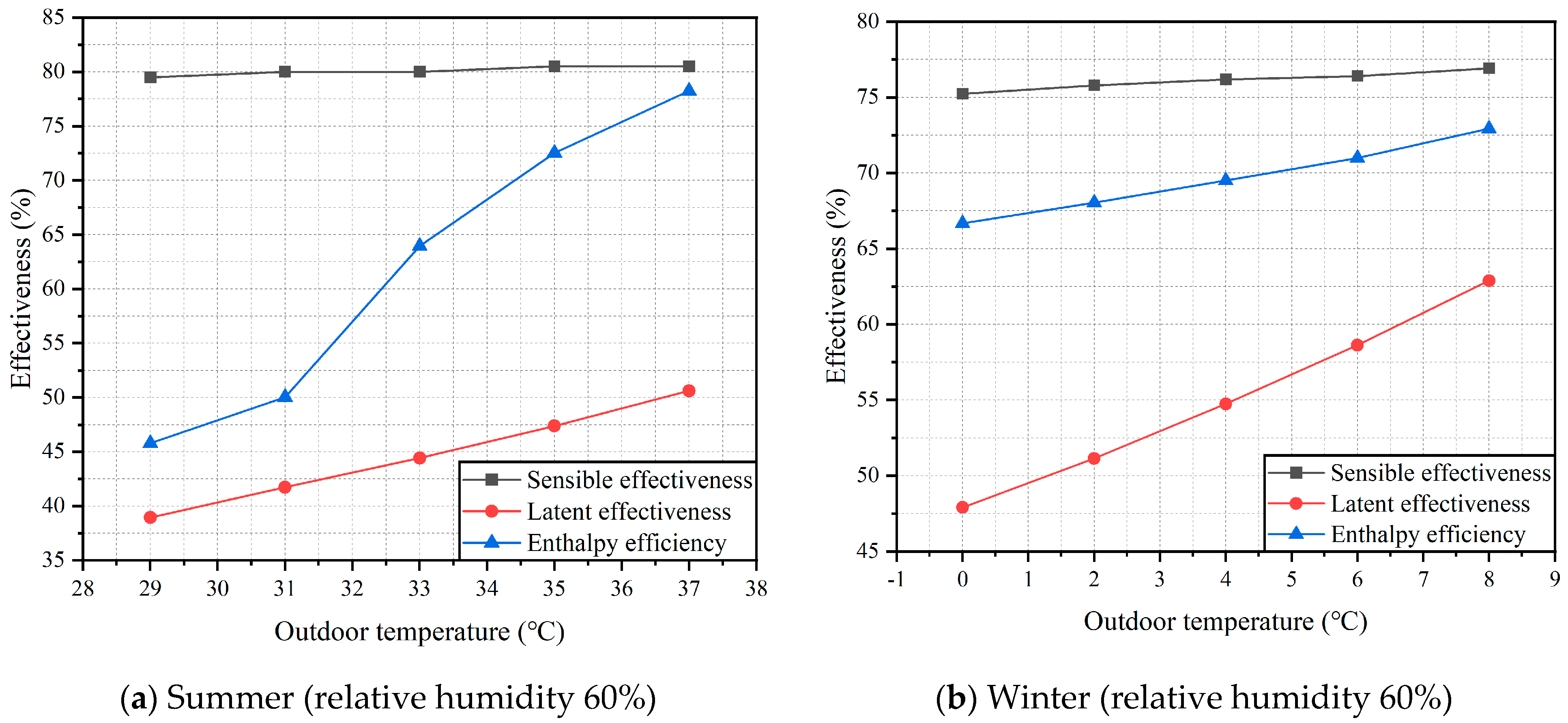
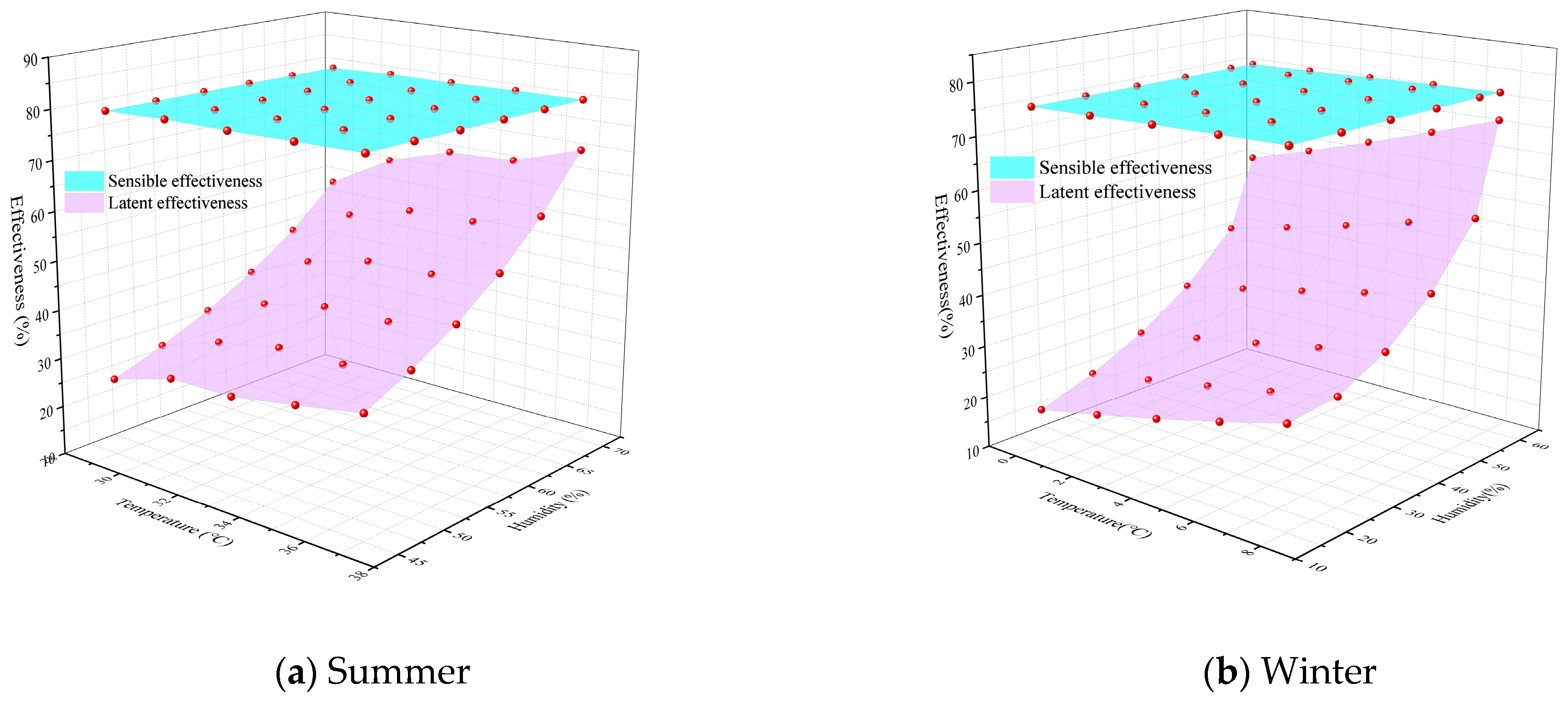
4.4. Effect of Simultaneous Changes in Temperature and Relative Humidity on Efficiency
4.5. Effect of Airflow Rate on Efficiency
5. Conclusions
- Before using the model, it is essential to fully understand the physical structure of the heat exchange core (channel dimensions), the parameters of the fluid passing through (temperature, relative humidity, and velocity), and the material properties of the core (thermal conductivity, moisture permeability, density, and mass diffusion coefficient) to ensure the accuracy of the calculations.
- As the thermal resistance constitutes a small proportion of the total resistance, the thermal conductivity of the heat transfer medium has little effect on the sensible effectiveness. In contrast, changes in the mass diffusion coefficient significantly affect the latent effectiveness, showing a clear upward trend as the mass diffusion coefficient increases. Consequently, mass diffusion resistance accounts for a large proportion of the total mass transfer resistance.
- The sensible effectiveness of the plate-fin heat exchanger is almost unaffected by outdoor air temperature differences and relative humidity. In contrast, latent effectiveness increases significantly with rising outdoor air temperature and relative humidity. This is because the changes in air temperature and humidity affect the heat and mass transfer capability, with mass diffusivity having a more significant impact.
- Both sensible and latent effectiveness are significantly influenced by the airflow rate. As the airflow rate increases around the design case, both efficiencies show a decreasing trend, with the impact on latent heat efficiency being more significant than that on sensible heat efficiency.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- United Nations Environment Programme. Not Just Another Brick in the Wall: The Solutions Exist—Scaling Them Will Build on Progress and Cut Emissions Fast. In Global Status Report for Buildings and Construction 2024/2025; United Nations Environment Programme: Nairobi, Kenya, 2025. [Google Scholar]
- Qiu, S.; Li, S.; Wang, F.; Wen, Y.; Li, Z.; Li, Z.; Guo, J. An Energy Exchange Efficiency Prediction Approach Based on Multivariate Polynomial Regression for Membrane-Based Air-to-Air Energy Recovery Ventilator Core. Build. Environ. 2019, 149, 490–500. [Google Scholar] [CrossRef]
- Xu, Q.; Riffat, S.; Zhang, S. Review of Heat Recovery Technologies for Building Applications. Energies 2019, 12, 1285. [Google Scholar] [CrossRef]
- Pandey, P.; Deng, Z.; Dong, B. Assessment of Indoor Air Quality and Ventilation Rate in Residential Dorms After Net-Zero Retrofit: 18th International Conference on Indoor Air Quality and Climate. In Proceedings of the 18th Conference of the International Society of Indoor Air Quality and Climate, INDOOR AIR 2024—Conference Program and Proceedings, Honolulu, HI, USA, 7–11 June 2024. [Google Scholar]
- Ma, X.; Zhang, Y.; Han, Z.; Zang, N.; Liu, Z. Performance Modelling on a Thermoelectric Air Conditioning System Using High Power Heat Sinks and Promoting Waste Heat Utilization. Energy 2023, 268, 126612. [Google Scholar] [CrossRef]
- Jouhara, H.; Khordehgah, N.; Almahmoud, S.; Delpech, B.; Chauhan, A.; Tassou, S.A. Waste Heat Recovery Technologies and Applications. Therm. Sci. Eng. Prog. 2018, 6, 268–289. [Google Scholar] [CrossRef]
- O’Connor, D.; Calautit, J.K.S.; Hughes, B.R. A Review of Heat Recovery Technology for Passive Ventilation Applications. Renew. Sustain. Energy Rev. 2016, 54, 1481–1493. [Google Scholar] [CrossRef]
- Min, J.; Su, M. Performance Analysis of a Membrane-Based Energy Recovery Ventilator: Effects of Outdoor Air State. Appl. Therm. Eng. 2011, 31, 4036–4043. [Google Scholar] [CrossRef]
- Li, J.; Shao, S.; Wang, Z.; Xie, G.; Wang, Q.; Xu, Z.; Han, L.; Gou, X. A Review of Air-to-Air Membrane Energy Recovery Technology for Building Ventilation. Energy Build. 2022, 265, 112097. [Google Scholar] [CrossRef]
- Zeng, C.; Liu, S.; Shukla, A. A Review on the Air-to-Air Heat and Mass Exchanger Technologies for Building Applications. Renew. Sustain. Energy Rev. 2017, 75, 753–774. [Google Scholar] [CrossRef]
- Yaïci, W.; Ghorab, M.; Entchev, E. Numerical Analysis of Heat and Energy Recovery Ventilators Performance Based on CFD for Detailed Design. Appl. Therm. Eng. 2013, 51, 770–780. [Google Scholar] [CrossRef]
- Lekshminarayanan, G.; Croal, M.; Maisonneuve, J. Recovering Latent and Sensible Energy from Building Exhaust with Membrane-Based Energy Recovery Ventilation. Sci. Technol. Built Environ. 2020, 26, 1000–1012. [Google Scholar] [CrossRef]
- Yang, T.; Guo, D.; Ge, T. Solid Desiccant Heat Pump Fresh Air Unit Using Composite Silica Gel. J. Therm. Sci. 2024, 33, 1286–1300. [Google Scholar] [CrossRef]
- Cho, H.-J.; Cheon, S.-Y.; Jeong, J.-W. Experimental Analysis on Energy Recovery Ventilator with Latent Heat Exchanger Using Hollow Fiber Membrane. Energy Convers. Manag. 2023, 278, 116706. [Google Scholar] [CrossRef]
- Armatis, P.D.; Fronk, B.M. Evaluation of Governing Heat and Mass Transfer Resistance in Membrane-Based Energy Recovery Ventilators with Internal Support Structures. Sci. Technol. Built Environ. 2017, 23, 912–922. [Google Scholar] [CrossRef]
- Song, H.-E.; Kang, Y.-H.; Yang, S.-K.; Ahn, Y. Prediction of Pressure Drop from Multivariate Polynomial Regression for Membrane-Based Air-to-Air Energy Recovery Ventilator Cores. Sci. Technol. Built Environ. 2023, 29, 339–346. [Google Scholar] [CrossRef]
- Gao, H.; Li, Z.; Qiu, S.; Yang, B.; Li, S.; Wen, Y. Energy Exchange Efficiency Prediction from Non-Linear Regression for Membrane-Based Energy-Recovery Ventilator Cores. Appl. Therm. Eng. 2021, 197, 117353. [Google Scholar] [CrossRef]
- Gao, Z.; Rendall, J.; Nawaz, K.; Abuheiba, A.; Abdelaziz, O. Innovative Modeling and Simulation of Membrane-Based Dehumidification and Energy Recovery Equipment. Case Stud. Therm. Eng. 2023, 43, 102783. [Google Scholar] [CrossRef]
- Zhang, C.; Xu, J.; Ge, T.; Dai, Y.; Wang, R. A Study on Enthalpy Exchanger with Modified Functional Layers Based on Chloride. Sci. Technol. Built Environ. 2017, 23, 72–80. [Google Scholar] [CrossRef]
- Zhang, L.-Z. Heat and Mass Transfer in a Quasi-Counter Flow Membrane-Based Total Heat Exchanger. Int. J. Heat Mass Transf. 2010, 53, 5478–5486. [Google Scholar] [CrossRef]
- Zhang, L.; Chen, Z. Convective Heat Transfer in Cross-Corrugated Triangular Ducts under Uniform Heat Flux Boundary Conditions. Int. J. Heat Mass Transf. 2011, 54, 597–605. [Google Scholar] [CrossRef]
- Liu, P.; Alonso, M.J.; Mathisen, H.M.; Simonson, C. Performance of a Quasi-Counter-Flow Air-to-Air Membrane Energy Exchanger in Cold Climates. Energy Build. 2016, 119, 129–142. [Google Scholar] [CrossRef]
- Zhang, L.-Z.; Liang, C.-H.; Pei, L.-X. Heat and Moisture Transfer in Application Scale Parallel-Plates Enthalpy Exchangers with Novel Membrane Materials. J. Membr. Sci. 2008, 325, 672–682. [Google Scholar] [CrossRef]
- Jeong, C.H.; Kim, H.R.; Ha, M.Y.; Son, S.W.; Lee, J.S.; Kim, P.Y. Numerical Investigation of Thermal Enhancement of Plate Fin Type Heat Exchanger with Creases and Holes in Construction Machinery. Appl. Therm. Eng. 2014, 62, 529–544. [Google Scholar] [CrossRef]
- Cuce, P.M.; Riffat, S. A Comprehensive Review of Heat Recovery Systems for Building Applications. Renew. Sustain. Energy Rev. 2015, 47, 665–682. [Google Scholar] [CrossRef]
- Mardiana-Idayu, A.; Riffat, S.B. Review on Heat Recovery Technologies for Building Applications. Renew. Sustain. Energy Rev. 2012, 16, 1241–1255. [Google Scholar] [CrossRef]
- Chaudhry, H.N.; Hughes, B.R.; Ghani, S.A. A Review of Heat Pipe Systems for Heat Recovery and Renewable Energy Applications. Renew. Sustain. Energy Rev. 2012, 16, 2249–2259. [Google Scholar] [CrossRef]
- Al-Waked, R.; Nasif, M.S.; Morrison, G.; Behnia, M. CFD Simulation of Air to Air Enthalpy Heat Exchanger. Energy Convers. Manag. 2013, 74, 377–385. [Google Scholar] [CrossRef]
- Nasif, M.; AL-Waked, R.; Morrison, G.; Behnia, M. Membrane Heat Exchanger in HVAC Energy Recovery Systems, Systems Energy Analysis. Energy Build. 2010, 42, 1833–1840. [Google Scholar] [CrossRef]
- Liu, Z.; Li, W.; Chen, Y.; Luo, Y.; Zhang, L. Review of Energy Conservation Technologies for Fresh Air Supply in Zero Energy Buildings. Appl. Therm. Eng. 2019, 148, 544–556. [Google Scholar] [CrossRef]
- Zender–Świercz, E. A Review of Heat Recovery in Ventilation. Energies 2021, 14, 1759. [Google Scholar] [CrossRef]
- Saleh, K.; Aute, V.; Radermacher, R.; Azarm, S. Chevron Plate Heat Exchanger Optimization Using Efficient Approximation-Assisted Multi-Objective Optimization Techniques. HVACR Res. 2013, 19, 788–799. [Google Scholar] [CrossRef]
- Liang, C.; Tong, X.; Lei, T.; Li, Z.; Wu, G. Optimal Design of an Air-to-Air Heat Exchanger with Cross-Corrugated Triangular Ducts by Using a Particle Swarm Optimization Algorithm. Appl. Sci. 2017, 7, 554. [Google Scholar] [CrossRef]
- American Society of Heating, Refrigerating and Air-Conditioning Engineers, Inc. 2024 ASHRAE Handbook: Heating, Ventilating, and Air-Conditioning Systems and Equipment, Inch-Pound Edition; ASHRAE: Atlanta, GA, USA, 2024; ISBN 978-1-5231-3507-3. [Google Scholar]
- Charles, N.T.; Johnson, D.W. The Occurrence and Characterization of Fouling during Membrane Evaporative Cooling. J. Membr. Sci. 2008, 319, 44–53. [Google Scholar] [CrossRef]
- Engarnevis, A.; Huizing, R.; Green, S.; Rogak, S. Particulate Fouling Assessment in Membrane Based Air-to-Air Energy Exchangers. Energy Build. 2017, 150, 477–487. [Google Scholar] [CrossRef]
- Karlsson, H.O.E.; Trägårdh, G. Heat Transfer in Pervaporation. J. Membr. Sci. 1996, 119, 295–306. [Google Scholar] [CrossRef]
- Niu, J.L.; Zhang, L.Z. Membrane-Based Enthalpy Exchanger: Material Considerations and Clarification of Moisture Resistance. J. Membr. Sci. 2001, 189, 179–191. [Google Scholar] [CrossRef]
- Standardization Administration of China. GB/T 20187-2020; Energy Recovery Ventilators for Outdoor Air Handling. SAC: Beijing, China, 2020.
- Zhang, L.-Z. Heat and Mass Transfer in Plate-Fin Enthalpy Exchangers with Different Plate and Fin Materials. Int. J. Heat Mass Transf. 2009, 52, 2704–2713. [Google Scholar] [CrossRef]



| Item | Symbol (Unit) | Value |
|---|---|---|
| Channel length | A (mm) | 140 |
| Core height | H (mm) | 70 |
| Channel height | H (mm) | 5 |
| Membrane thickness | Δ (mm) | 0.05 |
| Triangle base length | A (mm) | 0.8 |
| Conditions | Indoor Air Inlet | Fresh Air Inlet | ||
|---|---|---|---|---|
| Dry Bulb Temperature | Wet Bulb Temperature | Dry Bulb Temperature | Wet Bulb Temperature | |
| Summer Winter | 27 21 | 19.5 13 | 35 2 | 28 1 |
| Heat Exchanger Structural Parameters | Core Material Parameters | ||||
|---|---|---|---|---|---|
| Structure | Unit | Value | Items | Unit | Value |
| Channel length | m | 0.18 | Maximum moisture absorption | Wmax (kg/kg) | 0.92 |
| Channel height | mm | 5 | Adsorption curve constant | —— | 6 |
| Core height | m | 0.7 | Diffusivity | Dw (m2/s) | 6.08 × 10−12 |
| Diffusion coefficient | m2/s | 2.82 × 10−5 | Thickness | δ (μm) | 55 |
| Outdoor air | °C | 35/2 | Density | ρ (kg/m3) | 876 |
| Indoor air | °C | 27/21 | Thermal conductivity | λ (Wm−1 K−1) | 0.44 |
| Parameters | Airflow Rates | Heat Transfer Coefficients | Mass Transfer Coefficients | Residence Time |
|---|---|---|---|---|
| m3/h | W/ (m2·K) | kg/ (m2·s) | S | |
| Summer | 100 | 23.25 | 2.75 × 10–3 | 0.41 |
| 200 | 28.06 | 2.81 × 10–3 | 0.20 | |
| 300 | 31.96 | 2.84 × 10–3 | 0.14 | |
| 400 | 35.28 | 2.87 × 10–3 | 0.10 | |
| 500 | 38.20 | 2.89 × 10–3 | 0.08 | |
| Winter | 100 | 22.04 | 3.52 × 10–3 | 0.41 |
| 200 | 26.88 | 3.63 × 10–3 | 0.20 | |
| 300 | 30.73 | 3.69 × 10–3 | 0.14 | |
| 400 | 33.99 | 3.74 × 10–3 | 0.10 | |
| 500 | 36.84 | 3.77 × 10–3 | 0.08 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, H.; Nie, J.; Sheng, Y.; Li, H.; Zhang, W.; Huang, H. Theoretical Modeling and Analysis of Energy Recovery Efficiency and Influencing Factors of Energy Recovery Ventilators Under Dynamic Thermal Environments. Sustainability 2025, 17, 4509. https://doi.org/10.3390/su17104509
Wang H, Nie J, Sheng Y, Li H, Zhang W, Huang H. Theoretical Modeling and Analysis of Energy Recovery Efficiency and Influencing Factors of Energy Recovery Ventilators Under Dynamic Thermal Environments. Sustainability. 2025; 17(10):4509. https://doi.org/10.3390/su17104509
Chicago/Turabian StyleWang, Huanyu, Jinzhe Nie, Ying Sheng, Hanlong Li, Wen Zhang, and Honghao Huang. 2025. "Theoretical Modeling and Analysis of Energy Recovery Efficiency and Influencing Factors of Energy Recovery Ventilators Under Dynamic Thermal Environments" Sustainability 17, no. 10: 4509. https://doi.org/10.3390/su17104509
APA StyleWang, H., Nie, J., Sheng, Y., Li, H., Zhang, W., & Huang, H. (2025). Theoretical Modeling and Analysis of Energy Recovery Efficiency and Influencing Factors of Energy Recovery Ventilators Under Dynamic Thermal Environments. Sustainability, 17(10), 4509. https://doi.org/10.3390/su17104509







