The Influence Depth of Pile Base Resistance in Sand-Layered Clay
Abstract
1. Introduction
2. Difference in Calculation Formula of Pile Base Resistance
2.1. Engineering Example
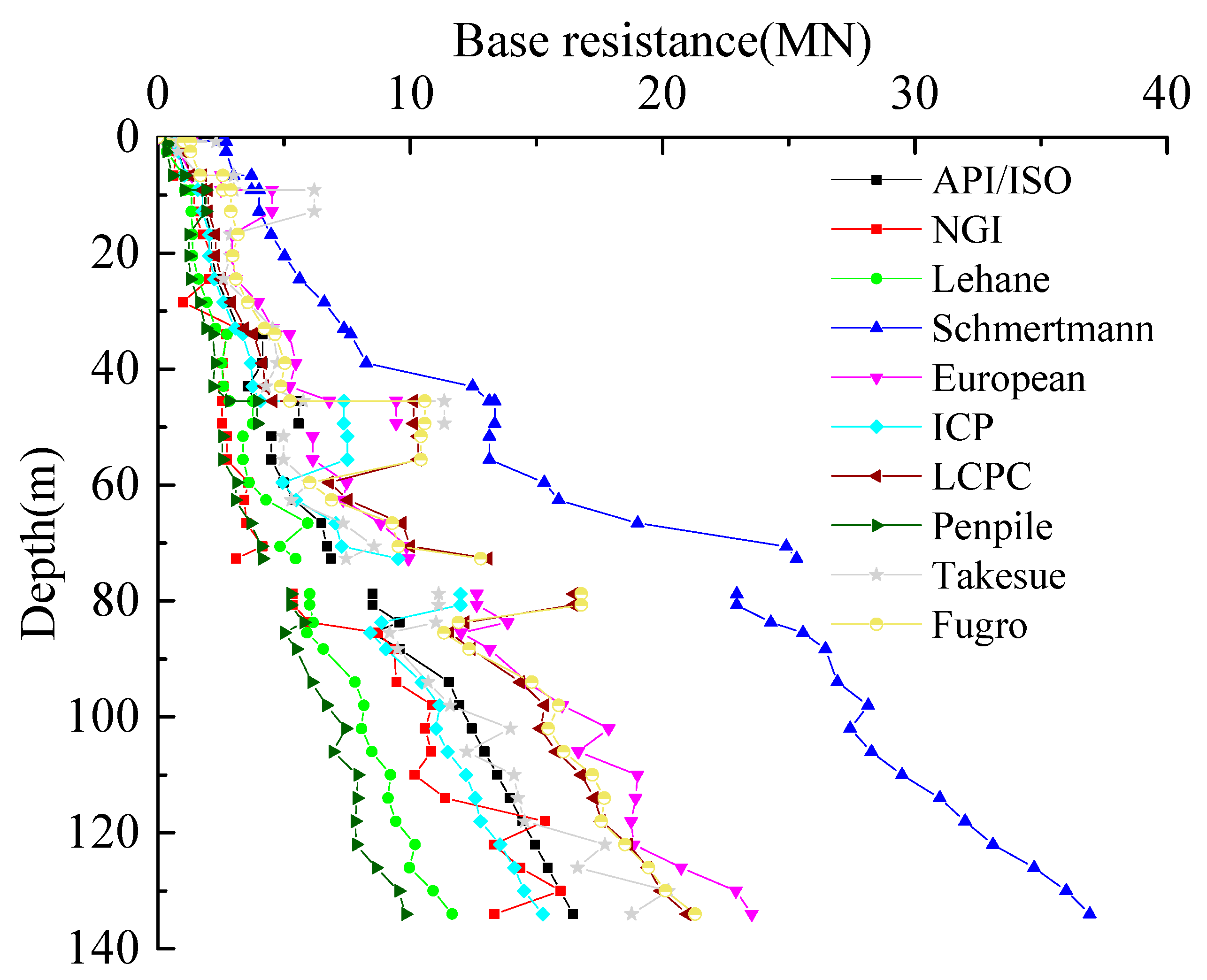
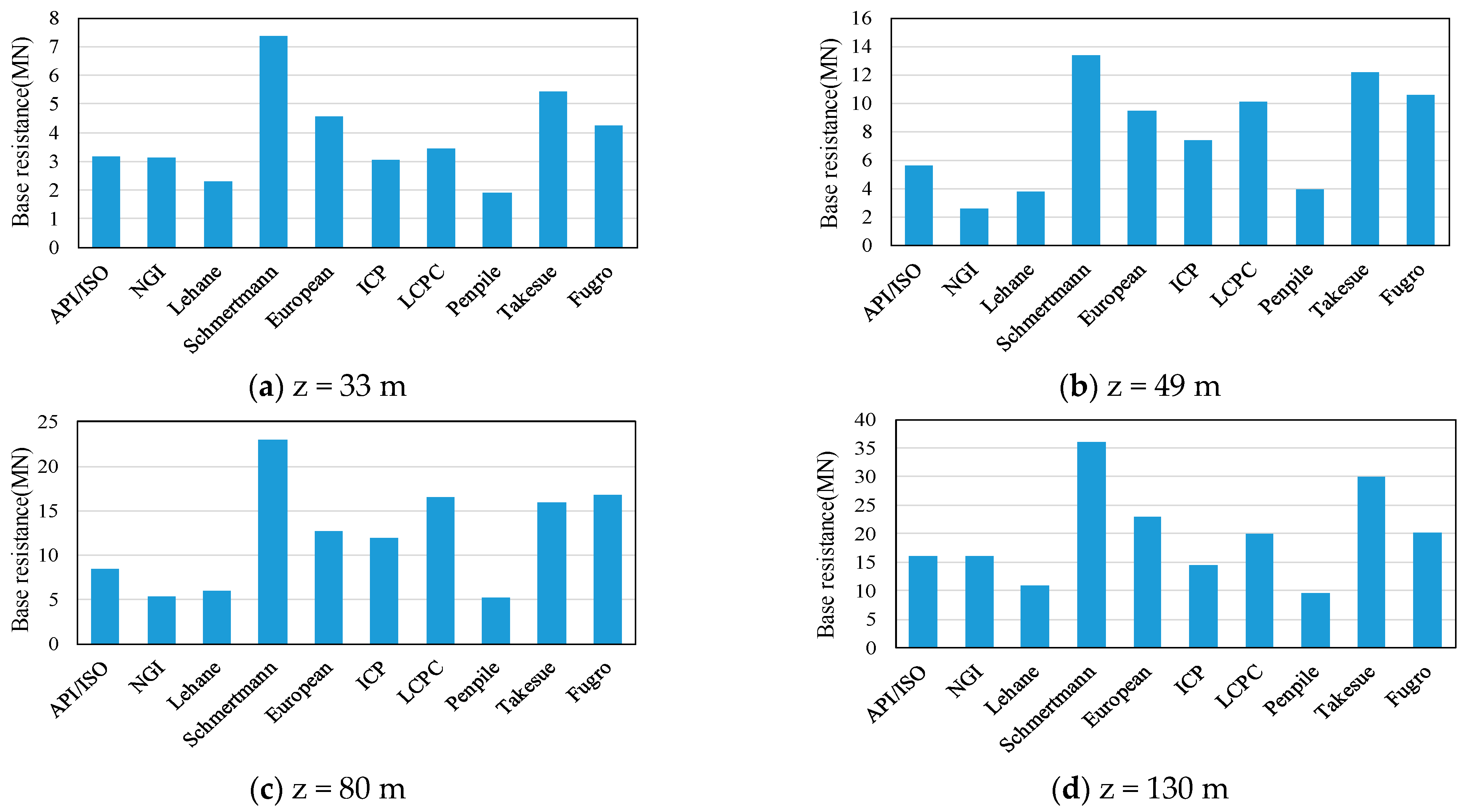
2.2. Results of Pile Base Resistance
3. Numerical Simulation on Influence Range of Pile Base Resistance
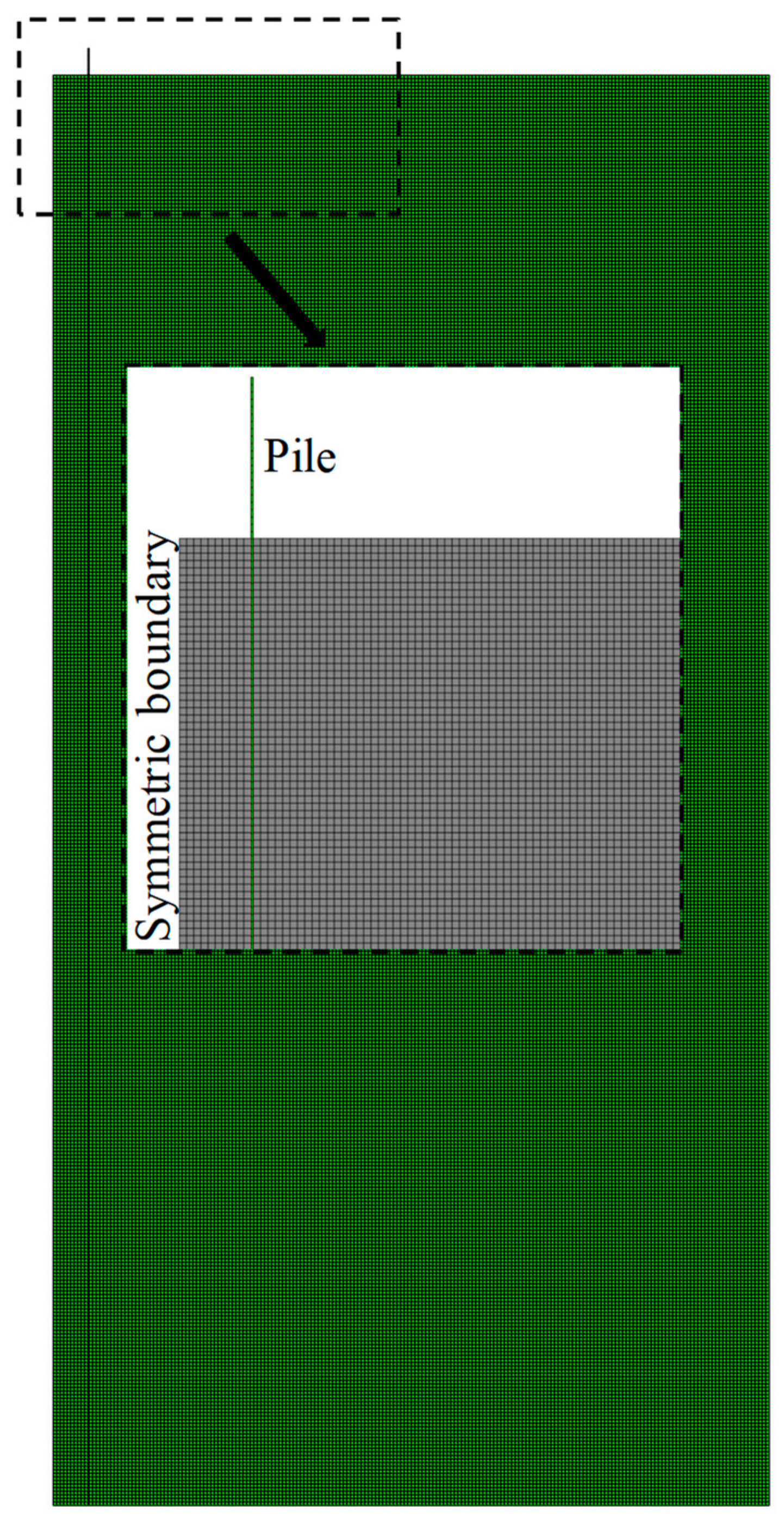
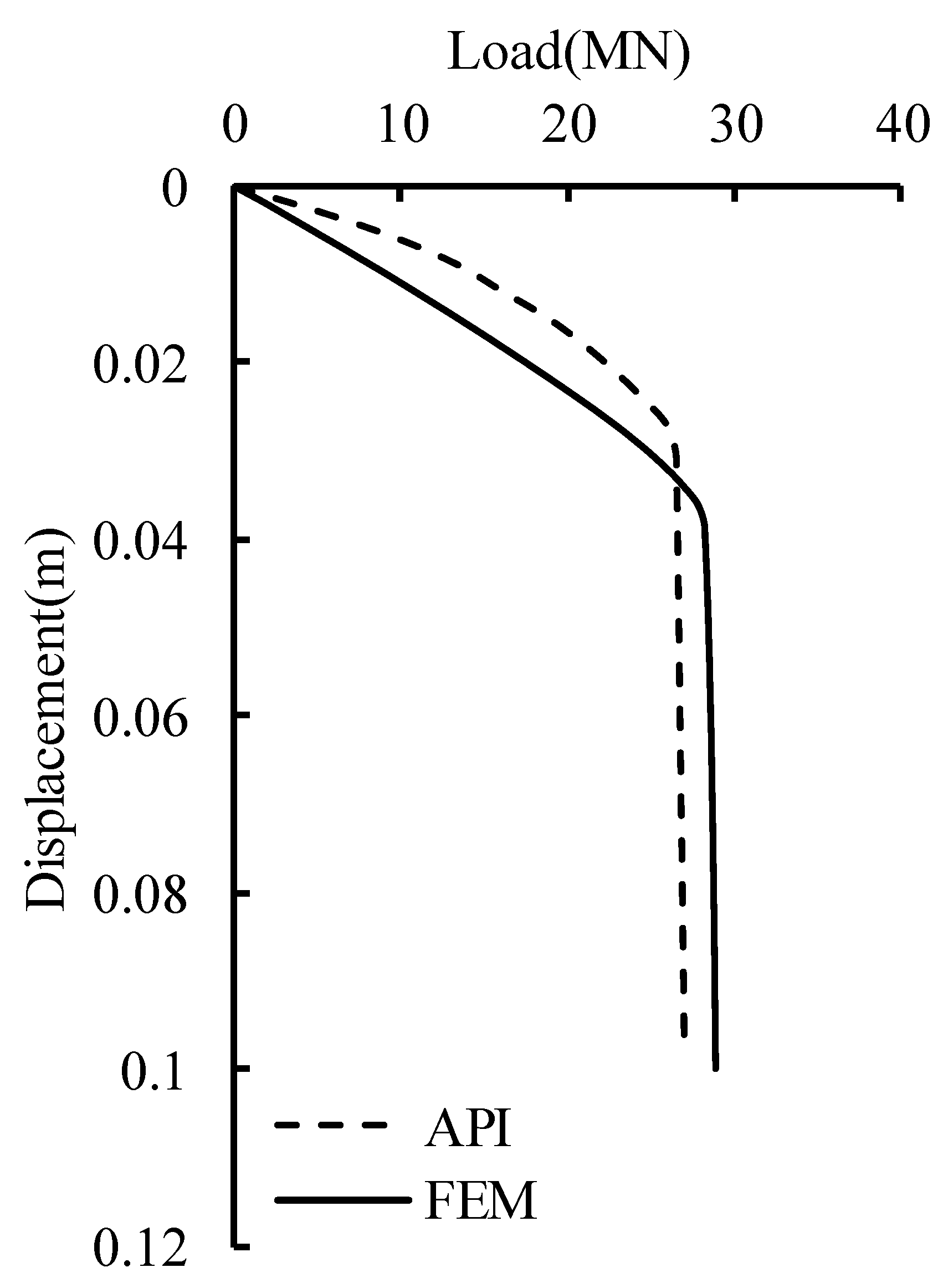
3.1. Numerical Model and Verification
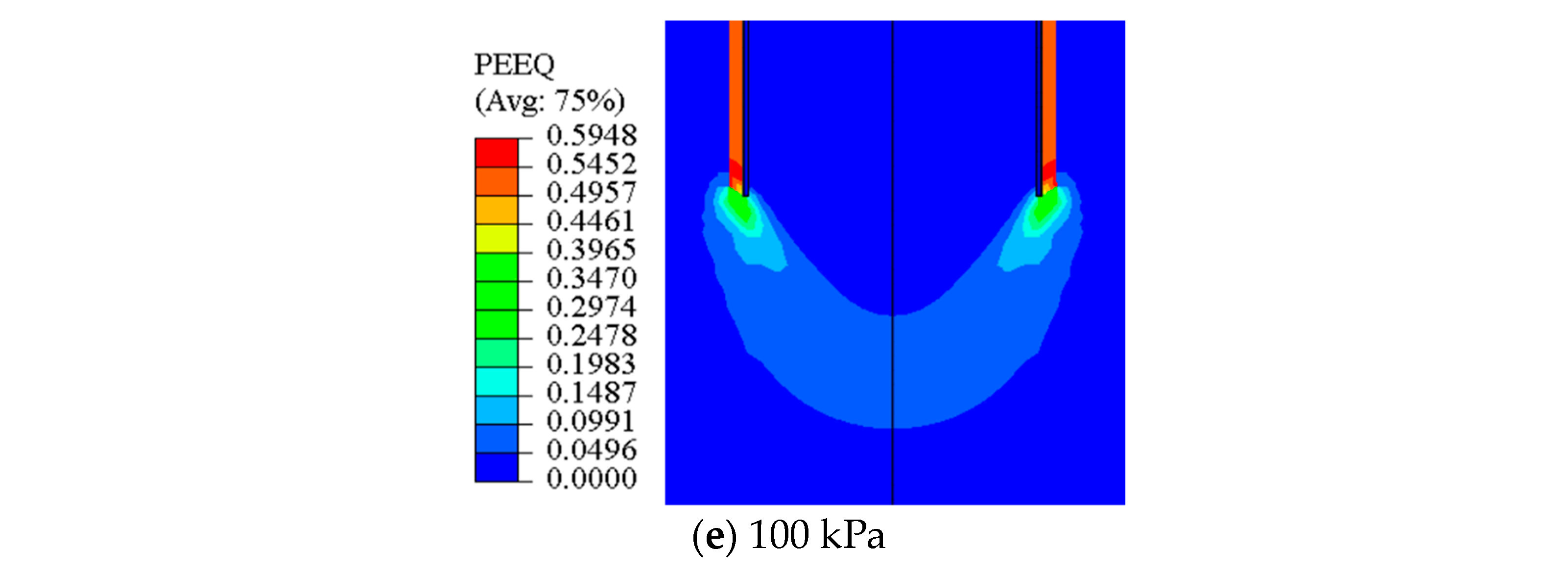
3.2. Results in Homogeneous Clay
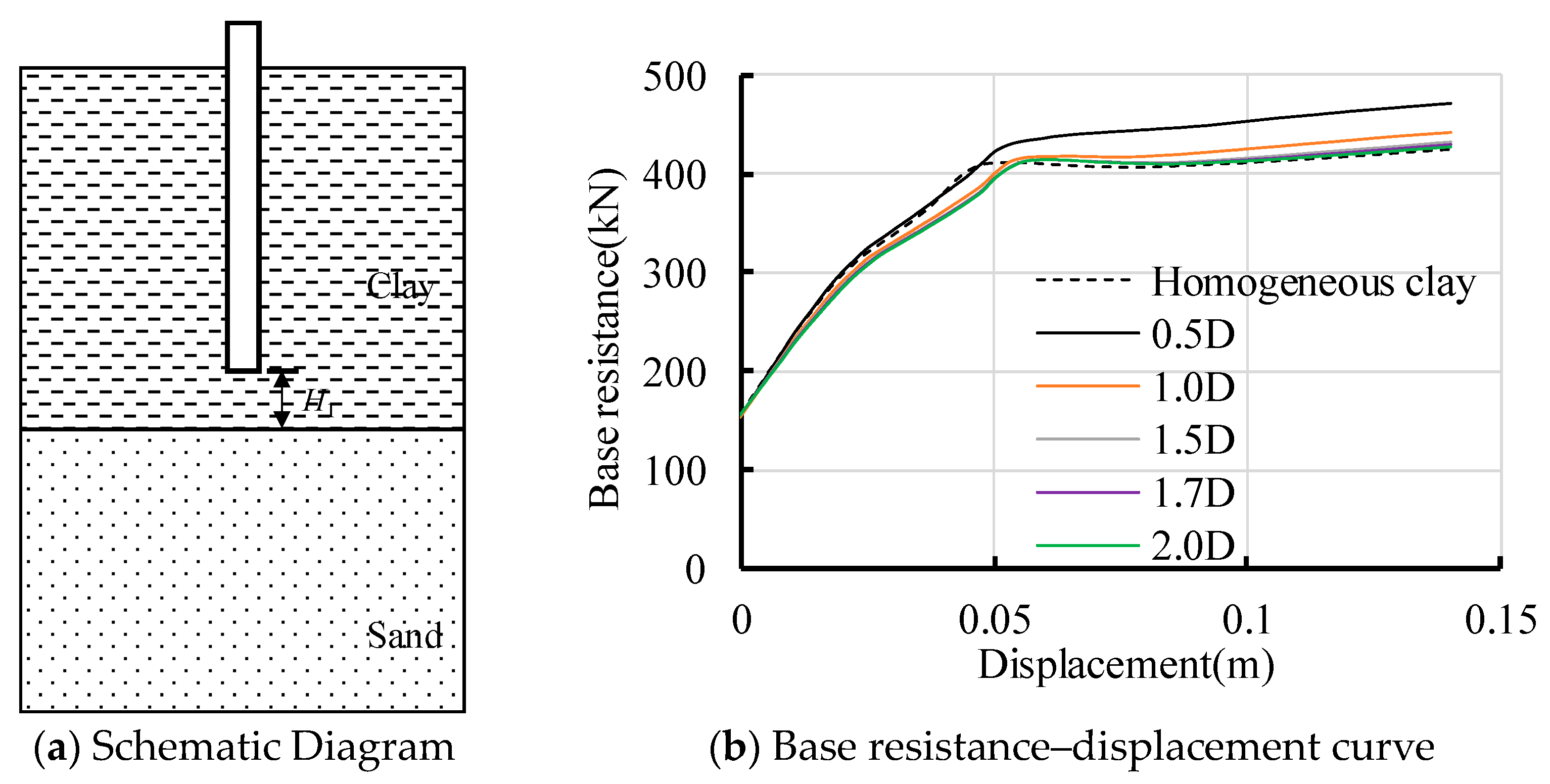
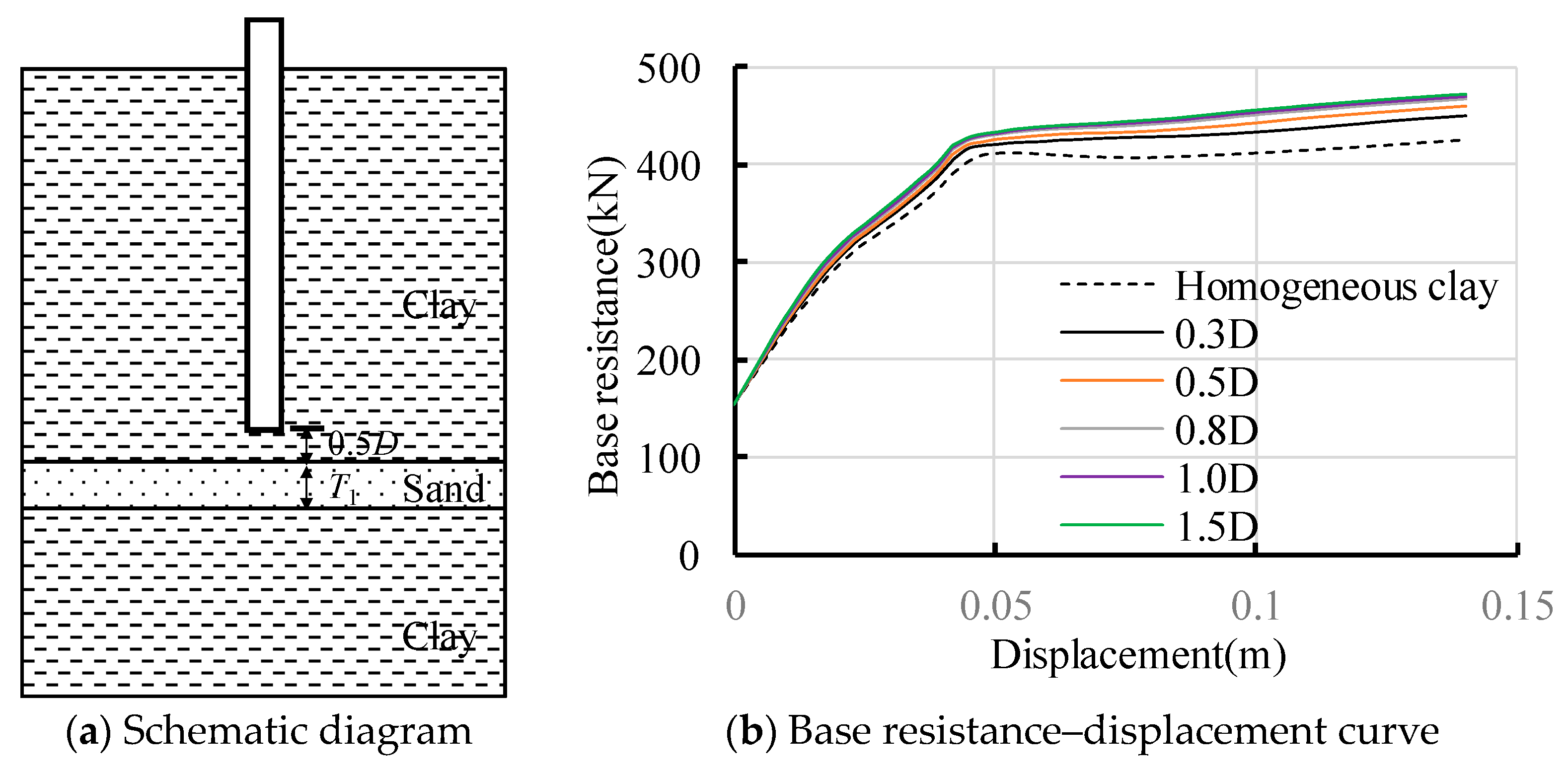
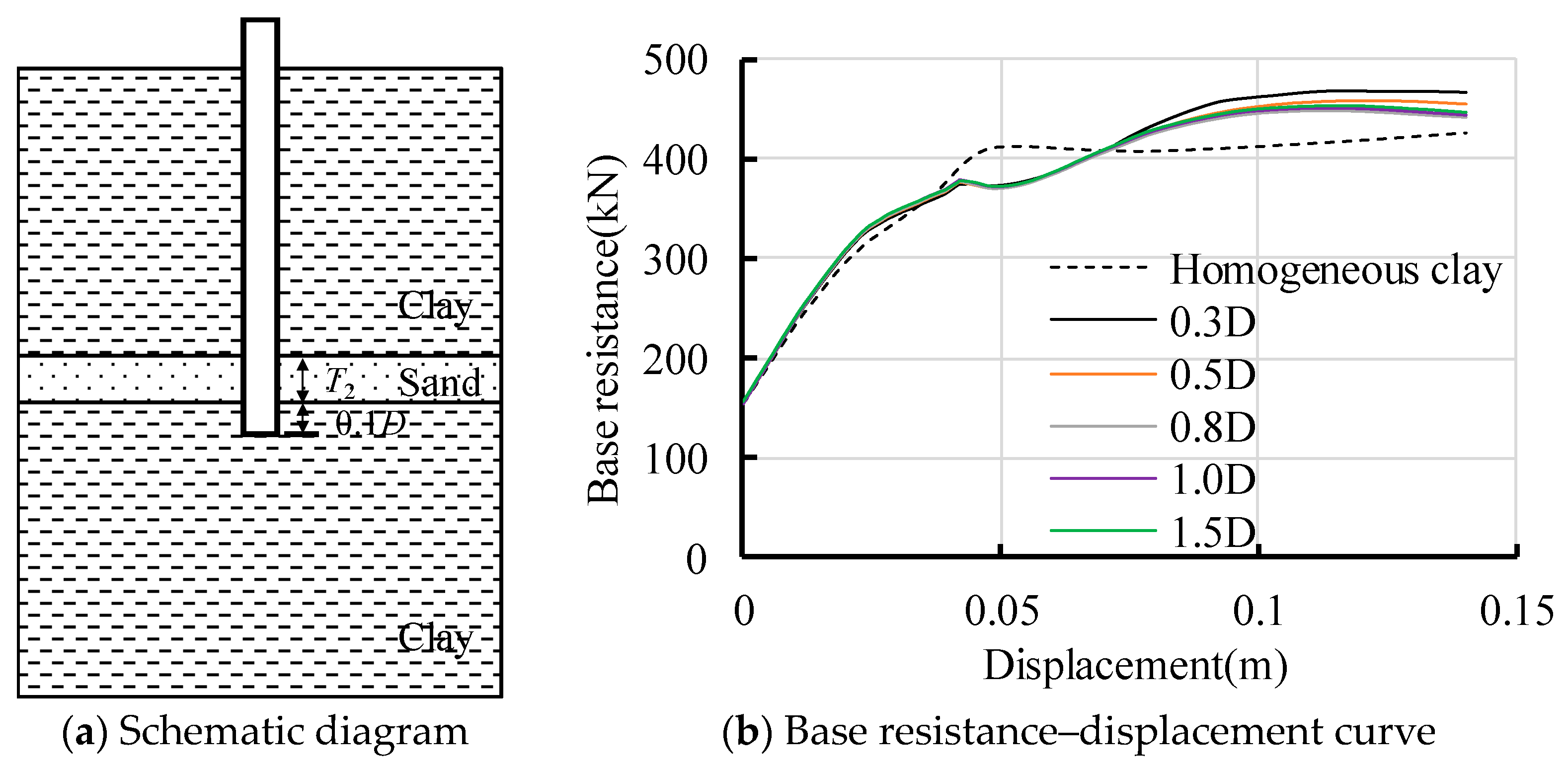
3.3. Results when the Clay Is above the Sand
3.3.1. Influence of Distance between Sand and the Pile End
3.3.2. Influence of Sand Thickness
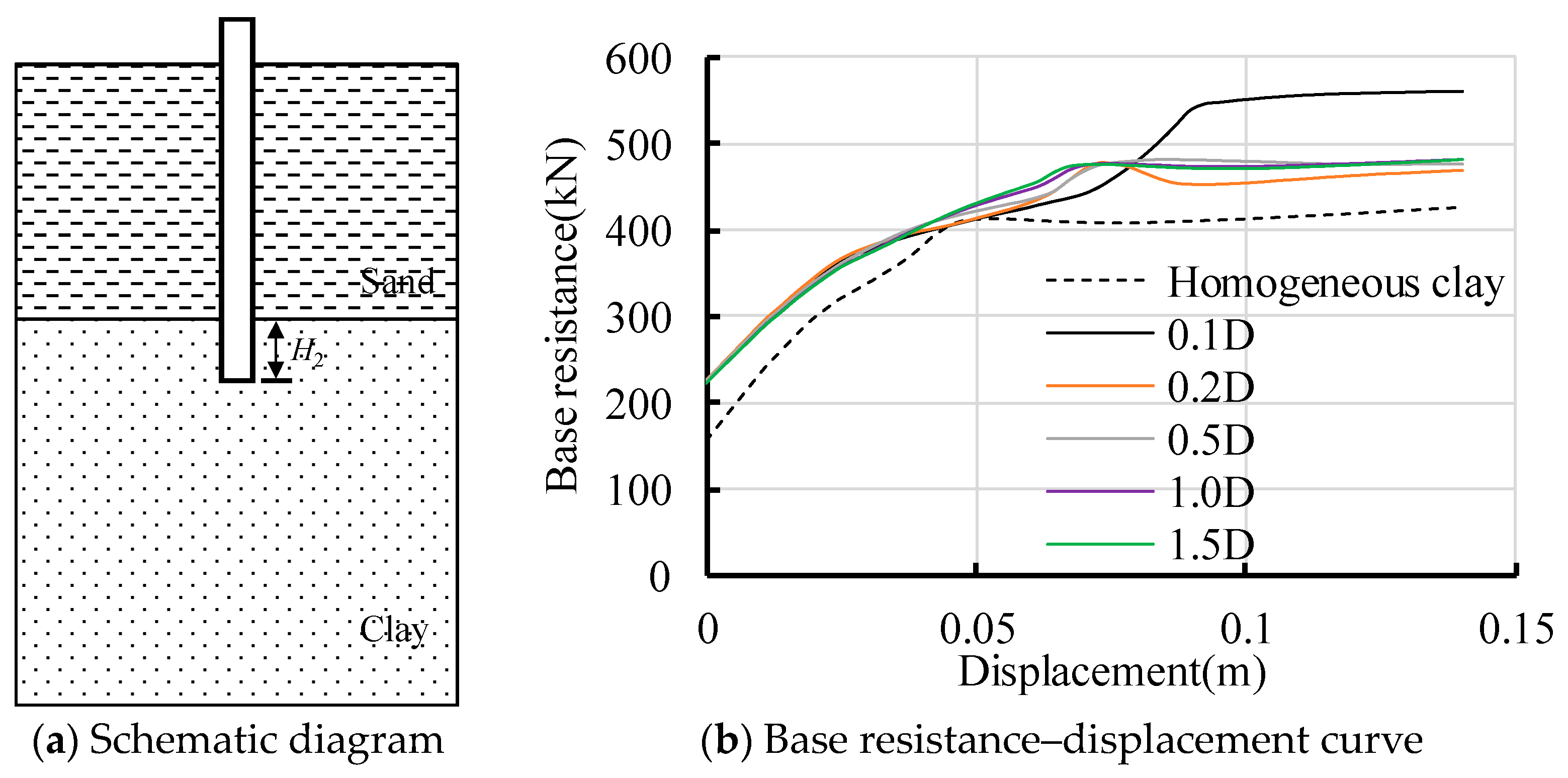
3.4. Results when the Clay Is under the Sand
3.4.1. Influence of Distance between Sand and the Pile End
3.4.2. Influence of Sand Thickness
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Wu, X.; Hu, Y.; Li, Y.; Yang, J. Foundations of offshore wind turbines: A review. Renew. Sustain. Energy Rev. 2019, 104, 379–393. [Google Scholar] [CrossRef]
- Li, L.; Zheng, M.; Liu, X.; Wu, W. Numerical analysis of the cyclic loading behavior of monopile and hybrid pile foundation. Comput. Geotech. 2022, 144, 104635. [Google Scholar] [CrossRef]
- Basack, S.; Goswami, G.; Dai, Z.H.; Baruah, P. Failure-mechanism and design techniques of offshore wind turbine pile foundation: Review and research directions. Sustainability 2022, 14, 12666. [Google Scholar] [CrossRef]
- Zhang, X.; Liu, C.; Wang, P.; Zhang, G. Experimental simulation study on dynamic response of offshore wind power pile foundation under complex marine loadings. Soil Dyn. Earthq. Eng. 2022, 156, 107232. [Google Scholar]
- American Petroleum Institute. Geotechnical and Foundation Design Considerations. In ANSI/API Recommended Practice 2GEO, 1st ed.; API Publishing Services: Washington, DC, USA, 2014. [Google Scholar]
- Terzaghi, K.T. Theoretical Soil Mechanics; John Wiley and Sons Inc.: Hoboken, NJ, USA, 1943. [Google Scholar]
- Meyerhof, G.G. The ultimate bearing capacity of foundations. J. Geotech. 1951, 2, 301–332. [Google Scholar] [CrossRef]
- Meyerhof, G.G. The ultimate bearing capacity of foundations under eccentric and inclined loads. In Proceedings of the third International Conference on Soil Mechanics and Foundation Engineering, Zurich, Switzerland, 16–27 August 1953; Volume 1. [Google Scholar]
- Meyerhof, G.G. Influence of roughness of base and ground-water conditions on the ultimate bearing capacity of foundations. J. Geotech. 1955, 5, 227–242. [Google Scholar] [CrossRef]
- Meyerhof, G.G. Bearing capacity and settlement of pile foundations. J. Geotech. Eng. Div. 1976, 102, 197–228. [Google Scholar] [CrossRef]
- Berezantzev, V.G. Load bearing capacity and deformation of piled foundations. In Proceedings of the 5th International Conference on Soil Mechanics and Foundation Engineering ISSMFE, Paris, France, 17–22 July 1961; Volume 2, pp. 11–12. [Google Scholar]
- Hu, G.C.Y. Variable-factors theory of bearing capacity. J. Soil Mech. Found. Div. 1964, 90, 85–95. [Google Scholar] [CrossRef]
- Vesic, A.S. Design of Pile Foundations; Transportation Research Board, National Research Council: Washington, DC, USA, 1977. [Google Scholar]
- Wilson, G. The Bearing Capacity of Screw Piles and Screwcrete Cylinders. J. Inst. Civ. Eng. 1950, 34, 4–73. [Google Scholar] [CrossRef]
- Meigh, A.C. Model Footing Tests on Clay. Master’s Thesis, Imperial College London, London, UK, 1950. [Google Scholar]
- Yassin, A.A. Model Studies on Bearing Capacity of Piles. Ph.D. Thesis, Imperial College London, London, UK, 1950. [Google Scholar]
- Skempton, A.W. The Bearing Capacity of Clays. Build. Res. Congr. 1951, 1, 180–189. [Google Scholar]
- Randolph, M.; Gourvenec, S. Offshore Geotechnical Engineering; CRC Press: Boca Raton, FL, USA, 2017. [Google Scholar]
- Gavin, K.; Kovacevic, M.S.; Igoe, D. A review of CPT based axial pile design in The Netherlands. Undergr. Space 2019, 6, 85–99. [Google Scholar] [CrossRef]
- Amirmojahedi, M.; Abu-Farsakh, M. Evaluation of 18 Direct CPT Methods for Estimating the Ultimate Pile Capacity of Driven Piles. Transp. Res. Rec. J. Transp. Res. Board 2019, 2673, 127–141. [Google Scholar] [CrossRef]
- Leetsaar, L.; Korkiala-Tanttu, L.; Kurnitski, J. CPT, CPTu and DCPT methods for predicting the ultimate bearing capacity of cast in situ displacement piles in silty soils. Geotech. Geol. Eng. 2022, 41, 631–652. [Google Scholar] [CrossRef]
- Heidari, P.; Ghazavi, M. Statistical Evaluation of CPT and CPTu Based Methods for Prediction of Axial Bearing Capacity of Piles. Geotech. Geol. Eng. 2021, 39, 1259–1287. [Google Scholar] [CrossRef]
- Schmertmann, J.H. Guidelines for Cone Penetration Test: Performance and Design; Federal Highway Administration: Washington, DC, USA, 1978. [Google Scholar]
- De Kuiter, J.; Beringen, F.L. Pile foundations for large North Sea structures. J. Mar. Georesour. Geotechnol. 1979, 3, 267–314. [Google Scholar] [CrossRef]
- Jardine, R.; Chow, F.; Overy, R. ICP Design Methods for Driven Piles in Sands and Clays; Thomas Telford: London, UK, 2005. [Google Scholar]
- Bustamante, M.; Gianeselli, L. Pile bearing capacity prediction by means of static penetrometer CPT. In Proceedings of the 2nd European Symposium on Penetration testing, Amsterdam, The Netherlands, 24–27 May 1982; Volume 2, pp. 493–500. [Google Scholar]
- Lehane, B.M.; Liu, Z.; Bittar, E.J. CPT-based axial capacity design method for driven piles in clay. J. Geotech. Geoenviron. Eng. 2022, 148, 04022069. [Google Scholar] [CrossRef]
- Van Dijk, B.F.J.; Kolk, H.J. CPT-based design method for axial capacity of offshore piles in clays. J. Front. Offshore Geotech. II 2011, 555–560. [Google Scholar]
- Price, G.; Wardle, I.F. A comparison between cone penetration test results and the performance of small diameter instrumented piles in stiff clay. In Proceedings of the 2nd European Symposium on Penetration Testing, Amsterdam, The Netherlands, 24–27 May 1982; Volume 2, pp. 775–780. [Google Scholar]
- Takesue, K.; Sasao, H.; Matsumoto, T. Correlation between ultimate pile skin friction and CPT data. In Proceedings of the First International Conference on Site Characterization, Atlanta, Georgia, 19–22 April 1998; pp. 1177–1182. [Google Scholar]





| Pile Length (m) | Length of Embedded Pile (m) | Diameter (m) | Thickness (mm) |
|---|---|---|---|
| 171 | 134 | 2.743 | 70 |
| Stratum | Soil Description | Thickness (m) | Submerged Unit Weight γ′ (kN/m3) | Internal Friction Angle φ (°) | Design Shear Strength Su (kPa) |
|---|---|---|---|---|---|
| 1 | Very soft sandy clay | 0.8 | 8.2 | — | 11 |
| 2 | Soft calcareous silty clay | 5.8 | 7.7 | — | 12–24 |
| 3 | Slightly hard calcareous sandy clay | 2.5 | 7.0 | — | 28–38 |
| 4 | Slightly hard to hard calcareous silty clay | 36.4 | 7.6 | — | 30–75 |
| 5 | Hard to very hard calcareous sandy clay | 3.9 | 9.8 | — | 90–120 |
| 6 | Loose to medium dense clayey sand | 2.2 | 9.3 | 25 | — |
| 7 | Hard to very hard calcareous silty clay | 21.1 | 8.6 | — | 80–130 |
| 8 | Medium dense carbonate silty fine sand | 6.1 | 6.5 | 30 | — |
| 9 | Very hard to hard calcareous silty clay | 91.4 | 9.6 | — | 160–400 |
| Method | Formula | Kernel Parameter | Range of Soil |
|---|---|---|---|
| API/ISO | Design Shear Strength, Su | Pile end | |
| NGI | UU Shear Strength, SuUU | Pile end | |
| Lehane | qt | 20 times the pile wall thickness below the pile end | |
| Schmertmann | qc | 8 D above the pile end, 0.7 D–4 D below the pile end | |
| European | qc | 0.7 D–4 D below the pile end | |
| ICP | Closed: q = 0.8 qc (undrained) q = 0.8 qc (drained) Open (fully plugged): q = 0.4 qc (undrained) q = 0.65 qc (drained) Open (unplugged): q = 1.0 qc (undrained) q = 1.6 qc (drained) | qc | ±1.5 D at the pile end |
| LCPC | qc | ±1.5 D at the pile end | |
| Penpile [29] | qc | Pile end | |
| Takesue [30] | qt | Pile end | |
| Fugro | qn | ±1.5 D at the pile end |
| Depth (m) | Maximum (MN) | Minimum (MN) | Mean (MN) | Variance | COV |
|---|---|---|---|---|---|
| 33 | 7.4 | 1.9 | 3.9 | 1.6 | 0.41 |
| 49 | 13.4 | 2.5 | 7.9 | 3.8 | 0.48 |
| 80 | 22.9 | 5.3 | 12.2 | 5.9 | 0.49 |
| 130 | 36.0 | 9.5 | 19.6 | 8.3 | 0.42 |
| Model | Effective Density (kg/m3) | Elastic Modulus (kPa) | Poisson’s Ratio | Shear Strength (kPa) | Internal Friction Angle (°) |
|---|---|---|---|---|---|
| Pile | 6850 | 210 × 106 | 0.25 | — | — |
| Clay | 600 | 500 Su | 0.49 | 20–100 | — |
| Sand | 900 | 50,000 | 0.3 | — | 30 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fu, D.; Li, S.; Zhang, H.; Jiang, Y.; Liu, R.; Li, C. The Influence Depth of Pile Base Resistance in Sand-Layered Clay. Sustainability 2023, 15, 7221. https://doi.org/10.3390/su15097221
Fu D, Li S, Zhang H, Jiang Y, Liu R, Li C. The Influence Depth of Pile Base Resistance in Sand-Layered Clay. Sustainability. 2023; 15(9):7221. https://doi.org/10.3390/su15097221
Chicago/Turabian StyleFu, Dianfu, Shuzhao Li, Hui Zhang, Yu Jiang, Run Liu, and Chengfeng Li. 2023. "The Influence Depth of Pile Base Resistance in Sand-Layered Clay" Sustainability 15, no. 9: 7221. https://doi.org/10.3390/su15097221
APA StyleFu, D., Li, S., Zhang, H., Jiang, Y., Liu, R., & Li, C. (2023). The Influence Depth of Pile Base Resistance in Sand-Layered Clay. Sustainability, 15(9), 7221. https://doi.org/10.3390/su15097221






