Hydraulic Characteristics of Continuous Submerged Jet Impinging on a Wall by Using Numerical Simulation and PIV Experiment
Abstract
:1. Introduction
2. Governing Equations and Turbulence Model
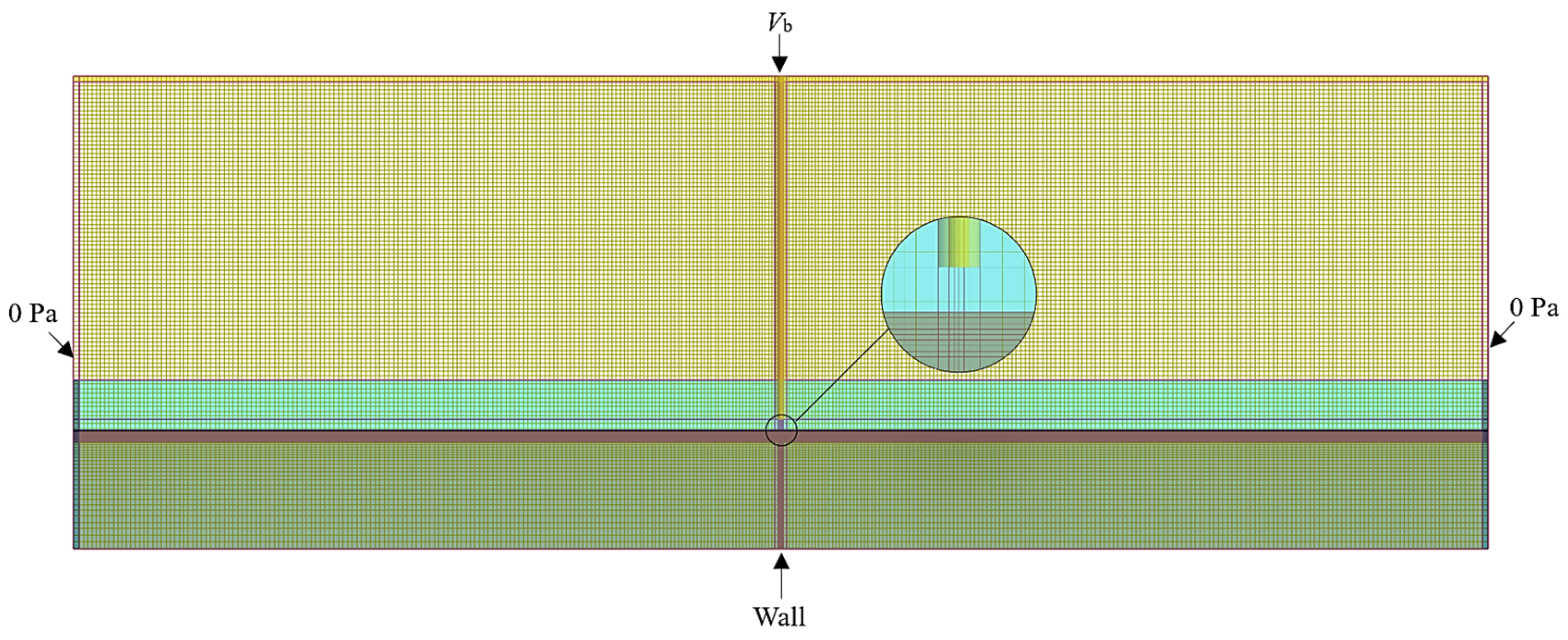
3. Calculation Regions and Boundary Conditions
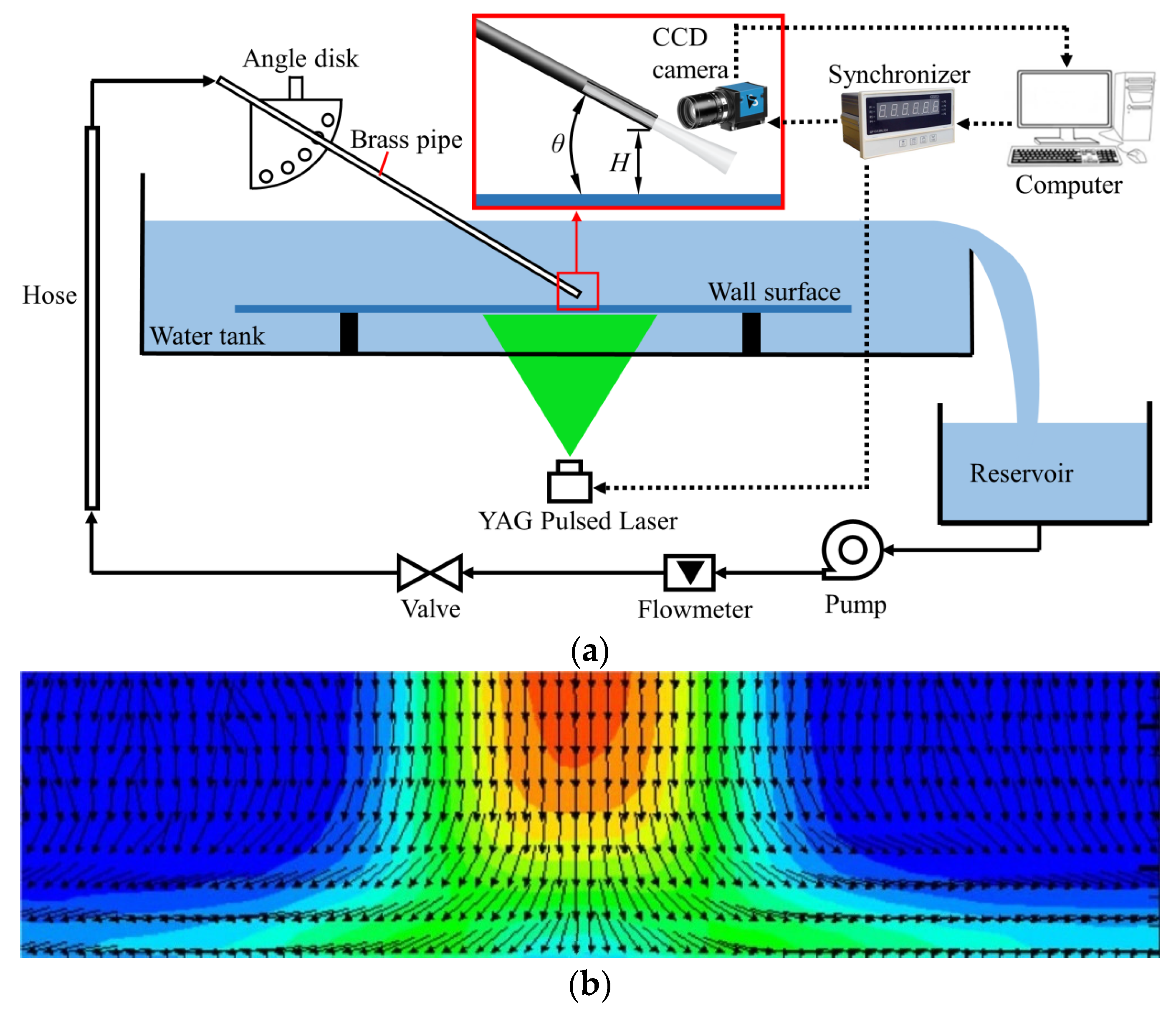
4. Experimental Verification
5. Results and Discussion
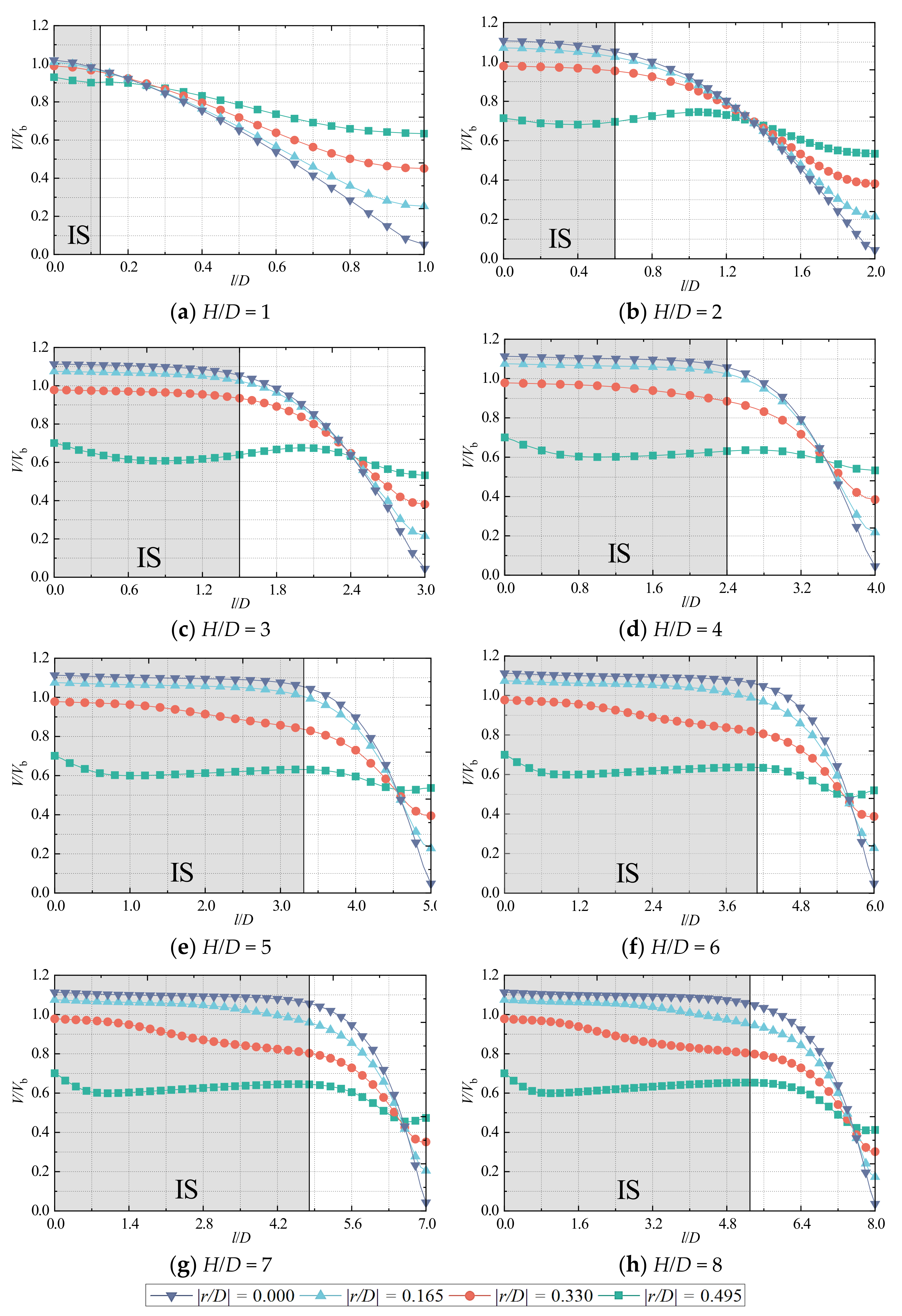
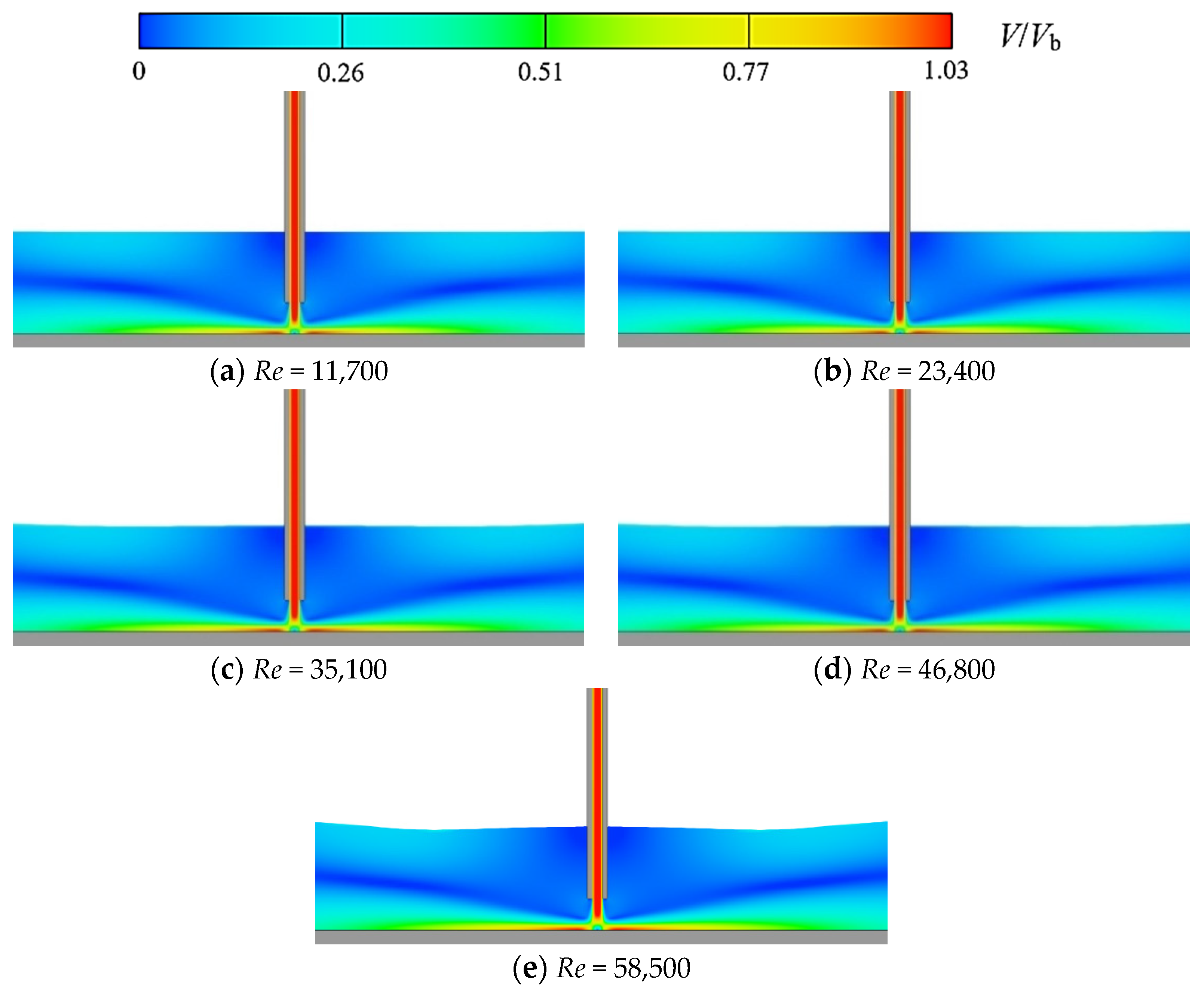
5.1. Flow Field Analysis of Submerged Jet under Different Impinging Distances
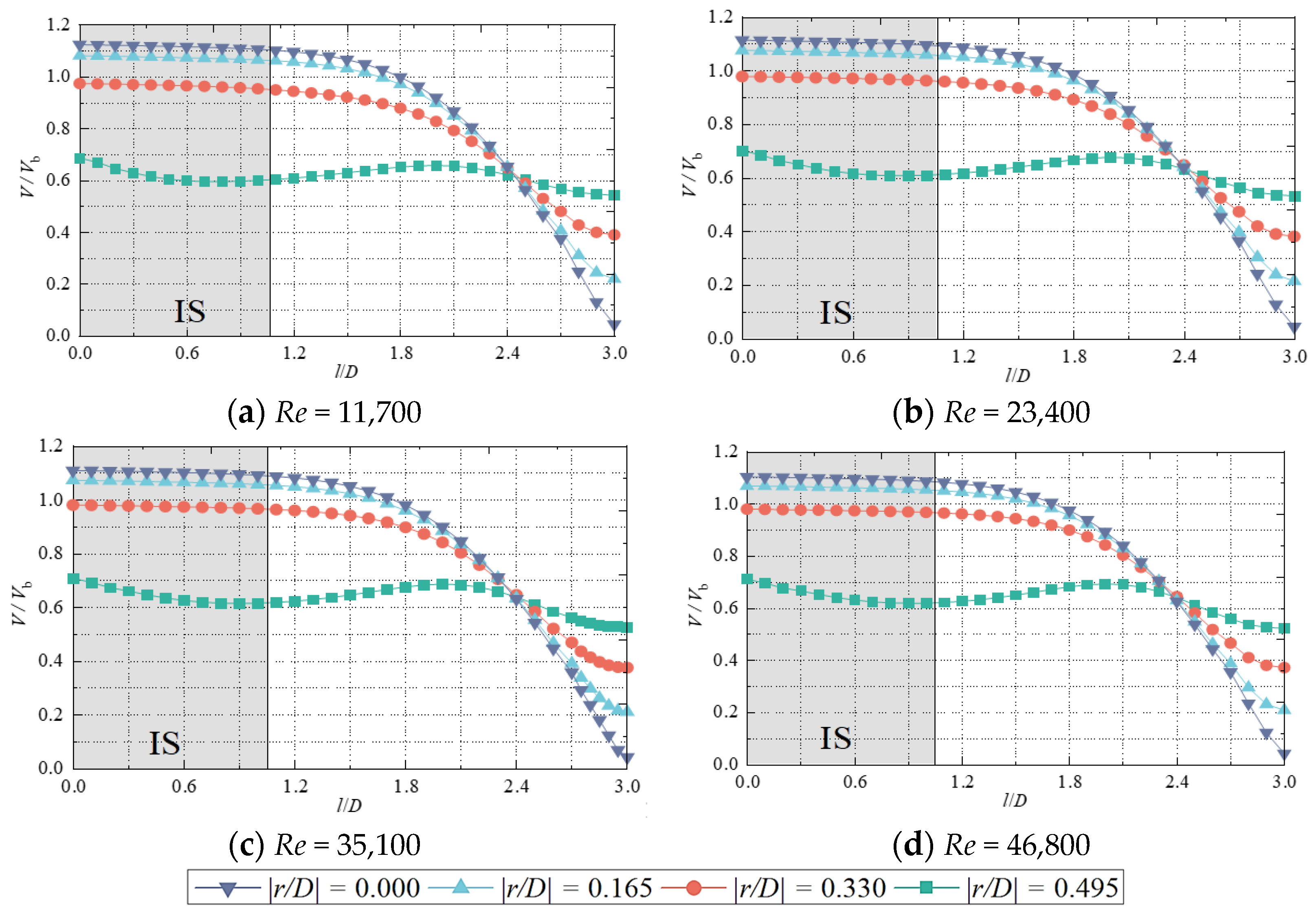
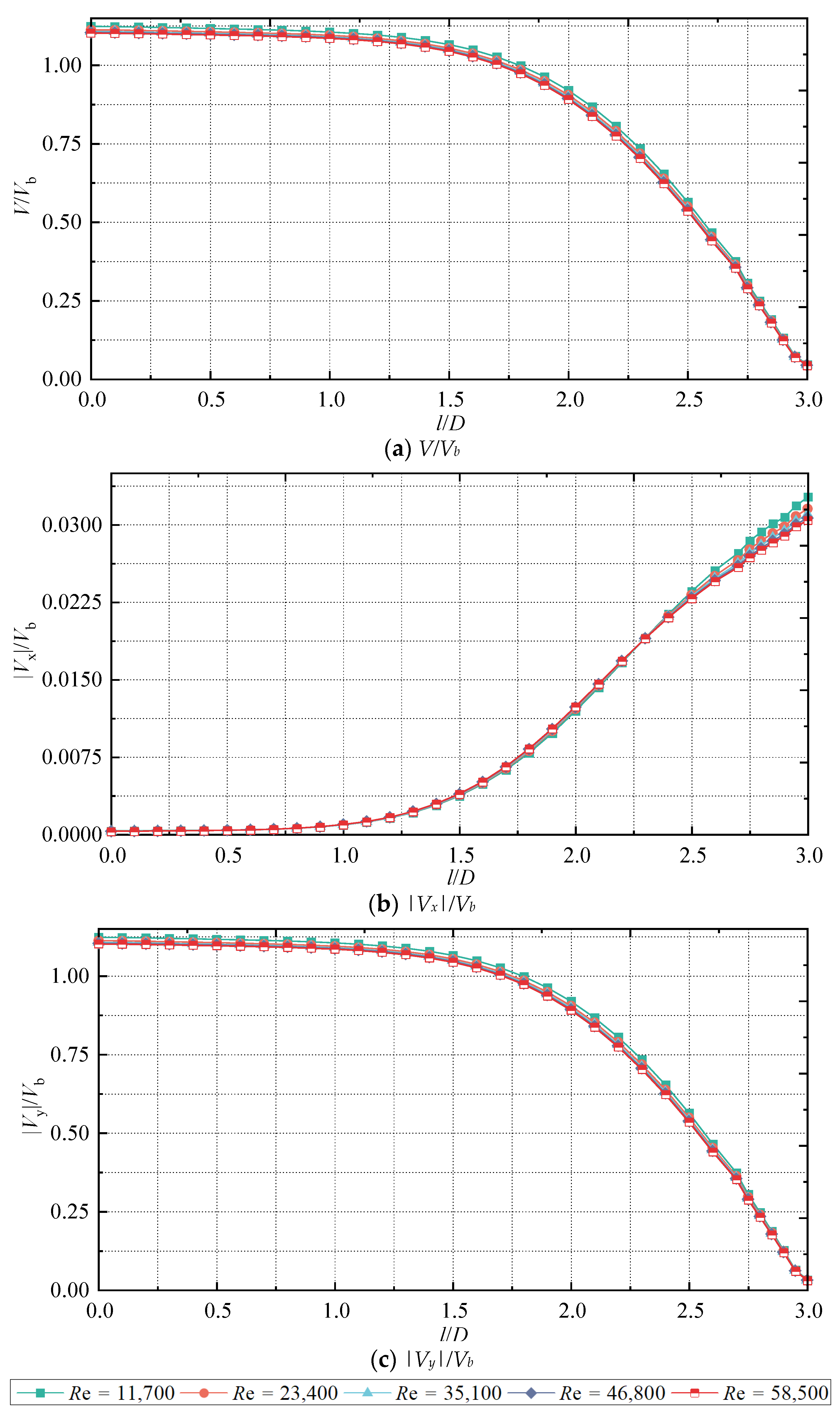
5.2. Analysis of Submerged Jet Flow Field under Different Reynolds Numbers
6. Conclusions
- (1)
- Flow-3D can properly simulate the flow of continuous submerged jets impacting a wall. Meanwhile, the prediction results based on the RNG k-ε turbulence model are in good agreement with the empirical equations and the results of the model experiments, indicating that the RNG k-ε turbulence model is suitable for the numerical simulation of the submerged impinging jet.
- (2)
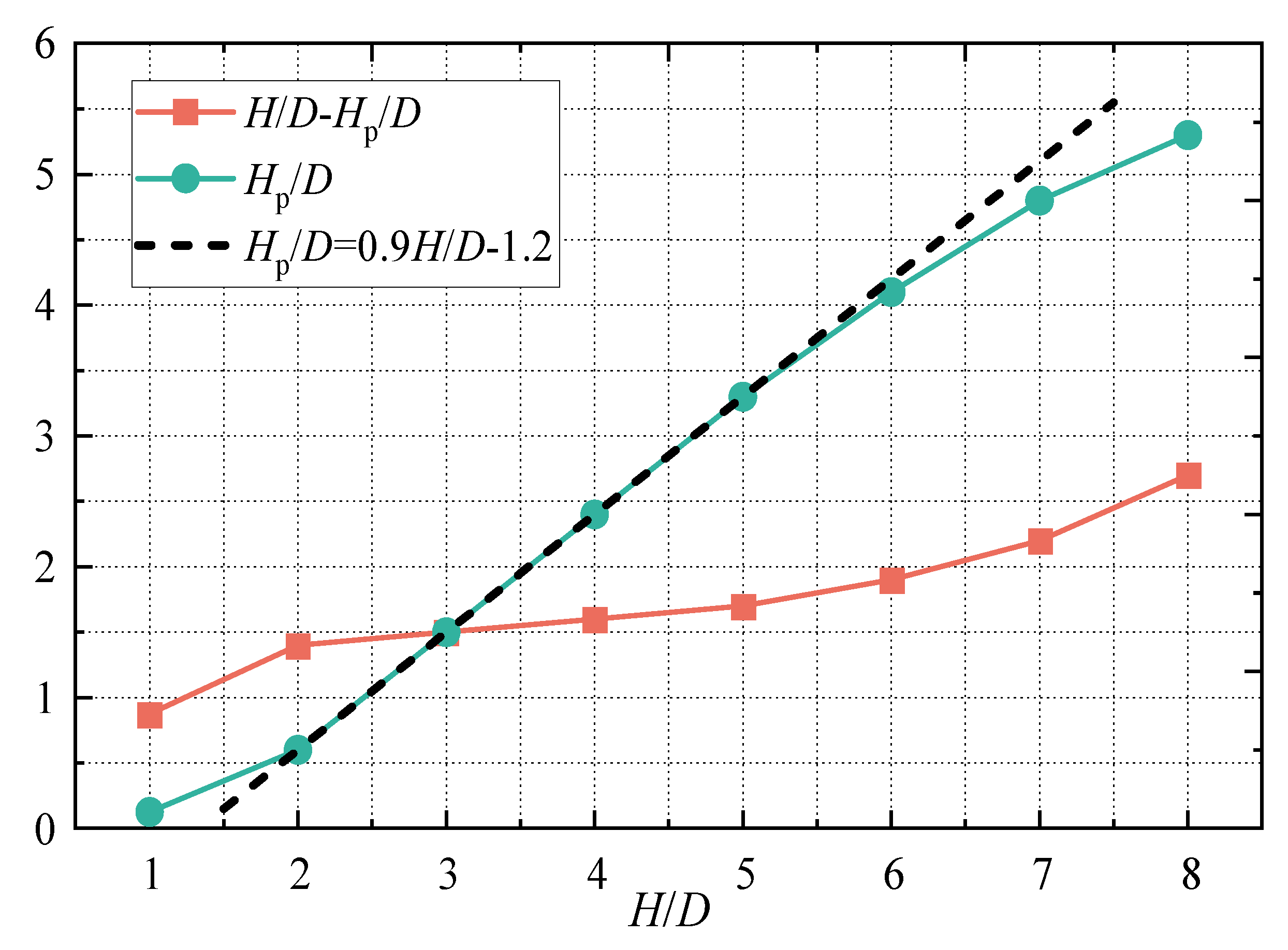
- The length of the potential core region Hp/D increases with the increase of the impinging distance H/D. Among them, when 2 ≤ H/D ≤ 5, the length of the potential core region Hp/D increases linearly with the increase of the impinging distance H/D; when H/D > 5, the length of the potential core region Hp/D still increases with the increase of the impinging distance H/D, but the increase decreases.
- (3)
- The length of the potential core region can be defined as the length at which the flow angle in the axial direction of the jet is kept near 90°. As the impinging distance H/D increases, the component in the direction of the flow axis at the end of the potential core region gradually increases.
- (4)
- The velocity V/Vb of the jet axis in the impact zone decreases linearly with the development of the jet. At the same time, the action range of the vortex caused by the wall flow of the jet after impacting the flat plate is gradually reduced inward with the increase of the impinging distance H/D.
- (5)
- The flow structure and flow characteristics of continuous submerged impinging jets are relatively independent of the Reynolds number Re; i.e., the length of the potential core region and the relationship between the axial velocity of the jet within the impinging zone and the impinging distance are also applicable to the conditions at other Reynolds numbers.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Zhang, J.; Li, Y.; Zhang, Y.; Yang, F.; Liang, C.; Tan, S. Using a high-pressure water jet-assisted tunnel boring machine to break rock. Adv. Mech. Eng. 2020, 12, 1687814020962290. [Google Scholar] [CrossRef]
- Shi, X.; Zhang, G.; Xu, G.; Ma, Y.; Wu, X. Inactivating Microorganism on Medical Instrument Using Plasma Jet. High Volt. Eng. 2009, 35, 632–635. [Google Scholar]
- Gao, Y.; Han, P.; Wang, F.; Cao, J.; Zhang, S. Study on the Characteristics of Water Jet Breaking Coal Rock in a Drilling Hole. Sustainability 2022, 14, 8258. [Google Scholar] [CrossRef]
- Xu, W.; Wang, C.; Zhang, L.; Ge, J.; Zhang, D.; Gao, Z. Numerical study of continuous jet impinging on a rotating wall based on Wray—Agarwal turbulence model. J. Braz. Soc. Mech. Sci. Eng. 2022, 44, 433. [Google Scholar] [CrossRef]
- Hu, B.; Wang, C.; Wang, H.; Yu, Q.; Liu, J.; Zhu, Y.; Ge, J.; Chen, X.; Yang, Y. Numerical Simulation Study of the Horizontal Submerged Jet Based on the Wray—Agarwal Turbulence Model. J. Mar. Sci. Eng. 2022, 10, 1217. [Google Scholar] [CrossRef]
- Dahiya, A.K.; Bhuyan, B.K.; Kumar, S. Perspective study of abrasive water jet machining of composites—A review. J. Mech. Sci. Technol. 2022, 36, 213–224. [Google Scholar] [CrossRef]
- Abushanab, W.S.; Moustafa, E.B.; Harish, M.; Shanmugan, S.; Elsheikh, A.H. Experimental investigation on surface characteristics of Ti6Al4V alloy during abrasive water jet machining process. Alex. Eng. J. 2022, 61, 7529–7539. [Google Scholar] [CrossRef]
- Hu, B.; Wang, H.; Liu, J.; Zhu, Y.; Wang, C.; Ge, J.; Zhang, Y. A numerical study of a submerged water jet impinging on a stationary wall. J. Mar. Sci. Eng. 2022, 10, 228. [Google Scholar] [CrossRef]
- Peng, J.; Shen, H.; Xie, W.; Zhai, S.; Xi, G. Influence of flow fluctuation characteristics on flow and heat transfer in different regions. J. Drain. Irrig. Mach. Eng. 2022, 40, 826–833. [Google Scholar]
- Zhai, S.; Xie, F.; Yin, G.; Xi, G. Effect of gap ratio on vortex-induced vibration characteristics of different blunt bodies near-wall. J. Drain. Irrig. Mach. Eng. 2021, 39, 1132–1138. [Google Scholar]
- Lin, W.; Zhou, Y.; Wang, L.; Tao, L. PIV experiment and numerical simulation of trailing vortex structure of improved INTER-MIG impeller. J. Drain. Irrig. Mach. Eng. 2021, 39, 158–164. [Google Scholar]
- Han, B.; Yao, Z.; Tang, R.; Xu, H. On the supersonic impinging jet by laser Doppler velocimetry. Exp. Meas. Fluid Mech. 2002, 16, 99–103. [Google Scholar]
- Darisse, A.; Lemay, J.; Benaissa, A. LDV measurements of well converged third order moments in the far field of a free turbulent round jet. Exp. Therm. Fluid Sci. 2013, 44, 825–833. [Google Scholar] [CrossRef]
- Kumar, S.; Kumar, A. Effect of initial conditions on mean flow characteristics of a three dimensional turbulent wall jet. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2021, 235, 6177–6190. [Google Scholar] [CrossRef]
- Tao, D.; Zhang, R.; Ying, C. Development and application of the pollutant diffusion testing apparatus based on the image analysis. J. Saf. Environ. 2016, 16, 247–251. [Google Scholar]
- Seo, H.; Kim, K.C. Experimental study on flow and turbulence characteristics of bubbly jet with low void fraction. Int. J. Multiph. Flow 2021, 142, 103738. [Google Scholar] [CrossRef]
- Wen, Q.; Sha, J.; Liu, Y. TR-PIV measurement of the turbulent submerged jet and POB analysis of the dynamic structure. J. Exp. Fluid Mech. 2014, 4, 16–24. [Google Scholar]
- Yang, Y.; Zhou, L.; Shi, W.; He, Z.; Han, Y.; Xiao, Y. Interstage difference of pressure pulsation in a three-stage electrical submersible pump. J. Petrol. Sci. Eng. 2021, 196, 107653. [Google Scholar] [CrossRef]
- Tang, S.; Zhu, Y.; Yuan, S. An improved convolutional neural network with an adaptable learning rate towards multi-signal fault diagnosis of hydraulic piston pump. Adv. Eng. Inform. 2021, 50, 101406. [Google Scholar] [CrossRef]
- Han, Y.; Song, X.; Li, K.; Yan, X. Hybrid modeling for submergence depth of the pumping well using stochastic configuration networks with random sampling. J. Petrol. Sci. Eng. 2022, 208, 109423. [Google Scholar] [CrossRef]
- Tang, S.; Zhu, Y.; Yuan, S. A novel adaptive convolutional neural network for fault diagnosis of hydraulic piston pump with acoustic images. Adv. Eng. Inform. 2022, 52, 101554. [Google Scholar] [CrossRef]
- Long, J.; Song, X.; Shi, J.; Chen, J. Optimization and CFD Analysis on Nozzle Exit Position of Two-phase Ejector. J. Refrig. 2022, 43, 39–45. [Google Scholar]
- Ni, Q.; Ruan, W. Optimization design of desilting jet pump parameters based on response surface model. J. Ship Mech. 2022, 26, 365–374. [Google Scholar]
- Zhang, K.; Zhu, X.; Ren, X.; Qiu, Q.; Shen, S. Numerical investigation on the effect of nozzle position for design of high performance ejector. Appl. Therm. Eng. 2017, 126, 594–601. [Google Scholar] [CrossRef]
- Fu, W.; Liu, Z.; Li, Y.; Wu, H.; Tang, Y. Numerical study for the influences of primary steam nozzle distance and mixing chamber throat diameter on steam ejector performance. Int. J. Therm. Sci. 2018, 132, 509–516. [Google Scholar] [CrossRef]
- Lucas, C.; Rusche, H.; Schroeder, A.; Koehler, J. Numerical investigation of a two-phase CO2 ejector. Int. J. Refrigeration 2014, 43, 154–166. [Google Scholar] [CrossRef]
- Ma, X.; Zhu, T.; Fu, Y.; Yan, Y.; Chen, W. Numerical simulation of rock breaking by abrasive water jet. J. Coast. Res. 2019, 93, 274–283. [Google Scholar] [CrossRef]
- He, L.; Liu, Y.; Shen, K.; Yang, X.; Ba, Q.; Xiong, W. Numerical research on the dynamic rock-breaking process of impact drilling with multi-nozzle water jets. J. Pet. Sci. Eng. 2021, 207, 109145. [Google Scholar] [CrossRef]
- Yu, Z.; Wang, Z.; Lei, C.; Zhou, Y.; Qiu, X. Numerical Simulation on Internal Flow Field of a Self-excited Oscillation Pulsed Jet Nozzle with Back-flow. Mech. Sci. Technol. Aerosp. Eng. 2022, 41, 998–1002. [Google Scholar]
- Huang, J.; Ni, F.; Gu, L. Numerical method of FLOW-3D for sediment erosion simulation. China Harb. Eng. 2019, 39, 6–11. [Google Scholar]
- Al Shaikhli, H.I.; Khassaf, S.I. Using of flow 3d as CFD materials approach in waves generation. Mater. Today Proc. 2022, 49, 2907–2911. [Google Scholar] [CrossRef]
- Kosaj, R.; Alboresha, R.S.; Sulaiman, S.O. Comparison Between Numerical Flow3d Software and Laboratory Data, For Sediment Incipient Motion. IOP Conf. Ser. Earth Environ. Sci. 2022, 961, 012031. [Google Scholar] [CrossRef]
- Du, C.; Liu, X.; Zhang, J.; Wang, B.; Chen, X.; Yu, X. Long-distance water hammer protection of pipeline after pump being first lowered and then rasied. J. Drain. Irrig. Mach. Eng. 2022, 40, 1248–1253, 1267. [Google Scholar]
- Gao, F.; Li, X.; Gao, Q. Experiment and numerical simulation on hydraulic characteristics of novel trapezoidal measuring weir. J. Drain. Irrig. Mach. Eng. 2022, 40, 1104–1111. [Google Scholar]
- Tu, A.; Nie, X.; Li, Y.; Li, H. Experimental and simulation study on water infiltration characteristics of layered red soil. J. Drain. Irrig. Mach. Eng. 2021, 39, 1243–1249. [Google Scholar]
- Chen, J.; Zeng, B.; Liu, L.; Tao, K.; Zhao, H.; Zhang, C.; Zhang, J.; Li, D. Investigating the anchorage performance of full-grouted anchor bolts with a modified numerical simulation method. Eng. Fail. Anal. 2022, 141, 106640. [Google Scholar] [CrossRef]
- Hu, B.; Yao, Y.; Wang, M.; Wang, C.; Liu, Y. Flow and Performance of the Disk Cavity of a Marine Gas Turbine at Varying Nozzle Pressure and Low Rotation Speeds: A Numerical Investigation. Machines 2023, 11, 68. [Google Scholar] [CrossRef]
- Yao, J.; Wang, X.; Zhang, S.; Xu, S.; Jin, B.; Ding, S. Orthogonal test of important parameters affecting hydraulic performance of negative pressure feedback jet sprinkler. J. Drain. Irrig. Mach. Eng. 2021, 39, 966–972. [Google Scholar]
- Wang, C.; Wang, X.; Shi, W.; Lu, W.; Tan, S.K.; Zhou, L. Experimental investigation on impingement of a submerged circular water jet at varying impinging angles and Reynolds numbers. Exp. Therm. Fluid Sci. 2017, 89, 189–198. [Google Scholar] [CrossRef]
- Speziale, C.G.; Thangam, S. Analysis of an RNG based turbulence model for separated flows. Int. J. Eng. Sci. 1992, 30, 1379–1388. [Google Scholar] [CrossRef]
- El Hassan, M.; Assoum, H.H.; Sobolik, V.; Vétel, J.; Abed-Meraim, K.; Garon, A.; Sakout, A. Experimental investigation of the wall shear stress and the vortex dynamics in a circular impinging jet. Exp. Fluids 2012, 52, 1475–1489. [Google Scholar] [CrossRef]
- Fairweather, M.; Hargrave, G. Experimental investigation of an axisymmetric, impinging turbulent jet. 1. Velocity field. Exp. Fluids 2002, 33, 464–471. [Google Scholar] [CrossRef]
- Ashforth-Frost, S.; Jambunathan, K. Effect of nozzle geometry and semi-confinement on the potential core of a turbulent axisymmetric free jet. Int. Commun. Heat Mass Transf. 1996, 23, 155–162. [Google Scholar] [CrossRef]
- Chen, M.; Huang, H.; Wang, D.; Lv, S.; Chen, Y. PIV tests for flow characteristics of impinging jet in a semi-closed circular pipe. J. Vib. Shock 2021, 40, 90–97, 113. [Google Scholar]


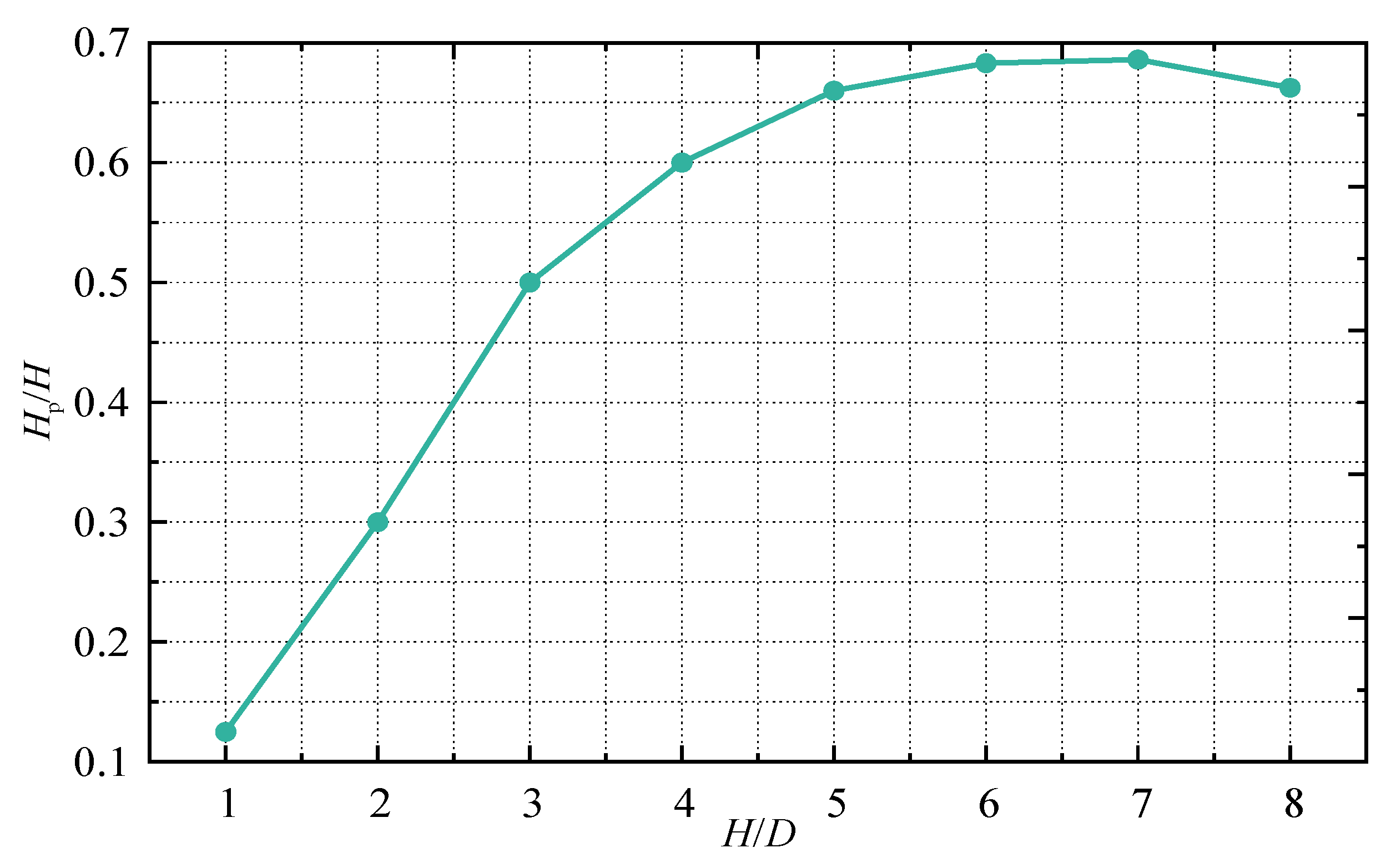


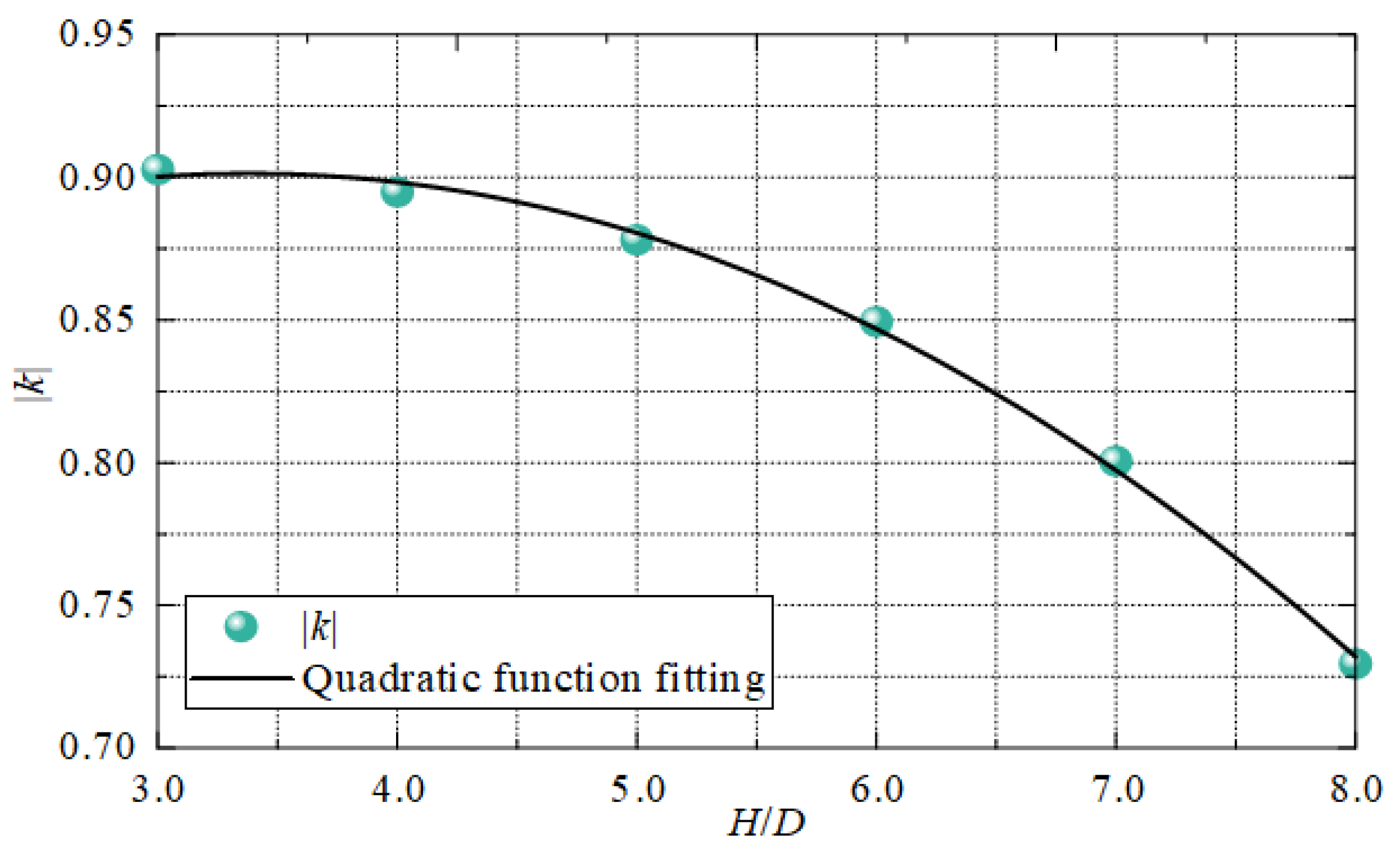
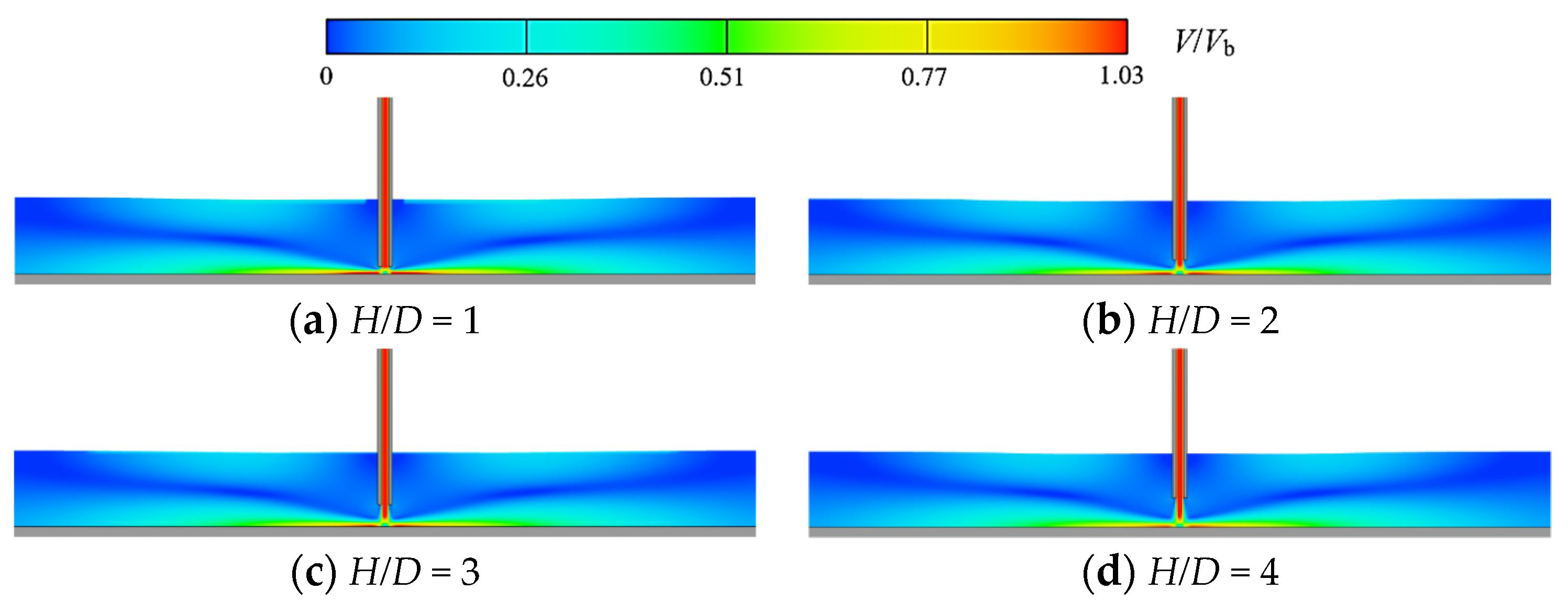
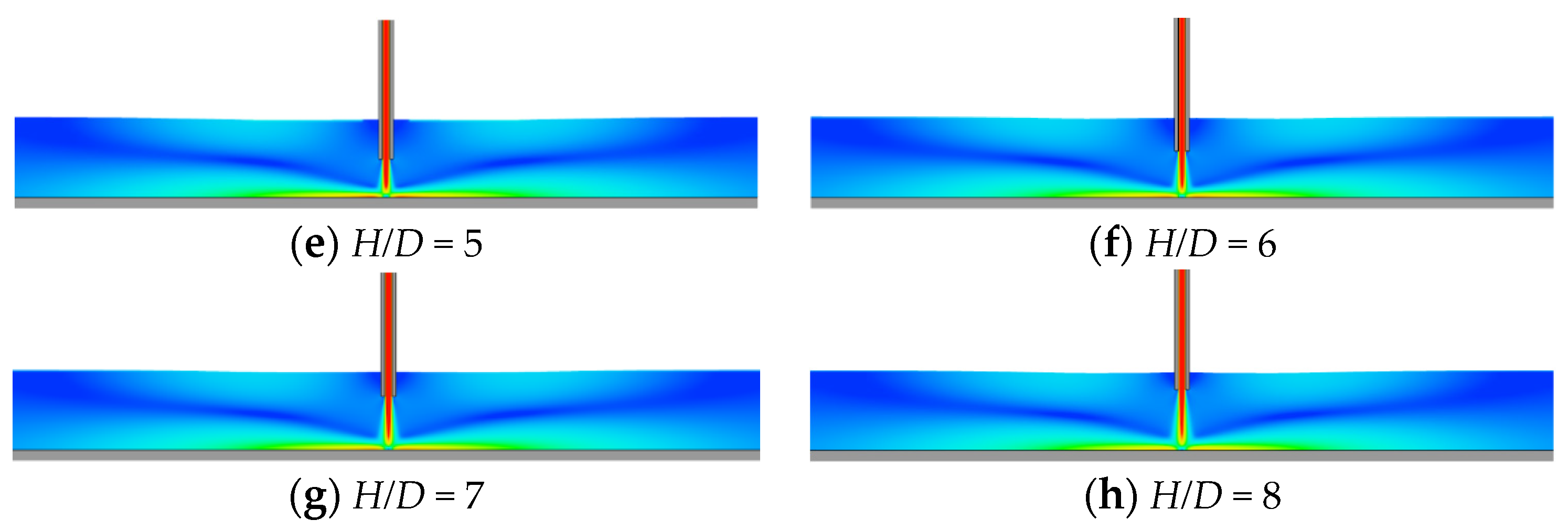

| Impinging Distance H/D | Slope k | (ki+1−ki) × 10−2 | Standard Error K | Intercept b | Standard Error b |
|---|---|---|---|---|---|
| 1 | −1.02709 | 0.03184 | 1.11785 | 0.01861 | |
| 11.10900 | |||||
| 2 | −0.91600 | 0.02053 | 1.89851 | 0.03141 | |
| 1.33400 | |||||
| 3 | −0.90266 | 0.02614 | 2.76997 | 0.06783 | |
| 0.78100 | |||||
| 4 | −0.89485 | 0.02336 | 3.65376 | 0.08207 | |
| 1.67900 | |||||
| 5 | −0.87806 | 0.02715 | 4.47909 | 0.12244 | |
| 2.86400 | |||||
| 6 | −0.84942 | 0.02813 | 5.19117 | 0.15494 | |
| 4.88700 | |||||
| 7 | −0.80055 | 0.02342 | 5.68175 | 0.1524 | |
| 7.09600 | |||||
| 8 | −0.72959 | 0.01698 | 5.89248 | 0.12745 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mi, H.; Wang, C.; Jia, X.; Hu, B.; Wang, H.; Wang, H.; Zhu, Y. Hydraulic Characteristics of Continuous Submerged Jet Impinging on a Wall by Using Numerical Simulation and PIV Experiment. Sustainability 2023, 15, 5159. https://doi.org/10.3390/su15065159
Mi H, Wang C, Jia X, Hu B, Wang H, Wang H, Zhu Y. Hydraulic Characteristics of Continuous Submerged Jet Impinging on a Wall by Using Numerical Simulation and PIV Experiment. Sustainability. 2023; 15(6):5159. https://doi.org/10.3390/su15065159
Chicago/Turabian StyleMi, Hongbo, Chuan Wang, Xuanwen Jia, Bo Hu, Hongliang Wang, Hui Wang, and Yong Zhu. 2023. "Hydraulic Characteristics of Continuous Submerged Jet Impinging on a Wall by Using Numerical Simulation and PIV Experiment" Sustainability 15, no. 6: 5159. https://doi.org/10.3390/su15065159
APA StyleMi, H., Wang, C., Jia, X., Hu, B., Wang, H., Wang, H., & Zhu, Y. (2023). Hydraulic Characteristics of Continuous Submerged Jet Impinging on a Wall by Using Numerical Simulation and PIV Experiment. Sustainability, 15(6), 5159. https://doi.org/10.3390/su15065159











