Numerical Implementation of Three-Dimensional Nonlinear Strength Model of Soil and Its Application in Slope Stability Analysis
Abstract
:1. Introduction
2. Three-Dimensional Nonlinear Strength Model
2.1. Failure Function on π Plane
2.2. Failure Function on the Triaxial Compression Meridian Plane
2.3. Nonlinear Strength Model in Principal Stress Space
3. Numerical Implementation of the Strength Model and UMAT Subroutine
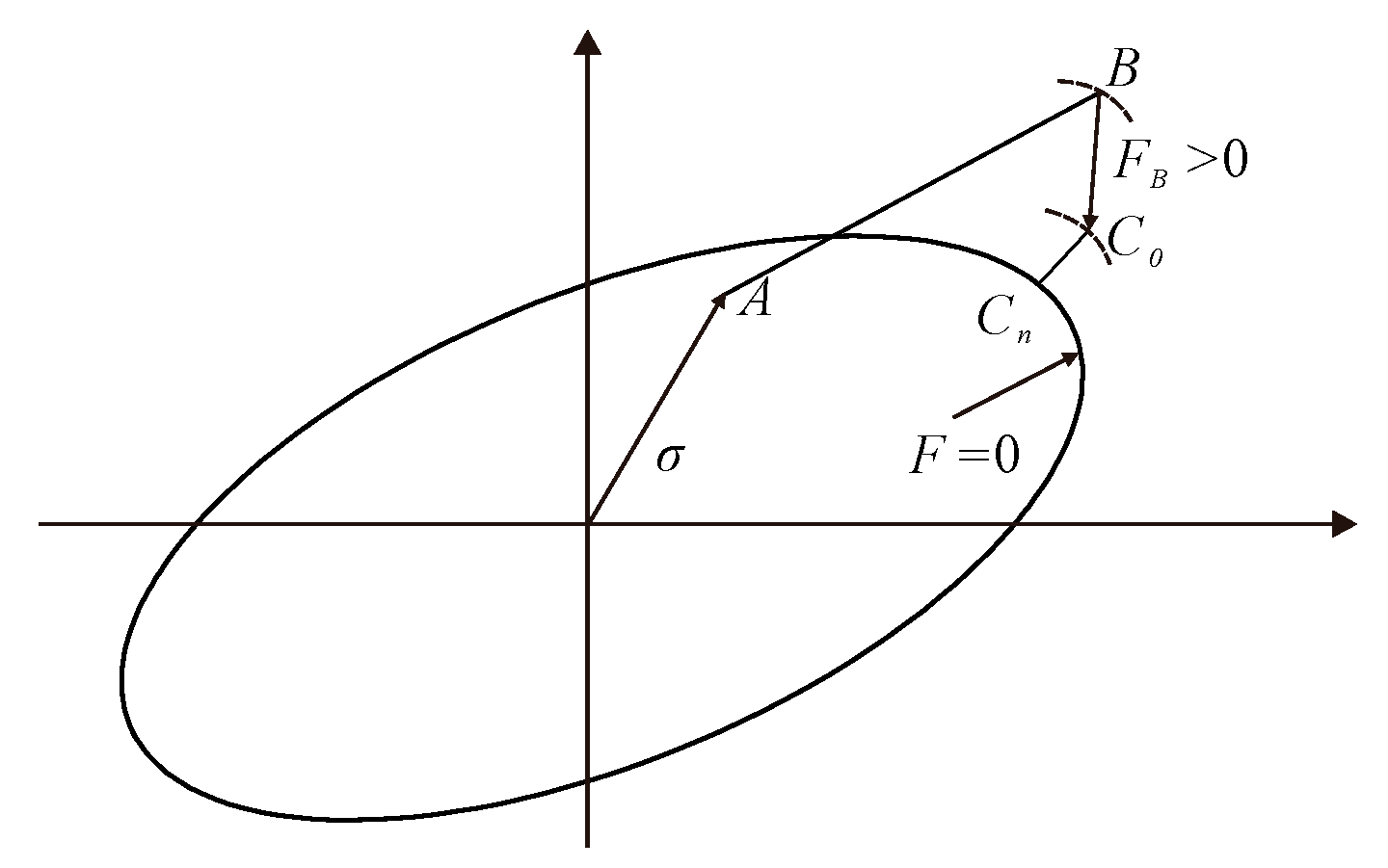
3.1. Stress Updating Algorithm
3.2. Secondary Development of UMAT
- (1)
- At the beginning of incremental loading step n, the ABAQUS main program transfers the stress tensor , the total strain , the total strain increment , and the time increment to the UMAT subroutine.
- (2)
- First, the elastic trial stress is calculated, and the plastic parameters are calculated with the implicit backward Euler integration algorithm. Second, the stress is pulled back to the yield surface through iterative calculation, and the consistent tangent modulus , the Jacobian matrix, is calculated. Finally, the stress increment can be calculated, and the stress is updated to .
- (3)
- At time , the ABAQUS main program uses the Newton–Raphson iterative method to perform the iterative calculations. If the calculation result converges, the next incremental step is performed with ; if not, the time increment of the incremental step is reduced until the calculation result converges.
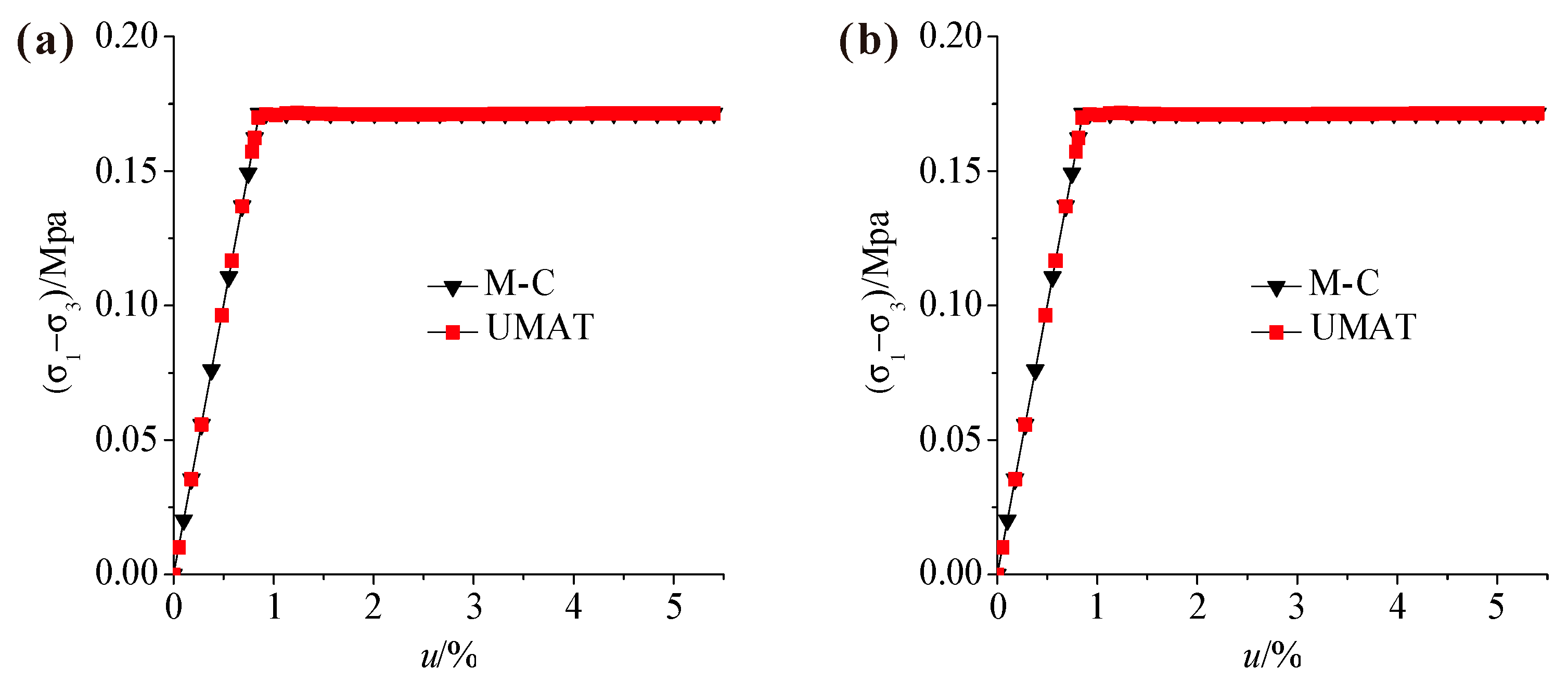
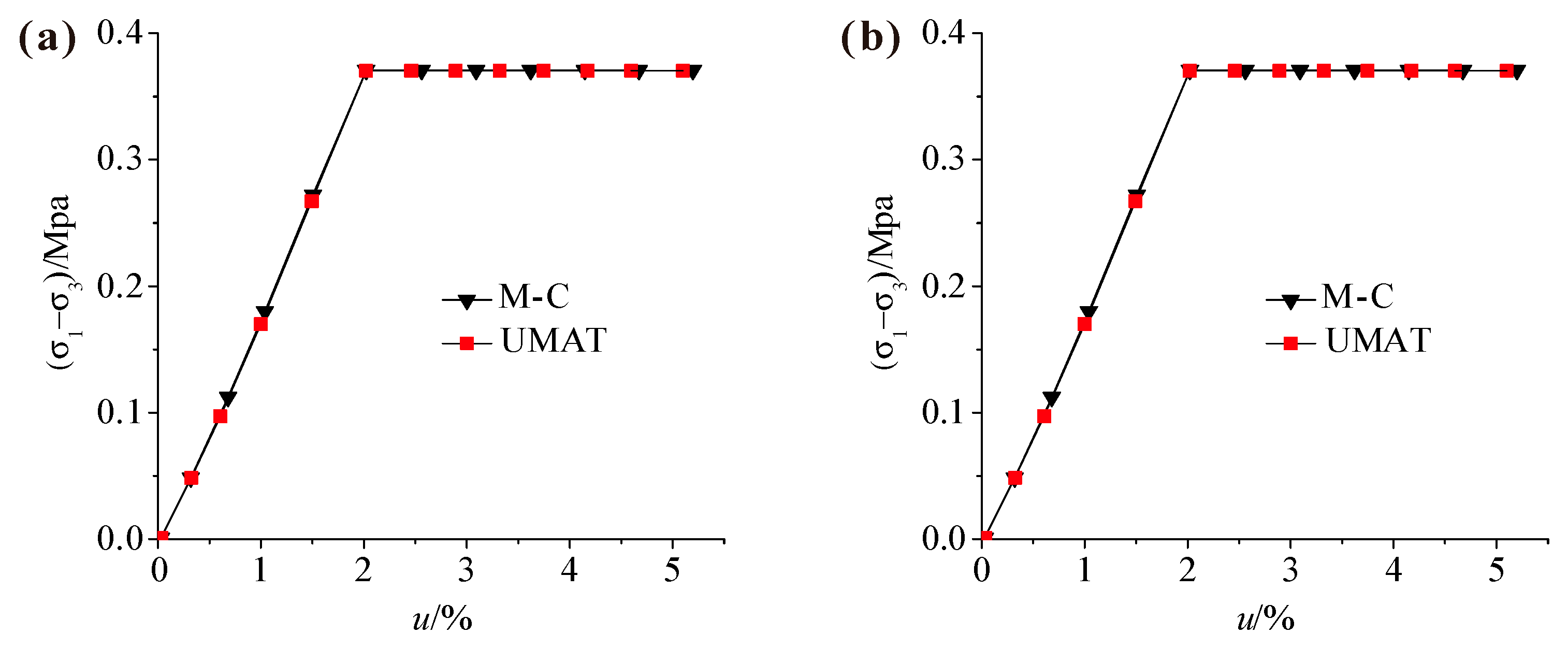
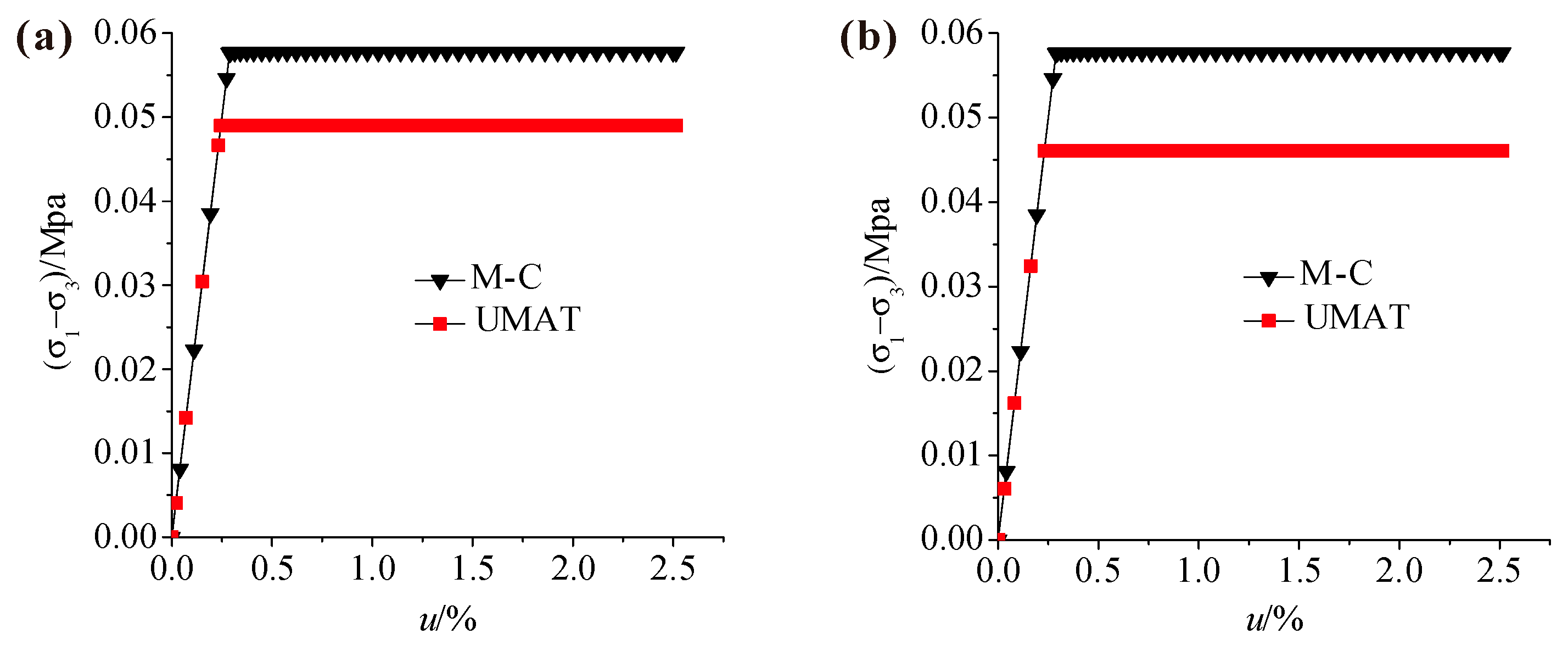
3.3. UMAT Subroutine Verification
3.3.1. Triaxial Compression Test
3.3.2. Uniaxial Tensile Test
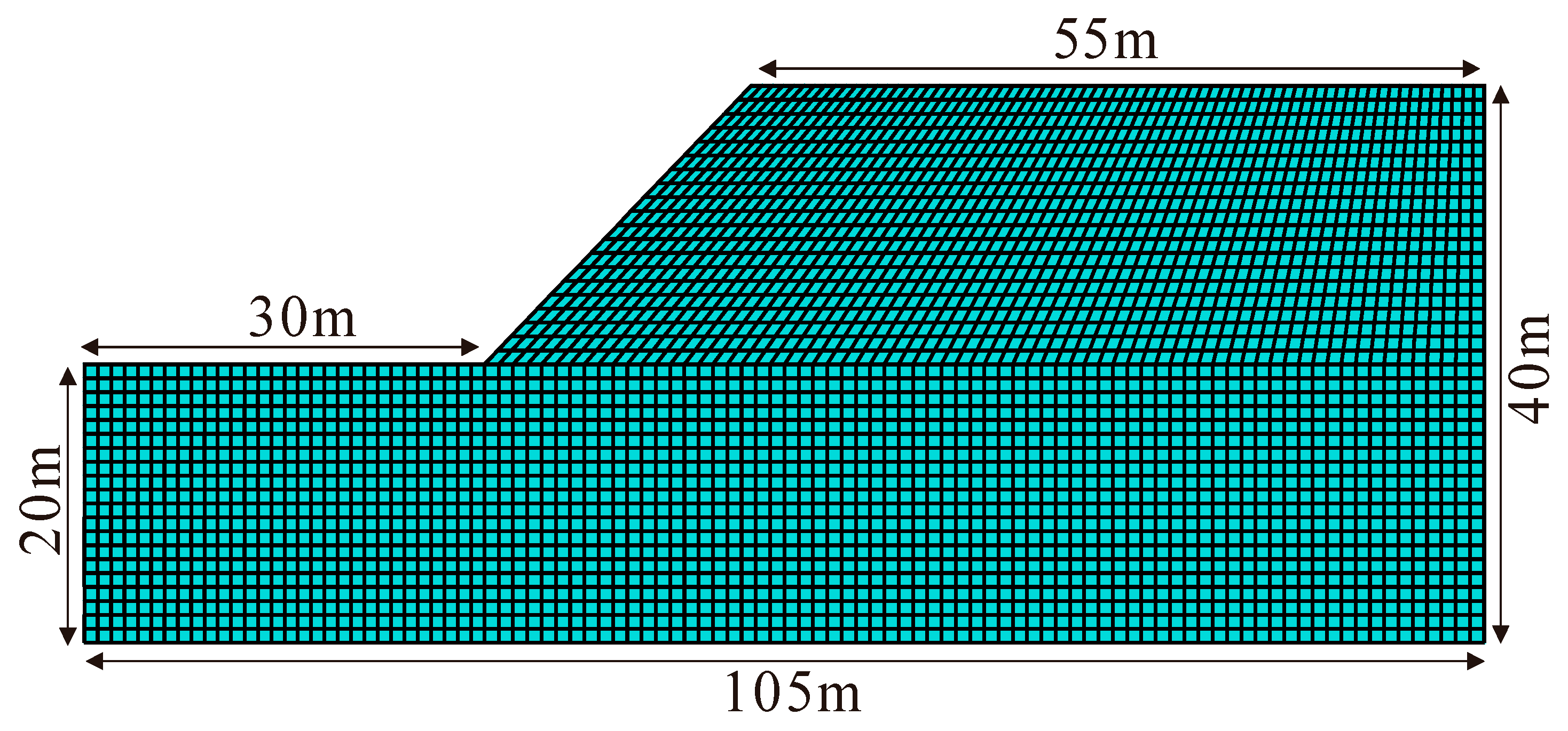
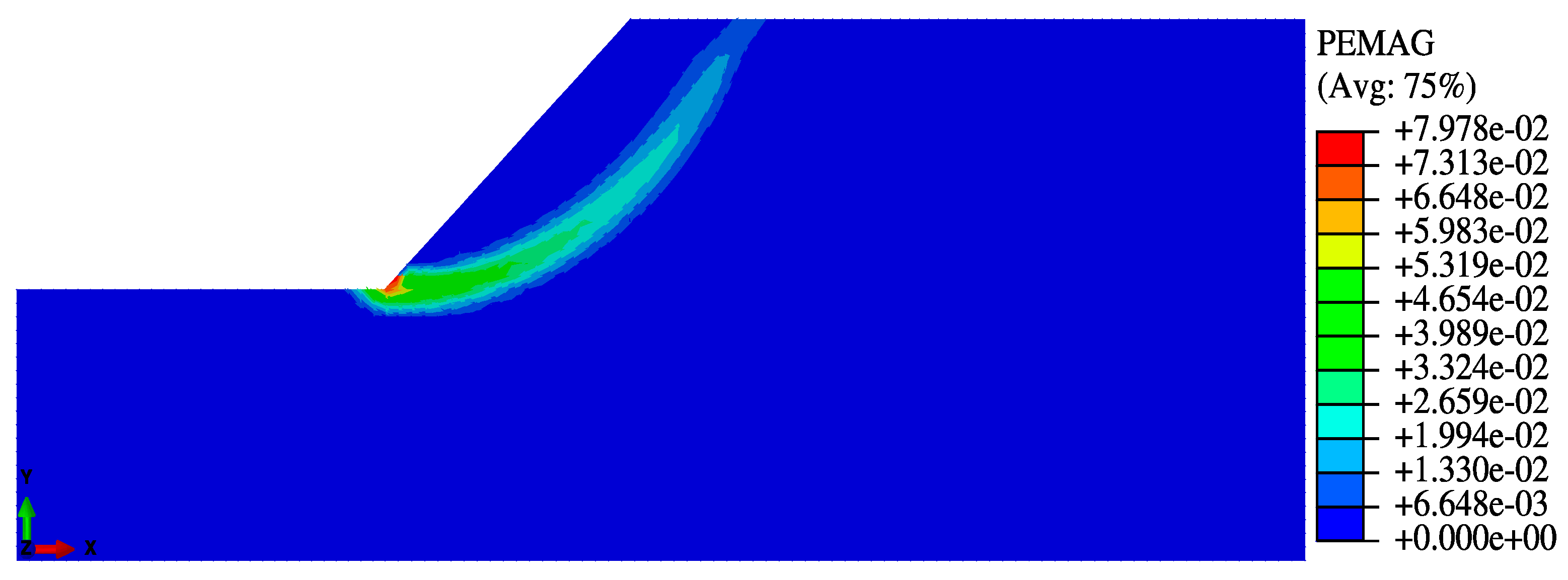
4. Numerical Examples of Saturated Slope
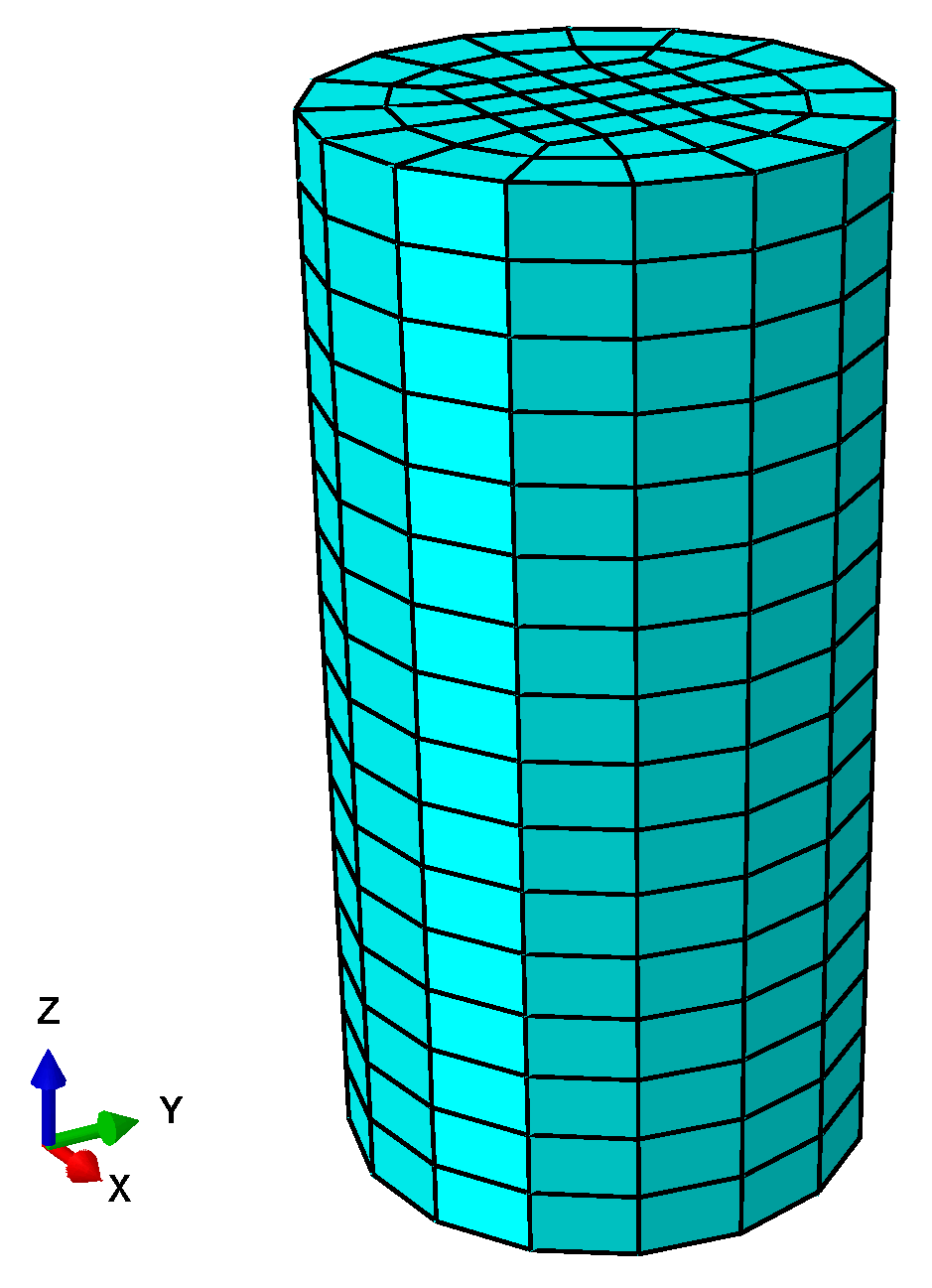
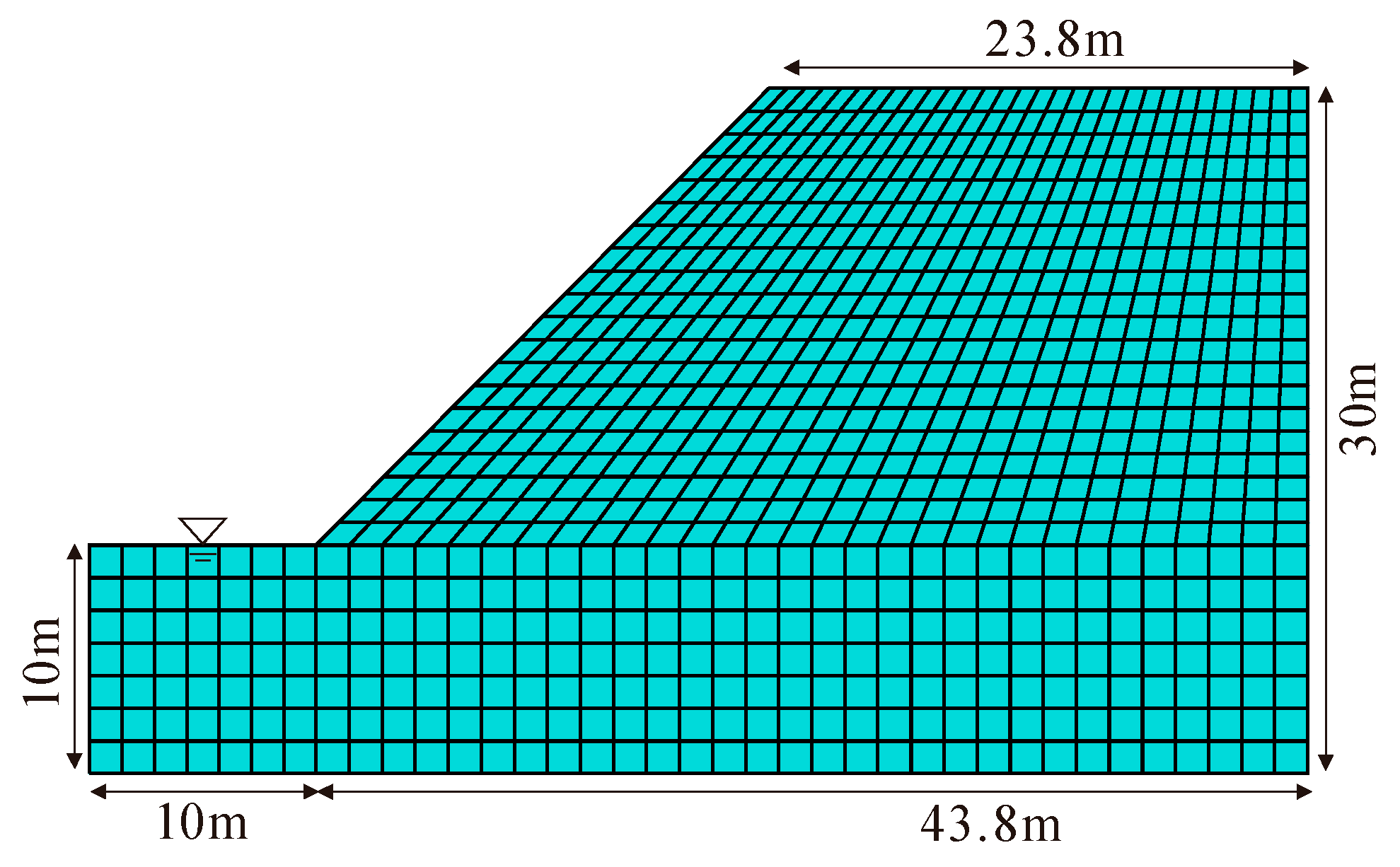
4.1. Analysis Model of Slope
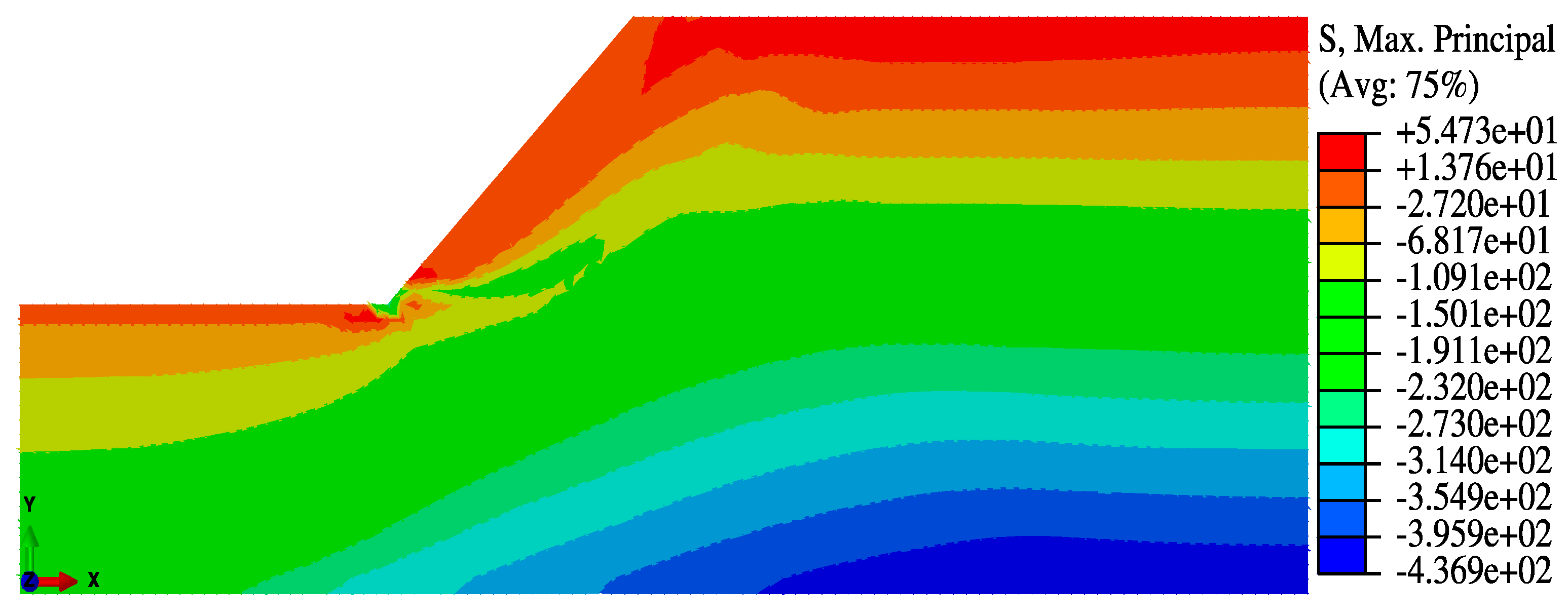
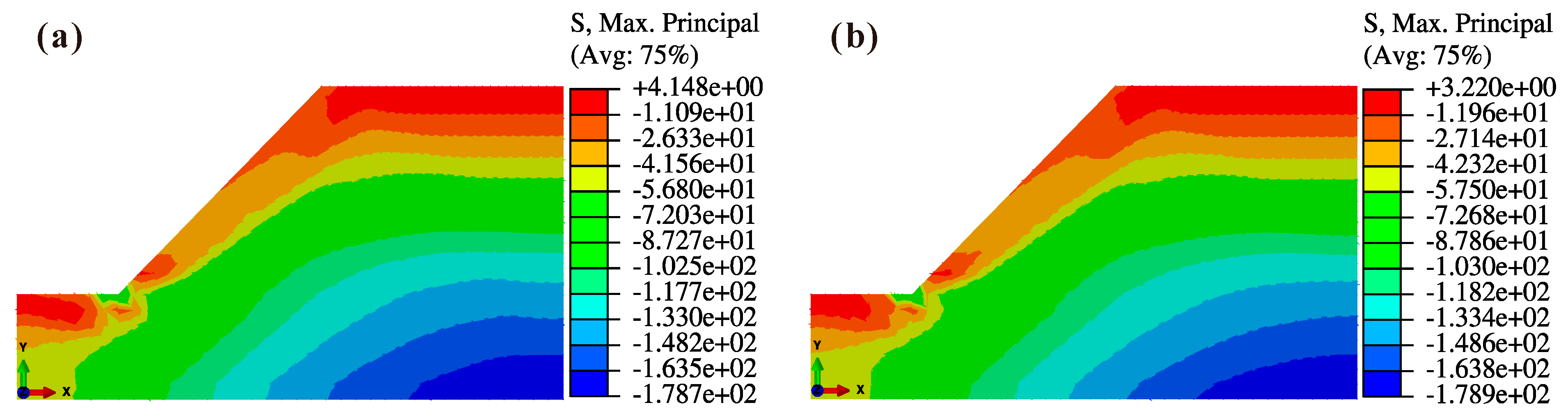
4.2. Slope Analysis Based on M-C Strength Model
4.3. Slope Analysis Based on Three-Dimensional Nonlinear Strength Model
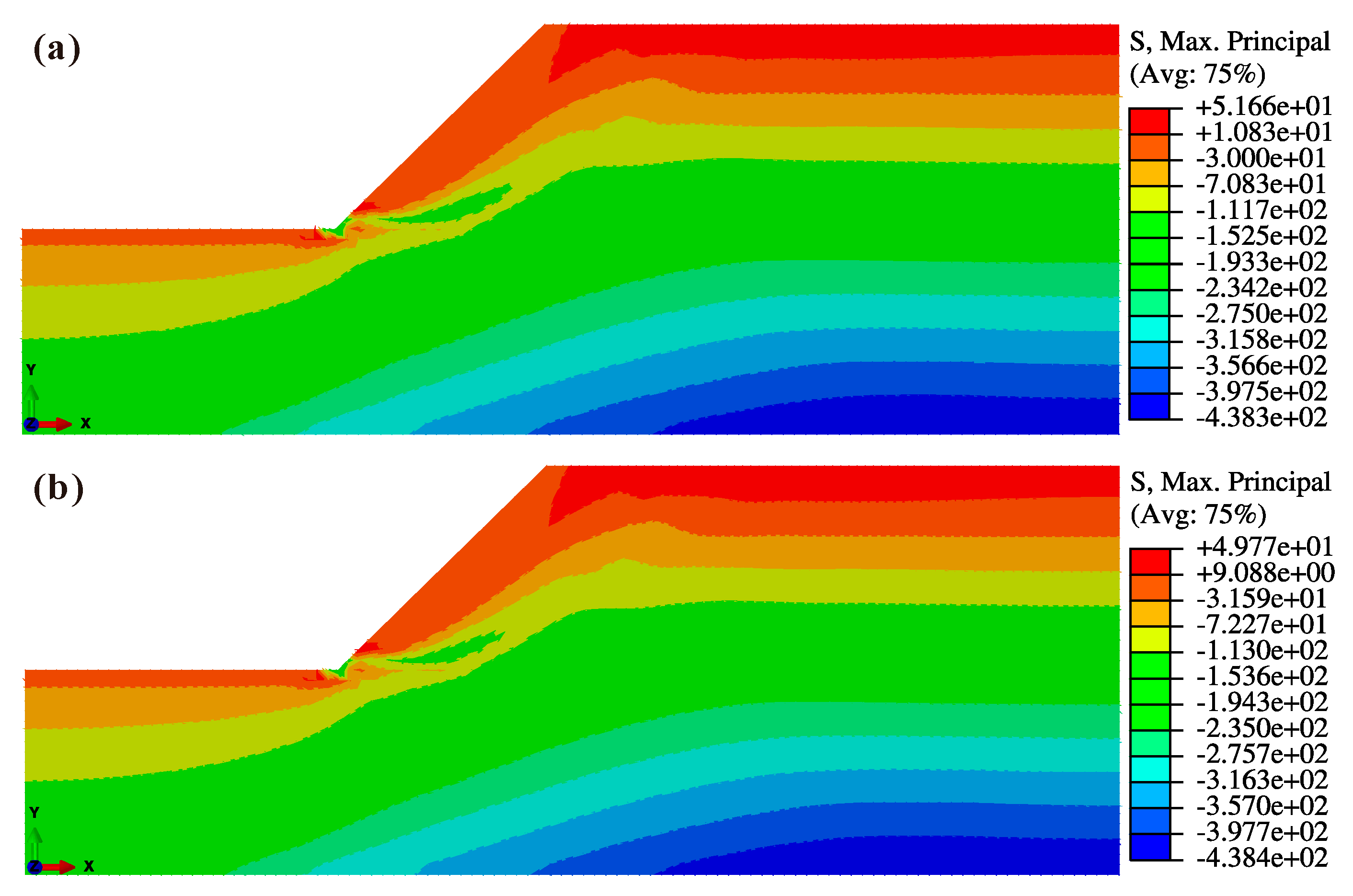
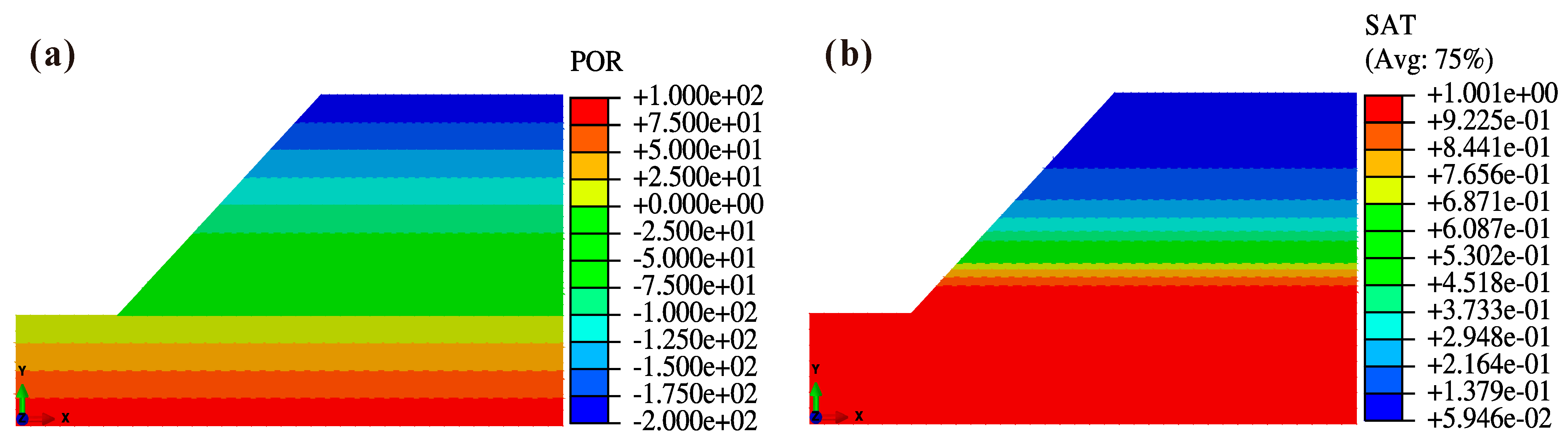
5. Numerical Examples of Unsaturated Slope
5.1. Analysis Model of Slope
5.2. Unsaturated Slope without Rainfall
5.3. Unsaturated Slope with Rainfall Infiltration
6. Conclusions
- (1)
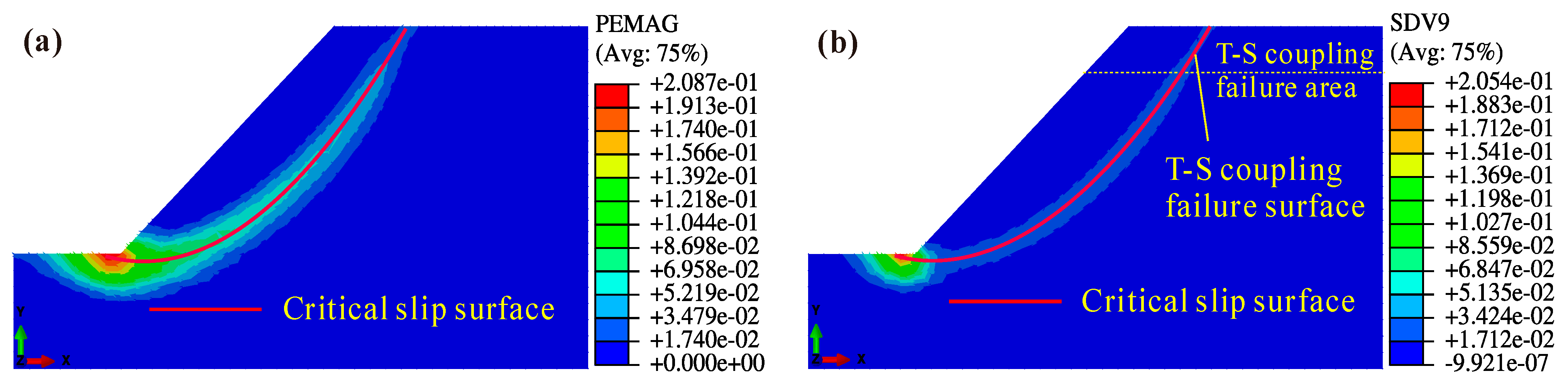
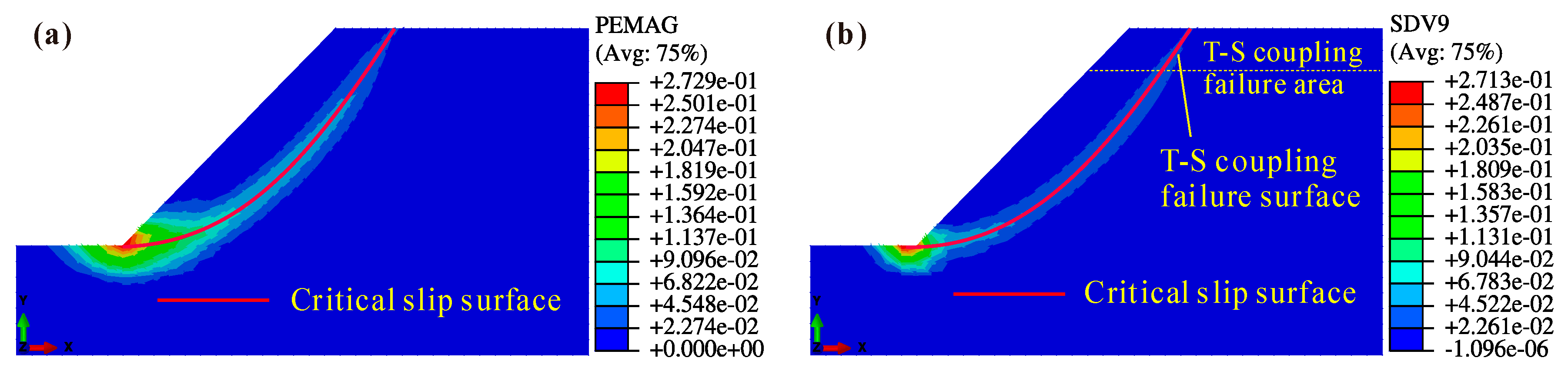
- For saturated slopes, a comparative analysis of the calculation results between the three-dimensional nonlinear strength model and the MC strength model, using the strength reduction finite element method, is conducted. The three-dimensional nonlinear strength model proposed in this paper can better describe the gradual development processes of the T-S coupling plastic zone and T-S coupling failure surface. The critical slip surface is a composite slip surface composed of a C-S slip surface and T-S coupling failure surface, and the obtained safety factor is small and inclined to be safe.
- (2)
- For unsaturated soil slopes, the stabilities of slopes under the conditions of no rainfall and rainfall infiltration are analyzed by using the strength reduction finite element method under fluid–solid coupling. Compared with the calculation results of the M-C strength model, the three-dimensional nonlinear strength model shows apparent advantages, which are the same as the conclusions from the analysis of saturated slopes.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A
References
- Yao, Y.P.; Lu, D.C.; Zhou, A.N.; Bo, Z. Generalized non-linear strength theory and transformed stress space. Sci. China Ser. E Technol. Sci. 2004, 47, 691–709. [Google Scholar] [CrossRef]
- Matsuoka, H.; Hoshikawa, T.; Ueno, K. A general failure criterion and stress-strain relation for granular materials to metals. Soils Found. 1990, 30, 119–127. [Google Scholar] [CrossRef] [Green Version]
- Lade, P.V.; Duncan, J.M. Elastoplastic stress-strain theory for cohesionless soil. J. Geotech. Eng. Div. 1975, 101, 1037–1053. [Google Scholar] [CrossRef]
- Lade, P.V. Estimating parameters from a single test for the three-dimensional failure criterion for frictional materials. J. Geotech. Geoenviron. Eng. 2014, 140, 4014038. [Google Scholar] [CrossRef]
- Yao, Y.P.; Zhou, A.N.; Lu, D.C. Extended transformed stress space for geomaterials and its application. J. Eng. Mech. 2007, 133, 1115–1123. [Google Scholar] [CrossRef]
- Choi, K.Y.; Cheung, R.W.M. Landslide disaster prevention and mitigation through works in Hong Kong. J. Rock Mech. Geotech. Eng. 2013, 5, 354–365. [Google Scholar] [CrossRef] [Green Version]
- Cai, G.Q.; Shi, P.X.; Kong, X.A.; Zhao, C.G.; Likos, W.J. Experimental study on tensile strength of unsaturated fine sands. Acta Geotech. 2020, 15, 1057–1065. [Google Scholar] [CrossRef]
- Zienkiewicz, O.C.; Humpheson, C.; Lewis, R.W. Associated and non-associated visco-plasticity and plasticity in soil mechanics. Geotechnique 1975, 25, 671–689. [Google Scholar] [CrossRef]
- Ugai, K. A method of calculation of total safety factor of slope by elasto-plastic FEM. Soils Found. 1989, 29, 190–195. [Google Scholar] [CrossRef] [Green Version]
- Ugai, K.; Leshchinsky, D.O.V. Three-dimensional limit equilibrium and finite element analyses: A comparison of results. Soils Found. 1995, 35, 1–7. [Google Scholar] [CrossRef]
- Griffiths, D.V.; Lane, P.A. Slope stability analysis by finite elements. Geotechnique 1999, 49, 387–403. [Google Scholar] [CrossRef]
- Lane, P.A.; Griffiths, D.V. Assessment of stability of slopes under drawdown conditions. J. Geotech. Geoenviron. Eng. 2000, 126, 443–450. [Google Scholar] [CrossRef]
- Cai, F.; Ugai, K. Numerical analysis of rainfall effects on slope stability. Int. J. Geomech. 2004, 4, 69–78. [Google Scholar] [CrossRef]
- Schneider-Muntau, B.; Medicus, G.; Fellin, W. Strength reduction method in Barodesy. Comput. Geotech. 2018, 95, 57–67. [Google Scholar] [CrossRef]
- Senior, R.B. Tensile strength, tension cracks, and stability of slopes. Soils Found. 1981, 21, 1–17. [Google Scholar] [CrossRef] [Green Version]
- Toyota, H.; Nakamura, K.; Sramoon, W. Failure criterion of unsaturated soil considering tensile stress under three-dimensional stress conditions. Soils Found. 2004, 44, 1–13. [Google Scholar] [CrossRef] [Green Version]
- Michalowski, R.L. Stability assessment of slopes with cracks using limit analysis. Can. Geotech. J. 2013, 50, 1011–1021. [Google Scholar] [CrossRef]
- Michalowski, R.L. Stability of intact slopes with tensile strength cut-off. Géotechnique 2017, 67, 720–727. [Google Scholar] [CrossRef]
- Yin, C.; Li, W.H.; Zhao, C.G.; Kong, X.A. Impact of tensile strength and incident angles on a soil slope under earthquake SV-waves. Eng. Geol. 2019, 260, 105192. [Google Scholar] [CrossRef]
- Tang, L.S.; Zhao, Z.L.; Luo, Z.G.; Sun, Y.L. What is the role of tensile cracks in cohesive slopes? J. Rock Mech. Geotech. Eng. 2019, 11, 314–324. [Google Scholar] [CrossRef]
- Clausen, J.; Damkilde, L.; Andersen, L. An efficient return algorithm for non-associated plasticity with linear yield criteria in principal stress space. Comput. Struct. 2007, 85, 1795–1807. [Google Scholar] [CrossRef]
- Mao, J.; Liu, X.; Zhang, C.; Jia, G.; Zhao, L. Runout prediction and deposit characteristics investigation by the distance potential-based discrete element method: The 2018 Baige landslides, Jinsha River, China. Landslides 2021, 18, 235–249. [Google Scholar] [CrossRef]
- Wang, X.; Wang, L.B.; Xu, L.M. Formulation of the return mapping algorithm for elastoplastic soil models. Comput. Geotech. 2004, 31, 315–338. [Google Scholar] [CrossRef]
- Aubertin, M.; Li, L.; Simon, R. A multiaxial stress criterion for short-and long-term strength of isotropic rock media. Int. J. Rock Mech. Min. Sci. 2000, 37, 1169–1193. [Google Scholar] [CrossRef]
- Murrell, S.A.F.; Digby, P.J. The theory of brittle fracture initiation under triaxial stress conditions—II. Geophys. J. Int. 1970, 19, 499–512. [Google Scholar] [CrossRef] [Green Version]
- Atkinson, J.H.; Richardson, D.; Robinson, P.J. Compression and extension of k0 normally consolidated kaolin clay. J. Geotech. Eng. 1987, 113, 1468–1482. [Google Scholar] [CrossRef]
- Li, R.J.; Liu, J.D.; Yan, R.; Zheng, W.; Shao, S.J. Characteristics of structural loess strength and preliminary framework for joint strength formula. Water Sci. Eng. 2014, 7, 319–330. [Google Scholar] [CrossRef]
- Lu, N.; Kim, T.H.; Sture, S.; Likos, W.J. Tensile strength of unsaturated sand. J. Eng. Mech. 2009, 135, 1410–1419. [Google Scholar] [CrossRef] [Green Version]
- Mitachi, T.; Kitago, S. Change in undrained shear strength characteristics of saturated remolded clay due to swelling. Soils Found. 1976, 16, 45–58. [Google Scholar] [CrossRef] [Green Version]
- Vesga, L.F. Direct tensile-shear test (DTS) on unsaturated kaolinite clay. Geotech. Test. J. 2009, 32, 397–409. [Google Scholar] [CrossRef]
- Murcia-Delso, J.; Benson Shing, P. Elastoplastic dilatant interface model for cyclic bond-slip behavior of reinforcing bars. J. Eng. Mech. 2016, 142, 4015082. [Google Scholar] [CrossRef] [Green Version]
- Sutharsan, T.; Muhunthan, B.; Liu, Y. Development and implementation of a constitutive model for unsaturated sands. Int. J. Geomech. 2017, 17, 4017103. [Google Scholar] [CrossRef]
- Chen, X.; Li, D.; Tang, X.; Liu, Y. A three-dimensional large-deformation random finite-element study of landslide runout considering spatially varying soil. Landslides 2021, 18, 3149–3162. [Google Scholar] [CrossRef]
- Zhang, Q.; Shi, Z.; Shan, J.; Shi, W. Secondary Development and Application of Bio-Inspired Isolation System. Sustainability 2019, 11, 278. [Google Scholar] [CrossRef] [Green Version]
- Sabetamal, H.; Salgado, R.; Carter, J.P.; Sheng, D. A two-surface plasticity model for clay; numerical implementation and applications to large deformation coupled problems of geomechanics. Comput. Geotech. 2021, 139, 104405. [Google Scholar] [CrossRef]
- Singh, V.; Stanier, S.; Bienen, B.; Randolph, M.F. Modelling the behaviour of sensitive clays experiencing large deformations using non-local regularisation techniques. Comput. Geotech. 2021, 133, 104025. [Google Scholar] [CrossRef]
- Duncan, J.M. State of the art: Limit equilibrium and finite-element analysis of slopes. J. Geotech. Eng. 1996, 123, 577–596. [Google Scholar] [CrossRef]
- Zheng, Y.R.; Deng, C.J.; Zhao, S.Y.; Tang, X.; Liu, M.; Zhang, L. Development of Finite Element Limiting Analysis Method and Its Applications in Geotechnical Engineering. Eng. Sci. 2007, 3, 10–36. [Google Scholar]
- Augusto Filho, O.; Fernandes, M.A. Landslide analysis of unsaturated soil slopes based on rainfall and matric suction data. Bull. Eng. Geol. Environ. 2019, 78, 4167–4185. [Google Scholar] [CrossRef]
- Guo, Z.; Zhao, Z. Numerical analysis of an expansive subgrade slope subjected to rainfall infiltration. Bull. Eng. Geol. Environ. 2021, 80, 5481–5491. [Google Scholar] [CrossRef]
- Fredlund, D.G.; Xing, A.; Fredlund, M.D.; Barbour, S.L. The relationship of the unsaturated soil shear to the soil-water characteristic curve. Can. Geotech. J. 1996, 33, 440–448. [Google Scholar] [CrossRef]
- Oberg, A.L.; Sallfors, G. Determination of Shear Strength Parameters of Unsaturated Silts and Sands Based on the Water Retention Curve. Geotech. Test. J. 1997, 20, 40–48. [Google Scholar] [CrossRef]
- Genuchten, M.T.V. A closed-form equation for predicting the hydraulic conductivity of unsaturated soils. Soil Sci. Soc. Am. J. 1980, 44, 892–898. [Google Scholar] [CrossRef] [Green Version]
- Mualem, Y. A new model for predicting the hydraulic conductivity of unsaturated porous media. Water Resour. Res. 1976, 12, 513–522. [Google Scholar] [CrossRef] [Green Version]
- Tagarelli, V.; Cotecchia, F. The Effects of Slope Initialization on the Numerical Model Predictions of the Slope-Vegetation-Atmosphere Interaction. Geosciences 2020, 10, 85. [Google Scholar] [CrossRef] [Green Version]


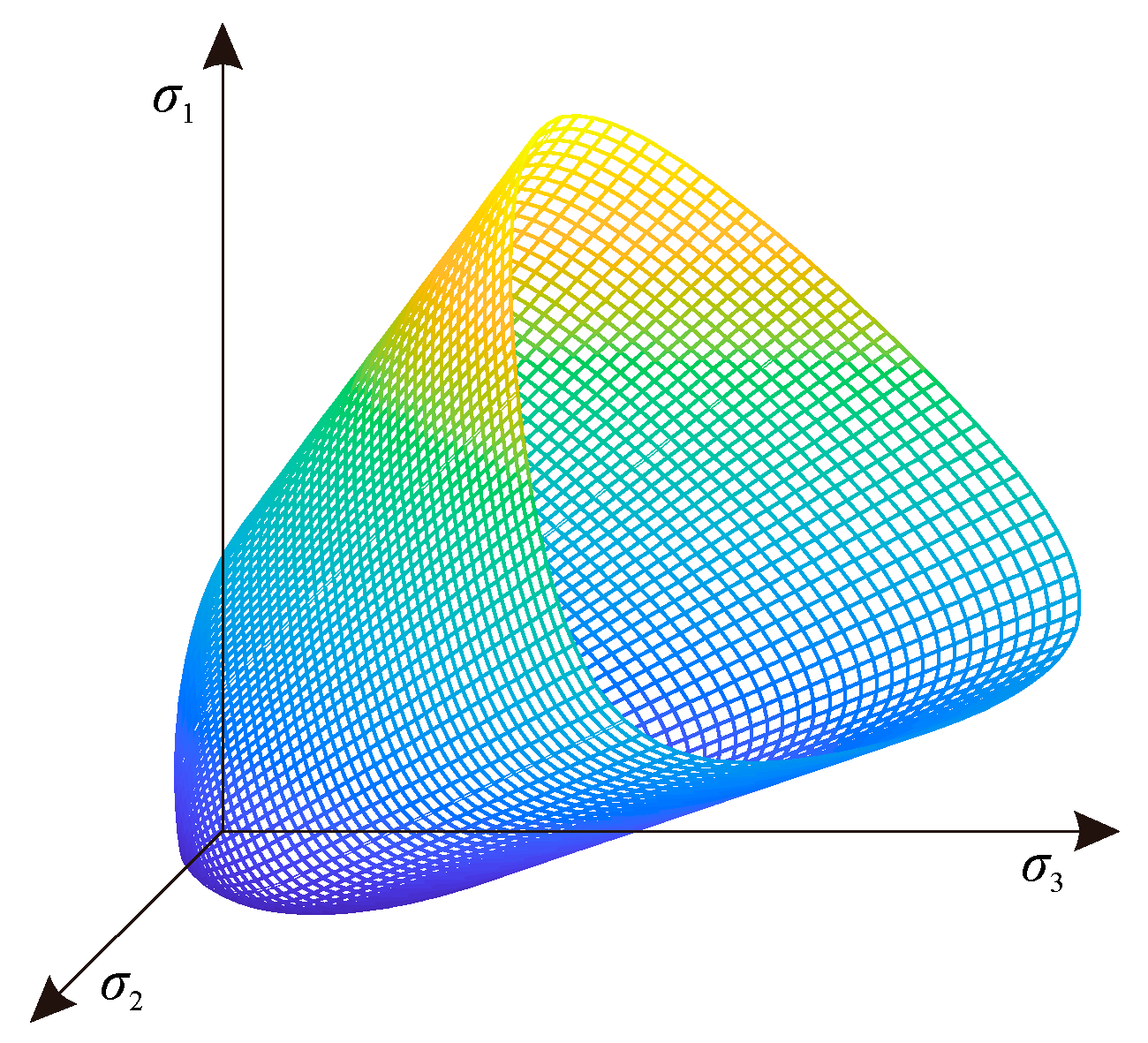
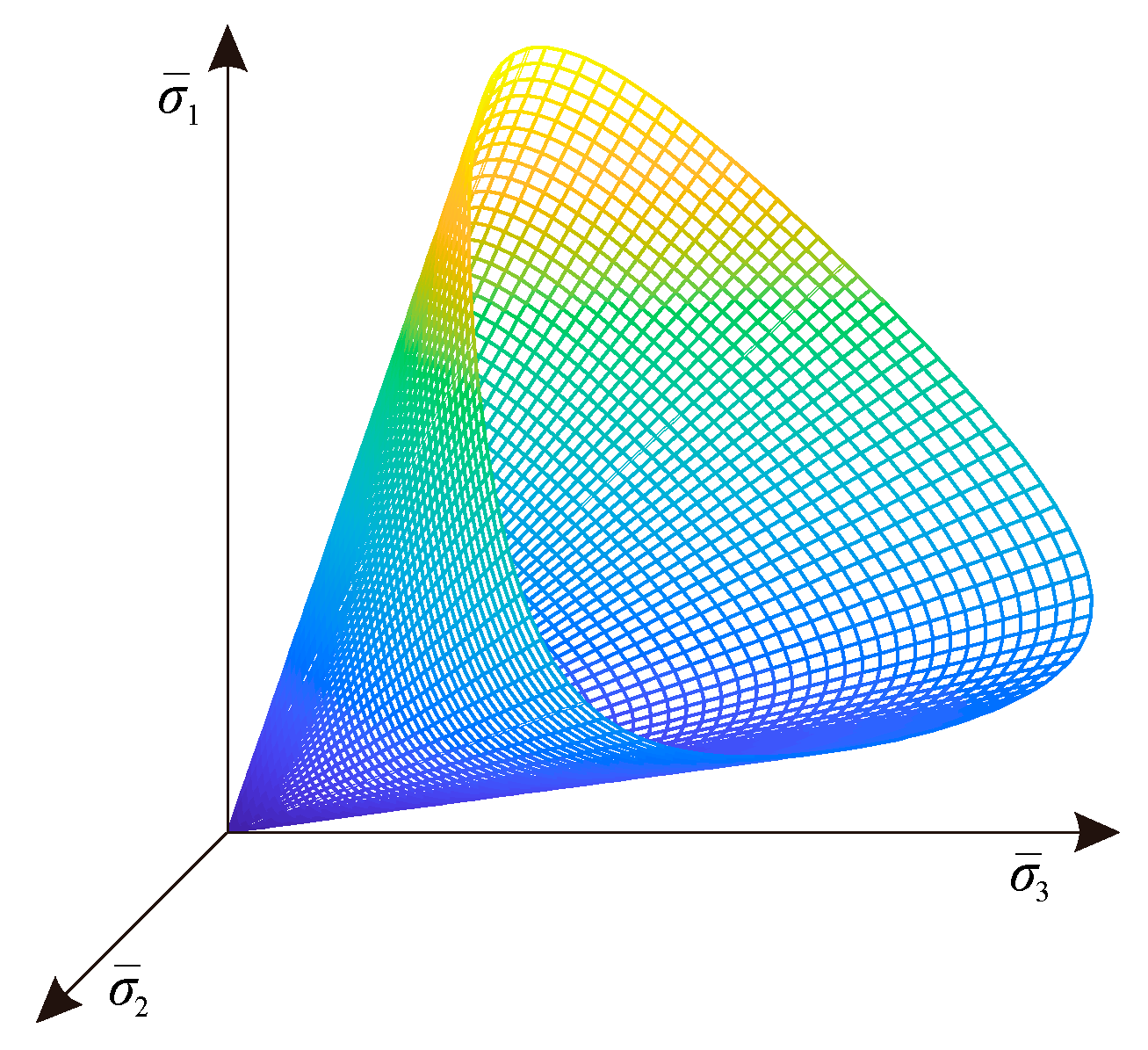





| Elastic Modulus (MPa) | Poisson’s Ratio | Cohesion (MPa) | Friction Angle (°) | Dilatancy Angle (°) |
|---|---|---|---|---|
| 20 | 0.3 | 0.05 | 30 | 30 |
| Elastic Modulus (MPa) | Poisson’s Ratio | Bulk Density (kN/m3) | Cohesion (MPa) | Friction Angle (°) |
|---|---|---|---|---|
| 100 | 0.3 | 20 | 0.042 | 17 |
| Mechanical Parameters | Hydraulic Parameters | |||
|---|---|---|---|---|
| (MPa) | 10 | parameters in Van Genuehten model | (1/m) | |
| 0.3 | ||||
| (kpa) | 15 | saturated permeability coefficient | (m/s) | 2 × 10−6 |
| (°) | 30 | |||
| 1 | ||||
| 2.71 | parameter in relative permeability coefficient | 3 | ||
| (kN/m3) | 14 | |||
| (kN/m3) | 19 | |||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kong, X.; Cai, G.; Cheng, Y.; Zhao, C. Numerical Implementation of Three-Dimensional Nonlinear Strength Model of Soil and Its Application in Slope Stability Analysis. Sustainability 2022, 14, 5127. https://doi.org/10.3390/su14095127
Kong X, Cai G, Cheng Y, Zhao C. Numerical Implementation of Three-Dimensional Nonlinear Strength Model of Soil and Its Application in Slope Stability Analysis. Sustainability. 2022; 14(9):5127. https://doi.org/10.3390/su14095127
Chicago/Turabian StyleKong, Xiaoang, Guoqing Cai, Yongfeng Cheng, and Chenggang Zhao. 2022. "Numerical Implementation of Three-Dimensional Nonlinear Strength Model of Soil and Its Application in Slope Stability Analysis" Sustainability 14, no. 9: 5127. https://doi.org/10.3390/su14095127
APA StyleKong, X., Cai, G., Cheng, Y., & Zhao, C. (2022). Numerical Implementation of Three-Dimensional Nonlinear Strength Model of Soil and Its Application in Slope Stability Analysis. Sustainability, 14(9), 5127. https://doi.org/10.3390/su14095127





