Dynamic Impact of the Perceived Value of Public on Panic Buying Behavior during COVID-19
Abstract
:1. Introduction
2. Theory Overview and Problem Description
2.1. Regret Theory
2.2. Problem Description
3. Evolutionary Game Analysis of Panic Buying Events
3.1. Model Hypothesis
3.2. The Critical Ability of Public
3.3. Model Building
- (1)
- In the germination stage of panic buying events, the game strategy combination is that the public does not participate in panic buying, and the government does not refute the rumors.
- (2)
- In the intensification stage of panic buying events, the game strategy combination is that the public participates in panic buying, and the government does not refute the rumors.
- (3)
- In the mitigation stage of panic buying events, the game strategy combination is that the public participates in panic buying, and the government does not refute the rumors.
- (4)
- In the regression stage of panic buying events, the game strategy combination is that the public participates in panic buying, and the government refutes the rumors.
- (1)
- In the germination stage of panic buying events, information related to the epidemic was released slowly, authoritative information was missing, and the public made their own speculations and had low information judgment literacy. The number of online rumors began to rise slowly and spread easily in a short time. Some potential factors that may induce large-scale panic buying are accumulating. If they are not highly valued or effectively solved, it may lead to further deterioration of the situation. However, in this stage, the spread scope of rumors about potential panic buying items is small, and the critical ability of the public to rumors and perceived value of panic buying items are low. Most of the public will still consume rationally, and they are not prone to panic buying behavior. The normal purchase volume is and the perceived value is . At the same time, if the government chooses to refute rumors, it may not only pay the cost of refuting rumors but also cause unnecessary panic due to premature attention. In this case, the cost to be paid by the government is 0.
- (2)
- In the intensification stage of panic buying events, the public’s critical consciousness began to improve gradually by popularizing the knowledge related to emergencies and emergency management and providing an open and effective platform for information inquiry and exchange. However, there was a sharp rise in the number of online rumors about the epidemic, which triggered the collective panic emotions of the public. The number of panic buying individuals has increased rapidly, the group scale has expanded sharply, and the relationship between supply and demand of panic buying items has been abnormal. In this stage, the spread of rumors gradually expanded, and the public’s trust in rumors and the perceived value of panic buying items increased, which promoted their panic buying willingness and behavior. The public’s panic buying willingness is , the purchase volume is and the perceived value is . At the same time, if the government insists on refuting the rumors, it will increase its own losses. The government has the motivation to choose not to refute the rumors in order to calm the incident on its own.
- (3)
- In the mitigation stage of panic buying events, the number of rumors about the development of the epidemic has decreased significantly due to the stable development of the epidemic, and the rumors are more from information misreading or subjective speculation. The government actively announced the epidemic information through press conferences and mainstream media to satisfy the public’s right to know and answered some rumors through official platforms and experts to minimize the ambiguity of information and curb the breeding of rumors. In this stage, the government actively refuted rumors, which reduced the public’s perceived value of panic buying items, improved their critical ability, and gradually lost their willingness to panic buying. The price of panic buying items rose, but part of the public returned to rationality and gave up participating in panic buying. Its perceived value is . At the same time, if the government does not refute the rumors, the social loss caused by panic buying events will be difficult to control. In this case, the government will pay a certain cost for refuting rumors and will be rewarded by higher government for the refuting rumors work.
- (4)
- In the regression stage of panic buying events, the government continued to release epidemic information openly and transparently, and the epidemic was effectively controlled. Online rumors about the epidemic gradually retreated steadily, and mainstream media effectively guided public opinion and controlled the development pace of public opinion. The public’s comprehensive literacy and ability to distinguish the authenticity of information are improved, the number of individuals involved in panic buying is significantly reduced, and panic buying behavior is basically calmed down. In this stage, the government keeps refuting rumors, and the public has strong critical consciousness. However, they still need to participate in the panic buying to meet their own normal epidemic prevention needs. Therefore, the public will increase the purchased quantity due to the decrease in the price of panic buying items and will still have the panic buying willingness . The purchase quantity of panic buying items is and the perceived value is . At the same time, the cost of refuting rumors caused by the government is , the social loss caused by the government is , and the reward for effectively guiding public opinion received by the higher government is .
3.4. Model Analysis
- (1)
- When , , and , the system has five equilibrium points, including three unstable points, a locally asymptotically stable point, and a saddle point. for a saddle point, namely the strategy combinations (The public does not participate in panic buying. The government does not refute the rumors). The practical significance of this evolutionary stabilization strategy is that the regret value of the public not participating in panic buying is the least, and the benefit is higher than participating in panic buying. This situation appeared mostly in the germination period of panic buying events; the government should strengthen the real-time monitoring of the rumors spreading situation and the public panic buying signs so as to make adequate preparations for timely policy adjustment. The determinant of the equilibrium point, trace, and stability are shown in Table 2.
- (2)
- When , , and , the system has five equilibrium points, including three unstable points, a locally asymptotically stable point, and a saddle point. for a saddle point, namely the strategy combinations (The public participates in panic buying. The government does not refute the rumors). The practical significance of this evolutionary stabilization strategy is that the public will be delighted to panic buy what they need, and the degree of regret is low. This situation appeared mostly during the intensification period of panic buying events; in order to encourage the government to actively perform its duties, the higher government should increase the punishment for its dereliction of duty and urge the government to adjust product production and balance product prices. The determinant of the equilibrium point, trace, and stability are shown in Table 3.
- (3)
- When , , and , the system has five equilibrium points, including three unstable points, a locally asymptotically stable point, and a saddle point. for a saddle point, namely the strategy combinations (The public does not participate in panic buying. The government refutes the rumors). The practical significance of this evolutionary stabilization strategy is that the price of panic buying items rises, and the public will be glad that the loss caused by not participating in panic buying is small. This situation appeared mostly in the mitigation period of panic buying events; the government should pay attention to repeatedly refuting the rumors and improving the intensity of refuting the rumors, strengthening the openness and transparency of information, reducing the public’s trust in rumors, and advocating reasonable public consumption. The determinant of the equilibrium point, trace, and stability are shown in Table 4.
- (4)
- When , , and , the system has five equilibrium points, including three unstable points, a locally asymptotically stable point, and a saddle point. . for a saddle point, namely the strategy combinations (the public participates in panic buying. The government refutes the rumors). The practical significance of this evolutionary stabilization strategy is that the public will be glad that the price of items will fall and the demand will be satisfied. This situation appeared mostly in the regression period of panic buying events; in order to curb the occurrence of panic buying behavior, the government should continue to promote refuting of the rumors, timely notify the market supply of items and the disposal progress of panic buying behavior, and enhance the public’s awareness of loss avoidance. The determinant of the equilibrium point, trace, and stability are shown in Table 5.
- (5)
- When and , this paper substitutes the equilibrium point into the Jacobi matrix, and the Jacobi matrix of this system is as follows:
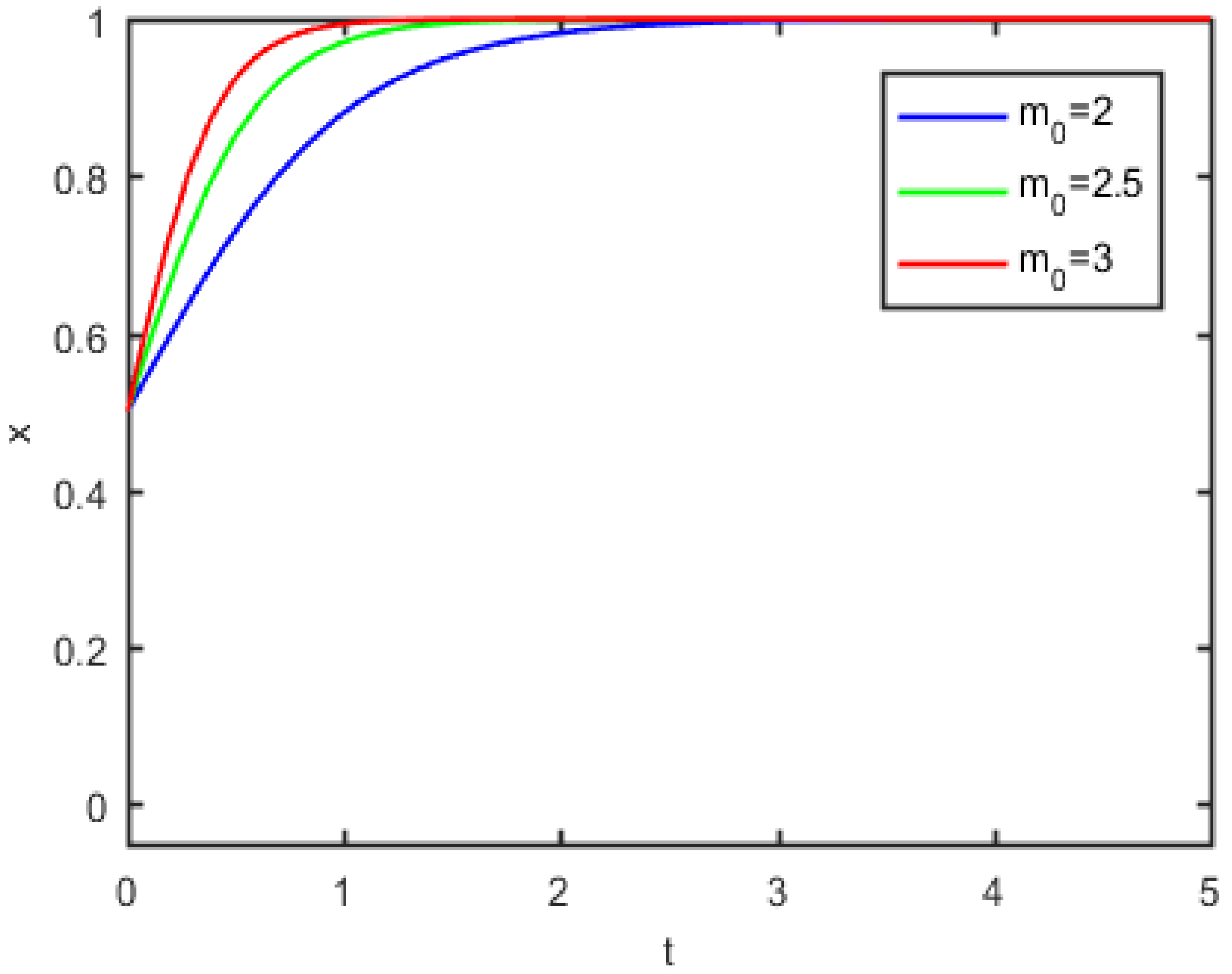
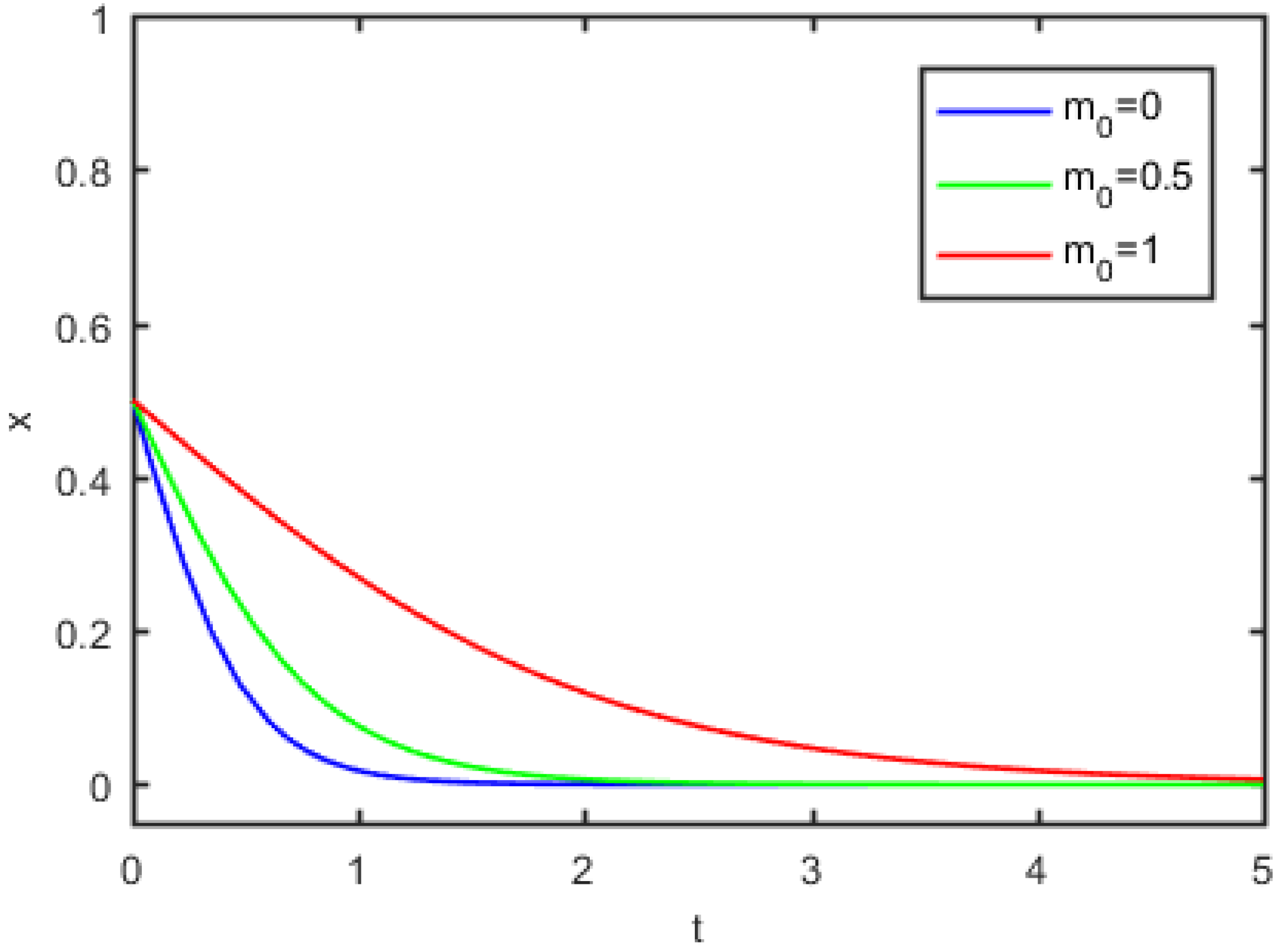
3.5. The Simulation Analysis of Panic Buying Masks Behavior
4. Discussion
5. Conclusions and Limitations
5.1. Conclusions
- (1)
- In order to effectively prevent and control the occurrence of panic buying behavior, the government should strengthen real-time monitoring of the rumors spreading situation and the public panic buying signs in the germination period of the epidemic and make adequate preparations for timely policy adjustment. During the intensification period, the government should actively perform its duties, adjust product production, and balance product prices. In the mitigation period, the government should pay attention to repeatedly refuting the rumors and improving the intensity of refuting the rumors, strengthening the openness and transparency of information, reducing the public’s trust in rumors, and advocating reasonable public consumption. In the regression period, the government should continue to promote refuting the rumors, timely notify the market supply of items and the disposal progress of panic buying behavior, enhance the public’s awareness of loss avoidance, and inhibit the occurrence of panic buying behavior.
- (2)
- In the group panic buying events, if the government does not take the strategy of refuting rumors, the public will have panic and blind obedience due to their poor self-cognition ability and unsatisfied information needs, which will lead to the panic buying events and interfere with the normal social and market order. If the government adopts the strategy of refuting rumors, it will have a significant impact on the volume of panic buying by the public, enhance the public’s critical awareness, and help the public to keep rational and make the right choice. Therefore, the implementation of government strategies will significantly affect the development of group panic buying events. The government should formulate relevant intervention measures at appropriate intervention opportunities to control the impact degree and scope of group buying events.
- (3)
- If the basic epidemic prevention needs of the majority of the public can be guaranteed, the impact scope of rumors spreading will be reduced, and group panic buying events will be more effectively controlled. Therefore, the government should actively meet the public’s demand for epidemic prevention, timely release the price and supply of epidemic prevention products and other information, quickly promote the resumption of work and production of relevant enterprises and effectively ensure the basic needs of the public.
5.2. Contributions
- (1)
- In a theoretical sense, it combines regret theory with an evolutionary game model to study the influence of the public’s perceived value on panic buying behavior, describes the characteristics of each stage of the rumors spreading process and the evolution process of panic buying events, and introduces the variable of public critical ability to measure the public’s panic buying willingness. This study provides a new theoretical perspective on the study of panic buying behavior, which can be considered from the public’s own perspective by considering their regret-joy level to judge their behavioral decisions. In addition, this study extends the application area of regret theory, enriches the study of the factors influencing panic buying behavior, and makes the integration of theory and model more reasonable.
- (2)
- In a practical sense, this study takes the COVID-19 epidemic as a background, and the study of its panic buying behavior will provide a reference for the establishment and effective implementation of the mechanism to guide public opinion on group panic buying behavior under emergencies and will help to further enhance the government’s crisis management capability, strengthen its comprehensive capacity building, actively guide the public to rational consumption, and promote the study of the governance of group panic buying behavior in major public health emergencies.
5.3. Limitations and Future Research
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Ma, X.; Liao, J. Buying to cope with scarcity during public emergencies: A serial mediation model based on cognition-affect theory. Front. Psychol. 2021, 12, 791850. [Google Scholar] [CrossRef]
- Arafat, S.M.Y.; Kar, S.K.; Kabir, R. Editorial: Panic buying: Human psychology and environmental influence. Front. Public Health 2021, 9, 694734. [Google Scholar] [CrossRef] [PubMed]
- Lee, Y.C.; Wu, W.L.; Lee, C.K. How COVID-19 Triggers our herding behavior? risk perception, state anxiety, and trust. Front. Public Health 2021, 9, 587439. [Google Scholar] [CrossRef]
- Arafat, S.Y.; Kar, S.K.; Menon, V.; Kaliamoorthy, C.; Mukherjee, S.; Alradie-Mohamed, A.; Sharma, P.; Marthoenis, M.; Kabir, R. Panic buying: An insight from the content analysis of media reports during COVID-19 pandemic. Neurol. Psychiatry Brain Res. 2020, 37, 100–103. [Google Scholar] [CrossRef] [PubMed]
- Wang, X.F.; Hao, Y.H.; Wu, Q.H. Incidence and influencing factors of public panic buying during epidemic of 2017–2018 seasonal influenza. Chin. J. Public Health 2019, 35, 746–749. [Google Scholar]
- Islam, T.; Pitafi, A.H.; Arya, V.; Wang, Y.; Akhtar, N.; Mubarik, S.; Xiaobei, L. Panic buying in the COVID-19 pandemic: A multi-country examination. J. Retail. Consum. Serv. 2020, 59, 102357. [Google Scholar] [CrossRef]
- Wilk, V.; Mat, R.S.; Jie, F. Supply chain insights from social media users’ responses to panic buying during COVID-19: The herd mentality. Asia Pac. J. Mark. Logist. 2021, ahead-of-print. [CrossRef]
- Motamed, J.; Meshkani, Z.; Mosavi, S.M.; Momenabadi, V.; Ahmadzadeh, M.S. Factors affecting panic buying during COVID-19 outbreak and strategies: A scoping review. Iran. J. Public Health 2021, 50, 2473–2485. [Google Scholar] [CrossRef]
- Lindenmeier, J.; Hodges, H.M.; Saliterer, I. Drivers of consumers’ panic purchase behaviour in the COVID-19 crisis: Validation of an affective and cognitive channel model. J. Mark. Manag. 2021, 37, 17–18. [Google Scholar] [CrossRef]
- Dulam, R.; Furuta, K.; Kanno, T. Consumer panic buying: Realizing its consequences and repercussions on the supply chain. Sustainability 2021, 13, 4370. [Google Scholar] [CrossRef]
- Wang, Z.Y.; Nie, H.F.; Yang, X.L. Evolutionary game analysis of sudden panic buying events considering the perceived value of the public. Chin. J. Manag. Sci. 2020, 28, 71–79. [Google Scholar] [CrossRef]
- Dai, Y.; Ji, W.K.; Jia, W.F. Health pandemic in the era of misinformation: Examining the utility of using victim narrative and social endorsement of user-generated content to reduce panic buying in the US. J. Appl. Commun. Res. 2022, 2043557. [Google Scholar] [CrossRef]
- Niu, J.Y.; Han, Q.Y.; Hao, Y.H.; Ning, L.W.; Liu, Z.; Zhang, Q.; Bi, X.J.; Wu, Q.H. Panic buying and stockpiling of emergency supplies among Chinese citizens during COVID-19 epidemic an online survey. Chin. J. Public Health 2021, 37, 1101–1106. [Google Scholar]
- Zhu, L.H.; Wang, B.X. Stability analysis of a SAIR rumor spreading model with control strategies in online social networks. Inf. Sci. 2020, 526, 1–19. [Google Scholar] [CrossRef]
- Li, Q.Q.; Chen, T.G.; Yang, J.J.; Cong, G.D. Based on computational communication paradigm: Simulation of public opinion communication process of panic buying during the COVID-19 pandemic. Psychol. Res. Behav. Manag. 2020, 13, 1027–1045. [Google Scholar] [CrossRef]
- Wang, W.; Tian, G.; Zhang, T.; Jabarullah, N.H.; Li, F.; Fathollahi-Fard, A.M.; Wang, D.; Li, Z. Scheme selection of design for disassembly (DFD) based on sustainability: A novel hybrid of interval 2-tuple linguistic intuitionistic fuzzy numbers and regret theory. J. Clean. Prod. 2021, 281, 124724. [Google Scholar] [CrossRef]
- Li, P.; Wang, N.; Wei, C.; Zhang, N. A two-sided matching method considering the lowest value of acceptability with regret theory for probabilistic linguistic term sets. Int. J. Mach. Learn. Cybern. 2021, 12, 917–930. [Google Scholar] [CrossRef]
- Ling, G.H.T.; Ho, C.M.C. Effects of the Coronavirus (COVID-19) pandemic on social behaviours: From a social dilemma perspective. Tech. Soc. Sci. J. 2020, 7, 312–320. [Google Scholar] [CrossRef]
- Johnson, T.; Dawes, C.; Fowler, J.; Smirnov, O. Slowing COVID-19 transmission as a social dilemma: Lessons for government officials from interdisciplinary research on cooperation. J. Behav. Public Adm. 2020, 3, 1–13. [Google Scholar] [CrossRef]
- Deng, J.G.; Wu, L.M.; Qi, J.Y.; Xu, X.K. Game analysis of online public opinion dissemination in public health Emergencies. Inf. Sci. 2021, 41, 139–148. [Google Scholar]
- Yan, J.D.; Yang, C.X.; Zhang, W.N. Study on the control and guidance of micro-blog public opinion based on the lifecycle theory. J. Intell. 2017, 36, 75,88–93. [Google Scholar]
- Yin, W.J.; Liu, P. Diffusion paths and mechanisms of mass emergencies in Ethnic Regions. Soc. Sci. Ningxia 2014, 2, 16–20. [Google Scholar]
- Zhuang, Y.M.; Yu, H.L. Research on characteristics of information propagation network of the mass unexpected incident: A case analysis of panic buying of candles. J. Intell. 2013, 32, 37–42. [Google Scholar]
- Chen, Y.S. Study on rumor spreading and public contact in public health emergency. J. Shanghai Norm. Univ. 2020, 49, 100–111. [Google Scholar]
- Song, Q.H.; Chen, J.H. Study on rumor propagation and intervention considering rumor refutation of public and feedback mechanism. China Saf. Sci. J. 2017, 27, 13–17. [Google Scholar]
- Daniel, F. On economic applications of evolutionary game theory. J. Evol. Econ. 1998, 8, 15–43. [Google Scholar]
- Li, J.; Zhang, R.; Xu, Y. Evolutionary game between E-commerce platform supervision and merchants selling fakes. J. Syst. Eng. 2018, 33, 649–661. [Google Scholar]
- Xu, H.; Tan, D.Q.; Zhang, J.Q.; Han, W. Evolutionary game analysison herding behavior of non-direct stakeholders in mass emergencies. Manag. Rev. 2019, 31, 254–266. [Google Scholar]
- Li, X.; Zhou, Y.S.; Wong, Y.D.; Wang, X.Q.; Yuen, K.F. What influences panic buying behaviour? A model based on dual-system theory and stimulus-organism-response framework. Int. J. Disaster Risk Reduct. 2021, 64, 102484. [Google Scholar] [CrossRef]

| Strategy Choice | Public | ||
|---|---|---|---|
| Government | ) | ) | ) |
| ) | ) | ) | |
| Equilibrium Point | Local Stability | ||
|---|---|---|---|
| ESS | |||
| Unstable | |||
| Unstable | |||
| Unstable | |||
| Saddle point |
| Equilibrium Point | Local Stability | ||
|---|---|---|---|
| Unstable | |||
| ESS | |||
| Unstable | |||
| Unstable | |||
| Saddle point |
| Equilibrium Point | Local Stability | ||
|---|---|---|---|
| Unstable | |||
| Unstable | |||
| ESS | |||
| Unstable | |||
| Saddle point |
| Equilibrium Point | Local Stability | ||
|---|---|---|---|
| Unstable | |||
| Unstable | |||
| Unstable | |||
| ESS | |||
| Saddle point |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mao, Q.-H.; Hou, J.-X.; Xie, P.-Z. Dynamic Impact of the Perceived Value of Public on Panic Buying Behavior during COVID-19. Sustainability 2022, 14, 4874. https://doi.org/10.3390/su14094874
Mao Q-H, Hou J-X, Xie P-Z. Dynamic Impact of the Perceived Value of Public on Panic Buying Behavior during COVID-19. Sustainability. 2022; 14(9):4874. https://doi.org/10.3390/su14094874
Chicago/Turabian StyleMao, Qing-Hua, Jiang-Xiao Hou, and Peng-Zhen Xie. 2022. "Dynamic Impact of the Perceived Value of Public on Panic Buying Behavior during COVID-19" Sustainability 14, no. 9: 4874. https://doi.org/10.3390/su14094874
APA StyleMao, Q.-H., Hou, J.-X., & Xie, P.-Z. (2022). Dynamic Impact of the Perceived Value of Public on Panic Buying Behavior during COVID-19. Sustainability, 14(9), 4874. https://doi.org/10.3390/su14094874








