Experimental and Theoretical Study on Mechanical Performance of a Sustainable Method to Simultaneously Generate Power and Fresh Water
Abstract
1. Introduction
2. Simultaneous Power and Water Generation
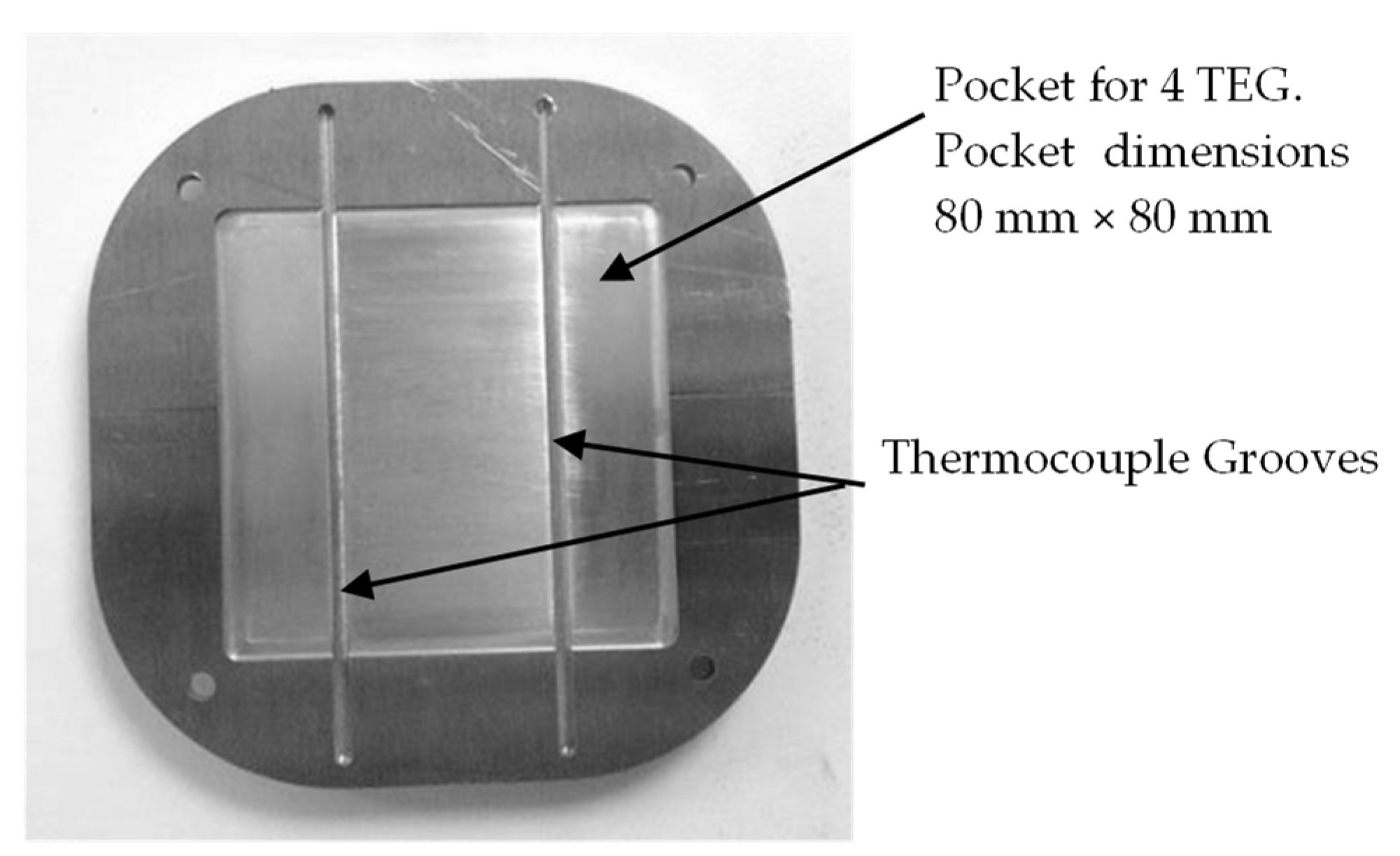
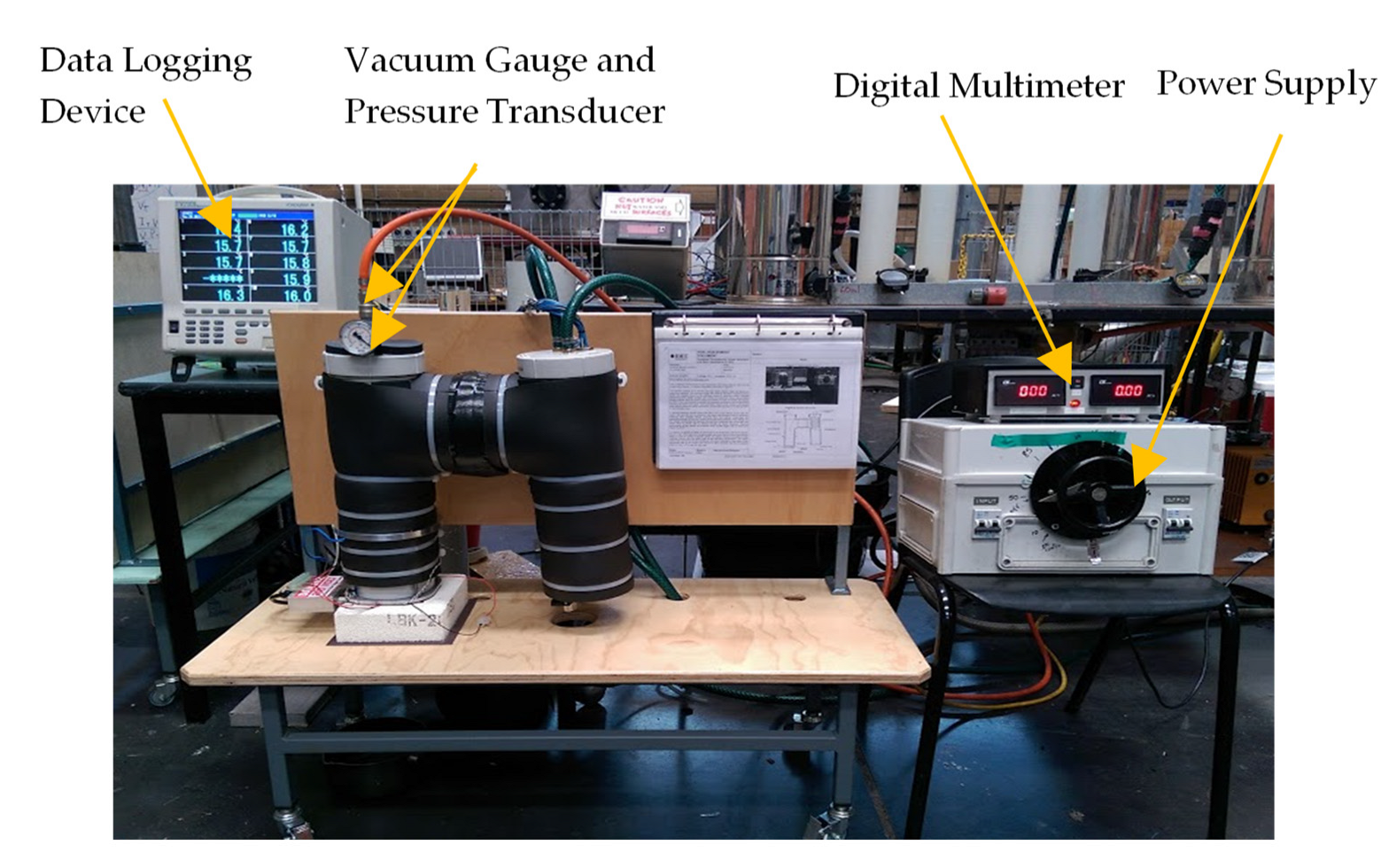
3. Experimental Set-Up and Data Acquisition System
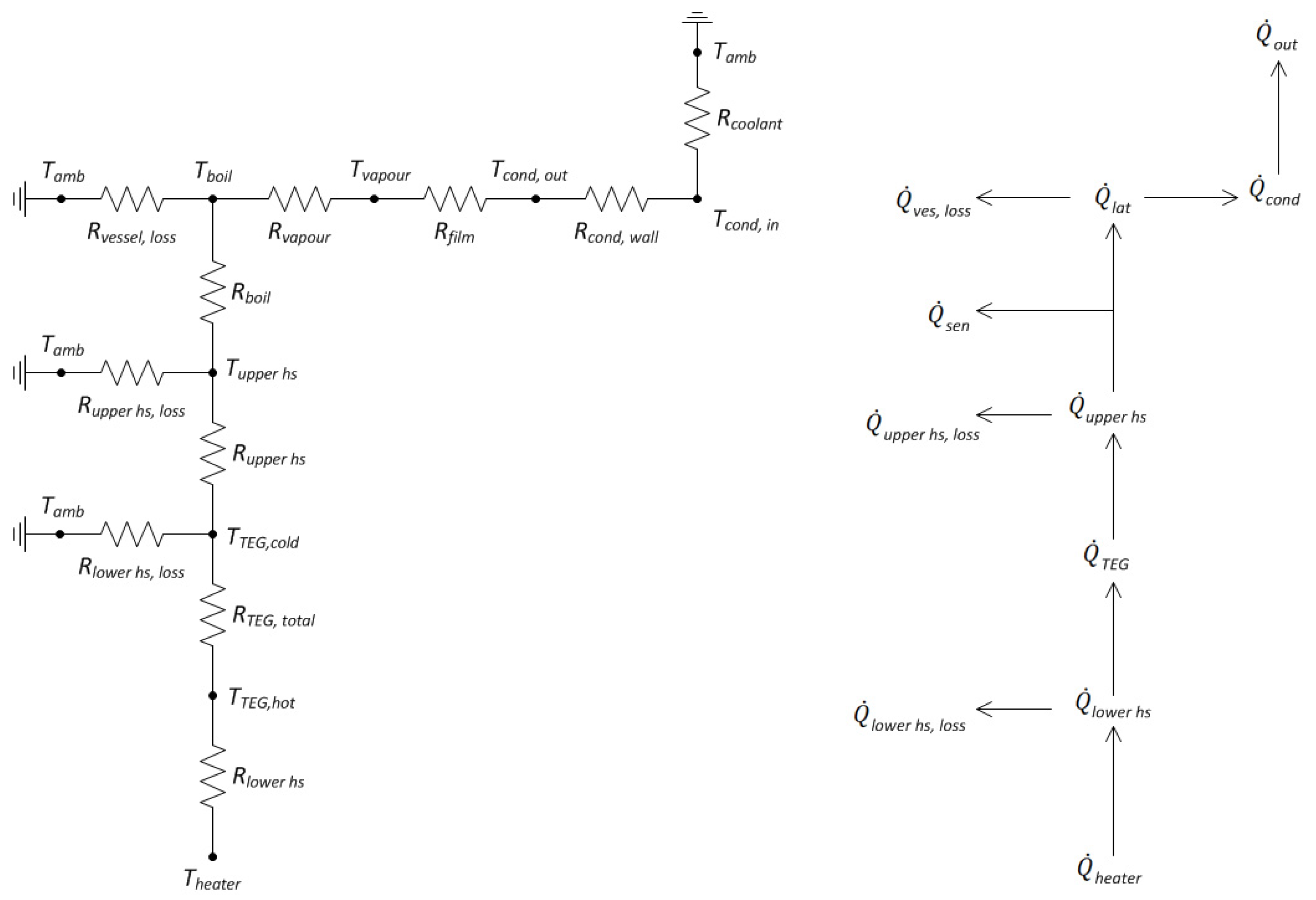
4. Energy Balance Modelling of the Proposed System
4.1. Heating of Saline Water and Vapour Generation
4.2. Fresh Water Production
4.3. Heat Loss
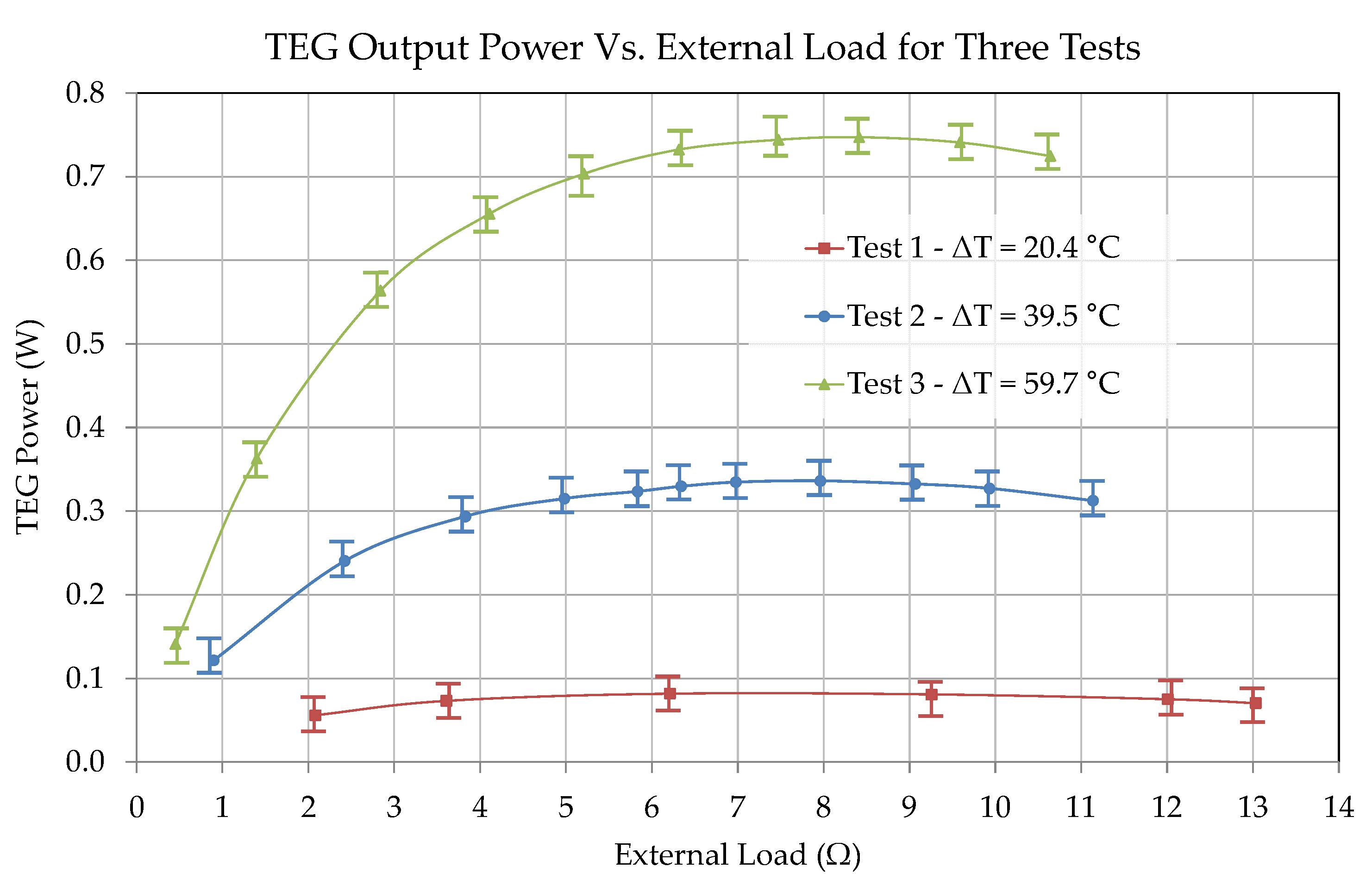
4.4. TEG Power Output
5. Experimental Procedure
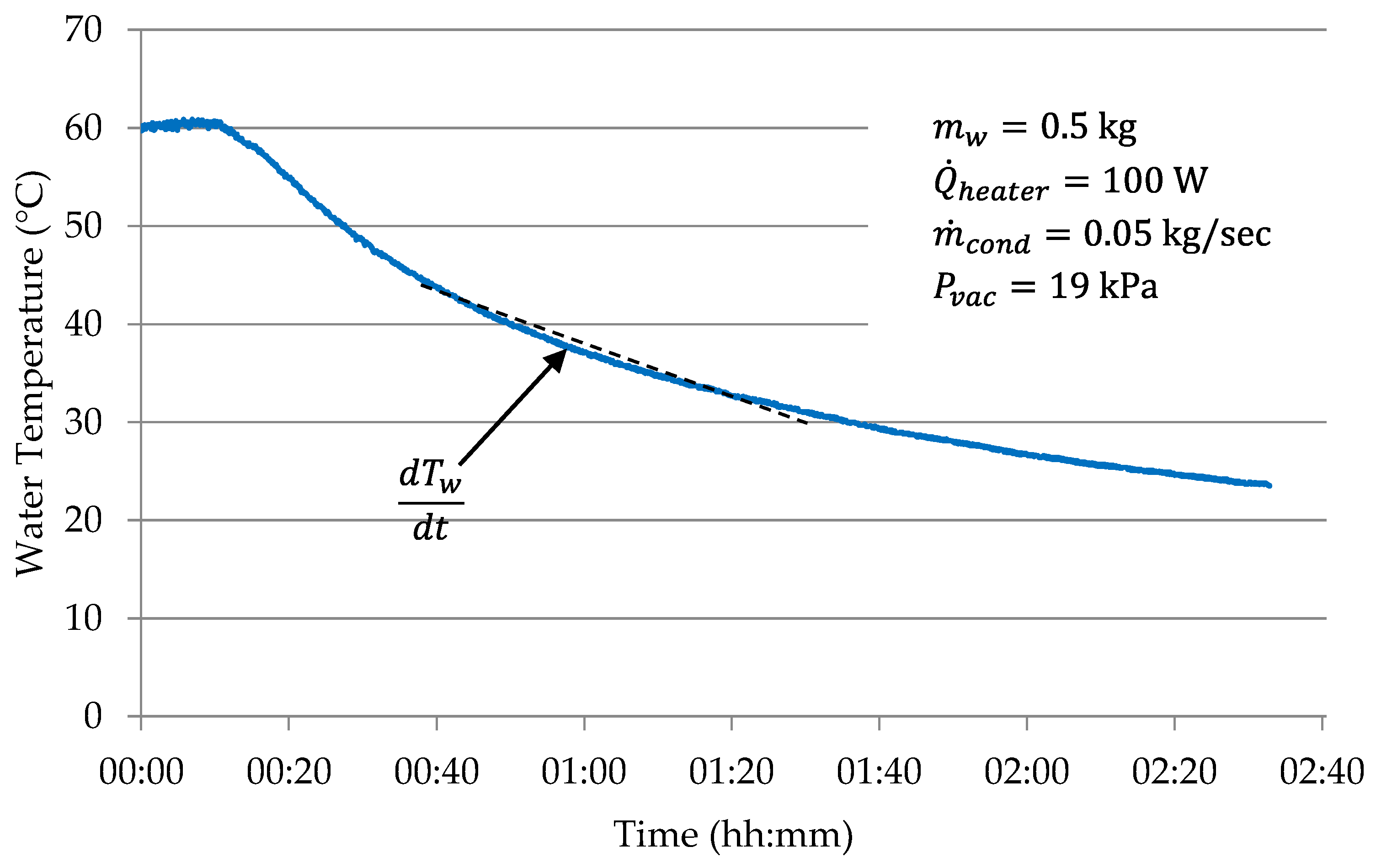
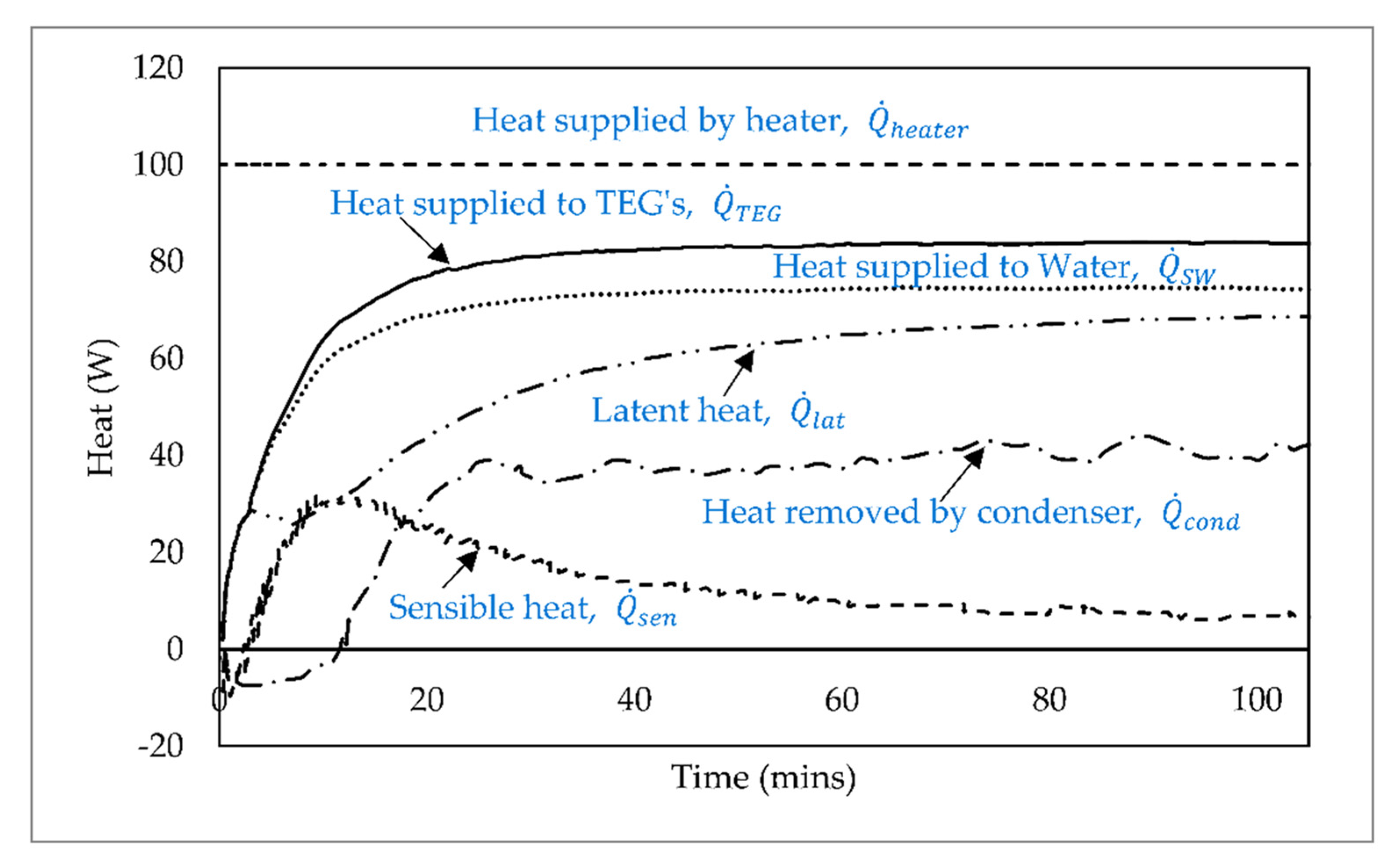
6. Results and Discussion
7. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- van Vliet, M.T.; Jones, E.R.; Flörke, M.; Franssen, W.H.; Hanasaki, N.; Wada, Y.; Yearsley, J.R. Global water scarcity including surface water quality and expansions of clean water technologies. Environ. Res. Lett. 2021, 16, 24020. [Google Scholar] [CrossRef]
- Turiel, A. The Energy Crisis in the World Today: Analysis of the World Energy Outlook 2021; International Energy Agency: Paris, France, 2022; Volume 1, pp. 1–21. [Google Scholar]
- IEA. World Energy Outlook 2021; OECD Publishing: Paris, France, 2021; pp. 1–386. [Google Scholar]
- Karagiannis, I.C.; Soldatos, P.G. Water desalination cost literature: Review and assessment. Desalination 2008, 223, 448–456. [Google Scholar] [CrossRef]
- Elimelech, M.; Phillip, W.A. The future of seawater desalination: Energy, technology, and the environment. Science 2011, 333, 712–717. [Google Scholar] [CrossRef] [PubMed]
- Lior, N. Advances in Water Desalination; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2013; pp. 1–712. [Google Scholar]
- Khawaji, A.D.; Kutubkhanah, I.K.; Wie, J.-M. Advances in seawater desalination technologies. Desalination 2008, 221, 47–69. [Google Scholar] [CrossRef]
- Stijepovic, M.Z.; Linke, P. Optimal waste heat recovery and reuse in industrial zones. Energy 2011, 36, 4019–4031. [Google Scholar]
- Brito, F.P.; Peixoto, J.S.; Martins, J.; Gonçalves, A.P.; Louca, L.; Vlachos, N.; Kyratsi, T. Analysis and Design of a Silicide-Tetrahedrite Thermoelectric Generator Concept Suitable for Large-Scale Industrial Waste Heat Recovery. Energies 2021, 14, 5655. [Google Scholar] [CrossRef]
- Khayyam, H. Automation, Control and Energy Efficiency in Complex Systems; MDPI Books: Basel, Switzerland, 2020. [Google Scholar]
- Gude, V.G. Energy storage for desalination processes powered by renewable energy and waste heat sources. Appl. Energy 2015, 137, 877–898. [Google Scholar] [CrossRef]
- Duke, M. Industrial waste heat powers desalination. Membr. Technol. 2012, 5, 9. [Google Scholar] [CrossRef]
- Asgharnejad, H.; Khorshidi Nazloo, E.; Madani Larijani, M.; Hajinajaf, N.; Rashidi, H. Comprehensive review of water management and wastewater treatment in food processing industries in the framework of water-food-environment nexus. Compr. Rev. Food Sci. Food Saf. 2021, 20, 4779–4815. [Google Scholar] [CrossRef]
- Bell, L.E. Cooling, heating, generating power, and recovering waste heat with thermoelectric systems. Science 2008, 321, 1457–1461. [Google Scholar] [CrossRef] [PubMed]
- Jaziri, N.; Boughamoura, A.; Müller, J.; Mezghani, B.; Tounsi, F.; Ismail, M. A comprehensive review of Thermoelectric Generators: Technologies and common applications. Energy Rep. 2020, 6, 264–287. [Google Scholar] [CrossRef]
- Bhathal Singh, B.S. Power Generation from Solar Pond Using Thermoelectric Generators. Ph.D. Thesis, RMIT University, Melbourne, Australia, 21 June 2015. [Google Scholar]
- Gude, V.G.; Nirmalakhandan, N. Desalination at low temperatures and low pressures. Desalination 2009, 244, 239–247. [Google Scholar] [CrossRef]
- Kumar, R.S.; Mani, A.; Kumaraswamy, S. Analysis of a jet-pump-assisted vacuum desalination system using power plant waste heat. Desalination 2005, 179, 345–354. [Google Scholar] [CrossRef]
- Tay, J.H.; Low, S.C.; Jeyaseelan, S. Vacuum desalination for water purification using waste heat. Desalination 1996, 106, 131–135. [Google Scholar] [CrossRef]
- Moharram, N.A.; Bayoumi, S.; Hanafy, A.A.; El-Maghlany, W.M. Hybrid desalination and power generation plant utilizing multi-stage flash and reverse osmosis driven by parabolic trough collectors. Case Stud. Therm. Eng. 2021, 23, 100807. [Google Scholar] [CrossRef]
- Ghaffar, A.; Imran, Q.; Hassan, M.; Usman, M.; Khan, M.U. Simultaneous solar water desalination and energy generation by high efficient graphene oxide-melanin photothermal membrane. J. Environ. Chem. Eng. 2022, 10, 108424. [Google Scholar] [CrossRef]
- Ng, K.C.; Burhan, M.; Chen, Q.; Ybyraiymkul, D.; Akhtar, F.H.; Kumja, M.; Shahzad, M.W. A thermodynamic platform for evaluating the energy efficiency of combined power generation and desalination plants. Npj Clean Water 2021, 4, 25. [Google Scholar] [CrossRef]
- Date, A.; Gauci, L.; Chan, R.; Date, A. Performance review of a novel combined thermoelectric power generation and water desalination system. Renew. Energy 2015, 83, 256–269. [Google Scholar] [CrossRef]
- Berenson, P.J. Experiments on pool-boiling heat transfer. Int. J. Heat Mass Transf. 1962, 5, 985–999. [Google Scholar] [CrossRef]
- Date, A.; Date, A.; Dixon, C.; Akbarzadeh, A. Theoretical and experimental study on heat pipe cooled thermoelectric generators with water heating using concentrated solar thermal energy. Sol. Energy 2014, 105, 656–668. [Google Scholar] [CrossRef]
- Robertson, C.R. Further Electrical and Electronic Principles, 3rd ed.; Taylor & Francis Group: Hoboken, NJ, USA, 2010; pp. 1–280. [Google Scholar]
- Wisniak, J. Historical development of the vapor pressure equation from dalton to antoine. J. Phase Equilibria 2001, 22, 622–630. [Google Scholar] [CrossRef]
- Çengel, Y.A.; Ghajar, A.J.; Kanoglu, M. Heat and Mass Transfer Fundamentals and Applications, 4th ed.; McGraw-Hill Education: New York, NY, USA, 2011; pp. 1–924. [Google Scholar]
- Zajaczkowski, B.; Halon, T.; Krolicki, Z. Experimental verification of heat transfer coefficient for nucleate boiling at sub-atmospheric pressure and small heat fluxes. Heat Mass Transf. 2016, 52, 205–215. [Google Scholar] [CrossRef]
- Rowe, D.M.; Min, G. Evaluation of thermoelectric modules for power generation. J. Power Sources 1998, 73, 193–198. [Google Scholar] [CrossRef]
- Faraji, A.; Akbarzadeh, A. Design of a Compact, Portable Test System for Thermoelectric Power Generator Modules. J. Electron. Mater. 2013, 42, 1535–1541. [Google Scholar] [CrossRef]
- Carmo, J.P.; Antunes, J.; Silva, M.F.; Ribeiro, J.F.; Goncalves, L.M.; Correia, J.H. Characterization of thermoelectric generators by measuring the load-dependence behavior. Measurement 2011, 44, 2194–2199. [Google Scholar] [CrossRef]



| Device/Sensor | Measured Quantity | Potential Error |
|---|---|---|
| Digital Display Module | Alternating Voltage/Current | ±0.1% of reading |
| Data Logger | Temperature | ±0.15% of reading +0.5 °C |
| Type-T Thermocouple | Temperature | Greater of 1.0 °C or 0.75% |
| Data Logger | DC Voltage | ±0.1% of reading + 2 digits |
| Pressure Sensor | Pressure | ±0.15% Typical, ±0.25% maximum best straight line (BSL) definition |
| 25 | 0.28 |
| 30 | 0.54 |
| 40 | 0.64 |
| 50 | 0.65 |
| Parameter | Test 1 | Test 2 | Test 3 |
|---|---|---|---|
| Heat power, (W) | 50 | 100 | 150 |
| Test duration, (min) | 118 | 116 | 120 |
| Condenser flow rate, (L/s) | 0.05 | 0.05 | 0.05 |
| Vacuum pressure, (kPa) | 5.4 | 5.6 | 5.8 |
| Theoretical freshwater output, (g) | 77.8 | 185.5 | 297.5 |
| Experimental freshwater output, (g) | 61.5 | 173.3 | 303.4 |
| Experimental freshwater mass flux, (kg/m2·h) | 4.8 | 13.5 | 23.7 |
| Experimental specific thermal energy consumption (MJ/kg) | 5.76 | 4.01 | 3.6 |
| Salinity of the feed water (ppm) | 9000 to 10,000 | ||
| Salinity of condensate—freshwater (ppm) | 5 to 10 | ||
| Parameter | Test 1 | Test 2 | Test 3 |
|---|---|---|---|
| Latent heating, (W) | 29 | 68 | 102 |
| Condenser heat removal, (W) | 10 | 35 | 95 |
| Conversion efficiency, (%) | 34 | 51 | 93 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Date, A.; Traisak, O.; Ward, M.; Rupakheti, E.; Hu, E.; Khayyam, H. Experimental and Theoretical Study on Mechanical Performance of a Sustainable Method to Simultaneously Generate Power and Fresh Water. Sustainability 2022, 14, 14039. https://doi.org/10.3390/su142114039
Date A, Traisak O, Ward M, Rupakheti E, Hu E, Khayyam H. Experimental and Theoretical Study on Mechanical Performance of a Sustainable Method to Simultaneously Generate Power and Fresh Water. Sustainability. 2022; 14(21):14039. https://doi.org/10.3390/su142114039
Chicago/Turabian StyleDate, Abhijit, Oranit Traisak, Matthew Ward, Eliza Rupakheti, Eric Hu, and Hamid Khayyam. 2022. "Experimental and Theoretical Study on Mechanical Performance of a Sustainable Method to Simultaneously Generate Power and Fresh Water" Sustainability 14, no. 21: 14039. https://doi.org/10.3390/su142114039
APA StyleDate, A., Traisak, O., Ward, M., Rupakheti, E., Hu, E., & Khayyam, H. (2022). Experimental and Theoretical Study on Mechanical Performance of a Sustainable Method to Simultaneously Generate Power and Fresh Water. Sustainability, 14(21), 14039. https://doi.org/10.3390/su142114039










