Study of Transformer Harmonic Loss Characteristic in Distribution Network Based on Field-Circuit Coupling Method
Abstract
:1. Introduction
2. Transformer Loss Model
2.1. Transformer Core Harmonic Loss
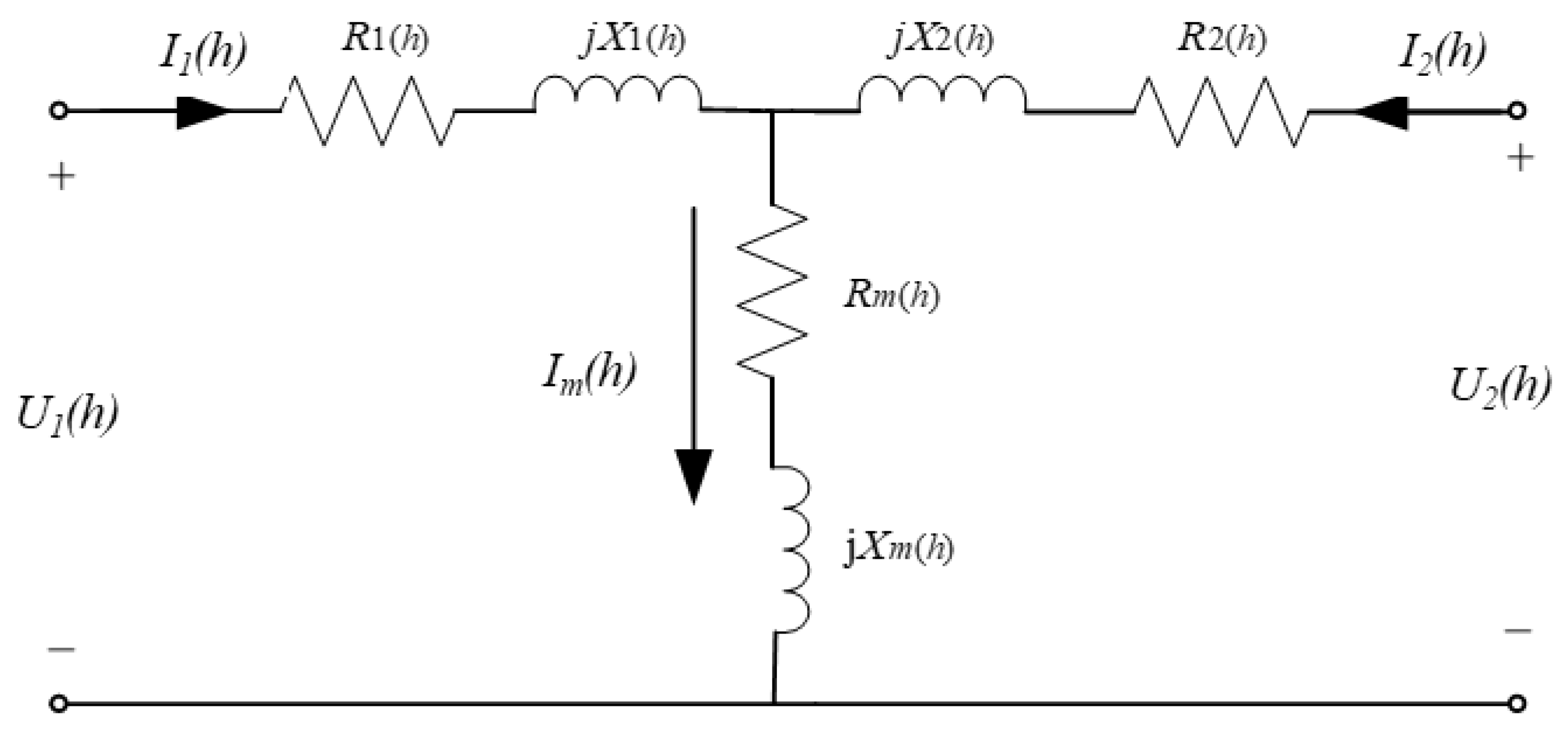
2.2. The Winding Harmonic Loss
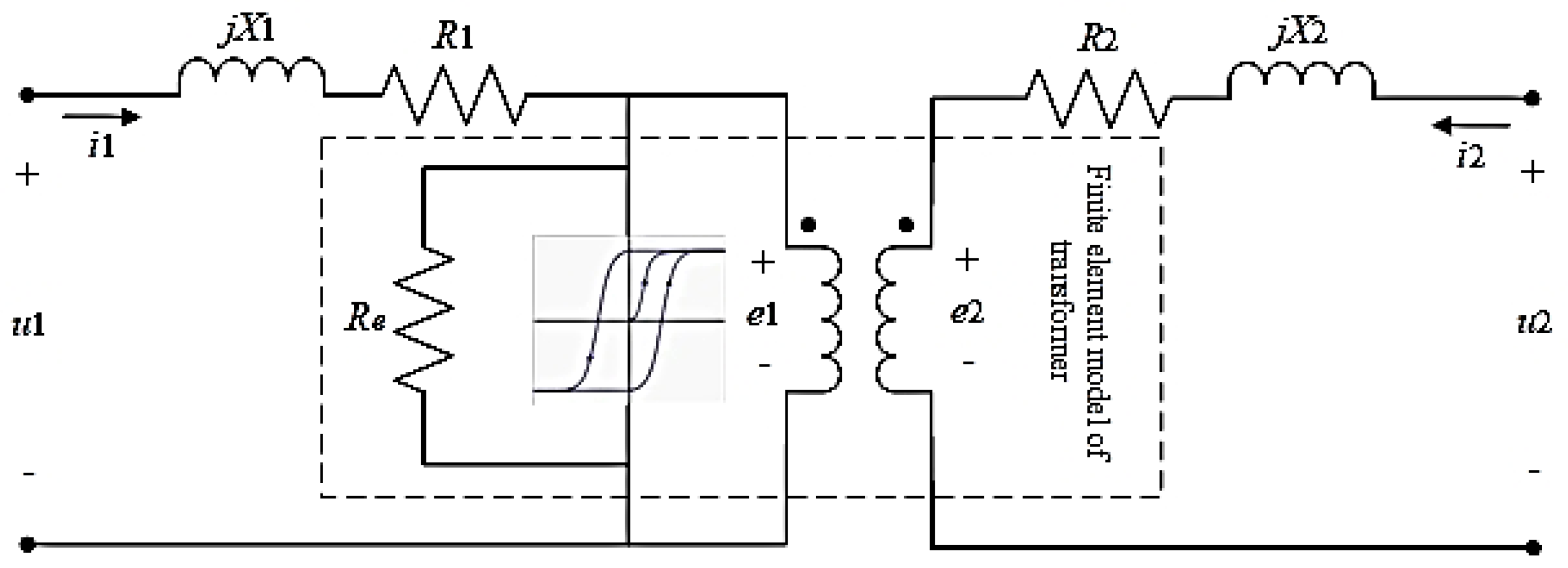
3. The Field-Circuit Coupling Model
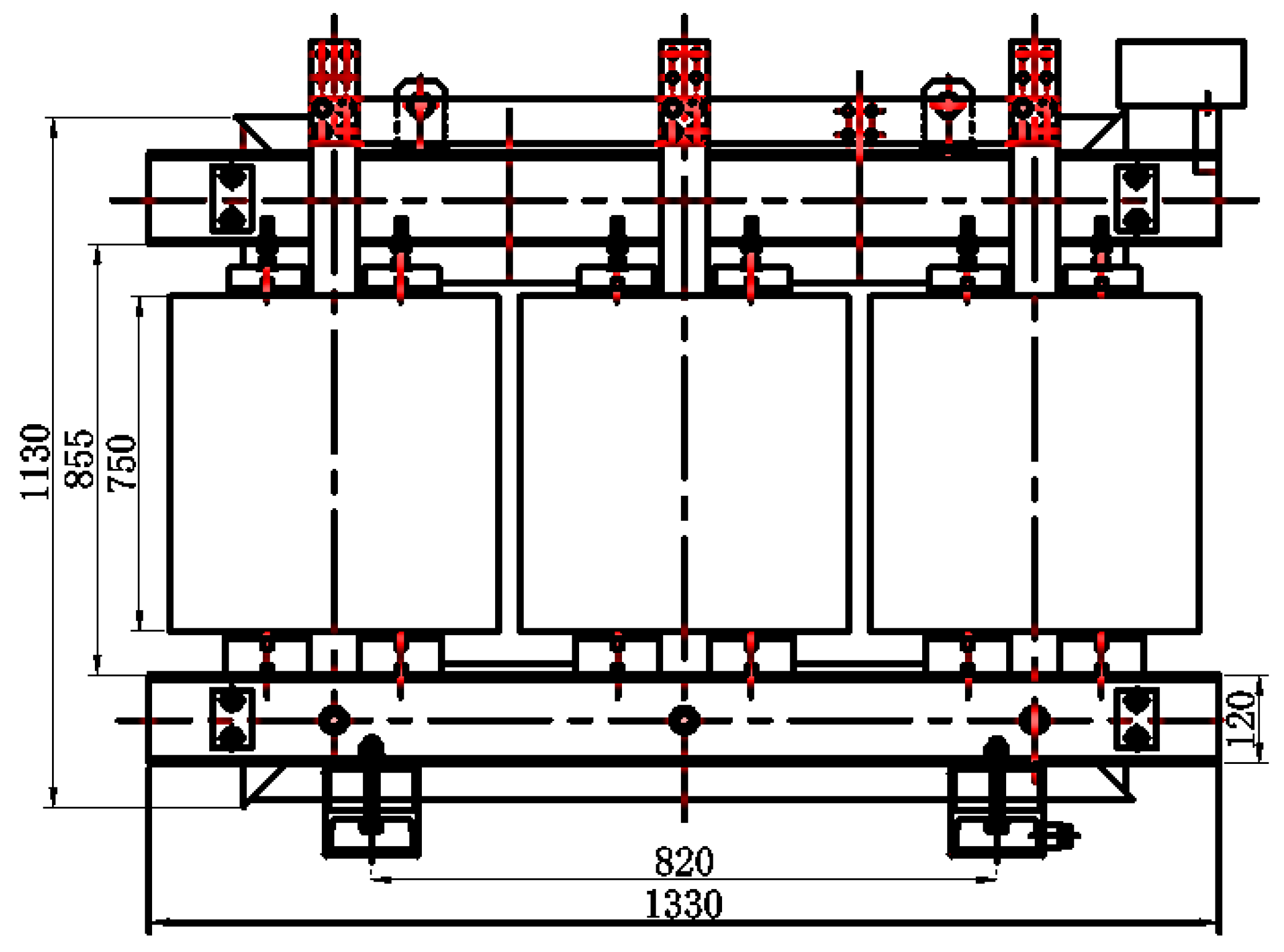
4. Simulation and Discussion
4.1. Transformer No-Load Loss Analysis
4.2. Transformer Load Loss Analysis
5. Conclusions
- (1)
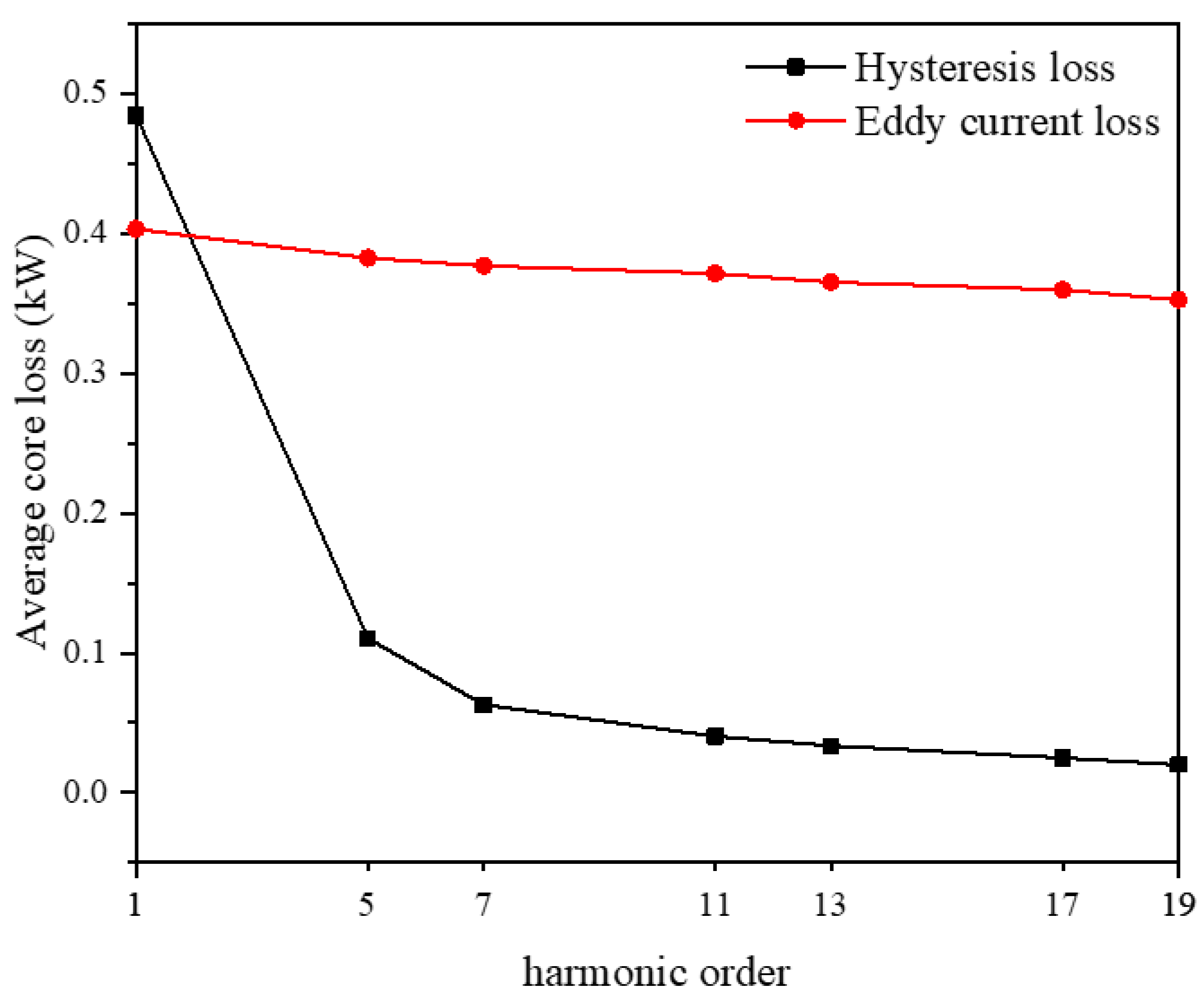
- As the voltage frequency increases, the hysteresis loss of the core of the three-phase coherent distribution trans-former decreases. As a result, when eliminating the line harmonic voltage in the transformer’s long-term no-load or light-load distribution line, the low harmonic voltage should be prioritized.
- (2)
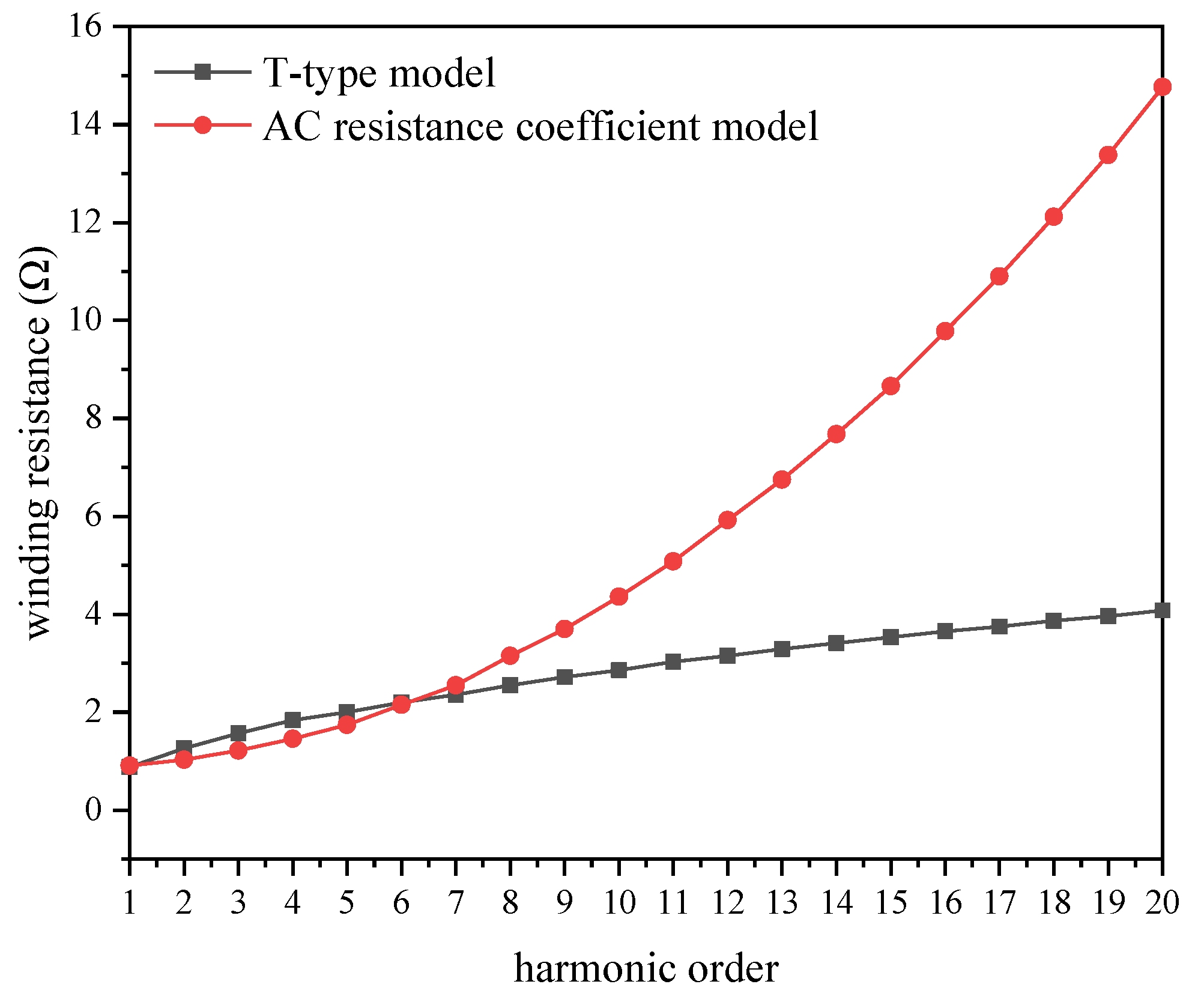
- By the finite element method of field-path coupling, the AC resistance coefficient model is more accurate than the conventional harmonic winding resistance model Rdc when calculating the high harmonic resistance of the winding of a three-phase dry-type distribution transformer due to skin and proximity effects. By using the AC resistance factor model, the accuracy of the calculation of high harmonic losses in transformer windings can be significantly improved. The errors in the 7th, 11th, 13th, 17th and 19th harmonic losses calculated using the AC resistance coefficient model are reduced by 31%, 87%, 91%, 61% and 89% respectively compared to the Rdc model.
- (3)
- Harmonic additional winding losses increase as harmonic currents increase. The more harmonic currents there are, the greater the harmonic additional losses. The extra losses are most noticeable at higher harmonic currents. Higher harmonic currents should be monitored more closely in distribution lines where transformers are heavily loaded or overloaded in order to reduce harmonic load losses in the transformer.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Chiu, H.-C.; Pao, H.-K.; Hsieh, R.-H.; Chiu, Y.-J.; Jang, J.-H. Estimation of the eddy current losses in a dry-type 3000 KVA transformer with machine learning. Energy Rep. 2020, 6, 447–451. [Google Scholar] [CrossRef]
- Attar, M.; Tezcan, S.S. Design and Cost Analysis of Two Different Types of 400 kVA Distribution Transformers. In Proceedings of the 13th International Conference on Electronics, Computers and Artificial Intelligence, ECAI 2021, Pitesti, Romania, 1–3 July 2021. [Google Scholar] [CrossRef]
- Liu, C.; Yang, R. Calculation and analysis of transformer’s harmonic loss. Power Syst. Prot. Control 2008, 36, 33–36. [Google Scholar]
- Qionglin, L.; Shuming, L.; Jiajing, W. Calculation model of 10 kV distribution network loss considering power quality impact and its experimental verification. Electr. Power Autom. Equip. 2022, 42, 212–220. [Google Scholar]
- Fang, S.L.; Li, J.H.; Huang, Y.; Qiu, W.; Sang, H.S. Research and Evaluation of the Transformer Harmonic Model. Proc. CSU-EPSA 2013, 25, 103–108. [Google Scholar]
- Zhang, Z.L.; Wang, K.; Li, D.W.; Zhou, J.; Wu, X.H.; Huang, S.; Tang, J. Transformer harmonic loss calculation and influence factor analysis. Power Syst. Prot. Control. 2011, 39, 68–78. [Google Scholar]
- IEEE. IEEE Recommended Practice for Establishing Liquid-Immersed and Dry-Type Power and Distribution Transformer Capability When Supplying Nonsinusoidal Load Currents: IEEE Std C57.110™-2018; Revision of IEEE Std C57.110-2008; IEEE: New York, NY, USA, 2018; pp. 1–68. [Google Scholar]
- Hurley, W.; Gath, E.; Breslin, J. Optimizing the AC resistance of multilayer transformer windings with arbitrary current waveforms. IEEE Trans. Power Electron. 2000, 15, 369–376. [Google Scholar] [CrossRef] [Green Version]
- Thango, B.A.; Jordaan, J.A.; Nnachi, A.F. Analysis of stray losses in transformers using finite element method modelling. In Proceedings of the 2021 IEEE PES/IAS PowerAfrica, PowerAfrica 2021, Nairobi, Kenya, 23–27 August 2021. [Google Scholar] [CrossRef]
- Qi, H.; Rong, X.Z.; Guo, X.Z. Influence of Harmonic Current on Load Loss of 27.5kV Dry Type Transformer. Transformer 2021, 58, 36–41. [Google Scholar]
- Wensi, W. Research on Parameters Distortion Characteristics and Loss of Transformer under Harmonic Background. Master’s Thesis, North China Electric Power University, Beijing, China, 2019. [Google Scholar]
- Chen, C.; Xin, L.; Xin, J. Research on Multiphysics Simulation of Transformer Winding Vibration Based on Finite Element Method. Transformer 2018, 55, 21–26. [Google Scholar]
- Le, W. Study on the Core Magnetic Loss of Harmonic Transformer based on Steinmetz Model. Master’s Thesis, Heibei University of Technology, Tianjin, China, 2020. [Google Scholar]
- Li, Y.; Li, Y.; Luo, L.; Rehtanz, C.; Wang, C.; Ruberg, S. Simulation of the Electromagnetic Response Characteristic of an Inductively Filtered HVDC Converter Transformer Using Field-Circuit Coupling. IEEE Trans. Ind. Electron. 2012, 59, 4020–4031. [Google Scholar] [CrossRef]
- Qiu, H.; Zhao, X.; Yang, C.; Yi, R.; Wei, Y. Influence of open-circuit fault on electromagnetic field of high-speed permanent magnet generator with Gramme ring windings. IET Electr. Power Appl. 2020, 14, 220–225. [Google Scholar] [CrossRef]
- Xu, J.; Gu, X.; Liang, C.; Bai, Z.; Kubis, A. Harmonic suppression analysis of a harmonic filtering distribution transformer with integrated inductors based on field–circuit coupling simulation. IET Gener. Transm. Distrib. 2018, 12, 615–623. [Google Scholar] [CrossRef]
- Zhang, Z. Analysis of new filtering transformers energy saving theory and calculation of harmonics eddy current loss. Chin. J. Power Sources 2016, 40, 444–447. [Google Scholar]
- Luo, G.; Zhang, R.; Chen, Z.; Tu, W.; Zhang, S.; Kennel, R. A Novel Nonlinear Modeling Method for Permanent-Magnet Synchronous Motors. IEEE Trans. Ind. Electron. 2016, 63, 6490–6498. [Google Scholar] [CrossRef]
- Gan, B.; Zhang, B.; Liu, Y.; Feng, G. Thermal Analysis of Modular Fault-Tolerant Permanent Magnet Motor Based on Electromagnetic-Thermal Bi-Directional Coupling. IEEJ Trans. Electr. Electron. Eng. 2022, 17, 454–469. [Google Scholar] [CrossRef]
- Zhu, S.; Hua, W.; Shi, B. Comparison of Methods Using Different Sources for Computing PWM Effects on Permanent Magnet Machines Considering Eddy Current Reaction. IEEE Trans. Magn. 2021, 57, 1–4. [Google Scholar] [CrossRef]
- Dawood, K.; Işik, F.; Kömürgöz, G. Analysis and optimization of leakage impedance in a transformer with additional winding: A numerical and experimental study. Alex. Eng. J. 2022, 61, 11291–11300. [Google Scholar] [CrossRef]
- Moradnouri, A.; Hajiaghapour-Moghimi, M.; Vakilian, M. A Framework for Economic and Low Harmonic Loss Distribution Transformer Selection based on Ecodesign Directive. In Proceedings of the 34th International Power System Conference, PSC 2019, Tehran, Iran, 9–11 December 2019; pp. 19–24. [Google Scholar] [CrossRef]
- Yazdani-Asrami, M.; Mirzaie, M.; Akmal, A.A.S. No-load loss calculation of distribution transformers supplied by nonsinusoidal voltage using three-dimensional finite element analysis. Energy 2013, 50, 205–219. [Google Scholar] [CrossRef]
- Irannezhad, F.; Heydari, H.; Faghihi, F. Precise appraisement of the harmonic loads impact on hysteresis losses in a 3-phase HTS transformer. Int. J. Electr. Power Energy Syst. 2021, 133, 107199. [Google Scholar] [CrossRef]
- Susnjic, L.; Haznadar, Z.; Valkovic, Z. 3D finite-element determination of stray losses in power transformer. Electr. Power Syst. Res. 2008, 78, 1814–1818. [Google Scholar] [CrossRef]
- Chiu, H.-C.; Pao, H.-K.; Hsieh, R.-H.; Chiu, Y.-J.; Jang, J.-H. Numerical Analysis on the Eddy Current Losses in a Dry-type 3000 KVA Transformer. Energy Procedia 2019, 156, 332–336. [Google Scholar] [CrossRef]
- Yazdani-Asrami, M.; Gholamian, S.A.; Mirimani, S.M.; Adabi, J. Influence of field-dependent critical current on harmonic AC loss analysis in HTS coils for superconducting transformers supplying non-linear loads. Cryogenics 2021, 113, 103234. [Google Scholar] [CrossRef]
- Ehsanifar, A.; Allahbakhshi, M.; Tajdinian, M.; Dehghani, M.; Montazeri, Z.; Malik, O.; Guerrero, J.M. Transformer inter-turn winding fault detection based on no-load active power loss and reactive power. Int. J. Electr. Power Energy Syst. 2021, 130, 107034. [Google Scholar] [CrossRef]
- Zhang, X.; Yang, S.; Guo, Q. Transformer Health Assessment Method Based on Loss Parameter Identification by Multivariate Linear Regression. 2019. Available online: https://ieeexplore.ieee.org/document/8984141/ (accessed on 5 September 2022).
- Qionglin, L.; Lei, Z.; Huijin, L.; Xue, C.; Zhenan, Z.; Shangbei, F. Simulation calculation and experimental research on harmonic loss of power transformers. Power Grid Technol. 2013, 37, 3521–3527. [Google Scholar] [CrossRef]
- Pan, C.; Wang, C.; Liu, Z.; Chen, X. Winding vibration analysis of unbalanced transformer based on electromagnetic-mechanical coupling. Int. J. Electr. Power Energy Syst. 2021, 134, 107459. [Google Scholar] [CrossRef]
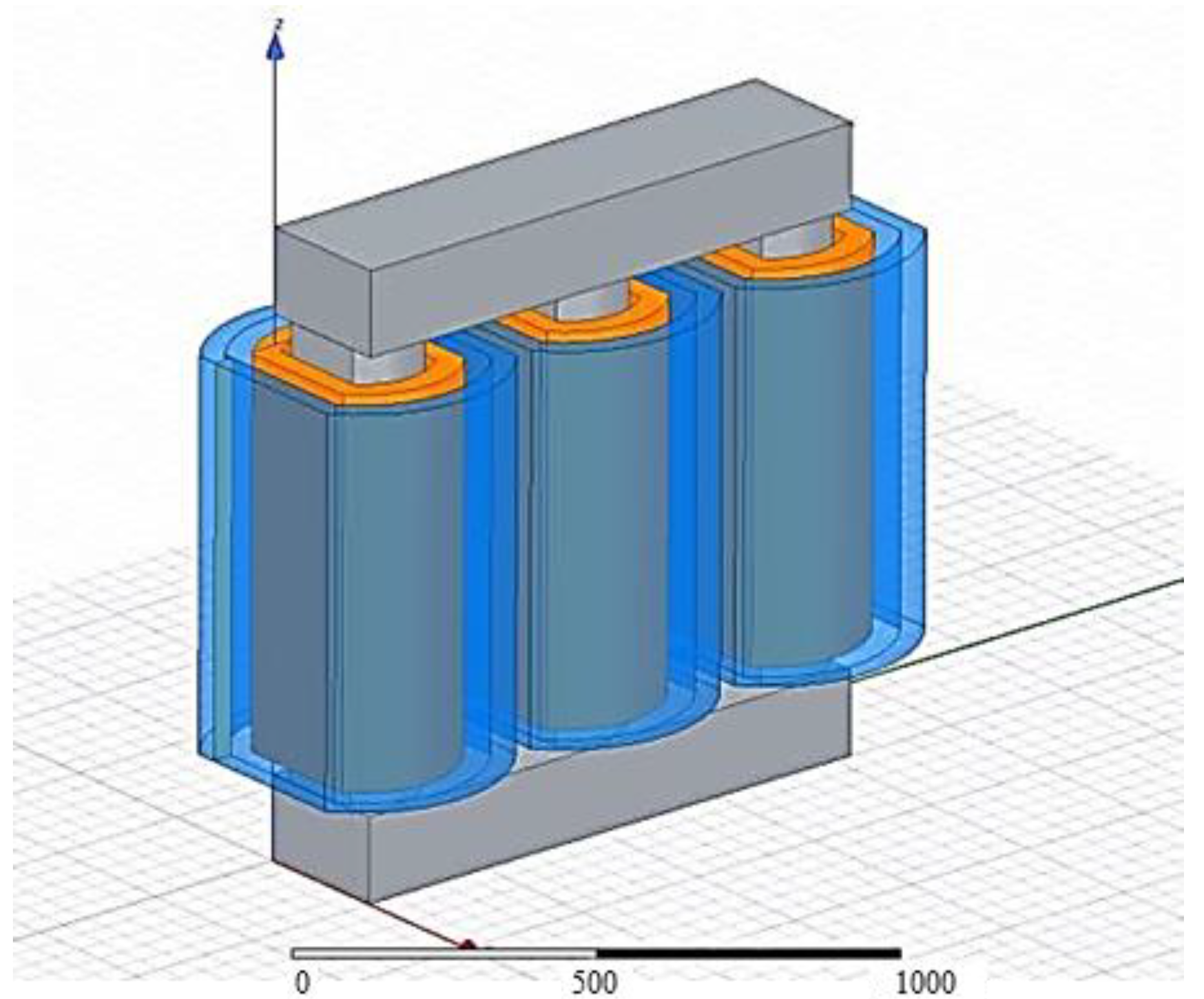
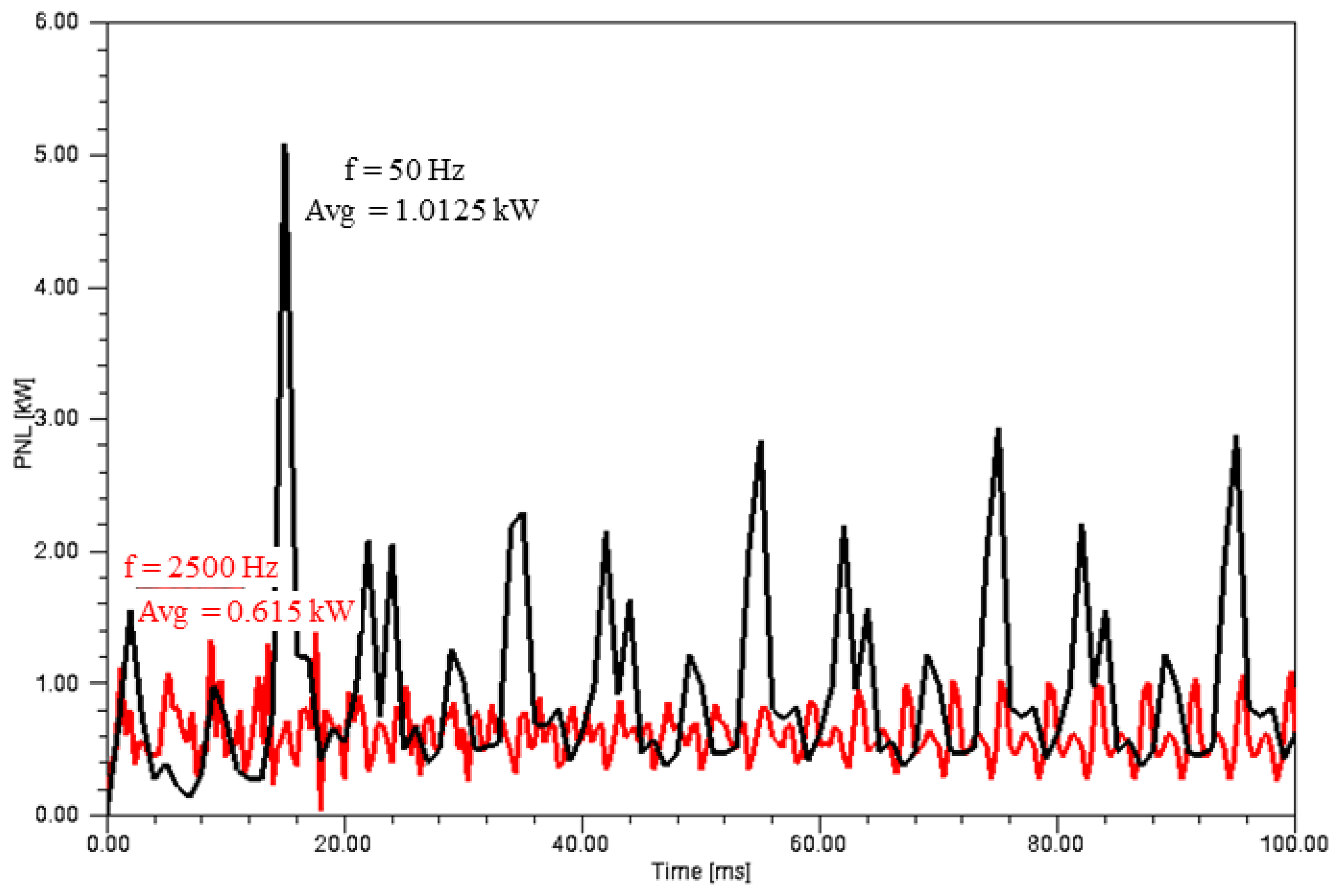

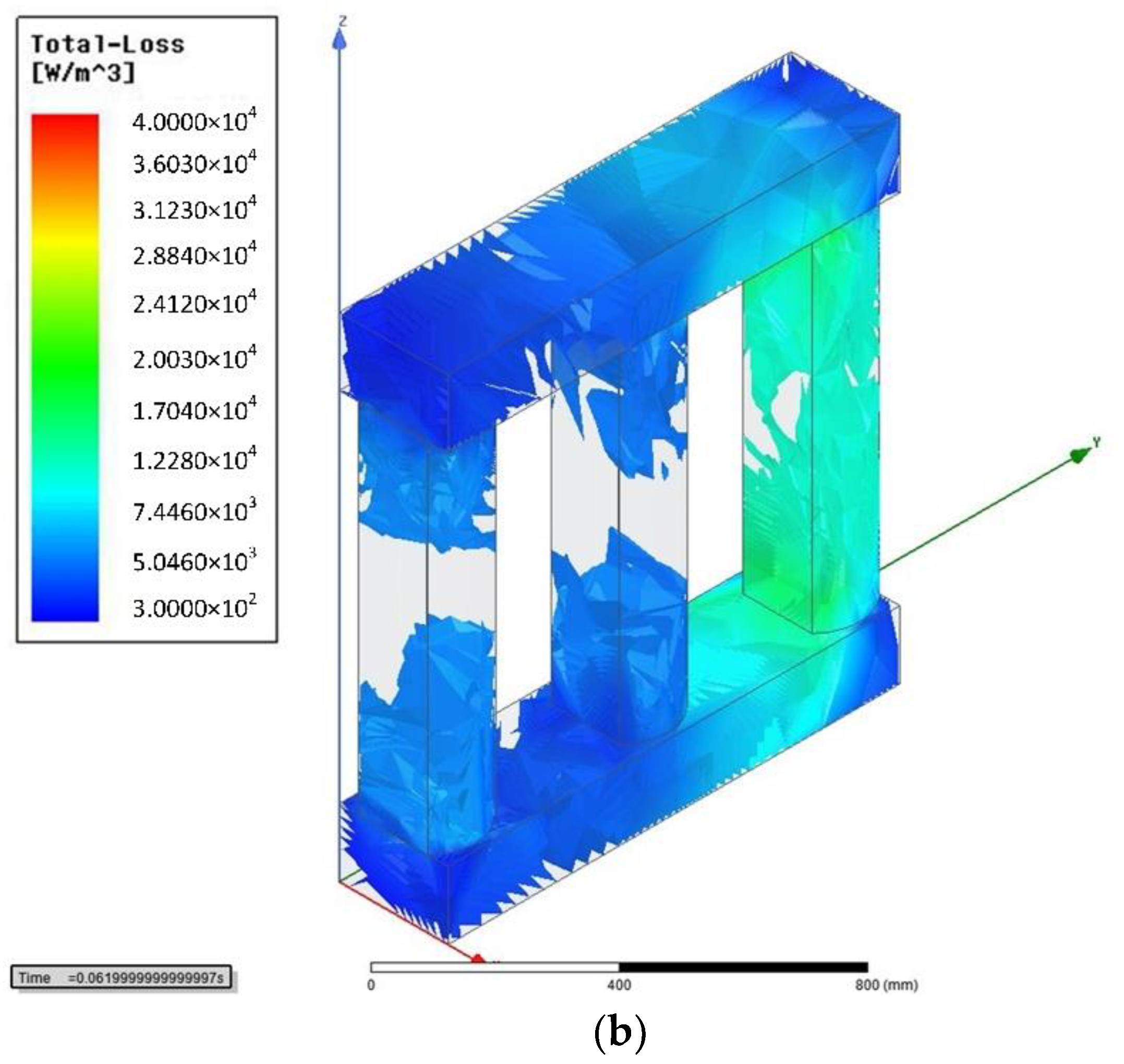




| Abbreviation | Transformer Parameters (Nameplate Value) |
|---|---|
| PTL | Total theoretical transformer losses |
| PNL | No-load core loss |
| PLL | Load winding losses |
| Working flux density amplitude of silicon steel sheet | |
| n | Hysteresis coefficient, generally 2 to 2.5 |
| t | Thickness of silicon steel sheet |
| G | Core quality |
| Additional losses in the iron core | |
| Transformer winding resistance losses | |
| Eddy current losses in windings | |
| Additional losses due to winding skin effect and proximity effect | |
| Hysteresis loss factor | |
| Eddy current loss factor | |
| Em | Transformer induced electromotive force amplitude |
| N | Number of winding turns |
| A | Effective cross-sectional area of the iron core |
| h | Number of harmonics |
| Rdc | Windings DC resistance |
| Electrical conductivity of copper conductors | |
| Copper conductor magnetic permeability | |
| Angular frequency of the hth harmonic | |
| P | Number of winding layers |
| d | Thickness of each layer of silicon steel sheet |
| A | Vector magnetic position |
| JS | Source current density |
| v | Magnetoresistivity |
| S | Cross-sectional area of windings |
| h | Coil tangent direction unit vector |
| Three-phase transformer core area |
| Parameter | Transformer Parameters (Nameplate Value) | Finite Element Simulation Values |
|---|---|---|
| capacity | 630 kV·A | 625.39 kV·A |
| Rated voltage | 10/0.4 kV | 9.83/0.388 kV |
| Connection group number | Yyn0 | Yyn0 |
| No-load loss | ≤1.36 kW | 1.01 kW |
| Load loss (120 °C) | ≤5.88 kW | 5.43 kW |
| Short-circuit impedance voltage Uk% | 4.0% | 4.3% |
| h | Hysteresis Loss | Eddy Current Loss | Total Core Loss |
|---|---|---|---|
| Fundamental | 0.4844 | 0.4031 | 1.013 |
| 5 | 0.1102 | 0.3825 | 0.615 |
| 7 | 0.0629 | 0.3769 | 0.562 |
| 11 | 0.0403 | 0.3714 | 0.537 |
| 13 | 0.0336 | 0.3651 | 0.521 |
| 17 | 0.0249 | 0.3597 | 0.503 |
| 19 | 0.0199 | 0.3525 | 0.484 |
| h | Calculation Result | Simulation Result | Error |
|---|---|---|---|
| Fundamental | 1.237 | 1.013 | 22.4% |
| 3 | 0.895 | 0.808 | 8.7% |
| 5 | 0.553 | 0.615 | 6.2% |
| 7 | 0.519 | 0.562 | 4.3% |
| 9 | 0.490 | 0.550 | 6% |
| 11 | 0.472 | 0.537 | 6.2% |
| 13 | 0.464 | 0.521 | 5.7% |
| 15 | 0.454 | 0.509 | 5.5% |
| 17 | 0.449 | 0.484 | 3.5% |
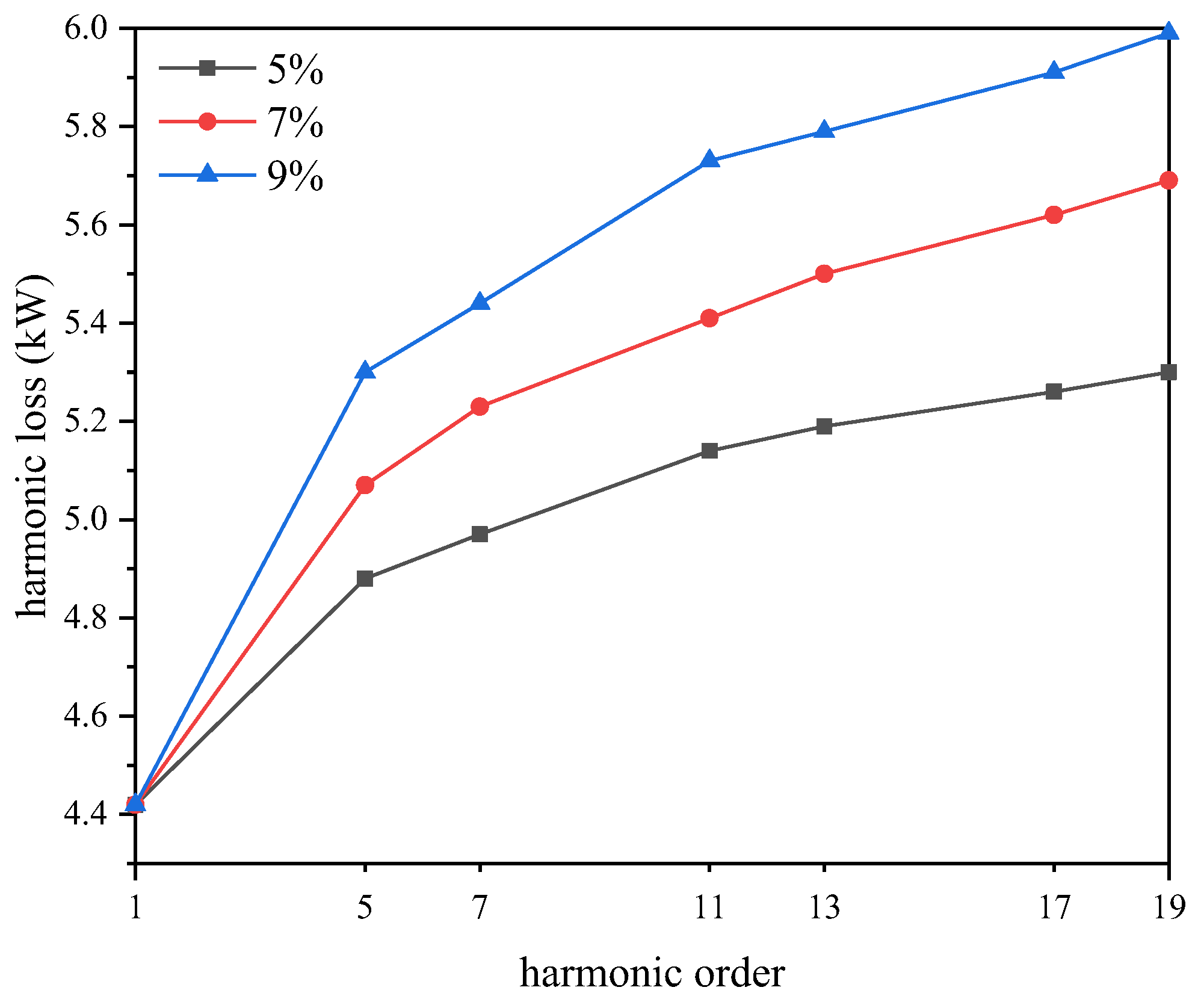
| h | 5% | 7% | 9% |
|---|---|---|---|
| Fundamental | 4.42 | 4.42 | 4.42 |
| 5 | 4.88 | 5.07 | 5.30 |
| 7 | 4.97 | 5.23 | 5.44 |
| 11 | 5.14 | 5.41 | 5.73 |
| 13 | 5.19 | 5.50 | 5.79 |
| 17 | 5.26 | 5.62 | 5.91 |
| 19 | 5.30 | 5.69 | 5.99 |
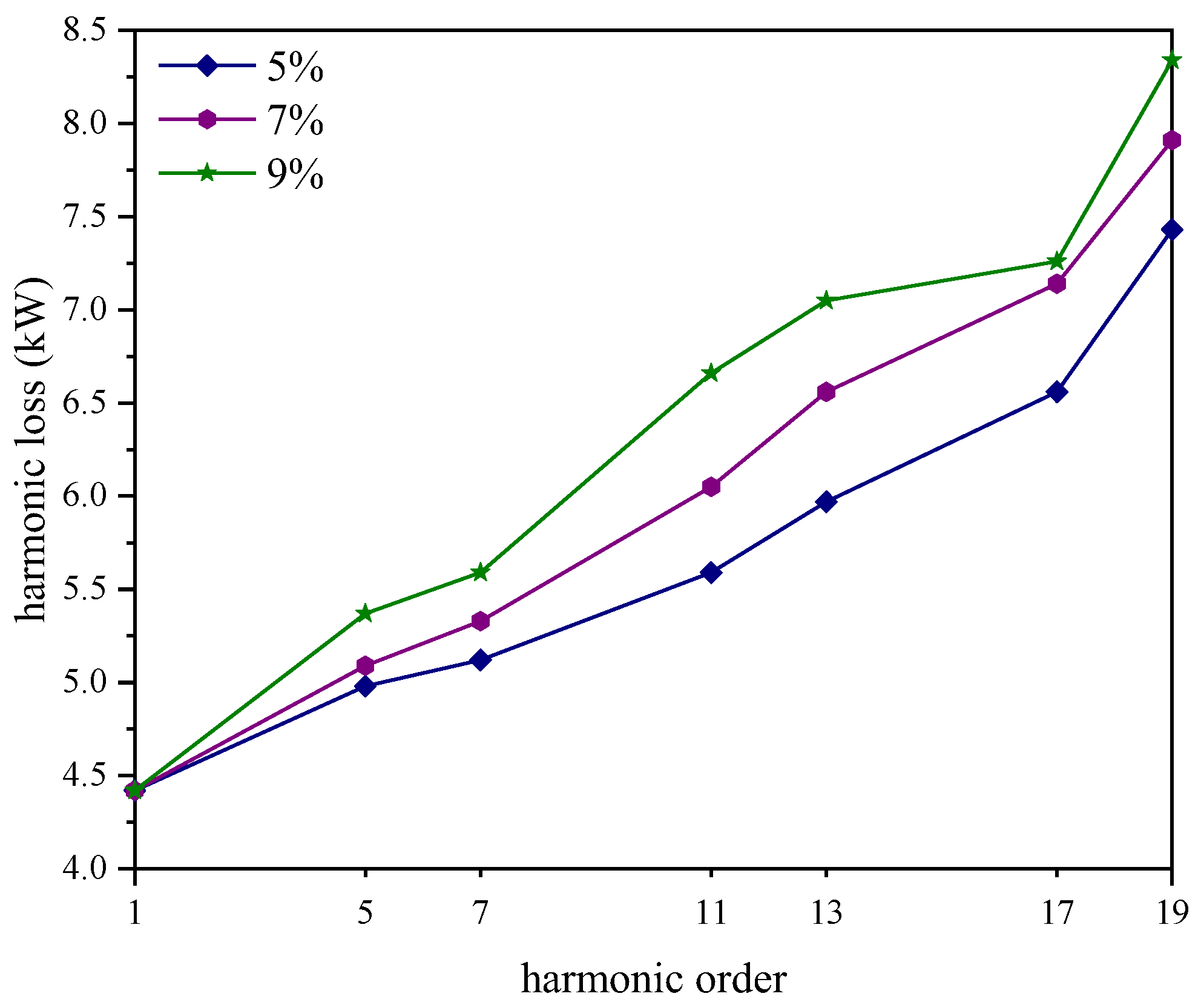
| h | 5% | 7% | 9% |
|---|---|---|---|
| Fundamental | 4.42 | 4.42 | 4.42 |
| 5 | 4.98 | 5.09 | 5.37 |
| 7 | 5.12 | 5.33 | 5.59 |
| 11 | 5.59 | 6.05 | 6.66 |
| 13 | 5.97 | 6.56 | 7.05 |
| 17 | 6.56 | 7.14 | 7.26 |
| 19 | 7.43 | 7.91 | 8.34 |
| h | AC Resistance Coefficient Model | |
|---|---|---|
| Fundamental | 0 | 0 |
| 5 | 1.8% | 3.8% |
| 7 | 2.9% | 2.0% |
| 11 | 9.7% | 1.3% |
| 13 | 15.6% | 1.4% |
| 17 | 20.1% | 7.8% |
| 19 | 28.1% | 3.2% |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ma, X.; Jia, R.; Liang, C.; Du, H.; Dong, X.; Ding, M. Study of Transformer Harmonic Loss Characteristic in Distribution Network Based on Field-Circuit Coupling Method. Sustainability 2022, 14, 12975. https://doi.org/10.3390/su142012975
Ma X, Jia R, Liang C, Du H, Dong X, Ding M. Study of Transformer Harmonic Loss Characteristic in Distribution Network Based on Field-Circuit Coupling Method. Sustainability. 2022; 14(20):12975. https://doi.org/10.3390/su142012975
Chicago/Turabian StyleMa, Xiping, Rong Jia, Chen Liang, Haodong Du, Xiaoyang Dong, and Man Ding. 2022. "Study of Transformer Harmonic Loss Characteristic in Distribution Network Based on Field-Circuit Coupling Method" Sustainability 14, no. 20: 12975. https://doi.org/10.3390/su142012975
APA StyleMa, X., Jia, R., Liang, C., Du, H., Dong, X., & Ding, M. (2022). Study of Transformer Harmonic Loss Characteristic in Distribution Network Based on Field-Circuit Coupling Method. Sustainability, 14(20), 12975. https://doi.org/10.3390/su142012975







