The Dynamics of Water-Based Nanofluid Subject to the Nanoparticle’s Radius with a Significant Magnetic Field: The Case of Rotating Micropolar Fluid
Abstract
1. Introduction
2. Mathematical Formulation
3. Solution Procedure
- ,
- ,
- ,
- ,
- ,
- ,
- ,
- ,
- .
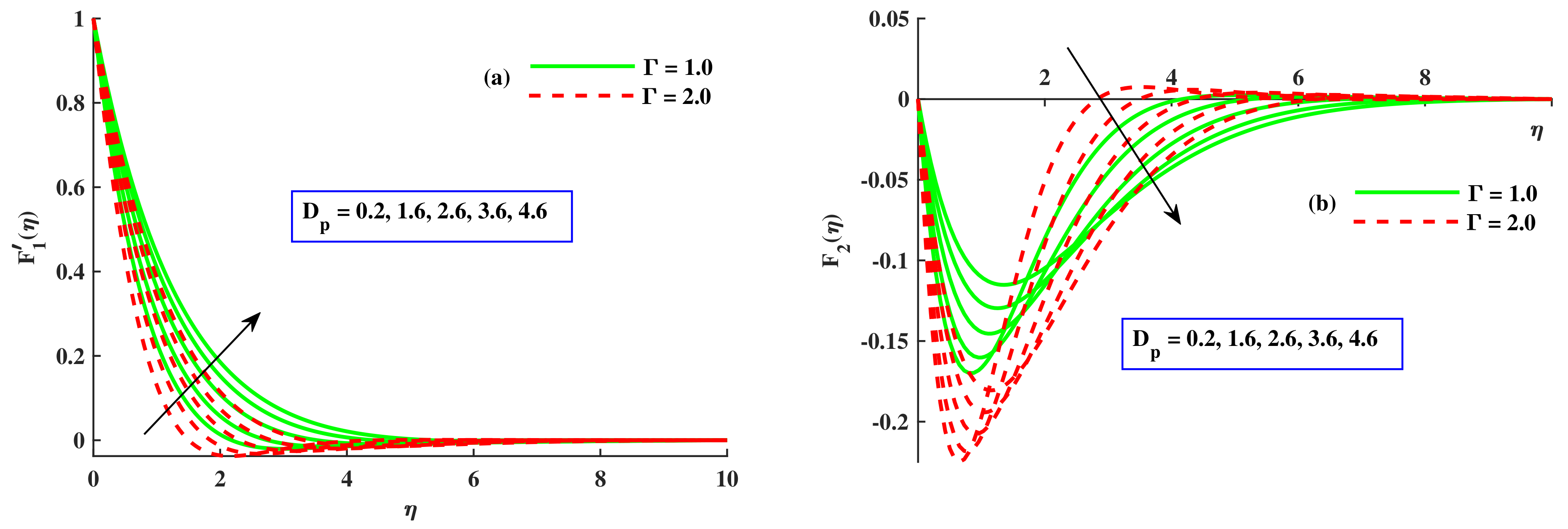
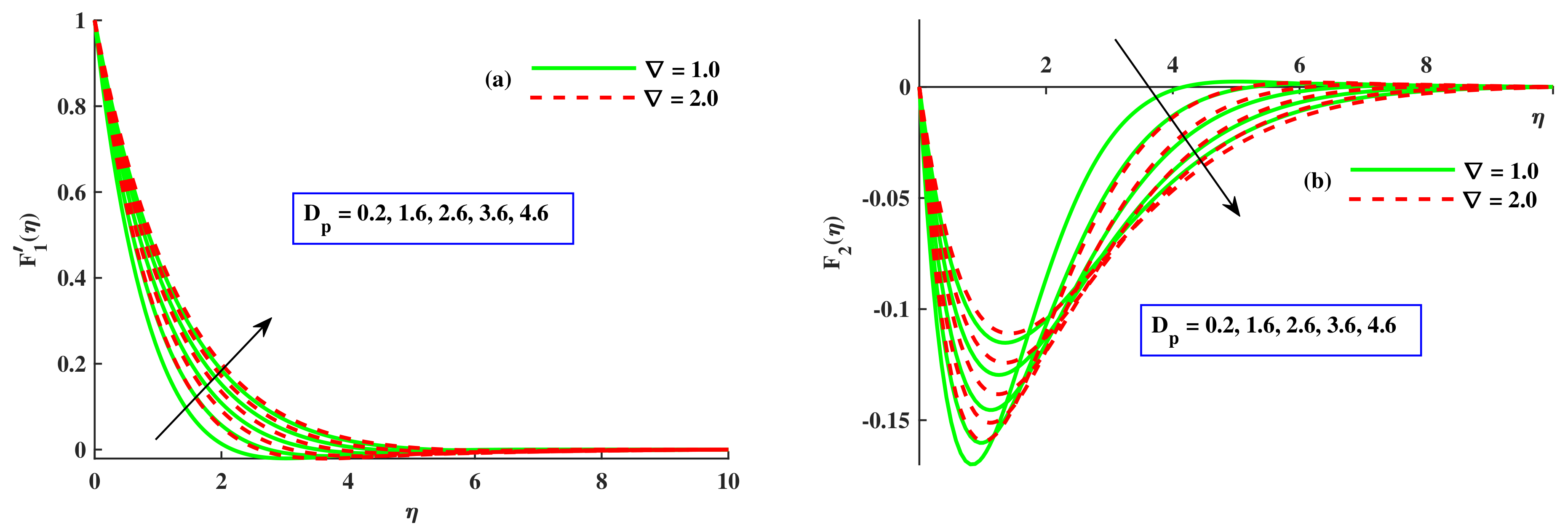
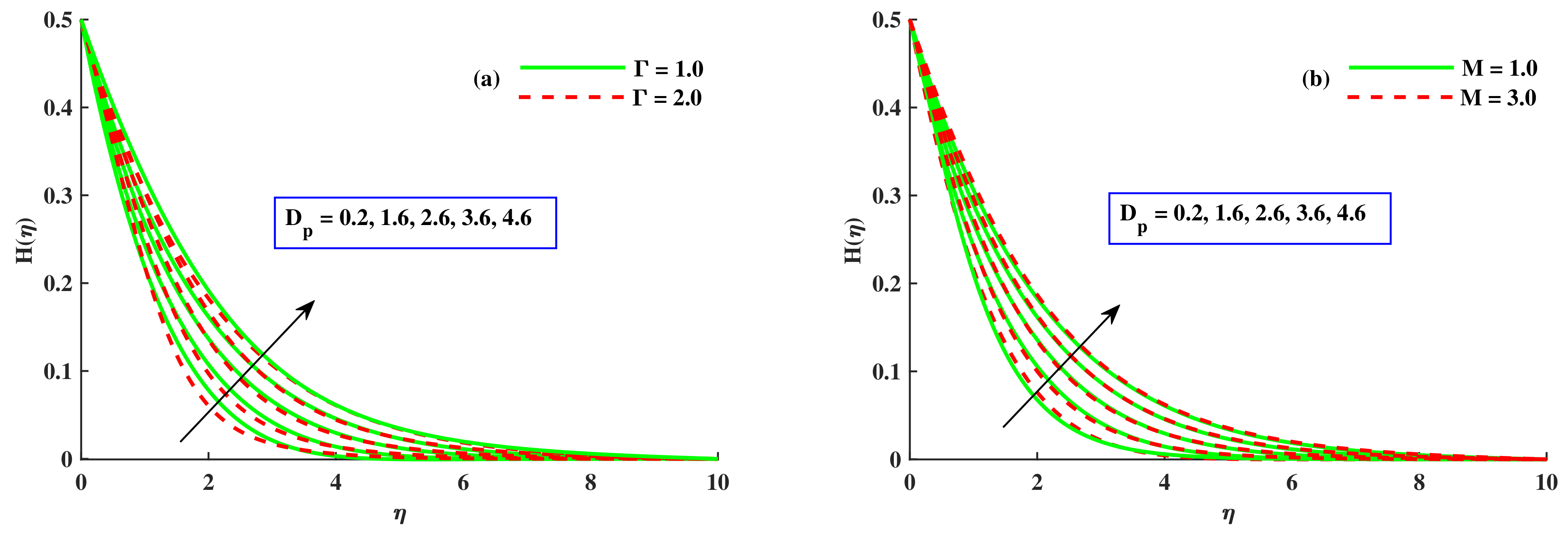
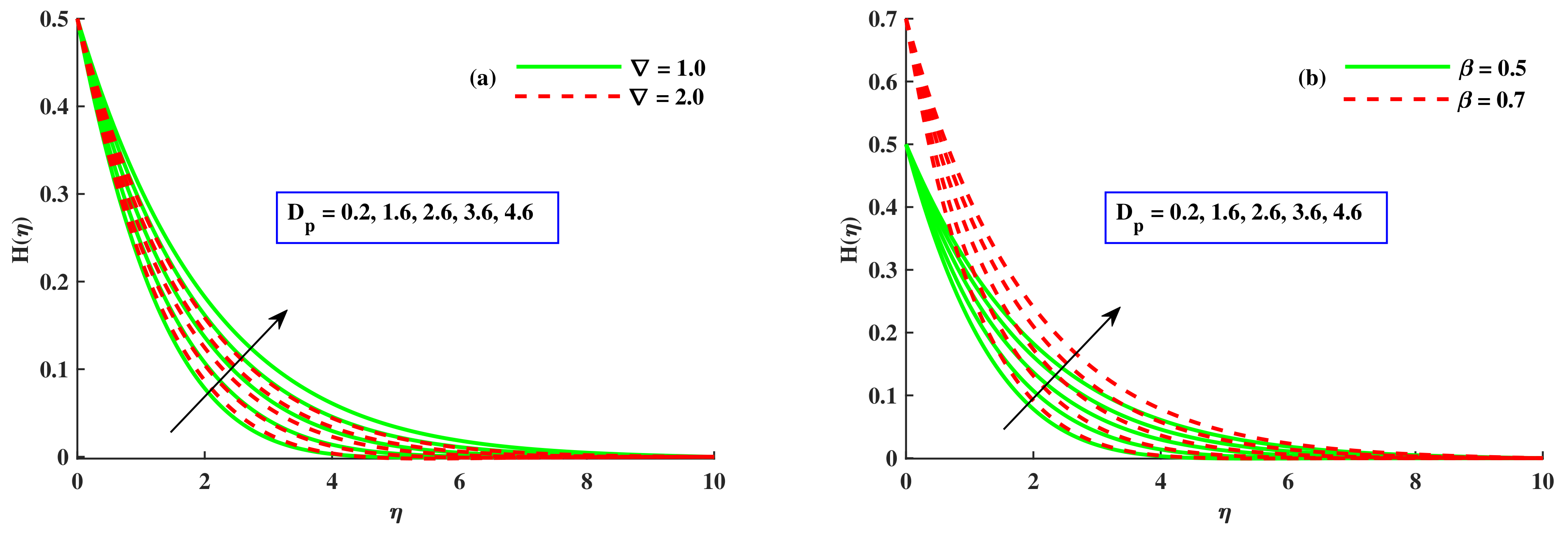
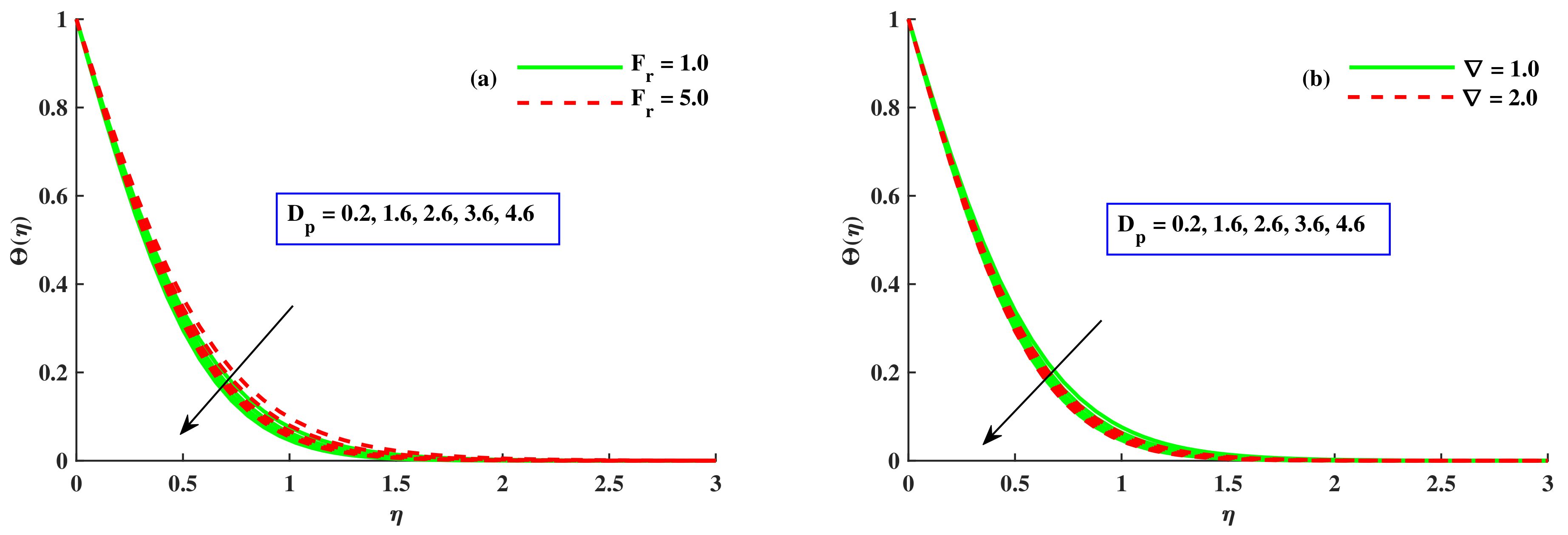
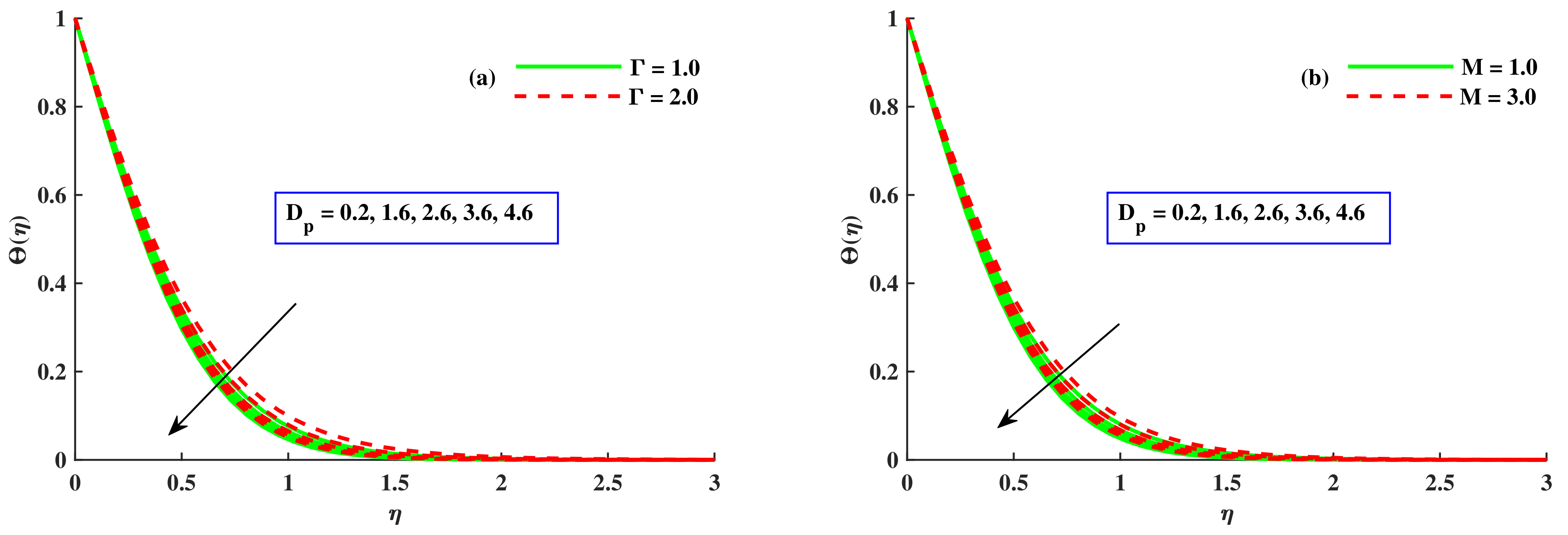
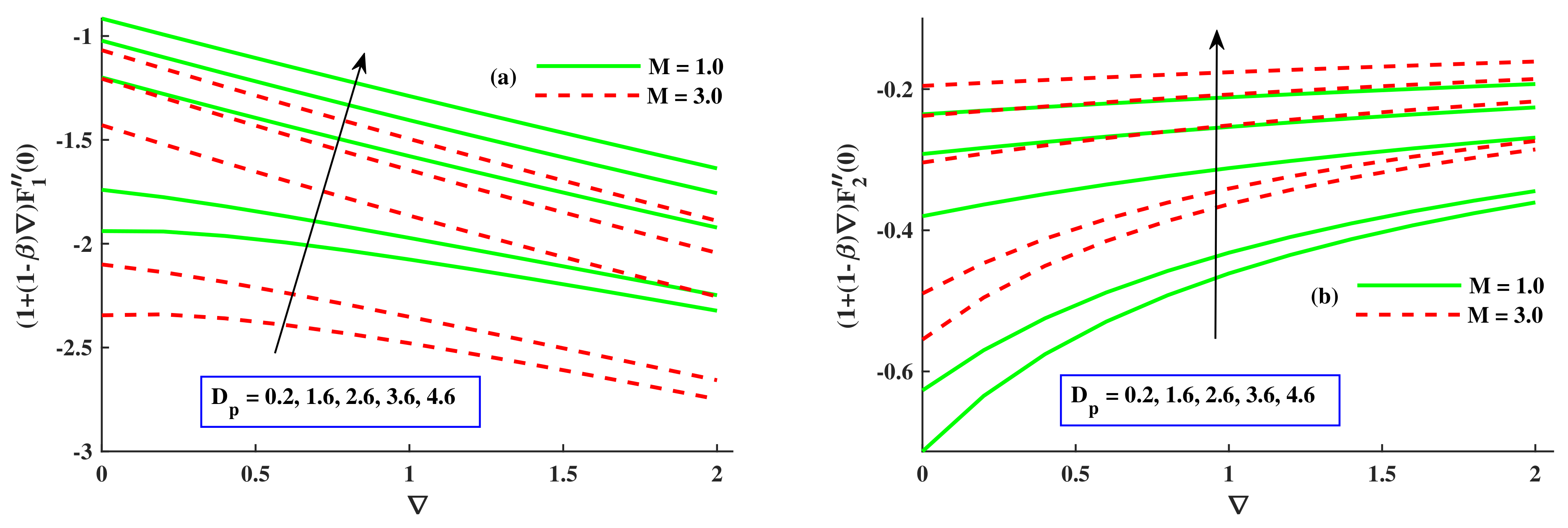
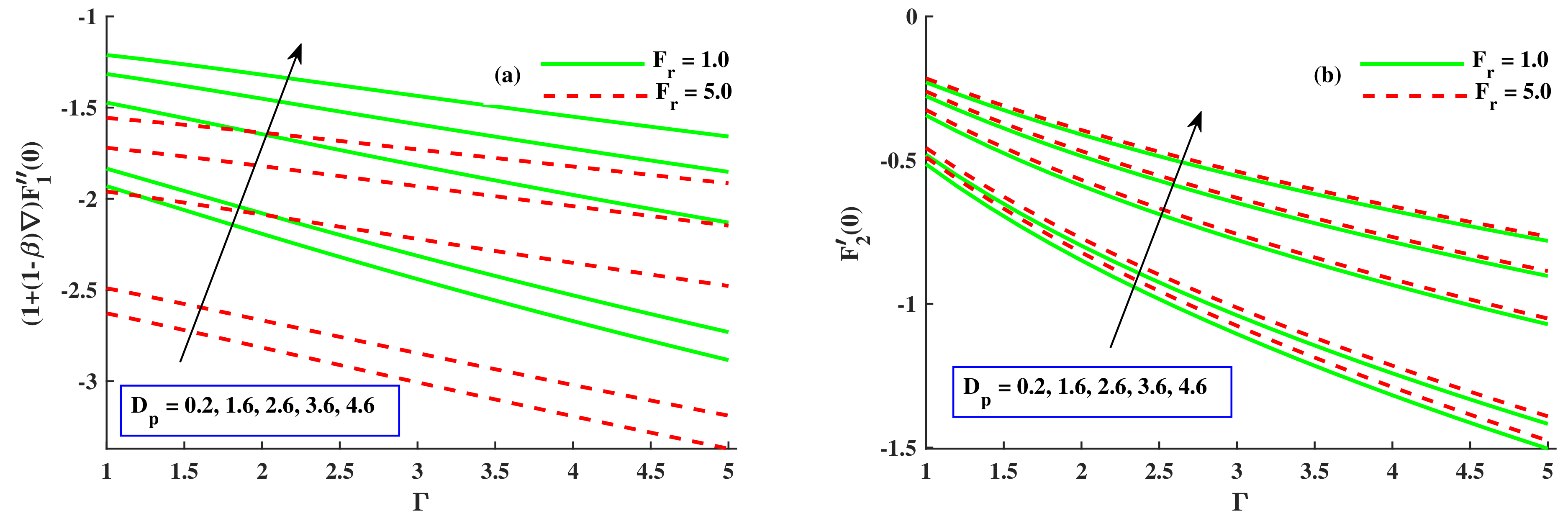
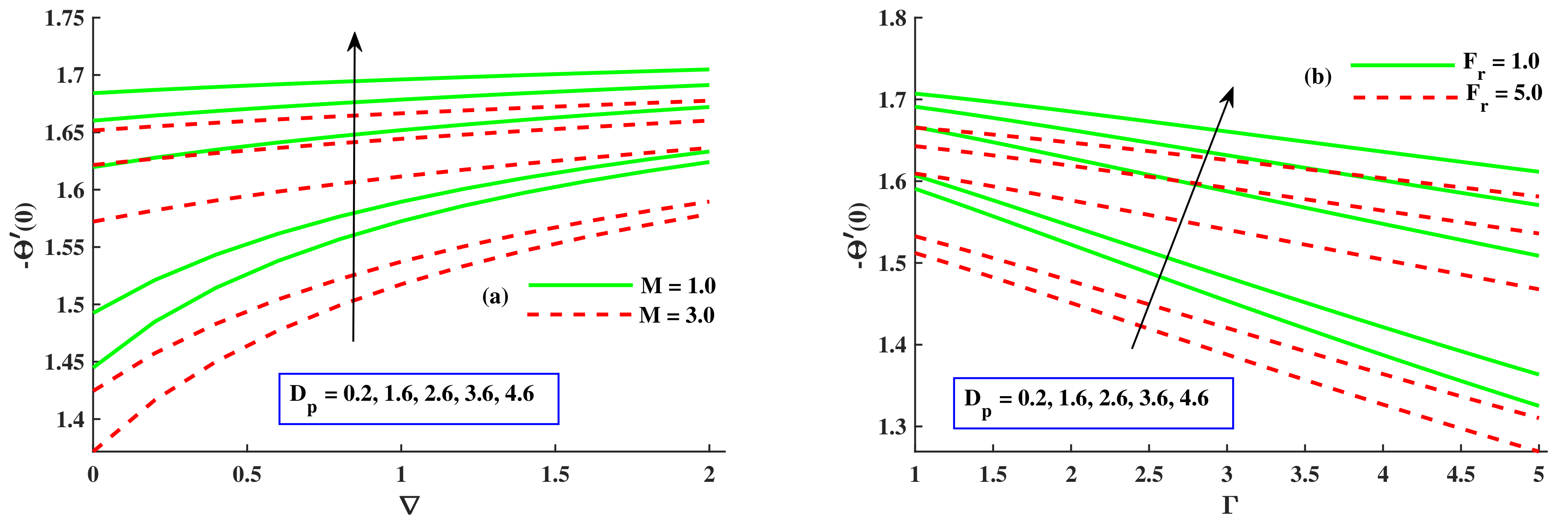
4. Results and Discussion
5. Conclusions
- The increase in the nanoparticle radius increased the velocity and microrotation H and
- Decreased the secondary velocity .
- Decreased the Cu-nanofluid’s temperature.
- Increased the skin friction factor.
- Increased the Nusselt number.
- The magnetic parameter M reduced the component of velocity , and
- Increased the microrotation of nanoparticles.
- Increased the temperature of the non-Newtonian fluid.
- Increased the skin friction coefficient but lessened the .
- Reduced the Nusselt number.
- The boundary concentration parameter increased the microrotation distribution.
- The rotational parameter lowered the , , and H and
- Enhanced the temperature profile.
- Decrease the skin friction coefficients and Nusselt number.
- The higher input of the Forchheimer number decreased the velocity , , and microrotation and
- Increased the temperature of the fluid.
- Increase the but reduced the and Nusselt number.
- The material parameter ∇ reduced the component of velocity , microrotation, and temperature and
- Enlarged the velocity component .
- Enlarged the but reduced the .
- Enlarged the Nusselt number.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Eringen, A.C. Theory of micropolar fluids. J. Math. Mech. 1966, 16, 1–18. [Google Scholar] [CrossRef]
- Reddy, S.; Reddy, P.B.A. Thermal radiation effect on unsteady three-dimensional mhd flow of micropolar fluid over a horizontal surface of a parabola of revolution. Propuls. Power Res. 2022, 11, 129–142. [Google Scholar] [CrossRef]
- Singh, K.; Pandey, A.K.; Kumar, M. Numerical solution of micropolar fluid flow via stretchable surface with chemical reaction and melting heat transfer using keller-box method. Propuls. Power Res. 2021, 10, 194–207. [Google Scholar] [CrossRef]
- Salahuddin, T.; Khan, M.; Al-Mubaddel, F.S.; Alam, M.M.; Ahmad, I. A study of heat and mass transfer micropolar fluid flow near the stagnation regions of an object. Case Stud. Therm. Eng. 2021, 26, 101064. [Google Scholar] [CrossRef]
- Nadeem, S.; Malik, M.; Abbas, N. Heat transfer of three-dimensional micropolar fluid on a riga plate. Can. J. Phys. 2020, 98, 32–38. [Google Scholar] [CrossRef]
- Ali, B.; Shafiq, A.; Siddique, I.; Al-Mdallal, Q.; Jarad, F. Significance of suction/injection, gravity modulation, thermal radiation, and magnetohydrodynamic on dynamics of micropolar fluid subject to an inclined sheet via finite element approach. Case Stud. Therm. Eng. 2021, 28, 101537. [Google Scholar] [CrossRef]
- Ali, B.; Rasool, G.; Hussain, S.; Baleanu, D.; Bano, S. Finite element study of magnetohydrodynamics (mhd) and activation energy in darcy–forchheimer rotating flow of casson carreau nanofluid. Processes 2020, 8, 1185. [Google Scholar] [CrossRef]
- He, J.-H.; Moatimid, G.M.; Sayed, A. Nonlinear ehd instabilitynonlinear ehd instability of two-superposed walters’ b fluids moving through porous media. Axioms 2021, 10, 258. [Google Scholar] [CrossRef]
- Lin, J.; Yang, H. A review on the flow instability of nanofluids. Appl. Math. Mech. 2019, 40, 1227–1238. [Google Scholar] [CrossRef]
- Jama, M.; Singh, T.; Gamaleldin, S.M.; Koc, M.; Samara, A.; Isaifan, R.J.; Atieh, M.A. Critical review on nanofluids: Preparation, characterization, and applications. J. Nanomater. 2016, 2016, 6717624. [Google Scholar] [CrossRef]
- Ashraf, M.A.; Peng, W.; Zare, Y.; Rhee, K.Y. Effects of size and aggregation/agglomeration of nanoparticles on the interfacial/interphase properties and tensile strength of polymer nanocomposites. Nanoscale Res. Lett. 2018, 13, 1–7. [Google Scholar] [CrossRef] [PubMed]
- Vishal, C.C.; Kanala, R.K.; Raju, C.S.K.; Madathil, P.K.; Saha, P.; Rao, B.R.; Sriganesh, G.; Ramesh, K. Sub-micron sized metal oxides based organic thermic fluids with enhanced thermo-physical properties. Appl. Therm. Eng. 2019, 163, 114337. [Google Scholar] [CrossRef]
- He, J.-H.; Abd Elazem, N.Y. The carbon nanotube-embedded boundary layer theory for energy harvesting. Facta Univ. Ser. Mech. Eng. 2022, 20, 211–235. [Google Scholar]
- Ali, B.; Siddique, I.; Khan, I.; Masood, B.; Hussain, S. Magnetic dipole and thermal radiation effects on hybrid base micropolar cnts flow over a stretching sheet: Finite element method approach. Results Phys. 2021, 25, 104145. [Google Scholar] [CrossRef]
- Yapici, K.; Osturk, O.; Uludag, Y. Dependency of nanofluid rheology on particle size and concentration of various metal oxide nanoparticles. Braz. J. Chem. Eng. 2018, 35, 575–586. [Google Scholar] [CrossRef]
- Namburu, P.; Kulkarni, D.; Dandekar, A.; Das, D. Experimental investigation of viscosity and specific heat of silicon dioxide nanofluids. Micro Nano Lett. 2007, 2, 67–71. [Google Scholar] [CrossRef]
- Shah, S.A.A.; Ahammad, N.A.; Din, E.M.T.E.; Gamaoun, F.; Awan, A.U.; Ali, B. Bio-convection effects on prandtl hybrid nanofluid flow with chemical reaction and motile microorganism over a stretching sheet. Nanomaterials 2022, 12, 2174. [Google Scholar] [CrossRef]
- Habib, D.; Salamat, N.; Abdal, S.H.S.; Ali, B. Numerical investigation for mhd prandtl nanofluid transportation due to a moving wedge: Keller box approach. Int. Commun. Heat Mass Transf. 2022, 135, 106141. [Google Scholar] [CrossRef]
- Wang, J.; Mustafa, Z.; Siddique, I.; Ajmal, M.; Jaradat, M.M.; Rehman, S.U.; Ali, B.; Ali, H.M. Computational analysis for bioconvection of microorganisms in prandtl nanofluid darcy–forchheimer flow across an inclined sheet. Nanomaterials 2022, 12, 1791. [Google Scholar] [CrossRef]
- Younis, O.; Alizadeh, M.; Hussein, A.K.; Ali, B.; Biswal, U.; Malekshah, E.H. Mhd natural convection and radiation over a flame in a partially heated semicircular cavity filled with a nanofluid. Mathematics 2022, 10, 1347. [Google Scholar] [CrossRef]
- Awan, A.U.; Abid, S.; Ullah, N.; Nadeem, S. Magnetohydrodynamic oblique stagnation point flow of second grade fluid over an oscillatory stretching surface. Results Phys. 2022, 18, 103233. [Google Scholar] [CrossRef]
- Jang, J.; Lee, S.S. Theoretical and experimental study of mhd (magnetohydrodynamic) micropump. Sensors Actuators A Phys. 2000, 80, 84–89. [Google Scholar] [CrossRef]
- Chabani, I.; Mebarek-Oudina, F.; Ismail, A.A.I. Mhd flow of a hybrid nano-fluid in a triangular enclosure with zigzags and an elliptic obstacle. Micromachines 2022, 13, 224. [Google Scholar] [CrossRef]
- Bhatti, M.; Arain, M.; Zeeshan, A.; Ellahi, R.; Doranehgard, M. Swimming of gyrotactic microorganism in mhd williamson nanofluid flow between rotating circular plates embedded in porous medium: Application of thermal energy storage. J. Energy Storage 2022, 45, 103511. [Google Scholar] [CrossRef]
- Al-Farhany, K.; Abdulkadhim, A.; Hamzah, H.K.; Ali, F.H.; Chamkha, A. Mhd effects on natural convection in a u-shaped enclosure filled with nanofluid-saturated porous media with two baffles. Prog. Nucl. Energy 2022, 145, 104136. [Google Scholar] [CrossRef]
- He, J.-H.; Moatimid, G.M.; Mostapha, D.R. Nonlinear instability of two streaming-superposed magnetic reiner-rivlin fluids by he-laplace method. J. Electroanal. Chem. 2021, 895, 115388. [Google Scholar] [CrossRef]
- Aziz, A.; Aziz, A.; Ullah, I.; Subhani, M. Numerical simulation for 3d rotating flow of nanofluid with entropy generation. Int. J. Model. Simul. 2022, 1–22. [Google Scholar] [CrossRef]
- Krishna, M.V.; Ahamad, N.A.; Chamkha, A.J. Numerical investigation on unsteady mhd convective rotating flow past an infinite vertical moving porous surface. Ain Shams Eng. J. 2021, 12, 2099–2109. [Google Scholar] [CrossRef]
- Yacob, N.A.; Dzulkifli, N.F.; Salleh, S.N.A.; Ishak, A.; Pop, I. Rotating flow in a nanofluid with cnt nanoparticles over a stretching/shrinking surface. Mathematics 2021, 10, 7. [Google Scholar] [CrossRef]
- Shahzad, F.; Jamshed, W.; Sajid, T.; Nisar, K.S.; Eid, M.R. Heat transfer analysis of mhd rotating flow of fe3o4 nanoparticles through a stretchable surface. Commun. Theor. Phys. 2021, 73, 075004. [Google Scholar] [CrossRef]
- Sajid, M.; Javed, T.; Hayat, T. Mhd rotating flow of a viscous fluid over a shrinking surface. Nonlinear Dyn. 2008, 51, 259–265. [Google Scholar] [CrossRef]
- Ali, B.; Siddique, I.; Ahmadian, A.; Senu, N.; Ali, L.; Haider, A. Significance of lorentz and coriolis forces on dynamics of water based silver tiny particles via finite element simulation. Ain Shams Eng. J. 2022, 13, 101572. [Google Scholar] [CrossRef]
- Rashid, S.; Hayat, T.; Qayyum, S.; Ayub, M.; Alsaedi, A. Three-dimensional rotating darcy–forchheimer flow with activation energy. Int. J. Numer. Methods Heat Fluid Flow 2018, 29, 935–948. [Google Scholar] [CrossRef]
- Javed, T.; Ahmad, I.; Abbas, Z.; Hayat, T. Rotating flow of a micropolar fluid induced by a stretching surface. Z. Naturforschung A 2010, 65, 829–843. [Google Scholar] [CrossRef][Green Version]
- Tiwari, R.K.; Das, M.K. Heat transfer augmentation in a two-sided lid-driven differentially heated square cavity utilizing nanofluids. Int. J. Heat Mass Transf. 2007, 50, 2002–2018. [Google Scholar] [CrossRef]
- Ali, B.; Khan, S.A.; Hussein, A.K.; Thumma, T.; Hussain, S. Hybrid nanofluids: Significance of gravity modulation, heat source/sink, and magnetohydrodynamic on dynamics of micropolar fluid over an inclined surface via finite element simulation. Appl. Math. Comput. 2022, 419, 126878. [Google Scholar] [CrossRef]
- Hayat, T.; Muhammad, T.; Mustafa, M.; Alsaedi, A. An optimal study for three-dimensional flow of maxwell nanofluid subject to rotating frame. J. Mol. Liq. 2017, 229, 541–547. [Google Scholar] [CrossRef]
- Ali, B.; Naqvi, R.A.; Ali, L.; Abdal, S.; Hussain, S. A comparative description on time-dependent rotating magnetic transport of a water base liquid H2O with hybrid nano-materials Al2O3-Cu and Al2O3-TiO2 over an extending sheet using buongiorno model: Finite element approach. Chin. J. Phys. 2021, 70, 125–139. [Google Scholar] [CrossRef]
- Graham, A.L. On the viscosity of suspensions of solid spheres. Appl. Sci. Res. 1981, 37, 275–286. [Google Scholar] [CrossRef]
- Lou, Q.; Ali, B.; Rehman, S.U.; Habib, D.; Abdal, S.; Shah, N.A.; Chung, J.D. Micropolar dusty fluid: Coriolis force effects on dynamics of mhd rotating fluid when lorentz force is significant. Mathematics 2022, 10, 2630. [Google Scholar] [CrossRef]
- Wei, Y.; Rehman, S.U.; Fatima, N.; Ali, B.; Ali, L.; Chung, J.D.; Shah, N.A. Significance of dust particles, nanoparticles radius, coriolis and lorentz forces: The case of maxwell dusty fluid. Nanomaterials 2022, 12, 1512. [Google Scholar] [CrossRef]
- Rehman, S.U.; Mariam, A.; Ullah, A.; Asjad, M.I.; Bajuri, M.Y.; Pansera, B.A.; Ahmadian, A. Numerical computation of buoyancy and radiation effects on mhd micropolar nanofluid flow over a stretching/shrinking sheet with heat source. Case Stud. Therm. Eng. 2021, 25, 100867. [Google Scholar] [CrossRef]
- Wang, C. Stretching a surface in a rotating fluid. Z. Angew. Math. Phys. 1988, 39, 177–185. [Google Scholar] [CrossRef]
- Butt, A.S.; Ali, A.; Mehmood, A. Study of flow and heat transfer on a stretching surface in a rotating casson fluid. Proc. Natl. Acad. Sci. India Sect. A Phys. Sci. 2015, 85, 421–426. [Google Scholar] [CrossRef]
- Ali, B.; Nie, Y.; Hussain, S.; Manan, A.; Sadiq, M.T. Unsteady magneto-hydrodynamic transport of rotating maxwell nanofluid flow on a stretching sheet with cattaneo–christov double diffusion and activation energy. Therm. Sci. Eng. Prog. 2020, 20, 100720. [Google Scholar] [CrossRef]
- Pastoriza-Gallego, M.J.; Lugo, L.; Legido, J.L.; Piñeiro, M.M. Thermal conductivity and viscosity measurements of ethylene glycol-based Al2O3 nanofluids. Nanoscale Res. Lett. 2011, 6, 1–11. [Google Scholar] [CrossRef] [PubMed]
- Pastoriza-Gallego, M.J.; Casanova, C.; Legido, J.A.; Piñeiro, M.M. Cuo in water nanofluid: Influence of particle size and polydispersity on volumetric behaviour and viscosity. Fluid Phase Equilibria 2011, 300, 188–196. [Google Scholar] [CrossRef]



| Physical Features | Density () | Specific Heat () | Thermal Conductivity () |
|---|---|---|---|
| HO | 0991.1 | 4179.0 | 00.613 |
| Cu | 8933.0 | 0385.0 | 0401.0 |
| Properties | Nanofluid |
|---|---|
| Viscosity | |
| Density | |
| Heat capacity | |
| Thermal conductivity (k) | |
| Electrical conductivity |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ali, B.; Ahammad, N.A.; Awan, A.U.; Oke, A.S.; Tag-ElDin, E.M.; Shah, F.A.; Majeed, S. The Dynamics of Water-Based Nanofluid Subject to the Nanoparticle’s Radius with a Significant Magnetic Field: The Case of Rotating Micropolar Fluid. Sustainability 2022, 14, 10474. https://doi.org/10.3390/su141710474
Ali B, Ahammad NA, Awan AU, Oke AS, Tag-ElDin EM, Shah FA, Majeed S. The Dynamics of Water-Based Nanofluid Subject to the Nanoparticle’s Radius with a Significant Magnetic Field: The Case of Rotating Micropolar Fluid. Sustainability. 2022; 14(17):10474. https://doi.org/10.3390/su141710474
Chicago/Turabian StyleAli, Bagh, N. Ameer Ahammad, Aziz Ullah Awan, Abayomi S. Oke, ElSayed M. Tag-ElDin, Farooq Ahmed Shah, and Sonia Majeed. 2022. "The Dynamics of Water-Based Nanofluid Subject to the Nanoparticle’s Radius with a Significant Magnetic Field: The Case of Rotating Micropolar Fluid" Sustainability 14, no. 17: 10474. https://doi.org/10.3390/su141710474
APA StyleAli, B., Ahammad, N. A., Awan, A. U., Oke, A. S., Tag-ElDin, E. M., Shah, F. A., & Majeed, S. (2022). The Dynamics of Water-Based Nanofluid Subject to the Nanoparticle’s Radius with a Significant Magnetic Field: The Case of Rotating Micropolar Fluid. Sustainability, 14(17), 10474. https://doi.org/10.3390/su141710474







