Fourth-Party Logistics Environmental Compliance Management: Investment and Logistics Audit
Abstract
:1. Introduction
- Strategies for the 3PL to make more efforts. The 4PL can use different strategies to motivate the 3PL to make efforts. Firstly, when the investment cost is lower, the 4PL can incentivize the 3PL to make efforts by investment. Secondly, when the investment cost is higher, the 4PL can incentivize the 3PL to make efforts by offering a high enough wholesale price that it incurs a potential loss if it loses the contract with the 4PL. Thirdly, the 4PL can use low investment and medium wholesale price to deter the 3PL from not making efforts, which should only be used in the commitment case, as the 3PL would like to adopt the medium wholesale price and make efforts only if it knows the 4PL’s investment.
- Factors affecting the 4PL’s strategies. Increasing the goodwill cost can improve the 3PL’s efforts. However, reducing the investment cost does not necessarily increase the 3PL’s efforts, as the 4PL may replace the price premium with an investment strategy. When the investment cost is higher, the 4PL is faced with high goodwill cost, and will offer a high wholesale price to increase the 3PL’s efforts. When the investment is lower, the 4PL may rely solely on the investment to improve the 3PL’s efforts, which could lead to a decrease in the 3PL’s efforts, since the investment strategy only partially increases the 3PL’s efforts. In addition, increasing the audit level can increase the 3PL’s effort level when its rectification costs are sufficiently high.
- The commitment to investment effort. Commitment to investment effort certainly improves the final logistics compliance level. The 4PL’s commitment to investment effort can help the 3PL make efforts by using a high-investment and medium wholesale price strategy. In addition, a commitment to investment effort enables the 4PL to use a high-investment strategy, which can complement the 3PL’s efforts.
2. Literature Review
2.1. Sustainability Supply Chain Management
2.2. Audits in the Supply Chain
2.3. Logistics Service Supply Chain Management
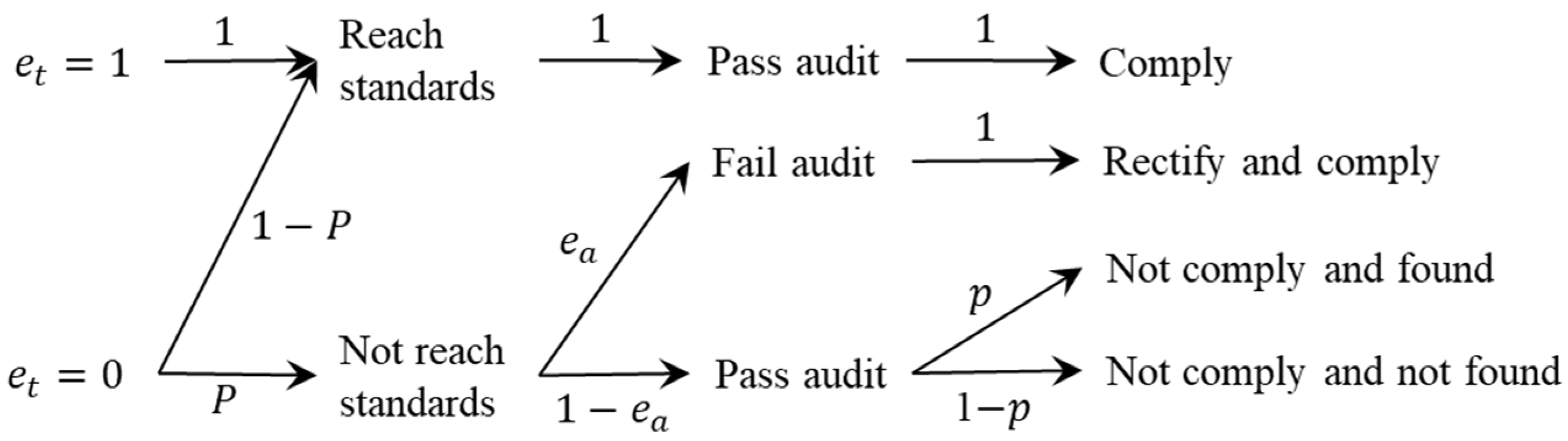
3. The Model
4. Base Model
- The 4PL provided a wholesale price contract to the 3PL;
- The 3PL accepted the contract if its expected profits were higher than the reservation profit;
- The 3PL chose the efforts level, , and delivered, whereas the 4PL chose its investment level, ev, and invested; we assumed that their decisions were simultaneous.
- The 4PL conducted the process audit.
- After the delivery was completed, the 4PL paid to the 3PL, and may have suffered from goodwill cost, .
4.1. Equilibrium in a Subgame
4.2. Subgame-Perfect Equilibrium
- (i)
- is decreasing in;
- (ii)
- If , is increasing in ; if , is decreasing in ;
- (iii)
- If , is increasing in ; If , is decreasing in .
5. Commitment to the 4PL Effort
- The 4PL provided a wholesale price, , and committed the investment level, , to the 3PL;
- The 3PL accepted the contract if its expected profits were higher than the reservation profit;
- The 3PL chose the effort level, , and delivered, whereas the 4PL conducted its investment;
- The 4PL conducted a process audit;
- After the delivery was over, the 4PL paid to the 3PL, and could suffer from the goodwill cost, .
5.1. Subgame-Perfect Equilibrium
- (i)
- When , the subgame-perfect equilibrium in the commitment scenario is:
- ii
- When , the subgame-perfect equilibrium in the commitment scenario is:
- (i)
- When , :
- (a)
- If the rectification cost , then and are increasing in the audit level, while ,, are decreasing in the audit level;
- (b)
- If the rectification cost , then and are decreasing in the audit level, while ,, are increasing in the audit level.
- (ii)
- When :
- (a)
- If the rectification cost , then , and are increasing in the audit level, while and are decreasing in the audit level;
- (b)
- If the rectification cost , then , and are decreasing in the audit level, while and are increasing in the audit level.
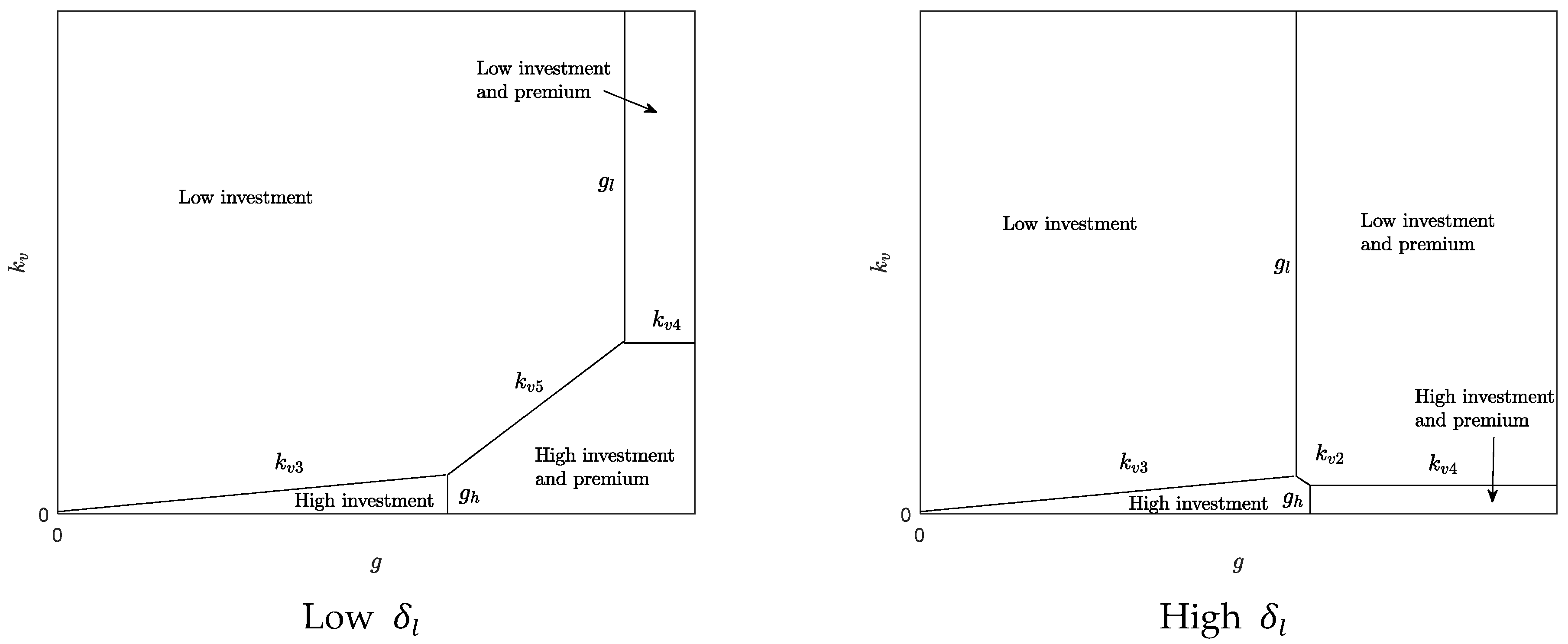
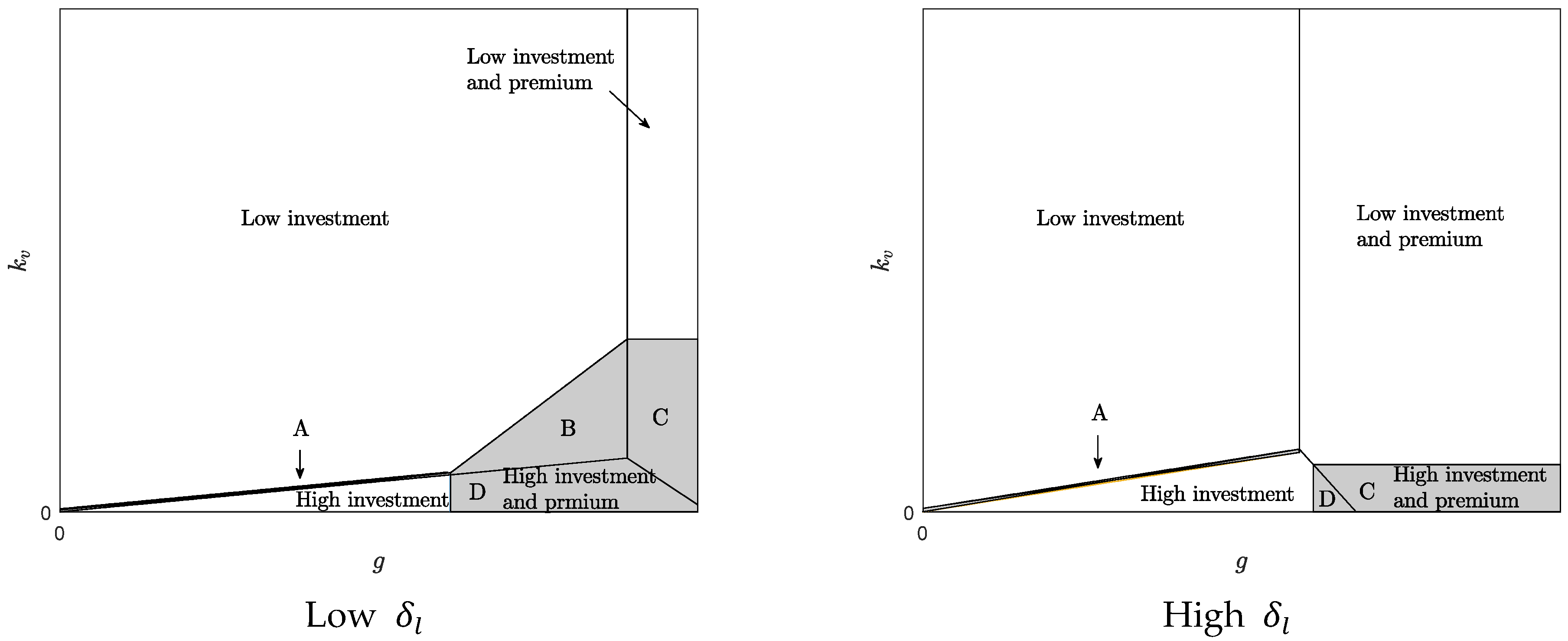
5.2. Effects of Commitment
- , low-investment high-investment.
- , low-investment high-investment-and-premium.
- , low-investment-and premium high-investment-and-premium.
- , high-investment high-investment-and-premium.
- (i)
- The 3PL’s profit remains unchanged in A, decreases in C, and increases in B and D.
- (ii)
- The 4PL’s profit increases in all regions.
- (iii)
- The efforts made by the 3PL increase in A, B, and D, and remain the same in C.
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A
- (i)
- From , we obtained , and from , we obtained , since , we obtained ;
- (ii)
- .
- (i)
- If , the 4PL chooses when
- (ii)
- If , the 4PL chooses when
Appendix B
Appendix C
- (i)
- , , for ;
- (ii)
- , , for .
- (iii)
- , , for .
- (a)
- If , program [21] can be simplified as follows:
- (i)
- is the equilibrium outcome if, and only if, and
- (ii)
- is the equilibrium outcome if, and only if, , and
- (iii)
- is the equilibrium outcome if, and only if, , and
- (iv)
- is the equilibrium outcome if, and only if, and .
- (b)
- If , we obtained:
- (i)
- is the equilibrium outcome if, and only if, and
- (ii)
- is the equilibrium outcome if, and only if, , and ;
- (iii)
- is the equilibrium outcome if, and only if, , and
- (iv)
- is the equilibrium outcome if, and only if, , and . □
Appendix D
- (i)
- When , from , we obtained ; From , if , i.e., , we obtained ; From , we obtained , then is in the interval . We let .
- (ii)
- When , from , we obtained ; From , we had ; From , if , we obtained ; then is in the interval . We let .
References
- Bové, A.-T.; Swartz, S. Starting at the Source: Sustainability in Supply Chains. Available online: https://www.mckinsey.com/business-functions/sustainability/our-insights/starting-at-the-source-sustainability-in-supply-chains (accessed on 30 May 2022).
- He, N.; Jiang, Z.-Z.; Wang, J.; Sun, M.; Xie, G. Maintenance optimisation and coordination with fairness concerns for the service-oriented manufacturing supply chain. Enterp. Inf. Syst. 2021, 15, 694–724. [Google Scholar] [CrossRef]
- Chau, K.-Y.; Tang, Y.M.; Liu, X.; Ip, Y.-K.; Tao, Y. Investigation of critical success factors for improving supply chain quality management in manufacturing. Enterp. Inf. Syst. 2021, 15, 1418–1437. [Google Scholar] [CrossRef]
- PLS Blog. Sustainable Supply Chain: 3 Benefits of Green Logistics. Available online: https://www.plslogistics.com/blog/sustainable-supply-chain-3-benefits-of-green-logistics (accessed on 30 May 2022).
- Interlake Mecalux. Green Logistics: Definition, Challenges and Solutions. Available online: https://www.interlakemecalux.com/blog/green-logistics (accessed on 30 May 2022).
- Qian, X.; Fang, S.-C.; Yin, M.; Huang, M.; Li, X. Selecting green third party logistics providers for a loss-averse fourth party logistics provider in a multiattribute reverse auction. Inf. Sci. 2021, 548, 357–377. [Google Scholar] [CrossRef]
- Alizila Staff. Cainiao Unveils ‘Green Logistics’ Master Plan. Available online: https://www.alizila.com/cainiao-unveils-green-logistics-master-plan/ (accessed on 30 May 2022).
- Zarbakhshnia, N.; Soleimani, H.; Goh, M.; Razavi, S.S. A novel multi-objective model for green forward and reverse logistics network design. J. Clean. Prod. 2019, 208, 1304–1316. [Google Scholar] [CrossRef]
- Aktas, E.; Bloemhof, J.M.; Fransoo, J.C.; Günther, H.-O.; Jammernegg, W. Green logistics solutions. Flex. Serv. Manuf. J. 2018, 30, 363–365. [Google Scholar] [CrossRef]
- Ecostars Europe Blog. Why and How Carry out a Logistics Audit. Available online: http://www.ecostars-europe.eu/en/logistics-audit (accessed on 30 May 2022).
- Orji, I.J.; U-Dominic, C.M.; Okwara, U.K. Exploring the determinants in circular supply chain implementation in the Nigerian manufacturing industry. Sustain. Prod. Consump. 2022, 29, 761–776. [Google Scholar] [CrossRef]
- PwC. Sustainability Assurance. Available online: https://www.pwccn.com/en/services/sustainability-and-climate-change/sustainability-assurance.html (accessed on 30 May 2022).
- Khalid, M.K.; Agha, M.H.; Shah, S.T.H.; Akhtar, M.N. Conceptualizing Audit Fatigue in the Context of Sustainable Supply Chains. Sustainability 2020, 12, 9135. [Google Scholar] [CrossRef]
- Lee, H.-H.; Li, C. Supplier Quality Management: Investment, Inspection, and Incentives. Prod. Oper. Manag. 2018, 27, 304–322. [Google Scholar] [CrossRef]
- Rodrigue, J.-P. The Benefits of Logistics Investments Opportunities for Latin America and the Caribbean. Available online: https://publications.iadb.org/publications/english/document/The-Benefits-of-Logistics-Investments-Opportunities-for-Latin-America-and-the-Caribbean.pdf (accessed on 1 October 2021).
- Chen, L.; Lee, H.L. Sourcing under supplier responsibility risk: The effects of certification, audit, and contingency payment. Manage. Sci. 2017, 63, 2795–2812. [Google Scholar] [CrossRef]
- Niu, B.; Mu, Z. Sustainable efforts, procurement outsourcing, and channel co-opetition in emerging markets. Transp. Res. Part E Logist. Transp. Rev. 2020, 138, 101960. [Google Scholar] [CrossRef]
- Nikoofal, M.E.; Gümüş, M. Quality at the source or at the end? Managing supplier quality under information asymmetry. M&SOM-Manuf. Serv. Oper. Manag. 2018, 20, 498–516. [Google Scholar] [CrossRef]
- Seuring, S.; Müller, M. From a literature review to a conceptual framework for sustainable supply chain management. J. Clean. Prod. 2008, 16, 1699–1710. [Google Scholar] [CrossRef]
- Elkington, J. Enter the Triple Bottom Line, 1st ed.; Earthscan: London, UK, 2004. [Google Scholar]
- Qorri, A.; Gashi, S.; Kraslawski, A. A practical method to measure sustainability performance of supply chains with incomplete information. J. Clean. Prod. 2022, 341, 130707. [Google Scholar] [CrossRef]
- Ilgin, M.A.; Gupta, S.M. Environmentally conscious manufacturing and product recovery (ECMPRO): A review of the state of the art. J. Environ. Manag. 2010, 91, 563–591. [Google Scholar] [CrossRef]
- Min, H.; Kim, I. Green supply chain research: Past, present, and future. Logist. Res. 2012, 4, 39–47. [Google Scholar] [CrossRef]
- Krass, D.; Nedorezov, T.; Ovchinnikov, A. Environmental taxes and the choice of green technology. Prod. Oper. Manag. 2013, 22, 1035–1055. [Google Scholar] [CrossRef]
- Choi, T.-M.; Chiu, C.-H. Mean-downside-risk and mean-variance newsvendor models: Implications for sustainable fashion retailing. Int. J. Product. Econ. 2012, 135, 552–560. [Google Scholar] [CrossRef]
- Niu, B.; Dai, Z.; Liu, Y.; Jin, Y. The role of Physical Internet in building trackable and sustainable logistics service supply chains: A game analysis. Int. J. Product. Econ. 2022, 247, 108438. [Google Scholar] [CrossRef]
- Gouda, S.K.; Saranga, H. Sustainable supply chains for supply chain sustainability: Impact of sustainability efforts on supply chain risk. Int. J. Product. Res. 2018, 56, 5820–5835. [Google Scholar] [CrossRef]
- Christmann, P. Effects of “Best Practices” of Environmental Management on Cost Advantage: The Role of Complementary Assets. Acad. Manag. J. 2000, 43, 663–680. [Google Scholar] [CrossRef]
- Sodhi, M.S.; Tang, C.S. Corporate social sustainability in supply chains: A thematic analysis of the literature. Int. J. Product. Res. 2018, 56, 882–901. [Google Scholar] [CrossRef]
- Morais, D.; Gaspar, P.D.; Silva, P.D.; Andrade, L.P.; Nunes, J. Energy consumption and efficiency measures in the Portuguese food processing industry. J. Food Processing Preserv. 2020, 2020, e14862. [Google Scholar] [CrossRef]
- Caro, F.; Chintapalli, P.; Rajaram, K.; Tang, C.S. Improving Supplier Compliance Through Joint and Shared Audits with Collective Penalty. M&SOM-Manuf. Serv. Oper. Manag. 2018, 20, 363–380. [Google Scholar] [CrossRef]
- Plambeck, E.L.; Taylor, T.A. Supplier Evasion of a Buyer’s Audit: Implications for Motivating Supplier Social and Environmental Responsibility. M&SOM-Manuf. Serv. Oper. Manag. 2016, 18, 184–197. [Google Scholar] [CrossRef]
- Nikoofal, M.E.; Gümüş, M. Value of audit for supply chains with hidden action and information. Eur. J. Oper. Res. 2020, 285, 902–915. [Google Scholar] [CrossRef]
- Wang, Y.; Wallace, S.W.; Shen, B.; Choi, T.-M. Service supply chain management: A review of operational models. Eur. J. Oper. Res. 2015, 247, 685–698. [Google Scholar] [CrossRef]
- De, A.; Singh, S.P. A resilient pricing and service quality level decision for fresh agri-product supply chain in post-COVID-19 era. Int. J. Logist. Manag. 2021; ahead-of-print. [Google Scholar] [CrossRef]
- Zhang, Y.; Li, X.; Wang, L.; Zhao, X.; Gao, J. Financing capital-constrained third party logistic firms: Fourth party logistic driven financing mode vs. private lending driven financing mode. Int. J. Product. Res. 2021, 60, 2963–2982. [Google Scholar] [CrossRef]
- Wu, Q.; Mu, Y.; Feng, Y. Coordinating contracts for fresh product outsourcing logistics channels with power structures. Int. J. Product. Econ. 2015, 160, 94–105. [Google Scholar] [CrossRef]
- Gong, F.; Kung, D.S.; Zeng, T. The impact of different contract structures on IT investment in logistics outsourcing. Int. J. Product. Econ. 2018, 195, 158–167. [Google Scholar] [CrossRef]
- So, K.C. Price and time competition for service delivery. Manuf. Serv. Oper. Manag. 2000, 2, 392–409. [Google Scholar] [CrossRef]
- Huang, M.; Tu, J.; Chao, X.; Jin, D. Quality risk in logistics outsourcing: A fourth party logistics perspective. Eur. J. Oper. Res. 2019, 276, 855–879. [Google Scholar] [CrossRef]
- Liu, X.; Zhang, K.; Chen, B.; Zhou, J.; Miao, L. Analysis of logistics service supply chain for the One Belt and One Road initiative of China. Transp. Res. Part E Logist. Transp. Rev. 2018, 117, 23–39. [Google Scholar] [CrossRef]
- Liu, W.; Yan, X.; Wei, W.; Xie, D.; Wang, D. Altruistic preference for investment decisions in the logistics service supply chain. Eur. J. Ind. Eng. 2018, 12, 598–635. [Google Scholar] [CrossRef]
- Liu, W.; Xie, D.; Xu, X. Quality supervision and coordination of logistic service supply chain under multi-period conditions. Int. J. Product. Econ. 2013, 142, 353–361. [Google Scholar] [CrossRef]
- Kremer, M. The O-Ring Theory of Economic Development. Q. J. Econ. 1993, 108, 551–575. [Google Scholar] [CrossRef]
- Schaefer, S. Product design partitions with complementary components. J. Econ. Behav. Organ. 1999, 38, 311–330. [Google Scholar] [CrossRef]
- Siggelkow, N. Misperceiving Interactions Among Complements and Substitutes: Organizational Consequences. Manag. Sci. 2002, 48, 900–916. [Google Scholar] [CrossRef]
- Cho, S.-H.; Fang, X.; Tayur, S.; Xu, Y. Combating Child Labor: Incentives and Information Disclosure in Global Supply Chains. M&SOM-Manuf. Serv. Oper. Manag. 2019, 21, 692–711. [Google Scholar] [CrossRef]


| 4PL’s Investment Level | |||
|---|---|---|---|
| evl | evh | ||
| 3PL’s effort level | et = 0 | γevl | γevh |
| et = 1 | 1 | 1 | |
| 4PL’s Investment Level | |||
|---|---|---|---|
| evl | evh | ||
| 3PL’s effort level | et = 0 | B − α − Pl(1 − ea)(1 − p)g (1 − δlPl(1 − ea)(1 − p))(α − Pleaφ) | B − α – Ph(1 − ea)(1 − p)g − kv (1 – δhPh(1 − ea)(1 − p))(α – Pheaφ) |
| et = 1 | B − α α − kt | B − α − kv α − kt | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, H.; Huang, M.; Wang, H. Fourth-Party Logistics Environmental Compliance Management: Investment and Logistics Audit. Sustainability 2022, 14, 10106. https://doi.org/10.3390/su141610106
Wang H, Huang M, Wang H. Fourth-Party Logistics Environmental Compliance Management: Investment and Logistics Audit. Sustainability. 2022; 14(16):10106. https://doi.org/10.3390/su141610106
Chicago/Turabian StyleWang, Hongyan, Min Huang, and Hongfeng Wang. 2022. "Fourth-Party Logistics Environmental Compliance Management: Investment and Logistics Audit" Sustainability 14, no. 16: 10106. https://doi.org/10.3390/su141610106
APA StyleWang, H., Huang, M., & Wang, H. (2022). Fourth-Party Logistics Environmental Compliance Management: Investment and Logistics Audit. Sustainability, 14(16), 10106. https://doi.org/10.3390/su141610106







