Sensitivity Analysis of Factors Affecting the Bearing Capacity of Suction Bucket Foundation in Soft Clay
Abstract
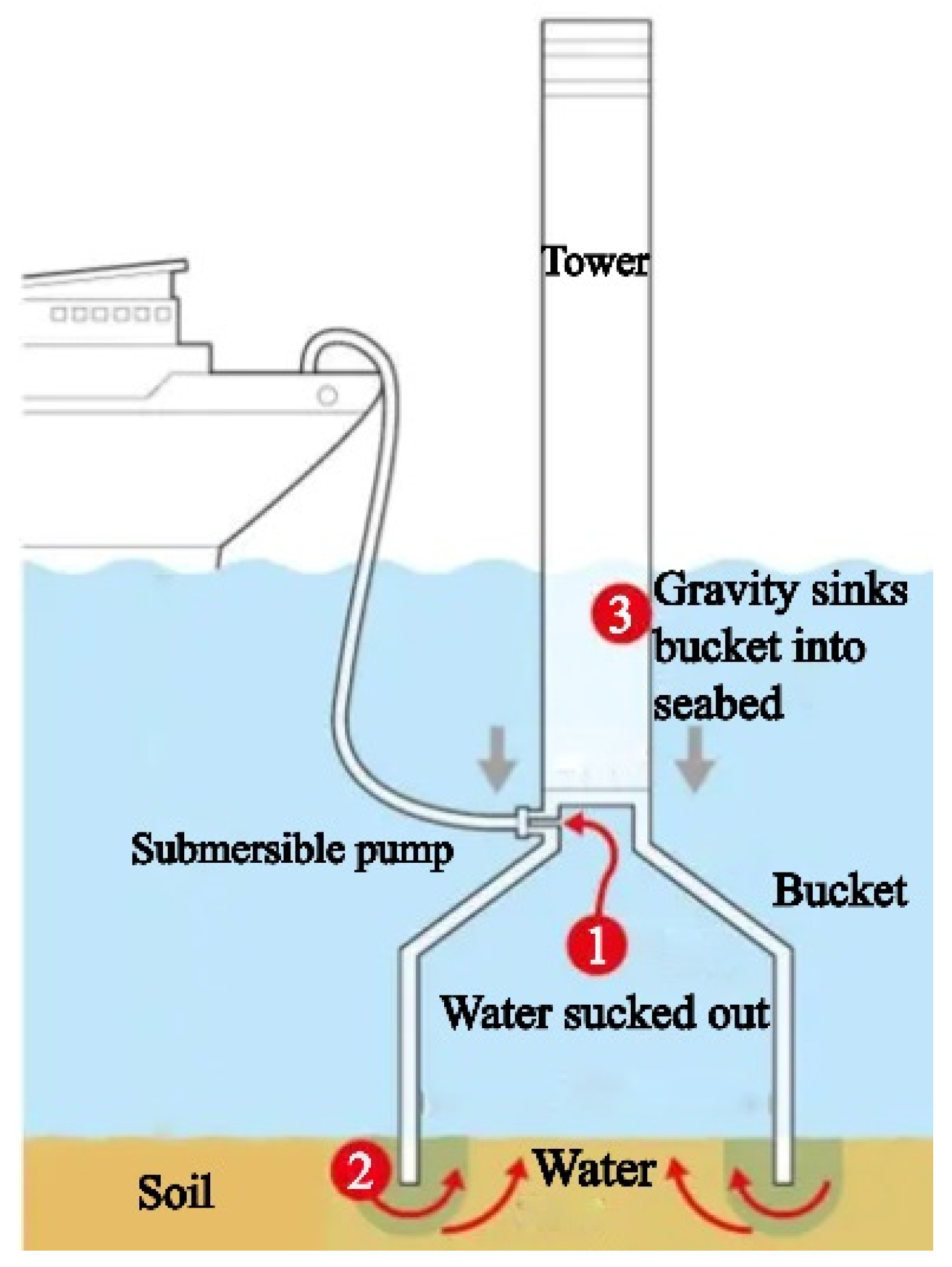
:1. Introduction
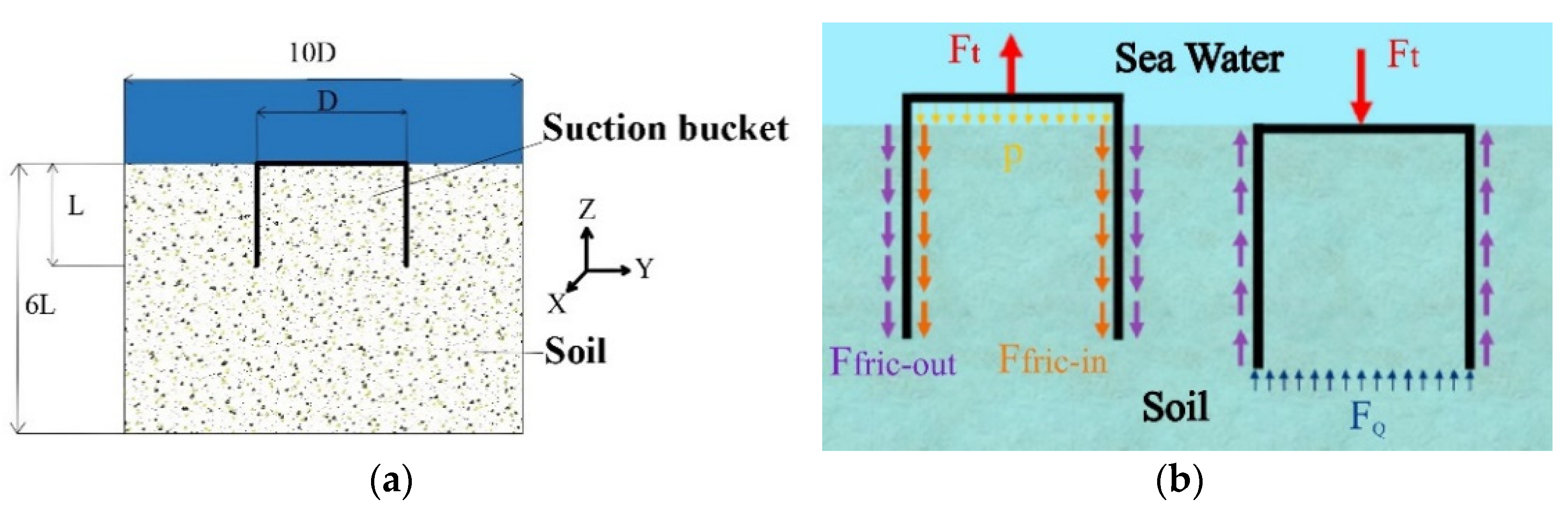
2. Establishment the Numerical Model
2.1. Overview of the Model
2.1.1. Parameters of the Model
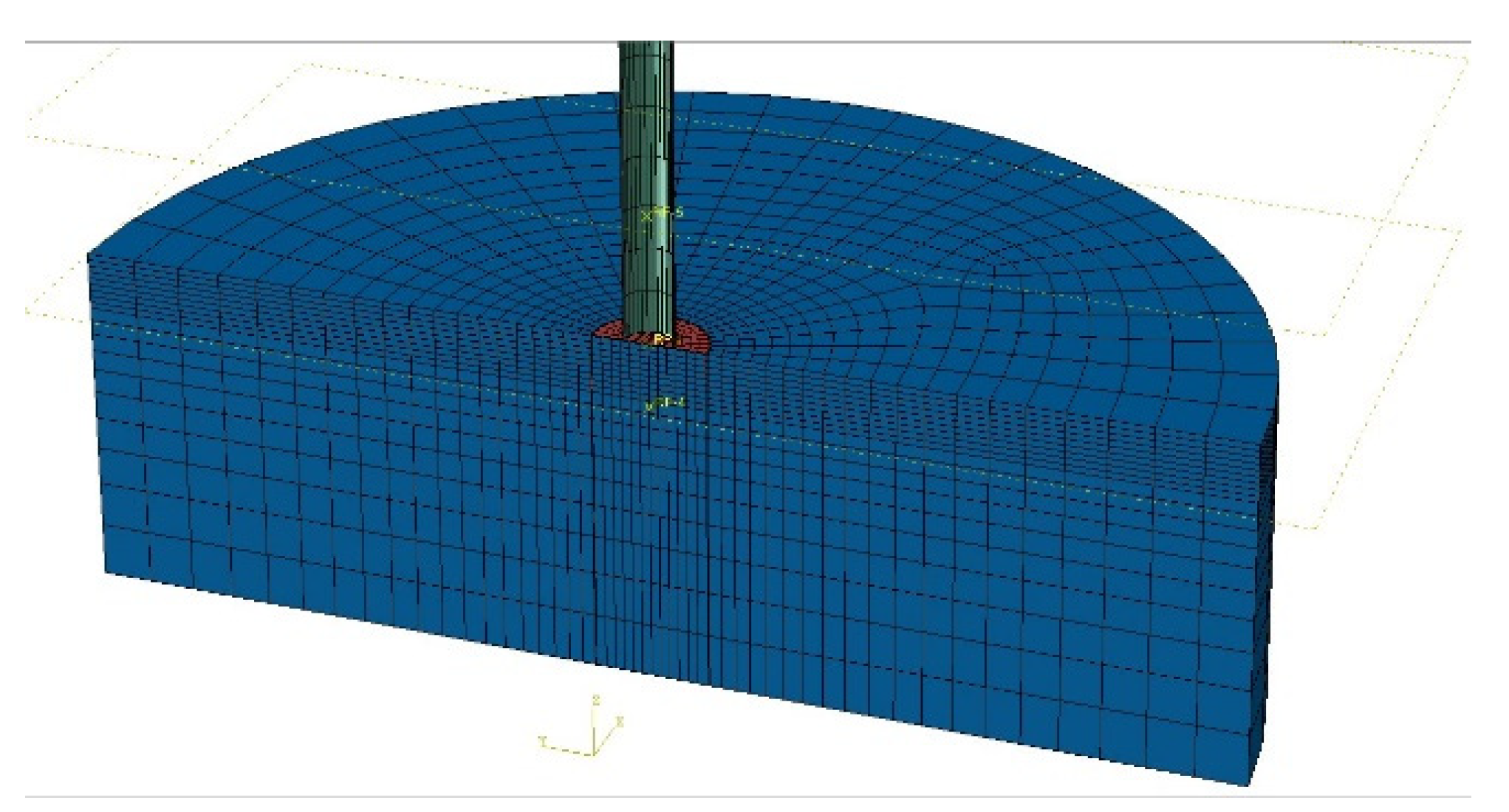
2.1.2. Mesh
2.1.3. Interaction between Contact Surfaces
2.1.4. Initial Geo–Stress Balance
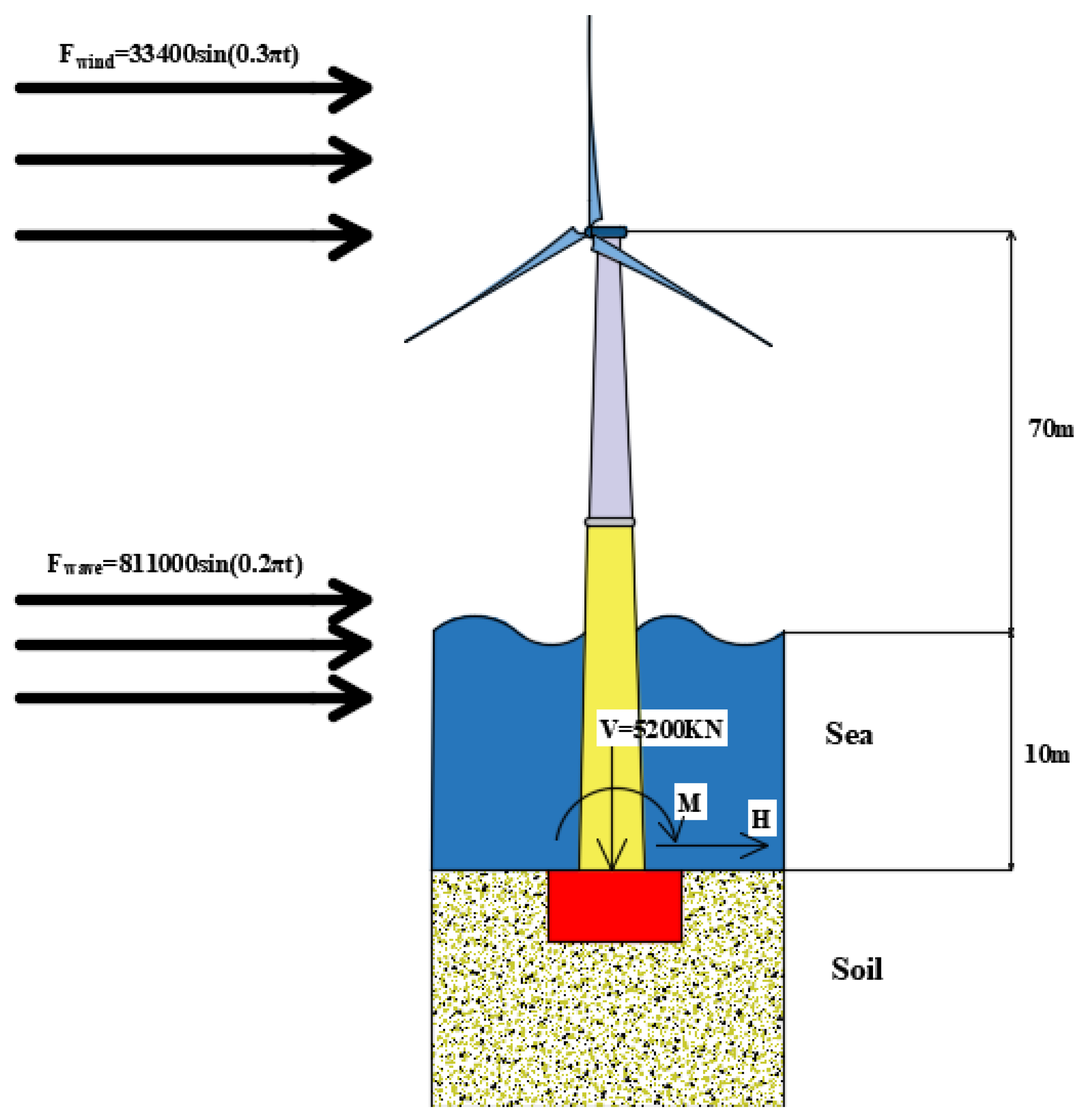
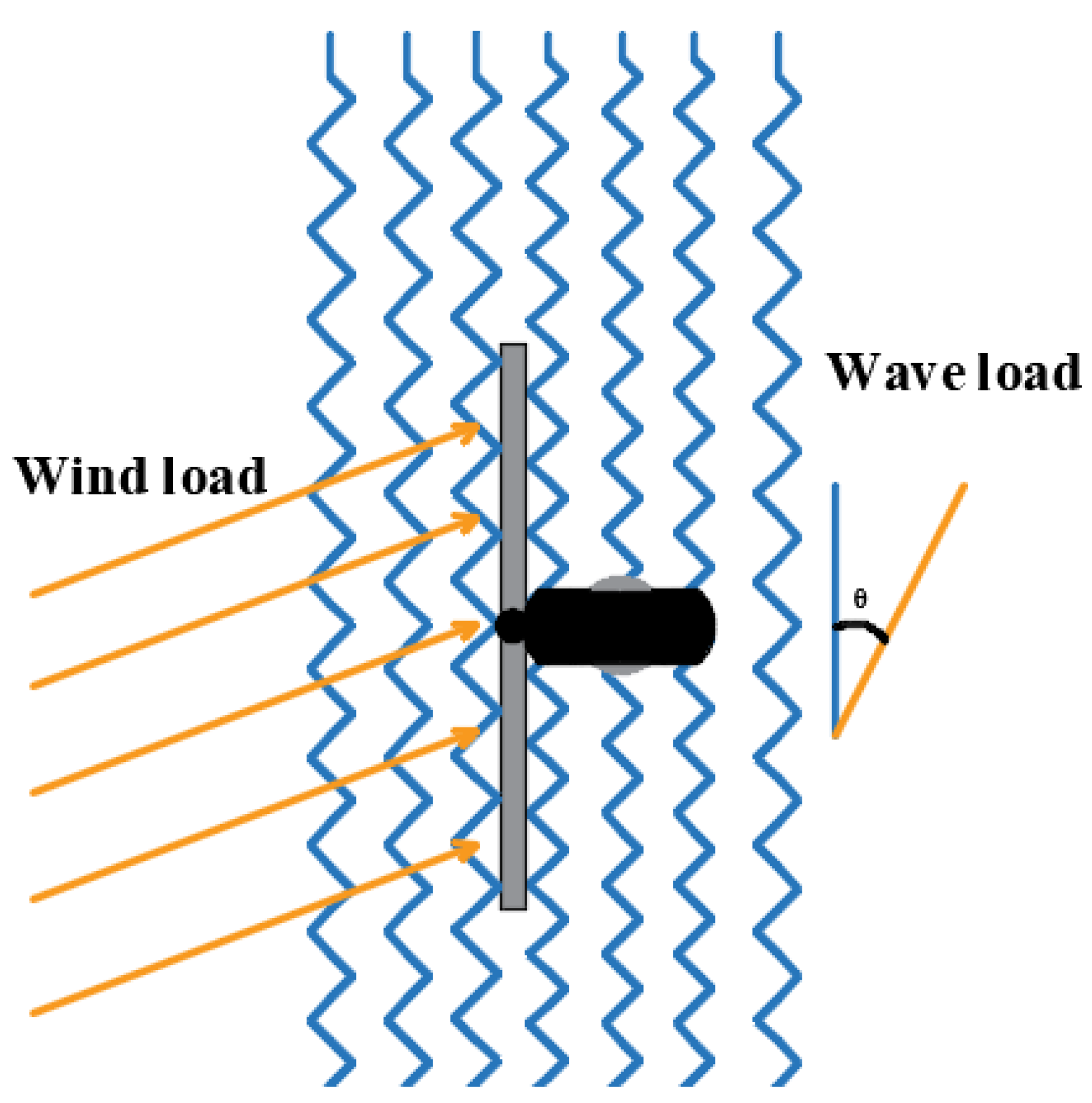
2.2. Expression of Load
2.3. Determination of Horizontal Cyclic Load
2.4. Verification of Finite Element Model
3. Results
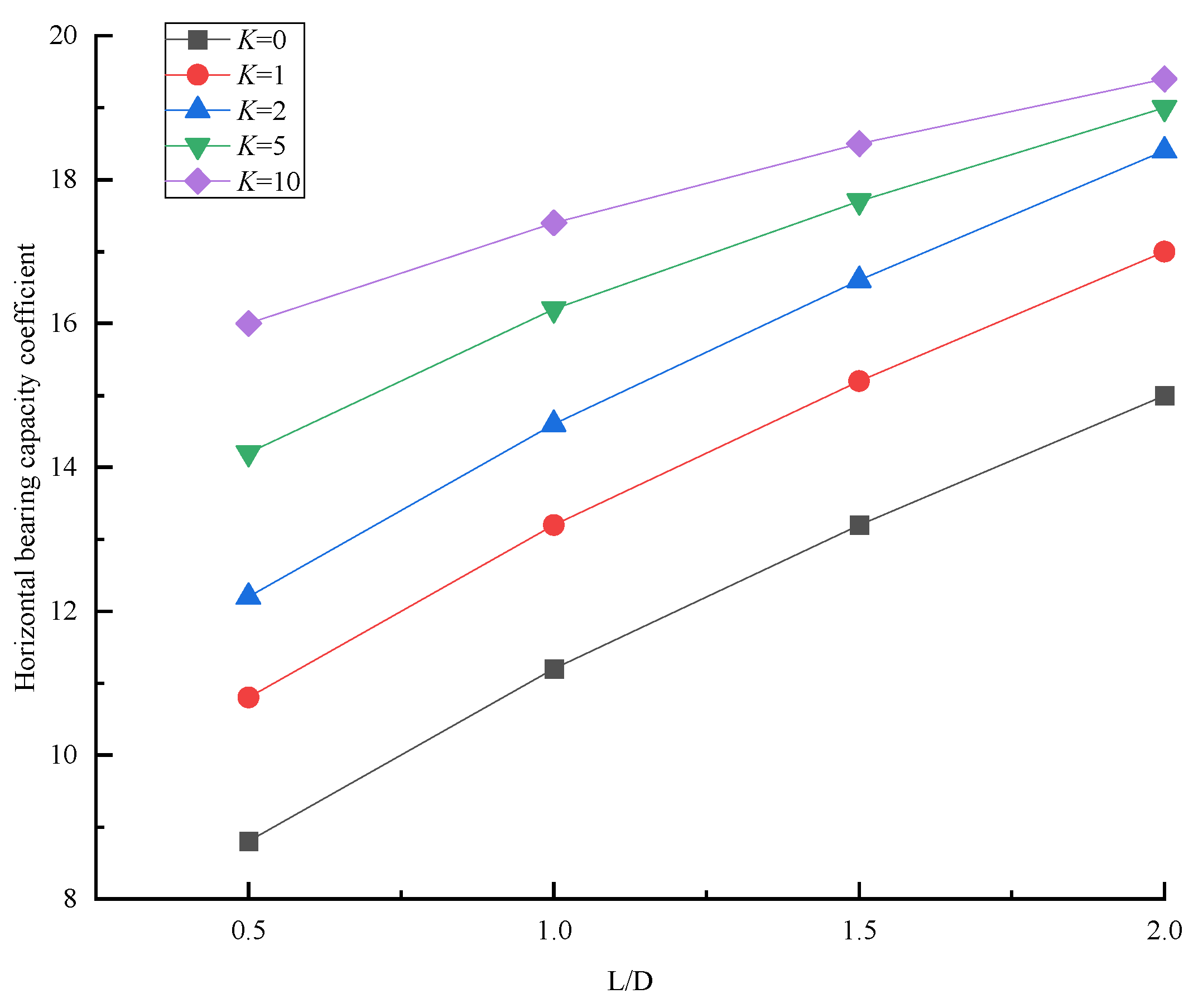
3.1. Bearing Capacity Characteristics with Different Length-Diameter Ratios
3.2. Bearing Capacity Characteristics with Different Soil Non-Uniformity Coefficients
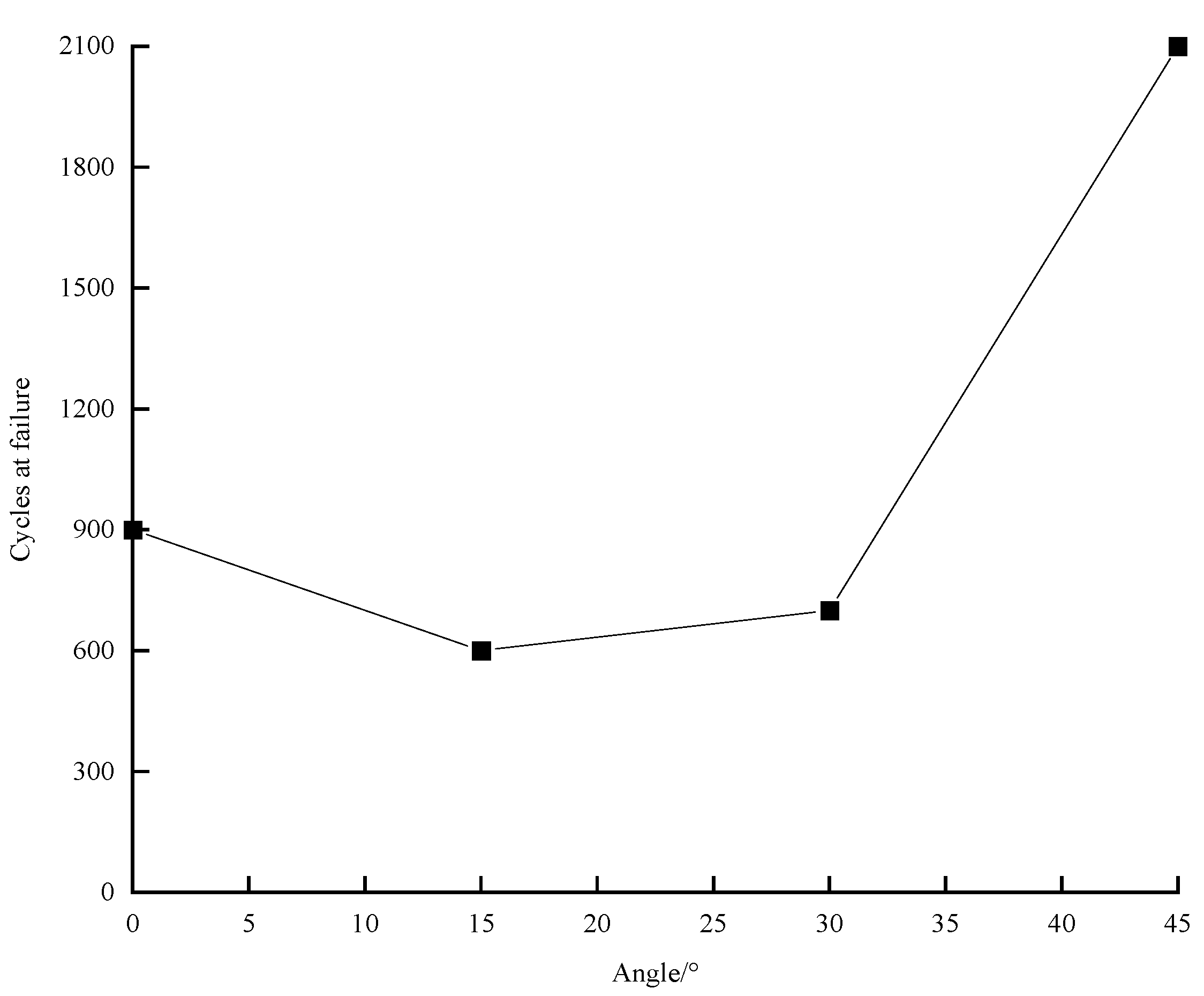
3.3. Bearing Capacity Characteristics with Different Angles between Wind and Wave Loads
3.4. Bearing Capacity Characteristics with Different Number of Cycles
4. Discussion
4.1. Sensitivity Analysis of Factor in Length–Diameter Ratios
4.2. Sensitivity Analysis of Factor in Soil Non-Uniformity Coefficients
4.3. Sensitivity Analysis of Factor in Angles between Wind and Wave Loads
4.4. Sensitivity Analysis of Factor in Number of Cycles
5. Conclusions
- The relationship between the horizontal bearing capacity coefficient and the length-diameter ratio and the soil unevenness coefficient has been established through numerical simulation, and the results show that the length–diameter ratio and the unevenness coefficient are positively correlated to the horizontal bearing capacity coefficient.
- When the length–diameter ratio of suction bucket is less than 1.0, the influence of the soil non-uniformity coefficient on the bearing capacity of the suction bucket foundation is greater than length–diameter ratio.
- When the length–diameter ratio of the suction bucket is more than 2.0, the influence of soil non-uniformity coefficient on the bearing capacity of the suction bucket foundation gradually diminishes.
- When the angle between wind and wave loads is 15° to 30°, the bearing capacity of the suction bucket foundation is lower than the bearing capacity when the wind and wave loads are collinear.
- The attenuation of soil strength is rapid within 60 cycles. Because the shallow soil layer is the primary provider of horizontal bearing capacity during the initial cycles, the rate of strength attenuation in the shallow soil is faster than that in the deep soil under initial cyclic loads.
- The attenuation of soil strength is slow after 100 cycles. Because the strength of shallow soil is relatively stable after 100 cycles, the reason for the subsequent slow strength attenuation is that the strength of deep soil decreases.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Zou, L.; Tang, H.Y.; Wang, Y.H.; Chen, P.; Huang, X.G.; Wu, W. Development of Foundation Structure Selection Program of Offshore Wind Turbines. Ship Eng. 2020, 42, 282–285. (In Chinese) [Google Scholar]
- Sun, Y.X. Experimental and Numerical Studies on a Laterally Loaded Monopile Foundation of Offshore Wind Turbine. Ph.D. Thesis, Zhejiang University, Zhejiang, China, 2016. (In Chinese). [Google Scholar]
- Xie, M.; Lopez-Querol, S. Numerical Simulations of the Monotonic and Cyclic Behavior of Offshore Wind Turbine Monopile Foundations in Clayey Soils. J. Mar. Sci. Eng. 2021, 9, 1036. [Google Scholar] [CrossRef]
- Amin, B.; Britta, B.; Domenico, L.; Shinji, S. Offshore Wind Turbine Foundations. Soils Found. 2021, 61, 621–622. [Google Scholar]
- Li, J.W.; Hu, G.Q.; Jin, G.Q. Hydrodynamic Performance of a Novel Floating Foundation for an Offshore Wind Turbine Under a Storm Condition. Int. J. Offshore Polar Eng. 2020, 30, 120–128. [Google Scholar] [CrossRef]
- Tjelta, T.L. Geotechnical Aspects of Bucket Foundations Replacing Piles for the Europipe 16/11-E Jacket. In Proceedings of the Offshore Technology Conference, Houston, TX, USA, 2 May 1994. [Google Scholar]
- Shi, X.C.; Xu, R.Q.; Gong, X.N.; Chen, G.X.; Yuan, Z.L. Introduction of Bucket Foundation. Chin. Civil Eng. J. 2000, 33, 68–92. (In Chinese) [Google Scholar]
- Yang, J.L.; Wang, S.F.; Kong, L.W.; Yuan, J.X.; Liu, Z.A.; Wang, Q. The 3-D Finite Element Analysis of Stability of Platform with Bucket Foundation. Rock Soil Mech. 2002, 23, 640–644. (In Chinese) [Google Scholar]
- Wu, K.; Xue, H.F.; Chen, R.; Li, S.C. Studies on Bearing Capacity of Composite Multi-Suction Bucket Structure of Suctional Suction Bucket Foundation. J. Disaster Prev. Mitigation Eng. 2008, 28, 484–491. (In Chinese) [Google Scholar]
- Li, D.Y.; Chen, Q.J.; Zhang, Y.K.; Chen, F.Q. Review of Horizontal Loading on Suction Caisson of Offshore Wind Turbines. Ocean Eng. 2020, 38, 137–147. (In Chinese) [Google Scholar]
- Wu, X.N.; Liao, Q.; Li, Y. A Review on the Bearing Capacity Studies of the Suction Bucket Foundation for Offshore Wind Turbines. J. Ocean Technol. 2020, 39, 91–106. (In Chinese) [Google Scholar]
- Jin, S.C.; Zhang, Y.T.; Yang, Y.H.; Li, B. Research on Horizontal Ultimate Bearing Capacity of Suction Bucket Foundation in Saturated Sand Ground in Saturated Sand Ground. Rock Soil Mech. 2013, 34, 221–227. (In Chinese) [Google Scholar]
- Sun, X.Y.; Luan, M.T.; Tang, X.W. Study of Horizontal Bearing Capacity of Bucket Foundation on Saturated Soft Clay Ground. Rock Soil Mech. 2010, 31, 667–672. (In Chinese) [Google Scholar]
- Wu, K.; Luan, M.T.; Fan, Q.L.; Wang, Z.Y. Numerical Analysis of Failure Envelopes of Bucket Foundation Subjected to Combined Loads Based on Elasto-plastic Fem. Eng. Mech. 2008, 25, 156–161. (In Chinese) [Google Scholar]
- Zhang, Y.K.; Wang, C.C.; Li, D.Y.; Qi, Y.S. Numerical Simulation of Bearing Characteristics of Modified Suction Cassions in Sand Under Combined Loads. Sci. Technol. Eng. 2021, 21, 1515–1521. (In Chinese) [Google Scholar]
- Wang, J.H.; Yang, H.M. Model test on Horizontal Cyclic Bearing Capacity of Bucket Foundations in Soft Clays. Rock Soil Mech. 2008, 29, 2606–2612. (In Chinese) [Google Scholar]
- Zhao, S.X.; Sun, B.B. Code for Design of Wind Turbine Foundations for Offshore Wind Power Projects (NB/T 10105-2018), 1st ed.; Yi, Y.C., Xie, H.W., Eds.; China Water & Power Press: Beijing, China, 2019; pp. 3–4. [Google Scholar]
- Hong, Z.B.; Tao, J.G.; Hu, D.; Li, F.; Xie, X.T. Research on the Stiffness Degradation Model of Saturated Soft Clay Seabed under Cyclic Loading. J. Railw. Sci. Eng. 2021, 18, 351–358. (In Chinese) [Google Scholar]
- Fan, Q.L. A Study on Stability of Deeply-Embedded Large-Diameter Cylindrical Structure in Soft Ground. Ph.D. Thesis, Dalian University of Technology, Liaoning, China, 2007. (In Chinese). [Google Scholar]
- Chen, G.; Beer, M.; Liu, Y. Modeling Response Spectrum Compatible Pulse-like Ground Motion. Mech. Syst. Signal Proc. 2022, 177, 109177. [Google Scholar] [CrossRef]
- Liu, J.C.; Xiong, G.; Zhu, B.; Ying, P.P. Bearing Capacity and Deflection Behaviors of Large Diameter Monopile Foundations in Sand Seabed. Rock Soil Mech. 2015, 36, 591–599. (In Chinese) [Google Scholar]
- Liu, Y.; Jiang, Y.; Xiao, H.; Lee, F.H. Determination of Representative Strength of Deep Cement-Mixed Clay from Core Strength Data. Géotechnique 2017, 67, 350–364. [Google Scholar] [CrossRef]
- Liu, Y.; Lee, F.H.; Quek, S.T.; Chen, E.J.; Yi, J.T. Effect of Spatial Variation of Strength and Modulus on the Lateral Compression Response of Cement-Admixed Clay Slab. Géotechnique 2015, 65, 851–865. [Google Scholar] [CrossRef]
- Wang, R.H.; Li, D.Q.; Chen, E.J.; Liu, Y. Dynamic Prediction of Mechanized Shield Tunneling Performance. Autom. Constr. 2021, 132, 103958. [Google Scholar] [CrossRef]
- Cao, J.F.; Shi, Y.P. Answers to Common Issues of Finite Element Analysis in ABAQUS, 1st ed.; Kong, J., Guo, J., Eds.; China Machine Press: Beijing, China, 2009; pp. 109–114. [Google Scholar]
- Jin, X.Y.; Wang, J. Load Code for the Design of Building Structures (GB50009-2012), 1st ed.; Chen, M.K., Wang, D.S., Eds.; China Architecture Publishing & Media Co., Ltd.: Beijing, China, 2012; pp. 30–62. [Google Scholar]
- Gelagoti, F.; Georgiou, I.; Kourkoulis, R.; Gazetas, G. Nonlinear Lateral Stiffness and Bearing Capacity of Suction Caissons for Offshore Wind-Turbines. Ocean Eng. 2018, 170, 445–465. [Google Scholar] [CrossRef]
- Zhou, S.J.; Zhang, Y.; Wang, D. Capacity Envelope of Caisson Foundation for on-bottom Pipelines. Mar. Sci. Bull. 2019, 38, 727–732. (In Chinese) [Google Scholar]
- Chen, X.J.; Li, D.Q.; Tang, X.S.; Liu, Y. A Three-Dimensional Large-Deformation Random Finite-Element Study of Landslide Runout Considering Spatially Varying Soil. Landslides 2021, 18, 3149–3162. [Google Scholar] [CrossRef]
- Liu, Y.; He, L.Q.; Jiang, Y.J.; Sun, M.M.; Chen, E.J.; Lee, F.H. Effect of in-Situ Water Content Variation on the Spatial Variation of Strength of Deep Cement-Mixed Clay. Géotechnique 2019, 69, 391–405. [Google Scholar] [CrossRef] [Green Version]
- Byrne, B.W.; Houlsby, G.T. Foundations for Offshore Wind Turbines. Philos. Trans. R. Soc. Lond. Ser. A 2003, 361, 2909–2930. [Google Scholar] [CrossRef]
- Seidel, M. Feasibility of Monopiles for Large Offshore Wind Turbines. In Proceedings of the 10th German Wind Energy Conference (DEWEK), Bremen, Germany, 17–18 November 2010. [Google Scholar]
- Fischer, T.; Rainey, P.; Bossanyi, E.; Kühn, M. Study on Control Concepts Suitable for Mitigation of Loads from Misaligned Wind and Waves on Offshore Wind Turbines Supported on Monopiles. Wind Eng. 2011, 35, 561–574. [Google Scholar] [CrossRef]
- Schaumann, P.; Lochte-Holtgreven, S.; Steppeler, S. Special Fatigue Aspects of Support Structures with Offshore Wind Turbines. Materialwiss. Werkstofftech. 2011, 42, 1075–1081. [Google Scholar] [CrossRef]
- Liu, Y.; Li, K.Q.; Li, D.Q.; Tang, X.S.; Gu, S.X. Coupled Thermal–Hydraulic Modeling of Artificial Ground Freezing with Uncertainties in Pipe Inclination and Thermal Conductivity. Acta Geotech. 2022, 17, 257–274. [Google Scholar] [CrossRef]





| Parameters | Elasticity Modulus/GPa | Poisson’s Ratio | Effective Weight/(KN·m−3) |
|---|---|---|---|
| Bucket | 210 | 0.125 | 23 |
| Parameters | Elasticity Modulus | Poisson’s Ratio | Effective Weight/(KN·m−3) | Cohesive Force/KPa | Internal Friction Angle/(°) | Dilatancy Angle/(°) |
|---|---|---|---|---|---|---|
| Soil | 500Su | 0.49 | 8 | 20 | 15 | 0.1 |
| K = pD/Su0 | p/(kPa·m−1) | Su0/kPa |
|---|---|---|
| 0 | 0 | 5 |
| 1 | 1 | 5 |
| 2 | 1.5 | 7.5 |
| 5 | 2 | 4 |
| 10 | 2.5 | 2.5 |
| Working Conditions | Wind Loading Direction | Wave Loading Direction |
|---|---|---|
| A | 0° | 0° |
| B | 0° | 15° |
| C | 0° | 30° |
| D | 0° | 45° |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, B.; Yuan, M.-H.; Li, L.; Yuan, C.-F.; Li, Y.; Shen, K.-M. Sensitivity Analysis of Factors Affecting the Bearing Capacity of Suction Bucket Foundation in Soft Clay. Sustainability 2022, 14, 9615. https://doi.org/10.3390/su14159615
Wang B, Yuan M-H, Li L, Yuan C-F, Li Y, Shen K-M. Sensitivity Analysis of Factors Affecting the Bearing Capacity of Suction Bucket Foundation in Soft Clay. Sustainability. 2022; 14(15):9615. https://doi.org/10.3390/su14159615
Chicago/Turabian StyleWang, Bin, Ming-Hui Yuan, Liang Li, Chang-Feng Yuan, Ying Li, and Kan-Min Shen. 2022. "Sensitivity Analysis of Factors Affecting the Bearing Capacity of Suction Bucket Foundation in Soft Clay" Sustainability 14, no. 15: 9615. https://doi.org/10.3390/su14159615
APA StyleWang, B., Yuan, M.-H., Li, L., Yuan, C.-F., Li, Y., & Shen, K.-M. (2022). Sensitivity Analysis of Factors Affecting the Bearing Capacity of Suction Bucket Foundation in Soft Clay. Sustainability, 14(15), 9615. https://doi.org/10.3390/su14159615






