Assessment of the Landfill Barrier System through Numerical Analysis: Rehabilitation and Expansion of Belgrade Landfill Case Study
Abstract
:1. Introduction
1.1. Vinča Landfill Rehabilitation and Expansion Plans
1.2. Geotechnical Aspects of the New Vinča Landfill Project
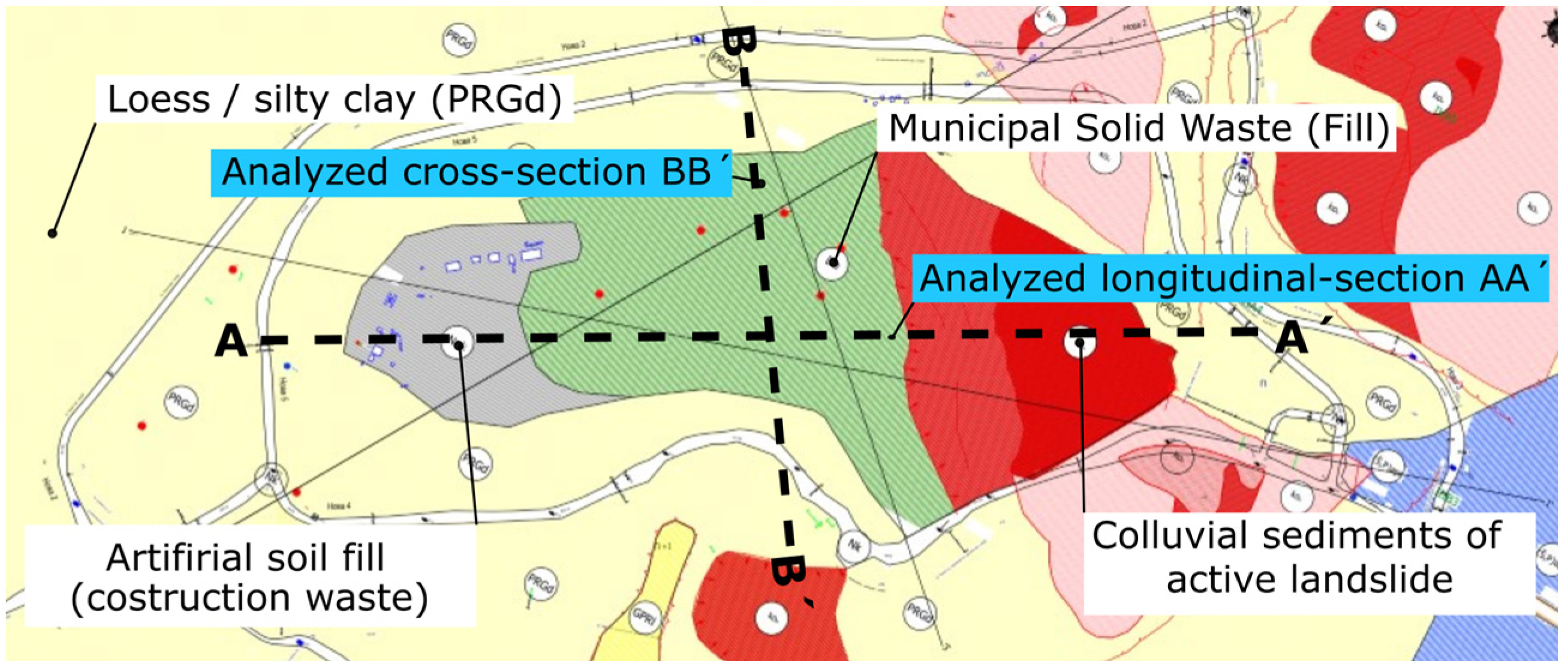
2. Geological and Geotechnical Conditions on the Site
2.1. Natural Soil Description
2.2. Hydrogeological Conditions at the Site
3. Numerical Calculation Assumptions
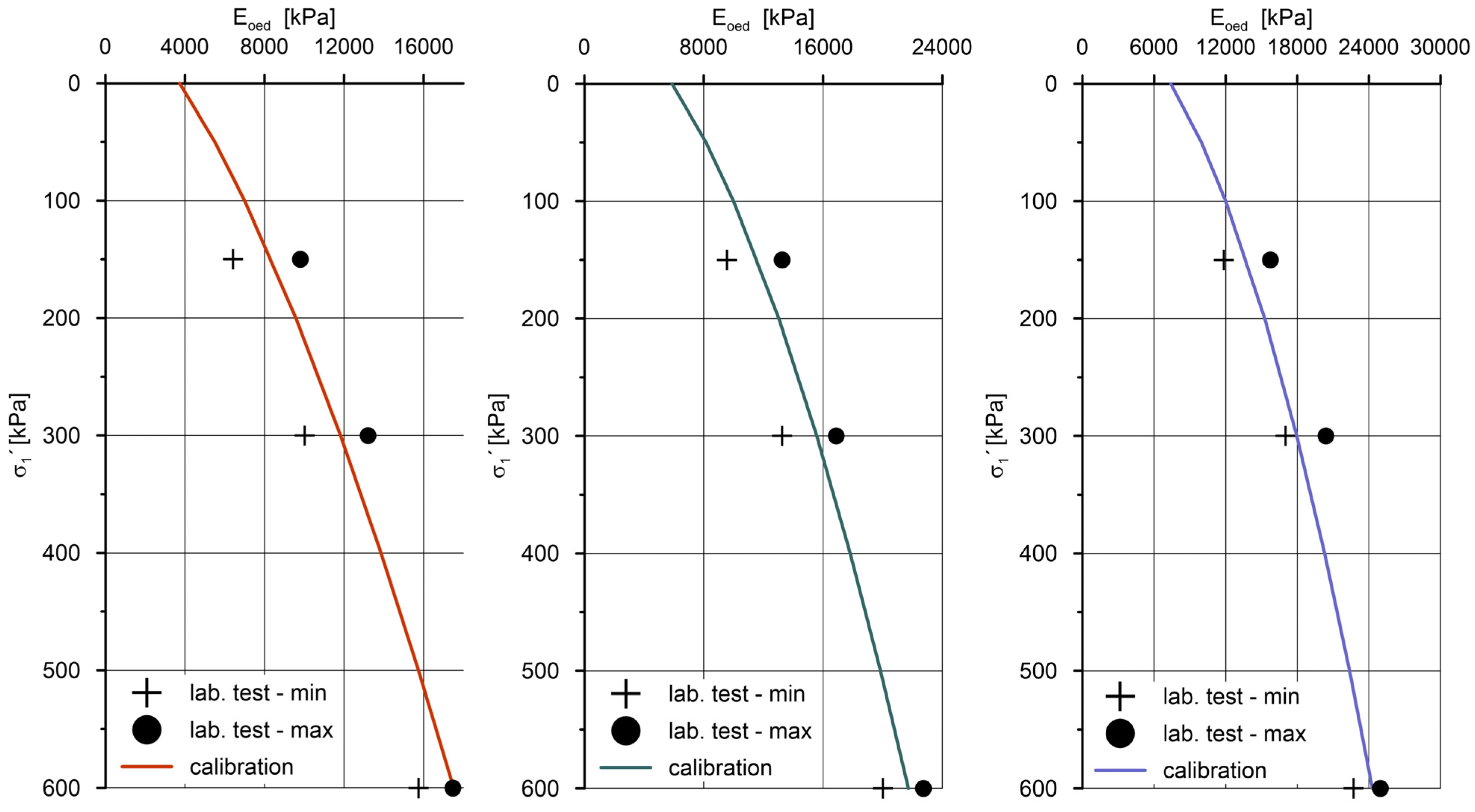
3.1. Material Models Used in Simulation
- The linear elastic—perfectly plastic Mohr–Coulomb MC model (MC);
- The Hardening Soil Model (HS)—an elastoplastic model with shear and volumetric hardening [68].
3.2. Input Values of Soil and Waste Material Parameters
4. Results
5. Discussion
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Ashford, S.A.; Visvanathan, C.; Husain, N.; Chomsurin, C. Design and construction of engineered municipal solid waste landfills in Thailand. Waste Manag. Res. J. Sustain. Circ. Econ. 2000, 18, 462–470. [Google Scholar] [CrossRef]
- Cvetkovic-Mrkic, S.; Janjic, I. Environmental aspects of interaction between structures and geologic milieu. In Proceedings of the International Symposium on Engineerin Geology and the Environment, Athens, Greece, 23 June 1997; The Greek International Group of IAEG. Volume 3, pp. 2647–2652. [Google Scholar]
- Samadder, S.; Prabhakar, R.; Khan, D.; Kishan, D.; Chauhan, M. Analysis of the contaminants released from municipal solid waste landfill site: A case study. Sci. Total Environ. 2017, 580, 593–601. [Google Scholar] [CrossRef] [PubMed]
- Cai, G.; Liu, S.; Tong, L.; Du, G. Assessment of direct CPT and CPTU methods for predicting the ultimate bearing capacity of single piles. Eng. Geol. 2009, 104, 211–222. [Google Scholar] [CrossRef]
- Han, Z.; Ma, H.; Shi, G.; He, L.; Wei, L.; Shi, Q. A review of groundwater contamination near municipal solid waste landfill sites in China. Sci. Total Environ. 2016, 569–570, 1255–1264. [Google Scholar] [CrossRef] [PubMed]
- Sarsby, R. Environmental Geotechnics, 2nd ed.; ICE Publishing: London, UK, 2013; pp. 231–284. [Google Scholar]
- Helping Belgrade Redevelop Its Previously Unmanaged Vinca Landfill, Including New Waste-to-Energy Project. Available online: https://www.arup.com/projects/vinca-landfill-redevelopment-serbia (accessed on 25 April 2022).
- Milanovic, A.; Kovacevic-Majkic, J.; Milivojevic, M. Water quality analysis of Danube river in Serbia: Pollution and protection problems. Glas. Srp. Geogr. Drus. 2010, 90, 47–68. [Google Scholar] [CrossRef]
- Brboric, M.; Stepanov, B.; Radonic, J.; Turk-Sekulic, M. Danube sediment contamination with polychlorinated biphenyls: New interpretation of sediment quality assessment. Acta Period. Technol. 2019, 43–50. [Google Scholar] [CrossRef] [Green Version]
- Sretenović, B.; Arnaut, F.; Vasiljević, I.; Cvetkov, V. 2D geoelectrical resistivity tomography application at the former city waste dump “Ada Huja”: Eco-geological problem. Podzemn. Rad. 2019, 59–76. [Google Scholar] [CrossRef]
- Bagchi, A. Design, Construction and Monitoring of Landfills, 2nd ed.; Wiley: New York, NY, USA, 1994; pp. 1–376. [Google Scholar]
- Karanac, M.; Jovanovic, M.; Timmermans, E.; Mulleneers, H.; Mihajlovic, M.; Jovanovic, J. Impermeable layers in landfill design. Hem. Ind. 2013, 67, 961–973. [Google Scholar] [CrossRef]
- Laner, D.; Crest, M.; Scharff, H.; Morris, J.; Barlaz, M. A review of approaches for the long-term management of municipal solid waste landfills. Waste Manag. 2012, 32, 498–512. [Google Scholar] [CrossRef]
- Josimović, B.; Marić, I.; Milijić, S. Multi-criteria evaluation in strategic environmental assessment for waste management plan, a case study: The city of Belgrade. Waste Manag. 2015, 36, 331–342. [Google Scholar] [CrossRef]
- Jovanovic, D.; Zivkovic, T. Public-private partnership as a possibility for improving municipal waste management. Spatium 2019, 41–48. [Google Scholar] [CrossRef] [Green Version]
- Nikolic, A.; Mikic, M.; Naunovic, Z. Broadening the urban sustainable energy diapason through energy recovery from waste: A feasibility study for the capital of Serbia. Renew. Sustain. Energy Rev. 2017, 69, 1–8. [Google Scholar] [CrossRef] [Green Version]
- Lee, U.; Han, J.; Wang, M. Evaluation of landfill gas emissions from municipal solid waste landfills for the life-cycle analysis of waste-to-energy pathways. J. Clean. Prod. 2017, 166, 335–342. [Google Scholar] [CrossRef]
- Alivojvodic, V.; Stanojevic, M.; Antonijevic, D. Belgrade solid waste management optimization—Potential of landfill gas recovery. Environ. Eng. Manag. J. 2015, 14, 2979–2984. [Google Scholar] [CrossRef]
- Ubavin, D.; Maoduš, N.; Milovanovič, D.; Stege, G.; Leatherwood, C. Preliminary estimate of methane production at Belgrade MSW landfill “Vinča”. In Proceedings of the 6th PSU-UNS International Conference on Engineering and Technology (ICET-2013), Novi Sad, Serbia, 15–17 May 2013. [Google Scholar]
- Beo Čista Energija d.o.o. Vinča Energy-From-Waste Facility, Construction of the New Landfill and Remediation of the Existing Landfill: Environmental and Social Impact Assessment, Non-Technical Sumary; Version 6; BC Beo Čista Energia: Beograd, Serbia, 2018; pp. 1–15. [Google Scholar]
- Pandey, L.; Shukla, S. An insight into waste management in Australia with a focus on landfill technology and liner leak detection. J. Clean. Prod. 2019, 225, 1147–1154. [Google Scholar] [CrossRef]
- Allen, A. Containment landfills: The myth of sustainability. Eng. Geol. 2001, 60, 3–19. [Google Scholar] [CrossRef]
- Rowe, R.; Sangam, H. Durability of HDPE geomembranes. Geotext. Geomembr. 2002, 20, 77–95. [Google Scholar] [CrossRef]
- Lavoie, F.; Kobelnik, M.; Valentin, C.; da Silva, J. Durability of HDPE Geomembranes: An Overview. Quím. Nova 2020, 43, 656–667. [Google Scholar] [CrossRef]
- Abdelaal, F.; Rowe, R.; Brachman, R. Brittle rupture of an aged HPDE geomembrane at local gravel indentations under simulated field conditions. Geosynth. Int. 2014, 21, 1–23. [Google Scholar] [CrossRef]
- Rowe, R.; Abdelaal, F. Antioxidant depletion in high-density polyethylene (HDPE) geomembrane with hindered amine light stabilizers (HALS) in low-pH heap leach environment. Can. Geotech. J. 2016, 53, 1612–1627. [Google Scholar] [CrossRef]
- Rowe, R.; AbdelRazek, A. Effect of interface transmissivity and hydraulic conductivity on contaminant migration through composite liners with wrinkles or failed seams. Can. Geotech. J. 2019, 56, 1650–1667. [Google Scholar] [CrossRef]
- Seymour, K. Landfill Lining for Leachate Containment. Water Environ. J. 1992, 6, 389–396. [Google Scholar] [CrossRef]
- Benson, C.; Daniel, D.; Boutwell, G. Field Performance of Compacted Clay Liners. J. Geotech. Geoenviron. Eng. 1999, 125, 390–403. [Google Scholar] [CrossRef]
- Marcotte, B.; Fleming, I. Design guidance for protection of geomembrane liners in landfill applications. Can. Geotech. J. 2022, 59, 743–757. [Google Scholar] [CrossRef]
- Gupt, C.; Bordoloi, S.; Sahoo, R.; Sekharan, S. Mechanical performance and micro-structure of bentonite-fly ash and bentonite-sand mixes for landfill liner application. J. Clean. Prod. 2021, 292, 126033. [Google Scholar] [CrossRef]
- Zhang, T.; Cai, G.; Liu, S. Assessment of mechanical properties in recycled lignin-stabilized silty soil as base fill material. J. Clean. Prod. 2018, 172, 1788–1799. [Google Scholar] [CrossRef]
- Rajesh, S.; Gourc, J.; Viswanadham, B. Evaluation of gas permeability and mechanical behaviour of soil barriers of landfill cap covers through laboratory tests. Appl. Clay Sci. 2014, 97–98, 200–214. [Google Scholar] [CrossRef]
- Leroueil, S.; Bihan, J.; Bouchard, R. Remarks on the design of clay liners used in lagoons as hydraulic barriers. Can. Geotech. J. 1992, 29, 512–515. [Google Scholar] [CrossRef]
- Marcotte, B.; Fleming, I. The role of undrained clay soil subgrade properties in controlling deformations in geomembranes. Geotext. Geomembr. 2019, 47, 327–335. [Google Scholar] [CrossRef]
- Brummermann, K.; Blumerl, W.; Stoewahse, C. Protection layers for geomembranes: Effectiveness and testing procedures. In Proceedings of the 5th Internation Conference on Goetextiles, Geomembranes and Related Products, Singapore, 5–9 September 1994. [Google Scholar]
- Reddy, K.; Bandi, S.; Rohr, J.; Finy, M.; Siebken, J. Field Evaluation of Protective Covers for Landfill Geomembrane Liners Under Contruction Loading. Geosynth. Int. 1996, 3, 679–700. [Google Scholar] [CrossRef]
- Zanzinger, H. Efficiency of Geosynthetic Protection Layers for Geomembrane Liners: Performance in a Large-Scale Model Test. Geosynth. Int. 1999, 6, 303–317. [Google Scholar] [CrossRef]
- Tognon, A.; Rowe, R.; Moore, I. Geomembrane Strain Observed in Large-Scale Testing of Protection Layers. J. Geotech. Geoenviron. Eng. 2000, 126, 1194–1205. [Google Scholar] [CrossRef]
- Koerner, R.; Soong, T. Analysis and design of veneer cover soils. Geosynth. Int. 2005, 12, 28–49. [Google Scholar] [CrossRef]
- Singh, M.; Fleming, I. Application of a hyperbolic model to municipal solid waste. Géotechnique 2011, 61, 533–547. [Google Scholar] [CrossRef]
- Krase, V.; Bente, S.; Kowalsky, U.; Dinkler, D. Modelling the stress–deformation behaviour of municipal solid waste. Géotechnique 2011, 61, 665–675. [Google Scholar] [CrossRef]
- Yin, D.; Wu, H.; Cheng, C.; Chen, Y. Fractional Order Constitutive Model of Geomaterials Under the Condition of Triaxial Test. In Proceedings of the 2011 ASME/IEEE International Conference on Mechatronic and Embedded Systems and Applications, Washington, DC, USA, 28–31 August 2011; Volume 3, pp. 257–265. [Google Scholar] [CrossRef]
- Kumar, G.; Reddy, K.; Foster, C. Modeling elasto-visco-bio-plastic mechanical behavior of municipal solid waste in landfills. Acta Geotech. 2021, 16, 1061–1081. [Google Scholar] [CrossRef]
- Kumar, G.; Reddy, K. Constitutive models for municipal solid waste in landfills. In Geotechnical Engineering in the XXI Century: Lessons Learned and Future Challenges, Proceedings of the XVI Pan-American Conference on Soil Mechanics and Geotechnical Engineering (XVI PCSMGE), Cancun, Mexico, 17–20 November 2019; IOS Press: Amsterdam, The Netherlands, 2019; pp. 654–662. [Google Scholar]
- Gao, W.; Kavazanjian, E. A constitutive model for municipal solid waste considering mechanical creep and biodegradation-induced compression. Acta Geotech. 2022, 17, 37–63. [Google Scholar] [CrossRef]
- Machado, S.; Vilar, O.; Carvalho, M. Constitutive model for long term municipal solid waste mechanical behavior. Comput. Geotech. 2008, 35, 775–790. [Google Scholar] [CrossRef]
- Feng, S.; Cao, B.; Bai, Z.; Yin, Z. Constitutive model for municipal solid waste considering the effect of biodegradation. Géotech. Lett. 2016, 6, 244–249. [Google Scholar] [CrossRef]
- Sivakumar Babu, G.; Reddy, K.; Chouskey, S.; Kulkarni, H. Prediction of long-term municipal solid waste landfill settelment using constitutive model. Pract. Period. Hazard. Toxic Radioact. Waste Manag. 2010, 14, 139–150. [Google Scholar] [CrossRef] [Green Version]
- Sharma, H.D.; De, A. Municipal Solid Waste Landfill Settlement: Postclosure Perspectives. J. Geotech. Geoenviron. Eng. 2007, 133, 619–629. [Google Scholar] [CrossRef]
- Bareither, C.A.; Breitmeyer, R.J.; Benson, C.H.; Barlaz, M.A.; Edil, T.B. Deer Track Bioreactor Experiment: Field-Scale Evaluation of Municipal Solid Waste Bioreactor Performance. J. Geotech. Geoenviron. Eng. 2012, 138, 658–670. [Google Scholar] [CrossRef]
- Wu, G.; Chen, Y.; Zhan, L.; Bian, X. Engineering properties for high kitchen waste content municipal solid waste. J. Rock Mech. Geotech. Eng. 2015, 7, 646–658. [Google Scholar] [CrossRef] [Green Version]
- Wu, G.; Xu, W.; Bian, X.; Chen, Y. A practical approach for calculating the settlement and storage capacity of landfills based on the space and time discretization of the landfilling process. Waste Manag. 2017, 69, 202–214. [Google Scholar] [CrossRef]
- Nobel, J.J.; Nunez-MCnally, T.; TanseL, B. The effects of mass transfer on landfill stabilisation rates. In Proceedings of the 43rd Annual Purdue Industrial Waste Conference, West Lafayette, IN, USA, 10–12 May 1988. [Google Scholar]
- Jessberger, H. Geotechnical aspects of landfill design and construction. Part 2: Material parameters and methods. Proc. ICE J. Geotech. Eng. 1994, 107, 105–113. [Google Scholar] [CrossRef]
- Cooke, S.; Walker, N.; Thomas, R. Calibrated waste settlement prediction data from numerical modelling of waste processes at some UK landfill sites. In Proceedings of the Sardinia 2007: 11th International Landfill Symposium, Sardinia, Italy, 1–5 October 2007; CISA (Environmental Sanitary Engineering Centre): Cagliari, Italy, 2007. [Google Scholar]
- Brachman, R.; Gudina, S. Gravel contacts and geomembrane strains for a GM/CCL composite liner. Geotext. Geomembr. 2008, 26, 448–459. [Google Scholar] [CrossRef]
- Fleming, I.; Sharma, J.; Jogi, M. Shear strength of geomembrane–soil interface under unsaturated conditions. Geotext. Geomembr. 2006, 24, 274–284. [Google Scholar] [CrossRef]
- Feng, S.-J.; Lu, S.-F. Repeated shear behaviors of geotextile/geomembrane and geomembrane/clay interfaces. Environ. Earth Sci. 2016, 75, 273. [Google Scholar] [CrossRef]
- Fox, P.J.; Kim, R.H. Effect of Progressive Failure on Measured Shear Strength of Geomembrane/GCL Interface. J. Geotech. Geoenviron. Eng. 2008, 134, 459–469. [Google Scholar] [CrossRef]
- Ling, H.I.; Pamuk, A.; Dechasakulsom, M.; Mohri, Y.; Burke, C. Interactions between PVC Geomembranes and Compacted Clays. J. Geotech. Geoenviron. Eng. 2001, 127, 950–954. [Google Scholar] [CrossRef]
- Palmeira, E.M. Soil–geosynthetic interaction: Modelling and analysis. Geotext. Geomembr. 2009, 27, 368–390. [Google Scholar] [CrossRef]
- McCartney, J.S.; Zornberg, J.G.; Swan, R.H. Analysis of a Large Database of GCL-Geomembrane Interface Shear Strength Results. J. Geotech. Geoenviron. Eng. 2009, 135, 209–223. [Google Scholar] [CrossRef] [Green Version]
- Šestak, A.; Stanimirovič, B. Report on Construction of Boreholes and Monitoring Wells with the Aim of Conducting Chemical and Geotechnical Testing of Groundwater and Soil in the Field of Communal Landfill Vinča in Belgrade: According to the Contract No. 7177062; Nikole Tesle No. 15, 22300; Institude MOL Ltd.: Stara Pazova, Serbia, 2016; pp. 1–269. [Google Scholar]
- Zivanovic, M. Report on Results of Geotechnical Investigation for Belgrade Solid Waste Treatment and Disposal PPP—Site Investigation Consultant—ECA PPP Team; TECHNOHIDROSFERA d.o.o.: Beočin, Serbija, 2016; pp. 1–232. [Google Scholar]
- Moric, P. Vinča Landfill: Preliminary Engineering Geology Investigation Report; Aquatis a.s.: Brno, Czech Republic, 2016; pp. 1–42. (In Czech) [Google Scholar]
- PLAXIS 2D: Reference Manual; Version 2018; build 9462; Plaxis: Delft, The Netherlands, 2016; pp. 2–494.
- Schanz, T.; Vermeer, P.; Bonnier, P. The hardening soil model: Formulation and verification: Formulation and verification. In Beyond 2000 in Computational Geotechnics: Ten Years of PLAXIS International, Proceedings of the International Symposium, Amsterdam, The Netherlands, 18–20 March 1999; Balkema: Rotterdam, The Netherlands, 1999; pp. 281–296. [Google Scholar]
- Duncan, J.; Chang, C. Nonlinear analysis of stress and strain in soils. J. Soil Mech. Found. Div. 1970, 96, 1629–1653. [Google Scholar] [CrossRef]
- Kondner, R.L.; Zelasko, J.S. A hyperbolic stress-strain formulation for sands. In Proceeding of the 2nd Pan American Conference on Soil Mechanics and Foundation Engineering, Sao Paulo, Brazil, 16–24 July 1963; Volume 1, pp. 289–324. [Google Scholar]
- Plaxis 2D: Material Models Manual; Version 201; build 9462; Plaxis: Delft, The Netherlands, 2016; pp. 1–256.
- Koerner, R. Designing with Geosynthetics, 5th ed.; Pearson Prentice Hall: Upper Saddle River, NJ, USA, 2005; pp. 1–818. [Google Scholar]
- Dixon, N.; Jones, D. Engineering properties of municipal solid waste. Geotext. Geomembr. 2005, 23, 205–233. [Google Scholar] [CrossRef]
- Machado, S.; Carvalho, M.; Vilar, O. Constitutive Model for Municipal Solid Waste. J. Geotech. Geoenviron. Eng. 2002, 128, 940–951. [Google Scholar] [CrossRef]
- Castelli, F.; Maugeri, M. Mechanical properties of Municipal Solid Waste by SDMT. Waste Manag. 2014, 34, 256–265. [Google Scholar] [CrossRef]
- Zekkos, D.; Bray, J.; Kavazanjian, E.; Matasovic, N.; Rathje, E.; Riemer, M.; Stokoe, K. Unit Weight of Municipal Solid Waste. J. Geotech. Geoenviron.Eng. 2006, 132, 1250–1261. [Google Scholar] [CrossRef]
- Hartwell, J.; Mousavi, M.; Eun, J.; Bartelt-Hunt, S. Assessment of in situ properties of municipal solid waste with a large-diameter borehole method. Waste Manag. Res. J. Sustain. Circ. Econ. 2021, 9, 987–997. [Google Scholar] [CrossRef]
- Yesiller, N.; Hanson, J.; Cox, J.; Noce, D. Determination of specific gravity of municipal solid waste. Waste Manag. 2014, 34, 848–858. [Google Scholar] [CrossRef] [Green Version]
- Bray, J.; Zekkos, D.; Kavazanjian, E.; Athanasopoulos, G.; Riemer, M. Shear Strength of Municipal Solid Waste. J. Geotech. Geoenviron. Eng. 2009, 135, 709–722. [Google Scholar] [CrossRef] [Green Version]
- Zhan, T.; Chen, Y.; Ling, W. Shear strength characterization of municipal solid waste at the Suzhou landfill, China. Eng. Geol. 2008, 97, 97–111. [Google Scholar] [CrossRef]
- Koelsch, F. Shear Strength of waste. In Proceedings of the Third International Workshop “Hydro-Physico Mechanics of Landfills”, Braunschweif, Germany, 10–13 March 2009; pp. 1–10. [Google Scholar]
- Zeng, G.; Liu, L.; Xue, Q.; Wan, Y.; Ma, J.; Zhao, Y. Experimental study of the porosity and permeability of municipal solid waste. Environ. Prog. Sustain. Energy 2017, 36, 1694–1699. [Google Scholar] [CrossRef]
- Zhang, Z.; Wang, Y.; Xu, H.; Fang, Y.; Wu, D. Influence of effective stress and dry density on the permeability of municipal solid waste. Waste Manag. Res. J. Sustain. Circ. Econ. 2018, 36, 471–480. [Google Scholar] [CrossRef] [PubMed]
- Jie, Y.; Xu, W.; Dunzhu, D.; Wei, Y.; Peng, T.; Zhou, Z. Laboratory testing of a densified municipal solid waste in Beijing. J. Cent. South Univ. 2013, 20, 1953–1963. [Google Scholar] [CrossRef]
- Miguel, M.; Mortatti, B.; da Paixão Filho, J.; Pereira, S. Saturated Hydraulic Conductivity of Municipal Solid Waste Considering the Influence of Biodegradation. J. Environ. Eng. 2018, 144. [Google Scholar] [CrossRef]
- Yang, R.; Xu, Z.; Chai, J. A Review of Characteristics of Landfilled Municipal Solid Waste in Several Countries: Physical Composition, Unit Weight, and Permeability Coefficient. Pol. J. Environ. Stud. 2018, 27, 2425–2435. [Google Scholar] [CrossRef]
- Durmusoglu, E.; Sanchez, I.; Corapcioglu, M. Permeability and compression characteristics of municipal solid waste samples. Environ. Geol. 2006, 50, 773–786. [Google Scholar] [CrossRef]
- Machado, S.; Karimpour-Fard, M.; Shariatmadari, N.; Carvalho, M.; Nascimento, J. Evaluation of the geotechnical properties of MSW in two Brazilian landfills. Waste Manag. 2010, 30, 2579–2591. [Google Scholar] [CrossRef] [Green Version]
- Travar, I. The Use of Air Pollution Control Residues in Landfill Covers and for Soil Stabilization. Ph.D. Thesis, Department of Civil, Environmental and Natural Resources Engineering, Geosciences and Environmental Engineering, Luleå University of Technology, Luleå, Sweden, 2015. [Google Scholar]
- Yu, Y.; Rowe, R.K. Development of geomembrane strains in waste containment facility liners with waste settlement. Geotext. Geomembr. 2018, 46, 226–242. [Google Scholar] [CrossRef]
- Xu, C.; Xiao, Y.Y.; Liao, X.Y.; Chen, T.T. Influence of Waste and Subgrade Settlement on Landfill Linier Stability and Integrity. In Geosynthetics in Civil and Environmental Engineering; Springer: Berlin/Heidelberg, Germany, 2009; pp. 564–568. ISBN 978-3-540-69312-3. [Google Scholar] [CrossRef]
- Shelton, D.A.; Schoenwolf, D.A.; Mohanan, N.P. Redevelopment of a Municipal Solid Waste Landfill: Engineering Design Challenges. In Proceedings of the 6th International Conference on Case Histories in Geotechnical Engineering, Arlington, VA, USA, 11–16 August 2008. [Google Scholar]
- Jedele, L.P.; Buschmeier, B. Ground Improvement for Redevelopment of Former Landfill. In Proceedings of the 7th International Conference on Case Histories in Geotechnical Engineering, Chicago, IL, USA, 29 April–4 May 2013. [Google Scholar]





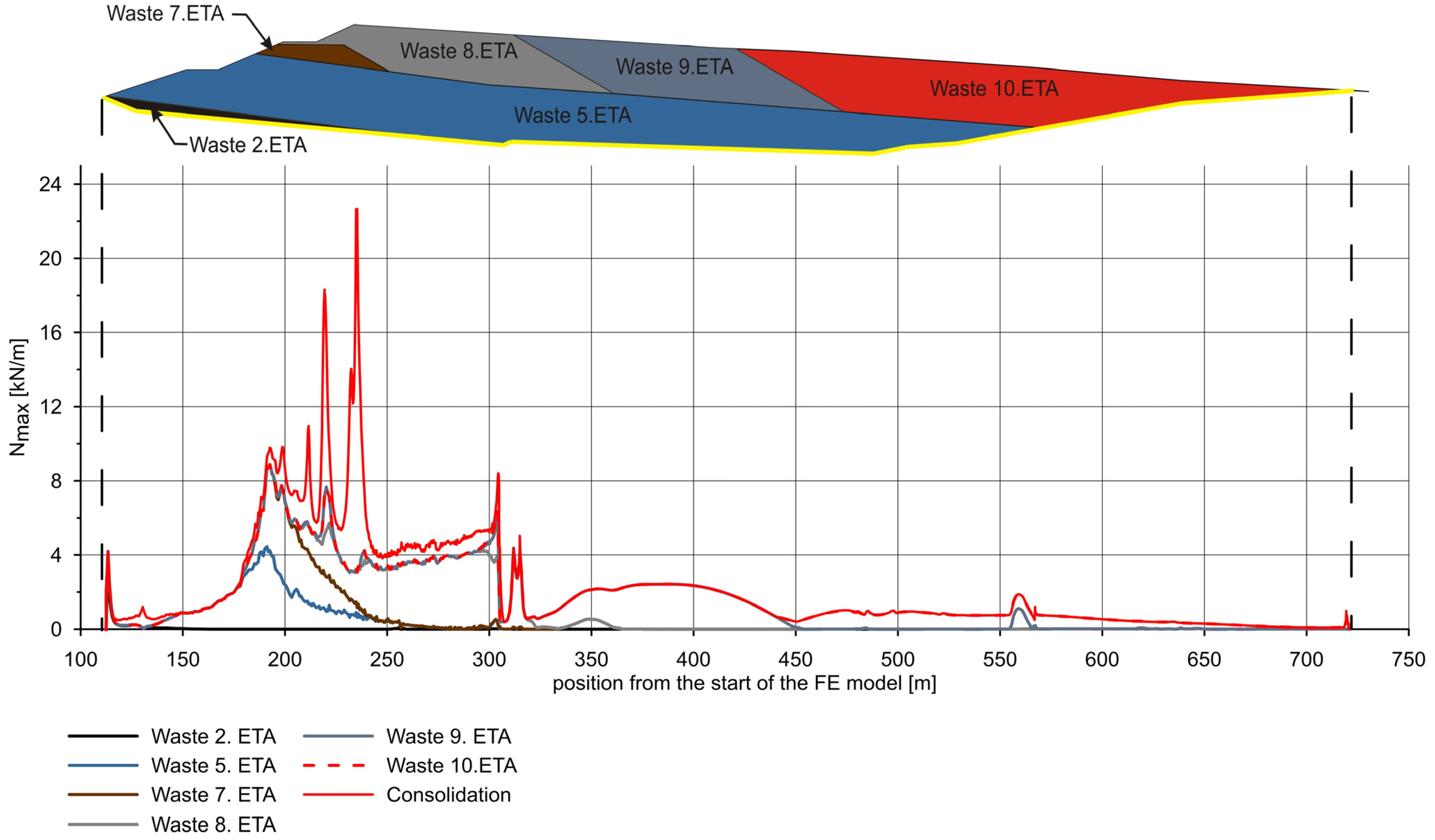



| FE Model | Model Width [m] | Model Height [m] | Number of Finite Elements [-] | Number of Nodes [-] |
|---|---|---|---|---|
| Longitudinal section A–A′ | 1580 | 258 | 5081 | 41,143 |
| Cross Section B–B′ | 920 | 202 | 3081 | 24,987 |
| No. | Description | Type of Calculation |
|---|---|---|
| 01 | Calculation of the original stress state before the current landfill began operation. | Gravity loading |
| 02 | Loading of the original valley by the weight of the waste deposited up to the moment before the landfill reconstruction. | Plastic |
| 03 | Ground zone of new landfill modification. | Plastic |
| 04 | Activation of geogrid finite elements simulating the installation of the liner system | Plastic |
| 05 | “Waste 2. ETA”—waste storage in the new landfill between the 2nd and 4th year of operation | Consolidation 2 years |
| 06 | “Waste 4 ETA”—waste storage in the new landfill between the 6th and 9th year of operation | Consolidation 3 years |
| 07 | “Waste 5. ETA”—waste storage in the new landfill between the 9th and 14th year of operation | Consolidation 5 years |
| 08 | “Waste 7. ETA”—waste storage in the new landfill between the 18th and 19th year of operation | Consolidation 1 year |
| 09 | “Waste 8. ETA”—waste storage in the new landfill between the 19th and 23rd year of operation | Consolidation 4 years |
| 10 | “Waste 9. ETA”—waste storage in the new landfill between the 23rd and 26th year of operation | Consolidation 3 years |
| 11 | “Waste 9. ETA”—waste storage in the new landfill between the 26th and 28th year of operation | Consolidation 2 years |
| 12 | Simulation of the consolidation process until the dissipation of pore pressures | Consolidation |
| No. | Description | Type of Calculation |
|---|---|---|
| 01 | Calculation of the original stress state before the current landfill began operation. | Gravity loading |
| 02 | Loading of the original valley by the weight of the waste deposited up to the moment before the landfill reconstruction. | Plastic |
| 03 | Ground zone of new landfill modification. | Plastic |
| 04 | Activation of geogrid finite elements simulating the installation of the liner system | Plastic |
| 05 | “Waste FGT Ash”—deposition of solidified FGT fly ash | Plastic |
| 06 | “Waste 01. ETA”—storage of waste in the new landfill between the 1st and 2nd year of operation | Consolidation 2 years |
| 07 | “Waste 10. ETA”—waste storage in the new landfill between the 26th and 28th year of operation | Consolidation 2 years |
| 08 | Simulation of the consolidation process until the dissipation of pore pressures | Consolidation |
| Material | Mass Unit Weight | Cohesion/Angle of Internal Friction | Hydraulic Conductivity Coefficient | ||
|---|---|---|---|---|---|
| γunsat [kN/m3] | γsat [kN/m3] | C′ [kPa] | φ′ [°] | kx,y sat [m/day] | |
| Modified terrain | 11.0 | 12.0 | 13.5 | 33.0 | 0.864 |
| MSW | 11.0 | 12.0 | 13.5 | 33.0 | 0.864 |
| FGT Ash | 15.0 | 15.0 | 34.0 | 29.0 | 0.864 |
| Clay; Gj | 19.4 | 21.0 | 24.3 | 21.6 | 1.36 × 10−6 |
| Marly clay; GLj | 19.6 | 21.0 | 26.0 | 20.5 | 1.36 × 10−6 |
| Loess (silty clay); PRGd | 19.75 | 21.0 | 25.0 | 21.0 | 1.36 × 10−6 |
| Material | MC | HS | ||||
|---|---|---|---|---|---|---|
| E′ [MPa] | ν′ [-] | Eoed,ref [MPa] | E50,ref [MPa] | Eur,ref [MPa] | m [-] | |
| Modified terrain | 10.0 | 0.35 | ||||
| MSW | 10.0 | 0.35 | ||||
| FGT Ash | 15.0 | 0.35 | ||||
| Clay; Gj | 10.0 | 10.0 | 30.0 | 0.55 | ||
| Marly clay; GLj | 12.0 | 12.0 | 36.0 | 0.50 | ||
| Loess (silty clay); PRGd | 7.0 | 7.0 | 21.0 | 0.65 | ||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Štefaňák, J.; Chalmovský, J. Assessment of the Landfill Barrier System through Numerical Analysis: Rehabilitation and Expansion of Belgrade Landfill Case Study. Sustainability 2022, 14, 7647. https://doi.org/10.3390/su14137647
Štefaňák J, Chalmovský J. Assessment of the Landfill Barrier System through Numerical Analysis: Rehabilitation and Expansion of Belgrade Landfill Case Study. Sustainability. 2022; 14(13):7647. https://doi.org/10.3390/su14137647
Chicago/Turabian StyleŠtefaňák, Jan, and Juraj Chalmovský. 2022. "Assessment of the Landfill Barrier System through Numerical Analysis: Rehabilitation and Expansion of Belgrade Landfill Case Study" Sustainability 14, no. 13: 7647. https://doi.org/10.3390/su14137647
APA StyleŠtefaňák, J., & Chalmovský, J. (2022). Assessment of the Landfill Barrier System through Numerical Analysis: Rehabilitation and Expansion of Belgrade Landfill Case Study. Sustainability, 14(13), 7647. https://doi.org/10.3390/su14137647






