Investigation of Resilience Characteristics of Unbound Granular Materials for Sustainable Pavements
Abstract
:1. Introduction
2. Experimental Program
2.1. Materials and Samples
2.2. Material Properties
2.3. Specimen Preparation
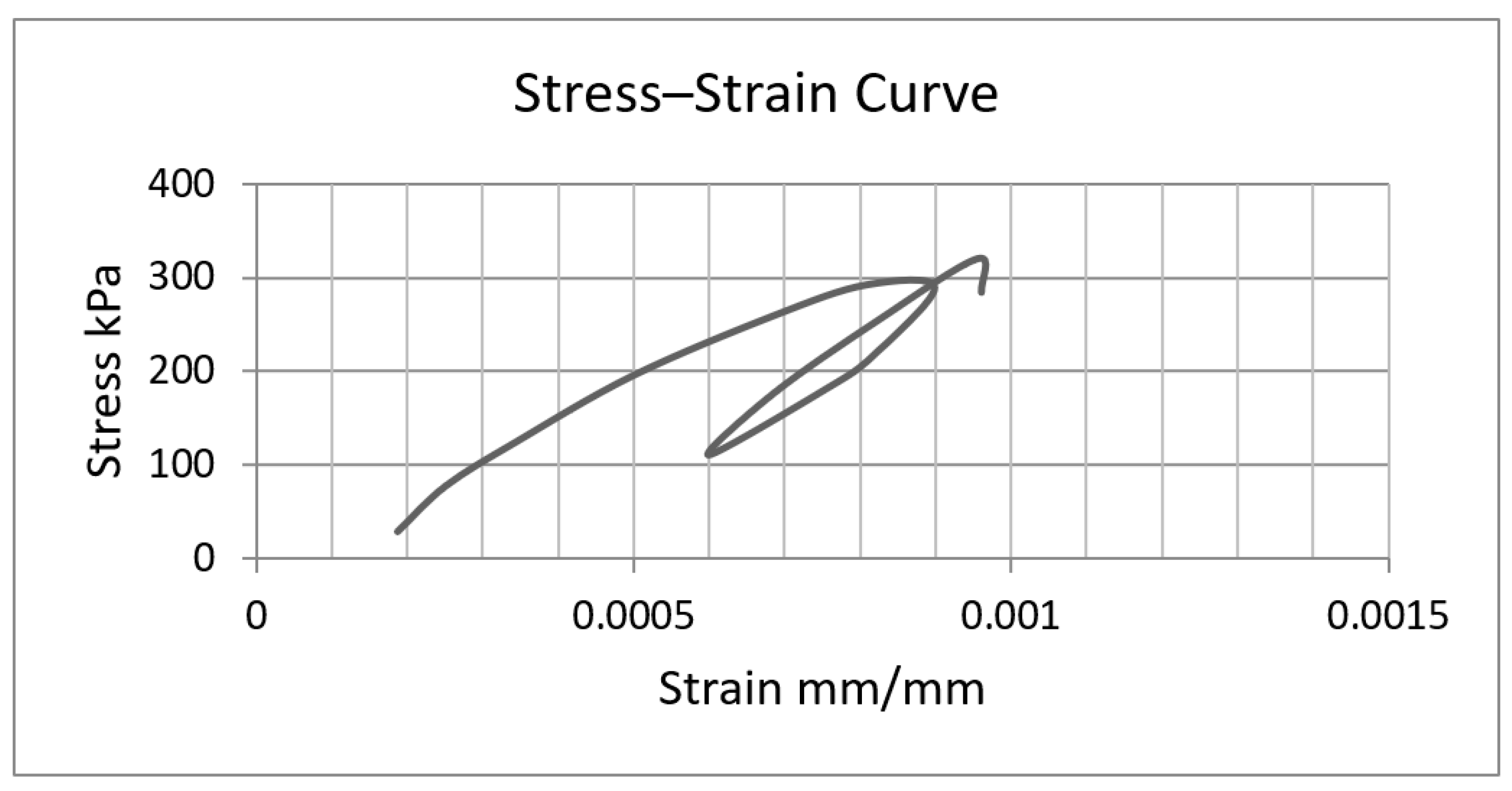
2.4. Resilient Modulus Test
2.5. Results and Discussion
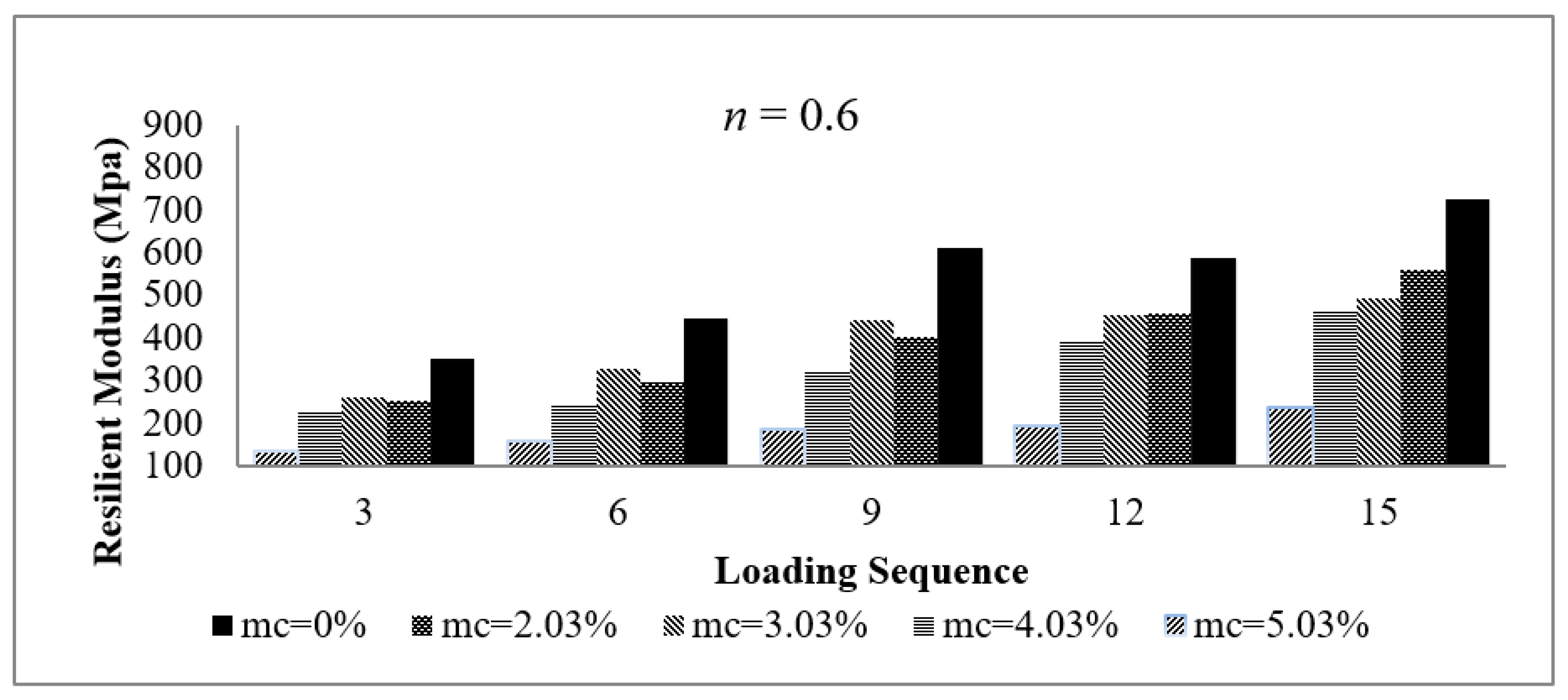
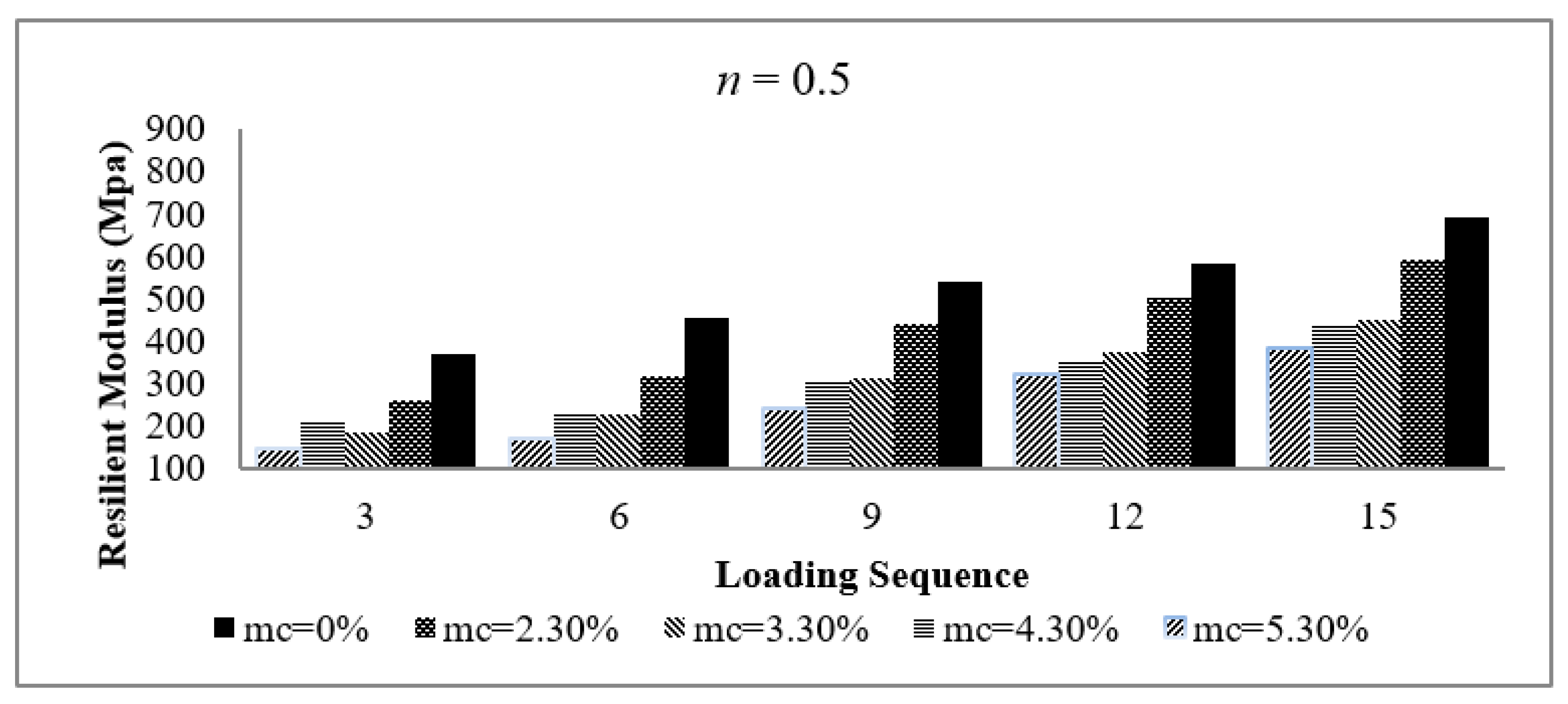
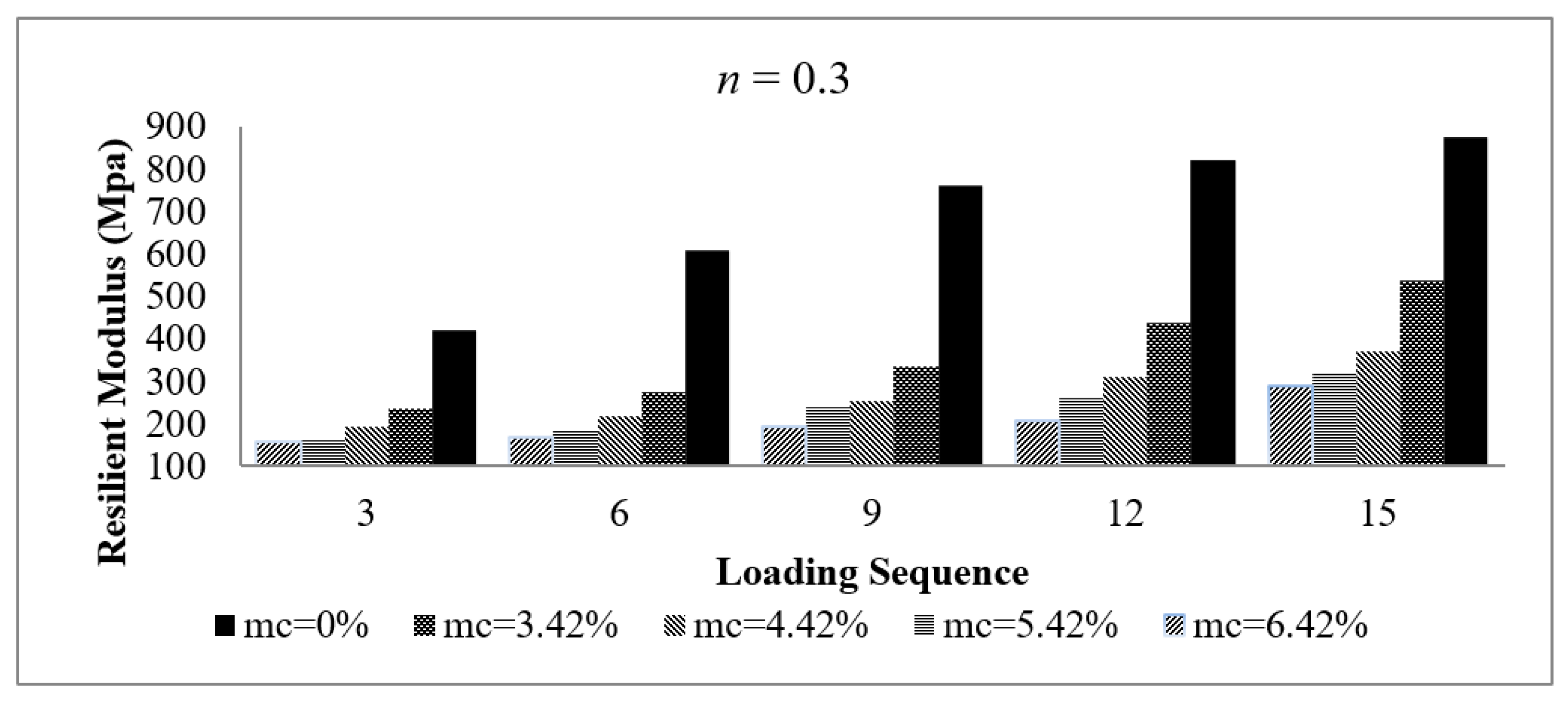
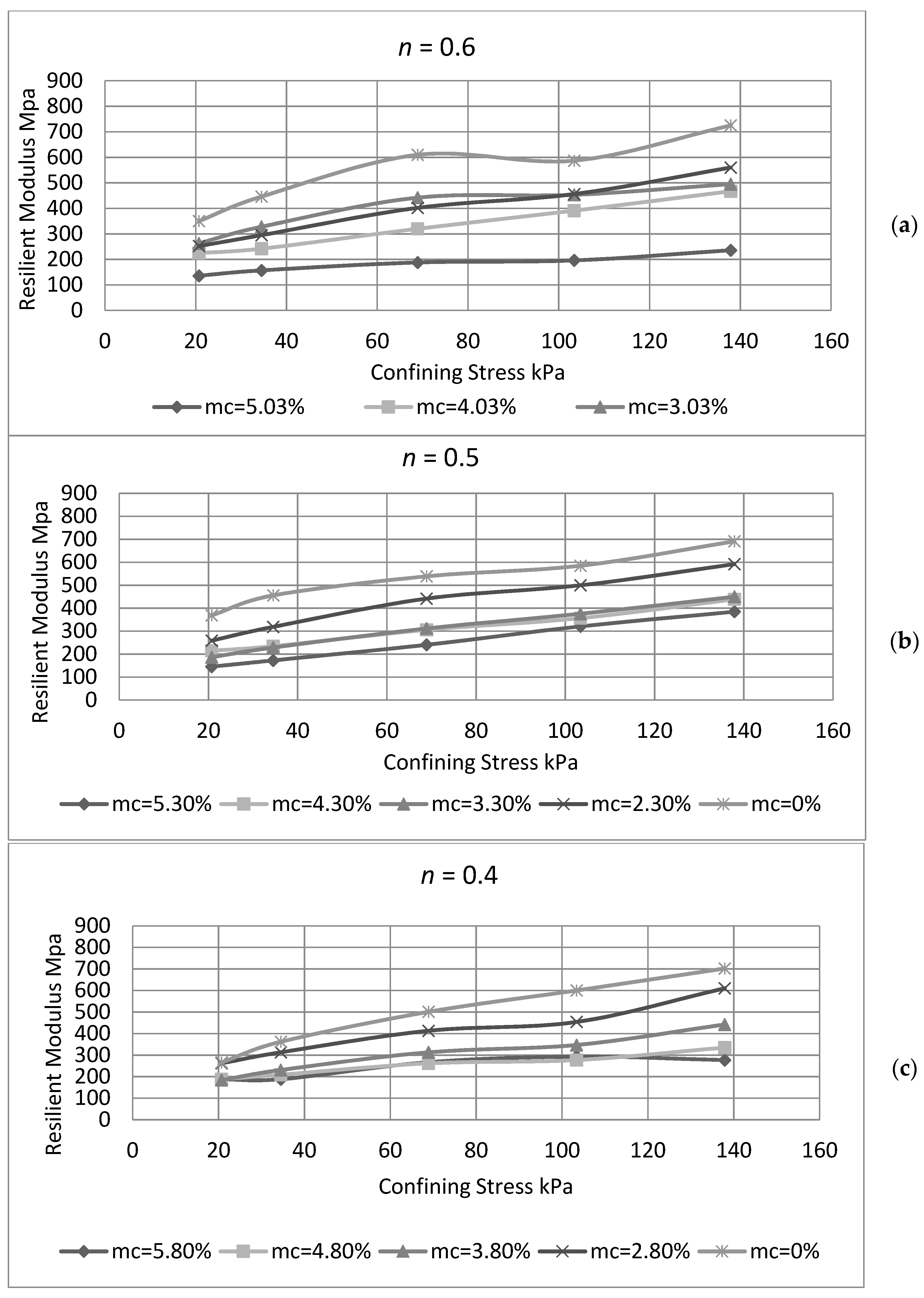
2.5.1. Effect of Moisture Content on the Resilient Modulus
2.5.2. Effect of Moisture Stresses on Resilient Modulus
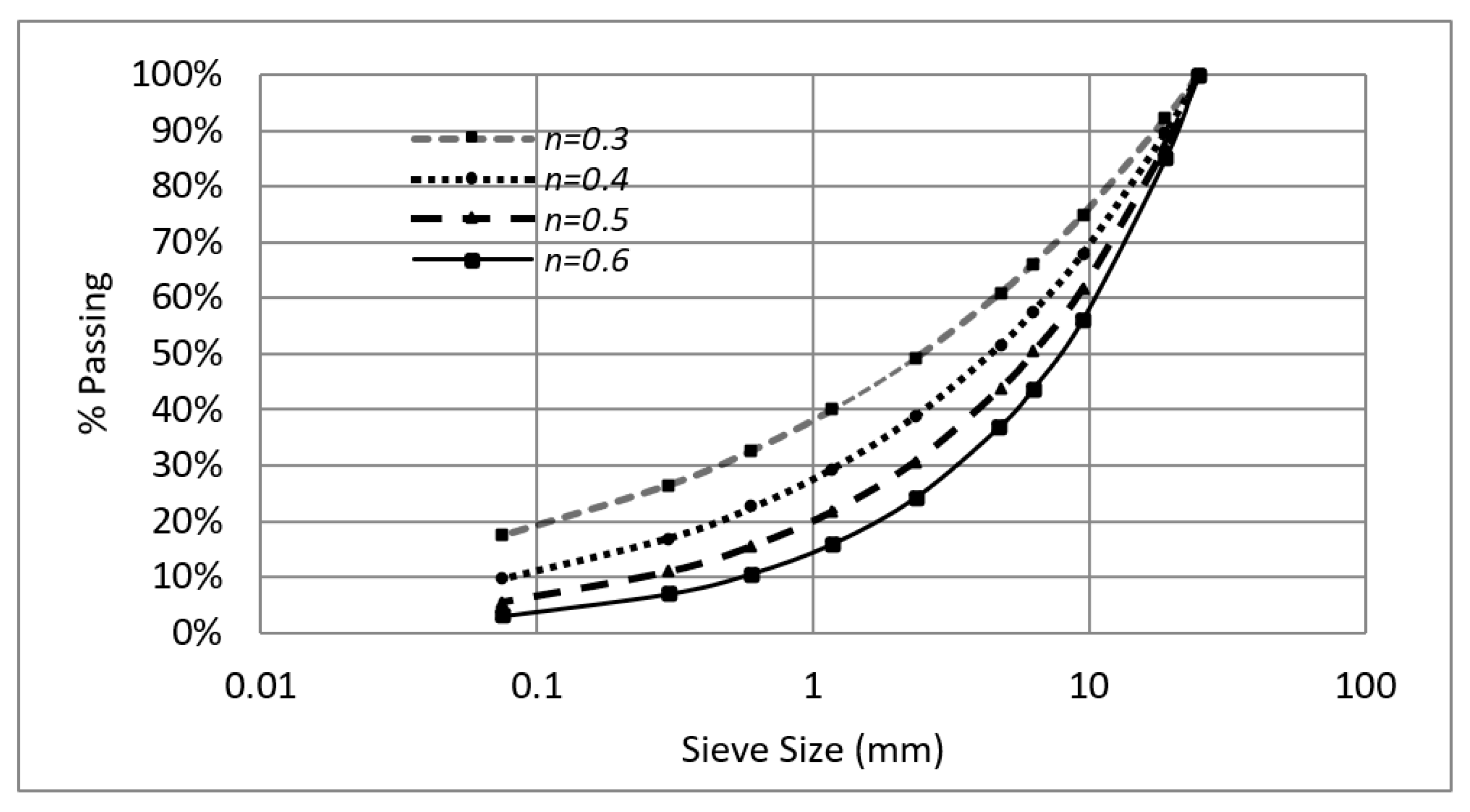
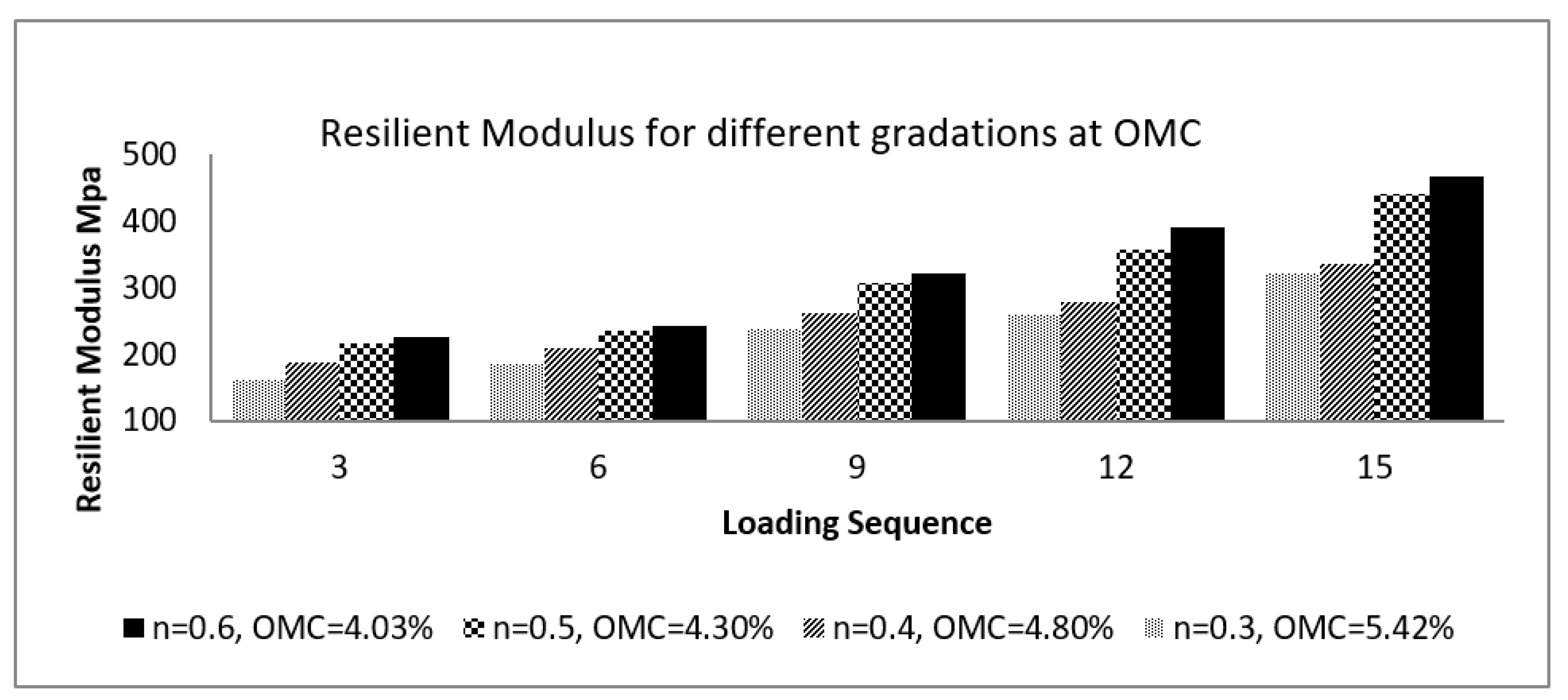
2.5.3. Effect of Material Gradation on the Resilient Modulus
3. Statistical Model
3.1. Statistical Model Evaluation
3.2. Relationship of Resilient Modulus and Moisture Content
3.3. Improved Relationship for Resilient Modulus, Stresses, and Moisture
4. Conclusions
- The resilient modulus decreases with an increase in the moisture content of unbound granular material, and vice versa. This is because at the dry side of optimum moisture content, the materials behave more stiffly, resulting in an increase in the resilient modulus, and at the wet side of optimum moisture content, the material becomes saturated and pore water pressure develops, resulting in a reduction in the stiffness.
- The resilient modulus increases significantly with the increase in both deviator and confining stresses of unbound granular material. It is shown in Figure 9b that by increasing the confining stress from 103 kPa to 137 kPa, the resilient modulus increases from 585 Mpa to 691 Mpa.
- The resilient modulus also decreases with an increase in finer gradation and increases with an increase in coarser gradation in unbound granular materials. A lower resilient modulus value is observed at (n) 0.3 and 0.4, and a higher resilient modulus value is seen at (n) 0.5 and 0.6. This is because the coarser gradations are stiffer than the finer gradation.
- A new relationship has been proposed in Equation (2), which depicts that the moisture content and resilient modulus of unbound granular material can be predicted through a linear relationship. However, the accuracy is better if Equation (3) is applied.
- The new relationship has been trained on three-fifth of the dataset, and regression parameters were calculated. The model was tested on the rest of the data with the trained parameters. It can be concluded that resilient modulus values predicted by the new relationship are in good agreement with repeated load triaxial test data. Therefore, new relationships can be used in the design process with greater confidence compared with the previous relationships, which only consider the stress tensors for the prediction of resilient modulus.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Ba, M.; Fall, M.; Sall, O.A.; Samb, F. Effect of Compaction Moisture Content on the Resilient Modulus of Unbound Aggregates from Senegal (West Africa). Geomaterials 2012, 2, 19–23. [Google Scholar] [CrossRef] [Green Version]
- Smith, W.; Nair, K. Development of Procedures for Characterization of Untreated Granular Base Course and Asphalt-Treated Base Course Materials; Materials Research: Oakland, CA, USA, 1973. [Google Scholar]
- Monismith, C.L.; Seed, F.G.; Mitry, F.G.; Chan, C. Predictions of pavement deflections from laboratory tests. In Second International Conference on the Structural Design of Asphalt Pavements; University of Michigan: Ann Arbor, MI, USA, 1967. [Google Scholar]
- Haynes, J.H. Effects of Repeated Loading on Gravel and Crushed Stone Base Course Materials Used in the AASHO Road Test; Purdue University: West Lafayette, IN, USA, 1961. [Google Scholar]
- Lekarp, F.; Isacsson, U.; Dawson, A. State of the Art. I: Resilient Response of Unbound Aggregates. J. Transp. Eng. 2000, 126, 66–75. [Google Scholar] [CrossRef] [Green Version]
- Jing, P. Experimental Study and Modelling of the Elastoplastic Behaviour of Unbound Granular Materials under Large Number of Cyclic Loadings at Various Initial Hydric States; Université de Strasbourg: Strasbourg, France, 2017. [Google Scholar]
- Sun, J.; Oh, E.; Ong, D. Influence of Degree of Saturation (DOS) on Dynamic Behavior of Unbound Granular Materials. Geosciences 2021, 11, 89. [Google Scholar] [CrossRef]
- Rahman, M.S. Characterising the Deformation Behaviour of Unbound Granular Materials in Pavement Structures; Royal Institute of Technology: Stockholm, Sweden, 2015. [Google Scholar]
- Rahman, M.S.; Erlingsson, S. Influence of moisture on Resilient Deformation behaviour of Unbound Granular Materials. In Asphalt Pavements; CRC Press: Boca Raton, FL, USA, 2014; pp. 595–604. [Google Scholar]
- Attia, M.; Abdelrahman, M. Modeling the Effect of Moisture on Resilient Modulus of Untreated Reclaimed Asphalt Pavement. Transp. Res. Rec. J. Transp. Res. Board 2010, 2167, 30–40. [Google Scholar] [CrossRef]
- Tamrakar, P.; Nazarian, S. Moisture effects on moduli of pavement bases. Int. J. Pavement Eng. 2021, 22, 1410–1422. [Google Scholar] [CrossRef]
- Rahman, M.S.; Erlingsson, S. Moisture influence on the resilient deformation behaviour of unbound granular materials. Int. J. Pavement Eng. 2016, 17, 763–775. [Google Scholar] [CrossRef]
- Rahman, M.S.; Erlingsson, S. Influence of Post Compaction on the Moisture Sensitive Resilient Modulus of Unbound Granular Materials. Procedia Eng. 2016, 143, 929–936. [Google Scholar] [CrossRef] [Green Version]
- Rokitowski, P.; Bzówka, J.; Grygierek, M. Influence of high moisture content on road pavement structure: A Polish case study. Case Stud. Constr. Mater. 2021, 15, e00594. [Google Scholar] [CrossRef]
- Dan, H.-C.; He, L.-H.; Zhao, L.-H. Experimental investigation on the resilient response of unbound graded aggregate materials by using large-scale dynamic triaxial tests. Road Mater. Pavement Des. 2020, 21, 434–451. [Google Scholar] [CrossRef]
- Bilodeau, J.-P.; Plamondon, C.O.; Doré, G. Estimation of resilient modulus of unbound granular materials used as pavement base: Combined effect of grain-size distribution and aggregate source frictional properties. Mater. Struct. 2016, 49, 4363–4373. [Google Scholar] [CrossRef]
- Mousa, R.; Gabr, A.; Arab, M.G.; Azam, A.; El-Badawy, S. Resilient modulus for unbound granular materials and subgrade soils in Egypt. MATEC Web Conf. 2017, 120, 06009. [Google Scholar] [CrossRef] [Green Version]
- Stolle, D.; Guo, P.; Liu, Y. Resilient modulus properties of granular highway materials. Can. J. Civ. Eng. 2009, 36, 639–654. [Google Scholar] [CrossRef]
- Li, N.; Tian, Y.; Ma, B.; Hu, D. Experimental Investigation of Water-Retaining and Mechanical Behaviors of Unbound Granular Materials under Infiltration. Sustainability 2022, 14, 1174. [Google Scholar] [CrossRef]
- Gu, F.; Sahin, H.; Luo, X.; Luo, R.; Lytton, R.L. Estimation of Resilient Modulus of Unbound Aggregates Using Performance-Related Base Course Properties. J. Mater. Civ. Eng. 2015, 27, 04014188. [Google Scholar] [CrossRef]
- Thai, H.N. Effects of Particle Size and Type of Aggregate on Mechanical Properties and Environmental Safety of Unbound Road Base and Subbase Materials: A Literature review. Int. J. Geomate J. 2021, 20, 148–157. [Google Scholar] [CrossRef]
- El-Ashwah, A.S.; Mousa, E.; El-Badawy, S.M.; Abo-Hashema, M.A. Advanced characterization of unbound granular materials for pavement structural design in Egypt. Int. J. Pavement Eng. 2020, 23, 476–488. [Google Scholar] [CrossRef]
- Esfahani, M.A.; Goli, A. Effects of Aggregate Gradation on Resilient Modulus and CBR in Unbound Granular Materials. Int. J. Trans. Eng. 2018, 5, 367–381. [Google Scholar] [CrossRef]
- Mneina, A.; Shalaby, A. Relating gradation parameters to mechanical and drainage performance of unbound granular materials. Transp. Geotech. 2020, 23, 100315. [Google Scholar] [CrossRef]
- Ekblad, J. Statistical evaluation of resilient models characterizing coarse granular materials. Mater. Struct. 2007, 41, 509–525. [Google Scholar] [CrossRef]
- Kamal, M.; Dawson, A.R.; Farouki, O.T.; Hughes, D.A.B.; Sha’at, A.A. Field and laboratory evaluation of the mechanical behavior of unbound granular materials in pavements. Transp. Res. Rec. 1993, 1406, 88. [Google Scholar]
- Kolisoja, P. Resilient Deformation Characteristics of Granular Materials; Tampere University of Technology Finland: Tampere, Finland, 1997. [Google Scholar]
- Barskale, R.D.; Itani, S.Y. Influence of aggregate shape on base behavior. Transp. Res. Rec. 1989, 1227, 173–182. [Google Scholar]
- Miao, Y.; Yu, W.; Hou, Y.; Liu, C.; Wang, L. Influences of Clay Brick Particles on the Performance of Cement Stabilized Recycled Aggregate as Pavement Base. Sustainability 2018, 10, 3505. [Google Scholar] [CrossRef] [Green Version]
- Jaffar, S.T.A.; Abid, M.M.; Khan, S.Z.; Jafri, T.; Rehman, Z.U.; Tariq, M.A.U.R.; Ng, A.W.M. Evaluation of Conventional and Sustainable Modifiers to Improve the Stiffness Behavior of Weak Sub-Grade Soil. Sustainability 2022, 14, 2493. [Google Scholar] [CrossRef]
- Cerni, G.; Corradini, A.; Pasquini, E.; Cardone, F. Resilient behaviour of unbound granular materials through repeated load triaxial test: Influence of the conditioning stress. Road Mater. Pavement Des. 2015, 16, 70–88. [Google Scholar] [CrossRef]
- Li, N.; Wang, H.; Ma, B.; Li, R. Investigation of unbound granular material behavior using precision unbound material analyzer and repeated load triaxial test. Transp. Geotech. 2019, 18, 1–9. [Google Scholar] [CrossRef]
- Nguyen, B.T.; Mohajerani, A. Possible simplified method for the determination of the resilient modulus of unbound granular materials. Road Mater. Pavement Des. 2016, 17, 841–858. [Google Scholar] [CrossRef]
- Gu, C.; Ye, X.; Cao, Z.; Cai, Y.; Wang, J.; Zhang, T. Resilient behavior of coarse granular materials in three dimensional anisotropic stress state. Eng. Geol. 2020, 279, 105848. [Google Scholar] [CrossRef]
- Gu, C.; Ye, X.; Wang, J.; Cai, Y.; Cao, Z.; Zhang, T. Resilient behavior of coarse granular materials in three-dimensional stress state. Can. Geotech. J. 2020, 57, 1280–1293. [Google Scholar] [CrossRef]
- Gu, C.; Zhan, Y.; Wang, J.; Cai, Y.; Cao, Z.; Zhang, Q. Resilient and permanent deformation of unsaturated unbound granular materials under cyclic loading by the large-scale triaxial tests. Acta Geotech. 2020, 15, 3343–3356. [Google Scholar] [CrossRef]
- Li, N.; Ma, B.; Wang, H.; Tang, J.; Wang, X.; Shao, Z. Influence of loading frequency on mechanical properties of unbound granular materials via repeated load tests. Constr. Build. Mater. 2021, 301, 124098. [Google Scholar] [CrossRef]
- Li, N.; Ma, B.; Wang, H.; Sun, W. Development of elasto-plastic constitutive model for unbound granular materials under repeated loads. Transp. Geotech. 2020, 23, 100347. [Google Scholar] [CrossRef]
- Zhang, Q.; Leng, W.; Zhai, B.; Xu, F.; Dong, J.; Yang, Q. Evaluation of Critical Dynamic Stress and Accumulative Plastic Strain of an Unbound Granular Material Based on Cyclic Triaxial Tests. Materials 2021, 14, 5722. [Google Scholar] [CrossRef] [PubMed]
- Chanpheng, P.; Sangpetngam, B. Influences of Moisture and Compaction on Resilient Modulus of an Unbound Base Material. In Proceedings of the 18th National Convention on Civil Engineering, Chiang Mai, Thailand, 8–10 May 2013. [Google Scholar]
- Robinson, R. Measurement of the Elastic Properties of Granular Materials using a Resonance Method; Transport and Road Research Laboratory (TRRL): Crowthorne, UK, 1974. [Google Scholar]
- Trollope, D.; Lee, I.; Morris, J. Stresses and deformation in two layer pavement structures under slow repeated loading. Proc. Aust. Road Res. Board 1962, 1, 693–721. [Google Scholar]
- Hopkins, C.; Cameron, D.; Rahman, M. On Site Improvement of Fines-Rich Unbound Granular Materials with Hydrophobic Polymer and Lime. Sustainability 2021, 13, 13479. [Google Scholar] [CrossRef]
- Uzan, J. Characterization of granular material. Transp. Res. Rec. 1985, 1022, 52–59. [Google Scholar]
- Uzan, J. Resilient characterization of pavement materials. Int. J. Numer. Anal. Methods Geomech. 1992, 16, 453–459. [Google Scholar] [CrossRef]
- Yaghoubi, E.; Mansourkhaki, E. Effect of “n” Exponent in Fuller Equation of Gradation on Hot Mix Asphalt Resistance to Permanent Deformation. Int. J. Pavement Res. Technol. 2010, 3, 336–342. [Google Scholar]
- ASTM D1557-12; Standard Test Methods for Laboratory Compaction Characteristics of Soil Using Modified Effort (56,000 Ft-Lbf/Ft3 (2,700 KN-M/M3)). ASTM International: West Conshohocken, PA, USA, 2009.
- Alnedawi, A.; Nepal, K.P.; Al-Ameri, R. Permanent Deformation Prediction Model of Unbound Granular Materials for Flexible Pavement Design. Transp. Infrastruct. Geotechnol. 2019, 6, 39–55. [Google Scholar] [CrossRef]
- ASTM C131-06; Standard Test Method for Resistance to Degradation of Small-Size Coarse Aggregate by Abrasion and Impact in the Los Angeles Machine. American Society for Testing and Materials: West Conshohocken, PA, USA, 1989.
- BSI. Methods for Determination of Aggregate Impact Value (AIV): BS 812–112; BSI: London, UK, 1990. [Google Scholar]
- ASTM D7172-14; Standard Test Method for Relative Density (Specific Gravity) and Absorption of Coarse Aggregate. ASTM: West Conshohocken, PA, USA, 2015.
- ASTM C127-12; Standard Test Method for Density, Relative Density (Specific Gravity), and Absorption of Coarse Aggregate: C127-12. ASTM: West Conshohocken, PA, USA, 2012.
- AASHTO T-307; Standard Method of Test for Determining the Resilient Modulus of Soils and Aggregate Materials. American Association of State Highway and Transportation Officials: Washington, DC, USA, 2003; p. 99.
- Cabrera, A. Evaluation of the Laboratory Resilient Modulus Test using a New Mexico Subgrade Soil; University of New Mexico: Albuquerque, NM, USA, 2012. [Google Scholar]
- LTPP Protocol. P46, Resilient Modulus of Unbound Granular Base/Subbase Materials and Subgrade Soils. In Long-Term Pavement Performance; U.S. Department of Transportation: McLean, VA, USA, 1996.
- Zhang, J.T.; Liang, X. One-way ANOVA for functional data via globalizing the pointwise F-test. Scand. J. Stat. 2014, 41, 51–71. [Google Scholar] [CrossRef]








| Model | Author | Equation | Variable | Remarks |
|---|---|---|---|---|
| (Uzan Model) [44] | Uzan | = k1 Pa (θ/Pa)k2 * (σd/Pa)k3 | k1, k2 = Material constants Pa = Atmospheric pressure | More precisely models the nonlinearity of granular soils |
| AASHTO Model [5] | = k1σd k2 | k1, k2 = Material constants | Uses least square regression analysis | |
| Universal Model (Modified Uzan model) (Uzan et al. 1992) [45] | Uzan | = k1Pa (θ/Pa)k2 * (/Pa)k3 | k1, k2 = Material constants Pa = Atmospheric pressure | Bulk stress and deviator stress effects are considered |
| K-Θ Model [44] | Uzan | = A(3pmax)B | A,B = Material constants Pmax = Max atmospheric pressure | Poisson’s ratio is assumed to be constant. No effect of dev. stress is considered |
| Boyce Model [5] | Boyce (1980) | εv = pA*(1/k1) [1 − β (q2/p2)] εs = (pB/3C)*(q/p) | A, C = Material constants controlled by B | The model is nonlinear elastic and isotropic |
| Gradation Coefficients (n) | Max Dry Density kg/m³ | Optimum Moisture Content % |
|---|---|---|
| 0.6 | 2432.40 | 4.03 |
| 0.5 | 2406.93 | 4.3 |
| 0.4 | 2395.72 | 4.8 |
| 0.3 | 2381.46 | 5.42 |
| Gradation Coefficient | OMC% | OMC + 1% | OMC − 1% | OMC − 2% | Dry |
|---|---|---|---|---|---|
| 0.6 | 4.03 | 5.03 | 3.03 | 2.03 | mc = 0 |
| 0.5 | 4.3 | 5.3 | 3.3 | 2.3 | mc = 0 |
| 0.4 | 4.8 | 5.8 | 3.8 | 2.8 | mc = 0 |
| 0.3 | 5.42 | 6.42 | 4.42 | 3.42 | mc = 0 |
| S. No | Description | Designation | Result | Allowable Limits |
|---|---|---|---|---|
| 1 | Aggregate Abrasion Value % [49] | C 131 | 21 | <40 |
| 2 | Aggregate Impact Value % [50] | BS 812–112 | 14 | <40 |
| 3 | Water Absorption of Coarse Aggregates % [51] | C 128 | 0.57 | <2 |
| 4 | Specific Gravity of Coarse Aggregate [52] | C 127 | 2.63 | 2.5–2.9 |
| Sequence Number | Confining Pressure, σ3 (kPa) | Maximum Axial Stress, σd (kPa) | Cyclic Stress, σcd (kPa) | Contact Stress, σcontact (kPa) | Number of Load Applications |
|---|---|---|---|---|---|
| Conditioning | 103.4 | 103.4 | 93.1 | 1.5 | 500–1000 |
| 1 | 20.7 | 20.7 | 18.6 | 2.1 | 100 |
| 2 | 20.7 | 41.4 | 37.3 | 4.1 | 100 |
| 3 | 20.7 | 62.1 | 55.9 | 6.2 | 100 |
| 4 | 34.5 | 34.5 | 31.0 | 3.5 | 100 |
| 5 | 34.5 | 68.9 | 62.0 | 6.9 | 100 |
| 6 | 34.5 | 103.4 | 93.1 | 10.3 | 100 |
| 7 | 68.9 | 68.9 | 62.0 | 6.9 | 100 |
| 8 | 68.9 | 137.9 | 124.1 | 13.8 | 100 |
| 9 | 68.9 | 206.8 | 186.1 | 20.7 | 100 |
| 10 | 103.4 | 68.9 | 62.0 | 6.9 | 100 |
| 11 | 103.4 | 103.4 | 93.1 | 10.3 | 100 |
| 12 | 103.4 | 206.8 | 186.1 | 20.7 | 100 |
| 13 | 137.9 | 103.4 | 93.1 | 10.3 | 100 |
| 14 | 137.8 | 137.9 | 124.1 | 13.8 | 100 |
| 15 | 137.9 | 275.8 | 248.2 | 27.6 | 100 |
| Effect of Moisture on UGM by Changing Theta and Gradation | |||||||
|---|---|---|---|---|---|---|---|
| Theta | n | k1 | k2 | p- | F- | ||
| 196.5 | 0.3 | 5705.8 | −712.35 | 0.94 | 0 | 0.94 | 41,092 |
| 392.7 | 0.3 | 7128.4 | −907.1 | 0.93 | 0 | 0.93 | 53,360 |
| 496.2 | 0.3 | 7956.9 | −986.01 | 0.98 | 0 | 0.98 | 33,749 |
| 661.7 | 0.3 | 8581.4 | −963.76 | 0.97 | 0 | 0.97 | 37,130 |
| 196.5 | 0.4 | 3709.3 | −319.99 | 0.93 | 0 | 0.93 | 17,324 |
| 392.7 | 0.4 | 5057.3 | −448.19 | 0.93 | 0 | 0.93 | 23,958 |
| 496.2 | 0.4 | 5961.9 | −585.82 | 0.94 | 0 | 0.94 | 28,193 |
| 661.7 | 0.4 | 7404 | −774.95 | 0.92 | 0 | 0.92 | 45,509 |
| 196.5 | 0.5 | 4433.8 | −528.15 | 0.95 | 0 | 0.95 | 20,986 |
| 392.7 | 0.5 | 5434 | −575.46 | 0.95 | 0 | 0.95 | 23,711 |
| 496.2 | 0.5 | 5886.9 | −527.86 | 0.94 | 0 | 0.94 | 23,396 |
| 661.7 | 0.5 | 6949.2 | −602.11 | 0.94 | 0 | 0.94 | 26,509 |
| 196.5 | 0.6 | 4422.6 | −526.41 | 0.91 | 0 | 0.91 | 29,166 |
| 392.7 | 0.6 | 6093.8 | −768.35 | 0.91 | 0 | 0.91 | 41,358 |
| 496.2 | 0.6 | 6095.1 | −683.1 | 0.86 | 0 | 0.86 | 47,719 |
| 661.7 | 0.6 | 7430.4 | −872.65 | 0.91 | 0 | 0.91 | 46,875 |
| Model Training Regression Parameters and Goodness-of-Fit Tests | |||||||||
|---|---|---|---|---|---|---|---|---|---|
| n | k1 | k2 | k3 | k4 | R2 | adj R2 | p-Value | F-Value | RMSE |
| 0.3 | 5191.7 | 0.0691 | 0.1652 | −714.36 | 0.89 | 0.88 | 0.00 | 0.89 | 73,667 |
| 0.4 | 3687.7 | 0.1530 | 0.1654 | −410.53 | 0.80 | 0.78 | 0.00 | 0.81 | 64,009 |
| 0.5 | 3448.6 | 0.0616 | 0.2667 | −433.28 | 0.91 | 0.91 | 0.00 | 0.93 | 39,910 |
| 0.6 | 4918.6 | 0.2397 | −0.0061 | −551.76 | 0.78 | 0.76 | 0.00 | 0.79 | 71,683 |
| Model Testing Regression Parameters and Goodness-of-Fit Tests | |||||||||
|---|---|---|---|---|---|---|---|---|---|
| n | k1 | k2 | k3 | k4 | R2 | adj R2 | p-Value | F-Value | RMSE |
| 0.3 | 5191.7 | 0.0691 | 0.1652 | −714.36 | 0.76 | 0.73 | 0.00 | 1.23 | 50,008 |
| 0.4 | 3687.7 | 0.1530 | 0.1654 | −410.53 | 0.76 | 0.72 | 0.00 | 0.90 | 48,587 |
| 0.5 | 3448.6 | 0.0616 | 0.2667 | −433.28 | 0.88 | 0.86 | 0.00 | 0.93 | 36,376 |
| 0.6 | 4918.6 | 0.2397 | −0.0061 | −551.76 | 0.55 | 0.47 | 0.00 | 0.90 | 65,674 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ullah, S.; Jamal, A.; Almoshaogeh, M.; Alharbi, F.; Hussain, J. Investigation of Resilience Characteristics of Unbound Granular Materials for Sustainable Pavements. Sustainability 2022, 14, 6874. https://doi.org/10.3390/su14116874
Ullah S, Jamal A, Almoshaogeh M, Alharbi F, Hussain J. Investigation of Resilience Characteristics of Unbound Granular Materials for Sustainable Pavements. Sustainability. 2022; 14(11):6874. https://doi.org/10.3390/su14116874
Chicago/Turabian StyleUllah, Salamat, Arshad Jamal, Meshal Almoshaogeh, Fawaz Alharbi, and Jawad Hussain. 2022. "Investigation of Resilience Characteristics of Unbound Granular Materials for Sustainable Pavements" Sustainability 14, no. 11: 6874. https://doi.org/10.3390/su14116874
APA StyleUllah, S., Jamal, A., Almoshaogeh, M., Alharbi, F., & Hussain, J. (2022). Investigation of Resilience Characteristics of Unbound Granular Materials for Sustainable Pavements. Sustainability, 14(11), 6874. https://doi.org/10.3390/su14116874








