Quantitative Study on the Law of Surface Subsidence Zoning in Steeply Inclined Extra-Thick Coal Seam Mining
Abstract
:1. Introduction
2. Materials and Methods
2.1. Overview of Study Area
2.2. Numerical Simulation
2.2.1. Introduction of 3DEC Numerical Simulation Software
2.2.2. Scheme Design and Parameter Determination
- (1)
- Scheme design
- (2)
- Parameter determination
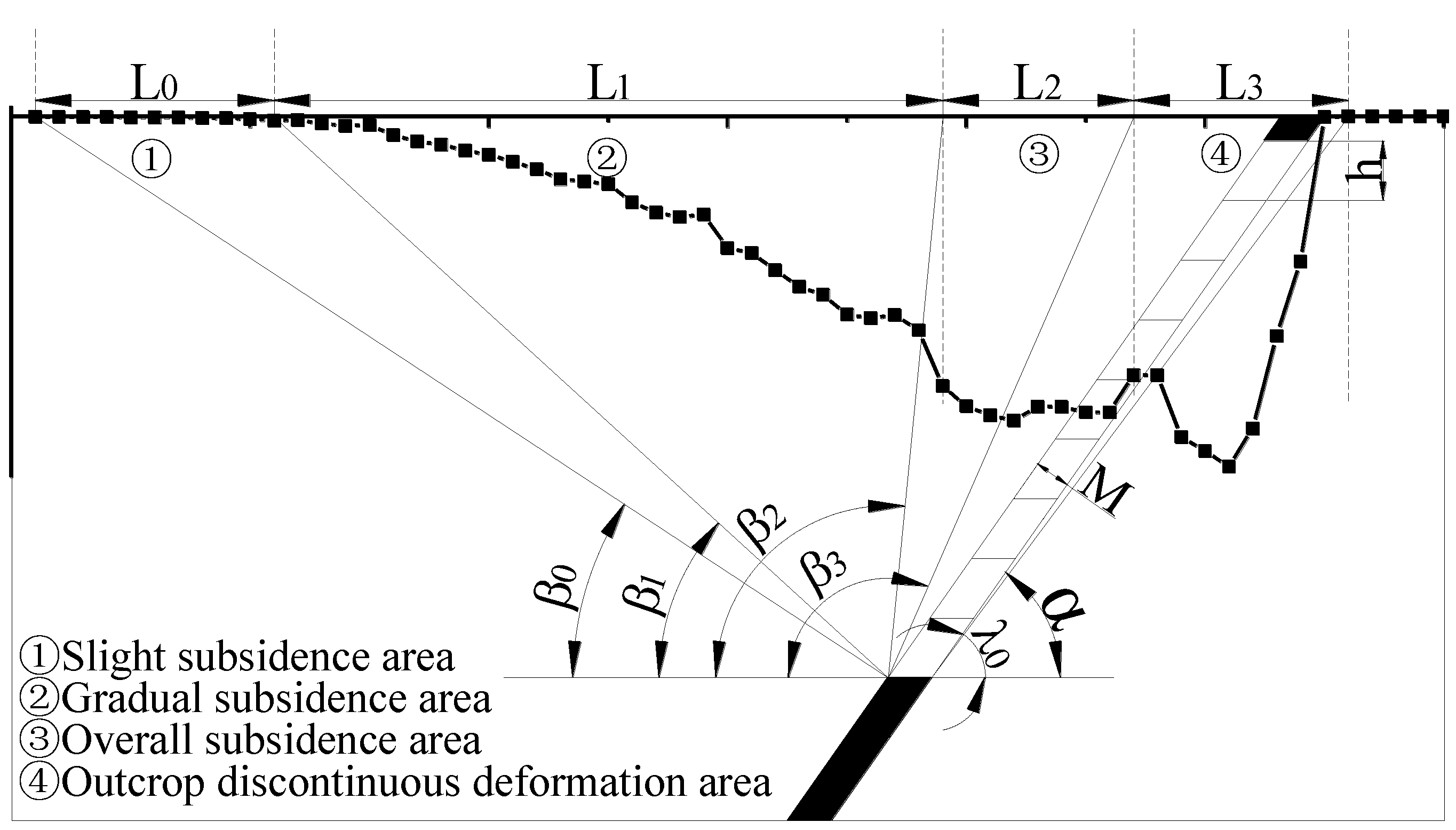
3. Quantitative Method of Surface Subsidence Zoning
- (1)
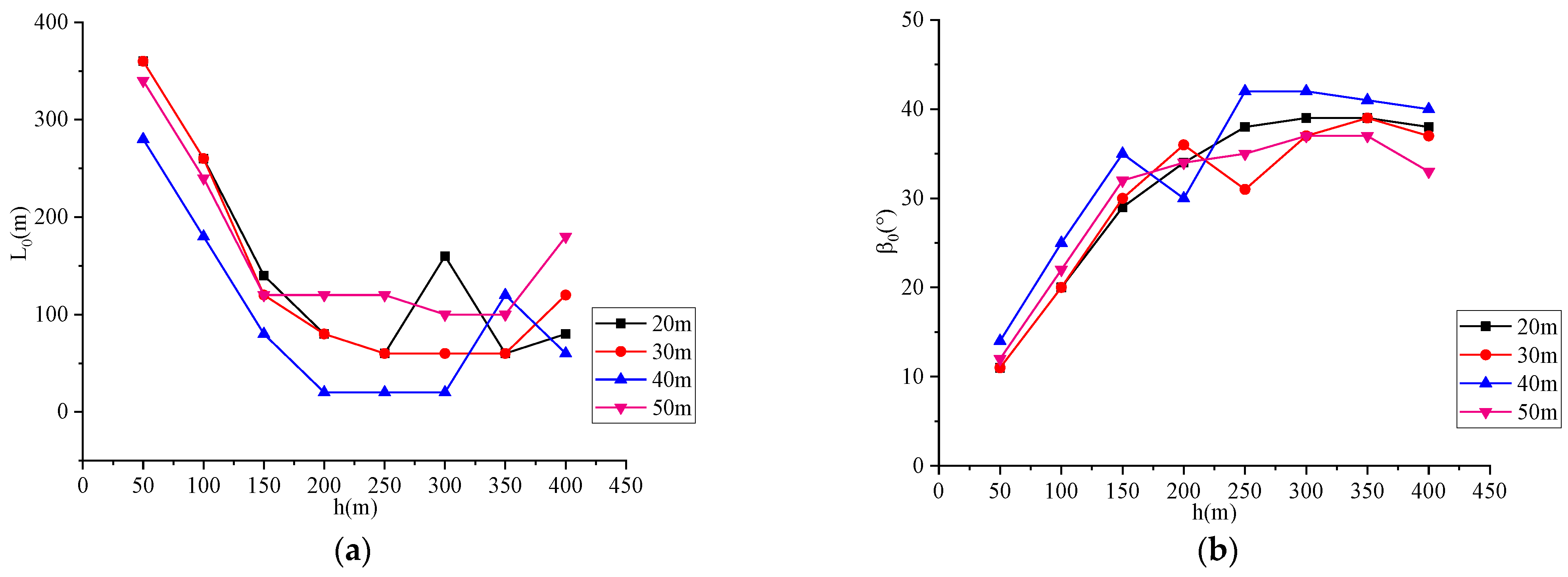
- Floor boundary angle (λ0)
- (2)
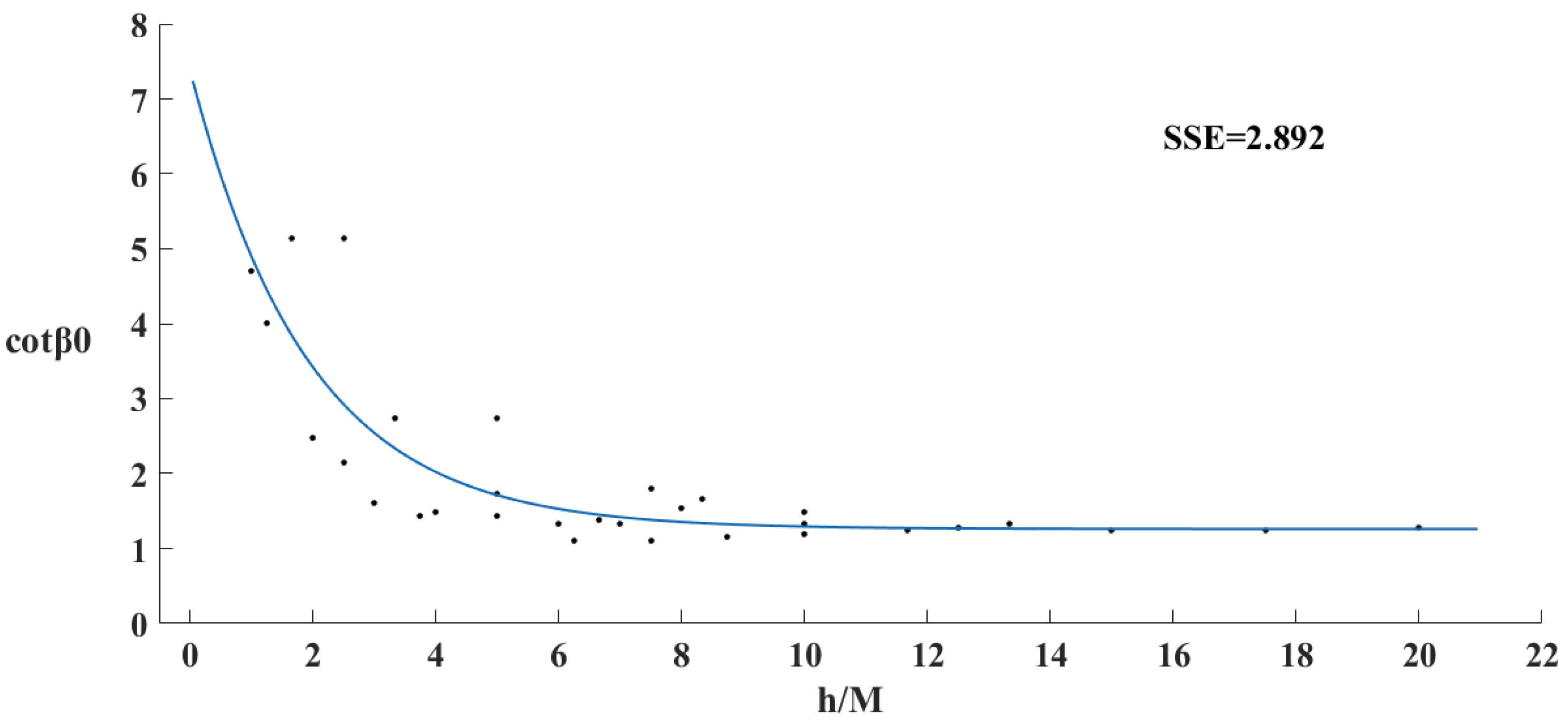
- Roof boundary angle (β0)
- (3)
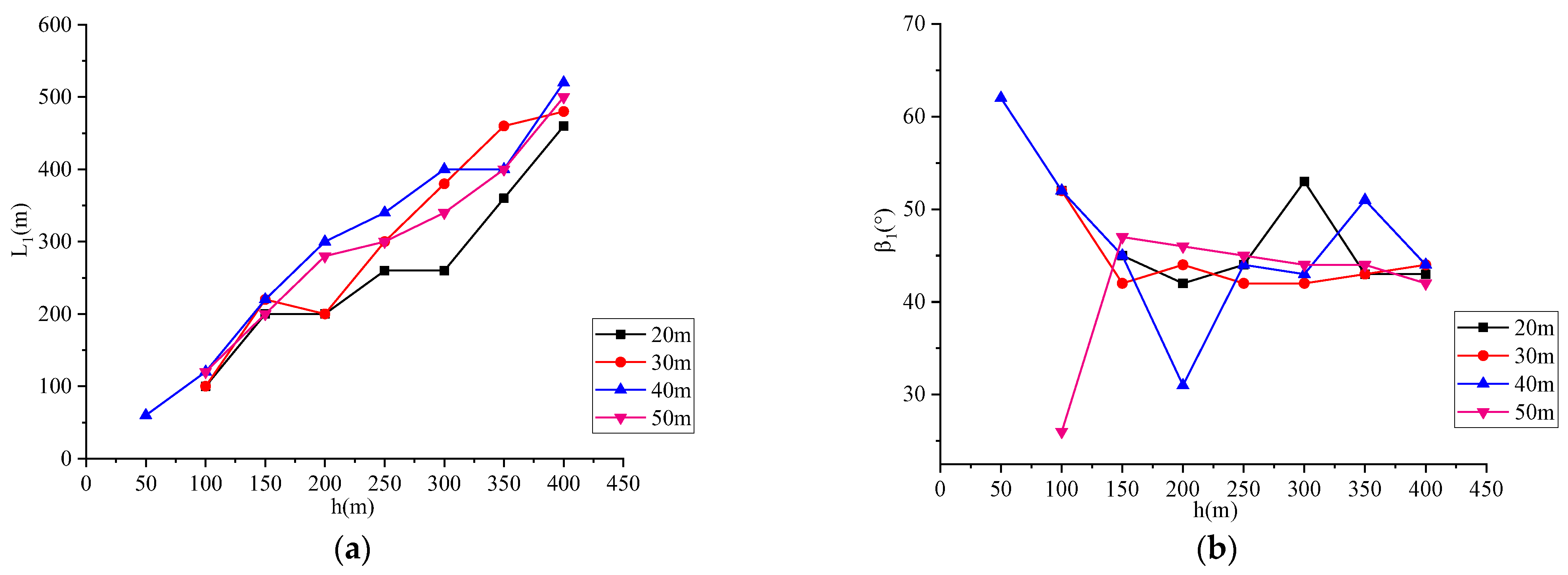
- Zoning angle of the gradual subsidence area (β1)
- (4)
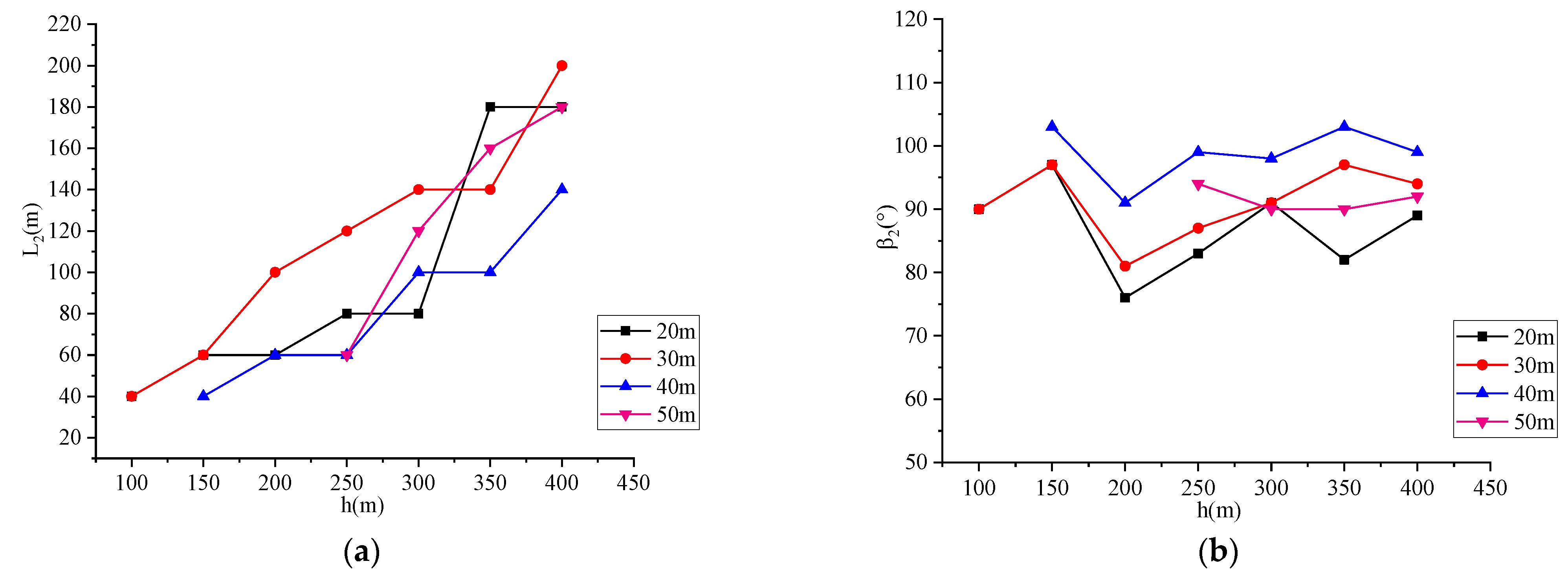
- Zoning angle of the overall subsidence area (β2)
- (5)
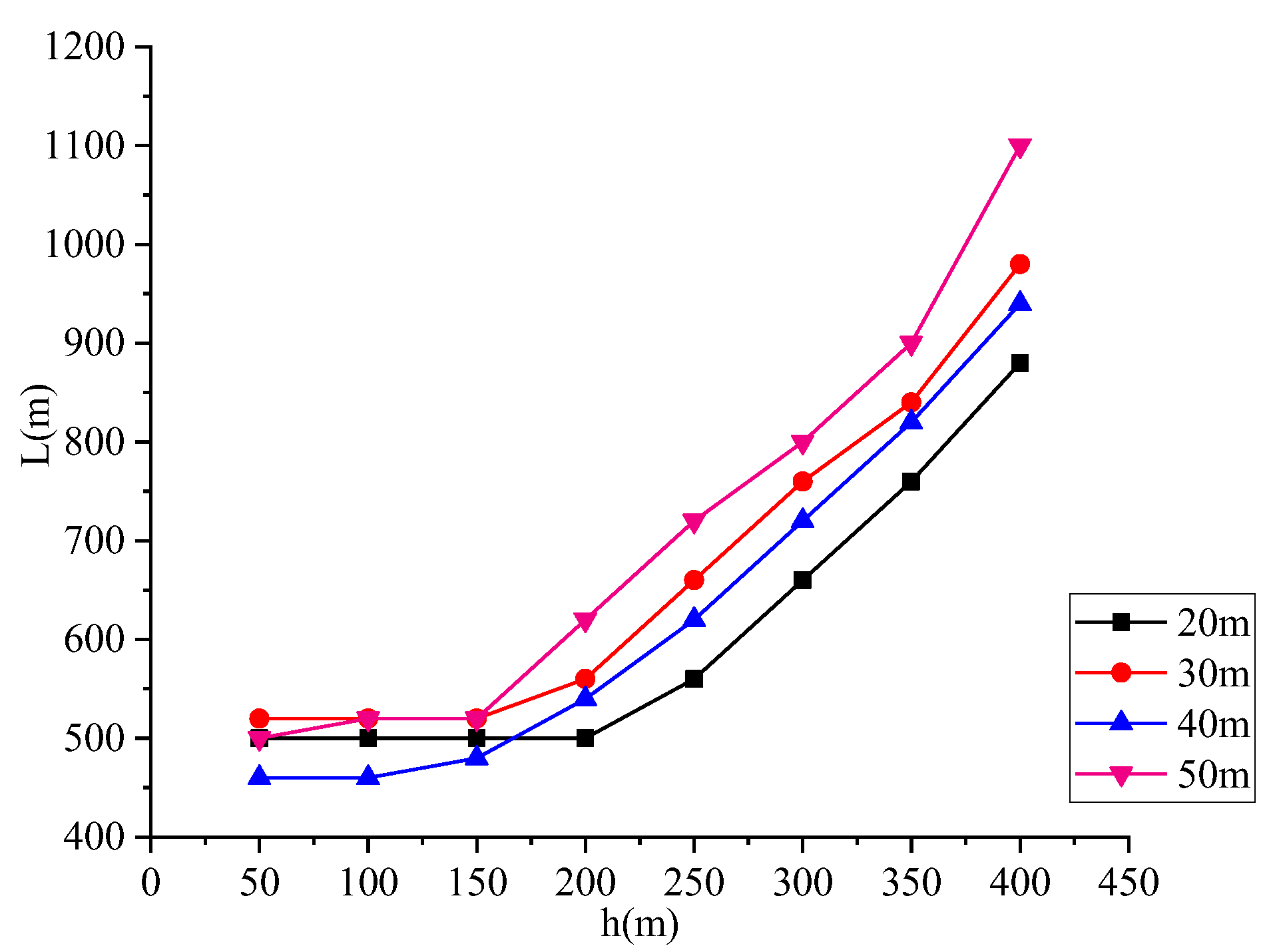
- Zoning angle of the outcrop discontinuous deformation area (β3)
- (6)
- L3: range of the outcrop discontinuous deformation area; L2: range of the overall subsidence area; L1: range of the gradual subsidence area; L0: range of the slight subsidence area.
4. Results and Analysis
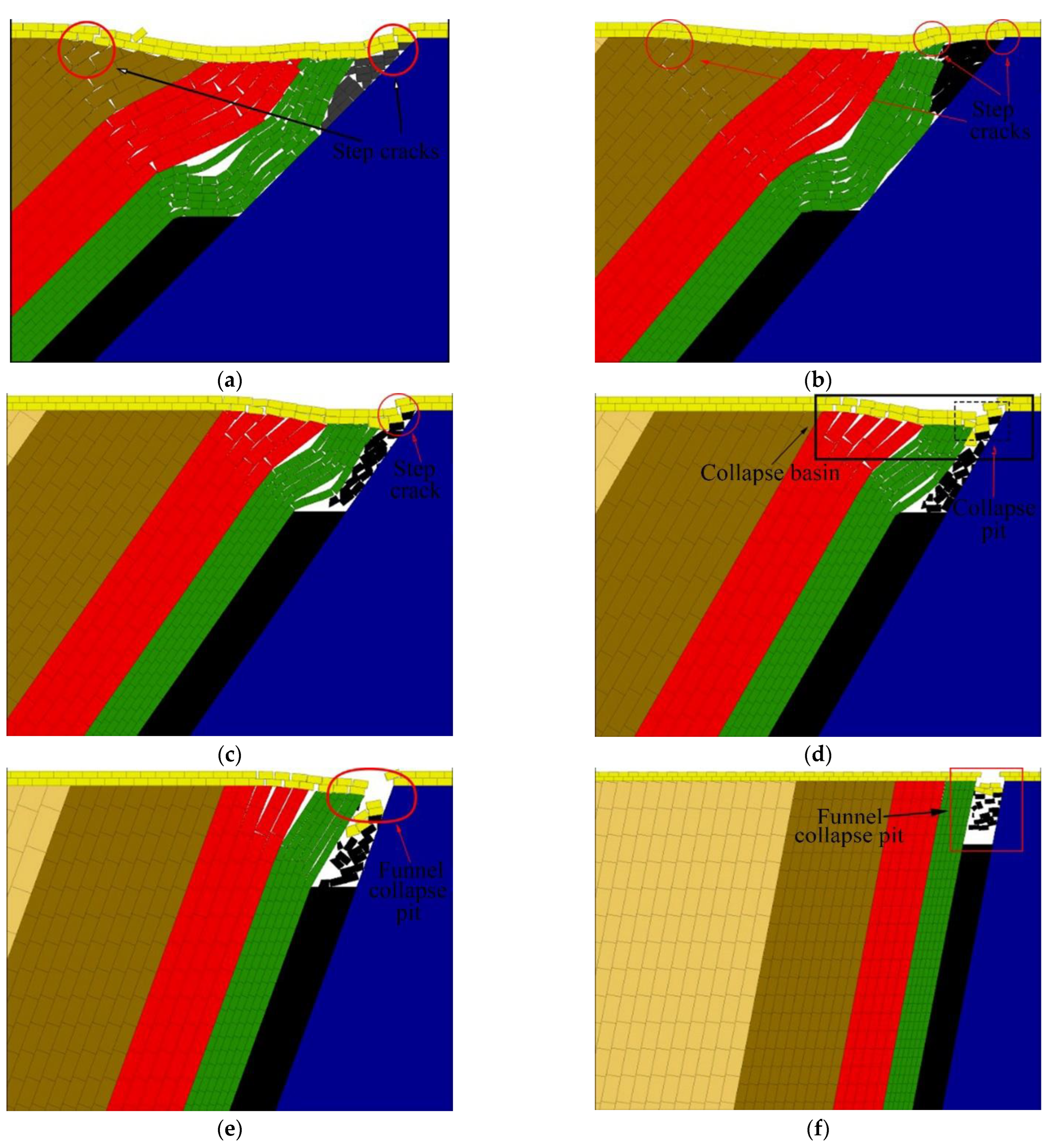
4.1. Division of Surface Subsidence Zoning
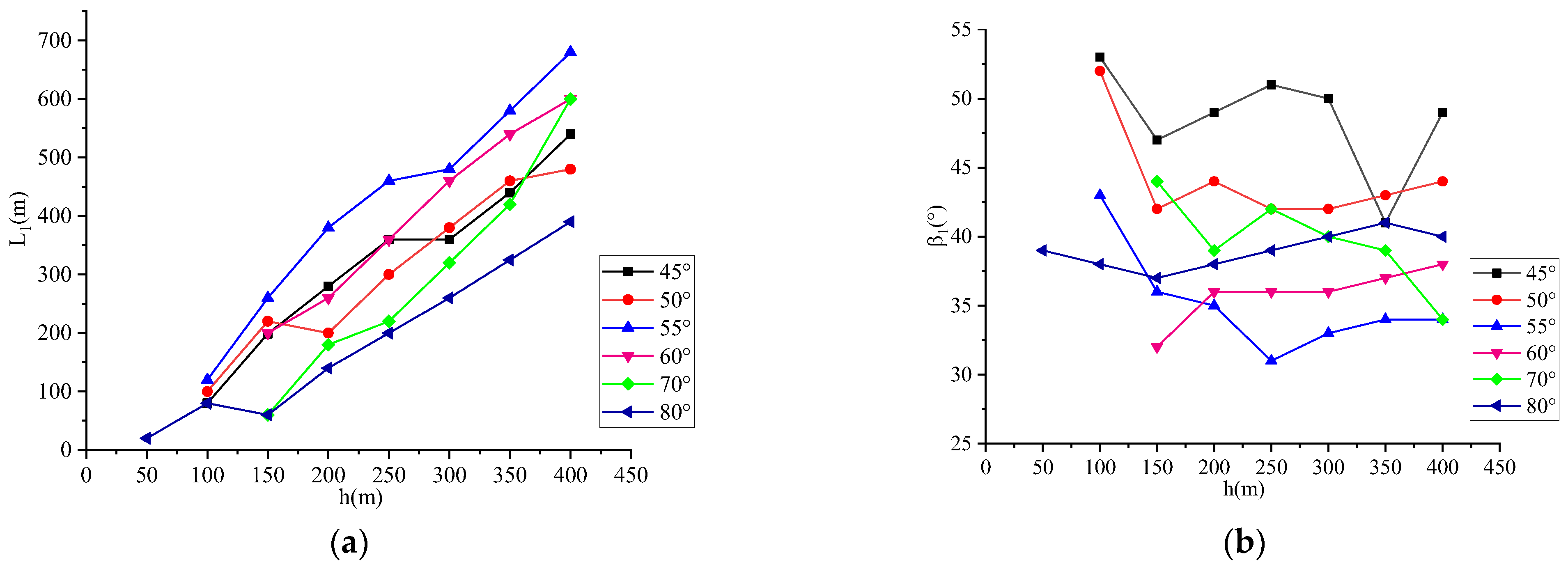
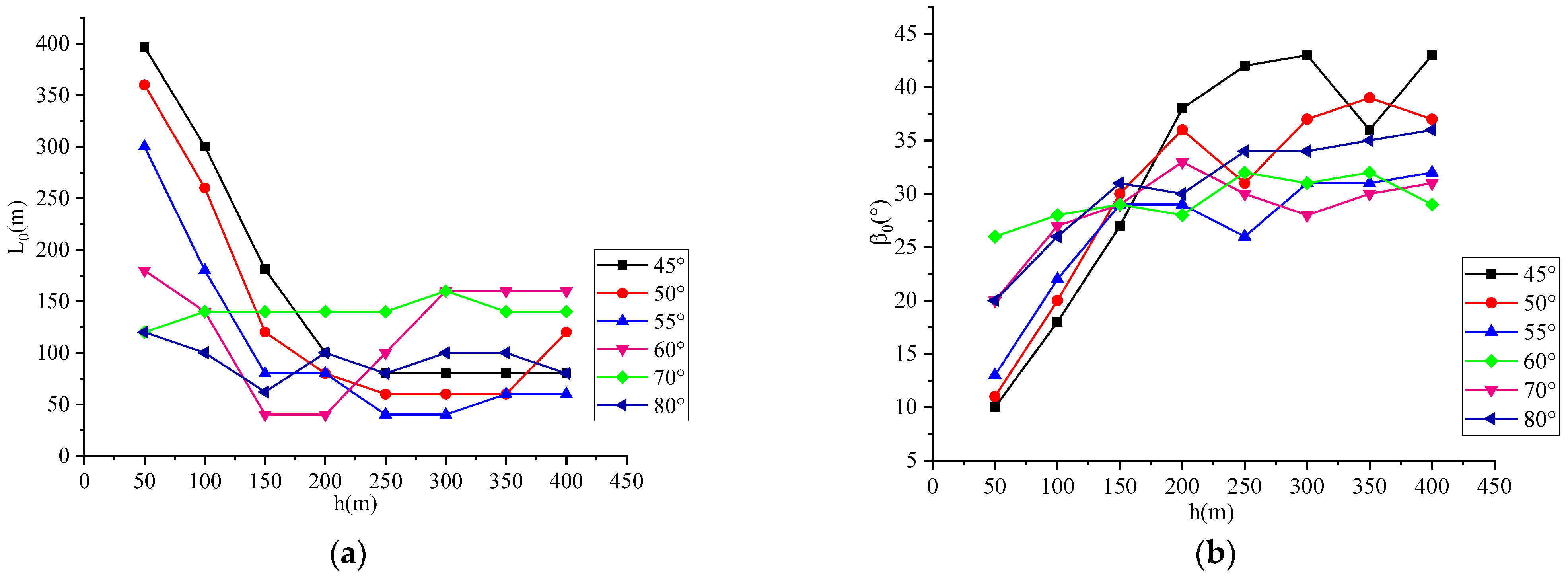
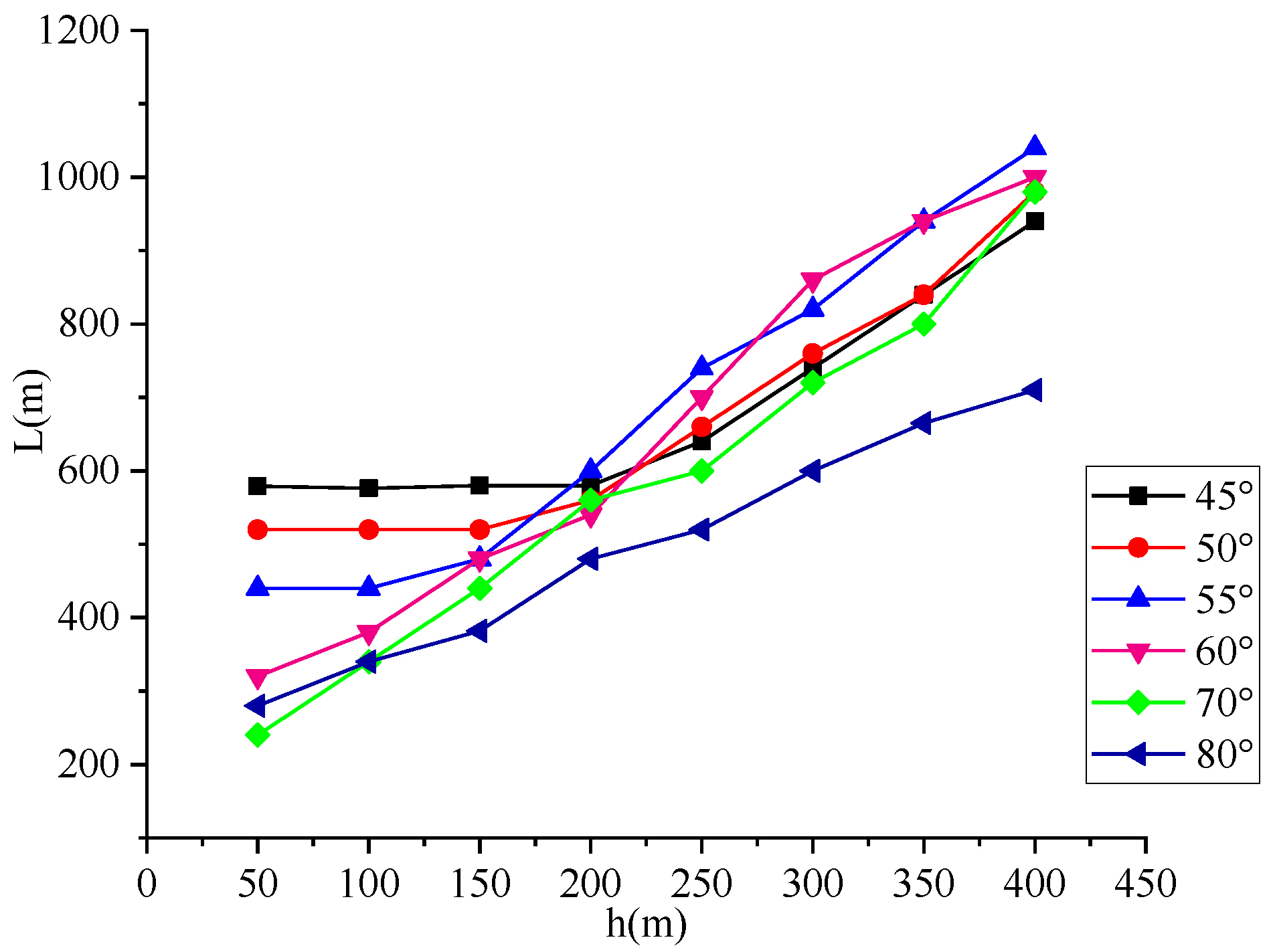
4.2. Analysis of the Law of Surface Subsidence Zoning with the Change in the Coal Seam Dip Angle
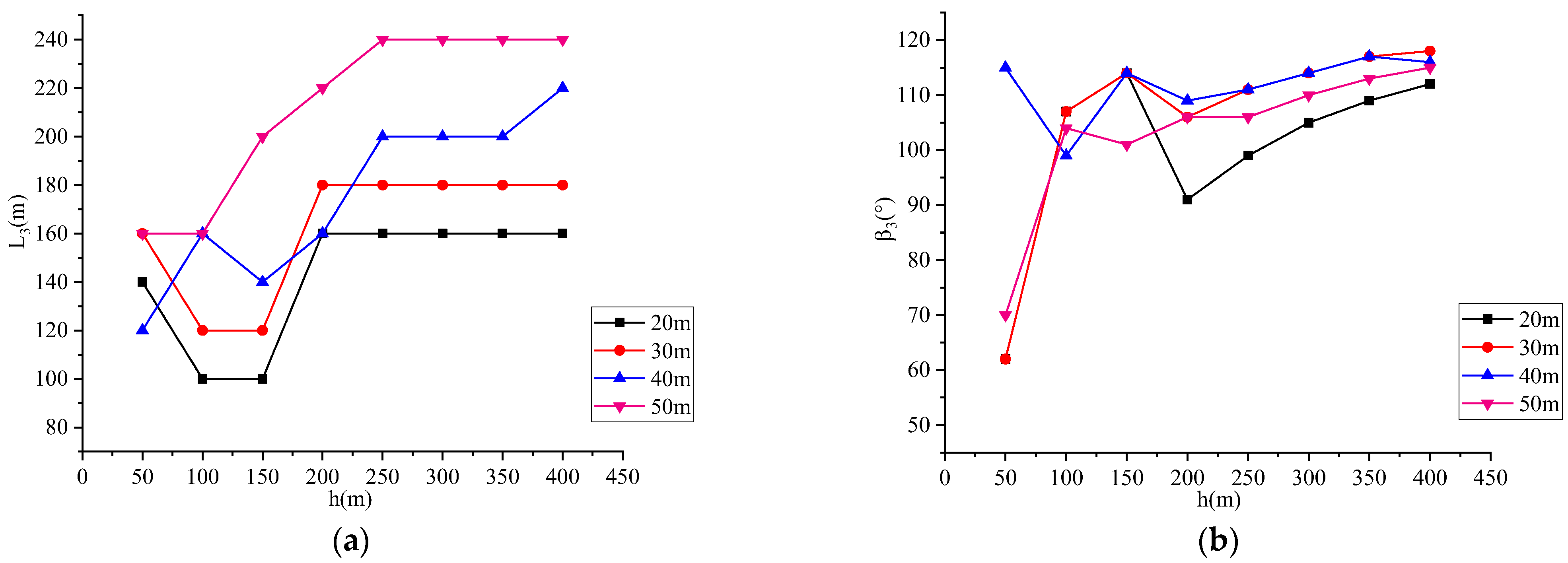
4.3. Analysis of the Law of Surface Subsidence Zoning with the Change in the Coal Thickness
5. Discussion
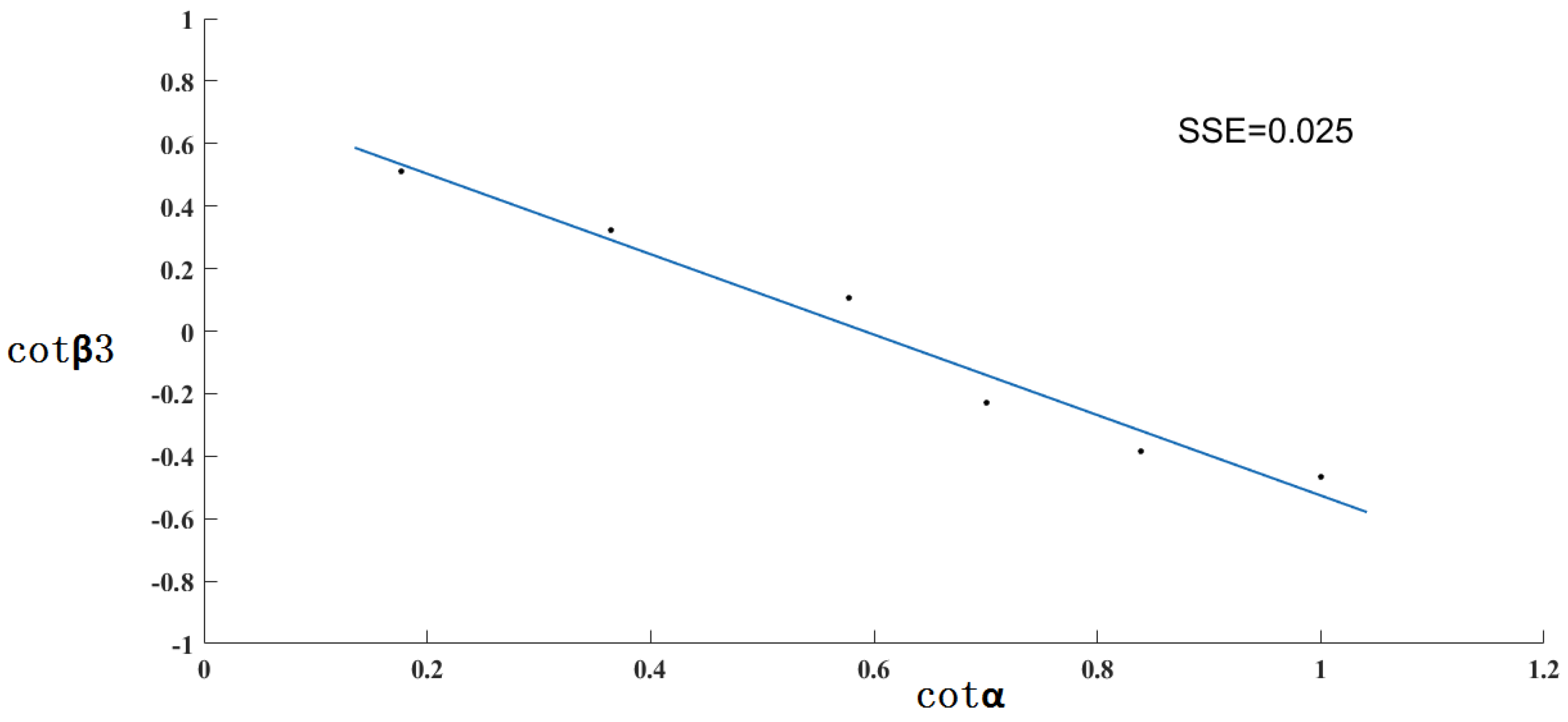
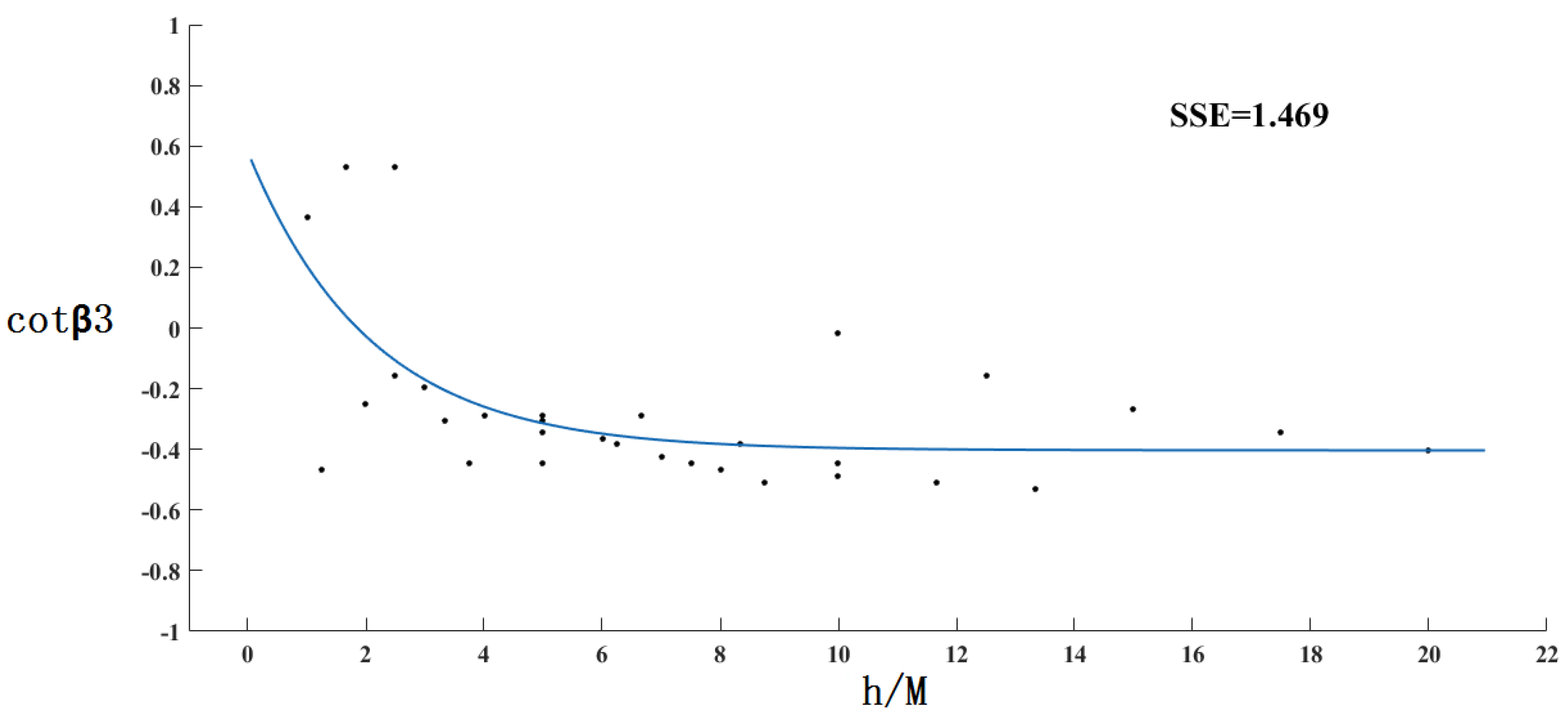
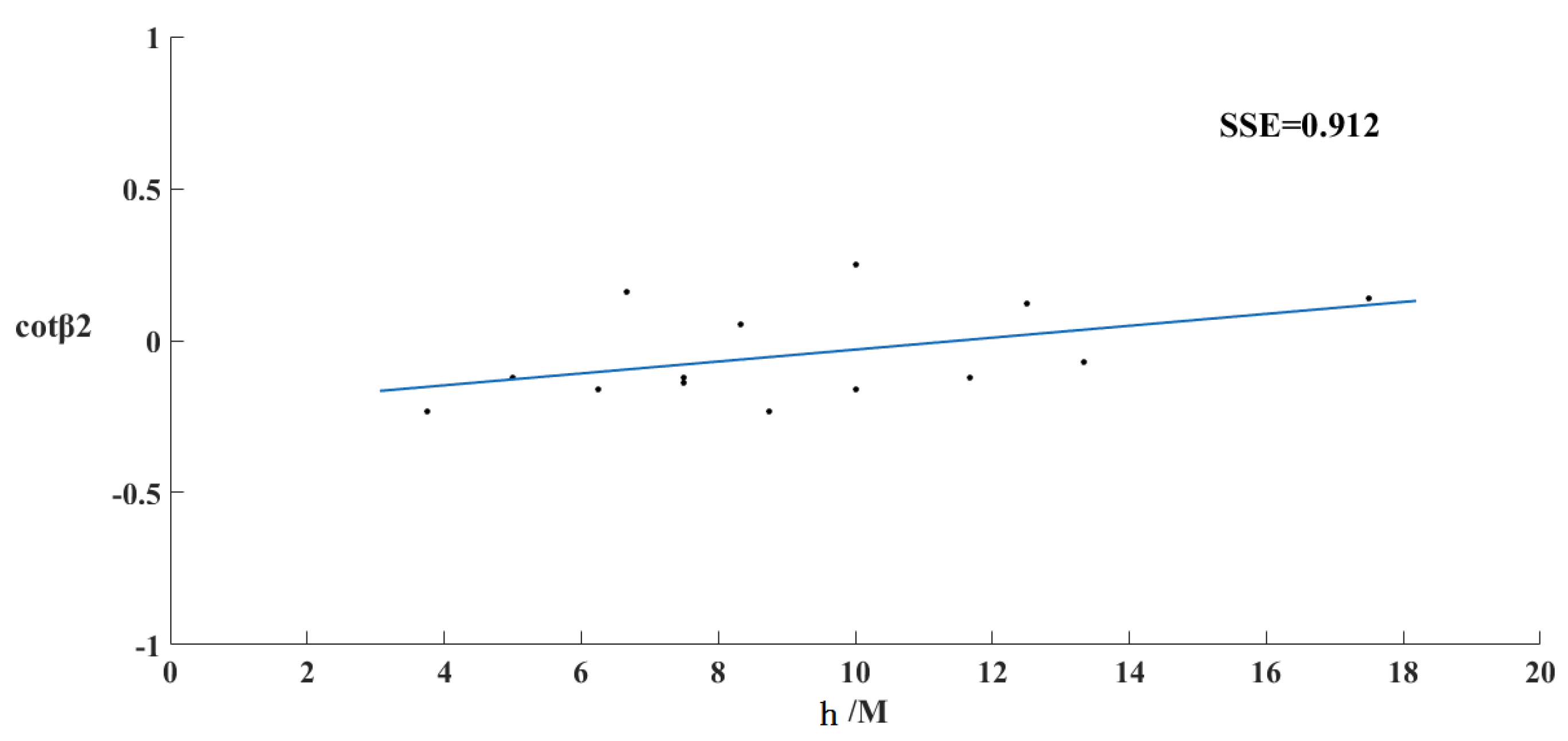
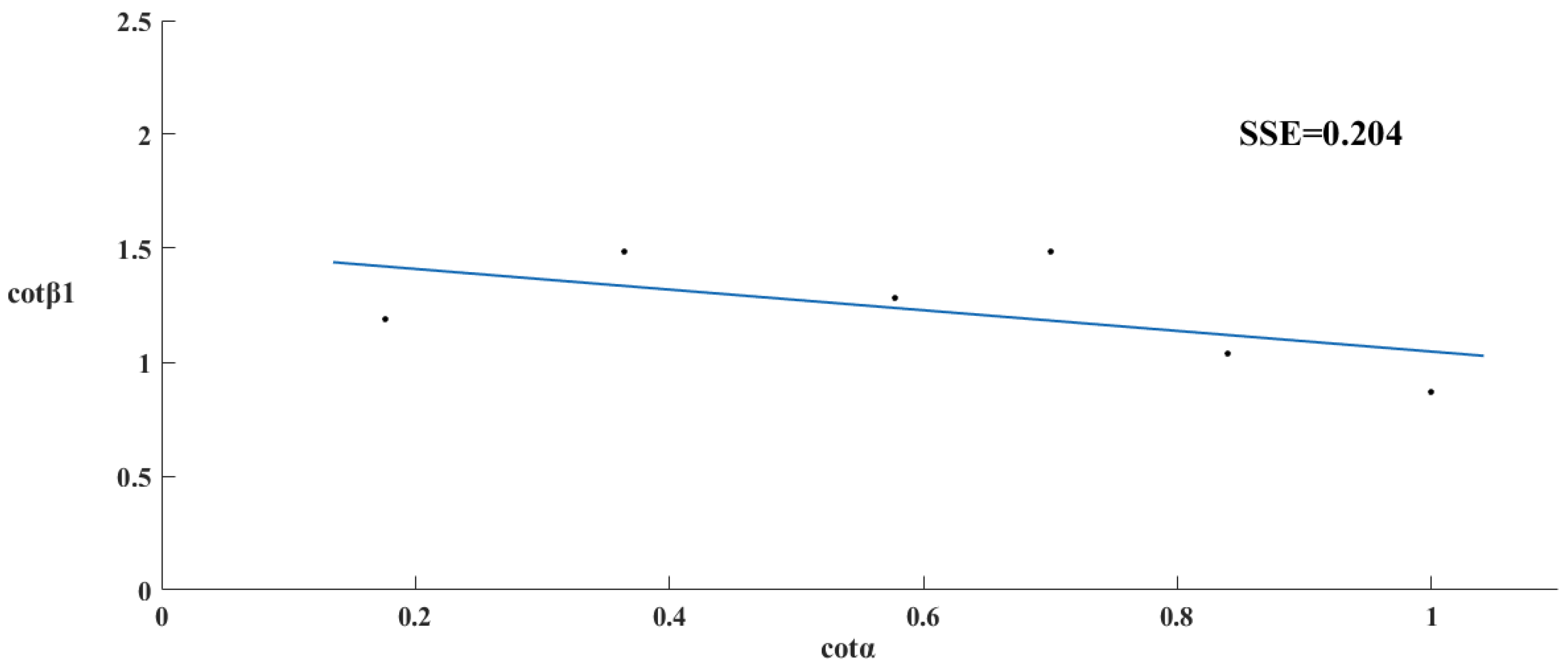
5.1. Variation Law of the Outcrop Discontinuous Deformation Area
5.2. Variation Law of the Overall Subsidence Area
5.3. Variation Law of the Gradual Subsidence Area
5.4. Variation Law of the Slight Subsidence Area
6. Conclusions
- (1)
- Surface subsidence basins can be divided into four areas: the outcrop discontinuous deformation area, the overall subsidence area, the gradual subsidence area, and the slight subsidence area;
- (2)
- The influence of the coal seam dip angle on surface subsidence zoning of steeply inclined extra-thick coal seam mining is mainly reflected in the range of the affected area and the form of damage;
- (3)
- The influence of coal thickness on surface subsidence zoning of steeply inclined thick coal seam mining is mainly reflected in the surface subsidence and the degree of damage. On the whole, a larger coal thickness can increase the range of surface subsidence basins and subsidence zoning.
Author Contributions
Funding
Conflicts of Interest
References
- Lai, X.P.; Dai, J.J.; Li, C. Analysis on hazard characteristics of overburden structure in steeply inclined coal seam. J. China Coal Soc. 2020, 45, 122–130. [Google Scholar]
- Sun, C.; Chen, D.X.; Cheng, Y.H.; Lu, J.X. Study on collapse rule and control of hard roofs in steeply inclined coal seams. Chin. J. Rock Mech. Eng. 2019, 38, 1647–1658. [Google Scholar]
- Wang, J.C.; Yang, S.L.; Li, L.H. Toppling-slumping failure mode in horizontal sublevel top-coal caving face in steeply-inclined seam. J. China Univ. Min. Technol. 2018, 47, 1175–1184. [Google Scholar]
- Zhang, J.W. The fracture mechanism of main roof stratum and strong mine pressure control method in long wall mining of steeply inclined coal seam in Wangjiashan colliery. Chin. J. Rock Mech. Eng. 2018, 37, 1776. [Google Scholar]
- Huang, X.C. Study on stress and fracture evolution law of steeply inclined coal seam. Coal Sci. Technol. 2018, 46, 93–96. [Google Scholar]
- Zhong, H.L. Experience of Coal Mining under Buildings in Dunbas Mining Area of Soviet Union. Coal Min. Technol. 1991, 2, 55–58. [Google Scholar]
- Afsari, N. Numerical modelling of surface subsidence arising from longwall mining of steeply inclined coal seams. In Proceedings of the Turkish Coal Congress, Bartm-Amasra, Turkey, 10–12 June 1998. [Google Scholar]
- Alejano, L.R.; Ramírez-Oyanguren, P.; Taboada, J. FDM predictive methodology of subsidence due to flat and inclined coal seam mining. Int. J. Rock Mech. Min. Sci. 1999, 36, 475–491. [Google Scholar] [CrossRef]
- Asadi, A.; Shahriar, K.; Goshtasbi, K.; Najm, K. Development of a new mathematical model for prediction of surface subsidence due to inclined coal-seam mining. J. S. Afr. Inst. Min. Metall. 2005, 105, 15–20. [Google Scholar]
- Ma, F.; Sun, L.; Li, D. Numerical simulation analysis of covering rock strata as mining steep-inclined coal seam under fault movement. Trans. Nonferrous Met. Soc. China 2011, 21, 556–561. [Google Scholar] [CrossRef]
- Wang, M.L.; Hu, B.N.; Cheng, X.H.; Zhang, G.Y. Numerical simulation of overburden failure and coal pillar stabilityin steep multi-seam mining. Coal Min. Technol. 2005, 10, 64–66. [Google Scholar]
- Yang, F. Study on Overburden Strata’s Movement Pattern and Mechanism of Mining Steeply Inclined Seams. Ph.D. Thesis, Liaoning Technical University, Shenyang, China, 2006. [Google Scholar]
- Yi, S.H. The Research on Surface Subsidence Due to Mining Horizontal Slice of Extra-Thick and Steep Coal Seam. Ph.D. Thesis, China University of Mining and Technology, Beijing, China, 2009. [Google Scholar]
- Dai, H.Y.; Tiao, X.G.; Zhao, S.L. Rules of surface movement induced by mining steeply inclined coal seam in majiagou colliery. Coal Min. Technol. 2010, 15, 48–50. [Google Scholar]
- Dai, H.Y. Study on seam inclination based mining subsidence model and its visualized application with GIS. Chin. J. Rock Mech. Eng. 2002, 21, 148. [Google Scholar]
- Yan, Y.G.; Guo, S.C.; Liu, J.B.; Zhang, G.G.; Wang, H.L. Principle and rock strata control effect based on mining mixed with backfilling and keeping. Coal Min. Technol. 2016, 21, 64–68. [Google Scholar]
- Yan, Y.G.; Dai, H.Y.; Wang, Z.W.; Jing, S.Q.; Chen, H.Z. Ground subsidence zone and surrounding rock failure mechanism due to steep multiple coal seam mining: A case study at Muchenyjian Datai Mine. J. China Univ. Min. Technol. 2013, 42, 547–553. [Google Scholar]
- Yan, Y.G.; Dai, H.Y.; Wang, Z.W.; Zhu, G.H.; Chen, H.Z.; Xu, C. Study on failure characteristics and normal height of caving zone due to steep seam mining. Coal Sci. Technol. 2015, 43, 23–26. [Google Scholar]
- Zhang, C.; Zhao, Y.X.; Han, P.H.; Bai, Q.S. Coal pillar failure analysis and instability evaluation methods: A short review and prospect. Eng. Fail. Anal. 2022, 138, 06344. [Google Scholar] [CrossRef]
- Zhang, Y.; Yan, Y.; Dai, H.; Zhu, Y.; Wu, T. Stability and force chain characteristics of “inclined step cutting body” in stope. Appl. Sci. 2021, 11, 10276. [Google Scholar] [CrossRef]
- Emad, M.Z.; Mitri, H.; Kelly, C. Dynamic model validation using blast vibration monitoring in mine backfill. Int. J. Rock Mech. Min. Sci. 2018, 107, 48–54. [Google Scholar] [CrossRef]
- Wang, Y.; Ye, H.W.; Li, Y.Z. Numerical simulation on jointed and fractured rock blasting. Blasting 2012, 29, 20–22. [Google Scholar]
- Wang, H.; Gao, Y.T.; Jin, A.B.; Zhang, K. Determination of stiffness parameters of jointed rock masses with 3dec simulations. Chin. J. Rock Mech. Eng. 2014, 33, 2894–2900. [Google Scholar]
- Yan, Q.R.; Feng, J.; Yang, T.; Deng, W.D. Numerical experiment method to determining mechanical parameters of jointed rock mass. J. Tongji Univ. 2017, 45, 187–194. [Google Scholar]
- Yang, F.; Yu, H.F.; Guo, J.T. Mechanism of mining-induced surface crack by numerical simulation method. J. Liaoning Tech. Univ. 2016, 35, 566–570. [Google Scholar]
- Xu, F.Y. Surface Cracks Distribution Characteristics and Development Mechanism in High-Intensity Mining of Nearly Shallow Thick Coal Seam. Ph.D. Thesis, Henan Polytechnic University, Jiaozuo, China, 2019. [Google Scholar]
- Guo, W.B.; Xu, F.Y. Numerical simulation of overburden and surface movements for Wongawilli strip pillar mining. Int. J. Min. Sci. Technol. 2016, 26, 71–76. [Google Scholar] [CrossRef]
- Xu, Z.H.; Zhu, R.S.; He, W.R.; Li, X.B. Subsidence model of large working face in shallow buried coal seam with thick loose layer. J. Min. Saf. Eng. 2020, 37, 264–271. [Google Scholar]
- Wang, T.B. Research on the influence range of coal mining in mountainous areas of Guizhou. Coal Eng. 2018, 50, 141–144. [Google Scholar]
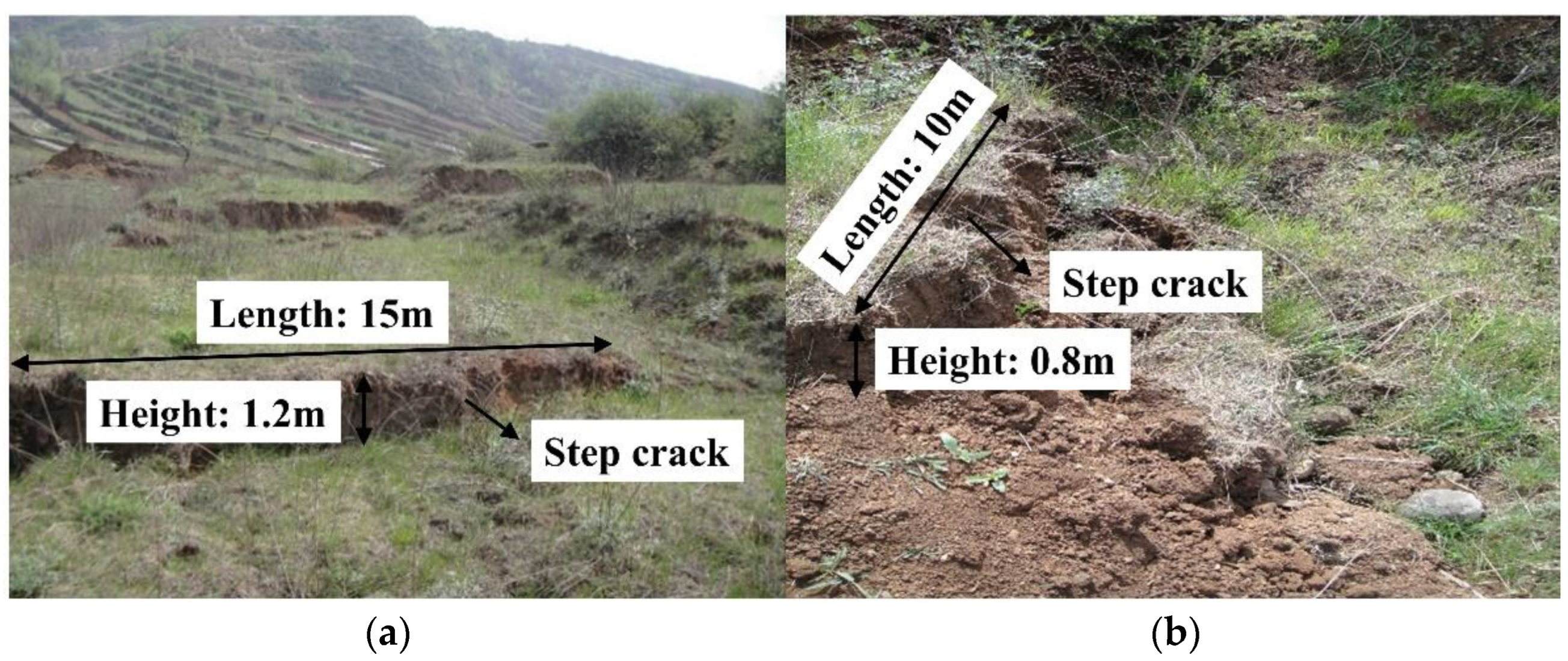

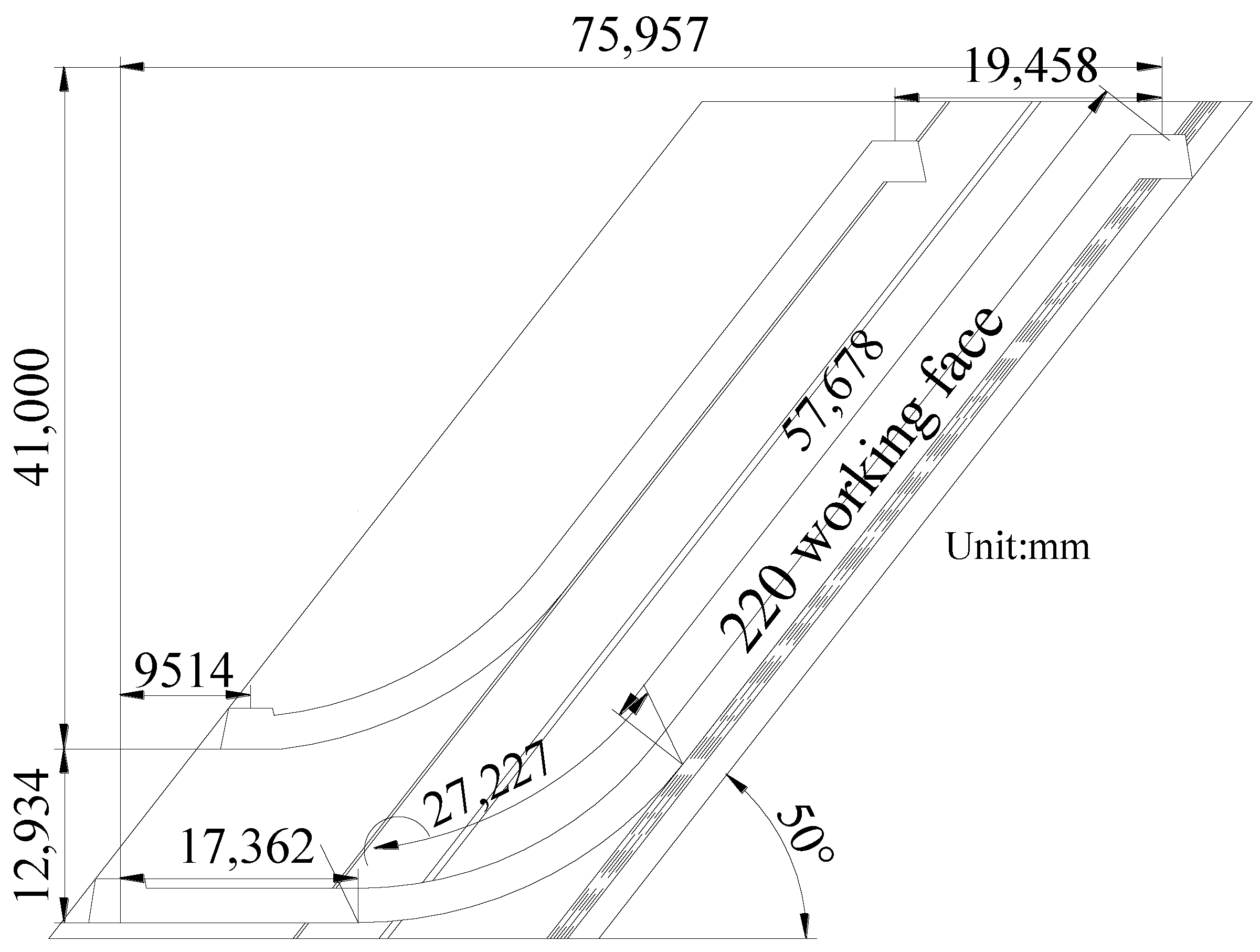








| Line Name | Time of Observation | Length (m) | Points Number | Observation Number | Remark |
|---|---|---|---|---|---|
| Q line | 2012.7~2014.5 | 820 | 28 | 7 | Vertical to the working face |
| Number | Coal Seam Thickness (m) | Dip Angle (°) | Stage Mining Depth (m) | Stage Number | Mining Depth (m) |
|---|---|---|---|---|---|
| 1 | 30 | 50 | 50 | 10 | 20~520 |
| 2 | 30 | 45 | 50 | 10 | 20~520 |
| 3 | 30 | 55 | 50 | 10 | 20~520 |
| 4 | 30 | 60 | 50 | 10 | 20~520 |
| 5 | 30 | 70 | 50 | 10 | 20~520 |
| 6 | 30 | 80 | 50 | 10 | 20~520 |
| 7 | 20 | 50 | 50 | 10 | 20~520 |
| 8 | 40 | 50 | 50 | 10 | 20~520 |
| 9 | 50 | 50 | 50 | 10 | 20~520 |
| Lithology | Thickness (m) | Volume Weight (kg/m3) | Bulk Modulus (GPa) | Shear Modulus (GPa) | Cohesion (MPa) | Friction Angle (°) | Tension (MPa) |
|---|---|---|---|---|---|---|---|
| Sandy shale | 100 | 2400 | 3.2 | 1.48 | 0.60 | 30 | 0.12 |
| Coal | 30 | 1350 | 1.62 | 0.62 | 0.28 | 28 | 0.10 |
| Sandy mudstone and sandstone | 30 | 2400 | 3.04 | 1.58 | 0.60 | 30 | 0.13 |
| Sandy mudstone | 50 | 2450 | 4.30 | 2.60 | 0.56 | 35 | 0.20 |
| Siltstone | 100 | 2300 | 2.80 | 1.28 | 0.48 | 34 | 0.24 |
| Fine sandstone | 120 | 2200 | 2.48 | 1.02 | 0.24 | 23 | 0.21 |
| Loess | 10 | 1600 | 0.16 | 0.06 | 0.20 | 17 | 0.10 |
| Lithology | Normal Stiffness (GPa) | Tangential Stiffness (GPa) | Friction Angle (°) | Cohesion (MPa) | Tension (MPa) |
|---|---|---|---|---|---|
| Sandy shale | 3.2 | 1.48 | 22 | 0.21 | 0.12 |
| Coal | 1.62 | 0.62 | 20 | 0.13 | 0.10 |
| Sandy mudstone and sandstone | 3.01 | 1.58 | 22 | 0.21 | 0.13 |
| Sandy mudstone | 4.40 | 2.60 | 27 | 0.56 | 0.20 |
| Siltstone | 3.20 | 1.28 | 22 | 0.25 | 0.24 |
| Fine sandstone | 2.40 | 1.02 | 21 | 0.20 | 0.21 |
| Loess | 0.16 | 0.06 | 12 | 0.10 | 0.10 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yan, Y.; Zhang, Y.; Zhu, Y.; Cai, J.; Wang, J. Quantitative Study on the Law of Surface Subsidence Zoning in Steeply Inclined Extra-Thick Coal Seam Mining. Sustainability 2022, 14, 6758. https://doi.org/10.3390/su14116758
Yan Y, Zhang Y, Zhu Y, Cai J, Wang J. Quantitative Study on the Law of Surface Subsidence Zoning in Steeply Inclined Extra-Thick Coal Seam Mining. Sustainability. 2022; 14(11):6758. https://doi.org/10.3390/su14116758
Chicago/Turabian StyleYan, Yueguan, Yanjun Zhang, Yuanhao Zhu, Jinchi Cai, and Junyao Wang. 2022. "Quantitative Study on the Law of Surface Subsidence Zoning in Steeply Inclined Extra-Thick Coal Seam Mining" Sustainability 14, no. 11: 6758. https://doi.org/10.3390/su14116758
APA StyleYan, Y., Zhang, Y., Zhu, Y., Cai, J., & Wang, J. (2022). Quantitative Study on the Law of Surface Subsidence Zoning in Steeply Inclined Extra-Thick Coal Seam Mining. Sustainability, 14(11), 6758. https://doi.org/10.3390/su14116758






