Joint Optimization of Routes and Container Fleets to Design Sustainable Intermodal Chains in Chile
Abstract
:1. Introduction
- Despite the numerous studies aiming to improve intermodal chains with a maritime leg, most of them have focused on the identification of the most suitable maritime routes to replace road transport with intermodal chains. These studies have considered two relevant initial assumptions: the technical and the operative features of the fleets are fixed [9]. Most of the vessels considered have been Ro-Ro vessels (Roll-on, Roll-off vessels; this refers to vessels with rolled cargo) with previous activity on other maritime routes. As a result, many studies have finally adapted the maritime routes to the current fleet instead adapting the fleets to the most interesting routes.
- Increasing discussion about the ‘green label’ of the Short Sea Shipping has also taken place in recent years [10,11,12]. A significant normative imbalance in terms of pollutant emissions damages the competitiveness of SSS in relation to road transport in the EU; the quick normative development in land transport demanding higher pollutant restrictions [13] along with the low impact of the economies of scale in SSS with small and quick vessels [10,14], have led to the intermodal chains not being as sustainable as was accepted several years ago [7].
2. Literature Review
3. Mathematical Model
- The kind of vessel for the fleet: According to the data published by the System of Public Companies of Chile (SEP) [34], in 2014, the containerization rate of general cargo (external and domestic traffic) in a large share of the Chilean ports reached and surpassed 70%. This, along with the good results obtained by a rising number of studies focused on the operation of feeder vessels under Short Sea Shipping conditions [6,7,35,36], led to the model presented in this paper optimizing uniquely container vessels for MoS. In this regard, the model published by Martínez-López et al. (2015) [19] has been simplified. The model has been tested for vessels from 85 to 200 m in length between perpendiculars; therefore, it is able to provide the technical and operative features of container vessels (Table 1 shows some of them) for its design in a conceptual step. Despite the numerous variables that are handled by the model (more than 150 without taking into account the inputs), only five of them are non-dependent (the first five variables of Table 1). This involves that the model collects many relationships among the search variables (linear and non-linear equations), but that the time invested in the resolution of the model (computation time) is low.
- The kind of truck for the unimodal option: Since the suitable cargo units for transport through container vessels are TEUs and FEUs, a unitary weight is assumed for each (Pp; ∀p ∈ PP): 12.5 and 20.5 t, respectively. Therefore, the trucks considered for this study must be able to transport containers with these net weights for the capillary hauls of the intermodal chain and for the unimodal option. The Economic Analysis of the Transport of National Cargo [37] published by the Undersecretary of Transport of the Government of Chile in 2009, indicated that a suitable truck is a vehicle with a maximum net weight of 25 tons (420 HP). This can be defined from the European regulation as a 5-axle ‘articulated vehicle’ (Directive 96/53/CE) and an N3 Heavy Duty Vehicle (Directive 2007/46/CE). On the other hand, taking into account the fact that, in 2013, 76% of Chilean trucks were less than 10 years old [38] the Euro-III technology (compulsory for trucks from 2000, Directive 98/69/EC) is assumed for the trucks (adopting a conservative attitude).
- Port operations: Containers will follow a direct route in the port [18]. This involves ideal conditions for the container movements in the port (minimum transit times for the containers in the port area).
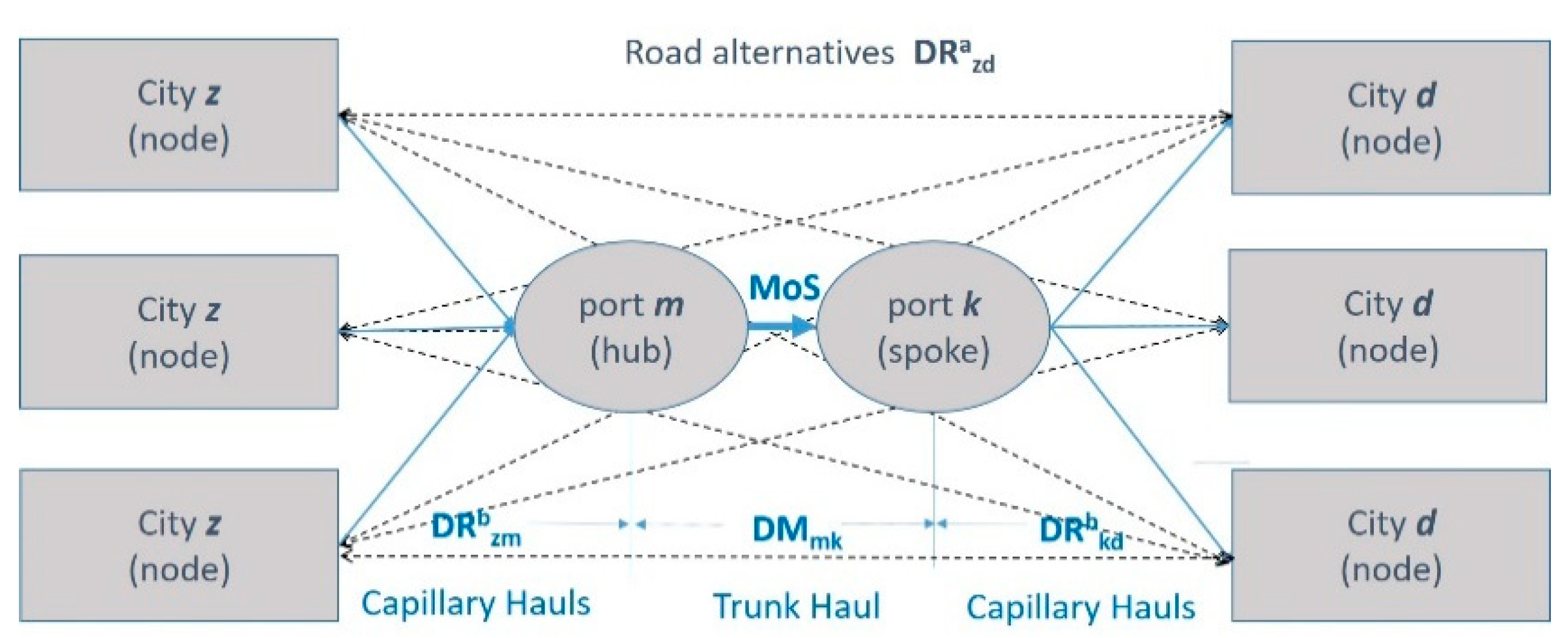
- Transport networks: A ‘many-to-many’ transport network [18] has been assumed as the transport model in this study (see Figure 1). This means that many possible origins and destinations (nodes) are considered as extreme points of the intermodal chains. This transport model is made up by one trunk haul to transport consolidated cargo (seaborne stretch) and capillary hauls (land hauls). Thus, the capillary hauls refer to land stretches from/to nodes and the ports (consolidation centres for the cargo). Whereas all nodes are extreme points of the chains, regardless of their localization and size, the port category is dependent of its geographical location and its capacity to receive deep sea vessels. Thereby, the ports that receive large vessels from deep sea activity (they are located in the central region of Chile in the application case) are hub ports. The other ports, peripheral ports, are known as spoke ports (see Figure 1).
3.1. Objective Functions
- Objective 1: The maximization of the relative competitiveness in terms of the overall transport costs (F1) of intermodality (CMU) in relation to unimodality (CU).
- Objective 2: The maximization of the relative competitiveness in terms of the transit time (F2) of intermodality (TVM) in relation to unimodality (TVU).
- Objective 3: The maximization of the relative competitiveness in terms of environmental costs (F3) of intermodality (MUE) in relation to unimodality (RE).
3.2. Constraints for the Mathematical Model
3.3. Resolution of the Model
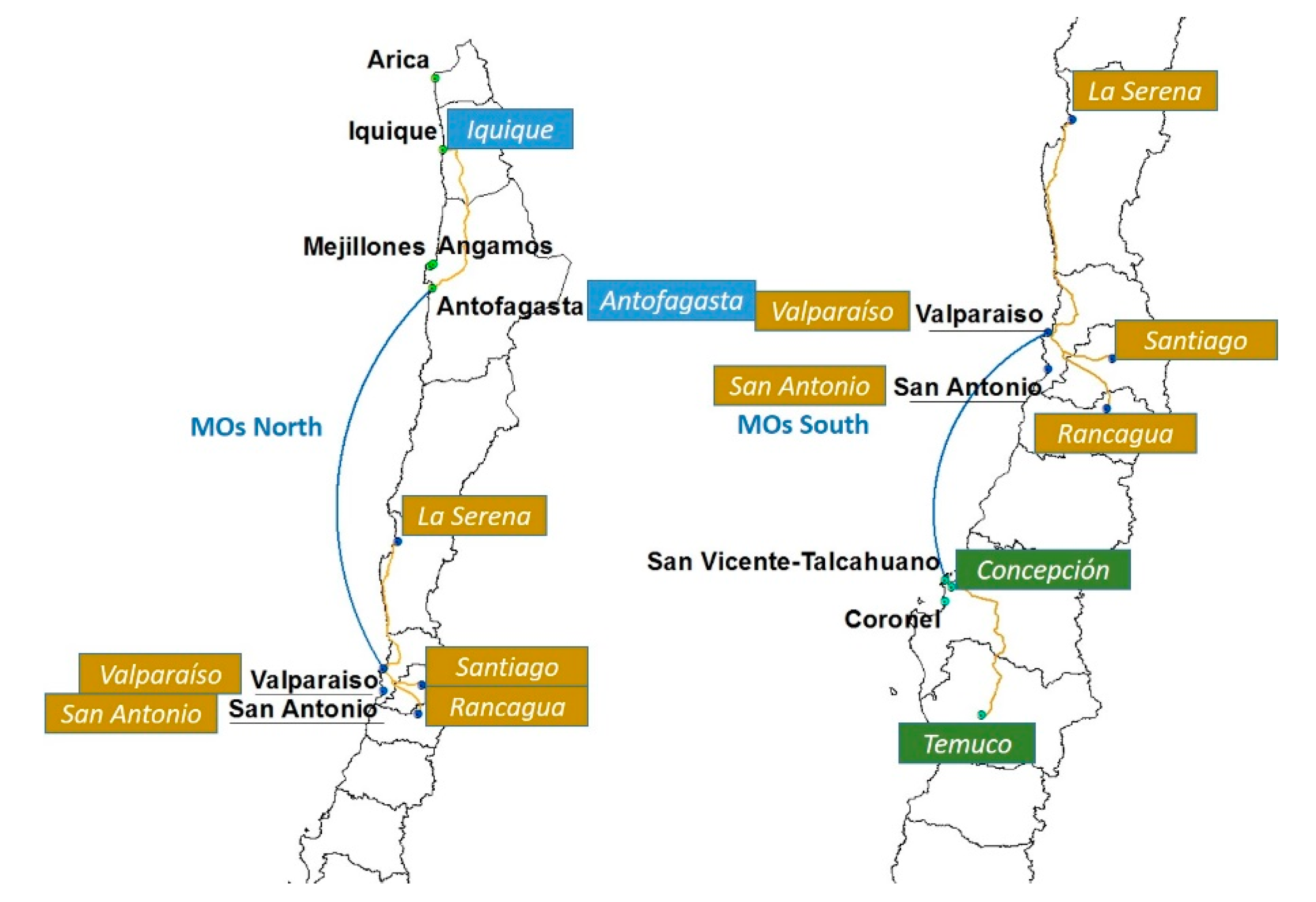
4. Application to Chile
- Preliminary scenarios: The competitiveness of the intermodal chains articulated through all possible MoS in the north and in the south will be evaluated using identical conditions (standard values for the port variables). In such a way, the spoke ports with the greatest opportunities for success are identified, by considering uniquely, their geographical localization.
- Current scenarios: From the previous selection of MoS, a new evaluation of the competitiveness of intermodal chains by operating with optimized fleets is carried out under the current port conditions (real port values). Consequently, the current port performances are included in this second assessment.
- Sensitivity analysis: The modification of the initial variables will lead to new scenarios. The new frameworks will permit the simulation of the performance and ruggedness of the intermodal chains when the initial scenario changes.
4.1. Data Description
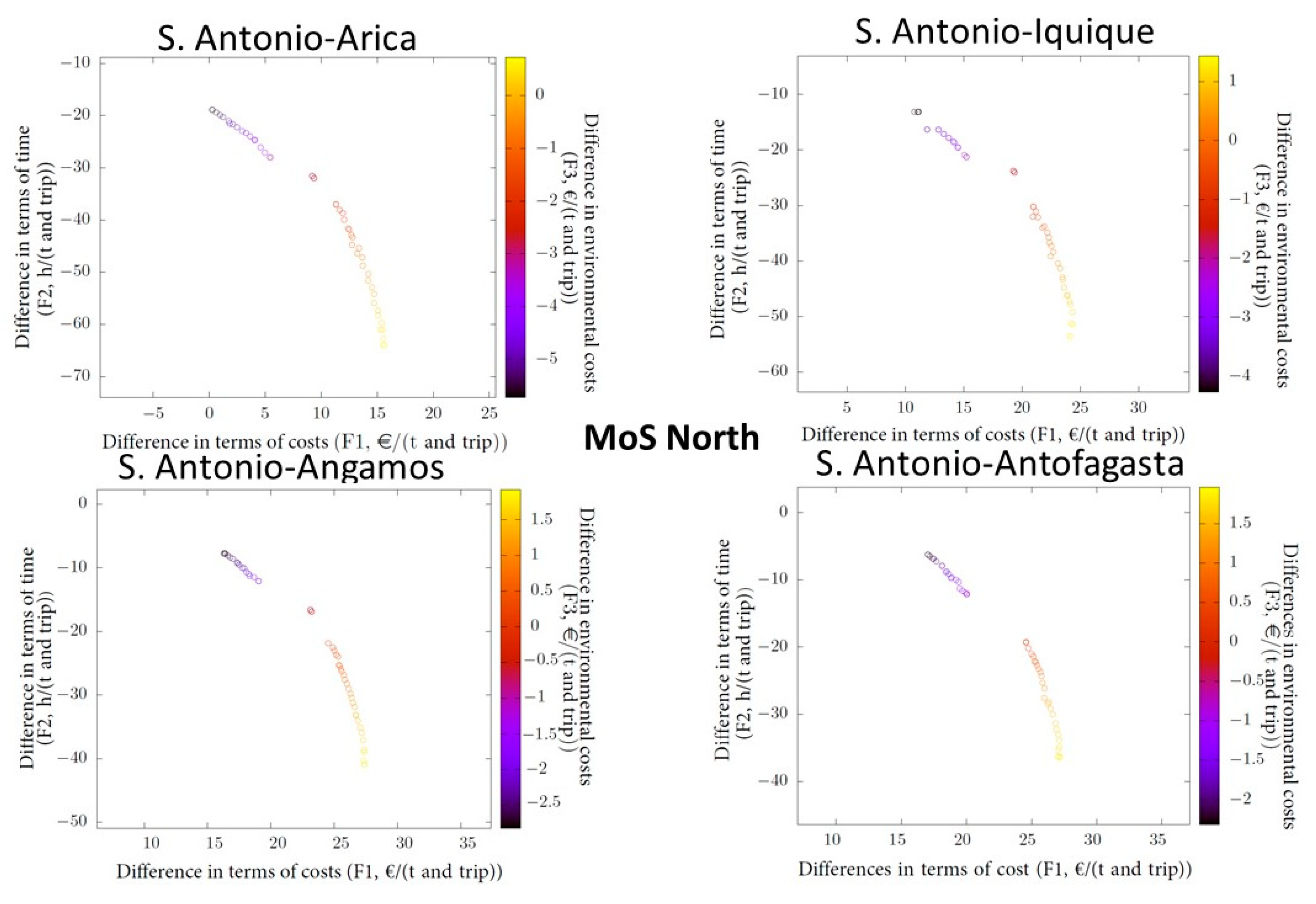
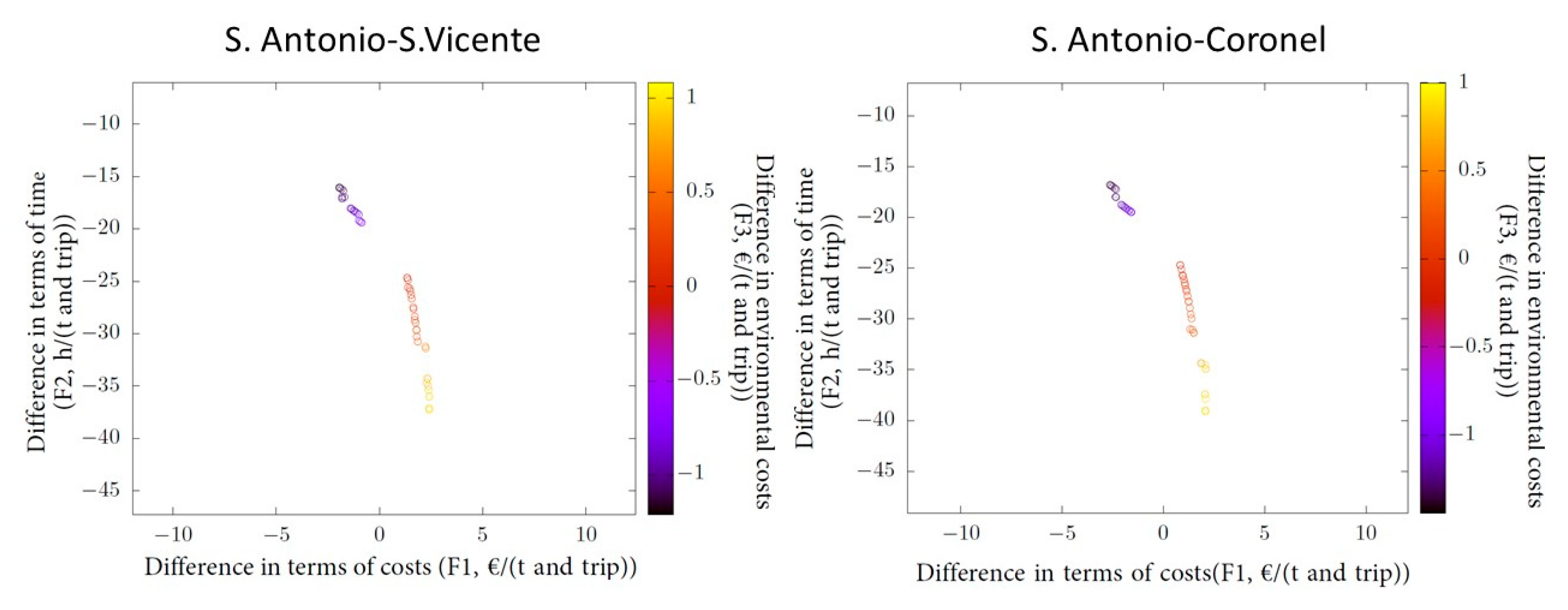
4.2. Preliminary Scenarios
4.3. Current Scenarios
4.4. Sensitivity Analysis
5. Discussion and Concluding Remarks
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Appendix A
| Subscripts: | |
| A = {1, …, a} | Different legs for the intermodal chains: capillary hauls (road haulage in both costs) and the trunk haul (maritime route). |
| BB = {1, …, b} | Installation of bow thruster: no or yes. |
| C = {1, …, c} | Cost inputs to reach the minimum required freight: amortization costs, financing costs, insurance costs, maintenance costs, crew costs, fuel costs, and port tariff costs (ship dues, cargo dues, pilot tariff, towing tariff, mooring dues, and loading/unloading costs). |
| DD = {1, …, d} | Final destinations on land (nodes). For the transport network of the application case, Iquique and Antofagasta are used in the northern region and Concepción and Temuco are used in the southern region. |
| EE = {1, …, e} | Kind of main engines: diesel engines and turbines. |
| GG = {1, …, g} | Cargo-handling systems: vessel cranes or port cranes. |
| H = {1, …, h} | Possible propellers: screws or waterjets. |
| I = {1, …, i} | Number of main engines. |
| J = {1, …, j} | Direction for the transport (north–south and south–north). |
| K = {1, …, k} | Possible spoke ports. For the application case, they are Arica, Iquique, Mejillones, and Antofagasta in the northern region and San Vicente and Coronel in the southern region. |
| M = {1, …, m} | Possible hub ports. For the application case, they are Valparaíso and San Antonio in the V region. |
| N = {1, …, n} | Number of shaft lines in the machine room. |
| PP = {1, …, p} | Types of cargo units for container vessels: TEUs and FEUs. |
| SS = {1, …, s} | Stages during maritime transport: free sailing, maneuvering (pilotage time, towing time, and mooring time), and berthing (loading and unloading operations). |
| U = {1, …, u} | Group of evaluated pollutants: SO2, NOx, PM2.5, and CO2. |
| V = {1, …, v} | Classification of the zones according to the harmful impact of the emissions: metropolitan zone and urban zone. |
| WW = {1, …, w} | Port services for the maneuvering stage: pilotage, towing, and mooring services. |
| Z = {1, …, z} | The origins on land (nodes). For the transport network of the application case, in the central region, are Santiago, Valparaíso hub port (Valparaíso or San Antonio), La Serena, and Rancagua are used. |
| Superscripts: | |
| ST = {c} | The MoS analyzed: MoS North and MoS South. |
| DIS = {d} | Compulsory driving with two drivers (no and yes). |
| Variables: | |
| B | Breadth of the vessels (m). |
| CTc | Cost of the items for the maritime required freight of the trunk haul (€); ∀c ∈ C |
| CMU | Overall transport costs for the intermodal chain (€/(t×trip)). |
| CU | Overall transport costs for the unimodal alternative (€/(t×trip)). |
| D | Depth to the upper deck (m). |
| EGsu | Emission coefficients for container vessels during the different maritime stages (kg/nm and in kg/h); ∀s ∈ SS ∧ ∀u ∈ U. |
| EGUu | Emission coefficients for trucking (gr of pollutant/kg of fuel consumed); ∀u ∈ U. |
| FB | Freeboard (m). |
| FCp | Fuel consumption for trucks by considering the cargo unit transported (gr fuel/km); ∀p ∈ PP. |
| Gp | Cargo units related to the cargo capacity of the vessel; ∀p ∈ PP. |
| GT | Gross Tonnage. |
| L | length of the vessels (m). |
| MGg | Cargo handling systems ∀g ∈ GG. |
| MMb | Bow thruster installation ∀b ∈ BB. |
| MUE | Environmental costs for the intermodal chains (€/(t×trip)). |
| NB | Number of vessels. |
| NCk | Number of cranes per vessel ∀k ∈ K. |
| NCm | Number of cranes per vessel ∀m ∈ M. |
| Ntrips | Annual trips for the fleet. |
| NMEi | Number of main engines for the vessels ∀i ∈ I. |
| NSLn | Number of shaft lines in the vessels; ∀n ∈ N. |
| RE | Environmental costs (€/(t×trip)) for the road transport. |
| REa | Environmental costs (€/(t×trip)) for the stretches of the intermodal chain ∀a ∈ A. |
| T | Summer draught (m). |
| PB | Propulsion Power for the vessels (HP). |
| TMEe | Type of Main Engines; ∀e ∈ EE. |
| TPh | Type of propeller; ∀h ∈ H. |
| TPM | Deadweight (t). |
| TSw | Time for every port operation during the maneuvering stage (h); ∀w ∈ WW. |
| TVM | Time invested in the intermodal transport (h). |
| TVU | Time invested in the unimodal alternative (road haulage) (h). |
| VB | Speed of the vessel (knots). |
| VT | Speed of the truck (km/h). |
| ∇ | Buoyancy volume (m3). |
| Inputs: | |
| CF1u | Unitary costs for the pollutants during free sailing (€/kg); ∀u ∈ U. |
| CFsuv | Unitary costs for the pollutants considering the maritime stages and the affected zones (€/kg); ∀s ∈ SS ∧ ∀u ∈ U ∧ ∀v ∈ V. |
| CKdp | Unitary cost per kilometer by road (unimodal; the value is dependent on the required number of drivers and the cargo unit transported (€/km)); ∀p ∈ PP ∧ ∀d ∈ DIS. |
| DMmk | Maritime distance for the trunk haul (km); ∀m ∈ M ∧ ∀k ∈ K. |
| DRazd | Road distance for the unimodal alternative (km); ∀z ∈ Z ∧ ∀d ∈ DD. |
| DRbzm | Road distance for the capillary hauls in the intermodal chains from/to hub ports (km); ∀z ∈ Z ∧ ∀m ∈ M. |
| DRbkd | Road distance for the capillary hauls in the intermodal chains from/to spoke ports (km); ∀k ∈ K ∧ ∀d ∈ DD. |
| Xd | Relative probability of delivering/receiving a load for each node of the northern and southern regions (%) regarding other alternative nodes ∀d ∈ DD. |
| Xcjz | Relative probability of delivering/receiving a load for each node of the central region (%) regarding other alternative nodes for each MoS (MoS North and MoS South) and direction (north–south and south–north) ∀z ∈ Z ∧ ∀c ∈ ST ∧ ∀j ∈ J. |
| Pp | weights for the cargo units (tons); (∀p ∈ PP) |
| Vk | Average speed of the cranes for the spoke ports ∀k ∈ K (cycles/h). |
| Vm | Average speed of the cranes for the hub ports ∀m ∈ M (cycles/h). |
Appendix B
| Preliminary Scenarios | Current Scenarios | |||||||
|---|---|---|---|---|---|---|---|---|
| For Every Port | Valparaíso | S. Antonio | Antofagasta | S. Vicente | Coronel | |||
| Maximum Number of cranes/vessels (NCk and NCm) | units | no limit | 6 | 6 | 3 | 7 | 4 | |
| Average speed/crane (Vk and Vm) | (cycle/h) | 27 | 27 | 27 | 18 | 18 | 22.5 | |
| Ship dues (CT7) | (€/GT h) | 0.0167 | 1.536 | 1.574 | 1.954 | 2.618 | 2.772 | |
| Cargo dues (CT8) | (€/TEU) | 32.73 | 7.95 | 8.3 | 63.86 | 33.86 | 34.08 | |
| (€/FEU) | 49.104 | 13.04 | 13.61 | 104.72 | 55.53 | 55.9 | ||
| Pilot dues (CT9) | (GT < 10,000) | (€) | 231.28 | 112.68 | 112.68 | 112.68 | 112.68 | 112.68 |
| (GT ≥ 10,000) | (€) | 460.6 | 337.71 | 337.71 | 0 | 0 | 0 | |
| Towing dues (CT10) | (GT < 13,000) | (€/tug) | 420.12 | 995.35 | 995.35 | 995.35 | 995.35 | 995.35 |
| (GT ≥ 13,000) | (€/tug) | 1093.42 | 2190.69 | 2190.69 | 2190.69 | 2190.69 | 2190.69 | |
| Mooring dues (CT11) | (GT < 3000) | (€/mooring) | 96.35 | 0 | 0 | 0 | 0 | 390.87 |
| (GT ≥ 3000) | (€/mooring) | 316.15 | 0 | 0 | 0 | 0 | 390.87 | |
| Loading/unloading dues (CT12) | (€/TEU) | 24.81 | 83.35 | 73.05 | 82.54 | 63.63 | 72.72 | |
| (€/FEU) | 49.61 | 124.98 | 109.56 | 128.9 | 98.16 | 90.90 | ||
References
- Brooks, M.R.; Frost, J.D. Short Sea Shipping: A Canadian Perspective. Marit. Policy Manag. 2004, 31, 393–407. [Google Scholar] [CrossRef]
- Brooks, M.R.; Sanchez, R.J.; Wilmsmeier, G. Developing Short Sea Shipping in South America—Looking Beyond Traditional Perspectives. Ocean Yearb. Online 2014, 28, 495–526. [Google Scholar] [CrossRef] [Green Version]
- Brooks, M.; Wilmsmeier, G. A Chilean Maritime Highway: Is It a Possible Domestic Transport Option? Transp. Res. Rec. 2017, 2611, 32–40. [Google Scholar] [CrossRef]
- Puckett, S.; Hensher, D.; Brooks, M.; Trifts, V. Preferences for alternative short sea shipping opportunities. Transp. Res. Part E Log. Transp. Rev. 2011, 47, 182–189. [Google Scholar] [CrossRef]
- Bendall, H.; Brooks, M. Short sea shipping: Lessons for or from Australia. Int. J. Shipp. Transp. Logist. 2011, 3, 2011. [Google Scholar] [CrossRef]
- Chang, Y.; Lee, P.; Kim, H. Optimization Model for Transportation of Container Cargoes considering Short Sea Shipping and External Cost. South Korean Case. Transp. Res. Rec. 2010, 2166, 99–108. [Google Scholar] [CrossRef]
- Lee, P.; Hu, K.C.H.; Chen, T. External Costs of Domestic Container Transportation: Short-Sea Shipping versus Trucking in Taiwan. Transp. Rev. 2010, 30, 315–335. [Google Scholar] [CrossRef]
- Suárez-Alemán, A.; Trujillo, L.; Medda, F. Short sea shipping as intermodal competitor: A theoretical analysis of European transport policies. Marit. Policy Manag. 2015, 42, 317–334. [Google Scholar] [CrossRef]
- Takano, K.; Arai, M. A genetic algorithm for the hub-and-spoke problem applied to containerized cargo transport. J. Mar. Sci. Technol. 2009, 14, 256–274. [Google Scholar] [CrossRef]
- Hjelle, M.H. Short sea shipping’s green label at risk. Transp. Rev. 2010, 30, 617–640. [Google Scholar] [CrossRef]
- Hjelle, M.H. Atmospheric emissions of short sea shipping compared to road transport though the peaks and troughs of short-term market cycles. Transp. Rev. 2014, 34, 379–395. [Google Scholar] [CrossRef]
- Hjelle, M.H.; Fridell, E. When is short sea shipping environmentally competitive? In Environmental Health—Emerging Issues and Practice; Oosthuizen, J., Ed.; InTech: Rijeka, Croatia, 2012. [Google Scholar]
- Culliane, K.; Culliane, S. Atmospheric emissions from shipping: The need for regulation and approaches to compliance. Transp. Rev. 2013, 33, 377–401. [Google Scholar] [CrossRef]
- Bengtsson, S.; Fridell, E.; Andersson, K. Fuels for short sea shipping: Comparative assessment with focus on environment impact. Proc. Inst. Mech. Eng. M 2014, 228, 44–54. [Google Scholar] [CrossRef]
- Xie, X.; Xu, D.L.; Yang, J.B.; Wang, J.; Ren, J.; Yu, S. Ship selection using a multiple-criteria synthesis approach. J. Mar. Sci. Technol. 2008, 13, 50–62. [Google Scholar] [CrossRef]
- Giusti, R.; Iorfida Ch Li, Y.; Manerba, D.; Musso, S.; Perboli, G.; Tadei, R.; Yuan, S. Sustainable and De-Stressed International Supply-Chains Through the SYNCHRO-NET Approach. Sustainability 2019, 11, 1083. [Google Scholar] [CrossRef] [Green Version]
- Žgaljić, D.; Tijan, E.; Jugović, A.; Jugović, T.P. Implementation of Sustainable Motorways of the Sea Services Multi-Criteria Analysis of a Croatian Port System. Sustainability 2019, 11, 6827. [Google Scholar] [CrossRef] [Green Version]
- Daganzo, C. Many to many distribution. In Logistic Systems Analysis; Daganzo, C., Ed.; Springer: Berlin, Germany, 2005. [Google Scholar]
- Martínez-López, A.; Caamaño, P.; Castro, L. Definition of optimal fleets for Sea Motorways: The case of France and Spain on the Atlantic coast. Int. J. Shipp. Transp. Logist. 2015, 7, 89–113. [Google Scholar] [CrossRef]
- Martínez-López, A.; Caamaño, P.; Míguez, M. Influence of external costs on the optimisation of container fleets by operating under motorways of the sea conditions. Int. J. Shipp. Transp. Logist. 2016, 8, 653–686. [Google Scholar] [CrossRef]
- Yan, S.; Chen, C.Y.; Lin, S.C. Ship scheduling and container shipment planning for liners in short-term operations. J. Mar. Sci. Technol. 2009, 14, 417–435. [Google Scholar] [CrossRef]
- Fan, H.; Yu, J.; Liu, X. Tramp Ship Routing and Scheduling with Speed Optimization Considering Carbon Emissions. Sustainability 2019, 11, 6367. [Google Scholar] [CrossRef] [Green Version]
- Wang, S.; Meng, Q. Sailing speed optimization for container ships in a liner shipping network. Transp. Res. E Log. Transp. Rev. 2012, 48, 701–714. [Google Scholar] [CrossRef]
- Kim, J.-G.; Kim, H.-J.; Lee, P.T.-W. Optimising containership speed and fleet size under a carbon tax and an emission trading scheme. Int. J. Shipp. Transp. Logist. 2013, 5, 571–590. [Google Scholar] [CrossRef]
- Chandra, S.; Christiansen, M.; Fagerholt, K. Combined fleet deployment and inventory management in roll-on/roll-off shipping. Transp. Res. E Log. Transp. Rev. 2016, 92, 43–55. [Google Scholar] [CrossRef]
- Karsten, C.V.; Ropke, S.; Pisinger, D. Simultaneous Optimization of Container Ship Sailing Speed and Container Routing with Transit Time Restrictions. Transp. Sci. 2018, 52, 769–787. [Google Scholar] [CrossRef] [Green Version]
- Mansouri, S.A.; Lee, H.; Aluko, O. Multi-objective decision supports to enhance environmental sustainability in maritime shipping: A review and future directions. Transp. Res. Part E Log. Transp. Rev. 2015, 78, 3–18. [Google Scholar] [CrossRef]
- Wong, E.Y.; Yeung, H.S.; Lau, H.Y. Immunity-based hybrid evolutionary algorithm for multi-objective optimization in global container repositioning. Eng. Appl. Artif. Intel. 2009, 22, 842–854. [Google Scholar] [CrossRef]
- Wong, E.Y.C.; Lau, H.Y.; Mak, K.L. Immunity-based evolutionary algorithm for optimal global container repositioning in liner shipping. Or Spectrum 2010, 32, 739–763. [Google Scholar] [CrossRef] [Green Version]
- Chen, G.; Govindan, K.; Golias, M.M. Reducing truck emissions at container terminals in a low carbon economy: Proposal of a queueing-based Biobjective model for optimizing truck arrival pattern. Transp. Res. Part E Log. Transp. Rev. 2013, 55, 3–22. [Google Scholar] [CrossRef]
- Hu, Q.; Hu, Z.; Du, Y. Berth and quay-crane allocation problem considering fuel consumption and emissions from vessels. Comput. Ind. Eng. 2014, 70, 1–10. [Google Scholar] [CrossRef]
- Baykasoglu, A.; Subulan, K. A multi-objective sustainable load planning model for intermodal transportation networks with a real-life application. Transp. Res. Part E Log. Transp. Rev. 2016, 95, 207–247. [Google Scholar] [CrossRef]
- Martínez-López, A.; Caamaño, P.; Chica, M.; Trujillo, L. Optimization of a container vessel fleet and its propulsion plant to articulate sustainable intermodal chains versus road transport. Transp. Res. Part D Transp. Environ. 2018, 59, 134–147. [Google Scholar] [CrossRef]
- Ministry of Transportation of Chile. 2014. Available online: http://www.sepchile.cl/documentacion/estadisticas-portuarias/?no_cache=1 (accessed on 12 February 2020).
- Usabiaga, J.; Castells, M.; Martínez, X.; Olcer, A. A simulation model for road and maritime environmental performance assessment. J. Environ. Prot. Ecol. 2013, 4, 683–693. [Google Scholar] [CrossRef] [Green Version]
- Lupi, M.; Farina, A.; Orsi, D.; Pratelli, A. The capability of Motorways of the Sea of being competitive against road transport. The case of the Italian mainland and Sicily. J. Transp. Geogr. 2017, 58, 9–21. [Google Scholar] [CrossRef] [Green Version]
- Under Secretary of Transport, Ministry of Transport and Telecommunications of the Government of Chile. Analysis of the Transport. of National Cargo. 2009. Available online: http://www.subtrans.cl/subtrans/doc/Informefinalcorregido.pdf (accessed on 12 February 2020).
- National Institute of Statistics of Chile. Yearbook about the Road Transport. 2013. Available online: http://www.ine.cl/canales/chile_estadistico/estadisticas_economicas/transporte_y_comunicaciones/encuesta-estructural-transporte-carretera/2013/infografia_transporte_por_carretera_2015.pdf (accessed on 12 February 2020).
- Maibach, M.; Schreyer, C.; Sutter, D.; Essen, H.P.V.; Boon, B.H.; Smokers, R.; Schrote, A.; Doll, C.; Pawlowska, B.; Bak, M. Handbook on Estimation of External Costs in the Transport Sector; CE Delft: Delft, The Netherlands, 2008. [Google Scholar]
- Jiang, L.; Kronbak, J. The Model of Maritime External Costs; Project No. 2010-56, Emissionsbeslutingsstottesystem, Word Package 1, Report No. 06; University of Southern Denmark: Odense, Denmark, 2012. [Google Scholar]
- Jiang, L.; Kronbak, J.; Christensen, L. The cost and benefits of sulphur reduction measures:Sulphur scrubbers versus marine gas oil. Transp. Res. Part D Transp. Environ. 2014, 28, 19–27. [Google Scholar] [CrossRef]
- Ntziabchristos and Samaras. Passenger cars, light duty trucks, heavy duty vehicles including buses and motorbikes. In EMEP/EEA Air Pollutant Emission Inventory Guidebook 2009 (updated May, 2012); Technical Report No. 9/2009; The European Environment Agency: Copenhagen, Denmark, 2012. [Google Scholar]
- Kristensen, H. Energy Demand and Exhaust Gas Emissions of Ships; Work Package 2 of Project Emissionsbeslutningsstottesystem; Technical University of Denmark: Copenhagen, Denmark, 2012. [Google Scholar]
- Lützen, M.; Kristensen, H. A model for prediction of propulsion power and emissions tankers and bulk carriers. In Proceedings of the World Maritime Technology Conference, Saint Petersburg, Russia, 29 May–1 June 2012. [Google Scholar]
- Possel, B.; Wismans, L.; Van Berkum, E.; Bliemer, M. The multi-objective network design problem using minimizing externalities as objectives: Comparison of a genetic algorithm and simulated annealing framework. Transportation 2018, 45, 545–572. [Google Scholar] [CrossRef]
- Caamano, P.; Tedin, R.; Paz-Lopez, A.; Becerra, J.A. JEAF: A Java evolutionary algorithm framework. In Proceedings of the 2010 IEEE Congress on Evolutionary Computation (CEC), Barcelona, Spain, 18–23 July 2010; pp. 1–8. [Google Scholar]
- Directemar. Ministry of Transport and Telecommunications of Chile and Ports. 2012. Available online: http://web.directemar.cl/estadisticas/puertos/default.htm (accessed on 12 February 2020).
- Under Secretary of Transport; Ministry of Transport and Telecommunications of the Government of Chile. Analysis of the Costs and Competitiveness of the Modes of Interurban Land Transport. 2011. Available online: http://www.subtrans.cl/subtrans/doc/Informefinalcorregido.pdf (accessed on 12 February 2020).
- Reis, V. Analysis of mode choice variables in short-distance intermodal freight transport using an agent-based model. Transp. Res. Part A Policy Pract. 2014, 61, 100–120. [Google Scholar] [CrossRef]
- Asgari, N.; Zanjirani Farahani, R.; Goh, M. Network design approach for hub ports-shipping companies competition and cooperation. Transp. Res. Part A Policy Pract. 2013, 48, 1–18. [Google Scholar] [CrossRef]
| VB | Speed of the vessel (kn) |
| Gp: ∀p ∈ PP | Cargo Capacity for the vessels (units) |
| MGg; ∀g ∈ GG | Cargo handling systems |
| MMb; ∀b ∈ BB | Bow thruster installation |
| Ntrips | Annual trips for the fleet |
| NB | Number of vessels |
| L | Length between perpendiculars (m) |
| B | Breadth (m) |
| D | Depth to the upper deck (m) |
| T | Summer draught (m) |
| FB | Freeboard (m) |
| GT | Gross tonnage (t) |
| Buoyancy volume (m3) | |
| TPM | Deadweight (t) |
| PB | Propulsion Power (HP) |
| TMEe; ∀e ∈ EE | Type of Main Engines |
| TPh; ∀h ∈ H | Type of propeller |
| NSLn; ∀n ∈ N | Number of shaft lines |
| NMEi; ∀i ∈ I | Number of main engines |
| RR1 | |||
| RR2 | |||
| RR3 | |||
| RR4 | B ≥ 13.56 | ||
| RR5 | D ≥ | 7.15 if PB ≤ 33,794 | |
| if 33,794 < PB ≤ 53,600 | |||
| RR6 | |||
| RR7 | |||
| RR8 | |||
| RR9 | |||
| RR10 | |||
| RR11 | |||
| RR12 | Gp × Ntrips ≥ | MoS (North) | 370,566; if Gp = G1 |
| 225,955; if Gp = G2 | |||
| MoS (South) | 791,408; if Gp = G1 | ||
| 482,566; if Gp = G2 | |||
| RR13 | (Gp/2) × Ntrips ≤ | MoS (North) | 276,973; if Gp = G1 |
| 168,886; if Gp = G2 | |||
| MoS (South) | 709,542; if Gp = G1 | ||
| 432,648; if Gp = G2 | |||
| RR14 | |||
| Operator | Parameters | Values |
|---|---|---|
| Tournament Selection | Pool Size | 2 |
| SBX-Crossover | Probability | 5% |
| Polynomial Mutation | Probability | 60% |
| N | 1 |
| Ports of North | North–South & Centre | South & Centre–North |
| Region | (Exports and Cabotage) (t) | (Imports and Cabotage) (t) |
| Arica | 124,835 | 147,394 |
| Iquique | 412,948 | 75,311 |
| Mejillones | 122,186 | 121,964 |
| Angamos | 1,576,985 | 570,598 |
| Antofagasta | 1,225,215 | 254,636 |
| North Total | 3,462,168 | 1,169,903 |
| Ports of South | North & Centre–South | South–North & Centre |
| Region | (Imports and Cabotage) (t) | (Exports and Cabotage) (t) |
| Lirquen | 62,576 | 3,144,777 |
| San Vicente | 657,911 | 3,612,814 |
| Coronel | 302,832 | 2,111,696 |
| South Total | 1,023,319 | 8,869,287 |
| Fleets | Angamos | Antofagasta | S. Vicente | Coronel |
|---|---|---|---|---|
| Cargo units | FEUs | FEUs | FEUs | FEUs |
| Cargo capacity (Gp) | 761 TEUs | 608 TEUs | 1334 TEUs | 1330 TEUs |
| Speed of the vessels (kn) | 17.26 | 16.90 | 16.11 | 16.58 |
| Bow thruster | Yes (MM2) | Yes (MM2) | Yes (MM2) | Yes (MM2) |
| Cargo handling systems | Port cranes (MG2) | Port cranes (MG2) | Port cranes (MG2) | Port cranes (MG2) |
| Number of vessels (NB) | 5 | 4 | 3 | 3 |
| Yearly trips (N) | 668 | 670 | 672 | 672 |
| L (m) | 127.56 | 118.49 | 158.2 | 157.8 |
| B (m) | 21.45 | 20.15 | 27.41 | 27.34 |
| D (m) | 10.55 | 9.85 | 13.32 | 13.27 |
| GT (Ton) | 8.231 | 6.798 | 16.612 | 16.268 |
| Propeller | Conventional Screw (TP1) | Conventional Screw (TP1) | Conventional Screw (TP1) | Conventional Screw (TP1) |
| Number of shaft lines | 1 (NSL1) | 1 (NSL1) | 1 (NSL1) | 1 (NSL1) |
| Main engine | Diesel (TME1) | Diesel (TME1) | Diesel (TME1) | Diesel (TME1) |
| Number of main engines | 1 (NME1) | 1 (NME1) | 1 (NME1) | 1 (NME1) |
| RESULTS | ||||
| F1(€/(t×trip)) | 25.80 | 25.41 | 1.85 | 1.50 |
| F2(h/(t×trip)) | −27.58 | −22.73 | −30.75 | −31.36 |
| F3(€/(t×trip) | 1.02 | 1.04 | 0.46 | 0.37 |
| Spoke Ports | Objective Functions | Preliminary Scenarios | Current Scenarios | |
|---|---|---|---|---|
| S. Antonio | S. Antonio | Valparaíso | ||
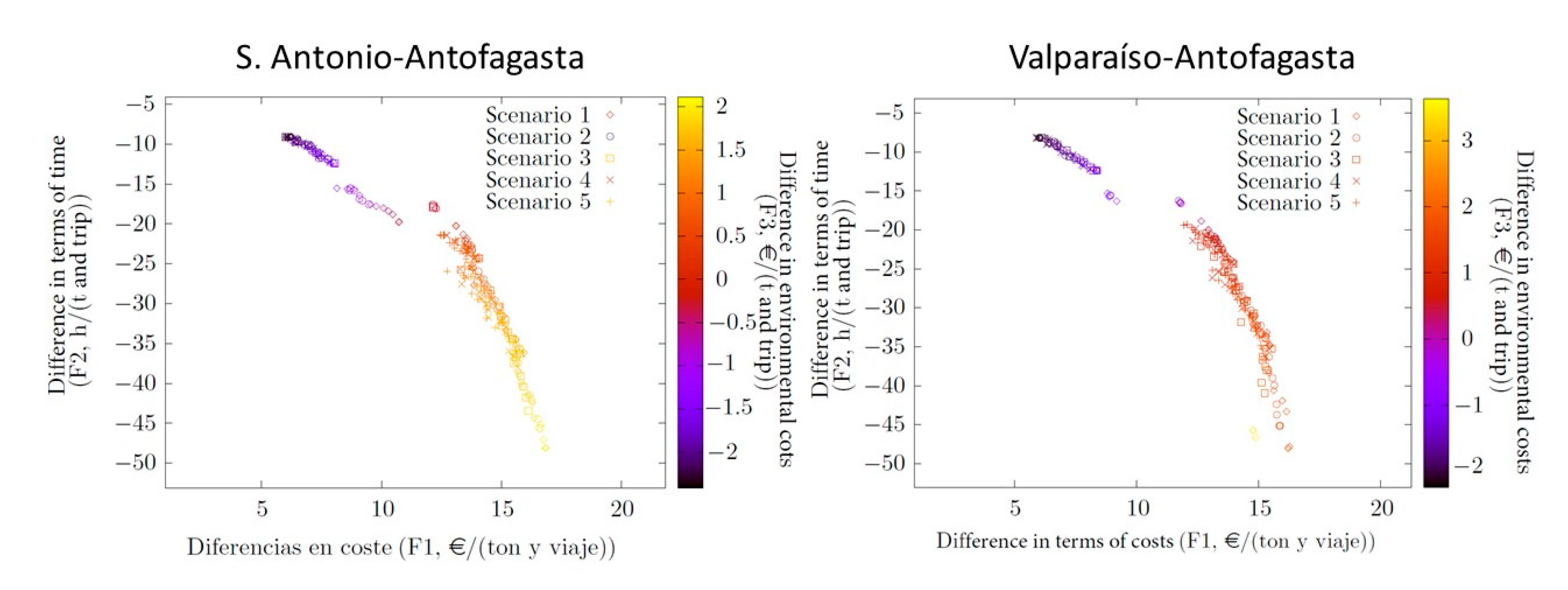
| Antofagasta | F1 (€/t and trip) | [17; 27] | [6.14.; 16.1] | [5.9.; 15.5] |
| F2 (h/t and trip) | [−36; −6] | [−44; −9.1] | [−38; −8] | |
| F3 (€/t and trip) | [−2; 2] | [−2; 2] | [−2; 2] | |
| S. Vicente | F1 (€/t and trip) | [−1.9; 2.4] | [−7.24; −3.1] | [−6.6; −2.0] |
| F2 (h/t and trip) | [−36; −16] | [−37.2; −16.0] | [−37.8; −16] | |
| F3 (€/t and trip) | [−1.1; 0.9] | [−1.1; 1.1] | [−1.2; 1.1] | |
| Coronel | F1 (€/t and trip) | [−2.6; 2.1] | [−8; −3.7] | [−7.5; −2,6] |
| F2 (h/t and trip) | [−34.9; −16.8] | [−41.7; −18.2] | [−42.6; −18.1] | |
| F3 (€/t and trip) | [−1.3; 0.8] | [−1.3; 0.9] | [−1.5; 1,0] | |
| Scenario 1 | Scenario 2 | Scenario 3 = Current Scenario | Scenario 4 | Scenario 5 |
|---|---|---|---|---|
| 120% | 110% | 100% | 90% | 80% |
| Base Value | Base Value | Base Value | Base Value | Base Value |
| Demand | ||||
|---|---|---|---|---|
| Antofagasta | F1(€/t Trip) | F2 (h/t Trip) | F3 (€/t Trip) | |
| Valparaíso | Scenario 3 (100%) = Base Value | 12.92 | −21.00 | 0.87 |
| Scenario 4 (90%) | 12.80 | −20.69 | 0.85 | |
| Scenario 5 (80%) | 12.56 | −20.55 | 0.85 | |
| S. Antonio | Scenario 3 (100%) = Base Value | 13.26 | −22.17 | 0.71 |
| Scenario 4 (90%) | 13.05 | −22.14 | 0.72 | |
| Scenario 5 (80%) | 13.10 | −22.01 | 0.71 | |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Martínez-López, A.; Chica, M. Joint Optimization of Routes and Container Fleets to Design Sustainable Intermodal Chains in Chile. Sustainability 2020, 12, 2221. https://doi.org/10.3390/su12062221
Martínez-López A, Chica M. Joint Optimization of Routes and Container Fleets to Design Sustainable Intermodal Chains in Chile. Sustainability. 2020; 12(6):2221. https://doi.org/10.3390/su12062221
Chicago/Turabian StyleMartínez-López, Alba, and Manuel Chica. 2020. "Joint Optimization of Routes and Container Fleets to Design Sustainable Intermodal Chains in Chile" Sustainability 12, no. 6: 2221. https://doi.org/10.3390/su12062221
APA StyleMartínez-López, A., & Chica, M. (2020). Joint Optimization of Routes and Container Fleets to Design Sustainable Intermodal Chains in Chile. Sustainability, 12(6), 2221. https://doi.org/10.3390/su12062221





