Experimental Validation and Parameter Study of a 2D Geometry-Based, Flexible Designed Thermal Motor Model for Different Cooled Traction Motor Drives
Abstract
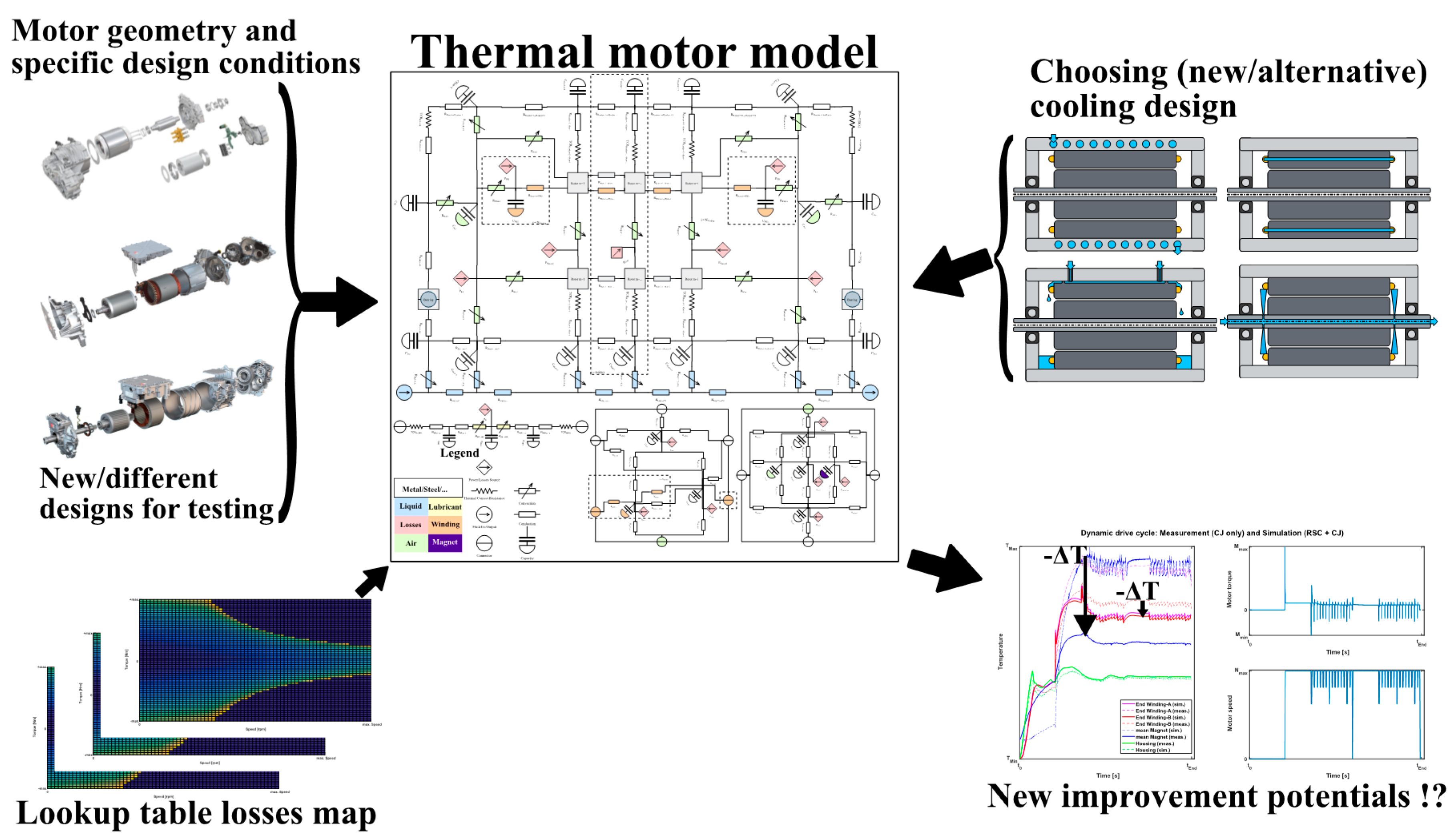
1. Introduction
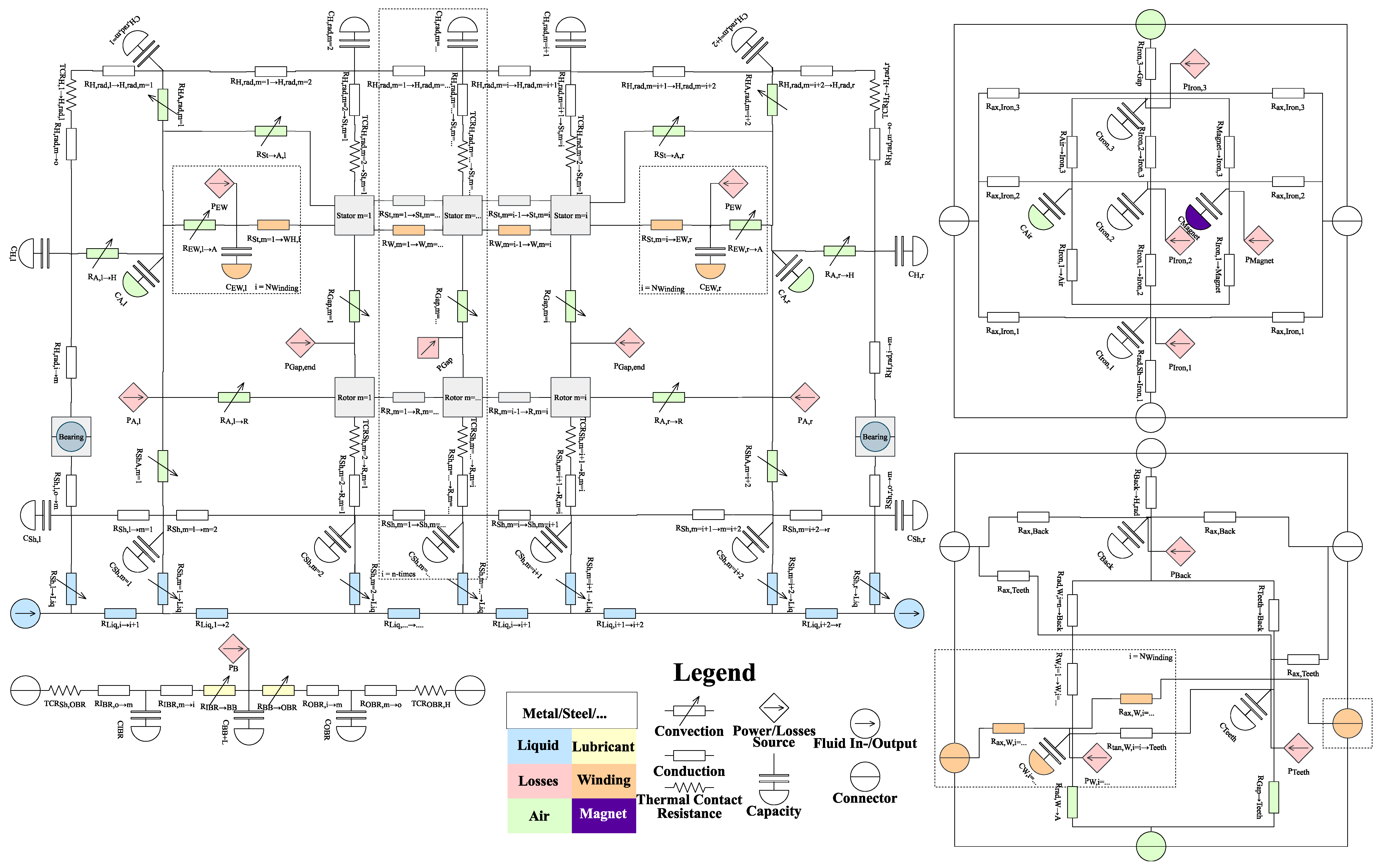
2. Thermal Model for Electric Traction Motors
3. Validation of the Thermal Model
3.1. Identification of the Best Heat Transfer Correlation Set
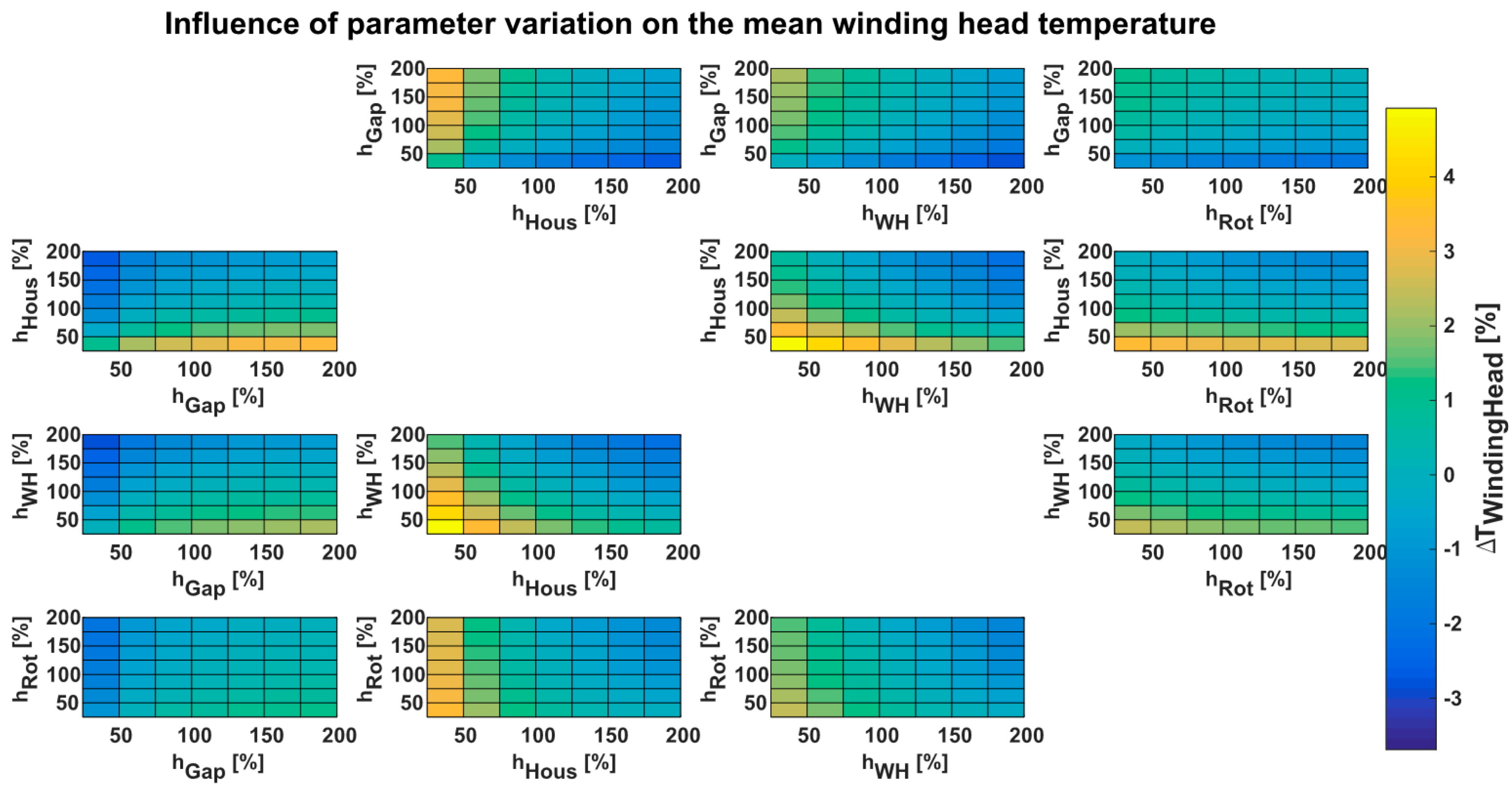
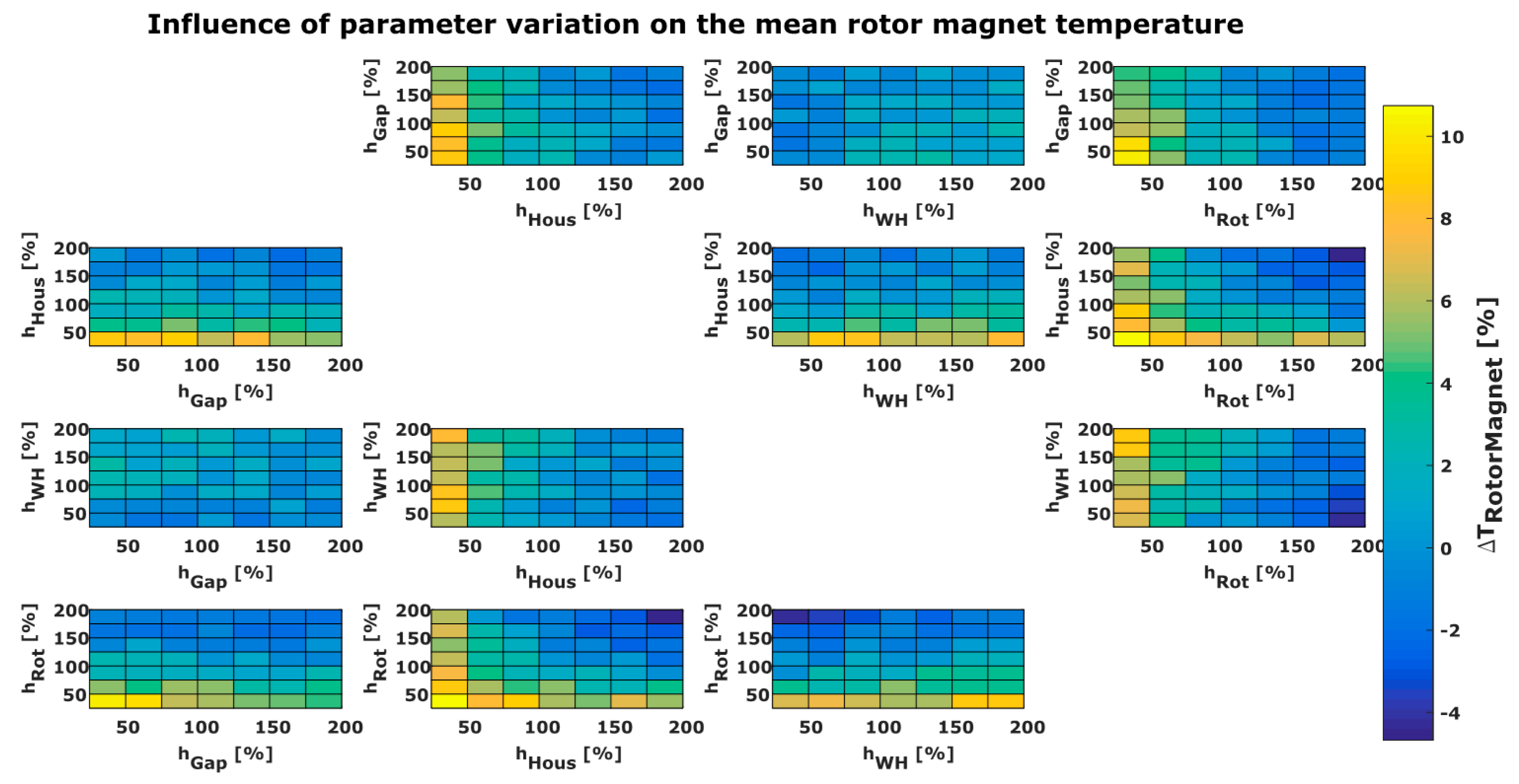
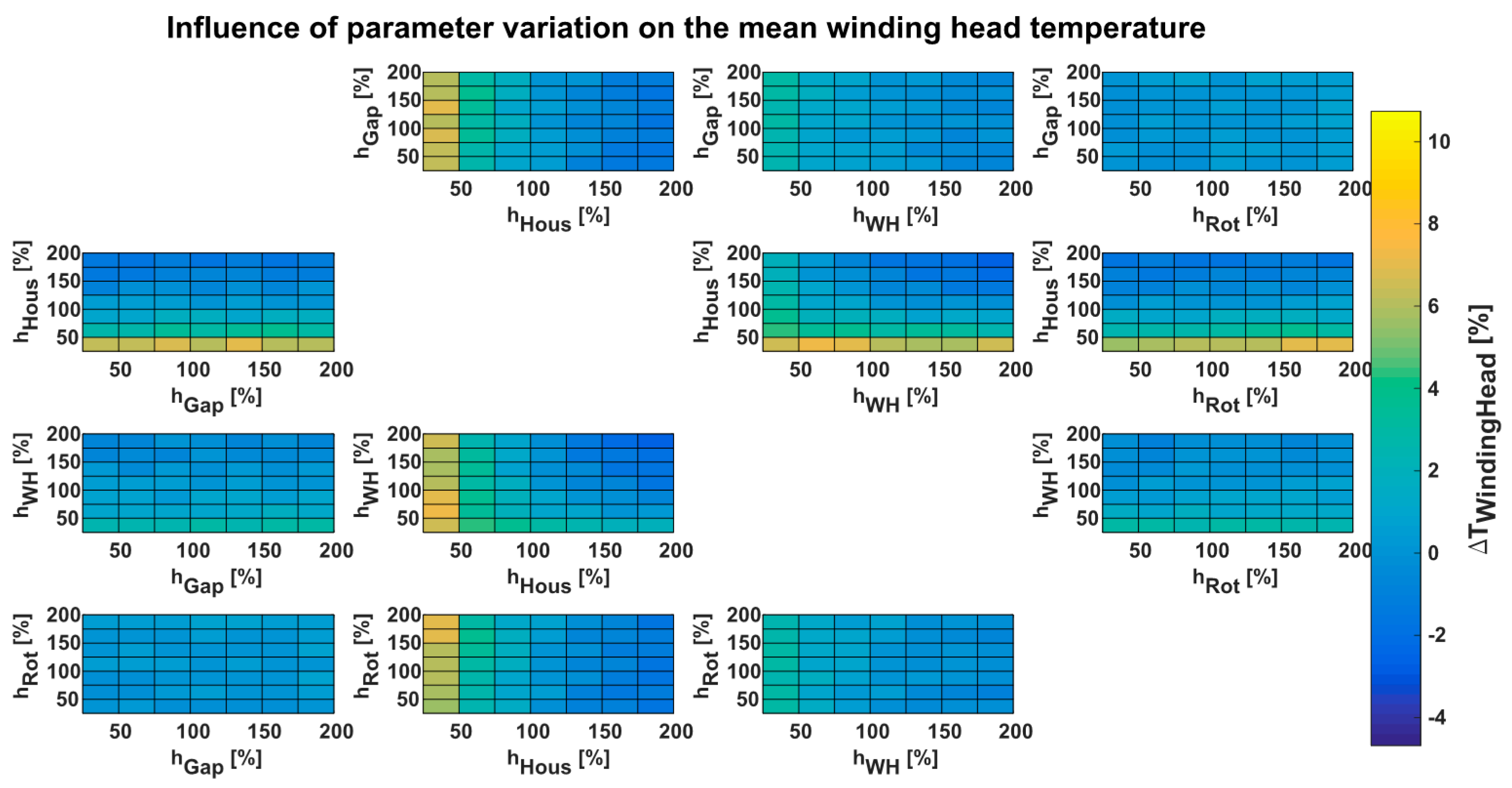
3.2. Parameter Studies of the Critical Heat Transfer Paths
3.3. Validation with Measurement Data from Different Electrical Machines
3.3.1. Simulation Process, Measurement System and Errors
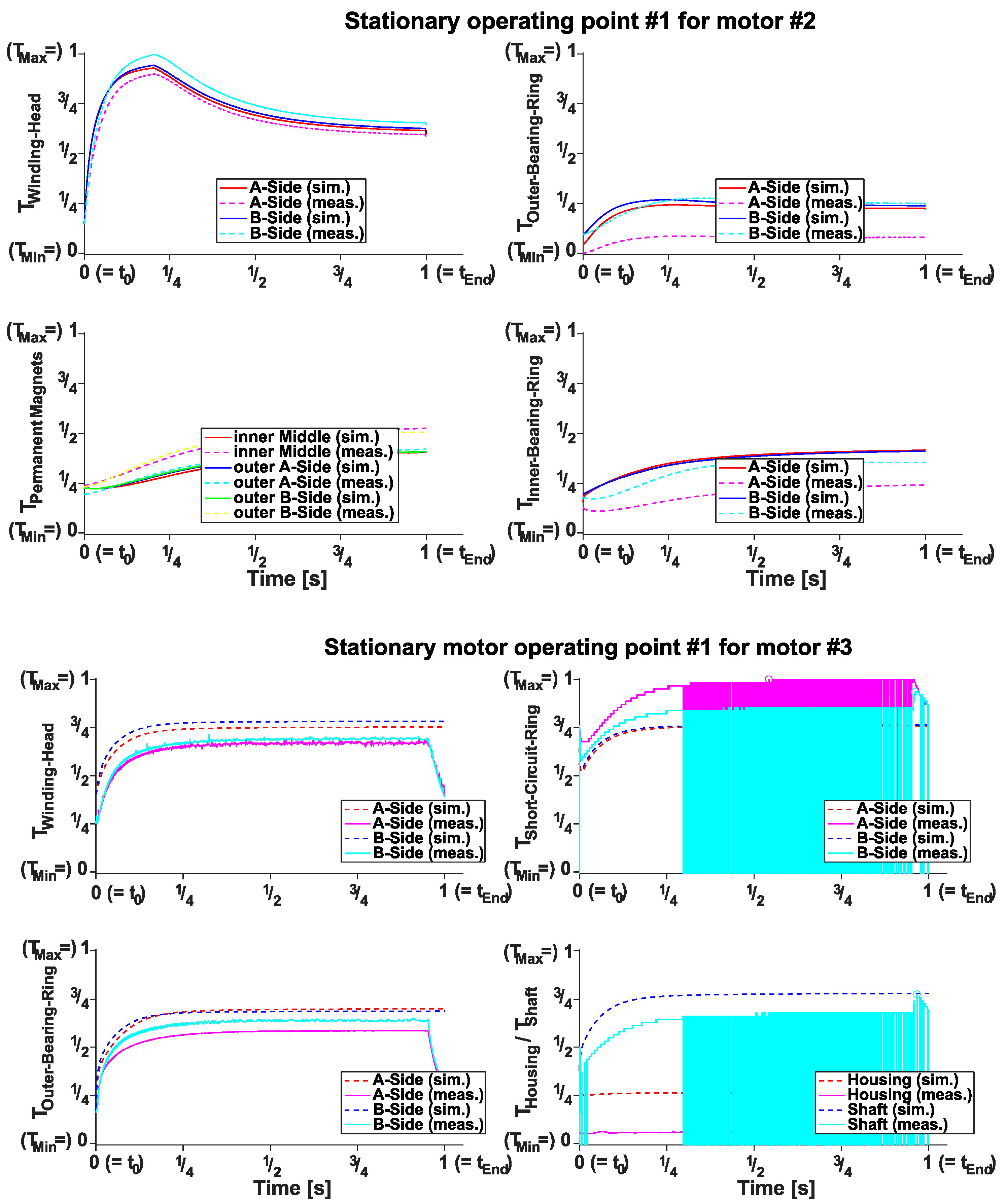
3.3.2. Stationary Operating Points
3.3.3. Peak Operating Points
3.3.4. Dynamic Drive Cycles
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
Nomenclature
| Symbols | |
| G | Geometrical factor |
| h | Heat transfer coefficient |
| Nu | Nusselt number |
| Pr | Prandtl number |
| Re | Reynolds number |
| t | Time |
| T | Temperature |
| Greek symbols | |
| Temperature change [%] | |
| Temperature difference [°C] | |
| Air gap ratio | |
| Temperature | |
| Subscripts | |
| 0 | Start time |
| 0, 1, 2,..., n | Index |
| A | Air |
| eff | effective |
| end | End time of measurement |
| Gap | Air gap |
| i | Index |
| i | inner |
| max | Maximum |
| min | Minimum |
| meas | measured |
| o | outer |
| R | Rotor |
| Rer | Rotor end ring |
| sim | simulated |
| Sh | Shaft |
| St | Stator |
| stat | stationary |
Abbreviations
| DU | Drive unit |
| ETM | Electric traction motor |
| Gap | Rotor stator air gap |
| Hous | Housing |
| HTC | Heat transfer coefficient |
| IM | Induction motor |
| LPTN | Lumped parameter thermal network |
| PMSM | Permanent magnet synchronous motor |
| RE | Relative error |
| Rot | Rotor |
| WH | Winding head (=end winding) |
References
- Bilgin, B.; Liang, J.; Terzic, M.V.; Dong, J.; Rodriguez, R.; Trickett, E.; Emadi, A. Modeling and Analysis of Electric Motors: State-of-the-Art Review. IEEE Trans. Transp. Electrific. 2019, 5, 602–617. [Google Scholar] [CrossRef]
- Gundabattini, E.; Kuppan, R.; Solomon, D.G.; Kalam, A.; Kothari, D.P.; Abu Bakar, R. A review on methods of finding losses and cooling methods to increase efficiency of electric machines. Ain. Shams. Eng. J. 2020. [Google Scholar] [CrossRef]
- Engelhardt, T.; Lange, J.; Oechslen, S.; Heitmann, A. Maximierung der Leistungsdichte elektrischer Maschinen durch elektromagnetische und thermische Maßnahmen. In Elektrische Antriebstechnologie für Hybrid und Elektrofahrzeuge; Schäfer, H., Ed.; Expert Verlag GmbH: Würzburg, Germany, 2019; pp. 13–24. ISBN 978-3-8169-8483-2. [Google Scholar]
- Grunditz, E.A.; Thiringer, T.; Saadat, N. Acceleration, Drive Cycle Efficiency, and Cost Tradeoffs for Scaled Electric Vehicle Drive System. IEEE Trans. Ind. Appl. 2020, 56, 3020–3033. [Google Scholar] [CrossRef]
- Hombitzer, M.; Franck, D.; von Pfingsten, G.; Hameyer, K. Permanentmagneterregter Traktionsantrieb für ein Elektrofahrzeug: Bauraum, Wirkungsgrad und Kosten–das Auslegungsdreieck. In Elektrische Antriebstechnologie für Hybrid-und Elektrofahrzeuge; Expert Verlag: Renningen, Germany, 2014; ISBN 978-3-8169-3239-0. [Google Scholar]
- Li, B.; Kuo, H.; Wang, X.; Chen, Y.; Wang, Y.; Gerada, D.; Worall, S.; Stone, I.; Yan, Y. Thermal Management of Electrified Propulsion System for Low-Carbon Vehicles. Automot. Innov. 2020, 3, 299–316. [Google Scholar] [CrossRef]
- Gronwald, P.; Kern, T.A. Traction motor cooling systems, a literature review and comparative study. IEEE Trans. Transp. Electrif. 2021. [Google Scholar] [CrossRef]
- Kylander, G. Thermal Modelling of Small Cage Induction Motors; Chalmers University of Technology: Göteborg, Sweden, 1995; ISBN 9171970614. [Google Scholar]
- Meksi, O.; Roberts, D.; Turner, D.R. Lumped parameter thermal model for electrical machines of TEFC design. IEE Proc. B Electr. Power Appl. 1991, 138, 205–218. [Google Scholar] [CrossRef]
- Tovar-Barranco, A.; Lopez-de-Heredia, A.; Villar, I.; Briz, F. Modeling of End-Space Convection Heat-Transfer for Internal and External Rotor PMSMs with Fractional-Slot Concentrated Windings. IEEE Trans. Ind. Electron. 2020, 1. [Google Scholar] [CrossRef]
- Boglietti, A.; Cavagnino, A. Analysis of the Endwinding Cooling Effects in TEFC Induction Motors. IEEE Trans. Ind. Appl. 2007, 43, 1214–1222. [Google Scholar] [CrossRef]
- Boutarfa, R.; Harmand, S. Local convective heat transfer for laminar and turbulent flow in a rotor-stator system. Exp. Fluids 2005, 38, 209–221. [Google Scholar] [CrossRef]
- Chen, Q.; Shao, H.; Huang, J.; Sun, H.; Xie, J. Analysis of Temperature Field and Water Cooling of Outer Rotor In-Wheel Motor for Electric Vehicle. IEEE Access 2019, 7, 140142–140151. [Google Scholar] [CrossRef]
- Cezário, C.; Verardi, M.; Borges, S.; Silva, J.; Antônio, A.; Oliveira, M. Transient Thermal Analysis of an induction Electric Motor. In Proceedings of the International Congress of Mechanical Engineering, Ouro Preto, Brazil, 6–11 November 2005. [Google Scholar]
- Kumar, R.R.; Singh, S.K.; Srivastava, R.K.; Saket, R.K. The thermal analysis of five-phase pmsg for small-scale wind power application. Int. J. Mech. Prod. Eng. Res. Dev. 2018, 8, 667–680. [Google Scholar]
- Cuiping, L.; Yulong, P.; Ronggan, N.; Shukang, C. Analysis of 3D Static Temperature Field of Water Cooling Induction Motor in Mini Electric Vehicle. In Proceedings of the 2011 International Conference on Electrical Machines and Systems, Beijing, China, 20–23 August 2011. [Google Scholar]
- Huang, Z.; Fang, J.; Liu, X.; Han, B. Loss Calculation and Thermal Analysis of Rotors Supported by Active Magnetic Bearings for High-Speed Permanent-Magnet Electrical Machines. IEEE Trans. Ind. Electron. 2016, 63, 2027–2035. [Google Scholar] [CrossRef]
- Jelden, H.; Lück, P.; Kruse, G.; Tousen, J. Der elektrische Antriebsbaukasten von Volkswagen. In Fahrerassistenzsysteme und Effiziente Antriebe; Siebenpfeiffer, W., Ed.; Springer Fachmedien Wiesbaden: Wiesbaden, Germany, 2015; pp. 84–93. ISBN 978-3-658-08161-4. [Google Scholar]
- Jelden, H.; Lück, P.; Kruse, G.; Tousen, J. The Electric Powertrain Matrix from Volkswagen. MTZ Worldw. 2014, 75, 4–9. [Google Scholar] [CrossRef]
- Blumenröder, K.; Bennewitz, K.; Lück, P.; Tousen, J.; Estorf, M. Der neue Modulare E-Antriebs-Baukasten von Volkswagen: Volkswagen’s new modular e-drive kit. In Proceedings of the 40th Internationales Wiener Motorensymposium, Vienna, Austria, 15–17 May 2019; Gerlinger, B., Lenz, P., Eds.; VDI Verlag: Düsseldorf, Germany, 2019. [Google Scholar]
- Helbing, C.; Bennewitz, K.; Lück, P.; Tousen, J.; Peter, J. Der Antriebsstrang des ID.CROZZ–Volkswagen erweitert das Portfolio des MEB: The powertrain of the ID.CROZZ–Volkswagen expands the portfolio of the MEB. In Proceedings of the 41th Internationales Wiener Motorensymposium, Vienna, Austria, 22–24 April 2020; Gerlinger, B., Lenz, P., Eds.; VDI Verlag: Düsseldorf, Germany, 2020. [Google Scholar]


| Motor Parts | Stationary Cooling | Rotating Cooling |
|---|---|---|
| Housing | Axial, circumferential and radial cooling jackets | |
| Stator | Spray cooling Jet impingement cooling Oil flushing Microchannel cooling Heat pipe cooling | |
| Winding heads | Spray cooling Jet impingement cooling Oil flushing | Rotating spray cooling (by rotor shaft and direct cooled rotor ducts) |
| Windings | Microchannel cooling with cooling channel inside the wire and between the wires | |
| Rotor | Spray cooling Jet impingement cooling | Rotor shaft cooling Direct cooled rotor Rotating heat pipes Rotor vent holes |
| Bearings | Liquid cooled bearings |
| Motor Parts | Heat Transfer Path | Number of Different HTCs Used |
|---|---|---|
| Winding head | Winding head→Air | 7 (same correlations used) |
| Stator back iron | Axial stator iron→Air | |
| Axial rotor balancing disc/ Short-circuit rings | Axial rotor sides→Air | 5 |
| Air gap | Rotor↔Air↔Stator | 6 |
| Motor housing | Air→Housing | 4 |
| Shaft | Shaft→Air | 1 |
| 840 combinations |
| Heat Transfer Path | PMSM | IM | |
|---|---|---|---|
| Winding head→Air | Tovar-Barranco et al. [10]: | Boglietti and Cavagnino [11]: | |
| Axial stator iron→Air | |||
| Axial rotor sides→Air | Boutarfa and Harmand [12] | Chen et al. [13]: Kaviany et al. [14] (for short circuit rings/rotor end rings): | |
| Rotor↔Air↔Stator | Kumar et al. [15] | Cuiping et al. [16] with: ; ; | |
| Air→Housing | Boutarfa and Harmand [12] (same equation as in line “Axial rotor sides→Air”) | ||
| Shaft→Air | Wang et al. (from [17]) | ||
| Motor | #1 | #2 and #2b | #3 | #4 | #5 |
|---|---|---|---|---|---|
| Motor type | PMSM | PMSM | IM | IM | PMSM |
| Motor peak power class | Class 3 100–140 kW | Class 4 140–180 kW | Class 2 60–100 kW | Class 5 >180 kW | |
| Nmax [rpm] | 12,000 | 16,000 | 14,000 | 14,000 | - |
| Cooling Concept | water jacket-cooling | Two different water jacket-cooling designs | water jacket-cooling and oil cooled short-circuit rings and winding heads | Water and oil cooled | Water jacket and direct oil cooled components |
| Information source | [18,19] | [20] | [21] | Internal | Internal |
| Schematic cooling system |  |  |  |  |  |
| Motor | #1 | #2 | #2b | #3 | #4 | #5 | #5 |
|---|---|---|---|---|---|---|---|
| Electric traction motor or drive unit? | DU | DU | ETM | DU | DU | ETM | DU |
| Winding head A | −5.49% | 2.57% | 3.65% | −8.06% | 6.16% | 1.60% | −13.01% |
| Winding head B | −8.51% | 3.44% | 1.78% | −6.77% | 6.86% | 2.69% | −11.74% |
| Winding head max | −6.63% | 7.70% | 2.17% | ||||
| Stator winding | 4.13% | ||||||
| Stator (back) iron | −28.70% | −16.87% | 8.43% | 5.7% to 17.8% | −1.09% | ||
| Outer Bearing A | −20.42% | −17.82% | 5.74% | 7.98% | −8.67% | −0.07% | |
| Outer Bearing B | −4.02% | 6.27% | 9.70% | −6.87% | 1.09% | ||
| Inner bearing A | −36.34% | −36.75% | −53.21% | −51.39% | −42.47% | ||
| Inner bearing B | −29.52% | −11.44% | −42.94% | −33.95% | |||
| Shaft A | −18.36% | −6.98% | −17.75% | ||||
| Shaft M | −11.70% | −11.37% | |||||
| Shaft B | −27.02% | 0.97% | −17.83% | ||||
| Rotor magnet A | 3.52% | −18.23% | |||||
| Rotor magnet M | 6.67% | 20.28% | −5.51% | −3.51% | |||
| Rotor magnet B | 9.81% | −10.92% | |||||
| Short circuit ring A | −1.75% | −3.29% | |||||
| Short circuit ring B | −4.48% | −0.99% | |||||
| Rotor sheet A | −1.93% | ||||||
| Rotor sheet B | −1.18% | ||||||
| Housing A | −33.68% | −22.64% | |||||
| Housing M | 4.18% | 5.45% | −4.42% | ||||
| Housing B | −16.69% | −9.10% | |||||
| Number of measurements | 9 | 85 | 17 | 12 | 28 | 47 | 21 |
| Motor | #2b | #3 | #4 | #5 | #5 |
|---|---|---|---|---|---|
| Electric traction motor or drive unit? | ETM | DU | DU | ETM | DU |
| Winding head A | 3.77% | −4.22% | −0.78% | 6.64% | −4.46% |
| Winding head B | −1.82% | −6.91% | −1.56% | −9.79% | |
| Winding head max | −4.75% | 1.32% | −4.49% | ||
| Stator winding | −2.11% | ||||
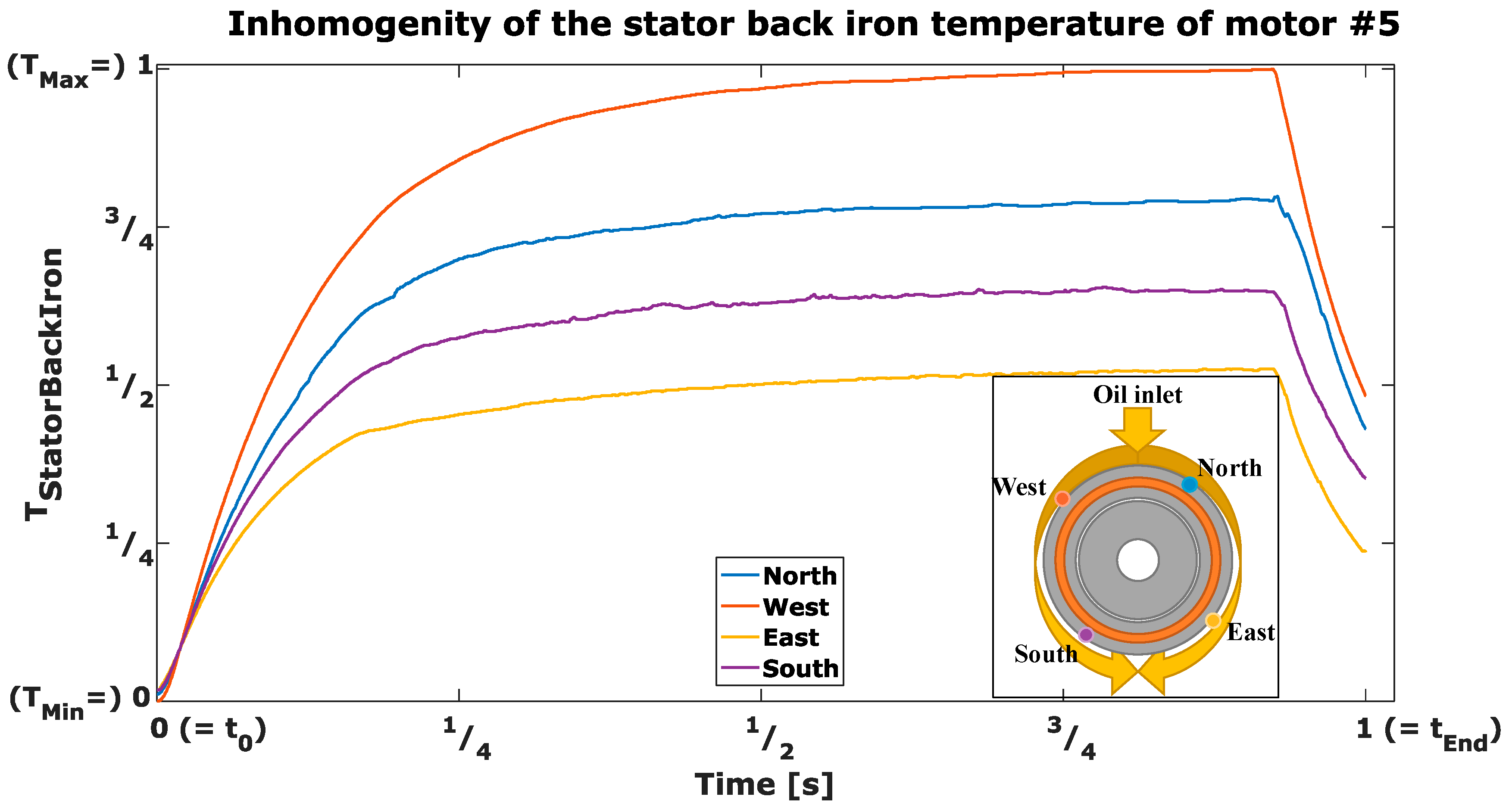
| Stator (back) iron max | 33.64% | −2.16% | |||
| Stator (back) iron mean | 2.15% | 15.26% | −8.70% | ||
| Stator (back) iron min | 2.22% | −14.64% | |||
| Outer Bearing A | −5.77% | −0.80% | −4.78% | ||
| Outer Bearing B | −4.38% | ||||
| Inner bearing A | 59.07% | 2.84% | −26.06% | ||
| Inner bearing B | 3.28% | −23.10% | |||
| Shaft A | 8.33% | ||||
| Shaft M | 10.78% | 2.73% | |||
| Shaft B | 7.04% | ||||
| Rotor magnet A | −10.76% | ||||
| Rotor magnet M | −0.97% | ||||
| Rotor magnet B | −4.50% | ||||
| Short circuit ring A | 30.50% | 11.20% | |||
| Short circuit ring B | 19.51% | 9.67% | |||
| Rotor sheet A | 11.41% | ||||
| Rotor sheet B | 10.51% | ||||
| Number of measurements | 17 | 30 | 32 | 48 | 12 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gronwald, P.-O.; Kern, T.A. Experimental Validation and Parameter Study of a 2D Geometry-Based, Flexible Designed Thermal Motor Model for Different Cooled Traction Motor Drives. World Electr. Veh. J. 2021, 12, 76. https://doi.org/10.3390/wevj12020076
Gronwald P-O, Kern TA. Experimental Validation and Parameter Study of a 2D Geometry-Based, Flexible Designed Thermal Motor Model for Different Cooled Traction Motor Drives. World Electric Vehicle Journal. 2021; 12(2):76. https://doi.org/10.3390/wevj12020076
Chicago/Turabian StyleGronwald, Peer-Ole, and Thorsten Alexander Kern. 2021. "Experimental Validation and Parameter Study of a 2D Geometry-Based, Flexible Designed Thermal Motor Model for Different Cooled Traction Motor Drives" World Electric Vehicle Journal 12, no. 2: 76. https://doi.org/10.3390/wevj12020076
APA StyleGronwald, P.-O., & Kern, T. A. (2021). Experimental Validation and Parameter Study of a 2D Geometry-Based, Flexible Designed Thermal Motor Model for Different Cooled Traction Motor Drives. World Electric Vehicle Journal, 12(2), 76. https://doi.org/10.3390/wevj12020076






