Impact of Processing Parameters on the Quality of Pharmaceutical Solid Dosage Forms Produced by Fused Deposition Modeling (FDM)
Abstract
:1. Introduction
2. Materials and Methods
2.1. Materials
2.2. Methods
2.2.1. Preparation of Drug Loaded Filaments by HME
2.2.2. FDM 3D Printing of Commercial Filaments and Drug-Loaded Filaments
2.2.3. In Vitro Drug Release Studies
2.2.4. Melt Flow Index Measurements
2.2.5. Leveling of the Build Plate of the Printer
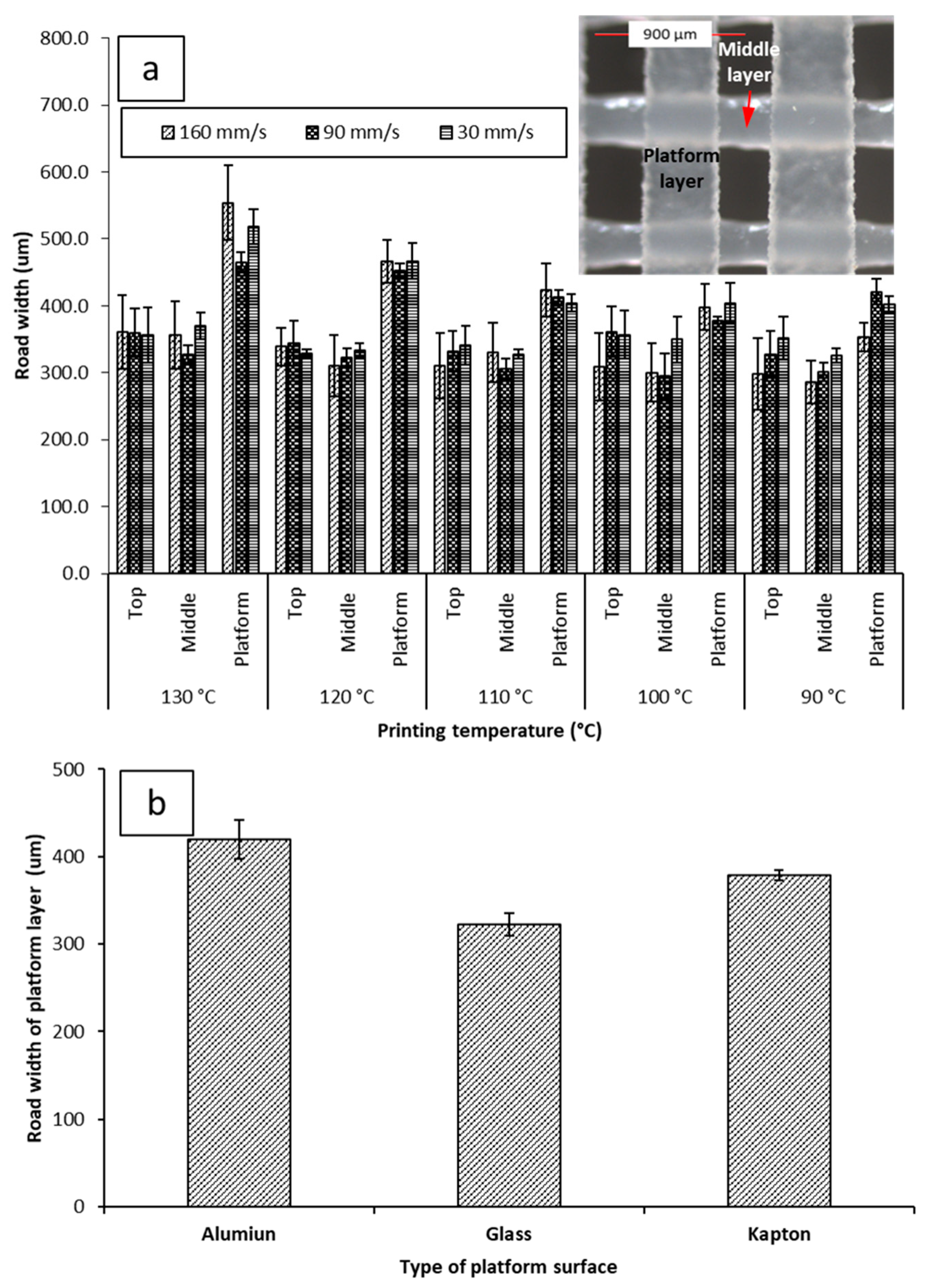
2.2.6. Printing on Different Surfaces
2.2.7. Characterization of Printed Solid Dosage Forms
2.2.8. Statistical Analysis
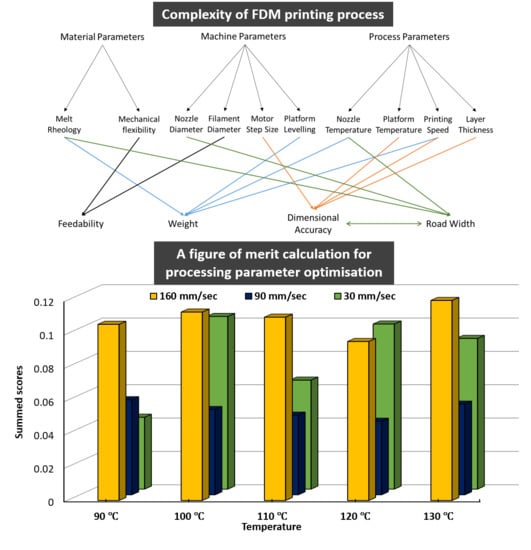
3. Results and Discussion
3.1. Impacts of Build Plate Leveling
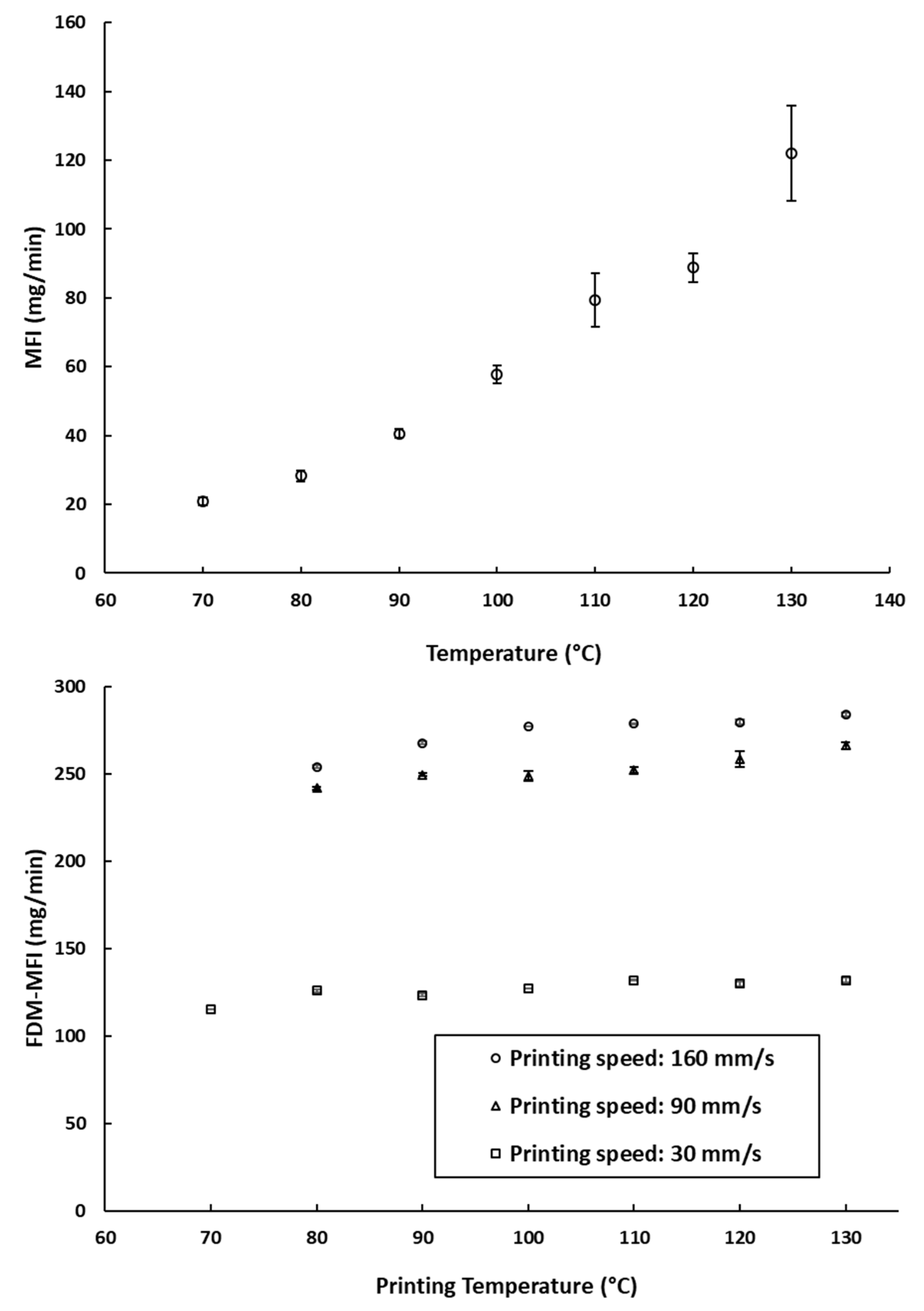
3.2. Impacts of Melt Flow of the Printed Materials
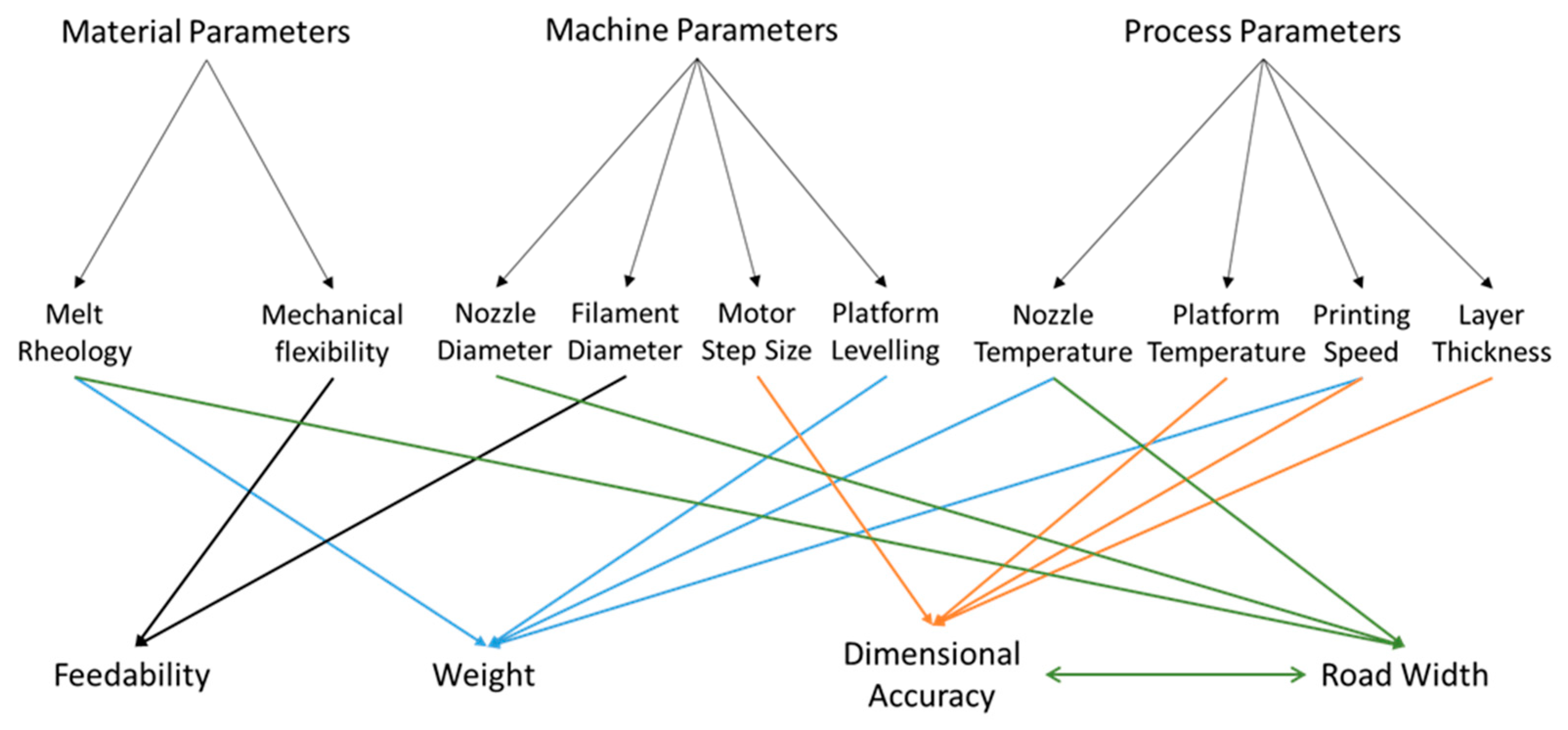
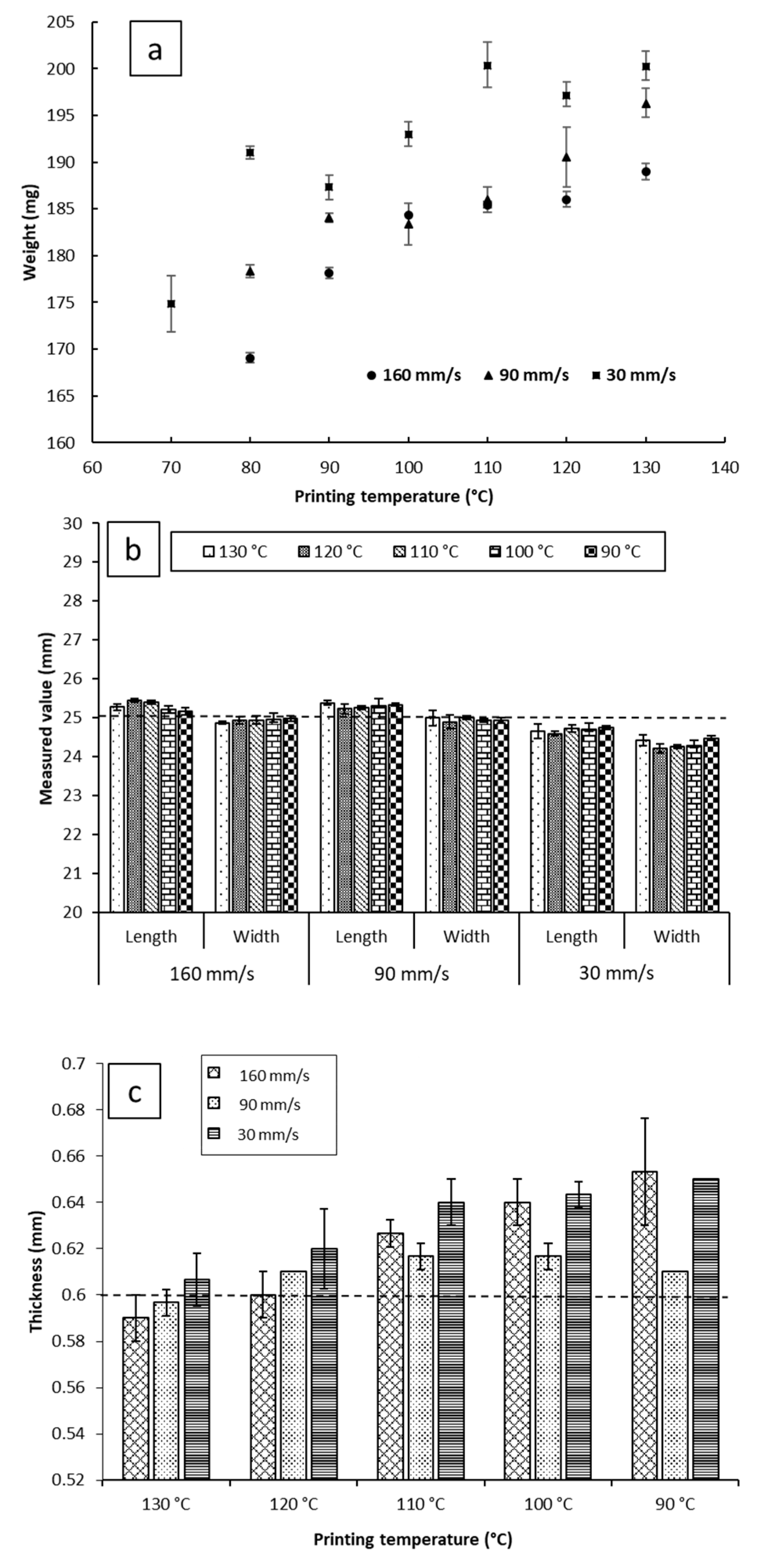
3.3. Impact of Processing Conditions on Weight Uniformity
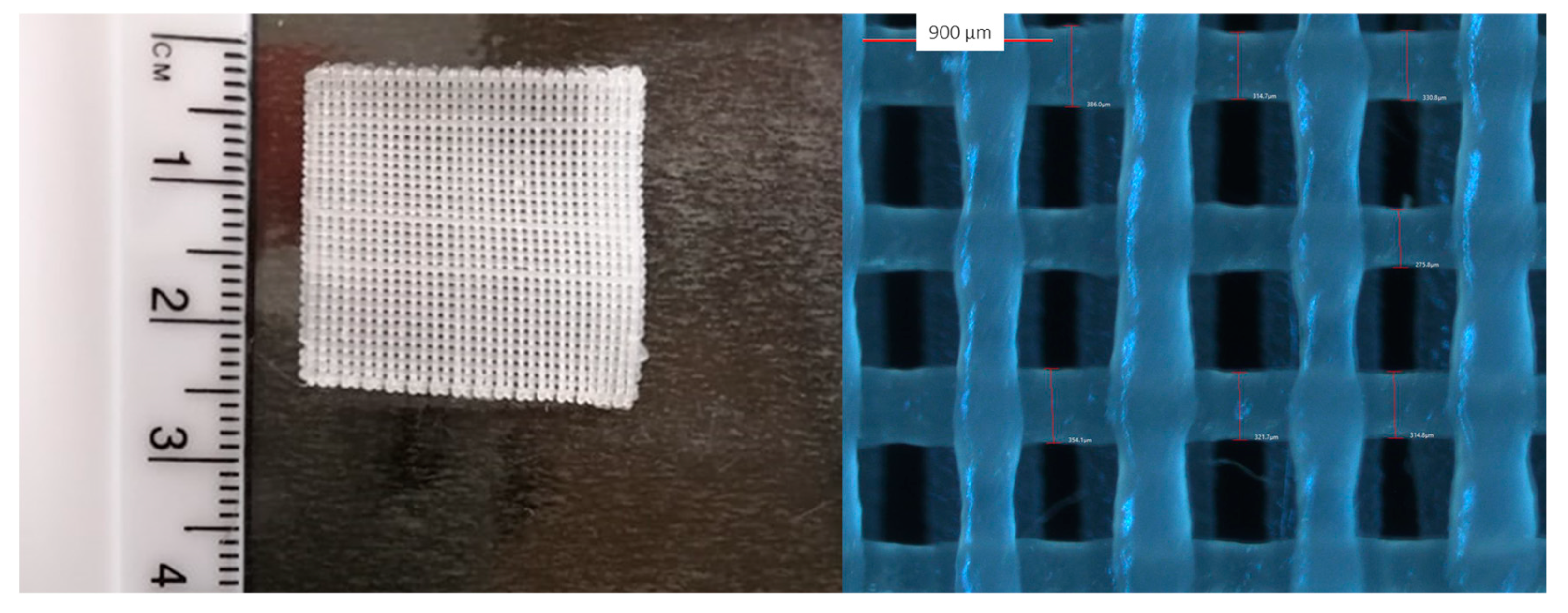
3.4. Impact of Processing Conditions on Dimensional Authenticity
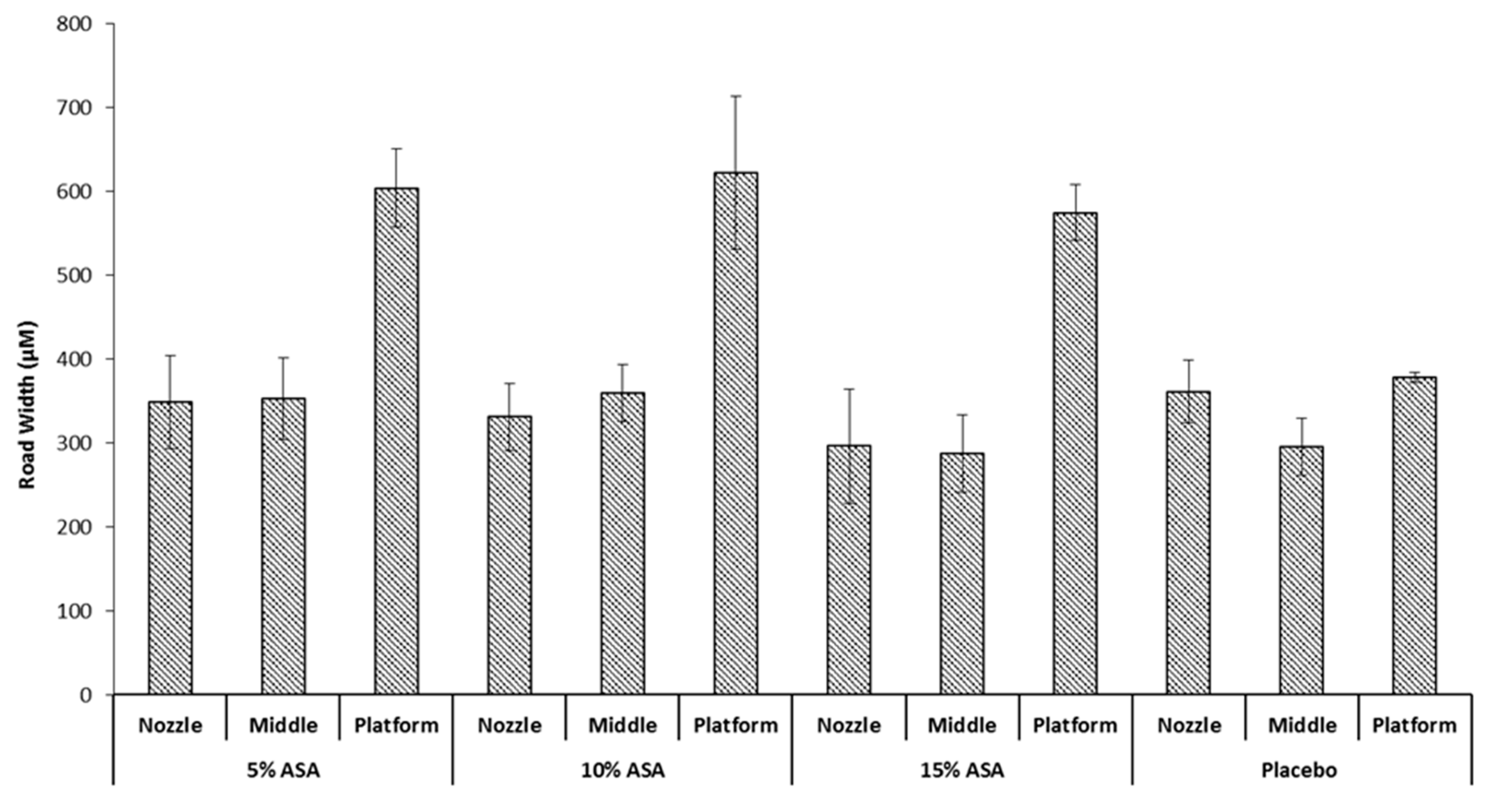
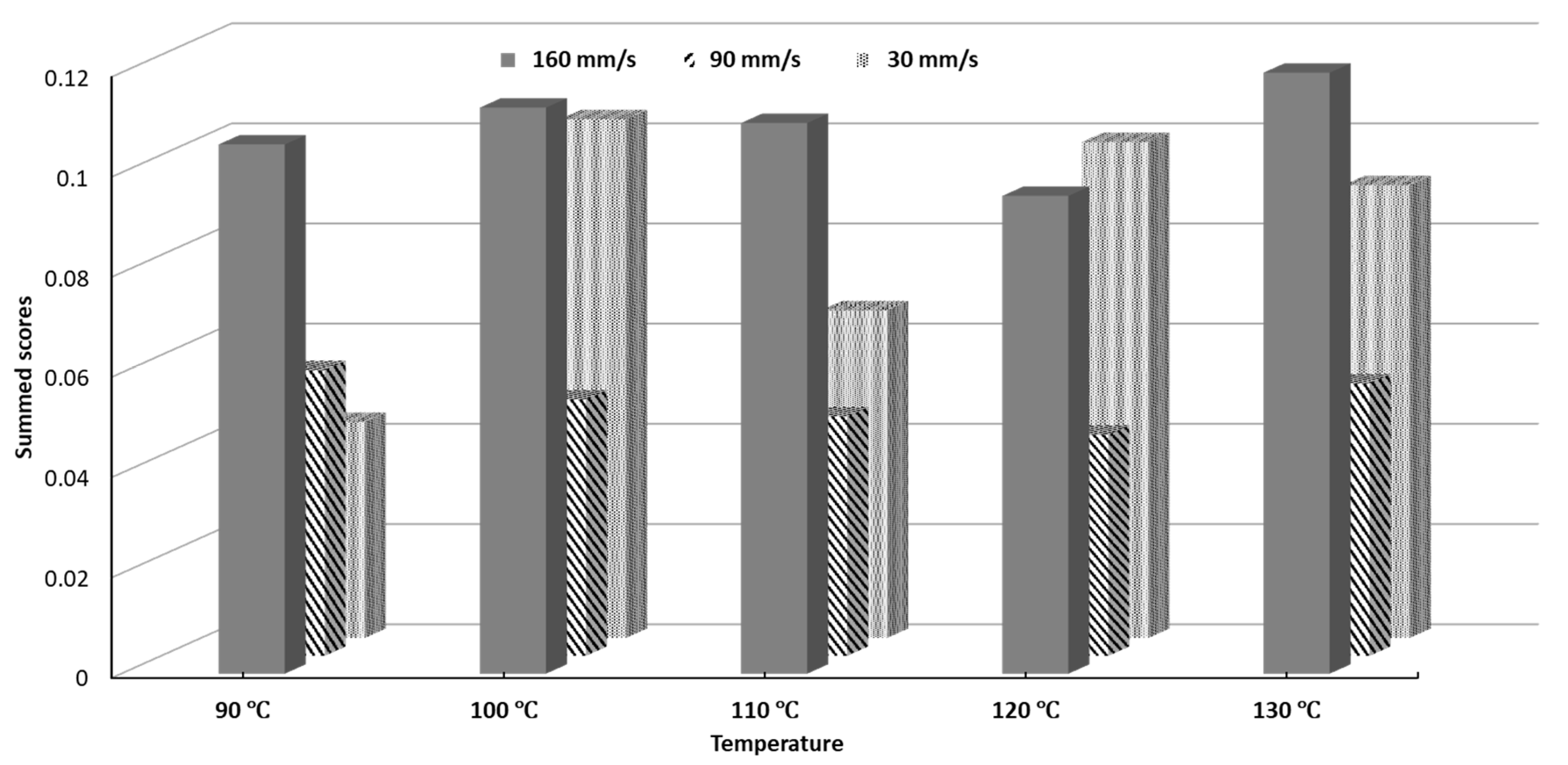
3.5. The First Layer Effect (FLE)
3.6. Impact of Drug Incorporation
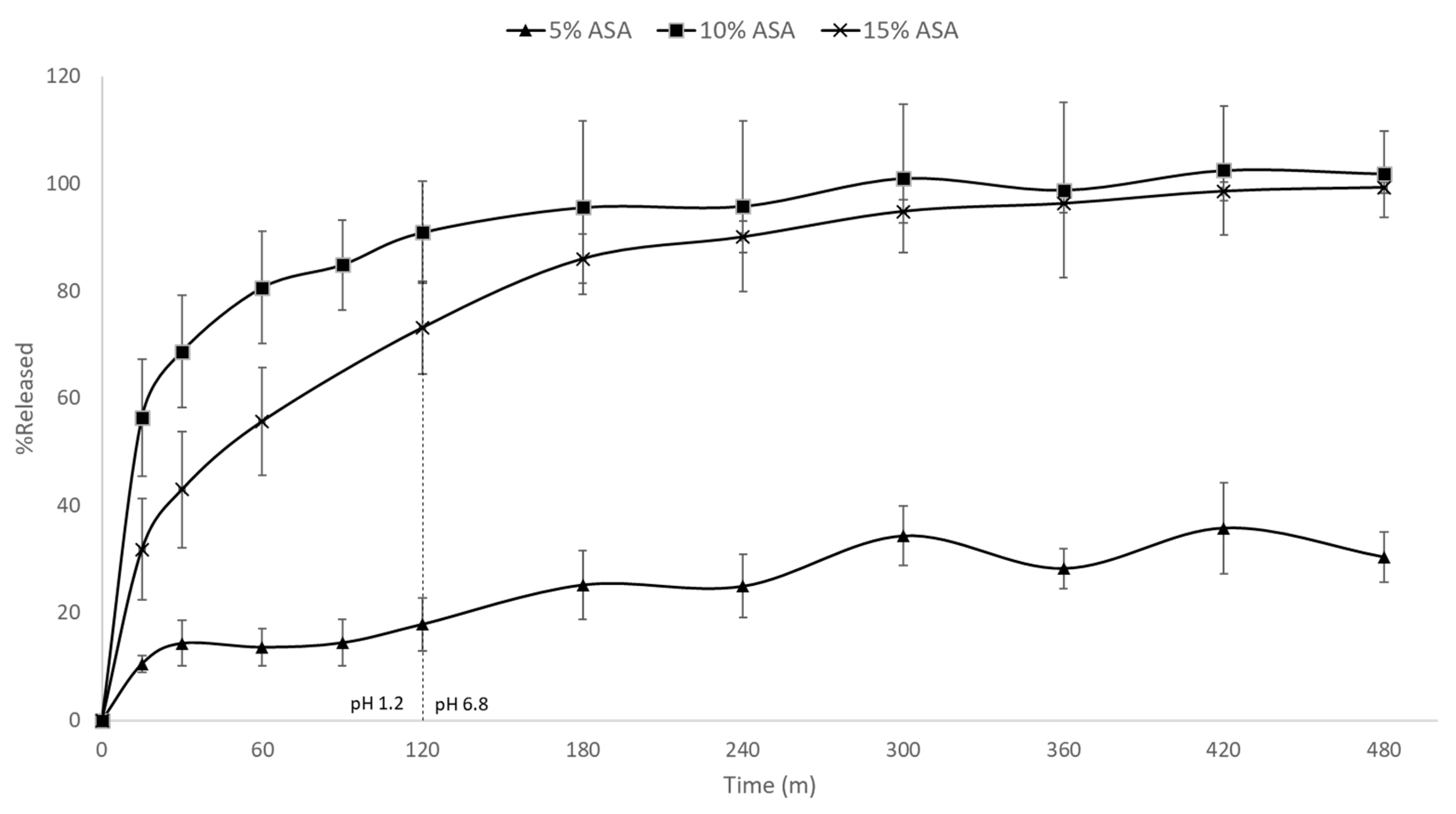
3.7. In Vitro Drug Release Studies
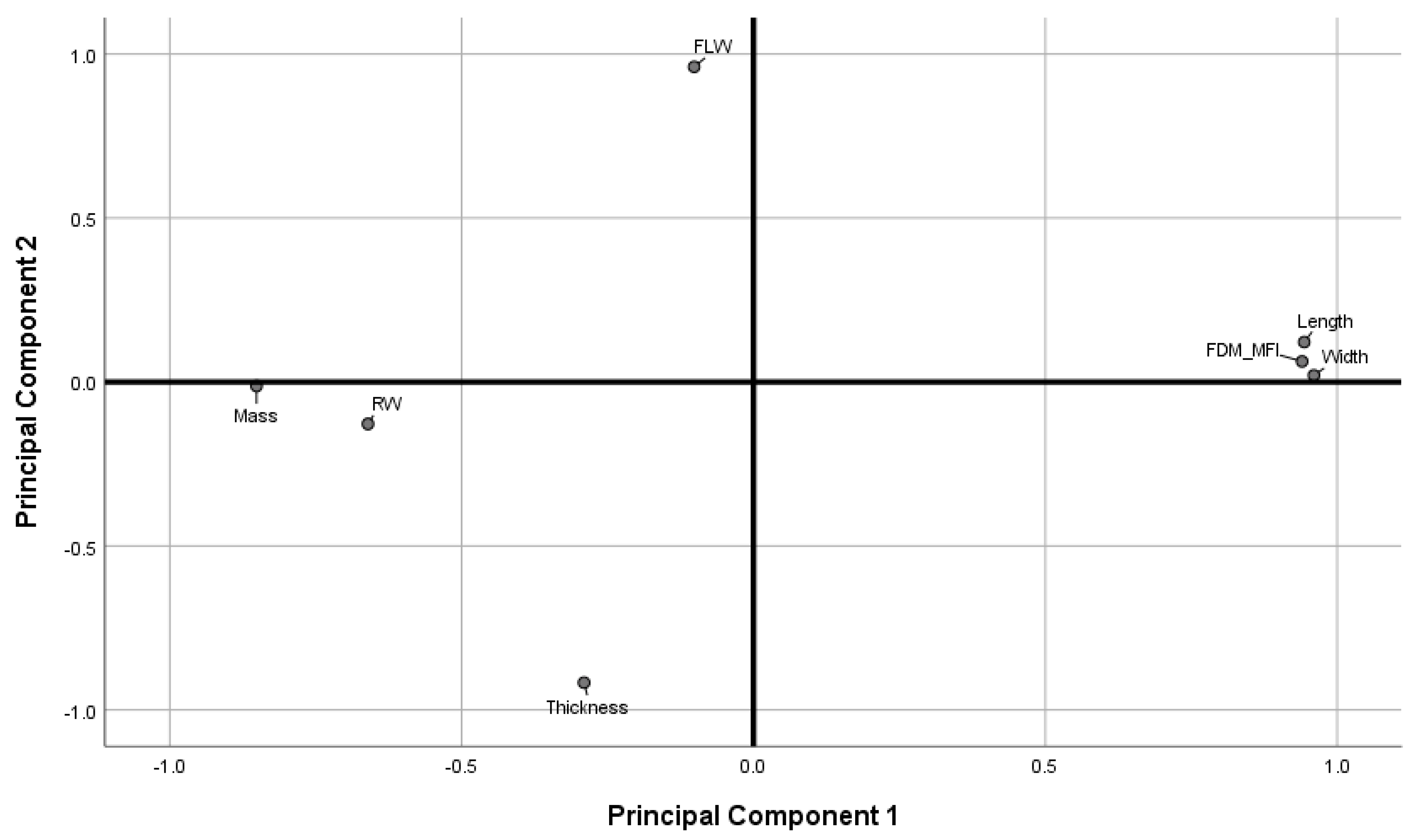
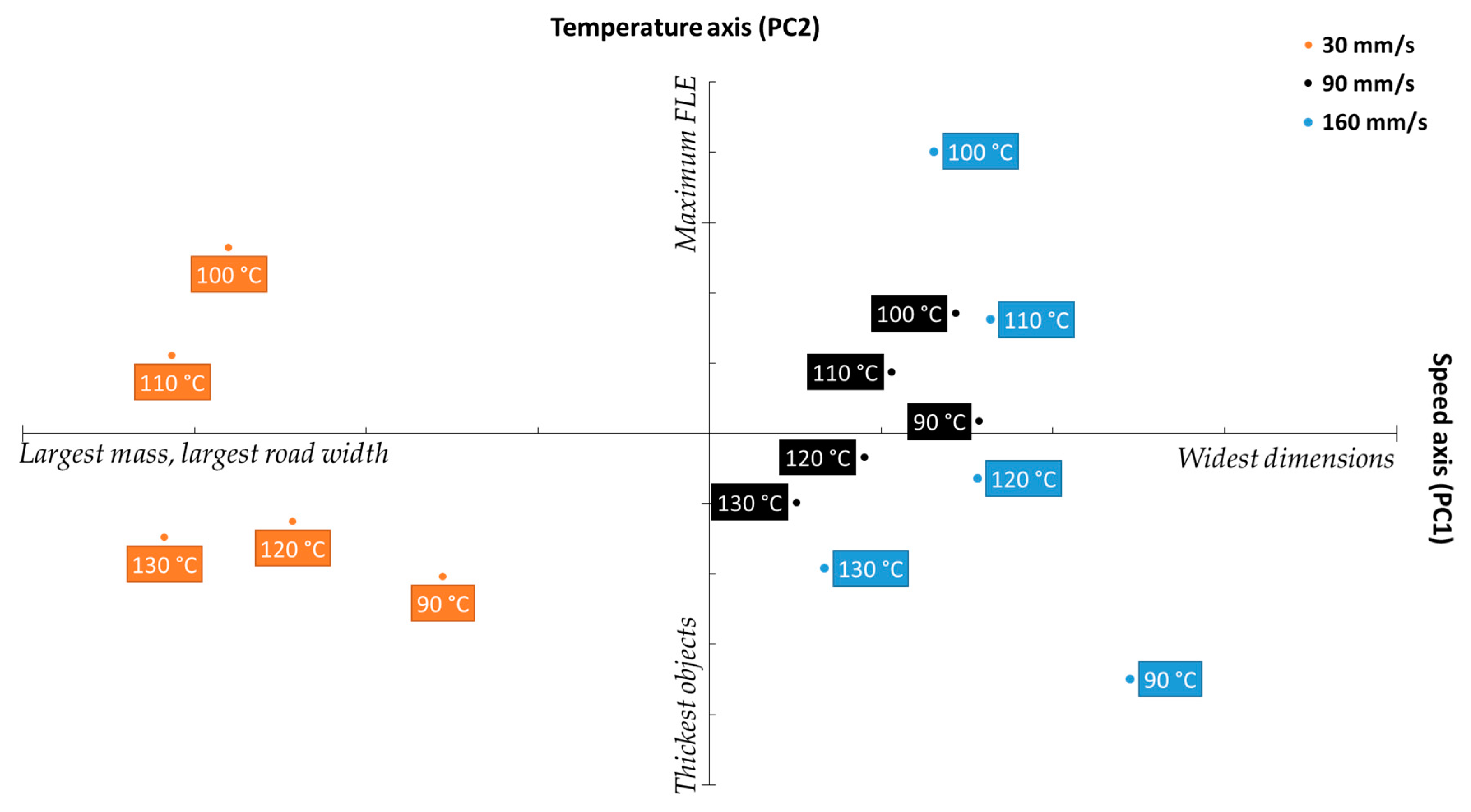
3.8. Statistical Analysis
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Hsiao, W.-K.; Lorber, B.; Reitsamer, H.; Khinast, J. 3D printing of oral drugs: A new reality or hype? Expert Opin. Drug Deliv. 2017, 15, 1–4. [Google Scholar] [CrossRef] [PubMed]
- Wening, K.; Breitkreutz, J. Oral drug delivery in personalized medicine: Unmet needs and novel approaches. Int. J. Pharm. 2011, 404, 1–9. [Google Scholar] [CrossRef] [PubMed]
- Joo, Y.; Shin, I.; Ham, G.; Abuzar, S.M.; Hyun, S.-M.; Hwang, S.-J. The advent of a novel manufacturing technology in pharmaceutics: Superiority of fused deposition modeling 3D printer. J. Pharm. Investig. 2019. [Google Scholar] [CrossRef]
- Khaled, S.A.; Burley, J.C.; Alexander, M.R.; Yang, J.; Roberts, C.J. 3D printing of five-in-one dose combination polypill with defined immediate and sustained release profiles. J. Control. Release 2015, 217, 308–314. [Google Scholar] [CrossRef]
- Awad, A.; Fina, F.; Trenfield, S.; Patel, P.; Goyanes, A.; Gaisford, S.; Basit, A. 3D Printed Pellets (Miniprintlets): A Novel, Multi-Drug, Controlled Release Platform Technology. Pharmaceutics 2019, 11, 148. [Google Scholar] [CrossRef]
- Robles-Martinez, P.; Xu, X.; Trenfield, S.J.; Awad, A.; Goyanes, A.; Telford, R.; Basit, A.W.; Gaisford, S. 3D printing of a multi-layered polypill containing six drugs using a novel stereolithographic method. Pharmaceutics 2019, 11, 274. [Google Scholar] [CrossRef] [PubMed]
- Alhnan, M.A.; Okwuosa, T.C.; Sadia, M.; Wan, K.W.; Ahmed, W.; Arafat, B. Emergence of 3D Printed Dosage Forms: Opportunities and Challenges. Pharm. Res. 2016, 33, 1817–1832. [Google Scholar] [CrossRef] [PubMed]
- Melocchi, A.; Parietti, F.; Maroni, A.; Foppoli, A.; Gazzaniga, A.; Zema, L. Hot-melt extruded filaments based on pharmaceutical grade polymers for 3D printing by fused deposition modeling. Int. J. Pharm. 2016, 509, 255–263. [Google Scholar] [CrossRef] [PubMed]
- Alhijjaj, M.; Belton, P.; Qi, S. An investigation into the use of polymer blends to improve the printability of and regulate drug release from pharmaceutical solid dispersions prepared via fused deposition modeling (FDM) 3D printing. Eur. J. Pharm. Biopharm. 2016, 108, 111–125. [Google Scholar] [CrossRef]
- Prasad, E.; Islam, M.T.; Goodwin, D.J.; Megarry, A.J.; Halbert, G.W.; Florence, A.J.; Robertson, J. Development of a hot-melt extrusion (HME) process to produce drug loaded AffinisolTM 15LV filaments for fused filament fabrication (FFF) 3D printing. Addit. Manuf. 2019, 29, 100776. [Google Scholar] [CrossRef]
- Norman, J.; Madurawe, R.D.; Moore, C.M.V.; Khan, M.A.; Khairuzzaman, A. A new chapter in pharmaceutical manufacturing: 3D-printed drug products. Adv. Drug Deliv. Rev. 2017, 108, 39–50. [Google Scholar] [CrossRef] [PubMed]
- Katstra, W.E.; Rowe, C.W.; Palazzolo, R.D.; Giritlioglu, B.; Teung, P.; Cima, M.J.; Katstra, W.E.; Palazzolo, R.D.; Giritlioglu, B.; Teung, P.; et al. Oral dosage forms fabricated by Three Dimensional Printing. J. Control. Release 2000, 66, 11–17. [Google Scholar] [CrossRef]
- Pietrzak, K.; Isreb, A.; Alhnan, M.A. A flexible-dose dispenser for immediate and extended release 3D printed tablets. Eur. J. Pharm. Biopharm. 2015, 96, 380–387. [Google Scholar] [CrossRef] [PubMed]
- Goyanes, A.; Robles Martinez, P.; Buanz, A.; Basit, A.W.; Gaisford, S. Effect of geometry on drug release from 3D printed tablets. Int. J. Pharm. 2015, 494, 657–663. [Google Scholar] [CrossRef] [PubMed]
- Goyanes, A.; Buanz, A.B.M.M.; Hatton, G.B.; Gaisford, S.; Basit, A.W. 3D printing of modified-release aminosalicylate (4-ASA and 5-ASA) tablets. Eur. J. Pharm. Biopharm. 2015, 89, 157–162. [Google Scholar] [CrossRef]
- Goyanes, A.; Buanz, A.B.M.; Basit, A.W.; Gaisford, S. Fused-filament 3D printing (3DP) for fabrication of tablets. Int. J. Pharm. 2014, 476, 88–92. [Google Scholar] [CrossRef]
- Korte, C.; Quodbach, J. Formulation development and process analysis of drug-loaded filaments manufactured via hot-melt extrusion for 3D-printing of medicines. Pharm. Dev. Technol. 2018, 23, 1117–1127. [Google Scholar] [CrossRef]
- Skowyra, J.; Pietrzak, K.; Alhnan, M.A. Fabrication of extended-release patient-tailored prednisolone tablets via fused deposition modelling (FDM) 3D printing. Eur. J. Pharm. Sci. 2015, 68, 11–17. [Google Scholar] [CrossRef]
- Nasereddin, J.M.; Wellner, N.; Alhijjaj, M.; Belton, P.; Qi, S. Development of a Simple Mechanical Screening Method for Predicting the Feedability of a Pharmaceutical FDM 3D Printing Filament. Pharm. Res. 2018, 35, 151. [Google Scholar] [CrossRef]
- Lim, S.H.; Kathuria, H.; Tan, J.J.Y.; Kang, L. 3D printed drug delivery and testing systems—A passing fad or the future? Adv. Drug Deliv. Rev. 2018. [Google Scholar] [CrossRef]
- Peng, A.; Xiao, X.; Yue, R. Process parameter optimization for fused deposition modeling using response surface methodology combined with fuzzy inference system. Int. J. Adv. Manuf. Technol. 2014, 73, 87–100. [Google Scholar] [CrossRef]
- Bakar, N.S.A.; Alkahari, M.R.; Boejang, H. Analysis on Fused Deposition Modelling Performance. J. Zhejiang Univ. Sci. A 2010, 11, 972–977. [Google Scholar] [CrossRef]
- Ziemian, S.; Okwara, M.; Ziemian, C.W. Tensile and fatigue behavior of layered acrylonitrile butadiene styrene. Rapid Prototyp. J. 2015, 21, 270–278. [Google Scholar] [CrossRef]
- Weeren, R.V.; Agarwala, M.; Jamalabad, V.R.; Bandyophadyay, A.; Vaidyanathan, R.; Langrana, N.; Ballard, C. Quality of Parts Processed by Fused Deposition. In Proceedings of the 1995 International Solid Freeform Fabrication Symposium, Austin, TX, USA, 1995; The University of Texas at Austin: Austin, TX, USA, 1995; pp. 314–321. [Google Scholar]
- Bharath Vasudevarao, B.; Dharma Prakash Natarajan, D.P.; Henderson, M.M.; Vasudevarao, B.; Natarajan, D.P.; Henderson, M.M. Sensitivitiy of RP Surface Finish to Process Parameter Variation. Solid Free. Fabr. Proc. 2000, 251–258. [Google Scholar]
- Dawson, P.C. Flow Properties of Molten Polymers. In Mechanical Properties and Testing of Polymers; Springer: Dordrecht, The Netherlands, 1999; pp. 88–95. [Google Scholar]
- Lu, L.; Chen, B.; Sharf, A.; Zhao, H.; Wei, Y.; Fan, Q.; Chen, X.; Savoye, Y.; Tu, C.; Cohen-Or, D. Build-to-last: Strength to Weight 3D Printed Objects. ACM Trans. Graph. 2014, 33, 1–10. [Google Scholar] [CrossRef]
- Gokstorp, D. Prototyping a Custom 3D Printing Slicer Implementation of a Prototype Modular Infill, Tool Path and Gcode Generator in Houdini. Master’s Thesis, Bournemouth University, Poole, UK, 2016. [Google Scholar]
- Wang, Y.; Xu, P.P.; Li, X.X.; Nie, K.; Tuo, M.F.; Kong, B.; Chen, J. Monitoring the hydrolyzation of aspirin during the dissolution testing for aspirin delayed-release tablets with a fiber-optic dissolution system. J. Pharm. Anal. 2012, 2, 386–389. [Google Scholar] [CrossRef]
- International Organization for Standardization. ISO 1133-1:2011–Plastics—Determination of the Melt Mass-Flow Rate (MFR) and Melt Volume-Flow Rate (MVR) of Thermoplastics—Part 1: Standard Method. Available online: https://www.iso.org/standard/44273.html (accessed on 21 July 2018).
- Makerbot LLC. (n.d.). User Manual|Replicator® 2X Dual Extrusion Experimental 3D Printer. Available online: http://downloads.makerbot.com/replicator2x/MakerBot_Replicator_2X_User_Manual.pdf (accessed on 4 October 2017).
- Ringnér, M. What is principal component analysis? Nat. Biotechnol. 2008, 26. [Google Scholar] [CrossRef]
- Williams, L.J. Principal Component Analysis. Wiley Interdiscip. Rev. Comput. Stat. 2010, 2, 433–470. [Google Scholar]
- Sadia, M.; Isreb, A.; Abbadi, I.; Isreb, M.; Aziz, D.; Selo, A.; Timmins, P.; Alhnan, M.A. From ‘fixed dose combinations’ to ‘a dynamic dose combiner’: 3D printed bi-layer antihypertensive tablets. Eur. J. Pharm. Sci. 2018, 123, 484–494. [Google Scholar] [CrossRef]
- Tian, Y.; Booth, J.; Meehan, E.; Jones, D.S.; Li, S.; Andrews, G.P. Construction of drug-polymer thermodynamic phase diagrams using flory-huggins interaction theory: Identifying the relevance of temperature and drug weight fraction to phase separation within solid dispersions. Mol. Pharm. 2013, 10, 236–248. [Google Scholar] [CrossRef]
- Rowe, R.; Sheskey, P.; Quinn, M. Handbook of Pharmaceutical Excipients, 6th ed.; Pharmaceutical Press: London, UK, 2009. [Google Scholar]

| Nozzle Temperature (°C) | Platform Temperature (°C) | Printing Speed (mm/s) |
|---|---|---|
| 100 | 30 | 30 |
| 100 | 30 | 90 |
| 100 | 30 | 160 |
| 110 | 30 | 30 |
| 110 | 30 | 90 |
| 110 | 30 | 160 |
| 120 | 30 | 30 |
| 120 | 30 | 90 |
| 120 | 30 | 160 |
| 100 | 45 | 30 |
| 100 | 45 | 90 |
| 100 | 45 | 160 |
| Operator I | ||||
| n | Thickness (mm) | Length (mm) | Width (mm) | Weight (mg) |
| 1 | 1.09 | 9.92 | 10.01 | 110.6 |
| 2 | 1.04 | 10.03 | 10.10 | 114.6 |
| 3 | 1.07 | 10.00 | 10.16 | 113.4 |
| 4 | 1.05 | 10.00 | 10.09 | 111.0 |
| 5 | 1.10 | 9.91 | 10.02 | 109.8 |
| 6 | 1.05 | 9.99 | 10.20 | 111.0 |
| RSD | 2.25% | 0.48% | 0.74% | 1.65% |
| Operator II | ||||
| n | Thickness (mm) | Length (mm) | Width (mm) | Weight (mg) |
| 1 | 1.03 | 10.05 | 10.16 | 107.7 |
| 2 | 1.08 | 10.05 | 10.23 | 112.6 |
| 3 | 1.04 | 10.06 | 10.13 | 111.1 |
| 4 | 1.06 | 10.05 | 10.20 | 112.5 |
| 5 | 1.04 | 9.96 | 10.18 | 108.7 |
| 6 | 1.06 | 9.94 | 10.10 | 110.1 |
| RSD | 1.71% | 0.53% | 0.46% | 1.8% |
| Independent Sample T-Test | ||||
| p-Value (Length) | p-Value (Width) | p-Value (Thickness) | p-Value (Weight) | |
| 0.223 | 0.101 | 0.127 | 0.137 | |
| Day 1 | ||||
| n | Thickness (mm) | Length (mm) | Width (mm) | Weight (mg) |
| 1 | 1.09 | 9.92 | 10.01 | 110.6 |
| 2 | 1.04 | 10.03 | 10.10 | 114.6 |
| 3 | 1.07 | 10.00 | 10.16 | 113.4 |
| 4 | 1.05 | 10.00 | 10.09 | 111.0 |
| 5 | 1.10 | 9.91 | 10.02 | 109.8 |
| 6 | 1.05 | 9.99 | 10.20 | 111.0 |
| RSD | 2.25% | 0.48% | 0.74% | 1.65% |
| Day 2 | ||||
| n | Thickness (mm) | Length (mm) | Width (mm) | Weight (mg) |
| 1 | 1.02 | 10.08 | 10.11 | 108.7 |
| 2 | 1.04 | 10.09 | 10.12 | 108.4 |
| 3 | 1.07 | 10.03 | 10.14 | 105.2 |
| 4 | 1.03 | 10.06 | 10.11 | 107.9 |
| 5 | 1.02 | 10.03 | 10.08 | 104.5 |
| 6 | 1.04 | 10.06 | 10.14 | 109.7 |
| RSD | 1.83% | 0.25% | 0.23% | 1.93% |
| Independent Sample T-Test | ||||
| p-Value (Length) | p-Value (Width) | p-Value (Thickness) | p-Value (Weight) | |
| 0.004 | 0.213 | 0.04 | 0.006 | |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Alhijjaj, M.; Nasereddin, J.; Belton, P.; Qi, S. Impact of Processing Parameters on the Quality of Pharmaceutical Solid Dosage Forms Produced by Fused Deposition Modeling (FDM). Pharmaceutics 2019, 11, 633. https://doi.org/10.3390/pharmaceutics11120633
Alhijjaj M, Nasereddin J, Belton P, Qi S. Impact of Processing Parameters on the Quality of Pharmaceutical Solid Dosage Forms Produced by Fused Deposition Modeling (FDM). Pharmaceutics. 2019; 11(12):633. https://doi.org/10.3390/pharmaceutics11120633
Chicago/Turabian StyleAlhijjaj, Muqdad, Jehad Nasereddin, Peter Belton, and Sheng Qi. 2019. "Impact of Processing Parameters on the Quality of Pharmaceutical Solid Dosage Forms Produced by Fused Deposition Modeling (FDM)" Pharmaceutics 11, no. 12: 633. https://doi.org/10.3390/pharmaceutics11120633
APA StyleAlhijjaj, M., Nasereddin, J., Belton, P., & Qi, S. (2019). Impact of Processing Parameters on the Quality of Pharmaceutical Solid Dosage Forms Produced by Fused Deposition Modeling (FDM). Pharmaceutics, 11(12), 633. https://doi.org/10.3390/pharmaceutics11120633