Determination of the Dynamic Modulus of Elasticity of Pine Based on the PZT Transducer
Abstract
1. Introduction
2. Materials and Methods
2.1. Materials
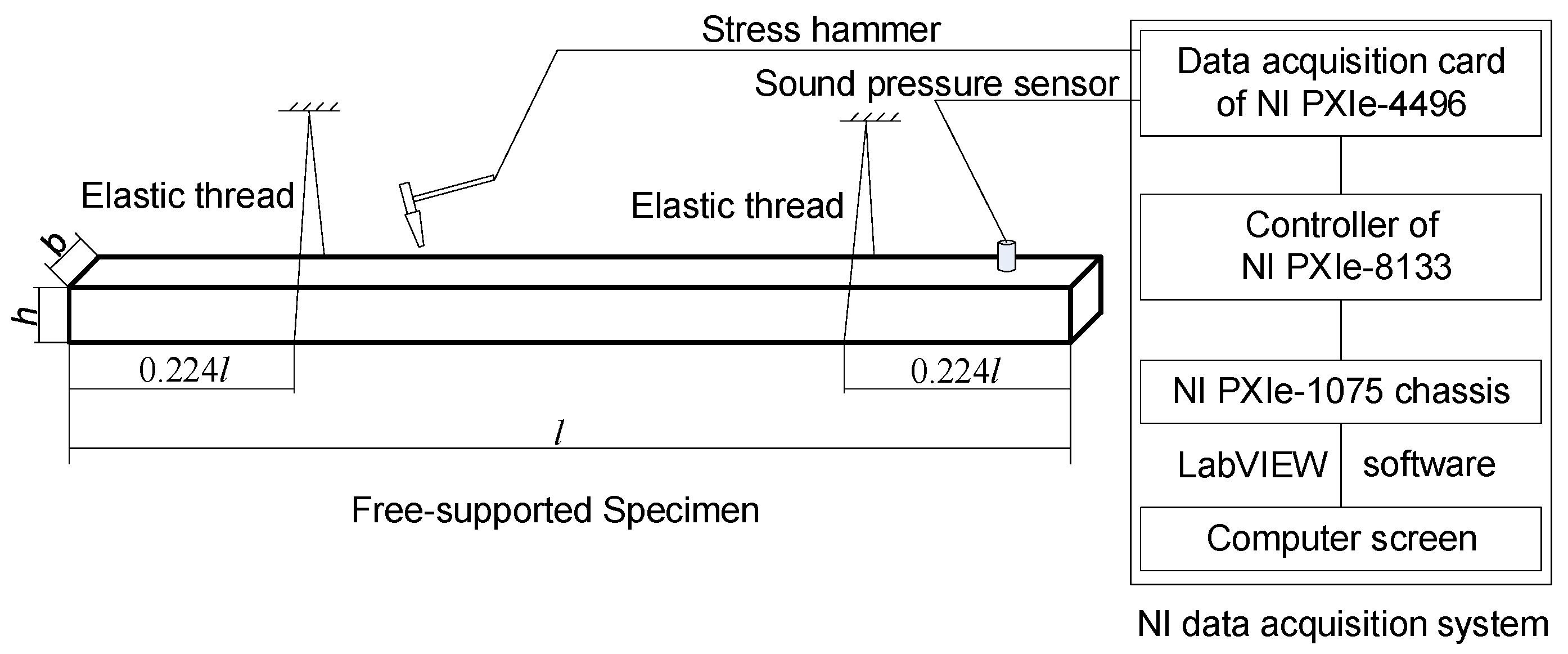
2.2. Ed Calculation of the Transverse Vibration Method
2.3. Theoretical Basis of the EMI Method
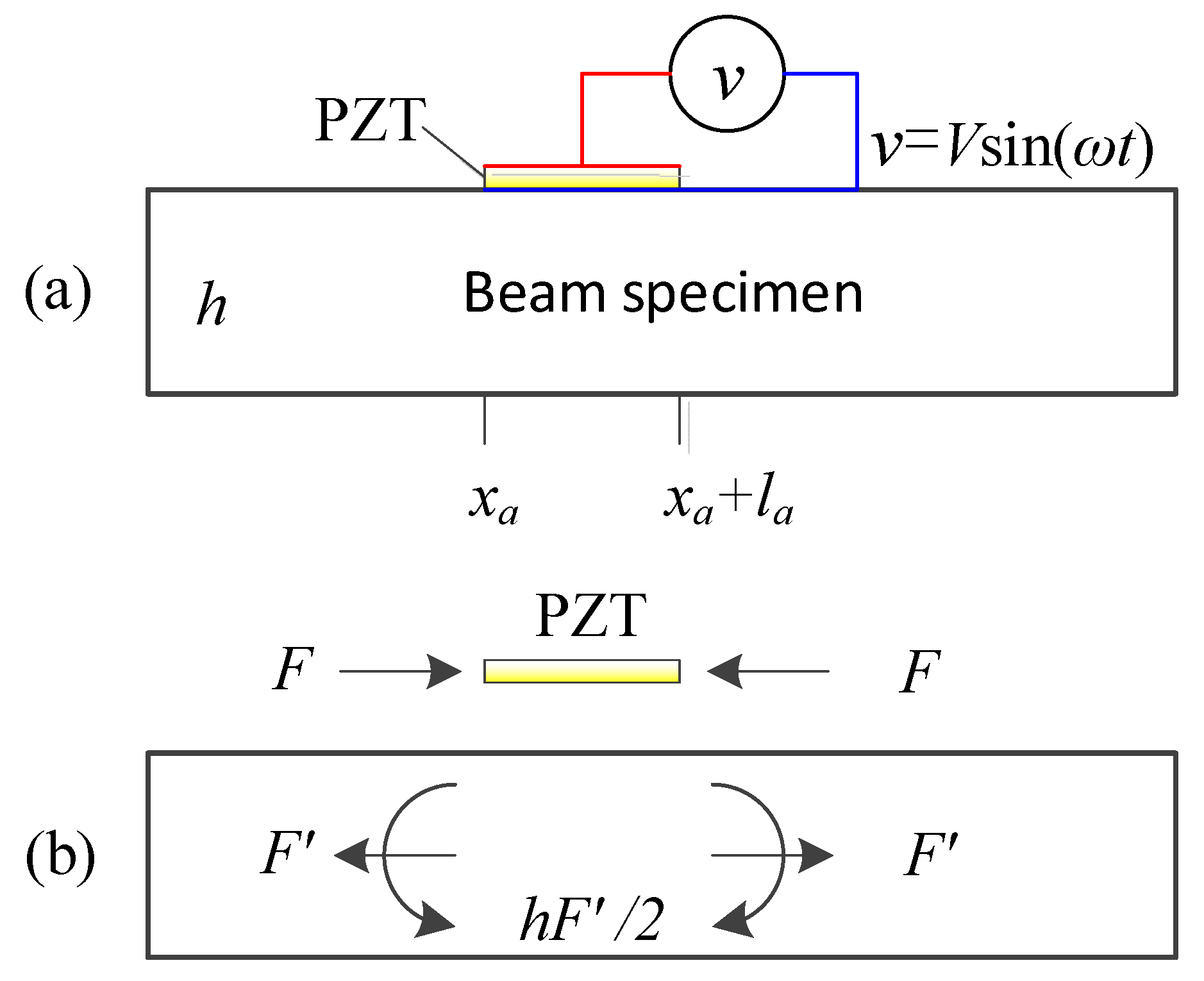
2.4. Ed Detection Principle Based on the EMI Method
3. Results and Discussion
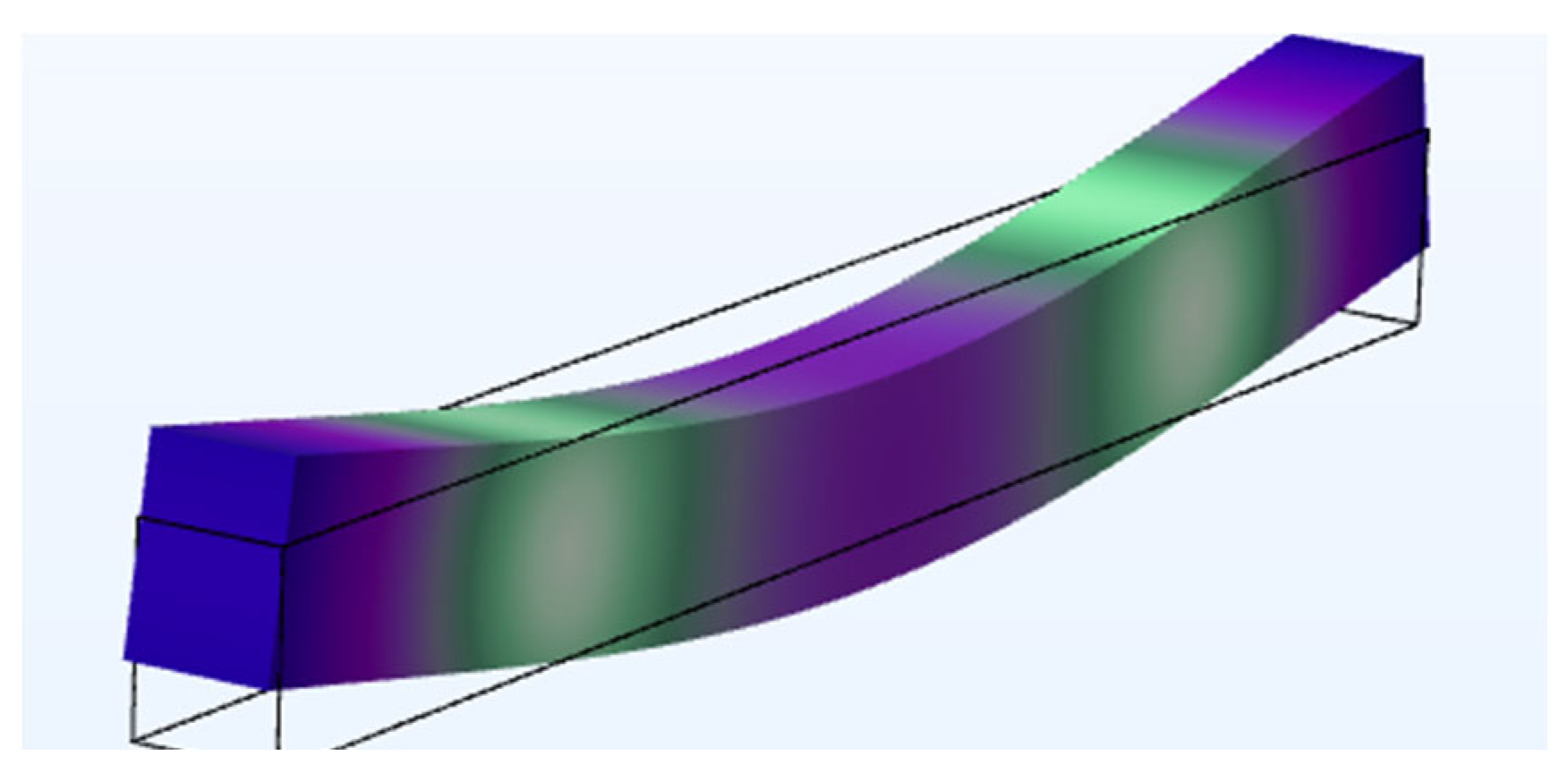
3.1. Size Optimization of the Specimen
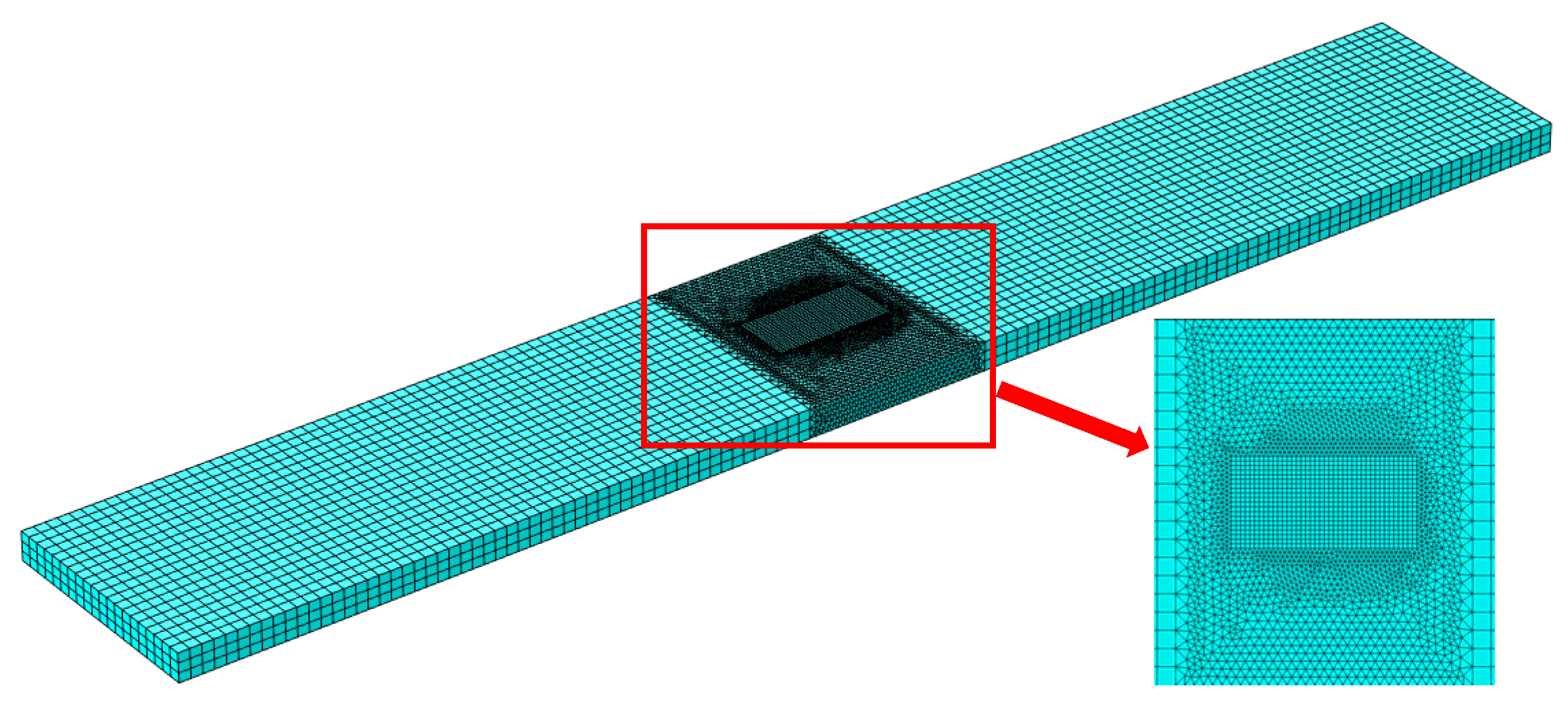
3.2. Verification of the Validity of the Proposed Method Based on the EMI Simulation
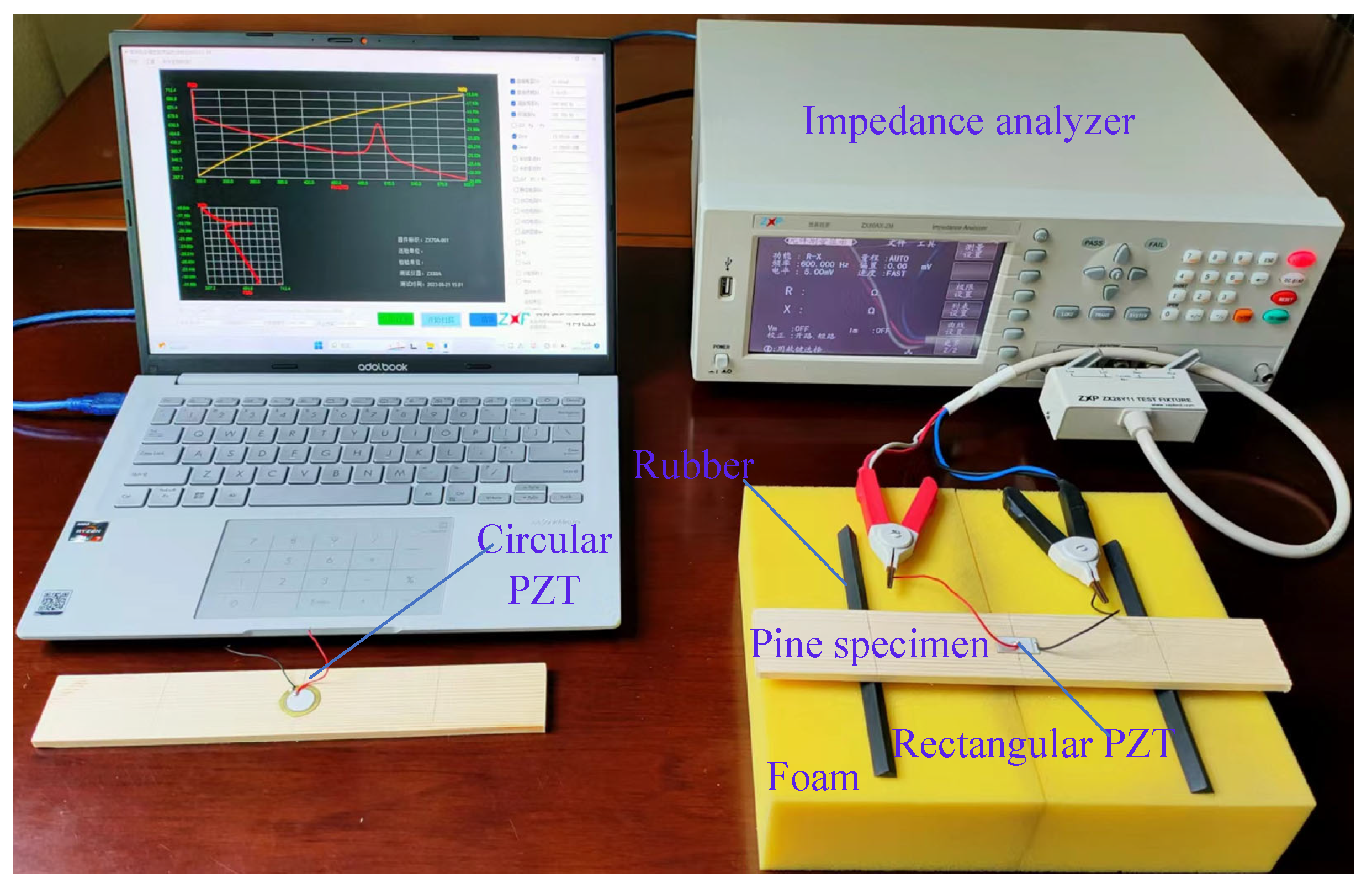
3.3. Validation of the Effectiveness of the Proposed Method Based on the Test
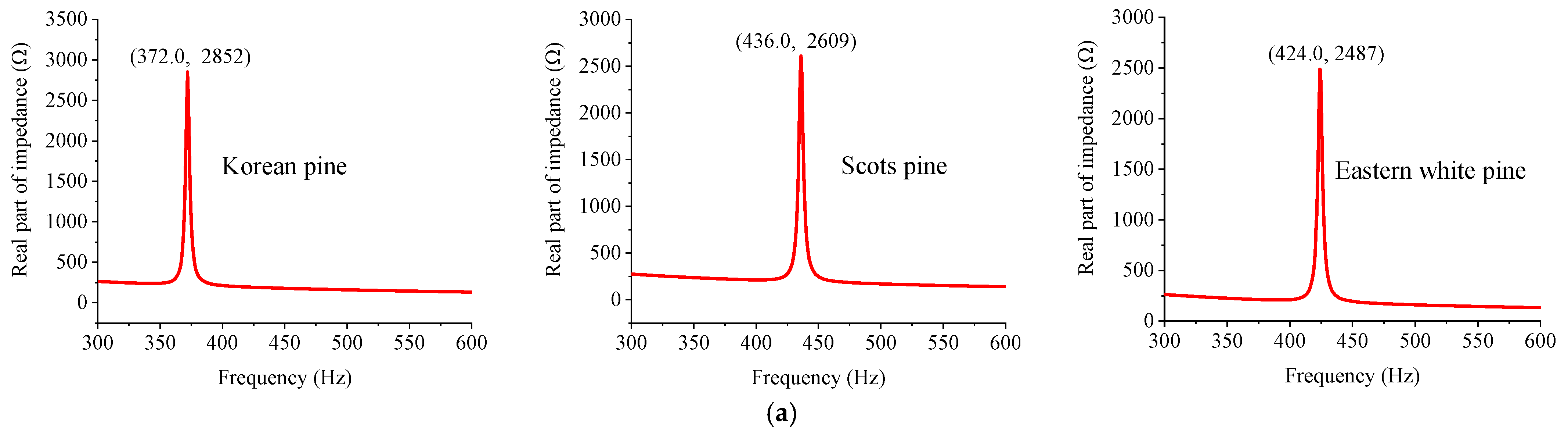
3.3.1. Elastic Modulus Determination by the EMI Method
3.3.2. Verification of the Effectiveness of the EMI Method
4. Conclusions
- The results of the modal simulation on the transverse vibration method indicate that the detection accuracy of the dynamic modulus of elasticity is higher when the length to thickness ratio of the pine specimen is larger. When the length to thickness ratio of the pine specimen reaches about 50, the detection accuracy meets the actual demand, with which the size of the pine specimen was optimized for impedance measurement.
- The scanning frequency range of the EMI detection is determined to be 300–600 Hz based on the mode frequencies of three kinds of pine specimens, which nearly cover the first-order bending mode frequencies of all the pine specimens and cannot reach other vibration modes of the pine specimen.
- The EMI simulation results illustrate that a unique and significant formant appears in the real part of each EMI response curve, and the maximum relative errors using the rectangular PZT patch and the circular PZT patch are 1.34% and 1.81%, respectively, which verifies the feasibility and validity of the proposed method.
- The EMI test results indicate that the maximum relative errors using the rectangular PZT patch and the commercial buzzer are 1.41% and 1.68%, respectively, compared with the corresponding results obtained using the traditional transverse vibration method, which verifies the effectiveness and practicality of the EMI method.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Kharrat, W.; Koubaa, A.; Khlif, M.; Bradai, C. Intra-ring Wood Density and Dynamic Modulus of Elasticity Profiles for Black Spruce and Jack Pine from X-ray Densitometry and Ultrasonic Wave Velocity Measurement. Forests 2019, 10, 569. [Google Scholar] [CrossRef]
- Hearmon, R.F.S. The Elasticity of Wood and Plywood; Forest Products Research, Special Report, No. 7; H.M. Stationery Office: London, UK, 1948. [Google Scholar]
- Radu, A.; Brenndörfer, D. Zur zerstörungsfreien Prüfung des Holzes durch Schwingungsversuche. Holz Roh Werkst. 1976, 34, 219–222. [Google Scholar] [CrossRef]
- Haines, D.W.; Leban, J.-M.; Herbé, C. Determination of Young’s Modulus for Spruce, Fir and Isotropic Materials by the Resonance Flexure Method with Comparisons to Static Fexure and Other Dynamic Methods. Wood Sci. Technol. 1996, 30, 253–263. [Google Scholar] [CrossRef]
- Yin, Y.; Lu, J.; Ni, C.; Ren, H. Evaluation of Bending, Tensile and Compressive Strength of Structural Lumber with Transverse Vibration Technique. J. Build. Mater. 2007, 10, 543–547. [Google Scholar]
- Wang, S.Y.; Chen, J.H.; Tsai, M.J.; Lin, C.J.; Yang, T.H. Grading of Softwood Lumber Using Non-destructive Techniques. J. Mater. Process. Technol. 2008, 208, 149–158. [Google Scholar] [CrossRef]
- ASTM D6874; Standard Test Methods for Nondestructive Evaluation of Wood-Based Flexural Members Using Transverse Vibration. American Society for Testing and Materials: West Conshohocken, PA, USA, 2012. [CrossRef]
- Li, W.; Liu, T.; Zou, D.; Wang, J.; Yi, T.H. PZT Based Smart Corrosion Coupon Using Electromechanical Impedance. Mech. Syst. Sig. Process. 2019, 129, 455–469. [Google Scholar] [CrossRef]
- Ritdumrongkul, S.; Fujino, Y. Identification of the Location and Size of Cracks in Beams by a Piezoceramic Actuator–sensor. Struct. Control Health Monit. 2007, 14, 931–943. [Google Scholar] [CrossRef]
- Ai, D.; Zhu, H.; Luo, H.; Wang, C. Mechanical Impedance Based Embedded Piezoelectric Transducer for Reinforced Concrete Structural Impact Damage Detection: A Comparative Study. Constr. Build. Mater. 2018, 165, 472–483. [Google Scholar] [CrossRef]
- Fan, X.; Li, J. Damage Identification in Plate Structures Using Sparse Regularization Based Electromechanical Impedance Technique. Sensors 2020, 20, 7069. [Google Scholar] [CrossRef] [PubMed]
- Huo, L.; Chen, D.; Liang, Y.; Li, H.; Feng, X.; Song, G. Impedance Based Bolt Pre-Load Monitoring Using Piezoceramic Smart Washer. Smart Mater. Struct. 2017, 26, 057004. [Google Scholar] [CrossRef]
- Du, F.; Wu, S.; Xu, C.; Yang, Z.; Su, Z. Electromechanical Impedance Temperature Compensation and Bolt Loosening Monitoring Based on Modified Unet and Multitask Learning. IEEE Sens. J. 2023, 23, 4556–4567. [Google Scholar] [CrossRef]
- Ferreira, F.I.; de Aguiar, P.R.; da Silva, R.B.; Jackson, M.J.; Baptista, F.G.; Bianchi, E.C. Monitoring of Cylindrical Plunge Grinding Process by Electromechanical Impedance. IEEE Sens. J. 2022, 22, 12314–12322. [Google Scholar] [CrossRef]
- Marchi, M.; Baptista, F.G.; de Aguiar, P.R.; Bianchi, E.C. Grinding Process Monitoring Based on Electromechanical Impedance Measurements. Meas. Sci. Technol. 2015, 26, 045601. [Google Scholar] [CrossRef]
- Çakir, F.H.; Er, Ü.; Tekkalmaz, M. Monitoring the Wear of Turning Tools with the Electromechanical Impedance Technique. J. Intell. Mater. Syst. Struct. 2023, 34, 1341–1352. [Google Scholar] [CrossRef]
- Wang, J.; Li, W.; Lan, C.; Wei, P.; Luo, W. Electromechanical Impedance Instrumented Piezoelectric Ring for Pipe Corrosion and Bearing Wear Monitoring: A Proof-of-concept Study. Sens. Actuators A Phys. 2020, 315, 112276. [Google Scholar] [CrossRef]
- Wu, J.; Yang, G.; Wang, X.; Li, W. PZT-Based Soil Compactness Measuring Sheet Using Electromechanical Impedance. IEEE Sens. J. 2020, 20, 10240–10250. [Google Scholar] [CrossRef]
- Zhang, J.; Zhang, C.; Xiao, J.; Jiang, J. A PZT-Based Electromechanical Impedance Method for Monitoring the Soil Freeze-Thaw Process. Sensors 2019, 19, 1107. [Google Scholar] [CrossRef]
- Zhu, X.; di Scalea, F.L. Sensitivity to Axial Stress of Electro-Mechanical Impedance Measurements. Exp. Mech. 2016, 56, 1599–1610. [Google Scholar] [CrossRef]
- Ong, C.-W.; Yang, Y.; Naidu, A.; Lu, Y.; Soh, C. Application of the Electromechanical Impedance Method for the Identification of In-situ Stress in Structures. Proc. SPIE 2002, 4935, 503–514. [Google Scholar]
- Carbajo, J.; Poveda, P.; Segovia, E.; Rincon, E.; Ramis, J. Determination of Dynamic Elastic Modulus of Materials Under a State of Simple Stresses by Using Electrodynamic Actuators in Beam-Type Mechanical Elements. Mater. Lett. 2022, 320, 132383. [Google Scholar] [CrossRef]
- Ferreira, D.; Fonseca, E.; Pinto, C.; Borges, P. Tensile Strength of pine and Ash Woods–Experimental and Numerical Study. In Proceedings of the 6th International Conference on Mechanics and Materials in Design (M2D), Ponta Delgada, Portugal, 26–30 July 2015. [Google Scholar]
- Fajdiga, G.; Rajh, D.; Necemer, B.; Glodez, S.; Sraml, M. Experimental and Numerical Determination of the Mechanical Properties of Spruce Wood. Forests 2019, 10, 1140. [Google Scholar] [CrossRef]
- Carreira, M.R.; Dias, A.A.; Segundinho, P.G.D. Nondestructive Evaluation of Corymbia Citriodora Logs by Means of the Free Transverse Vibration Test. J. Nondestruct. Eval. 2017, 36, 26. [Google Scholar] [CrossRef]
- Giurgiutiu, V.; Zagrai, A.N. Embedded Self-sensing Piezoelectric Active Sensors for On-line Structural Identification. J. Vib. Acoust. 2002, 124, 116–125. [Google Scholar] [CrossRef]
- Kouroussis, G.; Ben Fekih, L.; Descamps, T. Assessment of Timber Element Mechanical Properties Using Experimental Modal Analysis. Constr. Build. Mater. 2017, 134, 254–261. [Google Scholar] [CrossRef]
- Chui, Y.H.; Smith, I. Influence of Rotatory Inertia, Shear Deformation and Support Condition on Natural Frequencies of Wooden Beams. Wood Sci. Technol. 1990, 24, 233–245. [Google Scholar] [CrossRef]
- Yang, N.; Zhang, L. Investigation of Elastic Constants and Ultimate Strengths of Korean Pine from Compression and Tension Tests. J. Wood Sci. 2018, 64, 85–96. [Google Scholar] [CrossRef]
- Pencik, J. Modelling of Experimental Tests of Wooden Specimens from Scots Pine (Pinus sylvestris) with the Help of Anisotropic Plasticity Material Model. Drvna Ind. 2015, 66, 27–33. [Google Scholar] [CrossRef]
- Shahhosseini, S.; Crovella, P.; Smith, W.B. Comparing The Effect of Presence of the Knot and the Size of the Knot on the Rolling Shear Properties in Cross Laminated Timber (CLT) by Modified Planar Shear Test and FEM Analysis. In Proceedings of the 16th World Conference on Timber Engineering (WCTE), Santiago, Chile, 9–12 August 2021. [Google Scholar]
- Merhar, M. Determination of Elastic Properties of Beech Plywood by Analytical, Experimental and Numerical Methods. Forests 2020, 11, 1221. [Google Scholar] [CrossRef]
- Wang, L.; Yuan, B.; Xu, Z.; Sun, Q. Synchronous Detection of Bolts Looseness Position and Degree Based on Fusing Electro-mechanical Impedance. Mech. Syst. Sig. Process. 2022, 174, 109068. [Google Scholar] [CrossRef]
- Kubojima, Y.; Tonosaki, M.; Yoshihara, H. Effect of Additional Mass on the Young’s Modulus of a Wooden Beam. J. Test Eval. 2005, 33, 278–282. [Google Scholar] [CrossRef]
- Islam, M.; Huang, H. Understanding the effects of adhesive layer on the Electromechanical Impedance (EMI) of Bonded Piezoelectric Wafer Transducer. Smart Mater. Struct. 2014, 23, 125037. [Google Scholar] [CrossRef]
- Abbas, S.; Li, F.; Abbas, Z.; Abbasi, T.U.R.; Tu, X.; Pasha, R.A. Experimental study of effect of temperature variations on the impedance signature of PZT sensors for fatigue crack detection. Sound Vib. 2021, 55, 1–18. [Google Scholar] [CrossRef]
- Wang, J.; Li, W.; Lan, C.; Wei, P. Effective Determination of Young’s Modulus and Poisson’s Ratio of Metal Using Piezoelectric Ring and Electromechanical Impedance Technique: A Proof-of-concept Study. Sens. Actuators A Phys. 2021, 319, 112561. [Google Scholar] [CrossRef]



| Property | Korean Pine | Scots Pine | Eastern White Pine |
|---|---|---|---|
| ρ (Kg/m3) | 430 | 505 | 349 |
| EL (MPa) | 8856 | 14,300 | 9404 |
| ER (MPa) | 986 | 700 | 734 |
| ET (MPa) | 429 | 545 | 357 |
| GLR (MPa) | 499 | 1230 | 490 |
| GRT (MPa) | 42 | 500 | 47 |
| GLT (MPa) | 450 | 800 | 451 |
| νLR | 0.43 | 0.30 | 0.30 |
| νRT | 0.65 | 0.38 | 0.40 |
| νLT | 0.51 | 0.40 | 0.30 |
| No | Size (mm) | LTR | f1 (Hz) | ELd (MPa) | Relative Error |
|---|---|---|---|---|---|
| 1 | 800 × 40 × 80 | 10 | 516.7 | 6953 | 21.49% |
| 2 | 800 × 40 × 40 | 20 | 281.9 | 8279 | 6.52% |
| 3 | 800 × 40 × 25 | 32 | 179.8 | 8622 | 2.64% |
| 4 | 800 × 40 × 20 | 40 | 144.5 | 8701 | 1.75% |
| 5 | 800 × 40 × 16 | 50 | 116.0 | 8762 | 1.06% |
| No | Size (mm) | LTR | f1 (Hz) | ELd (MPa) | Relative Error |
|---|---|---|---|---|---|
| 1 | 800 × 40 × 80 | 10 | 610.9 | 11,415 | 20.17% |
| 2 | 800 × 40 × 40 | 20 | 331.4 | 13,437 | 6.03% |
| 3 | 800 × 40 × 25 | 32 | 211.0 | 13,945 | 2.48% |
| 4 | 800 × 40 × 20 | 40 | 169.6 | 14,077 | 1.56% |
| 5 | 800 × 40 × 16 | 50 | 136.1 | 14,165 | 0.94% |
| Species of Pine | Korean Pine | Scots Pine | Eastern White Pine |
|---|---|---|---|
| The first-order bending mode frequency f1 (Hz) | 371.3 | 435.4 | 424.4 |
| ELd (MPa) | 8766 | 14,157 | 9296 |
| Relative error | 1.02% | 1.00% | 1.15% |
| Species of Pine | Korean Pine | Scots Pine | Eastern White Pine |
|---|---|---|---|
| The first-order bending mode frequency (Hz) | 371.3 | 435.4 | 424.4 |
| The second-order bending mode frequency (Hz) | 1010.2 | 1185.7 | 1153.5 |
| The first-order longitudinal mode frequency (Hz) | 9053.4 | 10,622.0 | 10,367.0 |
| Parameters | Values |
|---|---|
| 3130/3130/3400 | |
| Piezoelectric strain coefficients (10−10 m/V) | −2.74/−2.74/5.93/7.41/7.41 |
| Compliance S11/S12/S13/S22/S23/S33/S44/S55/S66 (10−12 m2/N) | 16.50/−4.78/−8.45/16.50/−8.45/20.70/43.50/43.50/42.60 |
| Density ρ (kg/m3) | 7500 |
| Dielectric loss factor tanδ | 0.02 |
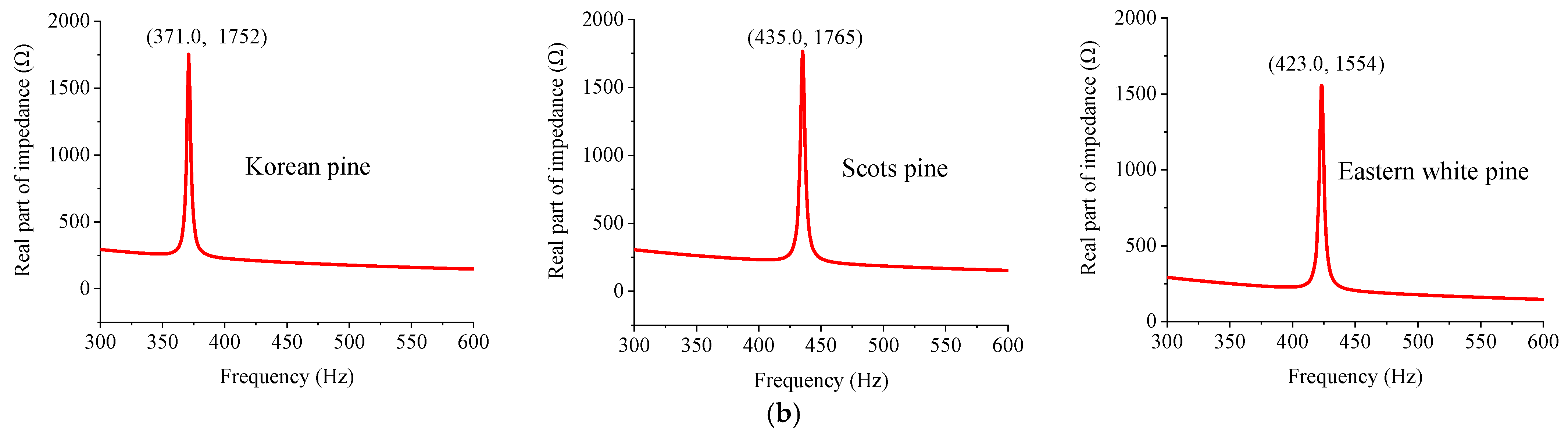
| Species of Pine | PZT Shape | f1 (Hz) | ELd (MPa) | EL (MPa) | Relative Error |
|---|---|---|---|---|---|
| Korean pine | Rectangular | 372.0 | 8799 | 8856 | 0.64% |
| Circular | 371.0 | 8752 | 1.17% | ||
| Scots pine | Rectangular | 436.0 | 14,196 | 14,300 | 0.73% |
| Circular | 435.0 | 14,131 | 1.18% | ||
| Eastern white pine | Rectangular | 424.0 | 9278 | 9404 | 1.34% |
| Circular | 423.0 | 9234 | 1.81% |
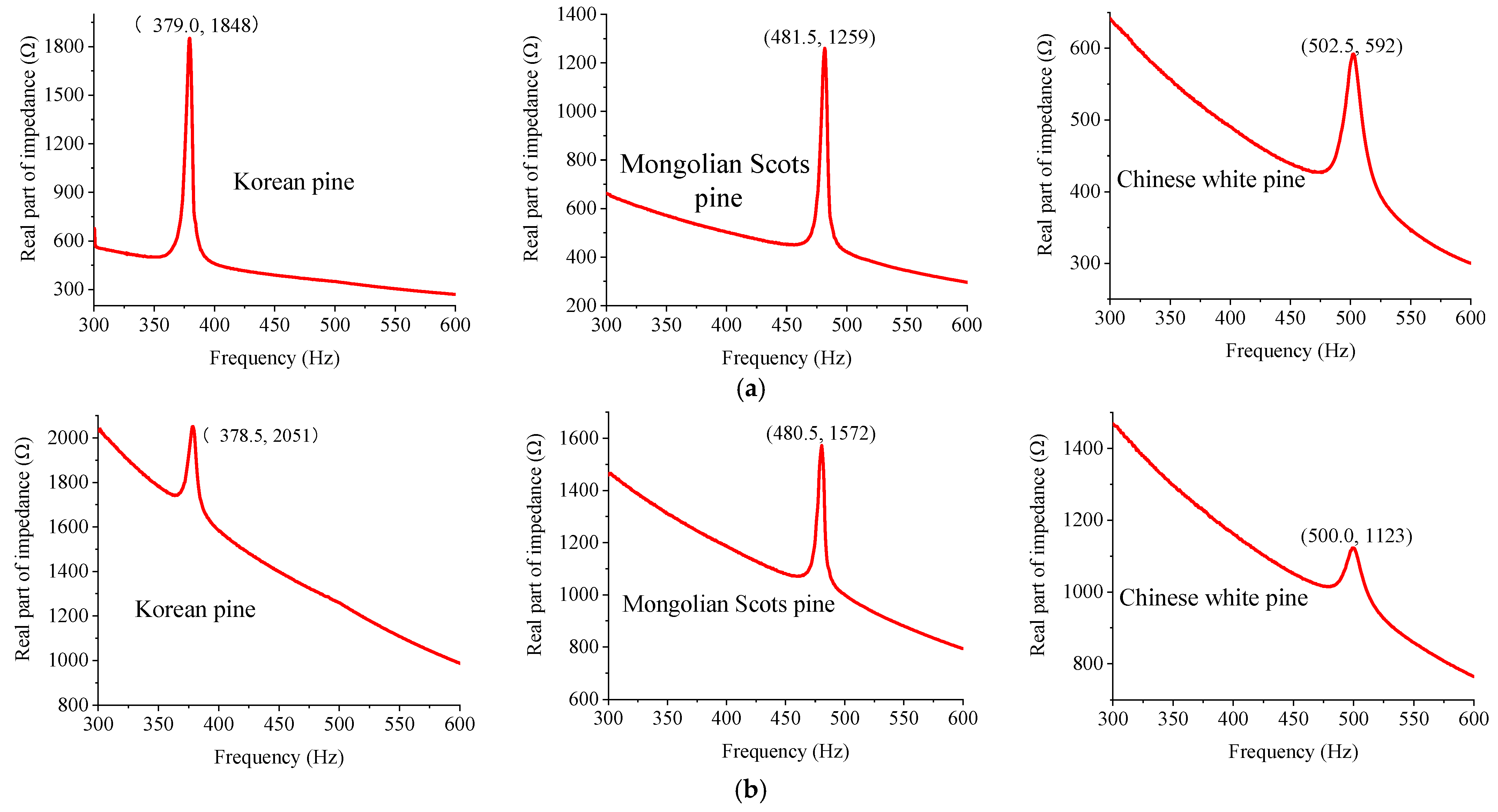
| Species of Pine | Size (mm) | Density (kg/m3) | PZT Shape | f1 (Hz) | ELd (MPa) |
|---|---|---|---|---|---|
| Korean pine | 250 × 40 × 5.30 | 454 | Rectangular | 379.0 | 8583 |
| Circular | 378.5 | 8560 | |||
| Mongolian Scots pine | 250 × 40 × 5.58 | 483 | Rectangular | 481.5 | 13,296 |
| Circular | 480.5 | 13,240 | |||
| Chinese white pine | 250 × 40 × 5.40 | 432 | Rectangular | 502.5 | 13,829 |
| Circular | 500.0 | 13,692 |
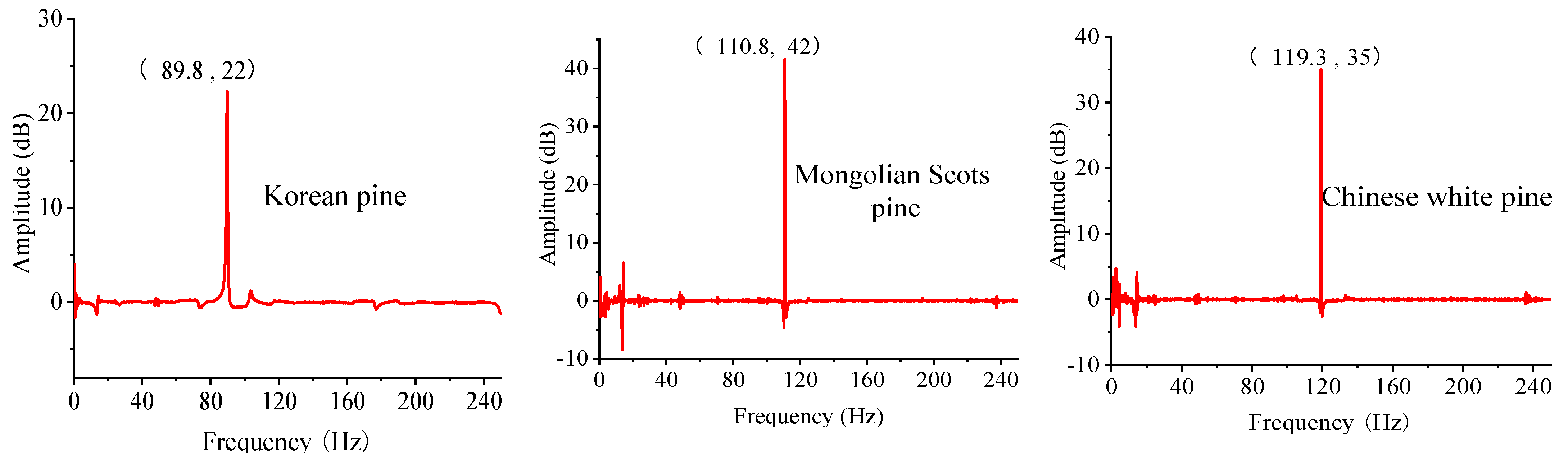
| Species of Pine | Size (mm) | Density (kg/m3) | f1 (Hz) | EL (MPa) |
|---|---|---|---|---|
| Korean pine | 1000 × 40 × 19.95 | 454 | 89.8 | 8706 |
| Mongolian Scots pine | 1000 × 40 × 20.42 | 483 | 110.8 | 13,458 |
| Chinese white pine | 1000 × 40 × 20.50 | 432 | 119.3 | 13,846 |
| Species of Pine | Results Using the Proposed Method | Results Using the Traditional Transverse Vibration Method | Relative Error | |
|---|---|---|---|---|
| PZT Shape | ELd (MPa) | EL (MPa) | ||
| Korean pine | Rectangular | 8583 | 8706 | 1.41% |
| Circular | 8560 | 1.68% | ||
| Mongolian Scots pine | Rectangular | 13,296 | 13,458 | 1.20% |
| Circular | 13,240 | 1.62% | ||
| Chinese white pine | Rectangular | 13,829 | 13,846 | 0.12% |
| Circular | 13,692 | 1.11% | ||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, S.; Xu, G.; Jiang, C.; Hu, H. Determination of the Dynamic Modulus of Elasticity of Pine Based on the PZT Transducer. Forests 2024, 15, 459. https://doi.org/10.3390/f15030459
Li S, Xu G, Jiang C, Hu H. Determination of the Dynamic Modulus of Elasticity of Pine Based on the PZT Transducer. Forests. 2024; 15(3):459. https://doi.org/10.3390/f15030459
Chicago/Turabian StyleLi, Shaocheng, Guangzhou Xu, Chenkan Jiang, and Hailong Hu. 2024. "Determination of the Dynamic Modulus of Elasticity of Pine Based on the PZT Transducer" Forests 15, no. 3: 459. https://doi.org/10.3390/f15030459
APA StyleLi, S., Xu, G., Jiang, C., & Hu, H. (2024). Determination of the Dynamic Modulus of Elasticity of Pine Based on the PZT Transducer. Forests, 15(3), 459. https://doi.org/10.3390/f15030459








