A Hybrid Model for Analysis of Laser Beam Distortions Using Monte Carlo and Shack–Hartmann Techniques: Numerical Study and Experimental Results
Abstract
1. Introduction
2. Materials and Methods
2.1. Radiative Transfer Equation
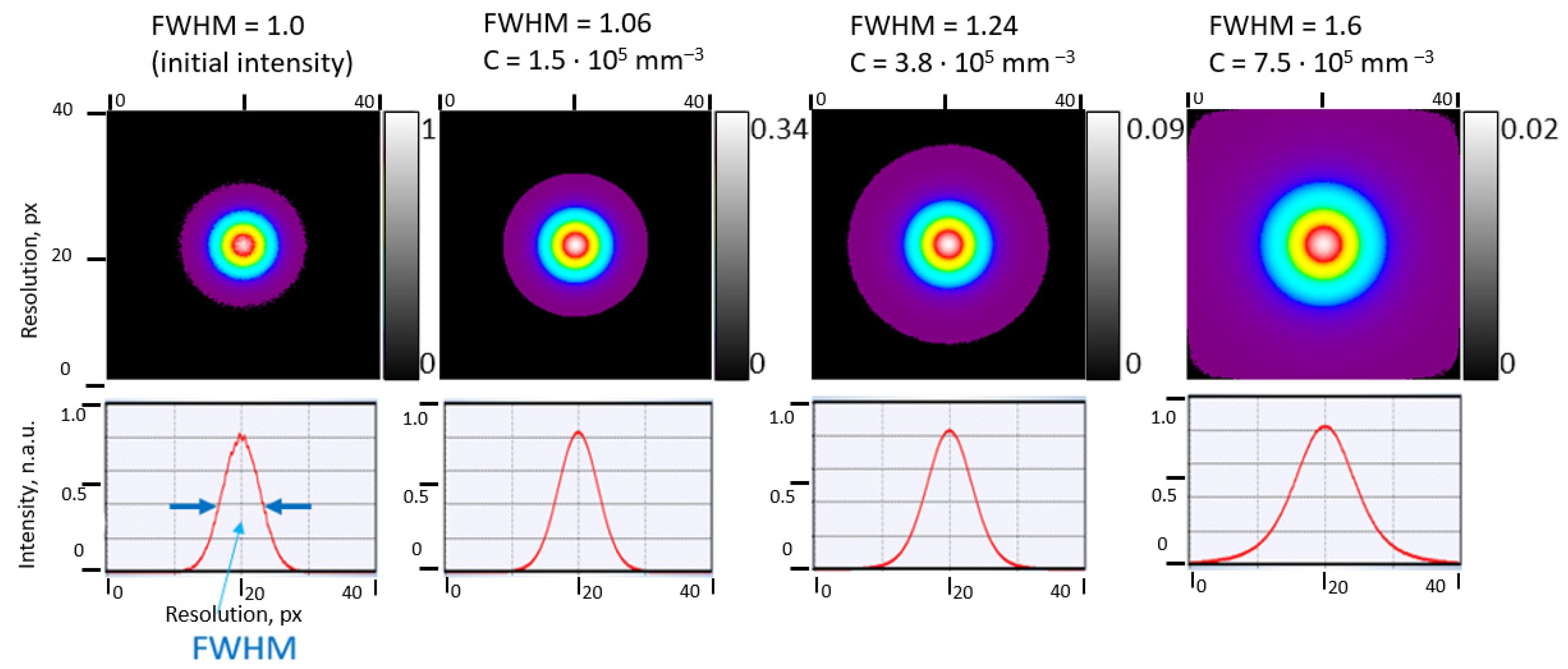
2.2. Monte Carlo Simulation
- Number of photons transmitted through and reflected from the medium.
- Distributions of photons per scattering order (number of photons undergone 1, 2, … etc. scattering events).
- Intensity distributions at the exit edge of the medium (in Table 1, we presented percentage of photons located within specified regions).
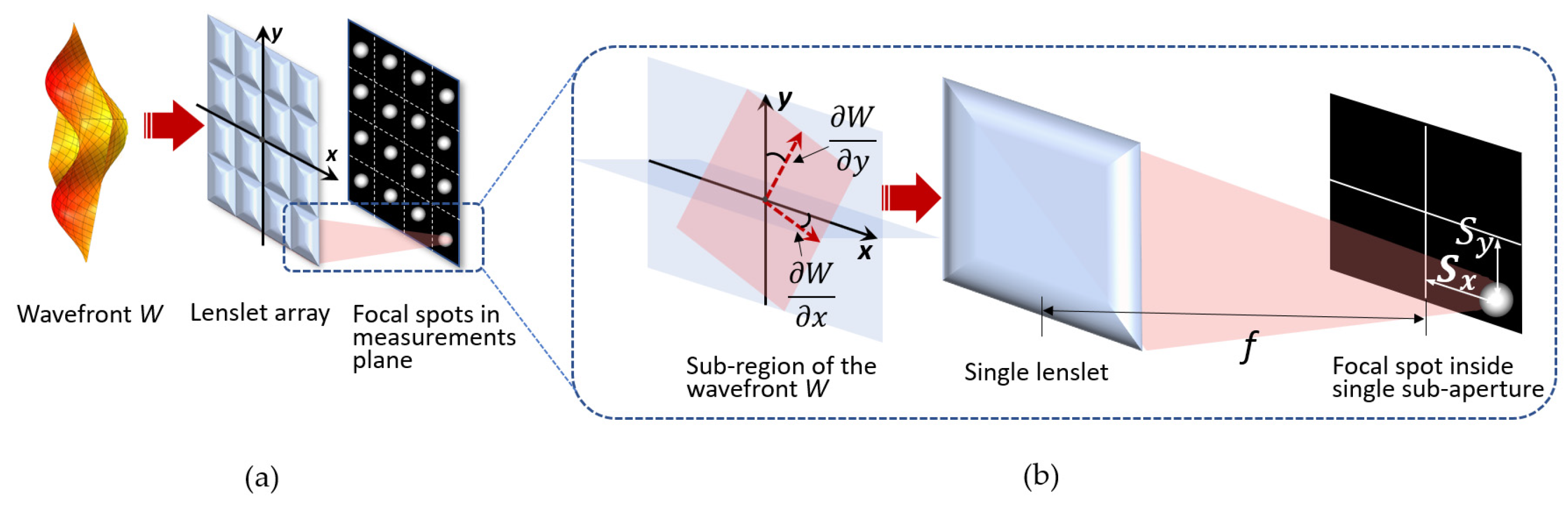
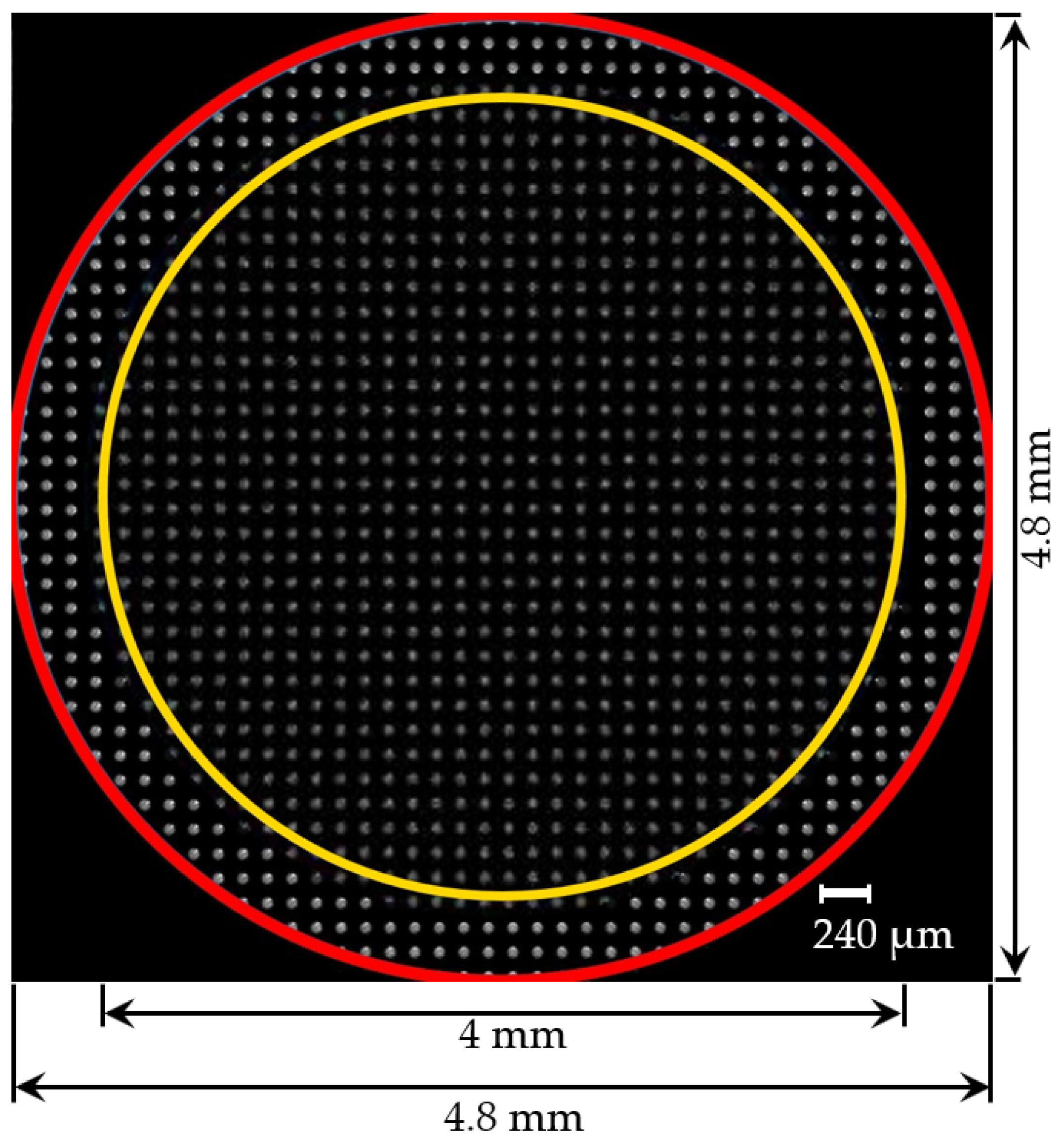
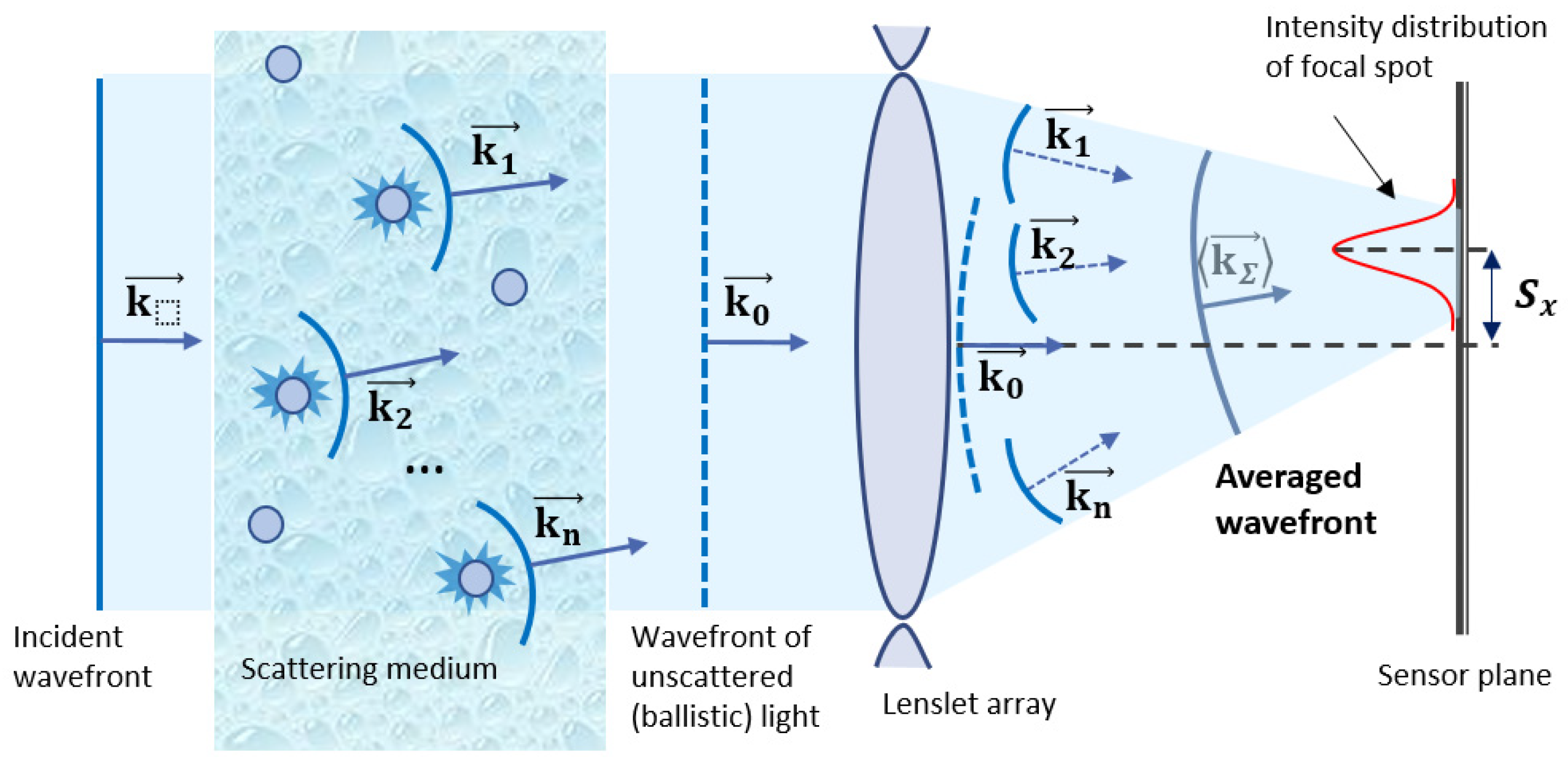
2.3. Shack–Hartmann Technique
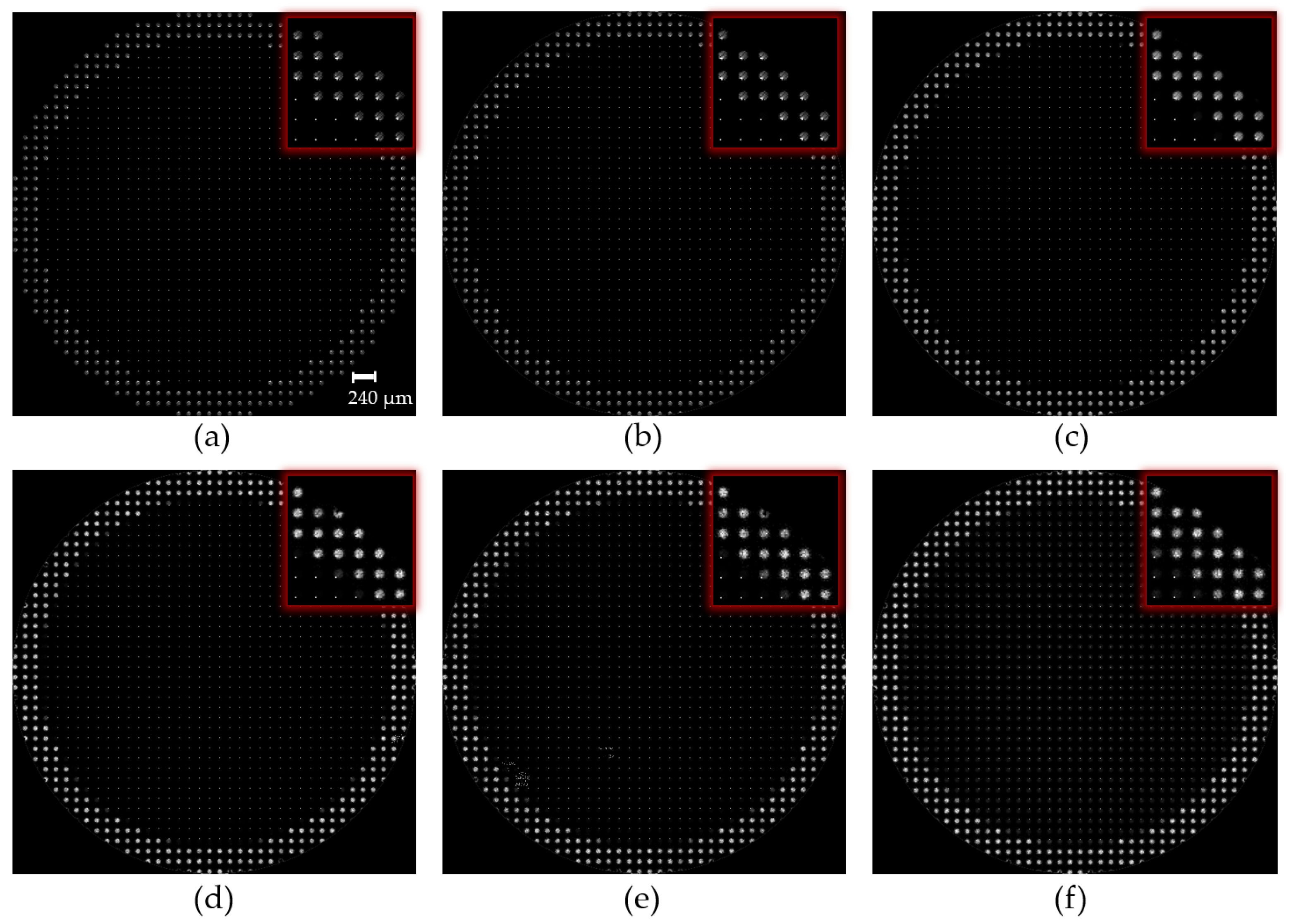
2.4. Hybrid Model Implementation
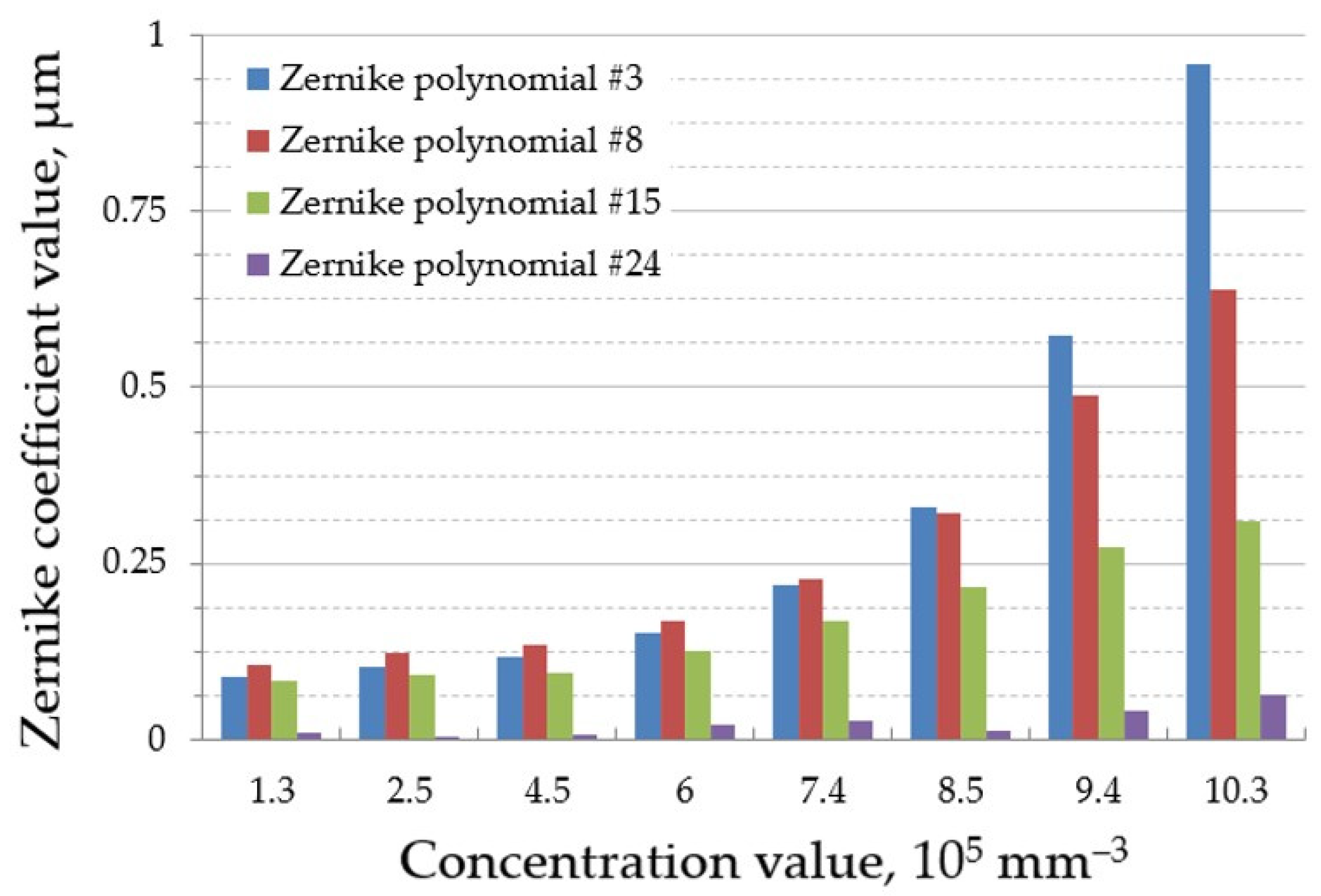
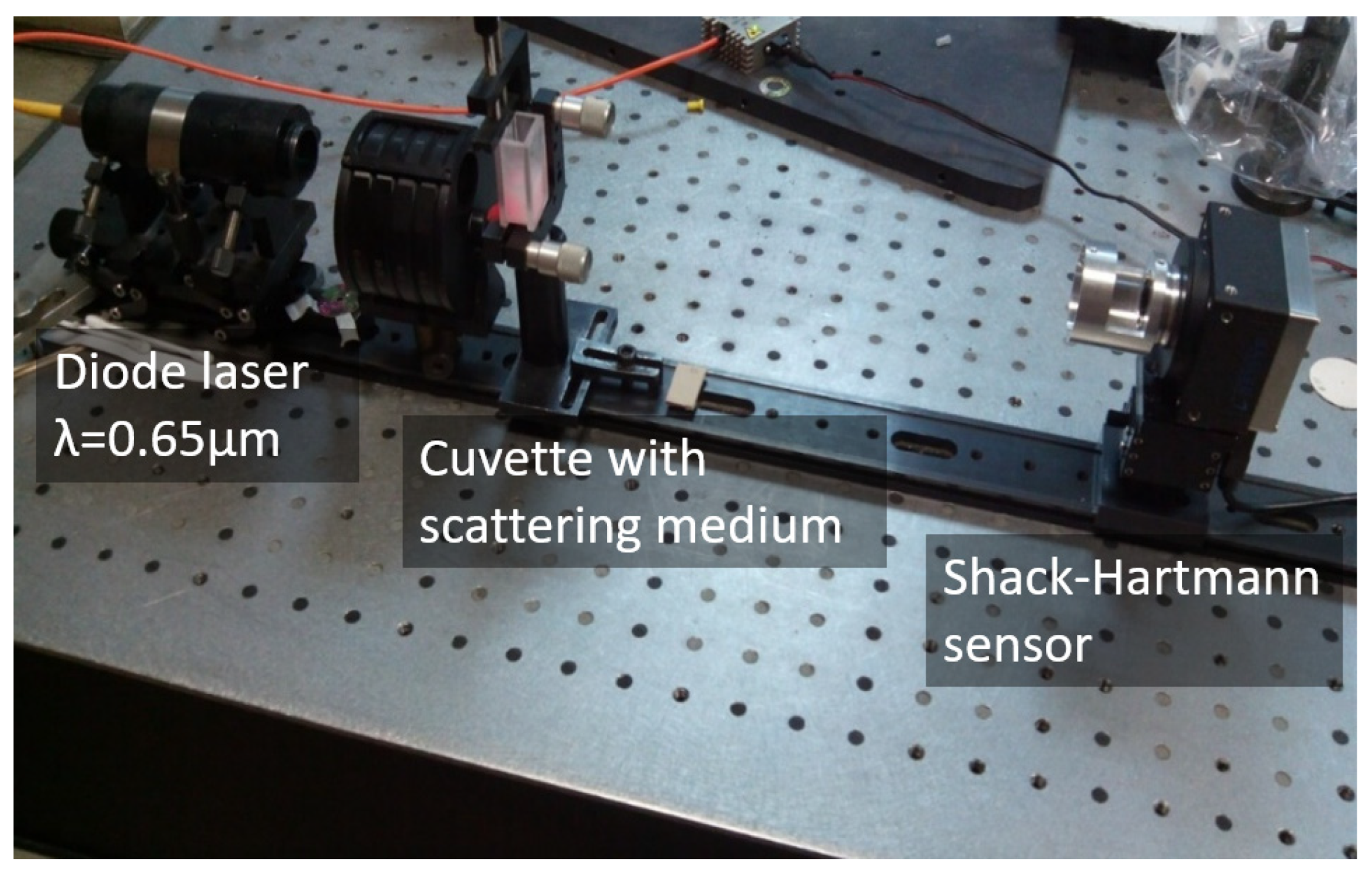
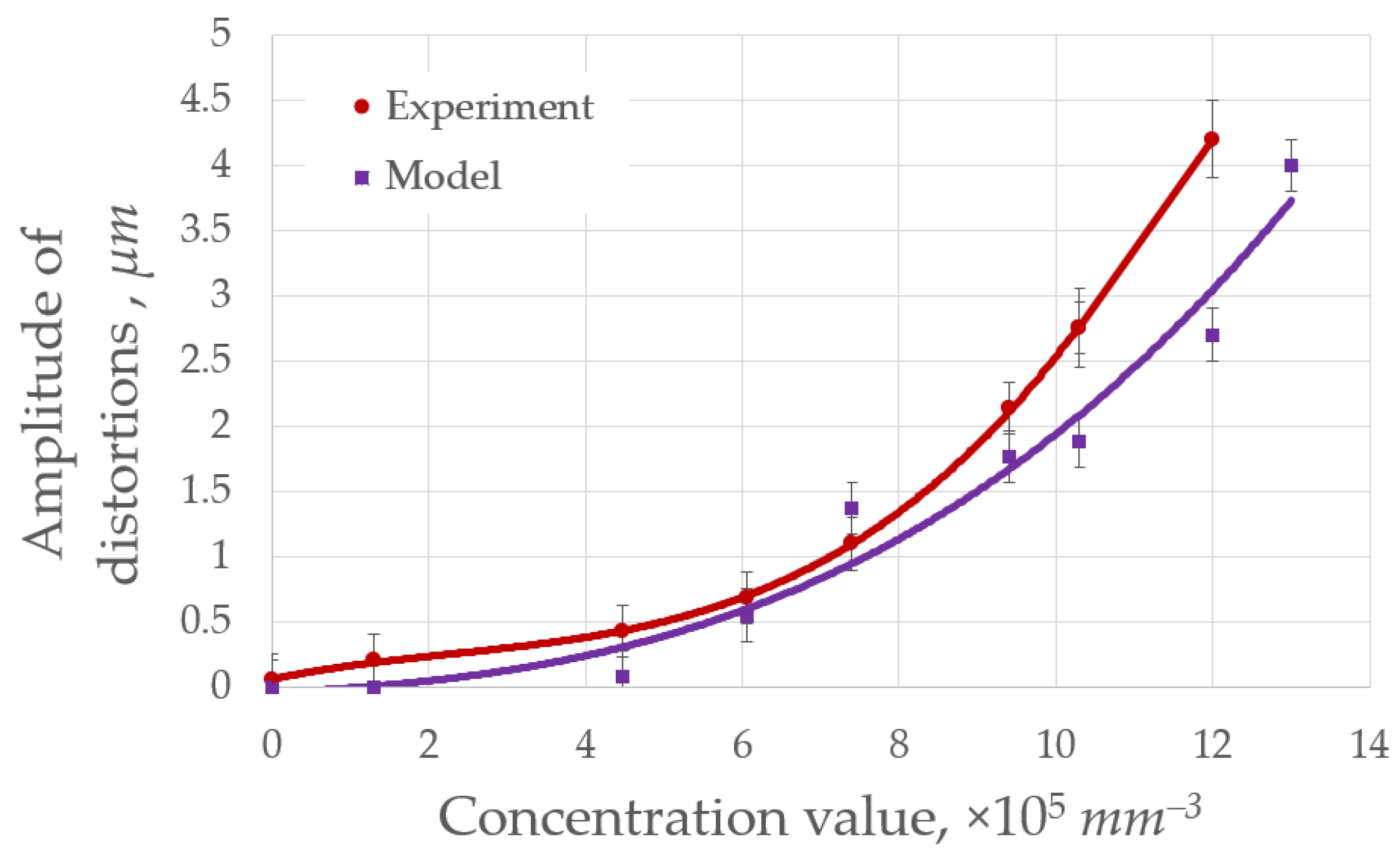
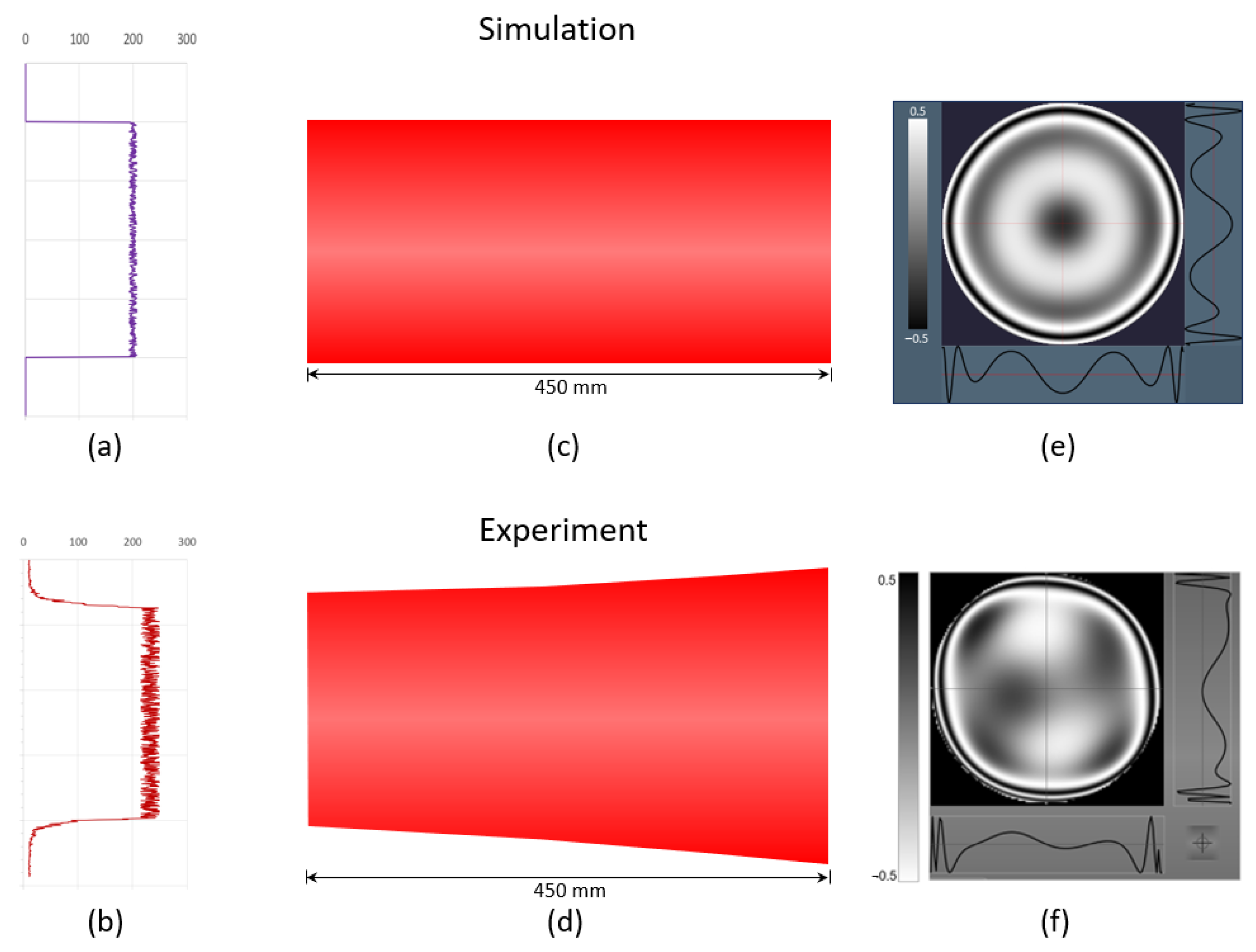
3. Hybrid Model Verification: Experimental Results and Discussion
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Berrocal, E.; Sedarsky, D.; Paciaroni, M.; Meglinski, I.; Linne, M. Laser light scattering in turbid media Part I: Experimental and simulated results for the spatial intensity distribution. Opt. Express 2007, 15, 10649–10665. [Google Scholar] [CrossRef] [PubMed]
- Measures, R.M. Laser Remote Sensing: Fundamentals and Applications; Krieger: Malabar, FL, USA, 1992. [Google Scholar]
- Shao, B.; Jaffe, J.S.; Chachisvilis, M.; Esener, S.C. Angular resolved light scattering for discriminating among marine picoplankton: Modeling and experimental measurements. Opt. Express 2006, 14, 12473–12484. [Google Scholar] [CrossRef] [PubMed]
- Tuchin, V.V. Handbook of Optical Biomedical Diagnostics; SPIE Press: Bellingham, WA, USA, 2002. [Google Scholar]
- Lee, C.-K.; Sun, C.-W.; Lee, P.-L.; Lee, H.-C.; Yang, C.; Jiang, C.-P.; Tong, Y.-P.; Yeh, T.-C.; Hsieh, J.-C. Study of photon migration with various source-detector separations in near-infrared spectroscopic brain imaging based on three-dimensional Monte Carlo modeling. Opt. Express 2005, 13, 8339–8348. [Google Scholar] [CrossRef]
- Berrocal, E. Multiple Scattering of Light in Optical Diagnostics of Dense Sprays and Other Complex Turbid Media. Ph.D. Thesis, Cranfield University, Cranfield, UK, 2006. [Google Scholar]
- Sobol, I. The Monte Carlo Method; The University of Chicago Press: Chicago, IL, USA, 1974. [Google Scholar]
- Wang, L.; Jacques, S.L.; Zheng, L. MCML—Monte Carlo modelling of light transport in multi-layered tissues. Comput. Methods Programs Biomed. 1995, 47, 131–146. [Google Scholar] [CrossRef]
- Churmakov, D.Y. Multipurpose Computational Model for Modern Optical Diagnostics and Its Biomedical Applications. Ph.D. Thesis, Cranfield University, Cranfield, UK, 2005. [Google Scholar]
- Boas, D.; Culver, J.; Stott, J.; Dunn, A. Three dimensional Monte Carlo code for photon migration through complex heterogeneous media including the adult human head. Opt. Express 2002, 10, 159–170. [Google Scholar] [CrossRef] [PubMed]
- Mosk, A.P.; Lagendijk, A.; Lerosey, G.; Fink, M. Controlling waves in space and time for imaging and focusing in complex media. Nat. Photonics 2012, 6, 283. [Google Scholar] [CrossRef]
- Bashkatov, A.N.; Priezzhev, A.V.; Tuchin, V.V. Laser technologies in biophotonics. Quantum Electron. 2012, 42, 379. [Google Scholar] [CrossRef]
- Vellekoop, I.M.; Mosk, A.P. Focusing coherent light through opaque strongly scattering media. Opt. Lett. 2007, 32, 2309. [Google Scholar] [CrossRef]
- Zhang, Y.; Chen, Y.; Yu, Y.; Xue, X.; Tuchin, V.V.; Zhu, D. Visible and near-infrared spectroscopy for distinguishing malignant tumor tissue from benign tumor and normal breast tissues in vitro. J. Biomed. Opt. 2013, 18, 077003. [Google Scholar] [CrossRef]
- Goodman, J.W.; Huntley, W.H., Jr.; Jackson, D.W.; Lehmann, M. Wavefront reconstruction imaging through random media. Appl. Phys. Lett. 1966, 8, 311–313. [Google Scholar] [CrossRef]
- Kogelnik, H.; Pennington, K.S. Holographic imaging through a random medium. J. Opt. Soc. Am. 1968, 58, 273–274. [Google Scholar] [CrossRef]
- Matthews, T.; Medina, M.; Maher, J.; Levinson, H.; Brown, W.; Wax, A. Deep tissue imaging using spectroscopic analysis of multiply scattered light. Optica 2014, 1, 105. [Google Scholar] [CrossRef]
- Bertolotti, J.; van Putten, E.G.; Blum, C.; Lagendijk, A.; Vos, W.L.; Mosk, A.P. Non-invasive imaging through opaque scattering layers. Nature 2012, 491, 232–234. [Google Scholar] [CrossRef]
- Katz, O.; Small, E.; Silberberg, Y. Looking around corners and through thin turbid layers in real time with scattered incoherent light. Nat. Photon. 2012, 6, 549–553. [Google Scholar] [CrossRef]
- Popoff, S.M.; Lerosey, G.; Carminati, R.; Fink, M.; Boccara, A.C.; Gigan, S. Measuring the transmission matrix in optics: An approach to the study and control of light propagation in disordered media. Phys. Rev. Lett. 2010, 104, 100601. [Google Scholar] [CrossRef]
- Conkey, D.B.; Caravaca-Aguirre, A.M.; Piestun, R. High-speed scattering medium characterization with application to focusing light through turbid media. Opt. Express 2012, 20, 1733–1740. [Google Scholar] [CrossRef] [PubMed]
- Hsieh, C.; Pu, Y.; Grange, R.; Laporte, G.; Psaltis, D. Imaging through turbid layers by scanning the phase conjugated second harmonic radiation from a nanoparticle. Opt. Express 2010, 18, 20723–20731. [Google Scholar] [CrossRef]
- Stockbridge, C.; Lu, Y.; Moore, J.; Hoffman, S.; Paxman, R.; Toussaint, K.; Bifano, T. Focusing through dynamic scattering media. Opt. Express 2012, 20, 15086–15092. [Google Scholar] [CrossRef]
- Xu, J.; Ruan, H.; Liu, Y.; Zhou, H.; Yang, C. Focusing light through scattering media by transmission matrix inversion. Opt. Express 2017, 25, 27234–27246. [Google Scholar] [CrossRef]
- Zhou, E.H.; Ruan, H.; Yang, C.; Judkewitz, B. Focusing on moving targets through scattering samples. Optica 2014, 1, 227–232. [Google Scholar] [CrossRef]
- Shen, Y.; Liu, Y.; Ma, C.; Wang, L.V. Focusing light through scattering media by full-polarization digital optical phase conjugation. Opt. Lett. 2016, 41, 1130–1133. [Google Scholar] [CrossRef] [PubMed]
- Feldkhun, D.; Tzang, O.; Wagner, K.H.; Piestun, R. Focusing and scanning through scattering media in microseconds. Optica 2019, 6, 72–75. [Google Scholar] [CrossRef]
- Galaktionov, I.; Kudryashov, A.; Sheldakova, J.; Nikitin, A. Laser beam focusing through the scattering medium using bimorph deformable mirror and spatial light modulator. Proc. SPIE 2019, 11135, 111350B. [Google Scholar]
- Galaktionov, I.; Sheldakova, J.; Kudryashov, A. Scattered laser beam control using bimorph deformable mirror. In Proceedings of the 18th International Conference “Laser Optics 2018”, St. Petersburg, Russia, 5–7 June 2018; p. 186. [Google Scholar]
- Galaktionov, I.; Sheldakova, J.; Toporovsky, V.; Samarkin, V.; Kudryashov, A. Bimorph vs stacked actuator deformable mirror for laser beam focusing through a moderately scattering medium. Proc. SPIE 2021, 11672, 1167214. [Google Scholar]
- Belousov, V.N.; Galaktionov, I.V.; Kudryashov, A.V.; Nikitin, A.N.; Otrubyannikova, O.V.; Rukosuev, A.L.; Samarkin, V.V.; Sivertceva, I.V.; Sheldakova, J.V. Adaptive optical system for correction of laser beam going through turbulent atmosphere. Proc. SPIE 2020, 11560, 1156026. [Google Scholar]
- Rukosuev, A.; Belousov, V.; Galaktionov, I.; Kudryashov, A.; Nikitin, A.; Samarkin, V.; Sheldakova, J. 1.5 kHz adaptive optical system for free-space communication tasks. Proc. SPIE 2020, 11272, 112721G. [Google Scholar]
- Nikitin, A.; Galaktionov, I.; Sheldakova, J.; Kudryashov, A.; Samarkin, V.; Rukosuev, A. Focusing laser beam through pinhole using bimorph deformable mirror. Proc. SPIE 2019, 10904, 109041I. [Google Scholar]
- Li, R.; Jin, D.; Pan, D.; Ji, S.; Xin, C.; Liu, G.; Fan, S.; Wu, H.; Li, J.; Hu, Y.; et al. Stimuli-Responsive Actuator Fabricated by Dynamic Asymmetric Femtosecond Bessel Beam for In Situ Particle and Cell Manipulation. ACS Nano 2020, 14, 5233–5242. [Google Scholar] [CrossRef]
- Galaktionov, I.; Nikitin, A.; Sheldakova, J.; Toporovsky, V.; Kudryashov, A. Focusing of a Laser Beam Passed through a Moderately Scattering Medium Using Phase-Only Spatial Light Modulator. Photonics 2022, 9, 296. [Google Scholar] [CrossRef]
- Sheldakova, J.; Toporovsky, V.; Galaktionov, I.; Nikitin, A.; Rukosuev, A.; Samarkin, V.; Kudryashov, A. Flat-top beam formation with miniature bimorph deformable mirror. Proc. SPIE 2020, 11486, 114860E. [Google Scholar]
- Galaktionov, I.; Sheldakova, J.; Nikitin, A.; Samarkin, V.; Parfenov, V.; Kudryashov, A. Laser beam focusing through a moderately scattering medium using a bimorph mirror. Opt. Express 2020, 28, 38061–38075. [Google Scholar] [CrossRef] [PubMed]
- Durant, S.; Calvo-Perez, O.; Vukadinovic, N.; Greffet, J.-J. Light scattering by a random distribution of particles embedded in absorbing media: Full-wave Monte Carlo solutions of the extinction coefficient. J. Opt. Soc. Am. A 2007, 24, 2953–2962. [Google Scholar] [CrossRef]
- Lacasa, J.; Hefley, T.J.; Otegui, M.E.; Ciampitti, I.A. A practical guide to estimating the light extinction coefficient with nonlinear models—A case study on maize. Plant Methods 2021, 17, 60. [Google Scholar] [CrossRef]
- Scattering Coefficient Definition. Available online: https://omlc.org/classroom/ece532/class3/musdefinition.html (accessed on 19 June 2023).
- Hohmann, M.; Lengenfelder, B.; Muhr, D.; Späth, M.; Hauptkorn, M.; Klämpfl, F.; Schmidt, M. Direct measurement of the scattering coefficient. Biomed. Opt. Express 2021, 12, 320–335. [Google Scholar] [CrossRef] [PubMed]
- Hsia, Y.; Fang, N.; Widatallah, H.; Wu, D.M.; Lee, X.M.; Zhang, J.R. Brownian motion of suspended particles in an anisotropic medium. Hyperfine Interact. 2000, 126, 401–406. [Google Scholar] [CrossRef]
- Vorob’eva, E.A.; Gurov, I.P. Models of Propagation and Scattering of Optical Radiation in Randomly Inhomogeneous Media; Meditsina: Moscow, Russia, 2006.
- Zege, E.P.; Ivanov, A.P.; Katsev, I.L. Image Transfer through a Scattering Medium; Springer: New York, NY, USA; Berlin/Heidelberg, Germany, 1991; p. 131. [Google Scholar]
- Jacques, S.L.; Prahl, S.A. Henyey-Greenstein Scattering Function. Available online: http://omlc.org/classroom/ece532/class3/hg.html (accessed on 7 June 2023).
- Wilson, B.C.; Adam, G. A Monte Carlo model for the absorption and flux distributions of light in tissue. Med. Phys. 1983, 10, 824–830. [Google Scholar] [CrossRef]
- Keijzer, M.; Jacques, S.L.; Prahl, S.A.; Welch, A.J. Light distributions in artery tissue: Monte Carlo simulations for finite-diameter laser beams. Lasers Surg. Med. 1989, 9, 148–154. [Google Scholar] [CrossRef]
- Prahl, S.A.; Keijzer, M.; Jacques, S.L.; Welch, A.J. A Monte Carlo model of light propagation in tissue. In Dosimetry of Laser Radiation in Medicine and Biology; SPIE Series; Muller, G., Sliney, D., Eds.; SPIE: Bellingham, WA, USA, 1989; pp. 102–111. [Google Scholar]
- Meglinski, I.V. Quantitative assessment of skin layers absorption and skin reflectance spectra simulation in the visible and near-infrared spectral regions. Physiol. Meas. 2002, 23, 741. [Google Scholar] [CrossRef]
- Kandidov, V.; Militsin, V.; Bykov, A.; Priezzhev, A. Application of corpuscular and wave Monte-Carlo methods in optics of dispersive media. Quantum Electron. 2006, 36, 1003. [Google Scholar] [CrossRef]
- Han, X.; Shen, J.; Yin, P.; Hu, S.; Bi, D. Influences of refractive index on forward light scattering. Opt. Commun. 2014, 316, 198–205. [Google Scholar] [CrossRef]
- Ma, X.; Lu, J.; Brocks, S.; Jacob, K.; Yang, P.; Xin, X.-H. Determination of complex refractive index of polystyrene microspheres from 370 to 1610 nm. Phys. Med. Biol. 2003, 48, 4165. [Google Scholar] [CrossRef]
- Piskozub, J.; McKee, D. Effective scattering phase functions for the multiple scattering regime. Opt. Express 2011, 19, 4786–4794. [Google Scholar] [CrossRef] [PubMed]
- Mishchenko, M.; Travis, L.D.; Lacis, A.A. Multiple Scattering of Light by Particles: Radiative Transfer and Coherent Backscattering; Cambridge University Press: Cambridge, UK, 2006. [Google Scholar]
- Zvereva, S. In the world of sunlight. Hydrometizdat 1988, 160. [Google Scholar]
- Fukutomi, D.; Ishii, K.; Awazu, K. Determination of the scattering coefficient of biological tissue considering the wavelength and absorption dependence of the anisotropy factor. Opt. Rev. 2016, 23, 291–298. [Google Scholar] [CrossRef]
- Lobanova, M.A.; Vasiliev, A.V.; Melnikova, I.N. Dependence of anisotropy factor of scattering phase function on the medium parameters. Mod. Probl. Remote Sens. Earth Space 2010, 7. [Google Scholar]
- Bashkatov, A.; Genina, E.; Tuchin, V.; Altshuler, G.; Yaroslavsky, I. Monte Carlo study of skin optical clearing to enhance light penetration in the tissue: Implications for photodynamic therapy of acne vulgaris. Proc. SPIE 2008, 7022, 702209. [Google Scholar]
- Vitkin, E.; Turzhitsky, V.; Qiu, L.; Guo, L.; Itzkan, I.; Hanlon, E.B.; Perelman, L.T. Photon diffusion near the point-of-entry in anisotropically scattering turbid media. Nat. Commun. 2011, 2, 587. [Google Scholar] [CrossRef]
- Tschukin, O.; Silberzahn, A.; Selzer, M.; Amos, P.G.K.; Schneider, D.; Nestler, B. Concepts of modeling surface energy anisotropy in phase-field approaches. Geotherm Energy 2017, 5, 19. [Google Scholar] [CrossRef]
- Platt, B.; Shack, R.J. History and principles of Shack-Hartmann wavefront sensing. Refr. Surg. 2001, 17, 15. [Google Scholar] [CrossRef] [PubMed]
- Liang, J.; Grimm, B.; Goelz, S.; Bille, J.F. Objective measurement of the wave aberrations of the human eye with the use of a Hartmann-Shack wave-front sensor. J. Opt. Soc. Am. A 1994, 11, 1949. [Google Scholar] [CrossRef]
- Lane, R.G. Wave-front reconstruction using a Shack–Hartmann sensor. Appl. Opt. 1992, 31, 6902. [Google Scholar] [CrossRef] [PubMed]
- Primot, J. Theoretical description of Shack–Hartmann wave-front sensor. Opt. Commun. 2003, 222, 81–92. [Google Scholar] [CrossRef]
- Konnik, M.; Doná, J. Waffle mode mitigation in adaptive optics systems: A constrained Receding Horizon Control approach. In Proceedings of the American Control Conference, Washington, DC, USA, 17–19 June 2013; pp. 3390–3396. [Google Scholar]
- Mauch, S.; Reger, J. Real-Time Spot Detection and Ordering for a Shack–Hartmann Wavefront Sensor With a Low-Cost FPGA. Proc. IEEE Trans. Instrum. Meas. 2014, 63, 2379–2386. [Google Scholar] [CrossRef]
- Vellekoop, I. Feedback-based wavefront shaping. Opt. Express 2015, 23, 12189–12206. [Google Scholar] [CrossRef]
- Galaktionov, I.V. Increase of Focusing Efficiency of Scattered Laser Radiation by Means of Adaptive Optics. Ph.D. Thesis, Institute of Applied Physics Russian Academy of Sciences, Nizhniy Novgorod, Russia, 2021. [Google Scholar]
- Galaktionov, I.; Nikitin, A.; Sheldakova, J.; Kudryashov, A. B-spline approximation of a wavefront measured by Shack-Hartmann sensor. Proc. SPIE 2021, 11818, 118180N. [Google Scholar]
- Malacara-Hernandez, D. Wavefront fitting with discrete orthogonal polynomials in a unit radius circle. Opt. Eng. 1990, 29. [Google Scholar] [CrossRef]
- Wyant, J.C.; Creath, K. Basic wavefront aberration theory for optical metrology. In Proceedings of Applied Optics and Optical Engineering; Academic Press, Inc.: Cambridge, MA, USA, 1992; pp. 27–39. [Google Scholar]
- Genberg, V.; Michels, G.; Doyle, K. Orthogonality of Zernike polynomials. Proc. SPIE 2002, 4771. [Google Scholar] [CrossRef]
- Lakshminarayanan, V.; Fleck, A. Zernike polynomials: A guide. J. Mod. Opt. 2011, 58, 545–561. [Google Scholar] [CrossRef]
- Linnik, J.V. Least Square Error Method and the Basics of Mathematical-Statistical Theory, 2nd ed.; Mir Publishers: Moscow, Russia, 1962. [Google Scholar]
- Ramachandran, H. Imaging through turbid media. Curr. Sci. 1999, 76, 1334. [Google Scholar]
- Polystyrene Microbeads. Available online: https://magsphere.com/Products/Polystyrene-Latex-Particles/polystyrene-latex-particles.html (accessed on 19 June 2023).
- Galaktionov, I.; Sheldakova, J.; Kudryashov, A. Laser beam focusing through the scattering medium using 14-, 32- and 48-channel bimorph mirrors. In Proceedings of the 18th International Conference “Laser Optics 2018”, St. Petersburg, Russia, 5–7 June 2018; p. 223. [Google Scholar]
- Liu, Y.; Hu, J. Investigation of Polystyrene-Based Microspheres from Different Copolymers and Their Structural Color Coatings on Wood Surface. Coatings 2021, 11, 14. [Google Scholar] [CrossRef]
- Galaktionov, I.V.; Kudryashov, A.V.; Sheldakova, J.V.; Byalko, A.A.; Borsoni, G. Measurement and correction of the wavefront of the laser light in a turbid medium. Quantum Electron. 2017, 47, 32–37. [Google Scholar] [CrossRef]
| Criteria | MCML | Developed Model |
|---|---|---|
| Transmittance, % | 94.380 | 94.377 |
| Reflectance, % | 5.620 | 5.623 |
| % of single scattered photons | 25.080 | 25.060 |
| % of double scattered photons | 23.910 | 23.910 |
| % of photons with scattering order = 3 | 16.280 | 16.288 |
| % of photons with scattering order = 4 | 9.110 | 9.120 |
| % of photons with scattering order = 5 | 4.660 | 4.660 |
| % of photons with scattering order = 6 | 2.410 | 2.410 |
| % of photons with scattering order = 7 | 1.370 | 1.370 |
| % of photons in circle (radius = 100 µm) | 16.540 | 16.550 |
| % of photons in ring (r1 = 100 µm, r2 = 200 µm) | 3.350 | 3.330 |
| % of photons in ring (r1 = 200 µm, r2 = 300 µm) | 3.410 | 3.420 |
| % of photons in ring (r1 = 300 µm, r2 = 400 µm) | 3.390 | 3.370 |
| % of photons in ring (r1 = 400 µm, r2 = 500 µm) | 3.300 | 3.290 |
| % of photons in ring (r1 = 500 µm, r2 = 600 µm) | 3.160 | 3.160 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Galaktionov, I.; Sheldakova, J.; Nikitin, A.; Toporovsky, V.; Kudryashov, A. A Hybrid Model for Analysis of Laser Beam Distortions Using Monte Carlo and Shack–Hartmann Techniques: Numerical Study and Experimental Results. Algorithms 2023, 16, 337. https://doi.org/10.3390/a16070337
Galaktionov I, Sheldakova J, Nikitin A, Toporovsky V, Kudryashov A. A Hybrid Model for Analysis of Laser Beam Distortions Using Monte Carlo and Shack–Hartmann Techniques: Numerical Study and Experimental Results. Algorithms. 2023; 16(7):337. https://doi.org/10.3390/a16070337
Chicago/Turabian StyleGalaktionov, Ilya, Julia Sheldakova, Alexander Nikitin, Vladimir Toporovsky, and Alexis Kudryashov. 2023. "A Hybrid Model for Analysis of Laser Beam Distortions Using Monte Carlo and Shack–Hartmann Techniques: Numerical Study and Experimental Results" Algorithms 16, no. 7: 337. https://doi.org/10.3390/a16070337
APA StyleGalaktionov, I., Sheldakova, J., Nikitin, A., Toporovsky, V., & Kudryashov, A. (2023). A Hybrid Model for Analysis of Laser Beam Distortions Using Monte Carlo and Shack–Hartmann Techniques: Numerical Study and Experimental Results. Algorithms, 16(7), 337. https://doi.org/10.3390/a16070337












