Masonry Columns Confined by Steel Fiber Composite Wraps
Abstract
:1. Introduction
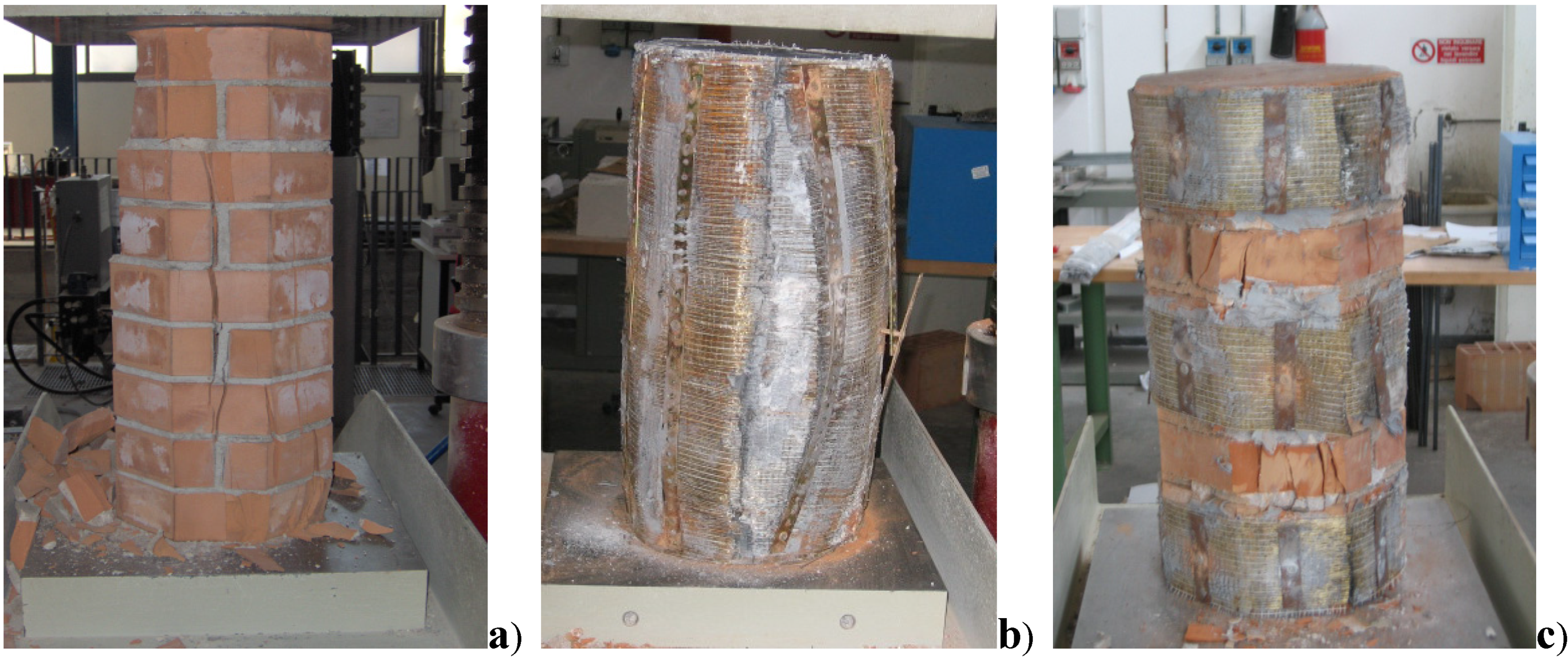
2. Test on Masonry Columns
2.1. Test Matrix
| Specimen | Shape of Cross-section | Matrix Type | Reinforcement Type | Reinforcing Scheme |
|---|---|---|---|---|
| 1 | octagonal | - | - | - |
| 2 | square | - | - | - |
| 3 | square | epoxy | Type 1 | Continuous wrap |
| 4 | square | epoxy | Type 1 | Continuous wrap |
| 5 | octagonal | epoxy | Type 2 | Continuous wrap |
| 6 | octagonal | epoxy | Type 2 | Discontinuous wrap |
| 7 | octagonal | epoxy | Type 2 | Continuous wrap |
| 8 | octagonal | epoxy | Type 2 | Discontinuous wrap |
| 9 | octagonal | epoxy | Type 1 | Continuous wrap |
| 10 | octagonal | epoxy | Type 1 | Discontinuous wrap |
| 11 | octagonal | epoxy | Type 1 | Continuous wrap |
| 12 | octagonal | epoxy | Type 2 | Discontinuous wrap |
| 13 | octagonal | epoxy | Type 1 | Discontinuous wrap |
| 14 | octagonal | epoxy | Type 1 | Continuous wrap |
| 15 | octagonal | epoxy | Type 2 | Discontinuous wrap |
| 16 | octagonal | epoxy | Type 2 | Continuous wrap |
| 17 | square | epoxy | Type 1 | Continuous wrap |
| 18 | square | epoxy | Type 1 | Continuous wrap |
| 19 | square | epoxy | Type 1 | Discontinuous wrap |
| 20 | square | epoxy | Type 1 | Discontinuous wrap |
| 21 | square | epoxy | Type 1 | Discontinuous wrap |
| 22 | square | epoxy | Type 1 | Discontinuous wrap |
| 23 | square | epoxy | Type 1 | Discontinuous wrap |


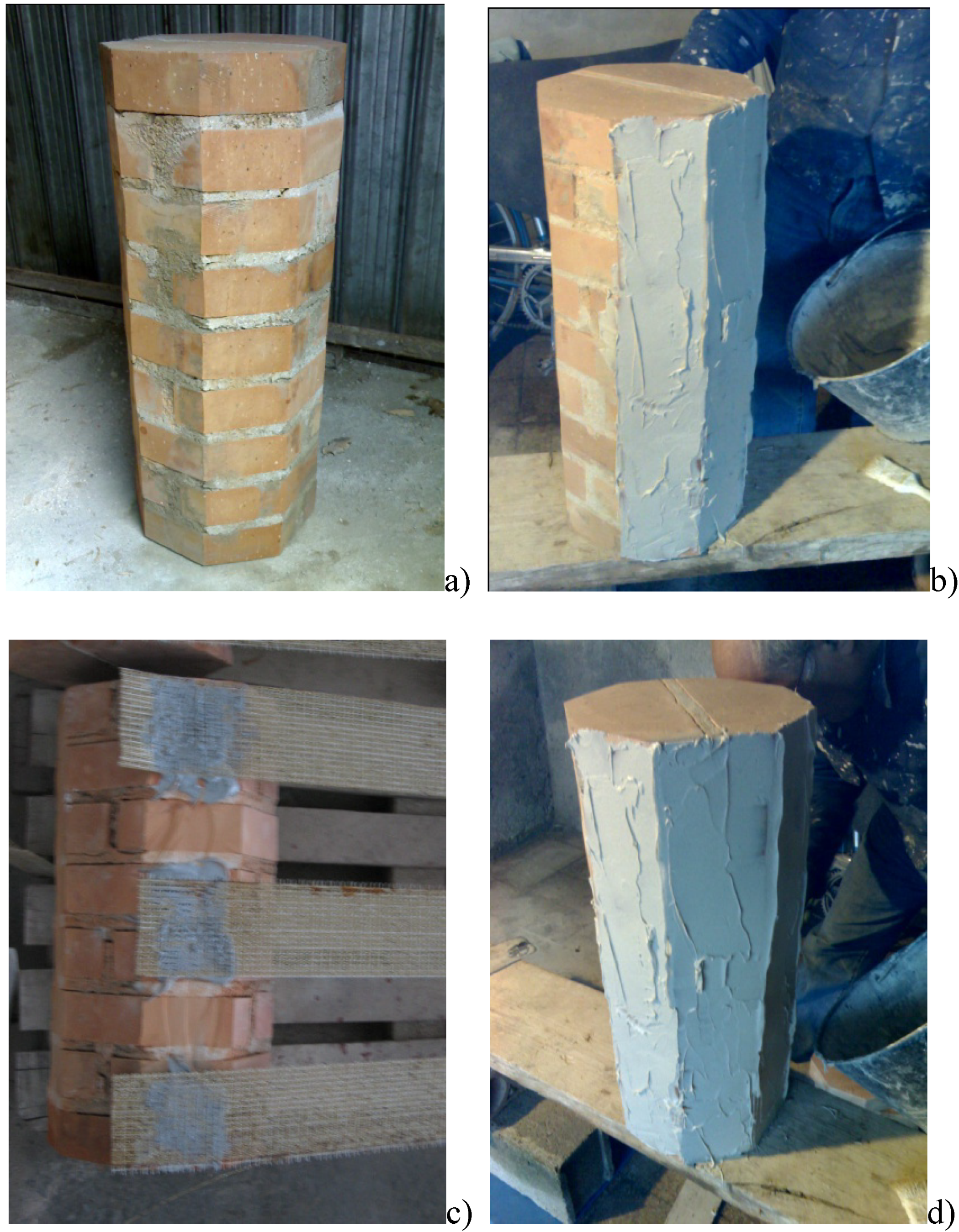

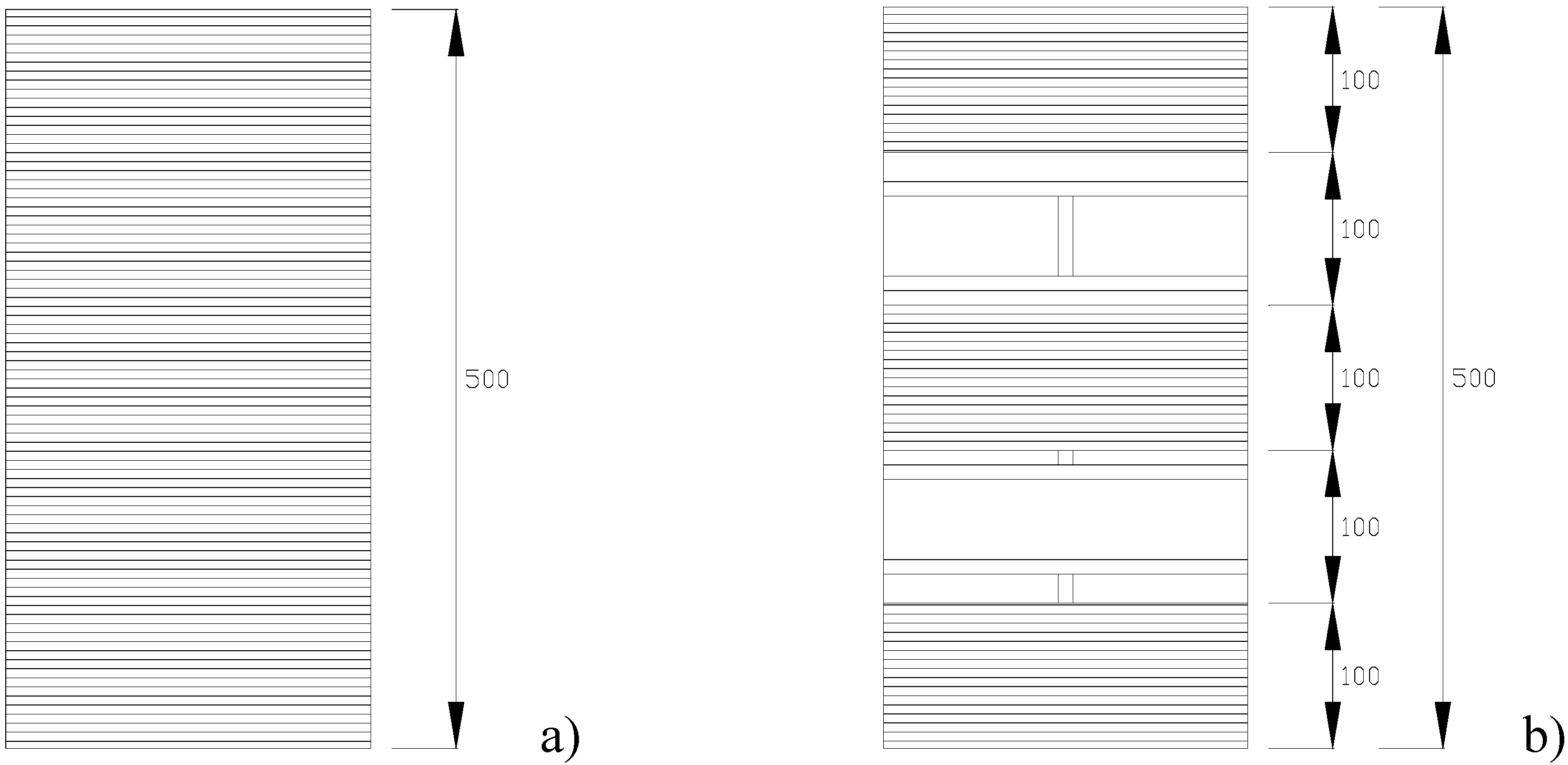
2.2. Mechanical Characterization of Materials
2.3. Cord Characterization
| Type 1 | Type 2 | |
|---|---|---|
| Cord sectional area (mm2) | 0.621 | 0.811 |
| Tensile strength (MPa) | 3199 | 2396 |
| Elastic Modulus (MPa) | 160,000 | 143,000 |
| Ultimate strain (%) | 1.55 | 1.16 |
2.4. Epoxy Resins
2.5. Bricks
| Number of Samples | 4 |
| Tensile Strength (MPa) | 25.21 |
| Young Modulus E (MPa) | 4510 |
| Number of Samples | 5 |
| Compressive Strength (MPa) | 65.54 |
| Young Modulus E (MPa) | 4634 |
2.6. Mortar
3. Test Setup

4. Experimental Results
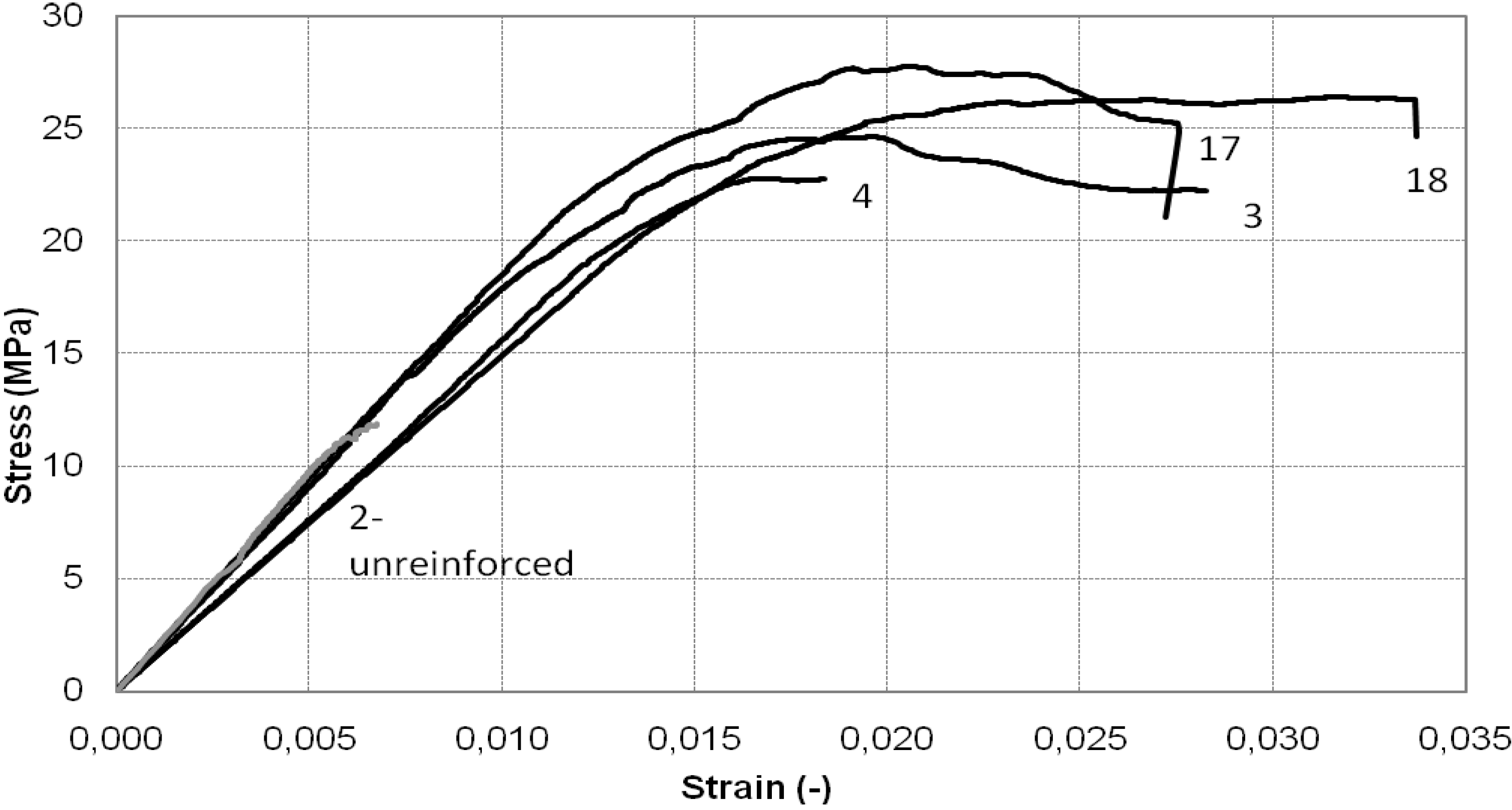
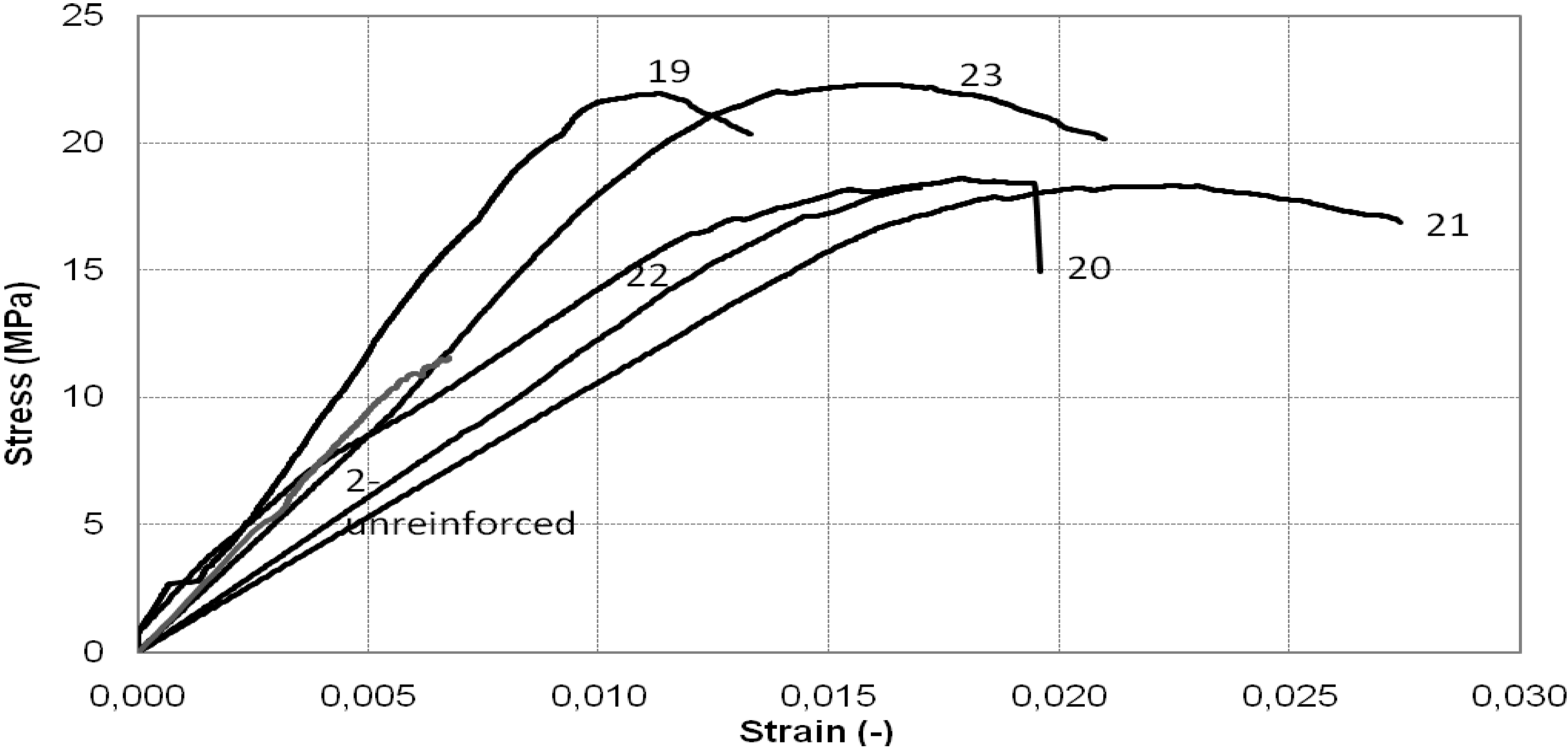
4.1. Square Cross-Section Masonry Specimens
| Series | Specimen | Volumetric ratio λ (%) | Average max compression load P (kN) | Average compressive strength (MPa) | Normalized strength Pconfined/Punconfined | Average load peak axial strain |
|---|---|---|---|---|---|---|
| Unconfined | 2 | - | 708 | 11.91 | 1.00 | 0.007 |
| S-3X2-C | 3, 4, 17, 18 | 0.60 | 1510 | 26.65 | 2.24 | 0.0225 |
| S-3X2-D | 19, 20, 21, 22, 23 | 0.40 | 1189 | 19.88 | 1.67 | 0.017 |

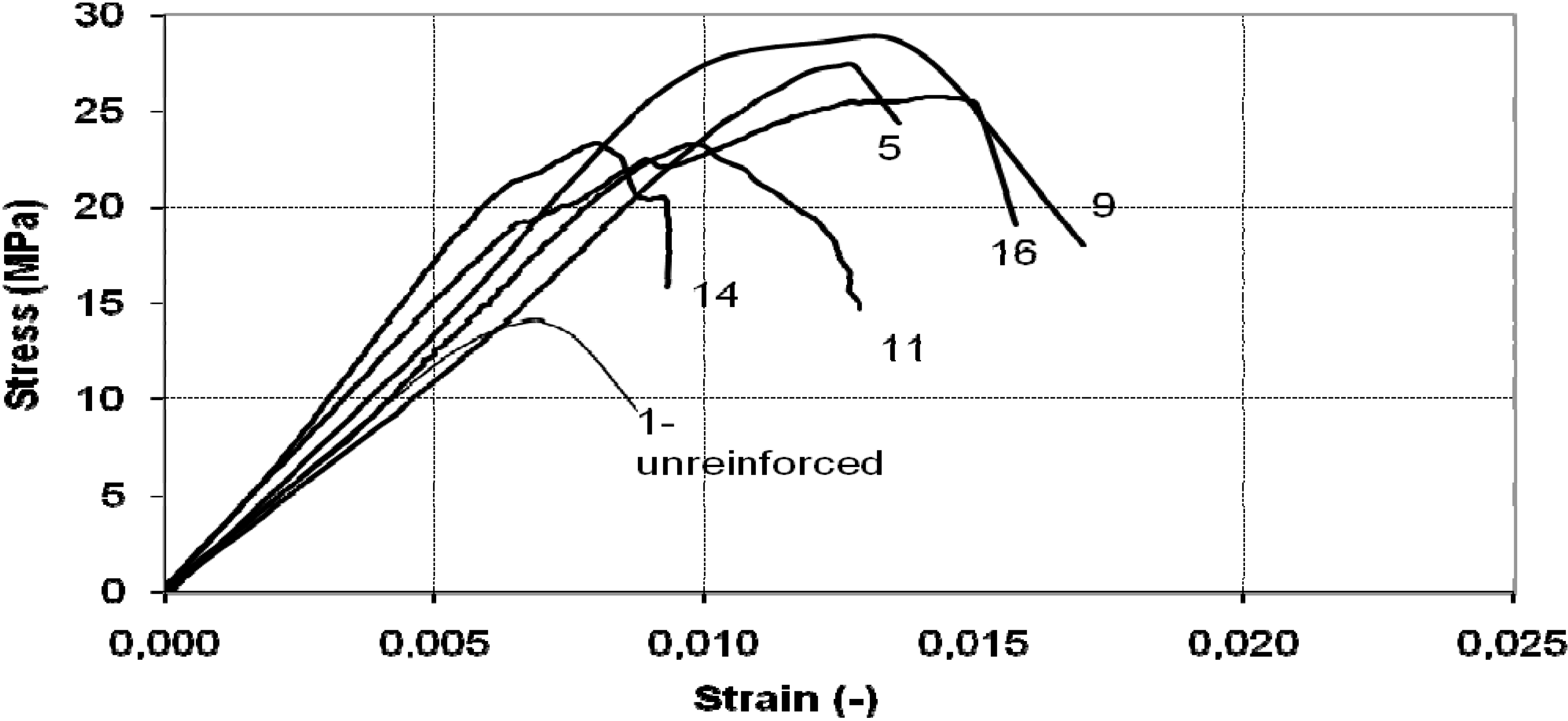
4.2. Octagonal Cross-Section Masonry Specimens
| Series | Specimen | Volumetric ratios λ (%) | Average max compression load P (kN) | Average compressive strength (MPa) | Normalized strength Pconfined/Punconfined | Average load peak axial strain |
|---|---|---|---|---|---|---|
| Unconfined | 1 | - | 719 | 14.0 | 1.00 | 0.0085 |
| O-3SX-C | 5, 7, 16 | 0.47 | 1361 | 26.6 | 1.90 | 0.0166 |
| O-3SX-D | 6, 8, 12, 15 | 0.31 | 1293 | 25.2 | 1.80 | 0.0128 |
| O-3X2-C | 9, 11, 14 | 0.62 | 1289 | 25.2 | 1.79 | 0.0117 |
| O-3X2-D | 10,13 | 0.41 | 1224 | 23.9 | 1.71 | 0.0127 |

5. Design
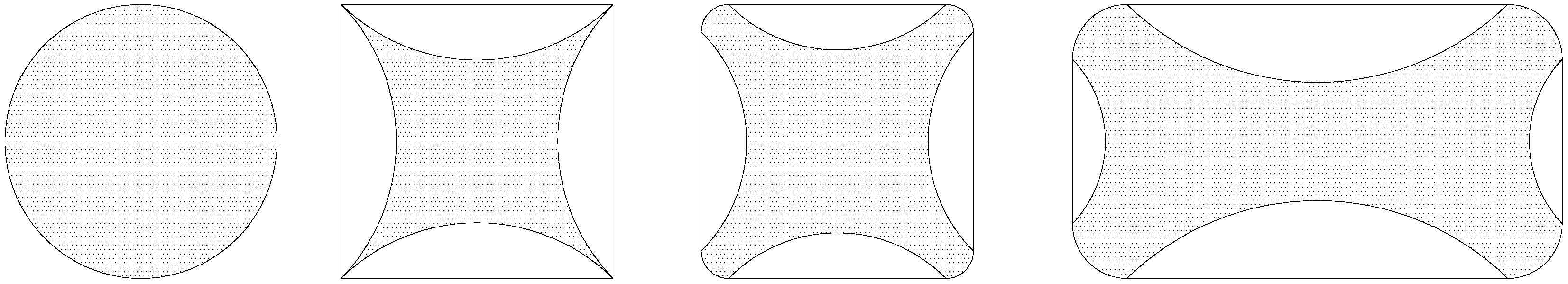
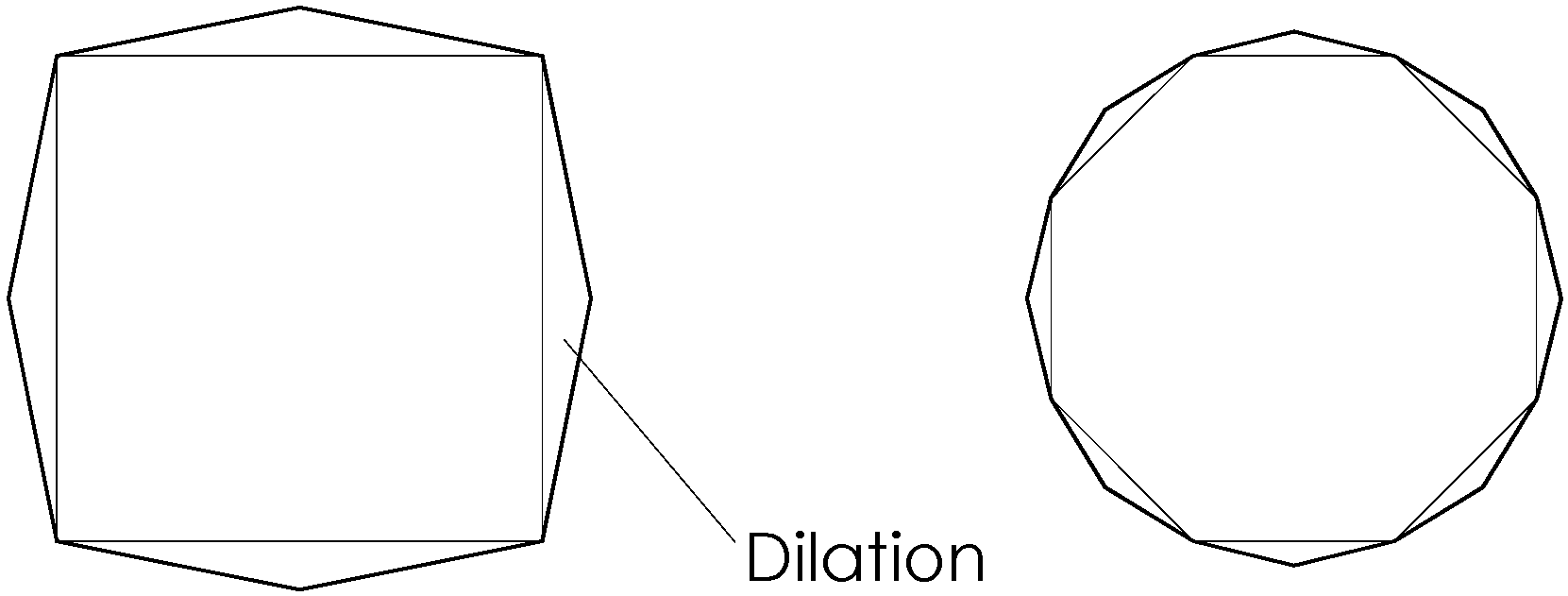
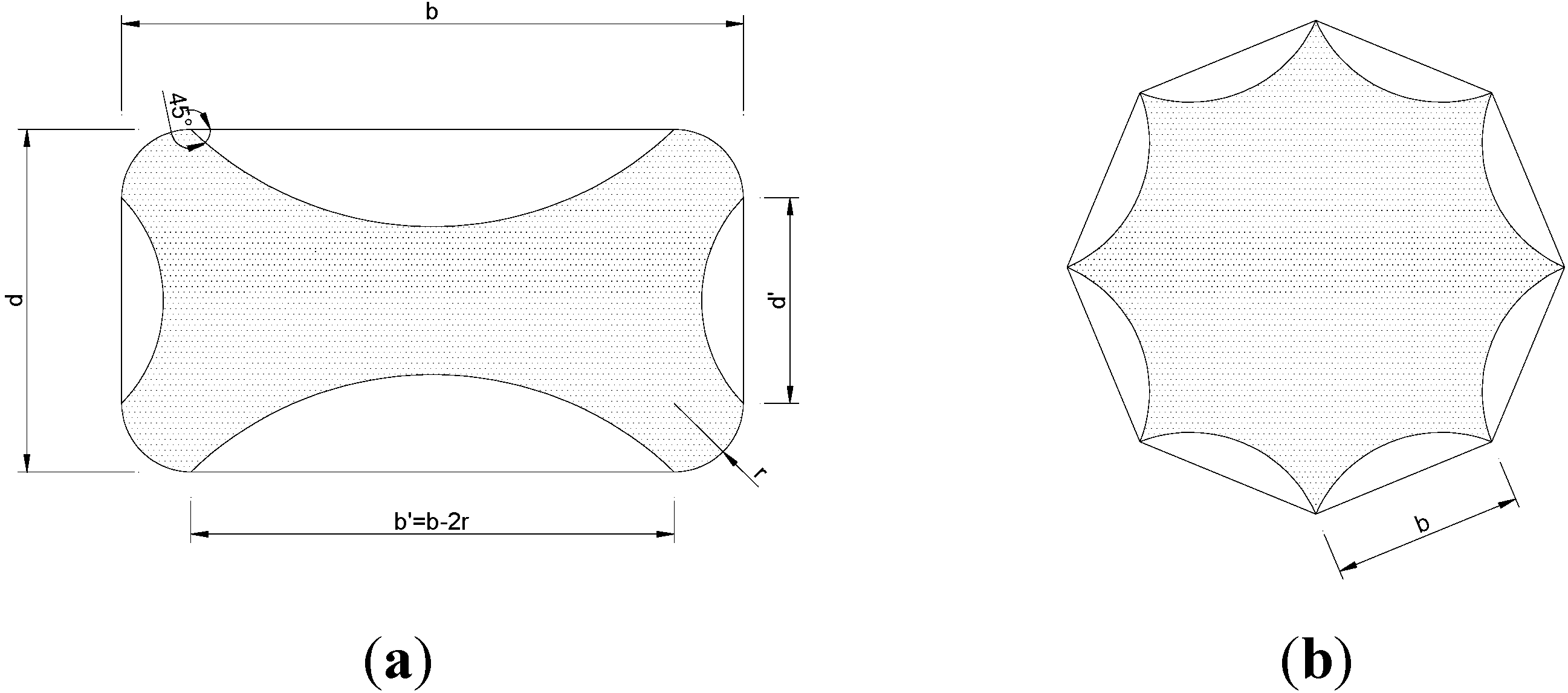
| Series | keff | f1 | f’1 | Maximum compression load Ptheoretical (kN) | Maximum compression load Pexperimental (kN) | Pexperimental /Ptheoretical |
|---|---|---|---|---|---|---|
| S-3X2-C | 0.497 | 13.95 | 6.93 | 1400 | 1510 | 1.07 |
| S-3X2-D | 0.315 | 7.11 | 2.83 | 952 | 1189 | 1.24 |
6. Conclusions
Acknowledgements
References
- Mastrodicasa, S. Dissesti Statici Delle Strutture Edilizie: Diagnosi, Consolidamento, Istituzioni Teoriche; Ulrico, H., Ed.; Sottotitolo: Milano, Italy, 1983. [Google Scholar]
- Ilyas, M.; Farooq, S.H.; Qazi, A.U.; Umair, R. Masonry confinement using steel strips. Pak. J. Eng. Appl. Sci. 2009, 5, 1–9. [Google Scholar]
- Krevaikas, T.D.; Triantafillou, T. Masonry confinement with fiber-reinforced polymers. J. Compos. Constr. 2005, 9, 128–135. [Google Scholar] [CrossRef]
- Triantafillou, T.C. Strengthening of masonry structures using epoxy-bonded FRP laminates. J. Compos. Constr. 1998, 2, 96–104. [Google Scholar] [CrossRef]
- Corradi, M.; Borri, A.; Grazini, A. Confinement of brick masonry columns with CFRP materials. Composites Sci. Technol. 2007, 67, 1772–1783. [Google Scholar]
- Aiello, M.A.; Sciolti, S.M. Bond analysis of masonry structures strengthened with CFRP sheets. Constr. Build. Mater. 2006, 20, 90–100. [Google Scholar]
- Micelli, F.; De Lorenzis, L.; La Tegola, A. FRP-confined masonry columns under axial loads: Experimental results and analytical model. Masonry Int. J. 2004, 17, 95–108. [Google Scholar]
- Di Ludovico, M.; D’Ambra, C.; Prota, A.; Manfredi, G. FRP confinement of tuff and clay brick columns: Experimental study and assessment of analytical models. J. Compos. Constr. 2010, 14, 583–596. [Google Scholar]
- Bieker, C.; Seim, W.; Stürz, J. Post-strengthening of masonry columns by use of fiber-reinforced polymers. In Presented at the 3rd International Conference of Composites in Infrastructure, San Francisco, CA, USA, 2002.
- ASTM D 638—10 Standard Test Method for Tensile Properties of Plastics; ASTM International—American Society for Testing and Materials: West Conshohocken, PA, USA, 1995.
- ASTM D 695M—Standard Test Method for Properties of Rigid Plastics; ASTM International—American Society for Testing and Materials: West Conshohocken, PA, USA, 1991.
- CNR-DT 200—Guide for the Design and Construction of Externally Bonded FRP Systems for Strengthening Existing Structures; Italian Council of Research (CNR): Rome, Italy, 2004.
- General rules for reinforced and unreinforced masonry structures. In EN 1996-1-1 Eurocode 6: Design of Masonry Structures; CEN—European Committee for Standardization: Brussels, Belgium, 2005; Part 1-1.
© 2011 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution license (http://creativecommons.org/licenses/by/3.0/).
Share and Cite
Borri, A.; Castori, G.; Corradi, M. Masonry Columns Confined by Steel Fiber Composite Wraps. Materials 2011, 4, 311-326. https://doi.org/10.3390/ma4010311
Borri A, Castori G, Corradi M. Masonry Columns Confined by Steel Fiber Composite Wraps. Materials. 2011; 4(1):311-326. https://doi.org/10.3390/ma4010311
Chicago/Turabian StyleBorri, Antonio, Giulio Castori, and Marco Corradi. 2011. "Masonry Columns Confined by Steel Fiber Composite Wraps" Materials 4, no. 1: 311-326. https://doi.org/10.3390/ma4010311
APA StyleBorri, A., Castori, G., & Corradi, M. (2011). Masonry Columns Confined by Steel Fiber Composite Wraps. Materials, 4(1), 311-326. https://doi.org/10.3390/ma4010311




