Electron Screening in Deuteron–Deuteron Reactions on a Zr Target with Oxygen and Carbon Contamination
Abstract
1. Introduction
2. Materials and Methods
2.1. The Experimental Setup
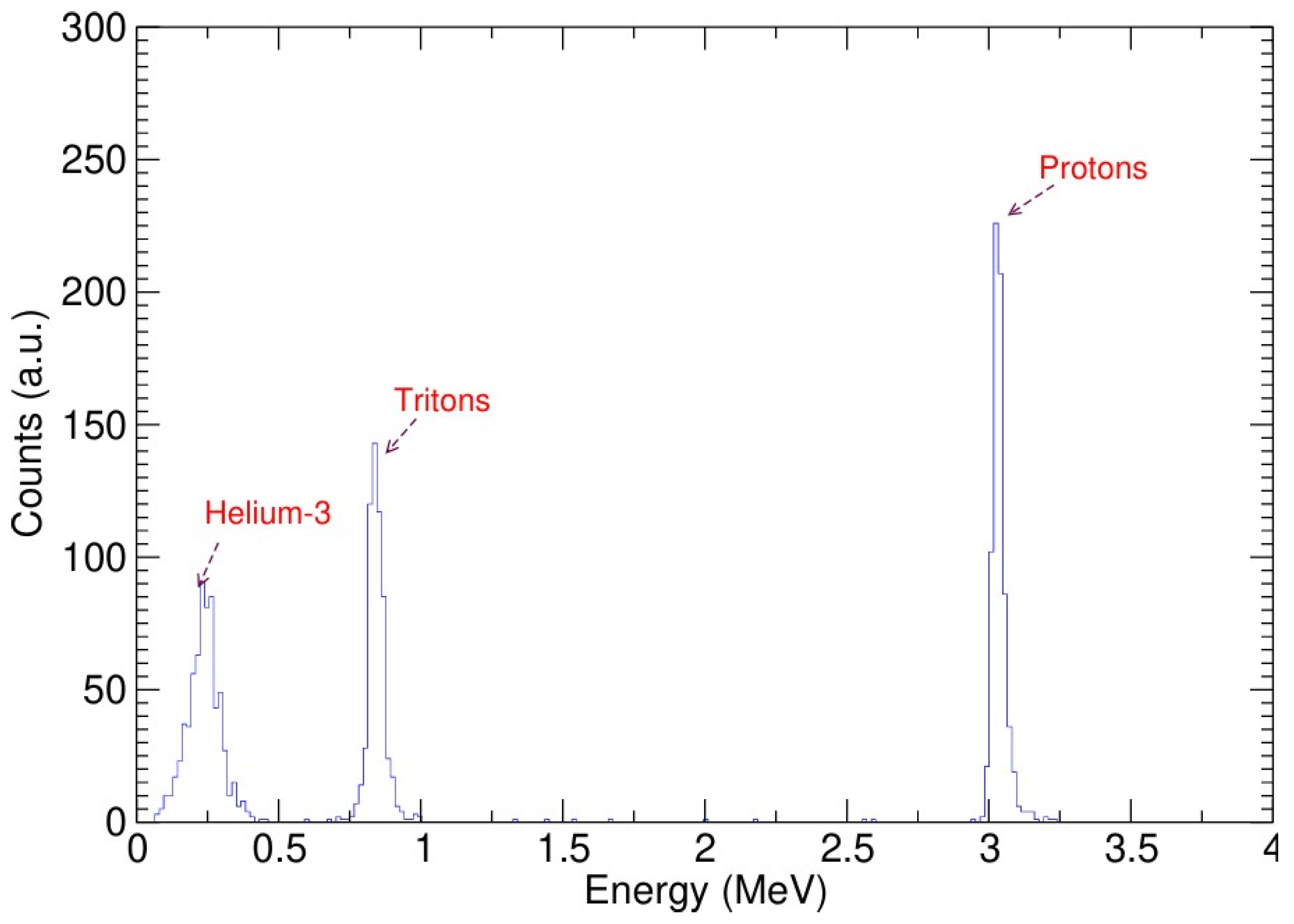
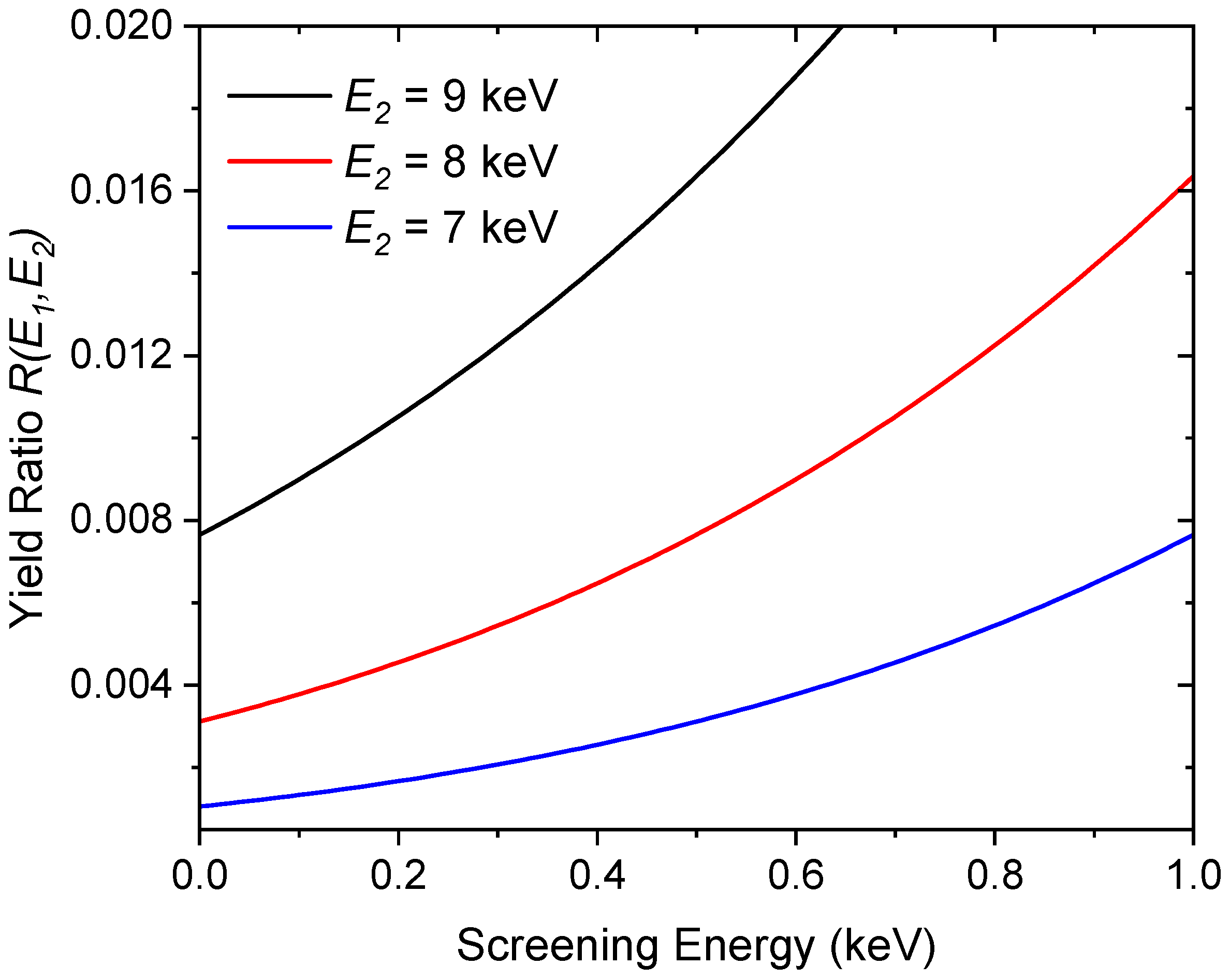
2.2. Determination of the Screening Energy Using the Two-Point Method
3. Results
3.1. Geant4 vs. SRIM/TRIM Calculations
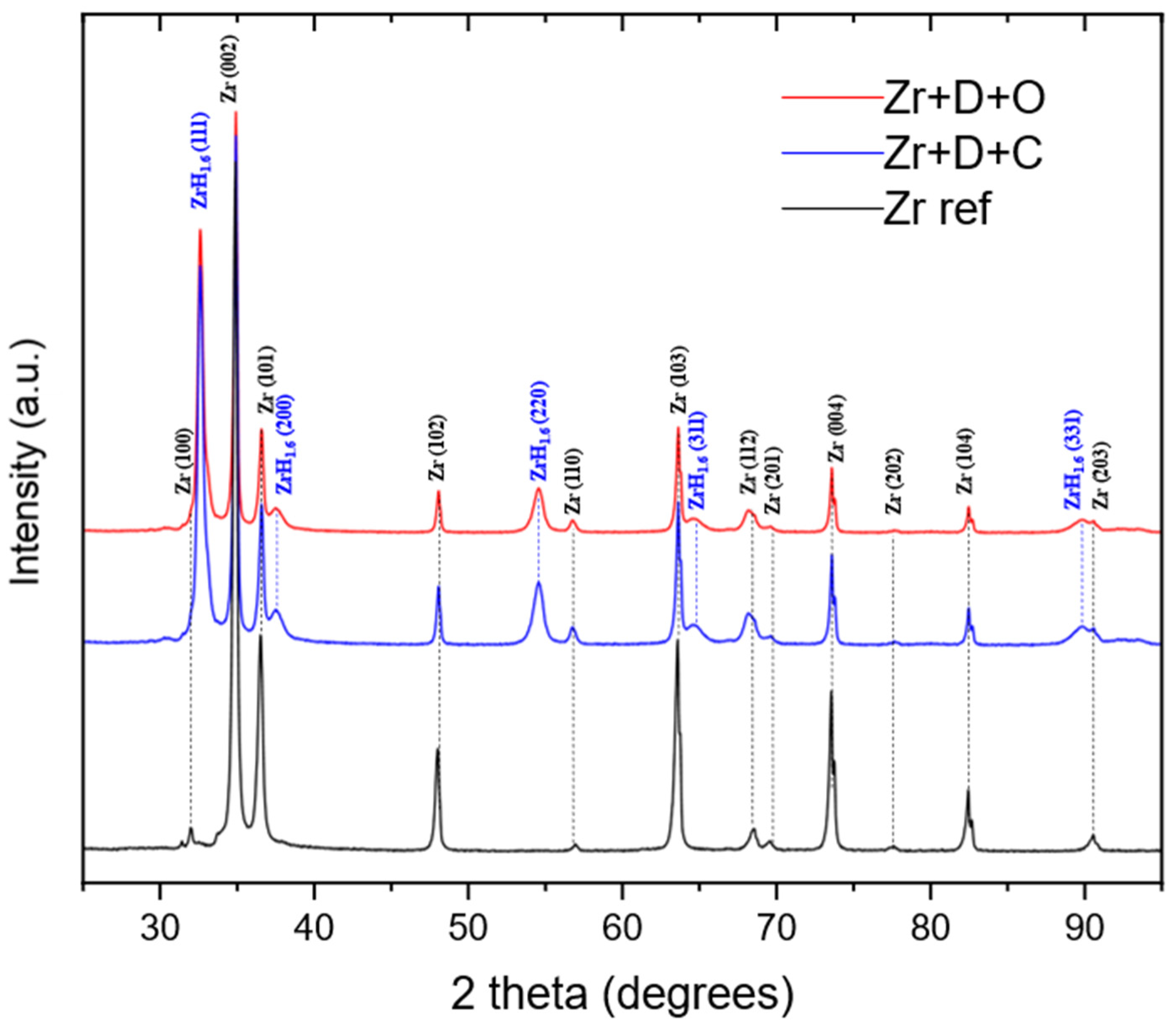
3.2. XRD Analysis
3.3. Electron Screening Energies

4. Discussion and Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Salpeter, E. Electrons Screening and Thermonuclear Reactions. Aust. J. Phys. 1954, 7, 373. [Google Scholar] [CrossRef]
- Ichimaru, S.; Kitamura, H. Pycnonuclear reactions in dense astrophysical plasmas. Phys. Plasmas 1999, 6, 2649. [Google Scholar] [CrossRef]
- Rolfs, C.; Somorjai, E. Status report on electron screening. Nucl. Instrum. Methods B 1995, 99, 297–300. [Google Scholar] [CrossRef]
- Czerski, K.; Huke, A.; Biller, A.; Heide, P.; Hoeft, M.; Ruprecht, G. Enhancement of the electron screening effect for d + d fusion reactions in metallic environments. Europhys. Lett. 2001, 54, 449–455. [Google Scholar] [CrossRef]
- Kasagi, J.; Yuki, H.; Baba, T.; Noda, T.; Ohtsuki, T.; Lipson, A.G. Strongly Enhanced DD Fusion Reaction in Metals Observed for keV D+ Bombardment. J. Phys. Soc. Jpn. 2002, 71, 2881. [Google Scholar] [CrossRef]
- Raiola, F.; Migliardi, P.; Gang, L.; Bonomo, C.; Gyürky, G.; Bonetti, R.; Broggini, C.; Christensen, N.E.; Corvisiero, P.; Cruz, J.; et al. Electron screening in d(d,p)t for deuterated metals and the periodic table. Phys. Lett. B 2002, 547, 193. [Google Scholar] [CrossRef]
- Huke, A.; Czerski, K.; Heide, P.; Ruprecht, G.; Targosz, N.; Żebrowski, W. Enhancement of deuteron-fusion reactions in metals and experimental implications. Phys. Rev. C 2008, 78, 015803. [Google Scholar] [CrossRef]
- Czerski, K.; Huke, A.; Heide, P.; Schiwietz, G. Solid-State Effects in d+d Fusion Reactions. Nucle. Instr. Meth. B 2002, 93, 183–187. [Google Scholar] [CrossRef]
- Ichimaru, S.; Tanaka, S. Theory of interparticle correlations in dense, high-temperature plasmas. Phys. Rev. A 1985, 32, 1790. [Google Scholar] [CrossRef]
- Czerski, K.; Huke, A.; Heide, P.; Ruprecht, G. The 2H(d,p)3H reaction in metallic media at very low energies. Europhys. Lett. 2004, 68, 363–369. [Google Scholar] [CrossRef]
- Raiola, F.; Burchard, B.; Fülöp, Z.; Gyürky, G. Enhanced d(d,p)t fusion reaction in metals. Eur. Phys. J. A 2006, 27, 79. [Google Scholar] [CrossRef]
- Bystritskii, V.M.; Bystritskii, V.M.; Dudkin, G.N.; Filipowicz, M.; Gazi, S.; Huran, J.; Kobzev, A.P.; Mesyats, G.A.; Nechaev, B.A.; Padalko, V.N.; et al. Investigation of temperature dependence of neutron yield and electron screening potential for the d(d,n)3He reaction proceeding in deuterides ZrD2 and TiD2. Phys. At. Nucl. 2012, 75, 913–922. [Google Scholar]
- Czerski, K.; Weissbach, D.; Kilic, A.I.; Ruprecht, G.; Huke, A.; Kaczmarski, M.; Targosz-Ślęczka, N.; Maass, K. Screening and resonance enhancements of the 2H(d,p)3H reaction yield in metallic environments. Europhys. Lett. 2016, 113, 22001. [Google Scholar] [CrossRef]
- Cvetinović, A.; Đeorđić, D.; Guardo, G.; Kelemen, M.; La Cognata, M.; Lamia, L.; Markelj, S.; Mikac, U.; Pizzone, R.; Schwarz-Selinger, T.; et al. Electron Screening in Palladium. Phys. Lett. B 2023, 838, 137684. [Google Scholar] [CrossRef]
- Cventovic, A.; Lipoglavsek, M.; Markelj, S.; Vesic, J. Molecular Screening in nuclear reactions. Phys. Rev. C 2015, 92, 065801. [Google Scholar]
- Kowalska, A.; Czerski, K.; Horodek, P.; Siemek, K.; Kaczmarski, M.; Targosz-Ślęczka, N.; Valat, M.; Dubey, R.; Pyszniak, K.; Turek, M.; et al. Crystal lattice defects in deuterated Zr in presence of O and C impurities studied by PAS and XRD for electron screening. Materials 2023, 16, 6255. [Google Scholar] [CrossRef]
- Agostinelli, S.; Allison, J.; Amako, K.A.; Apostolakis, J.; Araujo, H.; Arce, P.; Asai, M.; Axen, D.; Banerjee, S.; Barrand, G.J.N.I.; et al. Geant4—A Simulation Toolkit. Nucl. Instrum. Meth. A 2003, 506, 250–303. [Google Scholar] [CrossRef]
- Ziegler, J.F.; Ziegler, M.D.; Biersack, J.P. The stopping and range of ions in matter. Nucl. Instrum. Meth. Phys. Res. B 2010, 268, 1818–1823. [Google Scholar] [CrossRef]
- Malvern Pananalytical. Available online: https://www.malvernpanalytical.com/ (accessed on 1 December 2024).
- Waseda, Y.; Matsuba, E.; Shinoda, K. X-Ray Diffraction Crystallography, Introduction, Examples and Solved Problems, 1st ed.; Springer: Berlin/Heidelberg, Germany, 2011; pp. 134–137. [Google Scholar]
- Maxwell, C.; Pencer, J.; Torres, E. Atomistic simulation study of clustering and evolution of irradiation induced defects in zirconium. J. Int. Mater. 2020, 531, 151979. [Google Scholar] [CrossRef]
- Czerski, K. Deuteron-deuteron nuclear energies at extremely low energies. Phys. Rev. C 2022, 106, L011601. [Google Scholar] [CrossRef]
- You, D.; Ganorkar, S.; Joo, M.; Park, D.; Kim, S.; Kang, K.; Lee, D. Ab Initio Study of H, B, C, N, O, and Self-Interstitial Atoms in Hcp-Zr. J. Alloys Compd. 2019, 787, 631–637. [Google Scholar] [CrossRef]
- Feng, M.; Liu, G.; Liu, Z.; Hu, W.; He, X.; Deng, H. Interaction between impurity elements (C, N, and O) and hydrogen in hcp-Zr: A first-principle study. Model. Simul. Mater. Sci. Eng. 2020, 28, 085007. [Google Scholar] [CrossRef]
- Kittel, C. Chapter 20: Point defects. In Introduction to Solid State Physics, 8th ed.; Stuart, J., Ed.; John Wiley & Sons: New York, NY, USA, 2005; pp. 583–618. [Google Scholar]

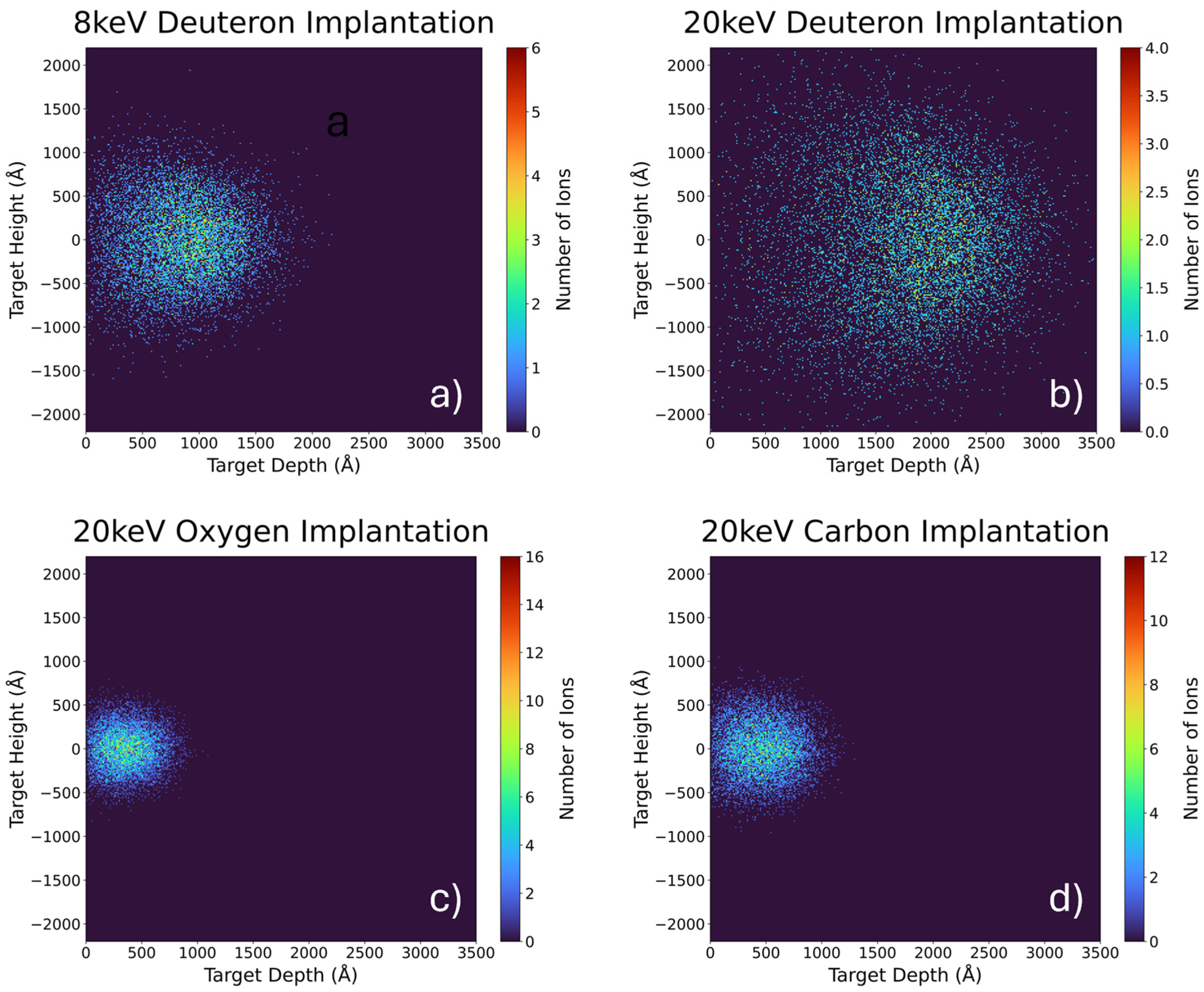
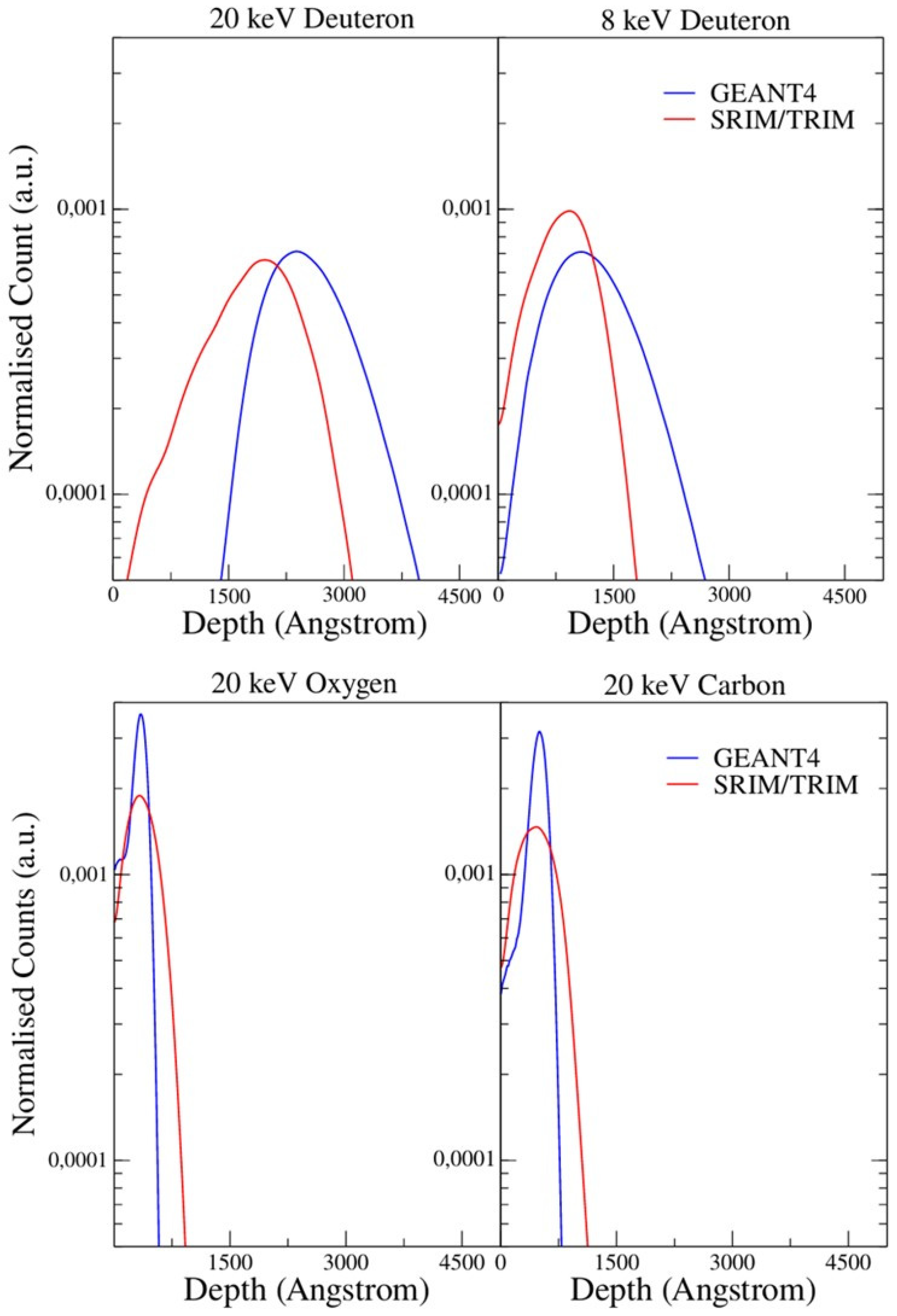
| Implanted Ion | Mean Range in SRIM [Å] | Mean Range in Geant4 [Å] |
|---|---|---|
| Deuteron at 20 keV | 1800 | 2500 |
| Deuteron at 8 keV | 860 | 1250 |
| Oxygen at 20 keV | 370 | 290 |
| Carbon at 20 keV | 470 | 446 |
| Target | a [Å] | c [Å] |
|---|---|---|
| Zr (reference) | 3.208 | 5.217 |
| Zr implanted with D | 3.209 | 5.243 |
| Zr implanted with D and O | 3.195 | 5.302 |
| Zr implanted with D and C | 3.195 | 5.294 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kowalska, A.; Kaczmarski, M.; Czerski, K.; Dubey, R.; Das Haridas, G.; Valat, M.; Targosz-Ślęczka, N.; Figiel, P.; Słowik, J.; Baranowska, J. Electron Screening in Deuteron–Deuteron Reactions on a Zr Target with Oxygen and Carbon Contamination. Materials 2025, 18, 1331. https://doi.org/10.3390/ma18061331
Kowalska A, Kaczmarski M, Czerski K, Dubey R, Das Haridas G, Valat M, Targosz-Ślęczka N, Figiel P, Słowik J, Baranowska J. Electron Screening in Deuteron–Deuteron Reactions on a Zr Target with Oxygen and Carbon Contamination. Materials. 2025; 18(6):1331. https://doi.org/10.3390/ma18061331
Chicago/Turabian StyleKowalska, Agata, Mateusz Kaczmarski, Konrad Czerski, Rakesh Dubey, Gokul Das Haridas, Mathieu Valat, Natalia Targosz-Ślęczka, Paweł Figiel, Justyna Słowik, and Jolanta Baranowska. 2025. "Electron Screening in Deuteron–Deuteron Reactions on a Zr Target with Oxygen and Carbon Contamination" Materials 18, no. 6: 1331. https://doi.org/10.3390/ma18061331
APA StyleKowalska, A., Kaczmarski, M., Czerski, K., Dubey, R., Das Haridas, G., Valat, M., Targosz-Ślęczka, N., Figiel, P., Słowik, J., & Baranowska, J. (2025). Electron Screening in Deuteron–Deuteron Reactions on a Zr Target with Oxygen and Carbon Contamination. Materials, 18(6), 1331. https://doi.org/10.3390/ma18061331






