Stability Analysis of Free Vibration of Gun Drill Rod
Highlights
- In this study, we present a Rayleigh rotor model for gun drill rods with asymmetric cross-sections.
- We identify distinct unstable speed ranges.
- Stability is considered based on the effects of aspect ratio, cutting fluid inlet, and chip groove dimensions.
- Our results confirm the reliability of using ANSYS simulation.
- The study provides engineering insights for gun drill rod design optimization.
Abstract
1. Introduction
2. Materials and Methods
2.1. Mechanical Model
2.2. Assumptions of the Rayleigh Rotor Model
- Small Displacements: The model assumes that the displacements of the rotor are small relative to its dimensions, allowing for linearization of the governing equations.
- Isotropic Material: The drill pipe is assumed to be made of an isotropic material, meaning its mechanical properties are uniform in all directions.
- Negligible Shear Deformation: The model neglects shear deformation, focusing primarily on bending vibrations.
- No Damping: The initial analysis assumes no external damping; however, damping effects can be incorporated in subsequent studies.
- Constant Rotational Speed: The rotor is assumed to rotate at a constant angular velocity, neglecting transient effects.
- These assumptions simplify the analysis while still providing accurate insights into the dynamic behavior of the gun drill rod.
2.3. Vibration Equation of the Rayleigh Rotor for Gun Drill Rod
2.4. Justification of Galerkin Method
- Accuracy: The Galerkin method provides high accuracy in approximating the solutions of PDEs by projecting the problem onto a finite-dimensional subspace spanned by a set of basis functions.
- Flexibility: The method allows for the use of various basis functions, enabling the capture of complex vibration modes in asymmetric rotors.
- Efficiency: By transforming the PDEs into a system of ordinary differential equations (ODEs), the Galerkin method significantly reduces computational complexity while maintaining the essential dynamics of the system.
- Validation: The method has been widely validated in rotor dynamics and structural vibration analysis, making it a reliable choice for this study.
3. Results
3.1. Reliability Analysis
3.2. Influence of Dimensional Factors on Vibration Stability
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A
Appendix B
Appendix B.1
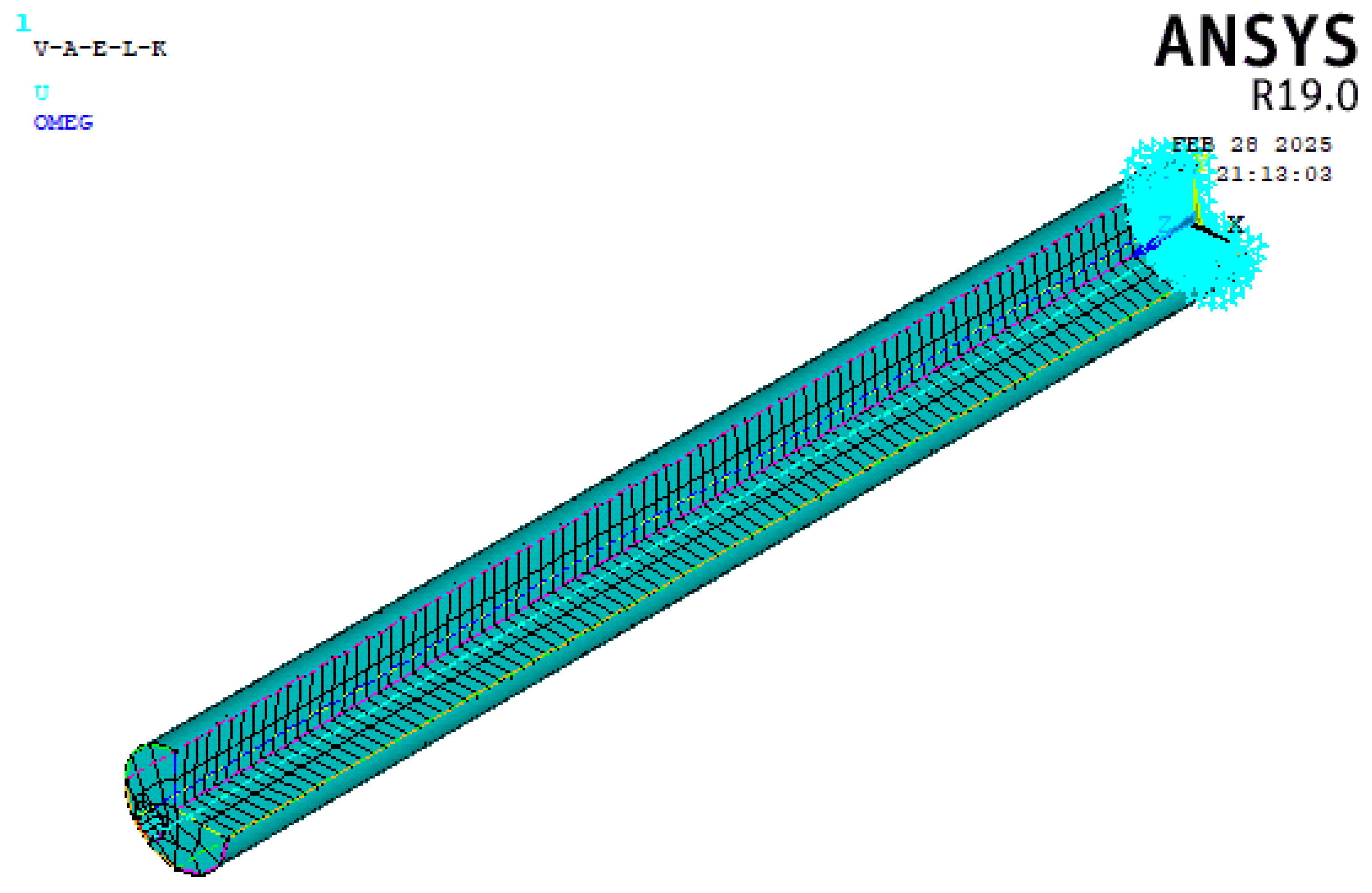
Appendix B.2
References
- Zapoměl, J. Special Issue on Rotor Dynamics: Theoretical Analysis, Computer and Experimental Modelling, Measurements. Appl. Sci. 2023, 13, 11551. [Google Scholar] [CrossRef]
- Khader, N. Stability Analysis for the Dynamic Design of Rotors. J. Sound Vib. 1997, 207, 287–299. [Google Scholar] [CrossRef]
- Rao, J.S.; Sreenivas, R. Dynamics of Asymmetric Rotors using Solid Models. In Proceedings of the International Gas Turbine Congress, Tokyo, Japan, 2–7 November 2003; pp. 1–6. [Google Scholar]
- You, H.Y.; Yang, S.C.; Yue, C.X. Application and Technology Research in Deep-Hole Drilling System. Mater. Sci. Forum 2014, 800–801, 506–510. [Google Scholar] [CrossRef]
- Gao, C.H.; Cheng, K.; Kirkwood, D. The Investigation on the Machining Process of BTA Deep Hole Drilling. J. Mater. Process. Technol. 2000, 107, 222–227. [Google Scholar] [CrossRef]
- Klocke, F.; Abouridouane, M.; Gerschwiler, K. 3D Modelling and Simulation of Gun Drilling. Adv. Mater. Res. 2011, 223, 12–19. [Google Scholar] [CrossRef]
- Lou, J.; Li, L.; Dong, X. Research on the Influencing Factors of Chip Forming in 304 Stainless Steel Deep Hole Gun Drilling. Int. J. Adv. Manuf. Technol. 2024, 134, 1447–1461. [Google Scholar] [CrossRef]
- Song, O.; Kim, S.K. Dynamic Responses of Composite H-Type Cross-Section Beams. In The Proceedings of The Computational Mechanics Conference 2010.23; The Japan Society of Mechanical Engineers: Sapporo, Japan, 2010; pp. 600–602. [Google Scholar] [CrossRef]
- Kim, K.T. Free Vibration of Composite Thin-Walled Beams with Chordwise Asymmetric Closed Cross-Sections. Int. J. Aerosp. Eng. 2019, 2019, 7503097. [Google Scholar] [CrossRef]
- Chattopadhyay, S. Warping Deformation Caused by Twisting Non-circular Shafts. In Proceedings of the 2015 ASEE Annual Conference & Exposition, Seattle, WA, USA, 14–17 June 2015. [Google Scholar] [CrossRef][Green Version]
- Jamshidi, P.; Jafari, A.A. Analytical Investigation on Nonlinear Vibration Behavior of an Unbalanced Asymmetric Rotor using the Method of Multiple Scales. J. Braz. Soc. Mech. Sci. Eng. 2019, 41, 456. [Google Scholar] [CrossRef]
- Bavi, R.; Mohammad-Sedighi, H.; Hajnayeb, A. Parametric Resonance and Bifurcation Analysis of Thin-Walled Asymmetric Gyroscopic Composite Shafts: An Asymptotic Study. Thin-Walled Struct. 2023, 184, 110508. [Google Scholar] [CrossRef]
- Binghao, L.; Chuanzhen, H.; Zhengyi, T. Effect of Drilling Parameters on the Hole Surface Integrity of Low Alloy Steel for Nuclear Power during BTA Deep Hole Drilling. Int. J. Adv. Manuf. Technol. 2023, 127, 565–577. [Google Scholar] [CrossRef]
- Strodick, S.; Schmidt, R.; Donnerbauer, K. Subsurface Conditioning in BTA Deep Hole Drilling for Improved Component Performance. Prod. Eng. Res. Dev. 2024, 18, 299–317. [Google Scholar] [CrossRef]
- Biermann, D.; Schmidt, R.; Strodick, S. Numerical Modelling of the BTA Deep Hole Drilling Process. Procedia CIRP 2024, 123, 470–475. [Google Scholar] [CrossRef]
- Licheng, L.; Can, N.; Liu, G. Analysis and Development of the CFRP Boring Bar for Stability Improvement. Fibers Polym. 2023, 24, 4413–4427. [Google Scholar] [CrossRef]
- Orgiyan, A.; Ivanov, V.; Tonkonogyi, V. The Efficiency of Dynamic Vibration Dampers for Fine Finishing Boring. In Proceedings of the International Conference on Advanced Manufacturing Engineering; Springer: Cham, Switzerland, 2023. [Google Scholar] [CrossRef]
- Li, L.; Sun, B.; Hua, H. Analysis of the Vibration Characteristics of a Boring Bar with a Variable Stiffness Dynamic Vibration Absorber. Shock Vib. 2019, 2019, 5284194. [Google Scholar] [CrossRef]
- Bonda, A.; Srinivas, J.; Nanda, B.K. Investigation of Stability in Internal Turning using a Boring Bar with a Passive Constrained Layer Damping. FME Trans. 2021, 49, 384–394. [Google Scholar]
- Hayati, S.; Shahrokhi, M.; Hedayati, A. Development of a Frictionally Damped Boring Bar for Chatter Suppression in Boring Process. Int. J. Adv. Manuf. Technol. 2021, 113, 2761–2778. [Google Scholar] [CrossRef]
- Prabhu, L.; Kumar, S.S.; Dinakaran, D. Improvement of Chatter Stability in Boring Operations with Semi Active Magneto-Rheological Fluid Damper. Mater. Today Proc. 2020, 33, 420–427. [Google Scholar] [CrossRef]
- Hou, J.; Niu, J.; Shen, Y. Dynamic Analysis and Vibration Control of Two-Degree-of-Freedom Boring Bar with Fractional-Order Model of Magnetorheological Fluid. J. Vib. Control 2022, 28, 3001–3018. [Google Scholar] [CrossRef]
- Quanbin, Z.; Wu, Z.; Shuangxi, Y. Transverse Vibration of the Boring Bar for BTA Deep Hole Machining under Stochastic Excitation. J. Mech. Sci. Technol. 2023, 37, 5635–5648. [Google Scholar] [CrossRef]
- Li, L.; Yang, D.L.; Cui, Y.M. Optimization of Machining Performance in Deep Hole Boring: A Study on Cutting Tool Vibration and Dynamic Vibration Absorber Design. Adv. Prod. Eng. Manag. 2023, 18, 371–380. [Google Scholar] [CrossRef]
- Bayly, P.V.; Metzler, S.A.; Schaut, A.J. Theory of Torsional Chatter in Twist Drills: Model, Stability Analysis and Composition to Test. J. Manuf. Sci. Eng. 2001, 123, 552–561. [Google Scholar] [CrossRef]
- Ouyang, H.; Wang, M. A Dynamic Model for a Rotating Beam Subjected to Axially Moving Forces. J. Sound Vib. 2007, 308, 674–682. [Google Scholar] [CrossRef]
- Sabuncu, M.; Evran, K. The Dynamic Stability of a Rotating Asymmetric Cross-Section Timoshenko Beam Subjected to Lateral Parametric Excitation. Finite Elem. Anal. Des. 2006, 42, 454–469. [Google Scholar] [CrossRef]
- Zhang, J.; Zhang, W.; Zhang, Y.F. Nonlinear Resonant Responses of Hyperelastic Cylindrical Shells with Initial Geometric Imperfections. Chaos Solitons Fractals 2023, 173, 113709. [Google Scholar] [CrossRef]
- Zou, R.; Luo, S.; Ma, W. A Study of the Compression Stability of a Coupler under Initial Asymmetric Conditions. Proc. Inst. Mech. Eng. Part F J. Rail Rapid Transit 2022, 236, 695–702. [Google Scholar] [CrossRef]
- Huang, Y.M.; Yang, M.L. Dynamic Analysis of a Rotating Beam Subjected to Repeating Axial and Transverse Forces for Simulating a Lathing Process. Int. J. Mech. Sci. 2009, 51, 256–268. [Google Scholar] [CrossRef]
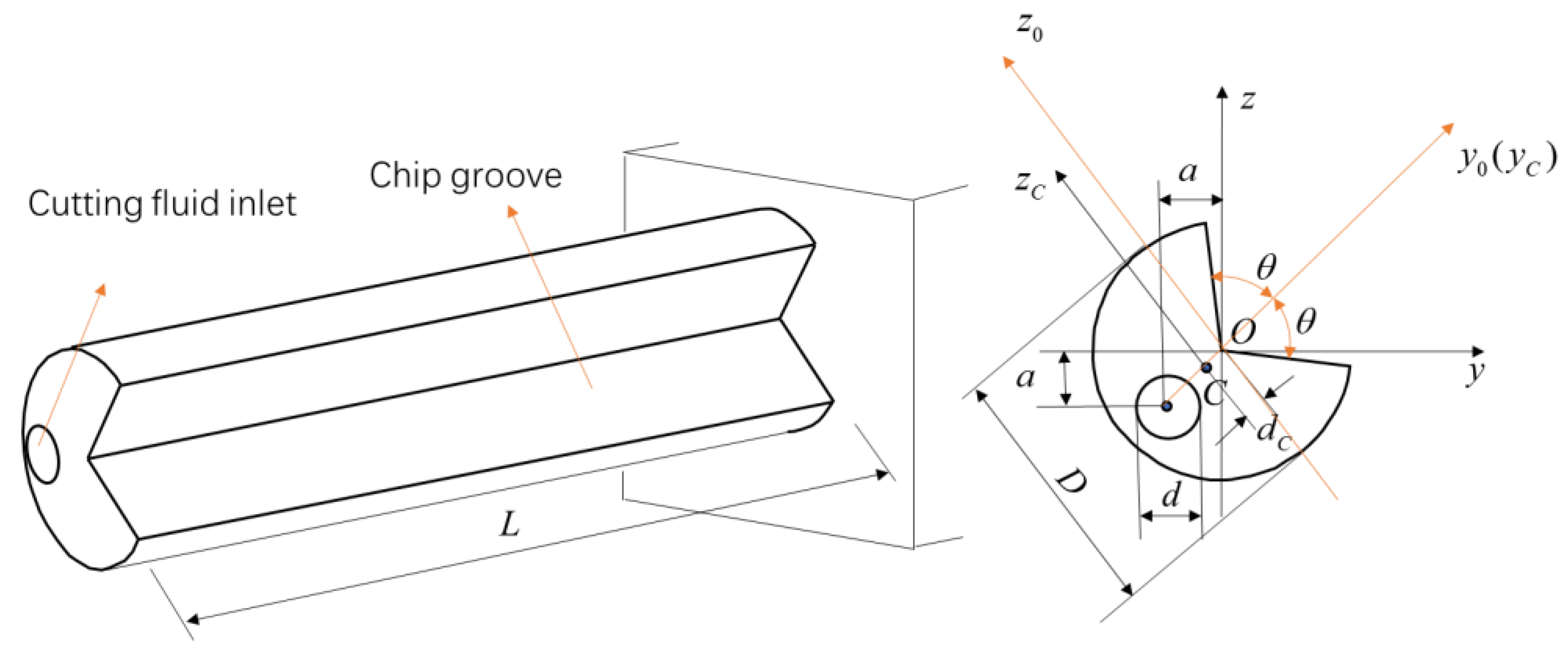
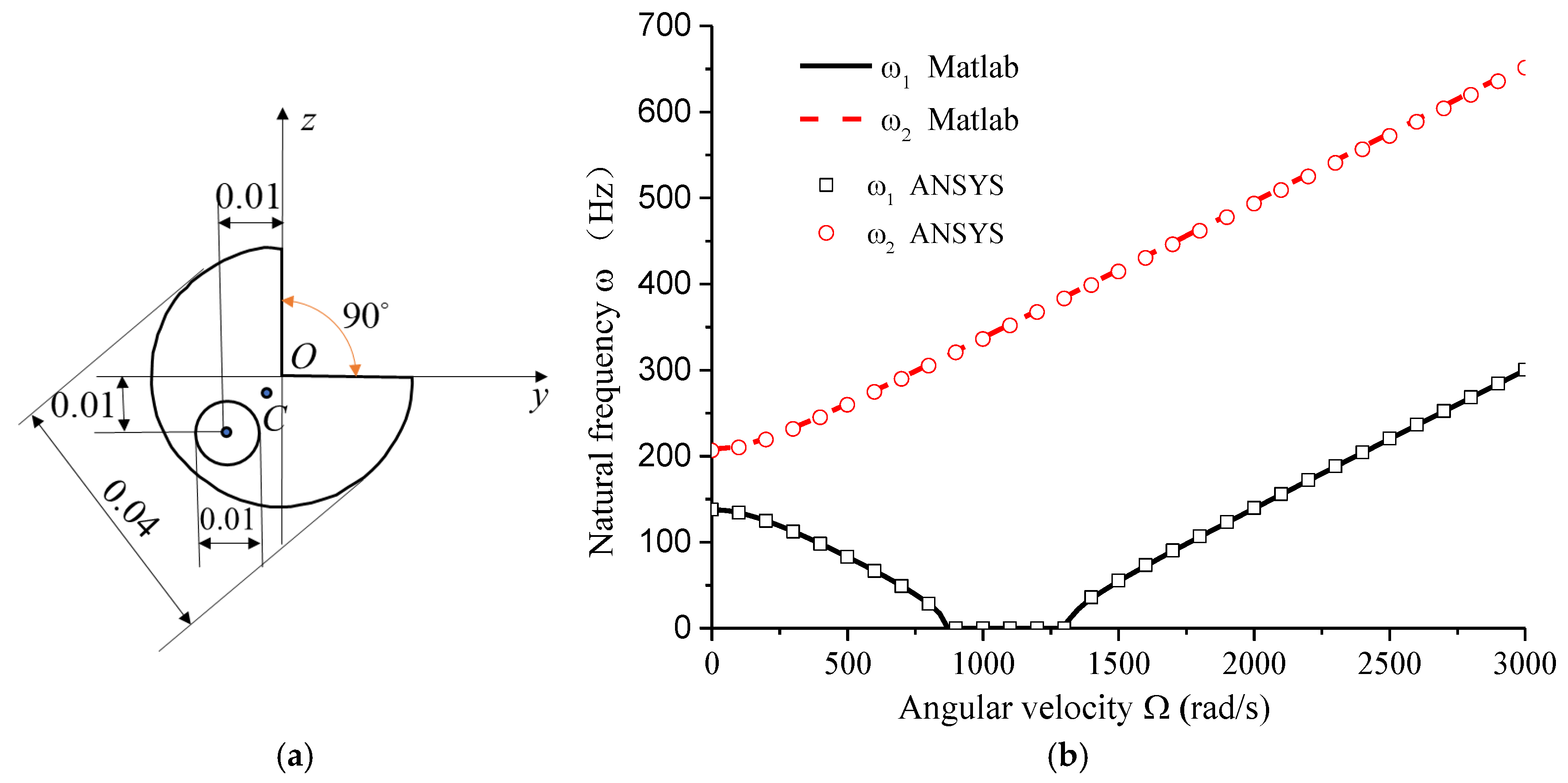
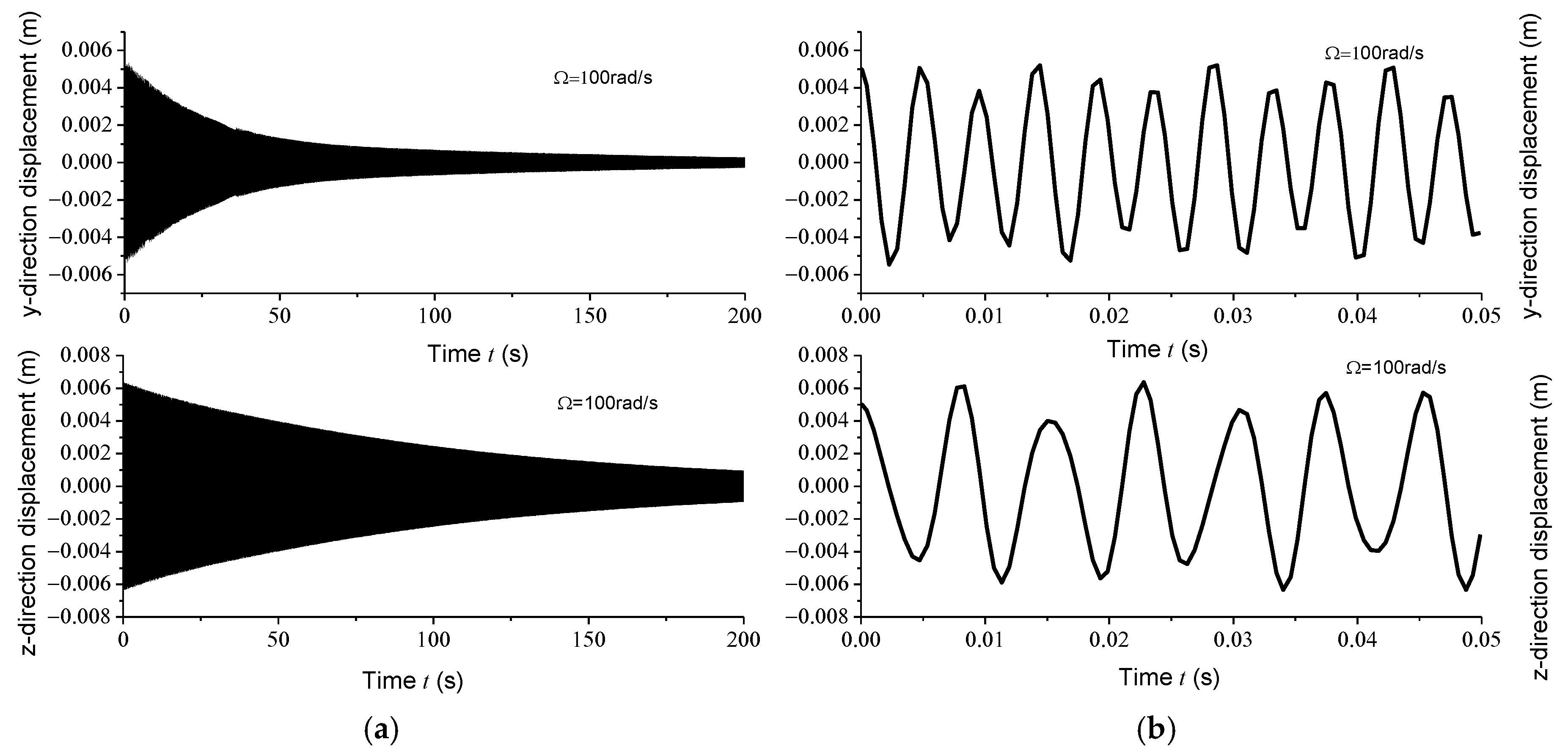
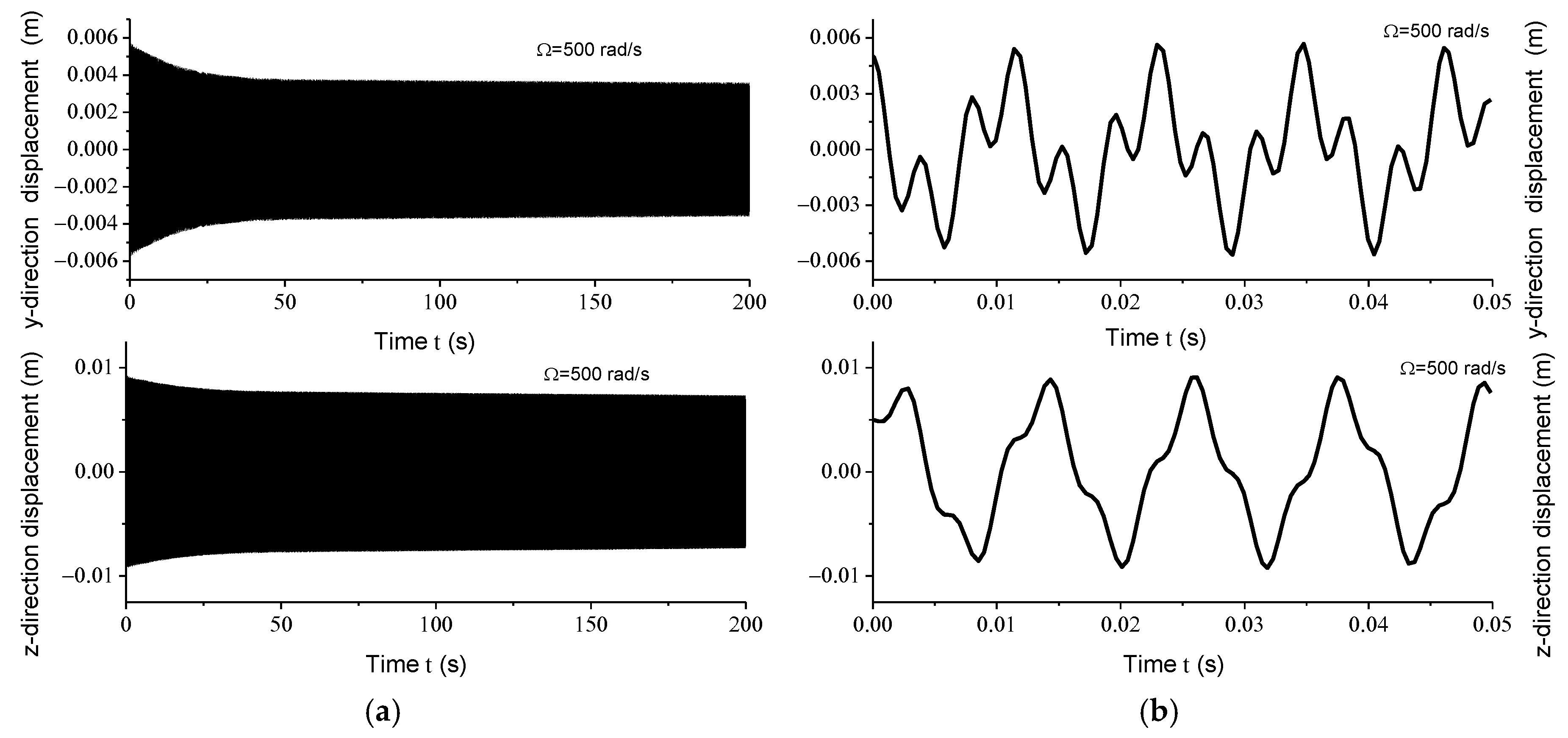
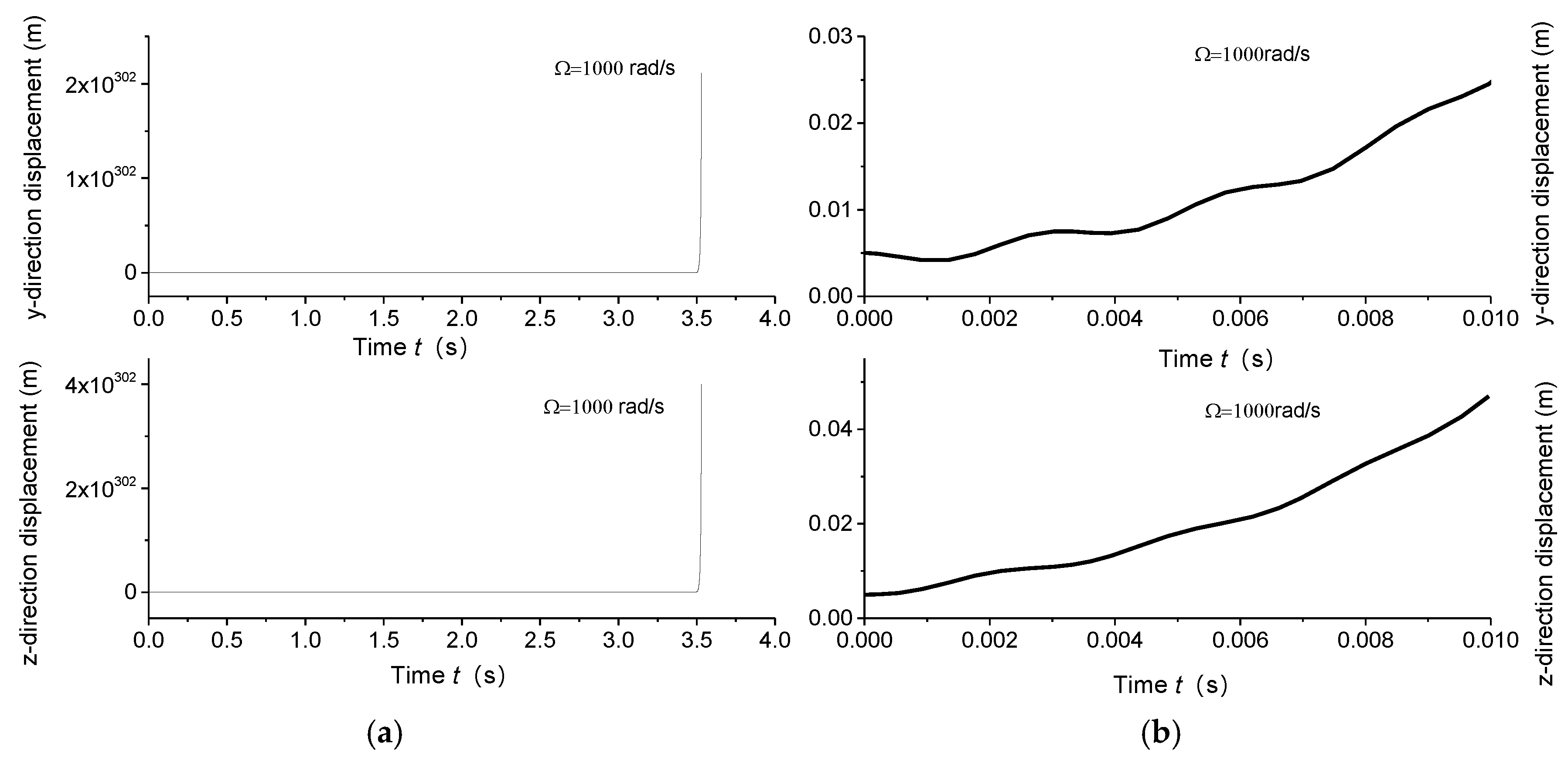
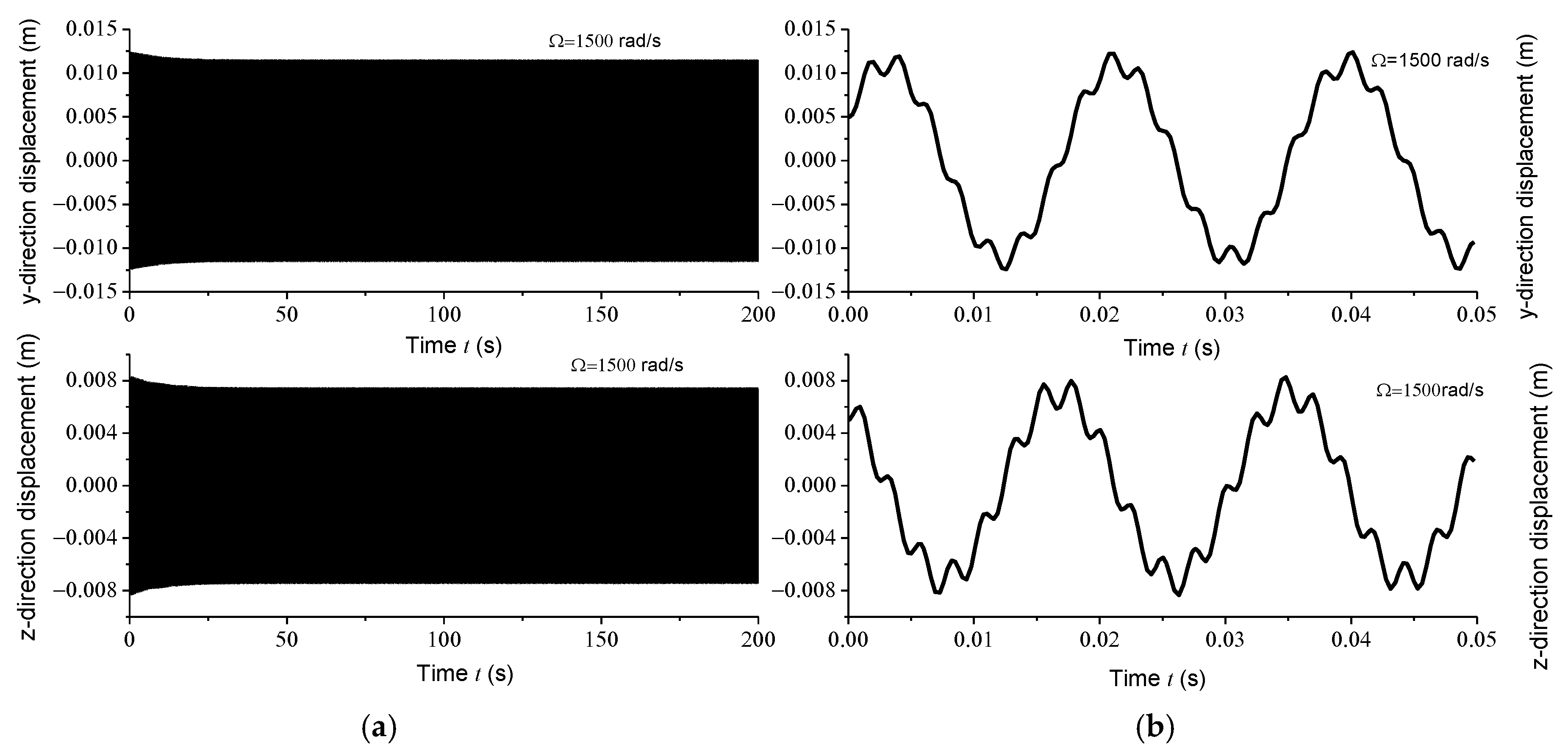
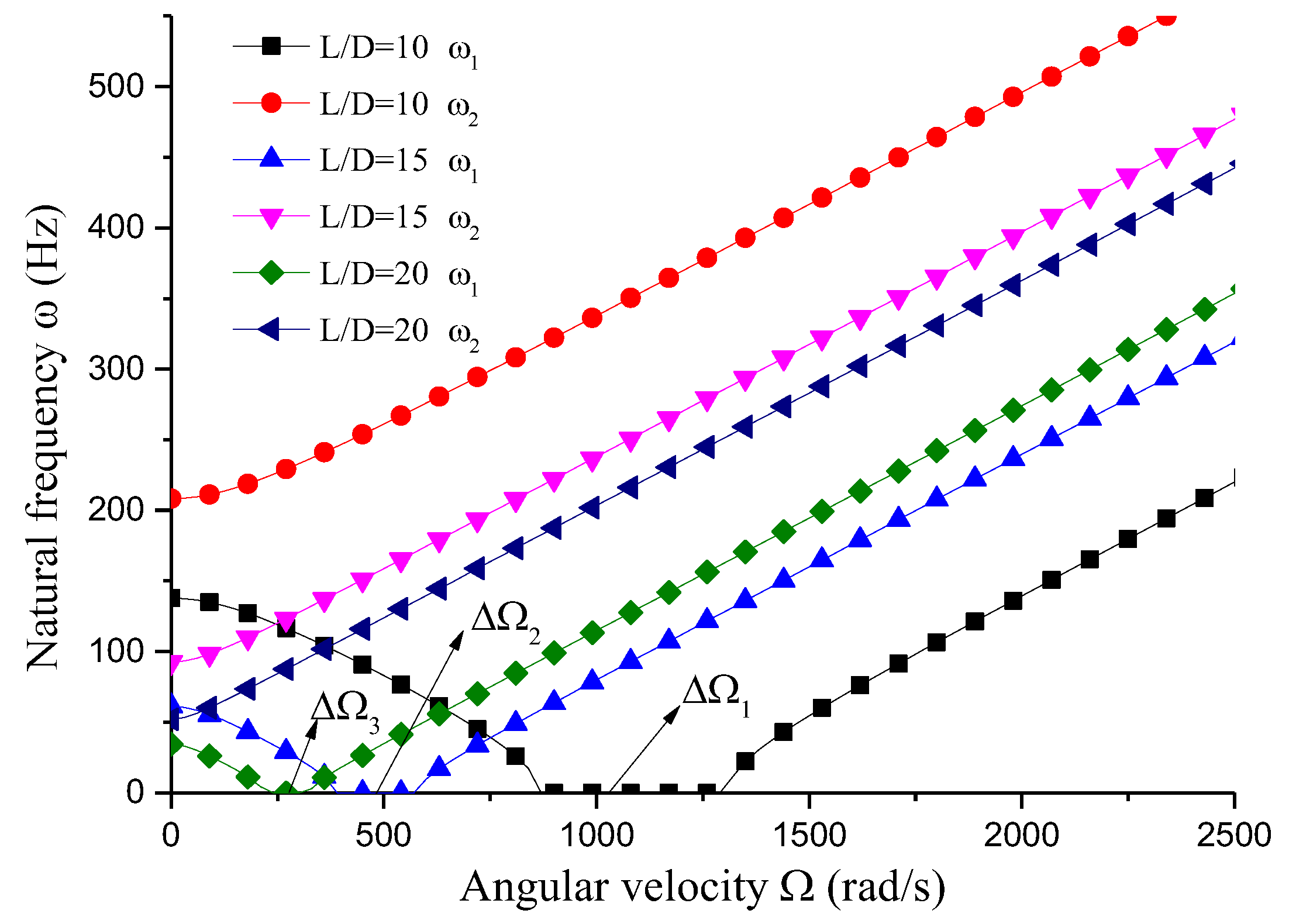
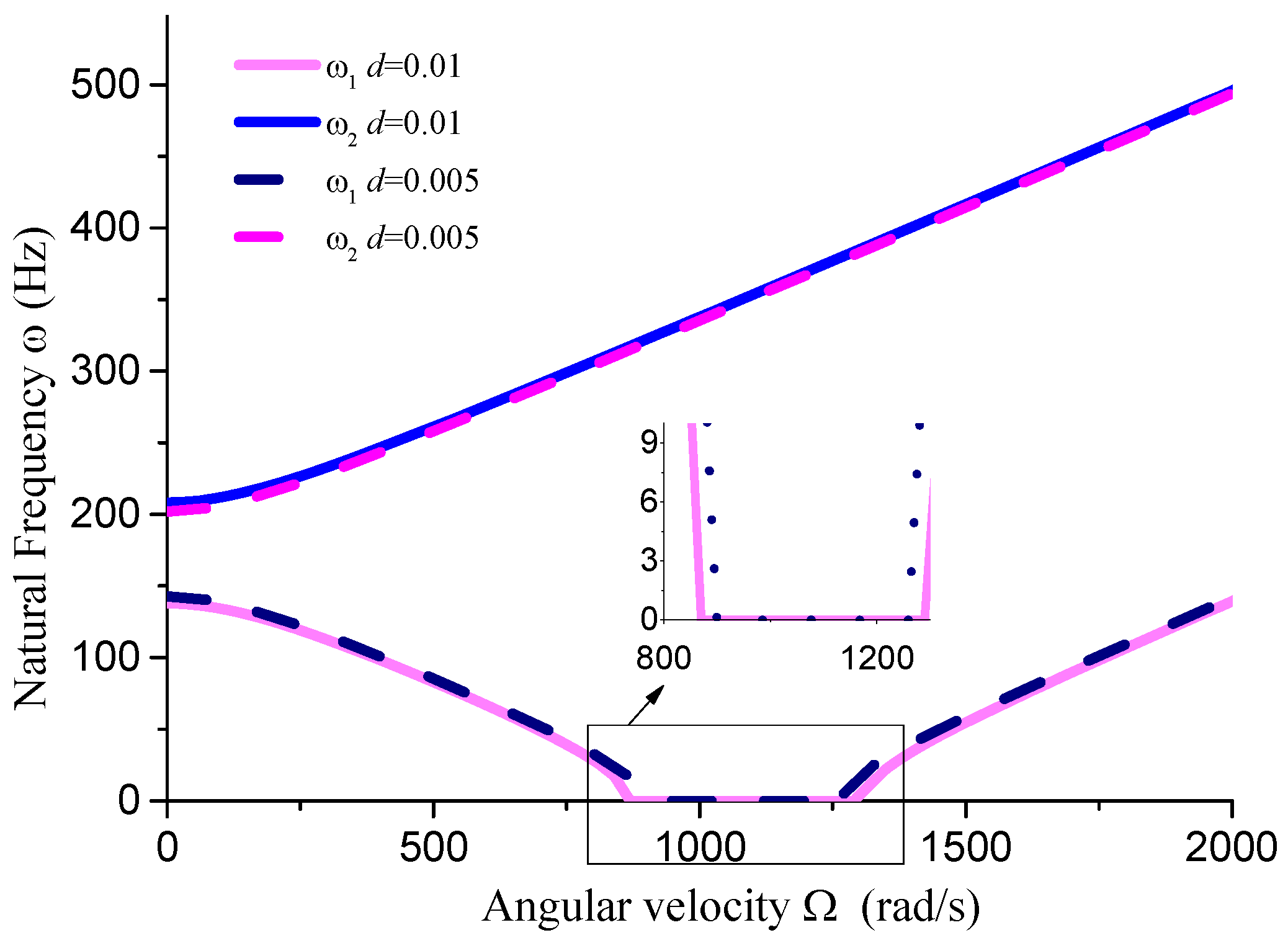

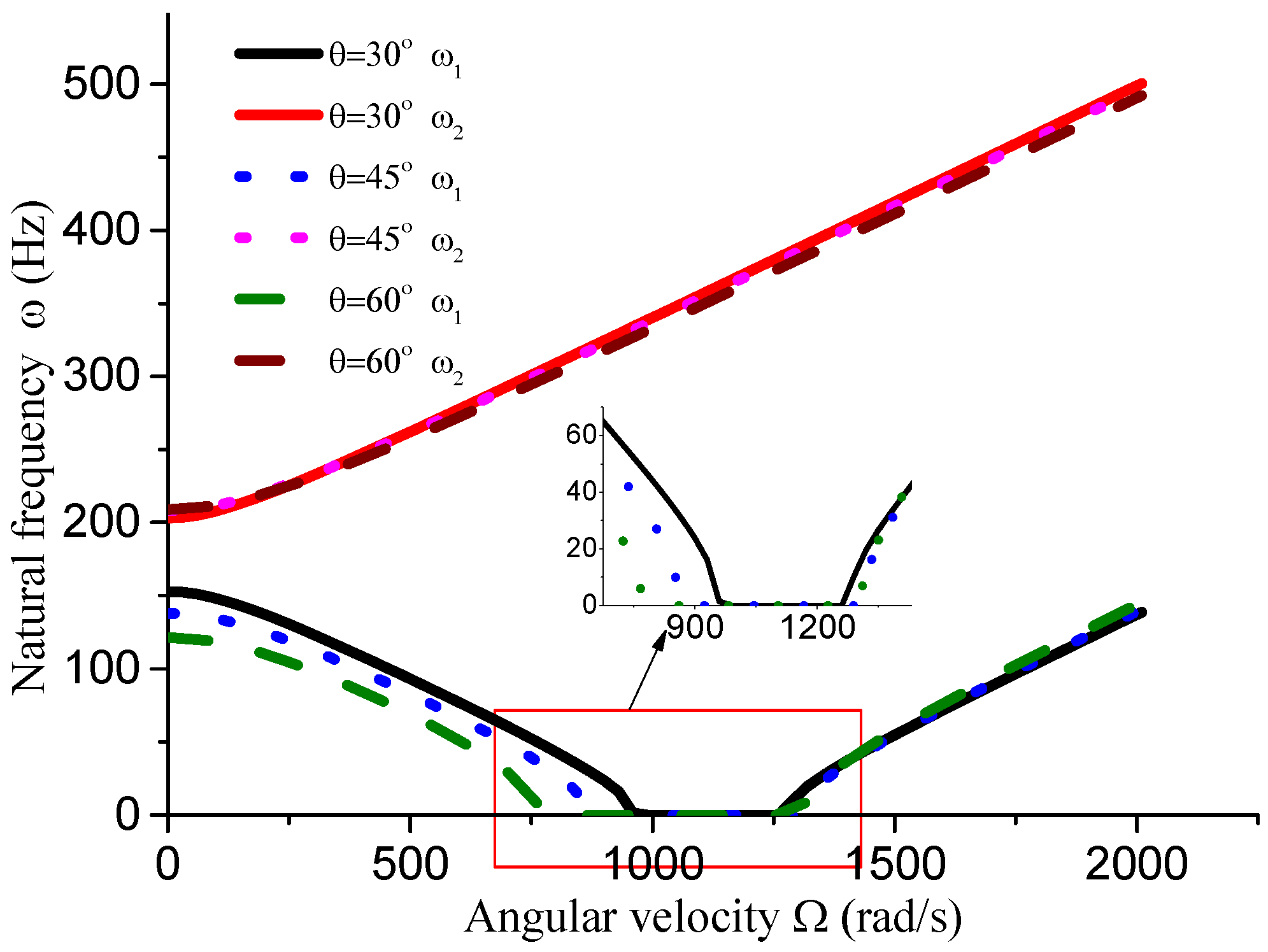
| Dimensional Parameters | ω1 (Hz, Ω = 0) | ω2 (Hz, Ω = 0) | ∆Ω (rad/s) |
|---|---|---|---|
| d = 0.01 m a = 0.01 m θ = 45° L = 0.4 m | 137.9 | 208.3 | 441 |
| d = 0.005 m a = 0.01 m θ = 45° L = 0.4 m | 142.7 | 202 | 371 |
| d = 0.01 m a = 0.005 m θ = 45° L = 0.4 m | 148.53 | 208.3 | 374 |
| d = 0.01 m a = 0.01 m θ = 30° L = 0.4 m | 152.85 | 203.35 | 316 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ma, J.; Yao, W. Stability Analysis of Free Vibration of Gun Drill Rod. Materials 2025, 18, 1241. https://doi.org/10.3390/ma18061241
Ma J, Yao W. Stability Analysis of Free Vibration of Gun Drill Rod. Materials. 2025; 18(6):1241. https://doi.org/10.3390/ma18061241
Chicago/Turabian StyleMa, Jingmin, and Wenli Yao. 2025. "Stability Analysis of Free Vibration of Gun Drill Rod" Materials 18, no. 6: 1241. https://doi.org/10.3390/ma18061241
APA StyleMa, J., & Yao, W. (2025). Stability Analysis of Free Vibration of Gun Drill Rod. Materials, 18(6), 1241. https://doi.org/10.3390/ma18061241









