Tensile Properties and Constitutive Model of BFRP–Steel–BFRP Composite Plates
Highlights
- Bonding BFRP layers enhances the tensile stiffness and yield strength of steel plates.
- The composite plates exhibit bilinear stress–strain behavior, maintaining ductility and stability.
- A validated three-segment model can accurately predict elastic, strengthening, and residual phases.
Abstract
1. Introduction
2. Specimen Design and Preparation
2.1. Details of Specimen
2.2. Preparation Process
3. Material Properties and Test Layout
4. Results Discussion
4.1. Failure Modes
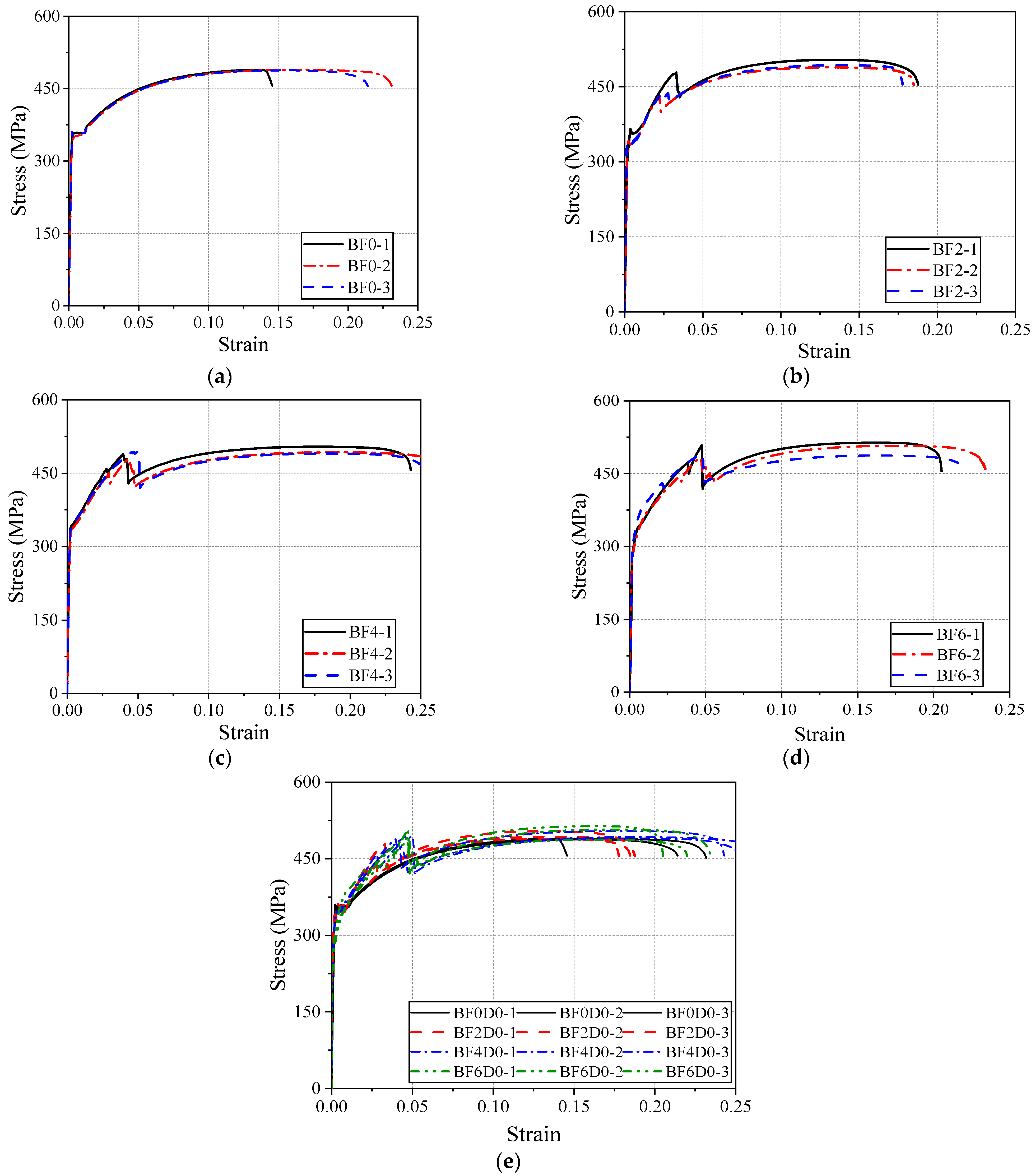
4.2. Stress–Strain Curves
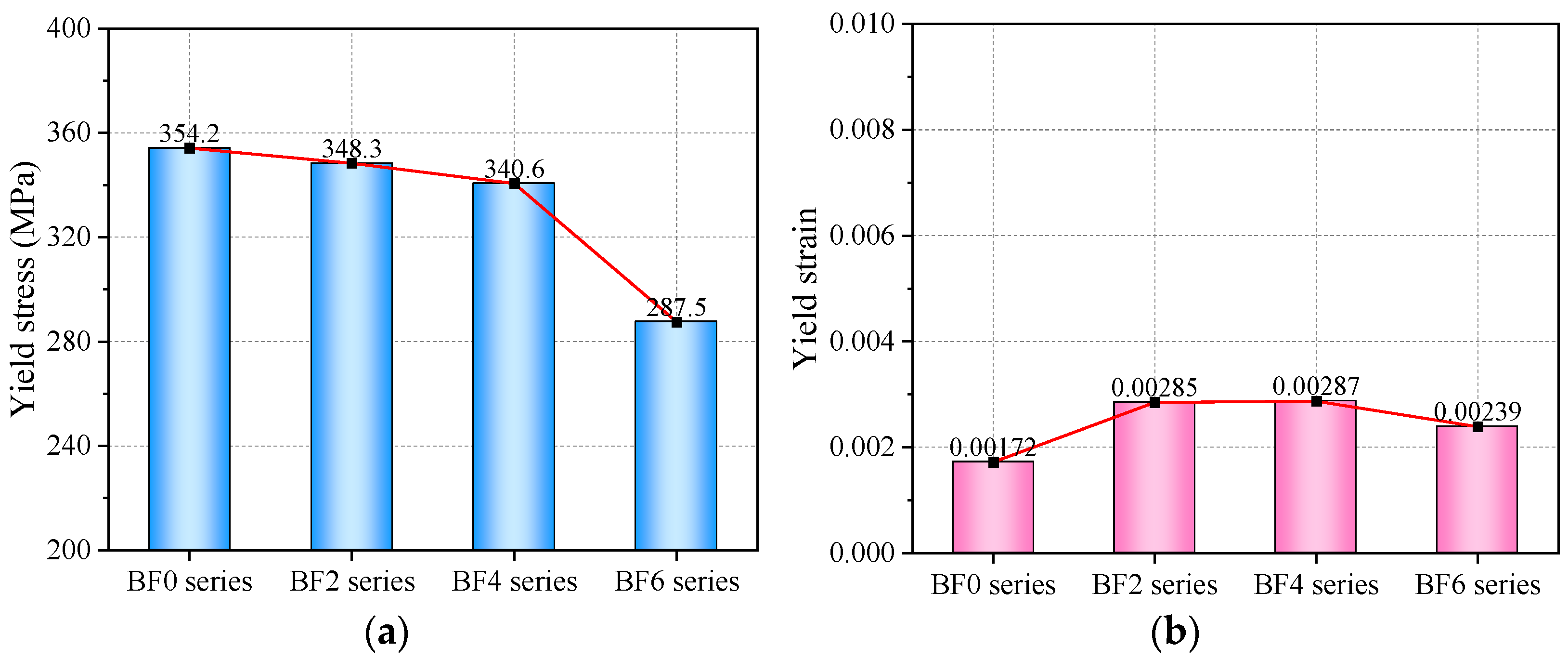
4.3. Analysis of Key Parameters
4.3.1. Yield Point
4.3.2. BFRP Fracture Point
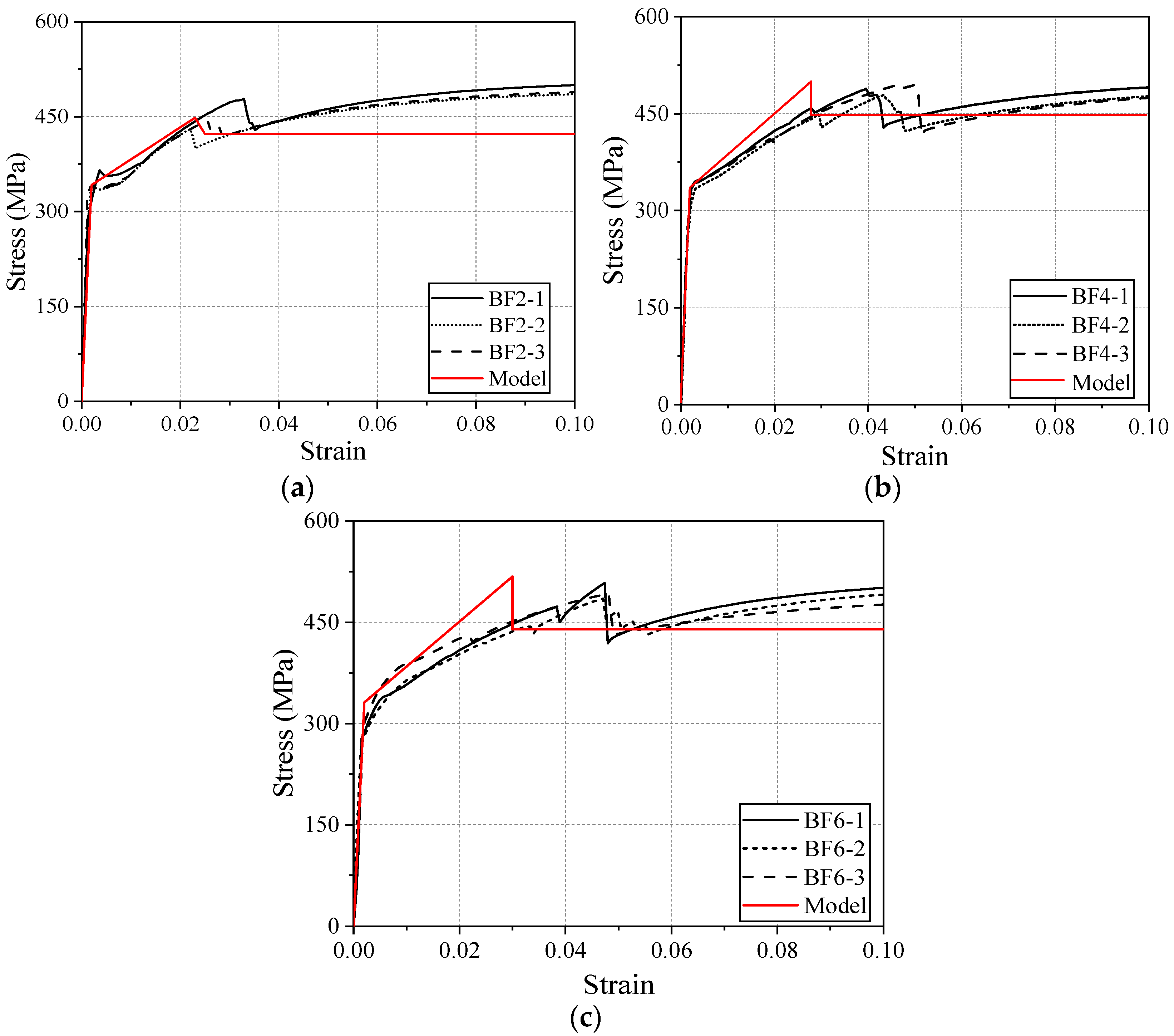
5. Full Stress–Strain Curve Model
6. Conclusions
- (1)
- Bonding BFRP sheets to steel plates significantly enhances the strengthening stiffness and yield strength while maintaining ductility. The composite plates exhibit a bilinear stress–strain relationship with distinct strengthening effects in the post-yield stage.
- (2)
- The fracture strain of the composite plates remains stable across specimens, and the addition of BFRP layers improves fracture stability. Failure modes indicate effective utilization of both steel and BFRP materials, with no premature debonding observed.
- (3)
- The proposed three-segment constitutive model accurately predicts the tensile behavior of composite plates, including the elastic, strengthening, and residual stages. The model demonstrates high reliability and practicality.
- (4)
- This study reveals a nonlinear relationship between the number of BFRP layers and tensile stress improvement. However, under compressive loads, the deformation and failure mechanisms of composites differ significantly from those of metals. Future research is needed to explore the compressive response and bonding behavior of hybrid composite systems.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Zhang, Y.; Ren, C.; Yi, J.; Wei, Y.; Li, G.; Zhao, H. Axial compression performance of concrete-filled steel tubular columns with damaged BFRP jackets. Constr. Build. Mater. 2024, 442, 137640. [Google Scholar] [CrossRef]
- Dong, Z.; Han, T.; Ji, J.; Zhu, H.; Wu, G. Durability of discrete BFRP needle-reinforced seawater sea-sand concrete-filled GFRP tubular columns in the ocean environment. Constr. Build. Mater. 2023, 365, 130017. [Google Scholar] [CrossRef]
- Cao, X.-Y.; Wu, G.; Ju, J.-W.W. Seismic performance improvement of existing RCFs using external PT-PBSPC frame sub-structures: Experimental verification and numerical investigation. J. Build. Eng. 2022, 46, 103649. [Google Scholar] [CrossRef]
- Tang, Y.; Wang, Y.; Sneed, L.H.; Sun, Z.; Wu, Z. Compressive performance of SFCB-reinforced and FRP-confined concrete cylinders. Constr. Build. Mater. 2024, 436, 136738. [Google Scholar] [CrossRef]
- Shi, J.; Qin, T.; Zhou, J.; Zhao, H.; Wang, H.; Fu, J.; Jin, L. Interlaminar shear behavior of basalt fiber-reinforced polymer tendons subjected to a combined effect of prestress, grout and seawater. Constr. Build. Mater. 2025, 462, 139982. [Google Scholar] [CrossRef]
- Liu, J.; Wei, Y.; Liu, Z.; Cui, Z. Effects of seawater corrosion on compression-induced buckling performance of FRP-CFST columns. Eng. Fail. Anal. 2024, 162, 108441. [Google Scholar] [CrossRef]
- Wang, Y.; Zheng, Y.; Li, J.; Zhang, L.; Deng, J. Experimental study on tensile behaviour of steel plates with centre hole strengthened by CFRP plates under marine environment. Int. J. Adhes. Adhes. 2018, 84, 18–26. [Google Scholar] [CrossRef]
- Zeng, J.-J.; Pan, B.-Z.; Fan, T.-H.; Zhuge, Y.; Liu, F.; Li, L.-J. Shear behavior of FRP-UHPC tubular beams. Compos. Struct. 2023, 307, 116576. [Google Scholar] [CrossRef]
- Lepretre, E.; Chataigner, S.; Dieng, L.; Gaillet, L. Stress Intensity Factor Assessment for the Reinforcement of Cracked Steel Plates Using Prestressed or Non-Prestressed Adhesively Bonded CFRP. Materials 2021, 14, 1625. [Google Scholar] [CrossRef] [PubMed]
- Doroudi, Y.; Fernando, D.; Hosseini, A.; Ghafoori, E. Behavior of cracked steel plates strengthened with adhesively bonded CFRP laminates under fatigue Loading: Experimental and analytical study. Compos. Struct. 2021, 266, 113816. [Google Scholar] [CrossRef]
- Romanowicz, P.J.; Szybiński, B.; Wygoda, M. Fatigue performance of open-hole structural elements reinforced by CFRP overlays. Int. J. Adhes. Adhes. 2024, 130, 103606. [Google Scholar] [CrossRef]
- Du, Y.; Shao, Y.; Wu, C.; Su, M.; Zhang, M.; Cao, Y. Behavior of steel plate shear walls reinforced with stiffened FRP plates. Thin-Walled Struct. 2024, 204, 112236. [Google Scholar] [CrossRef]
- Du, Y.; Liao, X.; Shao, Y.; Zhang, M.; Wu, C.; Cao, Y. Seismic performance of slit steel plate shear walls strengthened by FRP laminate. J. Build. Eng. 2024, 96, 110594. [Google Scholar] [CrossRef]
- Du, Y.; Gao, D.; Chen, Z.; Yan, J.-B.; Jia, P.; Dong, S. Experimental and theoretical investigation of FRP-steel composite plate under cyclic tensile loading. Thin-Walled Struct. 2023, 183, 110358. [Google Scholar] [CrossRef]
- Zheng, Z.; Du, Y.; Chen, Z.; Li, S.; Niu, J. Experimental and theoretical studies of FRP-Steel composite plate under static tensile loading. Constr. Build. Mater. 2021, 271, 121501. [Google Scholar] [CrossRef]
- Wang, H.-T.; Wu, G.; Jiang, J.-B. Fatigue Behavior of Cracked Steel Plates Strengthened with Different CFRP Systems and Configurations. J. Compos. Constr. 2016, 20, 04015078. [Google Scholar] [CrossRef]
- Hu, L.L.; Zhao, X.L.; Feng, P. Fatigue behavior of cracked high-strength steel plates strengthened by CFRP sheets. J. Compos. Constr. (ASCE) 2016, 20, 04016043. [Google Scholar] [CrossRef]
- Nhut, P.V.; Matsumoto, Y. Experimental analytical and theoretical investigations of CFRP strengthened thin-walled steel plates under shear loads. Thin-Walled Struct. 2020, 155, 106908. [Google Scholar] [CrossRef]
- Li, Y.; Wang, Y.; Ou, J. Mechanical Behavior of BFRP-Steel Composite Plate under Axial Tension. Polymers 2014, 6, 1862–1876. [Google Scholar] [CrossRef]
- Ji, J.; Wang, X.; Dong, Z.; Wang, J.; Li, J.; Li, K. Study on mechanical properties of curved prestressed BFRP bars for future application in PCCP. Constr. Build. Mater. 2024, 451, 138873. [Google Scholar] [CrossRef]
- Yang, C.; Liu, L.; Liu, Z.; Huang, Y.; Yu, S.; Fu, Y. Study on the mechanism of bond strength generation and debonding failure between basalt fiber and asphalt based on molecular dynamics. Case Stud. Constr. Mater. 2023, 19, e02493. [Google Scholar] [CrossRef]
- Zhang, B.; Zhu, H.; Chen, J. Bond durability between BFRP bars and seawater coral aggregate concrete under seawater corrosion environments. Constr. Build. Mater. 2023, 379, 131274. [Google Scholar] [CrossRef]
- Dong, Z.; Wu, G.; Zhao, X.; Zhu, H.; Wei, Y.; Yan, Z. Mechanical properties of discrete BFRP needles reinforced seawater sea-sand concrete-filled GFRP tubular stub columns. Constr. Build. Mater. 2020, 244, 118330. [Google Scholar] [CrossRef]
- Hu, Y.J.; Jiang, C.; Liu, W.; Yu, Q.Q.; Zhou, Y.L. Degradation of the In-plane Shear Modulus of Structural BFRP Laminates Due to High Temperature. Sensors 2018, 18, 3361. [Google Scholar] [CrossRef] [PubMed]
- ISO 6892-1:2019; Metallic Materials—Tensile Testing—Part 1: Method of Test at Room Temperature. International Organization for Standardization: Geneva, Switzerland, 2019.
- ASTM D3039/D3039M-17; Standard Test Method for Tensile Properties of Polymer Matrix Composite Materials. ASTM International: West Conshohocken, PA, USA, 2017.
- Wang, Q.; Shi, S.; Wang, L.; Wang, Z.; Ji, B.; Liu, J. Retarding effect on cracked steel plates strengthened by Fe-SMA and steel sheets. J. Constr. Steel Res. 2025, 225, 109190. [Google Scholar] [CrossRef]
- Wang, Z.; Wang, L.; Wang, Q.; Ji, B.; Liu, J.; Yao, Y. Strengthening Cracked Steel Plates with Shape Memory Alloy Patches: Numerical and Experimental Investigations. Materials 2023, 16, 7259. [Google Scholar] [CrossRef]
- Guo, S.C.; Liu, H.F. Elastic analysis on post-local buckling of steel plates in thin-walled rectangular concrete-filled steel tube Columns. Prog. Ind. Civ. Eng. 2012, 204–208, 912–916. [Google Scholar] [CrossRef]
- Aljabar, N.J.; Zhao, X.L.; Al-Mahaidi, R.; Ghafoori, E.; Motavalli, M.; Koay, Y.C. Experimental investigation on the CFRP strengthening efficiency of steel plates with inclined cracks under fatigue loading. Eng. Struct. 2018, 172, 877–890. [Google Scholar] [CrossRef]
- Wu, G.; Wu, Z.-S.; Luo, Y.-B.; Sun, Z.-Y.; Hu, X.-Q. Mechanical Properties of Steel-FRP Composite Bar under Uniaxial and Cyclic Tensile Loads. J. Mater. Civ. Eng. 2010, 22, 1056–1066. [Google Scholar] [CrossRef]



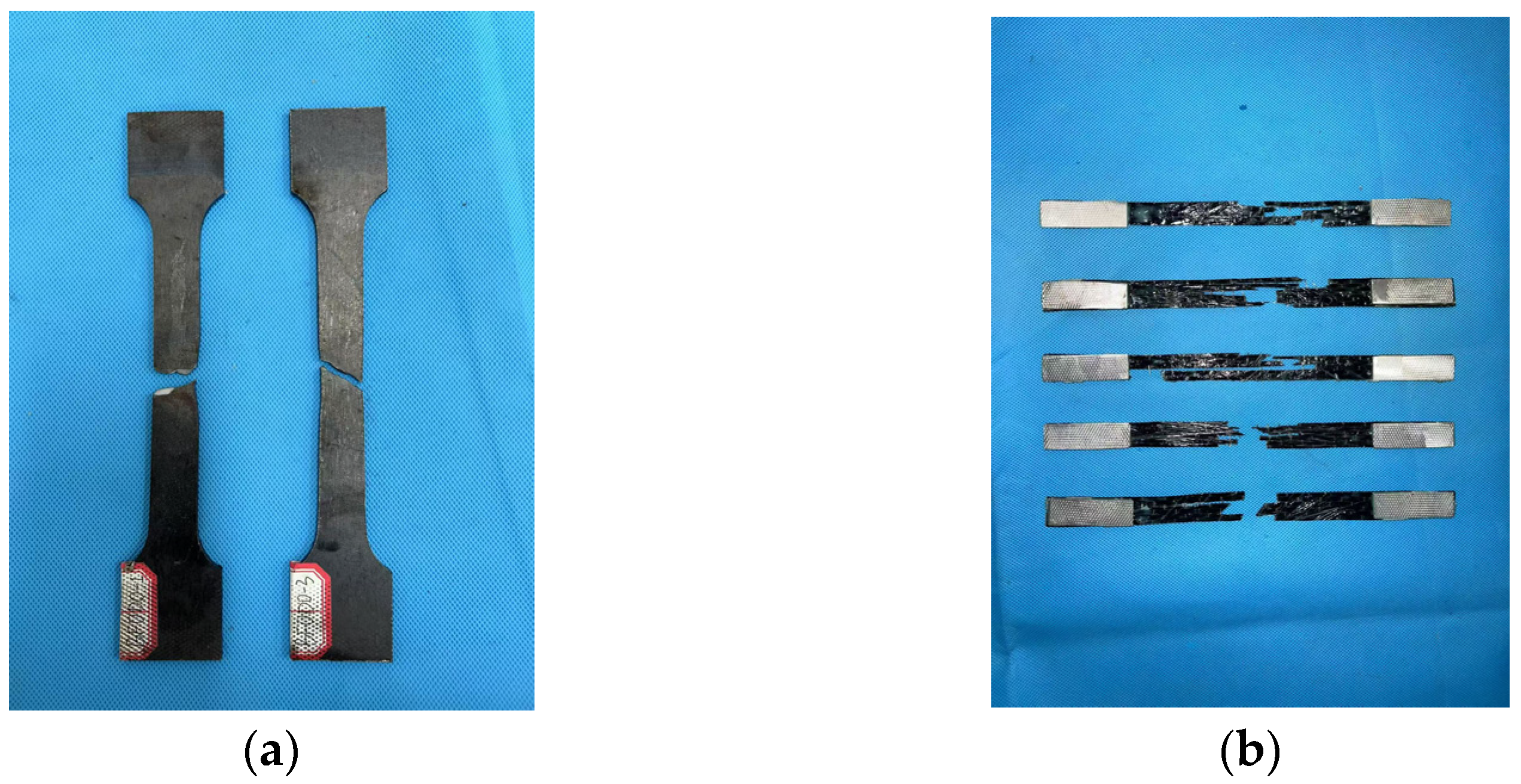
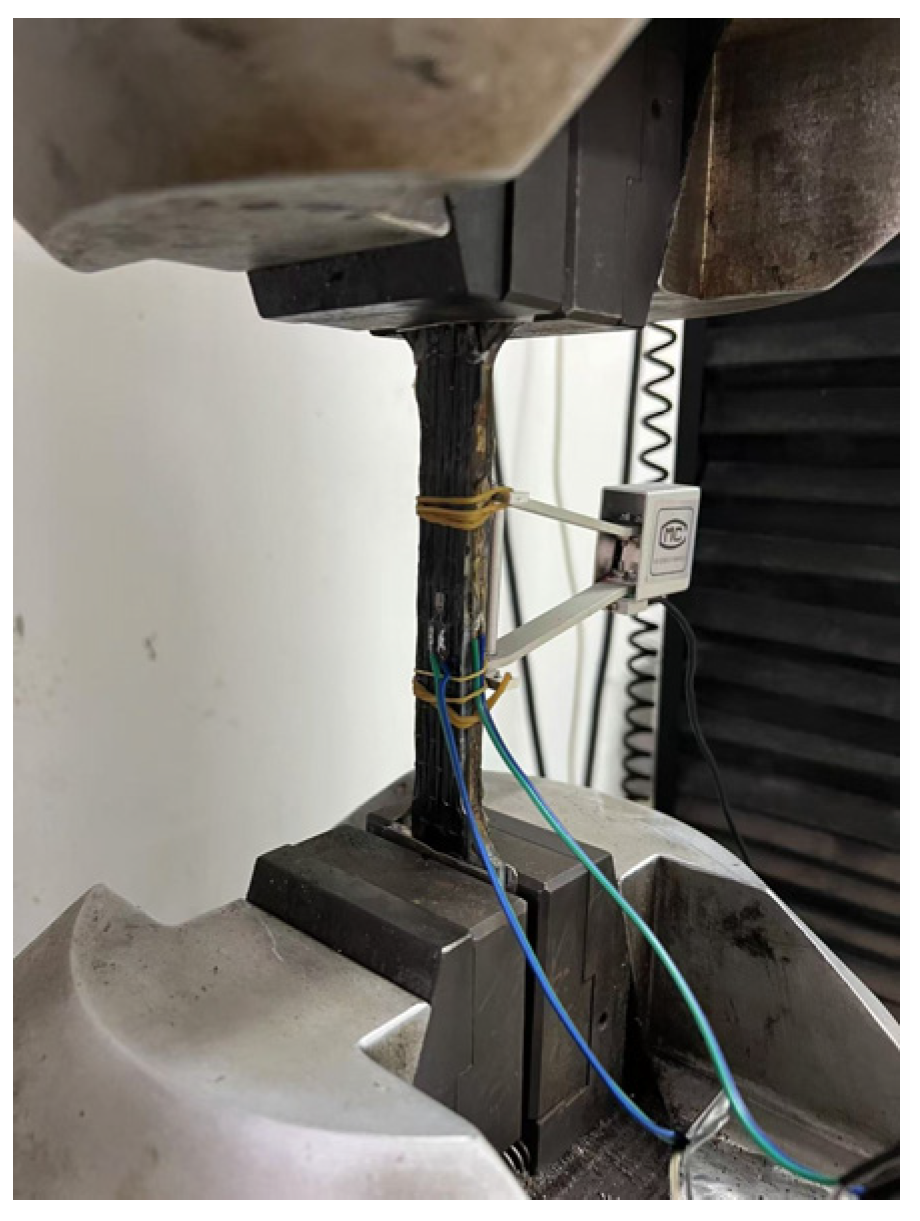
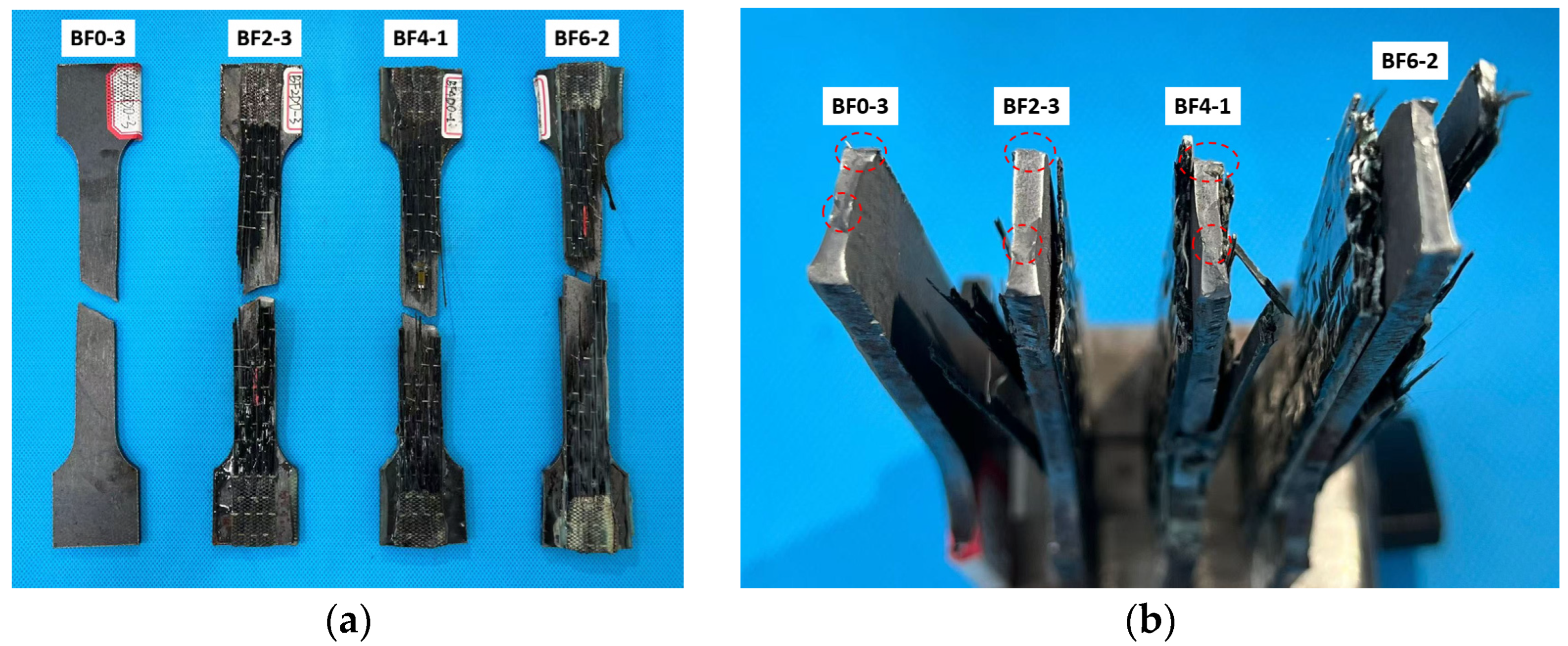
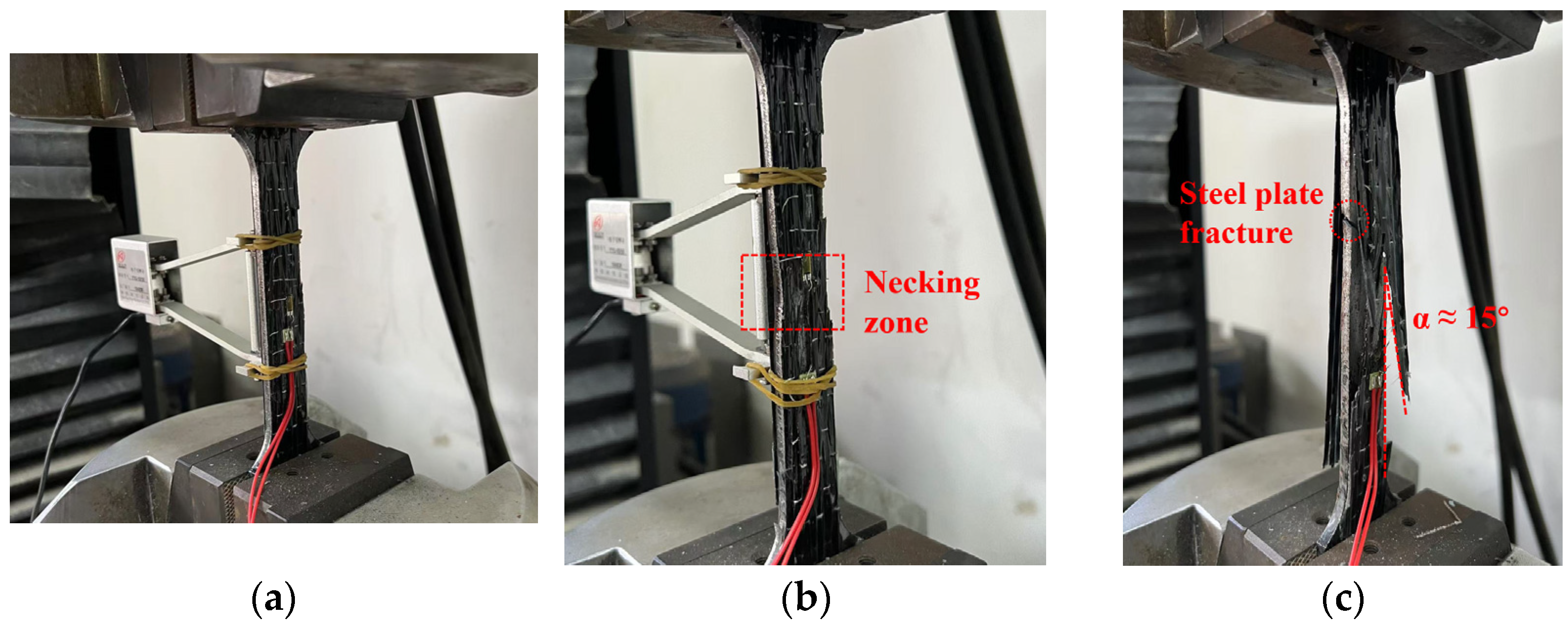



| Ref | Specimen | FRP Type | FRP Layers | Nominal Thickness of Steel Plate (mm) | Yield Strength (MPa) | Peak Strength (MPa) | Peak Strain (%) |
|---|---|---|---|---|---|---|---|
| [14] | FHA6-C1 | CFRP sheet | 1 | 6 | 312.6 | 356.5 | 1.05 |
| [14] | FHA6-2C1 | CFRP sheet | 2 | 6 | 300.2 | 430.7 | 1.11 |
| [14] | FHA6-3C1 | CFRP sheet | 3 | 6 | 296.2 | 429.3 | 1.08 |
| [14] | FHA6-G1 | GFRP sheet | 1 | 6 | 304.9 | 340.5 | 1.22 |
| [14] | FHA6-CP1 | CFRP plate | 1 | 8 | 271.9 | 559 | 1.57 |
| [14] | FHA8-C1 | CFRP sheet | 1 | 8 | 323.4 | 371 | 0.98 |
| [14] | FHA8-G1 | GFRP sheet | 1 | 8 | 329.8 | 382.9 | 1.45 |
| [14] | FHA8-3G1 | GFRP sheet | 3 | 8 | 306.6 | 415.4 | 1.64 |
| [14] | FHA8-5G1 | GFRP sheet | 5 | 8 | 273.5 | 402.3 | 1.52 |
| [14] | FHA8-CP1 | CFRP plate | 1 | 8 | 274.2 | 602.3 | 1.78 |
| [14] | FHA10-C1 | CFRP sheet | 1 | 10 | 358 | 359.6 | 0.96 |
| [14] | FHA10-G1 | GFRP sheet | 1 | 10 | 325.2 | 382.4 | 1.69 |
| [14] | FHA10-CP1 | CFRP plate | 1 | 10 | 279 | 651.5 | 4.25 |
| [14] | HA6-1 | - | - | 6 | 315.1 | - | - |
| [14] | HA8-1 | - | - | 8 | 309.5 | - | - |
| [14] | HA10-1 | - | - | 10 | 316 | - | - |
| [19] | BSP2 | BFRP sheet | 2 | 3.05 | 309.4 | 455.2 | 2.75 |
| [19] | BSP4 | BFRP sheet | 4 | 3.05 | 293 | 483.8 | 2.48 |
| [19] | BSP6 | BFRP sheet | 6 | 3.05 | 275.6 | 492 | 2.25 |
| [19] | BSP8 | BFRP sheet | 8 | 3.05 | 258.6 | 551.2 | 2.51 |
| Specimen No. | Steel Thickness (mm) | Layer of BFRP Sheet | Number of Composite Plates |
|---|---|---|---|
| BF0 | 4.0 | 0 | 3 |
| BF2 | 4.0 | 2 | 3 |
| BF4 | 4.0 | 4 | 3 |
| BF6 | 4.0 | 6 | 3 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, Y.; Yi, J.; Wei, Y.; Zhao, H. Tensile Properties and Constitutive Model of BFRP–Steel–BFRP Composite Plates. Materials 2025, 18, 756. https://doi.org/10.3390/ma18040756
Zhang Y, Yi J, Wei Y, Zhao H. Tensile Properties and Constitutive Model of BFRP–Steel–BFRP Composite Plates. Materials. 2025; 18(4):756. https://doi.org/10.3390/ma18040756
Chicago/Turabian StyleZhang, Yirui, Jiyang Yi, Yang Wei, and Hu Zhao. 2025. "Tensile Properties and Constitutive Model of BFRP–Steel–BFRP Composite Plates" Materials 18, no. 4: 756. https://doi.org/10.3390/ma18040756
APA StyleZhang, Y., Yi, J., Wei, Y., & Zhao, H. (2025). Tensile Properties and Constitutive Model of BFRP–Steel–BFRP Composite Plates. Materials, 18(4), 756. https://doi.org/10.3390/ma18040756









