Mechanism of Isotropic Behavior in Titanium Alloy Plates Formed by Axial Closed Die Rolling
Abstract
1. Introduction
2. Material and Methods
2.1. Test Materials
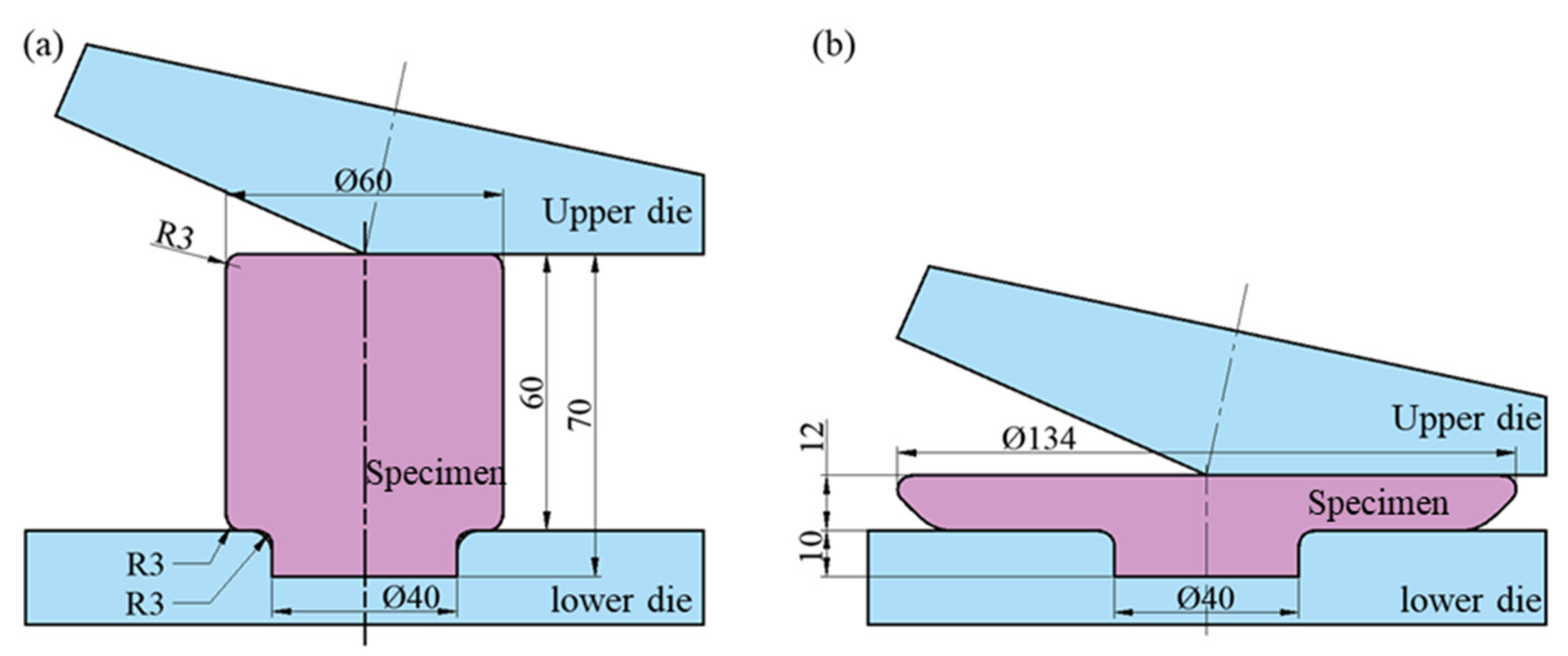
2.2. Test Scheme
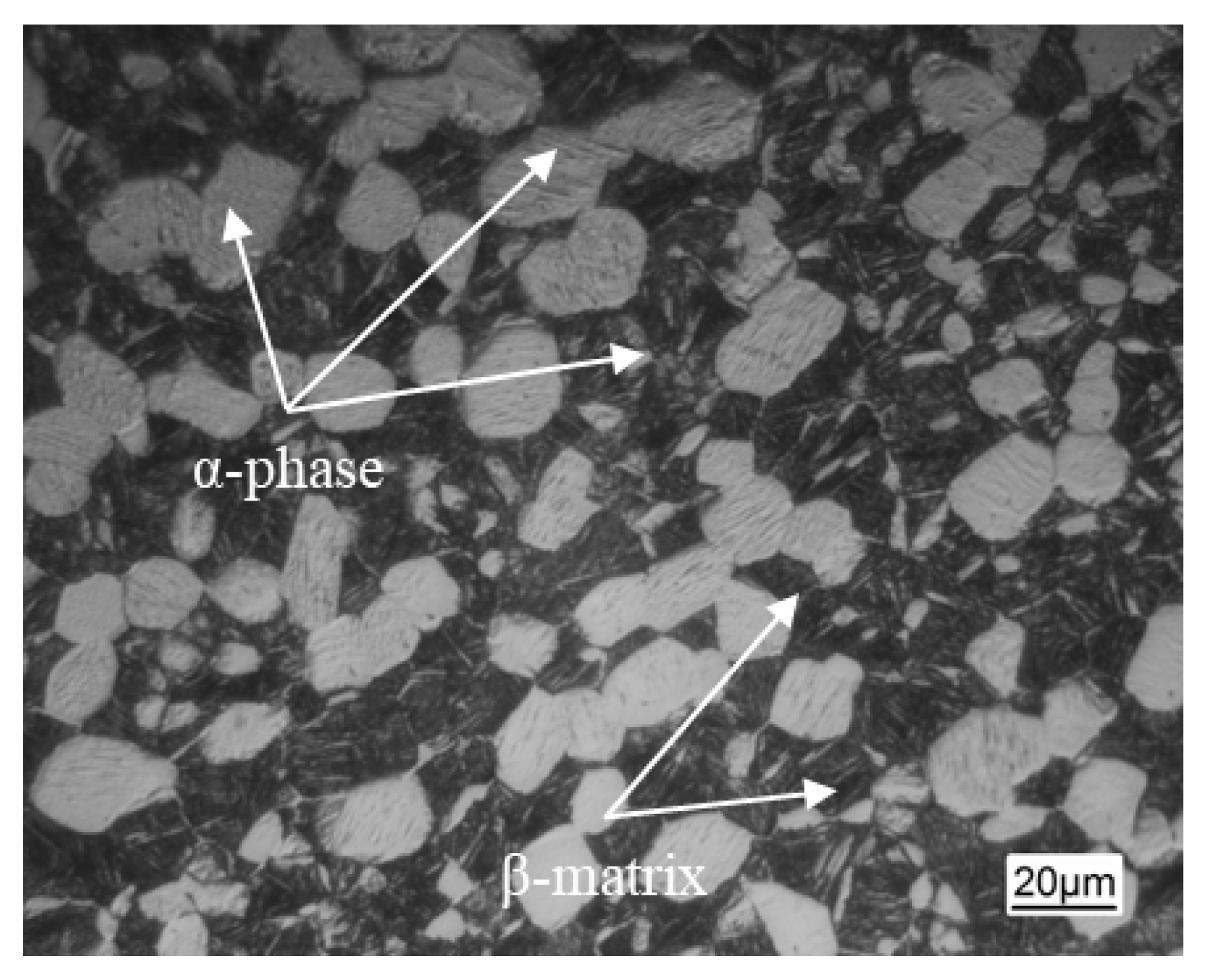
2.3. Microstructure and Performance Test Sampling Schemes
3. Experimental Results and Discussion
3.1. Reversal of Behavioral Characterization
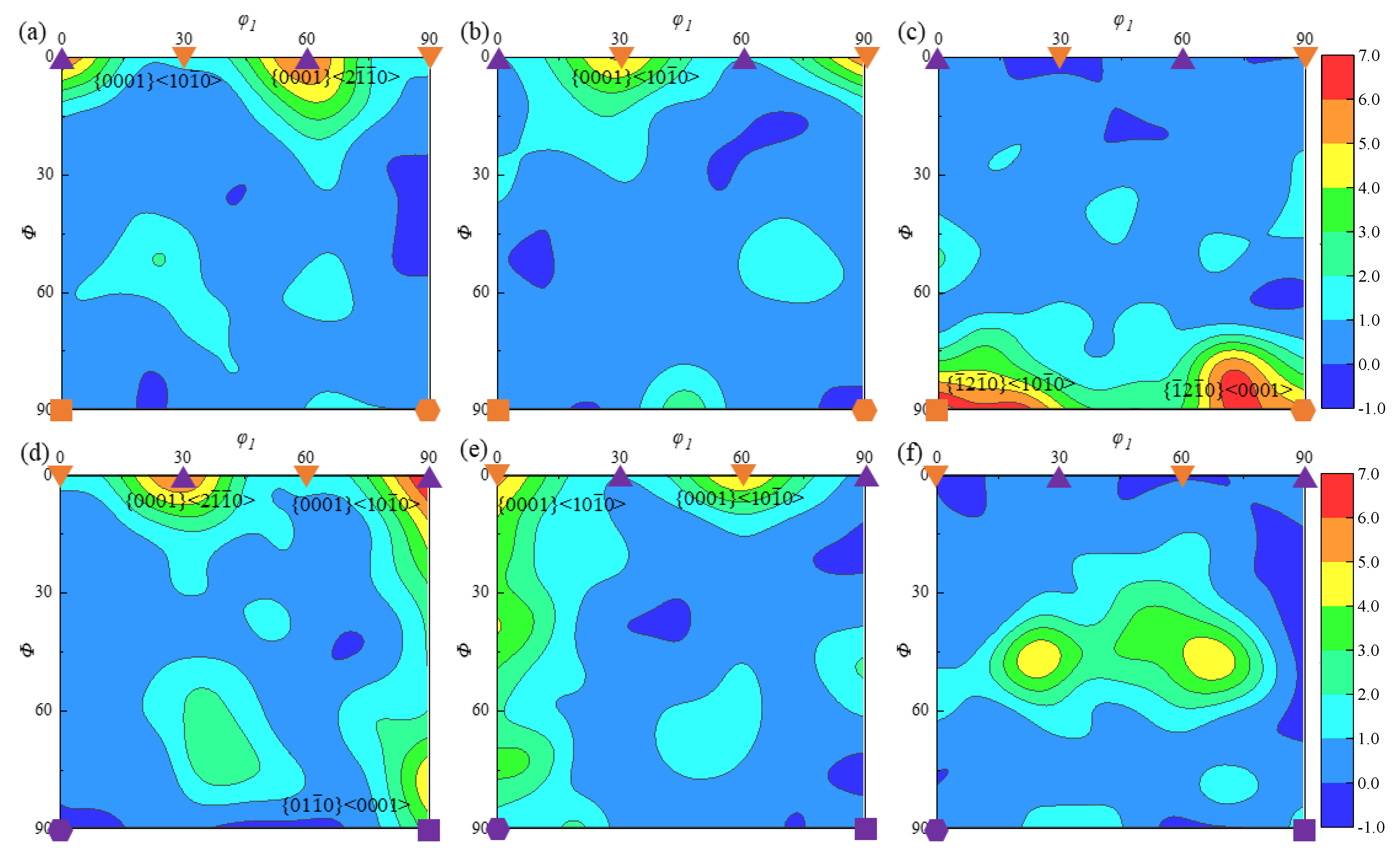
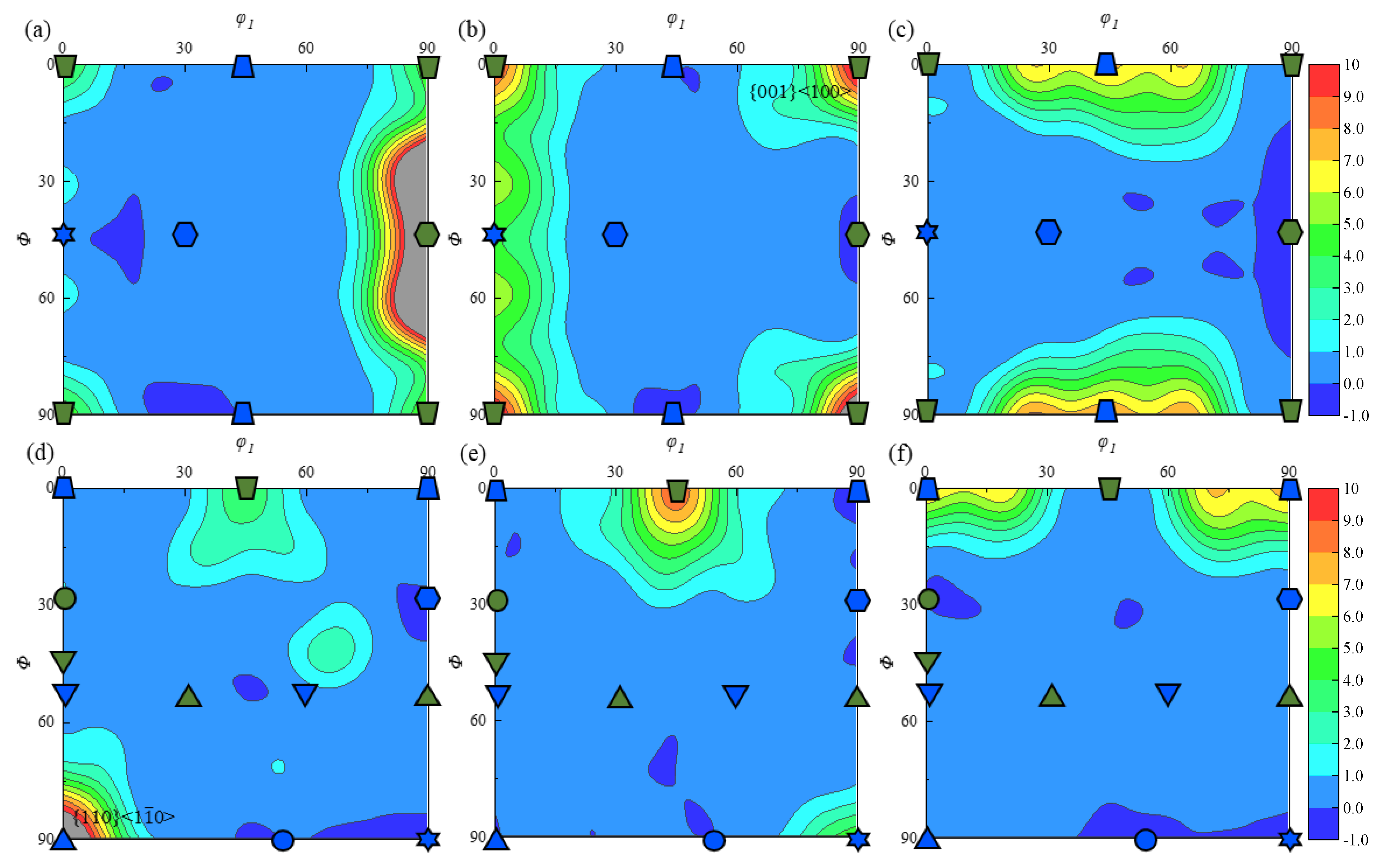
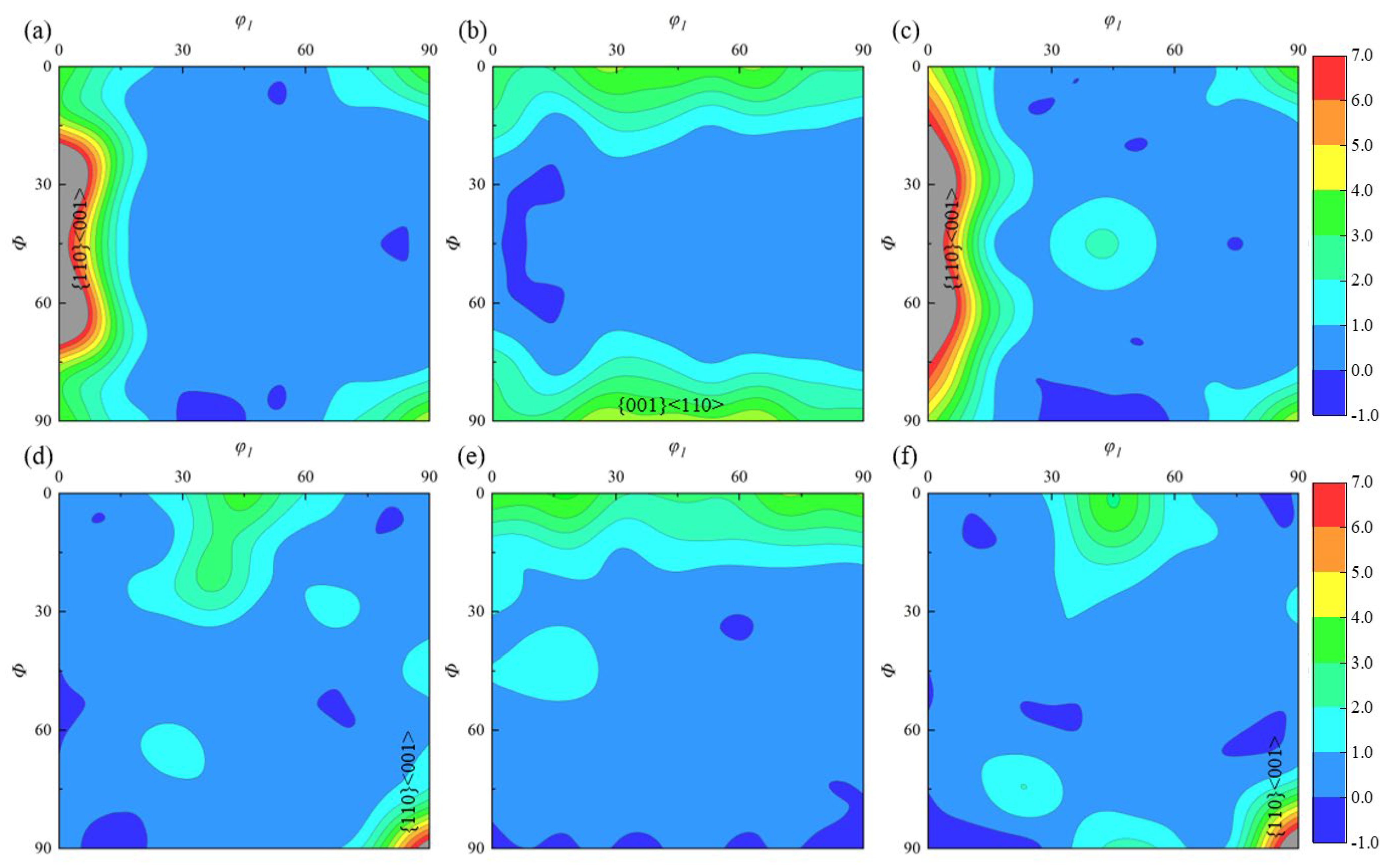
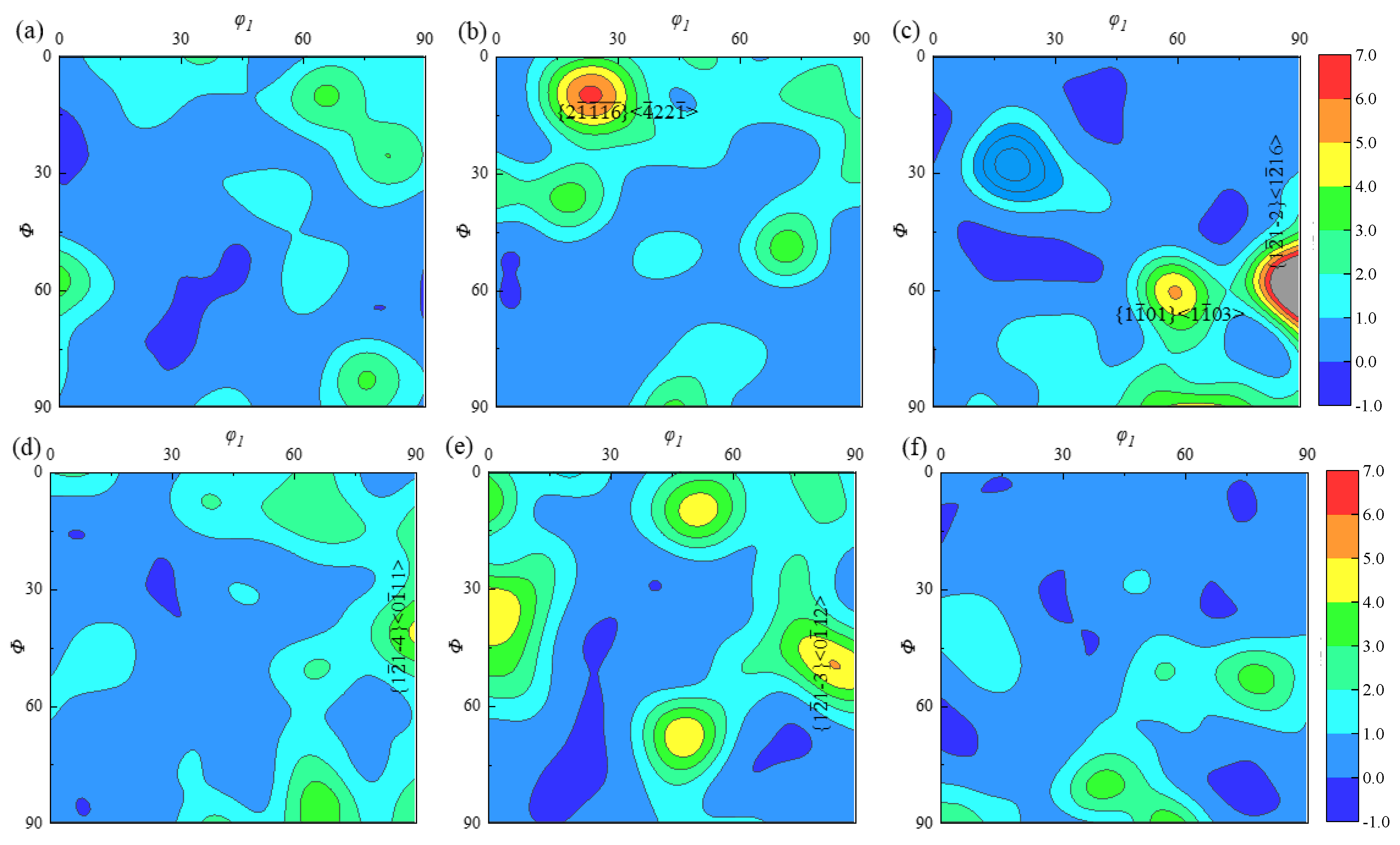
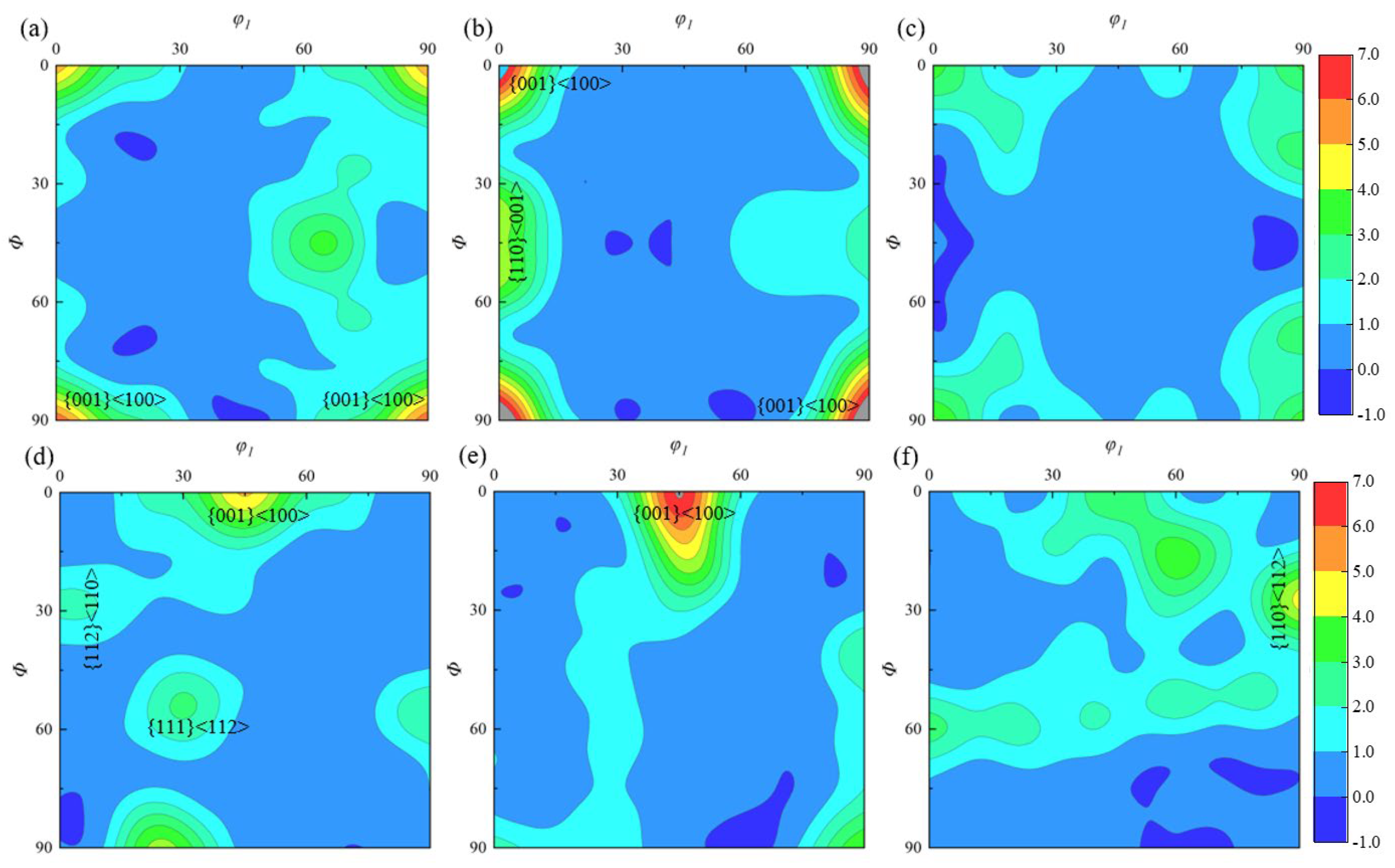
3.2. EBSD Characterization and Analysis of the Specimens
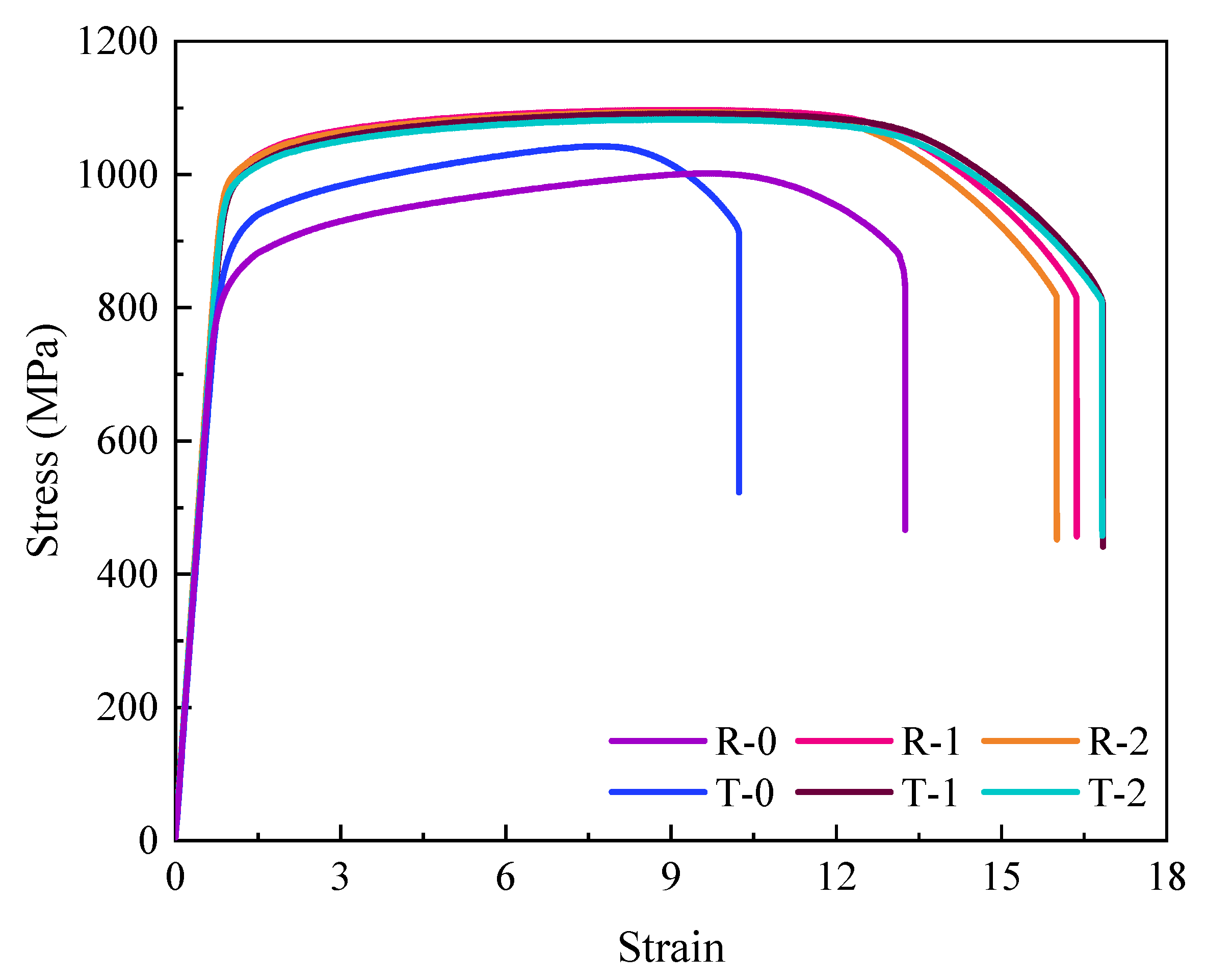
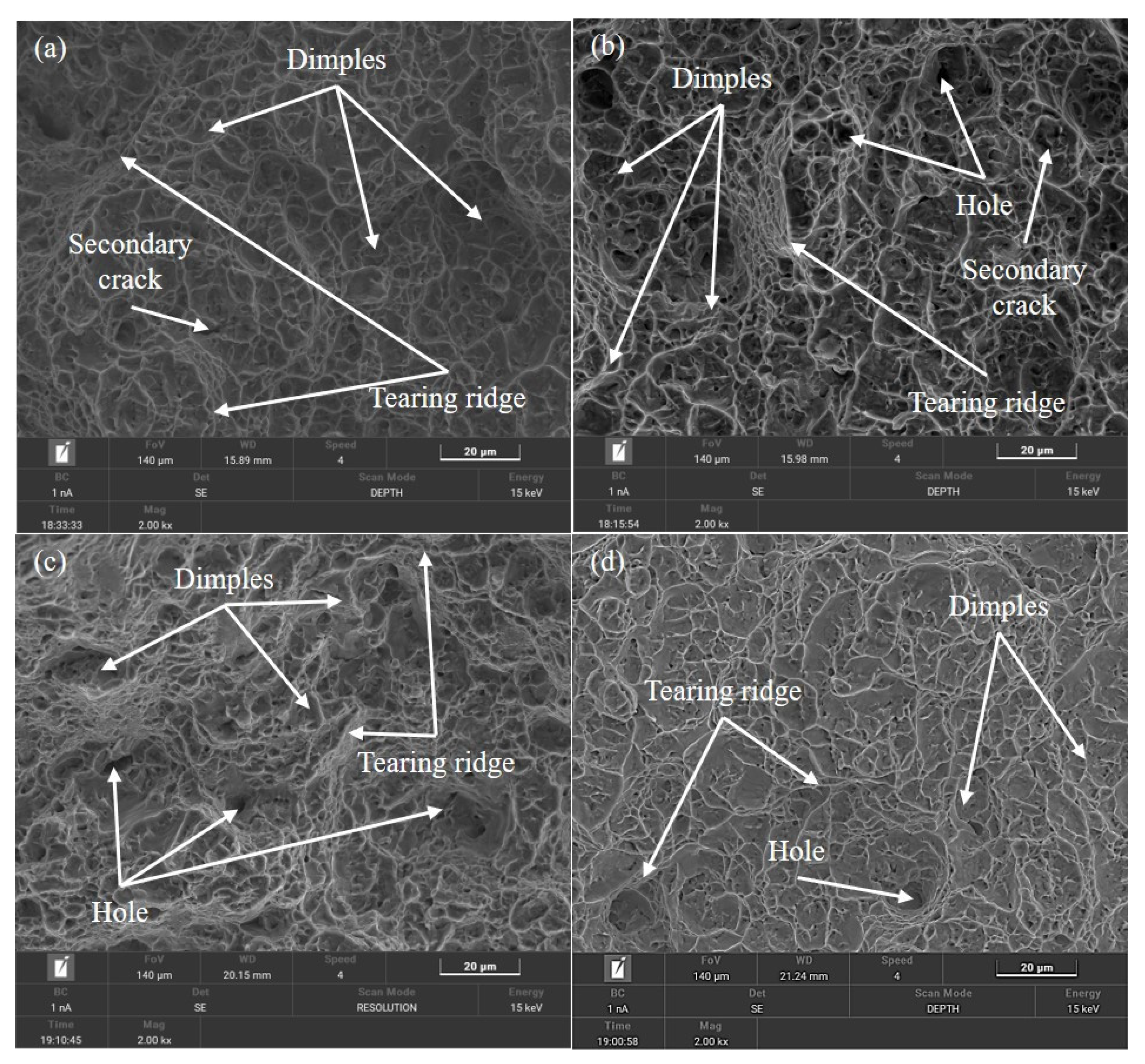
3.3. Room Temperature Tensile Tests and Isotropy Behavior
4. Conclusions
- (1)
- Since the ACDR is a volume incremental molding technique, strain is progressively accumulated from the specimen’s upper surface downward. Increases in deformation lead to an increase in the specimen’s axial and radial angles as well as the degree of torsion, which enhances the specimen’s strength and plasticity by increasing the amount of strain accumulation in each area.
- (2)
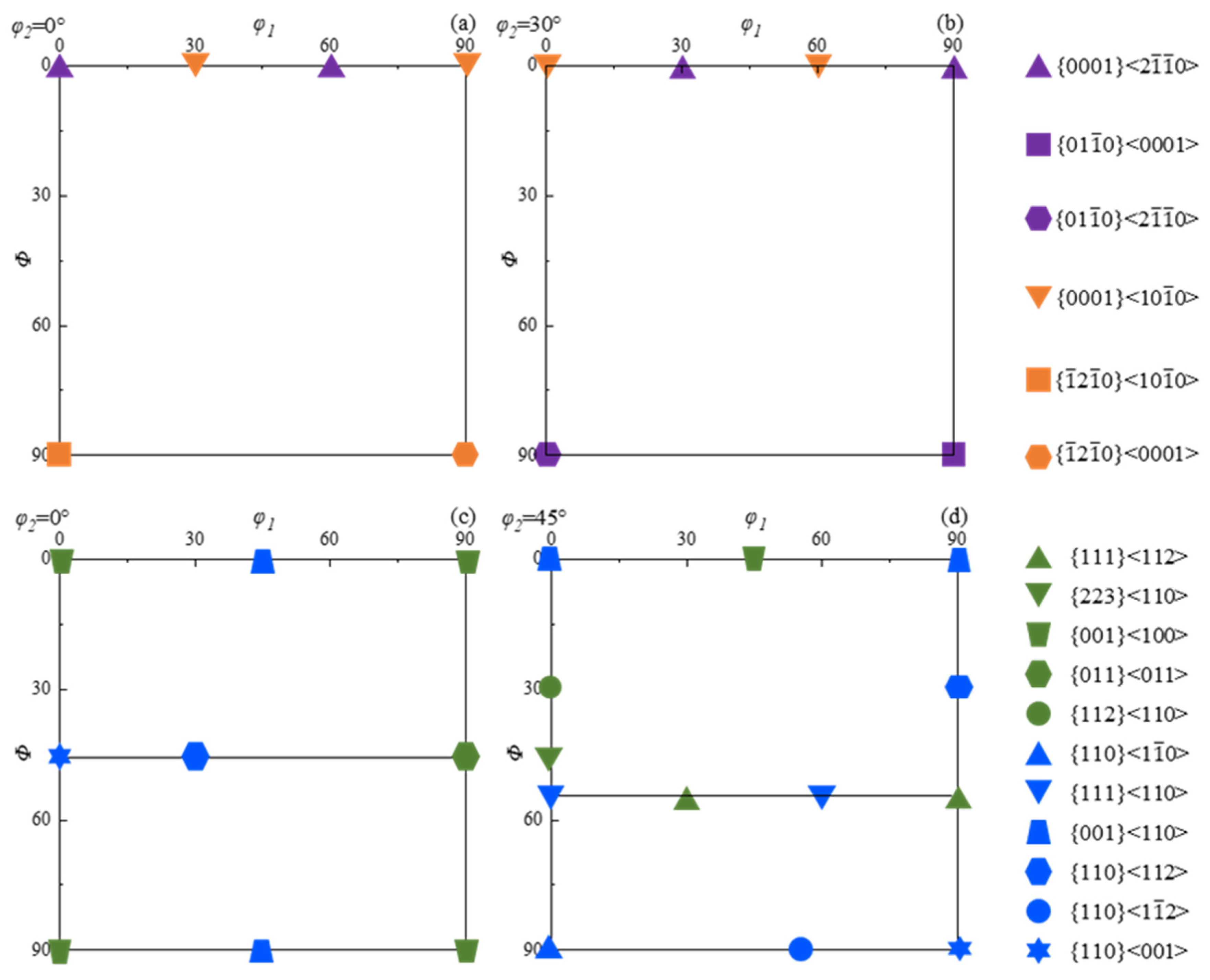
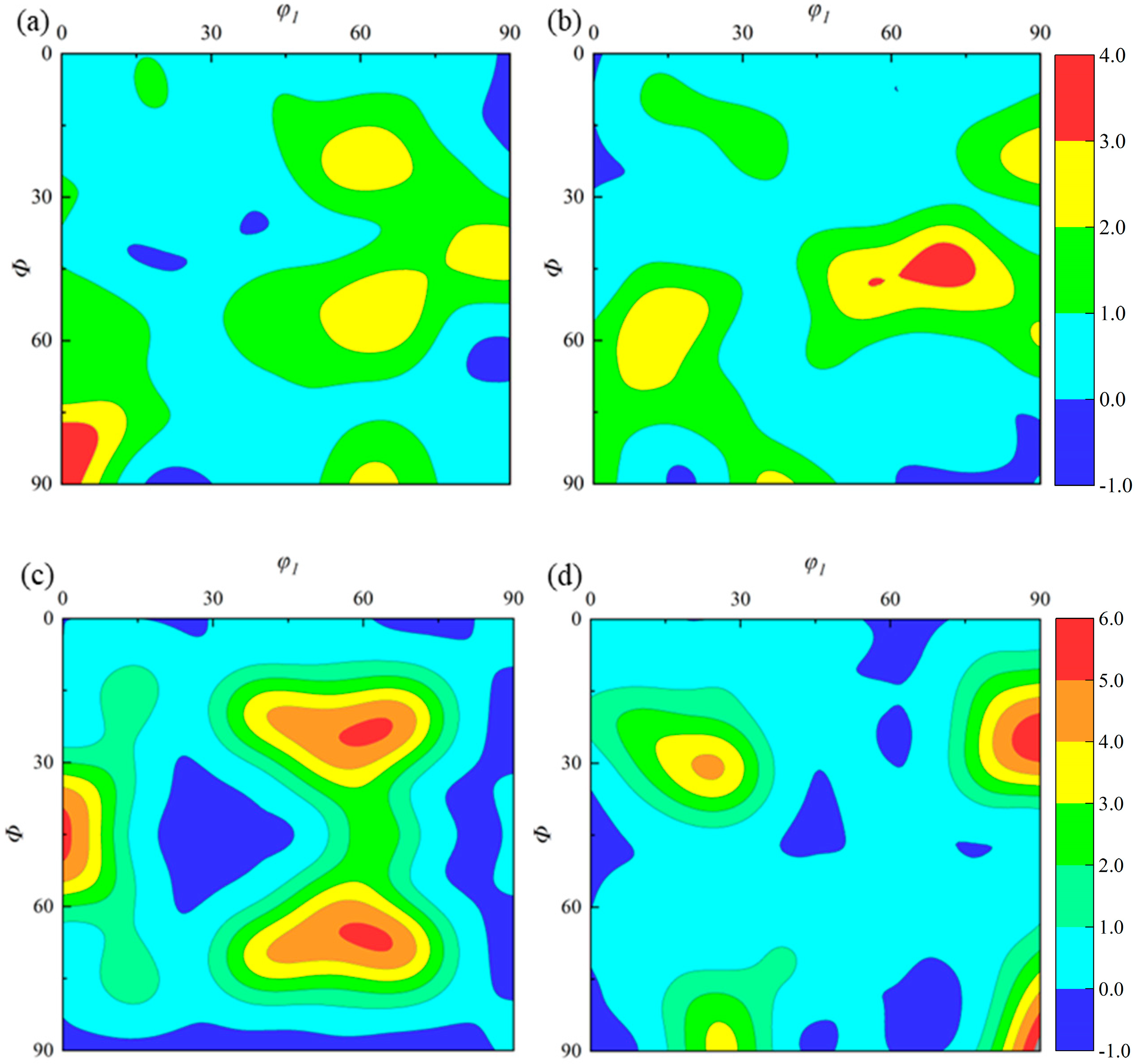
- As can be observed from the examination of the ODF plots, the specimen contains a variety of textures, each with a unique texture strength. The xz and yz planes are primarily composed of textures {0001} <20> and {010} <20>, which correspond to the strengths of 5.6 and 6.77, respectively, and are formed near the direction of the column plane. The specimen’s edge has a weaker {11} <011> texture, with a strength of roughly 4.9. {20} <100> and {010} <20> textures with strengths of roughly 8.2 are primarily dispersed along the cone’s direction in the xy plane. {11} <116> texture has strengths of roughly 13.7, meaning that the closer the specimen’s edge is to the texture’s location, the stronger the texture. Among them, the β-phase is mainly distributed with {001} <100>, {110} <10>, and {110} <001> texture, with approximately 8.5-fold texture strength within the specimen. Nevertheless, because the content of β-phase is too small in the specimen, the distribution pattern of its texture has a weak effect on the texture distribution of the overall specimen.
- (3)
- During the ACDR forming process, compression and torsional deformation occur simultaneously, resulting in the formation of uneven texture strengths and weakening the overall orientation of the specimen relationship. As a result, the specimen’s tensile properties exhibit high levels of anisotropy in all directions, with tensile strength and plasticity anisotropy exceeding 99 percent and 95 percent, respectively.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Zhao, W.G.; Li, X.; Lu, S.Q. Study on constitutive relationship of TC11 titanium alloy during high temperature deformation. J. Plast. Eng. 2018, 15, 123–127. [Google Scholar]
- Zhang, S.; Huang, W.; Yu, J.; Zhang, Z.; Zhang, J.; Yuan, R.; Zhang, C.; Han, J.; Meng, J.; Wang, T. Effect of Heterogeneous Deformation on Microstructure and Microtexture Evolution of Ti-6Al-4V Alloy during Multidirectional Isothermal Forging. Adv. Eng. Mater. 2024, 26, 12. [Google Scholar] [CrossRef]
- Pilchak, A.L.; Sargent, G.A.; Semiatin, S.L. Early Stages of Microstructure and Texture Evolution during Beta Annealing of Ti-6Al-4V. Metall. Mater. Trans. A 2018, 49, 908–919. [Google Scholar] [CrossRef]
- Gey, N.; Humbert, M.; Philippe, M.; Combres, Y. Investigation of the α- and β- texture evolution of hot rolled Ti-64 products. Mater. Sci. Eng. A 1996, 219, 80–88. [Google Scholar] [CrossRef]
- Li, S.; Yang, L.; Lei, L.; Wei, C.; Zhang, H. Development of Microstructure and Texture Heterogeneities during Static Annealing of Ti–6.5Al–3.5Mo–1.5Zr–0.3Si Alloy Preformed by Hot Working. J. Mater. Sci. Technol. 2012, 28, 1015–1024. [Google Scholar] [CrossRef]
- Dixit, S.; Dash, B.B.; Kumar, D.; Bhattacharjee, A.; Sankaran, S. Influence of phase morphology, static recrystallization, and crystallographic texture on room temperature tensile properties of Ti–6Al–4V alloy: Comparison between post-tested equiaxed, bimodal, and lamellar microstructures. Mater. Sci. Eng. A 2023, 873, 144990. [Google Scholar] [CrossRef]
- Dixit, S.; Kumar, D.; Dash, B.B.; Suwas, S.; Bhattacharjee, A.; Sankaran, S. Effect of solutionizing temperature and cooling rate on phase morphology, recrystallization and texture evolution in a heat treated Ti–6Al–4V alloy having different types of microstructure. J. Alloys Compd. 2022, 927, 166897. [Google Scholar] [CrossRef]
- Lu, L.; Zhang, Y.; Zhang, Z.; Song, K.; Li, S.; Li, Y.; Zhou, F.; Cao, Q.; Feng, Q.; Zhang, B. Investigation on microstructure and texture evolution of Ti–6Al–3Nb–2Zr–1Mo alloy during hot deformation. Mater. Res. Express 2021, 8, 096520. [Google Scholar] [CrossRef]
- Wang, Q.; Sha, A.; Huang, L.; Li, X. Influence of Heat Treatment Process on Microstructure and Mechanical Properties of Ti-55531 Alloy. Titan. Ind. Prog. 2014, 31, 16–19. [Google Scholar] [CrossRef]
- Li, W.; Peng, T.; Zhang, M.C. Effect of forging processes on the microstructure and texture distribution of Ti-6Al-4V alloy. Chin. J. Eng. 2015, 37, 345–352. [Google Scholar] [CrossRef]
- Kang, D.H.; Kim, T.W. Mechanical behavior and microstructural evolution of commercially pure titanium in enhanced multi-pass equal channel angular pressing and cold extrusion. Mater. Des. 2010, 31 (Suppl. S1), S54–S60. [Google Scholar] [CrossRef]
- Liu, C.; Yin, F.; Xie, L.; Qian, D.; Song, Y.; Wu, W.; Wang, L.; Zhang, L.-C.; Hua, L. Evolution of grain boundary and texture in TC11 titanium alloy under electroshock treatment. J. Alloy. Compd. 2022, 904, 163969. [Google Scholar] [CrossRef]
- Yang, P.; Yu, Y.; Chen, L.; Mao, W. Experimental determination and theoretical prediction of twin orientations in magnesium alloy AZ31. Scr. Mater. 2004, 50, 1163–1168. [Google Scholar] [CrossRef]
- Zheng, Y.; Liu, D.; Qiu, S.; Wang, S.; Wei, L.; Wang, Z.; Tian, D. Effect of deformation routes on torsion behavior via ACDR process. Int. J. Adv. Manuf. Technol. 2019, 104, 4105–4116. [Google Scholar] [CrossRef]
- Heilmaier, M.; Leetz, U.; Reppich, B. Order strengthening in the cast nickel-based superalloy IN 100 at room temperature—ScienceDirect. Mater. Sci. Eng. A 2001, 319–321, 375–378. [Google Scholar] [CrossRef]
- Seol, J.B.; Bae, J.W.; Kim, J.G.; Sung, H.; Li, Z.; Lee, H.H.; Shim, S.H.; Jang, J.H.; Ko, W.-S.; Hong, S.I.; et al. Short-range order strengthening in boron-doped high-entropy alloys for cryogenic applications. Acta Mater. 2020, 194, 366–377. [Google Scholar] [CrossRef]
- GB/T 228-2021; Metallic Materials—Tensile Testing—Part 1: Method of Test at Room Temperature. National Standards of the People’s Republic of China: Beijing, China, 2021.
- Wang, J.; Liu, D.; Yuan, Y.; Li, X.; Yang, Y.; Wang, H.; Wang, H.; Huang, J.; Zhang, Z. Deformation characteristic and microstructure evolution of GH4169 alloy induced by axial upsetting and axial rotary forging. Mater. Charact. 2022, 191, 112136. [Google Scholar] [CrossRef]
- Zheng, Y.; Liu, D.; Yang, Y.; Zhang, Z.; Li, X. PDZ evolution of hot ACDR and forging processes during titanium alloy disc forming. Int. J. Adv. Manuf. Technol. 2018, 95, 1635–1643. [Google Scholar] [CrossRef]
- Nan, J.; Liu, D.; Rao, H.; Zhang, Y.; Wu, J.; Wang, J. Study of Microstructure and Mechanical Properties by Torsional Behavior in Axial Closed Die Rolling Forming. Metals 2024, 14, 1418. [Google Scholar] [CrossRef]
- Xue, C.; Zeng, W.D.; Wang, W.; Liang, X.B.; Zhang, J.W. Coarsening behavior of lamellar orthorhombic phase and its effect on tensile properties for the Ti–22Al–25Nb Alloy. Mater. Sci. Eng. A 2014, 611, 320–325. [Google Scholar] [CrossRef]
- Sauer, C.; Luetjering, G. Thermo-mechanical processing of high strength β-titanium alloys and effects on microstructure and properties. J. Mater. Process. Technol. 2001, 117, 311–317. [Google Scholar] [CrossRef]
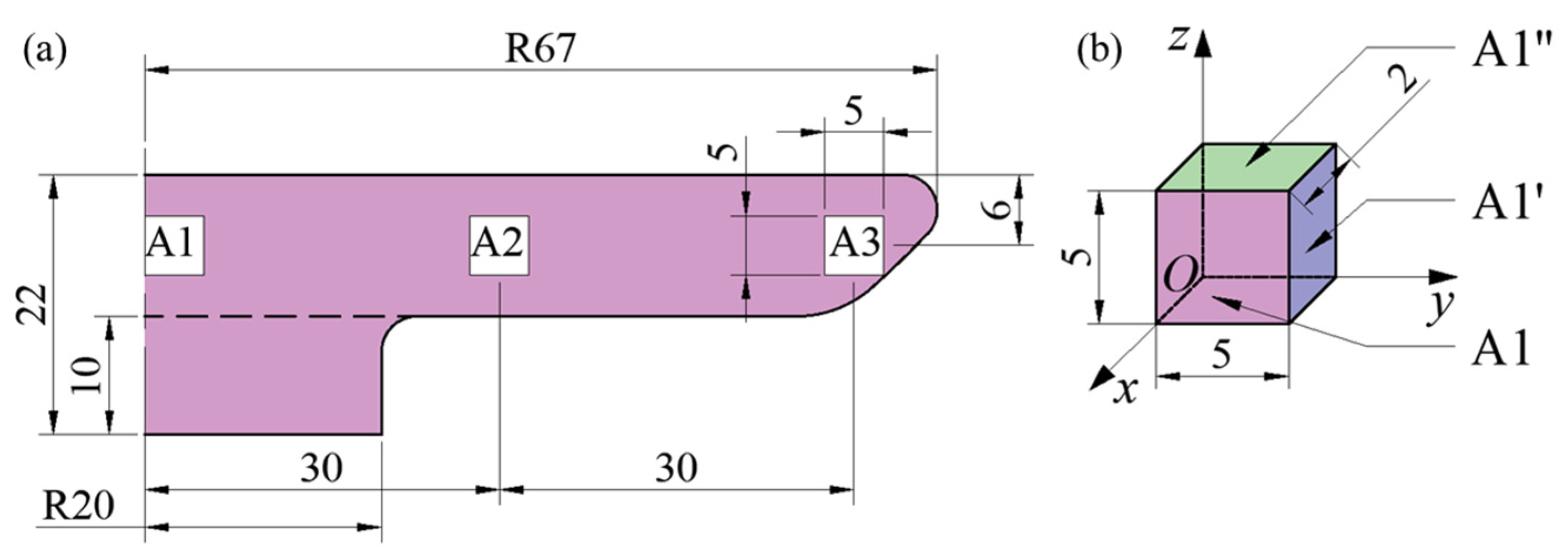
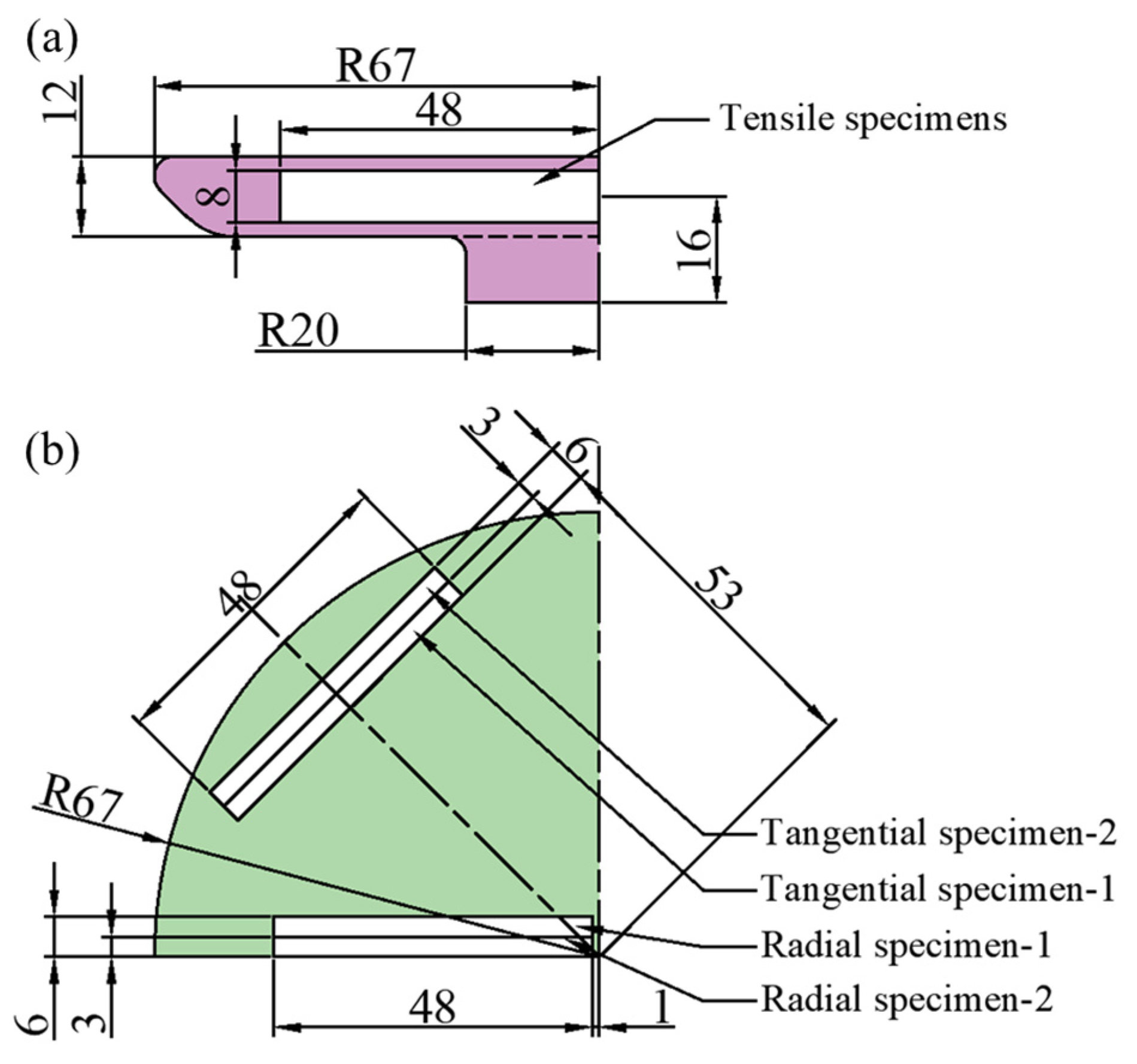
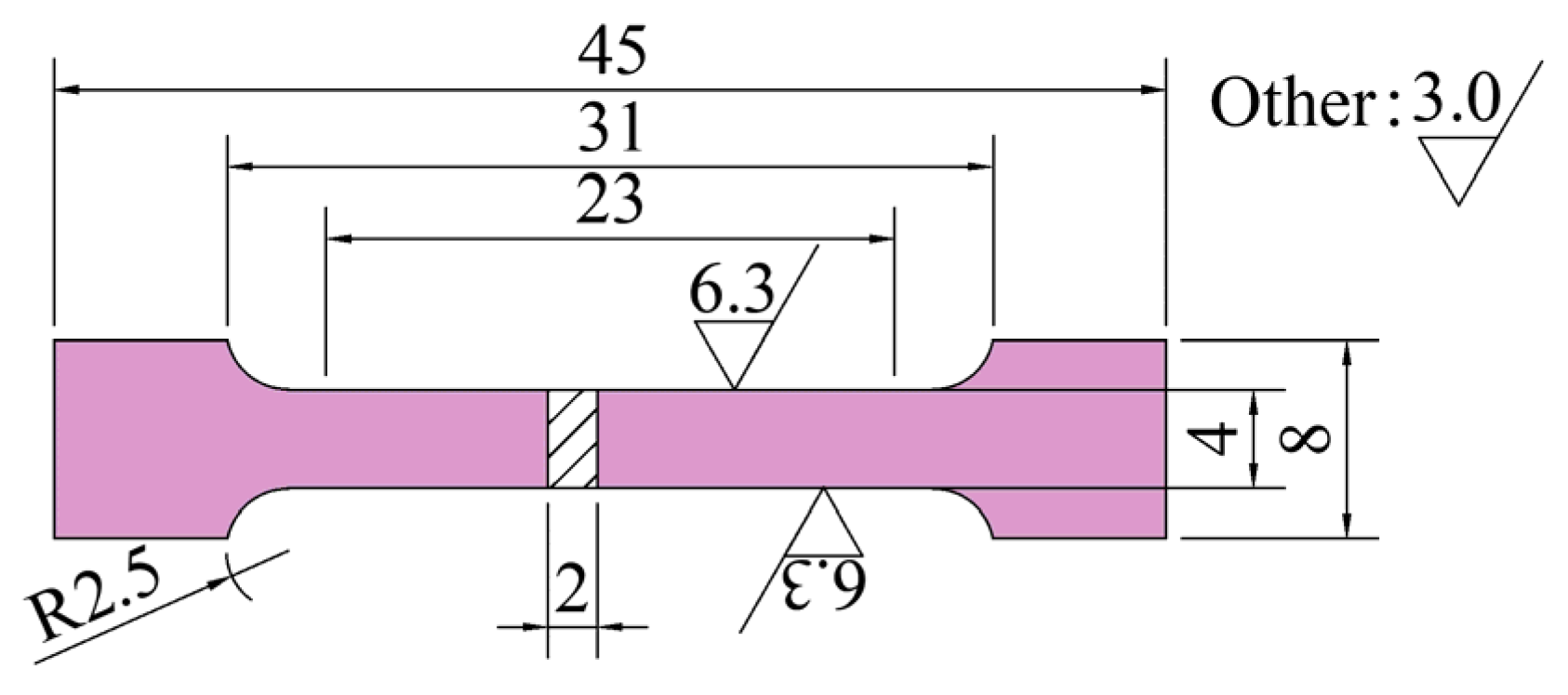
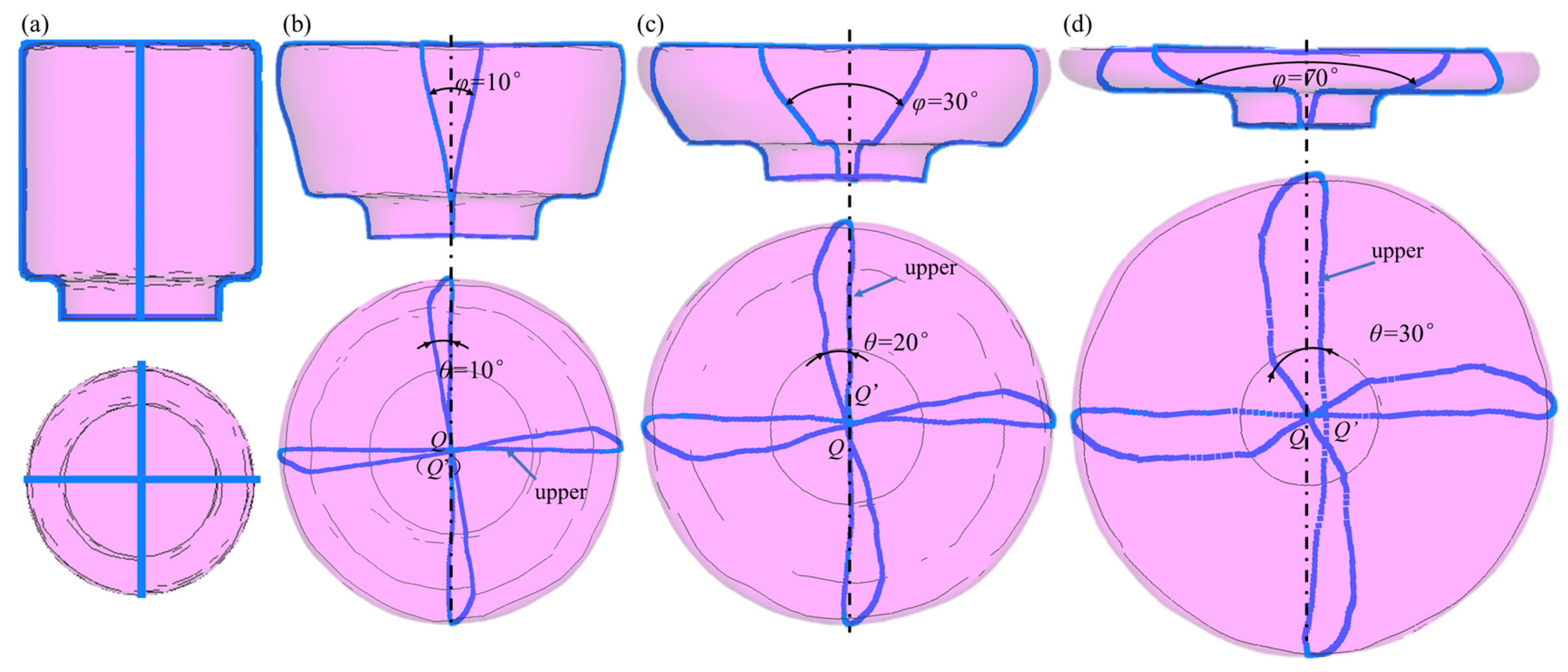


| Elements | Ti | Al | Mo | Zr | Si | Fe | C |
|---|---|---|---|---|---|---|---|
| Content (wt%) | Bal | 6.92 | 3.3 | 1.6 | 0.35 | 0.03 | 0.05 |
| Standard (wt%) | Bal | 5.8~7 | 2.8~3.8 | 0.8~2 | 0.2~0.35 | ≤0.25 | ≤0.1 |
| Parameters | ACDR |
|---|---|
| Billet size (Diameter (Φ) × Height (d) (mm)) | Φ60 × 60 |
| Positioning table size (Φ × d (mm)) | Φ40 × 10 |
| Heat temperature of the billet (°C) | 980 (Tβ − 30) |
| Preheating temperature of the dies (°C) | 200 |
| Axial reduction (%) | 80 |
| Rotation speed of the lower die (r/min) | 35 |
| Inclination angle of the upper die (°) | 6 |
| Feed speed of the upper die (mm/s) | 2.5 |
| Mold materials | 42CrMo |
| Processes | Yield Strength/MPa | Ultimate Tensile Strength/MPa | Uniform Elongation/% | Reduction of Area/% |
|---|---|---|---|---|
| Original | 3.23% | 2.77% | 14.94% | 5.80% |
| ACDR | 0.69% | 0.43% | 2.15% | 4.10% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Nan, J.; Liu, D.; Zhang, Y.; Zhang, Y.; Wang, J. Mechanism of Isotropic Behavior in Titanium Alloy Plates Formed by Axial Closed Die Rolling. Materials 2025, 18, 2528. https://doi.org/10.3390/ma18112528
Nan J, Liu D, Zhang Y, Zhang Y, Wang J. Mechanism of Isotropic Behavior in Titanium Alloy Plates Formed by Axial Closed Die Rolling. Materials. 2025; 18(11):2528. https://doi.org/10.3390/ma18112528
Chicago/Turabian StyleNan, Jungang, Dong Liu, Yonghao Zhang, Yu Zhang, and Jianguo Wang. 2025. "Mechanism of Isotropic Behavior in Titanium Alloy Plates Formed by Axial Closed Die Rolling" Materials 18, no. 11: 2528. https://doi.org/10.3390/ma18112528
APA StyleNan, J., Liu, D., Zhang, Y., Zhang, Y., & Wang, J. (2025). Mechanism of Isotropic Behavior in Titanium Alloy Plates Formed by Axial Closed Die Rolling. Materials, 18(11), 2528. https://doi.org/10.3390/ma18112528







