Simulation of the Penetration Process of 7xxx Aluminum Alloy Laminates with Different Configurations
Abstract
1. Introduction
2. Materials and Methods
2.1. Materials
2.2. Experimental Methods
3. Results
3.1. J-C Flow Stress Model
3.2. J-C Damage and Fracture Model
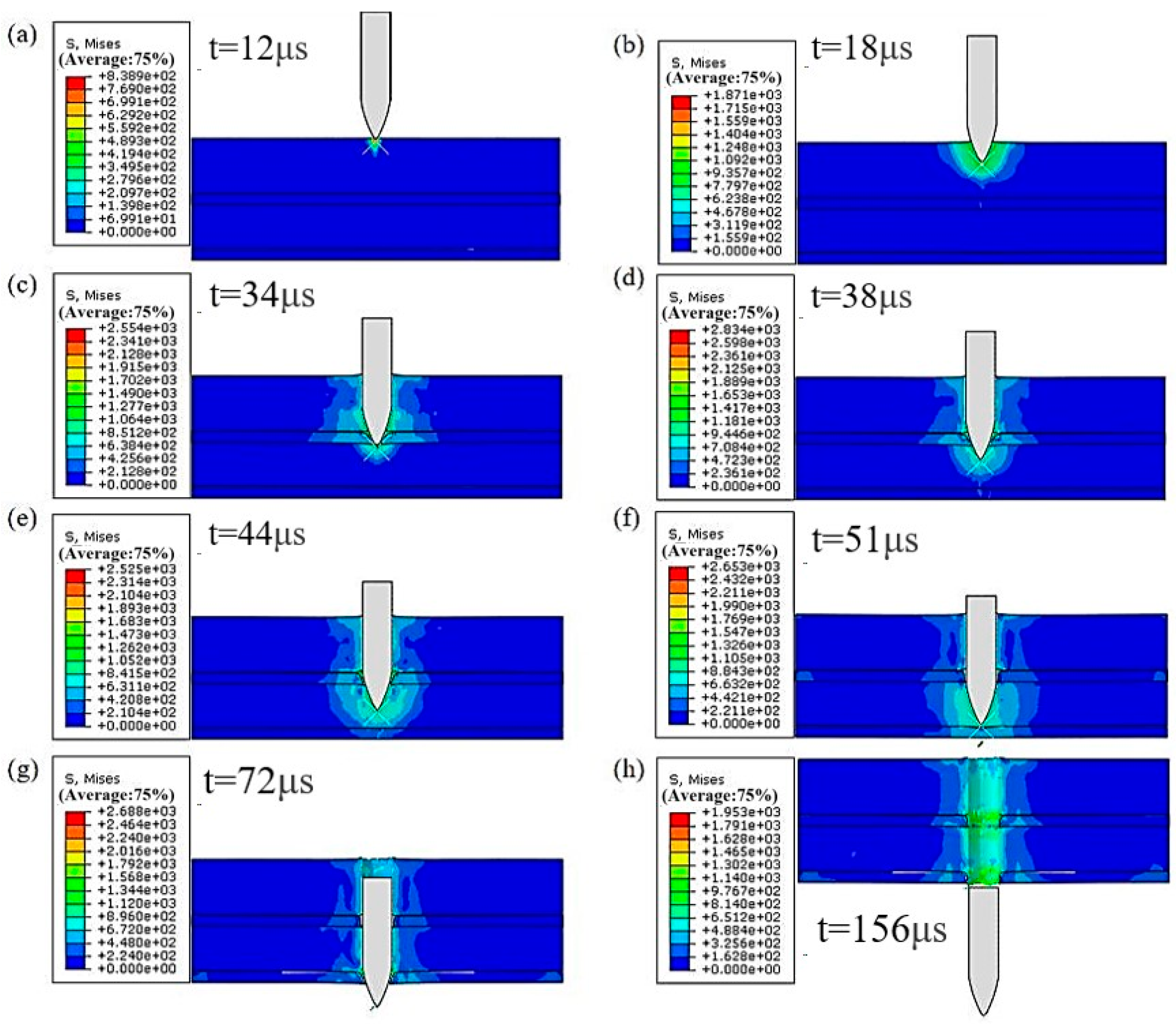
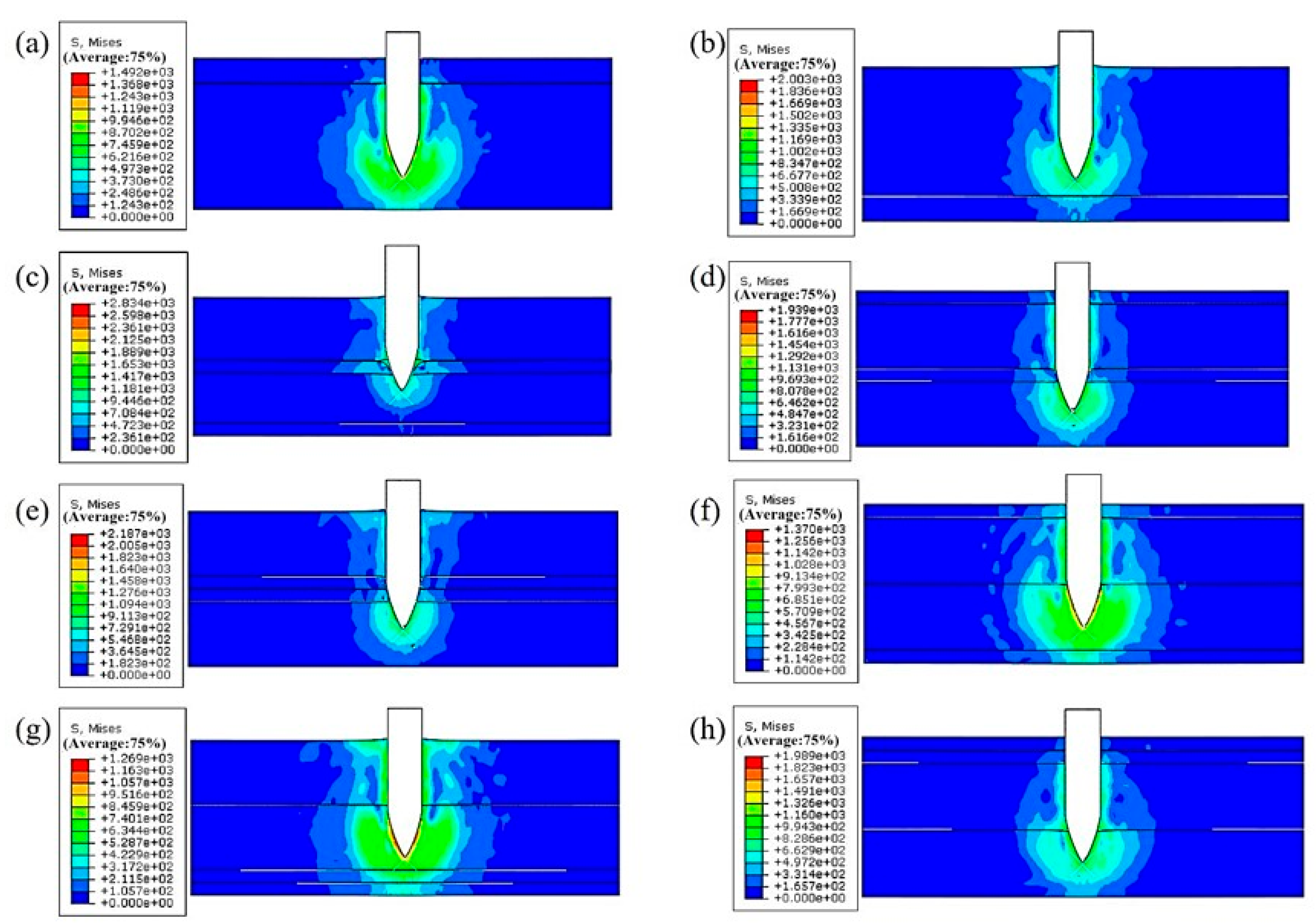
3.3. Simulation of the Penetration Process
4. Conclusions
- (1)
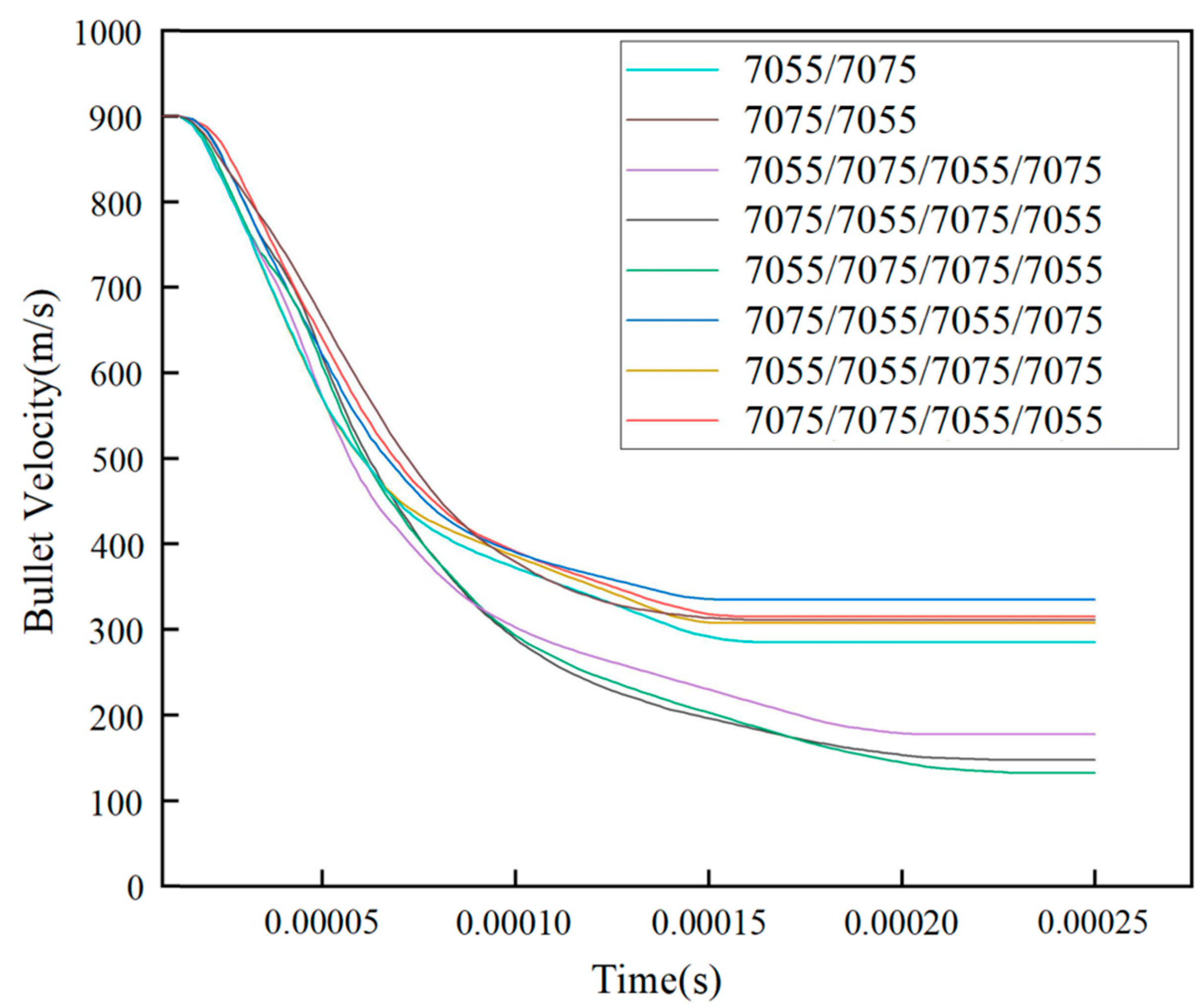
- From the velocity curve for bullet penetration, it can be seen that the velocity of the bullet sharply decreases in the initial stage of penetration, but in the later stage, it slows down. This indicates that the strain and stress generated by the deformation of the front end of the laminate may cause local instability of the rear part of the material, which reduces the anti-penetration performance of the laminate.
- (2)
- Numerical simulation was conducted on the bullet penetration process of 7055/7075 aluminum alloy laminates with different configurations, in order to evaluate their anti-penetration performance. The simulation results indicate that when the thickness ratio of 7075 and 7055 is 1:5, the optimal configuration of the composite plate is 7055/7075/7075/7055.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Jiang, A.; Li, Y.; Li, D.; Hou, H. Study on Anti-Penetration Performance of Semi-Cylindrical Ceramic Composite Armor against 12.7 mm API Projectile. Crystals 2022, 12, 1343. [Google Scholar] [CrossRef]
- Dhavale, O.V.; Kundurti, S.C.; Sharma, A. Ballistic response of additively manufactured AA6061/AA7075 multistack plate for armor-piercing projectile. Mater. Today Proc. 2024, 98, 194–199. [Google Scholar]
- Cheng, C.; Fu, Y.; Du, C.; Du, Z.; Jiang, Z.; Zhou, F.; Zhong, K.; Wang, X. Experimental and numerical study of tantalum-tungsten alloy rod penetrator impacting thick armor plate. Int. J. Refract. Met. Hard Mater. 2022, 107, 105873. [Google Scholar] [CrossRef]
- Tsirogiannis, E.C.; Daskalakis, E.; Hassan, M.H.; Omar, A.M.; Bartolo, P. Ballistic design and testing of a composite armour reinforced by CNTs suitable for armoured vehicles. Def. Technol. 2024, 32, 173–195. [Google Scholar] [CrossRef]
- Ben-Dor, G.; Dubinsky, A.; Elperin, T. On the order of plates providing the maximum ballistic limit velocity of a layered armor. Int. J. Impact Eng. 1999, 22, 741–755. [Google Scholar] [CrossRef]
- Deng, Y.F.; Zhang, W.; Yang, Y.G.; Shi, L.Z.; Wei, G. Experimental investigation on the ballistic performance of double-layered plates subjected to impact by projectile of high strength. Int. J. Impact Eng. 2014, 70, 38–49. [Google Scholar]
- Elek, P.; Jaramaz, S.; Micković, D. Modeling of perforation of plates and multi-layered metallic targets. Int. J. Solids Struct. 2005, 42, 1209–1224. [Google Scholar] [CrossRef]
- Liu, H.S.; Zhang, B.; Zhang, G.P. Enhanced toughness and fatigue strength of cold roll bonded Cu/Cu laminated composites with mechanical contrast. Scr. Mater. 2011, 65, 891–894. [Google Scholar] [CrossRef]
- Alic, J.A.; Danesh, A. Fracture of laminates combining 2024-T3 and 7075-T6 aluminum alloys. Eng. Fract. Mech. 1978, 10, 177–186. [Google Scholar] [CrossRef]
- Hassan, H.A.; Lewandowski, J.J. Laminated nanostructure composites with improved bend ductility and toughness. Scr. Mater. 2009, 61, 1072–1074. [Google Scholar] [CrossRef]
- Pozuelo, M.; Carreno, F.; Ruano, O.A. Delamination effect on the impact toughness of an ultrahigh carbon–mild steel laminate composite. Compos. Sci. Technol. 2006, 66, 2671–2676. [Google Scholar] [CrossRef]
- Pozuelo, M.; Carreno, F.; Cepeda-Jimenez, C.M.; Ruano, O.A. Effect of hot rolling on bonding characteristics and impact behavior of a laminated composite material based on UHCS-1.35 Pct C. Metall. Mater. Trans. A 2008, 39, 666–671. [Google Scholar] [CrossRef]
- Cepeda-Jimenez, C.M.; Pozuelo, M.; Garcia-Infanta, J.M.; Ruano, O.A.; Carreno, F. Influence of the alumina thickness at the interfaces on the fracture mechanisms of aluminium multilayer composites. Mater. Sci. Eng. A 2008, 496, 133–142. [Google Scholar] [CrossRef]
- Hassan, H.A.; Lewandowski, J.J.; Abdel-latif, M.H. Effects of lamination and changes in layer thickness on fatigue-crack propagation of lightweight laminated metal composites. Metall. Mater. Trans. A 2004, 35, 45–52. [Google Scholar] [CrossRef]
- Tekyeh-Marouf, B.; Bagheri, R. Fracture behavior of multi-layered composites under impact loading. Mater. Sci. Eng. A 2007, 448, 20–24. [Google Scholar] [CrossRef]
- Cepeda-Jimenez, C.M.; Alderliesten, R.C.; Ruano, O.A.; Carreno, F. Damage tolerance assessment by bend and shear tests of two multilayer composites: Glass fibre reinforced metal laminate and aluminium roll-bonded laminate. Compos. Sci. Technol. 2009, 69, 343–348. [Google Scholar] [CrossRef]
- Cepeda-Jimenez, C.M.; Hidalgo, P.; Pozuelo, M.; Ruano, O.A.; Carreno, F. Influence of constituent materials on the impact toughness and fracture mechanisms of hot-roll-bonded aluminum multilayer laminates. Metall. Mater. Trans. A 2010, 41, 61–72. [Google Scholar] [CrossRef]
- Tekyeh-Marouf, B.; Bagheri, R.; Mahmudi, R. Effects of number of layers and adhesive ductility on impact behavior of laminates. Mater. Lett. 2004, 58, 2721–2724. [Google Scholar] [CrossRef]
- Cepeda-Jimenez, C.M.; Pozuelo, M.; Ruano, O.A.; Carreno, F. Influence of the thermomechanical processing on the fracture mechanisms of high strength aluminium/pure aluminium multilayer laminate materials. Mater. Sci. Eng. A 2008, 490, 319–327. [Google Scholar] [CrossRef]
- Roghani, H.; Borhani, E.; Shams, S.A.A.; Lee, C.S.; Jafarian, H.R. Effect of concurrent accumulative roll bonding (ARB) process and various heat treatment on the microstructure, texture and mechanical properties of AA1050 sheet s. J. Mater. Res. Technol. 2022, 18, 1295–1306. [Google Scholar] [CrossRef]
- Song, L.; Xie, Z.; Gao, H.; Kong, C.; Yu, H. Microstructure and mechanical properties of ARB-processed AA1050/AA5052 multilayer laminate sheets during cryorolling. Mater. Lett. 2022, 307, 130998. [Google Scholar] [CrossRef]
- Liu, W.; Ke, Y.; Sugio, K.; Liu, X.; Guo, Y.; Sasaki, G. Microstructure and mechanical properties of Al2O3-particle-reinforced Al-matrix composite sheets produced by accumulative roll bonding (ARB). Mater. Sci. Eng. A 2022, 850, 143574. [Google Scholar] [CrossRef]
- Wang, X.; Xu, Y.; Yang, L.; Chen, C.; Song, Z.; Cui, J. Effect of Lubricating Oil and Wiper on Super-High Strength 7055 Aluminum Alloy Ingots. Crystals 2023, 13, 88. [Google Scholar] [CrossRef]
- Wang, K.; Hu, S.; Wang, T.; Xie, W.; Guo, T.; Li, F.; Luo, R. Microstructural Evolution and Mechanical Properties of 7075 Aluminium Alloy during Semi-Solid Compression Deformation. Crystals 2022, 12, 1119. [Google Scholar] [CrossRef]
- Li, Z.; Li, Y.; Zhou, R.; Xie, L.; Wang, Q.; Zhang, L.; Ji, Q.; Xu, B. Microstructure and Properties of Semi-Solid 7075 Aluminum Alloy Processed with an Enclosed Cooling Slope Channel. Crystals 2023, 13, 1102. [Google Scholar] [CrossRef]
- Roth, C.C.; Fras, T.; Mohr, D. Dynamic perforation of lightweight armor: Temperature-dependent plasticity and fracture of aluminum 7020-T6. Mech. Mater. 2020, 149, 103537. [Google Scholar] [CrossRef]
- Teng, X.; Wierzbicki, T.; Huang, M. Ballistic resistance of double-layered armor plates. Int. J. Impact Eng. 2008, 35, 870–884. [Google Scholar] [CrossRef]
- Dey, S.; Børvik, T.; Teng, X.; Wierzbicki, T.; Hopperstad, O. On the ballistic resistance of double-layered steel plates: An experimental and numerical investigation. Int. J. Solids Struct. 2007, 44, 6701–6723. [Google Scholar] [CrossRef]
- Teng, X.; Dey, S.; Børvik, T.; Wierzbicki, T. Protection performance of double-layered metal shields against projectile impact. J. Mech. Mater. Struct. 2007, 2, 1309–1329. [Google Scholar] [CrossRef]
- Holmen, J.K.; Johnsen, J.; Hopperstad, O.S.; Børvik, T. Influence of fragmentation on the capacity of aluminum alloy plates subjected to ballistic impact. Eur. J. Mech.-A/Solids 2016, 55, 221–233. [Google Scholar] [CrossRef]
- Han, J.; Shi, Y.; Ma, Q.; Vershinin, V.V.; Chen, X.; Xiao, X.; Jia, B. Experimental and numerical investigation on the ballistic resistance of 2024-T351 aluminum alloy plates with various thicknesses struck by blunt projectiles. Int. J. Impact Eng. 2022, 163, 104182. [Google Scholar] [CrossRef]
- Shen, W.; Xue, F.; Li, C.; Liu, Y.; Mo, X.; Gao, Q. Study on constitutive relationship of 6061 aluminum alloy based on Johnson-Cook model. Mater. Today Commun. 2023, 37, 106982. [Google Scholar] [CrossRef]
- Zhang, D.N.; Shangguan, Q.Q.; Xie, C.J.; Liu, F. A modified Johnson–Cook model of dynamic tensile behaviors for 7075-T6 aluminum alloy. J. Alloys Compd. 2015, 619, 186–194. [Google Scholar] [CrossRef]
- Wan, J.; Zhu, Y.; Zhang, Y.; Zhao, H. Strain rate effect and dynamic constitutive model of 7A04-T6 high-strength aluminium alloy. Structures 2023, 53, 1250–1266. [Google Scholar] [CrossRef]
- Tan, J.Q.; Zhan, M.; Liu, S.; Huang, T.; Guo, J.; Yang, H. A modified Johnson-Cook model for tensile flow behaviors of 7050-T7451 aluminum alloy at high strain rates. Mater. Sci. Eng. A 2015, 631, 214–219. [Google Scholar] [CrossRef]
- Chen, G.; Ren, C.Z.; Ke, Z.H.; Li, J.; Yang, X.P. Modeling of flow behavior for 7050-T7451 aluminum alloy considering microstructural evolution over a wide range of strain rates. Mech. Mater. 2016, 95, 146–157. [Google Scholar] [CrossRef]
- Johnson, G.R. A Constitutive Model and Data for Metals Subject to Large Strains, High Strain Rate and High Temperatures. In Proceedings of the 7th International Symposium on Ballistics, Hague, The Netherlands, 19–21 April 1983; pp. 541–547. [Google Scholar]
- Cao, Y.; Zhen, Y.; Song, M.; Yi, H.; Li, F.; Li, X. Determination of Johnson–Cook parameters and evaluation of Charpy impact test performance for X80 pipeline steel. Int. J. Mech. Sci. 2020, 179, 105627. [Google Scholar] [CrossRef]
- Miyambo, M.E.; Von Kallon, D.V.; Pandelani, T.; Reinecke, J.D. Review of the development of the split Hopkinson pressure bar. Procedia CIRP 2023, 119, 800–808. [Google Scholar] [CrossRef]
- Wang, B.; Xiao, X.; Astakhov, V.P.; Liu, Z. The effects of stress triaxiality and strain rate on the fracture strain of Ti6Al4V. Eng. Fract. Mech. 2019, 219, 106627. [Google Scholar] [CrossRef]
- Gambirasio, L.; Rizzi, E. On the calibration strategies of the Johnson–Cook strength model: Discussion and applications to experimental data. Mater. Sci. Eng. A 2014, 610, 370–413. [Google Scholar] [CrossRef]
- Senthil, K.; Iqbal, M.A.; Chandel, P.S.; Gupta, N.K. Study of the constitutive behavior of 7075-T651 aluminum alloy. Int. J. Impact Eng. 2017, 108, 171–190. [Google Scholar] [CrossRef]
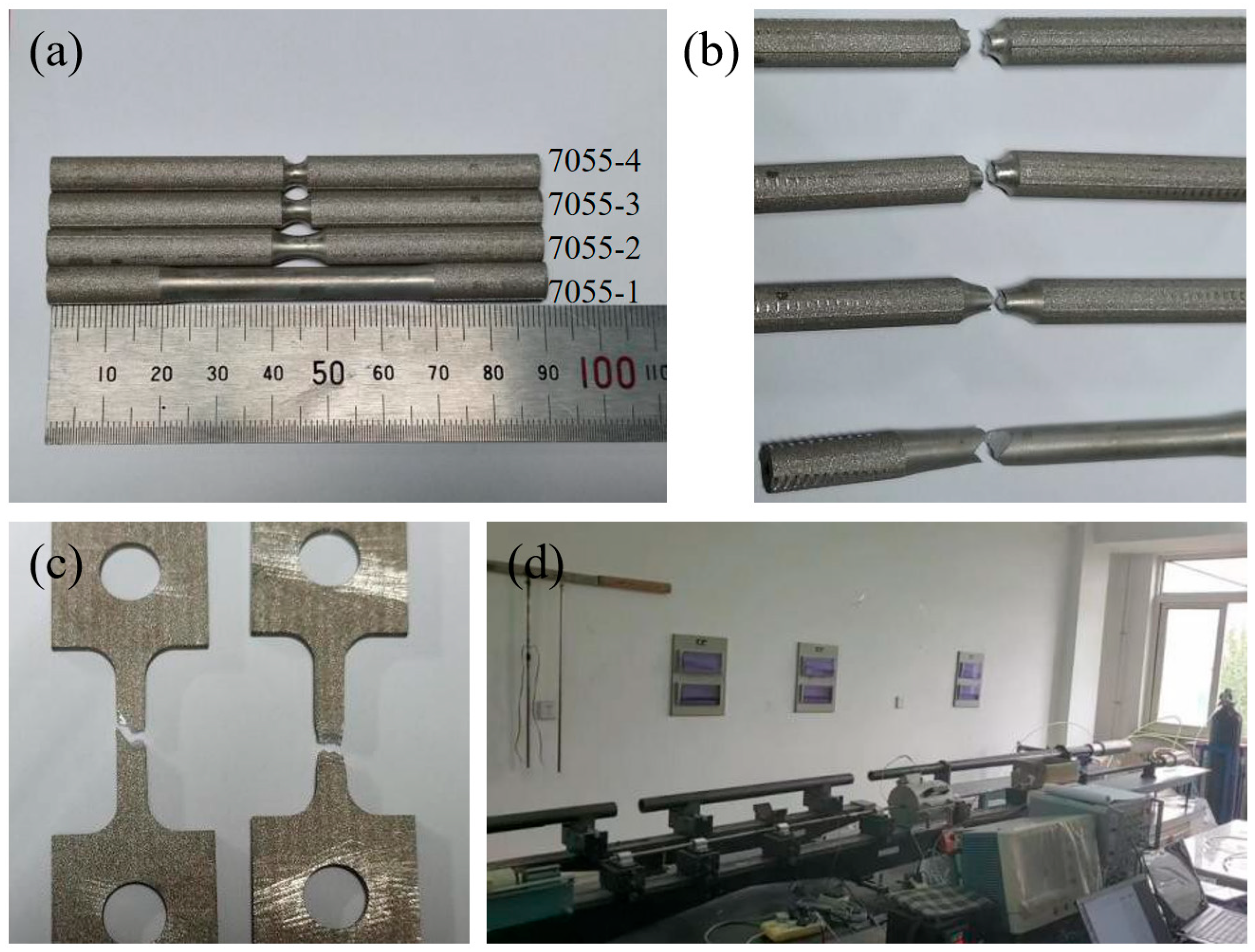
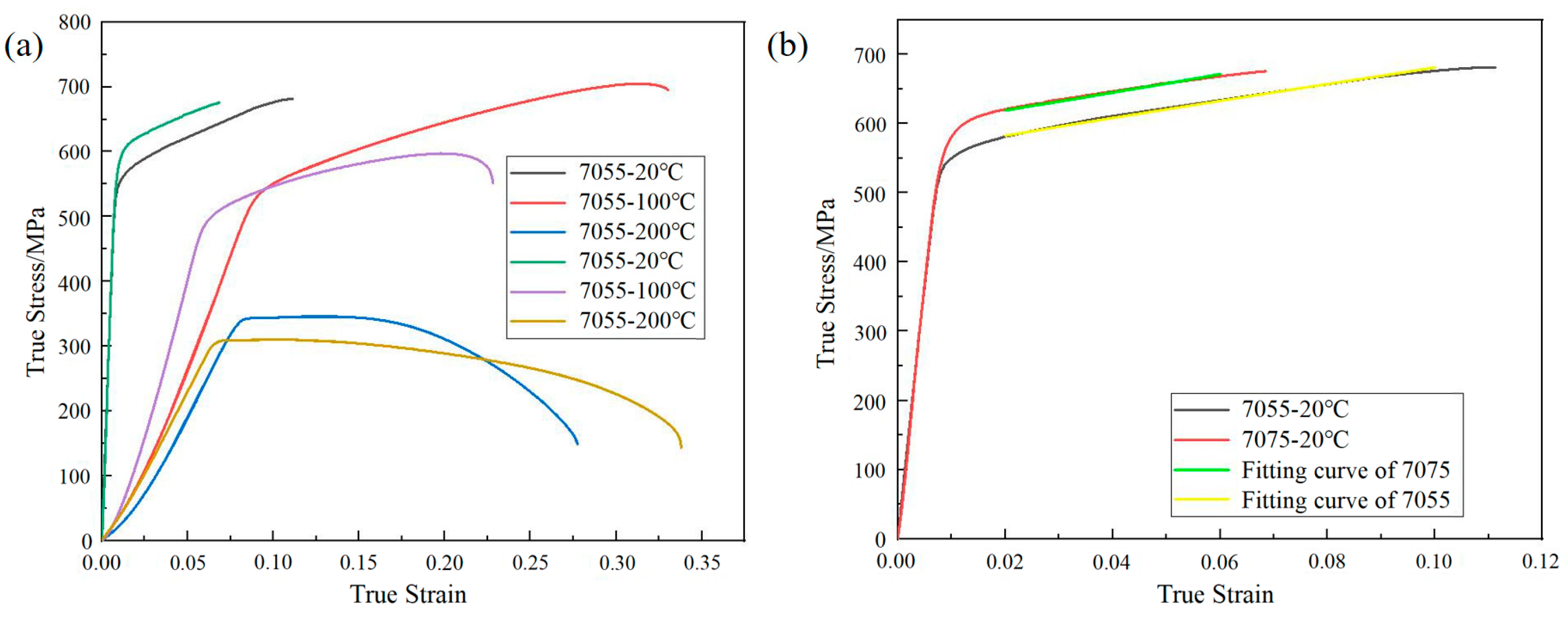
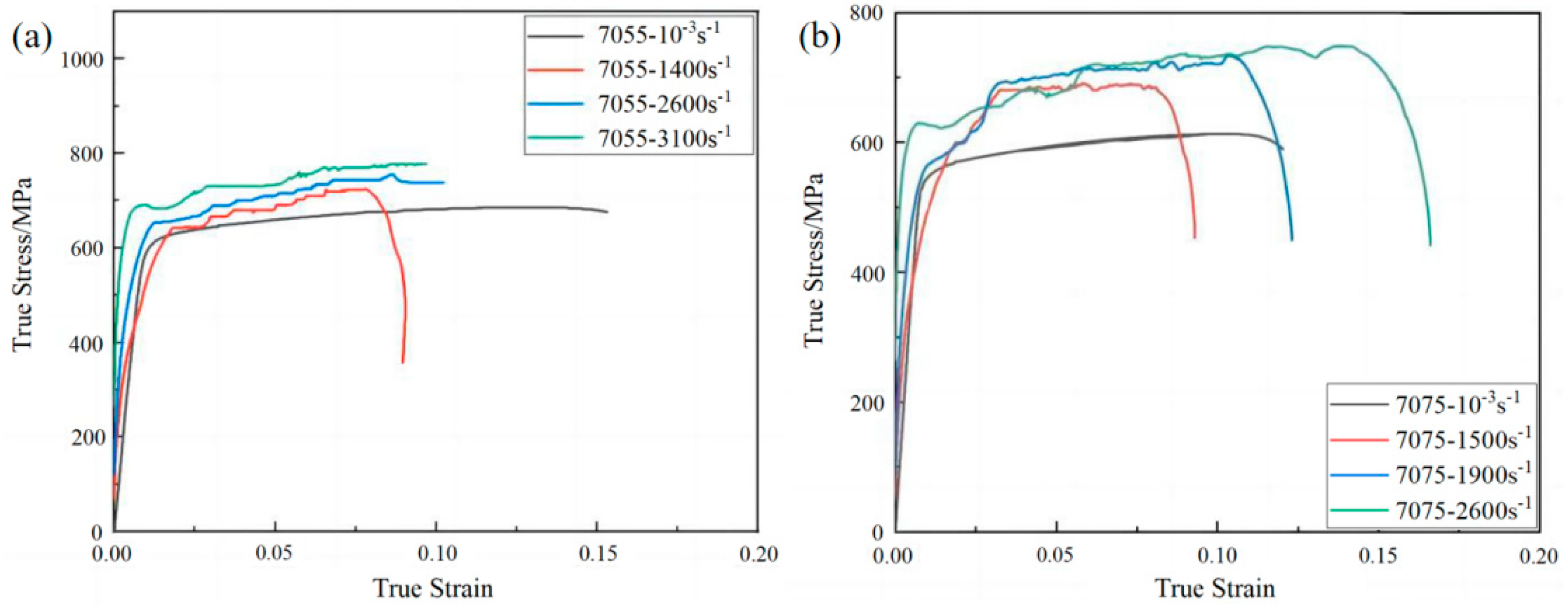
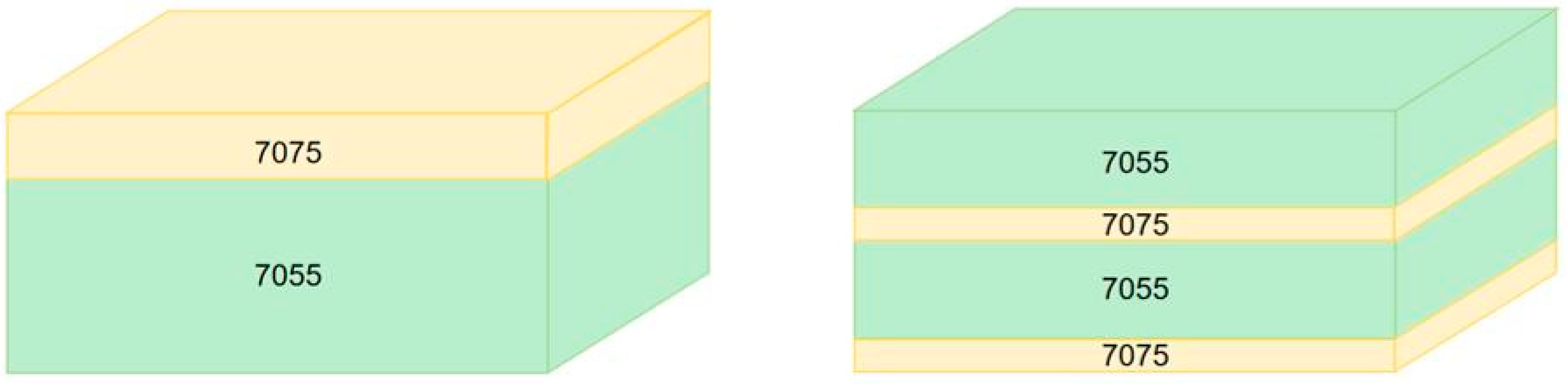

| Alloy | Element Content | ||||||||
|---|---|---|---|---|---|---|---|---|---|
| Si | Fe | Cu | Mn | Mg | Cr | Zn | Ti | Zr | |
| 7055 | 0.1 | 0.15 | 2.4 | 0.05 | 1.96 | 0.04 | 7.75 | 0.06 | 0.08 |
| 7075 | 0.4 | 0.5 | 1.8 | 0.3 | 2.4 | 0.1 | 5.8 | 0.1 | — |
| Parameters | Meaning |
|---|---|
| Flow stress | |
| Current experimental strain rate | |
| Reference strain rate | |
| Current temperature | |
| Material melting point | |
| Room temperature | |
| Yield strength at reference | |
| A | strain rate |
| B | Strain hardening factor |
| n | Strain hardening index |
| C | Factors related to strain rate reinforcement |
| m | Factors related to thermal softening |
| Alloy | A | B | n | C | m |
|---|---|---|---|---|---|
| 7055 | 555 | 1097 | 0.94 | 0.011 | 0.32 |
| 7075 | 595 | 1421 | 1.04 | 0.001 | 1.47 |
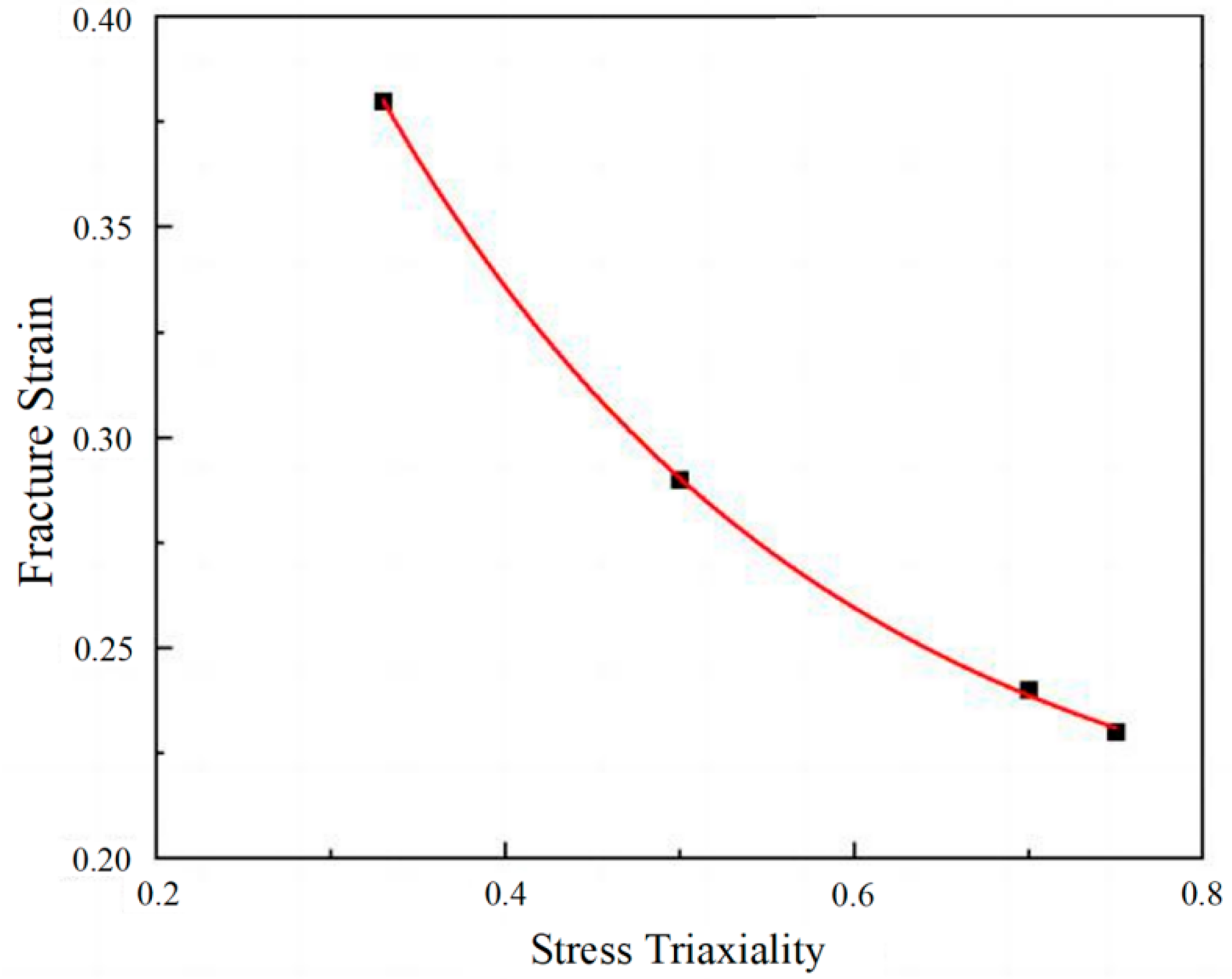
| Sample | Initial Minimum Cross-Sectional Diameter (mm) | Minimum Cross-Sectional Diameter After Fracture (mm) | Fracture Strain |
|---|---|---|---|
| 7055-1 | 6.01 | 4.97 | 0.38 |
| 7055-2 | 4.06 | 3.64 | 0.23 |
| 7055-3 | 4.03 | 3.56 | 0.24 |
| 7055-4 | 4.16 | 3.58 | 0.29 |
| 7075-1 | 6.02 | 5.12 | 0.16 |
| 7075-2 | 4.06 | 3.49 | 0.17 |
| 7075-3 | 4.06 | 3.34 | 0.20 |
| 7075-4 | 4.09 | 3.37 | 0.19 |
| Alloy | |||||
|---|---|---|---|---|---|
| 7055 | 0.195 | 0.670 | −3.900 | 0.04 | 4.72 |
| 7075 [42] | −0.428 | 0.757 | −3.408 | −0.003 | 24.93 |
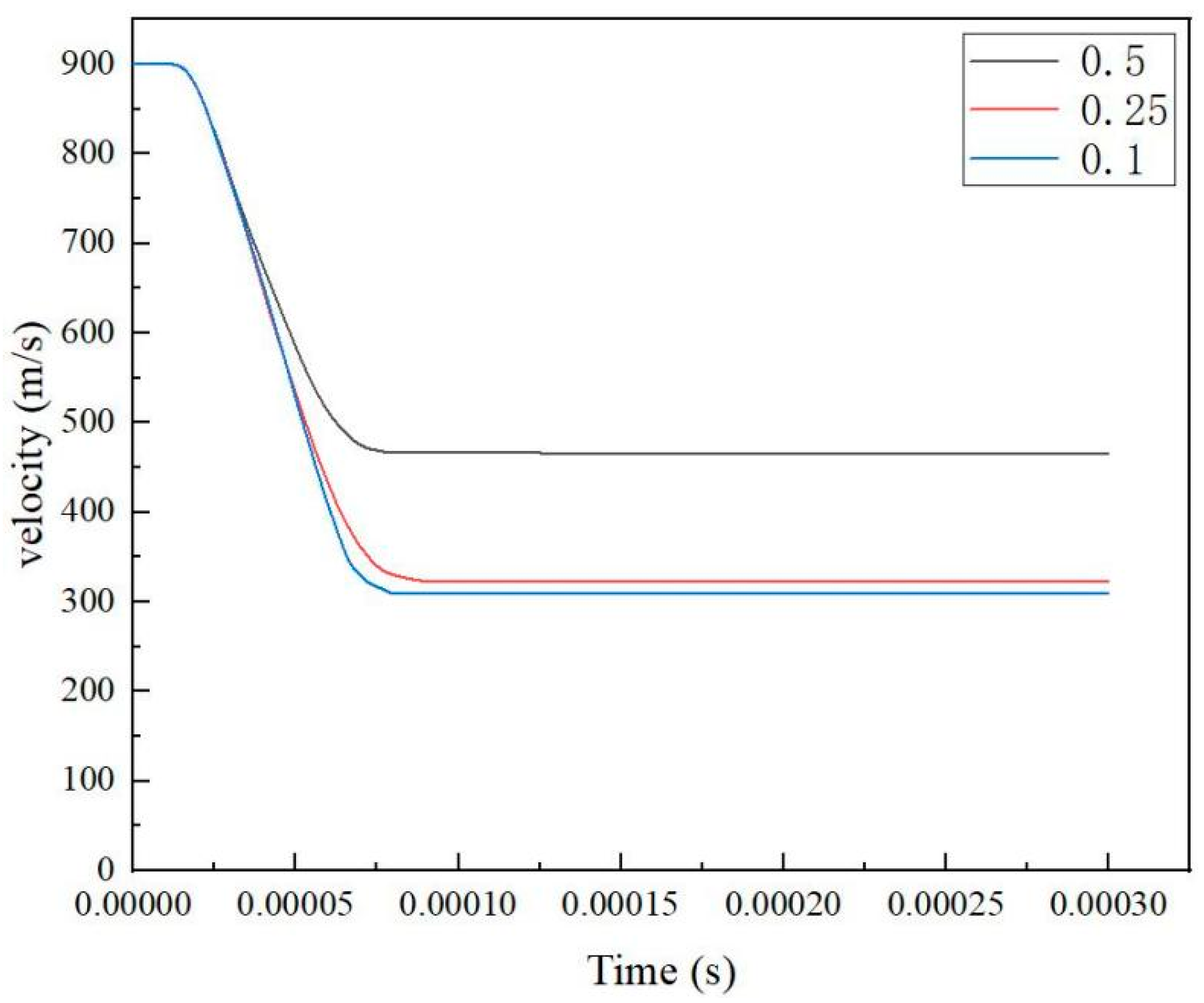
| Mesh Size | Residual Velocity (m/s) | Computational Time (h) |
|---|---|---|
| 0.5 | 466 | 0.2 |
| 0.25 | 322 | 0.45 |
| 0.1 | 309 | 1.5 |
| Initial Velocity (m/s) | Residual Velocity (m/s) |
|---|---|
| 800 | 0 |
| 850 | 81 |
| 900 | 225 |
| 1000 | 447 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, Q.; Zhang, S.; Yin, M.; Zhang, H.; Liu, X.; Mei, R.; Cong, F.; Zhang, Y.; Cao, Y. Simulation of the Penetration Process of 7xxx Aluminum Alloy Laminates with Different Configurations. Materials 2025, 18, 2357. https://doi.org/10.3390/ma18102357
Wang Q, Zhang S, Yin M, Zhang H, Liu X, Mei R, Cong F, Zhang Y, Cao Y. Simulation of the Penetration Process of 7xxx Aluminum Alloy Laminates with Different Configurations. Materials. 2025; 18(10):2357. https://doi.org/10.3390/ma18102357
Chicago/Turabian StyleWang, Qunjiao, Shuhan Zhang, Meilin Yin, Hui Zhang, Xinyu Liu, Ruibin Mei, Fuguan Cong, Yunlong Zhang, and Yu Cao. 2025. "Simulation of the Penetration Process of 7xxx Aluminum Alloy Laminates with Different Configurations" Materials 18, no. 10: 2357. https://doi.org/10.3390/ma18102357
APA StyleWang, Q., Zhang, S., Yin, M., Zhang, H., Liu, X., Mei, R., Cong, F., Zhang, Y., & Cao, Y. (2025). Simulation of the Penetration Process of 7xxx Aluminum Alloy Laminates with Different Configurations. Materials, 18(10), 2357. https://doi.org/10.3390/ma18102357






