First-Principles Study on the Electrical and Thermal Conductivities of Cu–Zn Binary Alloys
Abstract
:1. Introduction
2. Computational Methods
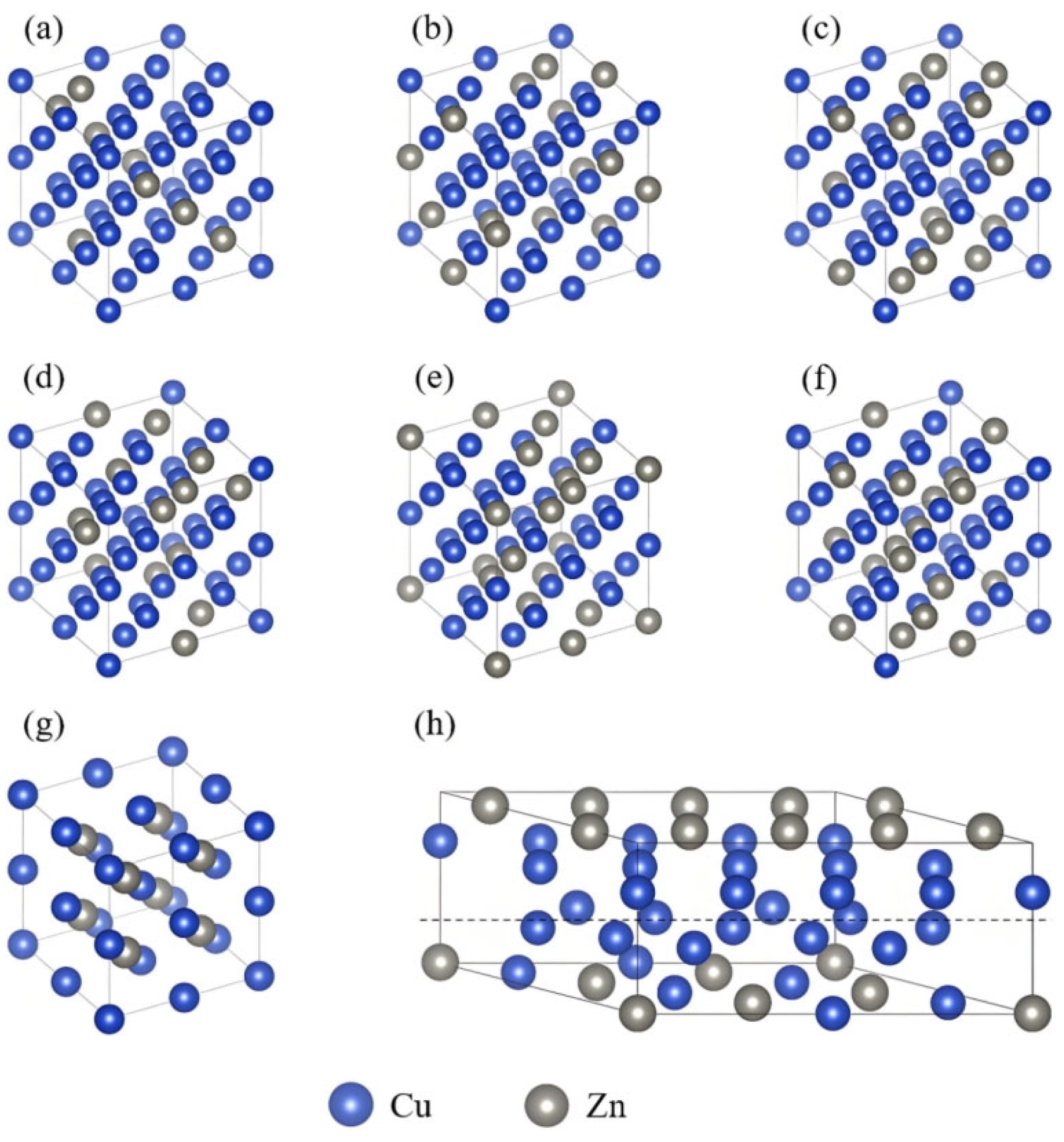
2.1. Computational Models
2.2. Electrical Conductivity Calculations
2.3. Thermal Conductivity Calculations
2.4. Computational Parameters
3. Results and Discussion
3.1. Structural Optimization Results
3.2. Electrical Conductivity: Results and Discussion
3.3. Effect of Zn Content on the Electronic Structure
3.4. Thermal Conductivity: Results and Discussion
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Berndorf, S.; Markelov, A.; Guk, S.; Mandel, M.; Krüger, L.; Prahl, U. Development of a Dezincification-Free Alloy System for the Manufacturing of Brass Instruments. Metals 2024, 14, 800. [Google Scholar] [CrossRef]
- Brans, K.; Kind, S.; Meurer, M.; Bergs, T. Influence of the Material Production Route on the Material Properties and the Machinability of the Lead-Free Copper-Zinc-Alloy CuZn40 (CW509L). Metals 2024, 14, 747. [Google Scholar] [CrossRef]
- Stavroulakis, P.; Toulfatzis, A.I.; Pantazopoulos, G.A.; Paipetis, A.S. Machinable leaded and eco-friendly brass alloys for high performance manufacturing processes: A critical review. Metals 2022, 12, 246. [Google Scholar] [CrossRef]
- Suárez, L.; Rodriguez-Calvillo, P.; Cabrera, J.; Martínez-Romay, A.; Majuelos-Mallorquín, D.; Coma, A. Hot working analysis of a CuZn40Pb2 brass on the monophasic (β) and intercritical (α+ β) regions. Mater. Sci. Eng. A 2015, 627, 42–50. [Google Scholar] [CrossRef]
- Garg, R.; Ranganathan, S.; Suwas, S. Effect of mode of rolling on development of texture and microstructure in two-phase (α+ β) brass. Mater. Sci. Eng. A 2010, 527, 4582–4592. [Google Scholar] [CrossRef]
- Yu, Y.; Chen, W.; Xie, L.; Song, Y. Effect of heat treatment on microstructure evolution, phase transformation and mechanical properties of dual phase Cu-Zn alloy. J. Alloys Compd. 2022, 904, 163960. [Google Scholar] [CrossRef]
- Choi, G.; Kim, H.S.; Lee, K.; Park, S.H.; Cha, J.; Chung, I.; Lee, W.B. Study on thermal conductivity and electrical resistivity of Al-Cu alloys obtained by Boltzmann transport equation and first-principles simulation: Semi-empirical approach. J. Alloys Compd. 2017, 727, 1237–1242. [Google Scholar] [CrossRef]
- Ho, C.; Ackerman, M.; Wu, K.; Oh, S.; Havill, T. Thermal conductivity of ten selected binary alloy systems. J. Phys. Chem. Ref. Data 1978, 7, 959–1178. [Google Scholar] [CrossRef]
- Ho, C.Y.; Ackerman, M.; Wu, K.; Havill, T.; Bogaard, R.; Matula, R.; Oh, S.; James, H. Electrical resistivity of ten selected binary alloy systems. J. Phys. Chem. Ref. Data 1983, 12, 183–322. [Google Scholar] [CrossRef]
- Jang, H.W.; Hong, J.-W. Influence of zinc content on the mechanical behaviors of Cu-Zn alloys by molecular dynamics. Materials 2020, 13, 2062. [Google Scholar] [CrossRef]
- Kong, G.-X.; Ma, X.-J.; Liu, Q.-J.; Li, Y.; Liu, Z.-T.; Matter, C. Structural stability, elastic and thermodynamic properties of Au–Cu alloys from first-principles calculations. Phys. B Condens. Matter 2018, 533, 58–62. [Google Scholar] [CrossRef]
- ASTM B36/B36M-23 (2023); Standard Specification for Brass Plate, Sheet, Strip, and Rolled Bar. ASTM International: West Conshohocken, PA, USA, 2023. Available online: https://cdn.standards.iteh.ai/samples/116325/cb60469514a0475baecfb788ef9a3d14/ASTM-B36-B36M-23.pdf (accessed on 9 May 2025).
- Dhaka, R.; Banik, S.; Shukla, A.; Vyas, V.; Chakrabarti, A.; Barman, S.; Ahuja, B.; Sharma, B. Electronic structure of α-and β-brass. Phys. Rev. B Condens. Matter Mater. Phys. 2008, 78, 073107. [Google Scholar] [CrossRef]
- Hong, H.; Wang, Q.; Dong, C.; Liaw, P.K. Understanding the Cu-Zn brass alloys using a short-range-order cluster model: Significance of specific compositions of industrial alloys. Sci. Rep. 2014, 4, 7065. [Google Scholar] [CrossRef] [PubMed]
- Liu, Q.; Cheng, L. Structural evolution and electronic properties of Cu-Zn alloy clusters. J. Alloys Compd. 2019, 771, 762–768. [Google Scholar] [CrossRef]
- Müller, S.; Zunger, A. Structure of ordered and disordered α-brass. Phys. Rev. B 2001, 63, 094204. [Google Scholar] [CrossRef]
- Temmerman, W.; Durham, P.; Szotek, Z.; Sob, M.; Larsson, C. Electronic effects in the order-disorder transition in β brass. J. Phys. F Met. Phys. 1988, 18, 2387. [Google Scholar] [CrossRef]
- Turchi, P.; Sluiter, M.; Pinski, F.; Johnson, D.D.; Nicholson, D.; Stocks, G.; Staunton, J. First-principles study of phase stability in Cu-Zn substitutional alloys. Phys. Rev. Lett. 1991, 67, 1779. [Google Scholar] [CrossRef]
- Upreti, D.; Basnet, R.; Sharma, M.M.; Chhetri, S.K.; Acharya, G.; Nabi, M.R.U.; Sakon, J.; Benamara, M.; Mortazavi, M.; Hu, J. Medium-Entropy Engineering of Magnetism in Layered Antiferromagnet CuxNi2(1−x)CrxP2S6. Adv. Funct. Mater. 2024, 35, 24018722. [Google Scholar] [CrossRef]
- Sharma, M.M.; Kumar, K.; Sang, L.; Wang, X.L.; Awana, V.P.S. Type-II Superconductivity Below 4 K in Sn0.4Sb0.6. J. Alloys Compd. 2020, 844, 156140. [Google Scholar] [CrossRef]
- Li, G.; Fu, C.; Shi, W.; Jiao, L.; Wu, J.; Yang, Q.; Saha, R.; Kamminga, M.E.; Srivastava, A.K.; Liu, E.; et al. Dirac nodal arc semimetal PtSn4: An ideal platform for understanding surface properties and catalysis for hydrogen evolution. Angew. Chem. 2019, 131, 13241–13246. [Google Scholar] [CrossRef]
- Li, H.; Zhou, D.; He, Q.; Si, N.; Xin, B.; Bu, S.; Ji, Q.; Li, H.; Fuchs, H.; Niu, T. Experimental realization and phase engineering of a two-dimensional SnSb binary honeycomb lattice. ACS Nano 2021, 15, 16335–16343. [Google Scholar] [CrossRef]
- Li, G.; Xu, Q.; Shi, W.; Fu, C.; Jiao, L.; Kamminga, M.E.; Yu, M.; Tüysüz, H.; Kumar, N.; Süß, V.; et al. Surface states in bulk single crystal of topological semimetal Co3Sn2S2 toward water oxidation. Sci. Adv. 2019, 5, eaaw9867. [Google Scholar] [CrossRef] [PubMed]
- Eastman, C.M.; Zhang, Q.; Zhao, J.-C. Diffusion coefficients and phase equilibria of the Cu-Zn binary system studied using diffusion couples. J. Phase Equilibria Diffus. 2020, 41, 642–653. [Google Scholar] [CrossRef]
- Van De Walle, A. Multicomponent multisublattice alloys, nonconfigurational entropy and other additions to the Alloy Theoretic Automated Toolkit. Calphad 2009, 33, 266–278. [Google Scholar] [CrossRef]
- van de Walle, A.; Tiwary, P.; de Jong, M.; Olmsted, D.L.; Asta, M.; Dick, A.; Shin, D.; Wang, Y.; Chen, L.-Q.; Liu, Z.-K. Efficient stochastic generation of special quasirandom structures. Calphad 2013, 42, 13–18. [Google Scholar] [CrossRef]
- Mahan, G.; Sofo, J. The best thermoelectric. Proc. Natl. Acad. Sci. USA 1996, 93, 7436–7439. [Google Scholar] [CrossRef]
- Madsen, G.K.; Singh, D.J. BoltzTraP. A code for calculating band-structure dependent quantities. Comput. Phys. Commun. 2006, 175, 67–71. [Google Scholar] [CrossRef]
- Van der Laag, N.J.; Fang, C.M.; De With, G.; De Wijs, G.A.; Brongersma, H.H. Geometry of {001} Surfaces of Spinel (MgAl2O4): First-Principles Simulations and Experimental Measurements. J. Am. Ceram. Soc. 2005, 88, 1544–1548. [Google Scholar] [CrossRef]
- Madsen, G.K.; Carrete, J.; Verstraete, M.J. BoltzTraP2, a program for interpolating band structures and calculating semi-classical transport coefficients. Comput. Phys. Commun. 2018, 231, 140–145. [Google Scholar] [CrossRef]
- Cui, Y.; Li, S.; Ying, T.; Bao, H.; Zeng, X. Research on the thermal conductivity of metals based on first principles. Acta Met. Sin 2020, 57, 375–384. [Google Scholar]
- Lavasani, A.; Bulmash, D.; Das Sarma, S. Wiedemann-Franz law and Fermi liquids. Phys. Rev. B 2019, 99, 085104. [Google Scholar] [CrossRef]
- Kresse, G.; Furthmüller, J. Efficiency of ab-initio total energy calculations for metals and semiconductors using a plane-wave basis set. Comput. Mater. Sci. 1996, 6, 15–50. [Google Scholar] [CrossRef]
- Kresse, G.; Hafner, J. Ab initio molecular-dynamics simulation of the liquid-metal–amorphous-semiconductor transition in germanium. Phys. Rev. B 1994, 49, 14251. [Google Scholar] [CrossRef]
- Blöchl, P.E. Projector augmented-wave method. Phys. Rev. B 1994, 50, 17953. [Google Scholar] [CrossRef]
- Kresse, G.; Joubert, D. Projector augmented-wave method. Phys. Rev. B 1999, 59, 1758. [Google Scholar] [CrossRef]
- Shewchuk, J.R. Classroom Figures for the Conjugate Gradient Method Without the Agonizing Pain; School of Computer Science Carnegie Mellon University: Pittsburgh, PA, USA, 1994. [Google Scholar]
- Beck, L.H.; Smith, C.S. Copper-zinc constitution diagram, redetermined in the vicinity of the beta phase by means of quantitative metallography. JOM 1952, 4, 1079–1083. [Google Scholar] [CrossRef]
- Jette, E.R.; Foote, F. Precision determination of lattice constants. J. Chem. Phys. 1935, 3, 605–616. [Google Scholar] [CrossRef]
- Keast, V.; Ewald, J.; De Silva, K.; Cortie, M.; Monnier, B.; Cuskelly, D.; Kisi, E. Optical properties and electronic structure of the Cu–Zn brasses. J. Alloys Compd. 2015, 647, 129–135. [Google Scholar] [CrossRef]
- Lu, S.; Chang, Y. The accurate evaluation of lattice spacings from back-reflection powder photographs. Proc. Phys. Soc. 1941, 53, 517. [Google Scholar] [CrossRef]
- Owen, E.A.; Yates, E.L. Precision measurements of crystal parameters. Lond. Edinb. Dublin Philos. Mag. J. Sci. 1933, 15, 472–488. [Google Scholar] [CrossRef]
- Deinzer, G.; Birner, G.; Strauch, D. Ab initio calculation of the linewidth of various phonon modes in germanium and silicon. Phys. Rev. B 2003, 67, 144304. [Google Scholar] [CrossRef]
- Gall, D. Electron mean free path in elemental metals. J. Appl. Phys. 2016, 119, 085101. [Google Scholar] [CrossRef]
- Wang, Z.; Wang, S.; Obukhov, S.; Vast, N.; Sjakste, J.; Tyuterev, V.; Mingo, N. Thermoelectric transport properties of silicon: Toward an ab initio approach. Phys. Rev. B Condens. Matter Mater. Phys. 2011, 83, 205208. [Google Scholar] [CrossRef]



| Phase | Crystal Structure | Lattice Parameters (Å) | Ref. | |
|---|---|---|---|---|
| Cu | FCC | a = 3.6077 | Exp. [40] | |
| a = 3.6072 | Exp. [41] | |||
| a = 3.615 | This study | |||
| Cu3Zn-α | FCC | a = 3.6718 | Exp. [43] | |
| a = 3.677 | This study | |||
| CuZn-β’ | BCC | a = 2.9575 | Exp. [44] | |
| a = 2.921 | This study | |||
| Zn | HCP | a = 2.6594 | c = 4.9368 | Exp. [42] |
| a = 2.6190 | c = 4.986 | This study | ||
| Zn at.% | 12.81 | 17.02 | 19.81 | 24.04 | 29.41 | 35.3 | 39.39 | 43.68 | 48.02 |
|---|---|---|---|---|---|---|---|---|---|
| Conductivity (experimental) 107 S/m | 3.56 | 3.08 | 3.04 | 2.83 | 2.46 | 2.39 | 3.28 | 4.73 | 8.25 |
| Zn at.% | 12.5 | 18.75 | 21.875 | 25 | 28.125 | 31.25 | 36 | - | 50 |
| Conductivity (computed) 107 S/m | 2.02 | 1.83 | 1.78 | 1.66 | 1.57 | 1.49 | 1.02 | - | 4.46 |
| Zn Doping Ratio | 12.5 at.% | 18.75 at.% | 21.875 at.% | 25 at.% | 28.125 at.% | 31.25 at.% |
|---|---|---|---|---|---|---|
| Integrated TDOS Value | 1.96 | 1.93 | 1.89 | 1.85 | 1.83 | 1.78 |
| Results of Wiedemann–Franz Law Calculation Method | ||||||||
| Zn at.% | 17.01 | 19.92 | 24.04 | 29.41 | 35.30 | 39.39 | 43.68 | 48.20 |
| κexp (W/m/K) | 71 | 69 | 62 | 54 | 52 | 64 | 90 | 156 |
| κWF (W/m/K) | 38.82 | 38.31 | 35.67 | 31.01 | 30.12 | 41.34 | 59.62 | 103.98 |
| Results of DFT calculation method | ||||||||
| Zn at.% | 12.5 | 18.75 | 21.875 | 25 | 28.125 | 31.25 | 36 | 50 |
| κDFT (W/m/K) | 37.74 | 34.97 | 33.92 | 31.26 | 30.83 | 29.73 | 19.55 | 80.99 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Huang, L.; Peng, B.; Yue, Q.; Huang, G.; Wang, C.; Wang, R.; Tian, N. First-Principles Study on the Electrical and Thermal Conductivities of Cu–Zn Binary Alloys. Materials 2025, 18, 2310. https://doi.org/10.3390/ma18102310
Huang L, Peng B, Yue Q, Huang G, Wang C, Wang R, Tian N. First-Principles Study on the Electrical and Thermal Conductivities of Cu–Zn Binary Alloys. Materials. 2025; 18(10):2310. https://doi.org/10.3390/ma18102310
Chicago/Turabian StyleHuang, Lei, Bo Peng, Qinchi Yue, Guojie Huang, Changhao Wang, Ruzhi Wang, and Ning Tian. 2025. "First-Principles Study on the Electrical and Thermal Conductivities of Cu–Zn Binary Alloys" Materials 18, no. 10: 2310. https://doi.org/10.3390/ma18102310
APA StyleHuang, L., Peng, B., Yue, Q., Huang, G., Wang, C., Wang, R., & Tian, N. (2025). First-Principles Study on the Electrical and Thermal Conductivities of Cu–Zn Binary Alloys. Materials, 18(10), 2310. https://doi.org/10.3390/ma18102310







