Experimental and Numerical Study of the Plasma Arc Melting of Titanium Alloys: Application to the Removal of High Density Inclusions (HDIs)
Abstract
1. Introduction
2. Methods
2.1. Trials Using the Pilot Furnace
2.1.1. Shape of the Liquid Pool
2.1.2. RTD Measurement
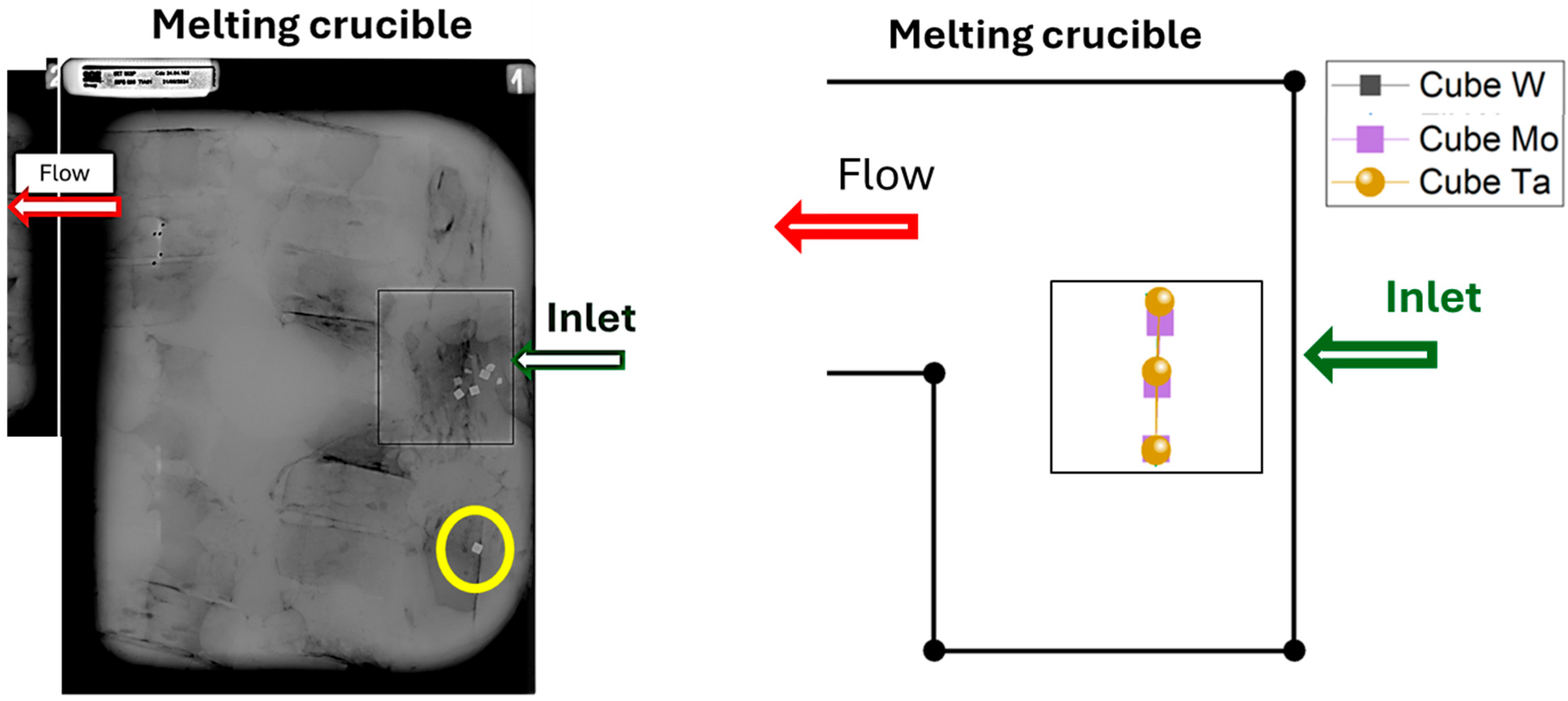
2.1.3. HDI Insemination
2.2. Mathematical Modeling
- (i)
- The surface of the liquid bath is considered to be flat; deformation of the surface under the plasma torch jet is neglected. The torch blast and its action on the bath are taken into account through a shear stress τs exerted on the bath surface and distributed as a function of the distance to the point of impact of each torch. The value of this stress has been estimated from a simplified 2D model of the plasma jet [9]. This 2D model and the work provided by Huang et al. [10] demonstrate that the deformation of the free surface can be neglected in a first stage of modeling.
- (ii)
- The effects of the electromagnetic forces are neglected. The electric current transferred between the plasma torch and the liquid bath induces an electromagnetic force. Using a 2D model, a comparison between electromagnetic and natural convection forces showed the predominance of the latter, and consequently the neglect of electromagnetic forces [23].
- (iii)
- The flow of liquid metal is mainly controlled by the movement of the torches. The torches move cyclically along a continuous trajectory with a configurable period. The heat flux and parietal stress distributions due to the torch jets follow a radial distribution around the moving impact point, and their expressions are detailed in [9].
2.2.1. Governing Transport Equations
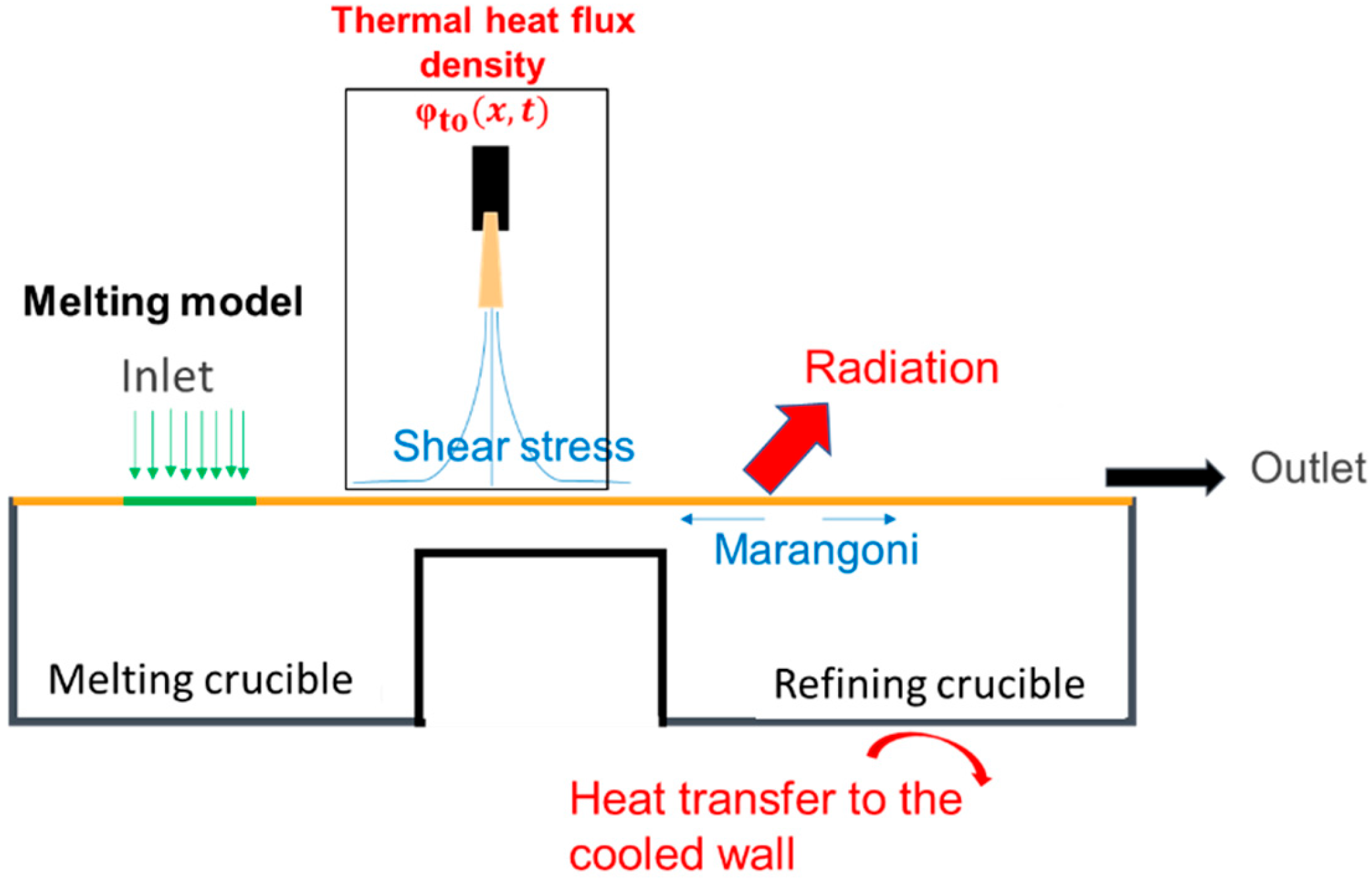
2.2.2. Boundary Conditions
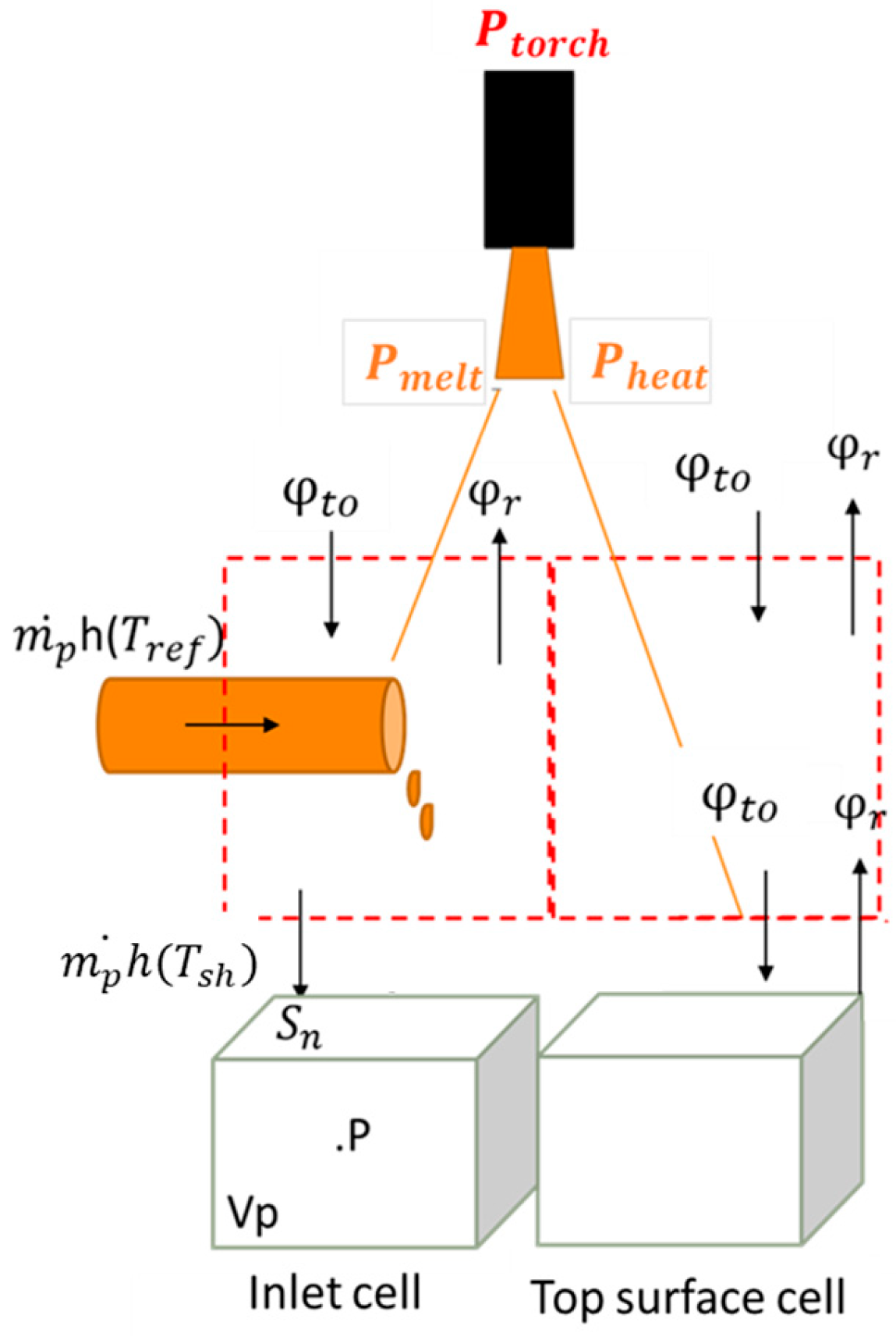
2.2.3. Melting Model
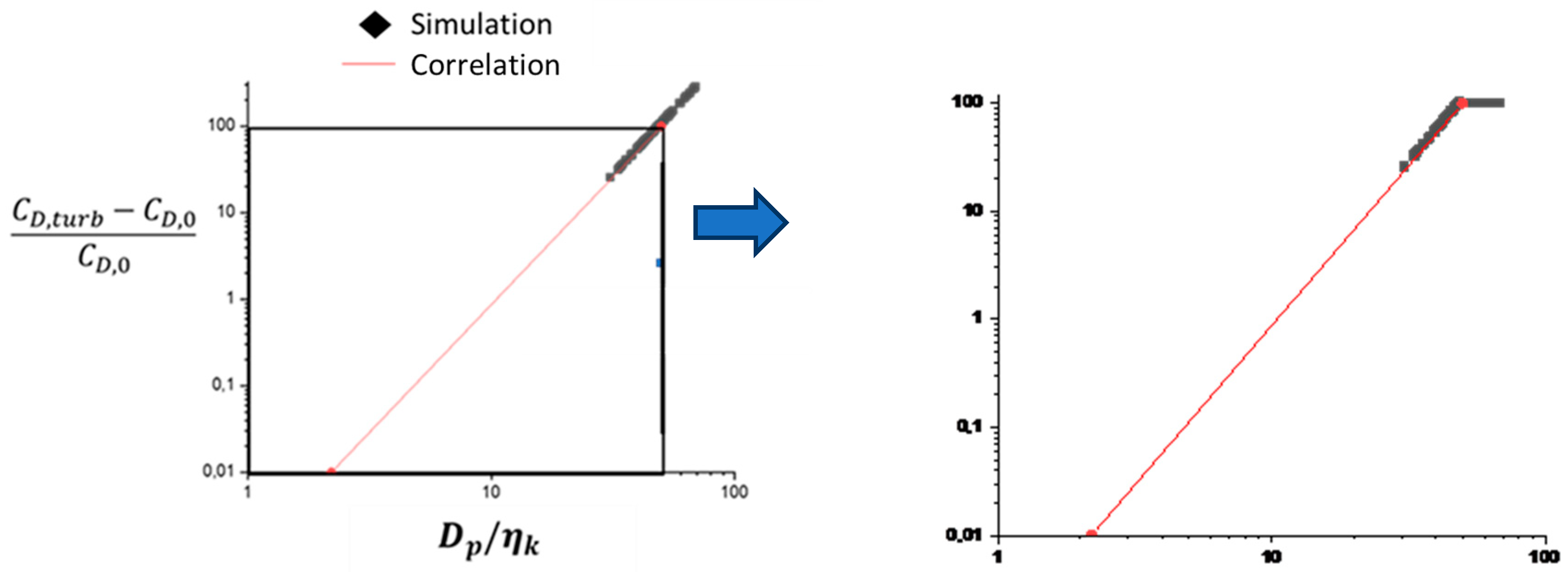
2.2.4. Trajectory of High-Density Inclusions (HDIs)
2.2.5. Numerical Procedure
3. Results and Discussion
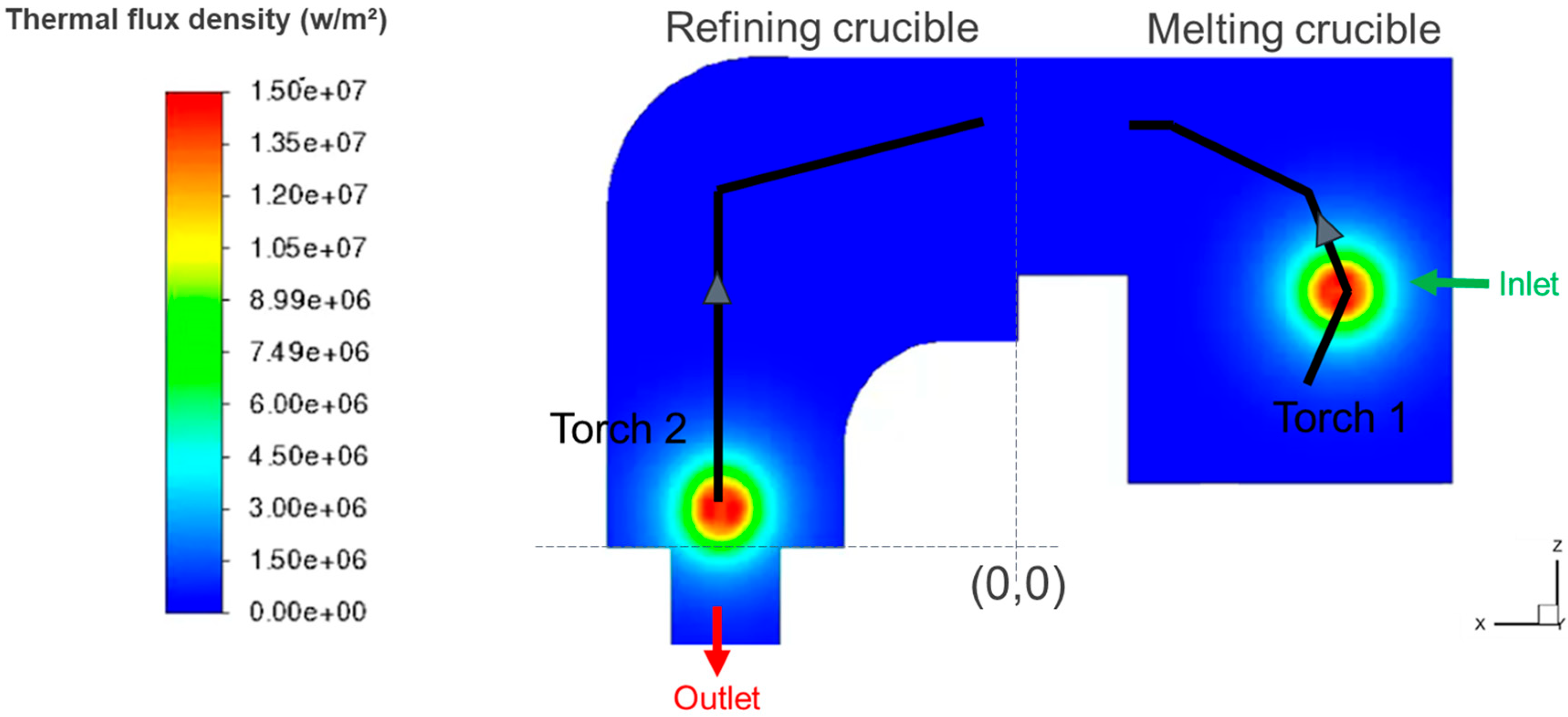
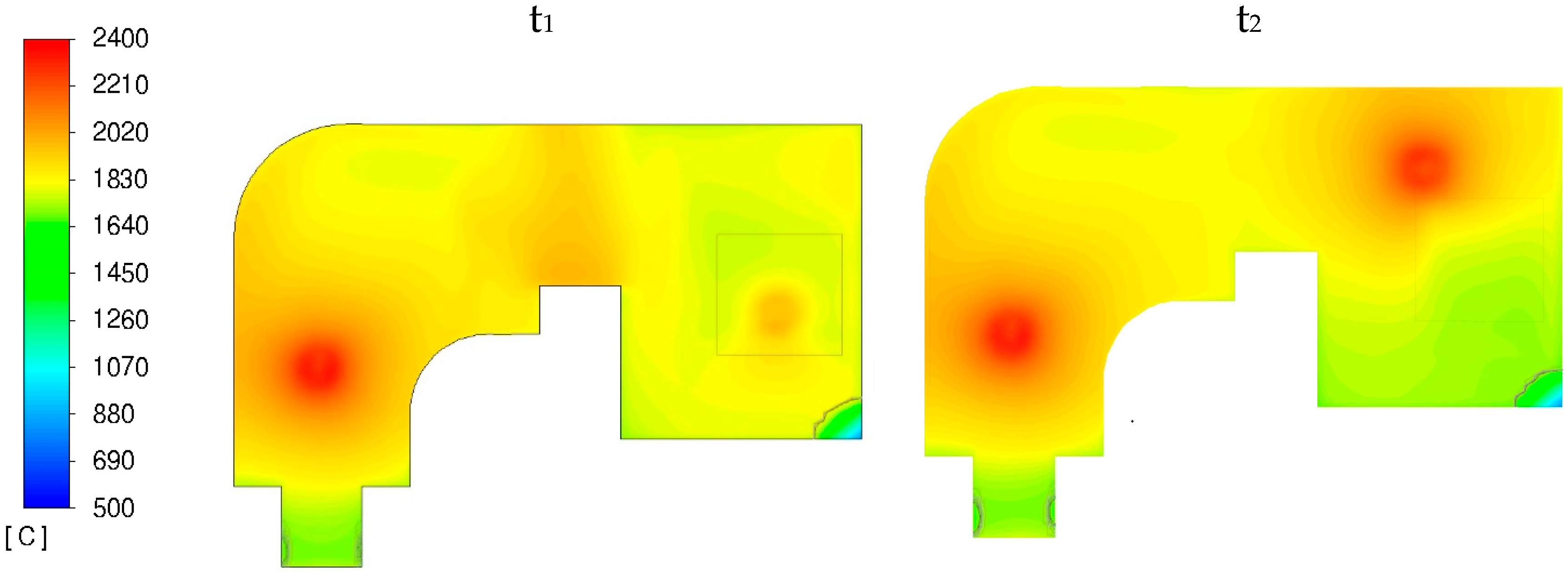
3.1. Heat Transfer and Fluid Flow
3.2. Global Balance
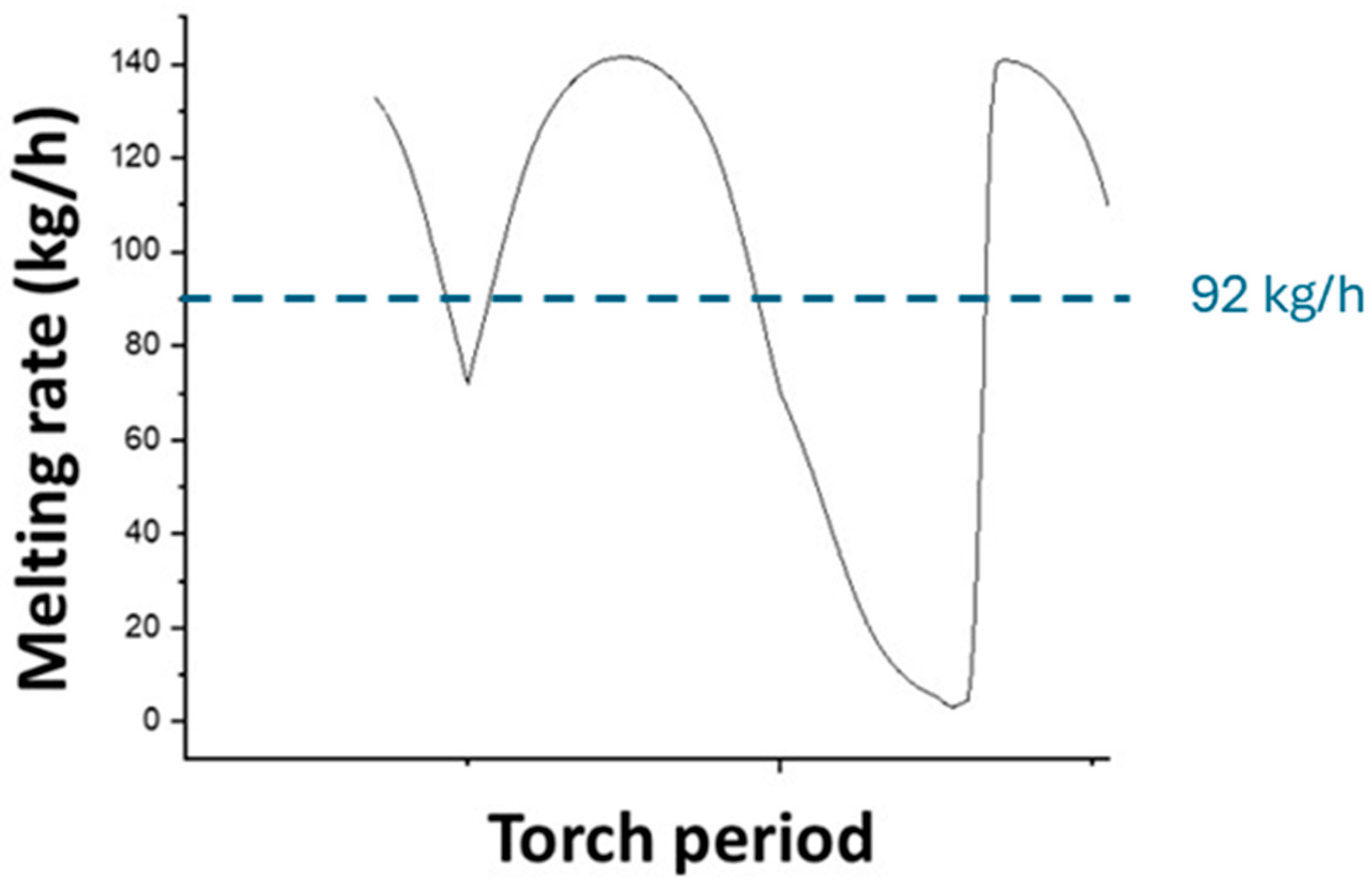
3.3. Melting Rate
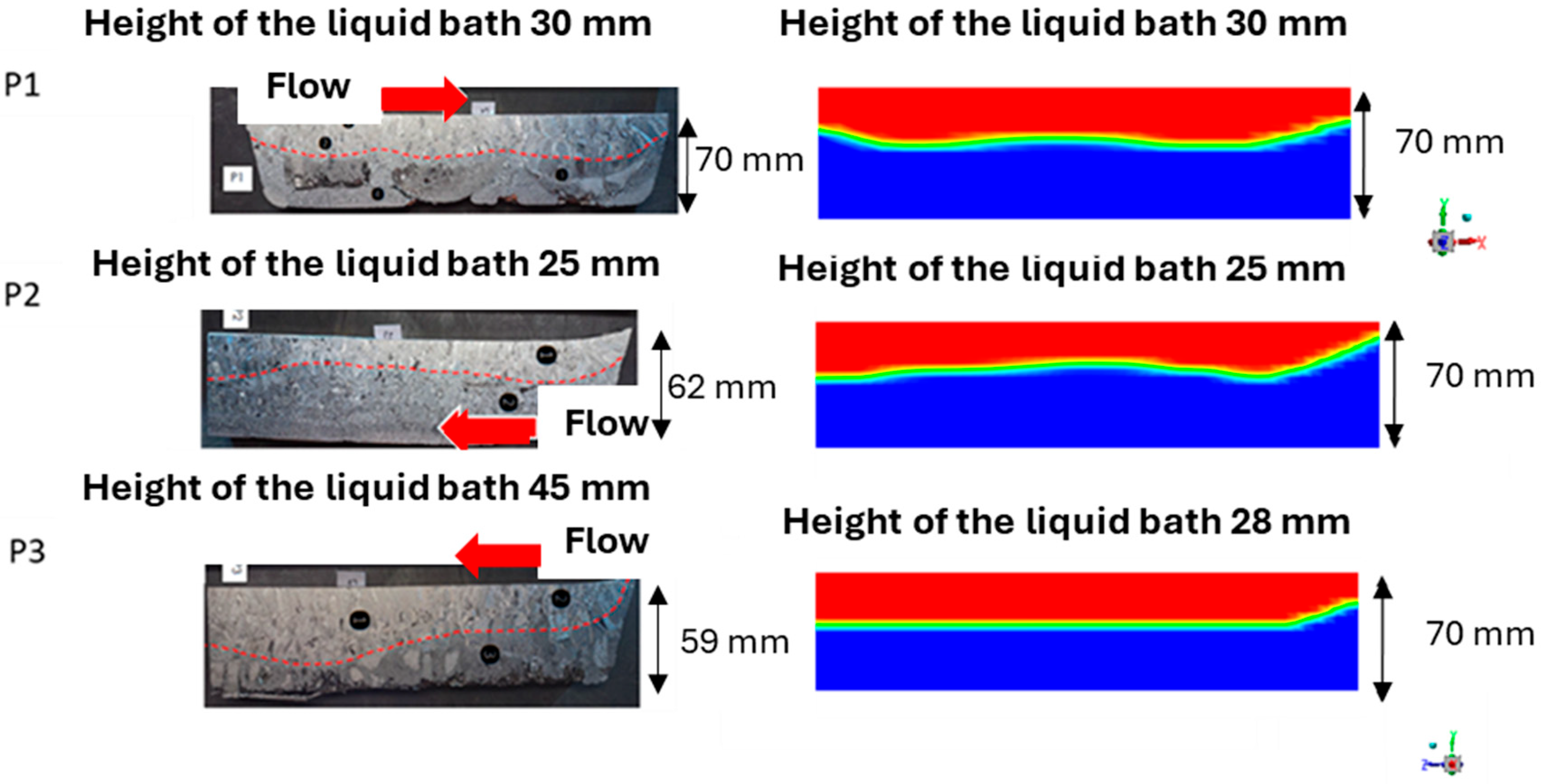
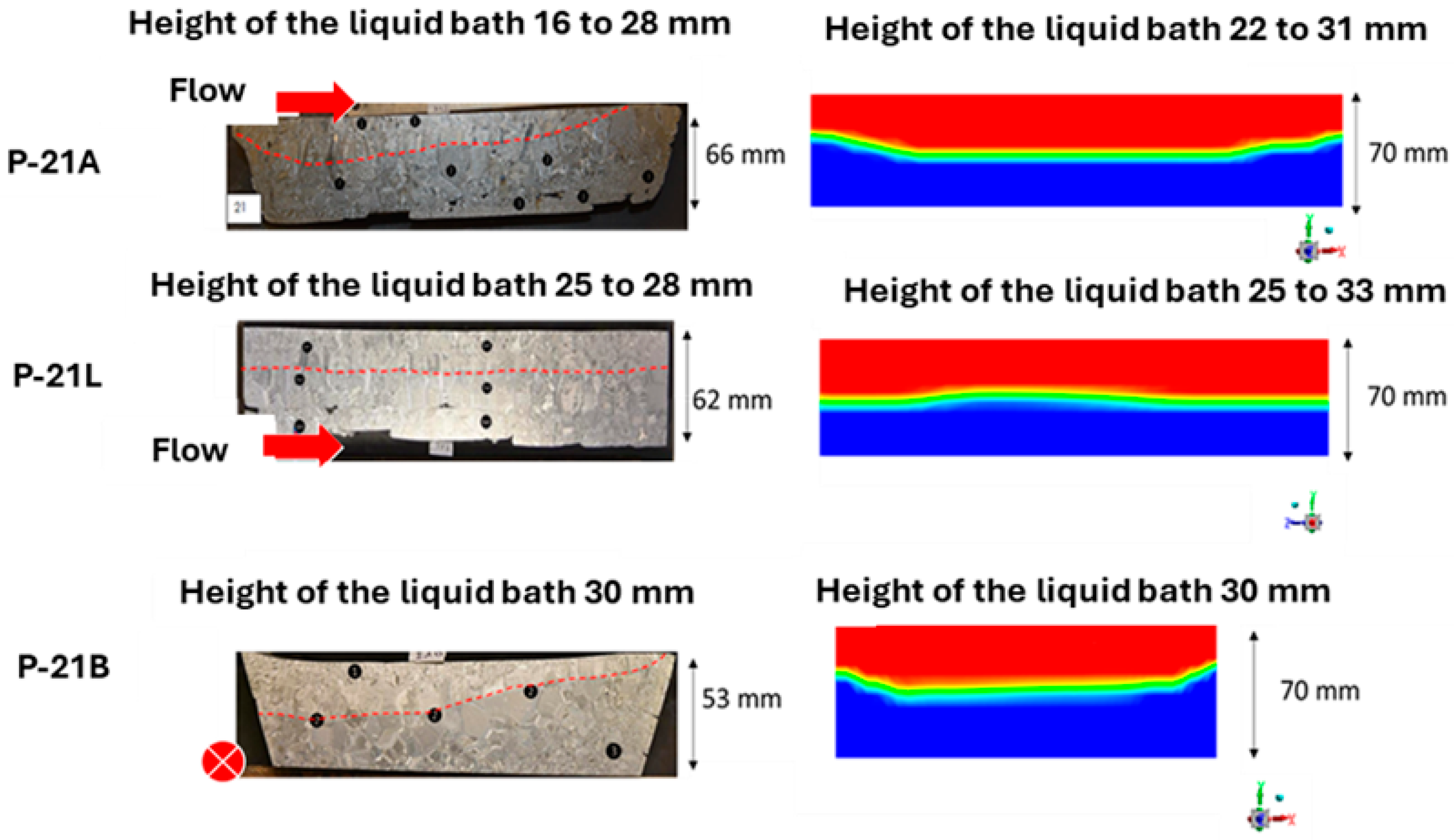
3.4. Liquid Pool Profiles
3.5. Residence Time Distribution (RTD)
3.6. Fate of HDIs
4. Conclusions and Perspectives
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Sibum, H. Titanium and Titanium Alloys—From Raw Material to Semi-finished Products. Adv. Eng. Mater. 2003, 5, 393–398. [Google Scholar] [CrossRef]
- Kenjer, A. De L’efficacité des Procédés SMAT et de Choc Laser dans L’amélioration de la Tenue à L’oxydation Haute Température D’alliages de Titane. Ph.D. Thesis, University Bourgogne-Franche Comté France, Besançon, France, 2017. [Google Scholar]
- Takanari, O.; Ouchi, T.; Okabe, T. Recent progress in titanium extraction and recycling. Metall. Mater. Trans. B 2020, 51B, 1315–1328. [Google Scholar]
- Sampath, K. The Use of Technical Cost Modeling for Titanium Alloy Process Selection. JOM 2005, 57, 25–32. [Google Scholar] [CrossRef]
- Wood, J.R. Producing Ti-6Al-4V plate from single-melt EBCHM ingot. JOM 2002, 54, 56–58. [Google Scholar] [CrossRef]
- Bellot, J.-P.; Hess, E.; Ablitzer, D. Aluminum volatilization and inclusion removal in the electron beam cold hearth melting of Ti alloys. Metall. Trans. B 2000, 31B, 845–854. [Google Scholar] [CrossRef]
- Reimer, C.; Jourdan, J.; Bellot, J.P. Ti-17 volatilization under vacuum: Investigation of the activity coefficients at 1900 °C. Calphad 2017, 58, 122–127. [Google Scholar] [CrossRef]
- Truong, V.-D.; Hyun, Y.-T.; Woon, J.; Lee, W.; Yoon, J. Numerical simulation of the effects of scanning strategies on the aluminum evaporation of titanium alloy in the electron beam cold hearth melting process. Materials 2022, 15, 820. [Google Scholar] [CrossRef]
- Bellot, J.-P.; Decultot, L.; Jardy, A.; Hans, H.; Doridot, E.; Delfosse, J.; McDonald, N. Numerical simulation of the plasma arc melting cold hearth refining process (PAMCHR). Metall. Mater. Trans. B 2020, 51B, 1329–1338. [Google Scholar] [CrossRef]
- Huang, X.; Chou, J.; Tilly, D.; Yu, K. Computer Simulation of the Refining Hearth in a Plasma Arc Melting Process. In Proceedings of the Liquid Metal Processing and Casting Conferences, Santa Fe, NM, USA, 5–8 October 1997. [Google Scholar]
- Xu, X.; Chang, H.; Kou, H.; Yang, Z.; Li, J. Simulation of Temperature Field Distribution in Melting TiAl Alloy by PAM Process. Int. J. Innov. Tech. Explor. Eng. 2013, 2, 1123–1133. [Google Scholar]
- Ji, S.; Duan, J.; Yao, L.; Maijer, D.; Cockcroft, S.; Fiore, D.; Tripp, D. Quantification of the heat transfer during the plasma arc re-melting of titanium alloys. Int. J. Heat Mass Transfer. 2018, 119, 271–281. [Google Scholar] [CrossRef]
- Yao, L.; Maijer, D.; Cockcroft, S.; Fiore, L.; Tripp, D. Quantification of heat transfer phenomena within the melt pool during the plasma arc re-melting of titanium alloys. Int. J. Heat Mass Transf. 2018, 126, 1123–1133. [Google Scholar] [CrossRef]
- Blackburn, M.; Malley, D. Plasma arc melting of titanium alloys. Mater. Des. 1993, 14, 19–27. [Google Scholar] [CrossRef]
- Woodfield, A.; Lemaitre, G. Aerospace Titanium Alloy Melt Process Quality Improvements. In Proceedings of the MATEC Web of Conferences, Piešťany, Slovakia, 7–9 October 2020. [Google Scholar]
- Shamblen, C.; Hunter, G. Titanium Base Alloys Clean Melt Process Development. In the General Electric Aircraft Engines Series Report R89AERB141. 1989. [Google Scholar]
- Garrat, P.; Neal, D. The origin and dissolution of nitride and dense metal inclusions in vacuum arc remelting of titanium alloys. In Proceedings of the Liquid Metal Processing and Casting Conferences, Santa Fe, NM, USA, 11–14 September 1994. [Google Scholar]
- Cen, M.; Liu, Y.; Zhang, H.; Li, Y. Inclusions in melting process of titanium and titanium alloys. China Foundry 2019, 16, 223–231. [Google Scholar] [CrossRef]
- Bomberger, H.; Froes, F. The melting of titanium. JOM 1984, 36, 39–47. [Google Scholar] [CrossRef]
- Yamanaka, A.; Ichihashi, H. Dissolution of refractory elements to titanium alloy in var. ISIJ Int. 1992, 32, 600–606. [Google Scholar] [CrossRef]
- Ghazal, G.; Chapelle, P.; Jardy, A.; Jourdan, J.; Millet, Y. Dissolution of high density inclusions in titanium alloys. ISIJ Int. 2012, 52, 1–9. [Google Scholar] [CrossRef]
- Xu, X.; Kou, H.; Yang, Z.; Li, J. Simulation of HDIs behaviors in the plasma arc cold hearth melting process. Adv. Mat. Res. 2014, 893, 672–678. [Google Scholar] [CrossRef]
- Décultot, L. Etude et Modélisation du Procédé de Refusion par Plasma d’arc en Creuset Froid (PAMCHR) D’alliages de Titane Pour des Applications Aéronautiques. Ph.D. Thesis, Université de Lorraine, France, Nancy, 2021. [Google Scholar]
- Ansys, A. Fluent Solver Theory Guide; ANSYS Inc.: Canonsburg, PA, USA, 2021. [Google Scholar]
- Murakami, K.; Okamoto, T. Fluid flow in the mushy zone composed of granular grains. Acta Metall. 1984, 32, 1741–1744. [Google Scholar] [CrossRef]
- Choi, W.; Jourdan, J.; Matveichev, A.; Jardy, J.; Bellot, J.-P. Kinetics of Evaporation of Alloying Elements under Vacuum. High Temp. Mater. Process. 2017, 36, 815–823. [Google Scholar] [CrossRef]
- Huang, X.; Chou, J.; Pang, Y. Modeling of Plasma Arc Cold Hearth Melting and Refining of Ti Alloys. In Proceedings of the Liquid Metal Processing and Casting, Santa Fe, NM, USA, 21–24 February 1999. [Google Scholar]
- Brucato, A.; Grifasi, F.; Montante, G. Particle drag coefficients in turbulent fluids. Chem. Eng. Sci. 1998, 53, 3295–3315. [Google Scholar] [CrossRef]
- Morsi, S.; Alexander, A. An investigation of particle trajectories in two-phase flow systems. J. Fluid Mech. 1972, 55, 193–208. [Google Scholar] [CrossRef]
- Doridot, E.; Hans, S.; Jardy, A.; Bellot, J.-P. Industrial applications of modelling tools to simulate the PAMCHR casting and VAR process for Ti64. In Proceedings of the 14th Word Conference on Titanium, Nantes, France, 10–14 June 2019. [Google Scholar]
- Bellot, J.-P.; Foster, B.; Hans, S.; Hess, E.; Ablitzer, D.; Mitchell, A. Dissolution of hard-alpha inclusions in liquid titanium alloys. Met. Mat. Trans. B 1997, 28B, 1001–1010. [Google Scholar] [CrossRef]
- Mizukami, H.; Kitaura, T.; Shirai, Y. Nitriding behavior of titanium sponge studied using nitrogen gas and dissolution behavior of a titanium nitride sponge in titanium alloy melt. ISIJ Int. 2019, 59, 104–112. [Google Scholar] [CrossRef]







| 0.66 | |
| 0.05 | |
| 0.30 | |
| 0.65 |
| Electrical Power of the Two Torches (kW) | 716.59 | |
| Thermal power provided by the two torches on the crucibles (kW) | 281.82 | |
| Accumulation (kW) | 0.044 | ~0.01% |
| Power lost on crucible walls (kW) | 163.73 | 58% |
| Power lost by heat radiation (kW) | 63.08 | 22% |
| Enthalpy heat flux transferred to the ingot * (kW) | 52.4 | 18% |
| Residual | 2.57 | 0.9% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bellot, J.-P.; Ayadh, W.; Kroll-Rabotin, J.-S.; Marin, R.; Delfosse, J.; Cardon, A.; Biagi, A.; Hans, S. Experimental and Numerical Study of the Plasma Arc Melting of Titanium Alloys: Application to the Removal of High Density Inclusions (HDIs). Materials 2025, 18, 2051. https://doi.org/10.3390/ma18092051
Bellot J-P, Ayadh W, Kroll-Rabotin J-S, Marin R, Delfosse J, Cardon A, Biagi A, Hans S. Experimental and Numerical Study of the Plasma Arc Melting of Titanium Alloys: Application to the Removal of High Density Inclusions (HDIs). Materials. 2025; 18(9):2051. https://doi.org/10.3390/ma18092051
Chicago/Turabian StyleBellot, Jean-Pierre, Widad Ayadh, Jean-Sébastien Kroll-Rabotin, Raphaël Marin, Jérôme Delfosse, Amandine Cardon, Alessia Biagi, and Stéphane Hans. 2025. "Experimental and Numerical Study of the Plasma Arc Melting of Titanium Alloys: Application to the Removal of High Density Inclusions (HDIs)" Materials 18, no. 9: 2051. https://doi.org/10.3390/ma18092051
APA StyleBellot, J.-P., Ayadh, W., Kroll-Rabotin, J.-S., Marin, R., Delfosse, J., Cardon, A., Biagi, A., & Hans, S. (2025). Experimental and Numerical Study of the Plasma Arc Melting of Titanium Alloys: Application to the Removal of High Density Inclusions (HDIs). Materials, 18(9), 2051. https://doi.org/10.3390/ma18092051






