Modification and Aging Mechanism of Crumb Rubber Modified Asphalt Based on Molecular Dynamics Simulation
Abstract
1. Introduction
- (1)
- (2)
- In the MD study, there is still a need to deepen the research further in terms of the accuracy of CRMA model construction and the comprehensiveness of the mechanism of action study.
2. Modeling and Simulation Methods
2.1. Modeling of Base Asphalt Models
2.2. Modeling of Improved CRMA Models
2.3. MD Simulation Details
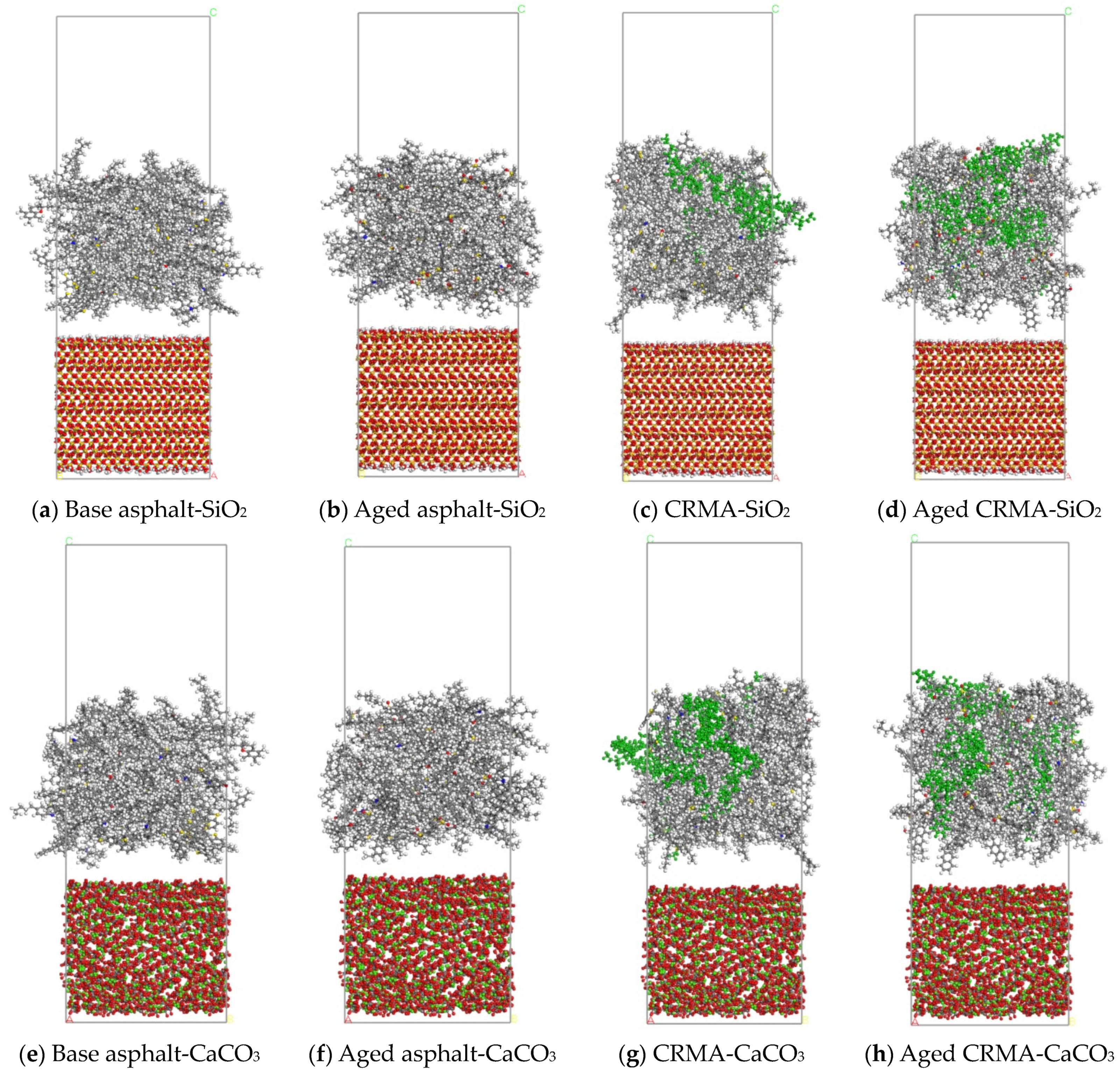
2.4. Modeling of Asphalt–Aggregate Interface Models
2.4.1. Aggregate Models
2.4.2. Asphalt–Aggregate Interface Models
3. Results and Discussion
3.1. Model Validation
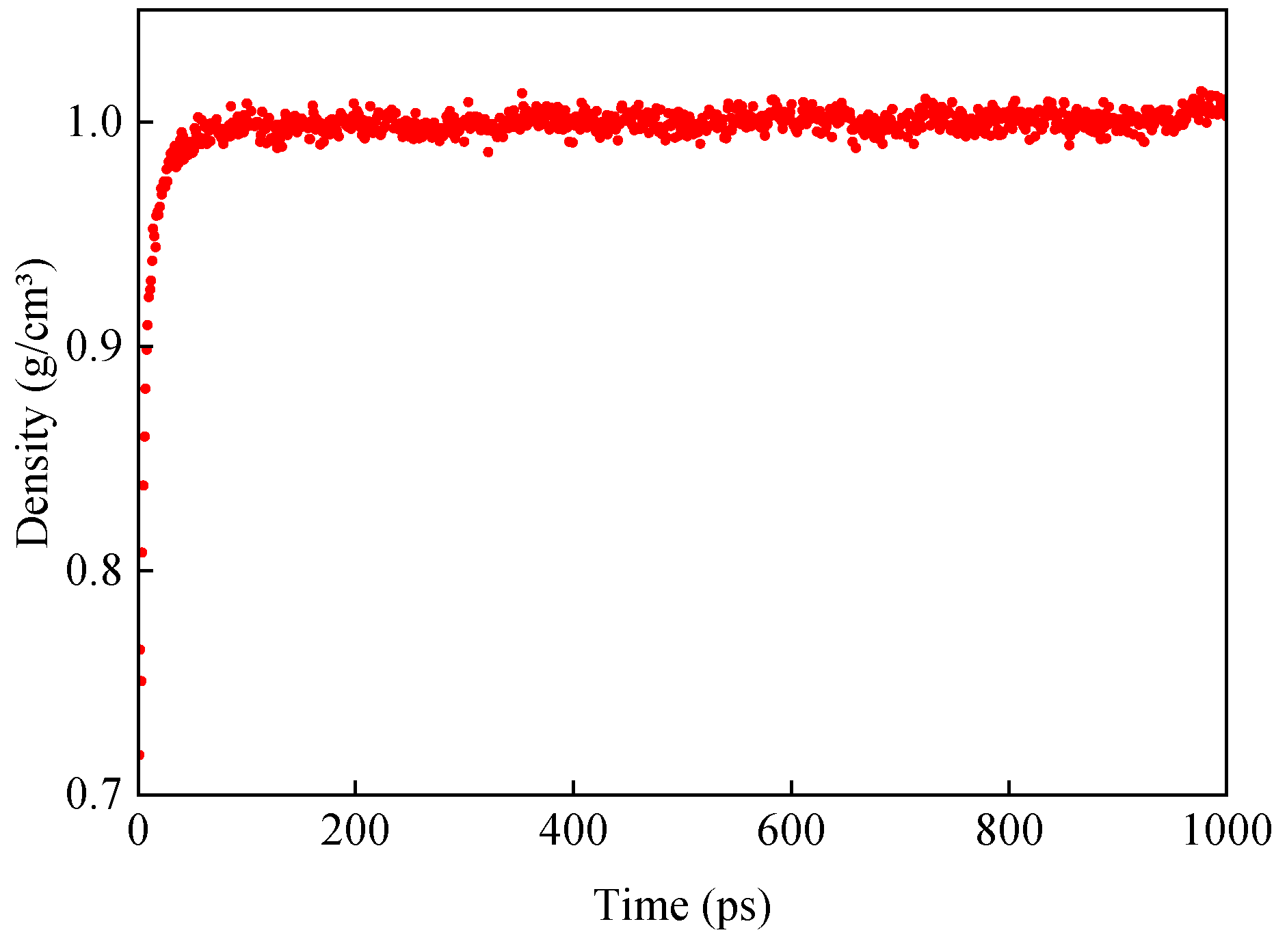
3.1.1. Density
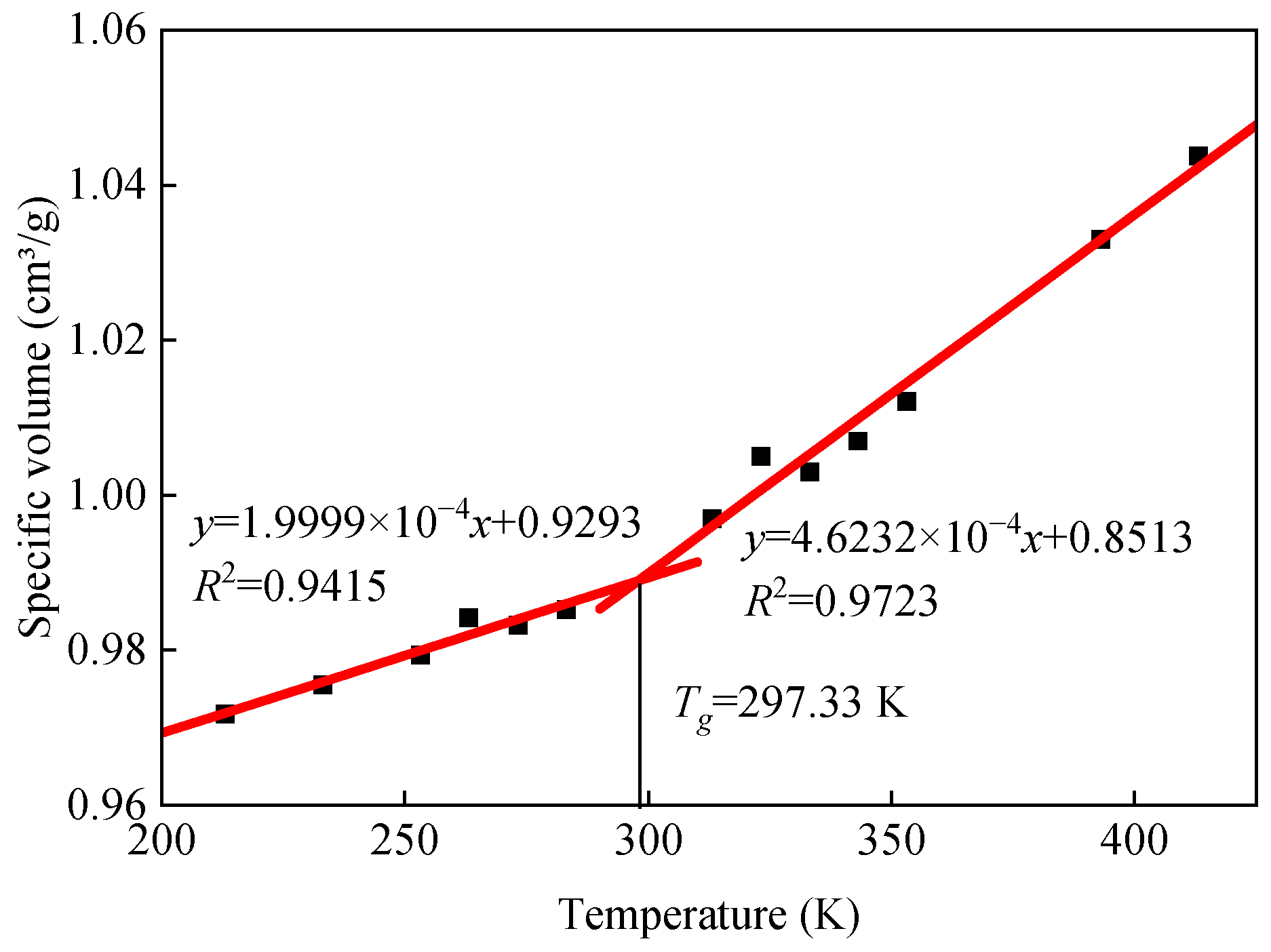
3.1.2. Tg
3.2. Physical Properties
3.2.1. Density
3.2.2. Compatibility
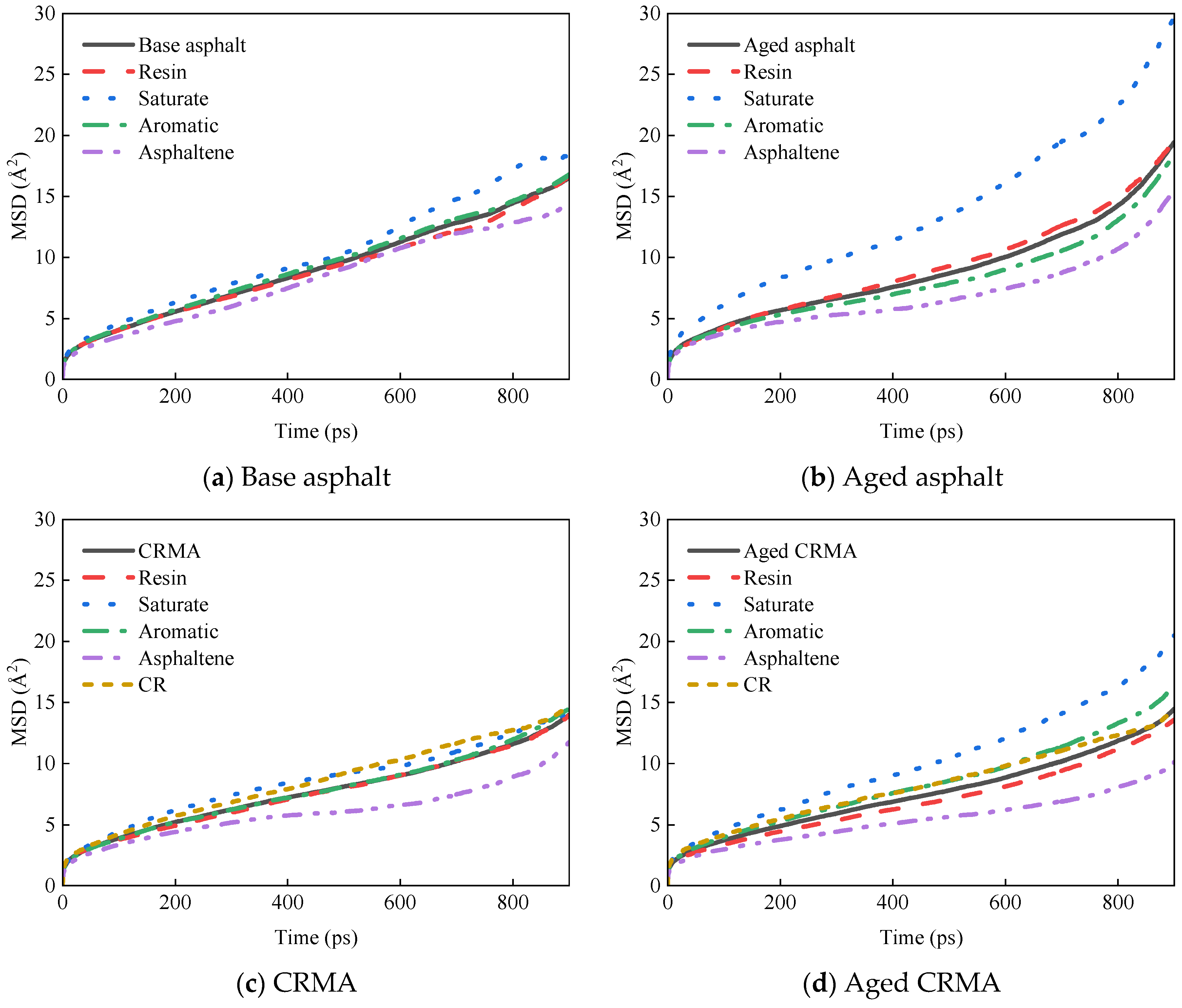
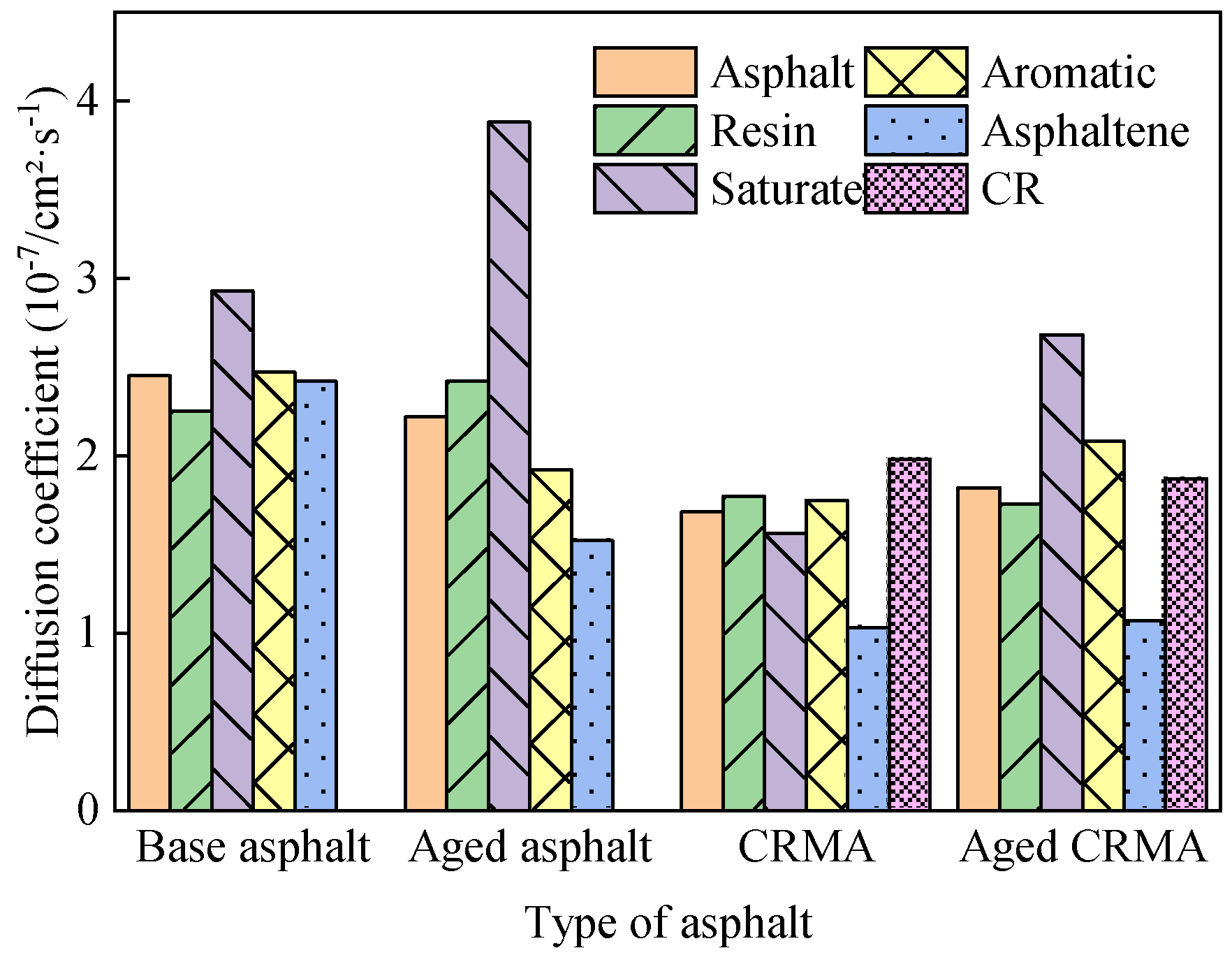
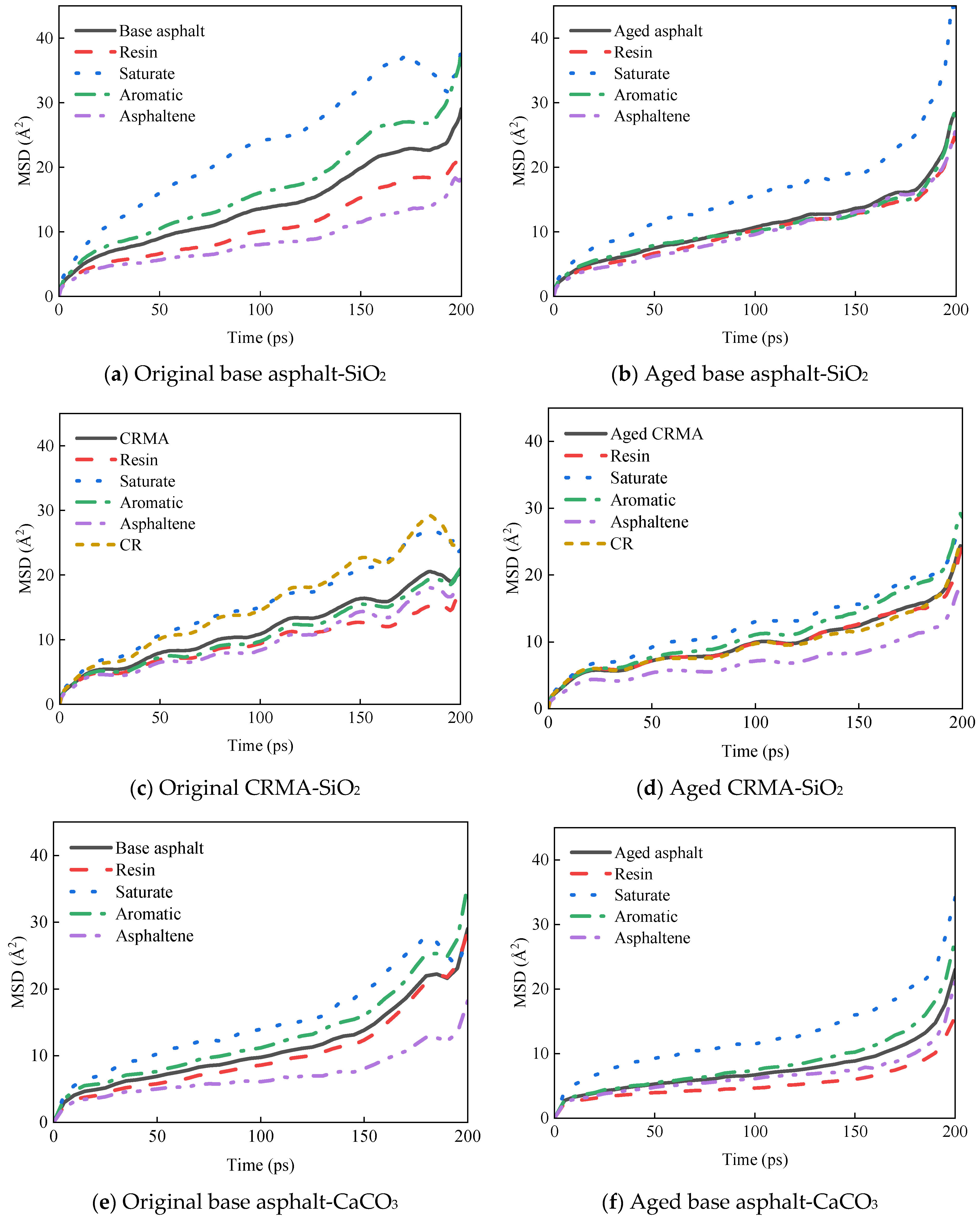
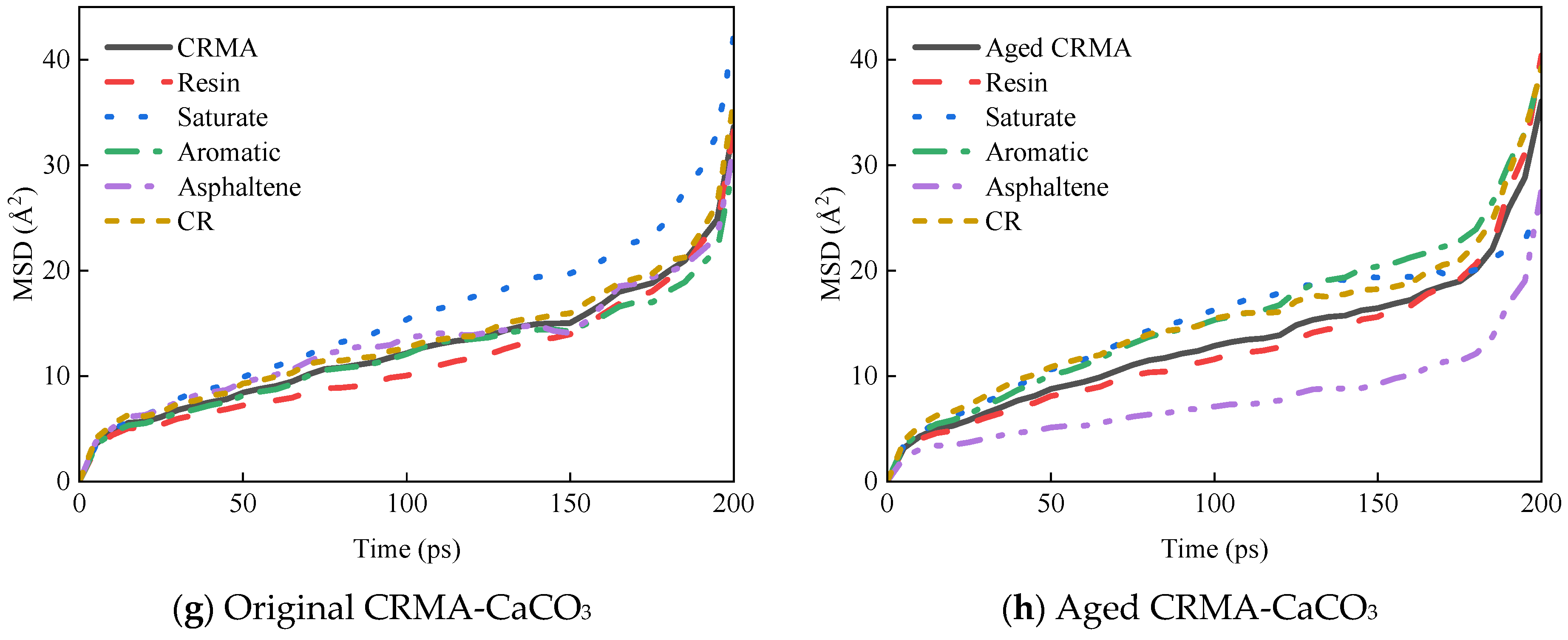
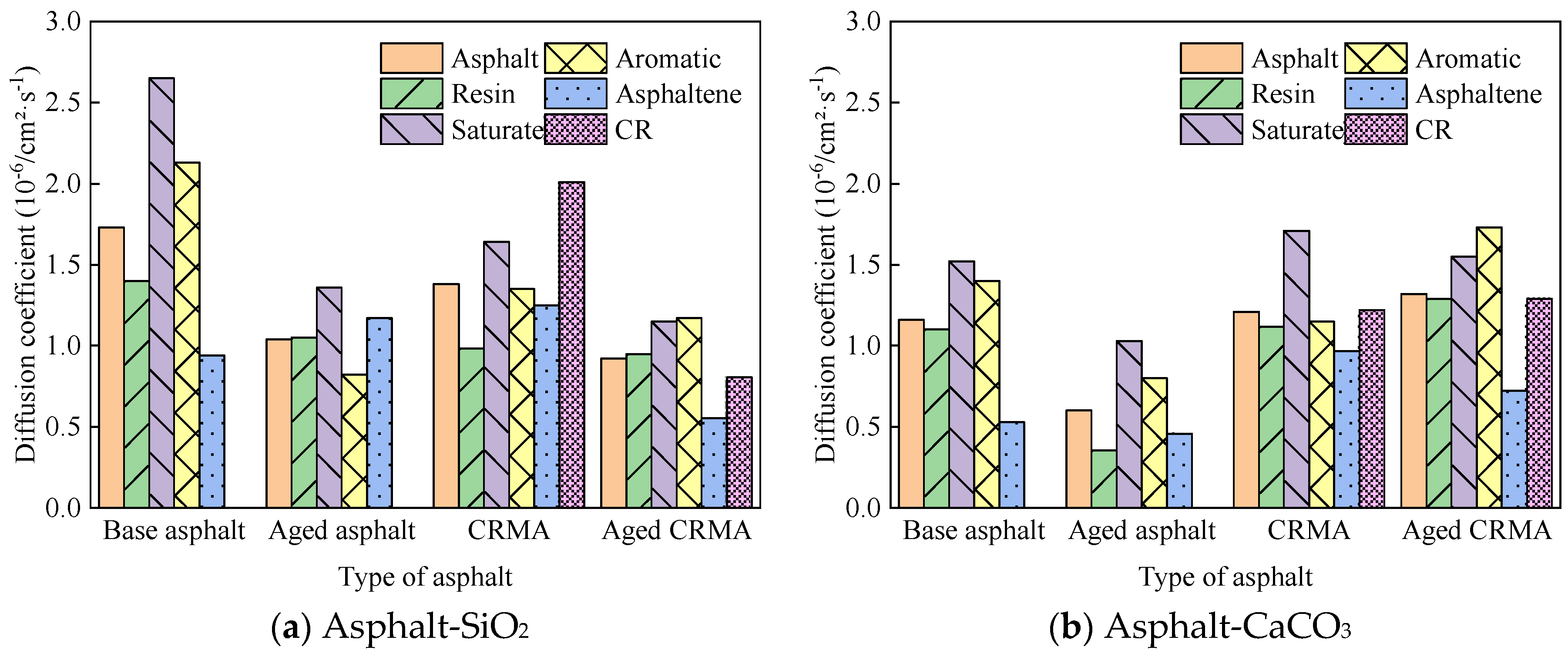
3.2.3. Diffusion Properties
Diffusion Properties of Asphalt Components
- (1)
- Diffusion rates varied for each fraction of asphalt. The saturate fraction moved the fastest and the asphaltene the slowest, and the law holds for most asphalt species. This was mainly related to the relative molecular weights of the asphalt fractions, where molecules with large relative molecular weights moved more slowly, and molecules with small relative molecular weights moved more quickly [36]. They were less active because molecules with high relative molecular weights had larger molecular structures and higher bond-stretching energies. Molecules with small relative molecular weights had small changes in their bond angle energies and were more reactive [45].
- (2)
- The addition of CR reduced the motion of asphalt molecules. The diffusion coefficient of CRMA decreased by about 31% compared to base asphalt, and the diffusion coefficients of the fractions of CRMA were also lower than those of base asphalt. Because the CR molecules were mixed with the fractions of the asphalt, the intermolecular interactions in the system inhibited the incorporation of CR, resulting in the slow molecular movement of CRMA. Therefore, CR would inhibit the movement of asphalt fractions and, to a certain extent, could improve the stability of CRMA. However, it was shown that there is a correlation between the asphalt diffusion coefficient and its self-healing properties. Smaller diffusion coefficients would weaken the self-healing performance of asphalt mixtures for microcracks [19].
- (3)
- The diffusion coefficient of the base asphalt decreased by about 10% after aging. However, the diffusion coefficient of CRMA did not change much. Because the diffusion coefficient of asphalt fractions affected their rheological properties [36], the rheological properties of CRMA were more stable, and the aging resistance was better than that of base asphalt.
Diffusion Properties of Asphalt Components in Asphalt-Aggregate Interface Model
- (1)
- The asphalt fractions of the asphalt–aggregate interface model had the fastest movement for the saturate fraction, followed by aromatic fraction and resin, and the slowest movement for asphaltene. The law of motion was consistent with the law mentioned above for the fractions of asphalt and held for most asphalt species. However, the diffusion coefficient of asphalt on the aggregate surface was nearly ten times greater than that of asphalt alone.
- (2)
- Different aggregates affected the asphalt diffusion coefficient for the asphalt–aggregate interface. The diffusion coefficient of asphalt in the asphalt–SiO2 interface was greater than that of asphalt in the asphalt–CaCO3 interface, and this law held for most asphalt species.
3.3. Mechanical Properties
- (1)
- Compared with base asphalt, CRMA increased each mechanical modulus. This included an increase in the bulk modulus of about 24%, shear modulus of about 23%, and Young’s modulus of about 43%. This indicated that CR improved the mechanical properties of asphalt and, through interaction with asphalt fractions, inhibited the movement of asphalt fractions.
- (2)
- The bulk modulus of the base asphalt increased by 21% after aging. The higher the bulk modulus, the smaller the material’s volume change and the greater the resistance to compressive force. This may be due to polymerization and oxidation within the asphalt after aging. The light fractions of asphalt are gradually converted to heavy fractions, so the asphalt becomes brittle and hard after aging. The shear modulus and Young’s modulus of base asphalt and CRMA decreased after aging. The shear modulus of the aged asphalt decreased by about 13% and Young’s modulus decreased by about 18%. The shear modulus of the aged CRMA decreased by about 3% and Young’s modulus decreased by about 13%. This indicated that the mechanical properties of both base asphalt and CRMA deteriorated after aging. However, CRMA showed a smaller reduction than base asphalt. This indicated that CRMA has better aging resistance.
3.4. Component Interaction Behavior
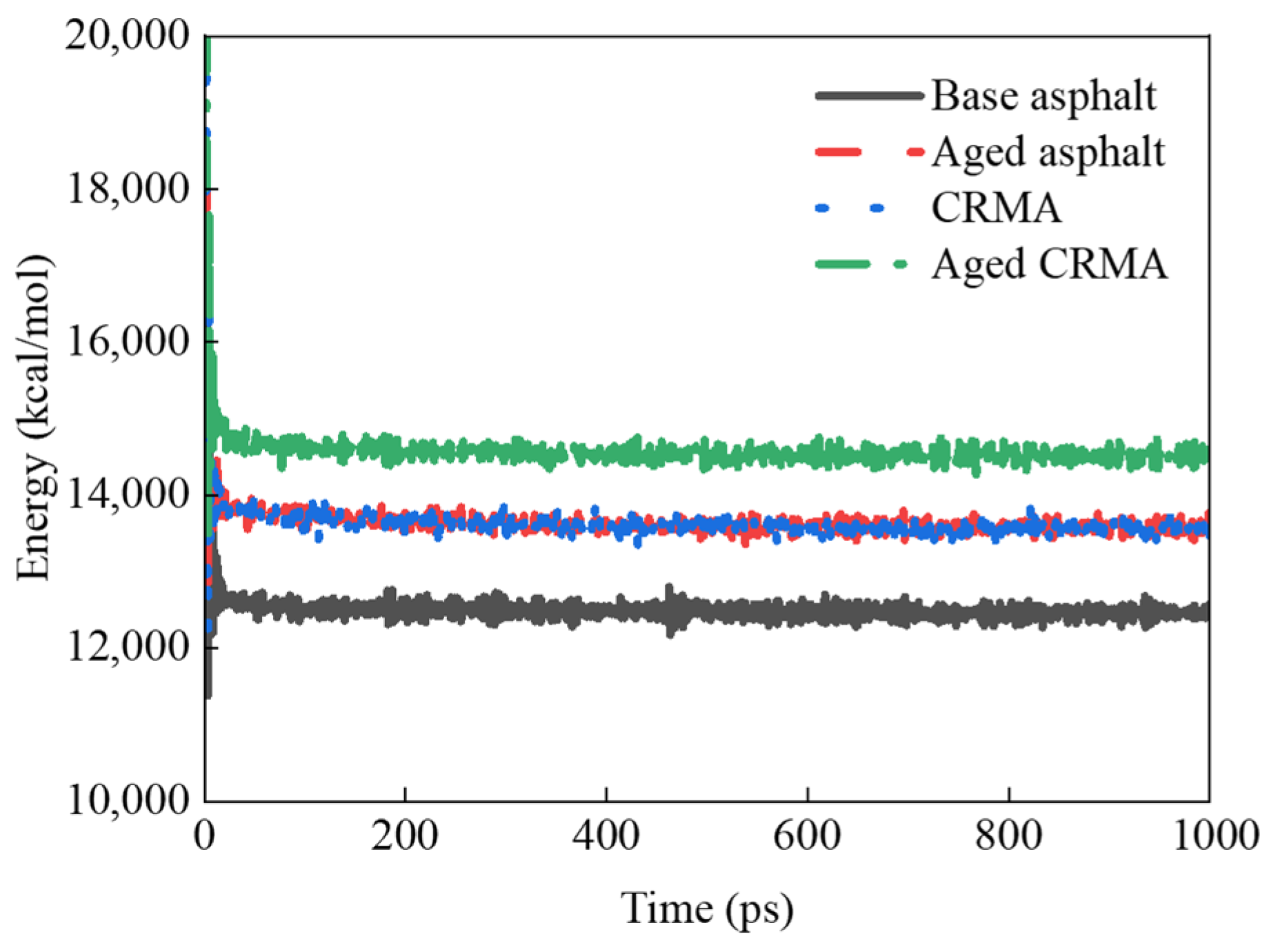
3.4.1. Energy Analysis
- (1)
- The energy size relationship of various types of asphalt was calculated as aged CRMA (14,507 kcal/mol) > aged asphalt (13,575 kcal/mol) > CRMA (13,564 kcal/mol) > base asphalt (12,465 kcal/mol). CRMA provided an 8.8% total energy gain over base asphalt. Because CR is a macromolecule, adding it to asphalt increases its energy.
- (2)
- The total energy of base asphalt and CRMA was improved by 8.9% and 6.9% after aging, respectively. The molecular mass of asphalt increased due to the oxidation of small molecules to form large molecules of asphaltene during the aging process. According to the mass–energy equation, an increase in mass at a given speed would increase the energy of the substance. This resulted in a greater relative atomic mass and higher energy in the aged asphalt than in the base asphalt [42].
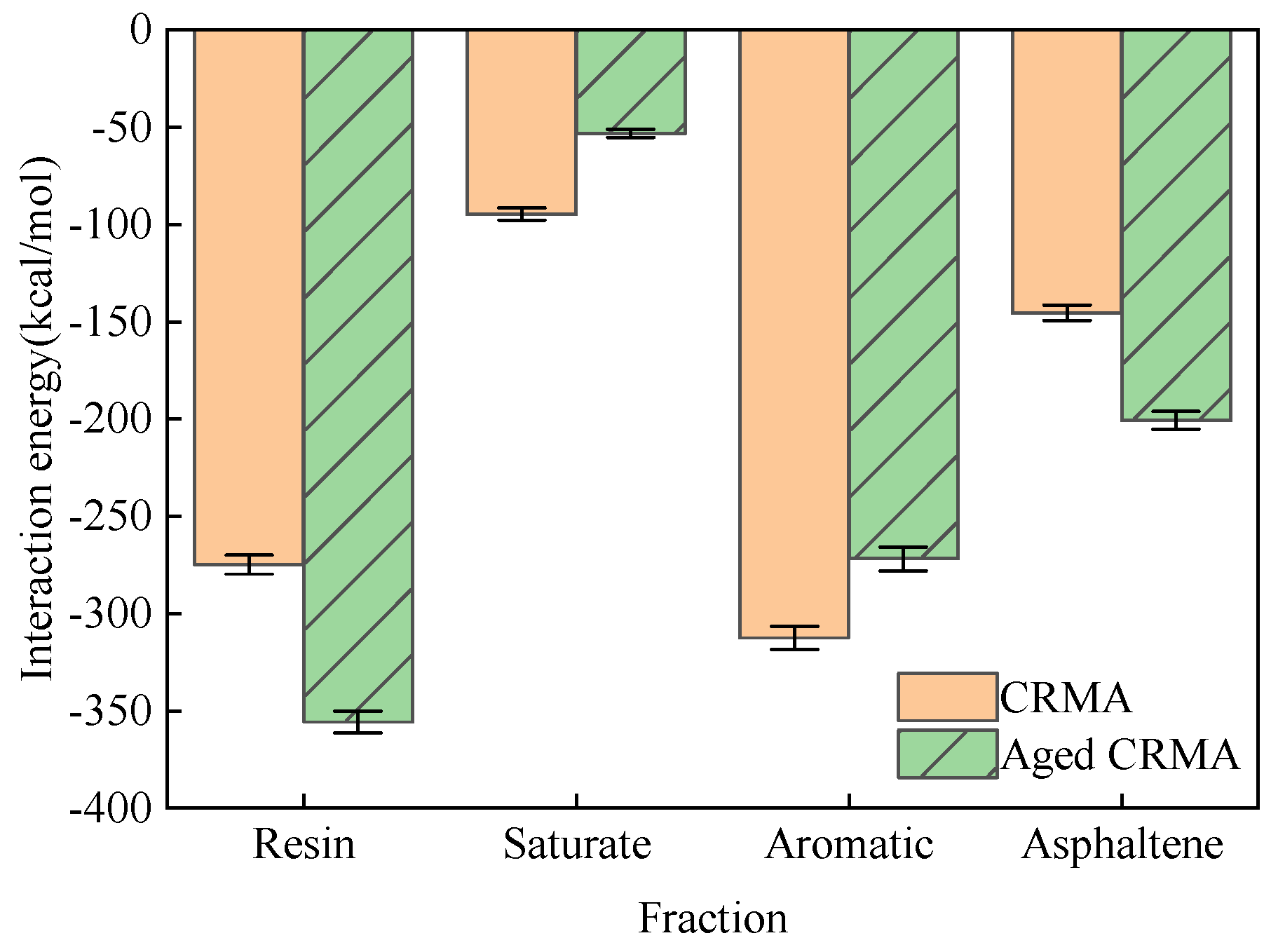
3.4.2. Interaction Energy Analysis
- (1)
- In CRMA, the absolute value of the interaction energy between CR and each fraction was calculated as aromatic > resin > asphaltene > saturate. Considering that the content of the four fractions in the asphalt was not uniform, the interaction energy was divided by the mass percentage of the four fractions (Table 1) to make the simulation results more instructive. The magnitude relationship of the normalized fitting results was obtained as aromatic (−976.98 kcal/mol) > saturate (−853.38 kcal/mol) > asphaltene (−840.98 kcal/mol) > resin (−694.42 kcal/mol). The interaction energy of light fractions such as saturate and aromatic with CR was stronger, indicating that CR will physically adsorb with light fractions.
- (2)
- In aged CRMA, the absolute value of the interaction energy between CR and each fraction was calculated as resin > aromatic > asphaltene > saturate. The magnitude relationship of the normalized fitting results was obtained as resin (−964.07 kcal/mol) > aromatic (−927.88 kcal/mol) > asphaltene (−832.68 kcal/mol) > saturate (−853.38 kcal/mol). The interaction energy between saturates and CR became weaker, and the interaction energy between resin and CR became stronger after aging.
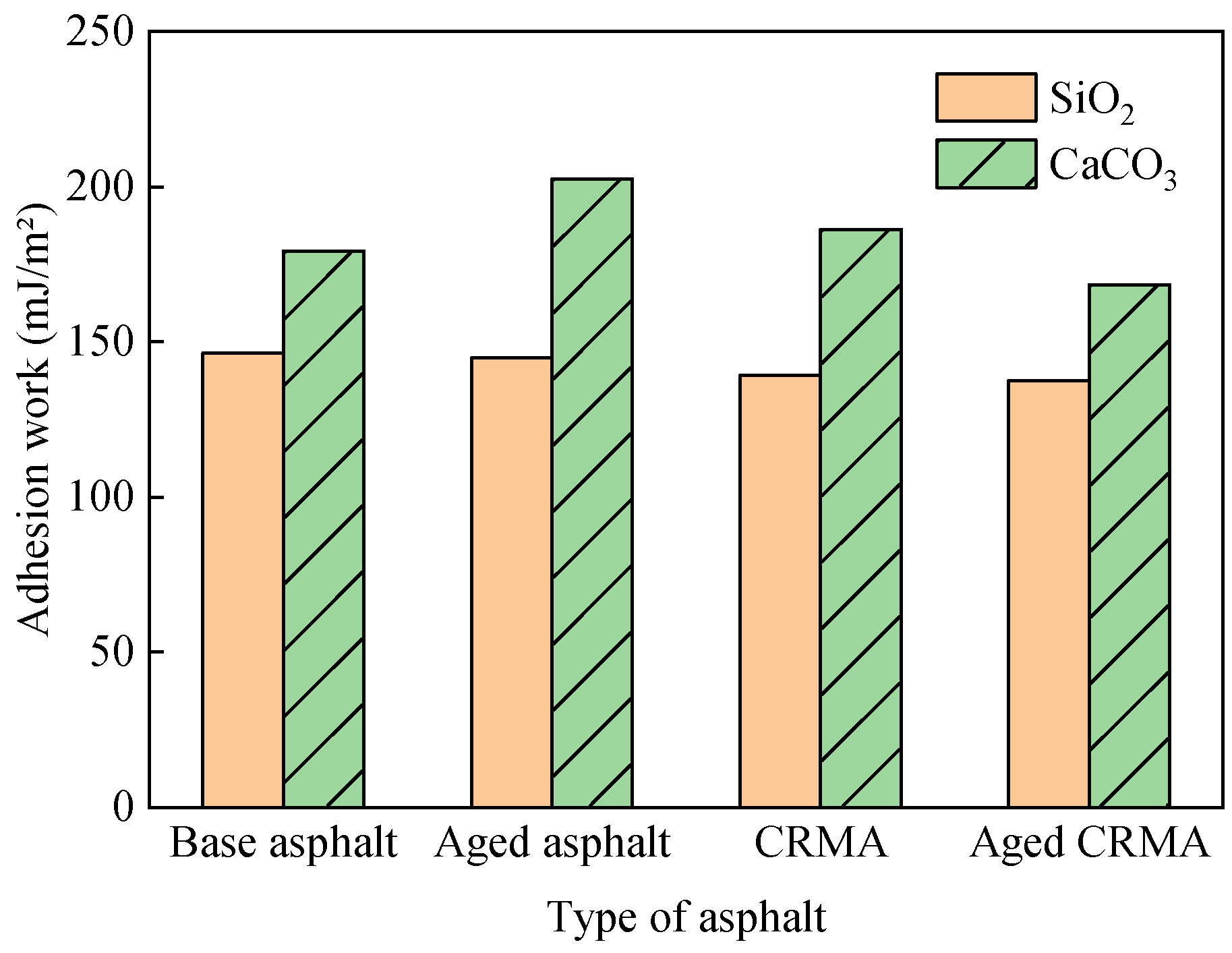
3.5. Asphalt–Aggregate Interface Interaction
- (1)
- The asphalt–aggregate interfacial adhesion work was affected by the aggregate type. The adhesion work of CaCO3 with asphalt was greater than that of SiO2. The difference between the adhesion work of the two aggregates with different asphalts was in the range of 22.5–39.9%. Because SiO2 was an acidic aggregate, and CaCO3 was a basic aggregate, acidic aggregates had worse adhesion properties with asphalt [47]. It can also be seen that the adhesion work diffusion law was consistent with the diffusion law of asphalt and its fractions on different aggregate surfaces. Aggregates with strong adherence were slower to diffuse. Aggregates with less adhesion work spread faster.
- (2)
- After adding CR, the asphalt–SiO2 interfacial adhesion work decreased by 5.2%, and the asphalt–CaCO3 interfacial adhesion work did not change much. This indicated that adding CR reduced the adhesion properties at the asphalt–aggregate interface. It should be emphasized that this conclusion presupposes that the mass of base asphalt in CRMA was equal to the modeled mass of base asphalt in the asphalt–aggregate adhesion model. This also indirectly verified that the optimal oil/stone ratio of CRMA mixes was larger than that of base asphalt mixes when designing CRMA mix ratios [48].
4. Conclusions
- (1)
- Compared to the base asphalt, the mechanical modulus of CRMA increased by 23–43%, and the diffusion coefficient decreased by about 31%. This indicated that the CR enhanced the interactions between the asphalt systems, improving the mechanical properties. The CR inhibited the movement of the asphalt components (especially saturate and aromatic) through interactions with the asphalt components.
- (2)
- The Sp difference between aged asphalt and CR was about four times that before aging. It indicated that the compatibility between asphalt and CR deteriorated obviously after aging. Therefore, compatibility should be a key concern when rubberized asphalt is recycled.
- (3)
- The shear modulus and Young’s modulus of both base asphalt and CRMA decreased after aging. The decrease for CRMA was smaller than that of base asphalt. The diffusion coefficient of the base asphalt decreased by about 10% after aging, but the CRMA remained stable. This indicated that CR could improve the mechanical stability of the base asphalt. This is attributed to the CR inhibiting the diffusion of asphalt components, providing CRMA with better aging resistance.
- (4)
- The adhesion work of CaCO3 with asphalt was greater than that of SiO2, and the difference between the adhesion work of the two aggregates with different asphalts was 22.5–39.9%. It can be seen that the diffusion law of adhesion work was consistent with the diffusion law of asphalt. Aggregates with stronger adhesion had slower diffusion rates. Aggregates with less adhesion had a faster diffusion rate.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Presti, D.L. Recycled Tyre Rubber Modified Bitumens for road asphalt mixtures: A literature review. Constr. Build. Mater. 2013, 49, 863–881. [Google Scholar] [CrossRef]
- Poovaneshvaran, S.; Hasan, M.R.M.; Jaya, R.P. Impacts of recycled crumb rubber powder and natural rubber latex on the modified asphalt rheological behaviour, bonding, and resistance to shear. Constr. Build. Mater. 2020, 234, 117357. [Google Scholar] [CrossRef]
- Shisong, R.; Xueyan, L.; Jian, X.; Peng, L. Investigating the role of swelling-degradation degree of crumb rubber on CR/SBS modified porous asphalt binder and mixture. Constr. Build. Mater. 2021, 300, 124048. [Google Scholar]
- Carlo, C.; Edoardo, B.; Emiliano, P.; Maurizio, B. Evaluation of the rheological and performance behaviour of bitumen modified with compounds including crumb rubber from waste tires. Constr. Build. Mater. 2022, 361, 129679. [Google Scholar]
- Wang, T.; Xiao, F.; Zhu, X.; Huang, B.; Wang, J.; Amirkhanian, S. Energy consumption and environmental impact of rubberized asphalt pavement. J. Clean. Prod. 2018, 180, 139–158. [Google Scholar] [CrossRef]
- Bakar, S.K.A.; Abdullah, M.E.; Kamal, M.M.; Rahman, R.A.; Buhari, R.; Jaya, R.P.; Sabri, S.; Ahmad, K.A. The effect of crumb rubber on the physical and rheological properties of modified binder. J. Phys. Conf. Ser. 2018, 1049, 012099. [Google Scholar] [CrossRef]
- Wenhui, Z.; Xiangbing, X.; Guanghui, L.; Jiuguang, G.; Meng, B.; Mingwei, W. Research on the Influence of Nanocarbon/Copolymer SBS/Rubber Powder Composite Modification on the Properties of Asphalt and Mixtures. Adv. Mater. Sci. Eng. 2020, 2020, 8820202. [Google Scholar]
- Tang, N.; Huang, W.; Hu, J.; Xiao, F. Rheological characterisation of terminal blend rubberised asphalt binder containing polymeric additive and sulphur. Road Mater. Pavement Des. 2018, 19, 1288–1300. [Google Scholar] [CrossRef]
- Peng, W.; Li, P.; Gao, J.; Liu, Z.; Wang, X.; Wang, S.; Wu, W. Long-term skid resistance evolution and influence mechanism of asphalt pavement based on self-developed wear equipment. Constr. Build. Mater. 2024, 453, 139085. [Google Scholar] [CrossRef]
- Jeong, K.; Lee, S.; Amirkhanian, S.N.; Kim, K.W. Interaction effects of crumb rubber modified asphalt binders. Constr. Build. Mater. 2010, 24, 824–831. [Google Scholar] [CrossRef]
- Peng, W.; Li, P.; Gong, W.; Tian, S.; Wang, Z.; Liu, S.; Liu, Z. Preparation and mechanism of rubber-plastic alloy crumb rubber modified asphalt with low viscosity and stabilized performance. Constr. Build. Mater. 2023, 388, 131687. [Google Scholar] [CrossRef]
- Su, J.; Li, P.; Zhu, G.; Wang, X.; Dong, S. Interface Interaction of Waste Rubber—Asphalt System. Buildings 2024, 14, 1868. [Google Scholar] [CrossRef]
- Wang, H.; You, Z.; Mills-Beale, J.; Hao, P. Laboratory evaluation on high temperature viscosity and low temperature stiffness of asphalt binder with high percent scrap tire rubber. Constr. Build. Mater. 2011, 26, 583–590. [Google Scholar] [CrossRef]
- Chen, Z.; Wang, T.; Pei, J.; Amirkhanian, S.; Xiao, F.; Ye, Q.; Fan, Z. Low Temperature and Fatigue Characteristics of Treated Crumb Rubber Modified Asphalt after a Long Term Aging Procedure. J. Clean. Prod. 2019, 234, 1262–1274. [Google Scholar] [CrossRef]
- Shen, A.; Zhai, C.; Guo, Y.; Yang, X. Mechanism of adhesion property between steel slag aggregate and rubber asphalt. J. Adhes. Sci. Technol. 2018, 32, 2727–2740. [Google Scholar] [CrossRef]
- Zhou, X.; Moghaddam, T.B.; Chen, M.; Wu, S.; Adhikari, S.; Wang, F.; Fan, Z. Nano-scale analysis of moisture diffusion in asphalt-aggregate interface using molecular simulations. Constr. Build. Mater. 2021, 285, 122962. [Google Scholar] [CrossRef]
- Zheng, C.; Shan, C.; Liu, J.; Zhang, T.; Yang, X.; Lv, D. Microscopic adhesion properties of asphalt-mineral aggregate interface in cold area based on molecular simulation technology. Constr. Build. Mater. 2021, 268, 121151. [Google Scholar] [CrossRef]
- Sonibare, K.; Rucker, G.; Zhang, L. Molecular dynamics simulation on vegetable oil modified model asphalt. Constr. Build. Mater. 2021, 270, 121687. [Google Scholar] [CrossRef]
- Hu, D.; Pei, J.; Li, R.; Zhang, J.; Jia, Y.; Fan, Z. Using thermodynamic parameters to study self-healing and interface properties of crumb rubber modified asphalt based on molecular dynamics simulation. Front. Struct. Civ. Eng. 2020, 14, 109–122. [Google Scholar] [CrossRef]
- Xu, G.; Wang, H. Molecular dynamics study of oxidative aging effect on asphalt binder properties. Fuel 2017, 188, 1–10. [Google Scholar] [CrossRef]
- Wang, L.; Liu, Y.; Zhang, L. Micro/Nanoscale Study on the Effect of Aging on the Performance of Crumb Rubber Modified Asphalt. Math. Probl. Eng. 2020, 2020, 1924349. [Google Scholar] [CrossRef]
- Qu, X.; Liu, Q.; Guo, M.; Wang, D.; Oeser, M. Study on the effect of aging on physical properties of asphalt binder from a microscale perspective. Constr. Build. Mater. 2018, 187, 718–729. [Google Scholar] [CrossRef]
- Chen, P.; Luo, X.; Gao, Y.; Zhang, Y. Modeling percentages of cohesive and adhesive debonding in bitumen-aggregate interfaces using molecular dynamics approaches. Appl. Surf. Sci. 2022, 571, 151318. [Google Scholar] [CrossRef]
- Gong, Y.; Xu, J.; Yan, E. Intrinsic temperature and moisture sensitive adhesion characters of asphalt-aggregate interface based on molecular dynamics simulations. Constr. Build. Mater. 2021, 292, 123462. [Google Scholar] [CrossRef]
- Li, D.D.; Greenfield, M.L. Chemical compositions of improved model asphalt systems for molecular simulations. Fuel 2014, 115, 347–356. [Google Scholar] [CrossRef]
- Xu, Z.; Wang, Y.; Cao, J.; Chai, J.; Cao, C.; Si, Z.; Li, Y. Adhesion between asphalt molecules and acid aggregates under extreme temperature: A ReaxFF reactive molecular dynamics study. Constr. Build. Mater. 2021, 285, 122882. [Google Scholar] [CrossRef]
- Chen, W.; Chen, S.; Zheng, C. Analysis of micromechanical properties of algae bio-based bio-asphalt-mineral interface based on molecular simulation technology. Constr. Build. Mater. 2021, 306, 124888. [Google Scholar] [CrossRef]
- Li, M.; Liu, L.; Xing, C.; Liu, L.; Wang, H. Influence of rejuvenator preheating temperature and recycled mixture’s curing time on performance of hot recycled mixtures. Constr. Build. Mater. 2021, 295, 123616. [Google Scholar] [CrossRef]
- He, L.; Zheng, Y.; Alexiadis, A.; Cannone Falchetto, A.; Li, G.; Valentin, J.; Van den Bergh, W.; Emmanuilovich Vasiliev, Y.; Kowalski, K.J.; Grenfell, J. Research on the self-healing behavior of asphalt mixed with healing agents based on molecular dynamics method. Constr. Build. Mater. 2021, 295, 123430. [Google Scholar] [CrossRef]
- Zhang, L.; Long, N.; Liu, Y.; Wang, L. Cross-scale study on the influence of moisture-temperature coupling conditions on adhesive properties of rubberized asphalt and steel slag. Constr. Build. Mater. 2022, 332, 127401. [Google Scholar] [CrossRef]
- Wang, L.; Zhang, L.; Liu, Y. Molecular Dynamics Study on the Effect of Mineral Composition on the Interface Interaction between Rubberized Asphalt and Aggregate. J. Mater. Civ. Eng. 2022, 34, 04022032. [Google Scholar] [CrossRef]
- Jiao, B.; Pan, B.; Che, T. Evaluating impacts of desulfurization and depolymerization on thermodynamics properties of crumb rubber modified asphalt through molecular dynamics simulation. Constr. Build. Mater. 2022, 323, 126360. [Google Scholar] [CrossRef]
- Guo, F.; Zhang, J.; Pei, J.; Ma, W.; Hu, Z.; Guan, Y. Evaluation of the compatibility between rubber and asphalt based on molecular dynamics simulation. Front. Struct. Civ. Eng. 2020, 14, 435–445. [Google Scholar] [CrossRef]
- Guo, F.; Zhang, J.; Pei, J.; Zhou, B.; Falchetto, A.C.; Hu, Z. Investigating the interaction behavior between asphalt binder and rubber in rubber asphalt by molecular dynamics simulation. Constr. Build. Mater. 2020, 252, 118956. [Google Scholar] [CrossRef]
- Zhang, X.; Han, C.; Otto, F.; Zhang, F. Evaluation of Properties and Mechanisms of Waste Plastic/Rubber-Modified Asphalt. Coatings 2021, 11, 1365. [Google Scholar] [CrossRef]
- Khabaz, F.; Khare, R. Glass Transition and Molecular Mobility in Styrene-Butadiene Rubber Modified Asphalt. J. Phys. Chem. B 2015, 119, 14261–14269. [Google Scholar] [CrossRef] [PubMed]
- Guo, F.; Pei, J.; Zhang, J.; Xue, B.; Sun, G.; Li, R. Study on the adhesion property between asphalt binder and aggregate: A state-of-the-art review. Constr. Build. Mater. 2020, 256, 119474. [Google Scholar] [CrossRef]
- Lesueur, D. The colloidal structure of bitumen: Consequences on the rheology and on the mechanisms of bitumen modification. Adv. Colloid Interface Sci. 2008, 145, 42–82. [Google Scholar] [CrossRef]
- You, L.; Spyriouni, T.; Dai, Q.; You, Z.; Khanal, A. Experimental and molecular dynamics simulation study on thermal, transport, and rheological properties of asphalt. Constr. Build. Mater. 2020, 265, 120358. [Google Scholar] [CrossRef]
- Zhou, K.; Huang, J.; Deng, Y.; Huang, L. Molecular dynamics simulation of the interface mechanical properties of graphene modified asphalt. J. Funct. Mater. 2021, 52, 12129–12136. [Google Scholar]
- Wang, P.; Dong, Z.; Tan, Y.; Liu, Z. Investigating the Interactions of the Saturate, Aromatic, Resin, and Asphaltene Four Fractions in Asphalt Binders by Molecular Simulations. Energy Fuels 2015, 29, 112–121. [Google Scholar] [CrossRef]
- Wang, L.; Zhang, L.; Liu, Y. Molecular Dynamics Study on Compatibility of Asphalt and Rubber Powders before and after Aging. J. Build. Mater. 2019, 22, 474–479. [Google Scholar]
- Wang, L.; Zhang, L.; Liu, Y. Compatibility of Rubber Powder and Asphalt in Rubber Powder Modified Asphalt by Molecular Dynamics. J. Build. Mater. 2018, 21, 689–694. [Google Scholar]
- Hu, D.; Gu, X.; Cui, B. Effect of styrene-butadiene-styrene copolymer on the aging resistance of asphalt: An atomistic understanding from reactive molecular dynamics simulations. Front. Struct. Civ. Eng. 2021, 15, 1261–1276. [Google Scholar] [CrossRef]
- Gao, Y.; Xie, Y.; Liao, M.; Li, Y.; Zhu, J.; Tian, W. Study on the mechanism of the effect of graphene on the rheological properties of rubber-modified asphalt based on size effect. Constr. Build. Mater. 2023, 364, 129815. [Google Scholar] [CrossRef]
- Su, M.; Zhang, H.; Zhang, Y.; Zhang, Z. Miscibility and mechanical properties of SBS and asphalt blends based on molecular dynamics simulation. J. Chang’an Univ. (Nat. Sci. Ed.) 2017, 37, 24–32. [Google Scholar]
- Yao, H.; Liu, J.; Xu, M.; Ji, J.; Dai, Q.; You, Z. Discussion on molecular dynamics (MD) simulations of the asphalt materials. Adv. Colloid Interface Sci. 2022, 299, 102565. [Google Scholar] [CrossRef]
- Kim, J.R. Characteristics of crumb rubber modified (CRM) asphalt concrete. KSCE J. Civ. Eng. 2001, 5, 157–164. [Google Scholar] [CrossRef]
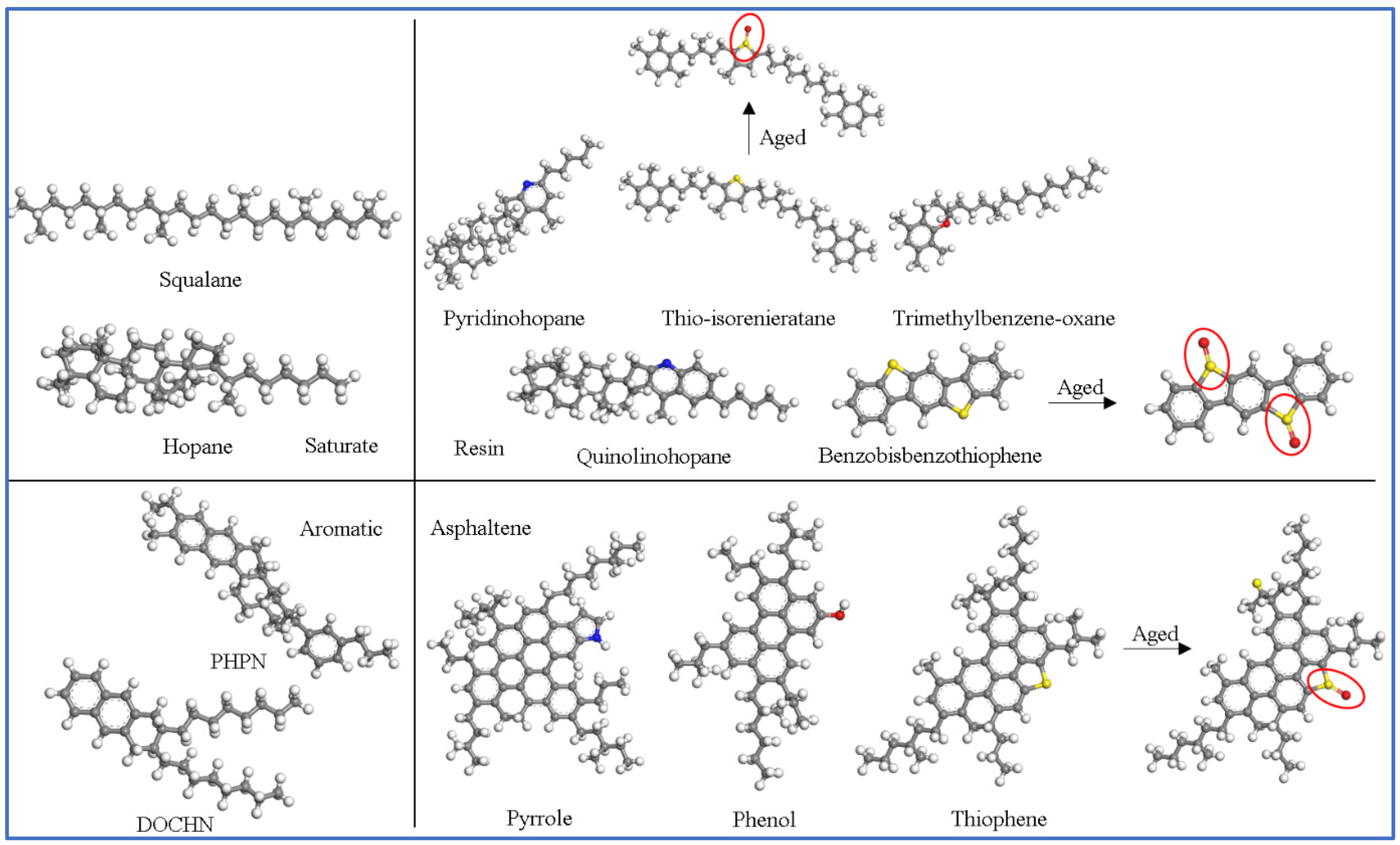
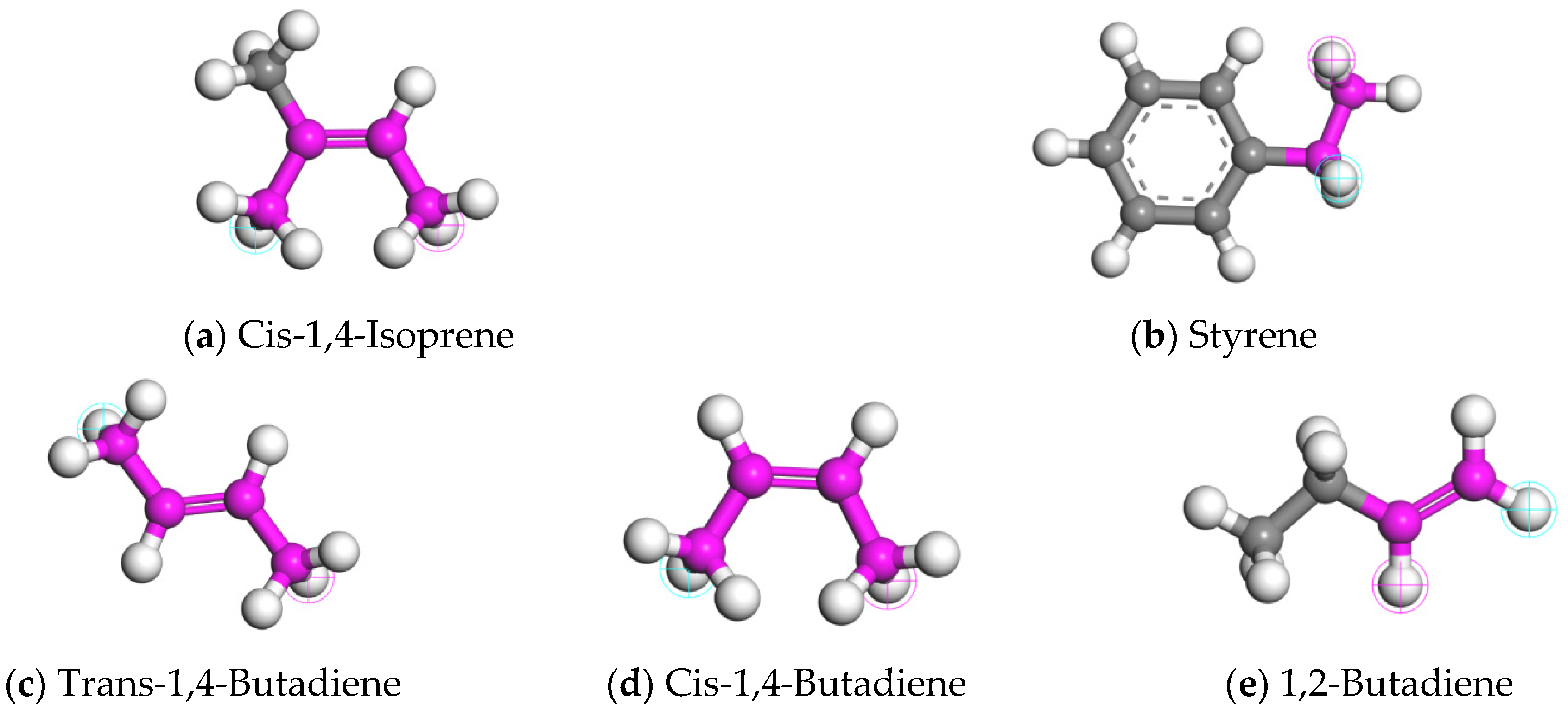
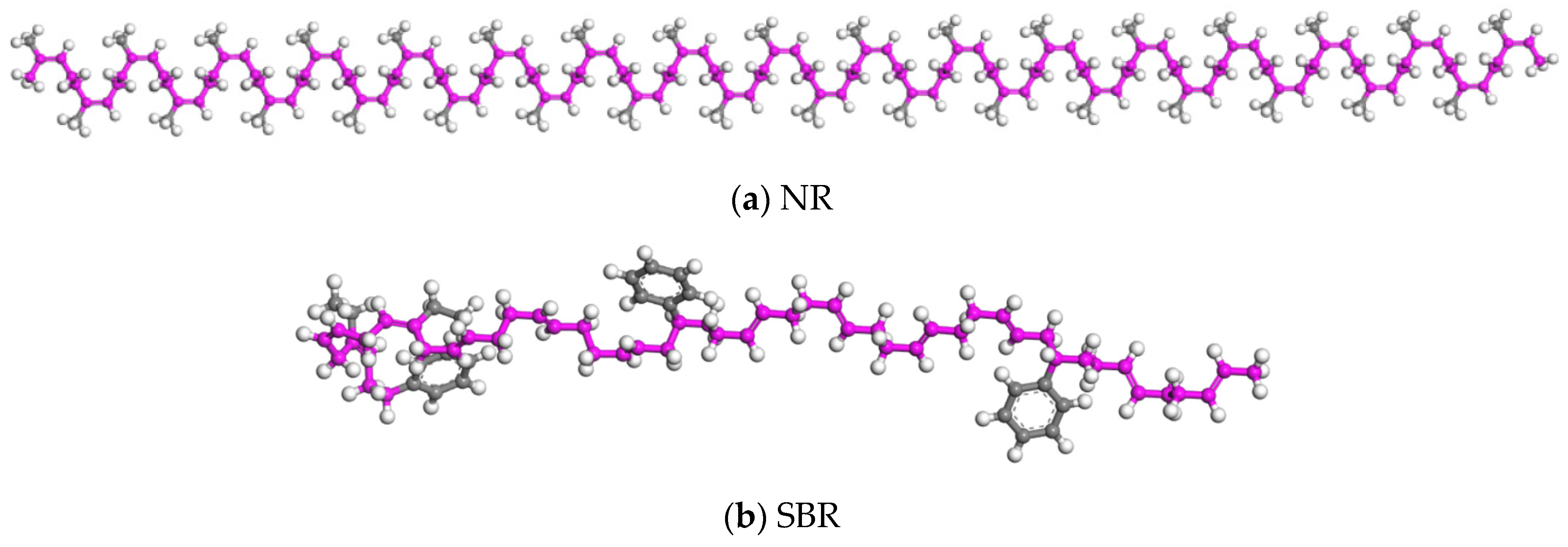



| Fraction | Molecular | Base Asphalt | Aged Asphalt | ||||
|---|---|---|---|---|---|---|---|
| Molecular Formula | Number of Molecules | Fraction Content (%) | Molecular Formula | Number of Molecules | Fraction Content (%) | ||
| Resin | Pyridinohopane | C36H57N | 4 | 39.6 | C36H57N | 3 | 36.9 |
| Thio-isorenieratane | C40H60S | 4 | C40H60SO | 4 | |||
| Trimethylbenzene-oxane | C29H50O | 5 | C29H50O | 4 | |||
| Quinolinohopane | C40H59N | 4 | C40H59N | 3 | |||
| Benzobisbenzothiophene | C18H10S2 | 15 | C18H10S2O2 | 15 | |||
| Saturate | Squalane | C30H62 | 4 | 11.1 | C30H62 | 4 | 9.7 |
| Hopane | C35H62 | 4 | C35H62 | 3 | |||
| Aromatic | PHPN | C35H44 | 11 | 32.0 | C35H44 | 10 | 29.3 |
| DOCHN | C30H46 | 13 | C30H46 | 12 | |||
| Asphaltene | Pyrrole | C66H81N | 2 | 17.3 | C66H81N | 3 | 24.1 |
| Phenol | C42H54O | 3 | C42H54O | 4 | |||
| Thiophene | C51H62S | 3 | C51H62SO | 4 | |||
| Styrene | Trans-1,4-Butadiene | Cis-1,4-Butadiene | 1,2-Butadiene | Others |
|---|---|---|---|---|
| 23.5 | 58 | 5.5 | 12 | 1 |
| Parameter | CR | Original Base Asphalt | Aged Base Asphalt |
|---|---|---|---|
| Sp/[(J/m−3)1/2] | 18.020 | 18.214 | 18.753 |
| |ΔSp|/[(J/m−3)1/2] | / | 0.194 | 0.733 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, J.; He, L. Modification and Aging Mechanism of Crumb Rubber Modified Asphalt Based on Molecular Dynamics Simulation. Materials 2025, 18, 197. https://doi.org/10.3390/ma18010197
Li J, He L. Modification and Aging Mechanism of Crumb Rubber Modified Asphalt Based on Molecular Dynamics Simulation. Materials. 2025; 18(1):197. https://doi.org/10.3390/ma18010197
Chicago/Turabian StyleLi, Jian, and Liang He. 2025. "Modification and Aging Mechanism of Crumb Rubber Modified Asphalt Based on Molecular Dynamics Simulation" Materials 18, no. 1: 197. https://doi.org/10.3390/ma18010197
APA StyleLi, J., & He, L. (2025). Modification and Aging Mechanism of Crumb Rubber Modified Asphalt Based on Molecular Dynamics Simulation. Materials, 18(1), 197. https://doi.org/10.3390/ma18010197







