Thermal Optimization of Edge-Emitting Lasers Arrays
Abstract
1. Introduction
2. Materials and Methods
2.1. Structure
2.2. Numerical Model
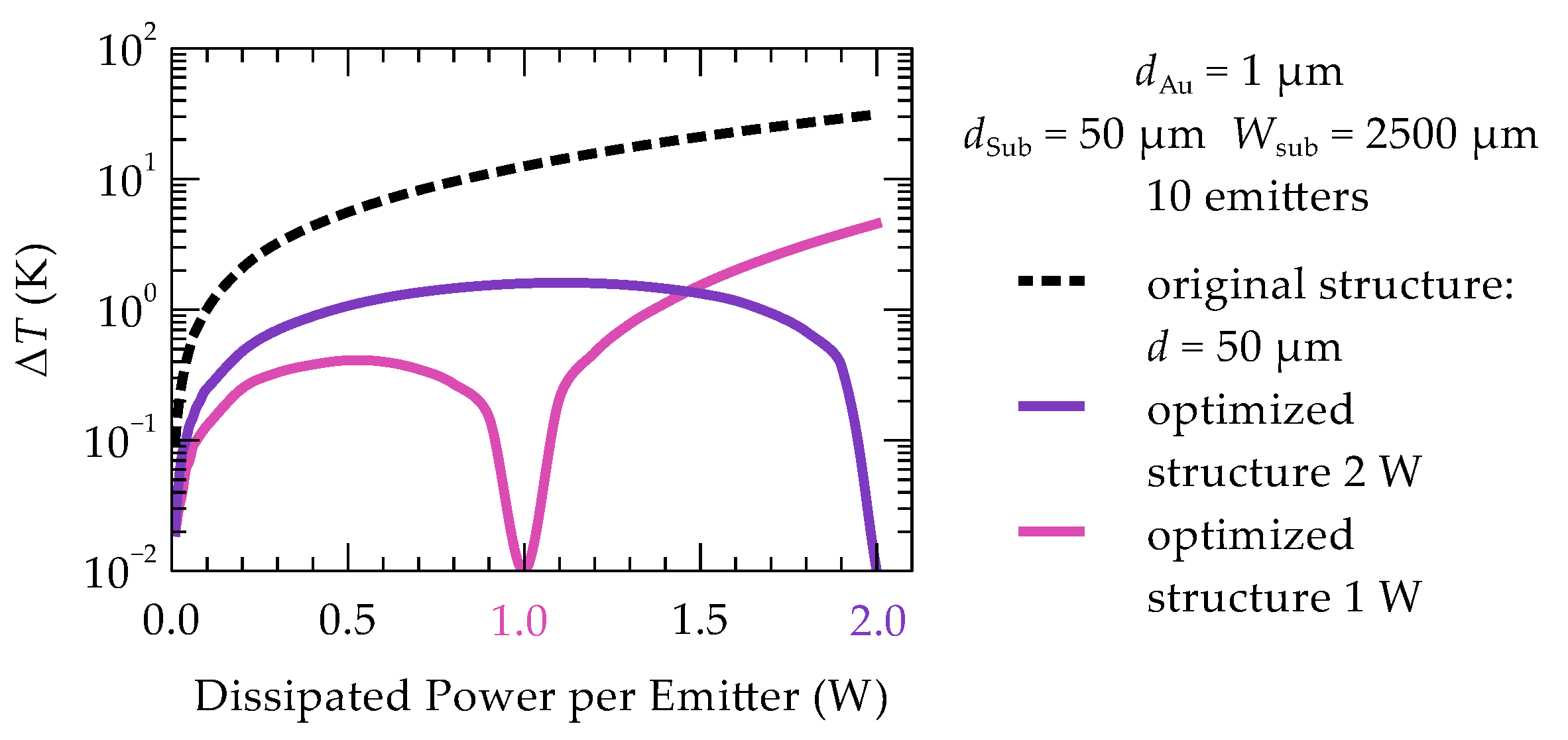
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Jiang, L.; Liu, J.; Tian, A.; Cheng, Y.; Li, Z.; Zhang, L.; Zhang, S.; Li, D.; Ikeda, M.; Yang, H. GaN-based green laser diodes. J. Semicond. 2016, 37, 111001. [Google Scholar] [CrossRef]
- Usman, M.; Munsif, M.; Mushtaq, U.; Anwar, A.R.; Muhammad, N. Green gap in GaN-based light-emitting diodes: In perspective. Crit. Rev. Solid State Mater. Sci. 2021, 46, 450–467. [Google Scholar] [CrossRef]
- Sizov, D.S.; Bhat, R.; en Zah, C. Challenges and approaches of fabricating GaN-based green lasers. In Proceedings of the 2011 International Semiconductor Device Research Symposium (ISDRS), College Park, MD, USA, 7–9 December 2011; pp. 1–2. [Google Scholar] [CrossRef]
- Sizov, D.; Bhat, R.; Zah, C.E. Gallium indium nitride-based green lasers. J. Light. Technol. 2012, 30, 679–699. [Google Scholar] [CrossRef]
- Nakatsu, Y.; Nagao, Y.; Kozuru, K.; Hirao, T.; Okahisa, E.; Masui, S.; Yanamoto, T.; ichi Nagahama, S. High-efficiency blue and green laser diodes for laser displays. In Proceedings of the SPIE, San Francisco, CA, USA, 1 March 2019; Volume 10918, p. 109181D. [Google Scholar] [CrossRef]
- Tian, A.; Hu, L.; Zhang, L.; Liu, J.; Yang, H. Design and growth of GaN-based blue and green laser diodes. Sci. China Mater. 2020, 63, 1348–1363. [Google Scholar] [CrossRef]
- Samonji, K.; Yoshida, S.; Hagino, H.; Yamanaka, K.; Takigawa, S. 6.3 W InGaN laser diode array with highly efficient wide-striped emitters. In Proceedings of the IEEE Photonic Society 24th Annual Meeting, Arlington, VA, USA, 9–13 October 2011; pp. 507–508. [Google Scholar]
- Suzuki, N.; Morimoto, K. 10-W CW blue-violet diode laser array on the micro-channel cooler. In Proceedings of the SPIE, San Francisco, CA, USA, 8 February 2012; Volume 8241, p. 82410J. [Google Scholar] [CrossRef]
- Perlin, P.; Marona, L.; Holc, K.; Wisniewski, P.; Suski, T.; Leszczynski, M.; Czernecki, R.; Najda, S.; Zajac, M.; Kucharski, R. InGaN Laser Diode Mini-Arrays. Appl. Phys. Express 2011, 4, 62103. [Google Scholar] [CrossRef]
- Goto, S.; Ohta, M.; Yabuki, Y.; Hoshina, Y.; Naganuma, K.; Tamamura, K.; Hashizu, T.; Ikeda, M. Super high-power AlGaInN-based laser diodes with a single broad-area stripe emitter fabricated on a GaN substrate. Phys. Status Solidi (A) 2003, 200, 122–125. [Google Scholar] [CrossRef]
- Najda, S.P.; Perlin, P.; Suski, T.; Marona, L.; Boćkowski, M.; Leszczyński, M.; Wisniewski, P.; Czernecki, R.; Kucharski, R.; Targowski, G. AlGaInN laser diode bar and array technology for high-power and individual addressable applications. In Proceedings of the SPIE, Brussels, Belgium, 28 April 2016; Volume 9892, p. 98920Z. [Google Scholar] [CrossRef]
- Samonji, K.; Yoshida, S.; Hagino, H.; Yamanaka, K.; Takigawa, S. High-power operation of a wide-striped InGaN laser diode array. In Proceedings of the SPIE, San Francisco, CA, USA, 8 February 2012; Volume 8277, p. 82771K. [Google Scholar] [CrossRef]
- Jawulski, K.; Kuc, M.; Sarzala, R.P. Simplified thermal analysis of impact of diamond heat spreader on InGaN laser diode arrays. In Proceedings of the SPIE, Szczecin, Poland, 22 January 2013; Volume 8702, p. 87020D. [Google Scholar] [CrossRef]
- Kozlowska, A.; Maląg, A. Investigations of transient thermal properties of conductively cooled diode laser arrays operating under quasicontinuous-wave conditions. Microelectron. Reliab. 2006, 46, 2079–2084. [Google Scholar] [CrossRef]
- Qiao, Y.; Feng, S.; Xiong, C.; Ma, X.; Zhu, H.; Guo, C.; Wei, G. The thermal properties of AlGaAs/GaAs laser diode bars analyzed by the transient thermal technique. Solid-State Electron. 2013, 79, 192–195. [Google Scholar] [CrossRef]
- Laff, R.A.; Comerford, L.D.; Crow, J.D.; Brady, M.J. Thermal performance and limitations of silicon–substrate packaged GaAs laser arrays. Appl. Opt. 1978, 17, 778–784. [Google Scholar] [CrossRef] [PubMed]
- Garmire, E.; Tavis, M. Heatsink requirements for coherent operation of high-power semiconductor laser arrays. IEEE J. Quantum Electron. 1984, 20, 1277–1283. [Google Scholar] [CrossRef]
- Murata, S.; Nishimura, K. Improvement in Thermal Properties of a Multi-Beam Laser Diode Array. Jpn. J. Appl. Phys. 1989, 28, 165. [Google Scholar] [CrossRef]
- Sato, K.; Murakami, M. Experimental investigation of thermal crosstalk in a distributed feedback laser array. IEEE Photonics Technol. Lett. 1991, 3, 501–503. [Google Scholar] [CrossRef]
- Osinski, M.; Nakwaski, W. Thermal analysis of closely-packed two-dimensional etched-well surface-emitting laser arrays. IEEE J. Sel. Top. Quantum Electron. 1995, 1, 681–696. [Google Scholar] [CrossRef]
- Wipiejewski, T.; Young, D.B.; Thibeault, B.J.; Coldren, L.A. Thermal crosstalk in 4 × 4 vertical-cavity surface-emitting laser arrays. IEEE Photonics Technol. Lett. 1996, 8, 980–982. [Google Scholar] [CrossRef]
- Nakwaski, W.; Osinski, M. Erratum: Thermal resistance of top-surface-emitting vertical-cavity semiconductor lasers and monolithic two-dimensional arrays. Electron. Lett. 1992, 28, 572–574. [Google Scholar] [CrossRef]
- Kuc, M.; Sarzała, R.P.; Nakwaski, W. Thermal crosstalk in arrays of III-N-based Lasers. Mater. Sci. Eng. B 2013, 178, 1395–1402. [Google Scholar] [CrossRef]
- Sarzała, R.P.; Sokół, A.K.; Kuc, M.; Nakwaski, W. How to enhance a room-temperature operation of diode lasers and their arrays. Opt. Appl. 2016, 46, 213–226. [Google Scholar]
- Dąbrówka, D.; Sarzała, R.; Wasiak, M.; Kafar, A.; Perlin, P.; Saba, K. Thermal analysis of a two-dimensional array with surface light emission based on nitride EEL lasers. Opto-Electron. Rev. 2022, 30, e144115. [Google Scholar] [CrossRef]
- Hu, J.J.; Zhang, S.M.; Li, D.Y.; Zhang, F.; Feng, M.X.; Wen, P.Y.; Liu, J.P.; Zhang, L.Q.; Yang, H. Thermal analysis of GaN-based laser diode mini-array*. Chin. Phys. B 2018, 27, 094208. [Google Scholar] [CrossRef]
- Vetrovec, J. Improved cooling for high-power laser diodes. In Proceedings of the SPIE, San Jose, CA, USA, 20 February 2008; Volume 6876, p. 687603. [Google Scholar] [CrossRef]
- Kozłowska, A.; Teodorczyk, M.; Dąbrowska-Tumańska, E.; Chmielewski, M.; Podniesiński, D.; Maląg, A. Novel micro-channel cooler for high-power diode laser arrays. Photonics Lett. Pol. 2014, 6, 23–25. [Google Scholar] [CrossRef]
- Dix, J.; Jokar, A.; Martinsen, R. Enhanced microchannel cooling for high-power semiconductor diode lasers. In Proceedings of the SPIE, San Jose, CA, USA, 13 February 2008; Volume 6876, p. 687606. [Google Scholar] [CrossRef]
- Pais, M.; Chang, M.; Morgan, M.; Chow, L. Spray Cooling of a High Power Laser Diode. In Proceedings of the Aerospace Atlantic Conference & Exposition. SAE International, Dayton, OH, USA, 18–22 April 1994. [Google Scholar] [CrossRef]
- Benett, W.J.; Freitas, B.L.; Ciarlo, D.R.; Beach, R.J.; Sutton, S.B.; Emanuel, M.A.; Solarz, R.W. Microchannel-cooled heatsinks for high-average-power laser diode arrays. In Proceedings of the SPIE, Tromsö, Norway, 30 June 1993; Volume 1865, pp. 144–153. [Google Scholar] [CrossRef]
- Krause, V.K.; Treusch, H.G.; Loosen, P.; Kimpel, T.; Biesenbach, J.; Koesters, A.; Robert, F.; Oestreicher, H.; Marchiano, M.; DeOdorico, B. Microchannel coolers for high-power laser diodes in copper technology. In Proceedings of the SPIE, Bergen, Norway, 13–15 June 1994; Volume 2148, pp. 351–358. [Google Scholar] [CrossRef]
- Huddle, J.J.; Chow, L.C.; Lei, S.; Marcos, A.; Rini, D.P.; Lindauer, S.J.; Bass, M.; Delfyett, P.J. Thermal management of diode laser arrays. In Proceedings of the Sixteenth Annual IEEE Semiconductor Thermal Measurement and Management Symposium (Cat. No.00CH37068), San Jose, CA, USA, 23–23 March 2000; pp. 154–160. [Google Scholar] [CrossRef]
- Wang, J.; Kang, L.; Zhang, P.; Nie, Z.; Li, X.; Xiong, L.; Liu, X. High-power semiconductor laser array packaged on microchannel cooler using gold-tin soldering technology. In Proceedings of the SPIE, San Francisco, CA, USA, 8 February 2012; Volume 8241, p. 82410H. [Google Scholar] [CrossRef]
- Liu, X.; Davis, R.W.; Hughes, L.C.; Rasmussen, M.H.; Bhat, R.; Zah, C.E.; Stradling, J. A study on the reliability of indium solder die bonding of high power semiconductor lasers. J. Appl. Phys. 2006, 100, 013104. [Google Scholar] [CrossRef]
- Barwolff, A.; Tomm, J.; Muller, R.; Weiß, S.; Hutter, M.; Oppermann, H.; Reichl, H. Spectroscopic measurement of mounting-induced strain in optoelectronic devices. IEEE Trans. Adv. Packag. 2000, 23, 170–175. [Google Scholar] [CrossRef]
- Mizuishi, K. Some aspects of bonding-solder deterioration observed in long-lived semiconductor lasers: Solder migration and whisker growth. J. Appl. Phys. 1984, 55, 289–295. [Google Scholar] [CrossRef]
- Dhamdhere, A.R.; Malshe, A.P.; Schmidt, W.F.; Brown, W.D. Investigation of reliability issues in high power laser diode bar packages. Microelectron. Reliab. 2003, 43, 287–295. [Google Scholar] [CrossRef]
- Kuc, M.; Piskorski, Ł.; Dems, M.; Wasiak, M.; Sokół, A.K.; Sarzała, R.P.; Czyszanowski, T. Numerical Investigation of the Impact of ITO, AlInN, Plasmonic GaN and Top Gold Metalization on Semipolar Green EELs. Materials 2020, 13, 1444. [Google Scholar] [CrossRef]
- Myzaferi, A.; Reading, A.H.; Farrell, R.M.; Cohen, D.A.; Nakamura, S.; DenBaars, S.P. Semipolar III-nitride laser diodes with zinc oxide cladding. Opt. Express 2017, 25, 16922–16930. [Google Scholar] [CrossRef] [PubMed]
- Myzaferi, A.; Mughal, A.J.; Cohen, D.A.; Farrell, R.M.; Nakamura, S.; Speck, J.S.; DenBaars, S.P. Zinc oxide clad limited area epitaxy semipolar III-nitride laser diodes. Opt. Express 2018, 26, 12490–12498. [Google Scholar] [CrossRef]
- Reading, A.H.; Richardson, J.J.; Pan, C.C.; Nakamura, S.; DenBaars, S.P. High efficiency white LEDs with single-crystal ZnO current spreading layers deposited by aqueous solution epitaxy. Opt. Express 2012, 20, A13–A19. [Google Scholar] [CrossRef] [PubMed]
- Mion, C.; Muth, J.; Preble, E.; Hanser, D. Accurate dependence of gallium nitride thermal conductivity on dislocation density. Appl. Phys. Lett. 2006, 89, 92123. [Google Scholar] [CrossRef]
- Oshima, Y.; Yoshida, T.; Eri, T.; Shibata, M.; Mishima, T. Thermal and electrical properties of high-quality freestanding GaN wafers with high carrier concentration. Phys. Status Solidi C 2007, 4, 2215–2218. [Google Scholar] [CrossRef]
- Daly, B.C.; Maris, H.J.; Nurmikko, A.V.; Kuball, M.; Han, J. Optical pump-and-probe measurement of the thermal conductivity of nitride thin films. J. Appl. Phys. 2002, 92, 3820–3824. [Google Scholar] [CrossRef]
- Pantha, B.N.; Dahal, R.; Li, J.; Lin, J.Y.; Jiang, H.X.; Pomrenke, G. Thermoelectric properties of InxGa1-xN alloys. Appl. Phys. Lett. 2008, 92, 042112. [Google Scholar] [CrossRef]
- Zhang, J.; Tong, H.; Liu, G.; Herbsommer, J.A.; Huang, G.S.; Tansu, N. Thermoelectric properties of MOCVD-grown AlInN alloys with various compositions. In Proceedings of the SPIE, San Francisco, CA, USA, 3 March 2011; Volume 7939, p. 79390O. [Google Scholar] [CrossRef]
- Osinski, M.; Nakwaski, W.; Leal, A. Effective thermal conductivity analysis of vertical-cavity top-surface-emitting lasers with semiconducting Bragg mirrors. In Proceedings of the SPIE, Los Angeles, CA, USA, 2 June 1994; Volume 2147, pp. 85–96. [Google Scholar] [CrossRef]
- Lide, D.R. (Ed.) CRC Handbook of Chemistry and Physics; CRC Press: Boca Raton, FL, USA, 2004; Volume 85. [Google Scholar]
- Shackelford, J.F.; Alexander, W. (Eds.) CRC Materials Science and Engineering Handbook; CRC Press: Boca Raton, FL, USA, 2000. [Google Scholar] [CrossRef]
- Wu, X.; Lee, J.; Varshney, V.; Wohlwend, J.L.; Roy, A.K.; Luo, T. Thermal Conductivity of Wurtzite Zinc-Oxide from First-Principles Lattice Dynamics–a Comparative Study with Gallium Nitride. Sci. Rep. 2016, 6, 22504. [Google Scholar] [CrossRef] [PubMed]
- Dems, M.; Beling, P.; Gębski, M.; Piskorski., Ł.; Walczak, J.; Kuc, M.; Frasunkiewicz, L.; Michał, W.; Sarzała, R.; Czyszanowski, T. VCSEL Modeling with Self-Consistent Models: From Simple Approximations to Comprehensive Numerical Analysis. In Proceedings of the SPIE, San Francisco, CA, USA, 4 March 2015; Volume 9381, p. 93810K. [Google Scholar] [CrossRef]
- PLaSK: A Comprehensive Tool for Numerical Analysis of a Broad Range of Physical Phenomena in Photonic Devices. 2024. Available online: https://plask.app (accessed on 19 December 2024).
- Czyszanowski, T.; Stańczyk, S.; Kafar, A.; Perlin, P. Optical Optimization of InGaN/GaN Edge-Emitting Lasers with Reduced AlGaN Cladding Thickness. Jpn. J. Appl. Phys. 2014, 53, 032701. [Google Scholar] [CrossRef]
- Janczak, M.; Sarzała, R.P.; Dems, M.; Kolek, A.; Bugajski, M.; Nakwaski, W.; Czyszanowski, T. Threshold Performance of Pulse-Operating Quantum-Cascade Vertical-Cavity Surface-Emitting Lasers. Opt. Express 2022, 30, 45054. [Google Scholar] [CrossRef] [PubMed]
- Więckowska, M.; Dems, M. Increasing Single-Mode Power in VCSELs With Antiresonant Oxide Island. IEEE Photonics J. 2024, 16, 1–7. [Google Scholar] [CrossRef]
- Dems, M.; Chung, I.S.; Nyakas, P.; Bischoff, S.; Panajotov, K. Numerical Methods for Modeling Photonic-Crystal VCSELs. Opt. Express 2010, 18, 16042. [Google Scholar] [CrossRef] [PubMed]
- Janaszek, A.; Wróbel, P.; Dems, M.; Ceylan, O.; Gurbuz, Y.; Kubiszyn, Ł.; Piotrowski, J.; Kotyński, R. Rigorous Optical Modelling of Long-Wavelength Infrared Photodetector with 2D Subwavelength Hole Array in Gold Film. Opto-Electron. Rev. 2024, 32, e148831. [Google Scholar] [CrossRef]
- Ekielski, M.; Głowadzka, W.; Bogdanowicz, K.; Rygała, M.; Mikulicz, M.; Śpiewak, P.; Kowalski, M.; Gębski, M.; Motyka, M.; Szerling, A.; et al. Monolithic High Contrast Grating Integrated with Metal: Infrared Electrode with Exceptionally High Conductivity and Transmission. Adv. Funct. Mater. 2023, 34, 2312392. [Google Scholar] [CrossRef]
- Pruszyńska-Karbownik, E.; Jandura, D.; Dems, M.; Zinkiewicz, Ł.; Broda, A.; Gębski, M.; Muszalski, J.; Pudiš, D.; Suffczyński, J.; Czyszanowski, T. Concept of Inverted Refractive-Index-Contrast Grating Mirror and Exemplary Fabrication by 3D Laser Micro-Printing. Nanophotonics 2023, 12, 3579–3588. [Google Scholar] [CrossRef]
- Mikulicz, M.; Rygała, M.; Smołka, T.; Janczak, M.; Badura, M.; Łozińska, A.; Wolf, A.; Emmerling, M.; Ściana, B.; Höfling, S.; et al. Enhancement of Quantum Cascade Laser Intersubband Transitions via Coupling to Resonant Discrete Photonic Modes of Subwavelength Gratings. Opt. Express 2023, 31, 26898–26909. [Google Scholar] [CrossRef] [PubMed]
- Schade, A.; Bader, A.; Huber, T.; Kuhn, S.; Czyszanowski, T.; Pfenning, A.; Rygała, M.; Smołka, T.; Motyka, M.; Sęk, G.; et al. Monolithic High Contrast Grating on GaSb/AlAsSb Based Epitaxial Structures for Mid-Infrared Wavelength Applications. Opt. Express 2023, 31, 16025–16034. [Google Scholar] [CrossRef] [PubMed]
- Virtanen, P.; Gommers, R.; Oliphant, T.E.; Haberland, M.; Reddy, T.; Cournapeau, D.; Burovski, E.; Peterson, P.; Weckesser, W.; Bright, J.; et al. SciPy 1.0: Fundamental Algorithms for Scientific Computing in Python. Nat. Methods 2020, 17, 261–272. [Google Scholar] [CrossRef]
- Kraft, D. A Software Package for Sequential Quadratic Programming; Tech. Rep. DFVLR-FB 88-28; DLR German Aerospace Center—Institute for Flight Mechanics: Koln, Germany, 1988. [Google Scholar]
- Ocaya, R.O. Thermal tuning of light-emitting diode wavelength as an implication of the Varshni equation. Measurement 2020, 162, 107910. [Google Scholar] [CrossRef]
- Madhusoodhanan, S.; Sabbar, A.; Al-kabi, S.; Atcitty, S.; Kaplar, R.; Dong, B.; Wang, J.; Yu, Q.; Chen, Z. High-Temperature Optical Characterization of Wide Band Gap Light Emitting Diodes and Photodiodes for Future Power Module Application. Adv. Sci. Technol. Eng. Syst. J. 2019, 4, 17–22. [Google Scholar] [CrossRef]
- Wang, G.; Tao, X.; Liu, J.; Jiang, F. Temperature-dependent electroluminescence from InGaN/GaN green light-emitting diodes on silicon with different quantum-well structures. Semicond. Sci. Technol. 2014, 30, 015018. [Google Scholar] [CrossRef]
- Jafar, N.; Jiang, J.; Lu, H.; Qasim, M.; Zhang, H. Recent Research on Indium-Gallium-Nitride-Based Light-Emitting Diodes: Growth Conditions and External Quantum Efficiency. Crystals 2023, 13, 1623. [Google Scholar] [CrossRef]
- Liu, J.; Tam, W.; Wong, H.; Filip, V. Temperature-dependent light-emitting characteristics of InGaN/GaN diodes. Microelectron. Reliab. 2009, 49, 38–41. [Google Scholar] [CrossRef]
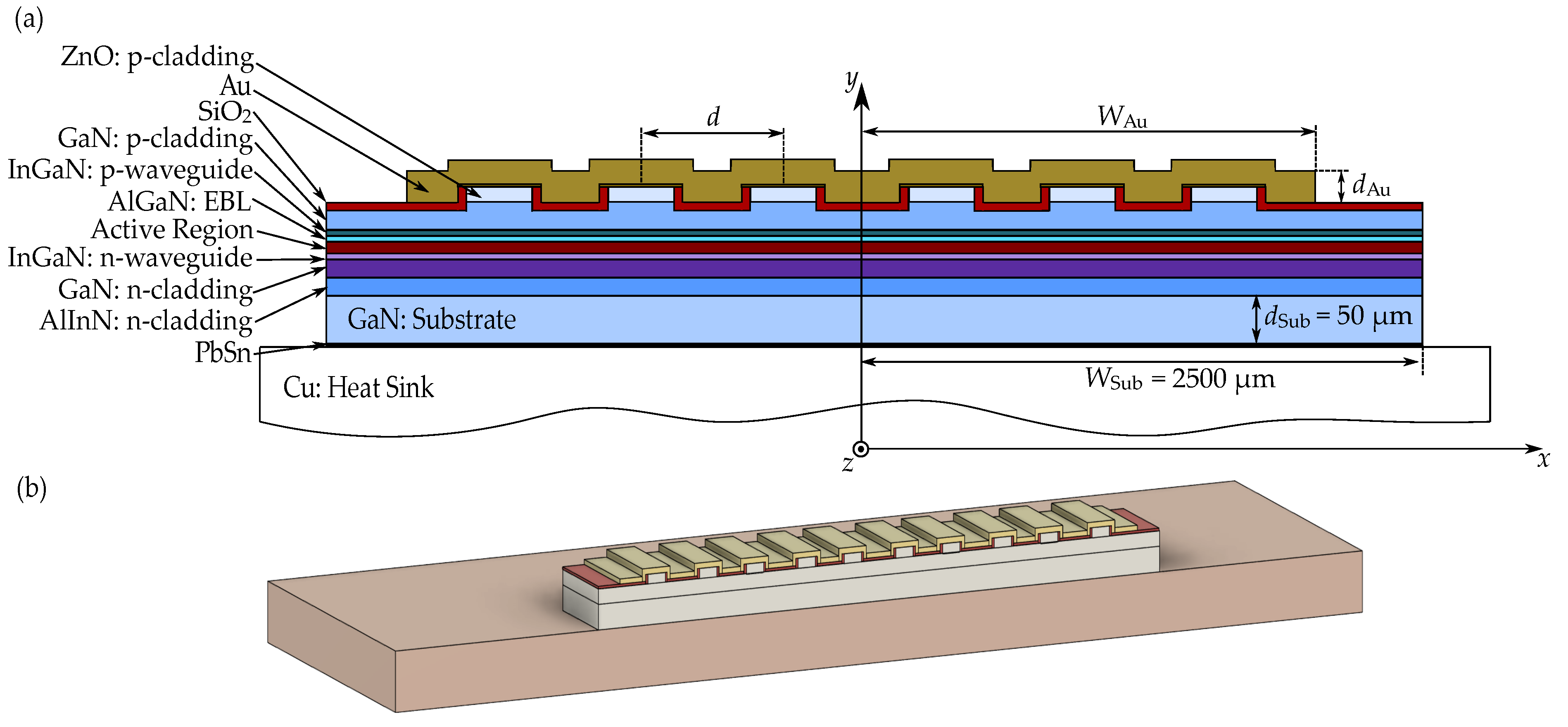
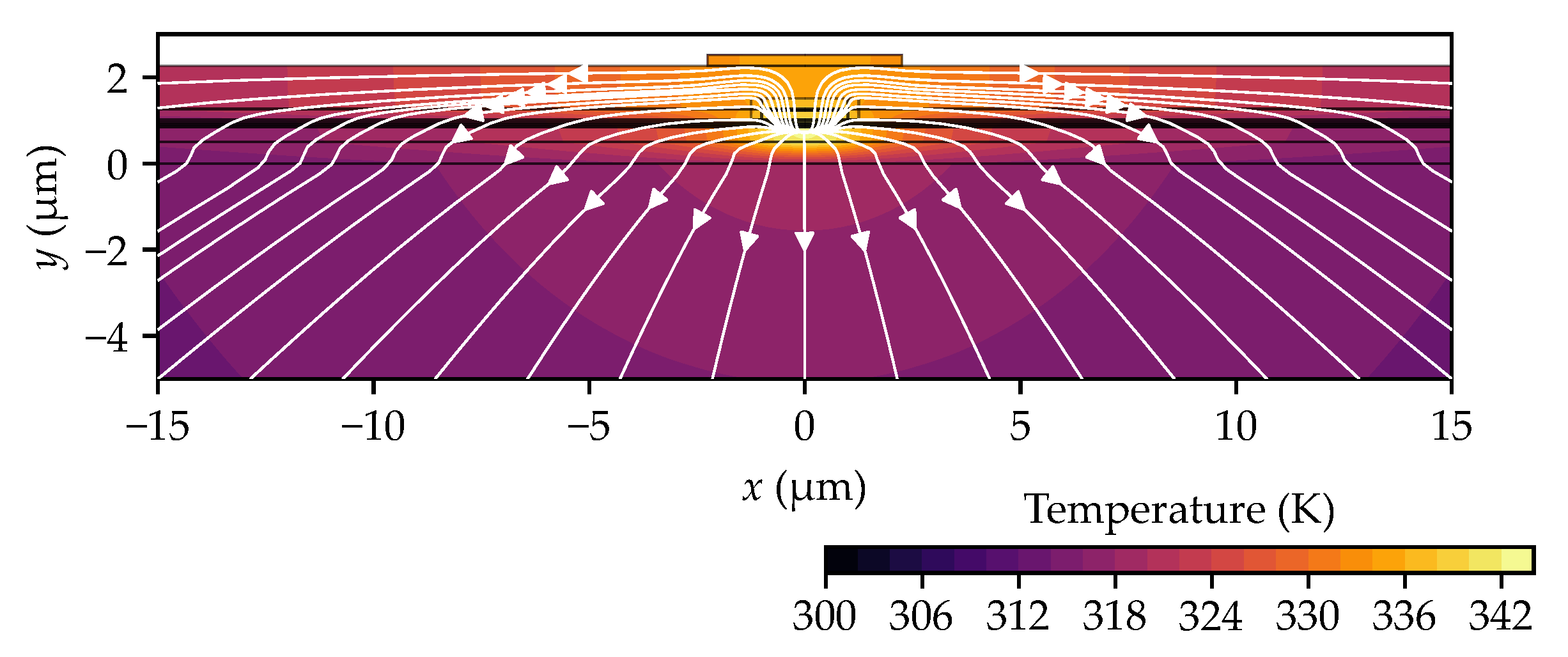
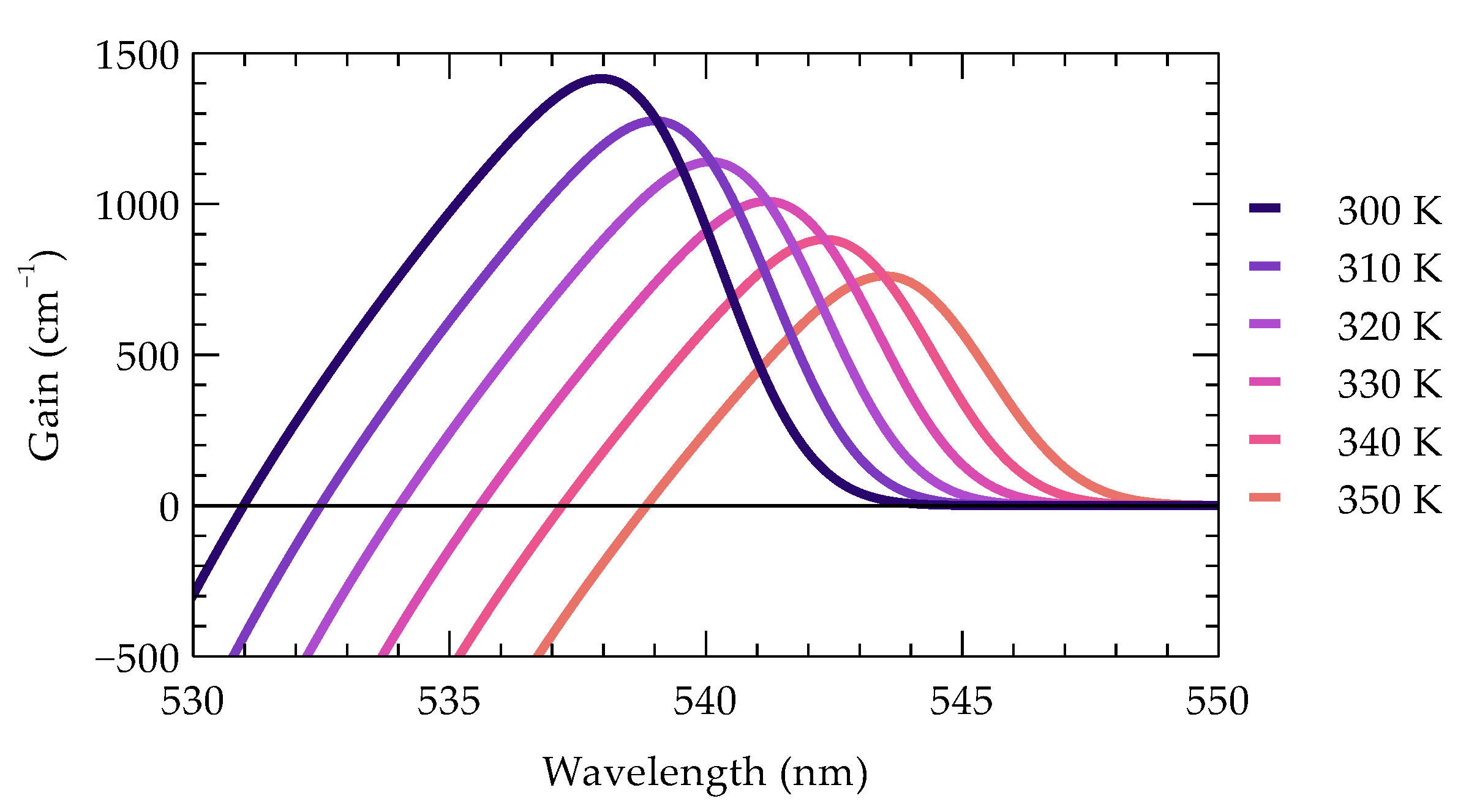


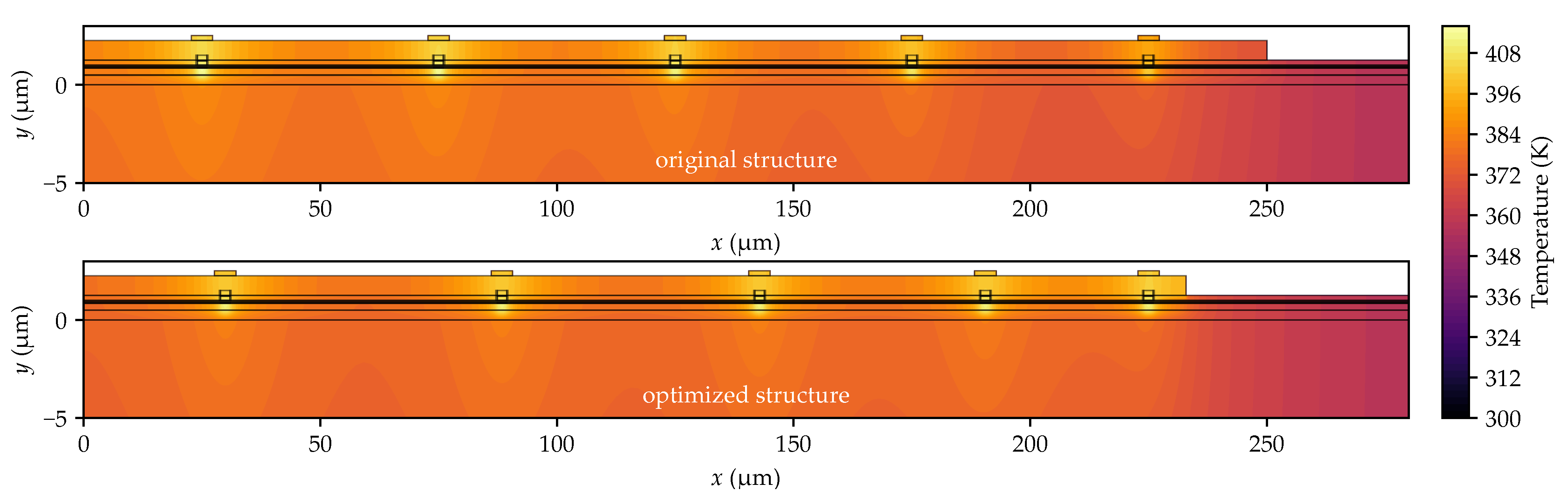

| Layer | Material | Thickness (µm) | Doping () | (W/(mK)) | (W/(mK)) | (W/(mK)) | ||||
|---|---|---|---|---|---|---|---|---|---|---|
| p-side contact | Au | 1.00 | - | 317.10 | 310.70 | 304.30 | ||||
| Insulator | 0.25 | - | 1.29 | 1.45 | 1.56 | |||||
| p-cladding | ZnO | 0.28 | - | 50.00 | 50.00 | 50.00 | ||||
| p-GaN | 0.28 | Mg: | 92.00 | 61.50 | 45.00 | |||||
| p-waveguide | p-N | 0.045 | Mg: | 23.00 | 15.37 | 11.25 | ||||
| EBL | p-N | 0.01 | Mg: | 13.00 | 8.69 | 6.36 | ||||
| Active region | 3 × QW 4 × barrier | N GaN | 0.0027 0.01 | Si-doped - | 48.46 | 8.39 | 32.39 | 5.61 | 23.70 | 4.10 |
| n-waveguide | n-N | 0.01 | Si: | 28.00 | 18.72 | 13.70 | ||||
| n-cladding | n-GaN | 0.35 | Si: | 50.00 | 50.00 | 50.00 | ||||
| n-N | 0.50 | Si: | 94.87 | 3.26 | 2.38 | |||||
| Substrate | n-GaN | 50.00 | Si: | 166.00 | 110.97 | 81.19 | ||||
| Solder | PbSn | 1.00 | - | 50.00 | 50.00 | 50.00 | ||||
| Heat sink | Cu | 5000 | - | 400.80 | 392.47 | 386.13 | ||||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sarzała, R.P.; Dąbrówka, D.; Dems, M. Thermal Optimization of Edge-Emitting Lasers Arrays. Materials 2025, 18, 107. https://doi.org/10.3390/ma18010107
Sarzała RP, Dąbrówka D, Dems M. Thermal Optimization of Edge-Emitting Lasers Arrays. Materials. 2025; 18(1):107. https://doi.org/10.3390/ma18010107
Chicago/Turabian StyleSarzała, Robert P., Dominika Dąbrówka, and Maciej Dems. 2025. "Thermal Optimization of Edge-Emitting Lasers Arrays" Materials 18, no. 1: 107. https://doi.org/10.3390/ma18010107
APA StyleSarzała, R. P., Dąbrówka, D., & Dems, M. (2025). Thermal Optimization of Edge-Emitting Lasers Arrays. Materials, 18(1), 107. https://doi.org/10.3390/ma18010107









