Multiscale Elastic Modulus Characterization of Ti0.5Fe0.45Mn0.05, an Iron–Titanium–Manganese Alloy Dedicated to Hydrogen Storage
Abstract
1. Introduction
2. Materials and Methods
2.1. Sample Preparation
2.2. Experimental Methods
2.2.1. Ultrasonic Elastic Characterization
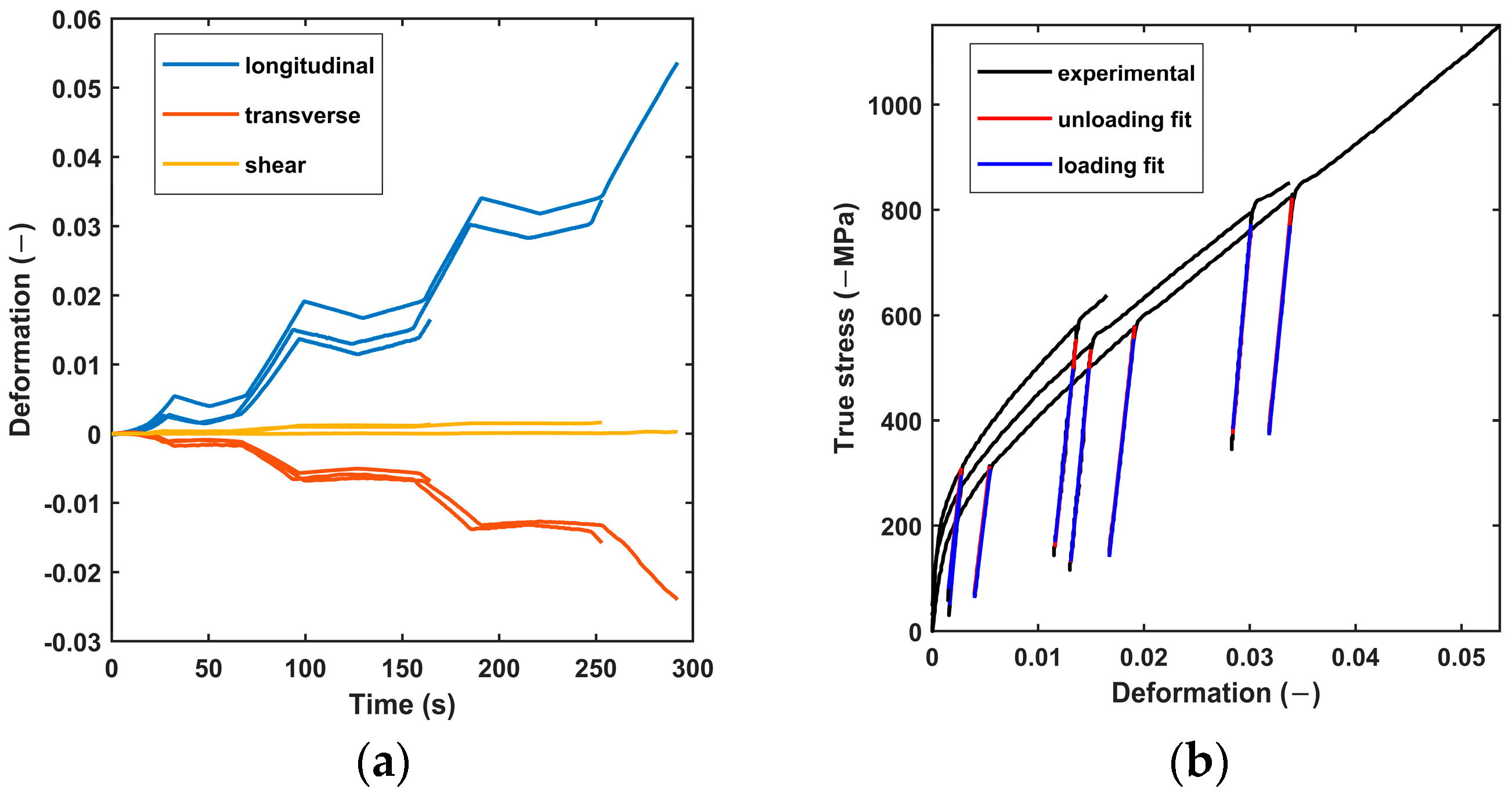
2.2.2. Compression Test
2.2.3. Micro-Scale Measurement
3. Results
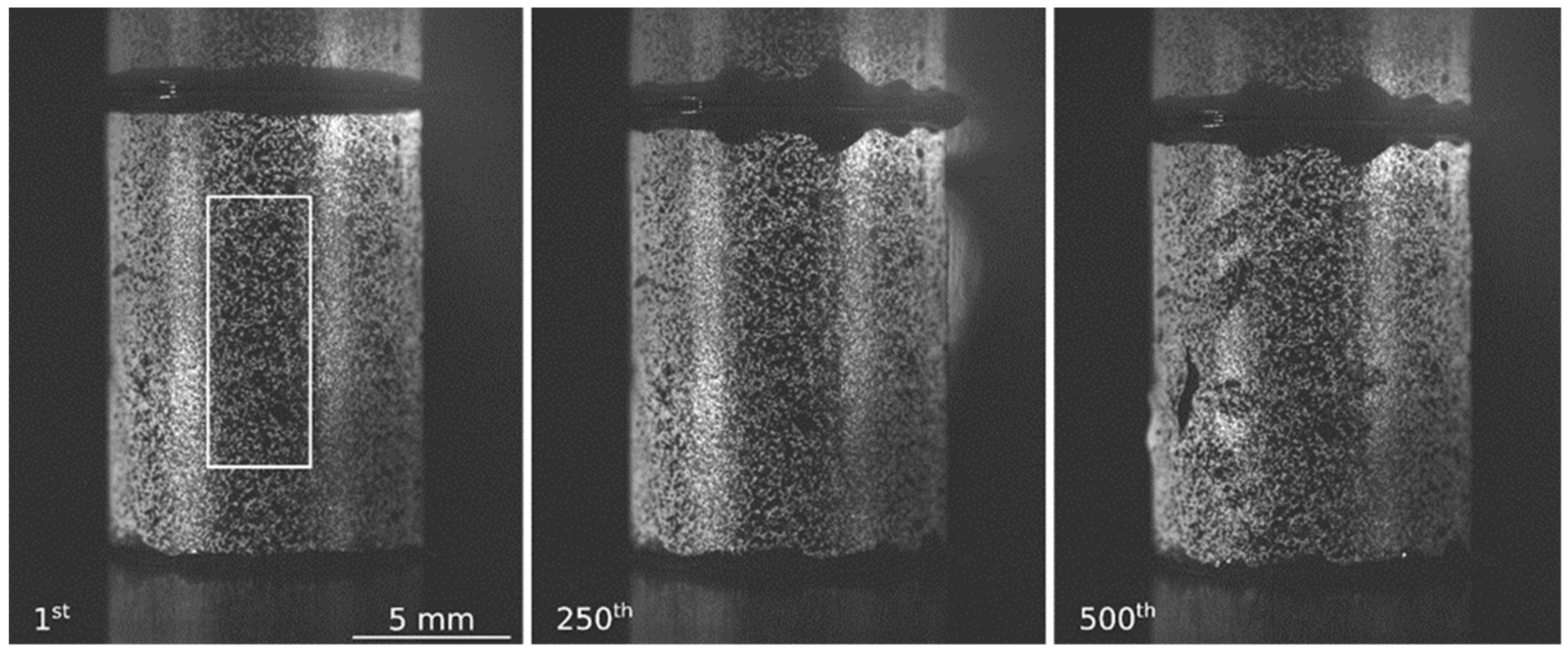
3.1. Macroscopic Structure
3.2. Ultrasonic Elastic Characterization Results
3.3. Compression Test Characterization Results
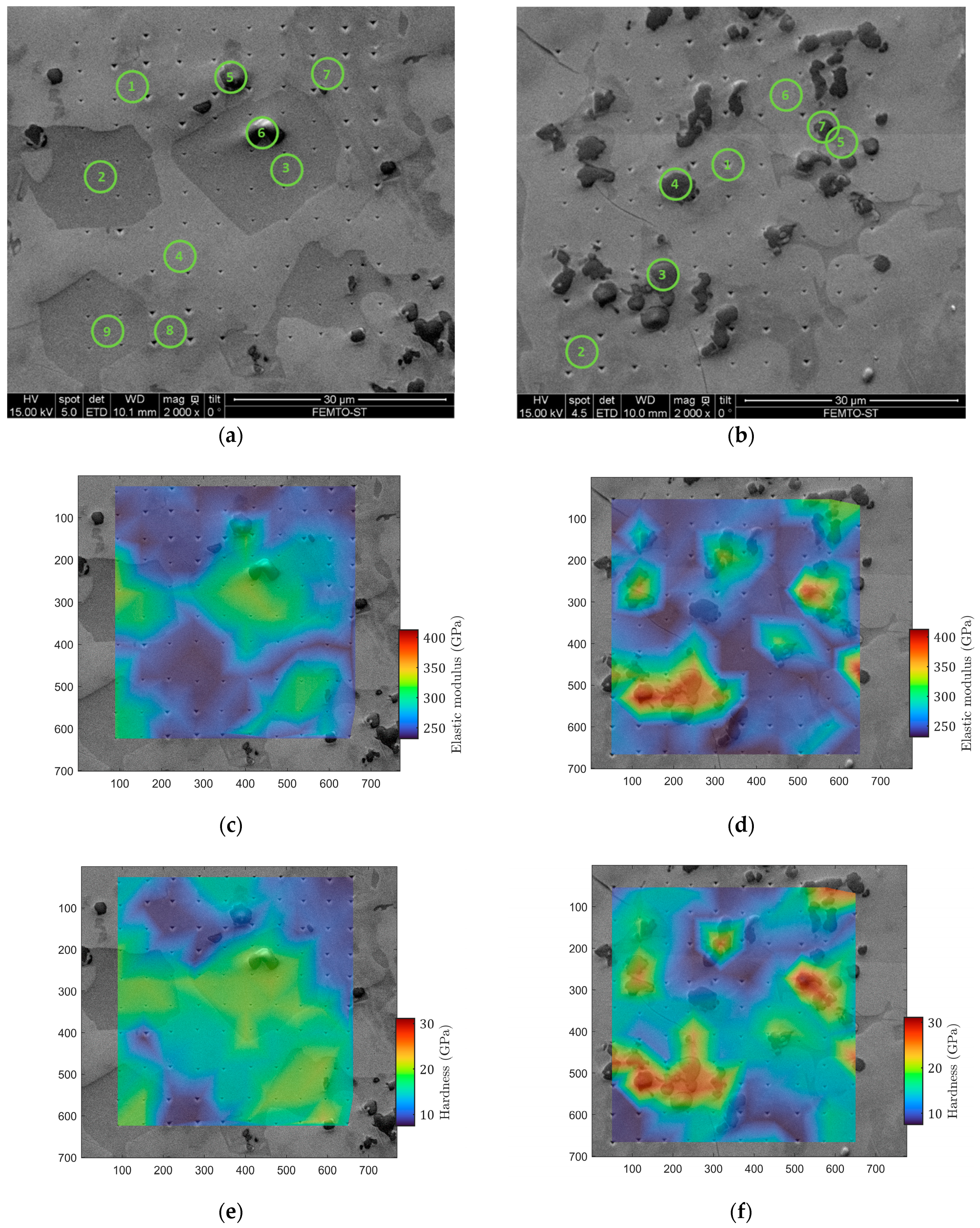
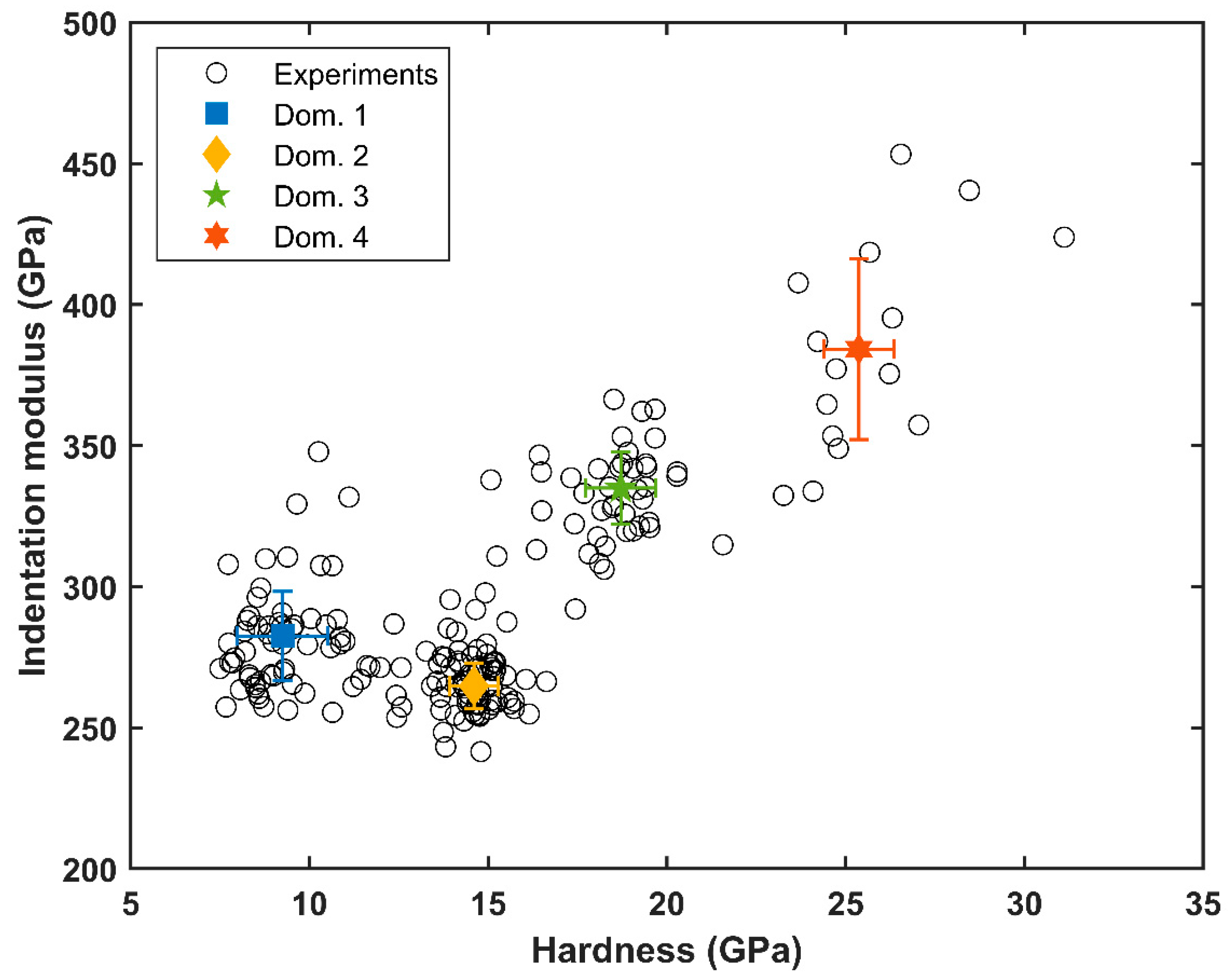
3.4. Nanoindentation Measurements and Energy-Dispersive Spectroscopy Results
4. Discussion
5. Conclusions
- Through compression testing, it was possible to assess to the elastic modulus representative of the macroscopic behavior of the bulk material, considering all its defects (pre-existing cracks) and its microstructural heterogeneity. The encountered value, between 163 and 229 GPa, seems to increase with deformation due to closure cracks. In this way, the values encountered on compression appear slightly low compared to those expected for a homogenous, isotropic and perfect material.
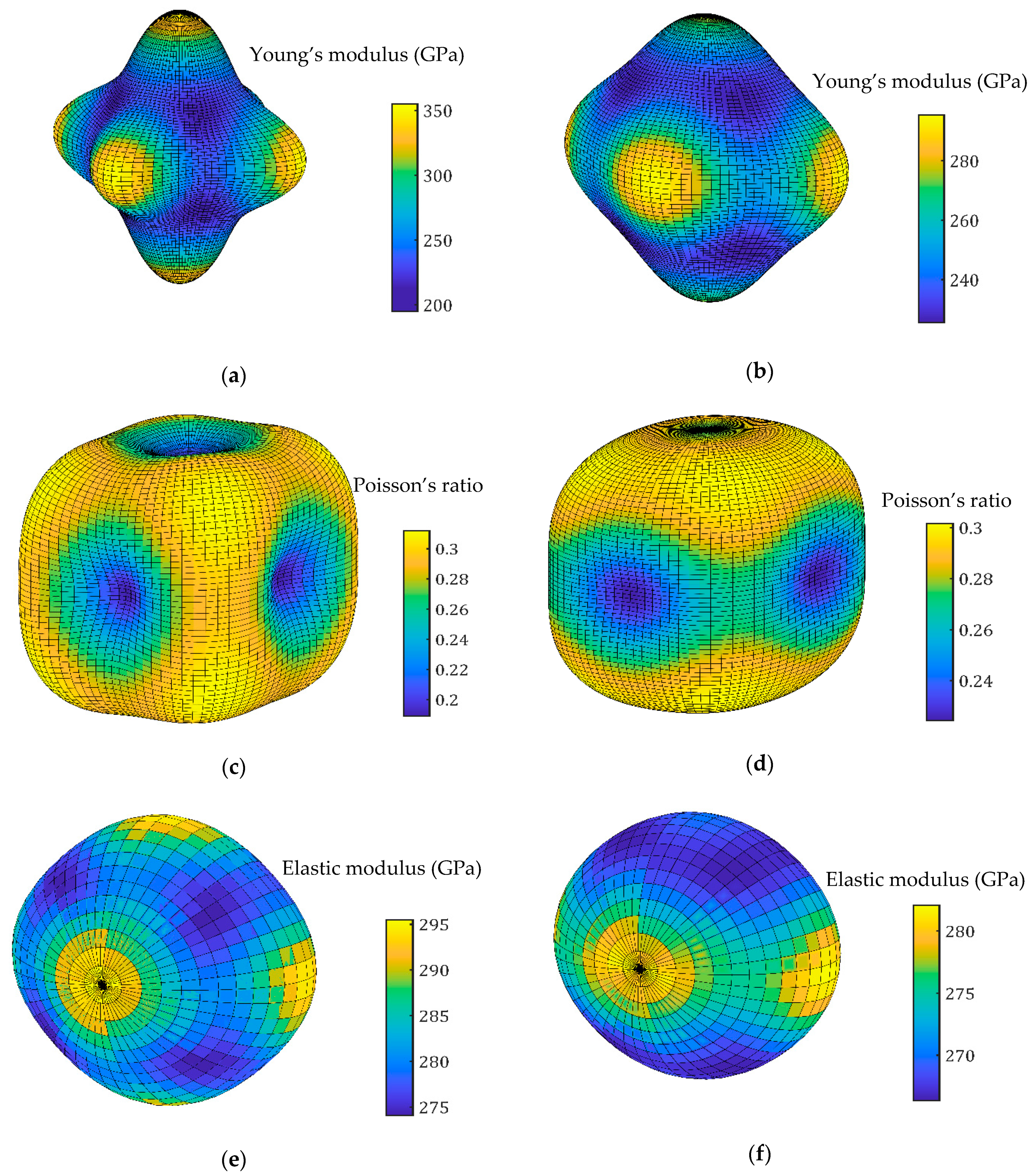
- Combining ultrasonic wave propagation and instrumented indentation, it was possible to extract a representative Young’s modulus and Poisson’s ratio for each of the different phase present in the material. In fact, both techniques allowed us to obtain a numerical value of the modulus as a function of the Poisson’s ratio. A Young’s modulus of 269 GPa and a Poisson’s ratio of 0.29 were found. These values are in agreement with the one encountered in the literature and determined by an ab initio technique, at least for TiFe and TiFe2, but with a high standard deviation due to phase heterogeneity among the different phases present in the material.
- A closer examination of the local indentation response together with microstructural and chemical analysis allowed us to attribute specific elastic behavior to each identified phase present in the material, four in total. In particular, depending on the local proportions of Fe, Ti and Mn, the indentation elastic modulus of the TiFeMn alloy may vary from 265 to 333 GPa and the hardness from 9.2 to 18.7 GPa. Additionally, the presence of nitride or carbide phase, having an indentation elastic modulus of 385 GPa and a hardness of 25.4 GPa, has also been evidenced.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Challet, S.; Latroche, M.; Heurtaux, F. Hydrogenation Properties and Crystal Structure of the Single BCC (Ti0.355V0.645)100−xMx Alloys with M = Mn, Fe, Co, Ni (X = 7, 14 and 21). J. Alloys Compd. 2007, 439, 294–301. [Google Scholar] [CrossRef]
- Gamo, T.; Moriwaki, Y.; Yanagihara, N.; Yamashita, T.; Iwaki, T. Formation and Properties of Titanium-Manganese Alloy Hydrides. Int. J. Hydrogen Energy 1985, 10, 39–47. [Google Scholar] [CrossRef]
- Lee and Perng, Effect of the second phase on the initiation of hydrogenation of TiFe1−xMx (M = Cr, Mn) alloys. Int. J. Hydrogen Energy 1994, 19, 259–262. [CrossRef]
- Lin, C.-K.; Chen, Y.-C. Effects of Cyclic Hydriding–Dehydriding Reactions of LaNi5 on the Thin-Wall Deformation of Metal Hydride Storage Vessels with Various Configurations. Renew. Energy 2012, 48, 404–410. [Google Scholar] [CrossRef]
- Joubert, J.-M.; Latroche, M.; Černý, R.; Percheron-Guégan, A.; Yvon, K. Hydrogen Cycling Induced Degradation in LaNi5-Type Materials. J. Alloys Compd. 2002, 330–332, 208–214. [Google Scholar] [CrossRef]
- Zeaiter, A.; Chapelle, D.; Cuevas, F.; Maynadier, A.; Latroche, M. Milling Effect on the Microstructural and Hydrogenation Properties of TiFe0.9Mn0.1 Alloy. Powder Technol. 2018, 339, 903–910. [Google Scholar] [CrossRef]
- Okumura, M.; Ikado, A.; Saito, Y.; Aoki, H.; Miura, T.; Kawakami, Y. Pulverization Mechanism of Hydrogen Storage Alloys on Microscale Packing Structure. Int. J. Hydrogen Energy 2012, 37, 10715–10723. [Google Scholar] [CrossRef]
- Charlas, B.; Chaise, A.; Gillia, O.; Doremus, P.; Imbault, D. Investigation of Hydride Powder Bed Swelling and Shrinking during Hydrogen Absorption/Desorption Cycles under Different Compressive Stresses. J. Alloys Compd. 2013, 580, S149–S152. [Google Scholar] [CrossRef]
- Duan, W.; Du, J.; Wang, Z.; Niu, Y.; Huang, T.; Li, Z.; Pu, C.; Wu, Z. Strain Variation on the Reaction Tank of High Hydrogen Content during Hydrogen Absorption-Desorption Cycles. Int. J. Hydrogen Energy 2013, 38, 2347–2351. [Google Scholar] [CrossRef]
- Nasako, K.; Ito, Y.; Hiro, N.; Osumi, M. Stress on a Reaction Vessel by the Swelling of a Hydrogen Absorbing Alloy. J. Alloys Compd. 1998, 264, 271–276. [Google Scholar] [CrossRef]
- Saito, T.; Suwa, K.; Kawamura, T. Influence of Expansion of Metal Hydride during Hydriding–Dehydriding Cycles. J. Alloys Compd. 1997, 253–254, 682–685. [Google Scholar] [CrossRef]
- Salque, B.; Chaise, A.; Iosub, V.; Gillia, O.; Charlas, B.; Dupuis, C.; Guenoux, L. Measure of the Hydride Breathing While Cyclically Absorbing and Desorbing Hydrogen. J. Alloys Compd. 2015, 645, S353–S356. [Google Scholar] [CrossRef]
- Qin, F. Pulverization, Expansion of La0.6Y0.4Ni4.8Mn0.2 during Hydrogen Absorption–Desorption Cycles and Their Influences in Thin-Wall Reactors. Int. J. Hydrogen Energy 2008, 33, 709–717. [Google Scholar] [CrossRef]
- Bebon, L.; Maynadier, A.; Chapelle, D.; Thiébaud, F. Modeling Progressive Absorption of a Hydride Material Particle Submitted to Hydrogen. Int. J. Hydrogen Energy 2021, 46, 10830–10837. [Google Scholar] [CrossRef]
- Cundall, P.A.; Strack, O.D.L. A Discrete Numerical Model for Granular Assemblies. Géotechnique 1979, 29, 47–65. [Google Scholar] [CrossRef]
- Charlas, B.; Kneib, F.; Gillia, O.; Imbault, D.; Doremus, P. A Tool for Modelling the Breathing of Hydride Powder in Its Container While Cyclically Absorbing and Desorbing Hydrogen. Int. J. Hydrogen Energy 2015, 40, 2283–2294. [Google Scholar] [CrossRef]
- Harthong, B.; Jérier, J.-F.; Dorémus, P.; Imbault, D.; Donzé, F.-V. Modeling of High-Density Compaction of Granular Materials by the Discrete Element Method. Int. J. Solids Struct. 2009, 46, 3357–3364. [Google Scholar] [CrossRef]
- Ono, Y.; Otani, T.; Okumura, M.; Hamanishi, S.; Saito, Y.; Matsushita, Y.; Aoki, H. Analysis for Packing State of the Packed Bed of Hydrogen Storage Alloy Using Discrete Element Method. J. Jpn. Inst. Energy 2020, 99, 41–51. [Google Scholar] [CrossRef]
- Benyelloul, K.; Bouhadda, Y.; Bououdina, M.; Faraoun, H.I.; Aourag, H.; Seddik, L. The Effect of Hydrogen on the Mechanical Properties of FeTi for Hydrogen Storage Applications. Int. J. Hydrogen Energy 2014, 39, 12667–12675. [Google Scholar] [CrossRef]
- Dematteis, E.M.; Dreistadt, D.M.; Capurso, G.; Jepsen, J.; Cuevas, F.; Latroche, M. Fundamental Hydrogen Storage Properties of TiFe-Alloy with Partial Substitution of Fe by Ti and Mn. J. Alloys Compd. 2021, 874, 159925. [Google Scholar] [CrossRef]
- Reilly, J.J.; Wiswall, R.H. Formation and Properties of Iron Titanium Hydride. Inorg. Chem. 1974, 13, 218–222. [Google Scholar] [CrossRef]
- Sandrock, G. A Panoramic Overview of Hydrogen Storage Alloys from a Gas Reaction Point of View. J. Alloys Compd. 1999, 293–295, 877–888. [Google Scholar] [CrossRef]
- Dematteis, E.M.; Berti, N.; Cuevas, F.; Latroche, M.; Baricco, M. Substitutional Effects in TiFe for Hydrogen Storage: A Comprehensive Review. Mater. Adv. 2021, 2, 2524–2560. [Google Scholar] [CrossRef]
- Sujan, G.K.; Pan, Z.; Li, H.; Liang, D.; Alam, N. An Overview on TiFe Intermetallic for Solid-State Hydrogen Storage: Microstructure, Hydrogenation and Fabrication Processes. Crit. Rev. Solid State Mater. Sci. 2020, 45, 410–427. [Google Scholar] [CrossRef]
- Filipczynski, L.; Pawłowski, Z.; Wehr, J. Ultrasonic Methods of Testing Materials; Butterworths: London, UK, 1966. [Google Scholar]
- Julien, R. UFreckles. 2018. Available online: https://zenodo.org/records/1433776 (accessed on 10 October 2024).
- Besnard, G.; Hild, F.; Roux, S. “Finite-Element” Displacement Fields Analysis from Digital Images: Application to Portevin–Le Châtelier Bands. Exp. Mech. 2006, 46, 789–803. [Google Scholar] [CrossRef]
- Maynadier, A.; Poncelet, M.; Lavernhe-Taillard, K.; Roux, S. One-Shot Measurement of Thermal and Kinematic Fields: InfraRed Image Correlation (IRIC). Exp. Mech. 2012, 52, 241–255. [Google Scholar] [CrossRef]
- Oliver, W.C.; Pharr, G.M. Measurement of Hardness and Elastic Modulus by Instrumented Indentation: Advances in Understanding and Refinements to Methodology. J. Mater. Res. 2004, 19, 3–20. [Google Scholar] [CrossRef]
- Ulm, F.; Vandamme, M.; Bobko, C.; Alberto Ortega, J.; Tai, K.; Ortiz, C. Statistical Indentation Techniques for Hydrated Nanocomposites: Concrete, Bone, and Shale. J. Am. Ceram. Soc. 2007, 90, 2677–2692. [Google Scholar] [CrossRef]
- Tromas, C.; Stinville, J.C.; Templier, C.; Villechaise, P. Hardness and Elastic Modulus Gradients in Plasma-Nitrided 316L Polycrystalline Stainless Steel Investigated by Nanoindentation Tomography. Acta Mater. 2012, 60, 1965–1973. [Google Scholar] [CrossRef]
- Campello, D.; Tardif, N.; Desquines, J.; Baietto, M.-C.; Coret, M.; Maynadier, A.; Chaudet, P. Validation of a Multimodal Set-up for the Study of Zirconium Alloys Claddings’ Behaviour under Simulated LOCA Conditions. Strain 2018, 54, e12279. [Google Scholar] [CrossRef]
- Nong, Z.-S.; Zhu, J.-C.; Cao, Y.; Yang, X.-W.; Lai, Z.-H.; Liu, Y. A First-Principles Study on the Structural, Elastic and Electronic Properties of the C14 Laves Phase Compounds TiX2 (X = Cr, Mn, Fe). Phys. B Condens. Matter 2013, 419, 11–18. [Google Scholar] [CrossRef]
- Vlassak, J.J.; Nix, W.D. Indentation Modulus of Elastically Anisotropic Half Spaces. Philos. Mag. A 1993, 67, 1045–1056. [Google Scholar] [CrossRef]
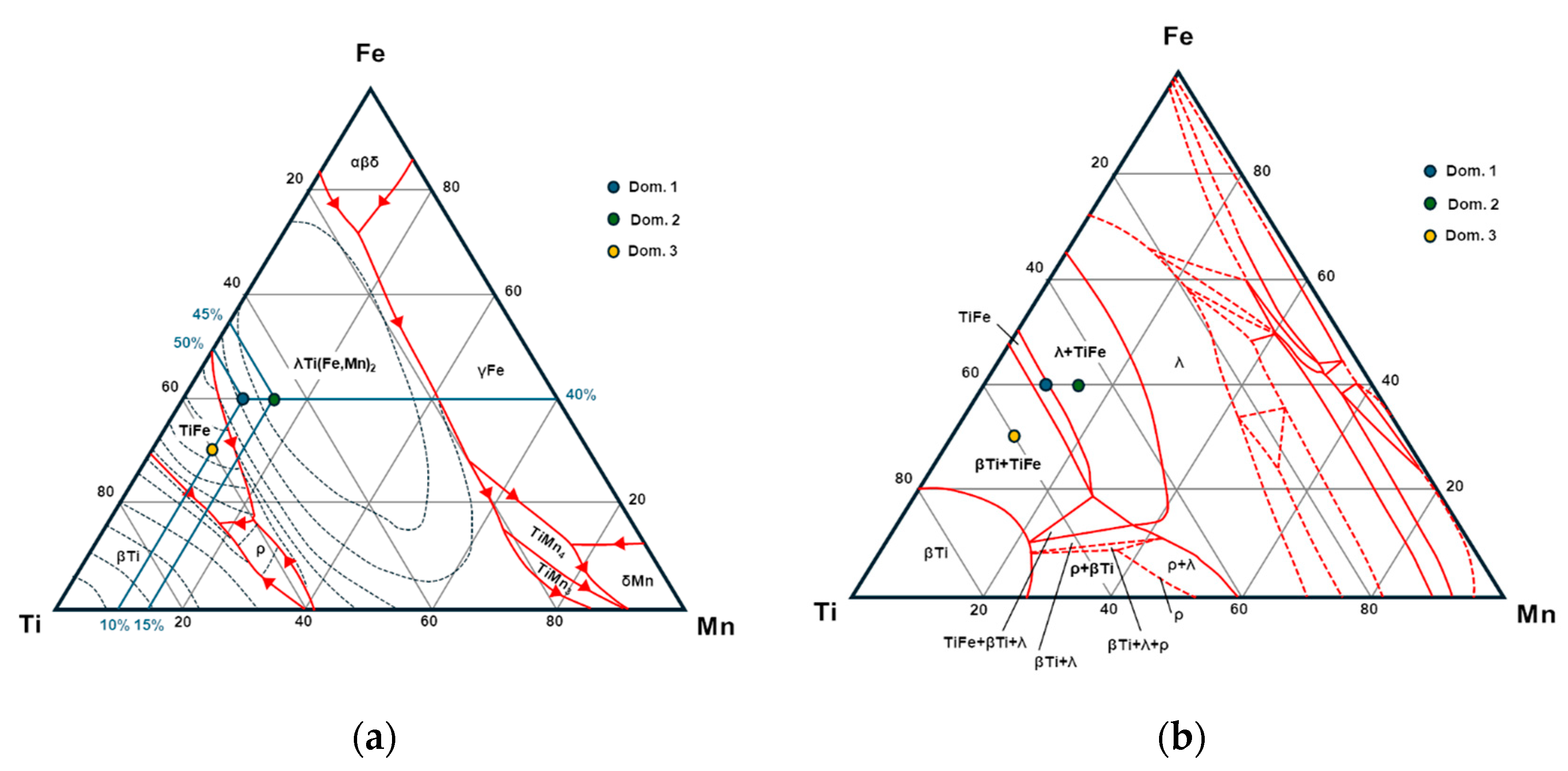
- Materials Science International Team, MSIT®; Ivanchenko, V.; Pryadko, T. Iron-Manganese-Titanium: Iron Systems: Phase Diagrams, Crystallographic and Thermodynamic Data. In Iron Systems, Part 4; Effenberg, G., Ilyenko, S., Eds.; Landolt-Börnstein-Group IV Physical Chemistry; Springer: Berlin/Heidelberg, Germany, 2008; Volume 11D4, pp. 359–373. ISBN 978-3-540-78643-6. [Google Scholar]


| First Cycle | Second Cycle | Third Cycle | |||||
|---|---|---|---|---|---|---|---|
| Sample | Unloading | Loading | Unloading | Loading | Unloading | Loading | Mean |
| 1 | 191 | 177 | 197 | 194 | 189 | ||
| 2 | 216 | 217 | 213 | 216 | 229 | 229 | 220 |
| 6 | 166 | 163 | 180 | 178 | 200 | 200 | 181 |
| Step mean E (GPa) | 191 | 186 | 197 | 196 | 215 | 215 | |
| Material mean E (GPa) | 198 | ||||||
| Domain | Indentation Elastic Modulus (GPa) | Hardness (GPa) | Corresponding Color on SEM Micrography | Proportion (%) |
|---|---|---|---|---|
| I | 265 ± 8 | 14.6 ± 0.7 | Tabby gray | 41.2 |
| II | 282 ± 16 | 9.2 ± 1.3 | Lightest gray | 31.4 |
| III | 333 ± 13 | 18.7 ± 1 | Darkest gray | 20.8 |
| IV | 384 ± 32 | 25.4 ± 1.5 | Black dots | 7.3 |
| Matrix | Dosing Point | Element (% at) | Prob. Domain | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| Ti | Mn | Fe | Cr | V | Al | O | C | N | |||
| 1 | 5 | 61 | 2 | 5 | 32 | IV | |||||
| 1 | 60 | 10 | 31 | I | |||||||
| 8 | 58 | 10 | 32 | III | |||||||
| 3 | 57 | 9 | 32 | <1 | III | ||||||
| 6 | 50 | 9 | 35 | <1 | 4 | I | |||||
| 2 | 50 | 8 | 28 | <1 | 13 | III | |||||
| 7 | 50 | 10 | 36 | 5 | I | ||||||
| 4 | 42 | 16 | 36 | <1 | 5 | II | |||||
| 2 | 4 | 85 | 4 | 10 | IV | ||||||
| 3 | 71 | 3 | 7 | 19 | IV | ||||||
| 7 | 69 | 3 | 10 | 18 | IV | ||||||
| 5 | 67 | 8 | 25 | III | |||||||
| 1 | 51 | 12 | 38 | I | |||||||
| 2 | 50 | 8 | 38 | 4 | I | ||||||
| 6 | 43 | 15 | 36 | <1 | 5 | II | |||||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bebon, L.; Maynadier, A.; Gaillard, Y.; Chapelle, D. Multiscale Elastic Modulus Characterization of Ti0.5Fe0.45Mn0.05, an Iron–Titanium–Manganese Alloy Dedicated to Hydrogen Storage. Materials 2024, 17, 6100. https://doi.org/10.3390/ma17246100
Bebon L, Maynadier A, Gaillard Y, Chapelle D. Multiscale Elastic Modulus Characterization of Ti0.5Fe0.45Mn0.05, an Iron–Titanium–Manganese Alloy Dedicated to Hydrogen Storage. Materials. 2024; 17(24):6100. https://doi.org/10.3390/ma17246100
Chicago/Turabian StyleBebon, Ludovic, Anne Maynadier, Yves Gaillard, and David Chapelle. 2024. "Multiscale Elastic Modulus Characterization of Ti0.5Fe0.45Mn0.05, an Iron–Titanium–Manganese Alloy Dedicated to Hydrogen Storage" Materials 17, no. 24: 6100. https://doi.org/10.3390/ma17246100
APA StyleBebon, L., Maynadier, A., Gaillard, Y., & Chapelle, D. (2024). Multiscale Elastic Modulus Characterization of Ti0.5Fe0.45Mn0.05, an Iron–Titanium–Manganese Alloy Dedicated to Hydrogen Storage. Materials, 17(24), 6100. https://doi.org/10.3390/ma17246100







