Enhanced Diffusion and Non-Gaussian Displacements of Colloids in Quasi-2D Suspensions of Motile Bacteria
Abstract
:1. Introduction
2. Materials and Methods
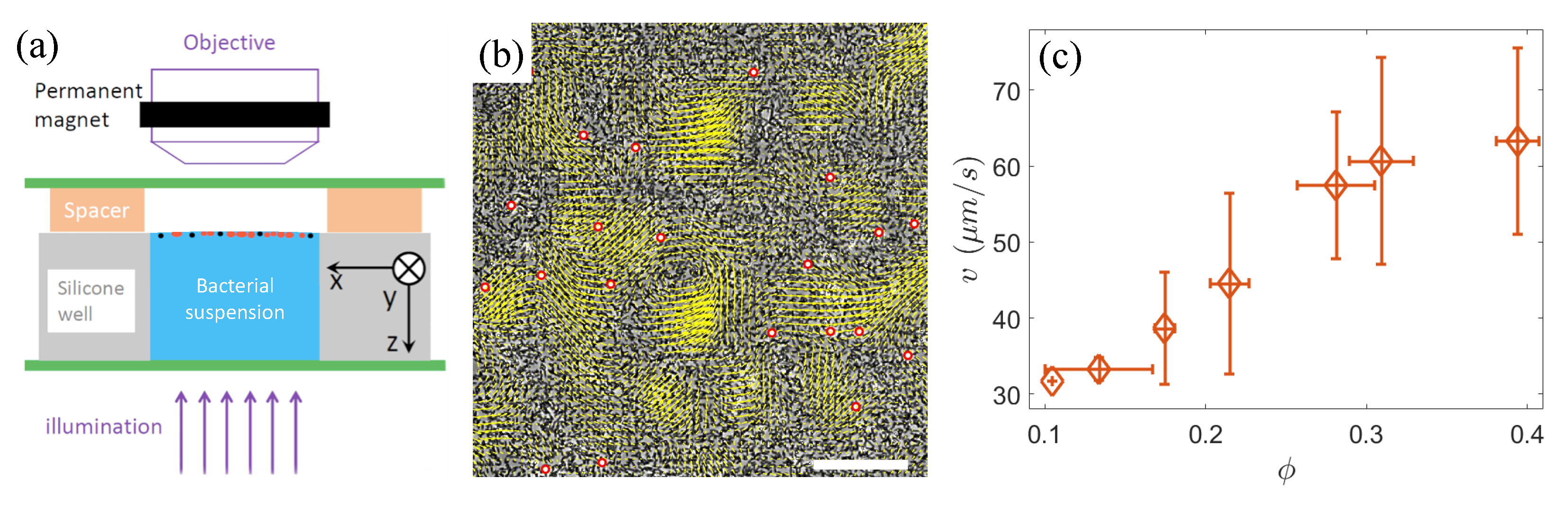
2.1. Experimental Setup
2.2. Image Analysis
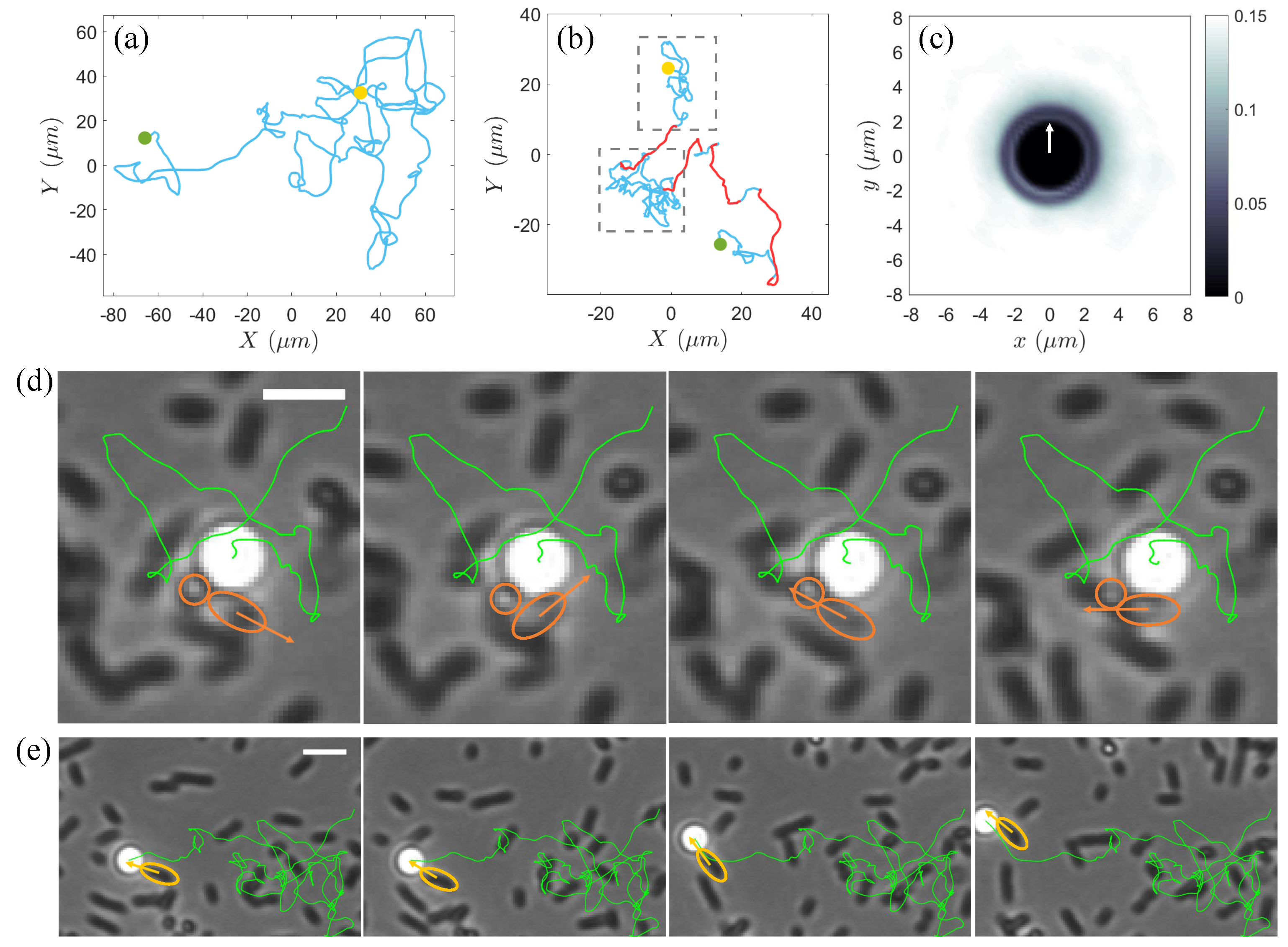
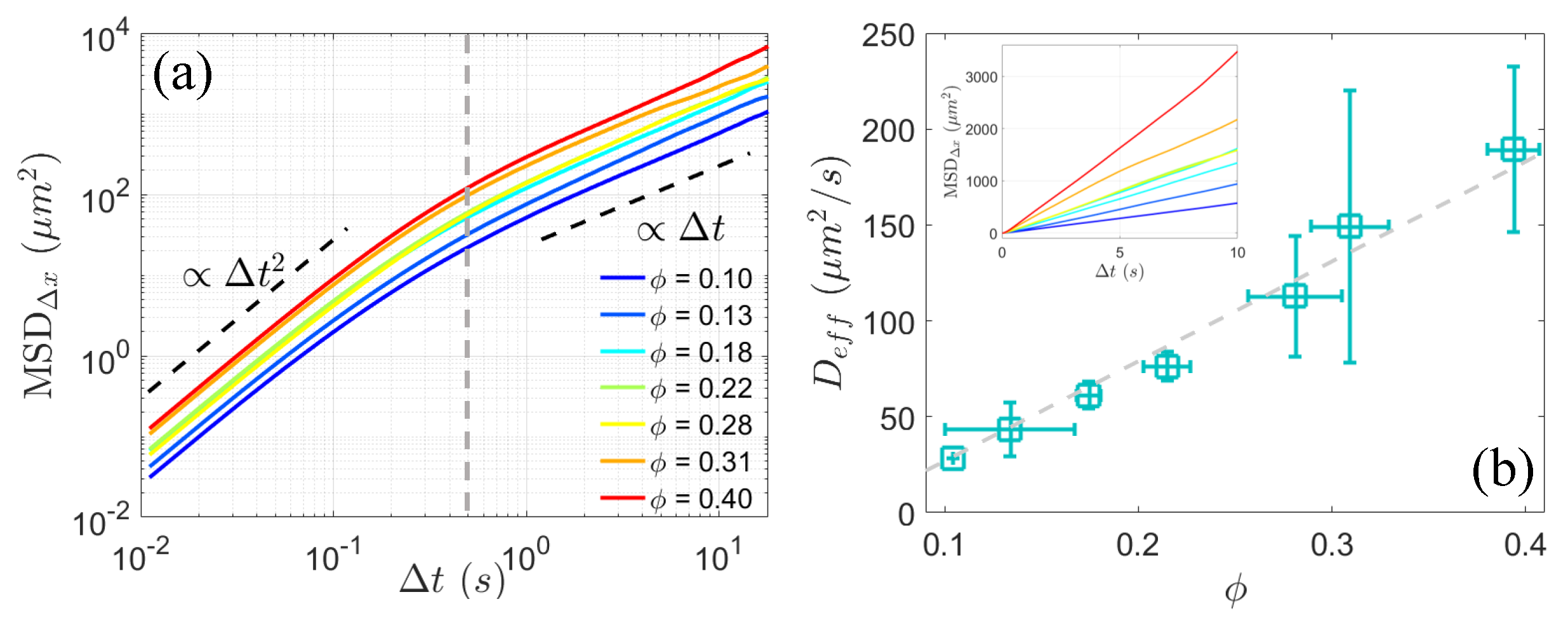
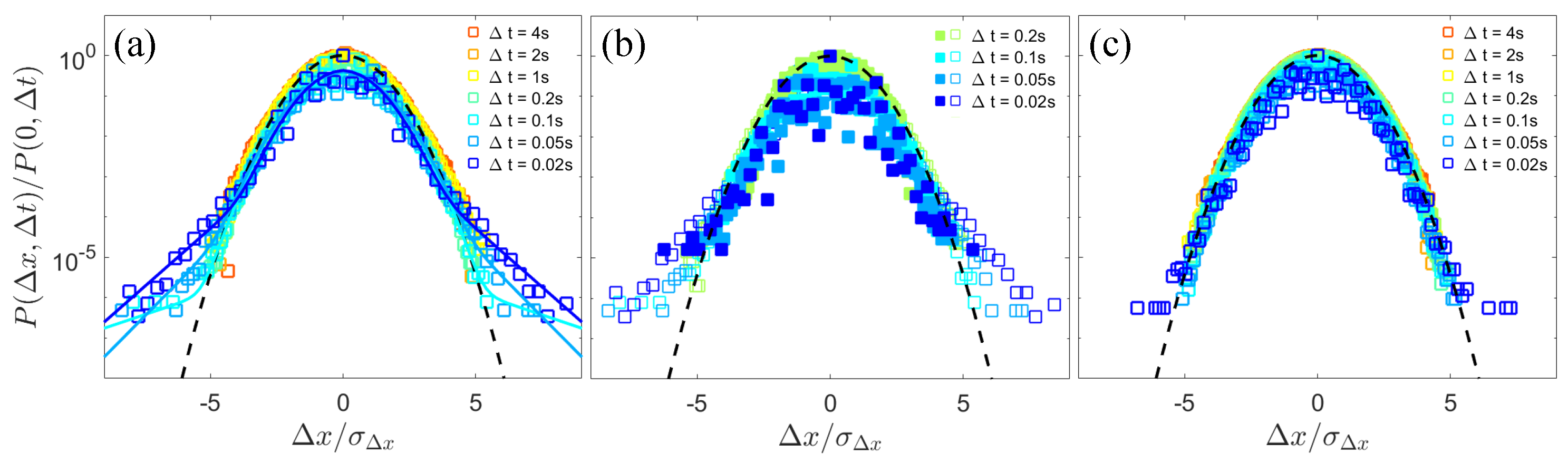
3. Results
4. Discussion and Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Martínez-Prat, B.; Alert, R.; Meng, F.; Ignés-Mullol, J.; Joanny, J.F.m.c.; Casademunt, J.; Golestanian, R.; Sagués, F. Scaling Regimes of Active Turbulence with External Dissipation. Phys. Rev. X 2021, 11, 031065. [Google Scholar] [CrossRef]
- Sokolov, A.; Aranson, I.S.; Kessler, J.O.; Goldstein, R.E. Concentration dependence of the collective dynamics of swimming bacteria. Phys. Rev. Lett. 2007, 98, 158102. [Google Scholar] [CrossRef]
- Zhang, H.P.; Be’er, A.; Florin, E.L.; Swinney, H.L. Collective motion and density fluctuations in bacterial colonies. Proc. Natl. Acad. Sci. USA 2010, 107, 13626–13630. [Google Scholar] [CrossRef]
- Goldstein, R.E. Green Algae as Model Organisms for Biological Fluid Dynamics. Annu. Rev. Fluid Mech. 2015, 47, 343–375. [Google Scholar] [CrossRef]
- Creppy, A.; Praud, O.; Druart, X.; Kohnke, P.L.; Plouraboué, F. Turbulence of swarming sperm. Phys. Rev. E 2015, 92, 032722. [Google Scholar] [CrossRef]
- Makris, N.C.; Ratilal, P.; Symonds, D.T.; Jagannathan, S.; Lee, S.; Nero, R.W. Fish population and behavior revealed by instantaneous continental shelf-scale imaging. Science 2006, 311, 660–663. [Google Scholar] [CrossRef]
- Cavagna, A.; Cimarelli, A.; Giardina, I.; Parisi, G.; Santagati, R.; Stefanini, F.; Viale, M. Scale-free correlations in starling flocks. Proc. Natl. Acad. Sci. USA 2010, 107, 11865–11870. [Google Scholar] [CrossRef]
- Sokolov, A.; Apodaca, M.M.; Grzybowski, B.A.; Aranson, I.S. Swimming bacteria power microscopic gears. Proc. Natl. Acad. Sci. USA 2010, 107, 969–974. [Google Scholar] [CrossRef]
- Chen, D.T.N.; Lau, A.W.C.; Hough, L.A.; Islam, M.F.; Goulian, M.; Lubensky, T.C.; Yodh, A.G. Fluctuations and Rheology in Active Bacterial Suspensions. Phys. Rev. Lett. 2007, 99, 148302. [Google Scholar] [CrossRef]
- Leptos, K.C.; Guasto, J.S.; Gollub, J.P.; Pesci, A.I.; Goldstein, R.E. Dynamics of Enhanced Tracer Diffusion in Suspensions of Swimming Eukaryotic Microorganisms. Phys. Rev. Lett. 2009, 103, 198103. [Google Scholar] [CrossRef]
- Mino, G.; Mallouk, T.E.; Darnige, T.; Hoyos, M.; Dauchet, J.; Dunstan, J.; Soto, R.; Wang, Y.; Rousselet, A.; Clement, E. Enhanced Diffusion due to Active Swimmers at a Solid Surface. Phys. Rev. Lett. 2011, 106, 048102. [Google Scholar] [CrossRef] [PubMed]
- Miño, G.L.; Dunstan, J.; Rousselet, A.; Clément, E.; Soto, R. Induced diffusion of tracers in a bacterial suspension: Theory and experiments. J. Fluid Mech. 2013, 729, 423–444. [Google Scholar] [CrossRef]
- Jepson, A.; Martinez, V.A.; Schwarz-Linek, J.; Morozov, A.; Poon, W.C.K. Enhanced diffusion of nonswimmers in a three-dimensional bath of motile bacteria. Phys. Rev. E 2013, 88, 041002. [Google Scholar] [CrossRef]
- Kurihara, T.; Aridome, M.; Ayade, H.; Zaid, I.; Mizuno, D. Non-Gaussian limit fluctuations in active swimmer suspensions. Phys. Rev. E 2017, 95, 030601. [Google Scholar] [CrossRef]
- Guzmán-Lastra, F.; Löwen, H.; Mathijssen, A.J.T.M. Active carpets drive non-equilibrium diffusion and enhanced molecular fluxes. Nat. Commun. 2021, 12, 1906. [Google Scholar] [CrossRef]
- Wu, X.L.; Libchaber, A. Particle diffusion in a quasi-two-dimensional bacterial bath. Phys. Rev. Lett. 2000, 84, 3017–3020. [Google Scholar] [CrossRef]
- Lagarde, A.; Dagès, N.; Nemoto, T.; Démery, V.; Bartolo, D.; Gibaud, T. Colloidal transport in bacteria suspensions: From bacteria collision to anomalous and enhanced diffusion. Soft Matter 2020, 16, 7503–7512. [Google Scholar] [CrossRef]
- Jeanneret, R.; Pushkin, D.O.; Kantsler, V.; Polin, M. Entrainment dominates the interaction of microalgae with micron-sized objects. Nat. Commun. 2016, 7, 12518. [Google Scholar] [CrossRef]
- Kurtuldu, H.; Guasto, J.S.; Johnson, K.A.; Gollub, J.P. Enhancement of biomixing by swimming algal cells in two-dimensional films. Proc. Natl. Acad. Sci. USA 2011, 108, 10391–10395. [Google Scholar] [CrossRef]
- Großmann, R.; Bort, L.S.; Moldenhawer, T.; Stange, M.; Panah, S.S.; Metzler, R.; Beta, C. Non-Gaussian Displacements in Active Transport on a Carpet of Motile Cells. Phys. Rev. Lett. 2024, 132, 088301. [Google Scholar] [CrossRef]
- Ortlieb, L.; Rafaï, S.; Peyla, P.; Wagner, C.; John, T. Statistics of Colloidal Suspensions Stirred by Microswimmers. Phys. Rev. Lett. 2019, 122, 148101. [Google Scholar] [CrossRef] [PubMed]
- Dhar, T.; Saintillan, D. Active transport of a passive colloid in a bath of run-and-tumble particles. Sci. Rep. 2024, 14, 11844. [Google Scholar] [CrossRef]
- Mathijssen, A.J.T.M.; Pushkin, D.O.; Yeomans, J.M. Tracer trajectories and displacement due to a micro-swimmer near a surface. J. Fluid Mech. 2015, 773, 498–519. [Google Scholar] [CrossRef]
- Mathijssen, A.J.T.M.; Guzmán-Lastra, F.; Kaiser, A.; Löwen, H. Nutrient Transport Driven by Microbial Active Carpets. Phys. Rev. Lett. 2018, 121, 248101. [Google Scholar] [CrossRef] [PubMed]
- Harris, L.S.T.; Fennell, J.; Fales, R.J.; Carrington, E. Spatial–Temporal Growth, Distribution, and Diffusion of Marine Microplastic Research and National Plastic Policies. Water Air Soil Pollut. 2021, 232, 400. [Google Scholar] [CrossRef]
- Patra, D.; Sengupta, S.; Duan, W.; Zhang, H.; Pavlick, R.; Sen, A. Intelligent, self-powered, drug delivery systems. Nanoscale 2013, 5, 1273–1283. [Google Scholar] [CrossRef]
- Bechinger, C.; Di Leonardo, R.; Löwen, H.; Reichhardt, C.; Volpe, G.; Volpe, G. Active particles in complex and crowded environments. Rev. Mod. Phys. 2016, 88, 045006. [Google Scholar] [CrossRef]
- Syzdek, L.D. Influence of Serratia marcescens Pigmentation on Cell Concentrations in Aerosols Produced by Bursting Bubbles. Appl. Environ. Microbiol. 1985, 49, 173–178. [Google Scholar] [CrossRef]
- Hejazi, A.; Falkiner, F.R. Serratia marcescens. J. Med. Microbiol. 1997, 46, 903–912. [Google Scholar] [CrossRef]
- Chen, X.; Dong, X.; Be’er, A.; Swinney, H.L.; Zhang, H.P. Scale-invariant correlations in dynamic bacterial clusters. Phys. Rev. Lett. 2012, 108, 148101. [Google Scholar] [CrossRef]
- Crocker, J.C.; Grier, D.G. Methods of Digital Video Microscopy for Colloidal Studies. J. Colloid Interface Sci. 1996, 179, 298–310. [Google Scholar] [CrossRef]
- Chen, X.; Yang, X.; Yang, M.; Zhang, H.P. Dynamic clustering in suspension of motile bacteria. EPL (Europhys. Lett.) 2015, 111, 54002. [Google Scholar] [CrossRef]
- Guasto, J.S.; Johnson, K.A.; Gollub, J.P. Oscillatory Flows Induced by Microorganisms Swimming in Two Dimensions. Phys. Rev. Lett. 2010, 105, 168102. [Google Scholar] [CrossRef] [PubMed]
- Drescher, K.; Goldstein, R.E.; Michel, N.; Polin, M.; Tuval, I. Direct Measurement of the Flow Field around Swimming Microorganisms. Phys. Rev. Lett. 2010, 105, 168101. [Google Scholar] [CrossRef]
- Kanazawa, K.; Sano, T.G.; Cairoli, A.; Baule, A. Loopy Lévy flights enhance tracer diffusion in active suspensions. Nature 2020, 579, 364–367. [Google Scholar] [CrossRef]
- Valeriani, C.; Li, M.; Novosel, J.; Arlt, J.; Marenduzzo, D. Colloids in a bacterial bath: Simulations and experiments. Soft Matter 2011, 7, 5228–5238. [Google Scholar] [CrossRef]
- Maggi, C.; Paoluzzi, M.; Angelani, L.; Di Leonardo, R. Memory-less response and violation of the fluctuation-dissipation theorem in colloids suspended in an active bath. Sci. Rep. 2017, 7, 17588. [Google Scholar] [CrossRef]
- Zhu, Z.; Liu, Q.X. Enhanced transport of nutrients powered by microscale flows of the self-spinning dinoflagellate Symbiodinium sp. J. Exp. Biol. 2019, 222, jeb197947. [Google Scholar] [CrossRef]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, X.; Yan, Y. Enhanced Diffusion and Non-Gaussian Displacements of Colloids in Quasi-2D Suspensions of Motile Bacteria. Materials 2024, 17, 5013. https://doi.org/10.3390/ma17205013
Chen X, Yan Y. Enhanced Diffusion and Non-Gaussian Displacements of Colloids in Quasi-2D Suspensions of Motile Bacteria. Materials. 2024; 17(20):5013. https://doi.org/10.3390/ma17205013
Chicago/Turabian StyleChen, Xiao, and Yaner Yan. 2024. "Enhanced Diffusion and Non-Gaussian Displacements of Colloids in Quasi-2D Suspensions of Motile Bacteria" Materials 17, no. 20: 5013. https://doi.org/10.3390/ma17205013
APA StyleChen, X., & Yan, Y. (2024). Enhanced Diffusion and Non-Gaussian Displacements of Colloids in Quasi-2D Suspensions of Motile Bacteria. Materials, 17(20), 5013. https://doi.org/10.3390/ma17205013




