Fatigue Life Prediction for Injection-Molded Carbon Fiber-Reinforced Polyamide-6 Considering Anisotropy and Temperature Effects
Abstract
1. Introduction
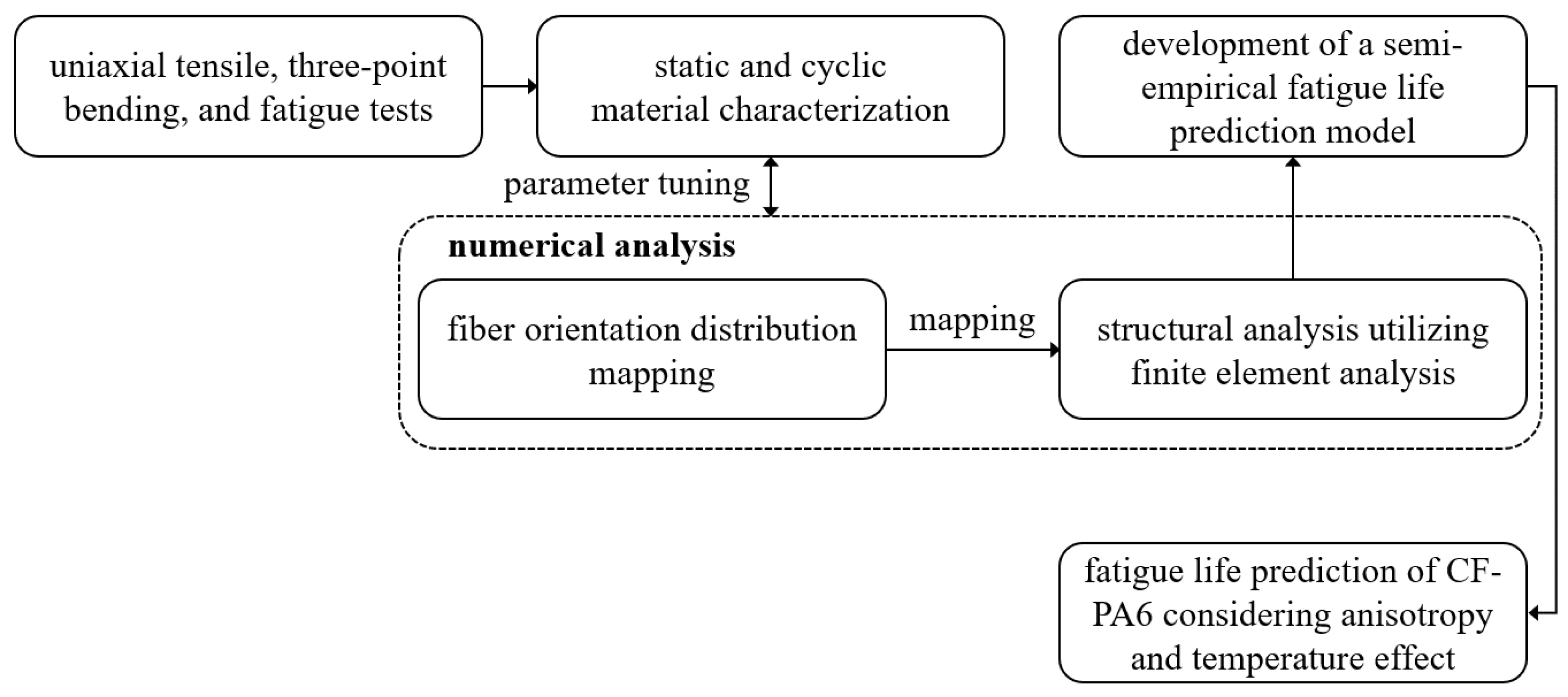
2. Fatigue Life Prediction Procedure
3. Material Characterization
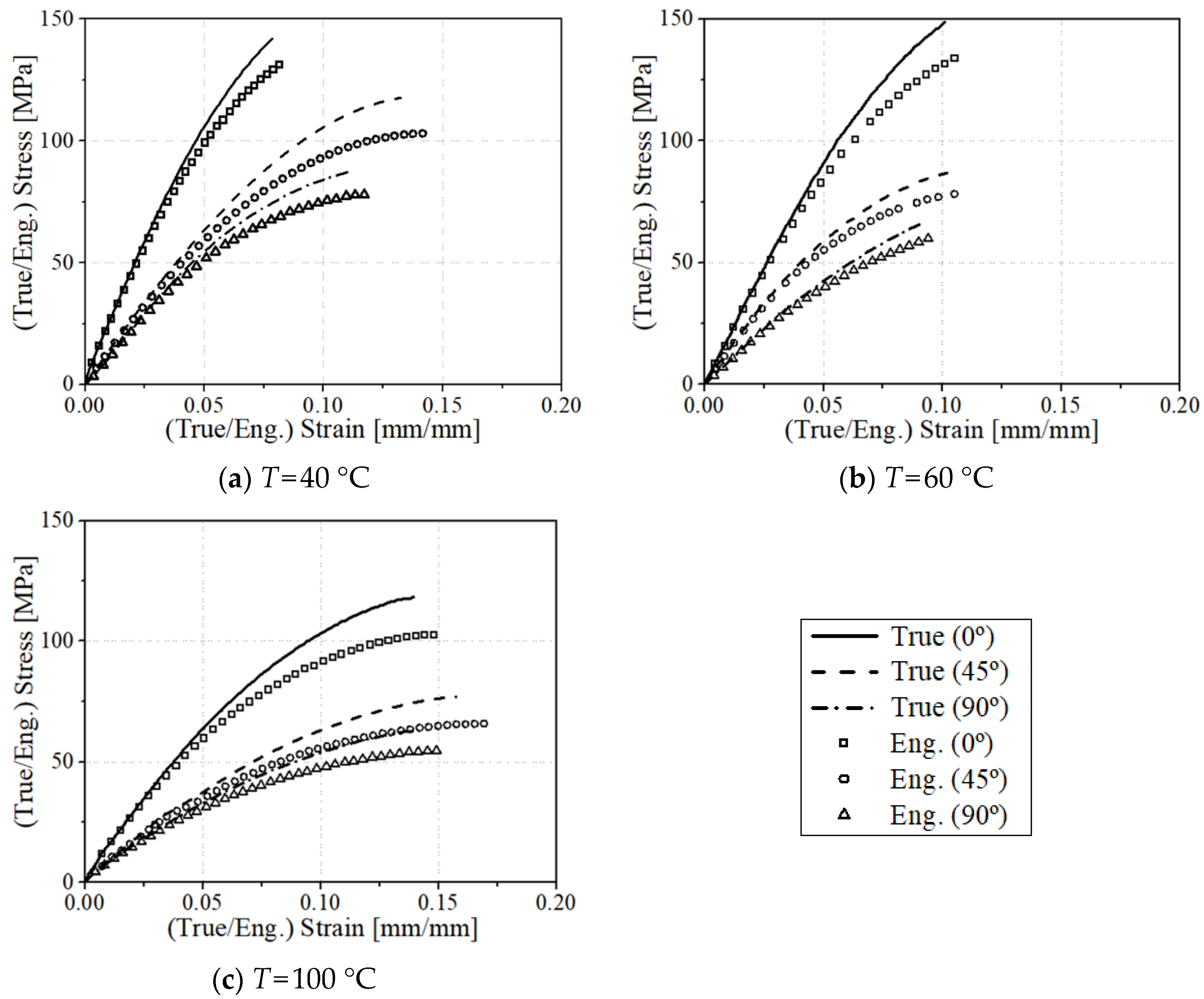
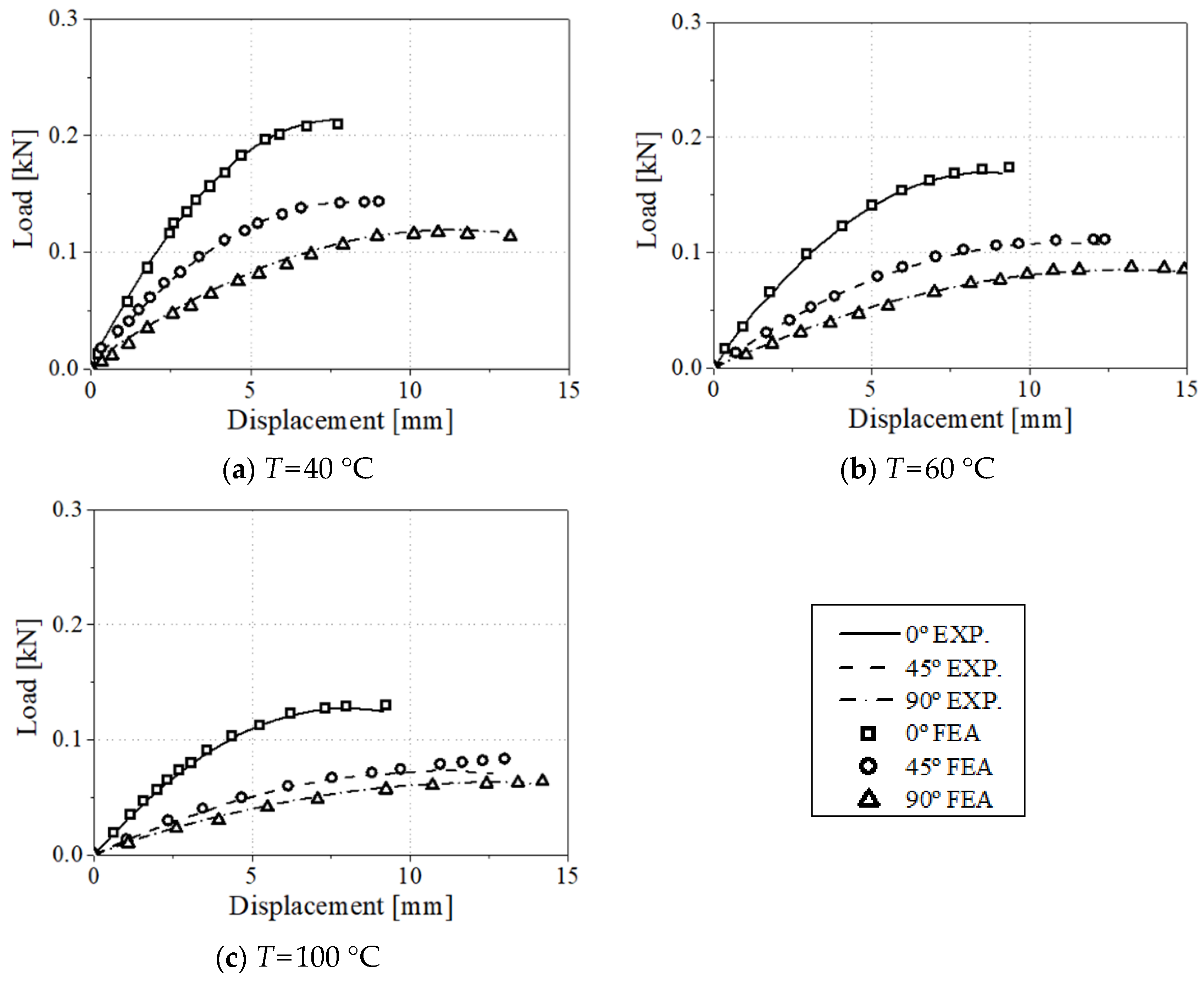
3.1. Static Mechanical Properties Characterization
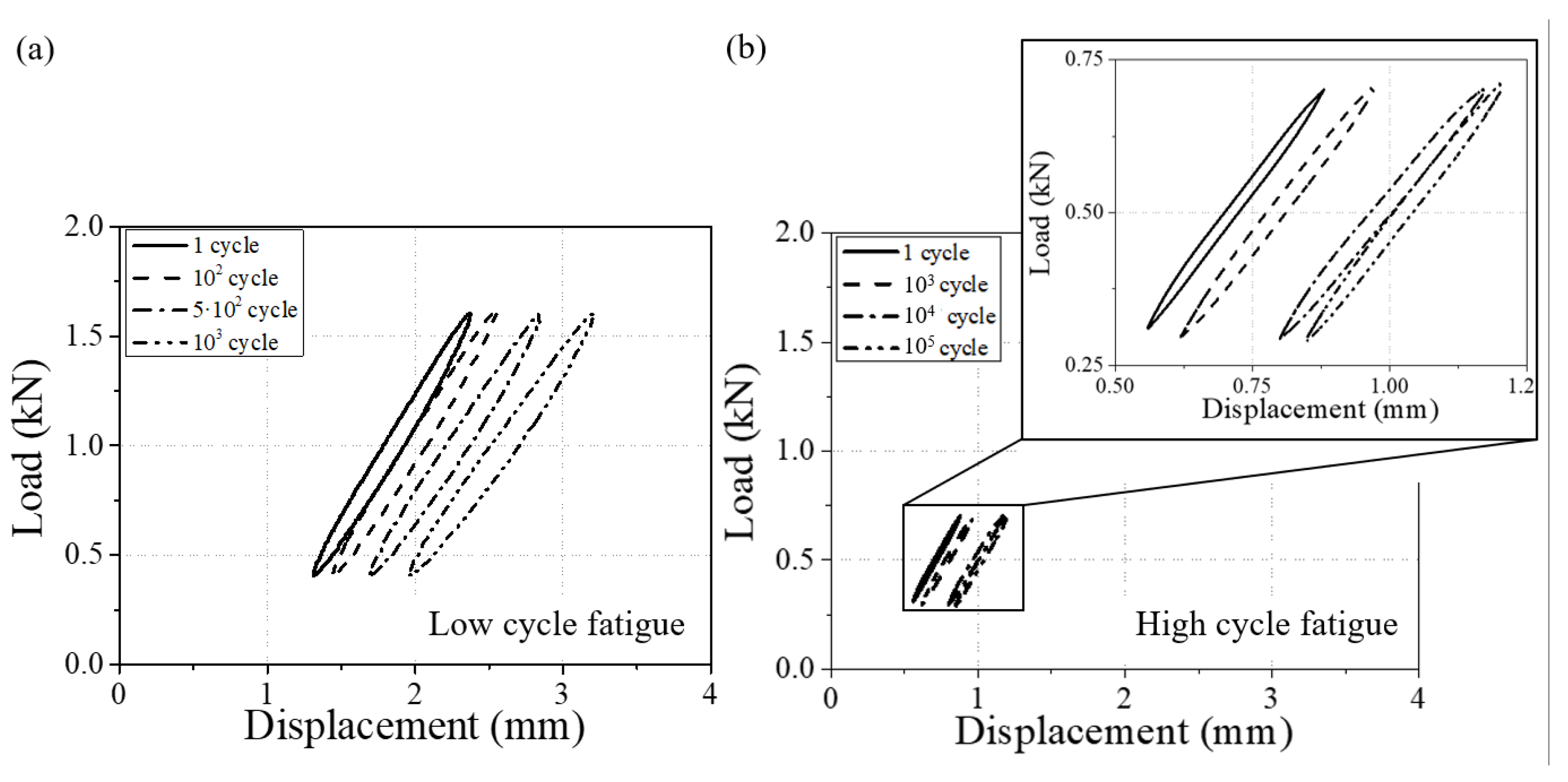
3.2. Cyclic Mechanical Properties Characterization
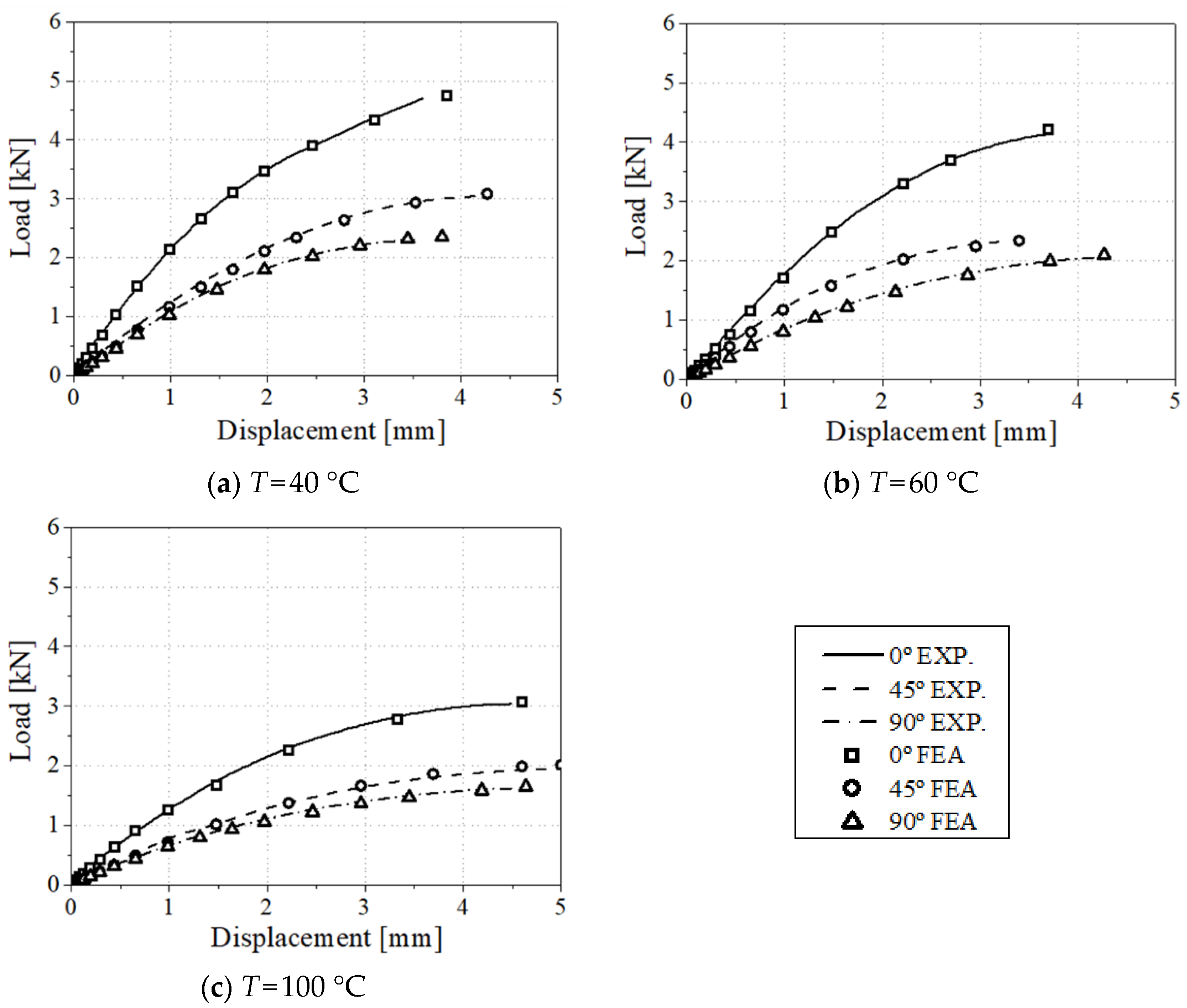
3.3. Numerical Analysis
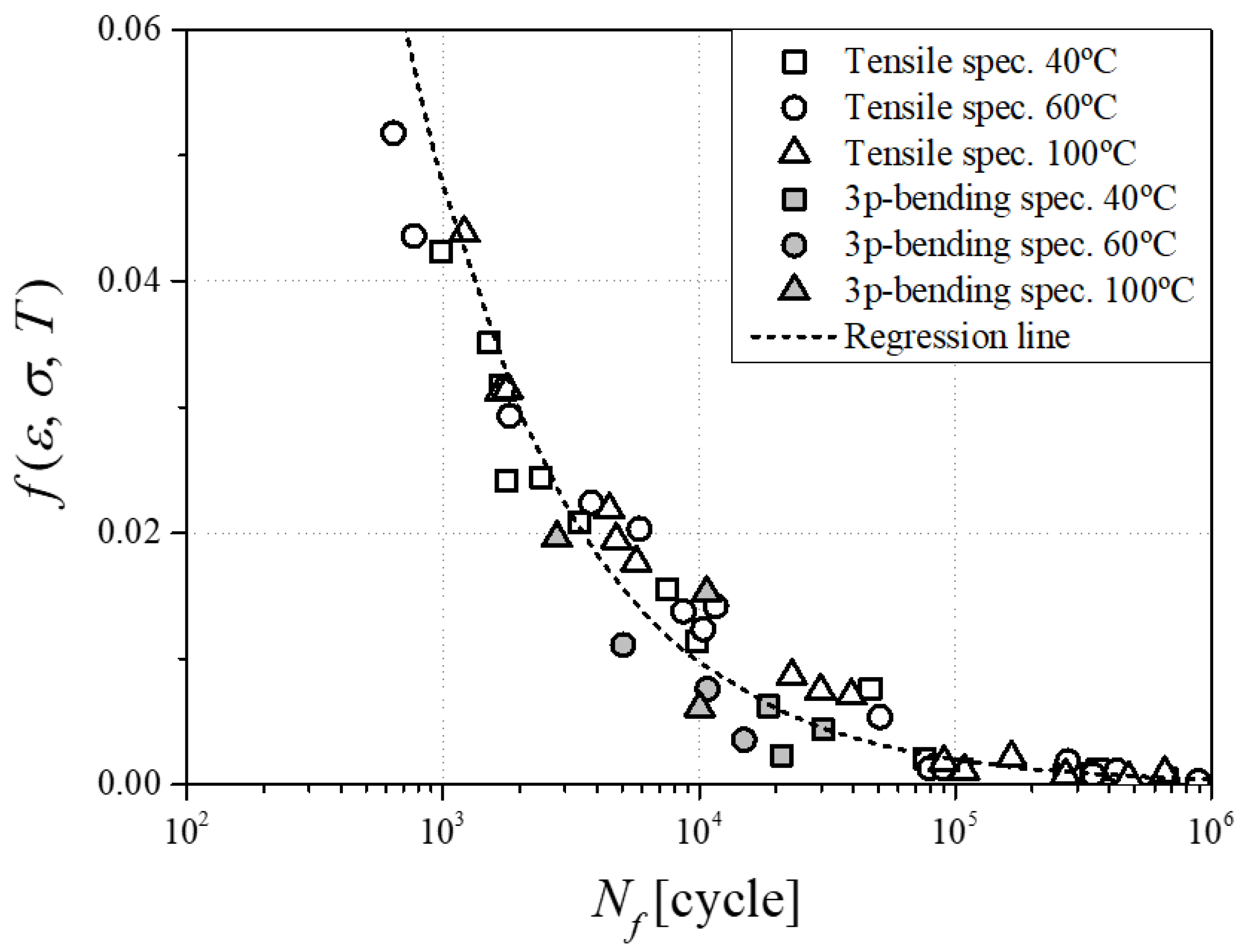
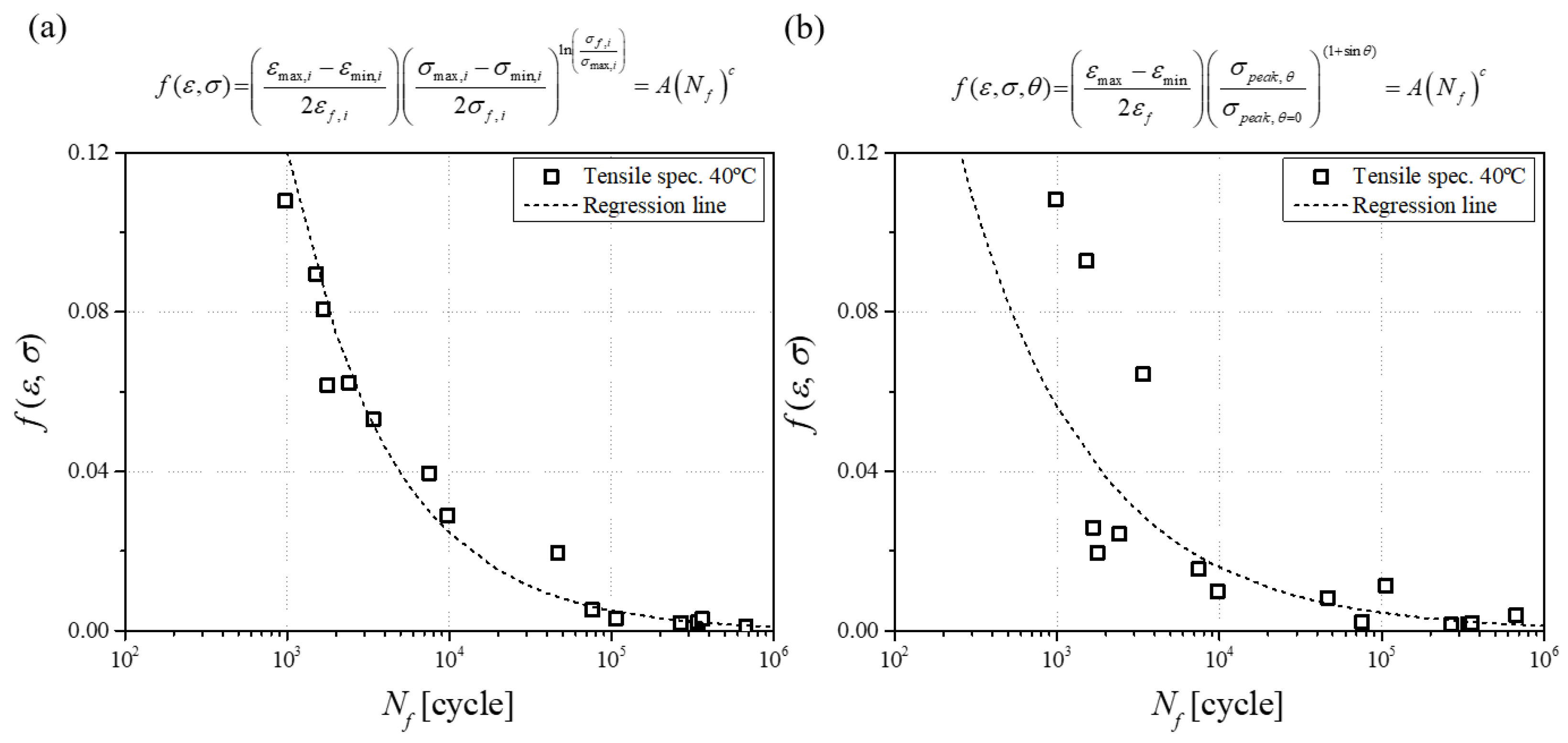
4. Fatigue Life Prediction Model
5. Results
6. Conclusions
- This study predicted the fatigue life expectancy of CF-PA6, a plastic reinforced with short fiber, through a strain-based semi-empirical model with a high correlation factor. A three-point bending test was performed to investigate various multi-axial stress states in actual components.
- A meaningful, intuitive fatigue life prediction model is proposed considering anisotropy as a stress term, which directly utilizes experimental results with a theoretical approach. It can be concluded that the fatigue life of materials with high temperature and anisotropy fiber orientation and polymers can be predicted with reasonable accuracy.
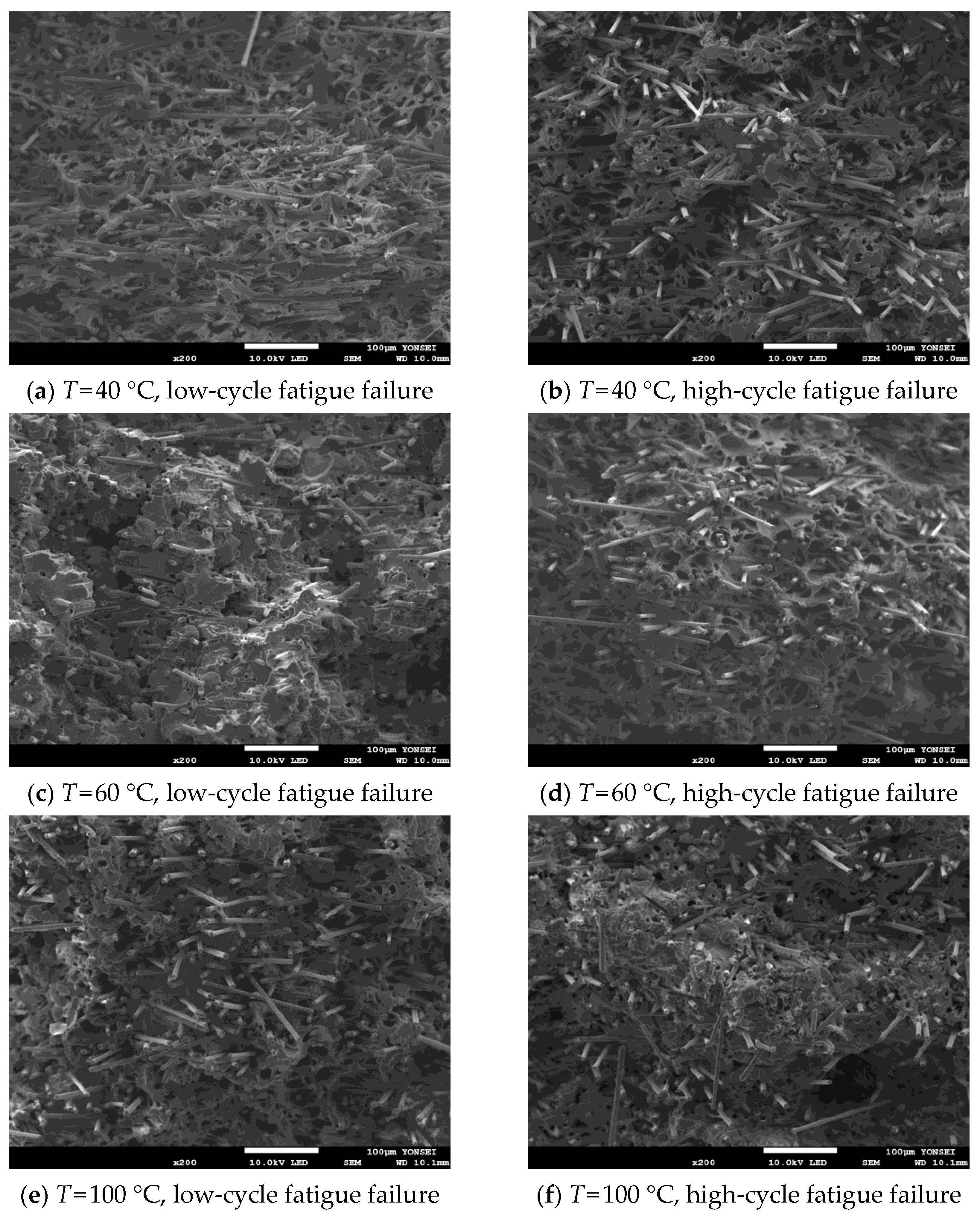
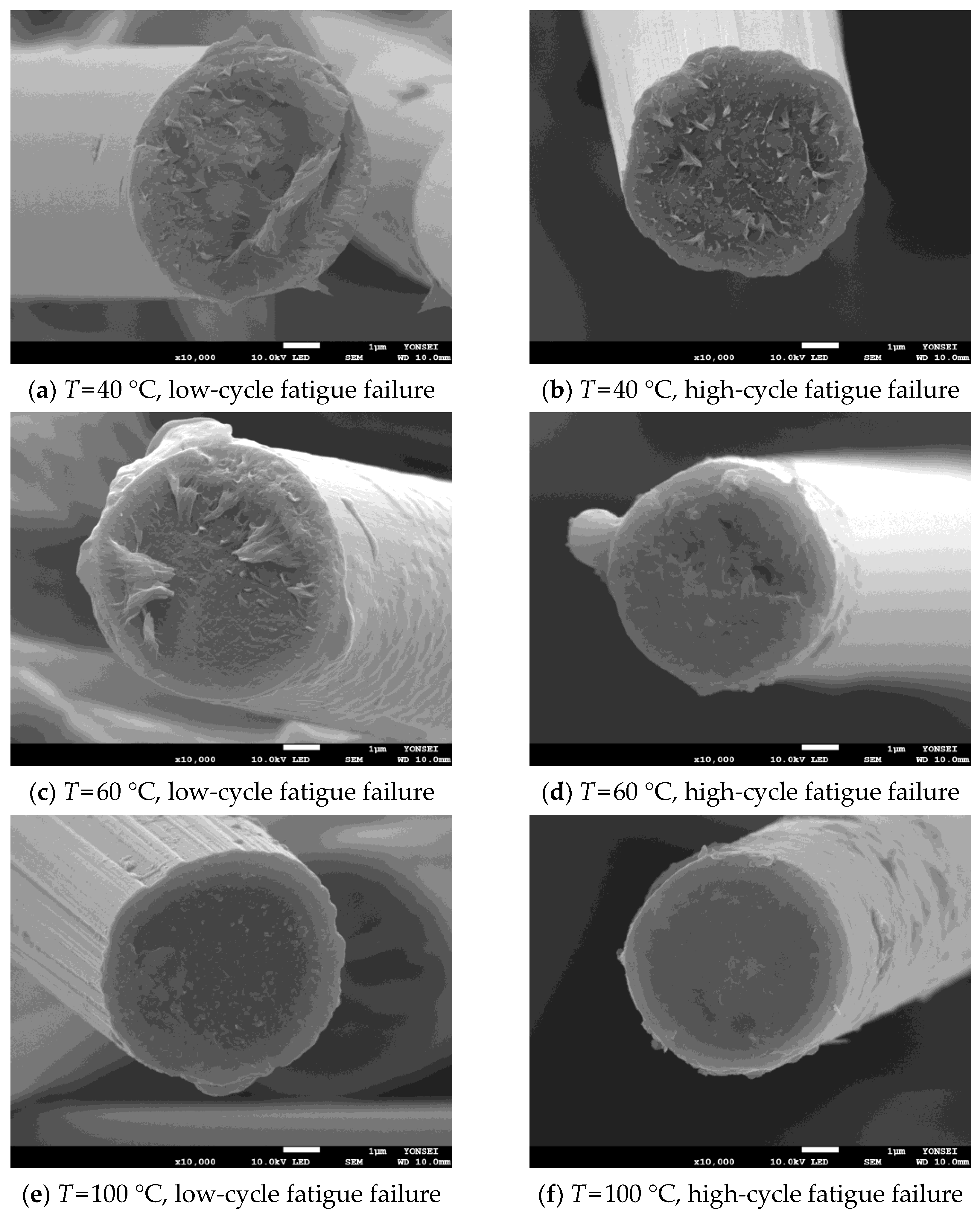
- SEM photography revealed that the higher the temperature and fatigue fracture cycle, the greater the deformation of the polymer matrix, and inversely, the more the deformation of the fiber. The higher the temperature, the more evenly the fiber’s fracture cross-section is.
- The developed numerical model and structural equation are highly consistent between experiments and FEA results. Furthermore, they could accurately export stress and strain as inputs to a semi-empirical model.
- The usefulness of the results proposed in this paper can be outlined in two parts. First, the paper summarizes the static and fatigue behavior considering the anisotropy and temperature of short fiber-reinforced plastic materials, which are increasingly utilized exponentially in the industry. Secondly, it provides insight into the availability of the developed semi-empirical model to predict the fatigue life of CF-PA6.
- The use of FRP affected by temperature and fiber orientation is a remaining challenge for research on much colder temperatures and compressive forces below 0 °C. In addition, using compressive force in testing and investigating the mechanical properties of FRP can accurately describe the complex stress states in industries. Therefore, it can be a better solution to predict the fatigue life and composite use of FRP considering low temperature and compression stress states in the future.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Offringa, A.R. Thermoplastic Composites—Rapid Processing Applications. Compos. Part A Appl. Sci. Manuf. 1996, 27, 329–336. [Google Scholar] [CrossRef]
- Pini, T.; Caimmi, F.; Briatico-Vangosa, F.; Frassine, R.; Rink, M. Fracture Initiation and Propagation in Unidirectional CF Composites Based on Thermoplastic Acrylic Resins. Eng. Fract. Mech. 2017, 184, 51–58. [Google Scholar] [CrossRef][Green Version]
- Li, L.; Xiao, N.; Guo, C.; Wang, F. A Study on Processing Defects and Parameter Optimization in Abrasive Suspension Jet Cutting of Carbon-Fiber-Reinforced Plastics. Materials 2023, 16, 7064. [Google Scholar] [CrossRef] [PubMed]
- Yardim, Y.; Yilmaz, S.; Corradi, M.; Thanoon, W.A. Strengthening of Reinforced Concrete Non-Circular Columns with FRP. Materials 2023, 16, 6973. [Google Scholar] [CrossRef] [PubMed]
- Tan, X.; Zhu, M.; Liu, W. Experimental Study and Numerical Analysis of the Seismic Performance of Glass-Fiber Reinforced Plastic Tube Ultra-High Performance Concrete Composite Columns. Materials 2023, 16, 6941. [Google Scholar] [CrossRef] [PubMed]
- Pan, X.; Tian, A.; Zhang, L.; Zheng, Z. A Study on the Performance of Prestressed Concrete Containment with Carbon Fiber-Reinforced Polymer Tendons under Internal Pressure. Materials 2023, 16, 6883. [Google Scholar] [CrossRef] [PubMed]
- Pastukhov, L.V.; Govaert, L.E. Crack-Growth Controlled Failure of Short Fibre Reinforced Thermoplastics: Influence of Fibre Orientation. Int. J. Fatigue 2021, 143, 105982. [Google Scholar] [CrossRef]
- Lazzarin, P.; Molina, G.; Molinari, L.; Quaresimin, M. Numerical Simulation of SMC Component Moulding. Key Eng. Mater. 1998, 144, 191–200. [Google Scholar] [CrossRef]
- Li, Y.; Li, W.; Shao, J.; Deng, Y.; Kou, H.; Ma, J.; Zhang, X.; Zhang, X.; Chen, L.; Qu, Z. Modeling the Effects of Interfacial Properties on the Temperature Dependent Tensile Strength of Fiber Reinforced Polymer Composites. Compos. Sci. Technol. 2019, 172, 74–80. [Google Scholar] [CrossRef]
- Fu, S.-Y.; Lauke, B. Effects of Fiber Length and Fiber Orientation Distributions on the Tensile Strength of Short-Fiber-Reinforced Polymers. Compos. Sci. Technol. 1996, 56, 1179–1190. [Google Scholar] [CrossRef]
- Launay, A.; Maitournam, M.H.; Marco, Y.; Raoult, I.; Szmytka, F. Cyclic Behaviour of Short Glass Fibre Reinforced Polyamide: Experimental Study and Constitutive Equations. Int. J. Plast. 2011, 27, 1267–1293. [Google Scholar] [CrossRef]
- Arif, M.F.; Saintier, N.; Meraghni, F.; Fitoussi, J.; Chemisky, Y.; Robert, G. Multiscale Fatigue Damage Characterization in Short Glass Fiber Reinforced Polyamide-66. Compos. Part B Eng. 2014, 61, 55–65. [Google Scholar] [CrossRef]
- Brighenti, R.; Carpinteri, A.; Scorza, D. Micromechanical Model for Preferentially-Oriented Short-Fibre-Reinforced Materials under Cyclic Loading. Eng. Fract. Mech. 2016, 167, 138–150. [Google Scholar] [CrossRef]
- Judt, P.O.; Zarges, J.C.; Ricoeur, A.; Heim, H.P. Anisotropic Fracture Properties and Crack Path Prediction in Glass and Cellulose Fiber Reinforced Composites. Eng. Fract. Mech. 2018, 188, 344–360. [Google Scholar] [CrossRef]
- Tanaka, K.; Kitano, T.; Egami, N. Effect of Fiber Orientation on Fatigue Crack Propagation in Short-Fiber Reinforced Plastics. Eng. Fract. Mech. 2014, 123, 44–58. [Google Scholar] [CrossRef]
- Tanaka, K.; Oharada, K.; Yamada, D.; Shimizu, K. Fatigue Crack Propagation in Short-Carbon-Fiber Reinforced Plastics Evaluated Based on Anisotropic Fracture Mechanics. Int. J. Fatigue 2016, 92, 415–425. [Google Scholar] [CrossRef]
- Russo, A.; Sellitto, A.; Curatolo, P.; Acanfora, V.; Saputo, S.; Riccio, A. A Robust Numerical Methodology for Fatigue Damage Evolution Simulation in Composites. Materials 2021, 14, 3348. [Google Scholar] [CrossRef]
- Qureshi, H.J.; Saleem, M.U.; Khurram, N.; Ahmad, J.; Amin, M.N.; Khan, K.; Aslam, F.; Al Fuhaid, A.F.; Arifuzzaman, M. Investigation of CFRP Reinforcement Ratio on the Flexural Capacity and Failure Mode of Plain Concrete Prisms. Materials 2022, 15, 7248. [Google Scholar] [CrossRef]
- Li, Y.; Deng, H.; Takamura, M.; Koyanagi, J. Durability Analysis of CFRP Adhesive Joints: A Study Based on Entropy Damage Modeling Using FEM. Materials 2023, 16, 6821. [Google Scholar] [CrossRef]
- Aklilu, G.; Adali, S.; Bright, G. Tensile Behaviour of Hybrid and Non-Hybrid Polymer Composite Specimens at Elevated Temperatures. Eng. Sci. Technol. Int. J. 2020, 23, 732–743. [Google Scholar] [CrossRef]
- Cheng, T. Ultra-High-Temperature Mechanical Behaviors of Two-Dimensional Carbon Fiber Reinforced Silicon Carbide Composites: Experiment and Modeling. J. Eur. Ceram. Soc. 2021, 41, 2335–2346. [Google Scholar] [CrossRef]
- Meng, J.; Wang, Y.; Yang, H.; Wang, P.; Lei, Q.; Shi, H.; Lei, H.; Fang, D. Mechanical Properties and Internal Microdefects Evolution of Carbon Fiber Reinforced Polymer Composites: Cryogenic Temperature and Thermocycling Effects. Compos. Sci. Technol. 2020, 191, 108083. [Google Scholar] [CrossRef]
- Jia, Z.; Li, T.; Chiang, F.P.; Wang, L. An Experimental Investigation of the Temperature Effect on the Mechanics of Carbon Fiber Reinforced Polymer Composites. Compos. Sci. Technol. 2018, 154, 53–63. [Google Scholar] [CrossRef]
- Carpier, Y.; Vieille, B.; Coppalle, A.; Barbe, F. About the Tensile Mechanical Behaviour of Carbon Fibers Fabrics Reinforced Thermoplastic Composites under Very High Temperature Conditions. Compos. Part B Eng. 2020, 181, 107586. [Google Scholar] [CrossRef]
- Jia, S.; Wang, F.; Zhou, J.; Jiang, Z.; Xu, B. Study on the Mechanical Performances of Carbon Fiber/Epoxy Composite Material Subjected to Dynamical Compression and High Temperature Loads. Compos. Struct. 2021, 258, 113421. [Google Scholar] [CrossRef]
- Li, Y.; Li, W.; Tao, Y.; Shao, J.; Deng, Y.; Kou, H.; Zhang, X.; Chen, L. Theoretical Model for the Temperature Dependent Longitudinal Tensile Strength of Unidirectional Fiber Reinforced Polymer Composites. Compos. Part B Eng. 2019, 161, 121–127. [Google Scholar] [CrossRef]
- Kawai, M.; Takeuchi, H.; Taketa, I.; Tsuchiya, A. Effects of Temperature and Stress Ratio on Fatigue Life of Injection Molded Short Carbon Fiber-Reinforced Polyamide Composite. Compos. Part A Appl. Sci. Manuf. 2017, 98, 9–24. [Google Scholar] [CrossRef]
- Milella, P.P. Fatigue and Corrosion in Metals; Springer: Milan, Italy, 2013; ISBN 9788578110796. [Google Scholar]
- Smith, P.; Topper, T.; Watson, P. A Stress-Strain Function for the Fatigue of Metals. J. Mater. 1970, 5, 767–778. [Google Scholar]
- Miao, C.; Tippur, H.V. Fracture Behavior of Carbon Fiber Reinforced Polymer Composites: An Optical Study of Loading Rate Effects. Eng. Fract. Mech. 2019, 207, 203–221. [Google Scholar] [CrossRef]
- Choi, J.; Quagliato, L.; Shin, J.; Kim, N. Investigation on the Static and Cyclic Anisotropic Mechanical Behavior of Polychloroprene Rubber (CR) Reinforced with Tungsten Nano-Particles. Eng. Fract. Mech. 2020, 235, 107183. [Google Scholar] [CrossRef]
- Choi, J.; Quagliato, L.; Lee, S.; Shin, J.; Kim, N. Multiaxial Fatigue Life Prediction of Polychloroprene Rubber (CR) Reinforced with Tungsten Nano-Particles Based on Semi-Empirical and Machine Learning Models. Int. J. Fatigue 2021, 145, 106136. [Google Scholar] [CrossRef]
- Choi, J.; Lee, H.; Lee, H.; Kim, N. A Methodology to Predict the Fatigue Life under Multi-Axial Loading of Carbon Fiber-Reinforced Polymer Composites Considering Anisotropic Mechanical Behavior. Materials 2023, 16, 1952. [Google Scholar] [CrossRef] [PubMed]
- Launay, A.; Maitournam, M.H.; Marco, Y.; Raoult, I. Multiaxial Fatigue Models for Short Glass Fibre Reinforced Polyamide. Part II: Fatigue Life Estimation. Int. J. Fatigue 2013, 47, 390–406. [Google Scholar] [CrossRef]
- Launay, A.; Maitournam, M.H.; Marco, Y.; Raoult, I. Multiaxial Fatigue Models for Short Glass Fiber Reinforced Polyamide—Part I: Nonlinear Anisotropic Constitutive Behavior for Cyclic Response. Int. J. Fatigue 2013, 47, 382–389. [Google Scholar] [CrossRef]
- Choi, J.; Choi, J.; Lee, K.; Hur, N.; Kim, N. Fatigue Life Prediction Methodology of Hot Work Tool Steel Dies for High-Pressure Die Casting Based on Thermal Stress Analysis. Metals 2022, 12, 1744. [Google Scholar] [CrossRef]
- Tang, H.C.; Nguyen, T.; Chuang, T.J.; Chin, J.; Wu, F.; Lesko, J. Temperature Effects on Fatigue of Polymer Composites. In Proceedings of the Composites Engineering, 7th Annual International Conference, ICCE/7, Denver, CO, USA, 2–8 July 2000. [Google Scholar]
- Mivehchi, H.; Varvani-Farahani, A. The Effect of Temperature on Fatigue Strength and Cumulative Fatigue Damage of FRP Composites. Procedia Eng. 2010, 2, 2011–2020. [Google Scholar] [CrossRef]
- Fouchier, N.; Nadot-Martin, C.; Conrado, E.; Bernasconi, A.; Castagnet, S. Fatigue Life Assessment of a Short Fibre Reinforced Thermoplastic at High Temperature Using a Through Process Modelling in a Viscoelastic Framework. Int. J. Fatigue 2019, 124, 236–244. [Google Scholar] [CrossRef]
- Okayasu, M.; Tsuchiya, Y. Mechanical and Fatigue Properties of Long Carbon Fiber Reinforced Plastics at Low Temperature. J. Sci. Adv. Mater. Devices 2019, 4, 577–583. [Google Scholar] [CrossRef]
- Yang, C.; Kim, Y.; Ryu, S.; Gu, G.X. Prediction of Composite Microstructure Stress-Strain Curves Using Convolutional Neural Networks. Mater. Des. 2020, 189, 108509. [Google Scholar] [CrossRef]
- Mortazavian, S.; Fatemi, A. Fatigue Behavior and Modeling of Short Fiber Reinforced Polymer Composites Including Anisotropy and Temperature Effects. Int. J. Fatigue 2015, 77, 12–27. [Google Scholar] [CrossRef]
- Murata, Y.; Kanno, R. Effects of Heating and Cooling of Injection Mold Cavity Surface and Melt Flow Control on Properties of Carbon Fiber Reinforced Semi-Aromatic Polyamide Molded Products. Polymers 2021, 13, 587. [Google Scholar] [CrossRef] [PubMed]
- Karsli, N.G.; Aytac, A. Tensile and Thermomechanical Properties of Short Carbon Fiber Reinforced Polyamide 6 Composites. Compos. Part B Eng. 2013, 51, 270–275. [Google Scholar] [CrossRef]



| Temperature [°C] | Selected Mean Load for Each Specimen Direction [kN] | ||
|---|---|---|---|
| 0° | 45° | 90° | |
| 40 | 2.000 | 1.250 | 1.125 |
| 60 | 1.750 | 1.125 | 1.000 |
| 100 | 1.500 | 1.000 | 0.750 |
| Temperature [°C] | Specimen Angle [°] | Max. Load [kN] | Min. Load [kN] | Mean Load [kN] | Amplitude Load [kN] | Nf [Cycles] |
|---|---|---|---|---|---|---|
| 40 | 0 | 3.20 | 0.80 | 2.00 | 1.20 | 1512 |
| 3.40 | 0.60 | 2.00 | 1.40 | 980 | ||
| 2.80 | 1.20 | 2.00 | 0.80 | 3400 | ||
| 1.40 | 0.60 | 1.00 | 0.40 | 106,263 | ||
| 2.40 | 2.20 | 2.30 | 0.10 | 674,785 | ||
| 45 | 1.90 | 0.60 | 1.25 | 0.65 | 7500 | |
| 2.10 | 0.40 | 1.25 | 0.85 | 2420 | ||
| 1.70 | 0.80 | 1.25 | 0.45 | 46,700 | ||
| 0.90 | 0.40 | 0.65 | 0.25 | 268,410 | ||
| 1.60 | 1.40 | 1.50 | 0.10 | 340,485 | ||
| 90 | 1.75 | 0.50 | 1.12 | 0.62 | 1774 | |
| 1.95 | 0.30 | 1.12 | 0.82 | 1670 | ||
| 1.55 | 0.70 | 1.12 | 0.42 | 9800 | ||
| 0.90 | 0.30 | 0.60 | 0.30 | 76,000 | ||
| 1.50 | 1.30 | 1.40 | 0.10 | 361,807 | ||
| 60 | 0 | 2.90 | 0.60 | 1.75 | 1.15 | 1820 |
| 3.25 | 0.25 | 1.75 | 1.50 | 770 | ||
| 2.60 | 0.90 | 1.75 | 0.85 | 8700 | ||
| 1.30 | 0.50 | 0.90 | 0.40 | 79,805 | ||
| 2.10 | 1.90 | 2.00 | 0.10 | 892,500 | ||
| 45 | 1.75 | 0.50 | 1.12 | 0.62 | 5840 | |
| 1.95 | 0.30 | 1.12 | 0.82 | 642 | ||
| 1.55 | 0.70 | 1.12 | 0.42 | 10,400 | ||
| 0.80 | 0.30 | 0.55 | 0.25 | 89,600 | ||
| 1.50 | 1.30 | 1.40 | 0.10 | 428,500 | ||
| 90 | 1.50 | 0.50 | 1.00 | 0.50 | 11,650 | |
| 1.60 | 0.40 | 1.00 | 0.600 | 3800 | ||
| 1.30 | 0.70 | 1.00 | 0.30 | 51,007 | ||
| 0.70 | 0.30 | 0.50 | 0.20 | 346,900 | ||
| 1.40 | 1.20 | 1.30 | 0.10 | 276,000 | ||
| 100 | 0 | 2.25 | 0.75 | 1.50 | 0.75 | 4753 |
| 2.50 | 0.50 | 1.50 | 1.00 | 1670 | ||
| 2.00 | 1.00 | 1.50 | 0.50 | 23,060 | ||
| 1.00 | 0.40 | 0.70 | 0.30 | 660,650 | ||
| 1.80 | 1.60 | 1.70 | 0.10 | 475,439 | ||
| 45 | 1.50 | 0.50 | 1.00 | 0.50 | 4460 | |
| 1.60 | 0.40 | 1.00 | 0.60 | 1790 | ||
| 1.30 | 0.70 | 1.00 | 0.30 | 29,800 | ||
| 0.70 | 0.30 | 0.50 | 0.20 | 108,699 | ||
| 1.40 | 1.20 | 1.30 | 0.10 | 90,335 | ||
| 90 | 1.15 | 0.35 | 0.75 | 0.40 | 5700 | |
| 1.35 | 0.15 | 0.75 | 0.60 | 1213 | ||
| 1.00 | 0.50 | 0.75 | 0.25 | 39,355 | ||
| 0.50 | 0.20 | 0.35 | 0.15 | 270,883 | ||
| 1.10 | 0.90 | 1.00 | 0.10 | 166,068 |
| Temperature [°C] | Specimen Angle [°] | Max. Load [kN] | Min. Load [kN] | Mean Load [kN] | Amplitude Load [kN] | Nf [Cycles] |
|---|---|---|---|---|---|---|
| 40 | 0 | 0.13 | 0.11 | 0.12 | 0.01 | 2109 |
| 45 | 0.10 | 0.80 | 0.90 | 0.01 | 905 | |
| 90 | 0.08 | 0.06 | 0.07 | 0.01 | 2160 | |
| 60 | 0 | 0.11 | 0.09 | 0.10 | 0.01 | 15,041 |
| 45 | 0.08 | 0.06 | 0.07 | 0.01 | 9800 | |
| 90 | 0.08 | 0.06 | 0.07 | 0.01 | 5061 | |
| 100 | 0 | 0.09 | 0.07 | 0.08 | 0.01 | 1200 |
| 45 | 0.06 | 0.04 | 0.05 | 0.01 | 671 | |
| 90 | 0.05 | 0.03 | 0.04 | 0.01 | 2780 |
| Symbol | Definition |
|---|---|
| εp,eff | Effective plastic strain |
| T | Temperature in the environmental chamber in °C |
| K | Strength coefficient |
| n | Hardening exponent |
| αm | Weight factor for the fiber direction |
| βm | Weight factor for the direction normal to the fibers |
| Em | Polymer matrix elastic modulus |
| Ef | Fiber’s elastic modulus |
| λm, I | The first eigenvalue of the fiber orientation matrix in the region with strong fiber alignment with the polymer flow |
| T [°C] | σ0 [MPa] | n | αm | βm | Em [GPa] | Ef [GPa] | λm,I |
|---|---|---|---|---|---|---|---|
| 40 | 250.10 | 3.34 | 18.85 | 11.33 | 0.78 | 60.02 | 0.85 |
| 60 | 215.76 | 4.14 | 7.13 | 8.40 | 0.56 | 30.37 | 0.85 |
| 100 | 227.12 | 4.46 | 16.71 | 17.65 | 0.40 | 30.48 | 0.85 |
| Temperature [°C] | Tensile Strength of Each Specimen Direction [MPa] | ||
|---|---|---|---|
| 0° | 45° | 90° | |
| 40 | 130.3 | 101.3 | 77.2 |
| 60 | 122.6 | 79.6 | 60.8 |
| 100 | 98.8 | 62.8 | 51.2 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Choi, J.; Andrian, Y.O.; Lee, H.; Lee, H.; Kim, N. Fatigue Life Prediction for Injection-Molded Carbon Fiber-Reinforced Polyamide-6 Considering Anisotropy and Temperature Effects. Materials 2024, 17, 315. https://doi.org/10.3390/ma17020315
Choi J, Andrian YO, Lee H, Lee H, Kim N. Fatigue Life Prediction for Injection-Molded Carbon Fiber-Reinforced Polyamide-6 Considering Anisotropy and Temperature Effects. Materials. 2024; 17(2):315. https://doi.org/10.3390/ma17020315
Chicago/Turabian StyleChoi, Joeun, Yohanes Oscar Andrian, Hyungtak Lee, Hyungyil Lee, and Naksoo Kim. 2024. "Fatigue Life Prediction for Injection-Molded Carbon Fiber-Reinforced Polyamide-6 Considering Anisotropy and Temperature Effects" Materials 17, no. 2: 315. https://doi.org/10.3390/ma17020315
APA StyleChoi, J., Andrian, Y. O., Lee, H., Lee, H., & Kim, N. (2024). Fatigue Life Prediction for Injection-Molded Carbon Fiber-Reinforced Polyamide-6 Considering Anisotropy and Temperature Effects. Materials, 17(2), 315. https://doi.org/10.3390/ma17020315






