A Novel Feedforward Model of Piezoelectric Actuator for Precision Rapid Cutting
Abstract
1. Introduction
2. MBW Model for PZAs
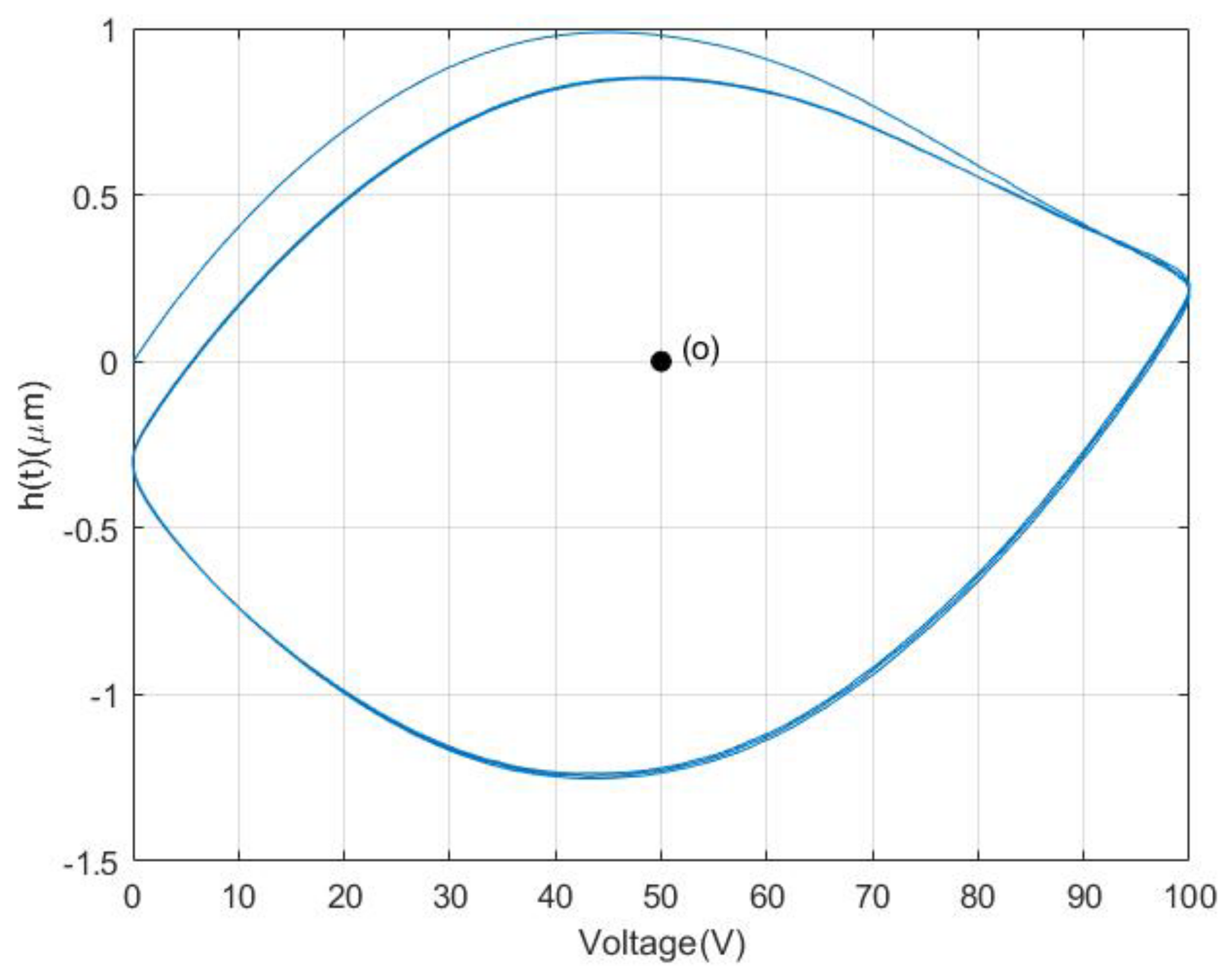
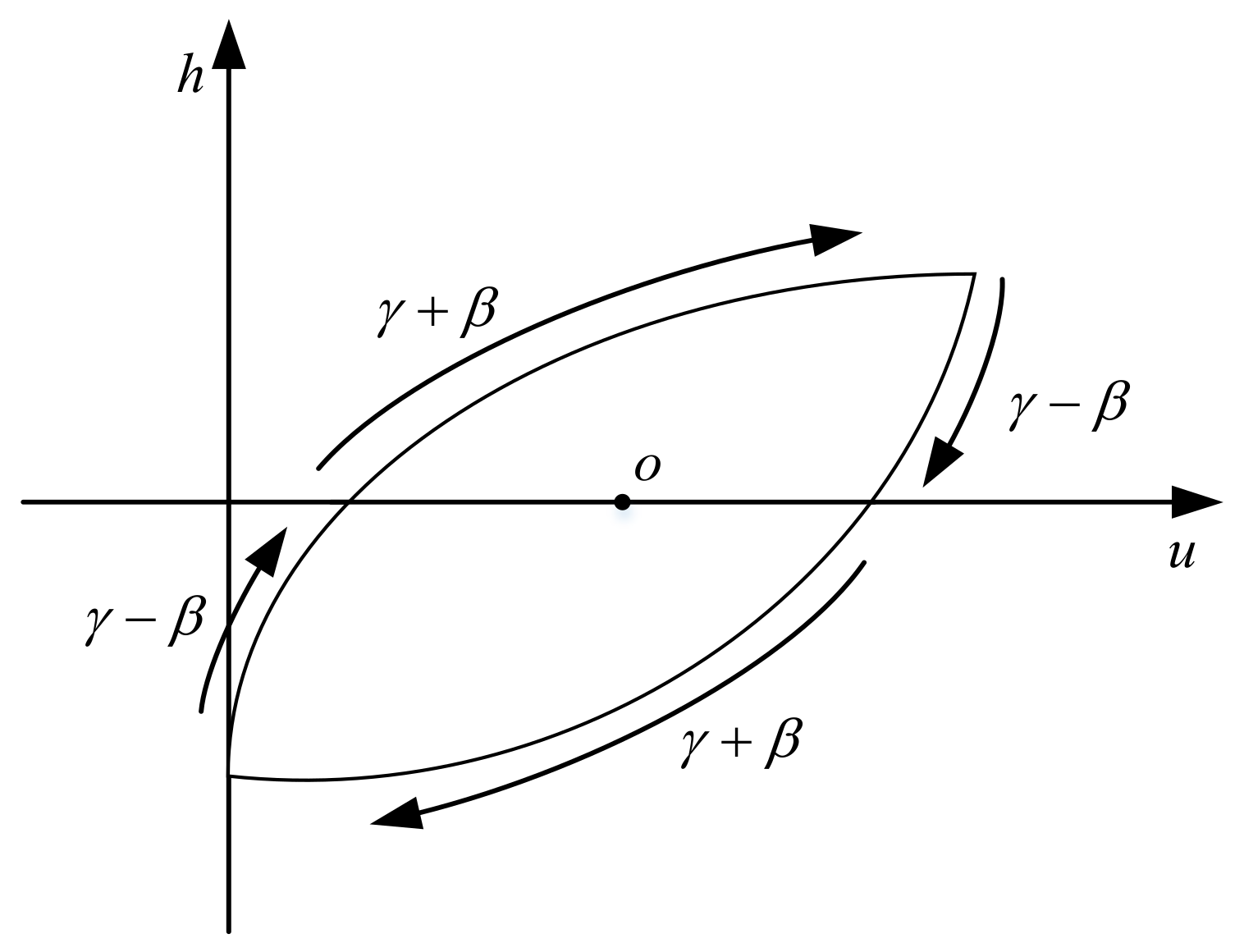
2.1. Hysteresis Analysis
2.2. CBW Model
2.3. MBW Model
3. Parameters Identification Using a Novel Modified PSO Algorithms
3.1. Parameters Identification with Traditional PSO Algorithm
3.2. Parameters Identification with Self-Adaptive Cooperative PSO Algorithm
4. Model Parameters Identification and Algorithm Evaluation
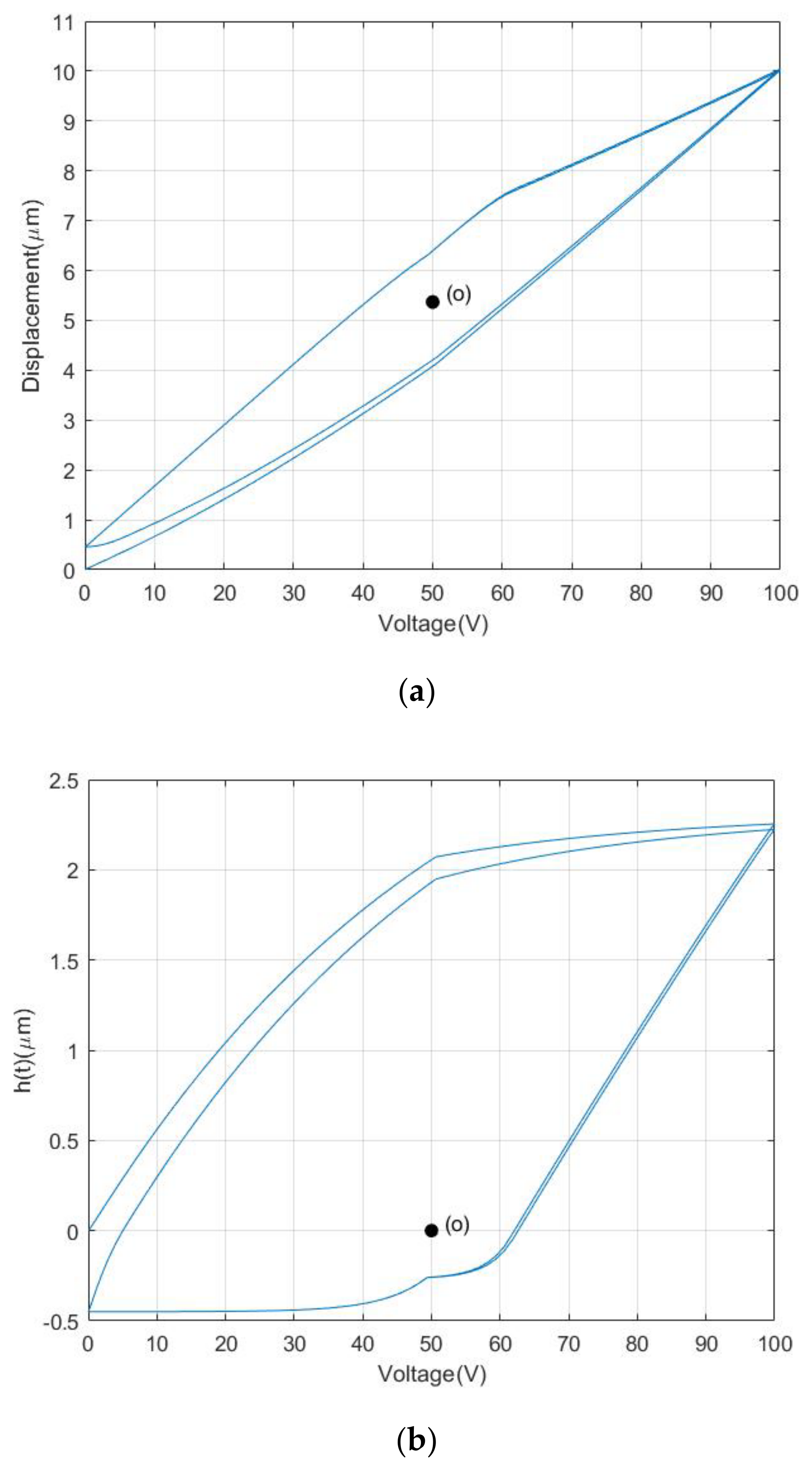
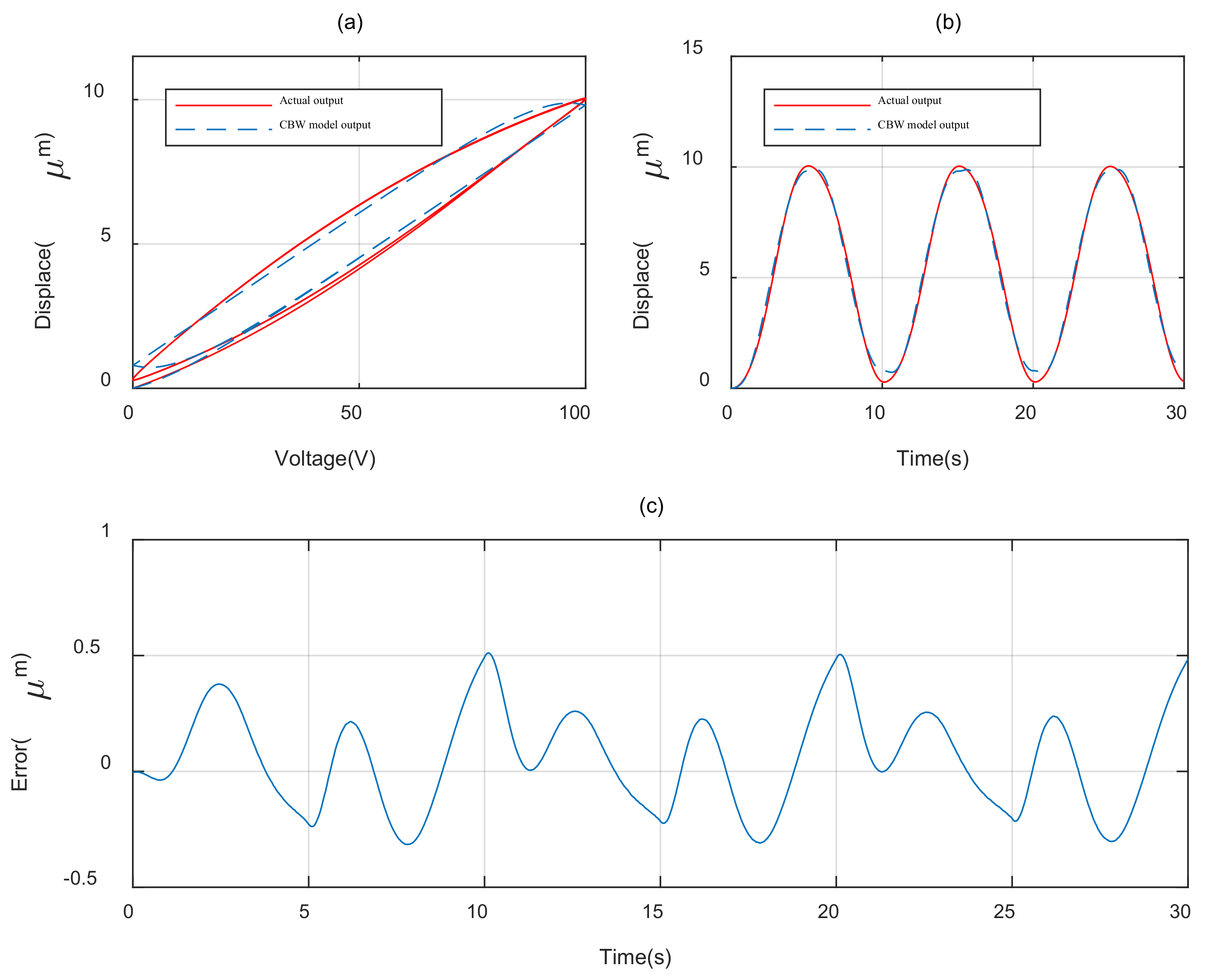
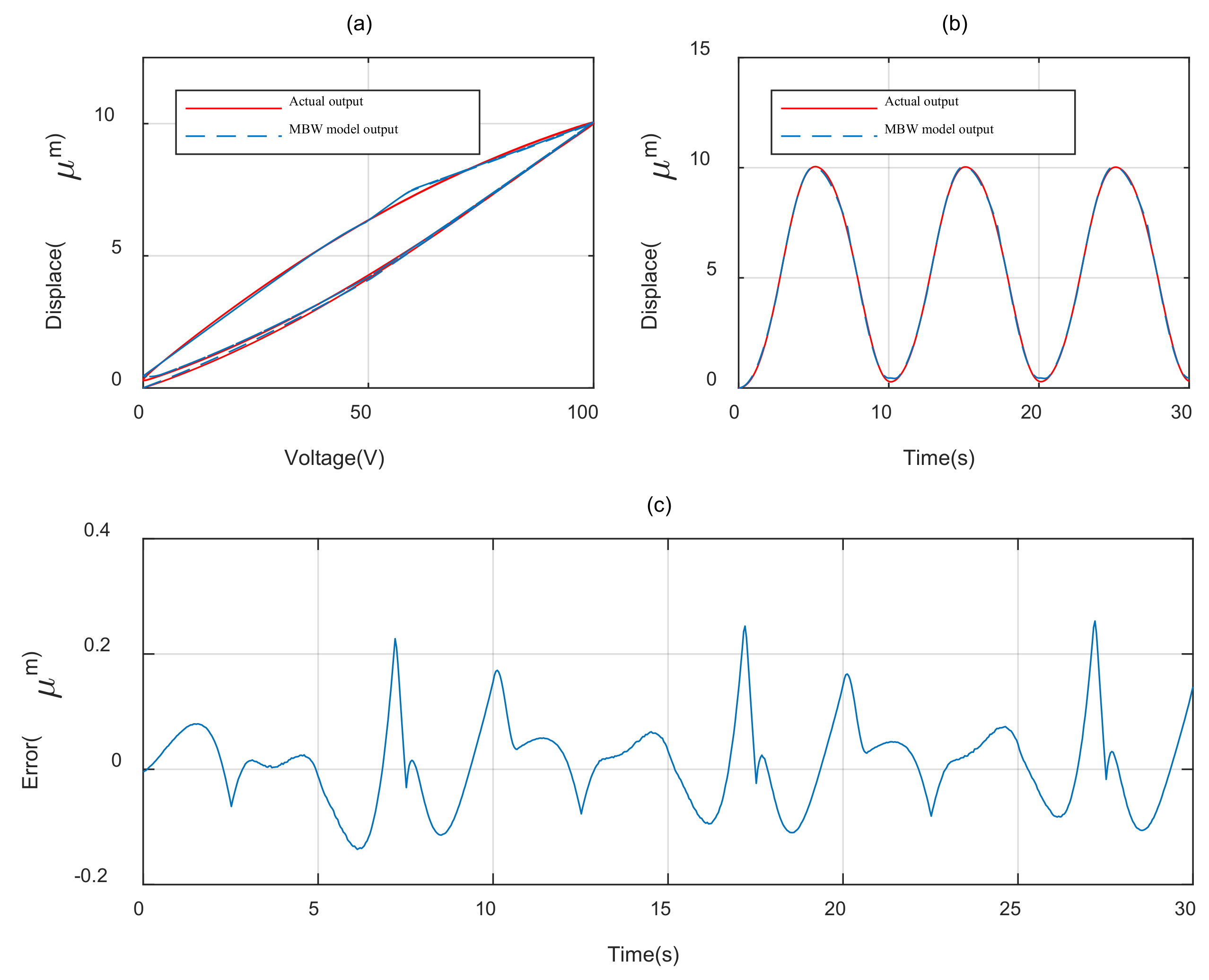
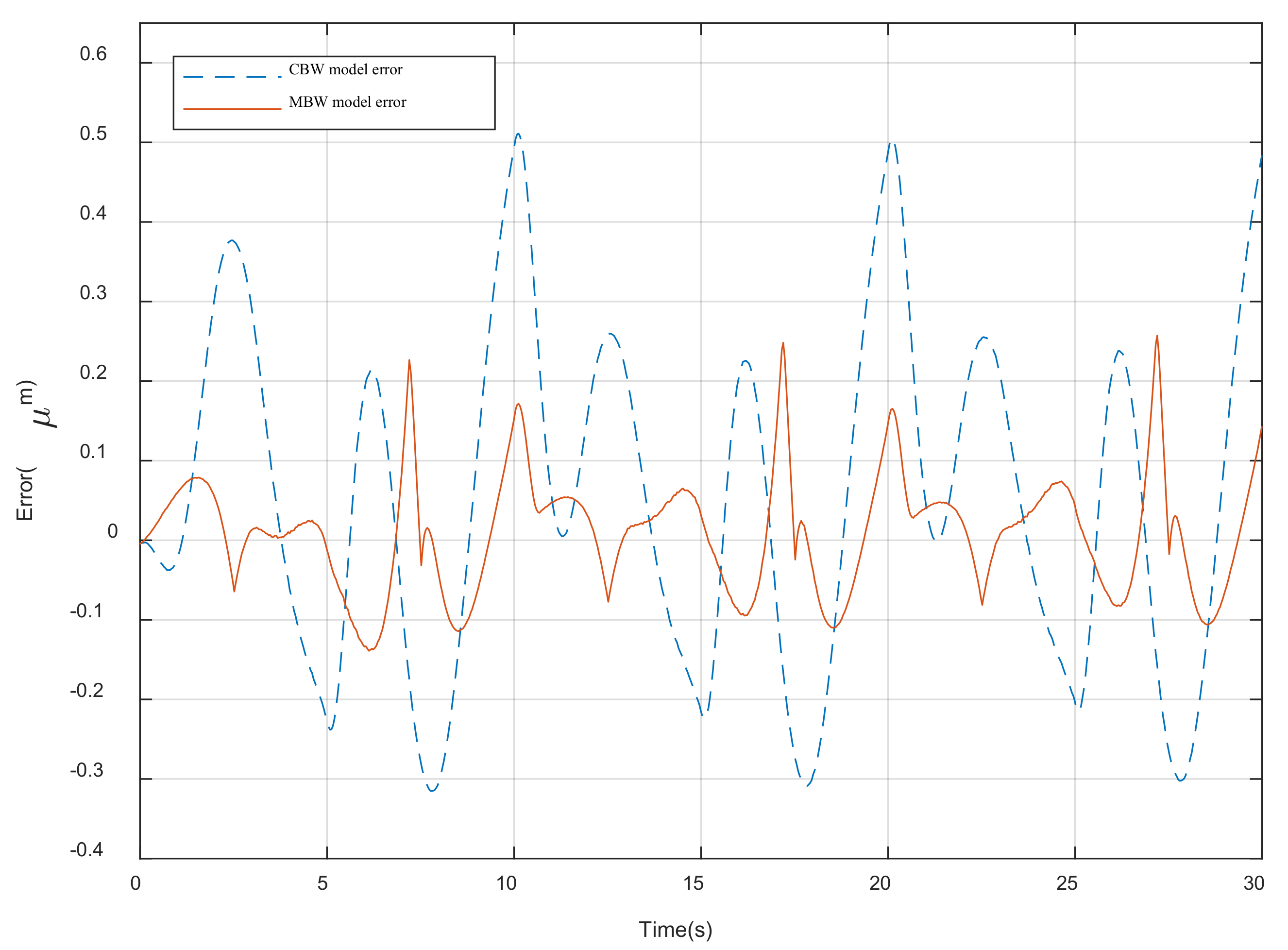
4.1. Hysteresis Model Evaluation
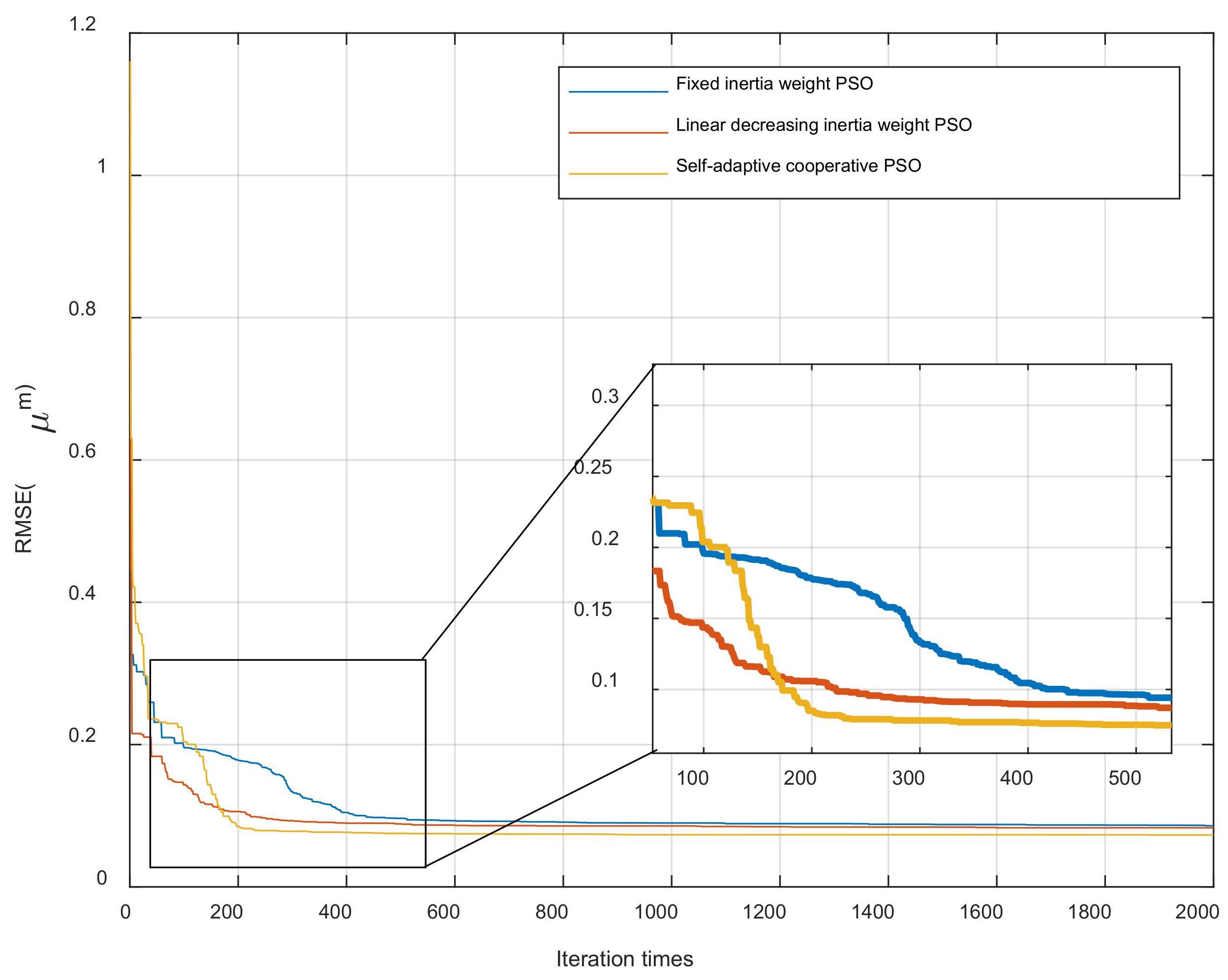
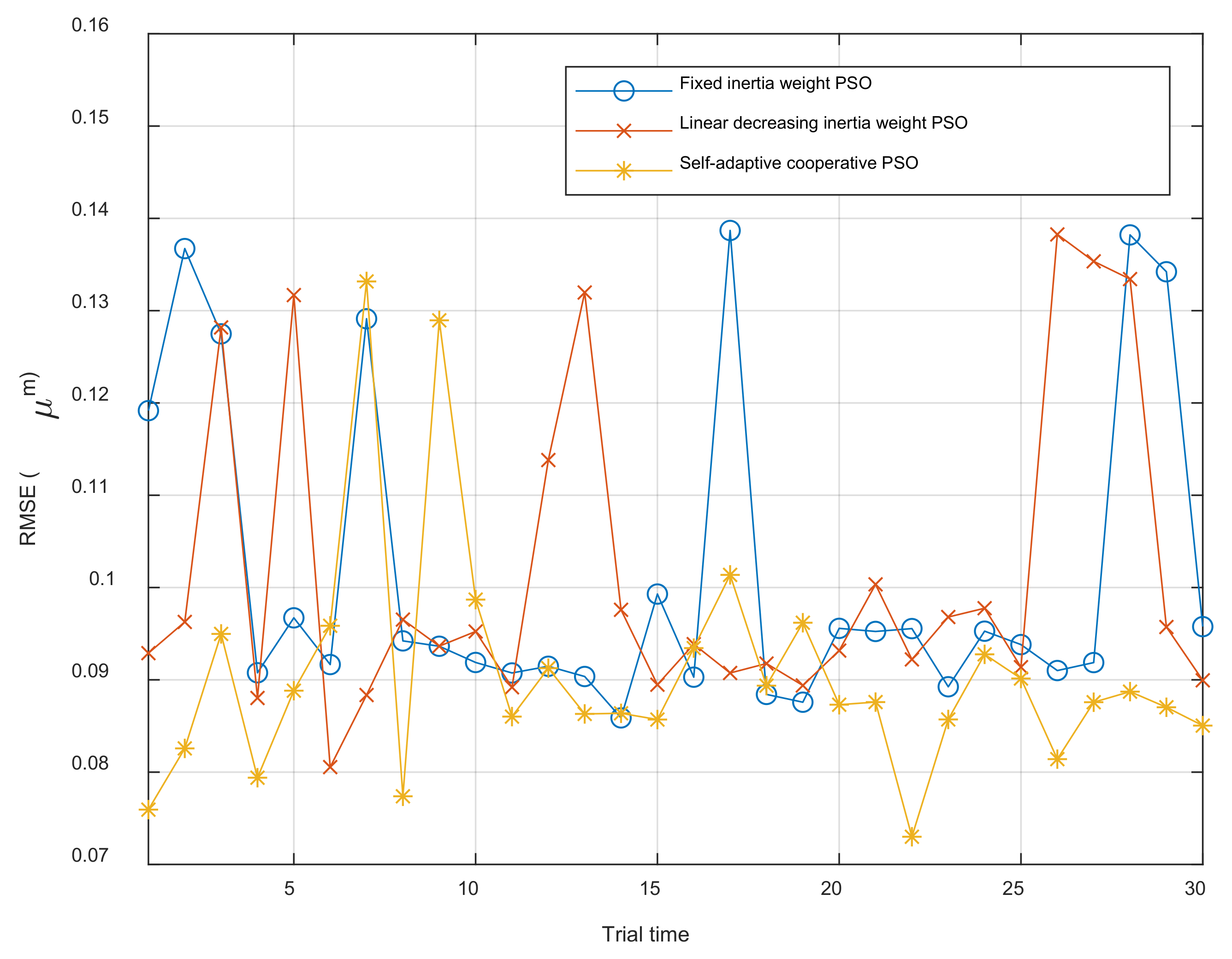
4.2. Parameter Identification Algorithm Evaluation
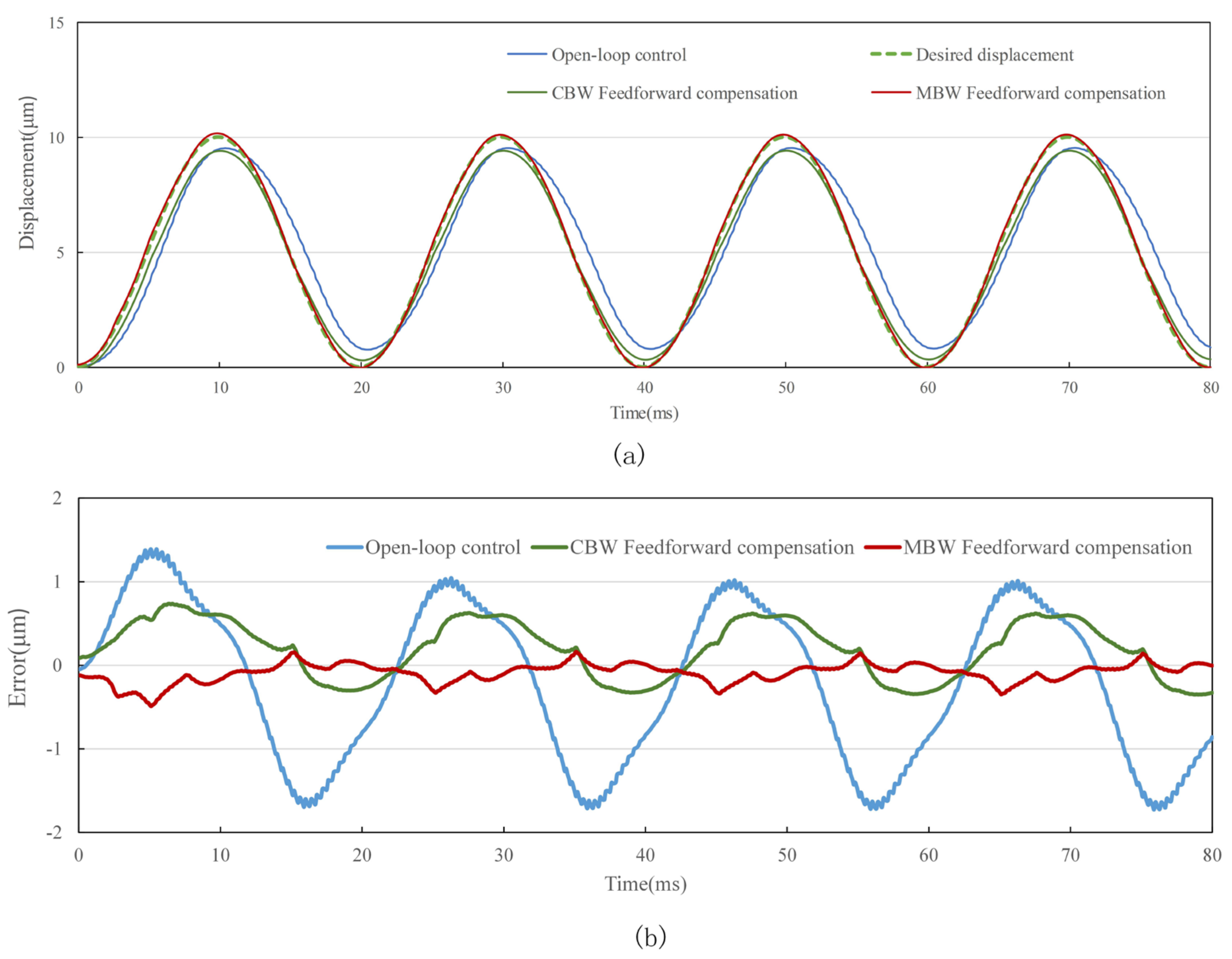
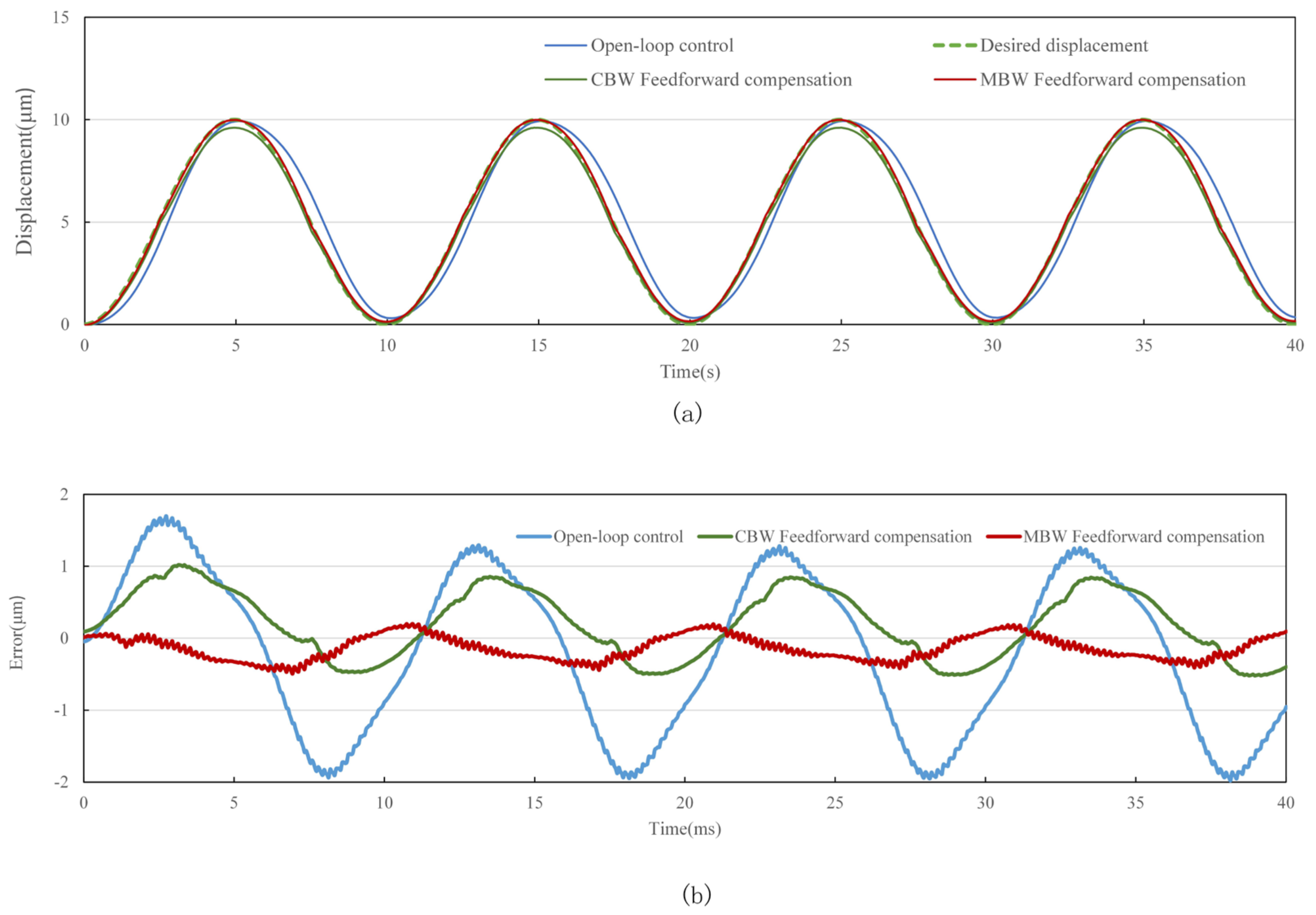
5. Feedforward Control Experiment
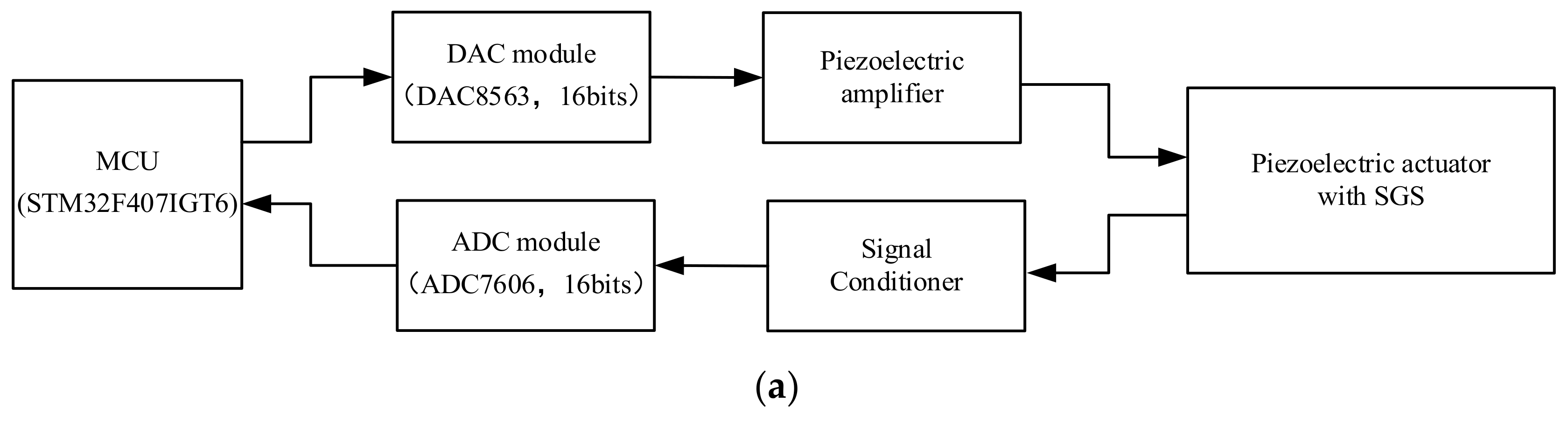
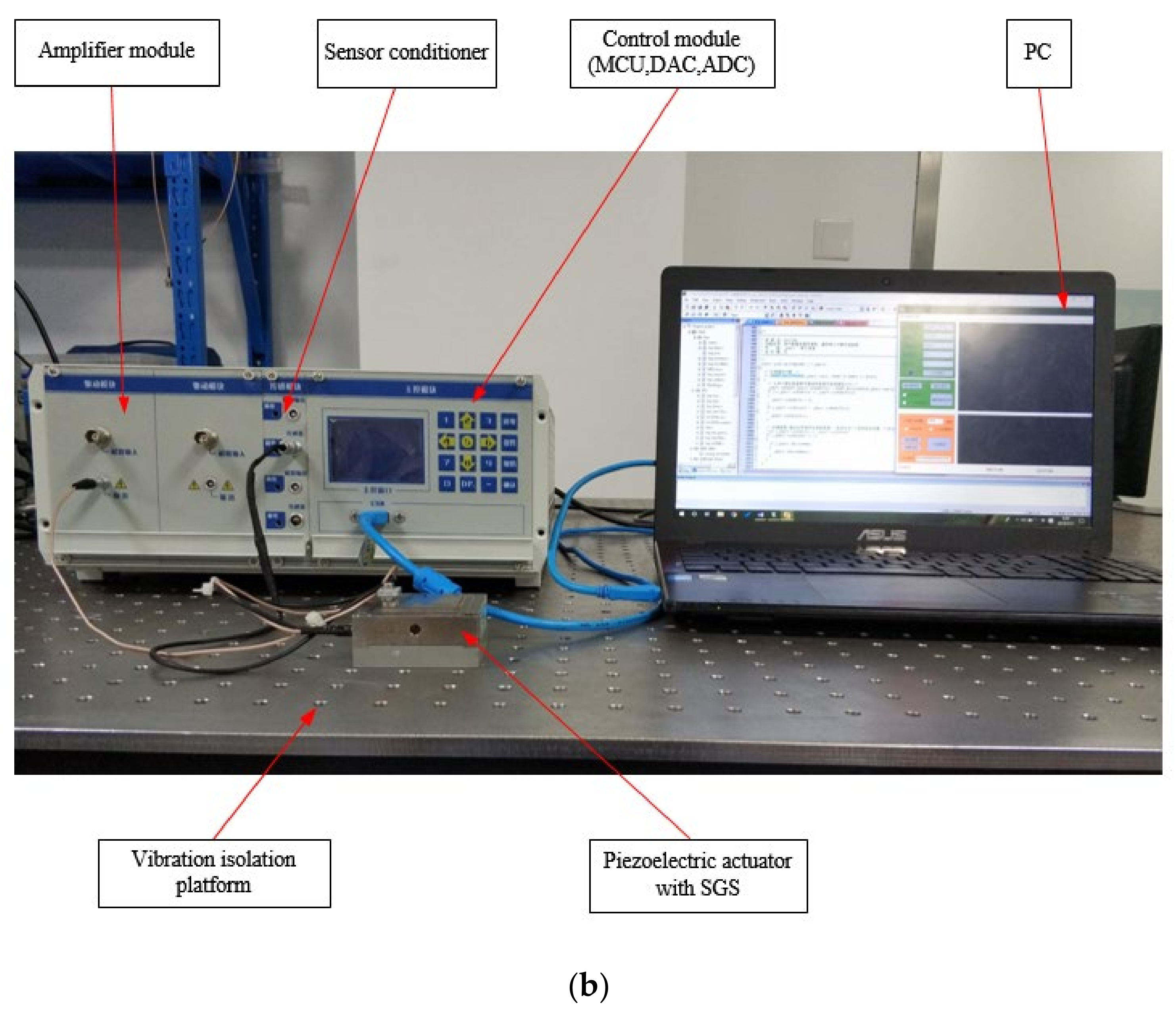
5.1. Experiment Setup
5.2. Feedforward Compensator Based on MBW Model
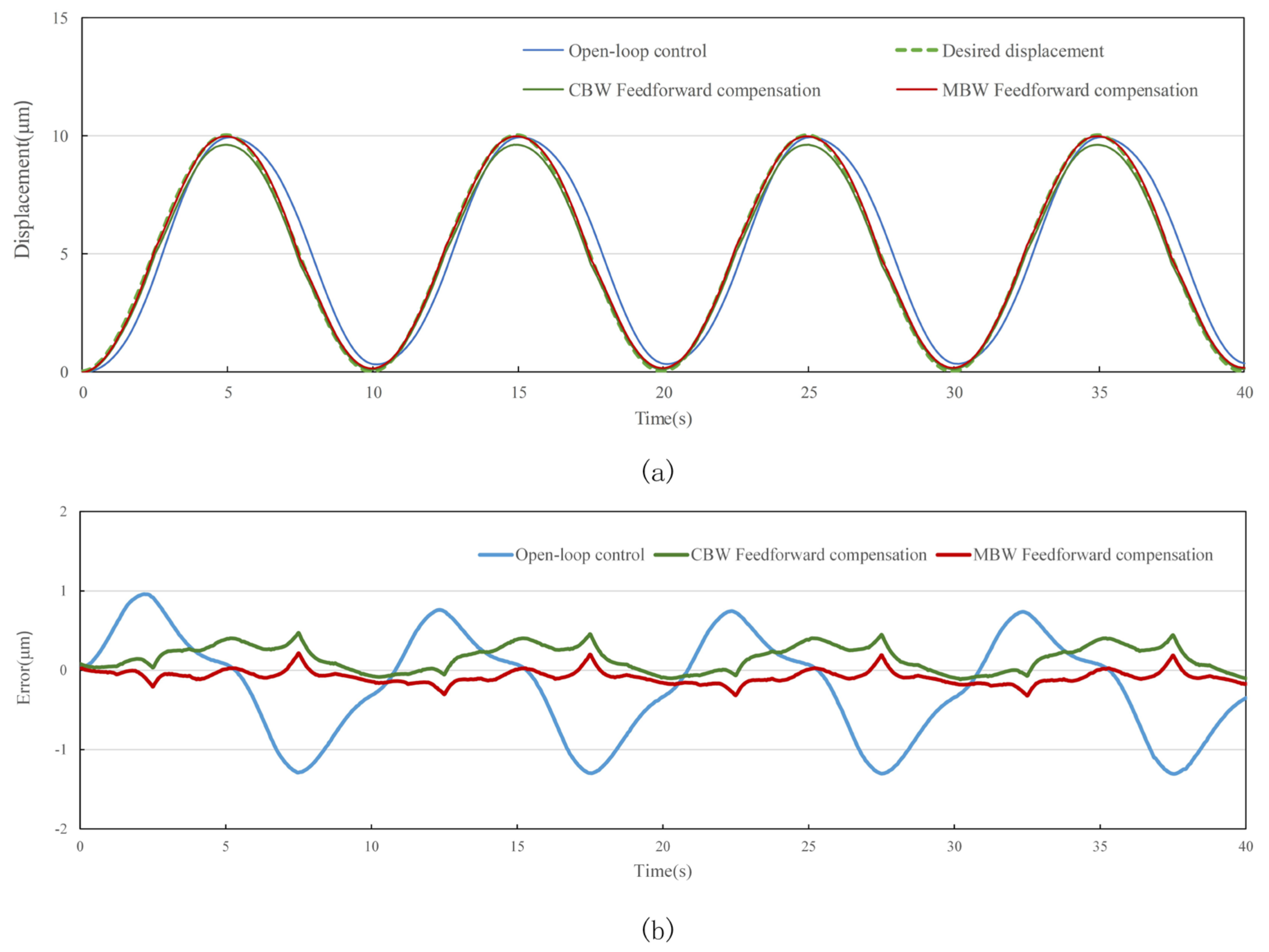
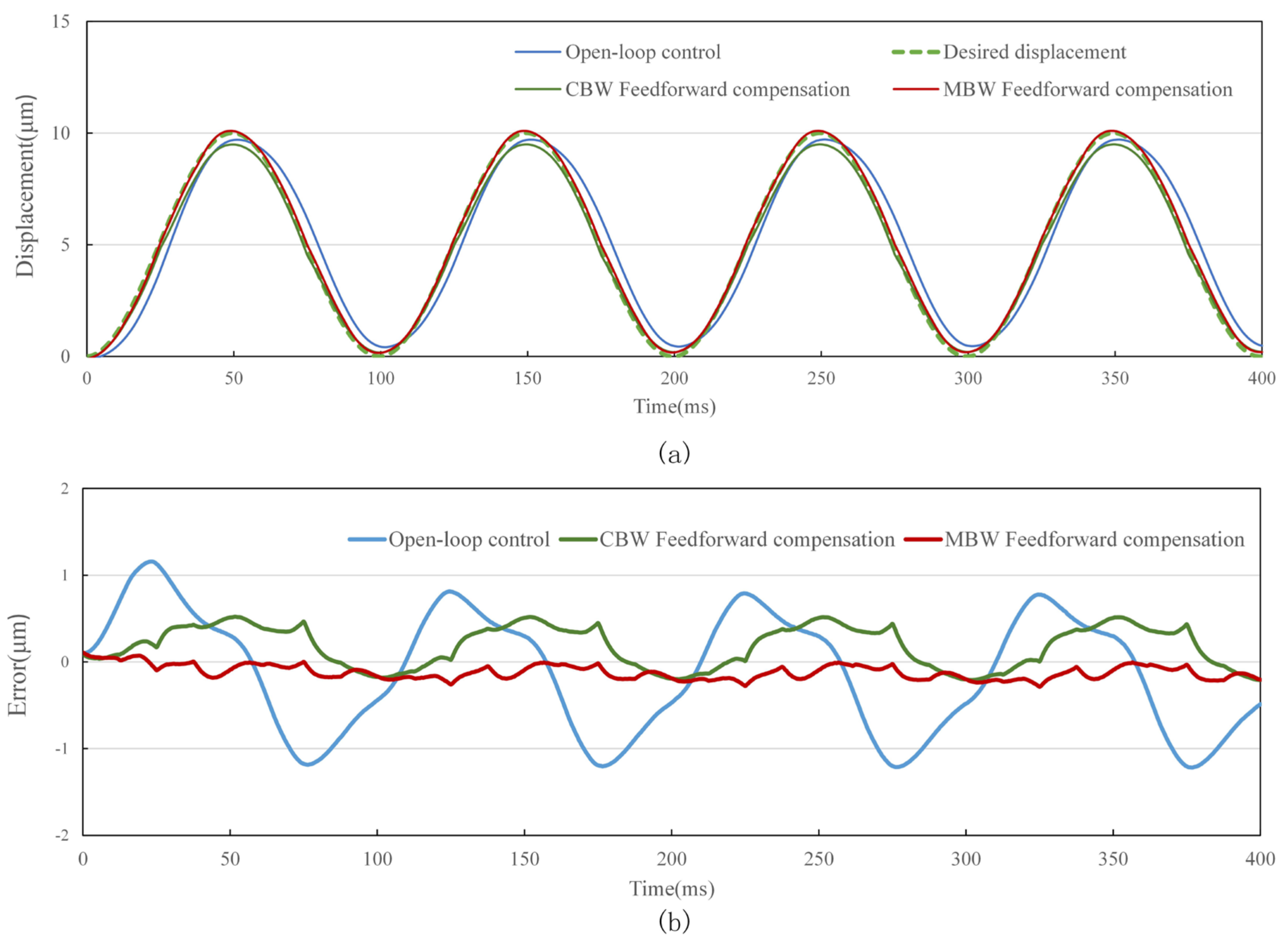
5.3. Control Experiment
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Dong, X.; Yang, Y.; Zhang, C.; Shan, X. A Novel Self-Sensing Stacking Piezoelectric Actuator Based on Structural Integration. In Proceedings of the 2018 5th International Conference on Information Science and Control Engineering (ICISCE), Zhengzhou, China, 20–22 July 2018; pp. 632–635. [Google Scholar] [CrossRef]
- Li, C.-X.; Gu, G.-Y.; Yang, M.-J.; Zhu, L.-M. Design, analysis and testing of a parallel-kinematic high-bandwidth XY nanopositioning stage. Rev. Sci. Instrum. 2013, 84, 125111. [Google Scholar] [CrossRef]
- Polit, S.; Dong, J. Development of a High-Bandwidth XY Nanopositioning Stage for High-Rate Micro-/Nanomanufacturing. IEEE/ASME Trans. Mechatron. 2011, 16, 724–733. [Google Scholar] [CrossRef]
- Hansma, P.K.; Schitter, G.; Fantner, G.E.; Prater, C. High-Speed Atomic Force Microscopy. Science 2006, 314, 601–602. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Y.; Lu, T.-F. Investigation on the Uniform-Electrical-Field Assumption for Modelling Multi-layer Piezoelectric Actuators. In Proceedings of the 2014 8th Asia Modelling Symposium, Taipei, Taiwan, 23–25 September 2014; pp. 251–254. [Google Scholar] [CrossRef]
- Liu, Y.; Wang, L.; Gu, Z.; Quan, Q.; Deng, J. Development of a Two-Dimensional Linear Piezoelectric Stepping Platform Using Longitudinal-Bending Hybrid Actuators. IEEE Trans. Ind. Electron. 2019, 66, 3030–3040. [Google Scholar] [CrossRef]
- Mazeika, D.; Vasiljev, P.; Borodinas, S.; Bareikis, R.; Yang, Y. Small size piezoelectric impact drive actuator with rectangular bimorphs. Sens. Actuators A Phys. 2019, 280, 76–84. [Google Scholar] [CrossRef]
- Gu, G.-Y.; Zhu, L.-M.; Su, C.-Y.; Ding, H. Motion Control of Piezoelectric Positioning Stages: Modeling, Controller Design, and Experimental Evaluation. IEEE/ASME Trans. Mechatron. 2013, 18, 1459–1471. [Google Scholar] [CrossRef]
- Lu, M.; Zhou, J.; Lin, J.; Gu, Y.; Han, J.; Zhao, D. Study on Ti-6Al-4V Alloy Machining Applying the Non-Resonant Three-Dimensional Elliptical Vibration Cutting. Micromachines 2018, 8, 306. [Google Scholar] [CrossRef]
- Lu, M.; Wang, H.; Guan, L.; Lin, J.; Gu, Y.; Chen, B.; Zhao, D. Modeling and analysis of surface topography of Ti6Al4V alloy machining by elliptical vibration cutting. Int. J. Adv. Manuf. Technol. 2018, 98, 2759–2768. [Google Scholar] [CrossRef]
- Suzuki, N.; Yokoi, H.; Shamoto, E. Micro/nano sculpturing of hardened steel by controlling vibration amplitude in elliptical vibration cutting. Precis. Eng. 2011, 1, 44–50. [Google Scholar] [CrossRef]
- Rakotondrabe, M. Bouc–Wen Modeling and Inverse Multiplicative Structure to Compensate Hysteresis Nonlinearity in Piezoelectric Actuators. IEEE Trans. Autom. Sci. Eng. 2011, 8, 428–431. [Google Scholar] [CrossRef]
- Gu, G.-Y.; Zhu, L.-M.; Su, C.-Y.; Ding, H.; Fatikow, S. Modeling and Control of Piezo-Actuated Nanopositioning Stages: A Survey. IEEE Trans. Autom. Sci. Eng. 2016, 13, 313–332. [Google Scholar] [CrossRef]
- Gu, G.-Y.; Zhu, L.-M.; Su, C.-Y. Modeling and Compensation of Asymmetric Hysteresis Nonlinearity for Piezoceramic Actuators with a Modified Prandtl–Ishlinskii Model. IEEE Trans. Ind. Electron. 2014, 61, 1583–1595. [Google Scholar] [CrossRef]
- Bhattacharjee, A.; Mohanty, A.K.; Chatterjee, A. Expansion of Preisach density in magnetic hysteresis using general basis functions. Appl. Math. Comput. 2019, 341, 418–427. [Google Scholar] [CrossRef]
- Kim, S.-Y.; Lee, C.-H. Description of asymmetric hysteretic behavior based on the Bouc-Wen model and piecewise linear strength-degradation functions. Eng. Struct. 2019, 181, 181–191. [Google Scholar] [CrossRef]
- Mohammad, N.F.; Ikhouane, F. Consistency of the duhem model with hysteresis. Math. Probl. Eng. 2013, 2013, 586130. [Google Scholar] [CrossRef]
- Qin, Y.; Zhao, X.; Zhou, L. Modeling and Identification of the Rate-Dependent Hysteresis of Piezoelectric Actuator Using a Modified Prandtl-Ishlinskii Model. Micromachines 2017, 8, 114. [Google Scholar] [CrossRef]
- Zhu, W.; Wang, D.-H. Non-symmetrical Bouc–Wen model for piezoelectric ceramic actuators. Sens. Actuators A Phys. 2012, 181, 51–60. [Google Scholar] [CrossRef]
- Angeline, P.J. Evolutionary Optimization Versus Particle Swarm Optimization: Philosophy and Performance Differences; Springer: Berlin/Heidelberg, Germany, 1998. [Google Scholar] [CrossRef]
- Song, J.; Der Kiureghian, A. Generalized Bouc–Wen Model for Highly Asymmetric Hysteresis. J. Eng. Mech. 2006, 132, 610–618. [Google Scholar] [CrossRef]
- Gu, G.; Zhu, L. Modeling of rate-dependent hysteresis in piezoelectric actuators using a family of ellipses. Sens. Actuators A Phys. 2011, 165, 303–309. [Google Scholar] [CrossRef]
- Ankita; Sahana, S.K. Ba-PSO: A Balanced PSO to solve multi-objective grid scheduling problem. Appl. Intell. 2022, 52, 4015–4027. [Google Scholar] [CrossRef]
- Li, M.; Chen, H.; Shi, X.; Liu, S.; Zhang, M.; Lu, S. A multi-information fusion “triple variables with iteration” inertia weight PSO algorithm and its application. Appl. Soft Comput. 2019, 84, 105677. [Google Scholar] [CrossRef]
- Shi, Y.; Eberhart, R.C. Empirical study of particle swarm optimization. In Proceedings of the 1999 Congress on Evolutionary Computation—CEC99 (Cat. No. 99TH8406), Washington, DC, USA, 6–9 July 1999; Volume 3, pp. 1945–1950. [Google Scholar] [CrossRef]




| Phase | ||||
|---|---|---|---|---|
| 1 | + | − | + | |
| 2 | + | + | + | |
| 3 | + | + | − | |
| 4 | − | + | − | |
| 5 | − | − | − | |
| 6 | − | − | + |
| Parameters | Value |
|---|---|
| 0.106037 | |
| 0.069699 | |
| −0.012006 | |
| 0.100000 |
| Parameters | Value |
|---|---|
| 0.124131 | |
| 0.062662 | |
| 0.062490 | |
| 0.099500 | |
| −0.003578 | |
| 0.083543 | |
| −0.090824 | |
| −0.084294 | |
| −0.050231 |
| Model | ||
|---|---|---|
| CBW model | 0.216506 | 0.511217 |
| MBW model | 0.072782 | 0.257119 |
| Algorithms | Traditional PSO (Fixed Inertia Weight) | Traditional PSO (Linear Decreased Inertia Weight) | Modified PSO |
|---|---|---|---|
| Fitness | 0.085877 | 0.080545 | 0.072782 |
| 0.120881 | 0.123564 | 0.124131 | |
| 0.054375 | 0.062080 | 0.062662 | |
| −0.011955 | 0.054668 | 0.062490 | |
| 0.073156 | 0.067579 | 0.099500 | |
| −0.022549 | −0.00865 | −0.003578 | |
| 0.014828 | 0.048177 | 0.083543 | |
| −0.081405 | −0.063953 | −0.090824 | |
| 0.001384 | −0.043963 | −0.084294 | |
| 0.039550 | −0.037160 | −0.050231 |
| Traditional PSO (Fixed Inertia Weight) | Traditional PSO (Linear Decreased Inertia Weight) | Modified PSO | |
|---|---|---|---|
| Min | 0.085877 | 0.080545 | 0.072782 |
| Max | 0.138687 | 0.138259 | 0.133166 |
| Average | 0.101663 | 0.101452 | 0.090603 |
| Frequency | Open-Loop Control | CBW Feedforward Compensation | MBW Feedforward Compensation |
|---|---|---|---|
| 0.1 Hz | 0.674 μm | 0.228 μm | 0.114 μm |
| 10 Hz | 0.693 μm | 0.297 μm | 0.143 μm |
| 50 Hz | 0.952 μm | 0.391 μm | 0.147 μm |
| 100 Hz | 1.112 μm | 0.501 μm | 0.236 μm |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhong, B.; Liu, S.; Wang, C.; Jin, Z.; Sun, L. A Novel Feedforward Model of Piezoelectric Actuator for Precision Rapid Cutting. Materials 2023, 16, 2271. https://doi.org/10.3390/ma16062271
Zhong B, Liu S, Wang C, Jin Z, Sun L. A Novel Feedforward Model of Piezoelectric Actuator for Precision Rapid Cutting. Materials. 2023; 16(6):2271. https://doi.org/10.3390/ma16062271
Chicago/Turabian StyleZhong, Bowen, Shilin Liu, Chenjun Wang, Ziqi Jin, and Lining Sun. 2023. "A Novel Feedforward Model of Piezoelectric Actuator for Precision Rapid Cutting" Materials 16, no. 6: 2271. https://doi.org/10.3390/ma16062271
APA StyleZhong, B., Liu, S., Wang, C., Jin, Z., & Sun, L. (2023). A Novel Feedforward Model of Piezoelectric Actuator for Precision Rapid Cutting. Materials, 16(6), 2271. https://doi.org/10.3390/ma16062271





