A Numerical Study of Crack Mixed Mode Model in Concrete Material Subjected to Cyclic Loading
Abstract
1. Introduction
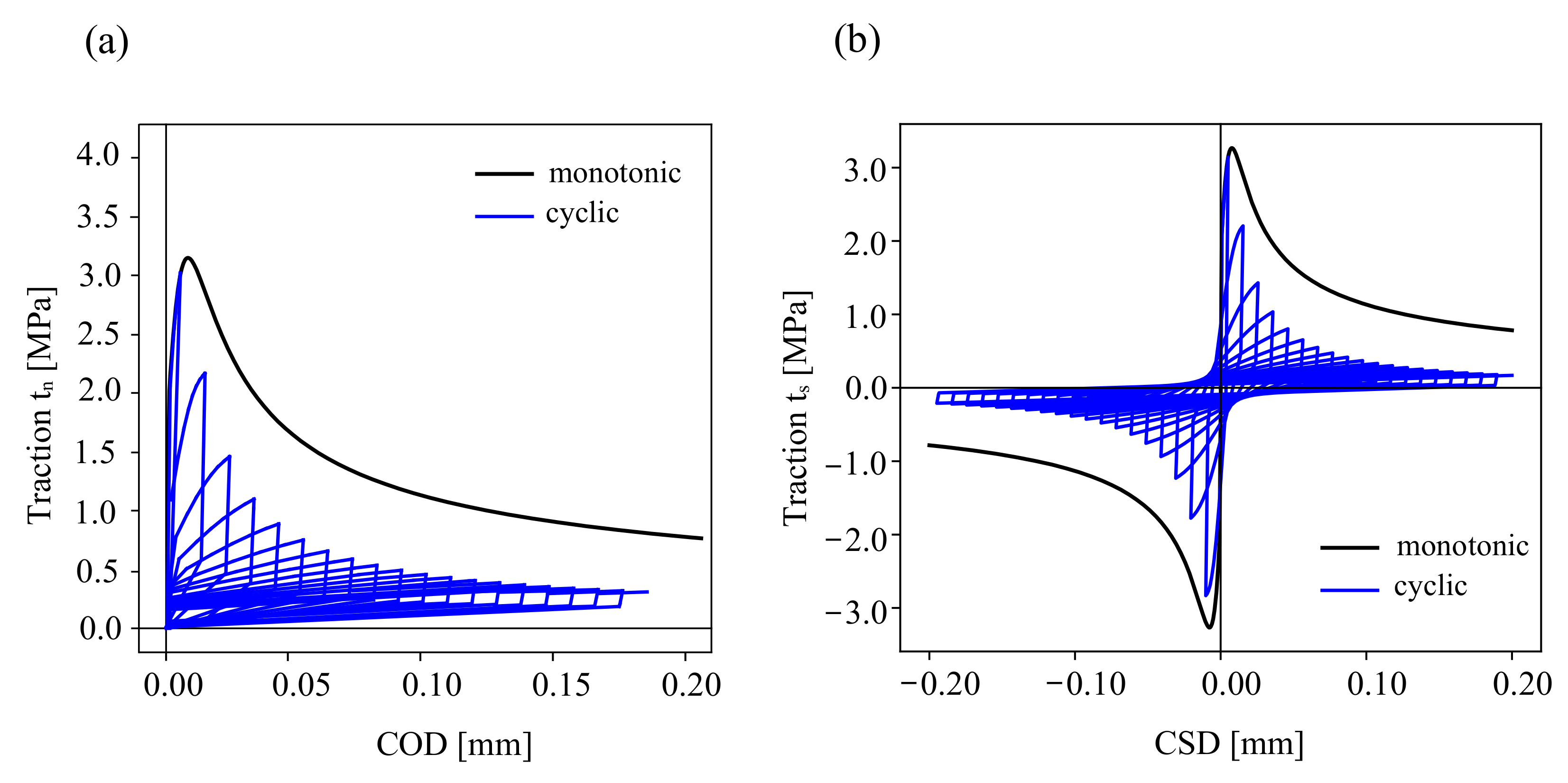
2. Constitutive Relation under Cyclic Loading
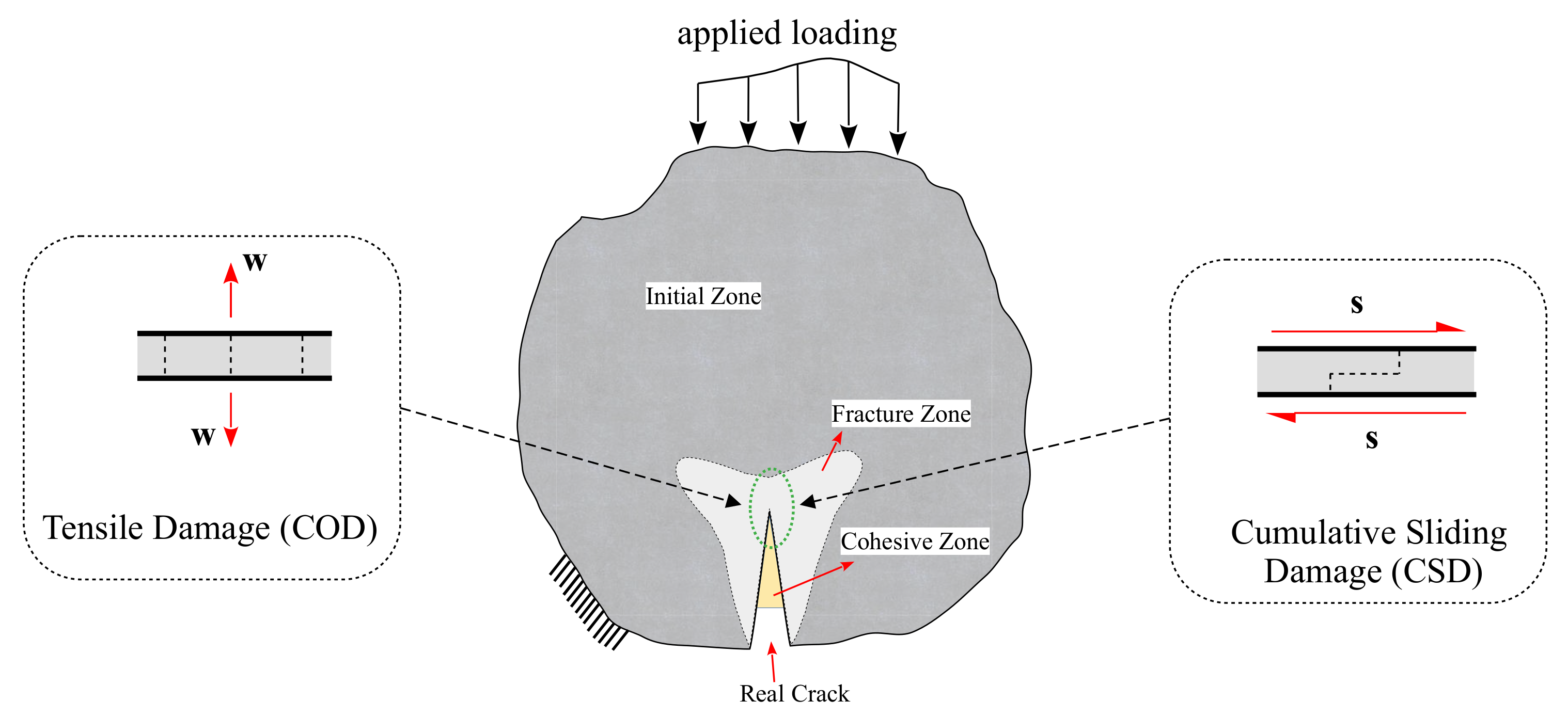
3. Modelling Crack Propagation
3.1. Crack Tip Stress Field in the Presence of Cohesive Traction
3.2. Crack Propagation
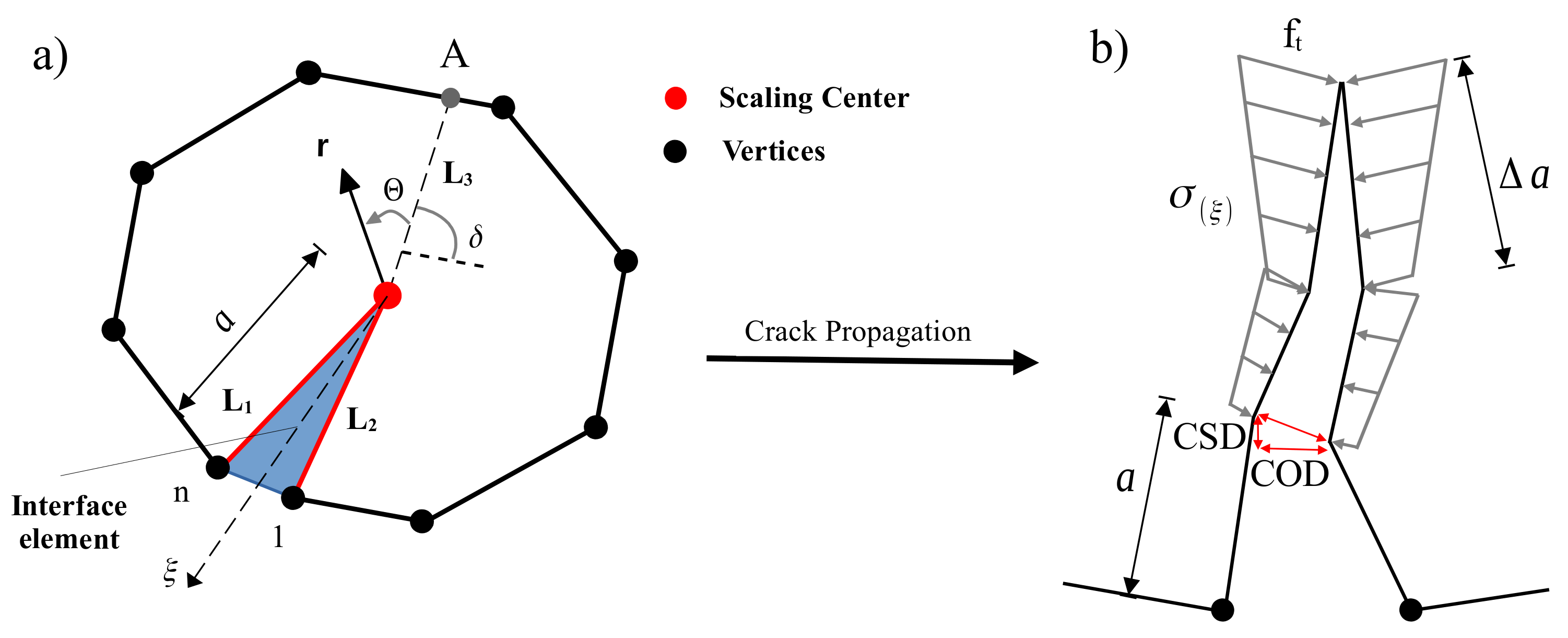
4. Implementation Procedure by SBFEM
- 1.
- Input the geometric dimensions of the specimen including; the span length L, height h, width b, and initial crack length a, along with the material parameters; the initial fracture toughness, Poisson’s ratio , Young’s modulus E, damage parameter , and the material plasticity and z under both static and cyclic loading P;
- 2.
- Establish the model (SBFEM) with the initial crack length a. Apply the external load P. Calculate the stress field of the domain, cohesive nodal traction, and the stress intensity factors (SIFs) and . Adjust the applied load until the initial cracking is reached;
- 3.
- Re-establish the SBFEM of the crack angle with crack length a. is the increment of crack length. If , the number of cyclic loading . Apply cyclic load and the cohesive force according to Equation (13). Finally, the single and mixed mode and for monotonic and cyclic crack propagation process can be calculated according to Equation (10);
- 4.
- Repeat step 3 until the structure fails and the numerical simulation is terminated. Output the necessary parameters, such as the crack propagation path, the number of cyclic loads N, and and displacements.
- (a)
- As shown in Figure 4, the linear elastic assumptions of SBFEM can be used to determine the relative displacement of the crack element when the structure is subject to the external force P. As a result, the corresponding cohesive traction can be acquired;
- (b)
- Both the external force and the cohesive force obtained in the previous step are applied to the structure, with the cohesive traction being applied in the form of a side-face force and formulated in accordance with Equation (13). Along the fracture process region, cohesive tractions are related to the relative opening and sliding displacements on the crack faces uwhere k is the stiffness of the softening laws.The stiffness matrix of an interface element in the local coordinate system is:where A is the crack surface area, is the one-dimensional Gaussian weight, is the number of integration points, and is the linear shape function matrix [33]. Based on Equation (14) the solution of the displacement and stress equations is calculated in Equations (7) and (8), respectively;
- (c)
- Proceed until the variation depicted in Figure 4 is consistent with the relationship between and .
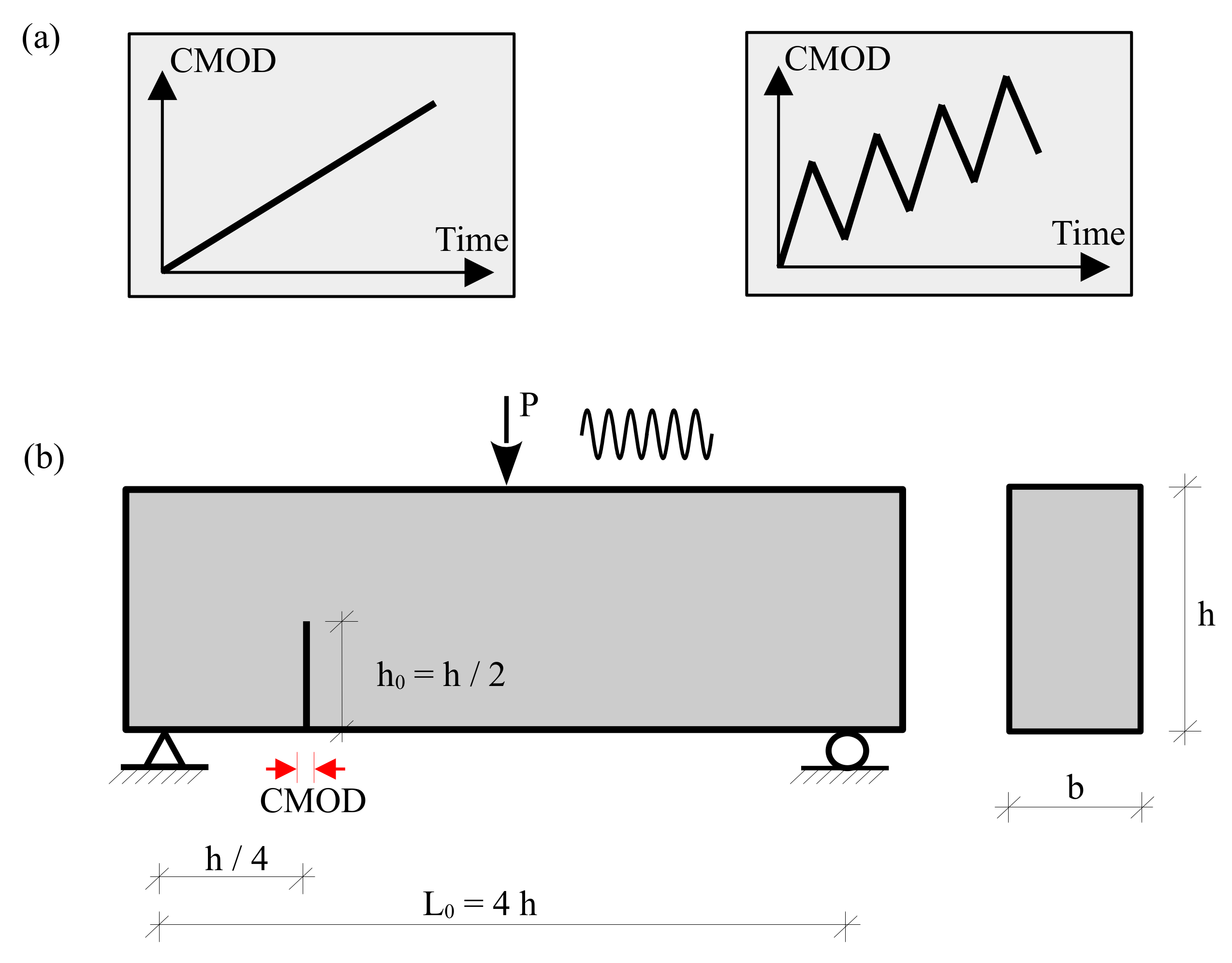
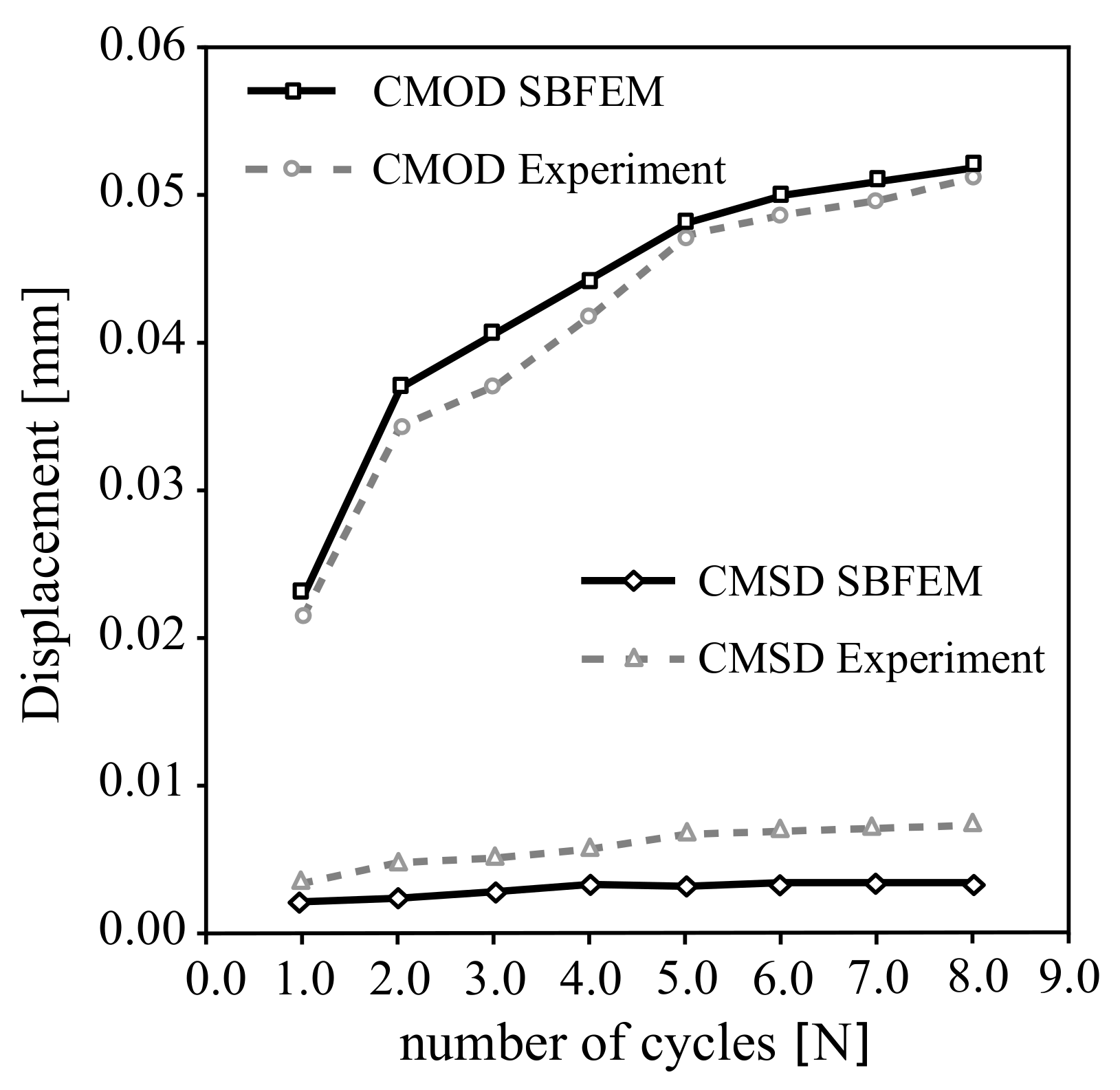
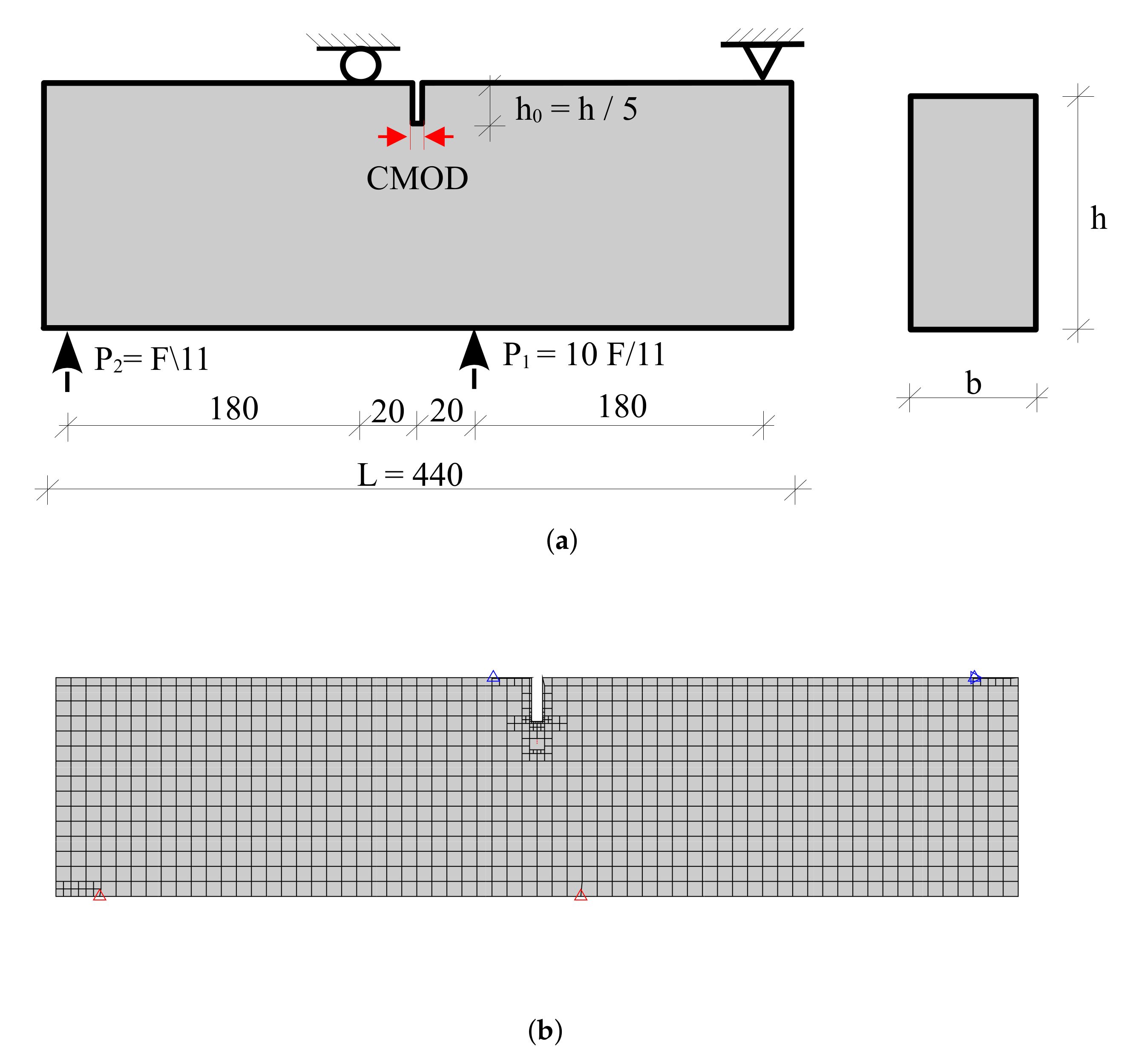
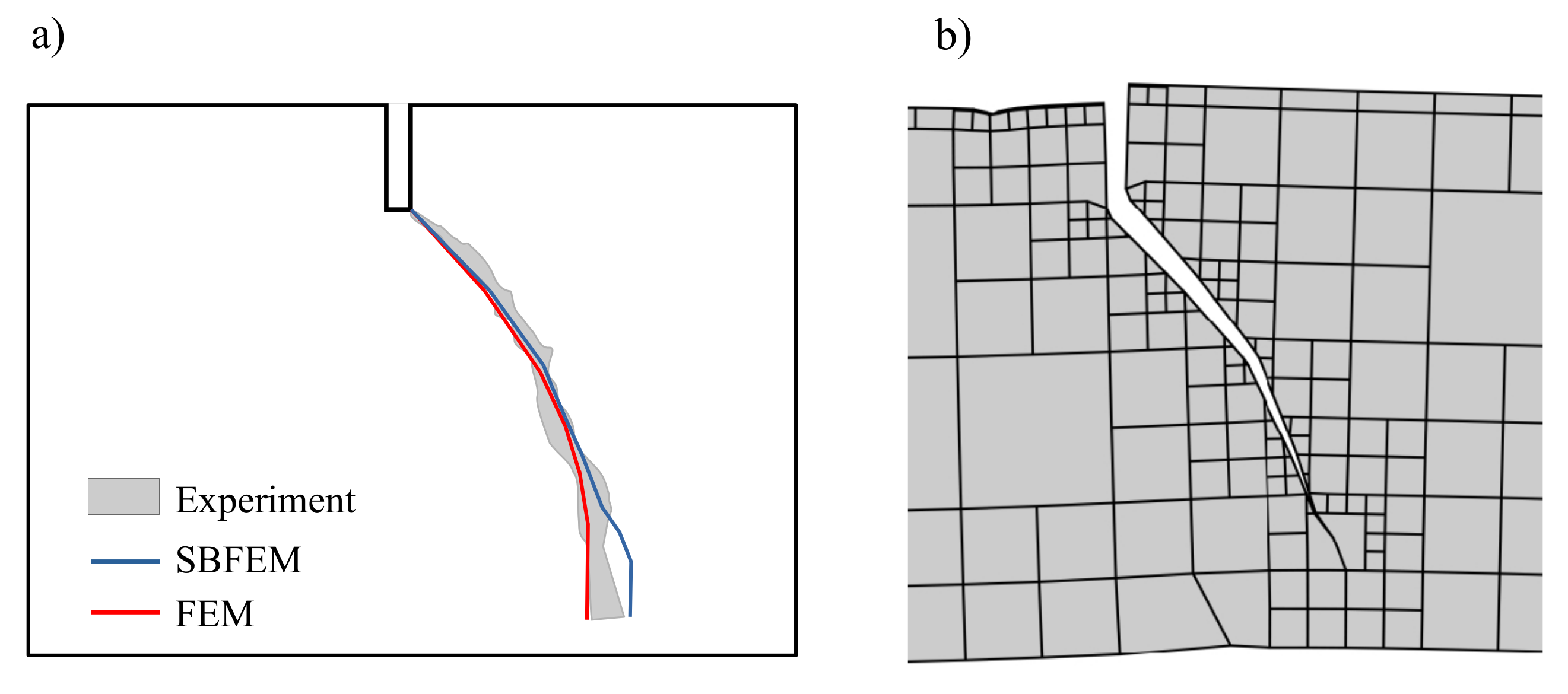
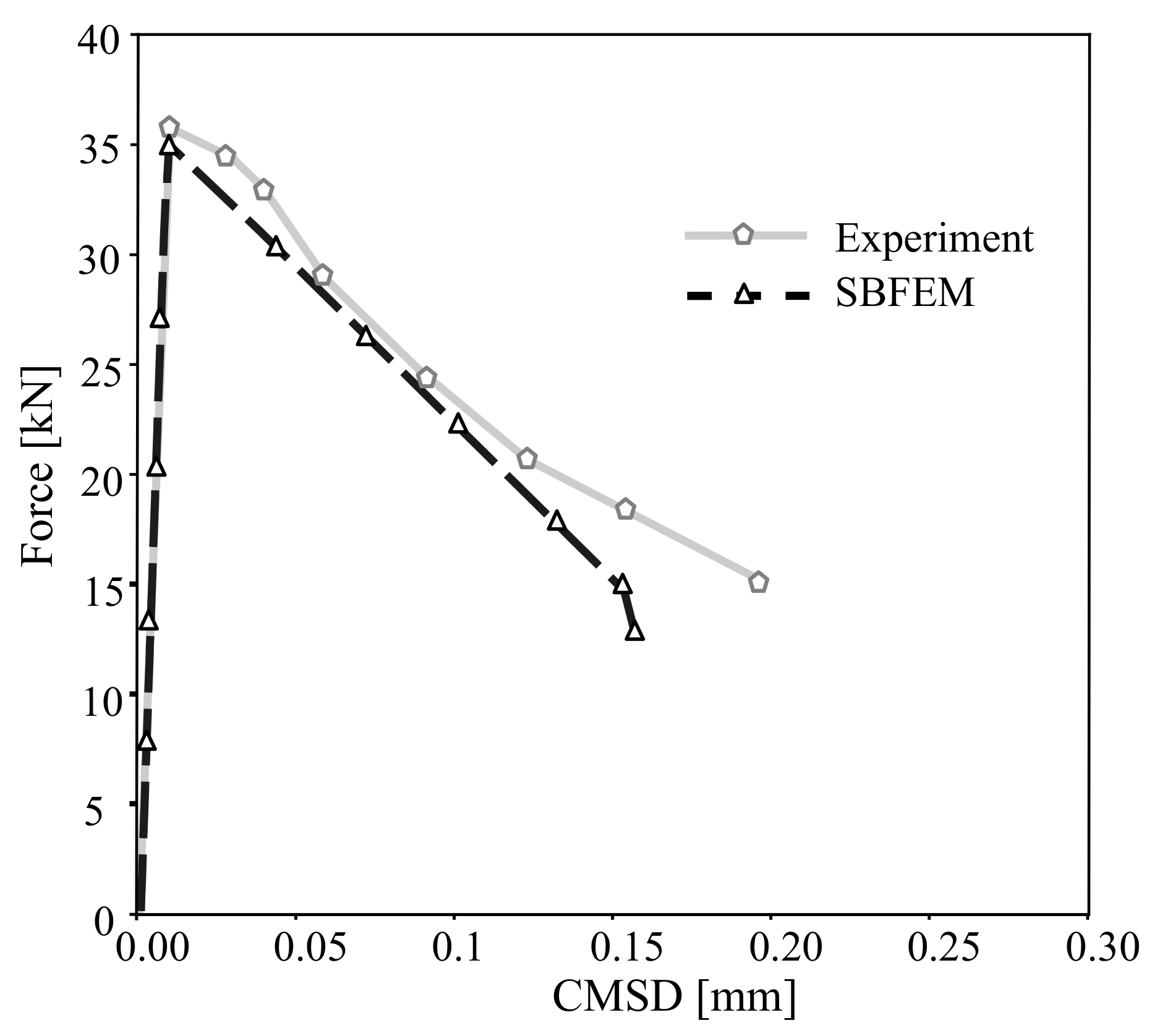
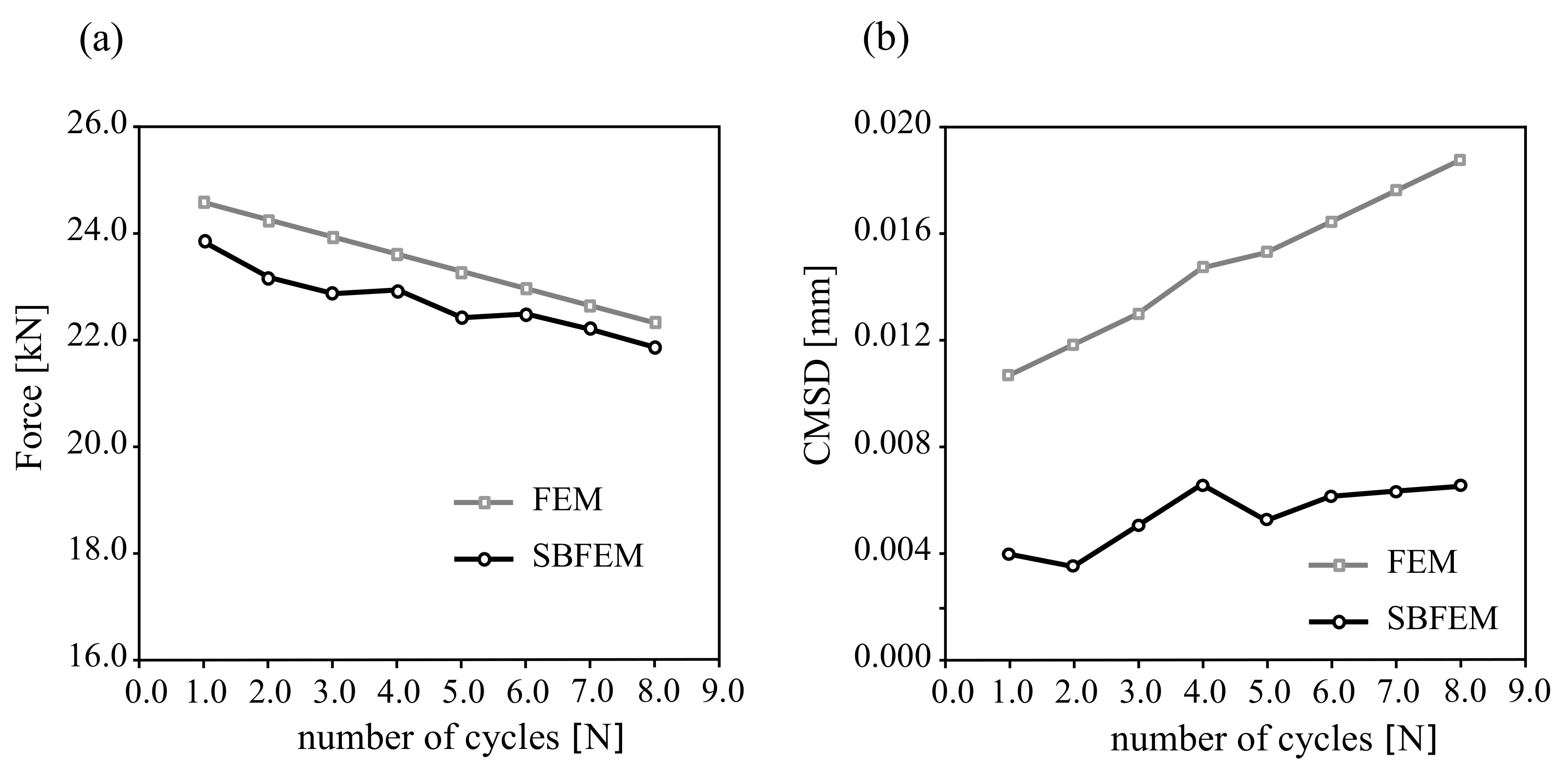
5. Numerical Simulation and Model Verification
5.1. Three-Point Bending Beam
5.2. Four-Point Bending Beam
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| Relative displacement | Damage variable | ||
| Crack opening at material point | Y | Energy release rate | |
| Crack sliding at material point | S | Damage strength parameter | |
| Exponential damage parameters | Effective stress limit | ||
| m | Material constant | E | Elastic stiffness |
| Hardening material variable | E | Elastic stiffness | |
| Cohesive normal stress | Cohesive tangential stress | ||
| Element stiffness of the interface | Isotropic and kinematic hardening moduli | ||
| Local coordinate system of SBFEM | Eigenvalue matrices | ||
| Displacement field | D | Material constitutive matrix | |
| Nodal shape function | Stiffens matrix of the domain | ||
| Eigenvector matrices | Integration constants of the SBFEM | ||
| Strain-displacement matrices of SBFEM system | M | Number of displacement modes | |
| P | External applied force | N | Number of load cycles |
| Crack propagation angle | Crack propagation length | ||
| Crack length | Stiffens matrix of interface element | ||
| A | Crack surface area | Gaussian weight function | |
| Crack mouth sliding displacement | Nodal side face load | ||
| Crack mouth opening displacement | Crack mode I & mode II stress intensity factors | ||
| Stress field |
References
- Baktheer, A.; Becks, H. Fracture mechanics based interpretation of the load sequence effect in the flexural fatigue behavior of concrete using digital image correlation. Constr. Build. Mater. 2021, 307, 124817. [Google Scholar] [CrossRef]
- Rosso, M.M.; Asso, R.; Aloisio, A.; Di Benedetto, M.; Cucuzza, R.; Greco, R. Corrosion effects on the capacity and ductility of concrete half-joint bridges. Constr. Build. Mater. 2022, 360, 129555. [Google Scholar] [CrossRef]
- Rots, J.G.; Nauta, P.; Kuster, G.; Blaauwendraad, J. Smeared crack approach and fracture localization in concrete. HERON 1985, 1985, 30. [Google Scholar]
- Blanco, N.; Gamstedt, E.K.; Asp, L.; Costa, J. Mixed-mode delamination growth in carbon–fibre composite laminates under cyclic loading. Int. J. Solids Struct. 2004, 41, 4219–4235. [Google Scholar] [CrossRef]
- Yang, B.; Mall, S.; Ravi-Chandar, K. A cohesive zone model for fatigue crack growth in quasibrittle materials. Int. J. Solids Struct. 2001, 38, 3927–3944. [Google Scholar] [CrossRef]
- Dekker, R.; van der Meer, F.; Maljaars, J.; Sluys, L. A cohesive XFEM model for simulating fatigue crack growth under mixed-mode loading and overloading. Int. J. Numer. Methods Eng. 2019, 118, 561–577. [Google Scholar] [CrossRef]
- Harper, P.W.; Hallett, S.R. A fatigue degradation law for cohesive interface elements – Development and application to composite materials. Int. J. Fatigue 2010, 32, 1774–1787. [Google Scholar] [CrossRef]
- Turon, A.; Costa, J.; Camanho, P.P.; Dávila, C.G. Simulation of Delamination Propagation in Composites under High-Cycle Fatigue by Means of Cohesive-Zone Models; Technical Report; NASA Langley Research Center: Hampton, VA, USA, 2006. Available online: https://ntrs.nasa.gov/search.jsp?R=20070004889 (accessed on 1 December 2006).
- Tracey, D. Finite elements for three-dimensional elastic crack analysis. Nucl. Eng. Des. 1974, 26, 282–290. [Google Scholar] [CrossRef]
- Pfister, T.; Petryna, Y.; Stangenberg, F. Damage modelling of reinforced concrete under multi-axial fatigue loading. In Computational Modelling of Concrete Structures; CRC Press: Boca Raton, FL, USA, 2006; pp. 421–429. [Google Scholar]
- Bittencourt, T.N.; Wawrzynek, P.; Ingraffea, A.; Sousa, J. Quasi-automatic simulation of crack propagation for 2D LEFM problems. Eng. Fract. Mech. 1996, 55, 321–334. [Google Scholar] [CrossRef]
- Hillerborg, A.; Modéer, M.; Petersson, P.E. Analysis of crack formation and crack growth in concrete by means of fracture mechanics and finite elements. Cem. Concr. Res. 1976, 6, 773–781. [Google Scholar] [CrossRef]
- Sato, Y.; Tadokoro, T.; Ueda, T. Diagonal tensile failure mechanism of reinforced concrete beams. J. Adv. Concr. Technol. 2004, 2, 327–341. [Google Scholar] [CrossRef]
- Segura, J.; Carol, I. Numerical modelling of pressurized fracture evolution in concrete using zero-thickness interface elements. Eng. Fract. Mech. 2010, 77, 1386–1399. [Google Scholar] [CrossRef]
- Xu, X.P.; Needleman, A. Numerical simulations of fast crack growth in brittle solids. J. Mech. Phys. Solids 1994, 42, 1397–1434. [Google Scholar] [CrossRef]
- Ruiz, G.; Pandolfi, A.; Ortiz, M. Three-dimensional cohesive modeling of dynamic mixed-mode fracture. Int. J. Numer. Methods Eng. 2001, 52, 97–120. [Google Scholar] [CrossRef]
- Su, X.; Yang, Z.; Liu, G. Finite element modelling of complex 3D static and dynamic crack propagation by embedding cohesive elements in Abaqus. Acta Mech. Solida Sin. 2010, 23, 271–282. [Google Scholar] [CrossRef]
- Xie, M.; Gerstle, W.H.; Rahulkumar, P. Energy-based automatic mixed-mode crack-propagation modeling. J. Eng. Mech. 1995, 121, 914–923. [Google Scholar] [CrossRef]
- Ciancio, D.; Carol, I.; Cuomo, M. Crack opening conditions at ‘corner nodes’ in FE analysis with cracking along mesh lines. Eng. Fract. Mech. 2007, 74, 1963–1982. [Google Scholar] [CrossRef]
- Khoei, A.; Barani, O.; Mofid, M. Modeling of dynamic cohesive fracture propagation in porous saturated media. Int. J. Numer. Anal. Methods Geomech. 2011, 35, 1160–1184. [Google Scholar] [CrossRef]
- Qiao, P.; Chen, Y. Cohesive fracture simulation and failure modes of FRP–concrete bonded interfaces. Theor. Appl. Fract. Mech. 2008, 49, 213–225. [Google Scholar] [CrossRef]
- Barsoum, R.S. Triangular quarter-point elements as elastic and perfectly-plastic crack tip elements. Int. J. Numer. Methods Eng. 1977, 11, 85–98. [Google Scholar] [CrossRef]
- Karihaloo, B.L.; Xiao, Q. Accurate determination of the coefficients of elastic crack tip asymptotic field by a hybrid crack element with p-adaptivity. Eng. Fract. Mech. 2001, 68, 1609–1630. [Google Scholar] [CrossRef]
- Prasad, M.; Krishnamoorthy, C. Computational model for discrete crack growth in plain and reinforced concrete. Comput. Methods Appl. Mech. Eng. 2002, 191, 2699–2725. [Google Scholar] [CrossRef]
- Moës, N.; Dolbow, J.; Belytschko, T. A finite element method for crack growth without remeshing. Int. J. Numer. Methods Eng. 1999, 46, 131–150. [Google Scholar] [CrossRef]
- Sancho, J.; Planas, J.; Gálvez, J.; Reyes, E.; Cendón, D. An embedded cohesive crack model for finite element analysis of mixed mode fracture of concrete. Fatigue Fract. Eng. Mater. Struct. 2006, 29, 1056–1065. [Google Scholar] [CrossRef]
- Unger, J.F.; Eckardt, S.; Könke, C. Modelling of cohesive crack growth in concrete structures with the extended finite element method. Comput. Methods Appl. Mech. Eng. 2007, 196, 4087–4100. [Google Scholar] [CrossRef]
- Song, C.; Wolf, J.P. The scaled boundary finite-element method—alias consistent infinitesimal finite-element cell method—for elastodynamics. Comput. Methods Appl. Mech. Eng. 1997, 147, 329–355. [Google Scholar] [CrossRef]
- Ooi, E.; Yang, Z. Modelling multiple cohesive crack propagation using a finite element–scaled boundary finite element coupled method. Eng. Anal. Bound. Elem. 2009, 33, 915–929. [Google Scholar] [CrossRef]
- Song, C.; Ooi, E.T.; Natarajan, S. A review of the scaled boundary finite element method for two-dimensional linear elastic fracture mechanics. Eng. Fract. Mech. 2018, 187, 45–73. [Google Scholar] [CrossRef]
- Wolf, J.P.; Song, C. The scaled boundary finite-element method—A fundamental solution-less boundary-element method. Comput. Methods Appl. Mech. Eng. 2001, 190, 5551–5568. [Google Scholar] [CrossRef]
- Ooi, E.; Song, C.; Tin-Loi, F.; Yang, Z. Automatic modelling of cohesive crack propagation in concrete using polygon scaled boundary finite elements. Eng. Fract. Mech. 2012, 93, 13–33. [Google Scholar] [CrossRef]
- Ooi, E.; Natarajan, S.; Song, C.; Ooi, E. Crack propagation modelling in concrete using the scaled boundary finite element method with hybrid polygon–quadtree meshes. Int. J. Fract. 2017, 203, 135–157. [Google Scholar] [CrossRef]
- Wang, Y.; Lin, G.; Hu, Z. A coupled FE and scaled boundary FE-approach for the earthquake response analysis of arch dam-reservoir-foundation system. In Proceedings of the IOP Conference Series: Materials Science and Engineering; IOP Publishing: Sydney, Australia, 2010; Volume 10, p. 012212. [Google Scholar]
- Liu, J.; Lin, G.; Li, J.; Zhong, H. Analysis of quadruple corner-cut ridged square waveguide using a scaled boundary finite element method. Appl. Math. Model. 2012, 36, 4797–4809. [Google Scholar] [CrossRef]
- Saputra, A.; Talebi, H.; Tran, D.; Birk, C.; Song, C. Automatic image-based stress analysis by the scaled boundary finite element method. Int. J. Numer. Methods Eng. 2017, 109, 697–738. [Google Scholar] [CrossRef]
- Ooi, E.T.; Song, C.; Tin-Loi, F.; Yang, Z. Polygon scaled boundary finite elements for crack propagation modelling. Int. J. Numer. Methods Eng. 2012, 91, 319–342. [Google Scholar] [CrossRef]
- Yang, Z.; Deeks, A. Fully-automatic modelling of cohesive crack growth using a finite element–scaled boundary finite element coupled method. Eng. Fract. Mech. 2007, 74, 2547–2573. [Google Scholar] [CrossRef]
- Egger, A.W.; Triantafyllou, S.P.; Chatzi, E.N. The scaled boundary finite element method for the efficient modeling of linear elastic fracture. In Proceedings of the 9th International Conference on Fracture Mechanics of Concrete and Concrete Structures, Berkley, CA, USA, 9 June 2016. [Google Scholar]
- Rabczuk, T.; Zi, G. A meshfree method based on the local partition of unity for cohesive cracks. Comput. Mech. 2007, 39, 743–760. [Google Scholar] [CrossRef]
- Baktheer, A.; Chudoba, R. Pressure-sensitive bond fatigue model with damage evolution driven by cumulative slip: Thermodynamic formulation and applications to steel-and FRP-concrete bond. Int. J. Fatigue 2018, 113, 277–289. [Google Scholar] [CrossRef]
- Becks, H.; Classen, M. Mode II Behavior of High-Strength Concrete under Monotonic, Cyclic and Fatigue Loading. Materials 2021, 14, 7675. [Google Scholar] [CrossRef]
- Ooi, E.; Shi, M.; Song, C.; Tin-Loi, F.; Yang, Z. Dynamic crack propagation simulation with scaled boundary polygon elements and automatic remeshing technique. Eng. Fract. Mech. 2013, 106, 1–21. [Google Scholar] [CrossRef]
- Baktheer, A.; Chudoba, R. Classification and evaluation of phenomenological numerical models for concrete fatigue behavior under compression. Constr. Build. Mater. 2019, 221, 661–677. [Google Scholar] [CrossRef]
- Baktheer, A.; Aguilar, M.; Hegger, J.; Chudoba, R. Microplane damage plastic model for plain concrete subjected to compressive fatigue loading. In Proceedings of the 10th International Conference on Fracture Mechanics of Concrete and Concrete Structures, FraMCoS-X, ayonne, France, 23–26 June 2019. [Google Scholar] [CrossRef]
- Aguilar, M.; Baktheer, A.; Chudoba, R. Numerical Investigation of Load Sequence Effect and Energy Dissipation in Concrete Due to Compressive Fatigue loading Using the New Microplane Fatigue Model MS1. In Proceedings of the XVI International Conference on Computational Plasticity. Fundamentals and Applications COMPLAS 2021, Barcelona, Spain, 7–10 September 2021. [Google Scholar] [CrossRef]
- Alrayes, O.; Könke, C.; Ooi, E.T.; Hamdia, K.M. Modeling Cyclic Crack Propagation in Concrete Using the Scaled Boundary Finite Element Method Coupled with the Cumulative Damage-Plasticity Constitutive Law. Materials 2023, 16, 863. [Google Scholar] [CrossRef]
- Alliche, A. Damage model for fatigue loading of concrete. Int. J. Fatigue 2004, 26, 915–921. [Google Scholar] [CrossRef]
- Petracca, M.; Pelà, L.; Rossi, R.; Zaghi, S.; Camata, G.; Spacone, E. Micro-scale continuous and discrete numerical models for nonlinear analysis of masonry shear walls. Constr. Build. Mater. 2017, 149, 296–314. [Google Scholar] [CrossRef]
- Desmorat, R.; Ragueneau, F.; Pham, H. Continuum damage mechanics for hysteresis and fatigue of quasi-brittle materials and structures. Int. J. Numer. Anal. Methods Geomech. 2007, 31, 307–329. [Google Scholar] [CrossRef]
- Baktheer, A.; Chudoba, R. Modeling of bond fatigue in reinforced concrete based on cumulative measure of slip. In Proceedings of the Computational Modelling of Concrete Structures, EURO-C 2018; CRC Press: Bad Hofgastein, Austria, 2018; pp. 767–776. [Google Scholar] [CrossRef]
- Baktheer, A.; Spartali, H.; Hegger, J.; Chudoba, R. High-cycle fatigue of bond in reinforced high-strength concrete under push-in loading characterized using the modified beam-end test. Cem. Concr. Compos. 2021, 118, 103978. [Google Scholar] [CrossRef]
- Lemaitre, J.; Desmorat, R. Engineering Damage Mechanics: Ductile, Creep, Fatigue and Brittle Failures; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2006. [Google Scholar]
- Baktheer, A.; Aguilar, M.; Chudoba, R. Microplane fatigue model MS1 for plain concrete under compression with damage evolution driven by cumulative inelastic shear strain. Int. J. Plast. 2021, 143, 102950. [Google Scholar] [CrossRef]
- Kobayashi, A.; Du, J. Fracture Process Zone Models for Concrete and Ceramics. In Computational Mechanics’ 86; Springer: Tokyo, Japan, 1986; pp. 987–993. [Google Scholar]
- Bazant, Z.P.; Li, Y.N. Stability of cohesive crack model: Part I—Energy principles. J. Appl. Mech. 1995, 62, 959–964. [Google Scholar] [CrossRef]
- Ooi, E.; Man, H.; Natarajan, S.; Song, C. Adaptation of quadtree meshes in the scaled boundary finite element method for crack propagation modelling. Eng. Fract. Mech. 2015, 144, 101–117. [Google Scholar] [CrossRef]
- Yang, Z. Fully automatic modelling of mixed-mode crack propagation using scaled boundary finite element method. Eng. Fract. Mech. 2006, 73, 1711–1731. [Google Scholar] [CrossRef]
- Benkemoun, N.; Poullain, P.; Al Khazraji, H.; Choinska, M.; Khelidj, A. Meso-scale investigation of failure in the tensile splitting test: Size effect and fracture energy analysis. Eng. Fract. Mech. 2016, 168, 242–259. [Google Scholar] [CrossRef]
- Jia, M.; Wu, Z.; Yu, R.C.; Zhang, X. Experimental and Numerical Study on Mixed Mode I–II Fatigue Crack Propagation in Concrete. J. Eng. Mech. 2022, 148, 04022044. [Google Scholar] [CrossRef]
- Chudoba, R.; Vořechovský, M.; Aguilar, M.; Baktheer, A. Coupled sliding–decohesion–compression model for a consistent description of monotonic and fatigue behavior of material interfaces. Comput. Methods Appl. Mech. Eng. 2022, 398, 115259. [Google Scholar] [CrossRef]
- Xu, Y.; Yuan, H. Computational analysis of mixed-mode fatigue crack growth in quasi-brittle materials using extended finite element methods. Eng. Fract. Mech. 2009, 76, 165–181. [Google Scholar] [CrossRef]
- Van Mier, J.; Schlangen, E.; Visser, J.; Vervuurt, A. Experimental and numerical analysis of cracking in concrete and sandstone. In Topics in Applied Mechanics; Springer: Dordrecht, The Netherlands, 1993; pp. 65–72. [Google Scholar]


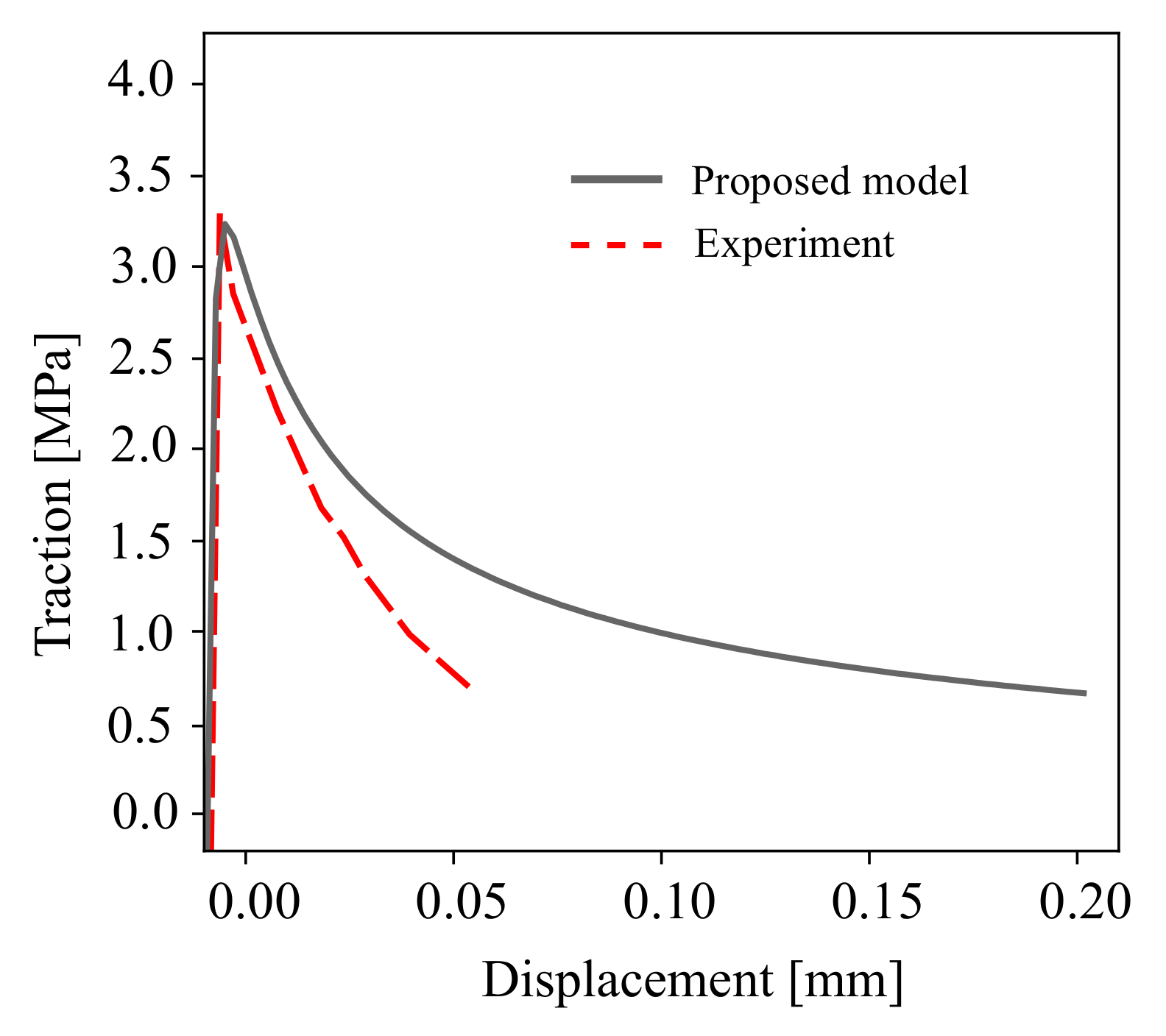
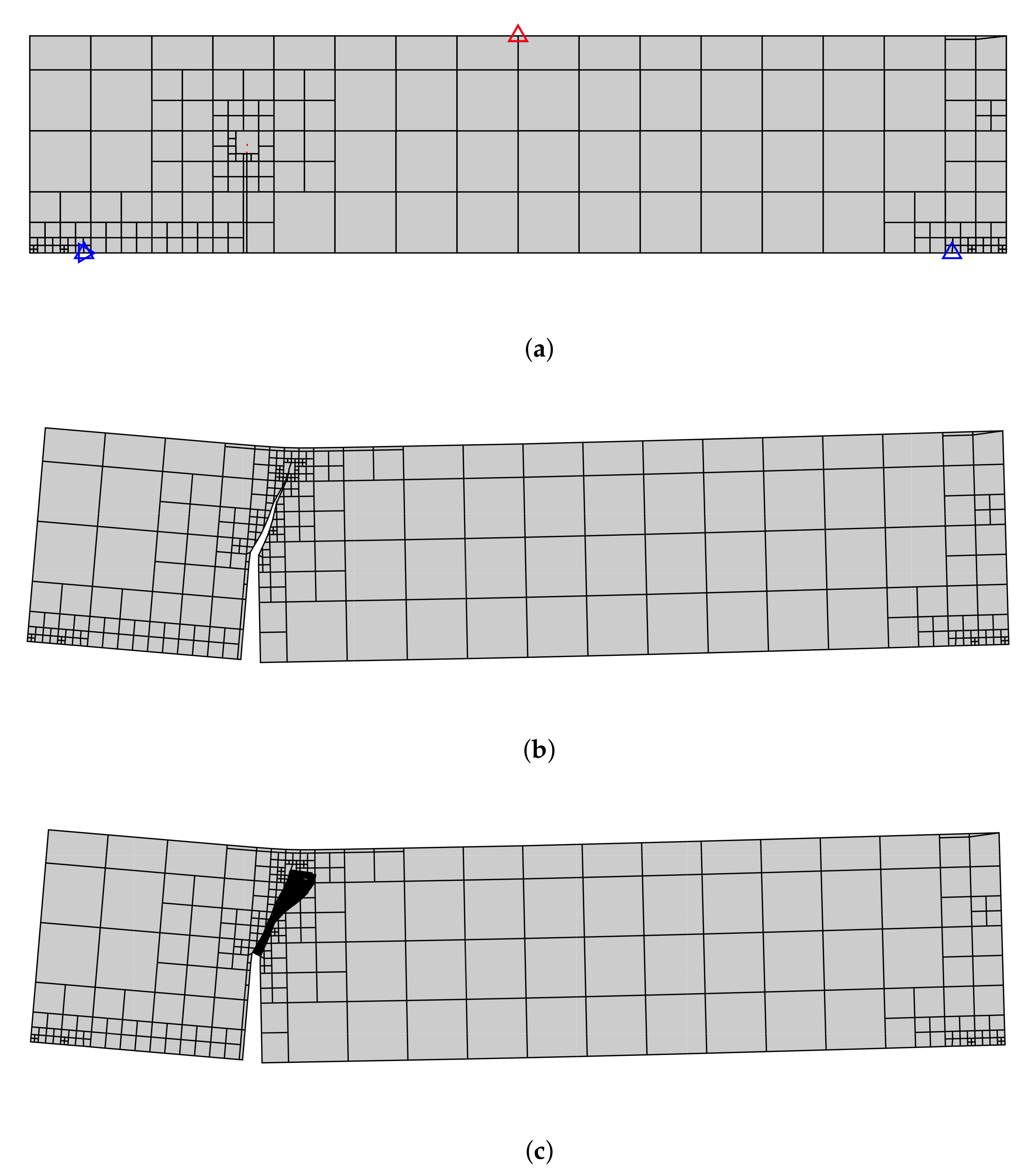
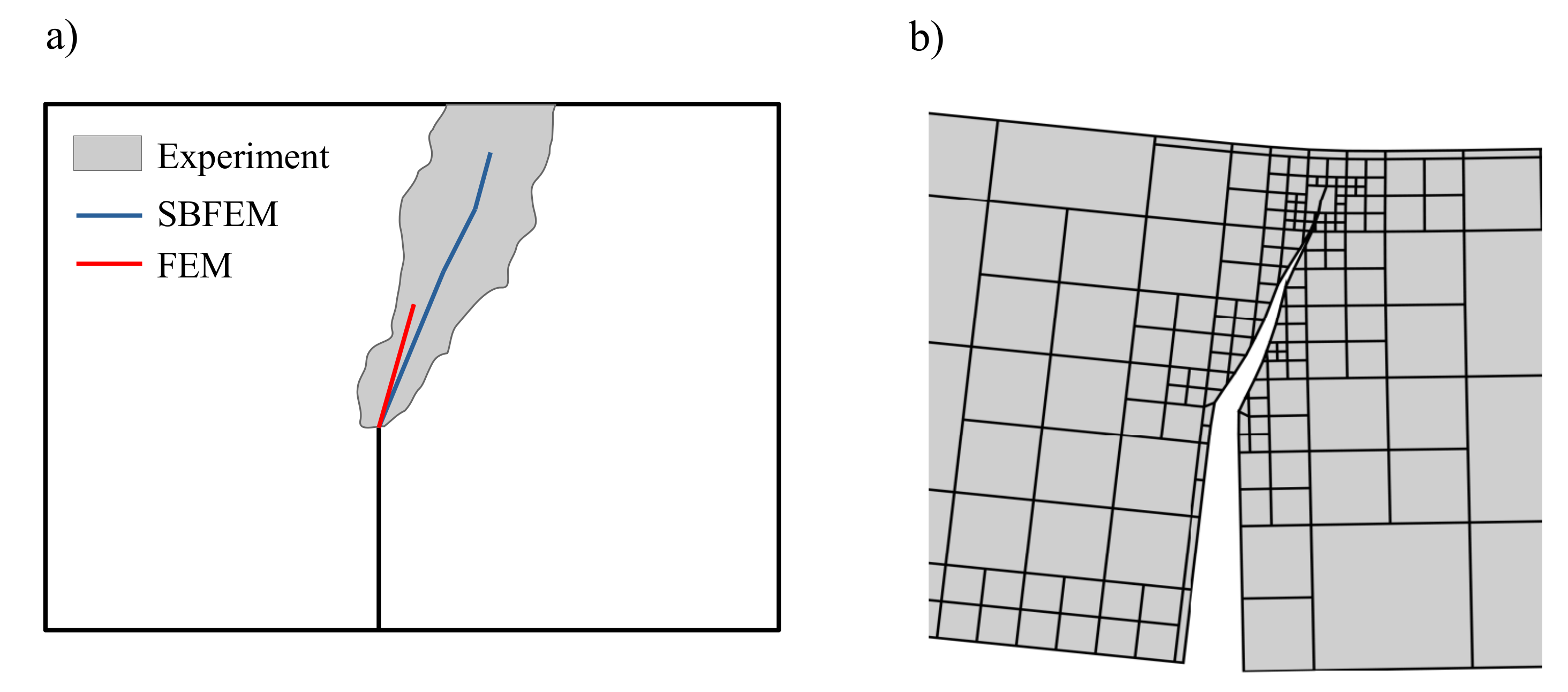
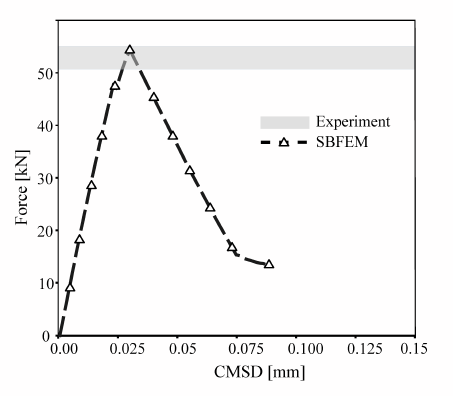


| Parameter | Denomination | Value | Unit |
|---|---|---|---|
| Compressive strength | 44.24 | [MPa] | |
| Tensile strength | 3.35 | [MPa] | |
| Young’s Modulus | 35.38 | [GPa] | |
| Poisson ratio | 0.21 | [-] |
| Parameter | Denomination | Value | Unit |
|---|---|---|---|
| E | Elastic cohesive modulus | 3000 | [MPa] |
| Reversibility limit | 2.0 | [MPa] | |
| K | Isotropic hardening modulus | 400.0 | [MPa] |
| Kinematic hardening modulus | 500.0 | [MPa] | |
| S | Damage strength | 0.25 × | [MPa] |
| r | Damage accumulation parameter | 1.0 | [-] |
| c | Damage accumulation parameter | 2.0 | [-] |
| Parameter | Denomination | Value | Unit |
|---|---|---|---|
| Tensile strength | 3.44 | [MPa] | |
| fracture energy | 0.126 | [N/mm] | |
| Young’s Modulus | 30.0 | [GPa] | |
| Poisson ratio | 0.20 | [-] |
| Parameter | Denomination | Value | Unit |
|---|---|---|---|
| E | Elastic cohesive modulus | 3500 | [MPa] |
| Reversibility limit | 2.0 | [MPa] | |
| K | Isotropic hardening modulus | 400.0 | [MPa] |
| Kinematic hardening modulus | 500.0 | [MPa] | |
| S | Damage strength | 0.25 × | [MPa] |
| r | Damage accumulation parameter | 1.0 | [-] |
| c | Damage accumulation parameter | 2.0 | [-] |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Alrayes, O.; Könke, C.; Hamdia, K.M. A Numerical Study of Crack Mixed Mode Model in Concrete Material Subjected to Cyclic Loading. Materials 2023, 16, 1916. https://doi.org/10.3390/ma16051916
Alrayes O, Könke C, Hamdia KM. A Numerical Study of Crack Mixed Mode Model in Concrete Material Subjected to Cyclic Loading. Materials. 2023; 16(5):1916. https://doi.org/10.3390/ma16051916
Chicago/Turabian StyleAlrayes, Omar, Carsten Könke, and Khader M. Hamdia. 2023. "A Numerical Study of Crack Mixed Mode Model in Concrete Material Subjected to Cyclic Loading" Materials 16, no. 5: 1916. https://doi.org/10.3390/ma16051916
APA StyleAlrayes, O., Könke, C., & Hamdia, K. M. (2023). A Numerical Study of Crack Mixed Mode Model in Concrete Material Subjected to Cyclic Loading. Materials, 16(5), 1916. https://doi.org/10.3390/ma16051916








