The Application of Mott’s Distribution in the Fragmentation of Steel Coaxial Cylinders
Abstract
:1. Introduction
2. Mathematical Deduction of Fragment Distribution Law
3. Critical Analysis of the Simplifying Assumptions
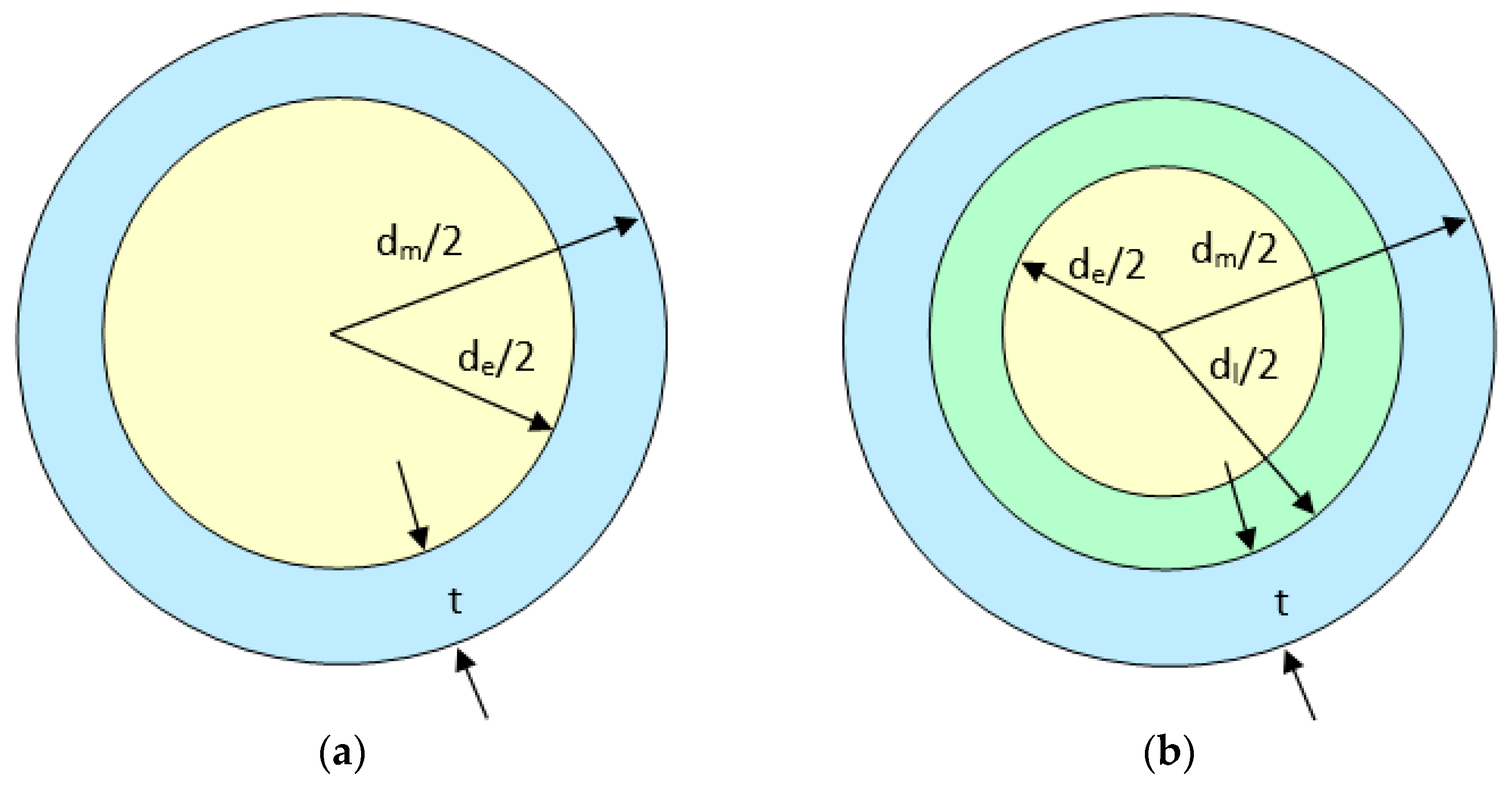
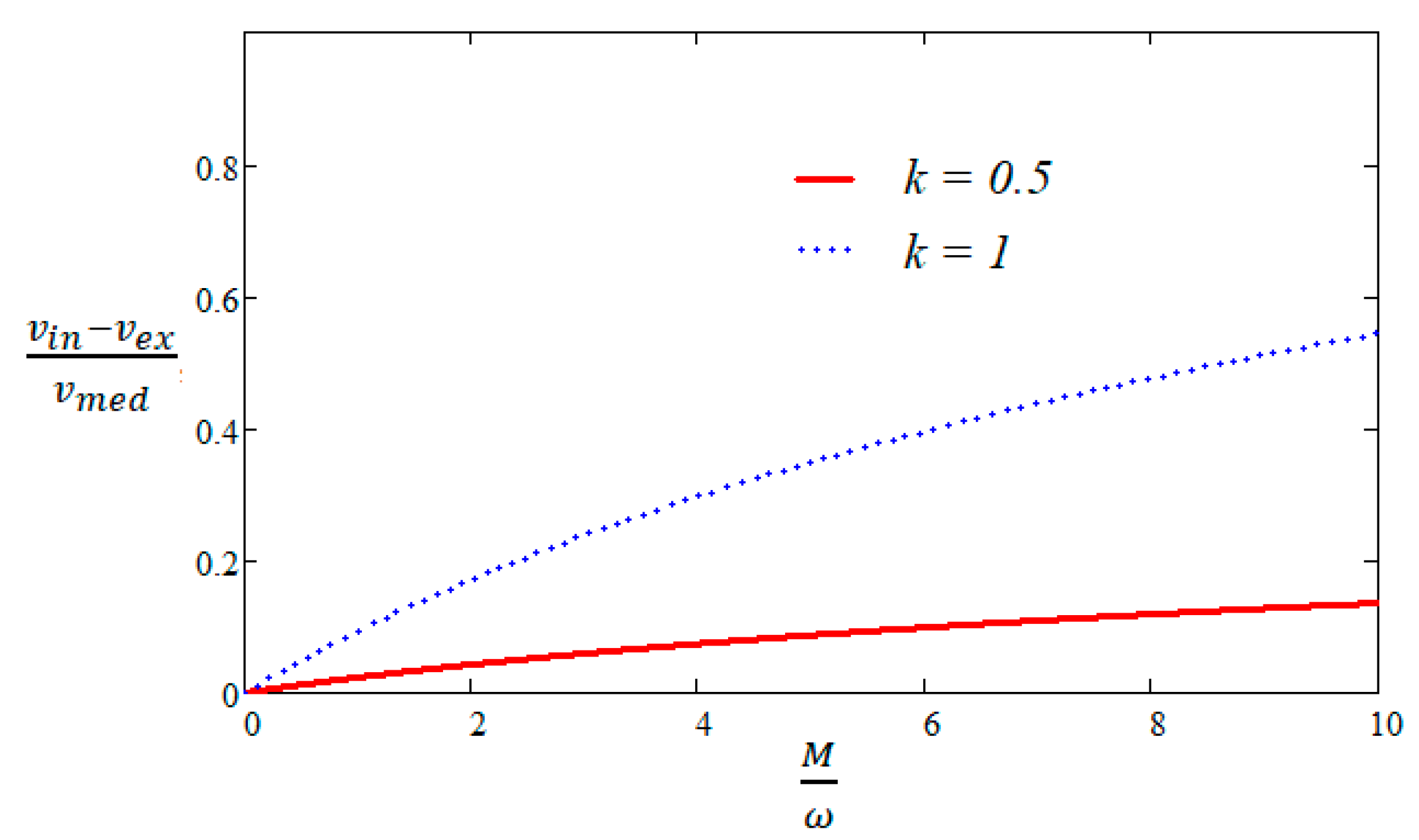
3.1. Equal Radial Velocity for All Cylinders
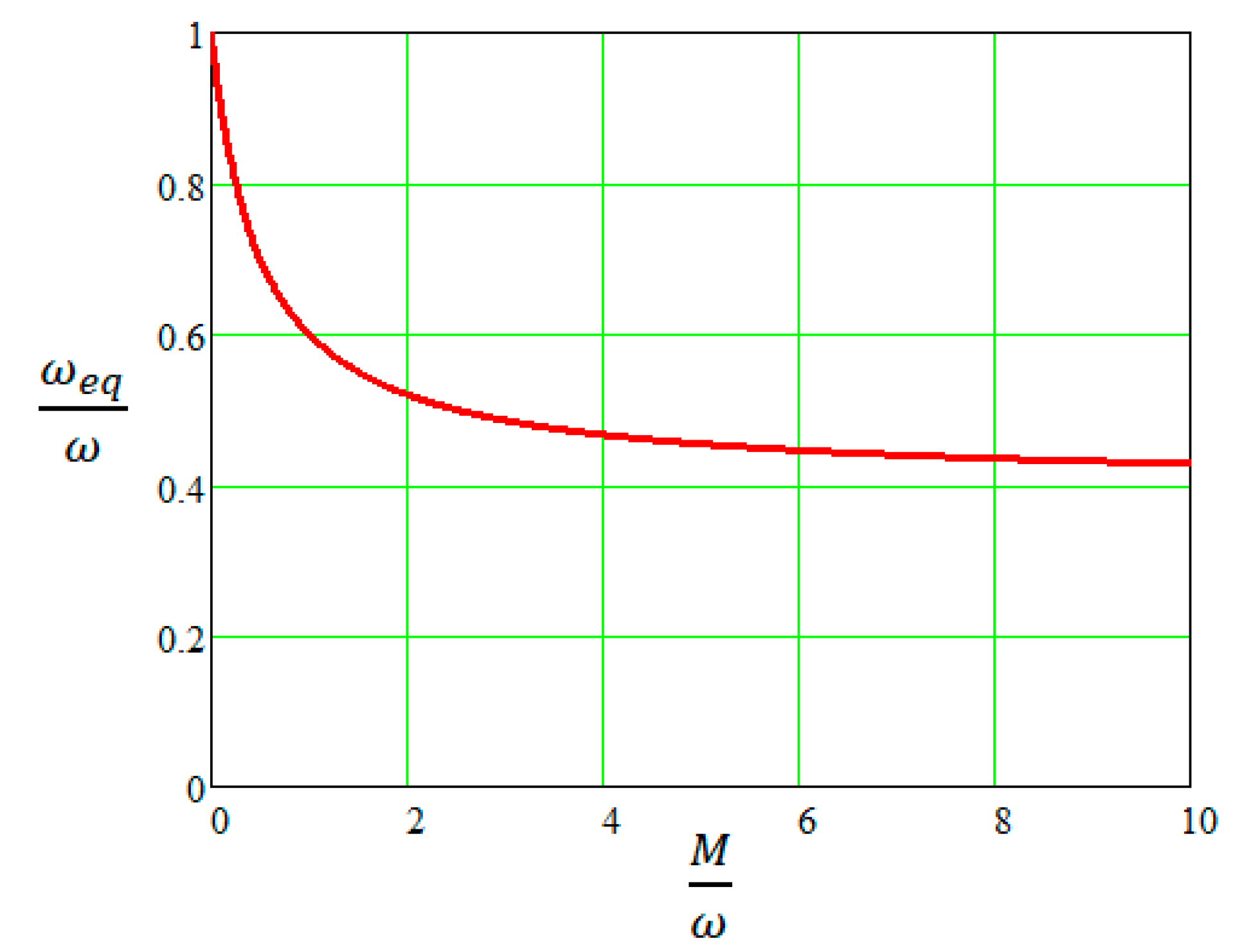
3.2. The Existence of the Characteristic
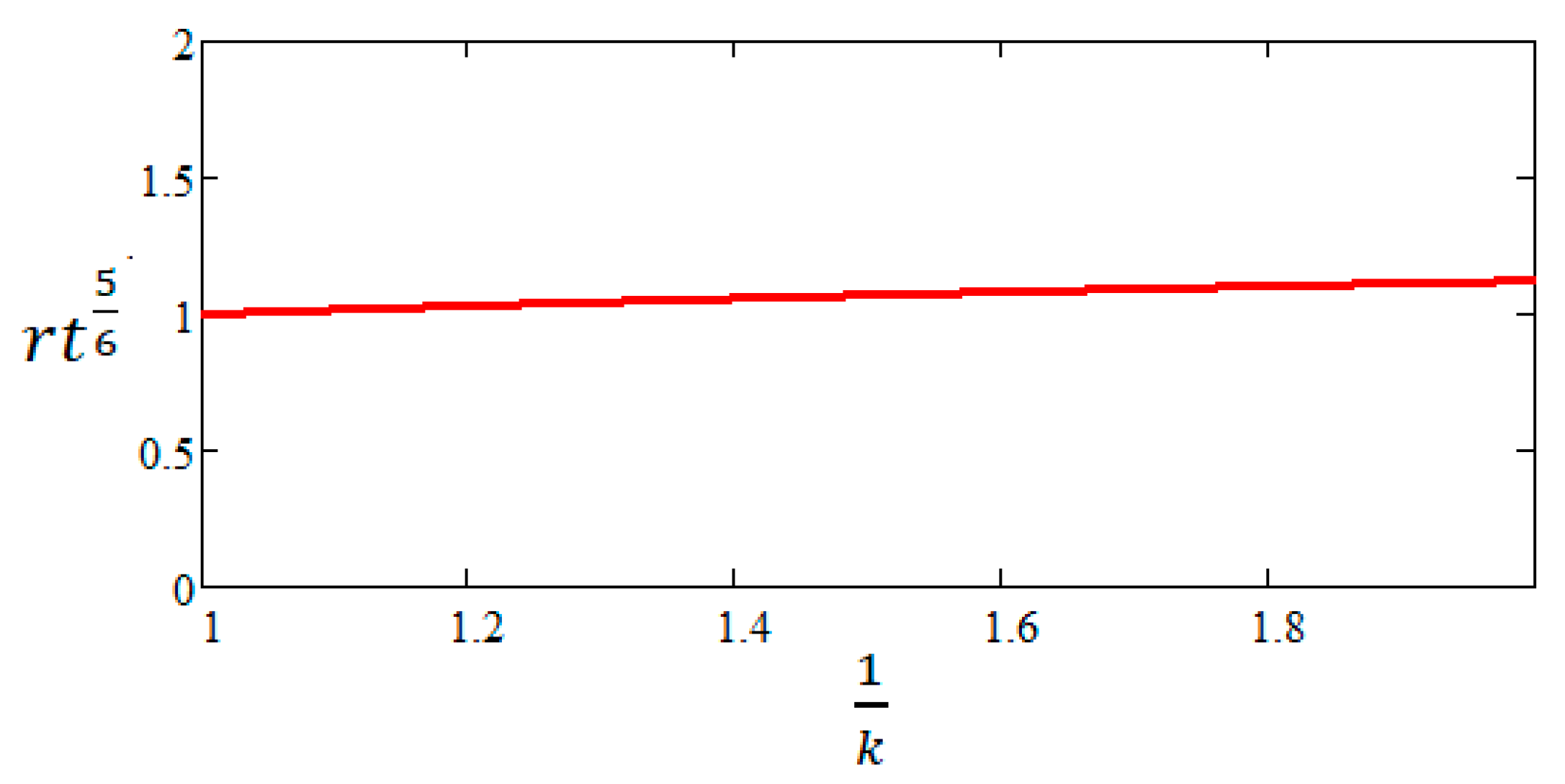
3.3. Using the Initial Values of r and t in Relation (12)
3.4. No Interference between the Cylinders’ Fragmentations Processes
4. Case Study on Structures with Two and Three Coaxial Cylinders
5. Conclusions
- (1)
- The fragmentation of several coaxial cylindrical bodies under the action of gases produced by detonation can be theoretically approached by adapting the theoretical model proposed by Mott for the fragmentation of a single cylindrical body. For this purpose, two additional hypotheses were used: equal radial velocity for all cylinders, and no interference between the fragmentation processes of the cylinders.
- (2)
- Thus, the formulas obtained show that in the calculation of the fragments’ mass scale parameters for each individual body, the influence of the other bodies is made through the dimensionless term that represents the ratio between the mass of the body in question and the total mass of all bodies.
- (3)
- For all simplifying hypotheses used in the development of the model, a justification analysis was presented.
- (4)
- For the “no interference” hypothesis, the results of a series of 2D planar numerical simulations were used. It was highlighted that the fragmentation process of two coaxial cylinders is not simultaneous, and there are some mutual influences; however, it can be approximated by accumulating the results obtained for each cylinder fragmented separately when the influence of the other cylinder fragmentation is cancelled by eliminating the failure and erosion criteria from the assigned material model. Such a result supports the use of the assumed hypothesis of the cylinders’ independent fragmentation.
- (5)
- Even if in the simulations the stochastic variance option was used in the plastic strain failure criterion to obtain an uneven distribution of the failure threshold, this approach fails to take in account the effect of the stress triaxiality on the value of the equivalent strain to fracture.
- (6)
- Theoretical calculations for configurations with two or three cylindrical bodies that have equal masses or equal wall thicknesses showed that the fragment mass distributions can be approximated by the generalized relation (21) when there are no large discrepancies between the cylindrical bodies in terms of their dimensions.
- (7)
- When there are major differences between the cylinders, Formula (16) is used to calculate the fragments’ mass distribution.
- (8)
- Although it does not fall into the category of controlled fragmentation solutions, in the absence of elements such as notches on the surfaces, which is why it does not have the same potential, the natural fragmentation of several coaxial cylinders can bring benefits from the point of view of terminal ballistics. Such a solution is justified to be used to the detriment of the single-body solution when it leads to a higher number of fragments that have a mass greater than , the minimum mass for which the fragment is relevant.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Kritzinger, H.H.; Stuhlmann, F. Artillerie und Ballistik in Stichworten; Springer: Berlin/Heidelberg, Germany, 1939. [Google Scholar]
- Mock, W.; Holt, W.H. Fragmentation behavior of Armco iron and HF1 steel explosive filled cylinders. J. Appl. Phys. 1983, 54, 2344–2351. [Google Scholar] [CrossRef]
- Justrow, K. Theorie der Splitterwirkung von Granaten, Wurfminen und ähnlicher Sprengkörper. In Technik und Wehrmacht; Mittler & Sohn: Berlin, Germany, 1921. [Google Scholar]
- Rosin, P.; Rammler, E. The laws governing the fineness of powdered coal. J. Inst. Fuel 1933, 7, 29–36. [Google Scholar]
- Mott, N.F. Fragmentation of H.E. Shells a Theoretical Formula for the Distribution of Weights of Fragments. In Fragmentation of Rings and Shells; A.O.R.G. Memorandum 24 Rep. no. AC3642; Springer: Berlin/Heidelberg, Germany, 1943. [Google Scholar]
- Lienau, C.C. Random fracture of a brittle solid. J. Frankl. Inst. 1936, 221, 769–787. [Google Scholar] [CrossRef]
- Grady, D.E. Fragmentation of rings and shells. In The Legacy of N.F. Mott; Springer: Berlin/Heidelberg, Germany, 2006. [Google Scholar]
- Grady, D.E.; Kipp, M.E. Geometric Statistics and Dynamic Fragmentation. J. Appl. Phys. 1985, 58, 1210–1222. [Google Scholar] [CrossRef]
- Elek, P.; Jaramaz, S. Fragment mass distribution of naturally fragmenting warheads. FME Trans. 2009, 37, 129–135. [Google Scholar]
- Odintsov, V.A. Hyper exponential Spectra of the Explosive Fracture of Metallic Cylinders. MTT 1992, 5, 48–55. [Google Scholar]
- Strømsøe, E.; Ingebrigtsen, K.O. A Modification of the Mott Formula for prediction of the fragment size distribution. PEP 1987, 12, 175–178. [Google Scholar] [CrossRef]
- Ahmed, K.; Malik, A.Q.; Hussain, A.; Ahmad, I.R.; Ahmad, I. Blast and Fragmentation Studied of a scaled down artillery shell-simulation and experimental approaches. Int. Jnl. Multiphys. 2021, 15, 49–71. [Google Scholar]
- Elshenawy, T.; Zaky, M.G.; Elbeih, A. Experimental and numerical studies of fragmentation shells flled with advanced HMX-plastic explosive compared to various explosive charges. Braz. J. Chem. Eng. 2023, 40, 481–492. [Google Scholar] [CrossRef]
- Nastasescu, V.; Marzavan, S. An overview of functionally graded material models. Proc. Rom. Acad. 2022, 23, 259–267. [Google Scholar]
- Trană, E.; Bucur, F.; Rotariu, A.N. On the fragmentation of explosively-driven plastic/steel layered cylinders. Mater. Plast. 2018, 55, 521–523. [Google Scholar] [CrossRef]
- Gurney, R.W. The Initial Velocities of Fragments from Bombs, Shell, and Grenades; BRL Report Number 405; Balistic Research Laboratory: Adelphi, MD, USA, 1943. [Google Scholar]
- Needham, C.E. Blast Waves; Springer: Berlin/Heidelberg, Germany, 2010. [Google Scholar]
- Fisher, E.M. The Effect of the Steel Case on the Air Blast from High Explosives; NAVORD Report 2753; Naval Ordnance Lab.: White Oak, MD, USA, 1953. [Google Scholar]
- Crowley, A.B. The effect of munition casings on reducing blast overpressures. In Proceedings of the Insensitive Munitions and Energetic Materials Technical Symposium, Bristol, UK, 24–28 April 2006. [Google Scholar]
- Wilkinson, C.R.; Andersonn, J.G. An Introduction to Detonation and Blast for the Non-Specialist; Australian Department of Defence location: Bungendore, Australia, 2003. [Google Scholar]
- Dunnett, J.; Flynn, D.; Wharton, J. Blast algorithm development: Definition of modified blast algorithms for PBX based explosives. In Proceedings of the Insensitive Munitions and Energetic Materials Technical Symposium IMEMTS, Bristol, UK, 24–28 April 2006. [Google Scholar]
- Locking, P.M.; Flynn, D.; Dunnett, J. Warhead Filling and Casing Interactions Affect the Blast Field Performance. In Proceedings of the 24th International Ballistics Symposium, New Orleans, LA, USA, 22–26 September 2008. [Google Scholar]
- Fikus, B. Preliminary Numerical Estimation of Selected Terminal Ballistic Effects Produced by Explosion of Mortar Projectile. Probl. Mechatron. Armament Aviat. Saf. Eng. 2018, 9, 49–60. [Google Scholar] [CrossRef]
- Zhang, X.T.; Jia, G.H.; Huang, H. A New Concept for Material Fragmentation Simulation—Nodes Separation. AMR 2010, 160–162, 558–563. [Google Scholar] [CrossRef]
- Ugrcic, M.; Ivanisevic, M. Characterization of the natural fragmentation of explosive ordnance using the numerical techniques based on the FEM. Sci. Tech. Rev. 2015, 65, 16–27. [Google Scholar] [CrossRef]
- Cullis, I.G.; Dunsmore, P.; Harrison, A.; Lewtas, I.; Townsley, R. Numerical simulation of the natural fragmentation of explosively loaded thick walled cylinders. Def. Technol. 2014, 10, 198–210. [Google Scholar] [CrossRef]
- Moxnes, J.F.; Borve, S. Simulation of natural fragmentation of rings cut from warheads. Def. Technol. 2015, 11, 319–329. [Google Scholar] [CrossRef]
- AUTODYN Theory Manual, Century Dynamics. 2005. Available online: https://www.researchgate.net/profile/Alireza_Rashiddel/post/Is_there_a_way_to_implement_a_large_strain_viscoelastic_material_model_in_AUTODYN/attachment/5f85890de66b860001a8503c/AS%3A946132159045637%401602586893515/download/0-theory_manual_AUTODYN.pdf (accessed on 15 June 2023).
- Trană, E.; Bucur, F.; Rotariu, A.N. Numerical simulation study on the ring fragmentation. In Proceedings of the International Conference ‘Greener and Safer Energetic and Ballistic Systems’, Bucharest, Romania, 22–23 May 2015. [Google Scholar]
- Taylor, G.I. The fragmentation of tubular bombs. In Scientific Papers of G.I. Taylor; Batchelor, G.K., Ed.; Cambridge University Press: Cambridge, UK, 1963; Volume 3, pp. 387–390. [Google Scholar]
- Al-Hassani, S.T.S.; Hopkins, H.G.; Johnson, W. A note on the fragmentation of tubular bombs. Int. J. Mech. Sci. 1969, 11, 545–549. [Google Scholar] [CrossRef]
- Zhao, C.; Wang, S.; Guo, C.; Liu, D.; Ma, F. Experimental study on fragmentation of explosive loaded steel projectile. Int. J. Impact Eng. 2020, 144, 103610. [Google Scholar] [CrossRef]
- Bao, Y.; Wierzbicki, T. On fracture locus in the equivalent strain and stress triaxiality space. Int. J. Mech. Sci. 2004, 46, 81–98. [Google Scholar] [CrossRef]
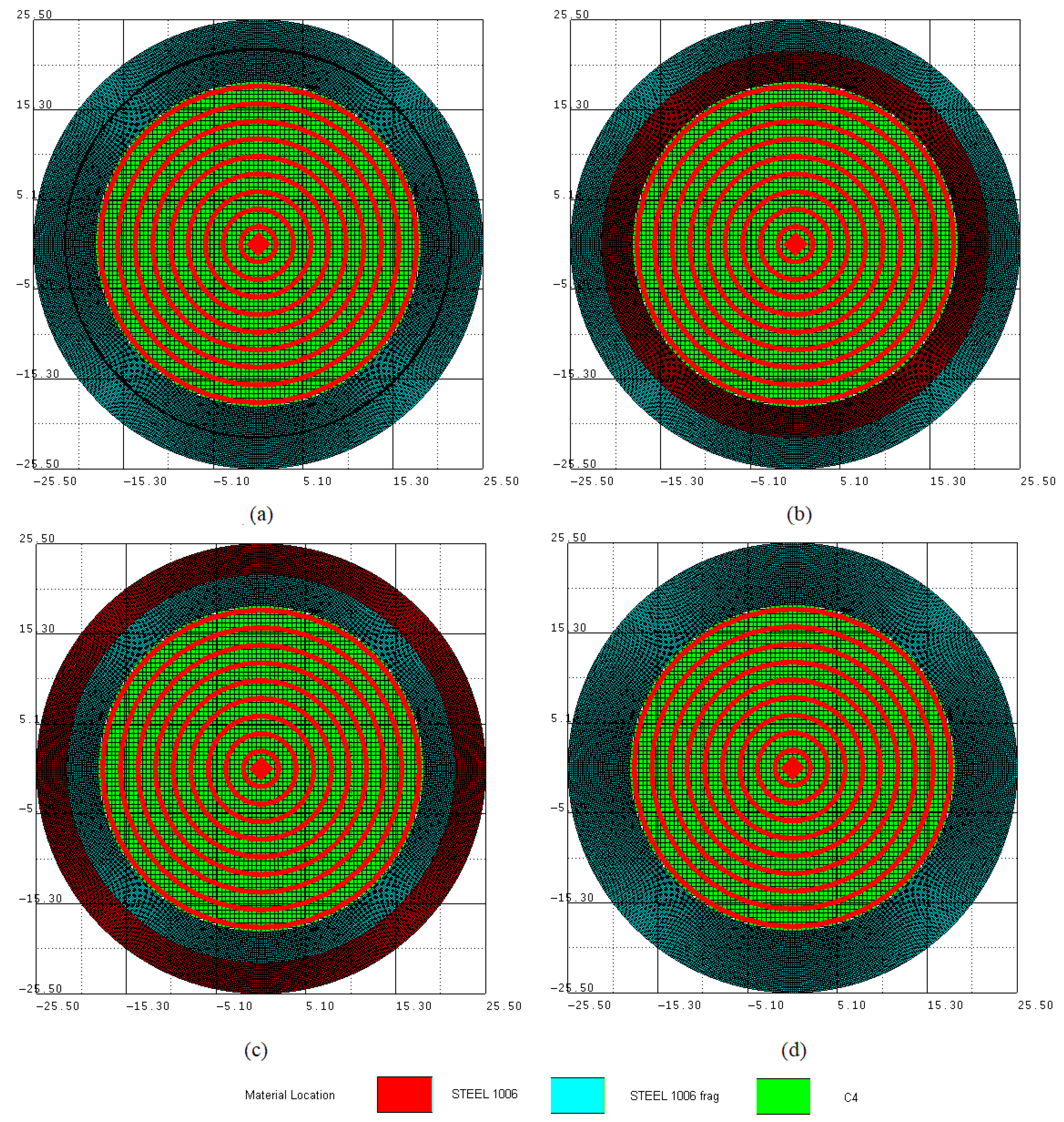
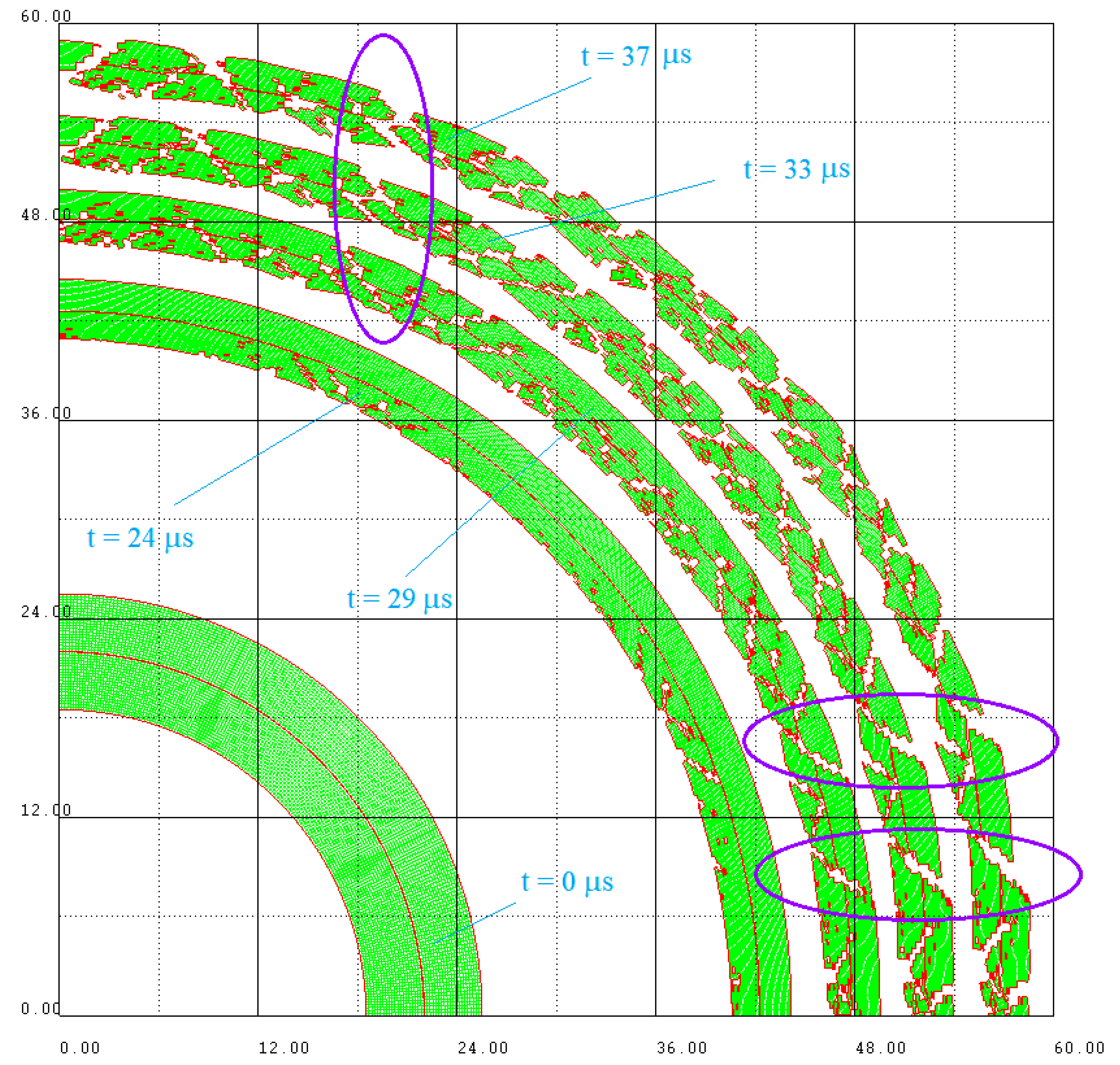

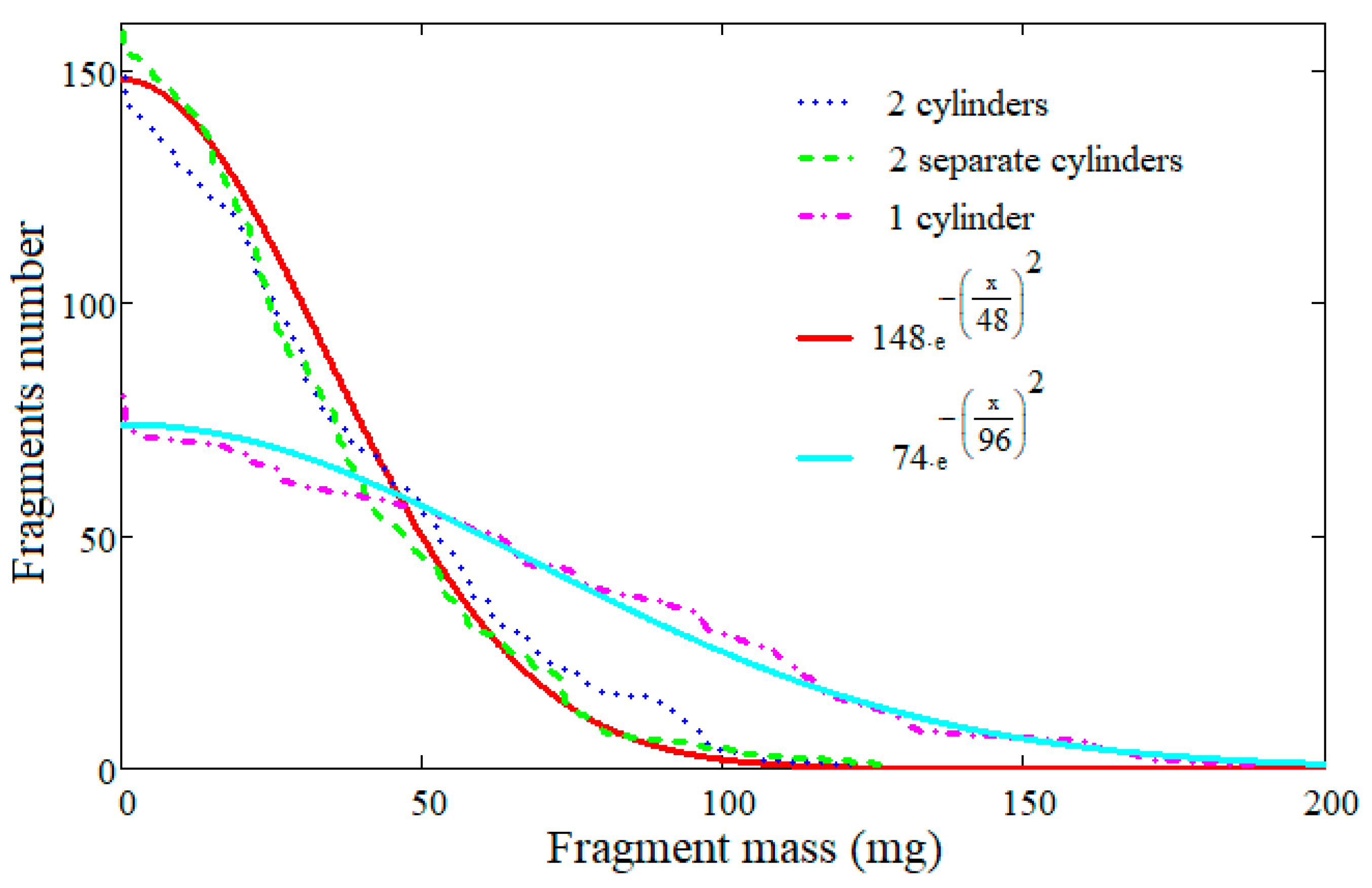

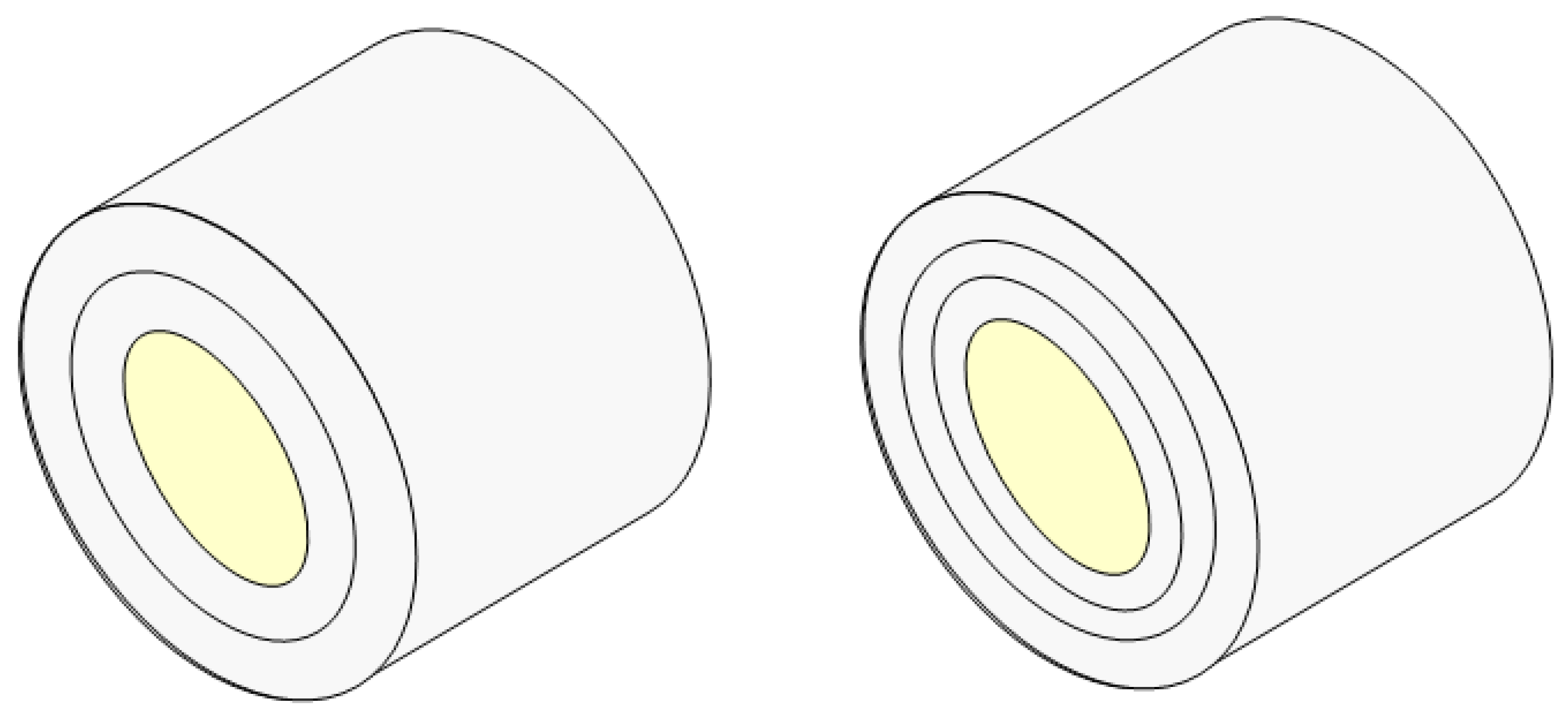

| No. | No. of Cylin. | Tip Conf. | Mean Diam. Cyl. No. 1 [mm] | Wall Thickness Cyl. No. 1 [mm] | MASS Ratio Cyl. No. 1 | Mean Diam. Cyl. No. 2 [mm] | Wall Thickness Cyl. No. 2 [mm] | Mass Ratio Cyl. No. 2 | Mean Diam. Cyl. No. 3 [mm] | Wall Thickness Cyl. No. 3 [mm] | Mass Ratio Cyl. No. 3 |
|---|---|---|---|---|---|---|---|---|---|---|---|
| 1 | 1 cyl. | Classic | 44 | 7 | - | - | - | - | - | - | - |
| 2 | 2 cyl. | Eq. thick. | 40.5 | 3.5 | 0.46 | 47.5 | 3.5 | 0.54 | - | - | - |
| 3 | 2 cyl. | Eq. mass | 40.78 | 3.78 | 0.5 | 47.78 | 3.22 | 0.5 | - | - | - |
| 4 | 3 cyl. | Eq. thick. | 39.33 | 2.33 | 0.298 | 44 | 2.33 | 0.333 | 48.67 | 2.33 | 0.369 |
| 5 | 3 cyl. | Eq. mass | 39.59 | 2.59 | 0.333 | 44.49 | 2.315 | 0.333 | 48.90 | 2.095 | 0.334 |
| 6 | 2 cyl. | Thin + thick | 38.5 | 1.5 | 0.188 | 45.5 | 5.5 | 0.812 | - | - | - |
| No. | No. of Cylinders | Configuration Type | The Total Number of Fragments | |||
|---|---|---|---|---|---|---|
| 1 | 1 cylinder | Classic | 2.713 × 10−4 kg | - | - | 907 |
| 2 | 2 cylinders | Equal thickness | 1.215 × 10−4 kg | 1.502 × 10−4 kg | - | 1815 |
| 3 | 2 cylinders | Equal mass | 1.325 × 10−4 kg | 1.392 × 10−4 kg | - | 1812 |
| 4 | 3 cylinders | Equal thickness | 7.787 × 10−5 kg | 9.046 × 10−5 kg | 1.034 × 10−4 kg | 2724 |
| 5 | 3 cylinders | Equal mass | 8.712 × 10−5 kg | 9.126 × 10−5 kg | 9.335 × 10−5 kg | 2717 |
| 6 | 2 cylinders | Thin + thick | 4.865 × 10−5 kg | 2.229 × 10−4 kg | - | 1844 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chiriac, O.-G.; Bucur, F.; Rotariu, A.-N.; Trană, E. The Application of Mott’s Distribution in the Fragmentation of Steel Coaxial Cylinders. Materials 2023, 16, 5783. https://doi.org/10.3390/ma16175783
Chiriac O-G, Bucur F, Rotariu A-N, Trană E. The Application of Mott’s Distribution in the Fragmentation of Steel Coaxial Cylinders. Materials. 2023; 16(17):5783. https://doi.org/10.3390/ma16175783
Chicago/Turabian StyleChiriac, Octavian-Gabriel, Florina Bucur, Adrian-Nicolae Rotariu, and Eugen Trană. 2023. "The Application of Mott’s Distribution in the Fragmentation of Steel Coaxial Cylinders" Materials 16, no. 17: 5783. https://doi.org/10.3390/ma16175783
APA StyleChiriac, O.-G., Bucur, F., Rotariu, A.-N., & Trană, E. (2023). The Application of Mott’s Distribution in the Fragmentation of Steel Coaxial Cylinders. Materials, 16(17), 5783. https://doi.org/10.3390/ma16175783






