Effect of Tendon-Related Variables on the Behavior of Externally CFRP Prestressed Concrete Beams
Abstract
1. Introduction
2. Materials, Method and Verification
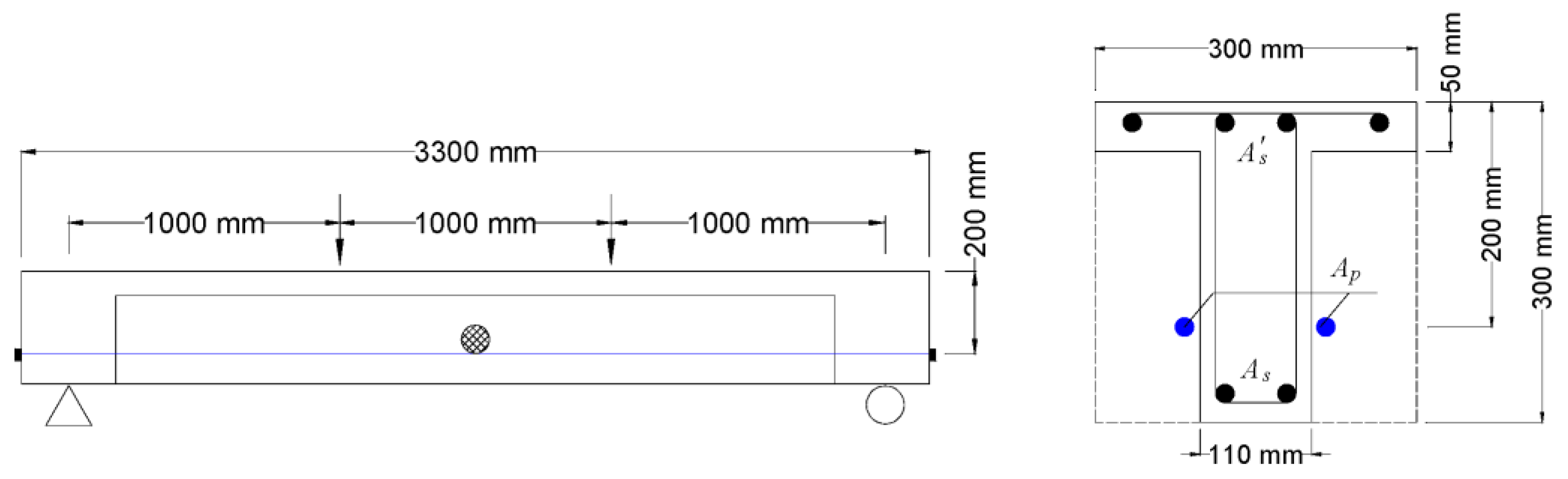
2.1. Materials and Method
2.2. Verification
3. Numerical Study
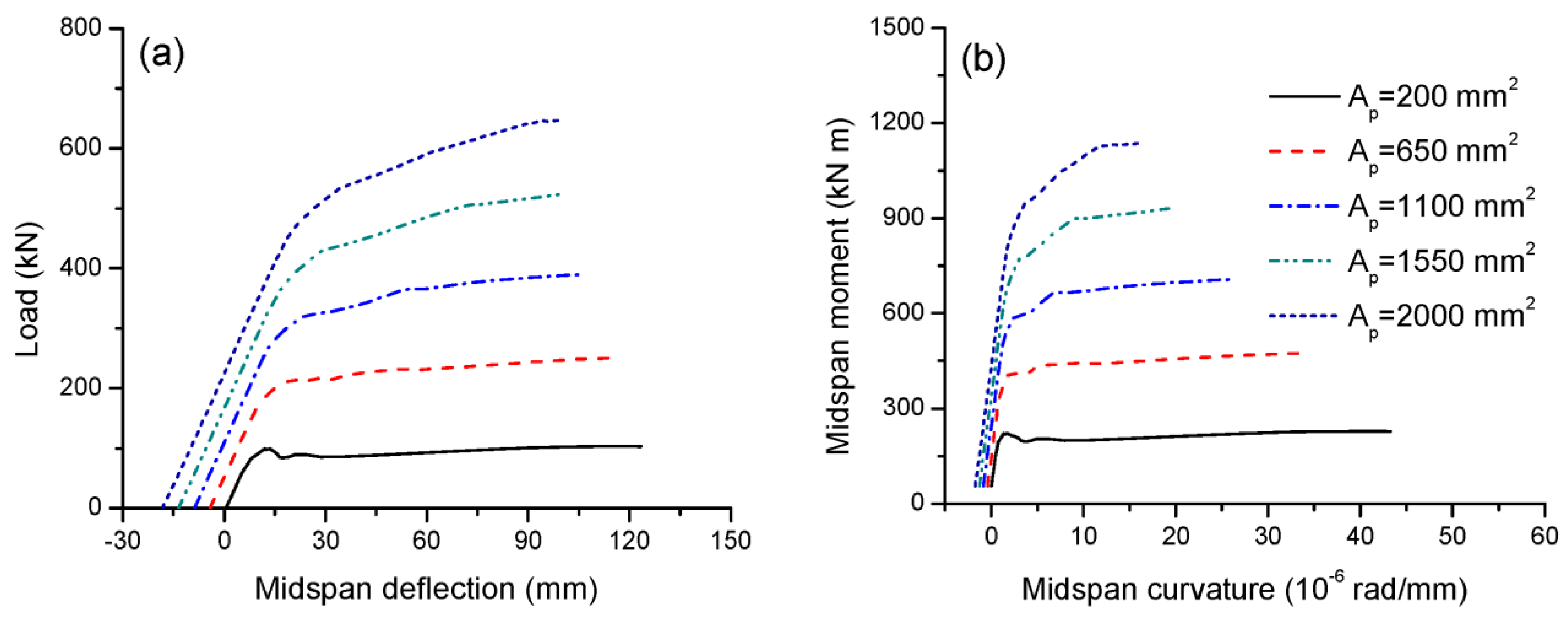
3.1. Effect of Tendon Area
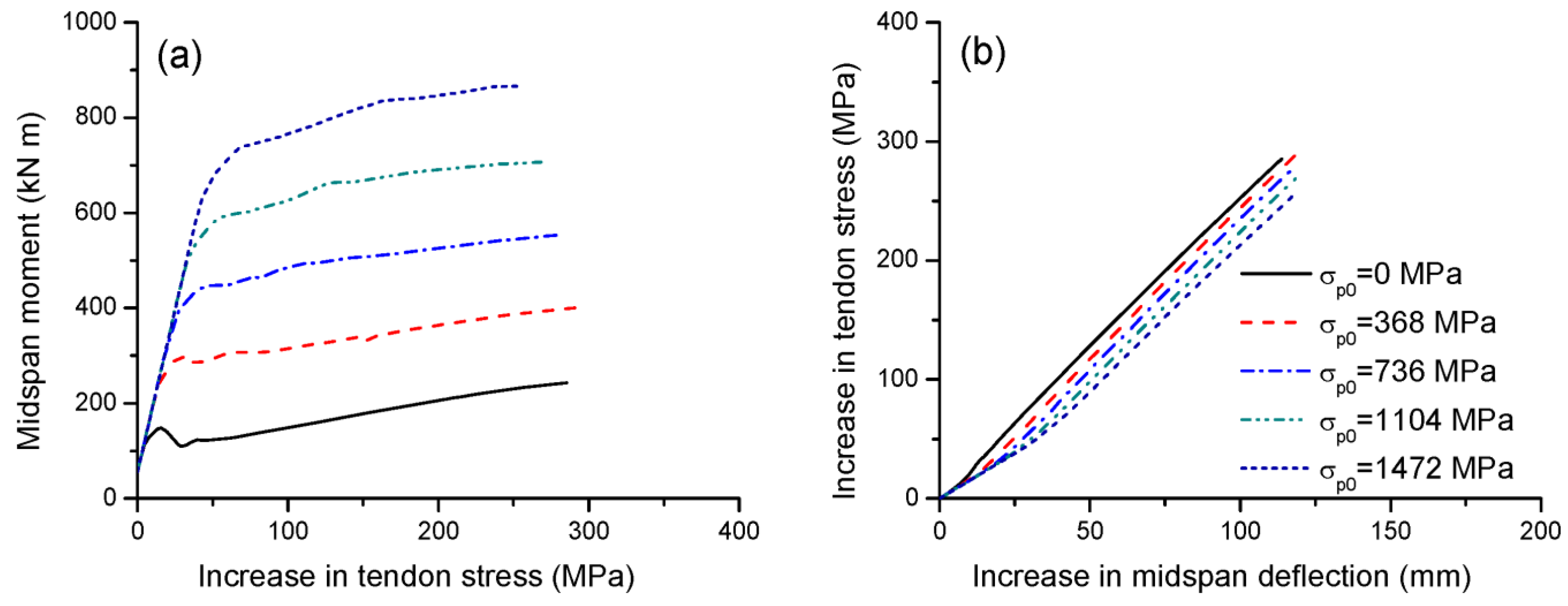
3.2. Effect of Prestress Level
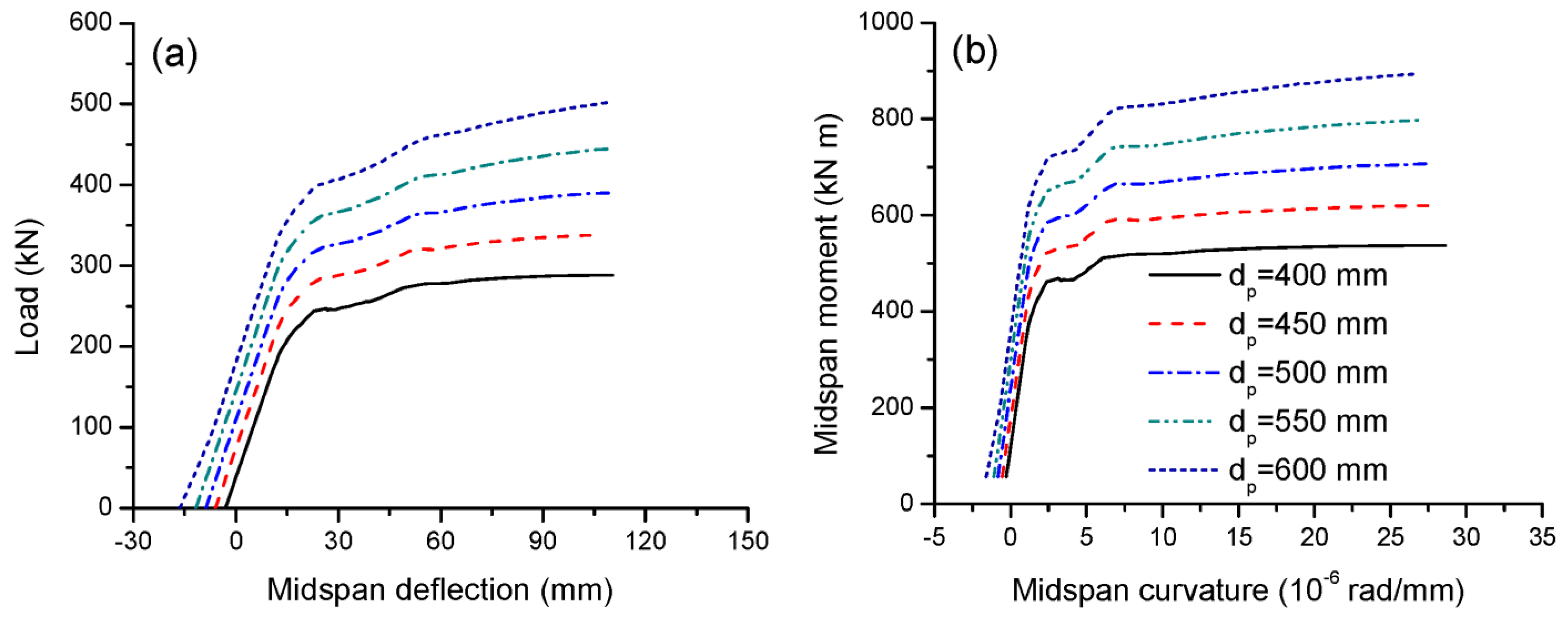
3.3. Effect of Tendon Depth
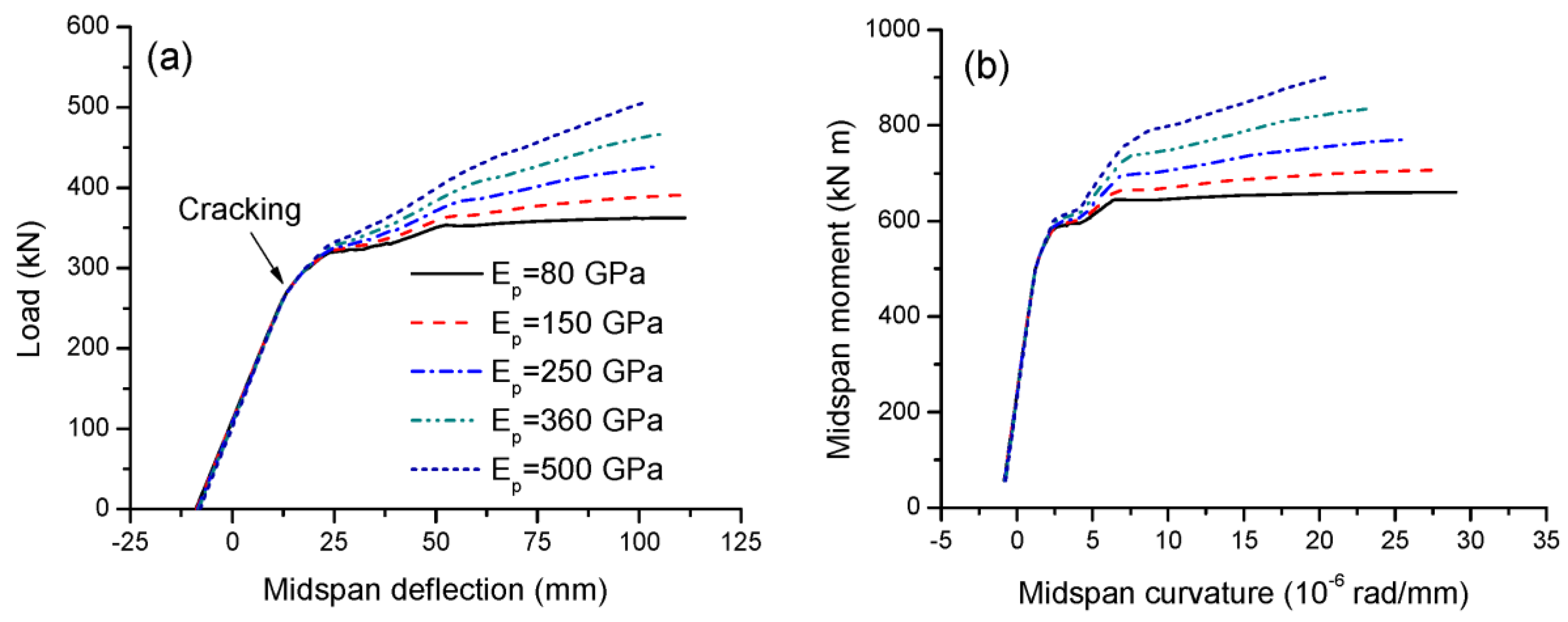
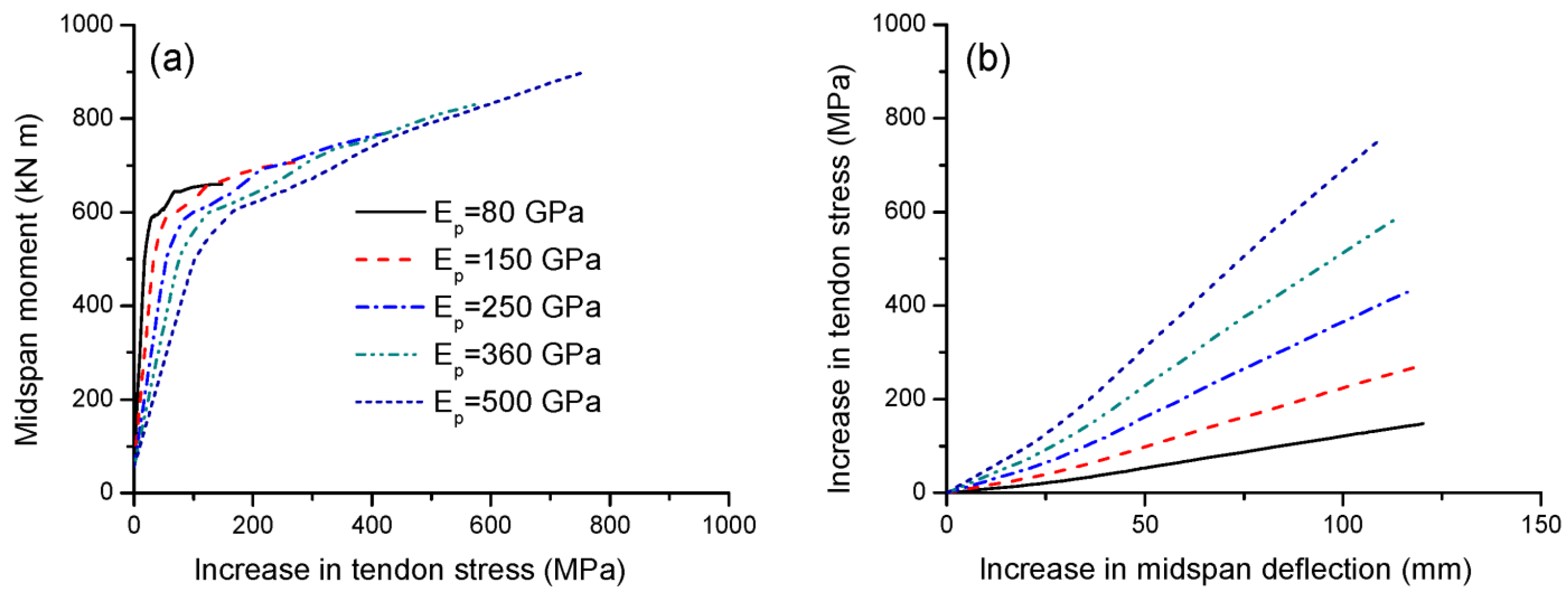
3.4. Effect of Tendon Elastic Modulus
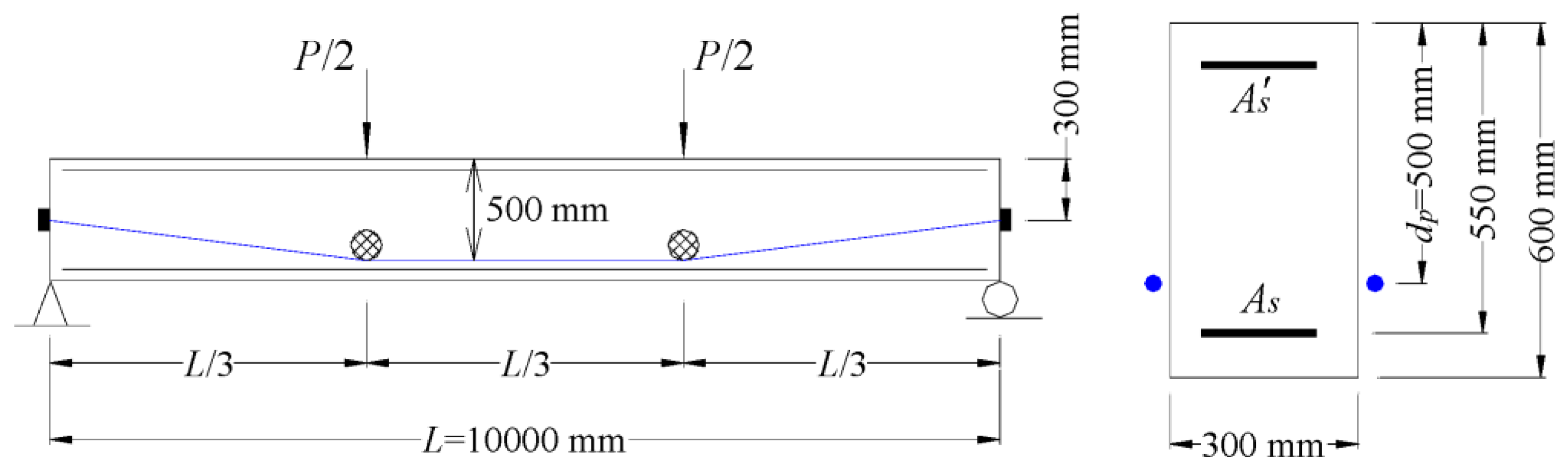
4. Analytical Study
4.1. Method
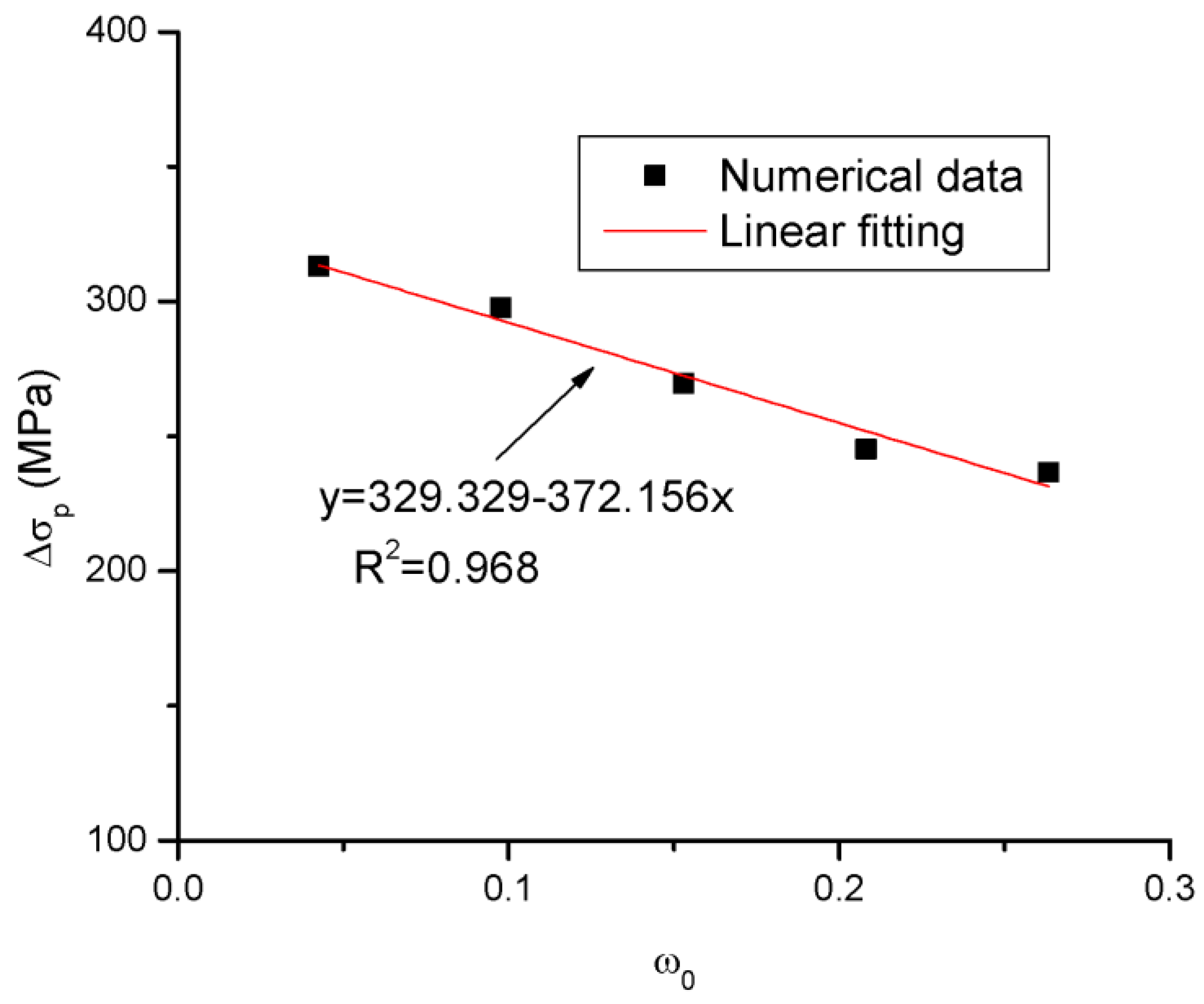
4.2. Results
5. Conclusions
- CFRP tendons play a crucial role in the structural performance of externally prestressed beams, including the flexural stiffness, ultimate load-carrying capacity, stress increase in external tendons, deformation and ductility. A higher tendon area, initial prestress or elastic modulus causes a lower flexural ductility. The Δσp decreases as the tendon area or initial prestress level increases or as the tendon depth or elastic modulus increases.
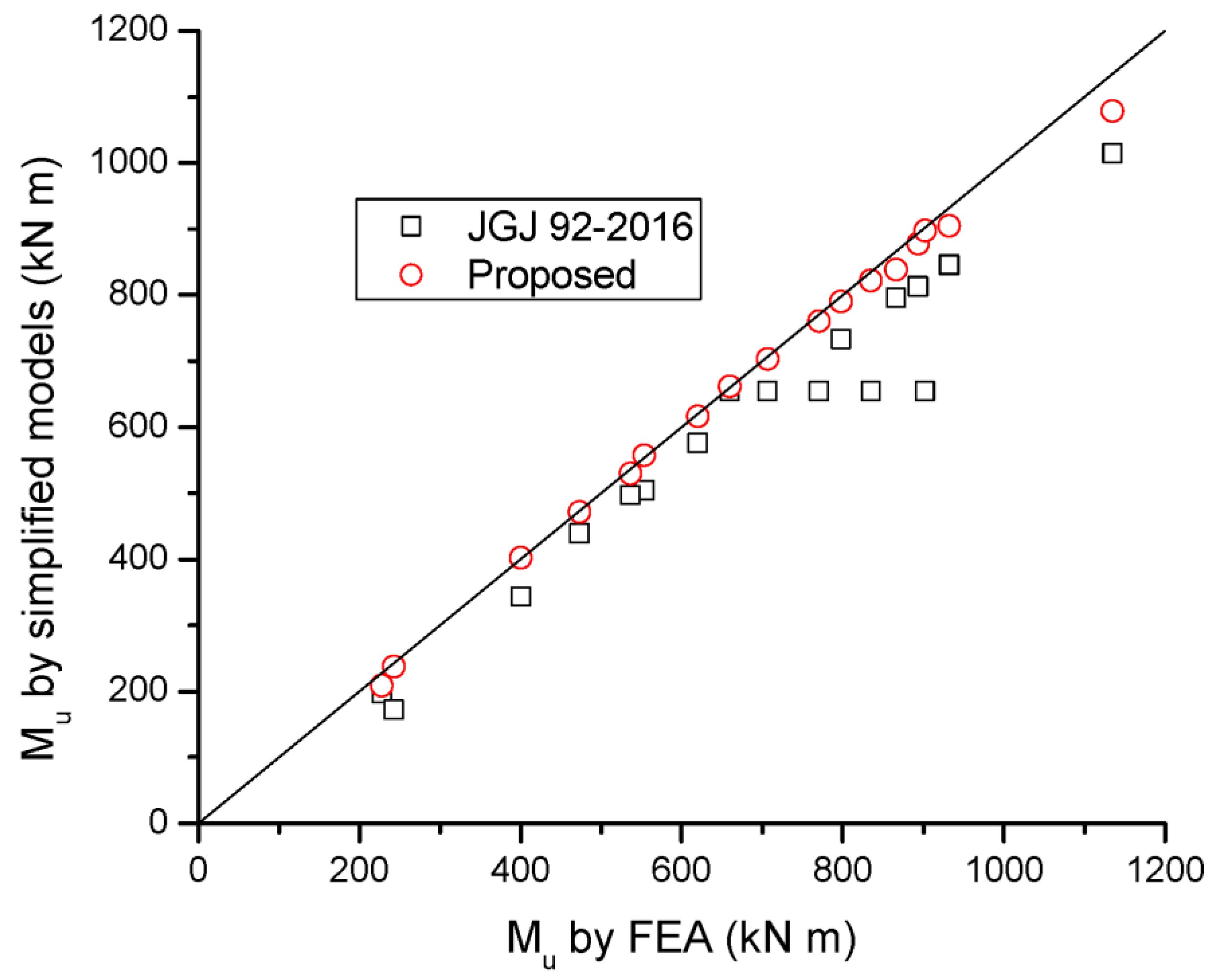
- JGJ 92-2016 significantly underestimates the ultimate tendon stress, and hence, this code is over-conservative for flexural strength predictions of externally CFRP prestressed beams. The predicted Δσp and Mu by JGJ 92-2016 are 53.5% and 88.6% of the FEA data, on average, respectively.
- An equation is proposed to calculate Δσp, considering the influence of the tendon area, effective prestress, tendon depth and modulus of elasticity. The proposed analytical model shows excellent predictions of tendon stress and flexural strength, i.e., the mean discrepancy for Δσp is 0.9% with a standard deviation of 11.1%, while the mean discrepancy for Mu is −1.6% with a standard deviation of 2.1%.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Recupero, A.; Spinella, N.; Colajanni, P.; Scilipoti, C.D. Increasing the capacity of existing bridges by using unbonded prestressing technology: A case study. Adv. Civil Eng. 2014, 2014, 840902. [Google Scholar] [CrossRef]
- Santarsiero, G.; Picciano, V. Durability enhancement of half-joints in RC bridges through external prestressed tendons: The Musmeci Bridge’s case study. Case Stud. Constr. Mater. 2023, 18, e01813. [Google Scholar] [CrossRef]
- Panahi, M.; Zareei, S.A.; Izadi, A. Flexural strengthening of reinforced concrete beams through externally bonded FRP sheets and near surface mounted FRP bars. Case Stud. Constr. Mater. 2021, 15, e00601. [Google Scholar] [CrossRef]
- Abdallah, M.; Al Mahmoud, F.; Khelil, A.; Mercier, J.; Almassri, B. Assessment of the flexural behavior of continuous RC beams strengthened with NSM-FRP bars, experimental and analytical study. Compos. Struct. 2020, 242, 112127. [Google Scholar] [CrossRef]
- Barris, C.; Torres, L.; Vilanova, I.; Mias, C.; Llorens, M. Experimental study on crack width and crack spacing for Glass-FRP reinforced concrete beams. Eng. Struct. 2017, 131, 231–242. [Google Scholar] [CrossRef]
- Efe, S.; Shokouhian, M.; Head, M.; Chinaka, E. Numerical study on the cyclic response of AFRP reinforced columns with externally unbonded energy dissipaters. Struct. Infrastruct. Eng. 2018, 14, 218–231. [Google Scholar] [CrossRef]
- Alkhraisha, H.; Mhanna, H.; Tello, N.; Abed, F. Serviceability and flexural behavior of concrete beams reinforced with basalt fiber-reinforced polymer (BFRP) bars exposed to harsh conditions. Polymers 2020, 12, 2110. [Google Scholar] [CrossRef]
- Alam, M.S.; Hussein, A. Relationship between the shear capacity and the flexural cracking load of FRP reinforced concrete beams. Constr. Build. Mater. 2017, 154, 819–828. [Google Scholar] [CrossRef]
- ACI Committee 440. Guide for the Design and Construction of Structural Concrete Reinforced with FRP Bars; ACI 440.1R-15; American Concrete Institute: Farmington Hills, MI, USA, 2015. [Google Scholar]
- FIB Model Code 2010; Bulletins 55 and 56; International Federation for Structural Concrete: Lausanne, Switzerland, 2012.
- Zhuge, P.; Tao, G.; Wang, B.; Jie, Z.; Zhang, Z. Effects of high temperatures on the performance of carbon fiber reinforced polymer (CFRP) composite cables protected with fire-retardant materials. Materials 2022, 15, 4696. [Google Scholar] [CrossRef]
- Xia, J.; Xu, Z.; Zhuge, P.; Wang, B.; Cai, W.; Fu, J. Testing and evaluation of flexural tensile strength of prestressed CFRP cables. Materials 2022, 15, 7065. [Google Scholar] [CrossRef]
- Elbelbisi, A.H.; El-Sisi, A.A.; Hassan, H.A.; Salim, H.A.; Shabaan, H.F. Parametric study on steel-concrete composite beams strengthened with post-tensioned CFRP tendons. Sustainability 2022, 14, 15792. [Google Scholar] [CrossRef]
- Jia, L.; Fang, Z.; Hu, R.; Pilakoutas, K.; Huang, Z. Fatigue behavior of UHPC beams prestressed with external CFRP tendons. ASCE J. Compos. Constr. 2022, 26, 04022066. [Google Scholar] [CrossRef]
- Abdel Aziz, M.; Abdel-Sayed, G.; Ghrib, F.; Grace, N.F.; Madugula, M.K.S. Analysis of concrete beams prestressed and post-tensioned with externally unbonded carbon fiber reinforced polymer tendons. Can. J. Civ. Eng. 2005, 31, 1138–1151. [Google Scholar] [CrossRef]
- Grace, N.F.; Singh, S.B.; Puravankara, S.; Sachidanandan, S. Behavior of prestressed concrete box-beam bridges using CFRP tendons. PCI J. 2006, 51, 26–41. [Google Scholar] [CrossRef]
- Bennitz, A.; Schmidt, J.W.; Nilimaa, J.; Taljsten, B.; Goltermann, P.; Ravn, D.L. Reinforced concrete T-beams externally prestressed with unbonded carbon fiber-reinforced polymer tendons. ACI Struct. J. 2012, 109, 521–530. [Google Scholar]
- Tan, K.H.; Ng, C.K. Effects of Deviators and tendon configuration on behavior of externally prestressed beams. ACI Struct. J. 1997, 94, 13–21. [Google Scholar]
- Lou, T.; Lopes, S.M.R.; Lopes, A.V. Numerical analysis of behaviour of concrete beams with external FRP tendons. Constr. Build. Mater. 2012, 35, 970–978. [Google Scholar] [CrossRef]
- Pang, M.; Li, Z.; Lou, T. Numerical study of using FRP and steel rebars in simply supported prestressed concrete beams with external FRP tendons. Polymers 2020, 12, 2773. [Google Scholar] [CrossRef]
- EN 1992-1-1; CEN Eurocode 2: Design of Concrete Structures—Part 1-1: General Rules and Rules for Buildings. European Committee for Standardization: Brussels, Belgium, 2004.
- Kwak, H.G.; Kim, S.P. Nonlinear analysis of RC beams based on moment-curvature relation. Comput. Struct. 2002, 80, 615–628. [Google Scholar] [CrossRef]
- Lou, T.; Xiang, Y. Finite element modeling of concrete beams prestressed with external tendons. Eng. Struct. 2006, 28, 1919–1926. [Google Scholar] [CrossRef]
- Bonopera, M.; Chang, K.C.; Tullini, N. Vibration of prestressed beams: Experimental and finite-element analysis of post-tensioned thin-walled box-girders. J. Constr. Steel Res. 2023, 205, 107854. [Google Scholar] [CrossRef]
- Kim, Y.J. Flexural response of concrete beams prestressed with AFRP tendons: Numerical investigation. ASCE J. Compos. Constr. 2010, 14, 647–658. [Google Scholar] [CrossRef]
- Lou, T.; Karavasilis, T.L.; Chen, B. Assessment of second-order effect in externally prestessed steel-concrete composite beams. ASCE J. Bridge Eng. 2021, 26, 04021024. [Google Scholar] [CrossRef]
- Lou, T.; Li, Z.; Pang, M. Behavior of externally prestressed continuous beams with FRP/steel rebars under symmetrical/unsymmetrical loading: Numerical study. Case Stud. Constr. Mater. 2022, 17, e01196. [Google Scholar] [CrossRef]
- Alqam, M.; Alkhairi, F.; Naaman, A. An improved methodology for the prediction of the stress at ultimate in unbonded internal and external steel tendons. Arab. J. Sci. Eng. 2020, 45, 7915–7954. [Google Scholar] [CrossRef]
- Ghallab, A. Calculating ultimate tendon stress in externally prestressed continuous concrete beams using simplified formulas. Eng. Struct. 2013, 46, 417–430. [Google Scholar] [CrossRef]
- He, Z.Q.; Liu, Z. Stresses in external and internal unbonded tendons: Unified methodology and design equations. ASCE J. Struct. Eng. 2010, 136, 1055–1065. [Google Scholar] [CrossRef]
- Roberts-Wollmann, C.L.; Kreger, M.E.; Rogowsky, D.M.; Breen, J.E. Stresses in external tendons at ultimate. ACI Struct. J. 2005, 102, 206–213. [Google Scholar]
- Maguire, M.; Chang, M.; Collins, W.; Sun, Y. Stress increase of unbonded tendons in continuous posttensioned members. ASCE J. Bridge Eng. 2017, 22, 04016115. [Google Scholar] [CrossRef]
- JGJ 92-2016; Technical Specification for Concrete Structures Prestressed with Unbonded Tendons. China Architecture & Building Press: Beijing, China, 2016.
- Hu, H.; Lopes, S.M.R.; Lopes, A.V.; Lou, T. Flexural response of axially restricted RC beams: Numerical and theoretical study. Materials 2022, 15, 6052. [Google Scholar] [CrossRef]
- ACI Committee 440. Prestressing Concrete Structures with FRP Tendons; ACI 440.4R-04; American Concrete Institute: Farmington Hills, MI, USA, 2004. [Google Scholar]







| Ap (mm2) | Deflection (mm) | Curvature (rad/mm) | Deflection Ductility | Curvature Ductility | ||
|---|---|---|---|---|---|---|
| Yielding | Ultimate | Yielding | Ultimate | |||
| 200 | 21.9 | 123.4 | 5.3 | 43.3 | 5.63 | 8.16 |
| 650 | 43.2 | 119.1 | 5.9 | 34.3 | 2.75 | 5.78 |
| 1100 | 52.7 | 110.0 | 6.6 | 27.8 | 2.09 | 4.24 |
| 1550 | 57.3 | 102.7 | 7.0 | 20.0 | 1.79 | 2.86 |
| 2000 | 61.4 | 100.9 | 7.5 | 15.9 | 1.64 | 2.13 |
| σp0 (MPa) | Deflection (mm) | Curvature (rad/mm) | Deflection Ductility | Curvature Ductility | ||
|---|---|---|---|---|---|---|
| Yielding | Ultimate | Yielding | Ultimate | |||
| 0 | 18.6 | 116.5 | 5.0 | 40.8 | 6.25 | 8.13 |
| 368 | 25.8 | 118.7 | 5.6 | 36.3 | 4.61 | 6.53 |
| 736 | 45.8 | 115.2 | 6.1 | 32.0 | 2.51 | 5.25 |
| 1104 | 52.7 | 110.0 | 6.6 | 27.8 | 2.09 | 4.24 |
| 1472 | 56.5 | 105.0 | 6.9 | 22.0 | 1.86 | 3.18 |
| dp (mm) | Deflection (mm) | Curvature (rad/mm) | Deflection Ductility | Curvature Ductility | ||
|---|---|---|---|---|---|---|
| Yielding | Ultimate | Yielding | Ultimate | |||
| 400 | 50.4 | 110.4 | 6.5 | 28.6 | 2.19 | 4.42 |
| 450 | 52.0 | 110.1 | 6.5 | 28.2 | 2.12 | 4.32 |
| 500 | 52.7 | 110.0 | 6.6 | 27.8 | 2.09 | 4.24 |
| 550 | 53.6 | 109.7 | 6.6 | 27.2 | 2.05 | 4.13 |
| 600 | 53.8 | 110.1 | 6.6 | 26.7 | 2.04 | 4.06 |
| Ep (GPa) | Deflection (mm) | Curvature (rad/mm) | Deflection Ductility | Curvature Ductility | ||
|---|---|---|---|---|---|---|
| Yielding | Ultimate | Yielding | Ultimate | |||
| 80 | 52.6 | 111.2 | 6.5 | 29.0 | 2.12 | 4.45 |
| 150 | 52.7 | 110.0 | 6.6 | 27.8 | 2.09 | 4.24 |
| 250 | 53.5 | 107.8 | 6.6 | 25.6 | 2.01 | 3.87 |
| 360 | 54.6 | 105.9 | 6.7 | 23.2 | 1.94 | 3.47 |
| 500 | 54.6 | 102.7 | 6.8 | 20.6 | 1.88 | 3.05 |
| Ap (mm2) | σp0 (MPa) | dp (mm) | Ep (GPa) | Δσp (MPa) | Mu (kN m) | (Δσp)sim/(Δσp)fea | (Mu)sim/(Mu)fea | ||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| JGJ | Pro | FEA | JGJ | Pro | FEA | JGJ | Pro | JGJ | Pro | ||||
| 200 | 1104 | 500 | 150 | 176 | 307 | 313 | 197 | 209 | 228 | 0.56 | 0.98 | 0.86 | 0.92 |
| 650 | 162 | 287 | 298 | 439 | 472 | 474 | 0.54 | 0.96 | 0.93 | 1.00 | |||
| 1100 | 147 | 267 | 270 | 655 | 703 | 707 | 0.55 | 0.99 | 0.93 | 0.99 | |||
| 1550 | 133 | 247 | 245 | 846 | 904 | 932 | 0.54 | 1.01 | 0.91 | 0.97 | |||
| 2000 | 118 | 227 | 237 | 1014 | 1078 | 1135 | 0.50 | 0.96 | 0.89 | 0.95 | |||
| 1100 | 0 | 500 | 150 | 182 | 316 | 285 | 172 | 238 | 243 | 0.64 | 1.11 | 0.71 | 0.98 |
| 368 | 171 | 300 | 292 | 343 | 402 | 400 | 0.59 | 1.03 | 0.86 | 1.01 | |||
| 736 | 159 | 283 | 283 | 504 | 558 | 554 | 0.56 | 1.00 | 0.91 | 1.01 | |||
| 1104 | 147 | 267 | 270 | 655 | 703 | 707 | 0.55 | 0.99 | 0.93 | 0.99 | |||
| 1472 | 135 | 251 | 255 | 795 | 838 | 867 | 0.53 | 0.98 | 0.92 | 0.97 | |||
| 1100 | 1104 | 400 | 150 | 137 | 253 | 188 | 497 | 530 | 537 | 0.73 | 1.35 | 0.93 | 0.99 |
| 450 | 143 | 261 | 228 | 576 | 616 | 620 | 0.63 | 1.14 | 0.93 | 0.99 | |||
| 500 | 147 | 267 | 270 | 655 | 703 | 707 | 0.55 | 0.99 | 0.93 | 0.99 | |||
| 550 | 151 | 272 | 313 | 734 | 790 | 798 | 0.48 | 0.87 | 0.92 | 0.99 | |||
| 600 | 154 | 276 | 363 | 813 | 877 | 894 | 0.42 | 0.76 | 0.91 | 0.98 | |||
| 1100 | 1104 | 500 | 80 | 147 | 164 | 148 | 655 | 661 | 660 | 1.00 | 1.11 | 0.99 | 1.00 |
| 150 | 147 | 267 | 270 | 655 | 703 | 707 | 0.55 | 0.99 | 0.93 | 0.99 | |||
| 250 | 147 | 414 | 429 | 655 | 761 | 770 | 0.34 | 0.96 | 0.85 | 0.99 | |||
| 360 | 147 | 575 | 590 | 655 | 822 | 835 | 0.25 | 0.97 | 0.78 | 0.98 | |||
| 500 | 147 | 780 | 763 | 655 | 897 | 902 | 0.19 | 1.02 | 0.73 | 0.99 | |||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lou, T.; Hu, H.; Pang, M. Effect of Tendon-Related Variables on the Behavior of Externally CFRP Prestressed Concrete Beams. Materials 2023, 16, 5197. https://doi.org/10.3390/ma16145197
Lou T, Hu H, Pang M. Effect of Tendon-Related Variables on the Behavior of Externally CFRP Prestressed Concrete Beams. Materials. 2023; 16(14):5197. https://doi.org/10.3390/ma16145197
Chicago/Turabian StyleLou, Tiejiong, Han Hu, and Miao Pang. 2023. "Effect of Tendon-Related Variables on the Behavior of Externally CFRP Prestressed Concrete Beams" Materials 16, no. 14: 5197. https://doi.org/10.3390/ma16145197
APA StyleLou, T., Hu, H., & Pang, M. (2023). Effect of Tendon-Related Variables on the Behavior of Externally CFRP Prestressed Concrete Beams. Materials, 16(14), 5197. https://doi.org/10.3390/ma16145197





