Eu3+ as a Powerful Structural and Spectroscopic Tool for Glass Photonics †
Abstract
1. Introduction
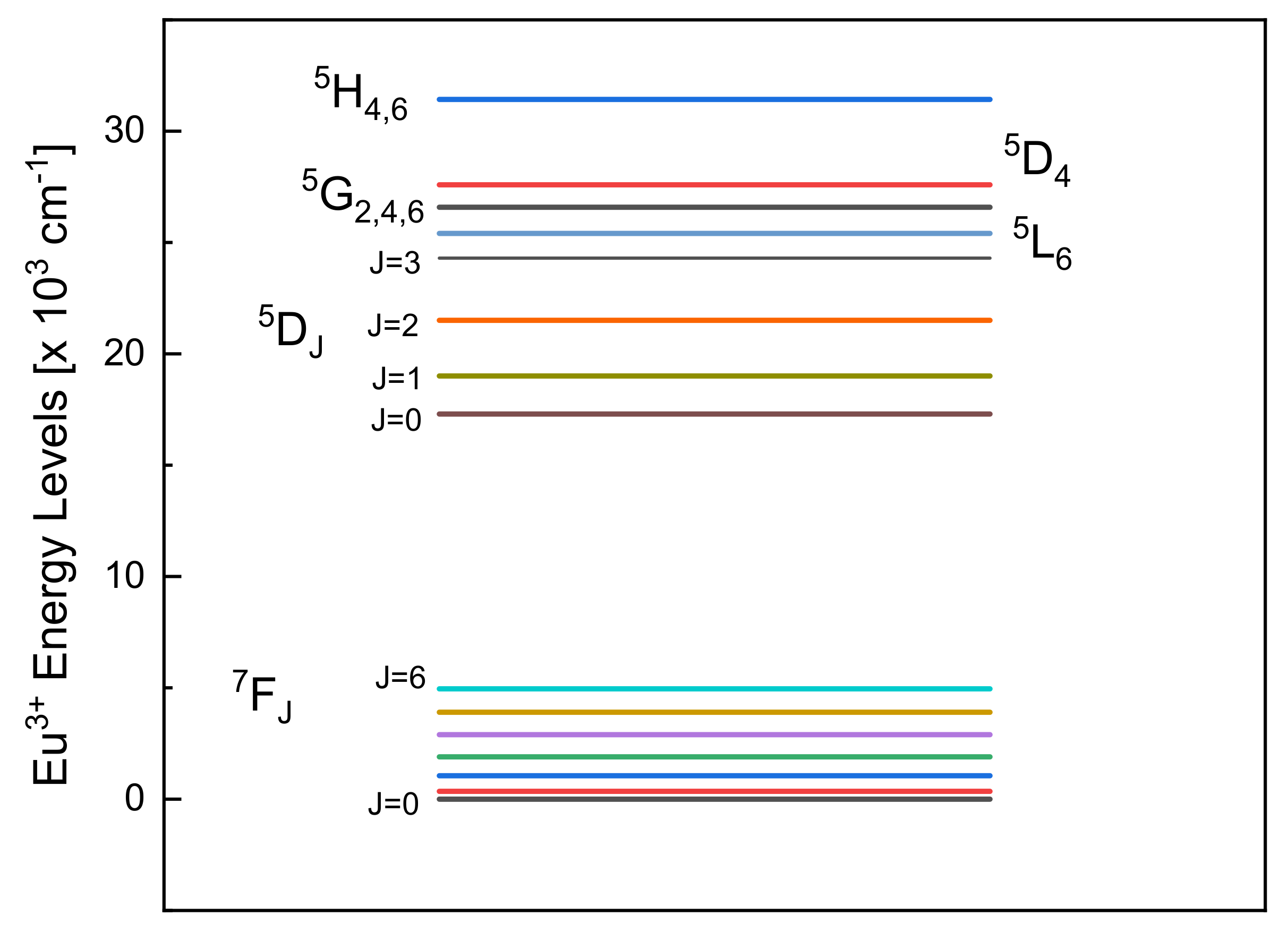
2. Eu3+ as Probe of Local Structure
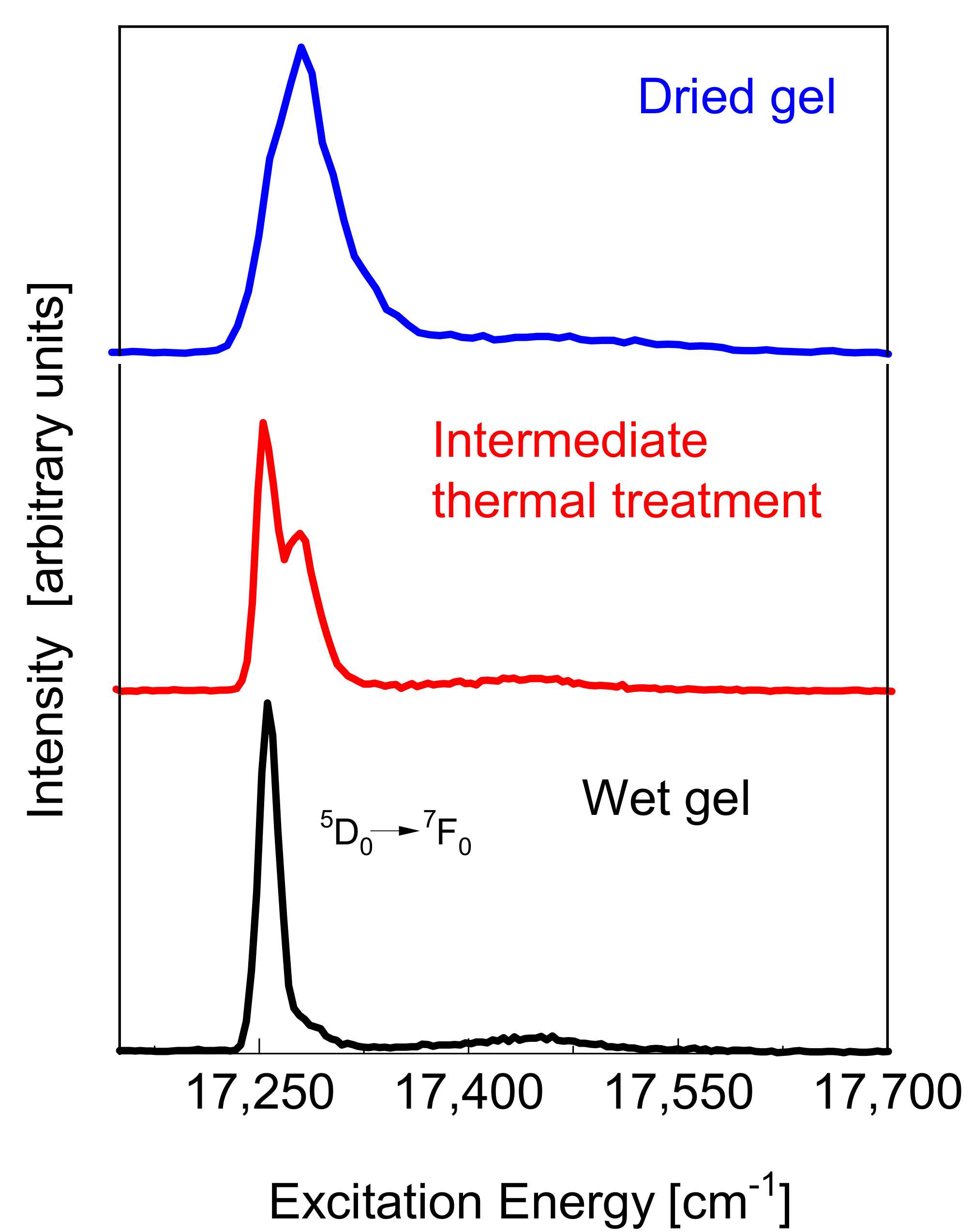
2.1. OH Coordination Sphere and Densification Process
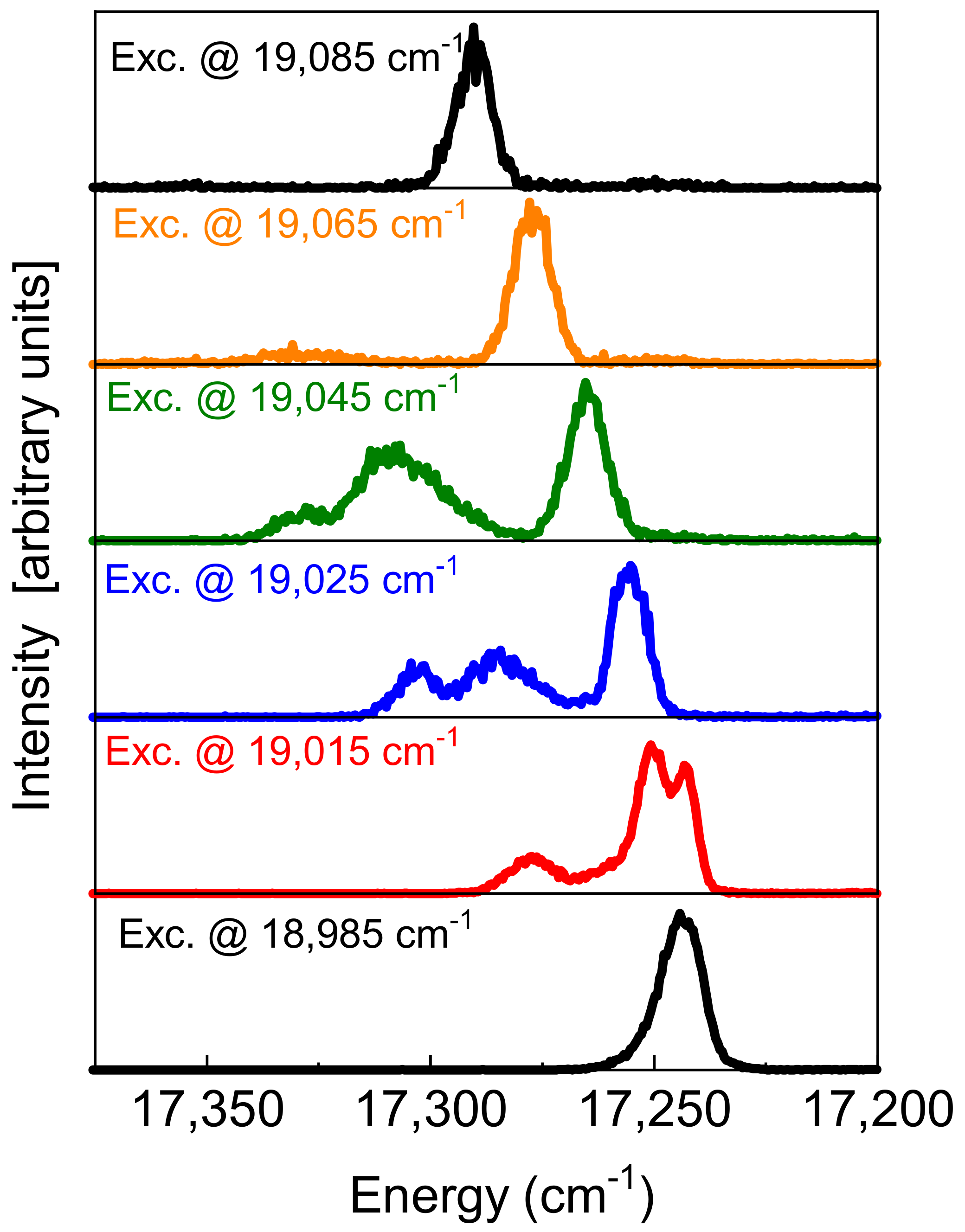
2.2. Dynamical Processes
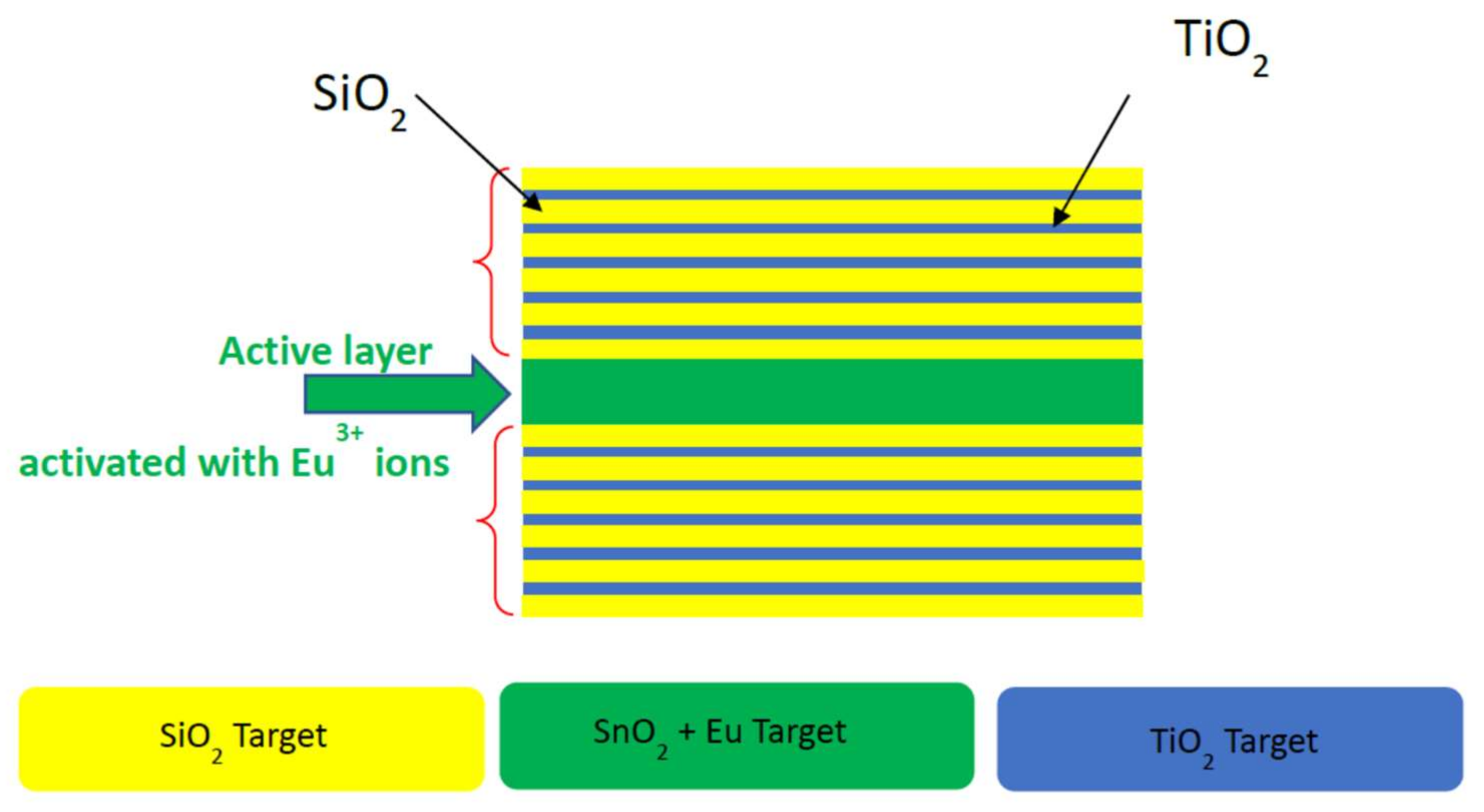
3. Eu3+ in Quantum Technologies
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Liu, G. Electronic Energy Level Structure. In Spectroscopic Properties of Rare Earths in Optical Materials; Liu, G., Jacquier, B., Eds.; Springer Series in Materials Science; Springer: Berlin/Heidelberg, Germany, 2005; pp. 1–94. [Google Scholar]
- Rajnak, K.; Wybourne, B.G. Configuration Interaction in Crystal Field Theory. J. Chem. Phys. 1964, 41, 565–569. [Google Scholar] [CrossRef]
- Lukowiak, A.; Chiasera, A.; Chiappini, A.; Righini, G.C.; Ferrari, M. Active Sol-Gel Materials, Fluorescence Spectra, and Lifetimes. In Handbook of Sol-Gel Science and Technology, 2nd ed.; Klein, L., Aparicio, M., Jitianu, A., Eds.; Springer: Cham, Switzeland, 2016; pp. 1–43. [Google Scholar]
- Campostrini, R.; Carturan, G.; Ferrari, M.; Montagna, M.; Pilla, O. Luminescence of Eu3+ ions during thermal densification of SiO2 gel. J. Mater. Res. 1992, 7, 745–753. [Google Scholar] [CrossRef]
- Hüfner, S. Optical Spectra of Transparent Rare-Earth Compounds; Academic Press: New York, NY, USA, 1978. [Google Scholar]
- Armellini, C.; Del Longo, L.; Ferrari, M.; Montagna, M.; Pucker, G.; Sagoo, P. Effect of Pr3+ doping on the OH content of silica xerogels. J. Sol Gel Sci. Technol. 1998, 13, 599–603. [Google Scholar] [CrossRef]
- Horrocks, W.D., Jr.; Sudnick, D.R. Lanthanide ion luminescence probes of the structure of biological macromolecules. Acc. Chem. Res. 1981, 14, 384–392. [Google Scholar] [CrossRef]
- Hazenkamp, M.F.; Blasse, G. Rare-earth ions adsorbed onto porous glass: Luminescence as a characterizing tool. Chem. Mater. 1990, 2, 105–110. [Google Scholar] [CrossRef]
- Popov, A.; Orlovskaya, E.; Shaidulin, A.; Vagapova, E.; Timofeeva, E.; Dolgov, L.; Iskhakova, L.; Uvarov, O.; Novikov, G.; Rähn, M.; et al. Stable aqueous colloidal solutions of Nd3+: LaF3 nanoparticles, promising for luminescent bioimaging in the near-infrared spectral range. Nanomaterials 2021, 11, 2847. [Google Scholar] [CrossRef] [PubMed]
- Aime, S.; Bettinelli, M.; Ferrari, M.; Razzano, E.; Terreno, E. NMR and luminescence studies on the formation of ternary adducts between HSA and Ln(III)/–malonate complexes (Ln=Eu, Gd, Tb). Biochim. Biophys. Acta 1998, 1385, 7–16. [Google Scholar] [CrossRef]
- Piazza, A.; Bouajaj, A.; Ferrari, M.; Montagna, M.; Campostrini, R.; Carturan, G. Optical spectroscopy of Eu3+ ion as a tool for the study of dehydration process in silica gels. J. Phys. IV 1994, C4, C4-569–C4-572. [Google Scholar] [CrossRef]
- Ferrari, M.; Piazza, A.; Montagna, M.; Carturan, G.; Campostrini, R. Site selection spectroscopy of SiO2:Eu3+ gels. J. Sol Gel Sci. Technol. 1994, 2, 783–786. [Google Scholar] [CrossRef]
- Blasse, G.; Dirksen, G.J. Scandium borate (ScBO3) as a host lattice for luminescent lanthanide and transition metal ions. Inorg. Chim. Acta 1988, 145, 303–308. [Google Scholar] [CrossRef]
- Tanabe, S.; Todoroki, S.; Hirao, K.; Soga, N. Phonon sideband of Eu3+ in sodium borate glasses. J. Non Cryst. Solids 1990, 122, 59–65. [Google Scholar] [CrossRef]
- Todoroki, S.; Hirao, K.; Soga, N. Phonon Sideband Spectra and Local Structure around Eu3+ Ions in Aluminosilicate Glasses. J. Ceram. Soc. Jpn. 1993, 101, 1065–1067. [Google Scholar] [CrossRef][Green Version]
- Habenschuss, A.; Spedding, F.H. The coordination (hydration) of rare earth ions in aqueous chloride solutions from x-ray diffraction. III. SmCl3, EuCl3, and series behavior. J. Chem. Phys. 1980, 73, 442–450. [Google Scholar] [CrossRef]
- Cormier, G.; Capobianco, J.A.; Morrison, C.A.; Monteil, A. Molecular-dynamics simulation of the trivalent europium ion doped in sodium disilicate glass: Electronic absorption and emission spectra. Phys. Rev. B 1993, 48, 16290. [Google Scholar] [CrossRef]
- Righini, G.C.; Ferrari, M. Photoluminescence of rare-earth-doped glasses. La Riv. Del Nuovo Cim. 2005, 28, 1–53. [Google Scholar]
- Bouajaj, A.; Monteil, A.; Bovier, C.; Ferrari, M.; Piazza, A. Site distribution and thermalization effects in Europium-doped silica glasses. J. Phys. IV 1994, C4, C4-579–C4-582. [Google Scholar] [CrossRef]
- Bouajaj, A.; Ferrari, M.; Montagna, M.; Moser, E.; Piazza, A.; Campostrini, R.; Carturan, G. Optical spectroscopy of Eu3+ doped silica gels. Philos. Mag. B 1995, 71, 633–640. [Google Scholar] [CrossRef]
- Mujahid, A.; Imran, M.; Fan, H.; Yuan, T.; Ali, H.; Li, P.; Zhang, Y. Multi-channel optical hybrid filter controlled by extrinsic parameters in various phases of Eu3+:BiPO4. J. Appl. Phys. 2021, 130, 243102. [Google Scholar] [CrossRef]
- Carlotto, A.; Babetto, L.; Carlotto, S.; Miozzi, M.; Seraglia, R.; Casarin, M.; Bottaro, G.; Rancan, M.; Armelao, L. Luminescent Thermometers: From a Library of Europium (III) β-Diketonates to a General Model for Predicting the Thermometric Behaviour of Europium-Based Coordination Systems. ChemPhotoChem 2020, 4, 674–684. [Google Scholar]
- Lu, D.; Gong, X.; Chen, Y.; Huang, J.; Lin, Y.; Luo, Z.; Huang, Y. Synthesis and photoluminescence characteristics of the LiGd3(MoO4)5:Eu3+ red phosphor with high color purity and brightness. Opt. Mat. Express 2018, 8, 259–269. [Google Scholar] [CrossRef]
- MacFarlane, R.M.; Shelby, R.M. Homogeneous line broadening of optical transitions of ions and molecules in glasses. J. Lumin. 1987, 36, 179–207. [Google Scholar] [CrossRef]
- Sbetti, M.; Moser, E.; Montagna, M.; Ferrari, M.; Chaussedent, S.; Bettinelli, M. Homogeneous line width in a zinc borate glass activated by Eu3+. J. Non-Cryst. Solids 1997, 220, 217–221. [Google Scholar] [CrossRef]
- Reineker, P.; Kassner, K. Temperature dependence of the homogeneous optical linewidth of impurities in glasses. J. Lumin. 1988, 40, 131–132. [Google Scholar] [CrossRef]
- Morgan, J.R.; Chock, E.P.; Hopewell, W.D.; El-Sayed, M.A.; Orbach, R. Origins of homogeneous and inhomogeneous line widths of the 5D0-7F0 transition of europium(3+) in amorphous solids. J. Phys. Chem. 1981, 85, 747–751. [Google Scholar] [CrossRef]
- Bhaktha, S.N.B.; Beclin, F.; Bouazaoui, M.; Capoen, B.; Chiasera, A.; Ferrari, M.; Kinowski, C.; Righini, G.C.; Robbe, O.; Turrell, S. Enhanced fluorescence from Eu3+ in low-loss silica glass-ceramic waveguides with high SnO2 content. Appl. Phys. Lett. 2008, 93, 211904. [Google Scholar] [CrossRef]
- Tran, T.N.L.; Armellini, C.; Varas, S.; Carpentiero, A.; Chiappini, A.; Głuchowski, P.; Iacob, E.; Ischia, G.; Scotognella, F.; Bollani, M.; et al. Assessment of SnO2-nanocrystal-based luminescent glass-ceramic waveguides for integrated photonics. Ceram. Int. 2021, 47, 5534–5541. [Google Scholar] [CrossRef]
- Zur, L.; Tran, T.N.L.; Meneghetti, M.; Tran, T.T.V.; Lukowiak, A.; Chiasera, A.; Zonta, D.; Ferrari, M.; Righini, G.C. Tin-dioxide nanocrystals as Er3+ luminescence sensitizers: Formation of glass-ceramics thin films and their characterization. Opt. Mat. 2017, 63, 95–100. [Google Scholar] [CrossRef]
- Cascales, C.; Balda, R.; García-Revilla, S.; Lezama, L.; Barredo-Zuriarrain, M.; Fernández, J. Site symmetry and host sensitization-dependence of Eu3+ real time luminescence in tin dioxide nanoparticles. Opt. Express 2018, 26, 16155–16170. [Google Scholar] [CrossRef]
- Kinos, A.; Hunger, D.; Kolesov, R.; Mølmer, K.; de Riedmatten, H.; Goldner, P.; Tallaire, A.; Morvan, L.; Berger, P.; Welinski, S.; et al. Roadmap for Rare-earth Quantum Computing. Available online: https://arxiv.org/abs/2103.15743v1 (accessed on 9 March 2021).
- Liu, S.; Serrano, D.; Fossati, A.; Tallaire, A.; Ferrier, A.; Goldner, P. Controlled size reduction of rare earth doped nanoparticles for optical quantum technologies. RSC Adv. 2018, 8, 37098–37104. [Google Scholar] [CrossRef]
- Ohlsson, N.; Mohan, R.K.; Kröll, S. Quantum computer hardware based on rare-earth-ion-doped inorganic crystals. Opt. Commun. 2002, 201, 71–77. [Google Scholar] [CrossRef]
- Sabooni, M.; Li, Q.; Kröll, S.; Rippe, L. Efficient Quantum Memory Using a Weakly Absorbing Sample. Phys. Rev. Lett. 2013, 110, 133604. [Google Scholar] [CrossRef]
- Yan, Y.; Karlsson, J.; Rippe, L.; Walther, A.; Serrano, D.; Lindgren, D.; Pistol, M.; Kröll, S.; Goldner, P.; Zheng, L.; et al. Measurement of linewidths and permanent electric dipole moment change of the Ce 4f-5d transition in Y2SiO5 for qubit readout scheme in rare-earth ion based quantum computing. Phys. Rev. B 2013, 87, 184205. [Google Scholar] [CrossRef]
- D’Amico, I.; Angelakis, D.G.; Bussières, F.; Caglayan, H.; Couteau, C.; Durt, T.; Kolaric, B.; Maletinsky, P.; Pfeiffer, W.; Rabl, P.; et al. Nanoscale quantum optics. La Riv. Del Nuovo Cim. 2019, 42, 153–195. [Google Scholar]
- Fan, H.; Goldschmidt, E.A. Narrowing of electromagnetically induced transparency in an inhomogeneously broadened solid-state atomic ensemble. In Proceedings of the Conference on Lasers and Electro-Optics (CLEO): QELS_Fundamental Science, San Jose, CA, USA, 13–18 May 2018. [Google Scholar]
- Mîrzac, A.; Macovei, M.A. Dynamics of a quantum oscillator coupled with a three-level Λ-type emitter. J. Opt. Soc. Am. B 2019, 36, 2473–2480. [Google Scholar] [CrossRef]
- Boucher, Y.G.; Al Sheikh, L. Universal Coupled-Mode Theory of Quasi-Normal Modes in a 1D Photonic Crystal. Opt. Quantum Electron. 2020, 52, 453. [Google Scholar] [CrossRef]
| Local Symmetry | Triclinic (C1, Ci) | Monoclinic (Cs, C2, C2h) | Rhombic (C2v, D2, D2h) |
|---|---|---|---|
| J = 0 | 1 | 1 | 1 |
| J = 1 | 3 | 3 | 3 |
| J = 2 | 5 | 5 | 5 |
| J = 3 | 7 | 7 | 7 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tran, T.N.L.; Chiasera, A.; Lukowiak, A.; Ferrari, M. Eu3+ as a Powerful Structural and Spectroscopic Tool for Glass Photonics. Materials 2022, 15, 1847. https://doi.org/10.3390/ma15051847
Tran TNL, Chiasera A, Lukowiak A, Ferrari M. Eu3+ as a Powerful Structural and Spectroscopic Tool for Glass Photonics. Materials. 2022; 15(5):1847. https://doi.org/10.3390/ma15051847
Chicago/Turabian StyleTran, Thi Ngoc Lam, Alessandro Chiasera, Anna Lukowiak, and Maurizio Ferrari. 2022. "Eu3+ as a Powerful Structural and Spectroscopic Tool for Glass Photonics" Materials 15, no. 5: 1847. https://doi.org/10.3390/ma15051847
APA StyleTran, T. N. L., Chiasera, A., Lukowiak, A., & Ferrari, M. (2022). Eu3+ as a Powerful Structural and Spectroscopic Tool for Glass Photonics. Materials, 15(5), 1847. https://doi.org/10.3390/ma15051847








