Semi-Infinite Structure Analysis with Bimodular Materials with Infinite Element
Abstract
:1. Introduction
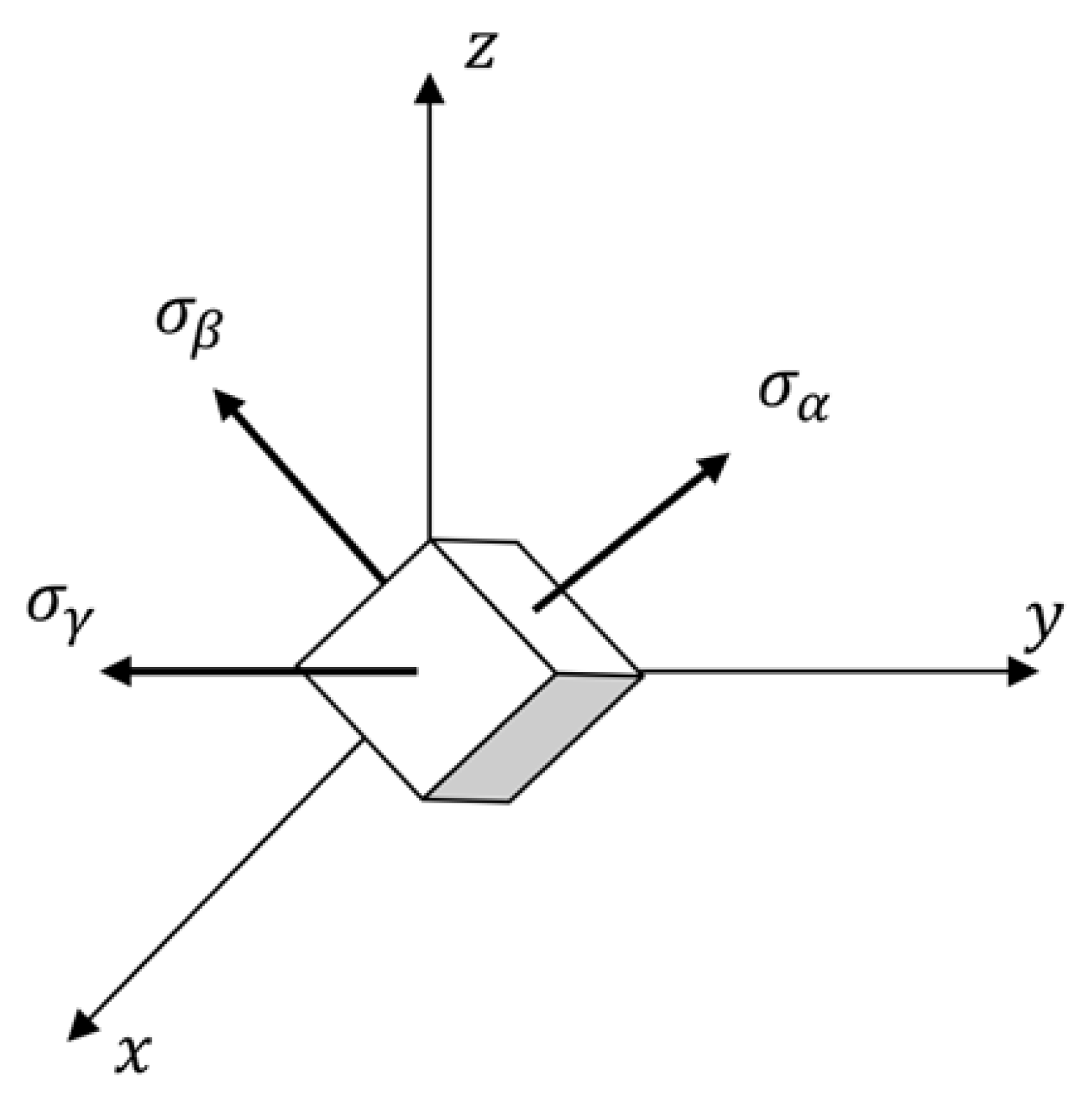
2. Bimodular Material Constitutive Equations
- (1)
- If all three principal stresses are equal, i.e., , we have
- a.
- If , then
- b.
- If , then
- (2)
- If only two of the three principal stresses are equal, i.e., , we hold
- (3)
- If all three principal stresses are not equal, i.e., , we have
3. The Meshless Finite Block Method
3.1. Lagrange Polynomial Interpolation
3.2. Partial Differential Matrix
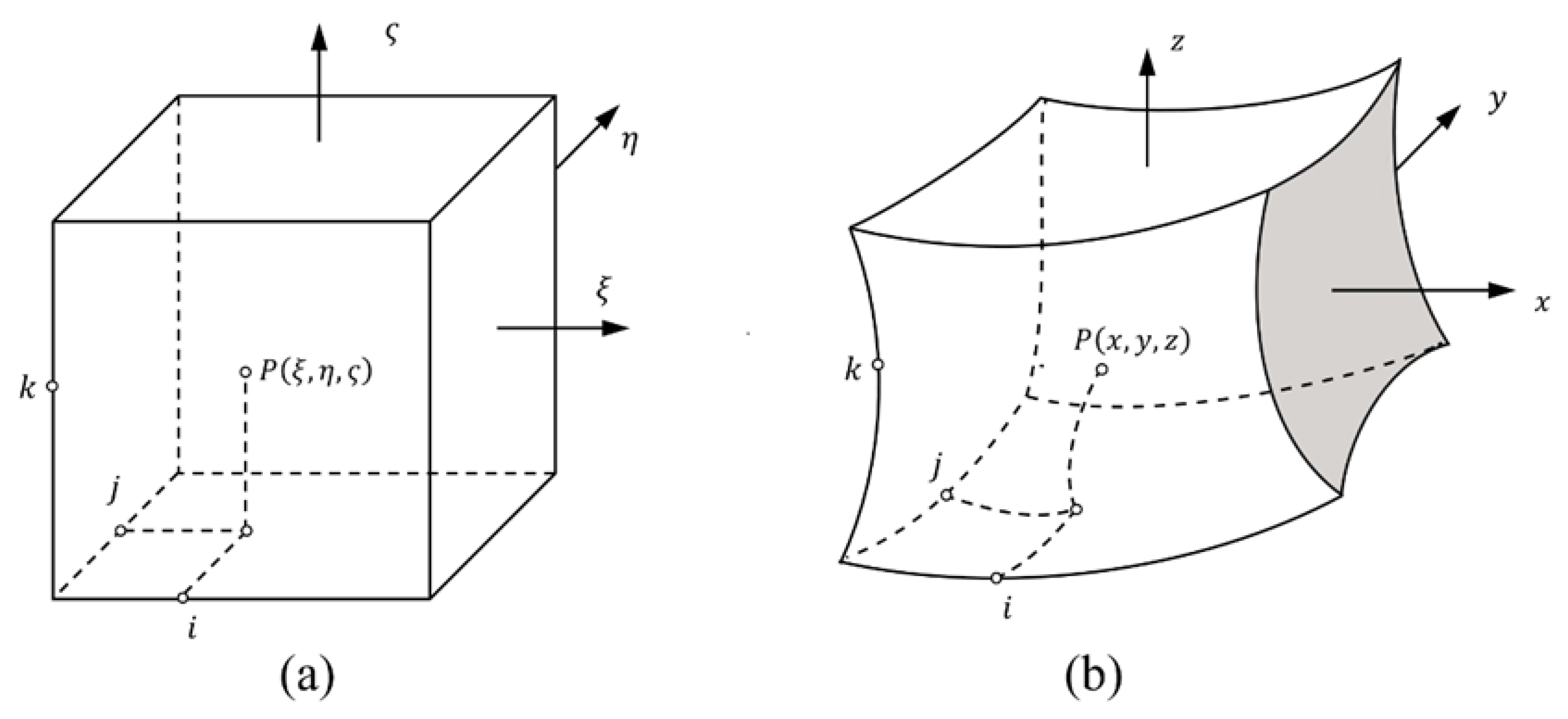
3.3. Mapping Differential Matrix
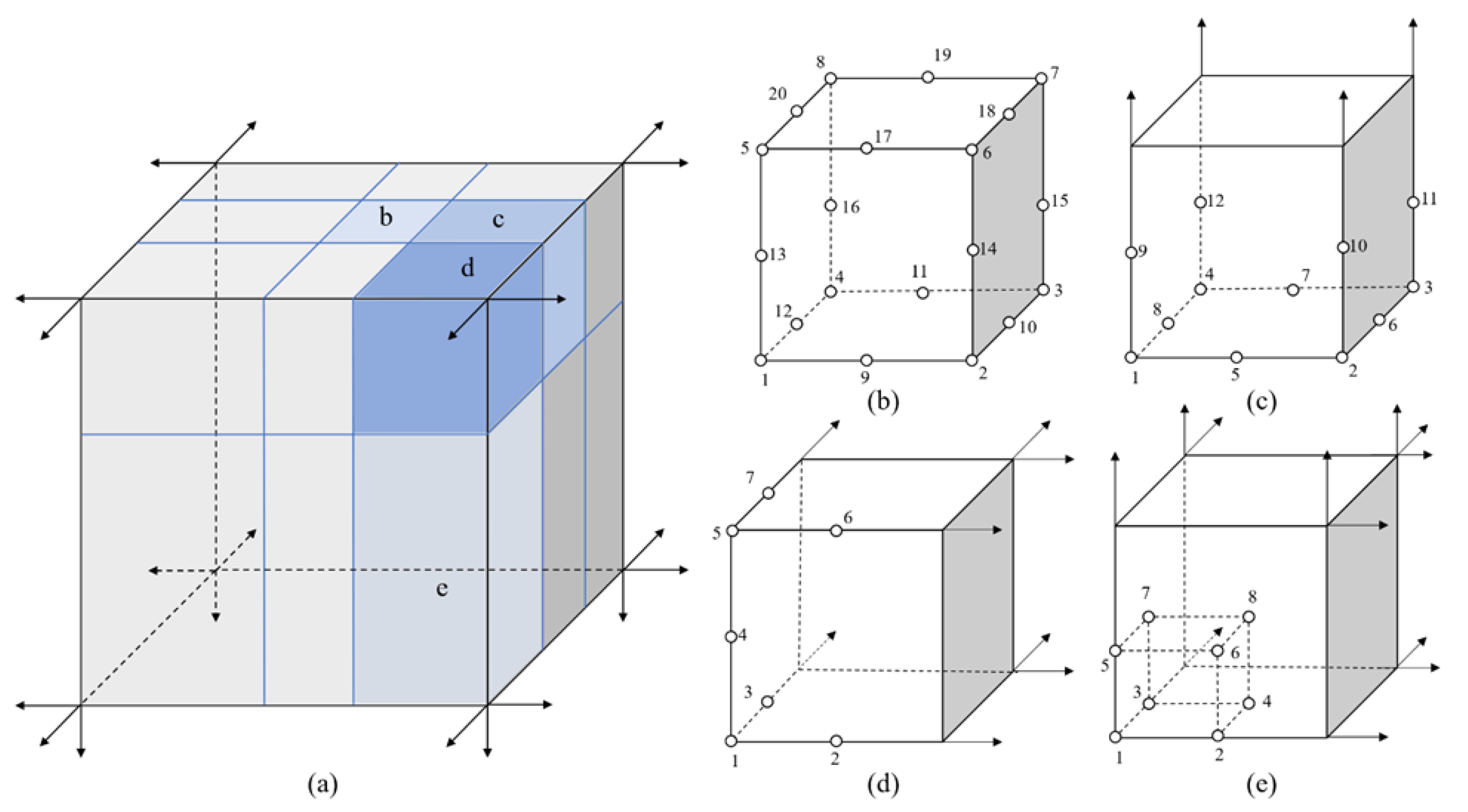
3.4. Mapping Technology with 3D Blocks
4. Formulations for Bimodular Material with Meshless FBM
5. Numerical Examples
5.1. Tensile Column with Gravity
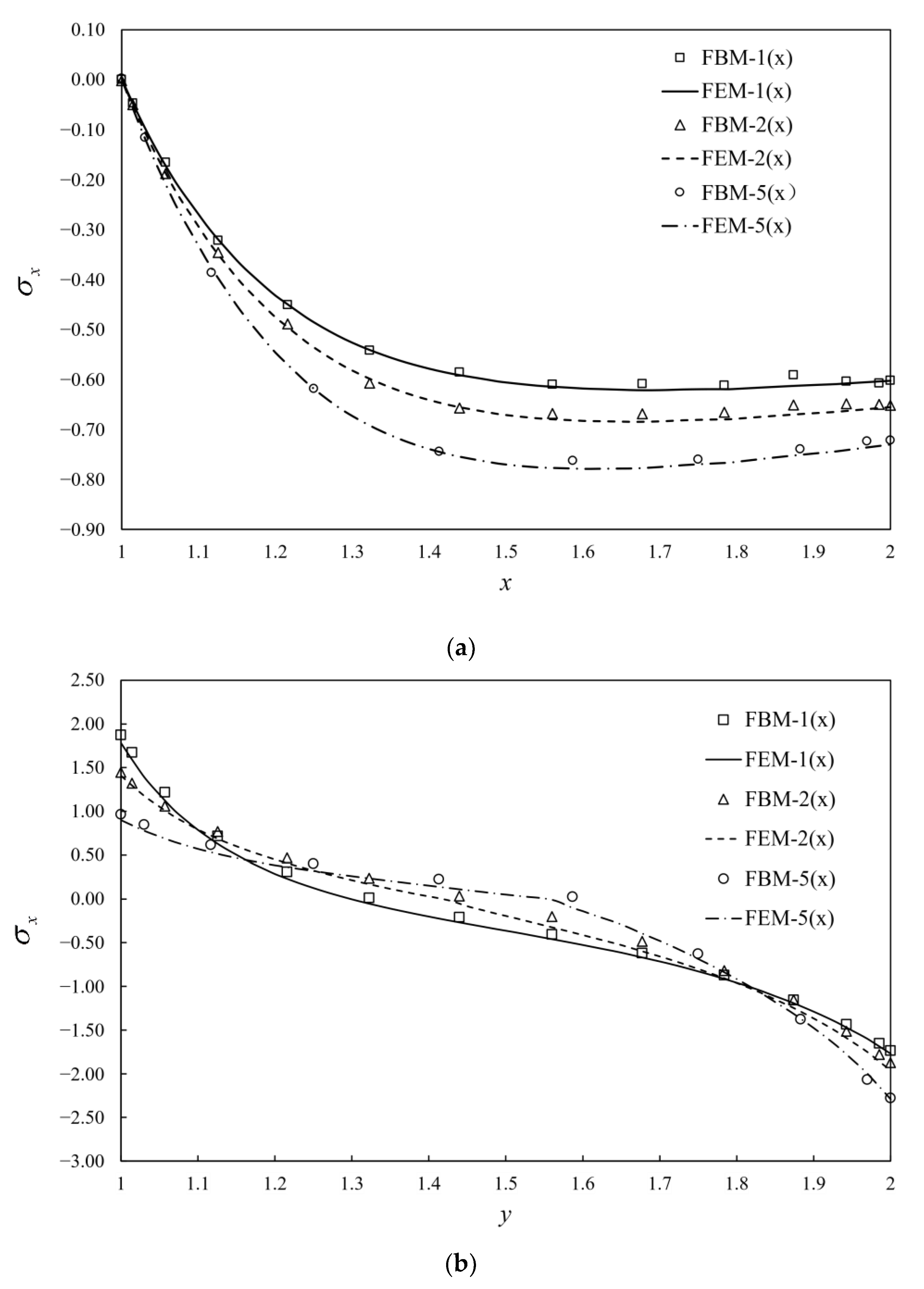
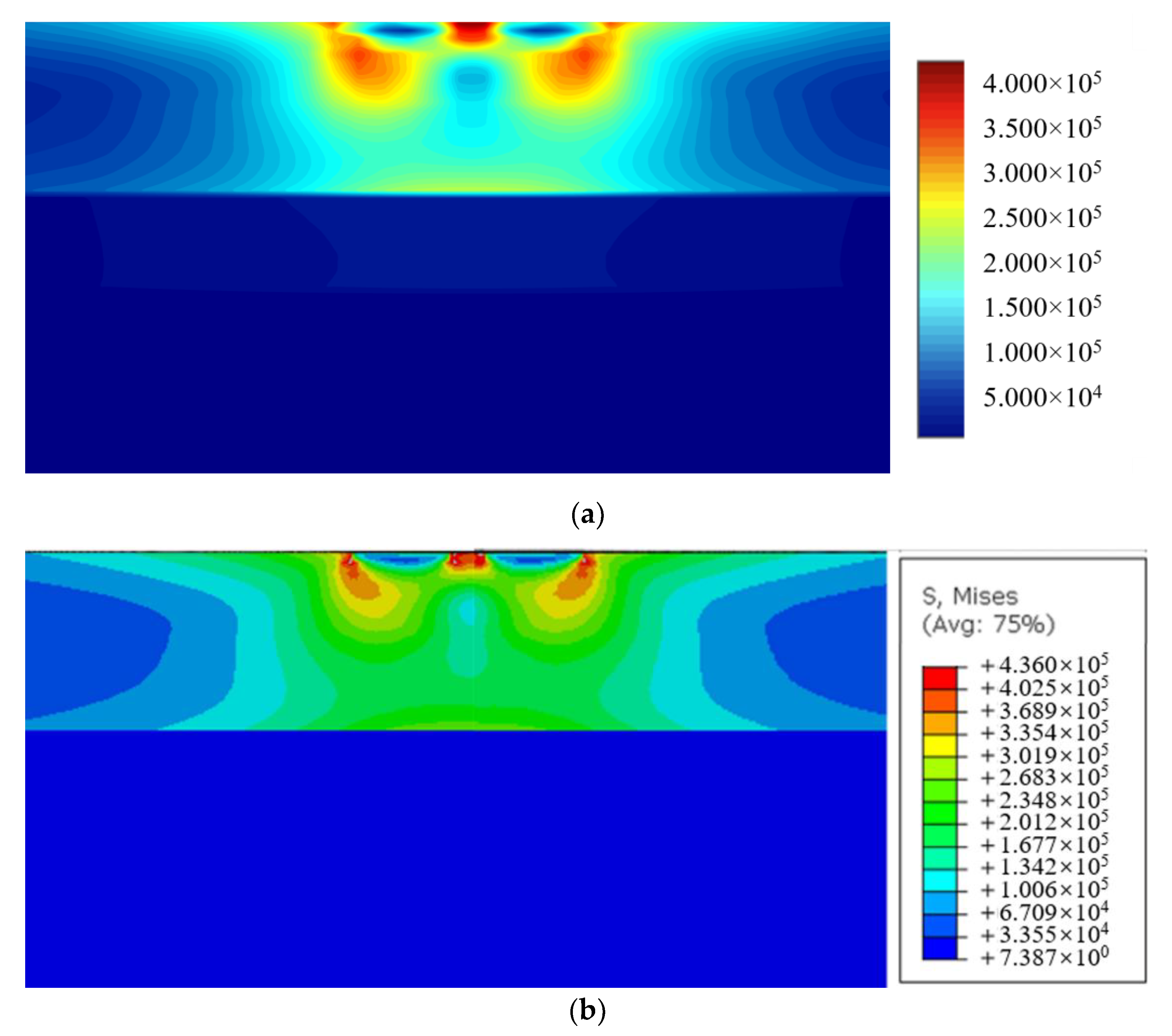
5.2. Arch Bridge in Bimodular Materials
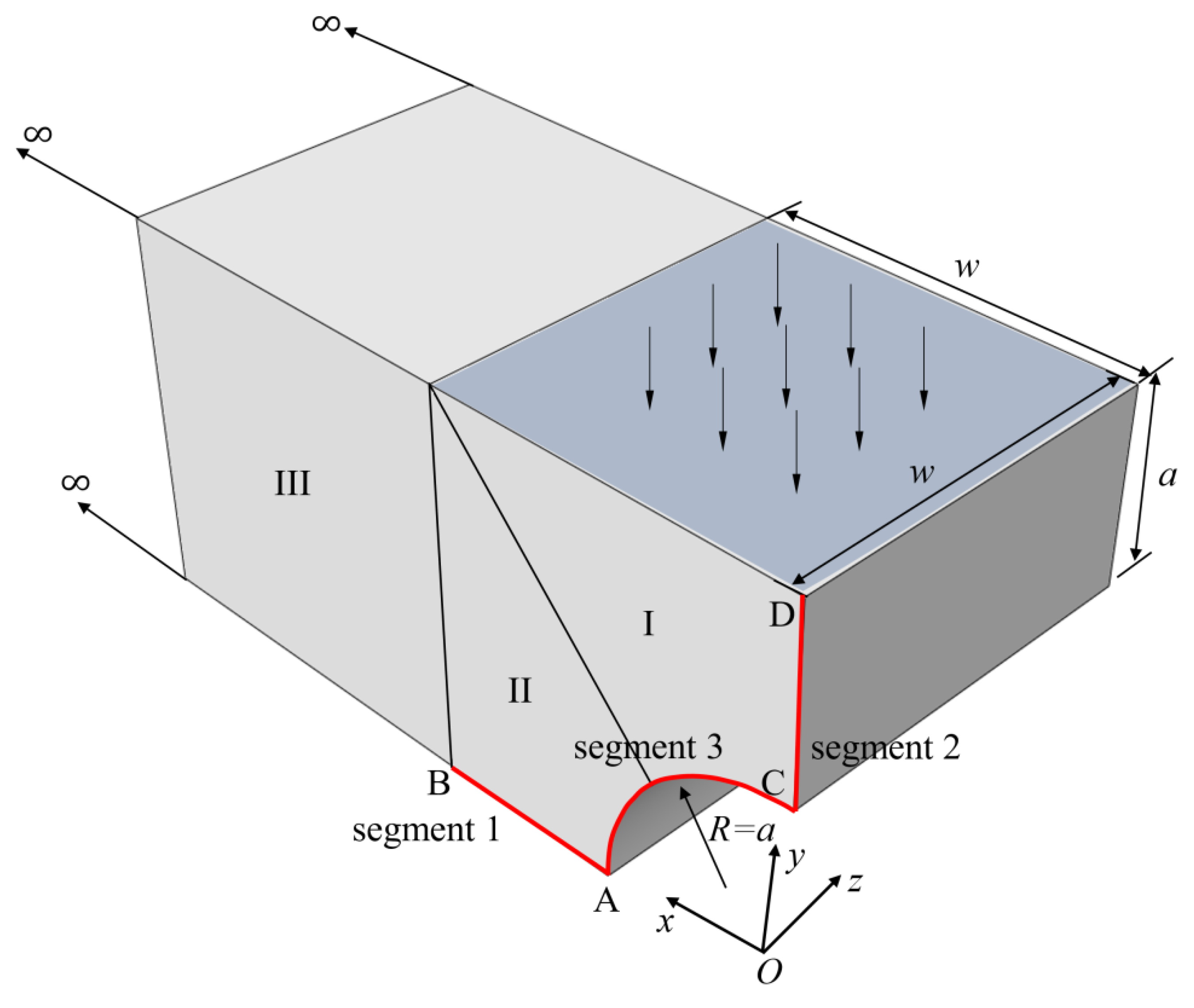
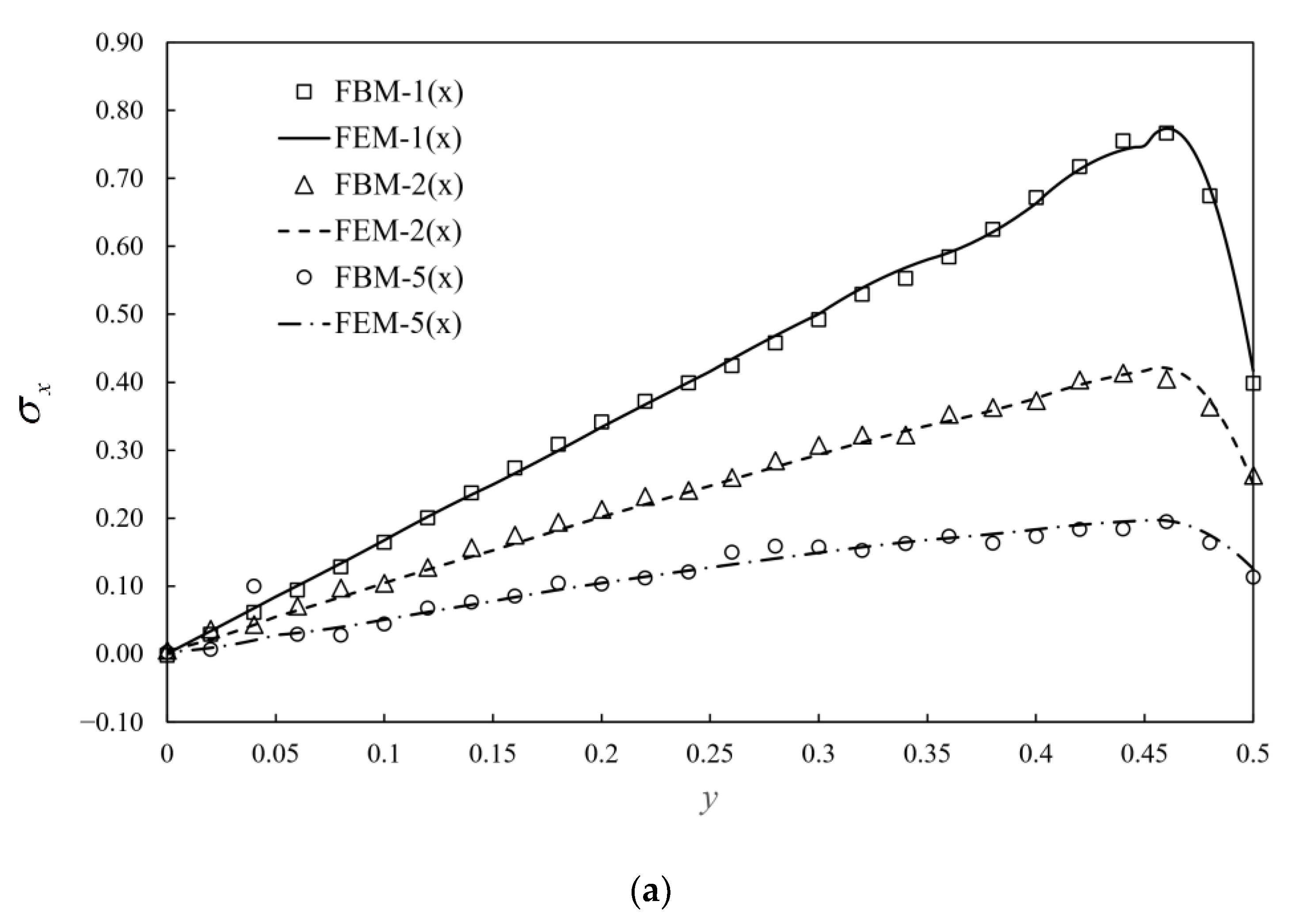
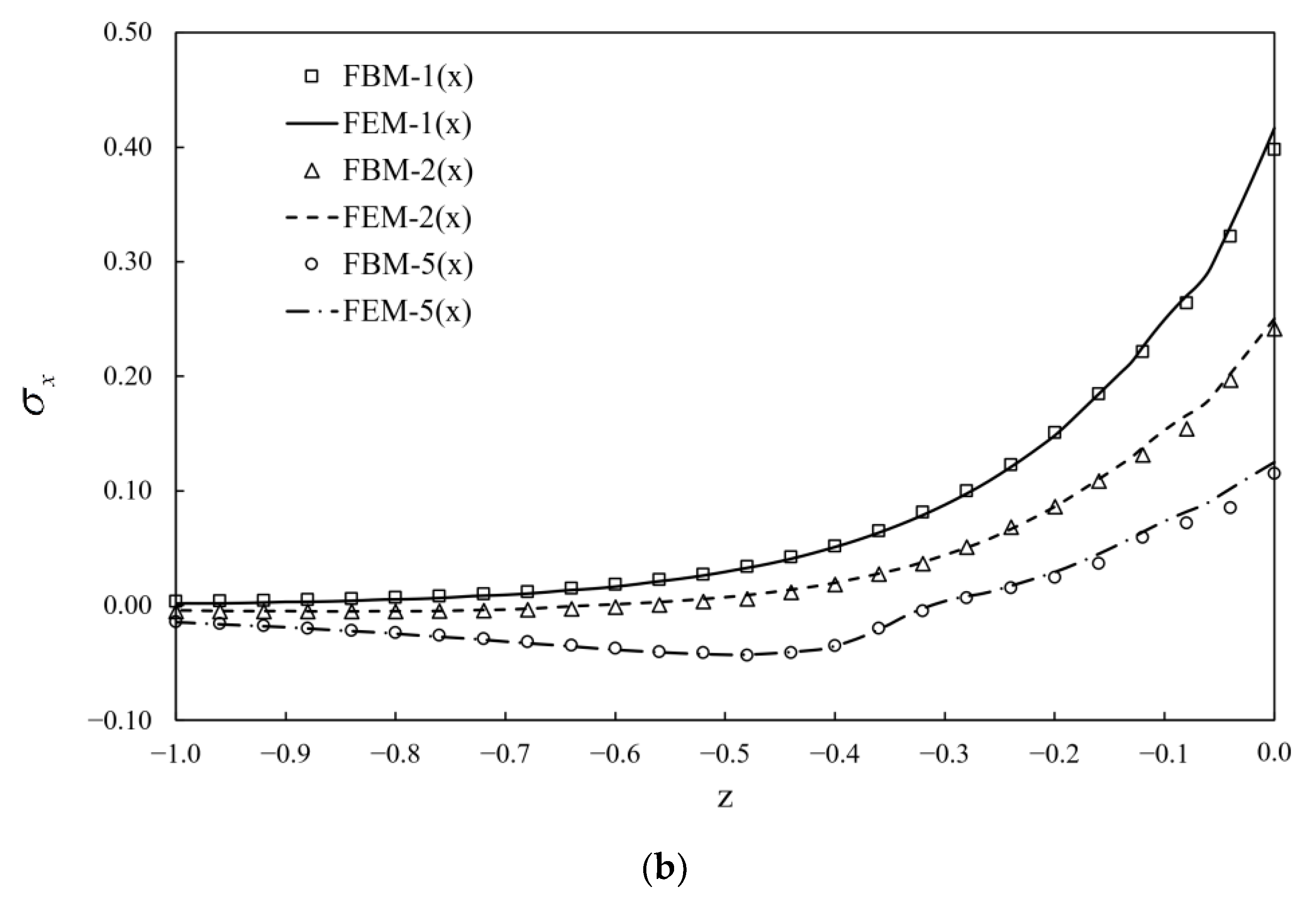
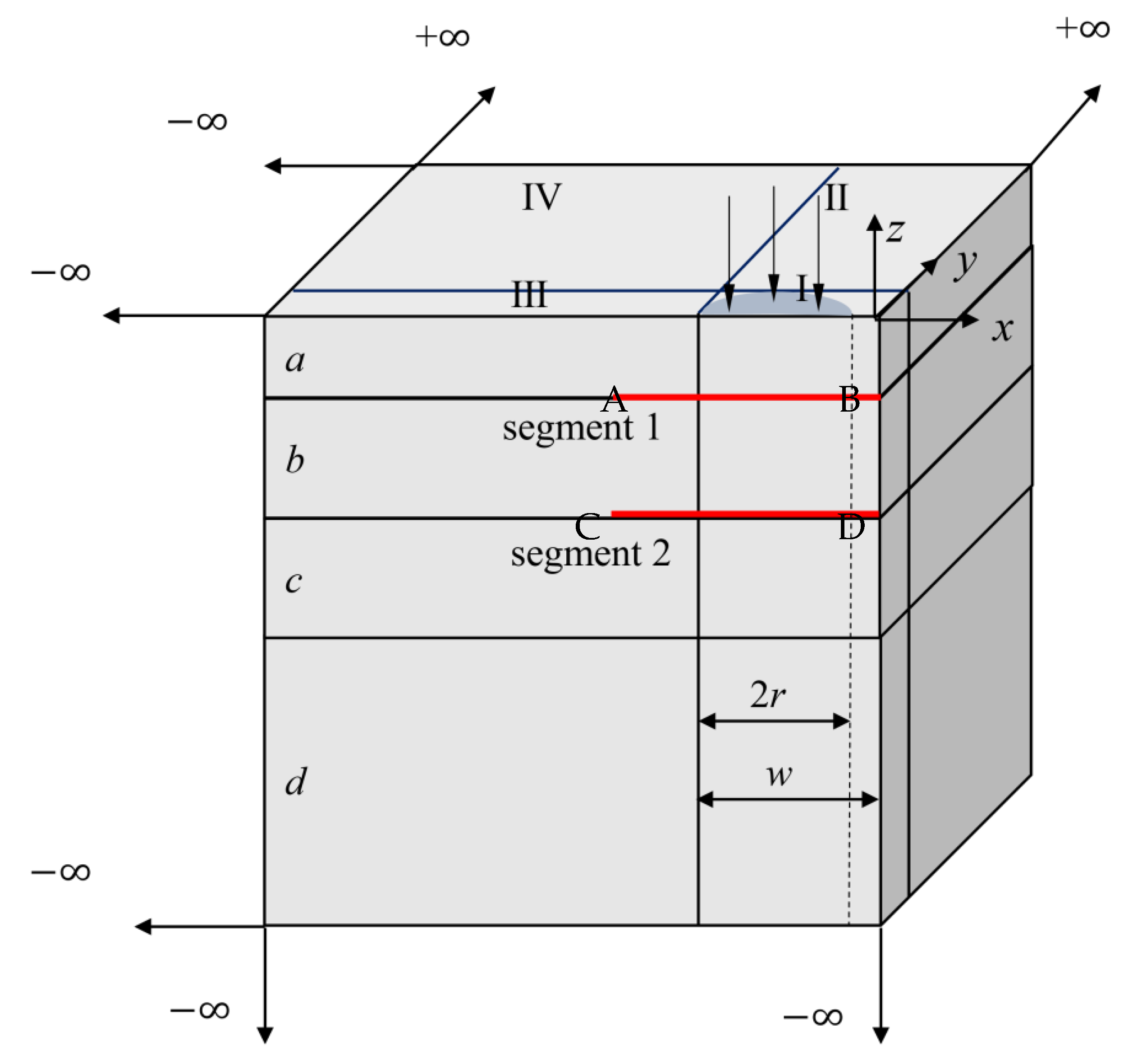
5.3. A Semi-Infinite Solid with Bimodular Materials
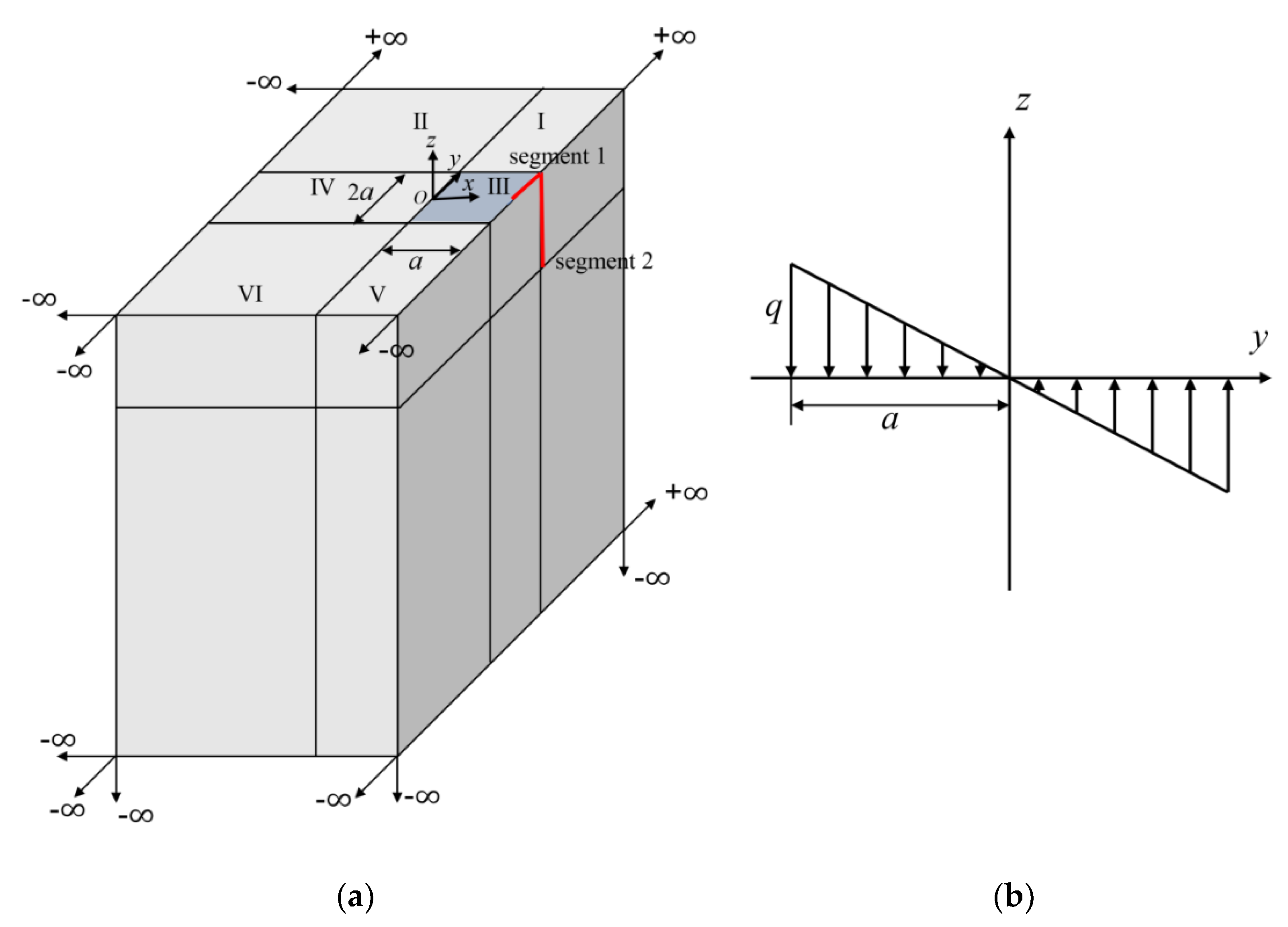
5.4. Multi-Layered Infinite Model with Bimodular Materials
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
- 1.
- 20-node finite block
- 2.
- 12-seed-one-edge-infinite block
- 3.
- 7-seed-two-edge-infinite block
- 4.
- 8-seed-three-edge-infinite block
References
- Ambartsumyan, S.A. Multimodulus Elasticity Theory; Nauka: Moscow, Russia, 1982. (In Russian) [Google Scholar]
- Jones, R.M. Stress-strain relations for materials with different moduli in tension and compression. Aiaa J. 1977, 15, 16–23. [Google Scholar] [CrossRef]
- Medri, G.A. Nonlinear Elastic Model for Isotropic Materials with Different Behavior in Tension and Compression. J. Eng. Mater. Technol. 1982, 104, 26–28. [Google Scholar] [CrossRef]
- Bert, C.W.; Gordaninejad, F. Transverse shear effects in bimodular composite laminates. J. Compos. Mater. 1983, 17, 282–298. [Google Scholar] [CrossRef] [Green Version]
- Bert, C.W. Models for fibrous composites with different properties in tension and compression. J. Eng. Mater. Technol. 1977, 99, 344–349. [Google Scholar] [CrossRef]
- Zheng, J.L. New structure design of durable asphalt pavement based on life increment [in Chinese]. China J. Highw. Transp. 2014, 27, 1–7. [Google Scholar]
- Wen, P.H.; Yang, J.J.; Huang, T.; Zheng, J.L.; Deng, Y.J. Infinite element in meshless approaches. Eur. J. Mech. -A/Solids 2018, 72, 175–185. [Google Scholar] [CrossRef]
- Von Estorff, O.; Firuziaan, M.; Friedrich, K.; Pflanz, G.; Schmid, G. A three-dimensional FEM/BEM model for the investigation of railway tracks. In Wave propagation Moving load–Vibration Reduction; CRC Press: Boca Raton, FL, USA, 2021; pp. 157–171. [Google Scholar]
- Zheng, C.J.; Bi, C.X.; Zhang, C.; Gao, H.F.; Chen, H.B. Free vibration analysis of elastic structures submerged in an infinite or semi-infinite fluid domain by means of a coupled FE–BE solver. J. Comput. Phys. 2018, 359, 183–198. [Google Scholar] [CrossRef]
- Wilson, E.L. Structural analysis of axisymmetric solids. Aiaa J. 1965, 3, 2269–2274. [Google Scholar] [CrossRef]
- Winnicki, L.A.; Zienkiewicz, O.C. Plastic (or visco-plastic) behaviour of axisymmetric bodies subjected to non-symmetric loading—semi-analytical finite element solution. Int. J. Numer. Methods Eng. 1979, 14, 1399–1412. [Google Scholar] [CrossRef]
- Liu, P.; Xing, Q.; Dong, Y.; Wang, D.; Oeser, M.; Yuan, S. Application of finite layer method in pavement structural analysis. Appl. Sci. 2017, 7, 611. [Google Scholar] [CrossRef] [Green Version]
- Liu, P.; Wang, D.; Oeser, M. Application of semi-analytical finite element method coupled with infinite element for analysis of asphalt pavement structural response. J. Traffic Transp. Eng. (Engl. Ed.) 2015, 2, 48–58. [Google Scholar] [CrossRef] [Green Version]
- Wood, W.L. On the finite element solution of an exterior boundary value problem. Int. J. Numer. Methods Eng. 1976, 10, 885–891. [Google Scholar] [CrossRef]
- Bettess, P.; Zienkiewicz, O.C. Diffraction and refraction of surface waves using finite and infinite elements. Int. J. Numer. Methods Eng. 1977, 11, 1271–1290. [Google Scholar] [CrossRef]
- Selvadurai, A.P.S.; Karpurapu, R. Composite infinite element for modeling unbounded saturated-soil media. J. Geotech. Eng. 1989, 115, 1633–1646. [Google Scholar] [CrossRef] [Green Version]
- Bettess, P. Infinite elements. Int. J. Numer. Methods Eng. 1977, 11, 53–64. [Google Scholar] [CrossRef]
- Damjanlć, F.; Owen, D.R.J. Mapped infinite elements in transient thermal analysis. Comput. Struct. 1984, 19, 673–687. [Google Scholar] [CrossRef]
- Zienkiewicz, O.C.; Bando, K.; Bettess, P.; Emson, C.; Chiam, T.C. Mapped infinite elements for exterior wave problems. Int. J. Numer. Methods Eng. 1985, 21, 1229–1251. [Google Scholar] [CrossRef]
- Simoni, L.; Schrefler, B.A. Mapped infinite elements in soil consolidation. Int. J. Numer. Methods Eng. 1987, 24, 513–527. [Google Scholar] [CrossRef]
- Du, Z.; Zhang, Y.; Zhang, W.; Guo, X. A new computational framework for materials with different mechanical responses in tension and compression and its applications. Int. J. Solids Struct. 2016, 100, 54–73. [Google Scholar] [CrossRef]
- Pan, Q.; Zheng, J.; Wen, P. Efficient algorithm for 3D bimodulus structures. Acta Mech. Sin. 2020, 36, 143–159. [Google Scholar] [CrossRef]
- Zhang, L.; Zhang, H.W.; Wu, J.; Yan, B. A stabilized complementarity formulation for nonlinear analysis of 3D bimodular materials. Acta Mech. Sin. 2016, 32, 481–490. [Google Scholar] [CrossRef]
- Zhang, L.; Dong, K.J.; Zhang, H.T.; Yan, B. A 3D PVP co-rotational formulation for large-displacement and small-strain analysis of bi-modulus materials. Finite Elem. Anal. Des. 2016, 110, 20–31. [Google Scholar] [CrossRef]
- Yang, H.; Wang, B. An analysis of longitudinal vibration of bimodular rod via smoothing function approach. J. Sound Vib. 2008, 317, 419–431. [Google Scholar] [CrossRef]
- He, X.T.; Cao, L.; Sun, J.Y.; Zheng, Z.L. Application of a biparametric perturbation method to large-deflection circular plate problems with a bimodular effect under combined loads. J. Math. Anal. Appl. 2014, 420, 48–65. [Google Scholar] [CrossRef]
- Nayroles, B.; Touzot, G.; Villon, P. Generalizing the finite element method: Diffuse approximation and diffuse elements. Comput. Mech. 1992, 10, 307–318. [Google Scholar] [CrossRef]
- Belytschko, T.; Lu, Y.Y.; Gu, L. Element-free Galerkin methods. Int. J. Numer. Methods Eng. 1994, 37, 229–256. [Google Scholar] [CrossRef]
- Belytschko, T.; Tabbara, M. Dynamic fracture using element-free Galerkin methods. Int. J. Numer. Methods Eng. 1996, 39, 923–938. [Google Scholar] [CrossRef]
- Fleming, M.; Chu, Y.A.; Moran, B.; Belytschko, T. Enriched element-free Galerkin methods for crack tip fields. Int. J. Numer. Methods Eng. 1997, 40, 1483–1504. [Google Scholar] [CrossRef]
- Belytschko, T.; Krongauz, Y.; Organ, D.; Fleming, M.; Krysl, P. Meshless methods: An overview and recent developments. Comput. Methods Appl. Mech. Eng. 1996, 139, 3–47. [Google Scholar] [CrossRef] [Green Version]
- Atluri, S.N.; Zhu, T. A new meshless local Petrov-Galerkin (MLPG) approach in computational mechanics. Comput. Mech. 1998, 22, 117–127. [Google Scholar] [CrossRef]
- Liu, W.K.; Jun, S.; Zhang, Y.F. Reproducing kernel particle methods. Int. J. Numer. Methods Fluids 1995, 20, 1081–1106. [Google Scholar] [CrossRef]
- Liu, W.K.; Jun, S.; Li, S.; Adee, J.; Belytschko, T. Reproducing kernel particle methods for structural dynamics. Int. J. Numer. Methods Eng. 1995, 38, 1655–1679. [Google Scholar] [CrossRef]
- Liu, W.K.; Chen, Y.; Jun, S.; Chen, J.S.; Belytschko, T.; Pan, C.; Uras, R.A.; Chang, C. Overview and applications of the reproducing kernel particle methods. Arch. Comput. Methods Eng. 1996, 3, 3–80. [Google Scholar] [CrossRef]
- Yang, J.J.; Zheng, J.L.; Wen, P.H. Generalized method of fundamental solutions (GMFS) for boundary value problems. Eng. Anal. Bound. Elem. 2018, 94, 25–33. [Google Scholar] [CrossRef]
- Chen, C.S.; Fan, C.M.; Wen, P.H. The method of approximate particular solutions for solving certain partial differential equations. Numer. Methods Part. Differ. Equ. 2012, 28, 506–522. [Google Scholar] [CrossRef]
- Chen, C.S.; Fan, C.M.; Wen, P.H. The method of approximate particular solutions for solving elliptic problems with variable coefficients. Int. J. Comput. Methods 2011, 8, 545–559. [Google Scholar] [CrossRef]
- Liu, G.R.; Zhang, G.Y.; Gu, Y.; Wang, Y.Y. A meshfree radial point interpolation method (RPIM) for three-dimensional solids. Comput. Mech. 2005, 36, 421–430. [Google Scholar] [CrossRef]
- Shivanian, E. Analysis of meshless local radial point interpolation (MLRPI) on a nonlinear partial integro-differential equation arising in population dynamics. Eng. Anal. Bound. Elem. 2013, 37, 1693–1702. [Google Scholar] [CrossRef]
- Wu, Y.L.; Liu, G.R. A meshfree formulation of local radial point interpolation method (LRPIM) for incompressible flow simulation. Comput. Mech. 2003, 30, 355–365. [Google Scholar]
- Fan, C.M.; Chien, C.S.; Chan, H.F.; Chiu, C.L. The local RBF collocation method for solving the double-diffusive natural convection in fluid-saturated porous media. Int. J. Heat Mass Transf. 2013, 57, 500–503. [Google Scholar] [CrossRef]
- Kosec, G.; Sarler, B. Local RBF collocation method for Darcy flow. Comput. Model. Eng. Sci. 2008, 25, 197. [Google Scholar]
- Li, M.; Chen, W.; Chen, C.S. The localized RBFs collocation methods for solving high dimensional PDEs. Eng. Anal. Bound. Elem. 2013, 37, 1300–1304. [Google Scholar] [CrossRef]
- Yang, J.; Zheng, J. Intervention-point principle of meshless method. Chin. Sci. Bull. 2013, 58, 478–485. [Google Scholar] [CrossRef] [Green Version]
- Wen, P.H.; Cao, P.; Korakianitis, T. Finite block method in elasticity. Eng. Anal. Bound. Elem. 2014, 46, 116–125. [Google Scholar] [CrossRef]
- Wang, Z.; Yu, T.; Wang, X.; Zhang, T.; Zhao, J.; Wen, P.H. Grinding temperature field prediction by meshless finite block method with double infinite element. Int. J. Mech. Sci. 2019, 153, 131–142. [Google Scholar] [CrossRef]
- Li, Y.; Li, J.; Wen, P.H. Finite and infinite block Petrov–Galerkin method for cracks in functionally graded materials. Appl. Math. Model. 2019, 68, 306–326. [Google Scholar] [CrossRef]
- Li, J.; Liu, J.Z.; Korakianitis, T.; Wen, P.H. Finite block method in fracture analysis with functionally graded materials. Eng. Anal. Bound. Elem. 2017, 82, 57–67. [Google Scholar] [CrossRef]
- Huang, T.; Pan, Q.X.; Jin, J.; Zheng, J.L.; Wen, P.H. Continuous constitutive model for bimodulus materials with meshless approach. Appl. Math. Model. 2019, 66, 41–58. [Google Scholar] [CrossRef]
- Marques, J.M.M.C. Finite and Infinite Elements in Static and Dynamic Structural Analysis. PhD Thesis, Swansea University (formerly University of Wales, Swansea), Wales, UK, 1984. [Google Scholar]
- Kay, S.; Bettess, P. Revised mapping functions for three-dimensional serendipity infinite elements. Commun. Numer. Methods Eng. 1996, 12, 181–184. [Google Scholar] [CrossRef]

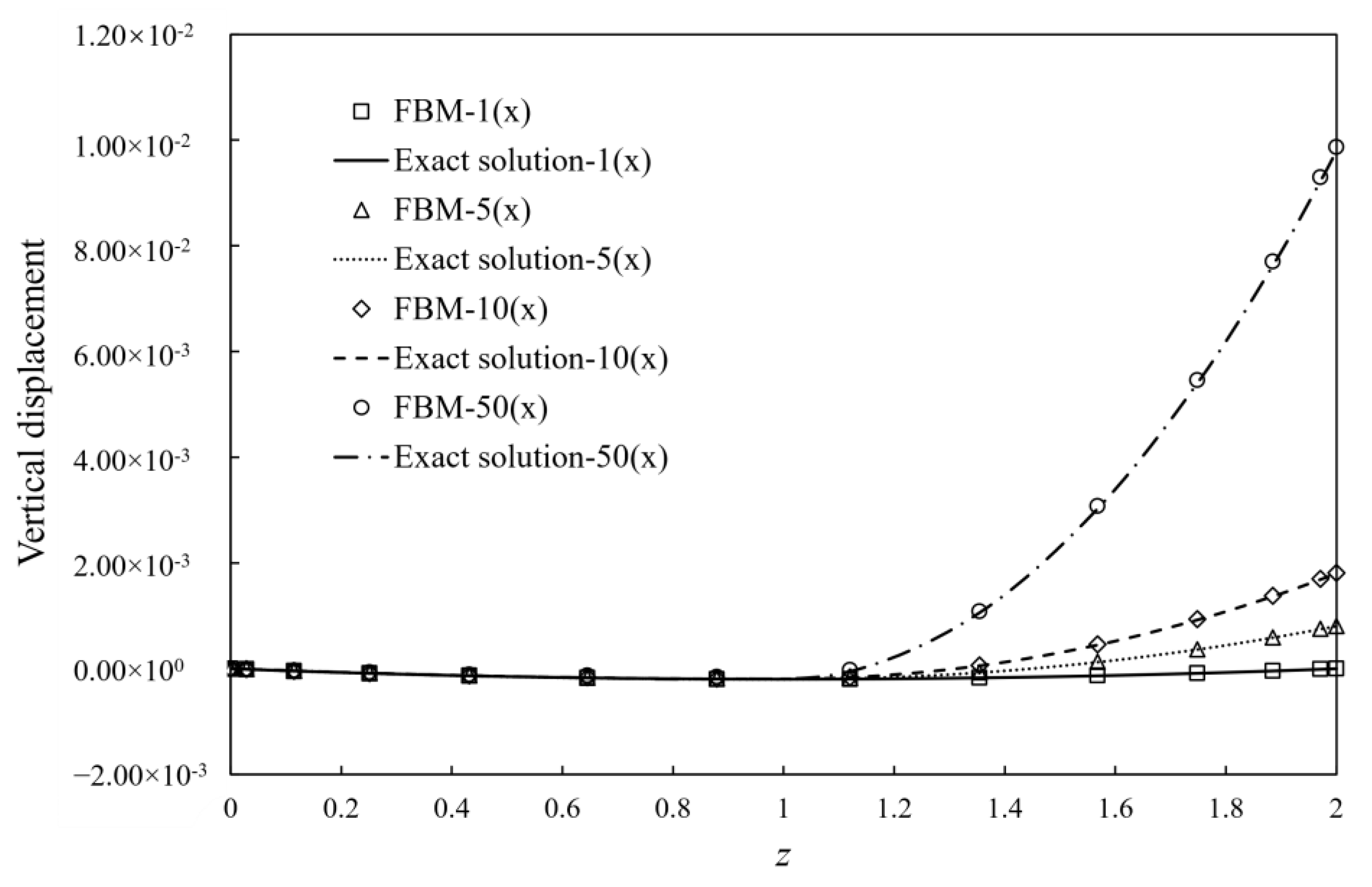




| z = 1.96 | Number of Iterations for Convergence | |||
|---|---|---|---|---|
| Exact Solution | FBM Solution | FEM | FBM | |
| 1 | 1.59 × 10−5 | 1.59 × 10−5 | 2 | 2 |
| 5 | 7.21 × 10−4 | 7.20 × 10−4 | 2 | 2 |
| 10 | 1.6 × 10−3 | 1.60 × 10−3 | 2 | 2 |
| 50 | 9.0 × 10−3 | 8.9 × 10−3 | 2 | 2 |
| Node Density | Number of Iterationsfor Convergence | |
|---|---|---|
| (3 × 3 × 6) | – | – |
| (4 × 4 × 8) | 5.20 × 10−5 | 2 |
| (5 × 5 × 10) | 1.29 × 10−5 | 2 |
| (7 × 7 × 14) | 6.24 × 10−6 | 2 |
| (9 × 9 × 18) | 3.65 × 10−6 | 2 |
| (11 × 11 × 22) | 2.39 × 10−6 | 2 |
| Case | Young’s Modulus | Poisson’s Ratio |
|---|---|---|
| 1 | 1/1 | 0.4/0.4 |
| 2 | 0.5/1 | 0.2/0.4 |
| 3 | 0.2/1 | 0.08/0.4 |
| Layer | Height (m) | ||
|---|---|---|---|
| a | 0.18 | 6000/9000 | 0.2/0.3 |
| b | 0.2 | 5000/8000 | 0.15625/0.25 |
| c | 0.2 | 300/300 | 0.35/0.35 |
| d | 80/80 | 0.4 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Huang, W.; Yang, J.; Sladek, J.; Sladek, V.; Wen, P. Semi-Infinite Structure Analysis with Bimodular Materials with Infinite Element. Materials 2022, 15, 641. https://doi.org/10.3390/ma15020641
Huang W, Yang J, Sladek J, Sladek V, Wen P. Semi-Infinite Structure Analysis with Bimodular Materials with Infinite Element. Materials. 2022; 15(2):641. https://doi.org/10.3390/ma15020641
Chicago/Turabian StyleHuang, Wang, Jianjun Yang, Jan Sladek, Vladimir Sladek, and Pihua Wen. 2022. "Semi-Infinite Structure Analysis with Bimodular Materials with Infinite Element" Materials 15, no. 2: 641. https://doi.org/10.3390/ma15020641
APA StyleHuang, W., Yang, J., Sladek, J., Sladek, V., & Wen, P. (2022). Semi-Infinite Structure Analysis with Bimodular Materials with Infinite Element. Materials, 15(2), 641. https://doi.org/10.3390/ma15020641







