Modulational Instability of Delocalized Modes in fcc Copper
Abstract
:1. Introduction
2. Simulation Procedure
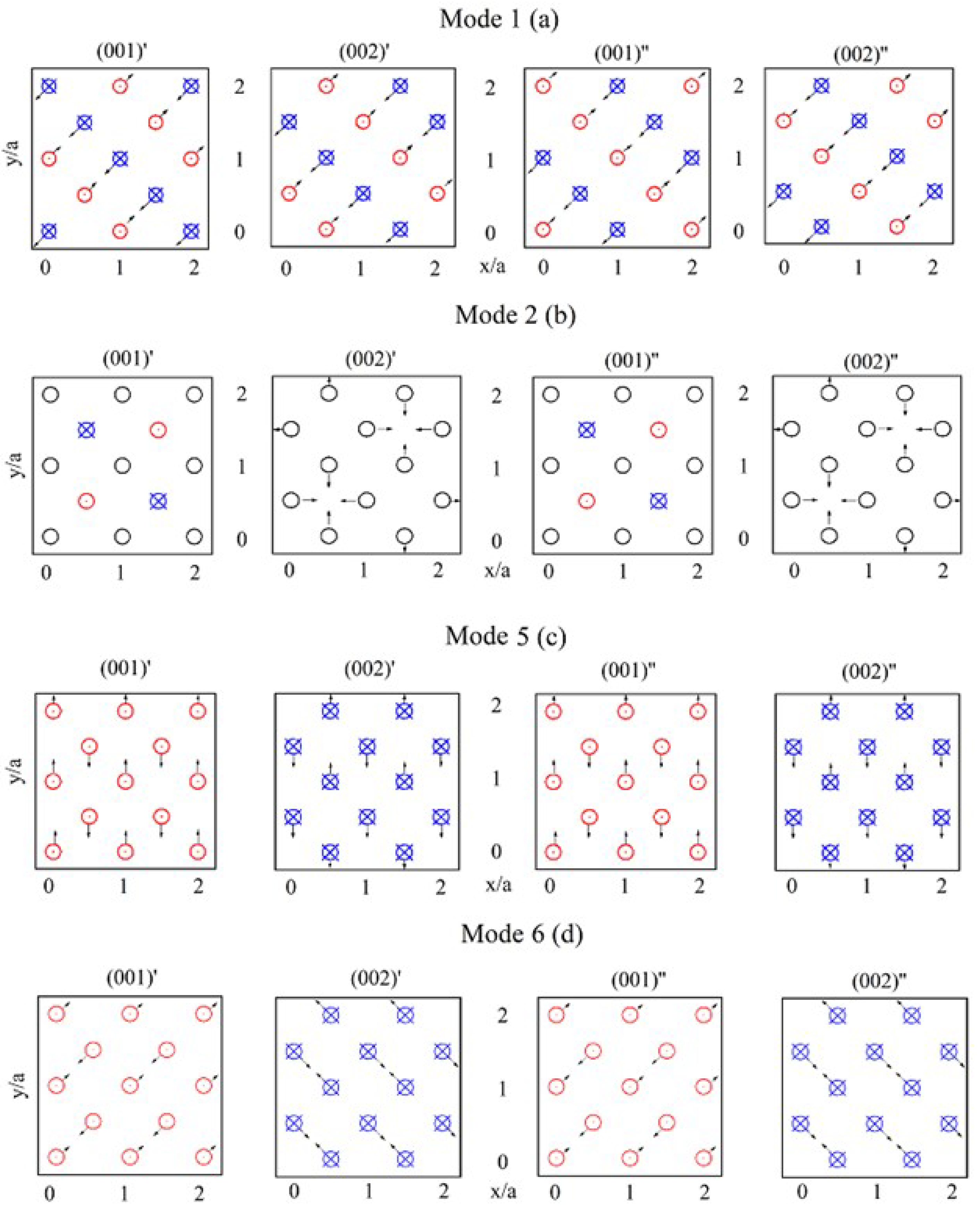
3. Results and Discussion
3.1. Frequency vs. Amplitude
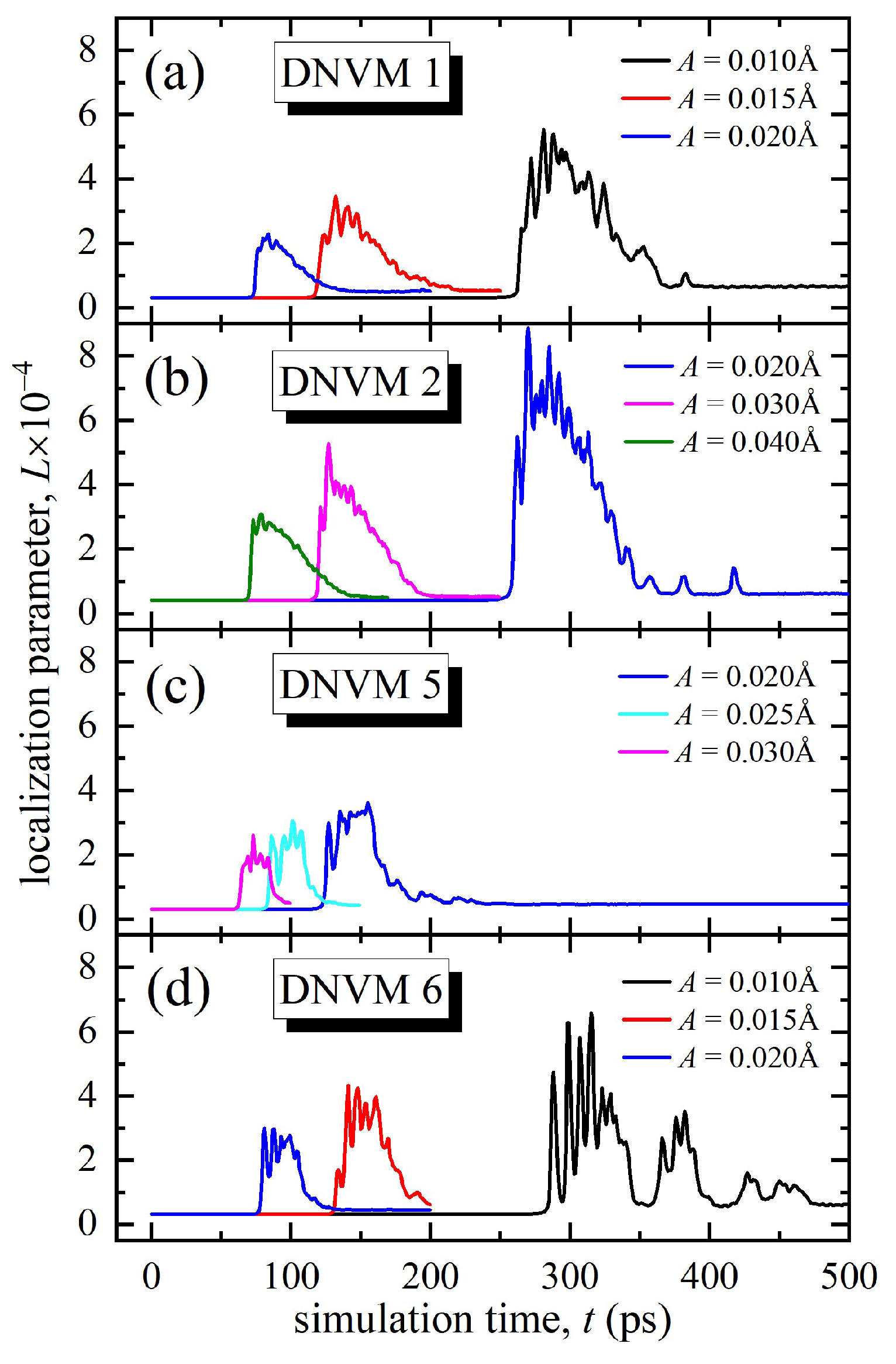
3.2. Localization Parameter
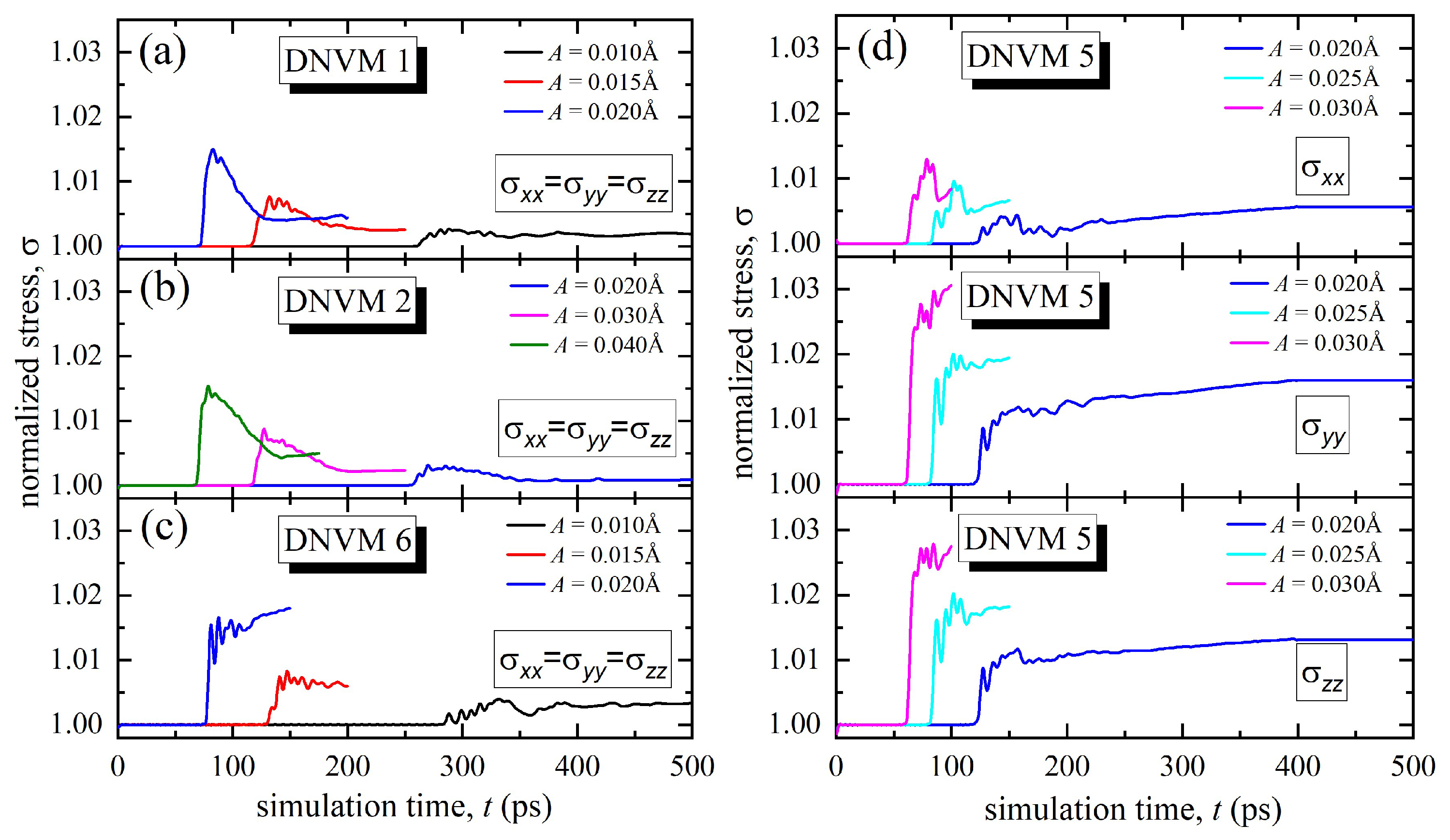
3.3. Stresses and Energies
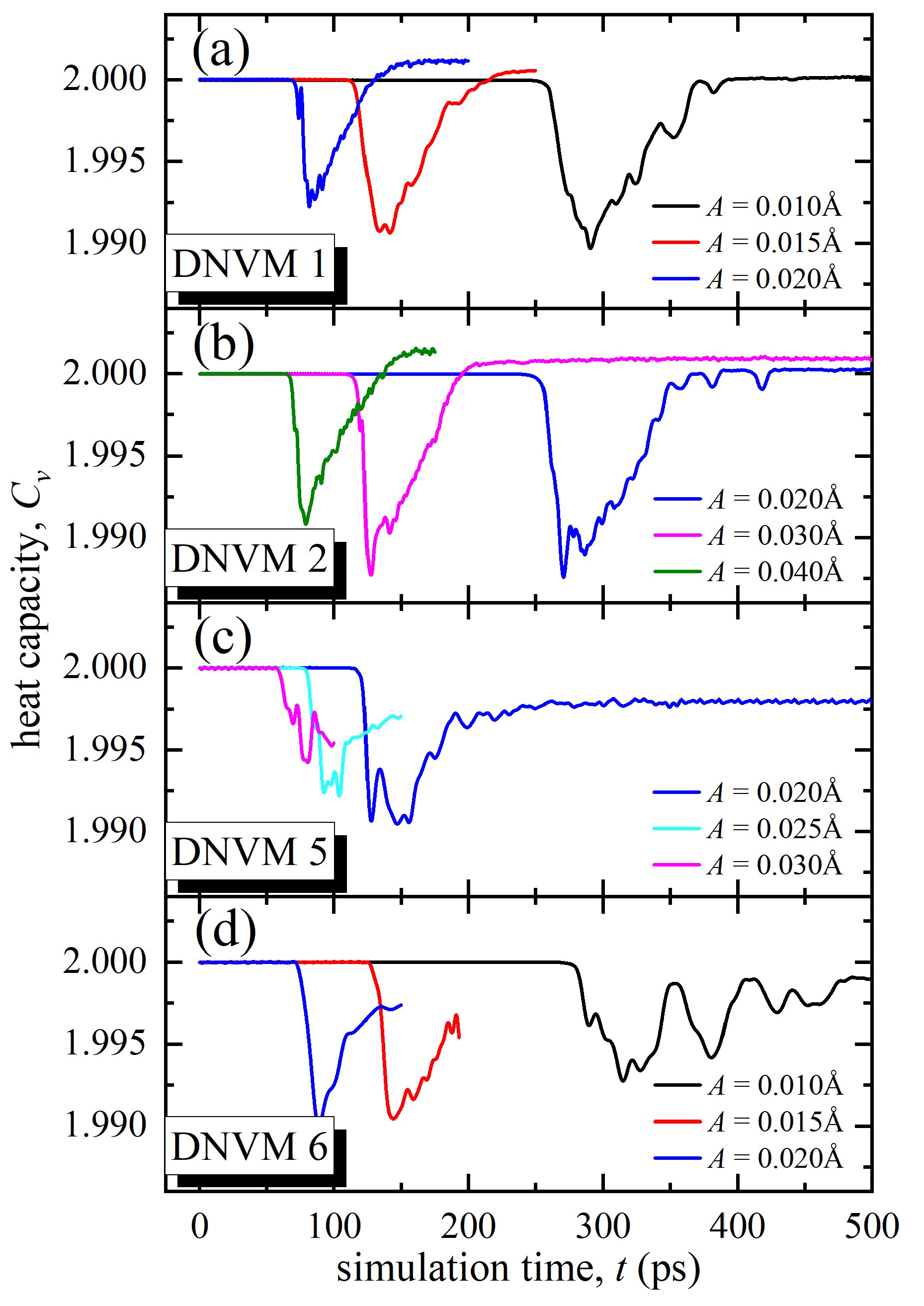
3.4. Heat Capacity
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| DB | Discrete breathers |
| DNVM | Delocalized nonlinear vibrational mode |
References
- Dolgov, A.S. The localization of vibrations in a nonlinear crystal structure. Sov. Phys. Solid State 1986, 28, 907–910. [Google Scholar]
- Takeno, S.; Sievers, A.J. Anharmonic Resonant Modes in Perfect Crystals. Solid State Commun. 1988, 67, 1023–1026. [Google Scholar] [CrossRef]
- Manley, M.E.; Yethiraj, M.; Sinn, H.; Volz, H.M.; Alatas, A.; Lashley, J.C.; Hults, W.L.; Lander, G.H.; Smith, J.L. Formation of a new dynamical mode in alpha-uranium observed by inelastic x-ray and neutron scattering. Phys. Rev. Lett. 2006, 96, 125501. [Google Scholar] [CrossRef] [PubMed]
- Manley, M.E.; Sievers, A.J.; Lynn, J.W.; Kiselev, S.A.; Agladze, N.I.; Chen, Y.; Llobet, A.; Alatas, A. Intrinsic localized modes observed in the high-temperature vibrational spectrum of NaI. Phys. Rev. B 2009, 79, 134304. [Google Scholar] [CrossRef]
- Manley, M.E. Impact of intrinsic localized modes of atomic motion on materials properties. Acta Mater. 2010, 58, 2926–2935. [Google Scholar] [CrossRef]
- Xiong, D.X.; Saadatmand, D.; Dmitriev, S.V. Crossover from ballistic to normal heat transport in the phi(4) lattice: If nonconservation of momentum is the reason, what is the mechanism? Phys. Rev. E 2017, 96, 042109. [Google Scholar] [CrossRef] [PubMed]
- Singh, M.; Morkina, A.Y.; Korznikova, E.A.; Dubinko, V.I.; Terentiev, D.A.; Xiong, D.X.; Naimark, O.B.; Gani, V.A.; Dmitriev, S.V. Effect of Discrete Breathers on the Specific Heat of a Nonlinear Chain. J. Nonlinear Sci. 2021, 31, 12. [Google Scholar] [CrossRef]
- Korznikova, E.A.; Morkina, A.Y.; Singh, M.; Krivtsov, A.M.; Kuzkin, V.A.; Gani, V.A.; Bebikhov, Y.V.; Dmitriev, S.V. Effect of discrete breathers on macroscopic properties of the Fermi-Pasta-Ulam chain. Eur. Phys. J. B 2020, 93, 123. [Google Scholar] [CrossRef]
- Chechin, G.M. Computers and Group-Theoretical Methods for Studying Structural Phase-Transitions. Comput. Math. Appl. 1989, 17, 255–278. [Google Scholar] [CrossRef]
- Chechin, G.M.; Ryabov, D.S.; Shcherbinin, S.A. Large-amplitude in-plane atomic vibrations in strained graphene monolayer: Bushes of nonlinear normal modes. Lett. Mater. 2017, 7, 367–372. [Google Scholar] [CrossRef]
- Chechin, G.M.; Sakhnenko, V.P. Interactions between normal modes in nonlinear dynamical systems with discrete symmetry. Exact results. Phys. D Nonlinear Phenom. 1998, 117, 43–76. [Google Scholar] [CrossRef]
- Krylova, K.A.; Lobzenko, I.P.; Semenov, A.S.; Kudreyko, A.A.; Dmitriev, S.V. Spherically localized discrete breathers in bcc metals V and Nb. Comp. Mater. Sci. 2020, 180, 109695. [Google Scholar] [CrossRef]
- Bachurina, O.V.; Murzaev, R.T.; Bachurin, D.V. Molecular dynamics study of two-dimensional discrete breather in nickel. J. Micromech. Mol. Phys. 2019, 3, 1950001. [Google Scholar] [CrossRef]
- Bachurina, O.V.; Kudreyko, A.A. Two-dimensional discrete breathers in fcc metals. Comp. Mater. Sci. 2020, 182, 109737. [Google Scholar] [CrossRef]
- Bachurina, O.V.; Kudreyko, A.A. Two-component localized vibrational modes in fcc metals. Eur. Phys. J. B 2021, 94, 218. [Google Scholar] [CrossRef]
- Shcherbinin, S.A.; Semenova, M.N.; Semenov, A.S.; Korznikova, E.A.; Chechin, G.M.; Dmitriev, S.V. Dynamics of a Three-Component Delocalized Nonlinear Vibrational Mode in Graphene. Phys. Solid State 2019, 61, 2139–2144. [Google Scholar] [CrossRef]
- Chechin, G.M.; Ryabov, D.S.; Zhukov, K.G. Stability of low-dimensional bushes of vibrational modes in the Fermi-Pasta-Ulam chains. Phys. D Nonlinear Phenom. 2005, 203, 121–166. [Google Scholar] [CrossRef]
- Chechin, G.M.; Sizintsev, D.A.; Usoltsev, O.A. Nonlinear atomic vibrations and structural phase transitions in strained carbon chains. Comp. Mater. Sci. 2017, 138, 353–367. [Google Scholar] [CrossRef]
- Barani, E.; Lobzenko, I.P.; Korznikova, E.A.; Soboleva, E.G.; Dmitriev, S.V.; Zhou, K.; Marjaneh, A.M. Transverse discrete breathers in unstrained graphene. Eur. Phys. J. B 2017, 90, 38. [Google Scholar] [CrossRef]
- Korznikova, E.A.; Shcherbinin, S.A.; Ryabov, D.S.; Chechin, G.M.; Ekomasov, E.G.; Barani, E.; Zhou, K.; Dmitriev, S.V. Delocalized nonlinear vibrational modes in graphene: Second harmonic generation and negative pressure. Phys. Status Solidi B 2018, 256, 1800061. [Google Scholar] [CrossRef]
- Chechin, G.M.; Ryabov, D.S.; Shcherbinin, S.A. Large-amplitude periodic atomic vibrations in diamond. J. Micromech. Mol. Phys. 2018, 3, 1850002. [Google Scholar] [CrossRef]
- Bachurina, O.V. Linear discrete breather in fcc metals. Comp. Mater. Sci. 2019, 160, 217–221. [Google Scholar] [CrossRef]
- Bachurina, O.V. Plane and plane-radial discrete breathers in fcc metals. Model. Simul. Mater Sci. Eng. 2019, 27, 055001. [Google Scholar] [CrossRef]
- Shcherbinin, S.A.; Krylova, K.A.; Chechin, G.M.; Soboleva, E.G.; Dmitriev, S.V. Delocalized nonlinear vibrational modes in fcc metals. Commun. Nonlinear Sci. 2022, 104, 106039. [Google Scholar] [CrossRef]
- Cretegny, T.; Dauxois, T.; Ruffo, S.; Torcini, A. Localization and equipartition of energy in the beta-FPU chain: Chaotic breathers. Phys. D Nonlinear Phenom. 1998, 121, 109–126. [Google Scholar] [CrossRef]
- Ikeda, K.; Doi, Y.; Feng, B.F.; Kawahara, T. Chaotic breathers of two types in a two-dimensional Morse lattice with an on-site harmonic potential. Phys. D Nonlinear Phenom. 2007, 225, 184–196. [Google Scholar] [CrossRef]
- Kavitha, L.; Mohamadou, A.; Parasuraman, E.; Gopi, D.; Akila, N.; Prabhu, A. Modulational instability and nano-scale energy localization in ferromagnetic spin chain with higher order dispersive interactions. J. Magn. Magn. Mater. 2016, 404, 91–118. [Google Scholar] [CrossRef]
- Kosevich, Y.A.; Lepri, S. Modulational instability and energy localization in anharmonic lattices at finite energy density. Phys. Rev. B 2000, 61, 299–307. [Google Scholar] [CrossRef]
- Mirnov, V.V.; Lichtenberg, A.J.; Guclu, H. Chaotic breather formation, coalescence, and evolution to energy equipartition in an oscillatory chain. Phys. D Nonlinear Phenom. 2001, 157, 251–282. [Google Scholar] [CrossRef]
- Ullmann, K.; Lichtenberg, A.J.; Corso, G. Energy equipartition starting from high-frequency modes in the Fermi-Pasta-Ulam beta oscillator chain. Phys. Rev. E 2000, 61, 2471–2477. [Google Scholar] [CrossRef]
- Korznikova, E.A.; Bachurin, D.V.; Fomin, S.Y.; Chetverikov, A.P.; Dmitriev, S.V. Instability of vibrational modes in hexagonal lattice. Eur. Phys. J. B 2017, 90, 23. [Google Scholar] [CrossRef]
- Upadhyaya, A.; Semenova, M.N.; Kudreyko, A.A.; Dmitriev, S.V. Chaotic discrete breathers and their effect on macroscopic properties of triangular lattice. Commun. Nonlinear Sci. 2022, 112, 106541. [Google Scholar] [CrossRef]
- Babicheva, R.I.; Semenov, A.S.; Soboleva, E.G.; Kudreyko, A.A.; Zhou, K.; Dmitriev, S.V. Discrete breathers in a triangular beta-Fermi-Pasta-Ulam-Tsingou lattice. Phys. Rev. E 2021, 103, 052202. [Google Scholar] [CrossRef]
- Semenov, A.S.; Murzaev, R.T.; Bebikhov, Y.V.; Kudreyko, A.A.; Dmitriev, S.V. New types of onedimensional discrete breathers in a two-dimensional lattice. Lett. Mater. 2020, 10, 185–188. [Google Scholar] [CrossRef]
- Korznikova, E.A.; Fomin, S.Y.; Soboleva, E.G.; Dmitriev, S.V. Highly symmetric discrete breather in a two-dimensional Morse crystal. JETP Lett. 2016, 103, 277–281. [Google Scholar] [CrossRef]
- Dmitriev, S.V.; Korznikova, E.A.; Bokij, D.I.; Zhou, K. Auxeticity from nonlinear vibrational modes. Phys. Status Solidi B-Basic Solid State Phys. 2016, 253, 1310–1317. [Google Scholar] [CrossRef]
- Babicheva, R.I.; Semenov, A.S.; Shcherbinin, S.A.; Korznikova, E.A.; Kudreyko, A.A.; Vivegananthan, P.; Zhou, K.; Dmitriev, S.V. Effect of the stiffness of interparticle bonds on properties of delocalized nonlinear vibrational modes in an fcc lattice. Phys. Rev. E 2022, 105, 064204. [Google Scholar] [CrossRef]
- LAMMPS. Available online: http://lammps.sandia.gov (accessed on 2 February 2022).
- Mendelev, M.I.; Kramer, M.J.; Becker, C.A.; Asta, M. Analysis of semi-empirical interatomic potentials appropriate for simulation of crystalline and liquid Al and Cu. Philos. Mag. 2008, 88, 1723–1750. [Google Scholar] [CrossRef]
- Sakhnenko, V.P.; Chechin, G.M. Symmetry selection rules in nonlinear dynamics of atomic systems. Dokl. Phys. 1993, 38, 219. [Google Scholar]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Morkina, A.Y.; Bachurin, D.V.; Dmitriev, S.V.; Semenov, A.S.; Korznikova, E.A. Modulational Instability of Delocalized Modes in fcc Copper. Materials 2022, 15, 5597. https://doi.org/10.3390/ma15165597
Morkina AY, Bachurin DV, Dmitriev SV, Semenov AS, Korznikova EA. Modulational Instability of Delocalized Modes in fcc Copper. Materials. 2022; 15(16):5597. https://doi.org/10.3390/ma15165597
Chicago/Turabian StyleMorkina, Alina Y., Dmitry V. Bachurin, Sergey V. Dmitriev, Aleksander S. Semenov, and Elena A. Korznikova. 2022. "Modulational Instability of Delocalized Modes in fcc Copper" Materials 15, no. 16: 5597. https://doi.org/10.3390/ma15165597
APA StyleMorkina, A. Y., Bachurin, D. V., Dmitriev, S. V., Semenov, A. S., & Korznikova, E. A. (2022). Modulational Instability of Delocalized Modes in fcc Copper. Materials, 15(16), 5597. https://doi.org/10.3390/ma15165597







