Reliability of Calculation of Dynamic Modulus for Asphalt Mixtures Using Different Master Curve Models and Shift Factor Equations
Abstract
:1. Introduction
2. Materials and Methods
2.1. Materials
2.2. Cyclic Indirect Tensile Test
2.2.1. Sample Preparation
2.2.2. Testing Procedure
2.3. Master Curve Construction
2.3.1. Master Curve Models
SLS Model
GLS Model
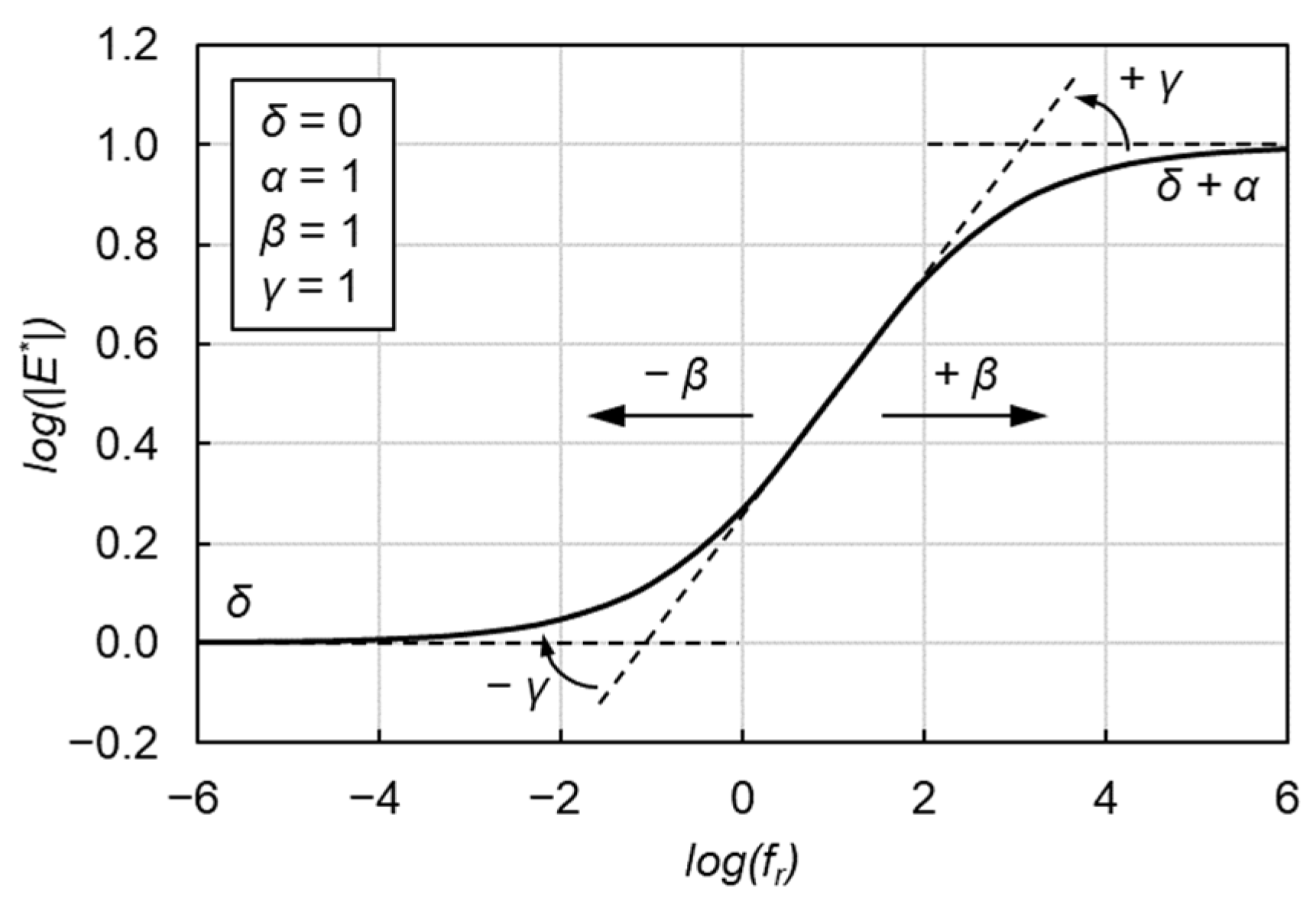
CAM Model
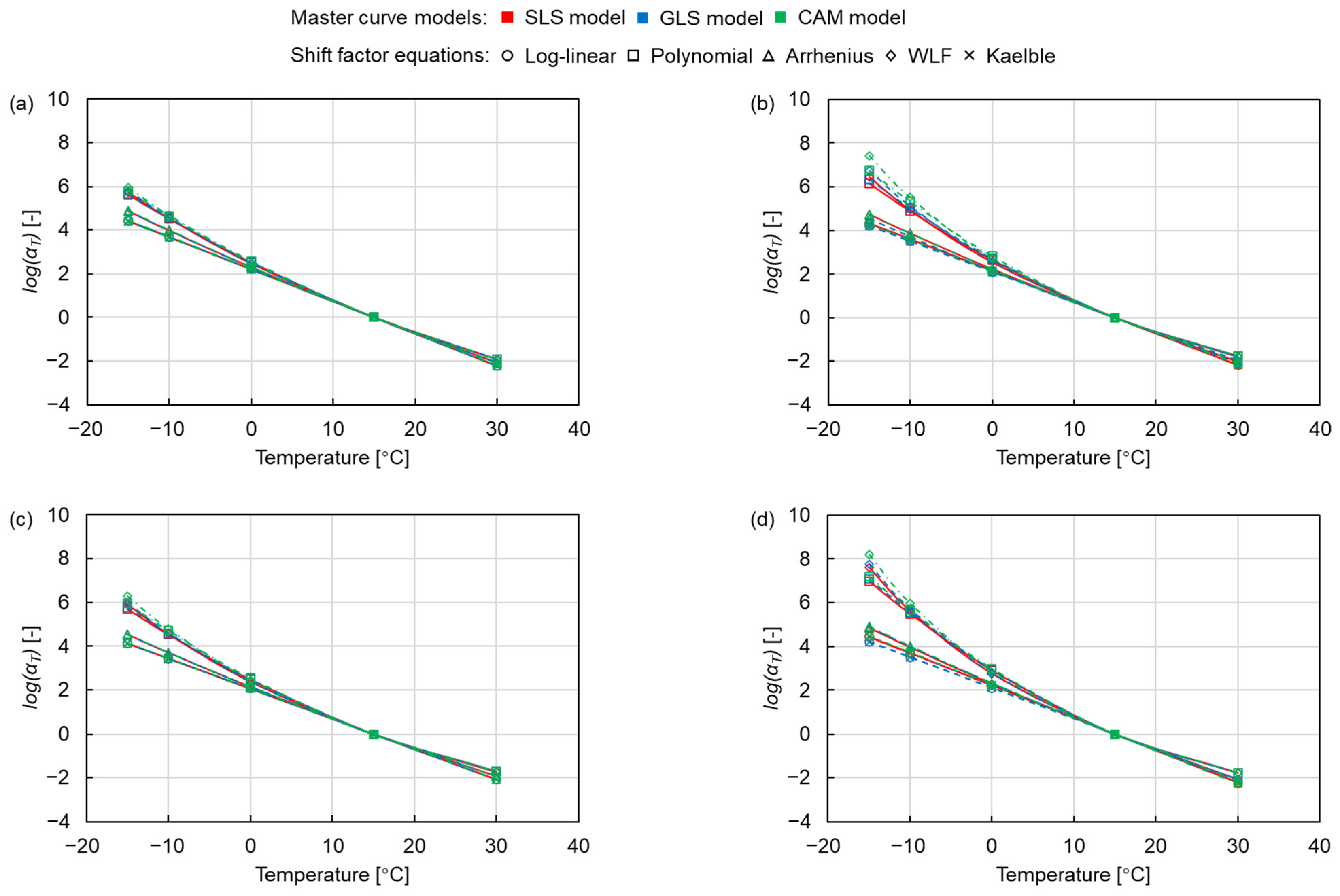
2.3.2. Shift Factor Equations
Log-Linear Equation
Quadratic Polynomial Equation
Arrhenius Equation
WLF Equation
Kaelble Equation
2.3.3. Fitting Procedure
2.4. Goodness of Fit Statistics
3. Results and Discussion
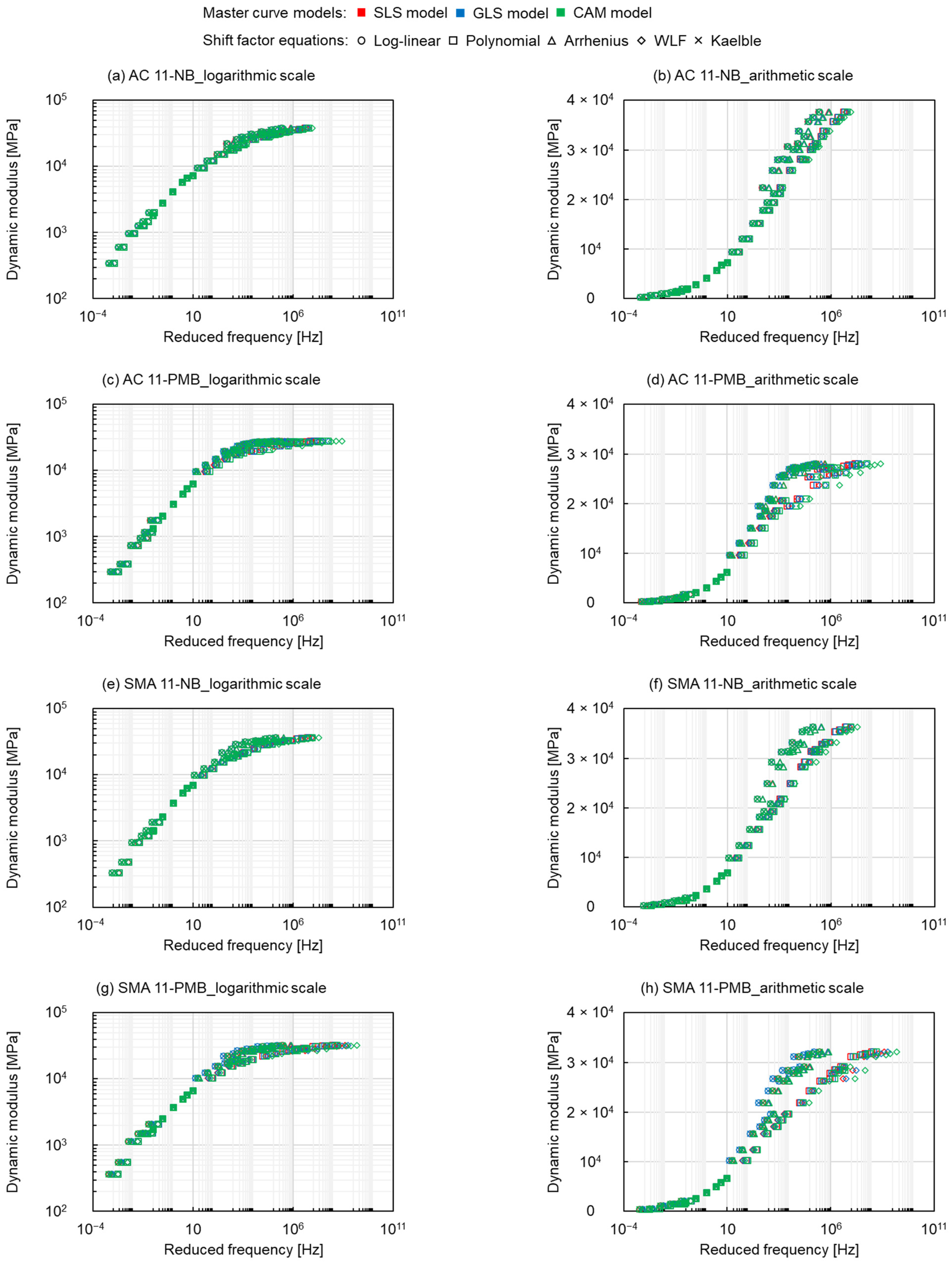
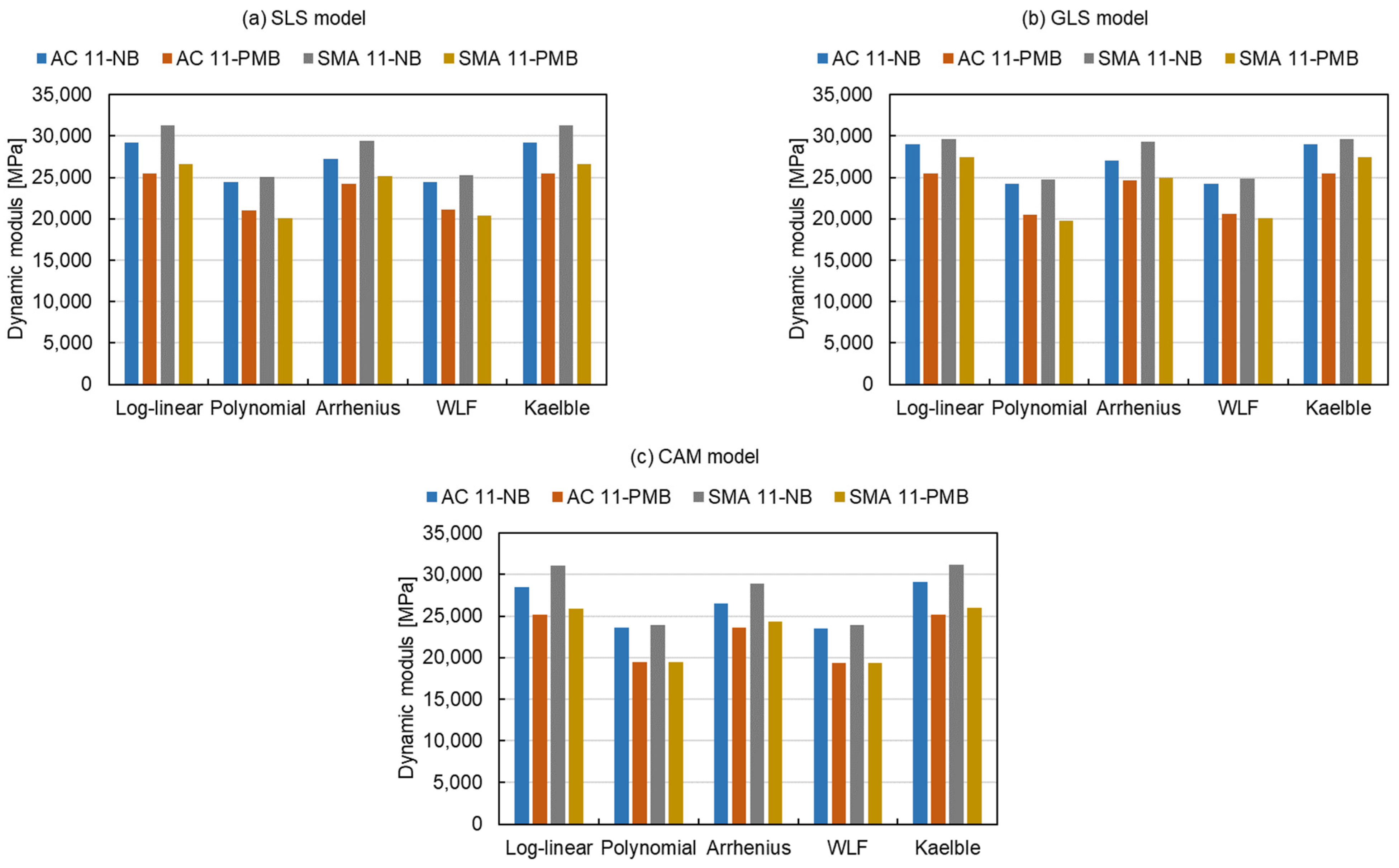
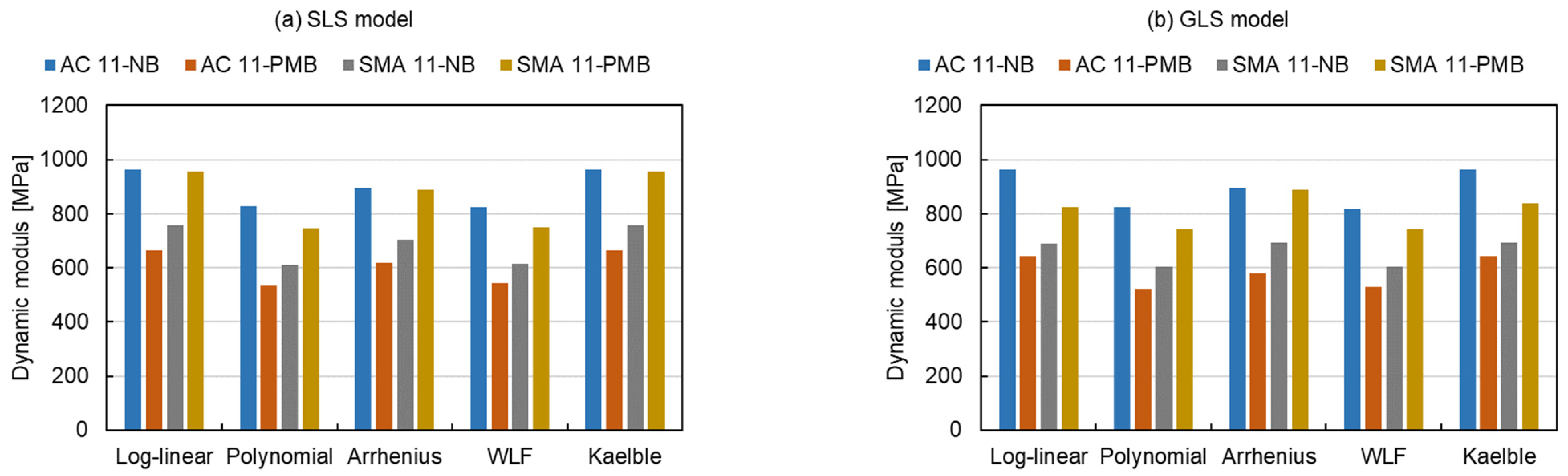
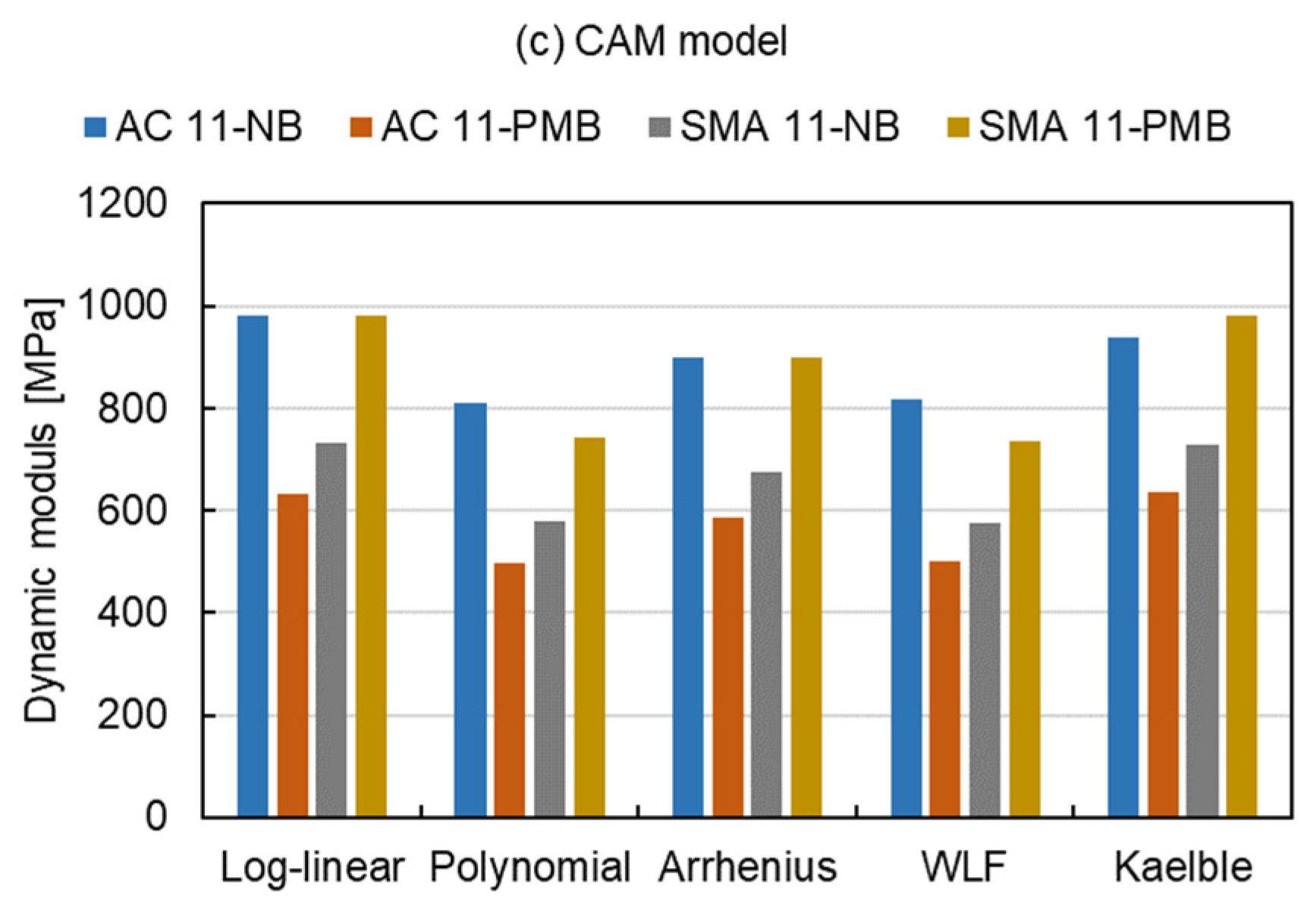
3.1. Dynamic Modulus Master Curve
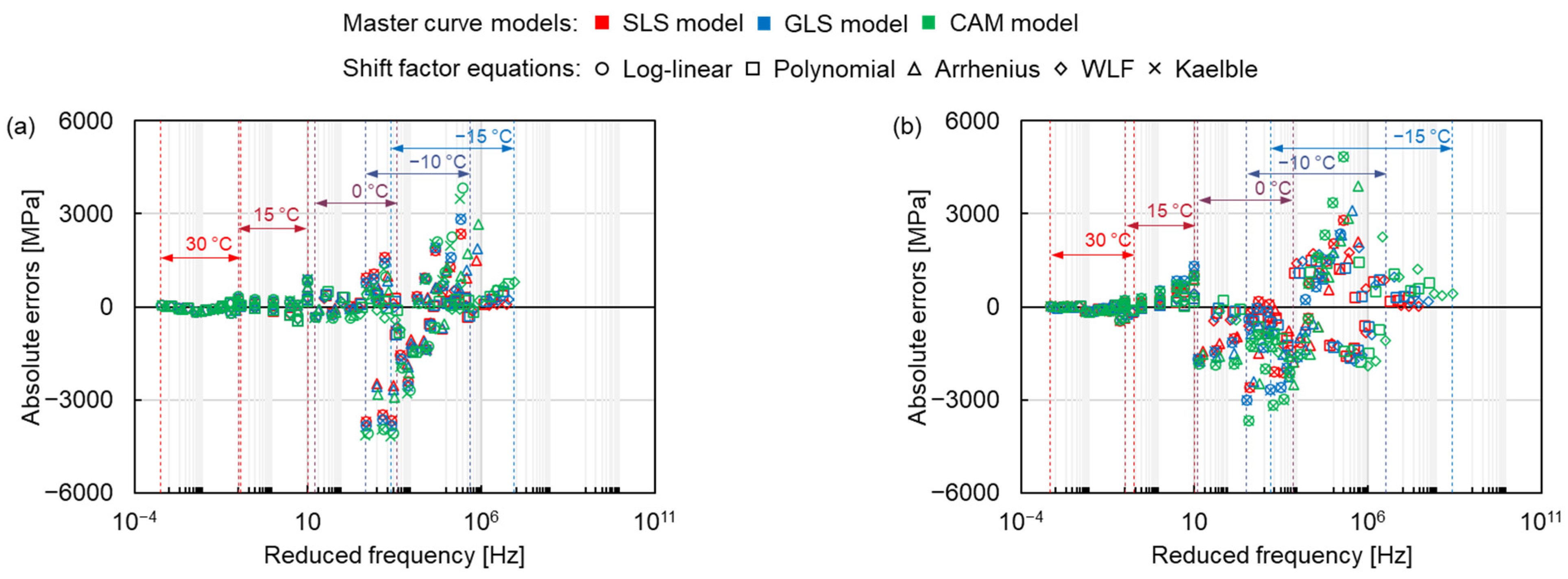
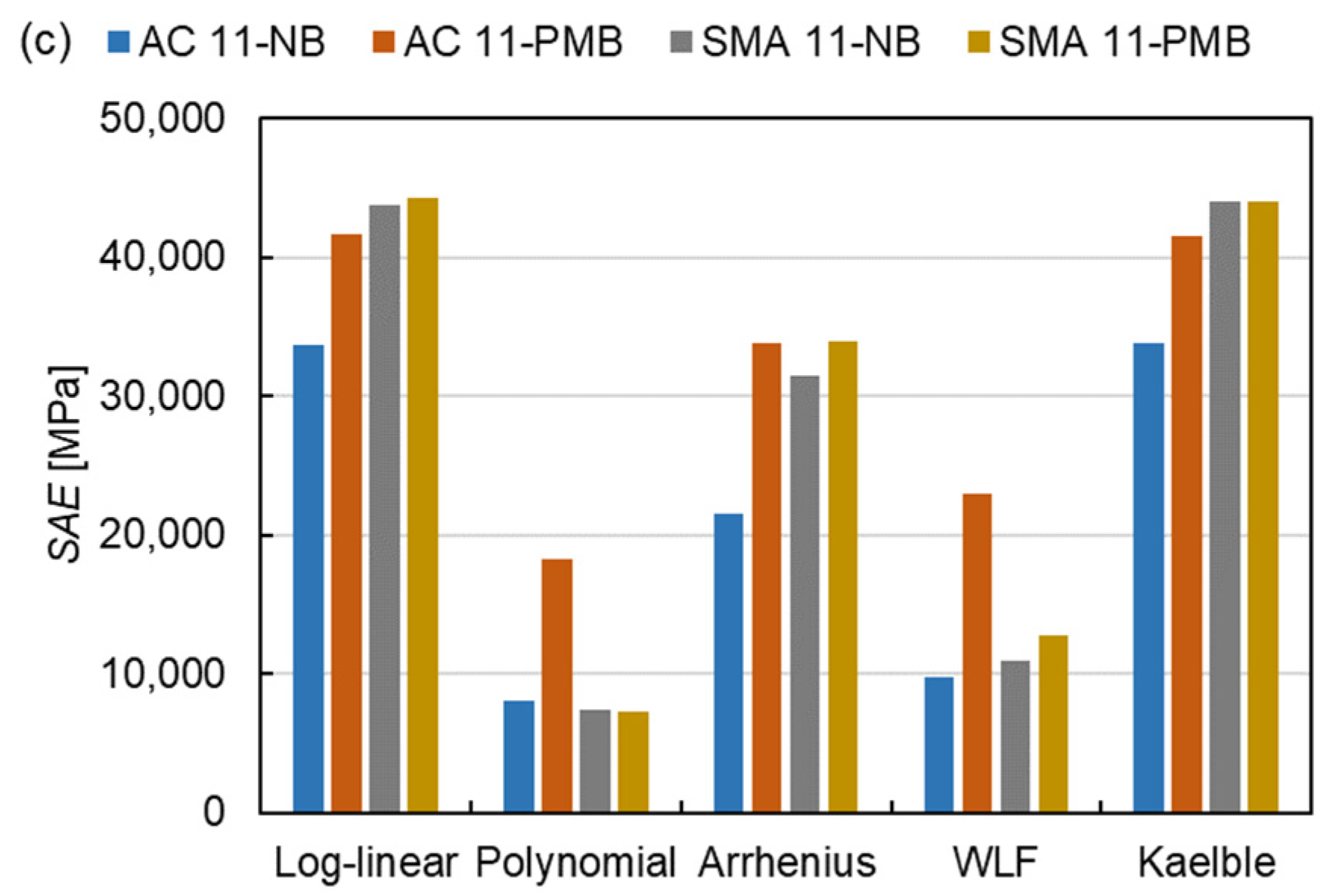
3.2. Error Analysis
3.2.1. Absolute Error
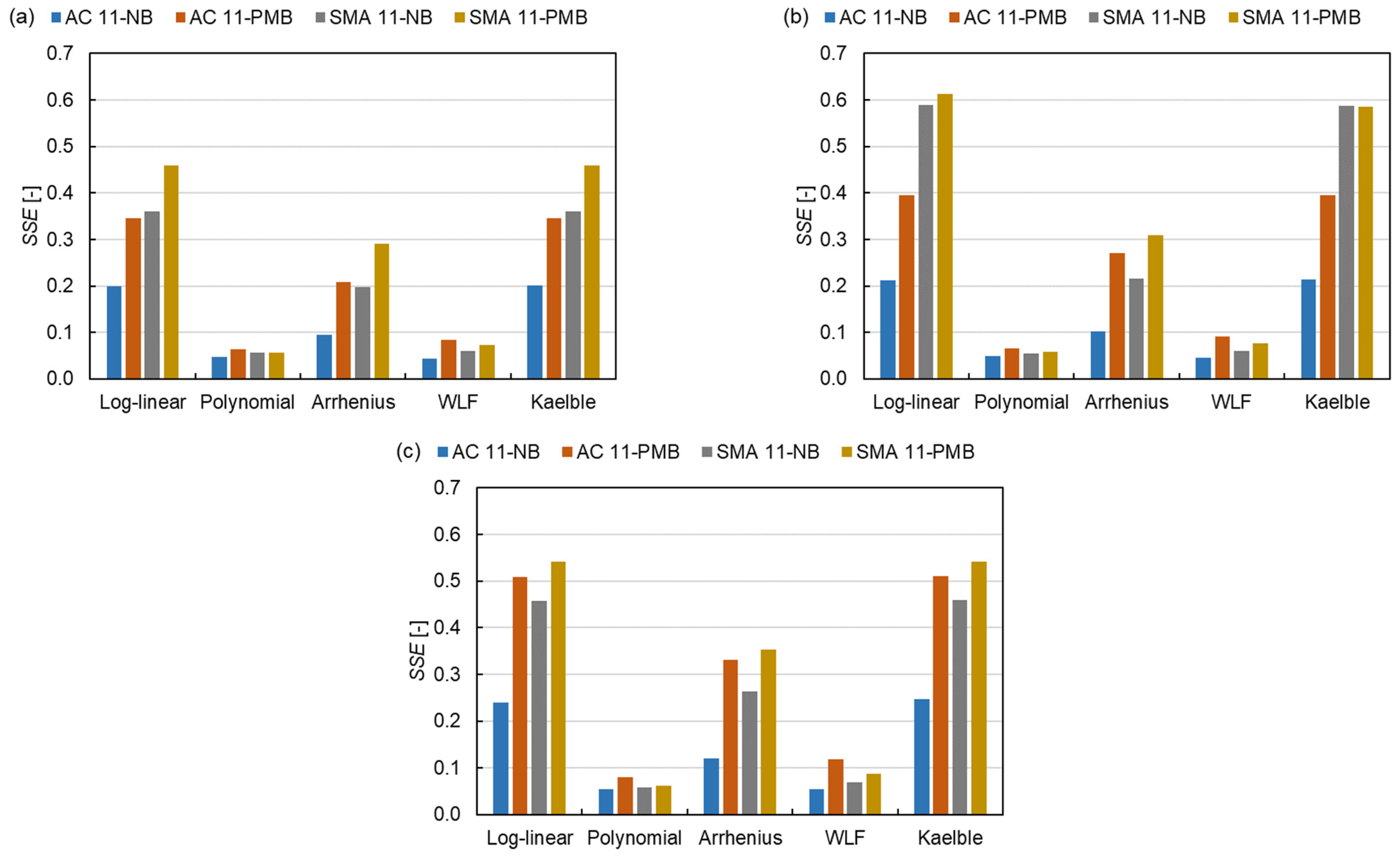
3.2.2. Normalised Square Error
3.3. Goodness of Fit
3.3.1. Master Curve Models
3.3.2. Shift Factor Equations
3.4. Comparison of Fits
4. Conclusions
- The selected shift factor equations were more influent with respect to the employed models in determining the final fitting reliability.
- The relationship between log(αT) and temperature of both the log-linear equation and the Kaelble equation were linear in the testing temperature range. These two shift factor equations had similar goodness-of-fit when extrapolating dynamic modulus master curves.
- Considering the results of absolute error, normalised square error, Se/Sy and R2, the combination of the SLS model and the polynomial equation had the best fitting quality index (1.94), while the combination of the CAM model and the Kaelble equation had the worst fitting quality index (14.25). Regarding the different asphalt mixtures, the fitting quality index of AC 11-NB (1.45) was the best, whereas the one of SMA 11-PMB (3.25) was the worst.
- The SLS model showed the best fitting quality and was considered to model the dynamic modulus of the asphalt mixtures most used as surface layer for Norwegian highway within the investigated CITT temperature range.
- Due to better goodness-of-fit and more convenience for temperature and frequency transform, the WLF equation was considered for modelling the dynamic modulus of the asphalt mixtures most adopted in Norway.
- The master curves constructed according to all the models and all shifting techniques were characterized by better goodness-of-fit for the asphalt mixtures containing NB than the ones comprised of PMB due to the effect of PMB structure on the dynamic modulus of the asphalt mixture. The modelling of dynamic modulus master curves for SMA mixtures has a better fit than the one for SMA mixtures because of the influence of more particle angularity on the dynamic modulus of the asphalt mixture. Therefore, the models can be developed further to be suitable for the asphalt mixtures containing the PMB and SMA types of asphalt mixtures.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- NPRA. VegDim. Available online: https://www.vegvesen.no/fag/fokusomrader/forskning-innovasjon-og-utvikling/pagaende-programmer-og-prosjekter/vegdim/ (accessed on 12 March 2022).
- Bahia, H.U.; Zhai, H.; Onnetti, K.; Kose, S. Non-linear viscoelastic and fatigue properties of asphalt binders. J. Assoc. Asph. Paving Technol. 1999, 68, 1–34. [Google Scholar]
- Lesueur, D.; Gerard, J.F.; Claudy, P.; Letoffe, J.M.; Planche, J.P.; Martin, D. A structure-related model to describe asphalt linear viscoelasticity. J. Rheol. 1996, 40, 813–836. [Google Scholar] [CrossRef]
- NCHRP. Guide for Mechanistic-Empirical Design of New and Rehabilitated Pavement Structures; National Cooperative Highway Research Program 1-37A: Washington, DC, USA, 2004. [Google Scholar]
- Schwartz, C.W.; Gibson, N.; Schapery, R.A. Time-temperature superposition for asphalt concrete at large compressive strains. Transp. Res. Rec. 2002, 1789, 101–112. [Google Scholar] [CrossRef]
- Chehab, G.; Kim, Y.; Schapery, R.; Witczak, M.; Bonaquist, R. Time-temperature superposition principle for asphalt concrete with growing damage in tension state. J. Assoc. Asph. Paving Technol. 2002, 71, 559–593. [Google Scholar]
- Pellinen, T.K.; Witczak, M.W.; Bonaquist, R.F. Asphalt mix master curve construction using sigmoidal fitting function with non-linear least squares optimization. In Recent Advances in Materials Characterization and Modeling of Pavement Systems; American Society of Civil Engineers: Reston, VA, USA, 2004; pp. 83–101. [Google Scholar]
- Pellinen, T.K.; Witczak, M.W.; Marasteanu, M.; Chehab, G.; Alavi, S.; Dongre, R. Stress Dependent Master Curve Construction for Dynamic (Complex) Modulus; Asphalt Paving Technology: Association of Asphalt Paving Technologists-Proceedings of the Technical Sessions; Association of Asphalt Paving Technologist: Colorado Springs, CO, USA, 2002; pp. 281–309. [Google Scholar]
- Rowe, G.; Baumgardner, G.; Sharrock, M. Functional forms for master curve analysis of bituminous materials. In Advanced Testing and Characterization of Bituminous Materials, Two Volume Set; CRC Press: London, UK, 2009; pp. 97–108. [Google Scholar]
- Zhang, F.; Wang, L.; Li, C.; Xing, Y. Predict the Phase Angle Master Curve and Study the Viscoelastic Properties of Warm Mix Crumb Rubber-Modified Asphalt Mixture. Materials 2020, 13, 5051. [Google Scholar] [CrossRef]
- Christensen, D.W.; Anderson, D.A. Interpretation of dynamic mechanical test data for paving grade asphalt cements (with discussion). J. Assoc. Asph. Paving Technol. 1992, 61, 67–116. [Google Scholar]
- Marasteanu, M.; Anderson, D. Improved Model for Bitumen Rheological Characterization; Eurobitume workshop on performance related properties for bituminous binders; European Bitumen Association: Brussels, Belgium, 1999. [Google Scholar]
- Wathne, P. Evaluation of Different Models for Construction of Master Curves for Dynamic E-module for Asphalt Materials; Norwegian University of Science and Technology: Trondheim, Norway, 2020. [Google Scholar]
- CholewiŃSka, M.; IwaŃSki, M.; Mazurek, G. The Impact of Ageing on the Bitumen Stiffness Modulus Using the Cam Model. Balt. J. Road Bridge Eng. 2018, 13, 34–39. [Google Scholar] [CrossRef] [Green Version]
- Williams, M.L.; Landel, R.F.; Ferry, J.D. The temperature dependence of relaxation mechanisms in amorphous polymers and other glass-forming liquids. J. Am. Chem. Soc. 1955, 77, 3701–3707. [Google Scholar] [CrossRef]
- Hutcheson, S.; McKenna, G. The measurement of mechanical properties of glycerol, m-toluidine, and sucrose benzoate under consideration of corrected rheometer compliance: An in-depth study and review. J. Chem. Phys. 2008, 129, 074502. [Google Scholar] [CrossRef]
- O’Connell, P.A.; McKenna, G.B. Arrhenius-type temperature dependence of the segmental relaxation below T g. J. Chem. Phys. 1999, 110, 11054–11060. [Google Scholar] [CrossRef] [Green Version]
- Kaelble, D.H. Computer Aided Design of Polymers and Composites; CRC Press: New York, NY, USA, 1985; Volume 7. [Google Scholar]
- Rowe, G.M.; Sharrock, M.J. Alternate Shift Factor Relationship for Describing Temperature Dependency of Viscoelastic Behavior of Asphalt Materials. Transp. Res. Rec. J. Transp. Res. Board 2011, 2207, 125–135. [Google Scholar] [CrossRef]
- Laukkanen, O.-V.; Winter, H.H. The dynamic fragility and apparent activation energy of bitumens as expressed by a modified Kaelble equation. J. Non-Cryst. Solids 2018, 499, 289–299. [Google Scholar] [CrossRef]
- Forough, S.A.; Nejad, F.M.; Khodaii, A. A comparative study of temperature shifting techniques for construction of relaxation modulus master curve of asphalt mixes. Constr. Build. Mater. 2014, 53, 74–82. [Google Scholar] [CrossRef]
- Bohn, M.A. The Connection Between the Parameters of WLF Equation and of Arrhenius Equation. Propellants Explos. Pyrotech. 2019, 44, 696–705. [Google Scholar] [CrossRef]
- Garcia, G.; Thompson, M. HMA Dynamic Modulus Predictive Models (A Review); IDEALS: Urbana, IL, USA, 2007. [Google Scholar]
- Angelone, S.; Borghi, M.; Casaux, M.C.; Martinez, F. Evaluation of Different Procedures and Models for the Construction of Dynamic Modulus Master Curves of Asphalt Mixtures. In Proceedings of the International Conferences on the Bearing Capacity of Roads Railways and Airfields, Trondheim, Norway, 25–27 June 2013; pp. 647–657. [Google Scholar]
- Tavassoti-Kheiry, P.; Boz, I.; Chen, X.; Solaimanian, M. Application of ultrasonic pulse velocity testing of asphalt concrete mixtures to improve the prediction accuracy of dynamic modulus master curve. In Proceedings of the Airfield and Highway Pavements, Philadelphia, PA, USA, 13 August 2017. [Google Scholar]
- Kim, M.; Mohammad, L.N.; Elseifi, M.A. Effects of various extrapolation techniques for abbreviated dynamic modulus test data on the MEPDG rutting predictions. J. Mar. Sci. Technol. 2015, 23, 12. [Google Scholar]
- Yildirim, Y. Polymer modified asphalt binders. Constr. Build. Mater. 2007, 21, 66–72. [Google Scholar] [CrossRef]
- CEN, EN 1426:2015; Bitumen and Bitumen Binders-Determination of Needle Penetration. European Committee for Standardization: Brussels, Belgium, 2015.
- CEN, EN 1427:2015; Bitumen and Bituminous Binders-Determination of the Softening Point-Ring and Ball Method. European Committee for Standardization: Brussels, Belgium, 2015.
- Arnevik, R.E.; Uthus, N.S.; Aurstad, J.; Aksnes, J.; Jørgensen, T. Guidelines Asphalt 2019; Norwegian Public Roads Administration: Oslo, Norway, 2019. [Google Scholar]
- CEN, EN 1097-1:2011; Tests for Mechanical Physical Properties of Aggregates-Part 1: Determination of the Resistance to Wear (Micro-Deval). European Committee for Standardization: Brussels, Belgium, 2011.
- CEN, EN 1097-2:2020; Tests for Mechanical and Physical Properties of Aggregates-Part 2: Methods for the Determination of Resistance to Fragmentation. European Committee for Standardization: Brussels, Belgium, 2020.
- Rowe, G. Phase angle determination and interrelationships within bituminous materials. In Advanced Testing and Characterization of Bituminous Materials, Two Volume Set; CRC Press: London, UK, 2009; pp. 59–68. [Google Scholar]
- Fan, B.; Kazmer, D.O. Low-temperature modeling of the time-temperature shift factor for polycarbonate. Adv. Polym. Technol. 2005, 24, 278–287. [Google Scholar] [CrossRef]
- Tran, N.; Hall, K. Evaluating the predictive equation in determining dynamic moduli of typical asphalt mixtures used in Arkansas. SAGE 2005, 2210, 7. [Google Scholar]
- Witczak, M.W. Simple Performance Test for Superpave Mix Design; Transportation Research Board: Washington, DC, USA, 2002; Volume 465. [Google Scholar]
- Veeraragavan, A. Dynamic mechanical characterization of asphalt concrete mixes with modified asphalt binders. Mater. Sci. Eng. A 2011, 528, 6445–6454. [Google Scholar]
- Dong, F.; Zhao, W.; Zhang, Y.; Wei, J.; Fan, W.; Yu, Y.; Wang, Z. Influence of SBS and asphalt on SBS dispersion and the performance of modified asphalt. Constr. Build. Mater. 2014, 62, 1–7. [Google Scholar] [CrossRef]
- Yu, H.; Shen, S. Impact of aggregate packing on dynamic modulus of hot mix asphalt mixtures using three-dimensional discrete element method. Constr. Build. Mater. 2012, 26, 302–309. [Google Scholar] [CrossRef]
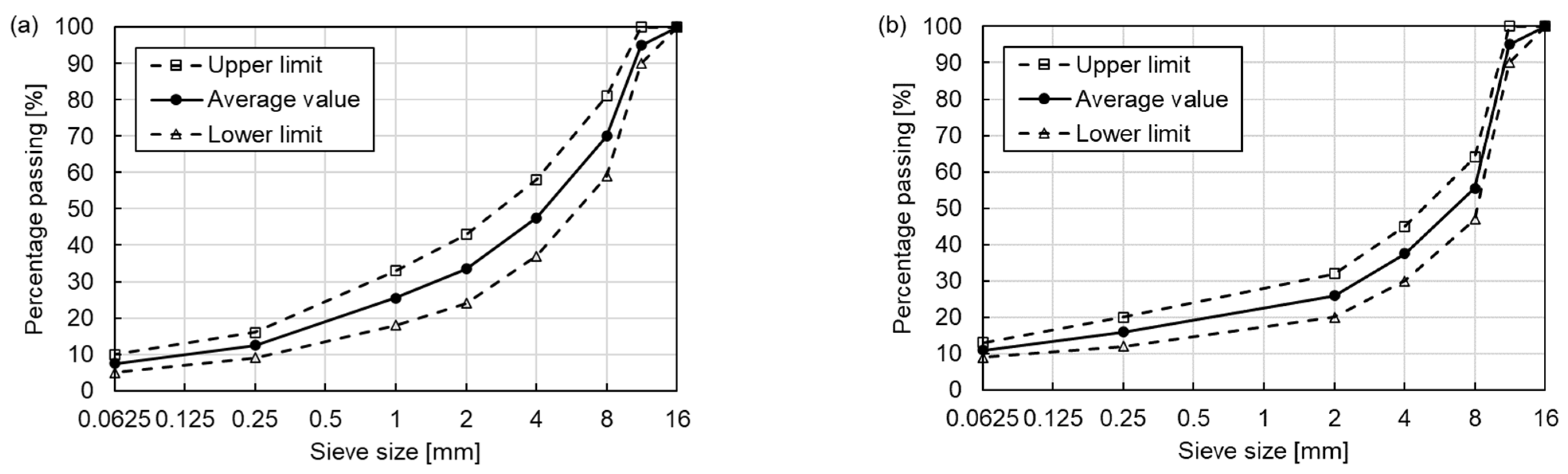









| Physical Properties | Bitumen | Test Standard | |
|---|---|---|---|
| NB | PMB | ||
| Penetration at 25 °C [0.1 mm] | 92 | 86 | EN 1426:2015 [28] |
| Softening point (Ring and Ball) [°C] | 46.0 | 62.6 | EN 1427:2015 [29] |
| Test | Value | Requirements for AADT > 15,000 | Test Standard |
|---|---|---|---|
| Micro-Deval coefficient | 14.2 | ≤20 | EN 1097-1:2011 [31] |
| Los Angeles value | 18.2 | ≤15 | EN 1097-2:2020 [32] |
| Mixture Type | Bitumen Type | Designation |
|---|---|---|
| AC 11 | NB | AC 11-NB |
| PMB | AC 11-PMB | |
| SMA 11 | NB | SMA 11-NB |
| PMB | SMA 11-PMB |
| Mixture | Maximum Density [Mg/m3] | Air Voids Content [%] | Voids in Mineral Aggregate [%] | Voids Filled with Bitumen [%] | |||
|---|---|---|---|---|---|---|---|
| Value | Standard Deviation | Value | Standard Deviation | Value | Standard Deviation | ||
| AC 11-NB | 2.753 | 3.5 | 0.197 | 16.9 | 0.170 | 79.1 | 0.947 |
| AC 11-PMB | 2.748 | 2.9 | 0.314 | 16.6 | 0.269 | 82.8 | 1.636 |
| SMA 11-NB | 2.740 | 4.4 | 0.252 | 18.1 | 0.216 | 75.8 | 1.101 |
| SMA 11-PMB | 2.740 | 3.1 | 0.296 | 17.1 | 0.254 | 81.6 | 1.438 |
| Shift Factor Equation | Master Curve Model | ||
|---|---|---|---|
| SLS Model | GLS Model | CAM Model | |
| Log-linear | 9.75 | 12.56 | 14.00 |
| Polynomial | 1.94 | 2.31 | 3.44 |
| Arrhenius | 6.81 | 8.50 | 9.75 |
| WLF | 3.31 | 4.00 | 6.19 |
| Kaelble | 10.75 | 12.44 | 14.25 |
 Poor (15).
Poor (15).| Mixture Type | Bitumen Type | |
|---|---|---|
| NB | PMB | |
| AC 11 | 1.45 | 2.92 |
| SMA 11 | 2.38 | 3.25 |
 Poor (4).
Poor (4).Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, H.; Barbieri, D.M.; Zhang, X.; Hoff, I. Reliability of Calculation of Dynamic Modulus for Asphalt Mixtures Using Different Master Curve Models and Shift Factor Equations. Materials 2022, 15, 4325. https://doi.org/10.3390/ma15124325
Chen H, Barbieri DM, Zhang X, Hoff I. Reliability of Calculation of Dynamic Modulus for Asphalt Mixtures Using Different Master Curve Models and Shift Factor Equations. Materials. 2022; 15(12):4325. https://doi.org/10.3390/ma15124325
Chicago/Turabian StyleChen, Hao, Diego Maria Barbieri, Xuemei Zhang, and Inge Hoff. 2022. "Reliability of Calculation of Dynamic Modulus for Asphalt Mixtures Using Different Master Curve Models and Shift Factor Equations" Materials 15, no. 12: 4325. https://doi.org/10.3390/ma15124325
APA StyleChen, H., Barbieri, D. M., Zhang, X., & Hoff, I. (2022). Reliability of Calculation of Dynamic Modulus for Asphalt Mixtures Using Different Master Curve Models and Shift Factor Equations. Materials, 15(12), 4325. https://doi.org/10.3390/ma15124325







