Novel Four-Cell Lenticular Honeycomb Deployable Boom with Enhanced Stiffness
Abstract
:1. Introduction
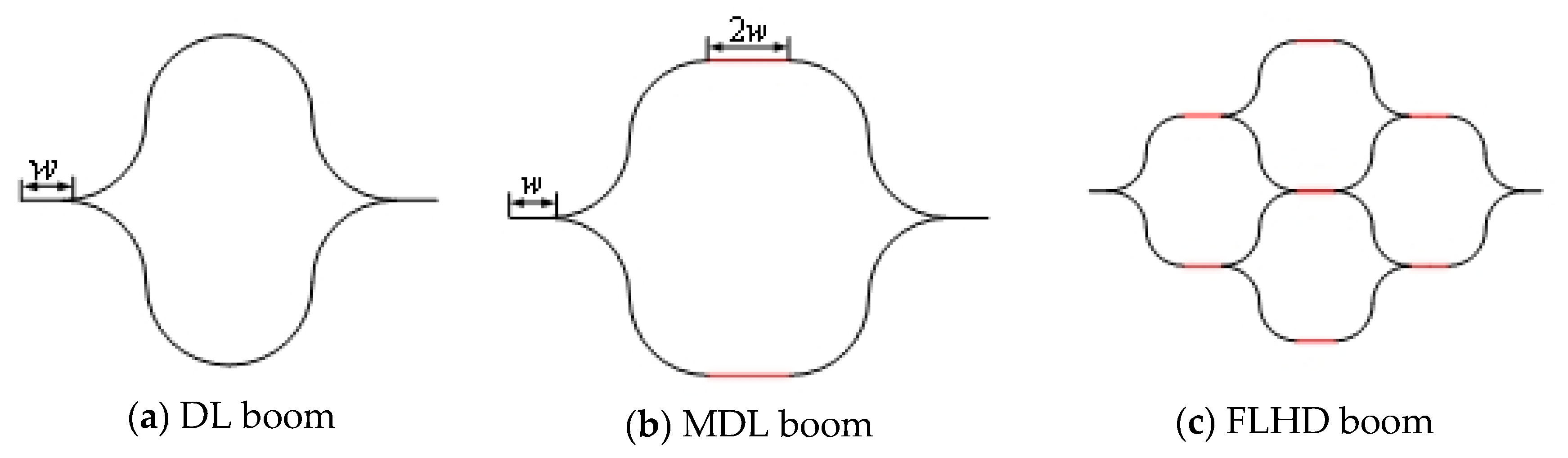
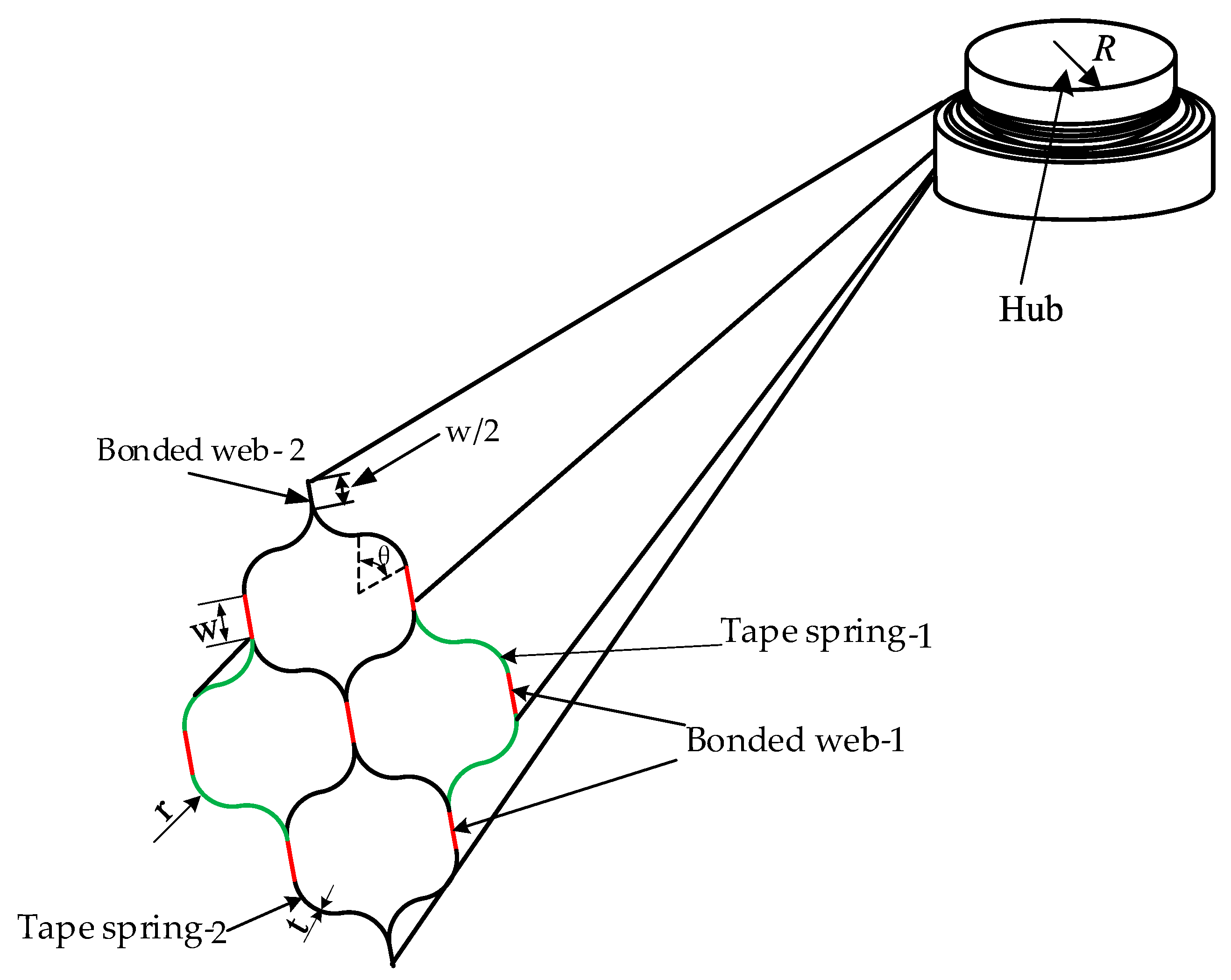
2. Problem Description
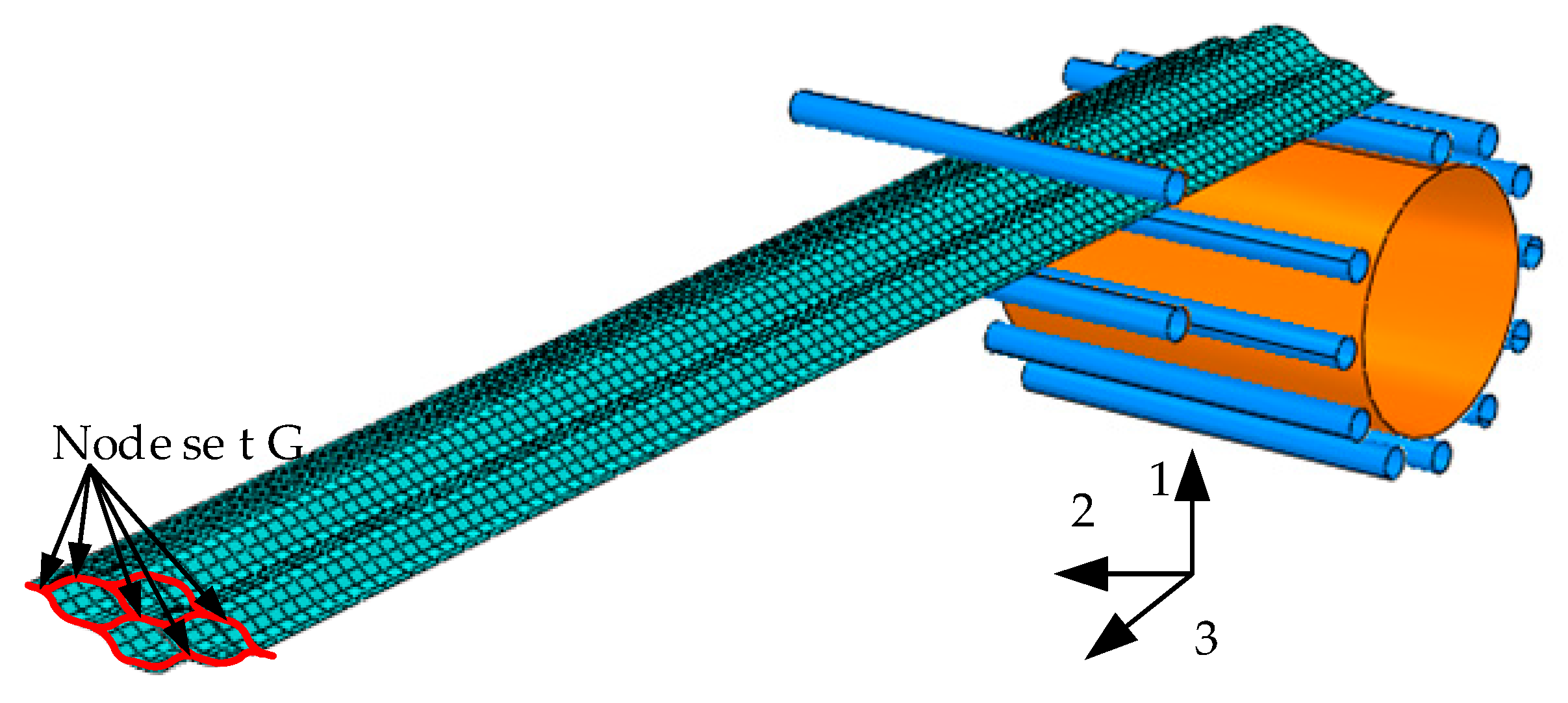
2.1. Behavior of FLHD Booms
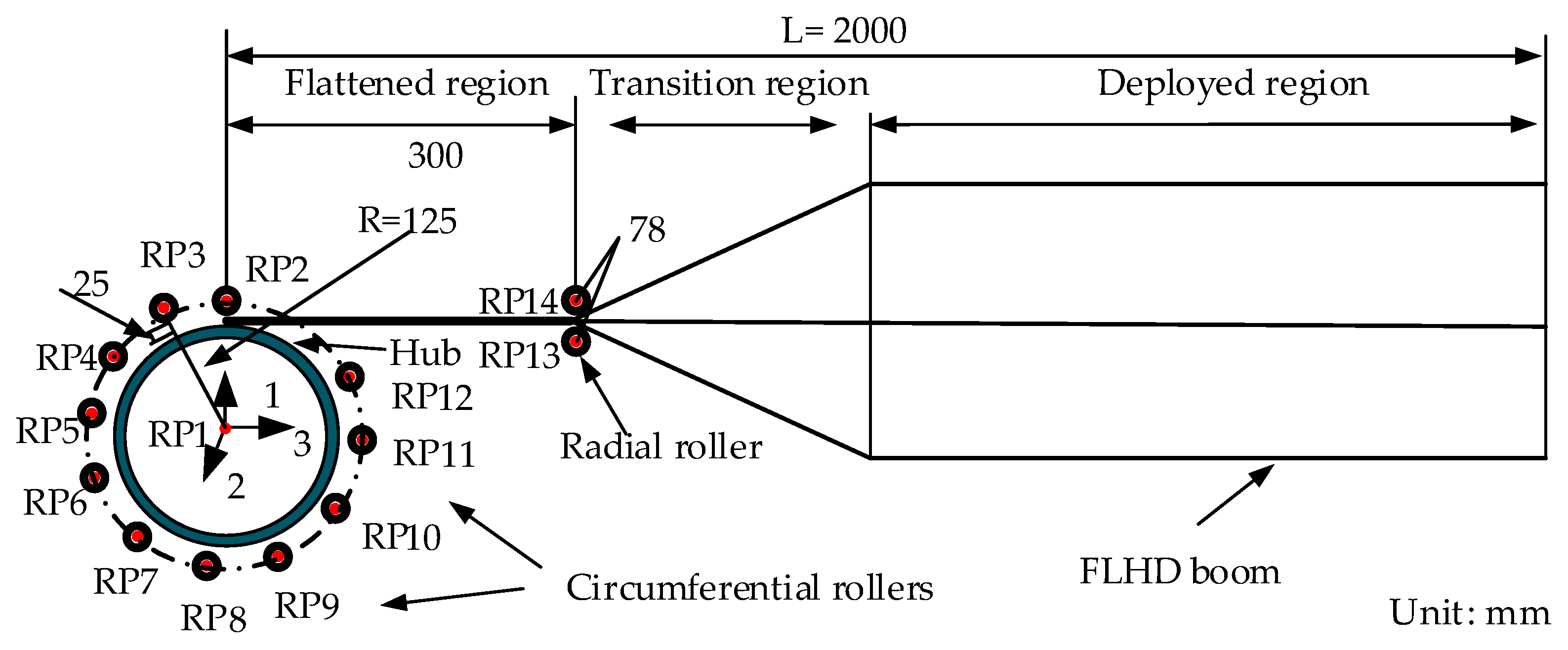
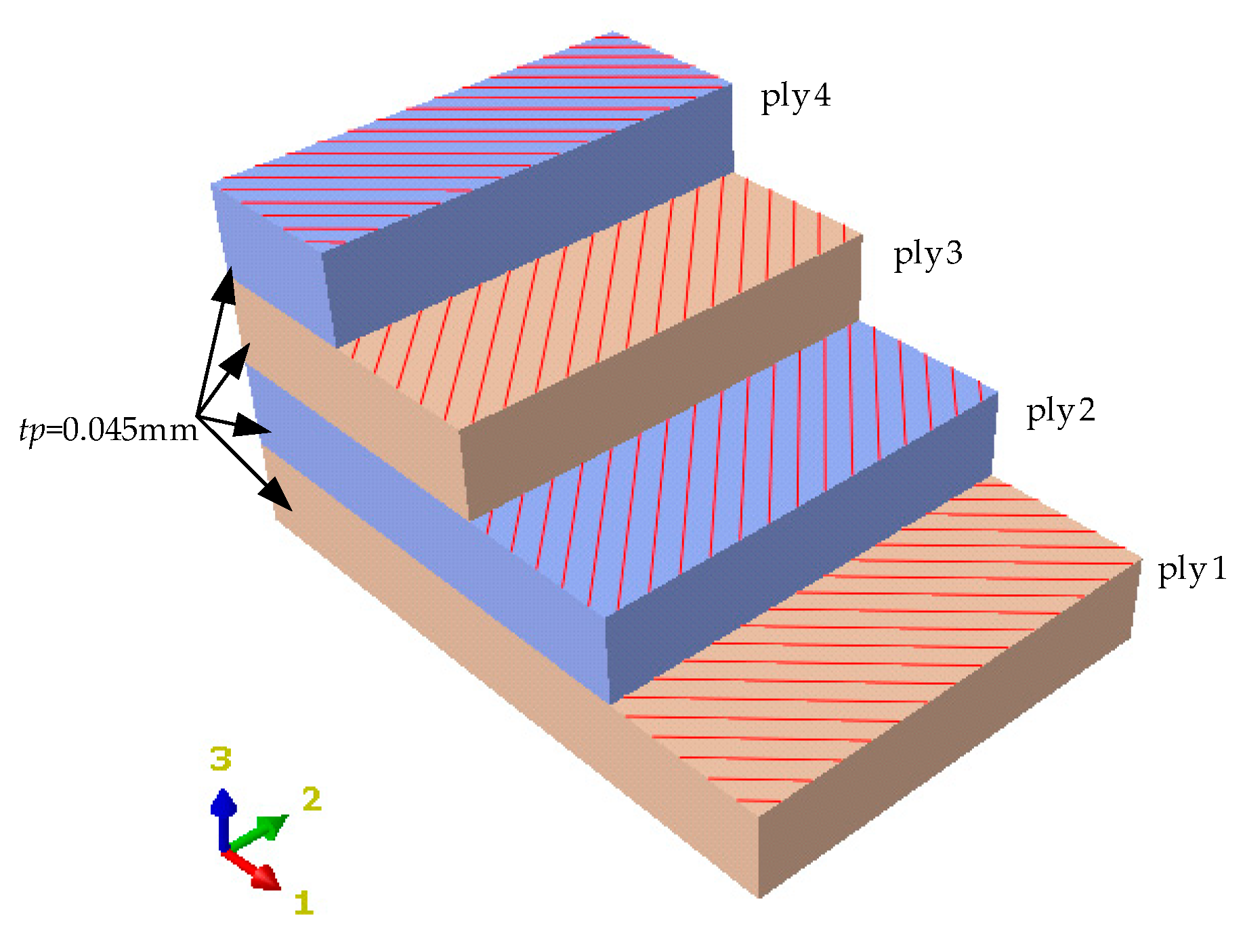
2.2. Analysis Steps
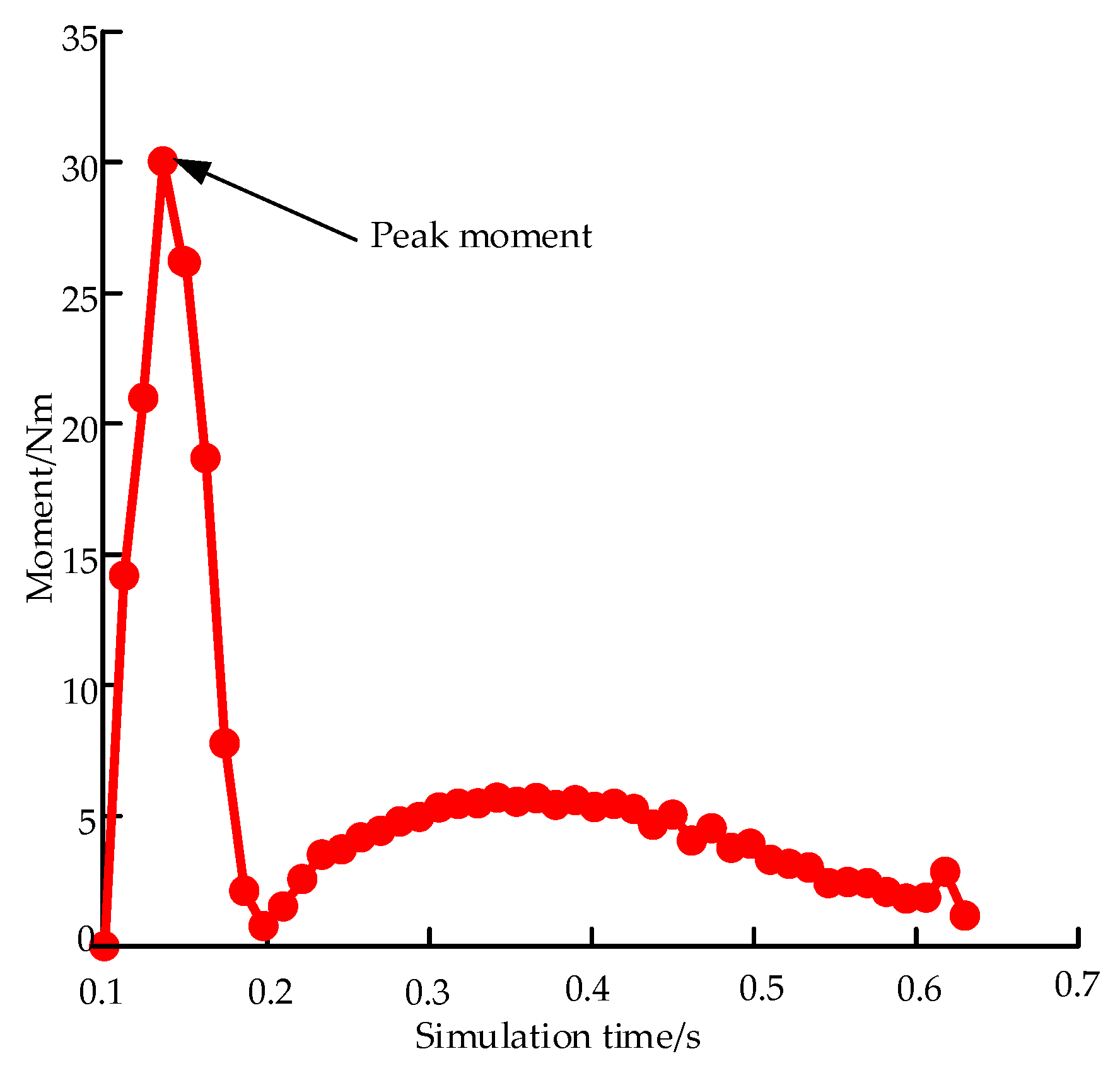
2.3. Numerical Results and Discussion
3. BPNN Surrogate Model Method
3.1. Description of the Optimization Problem
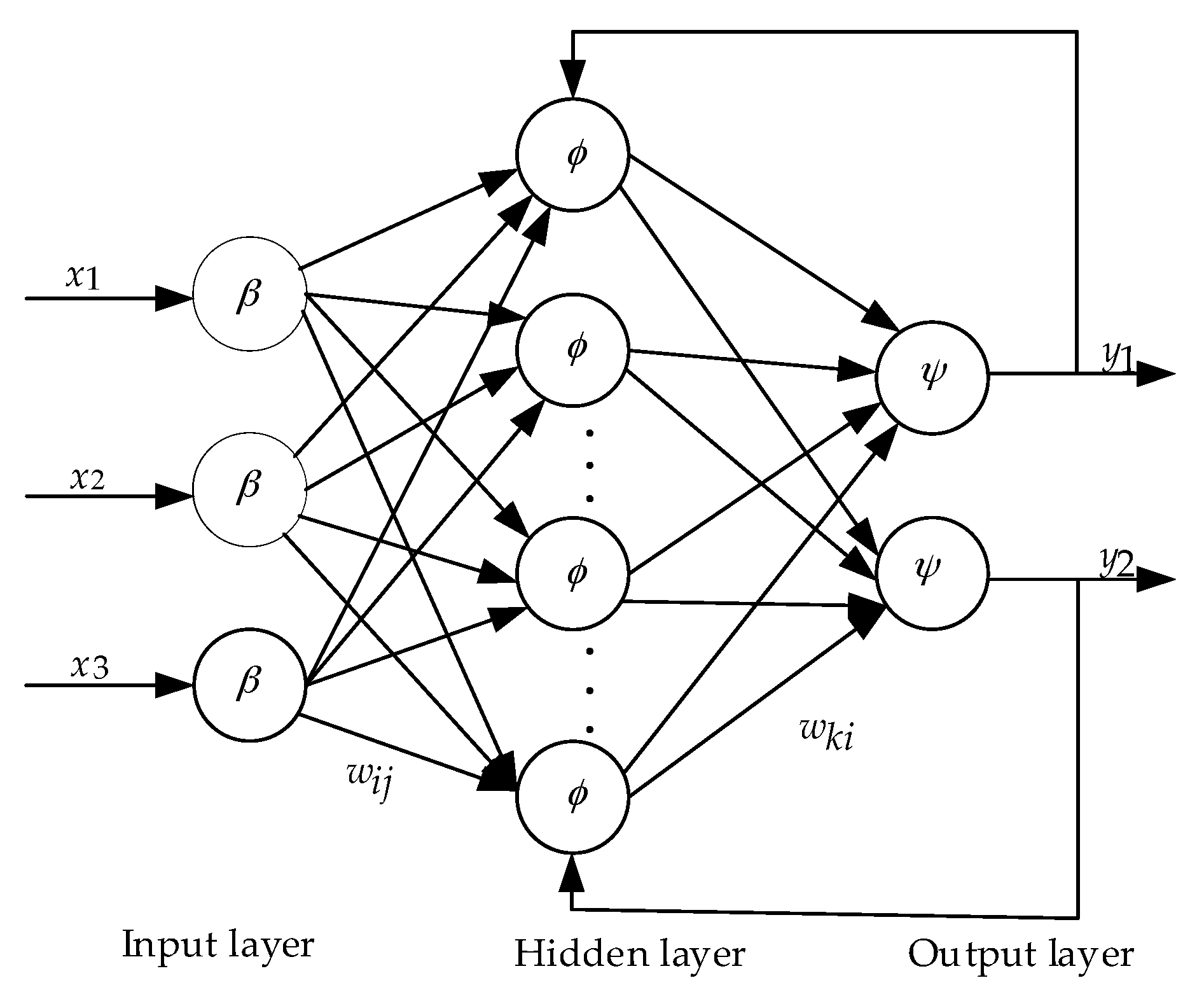
3.2. BPNN Surrogate Model
4. Build BPNN Surrogate Model of Mpeak and Smax
4.1. Sample Points
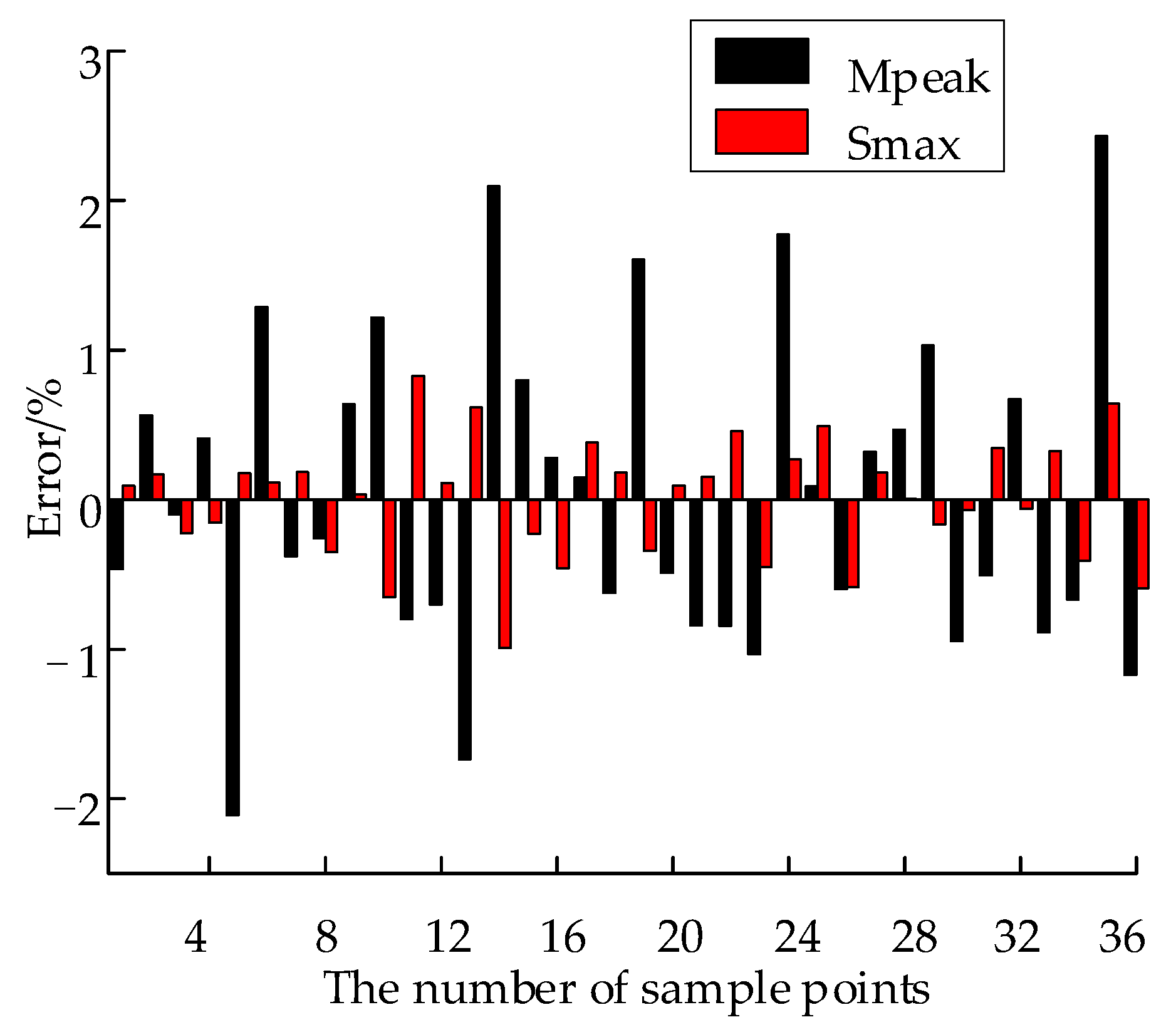
4.2. Error Analysis of the Surrogate Model
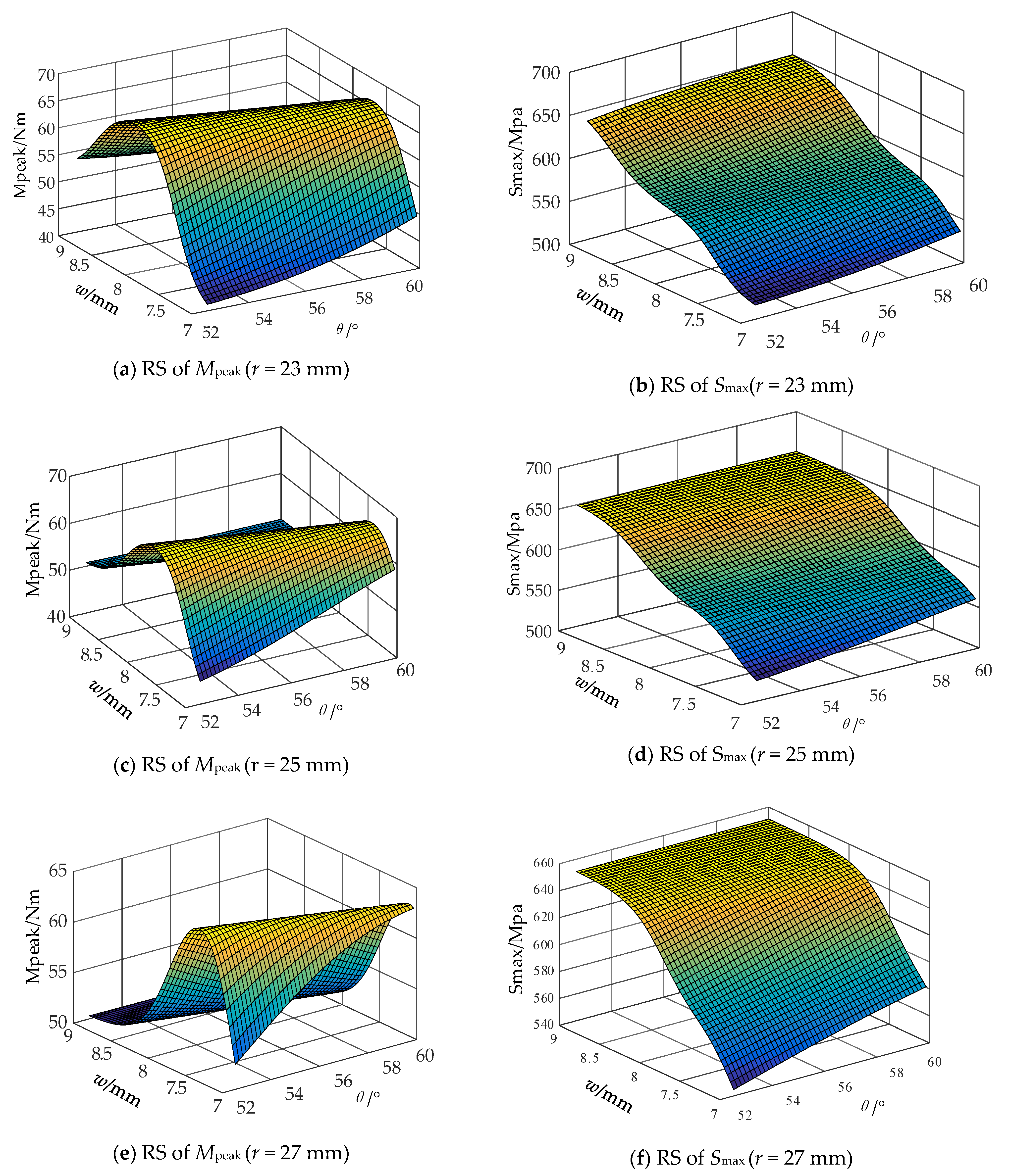
4.3. Response Surface
5. Multi-Objective Optimization Design
6. Conclusions
- A novel type of FLHD boom characterized by a high spreading ratio, light weight, and simple structure is proposed.
- A surrogate model of Mpeak and Smax is established by BPNN, and the REs of the Mpeak and Smax of 36 sample points do not exceed 3%, which verifies the accuracy of the surrogate model.
- NSGA-II is used to complete the multi-objective optimization design. Mpeak and mass are selected as objectives, Smax is selected as the constraint, and θ, r, and w are set as design variables. The optimal design structure is r = 23.00 mm, θ = 53.31°, and w = 7.52 mm. The REs of the optimal design results are less than −5.54%.
- The next step is to build an experimental platform to verify the reliability of the theory and simulation analysis. After the verification results are reliable, FLHD boom will be applied to the folding of large-aperture array antennas.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Hakkak, F.; Khodam, S. On calculation of preliminary design parameters for lenticular booms. Proc. IMechE Part G J. Aerosp. Eng. 2007, 221, 377–384. [Google Scholar] [CrossRef]
- Yang, H.; Liu, L.; Guo, H.; Lu, F.; Liu, Y. Wrapping dynamic analysis and optimization of deployable composite triangular rollable and collapsible booms. Struct. Multidiscip. Optim. 2018, 59, 1371–1383. [Google Scholar] [CrossRef]
- Yang, H.; Liu, R.; Wang, Y.; Deng, Z.; Guo, H. Experiment and multiobjective optimization design of tape-spring hinges. Struct. Multidiscip. Optim. 2015, 51, 1373–1384. [Google Scholar] [CrossRef]
- Stabile, A.; Laurenzi, S. Coiling dynamic analysis of thin-walled composite deployable boom. Compos. Struct. 2014, 113, 429–436. [Google Scholar] [CrossRef]
- Hoskin, A.; Viquerat, A.; Aglietti, G.S. Tip force during blossoming of coiled deployable booms. Int. J. Solids Struct. 2017, 118–119, 58–69. [Google Scholar] [CrossRef]
- Banik, J.; Hausgen, P. Roll-out solar array (ROSA): Next generation flexible solar array technology. In Proceedings of the AIAA SPACE and Astronautics Forum and Exposition, Orlando, FL, USA, 12–14 September 2017. [Google Scholar]
- Mallikarachchi, H.M.Y.C.; Pellegrino, S. Deployment Dynamics of Ultrathin Composite Booms with Tape-Spring Hinges. J. Spacecr. Rocket. 2014, 51, 604–613. [Google Scholar] [CrossRef]
- Mallikarachchi, H.M.Y.C.; Pellegrino, S. Design of Ultrathin Composite Self-Deployable Booms. J. Spacecr. Rocket. 2014, 51, 1811–1821. [Google Scholar] [CrossRef]
- Mallikarachchi, H. Predicting mechanical properties of thin woven carbon fiber reinforced laminates. Thin-Walled Struct. 2019, 135, 297–305. [Google Scholar] [CrossRef]
- Chu, Z.; Lei, Y. Design theory and dynamic analysis of a deployable boom. Mech. Mach. Theory 2014, 71, 126–141. [Google Scholar] [CrossRef]
- Chu, Z.; Lei, Y.; Li, D. Dynamics and robust adaptive control of a deployable boom for a space probe. Acta Astronaut. 2014, 97, 138–150. [Google Scholar] [CrossRef]
- Cai, J.G.; Zhou, Y.; Wang, X.Y.; Xu, Y.X.; Feng, J. Dynamic analysis of a cylindrical boom based on Miura Origami. Steel Compos. Struct. 2018, 28, 607–615. [Google Scholar]
- Bai, J.-B.; Chen, D.; Xiong, J.-J.; Shenoi, R.A. Folding analysis for thin-walled deployable composite boom. Acta Astronaut. 2019, 159, 622–636. [Google Scholar] [CrossRef] [Green Version]
- Angeletti, F.; Gasbarri, P.; Sabatini, M. Optimal design and robust analysis of a net of active devices for micro-vibration control of an on-orbit large space antenna. Acta Astronaut. 2019, 164, 241–253. [Google Scholar] [CrossRef]
- Chen, W.; Fang, G.; Hu, Y. An experimental and numerical study of flattening and wrapping process of deployable composite thin-walled lenticular tubes. Thin-Walled Struct. 2017, 111, 38–47. [Google Scholar] [CrossRef]
- Li, C.; Angeles, J.; Guo, H.; Tang, D.; Liu, R.; Qin, Z.; Xiao, H. On the Actuation Modes of a Multiloop Mechanism for Space Applications. IEEE/ASME Trans. Mechatron. 2021. [Google Scholar] [CrossRef]
- Liu, J.; Wen, G.; Zuo, H.Z.; Qing, Q. A simple reliability-based topology optimization approach for continuum structures using a topology description function. Eng. Optim. 2016, 48, 1182–1201. [Google Scholar] [CrossRef]
- Bessa, M.; Pellegrino, S. Design of ultra-thin shell structures in the stochastic post-buckling range using Bayesian machine learning and optimization. Int. J. Solids Struct. 2018, 139–140, 174–188. [Google Scholar] [CrossRef]
- Bessa, M.A.; Bostanabad, R.; Liu, Z.; Hu, A.; Apley, D.W.; Brinson, C.; Chen, W.; Liu, W.K. A framework for data-driven analysis of materials under uncertainty: Countering the curse of dimensionality. Comput. Methods Appl. Mech. Eng. 2017, 320, 633–667. [Google Scholar] [CrossRef]
- Seffen, K.; Wang, B.; Guest, S. Folded orthotropic tape-springs. J. Mech. Phys. Solids 2018, 123, 138–148. [Google Scholar] [CrossRef] [Green Version]
- Roybal, F.; Banik, J.; Murphey, T. Development of an Elastically Deployable Boom for Tensioned Planar Structures. In Proceedings of the 48th AIAA/ASME/ASCE/AHS/ASC Structures, Structural Dynamics, and Materials Conference, Honolulu, HI, USA, 23–26 April 2007. [Google Scholar]
- Yang, H.; Lu, F.S.; Guo, H.W.; Liu, R.Q. Design of a new N-shape composite ultra-thin deployable boom in the post-buckling range using response surface method and optimization. IEEE Access 2019, 7, 129659–129665. [Google Scholar] [CrossRef]
- Jiang, Z.; Gu, M. Optimization of a fender structure for the crashworthiness design. Mater. Des. 2010, 31, 1085–1095. [Google Scholar] [CrossRef]




| Material Properties | T800 |
|---|---|
| Longitudinal stiffness E1/GPa | 150 |
| Transverse stiffness E2 = E3/GPa | 9.4 |
| Shear stiffness G12 = G13/GPa | 9.4 |
| In-plane shear stiffness G23/GPa | 4.5 |
| Poisson’s ratio υ | 0.3 |
| Density ρ kg/m3 | 2500 |
| No. | r/mm | θ/° | w/mm | Mpeak/Nm | Smax/MPa |
|---|---|---|---|---|---|
| 1 | 27 | 52.5 | 7 | 30.01 | 620 |
| 2 | 27 | 52.5 | 8 | 30.02 | 632 |
| 3 | 27 | 52.5 | 9 | 38.32 | 635 |
| 4 | 27 | 55 | 7 | 27.81 | 632 |
| 5 | 27 | 55 | 8 | 30.88 | 634 |
| 6 | 27 | 55 | 9 | 33.67 | 654 |
| 7 | 27 | 57.5 | 7 | 26.08 | 702 |
| 8 | 27 | 57.5 | 8 | 31.79 | 713 |
| 9 | 27 | 57.5 | 9 | 32.87 | 675 |
| 10 | 27 | 60 | 7 | 26.44 | 620 |
| 11 | 27 | 60 | 8 | 38.28 | 679 |
| 12 | 27 | 60 | 9 | 34.43 | 719 |
| 13 | 25 | 52.5 | 7 | 29.86 | 647 |
| 14 | 25 | 52.5 | 8 | 34.94 | 685 |
| 15 | 25 | 52.5 | 9 | 33.89 | 682 |
| 16 | 25 | 55 | 7 | 25.58 | 663 |
| 17 | 25 | 55 | 8 | 25.62 | 687 |
| 18 | 25 | 55 | 9 | 29.88 | 675 |
| 19 | 25 | 57.5 | 7 | 27.23 | 674 |
| 20 | 25 | 57.5 | 8 | 27.91 | 671 |
| 21 | 25 | 57.5 | 9 | 28.60 | 674 |
| 22 | 25 | 60 | 7 | 30.03 | 656 |
| 23 | 25 | 60 | 8 | 28.14 | 649 |
| 24 | 25 | 60 | 9 | 31.60 | 694 |
| 25 | 23 | 52.5 | 7 | 29.45 | 624 |
| 26 | 23 | 52.5 | 8 | 31.18 | 620 |
| 27 | 23 | 52.5 | 9 | 31.35 | 673 |
| 28 | 23 | 55 | 7 | 37.76 | 704 |
| 29 | 23 | 55 | 8 | 39.33 | 714 |
| 30 | 23 | 55 | 9 | 28.64 | 730 |
| 31 | 23 | 57.5 | 7 | 30.52 | 681 |
| 32 | 23 | 57.5 | 8 | 32.62 | 695 |
| 33 | 23 | 57.5 | 9 | 29.32 | 729 |
| 34 | 23 | 60 | 7 | 34.57 | 642 |
| 35 | 23 | 60 | 8 | 35.17 | 736 |
| 36 | 23 | 60 | 9 | 41.01 | 668 |
| No | r/mm | θ/° | w/mm | Mpeak/Nm | RE/(%) | Smax/MPa | RE/(%) | ||
|---|---|---|---|---|---|---|---|---|---|
| FE Result | BPNN Result | FE Result | BPNN Result | ||||||
| 1 | 23 | 57 | 8.5 | 35.089 | 32.8801 | −6.30 | 714 | 737.5714 | 3.30 |
| 2 | 24 | 53 | 7.5 | 30.3478 | 29.3259 | −3.37 | 655 | 648.597 | −0.98 |
| 3 | 24.5 | 56 | 7.3 | 25.534 | 26.013 | 1.88 | 643 | 677.66 | 5.39 |
| 4 | 26.5 | 60 | 7.2 | 24.926 | 25.775 | 3.41 | 657 | 639.709 | −2.63 |
| 5 | 25.5 | 54 | 8.5 | 30.2272 | 29.7083 | −1.72 | 667 | 691.2579 | 3.64 |
| No. | r/mm | φ/° | w/mm | Mpeak/Nm | RE/(%) | Smax/MPa | RE/(%) | ||
|---|---|---|---|---|---|---|---|---|---|
| FE Result | BPNN Result | FE Result | BPNN Result | ||||||
| 1 | 23.00 | 53.31 | 7.52 | 33.72 | 34.02 | 0.87 | 688 | 650 | −5.54 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, H.; Fan, S.; Wang, Y.; Shi, C. Novel Four-Cell Lenticular Honeycomb Deployable Boom with Enhanced Stiffness. Materials 2022, 15, 306. https://doi.org/10.3390/ma15010306
Yang H, Fan S, Wang Y, Shi C. Novel Four-Cell Lenticular Honeycomb Deployable Boom with Enhanced Stiffness. Materials. 2022; 15(1):306. https://doi.org/10.3390/ma15010306
Chicago/Turabian StyleYang, Hui, Shuoshuo Fan, Yan Wang, and Chuang Shi. 2022. "Novel Four-Cell Lenticular Honeycomb Deployable Boom with Enhanced Stiffness" Materials 15, no. 1: 306. https://doi.org/10.3390/ma15010306
APA StyleYang, H., Fan, S., Wang, Y., & Shi, C. (2022). Novel Four-Cell Lenticular Honeycomb Deployable Boom with Enhanced Stiffness. Materials, 15(1), 306. https://doi.org/10.3390/ma15010306






