Experimental Study on Biaxial Dynamic Compressive Properties of ECC
Abstract
1. Introduction
2. Materials and Methods
2.1. Test Raw Materials and Mix Ratio
2.2. Test Device
2.3. Test Piece Preparation and Test Method
3. Results and Discussion
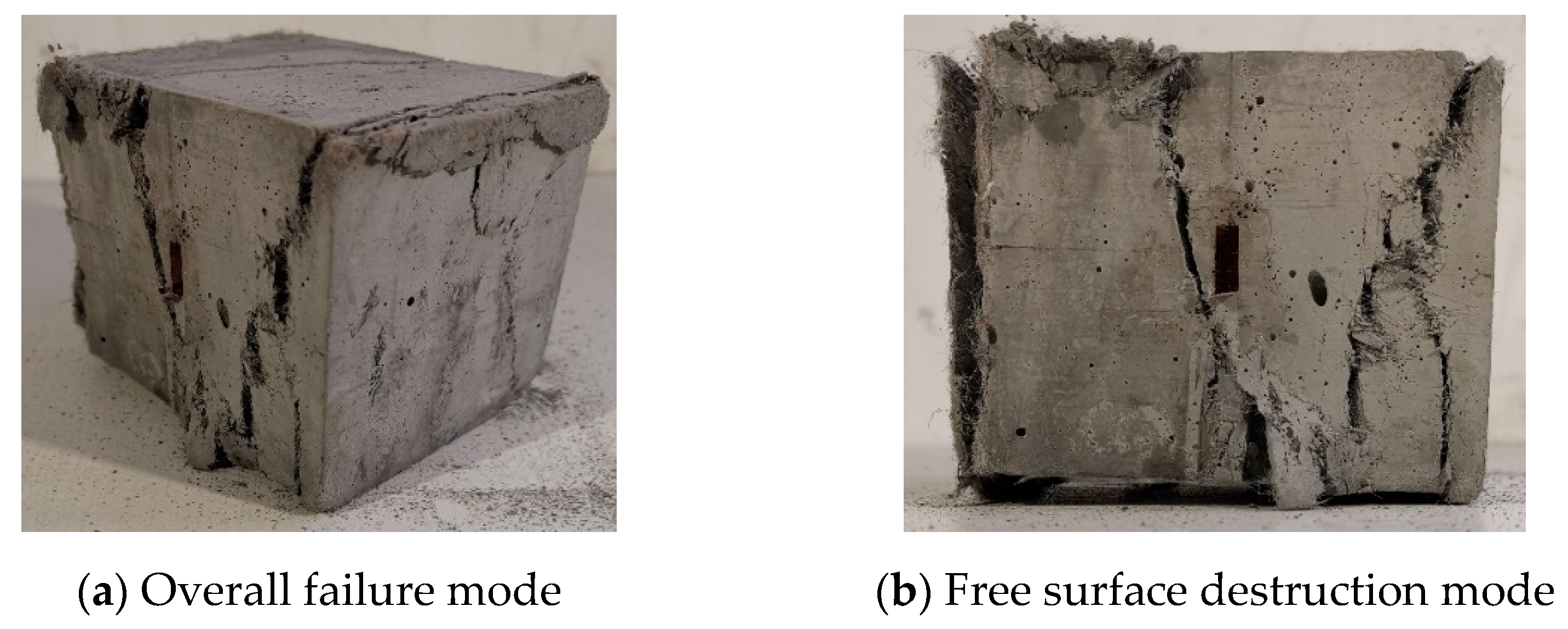
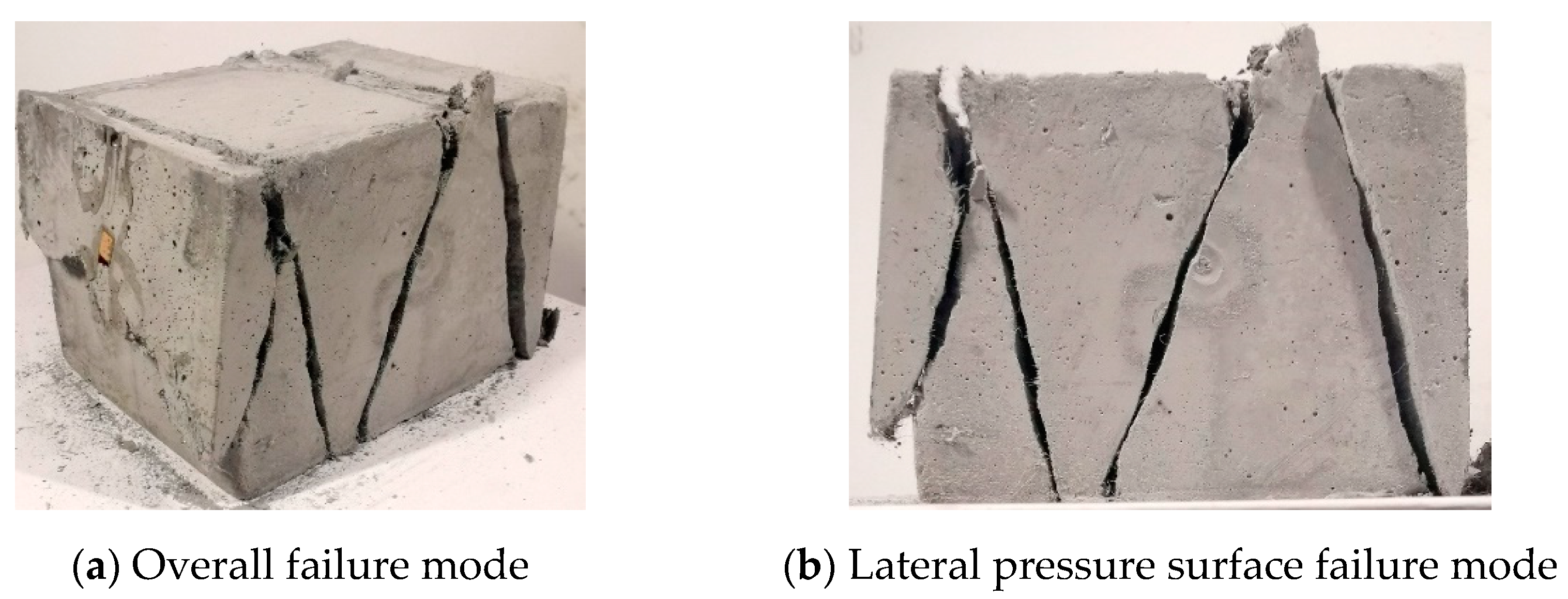
3.1. Damage Form
3.2. Peak Stress
3.2.1. ECC Biaxial Failure Criterion
3.2.2. Peak Stress under Different Lateral Pressure and Different Loading Rate
3.2.3. Dynamic Intensity Growth Factor DIF − σ
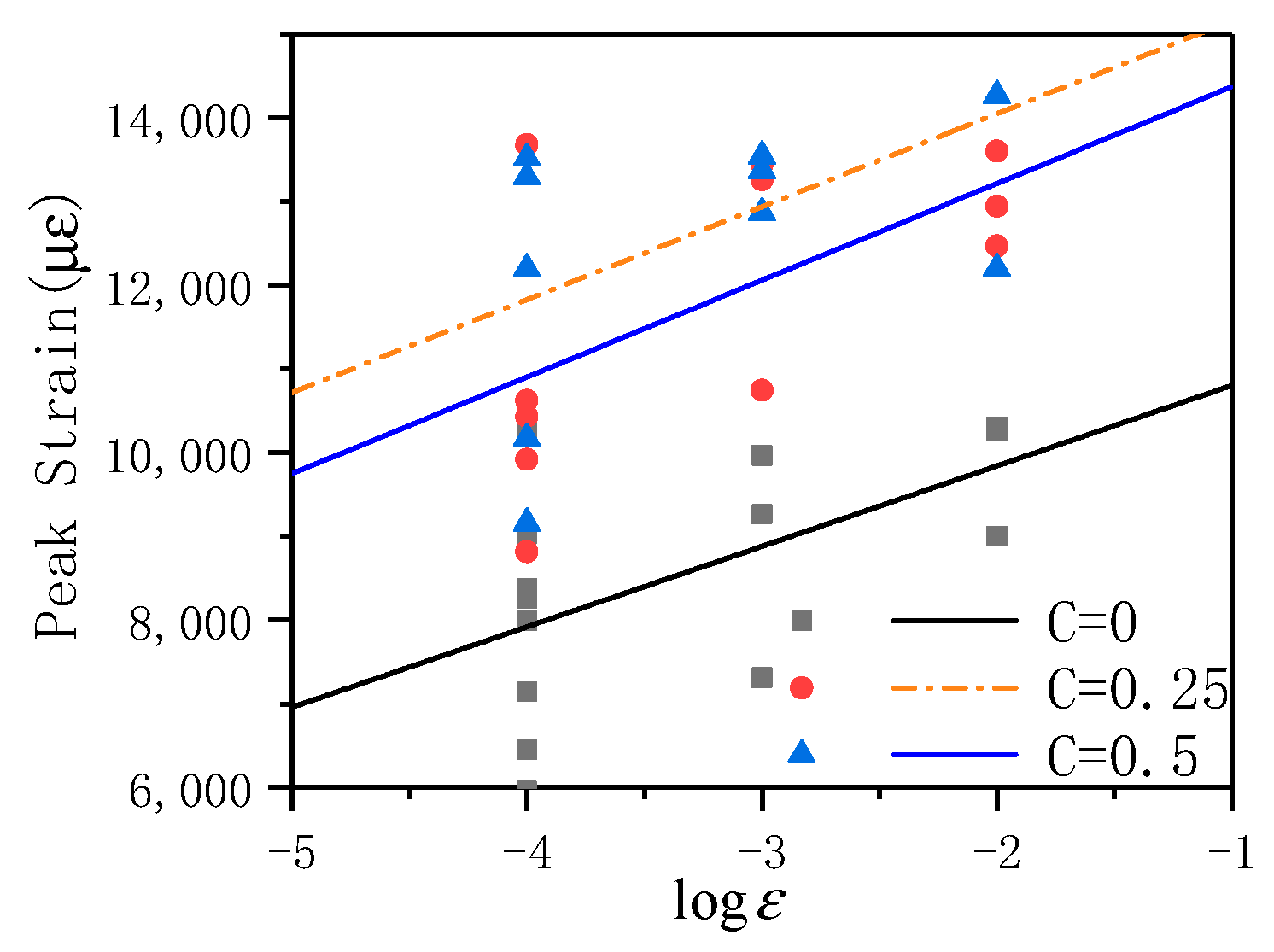
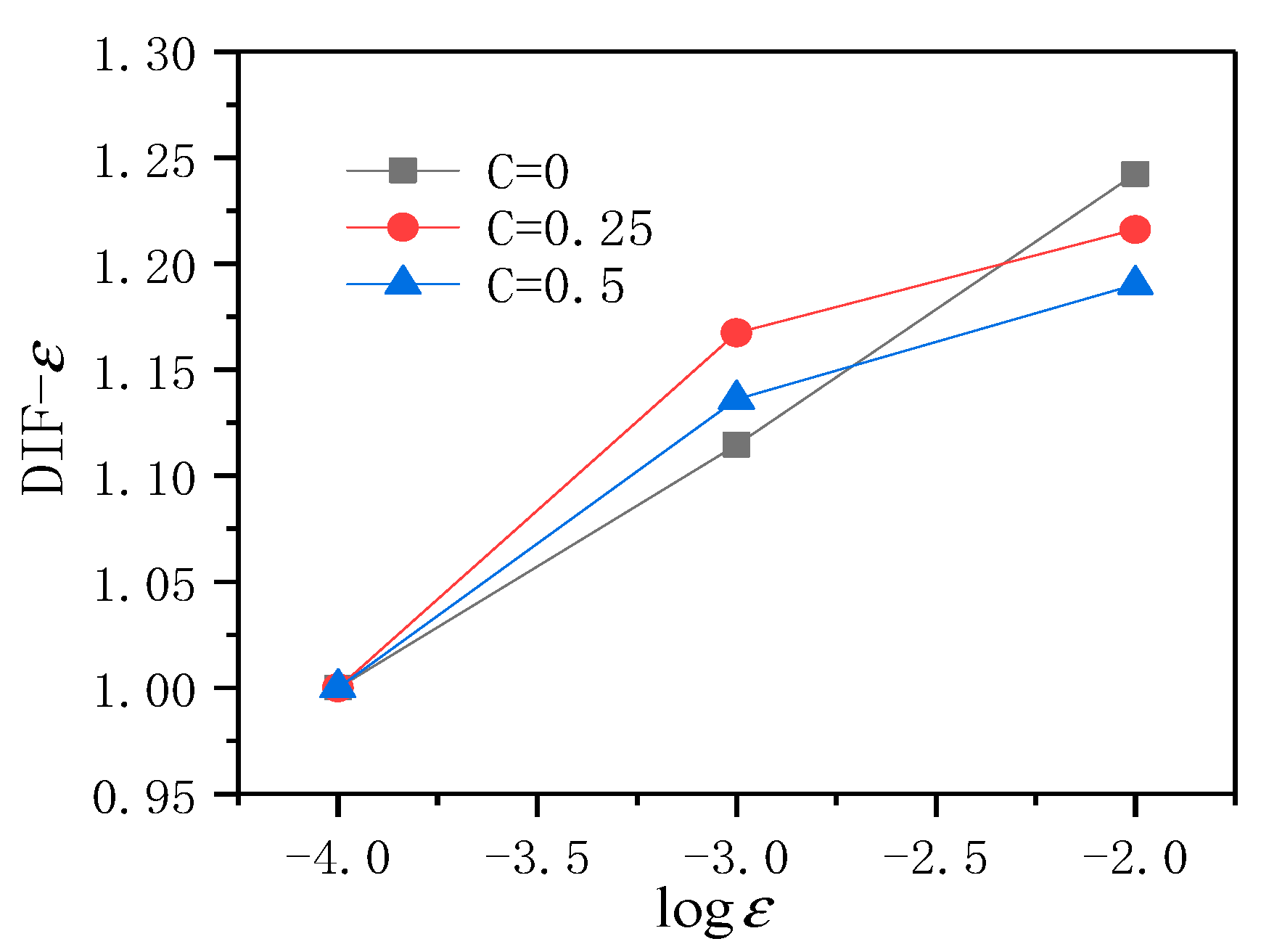
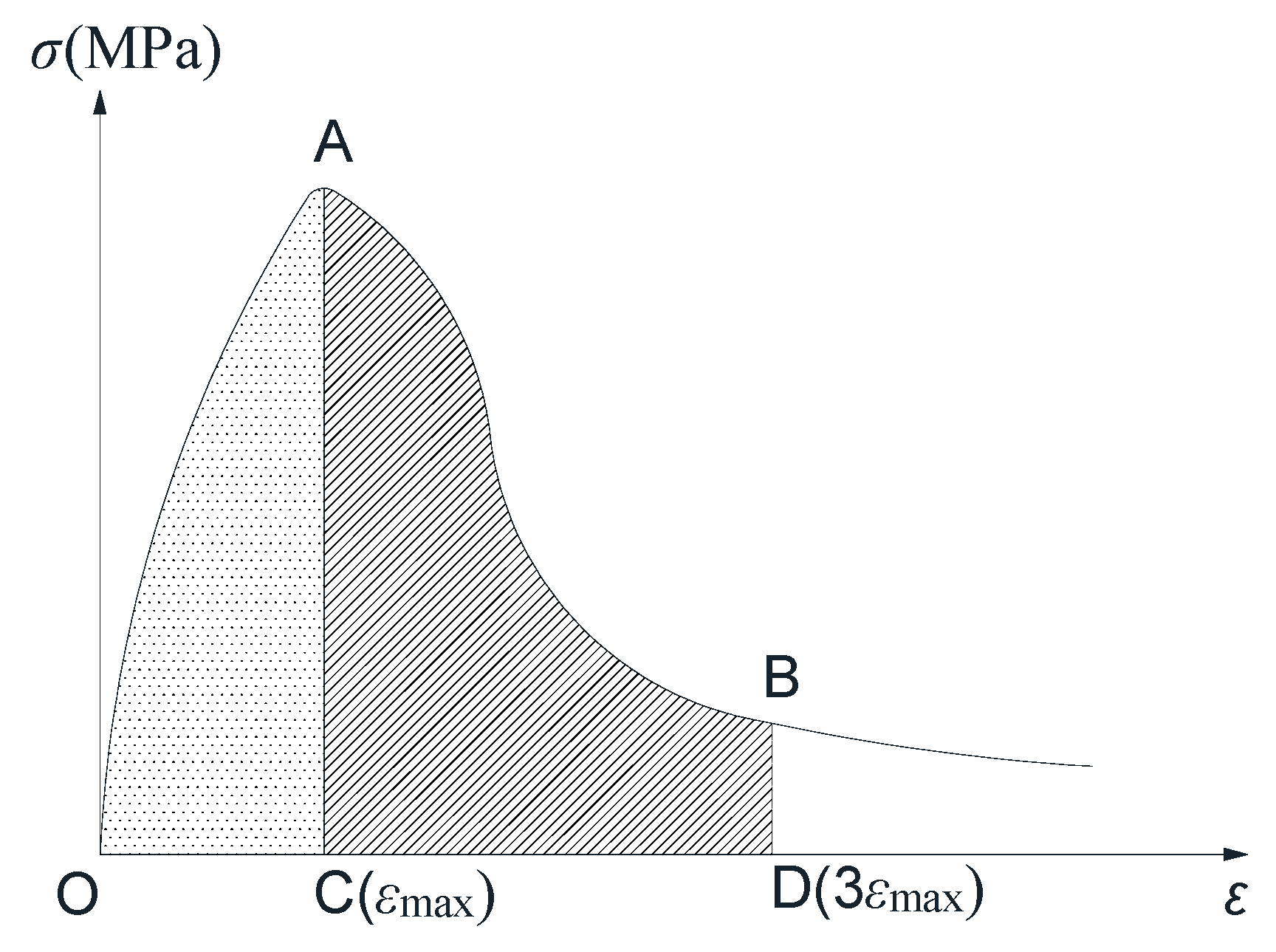
3.3. Peak Strain
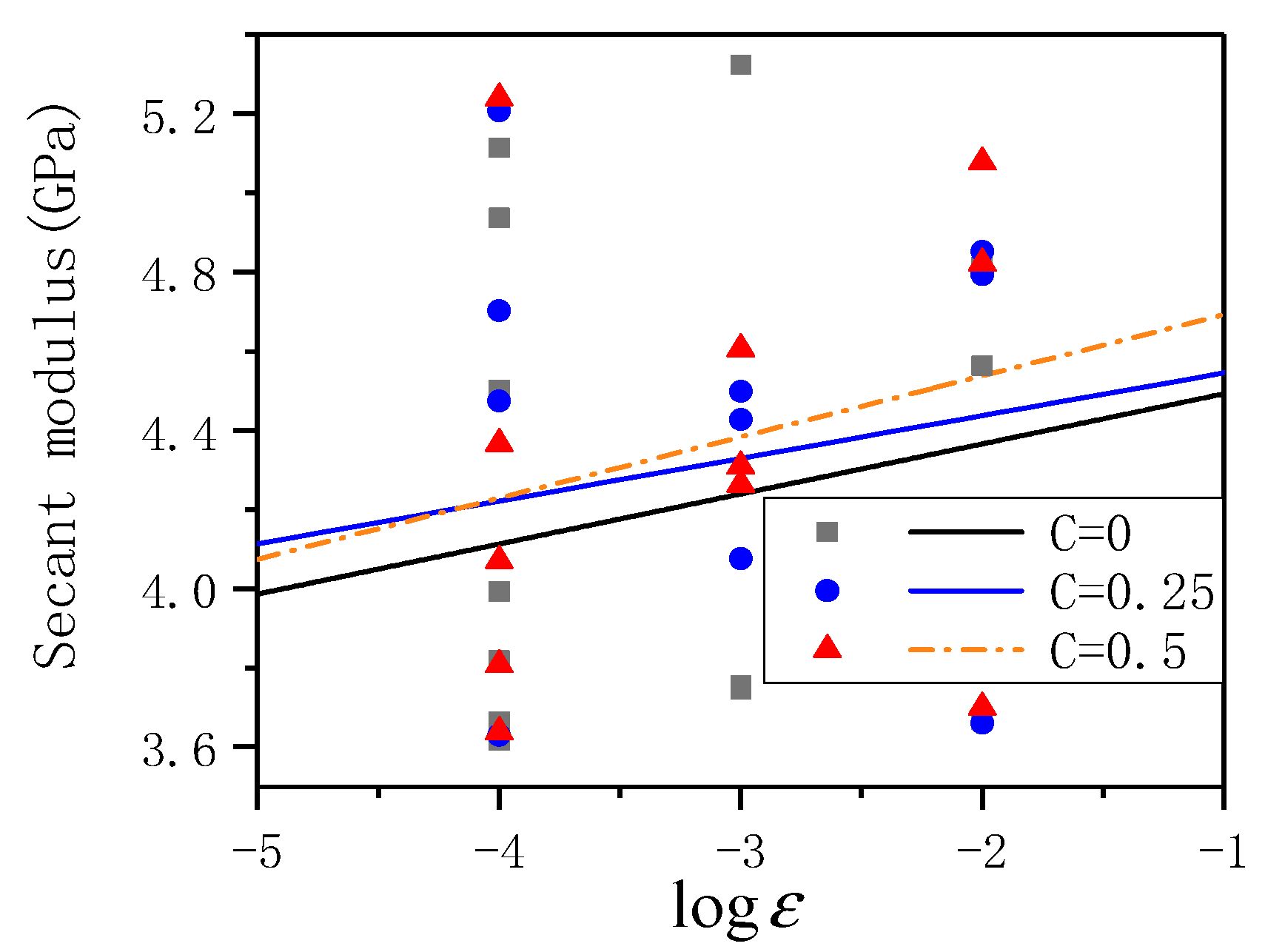
3.4. Deformation Modulus
- (1)
- ECC elastic modulus (origin modulus) . The slope of the tangent line at the origin of the ECC compressive stress-strain curve is the initial elastic modulus of the ECC.
- (2)
- The deformation modulus of ECC (secant modulus) , that is, ratio of peak stress to peak strain which is called the mean secant modulus of ECC.
- (3)
- The tangent modulus of ECC is the tangent slope at a specific stress value on the ECC stress-strain curve taken as the tangent modulus of ECC.
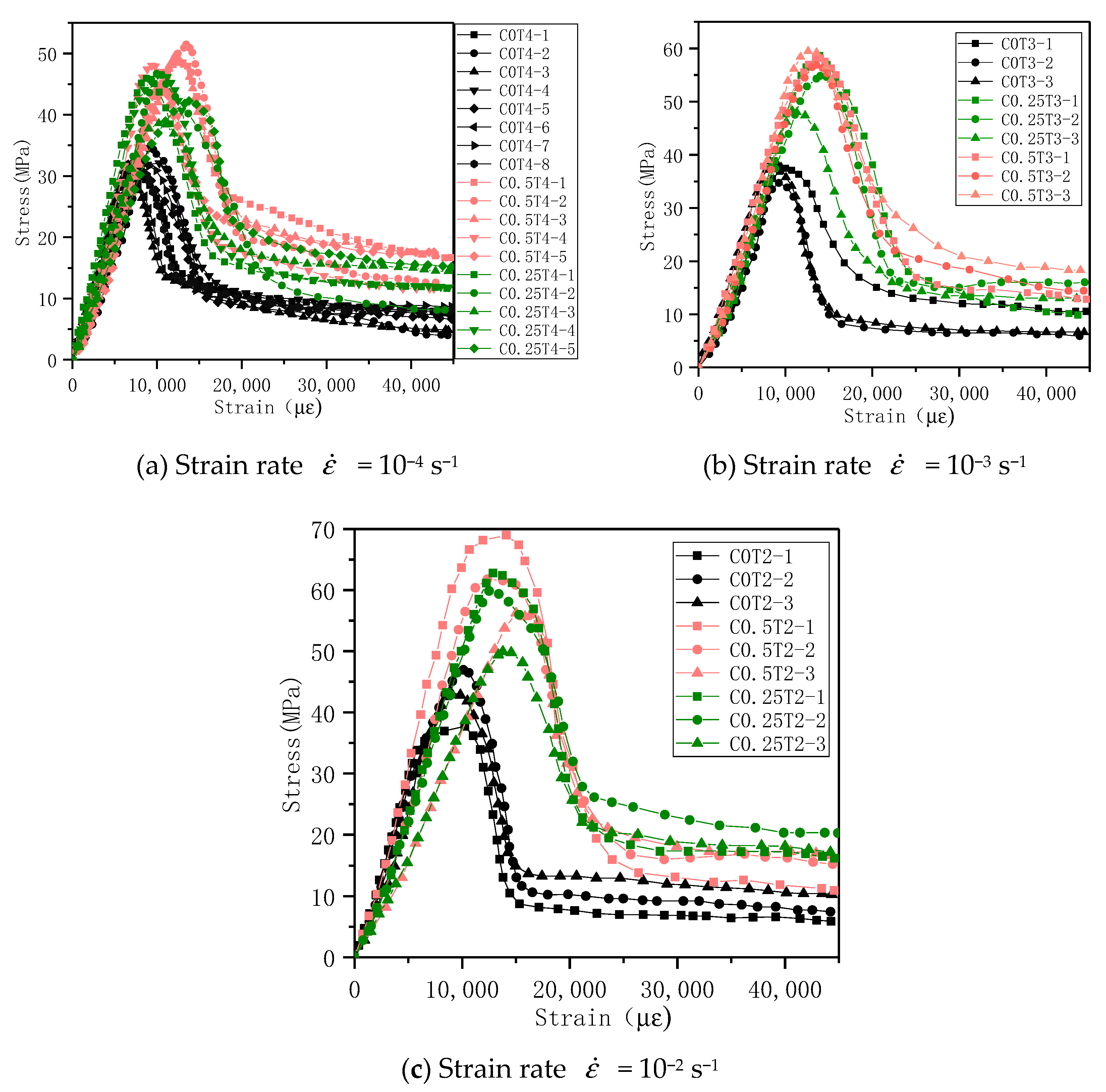
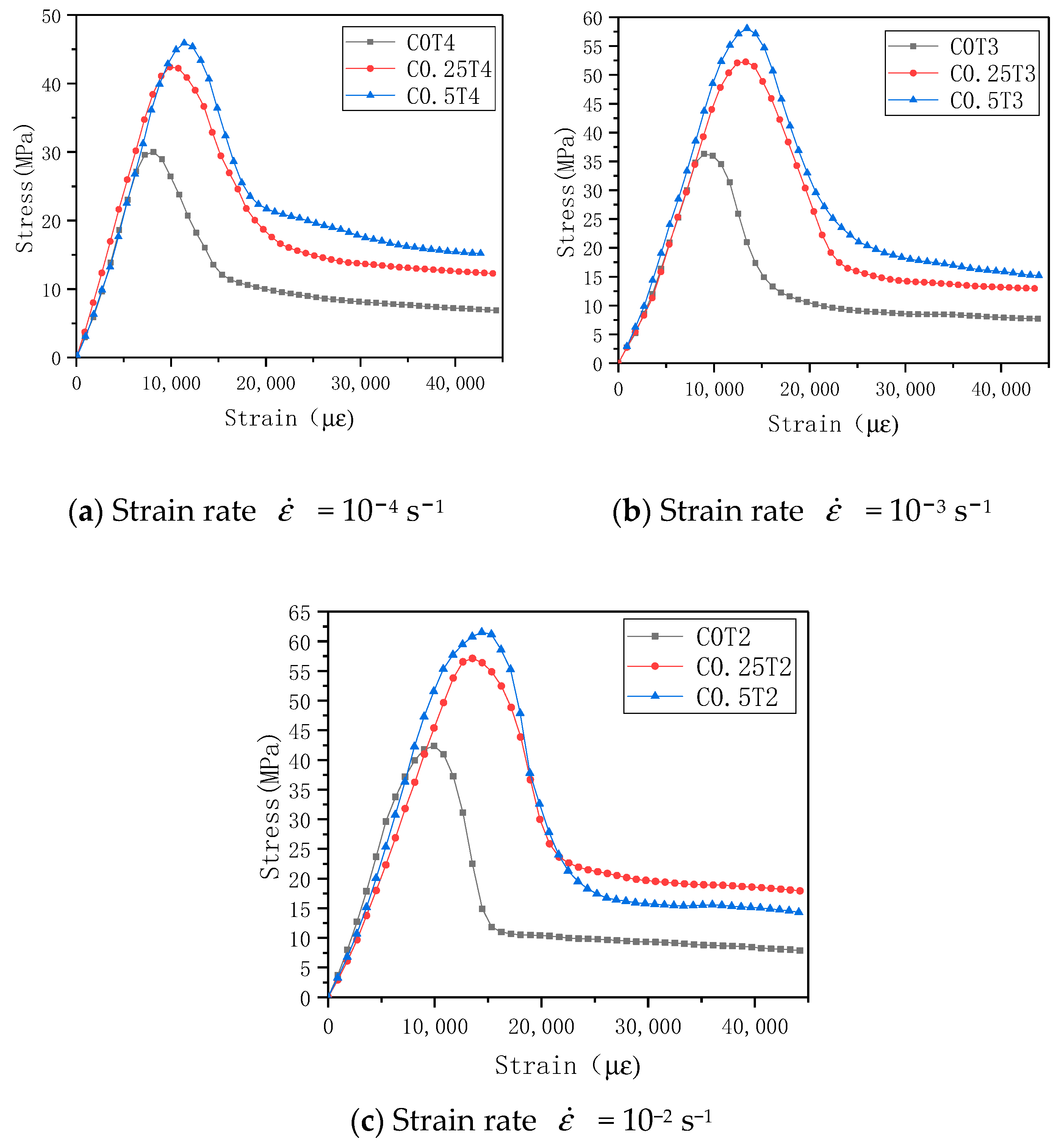
3.5. Stress-Strain Curve
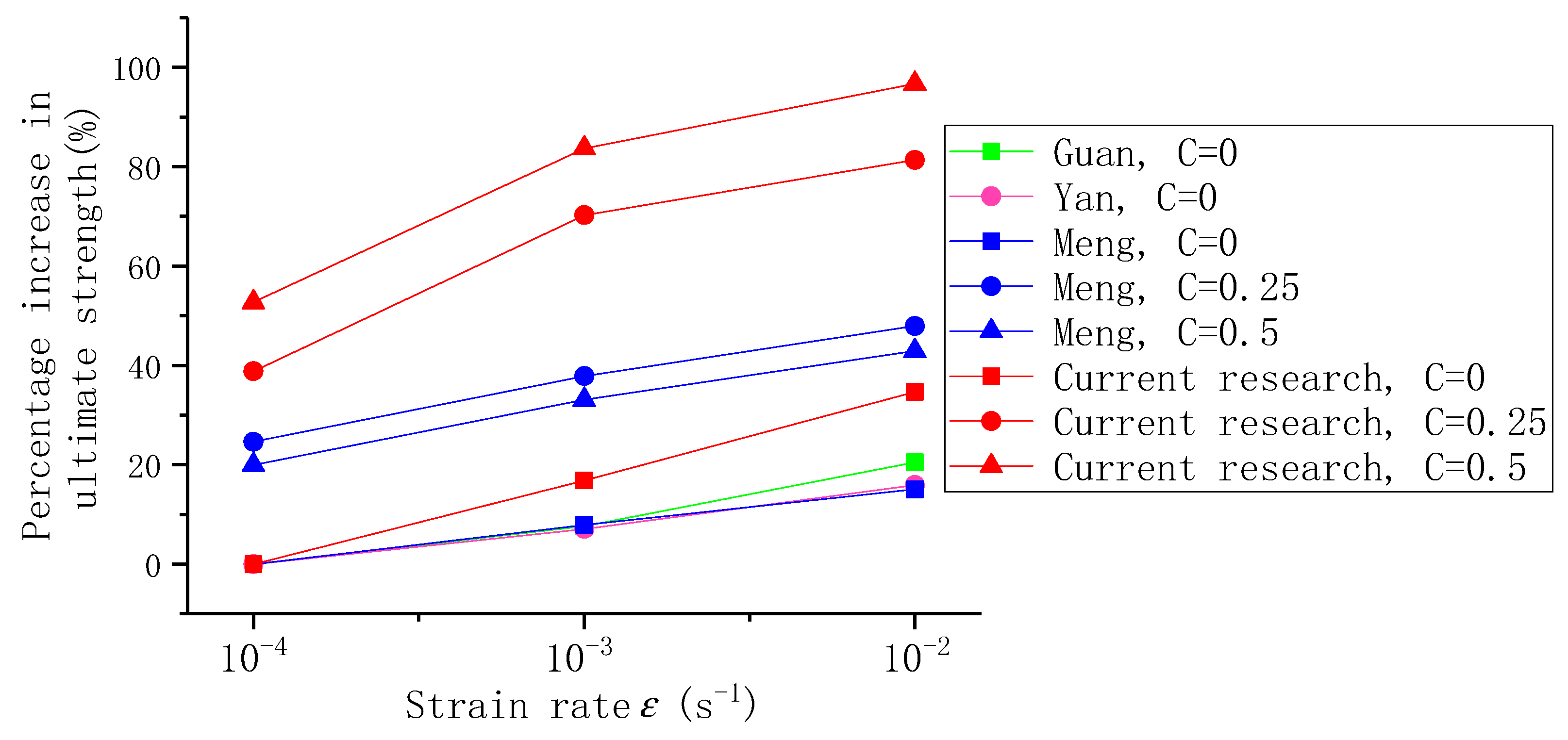
3.6. Comparison with Other Researchers
4. Conclusions
- (1)
- Under uniaxial compression, due to the internal bridging effect of the ECC specimen, the failure form of the specimen is a shear failure, and the main cracks of the specimen are generated on both surfaces. Under various lateral pressure stress levels, the failure form of the specimen is also shear failure, but the main crack direction appears on the lateral pressure surface. The significant cracks are generally 2–3, and the cracking direction is 15~30° with the longitudinal direction. The strain rate has little effect on the final failure form of the specimen.
- (2)
- Under the condition of biaxial compressive stress, ECC’s peak stress is higher than that under uniaxial. When the lateral pressure level is 0.7, the specimen’s peak stress reaches the highest, which is increased by 59%. Based on the normalized stress level, the failure criterion of ECC under biaxial is established, and the fitting effect is good, which can be used to predict the peak stress of ECC under different lateral pressures. Through dynamic strength growth factor DIF − σ and dynamic strain growth factor DIF − ε, the effect of strain rate on ECC peak stress and strain under different lateral pressure levels was evaluated. With the lateral pressure increases, the peak stress of ECC increases continuously. At the same time, under a certain level of lateral pressure, with the strain rate increases, the peak strain of ECC is also growing.
- (3)
- Under different lateral pressure levels and other stress levels, the deformation modulus of ECC has greater dispersion, but overall, the increase in lateral pressure and strain rate increases the average deformation modulus of ECC; however, the growth of deformation modulus is small. Through fitting, it is found that the ECC deformation modulus and the strain rate increase approximately linearly with the growth of the lateral pressure level.
- (4)
- The stress-strain curve of ECC under different lateral pressures and different strain rates can be divided into four stages: elastic stage, crack propagation stage, crack instability propagation stage, and residual stage. The compressive toughness index quantitatively evaluated the compressive toughness of ECC. When the strain rate is constant, as the lateral pressure level increases, the compressive toughness of ECC increases; when the lateral pressure level is constant, as the strain rate increases, ECC’s compressive toughness gradually decreases.
- (5)
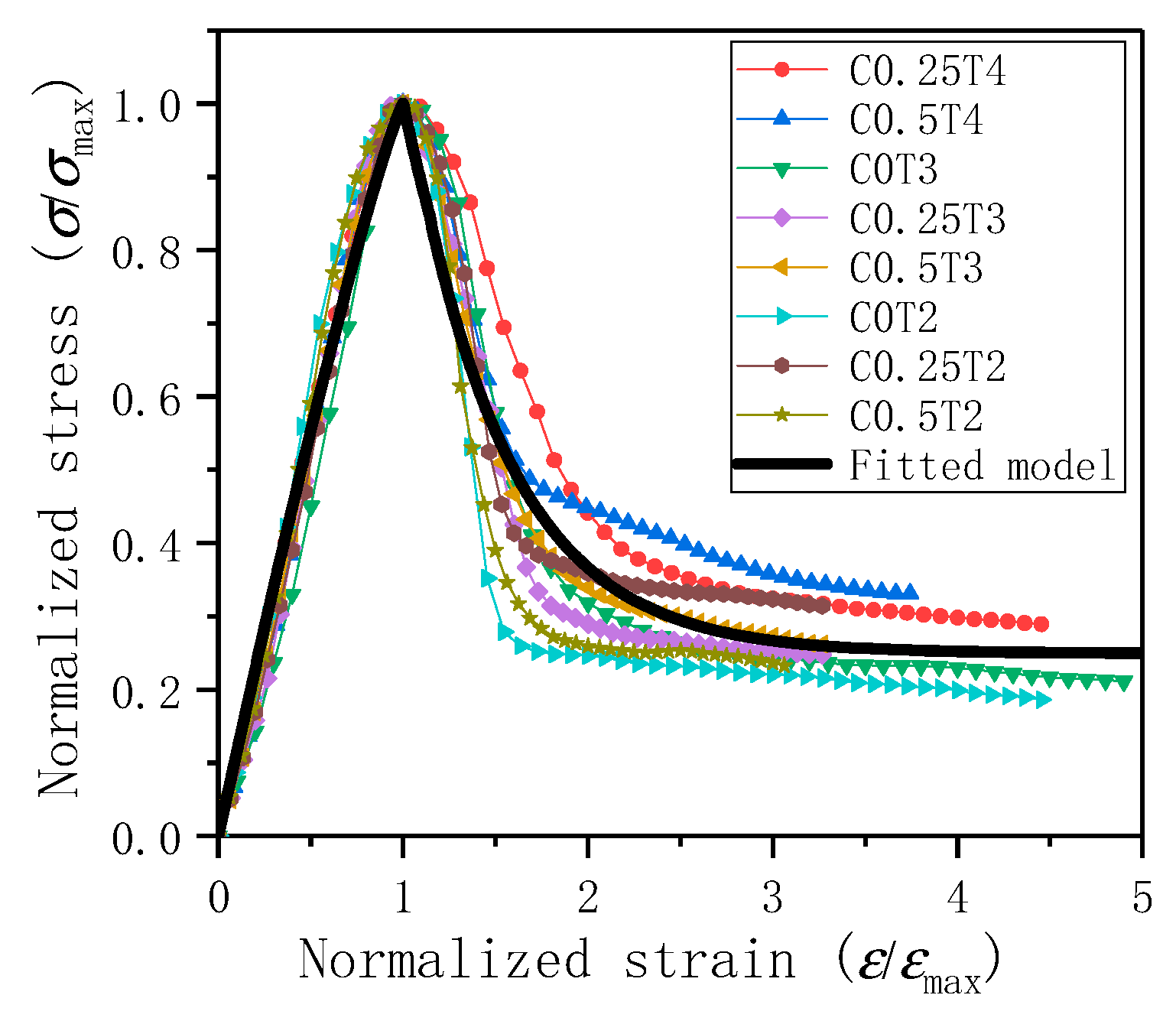
- A normalized ECC constitutive relationship is established. The known static compression test results can be used to estimate the full curve of ECC compressive stress and strain under different lateral pressure levels and different strain rates.
- (6)
- Compared with concrete, the ultimate strength improvement of ECC under biaxial dynamic conditions is significantly higher than that of plain concrete. In the future, we can explore the application of ECC structures in high strain rate and complex stress environments.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Li, V.C.; Leung, C.K.Y. Steady-State and Multiple Cracking of Short Random Fiber Composites. J. Eng. Mech. 1992, 118, 2246–2264. [Google Scholar] [CrossRef]
- Xu, S.; Hou, L.; Zhang, X. Flexural and shear behaviors of reinforced ultrahigh toughness cementitious composite beams without web reinforcement under concentrated load. Eng. Struct. 2012, 39, 176–186. [Google Scholar] [CrossRef]
- Kanda, T.; Nagai, S.; Maruta, M.; Yamamoto, Y. New high-rise R/C structure using ECC coupling beams. In Proceedings of the 2nd International RILEM Conference on Strain Hardening Cementitious Composites, Rio de Janeiro, Brazil, 12–14 December 2011; pp. 12–14. [Google Scholar]
- Li, V.C.; Wang, S.; Wu, C. Tensile strain-hardening behavior or polyvinyl alcohol engineered cementitious composite (PVA-ECC). ACI Mater. J. 2001, 98, 483–492. [Google Scholar]
- Fukuda, I.; Mitamura, H.; Imano, H.; Matsui, S. Effect of ECC overlay reinforcement method on steel plate deck attached with FRP dowels. Proc. Jpn. Concr. Inst. 2004, 26, 1693–1698. [Google Scholar]
- Kunieda, M.; Kamada, T.; Rokugo, K.; Bolander, J.E. Localized fracture of repaired material in patch repair systems. In Proceedings of the International Conference on Fracture Mechanics of Concrete Structures, Berkeley, CA, USA, 12–16 April 2004; pp. 765–772. [Google Scholar]
- Li, V.C.; Fischer, G.; Lepech, M. Shotcreting with ECC; Kusterle, W., Ed.; Spritzbeton Tagung: Innsbruck, Austria, 2009. [Google Scholar]
- Xu, S.; Li, Q. The Basic Application of Ultra-High Toughness Cement-Based Composite Materials in High-Performance Building Structures; Science Press: Beijing, China, 2010. [Google Scholar]
- Takeda, J.; Tachikawa, H.; Fujimoto, K. Mechanical behavior of concrete under higher rateloading than in static test. Mech. Behav. Mater. 1974, 2, 479–486. [Google Scholar]
- Yamaguchi, H.; Fujimoto, K.; Nomura, S. Strain rate effect on stress-strain relationships of concrete. In Proceedings of the 4th Symposium on the Interaction of Non-Nuclear Munitions with Structures, Palm Beach, FL, USA, 17–21 April 1989; pp. 290–295. [Google Scholar]
- Lu, P. Research on Uniaxial and Biaxial Dynamic Strength and Deformation of Concrete. Ph.D. Thesis, Dalian University of Technology, Dalian, China, November 2001. [Google Scholar]
- Yan, D. Experimental and Theoretical Research on the Dynamic Mechanical Properties of Concrete. Ph.D. Thesis, Dalian University of Technology, Dalian, China, 2006. [Google Scholar]
- Liang, C. Experimental study on concrete biaxial dynamic compression under constant lateral pressure. China Rural Water Hydropower 2013, 2. [Google Scholar] [CrossRef]
- Guan, P. Experimental study on the biaxial dynamic compressive performance of concrete under constant lateral pressure. China Civil Eng. J. 2009, 4. [Google Scholar]
- Meng, S. Research on Concrete Biaxial Dynamic Test. Master’s Thesis, Dalian University of Technology, Dalian, China, 2010. [Google Scholar]
- Foltz, R.R.; Lee, D.H.; LaFave, J.M. Biaxial behavior of high-performance fiber-reinforced cementitious composite plates. Constr. Build. Mater. 2017, 143, 501–514. [Google Scholar] [CrossRef]
- Pan, J.; He, J.; Wang, L.; Zhou, J. Experimental study on ECC biaxial compression performance and failure criteria. Eng. Mech. 2016, 33, 186–193. [Google Scholar]
- Li, Y.; Liang, X.W.; Liu, Z.J. Behavior of high performance PVA fiber reinforced cement composites in triaxial compression. J. Wuhan Univ. Tech. 2010, 32, 179–185. [Google Scholar]
- Sirijaroonchai, K.; El-Tawil, S.; Parra-Montesinos, G. Behavior of high performance fiber reinforced cement composites under multiaxial compressive loading. Cem. Concr. Compos. 2010, 32, 62–72. [Google Scholar] [CrossRef]
- Caverzan, A.; Cadoni, E.; Di Prisco, M. Tensile behaviour of high performance fibre-reinforced cementitious composites at high strain rates. Int. J. Impact Eng. 2012, 45, 28–38. [Google Scholar] [CrossRef]
- Yang, H. Study on Dynamic Mechanical Behavior and Constitutive Model of Fiber Reinforced Cemenitious Composites. Ph.D. Thesis, South China University of Technology, Guangzhou, China, 2016. [Google Scholar]
- Zhao, X. Experimental and Theoretical Research on Dynamic Mechanical Properties of Ultra-High Toughness Cement-Based Composites. Master’s Thesis, Zhejiang University, Hangzhou, China, 2018. [Google Scholar]
- Xu, S. Experimental Study on Dynamic Compression Mechanical Properties of Ultra-High Toughness Cement-Based Composites. Master’s Thesis, Zhejiang Ocean University, Zhejiang, China, 2017. [Google Scholar]
- Xu, S.; Chen, C.; Li, Q.; Zhao, X. Numerical simulation study on dynamic compression mechanical properties of ultra-high toughness cement-based composites. Eng. Mech. 2019, 36, 50–59. [Google Scholar] [CrossRef]
- Yin, W.S.; Su, E.C.; Mansur, M.A.; Hsu, T.T. Biaxial tests of plain and fiber concrete. Mater. J. 1989, 86, 236–243. [Google Scholar]
- Mansur, M.A.; Chin, M.S.; Wee, T.H. Stress-strain relationship of high-strength fiber concrete in compression. J. Mater. Civ. Eng. 1999, 11, 21–29. [Google Scholar] [CrossRef]
- Luo, F. A Meso-Numerical Study on the Effect of Spacing and Matrix Strength on the Drawing Performance of Twin Wires under Dynamic Loa. Master’s Thesis, Guangzhou University, Guangzhou, China, 2019. [Google Scholar]





| Diameter (mm) | Length (mm) | Length Diameter Ratio | Elongation (%) | Tensile Strength (MPa) | Young’s Modulus (GPa) | Density (kg/m3) |
|---|---|---|---|---|---|---|
| 0.04 | 12 | 300 | 6 | 1600 | 40 | 1300 |
| Number | Cement | Fly Ash | Silicon Sand | Silica Dume | Water | Reducer | PVA Volume Content |
|---|---|---|---|---|---|---|---|
| M8 | 1 | 3.9 | 1.25 | 0.1 | 1.25 | 0.725% | 2% |
| Sample Serial Number | Strain Rate (s−1) | Lateral Pressure Level C | Amount |
|---|---|---|---|
| C0T4-x | 10−4 | 0 | 8 |
| C0.125T4-x | 0.125 | 8 | |
| C0.25T4-x | 0.25 | 5 | |
| C0.5T4-x | 0.5 | 5 | |
| C0.7T4-x | 0.7 | 5 | |
| C0.8T4-x | 0.8 | 5 | |
| C0.9T4-x | 0.9 | 5 | |
| C1.0T4-x | 1 | 8 | |
| C0T3-x | 10−3 | 0 | 3 |
| C0.25T3-x | 0.25 | 3 | |
| C0.5T3-x | 0.5 | 3 | |
| C0T2-x | 10−2 | 0 | 3 |
| C0.25T2-x | 0.25 | 3 | |
| C0.5T2-x | 0.5 | 3 |
| Sample Serial Number | Lateral Pressure Level C | |||||||
|---|---|---|---|---|---|---|---|---|
| 0 | 0.125 | 0.25 | 0.5 | 0.7 | 0.8 | 0.9 | 1 | |
| 1 | 29.85 | 43.84 | 46.02 | 48.47 | 54.55 | 50.61 | 37.68 | 48.96 |
| 2 | 34.49 | 43.07 | 46.73 | 51.58 | 48.19 | 54.23 | 40.85 | 47.82 |
| 3 | 30.42 | 42.28 | 38.56 | 49.74 | 48.19 | 43.31 | 45.15 | 49.75 |
| 4 | 32.17 | 41.75 | 46.72 | 48.16 | 53.15 | 37.80 | 42.79 | 41.68 |
| 5 | 32.14 | 41.23 | 42.23 | 44.57 | 48.11 | 48.11 | 46.99 | 51.06 |
| 6 | 31.85 | 46.71 | 42.39 | |||||
| 7 | 31.93 | 37.06 | 48.37 | |||||
| 8 | 30.66 | 47.78 | 39.75 | |||||
| Average | 31.69 | 42.97 | 44.05 | 48.51 | 50.44 | 46.81 | 42.69 | 39.81 |
| Lateral Pressure Level C | Strain Rate(s−1) | ||||||
|---|---|---|---|---|---|---|---|
| No. | 10−4 | No. | 10−4 | No. | 10−3 | 10−2 | |
| 0 | 1 | 29.87 | 5 | 32.16 | 1 | 37.42 | 37.66 |
| 2 | 34.48 | 6 | 31.86 | 2 | 34.69 | 47.05 | |
| 3 | 30.46 | 7 | 31.92 | 3 | 38.94 | 43.33 | |
| 4 | 32.09 | 8 | 30.68 | ||||
| Average | 31.69 | 37.02 | 42.68 | ||||
| 0.25 | 1 | 45.92 | 4 | 46.67 | 1 | 58.71 | 62.80 |
| 2 | 46.66 | 5 | 42.18 | 2 | 54.79 | 59.78 | |
| 3 | 38.56 | 3 | 48.34 | 49.80 | |||
| Average | 44.00 | 53.95 | 57.46 | ||||
| 0.5 | 1 | 48.38 | 4 | 48.00 | 1 | 58.36 | 68.81 |
| 2 | 51.47 | 5 | 44.44 | 2 | 56.98 | 61.95 | |
| 3 | 49.66 | 3 | 59.26 | 56.26 | |||
| Average | 48.39 | 58.20 | 62.34 | ||||
| Lateral Pressure Level C | Strain Rate (s−1) | DIF − σ | Increasing Rate of DIF − σ |
|---|---|---|---|
| 0 | 10−4 | 1.00 | 0% |
| 10−3 | 1.17 | 17% | |
| 10−2 | 1.35 | 35% | |
| 0.25 | 10−4 | 1.00 | 0% |
| 10−3 | 1.23 | 23% | |
| 10−2 | 1.31 | 31% | |
| 0.5 | 10−4 | 1.00 | 0% |
| 10−3 | 1.20 | 20% | |
| 10−2 | 1.29 | 29% |
| Lateral Pressure Level C | Strain Rate (s−1) | ||||||
|---|---|---|---|---|---|---|---|
| No. | 10−4 | No. | 10−4 | No. | 10−3 | 10−2 | |
| 0 | 1 | 8259.43 | 5 | 7144.43 | 1 | 9964.45 | 10,267.59 |
| 2 | 9029.85 | 6 | 6453.55 | 2 | 9262.64 | 10,308.43 | |
| 3 | 5955.65 | 7 | 7993.91 | 3 | 7313.08 | 9001.68 | |
| 4 | 10,286.86 | 8 | 8373.49 | ||||
| Average | 7937.14 | 8846.72 | 9859.23 | ||||
| 0.25 | 1 | 8818.28 | 4 | 10,430.87 | 1 | 13,261.65 | 12,942.86 |
| 2 | 9921.37 | 5 | 13,676.69 | 2 | 13,441.80 | 12,469.28 | |
| 3 | 10,623.42 | 3 | 10,746.32 | 10,746.32 | |||
| Average | 10,694.12 | 12,483.26 | 13,005.03 | ||||
| 0.5 | 1 | 13,296.65 | 4 | 9160.04 | 1 | 13,543.13 | 14,267.89 |
| 2 | 13,519.30 | 5 | 10,176.52 | 2 | 13,366.30 | 12,199.68 | |
| 3 | 12,199.19 | 3 | 12,865.01 | 15,201.56 | |||
| Average | 11,670.34 | 13,258.15 | 13,889.71 | ||||
| Lateral Pressure Level C | Strain Rate (s−1) | DIF − ε | Increasing Rate of DIF − ε |
|---|---|---|---|
| 0 | 10−4 | 1.00 | 0% |
| 10−3 | 1.11 | 11% | |
| 10−2 | 1.24 | 24% | |
| 0.25 | 10−4 | 1.00 | 0% |
| 10−3 | 1.17 | 17% | |
| 10−2 | 1.22 | 22% | |
| 0.5 | 10−4 | 1.00 | 0% |
| 10−3 | 1.14 | 14% | |
| 10−2 | 1.19 | 19% |
| Lateral Pressure Level C | Strain Rate (s−1) | ||||||
|---|---|---|---|---|---|---|---|
| No. | 10−4 | No. | 10−4 | No. | 10−3 | 10−2 | |
| 0 | 1 | 3.62 | 5 | 4.50 | 1 | 3.76 | 3.67 |
| 2 | 3.82 | 6 | 4.94 | 2 | 3.75 | 4.56 | |
| 3 | 5.11 | 7 | 3.99 | 3 | 5.32 | 4.81 | |
| 4 | 3.12 | 8 | 3.66 | ||||
| Average | 4.10 | 4.28 | 4.35 | ||||
| 0.25 | 1 | 5.21 | 4 | 4.47 | 1 | 4.43 | 4.85 |
| 2 | 4.70 | 5 | 3.08 | 2 | 4.08 | 4.79 | |
| 3 | 3.63 | 3 | 4.50 | 3.66 | |||
| Average | 4.22 | 4.33 | 4.44 | ||||
| 0.5 | 1 | 3.64 | 4 | 5.24 | 1 | 4.31 | 4.82 |
| 2 | 3.81 | 5 | 4.37 | 2 | 4.26 | 5.08 | |
| 3 | 4.07 | 3 | 4.61 | 3.70 | |||
| Average | 4.22 | 4.39 | 4.53 | ||||
| Researcher | Uniaxial Ultimate Strength (MPa) | Material | Lateral Pressure Level C | The Percentage Increase of Ultimate Strength under Different Strain Rates (%) | ||
|---|---|---|---|---|---|---|
| 10−4 S−1 | 10−3 S−1 | 10−2 S−1 | ||||
| Guan [14] | 20.1 | Plain concrete | 0 | 0.00 | 7.63 | 20.49 |
| Yan [12] | 9.84 | Plain Concrete | 0 | 0.00 | 7.05 | 15.89 |
| Meng [15] | 34.36 | Plain Concrete | 0 | 0.00 | 7.87 | 15.03 |
| 0.25 | 24.64 | 37.82 | 47.91 | |||
| 0.5 | 19.96 | 33.11 | 42.91 | |||
| Current research | 31.69 | ECC | 0 | 0.00 | 16.82 | 34.68 |
| 0.25 | 38.85 | 70.24 | 81.32 | |||
| 0.5 | 52.70 | 83.65 | 96.72 | |||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gao, S.; Hu, G. Experimental Study on Biaxial Dynamic Compressive Properties of ECC. Materials 2021, 14, 1257. https://doi.org/10.3390/ma14051257
Gao S, Hu G. Experimental Study on Biaxial Dynamic Compressive Properties of ECC. Materials. 2021; 14(5):1257. https://doi.org/10.3390/ma14051257
Chicago/Turabian StyleGao, Shuling, and Guanhua Hu. 2021. "Experimental Study on Biaxial Dynamic Compressive Properties of ECC" Materials 14, no. 5: 1257. https://doi.org/10.3390/ma14051257
APA StyleGao, S., & Hu, G. (2021). Experimental Study on Biaxial Dynamic Compressive Properties of ECC. Materials, 14(5), 1257. https://doi.org/10.3390/ma14051257





