Single-Point Incremental Forming of Titanium and Titanium Alloy Sheets
Abstract
:1. Introduction
- The possibility of forming elements on a conventional CNC machine;
- Quick and easy consideration of design changes in the components being shaped;
- Significantly lower forming force values compared to conventional stamping;
- A higher value of the deformation limit of the sheet compared to conventional stamping.
2. Methods of Review
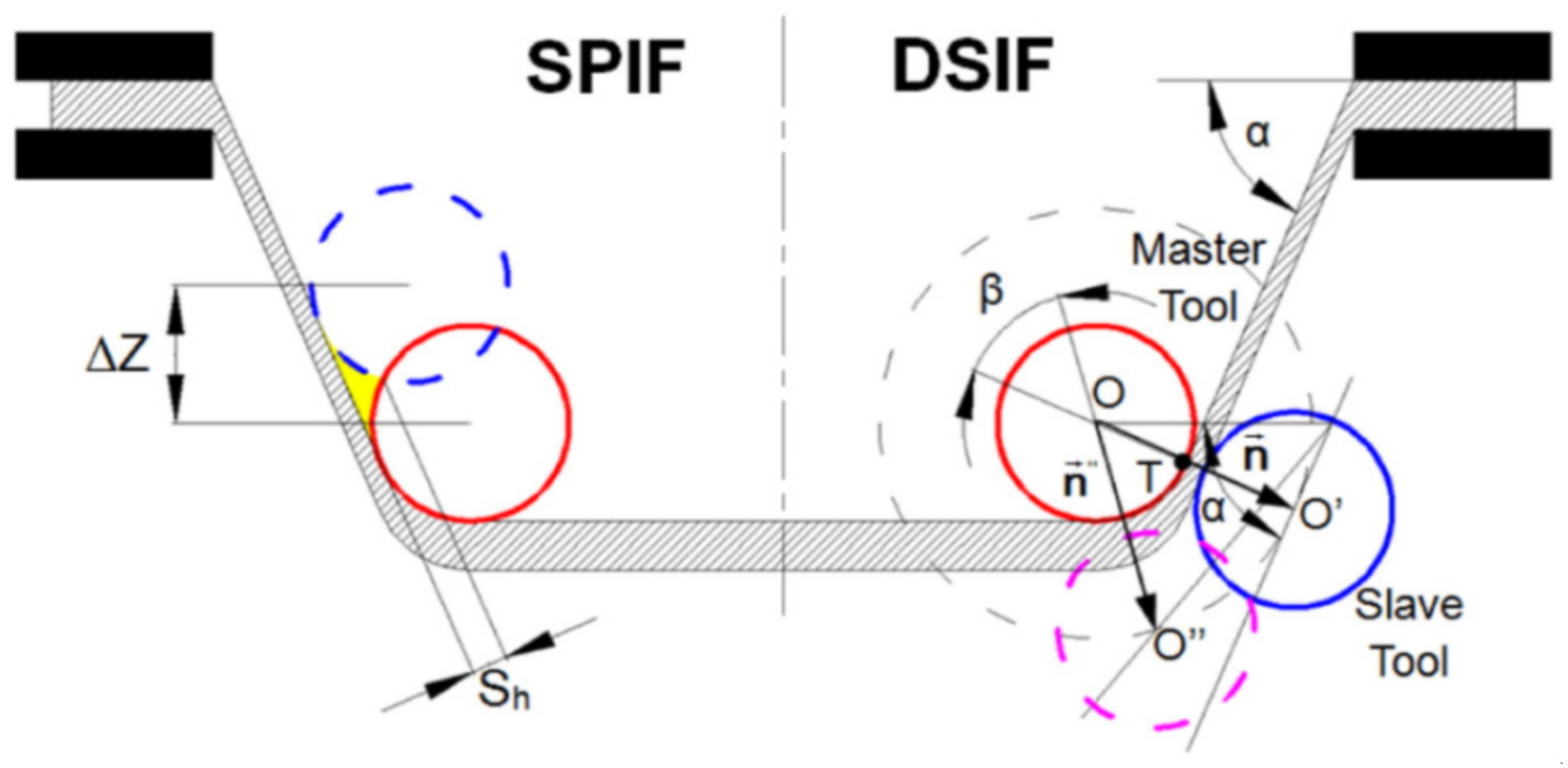
3. Incremental Sheet Forming Methods
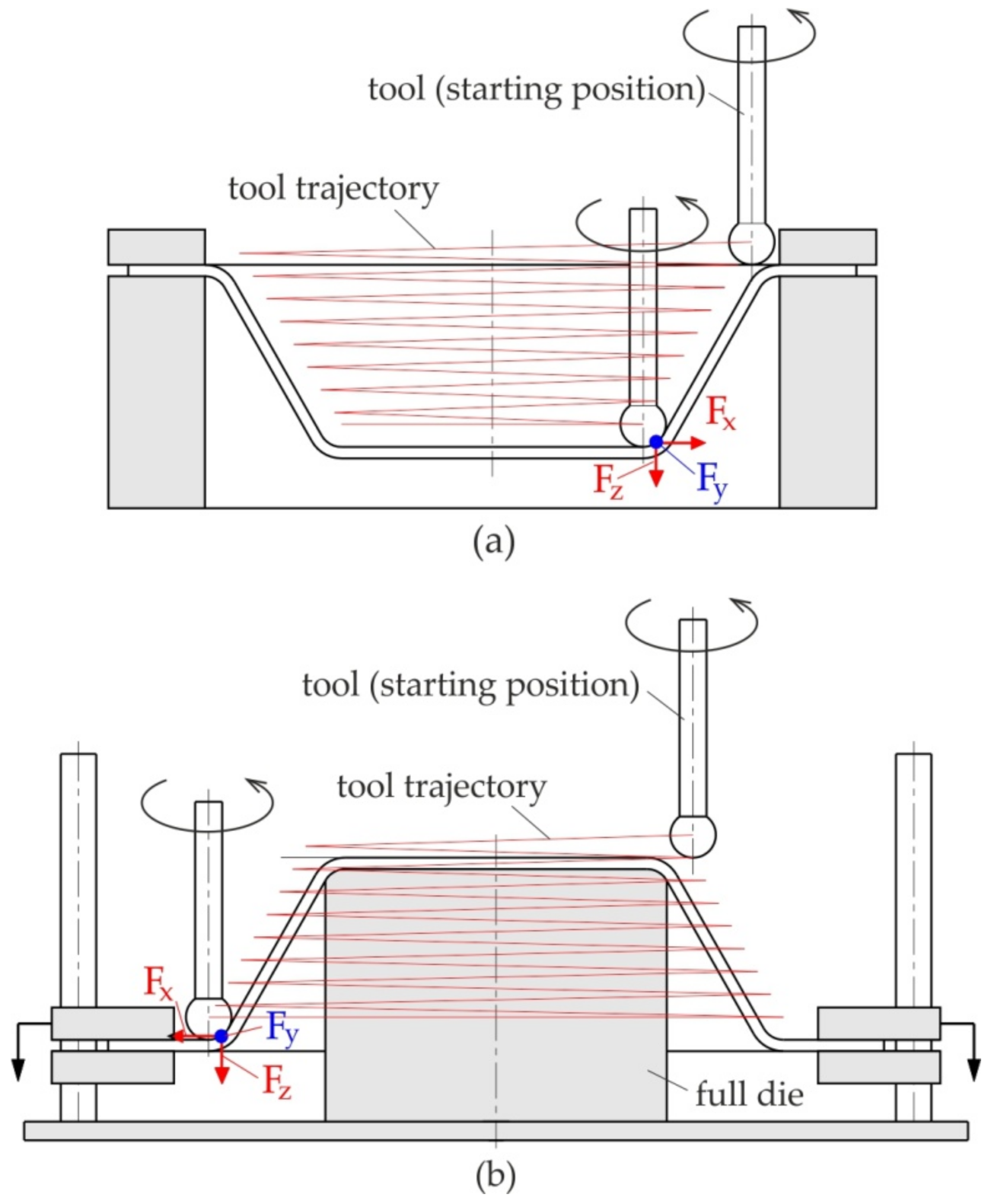
- Negative (Figure 2a), in which there is an additional element of the tool in the form of a template or a pin supporting the highest area of the detail being formed; forming takes place by appropriate mapping of the template geometry, by “arranging” the sheet on it, with a tool performing programmed movements,
- Positive (Figure 2b), in which the component is formed by the movement of the tool in accordance with the tool trajectory without the supporting template.
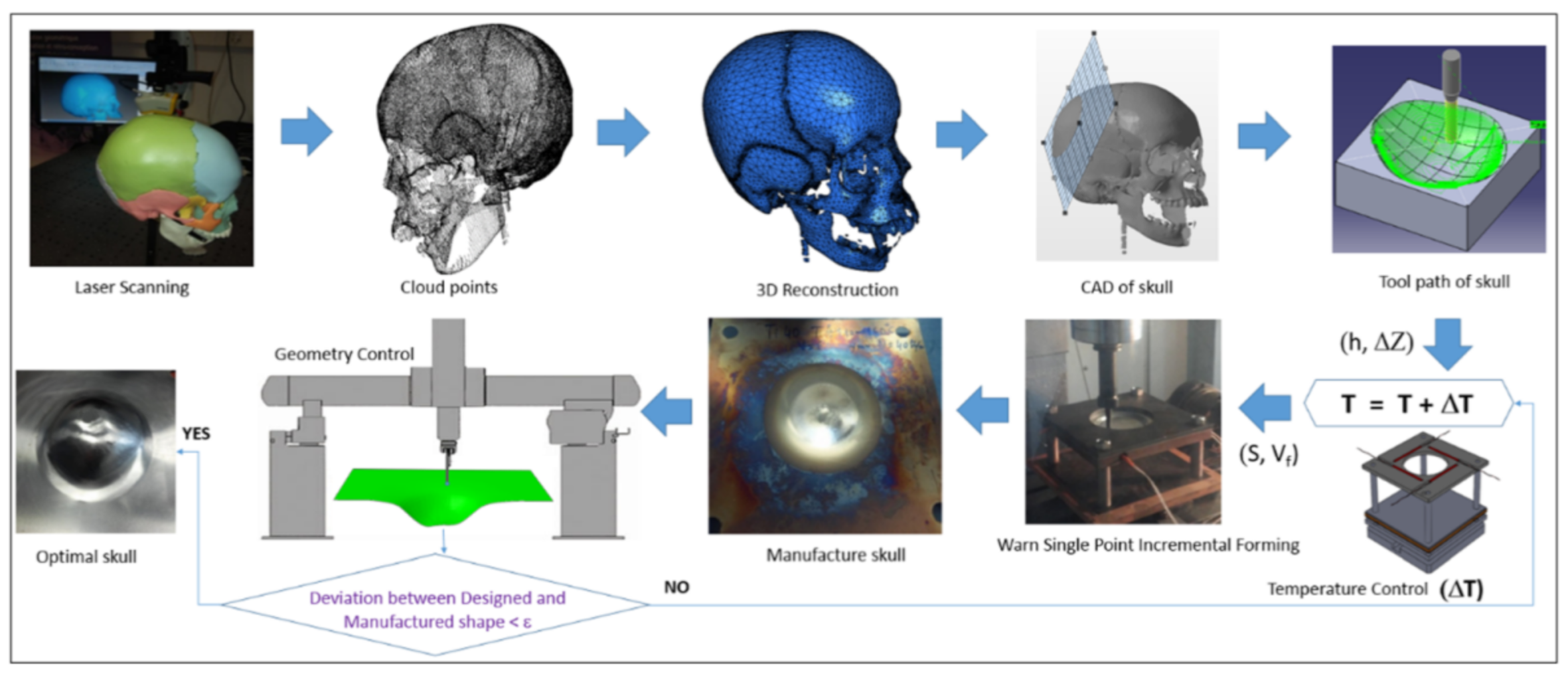
4. Incremental Sheet Forming towards Biomedical Applications
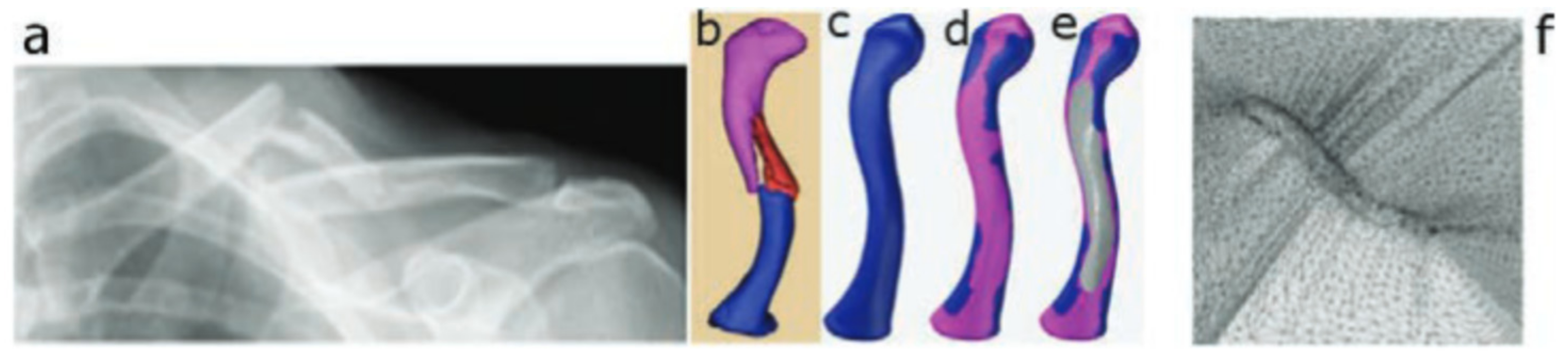
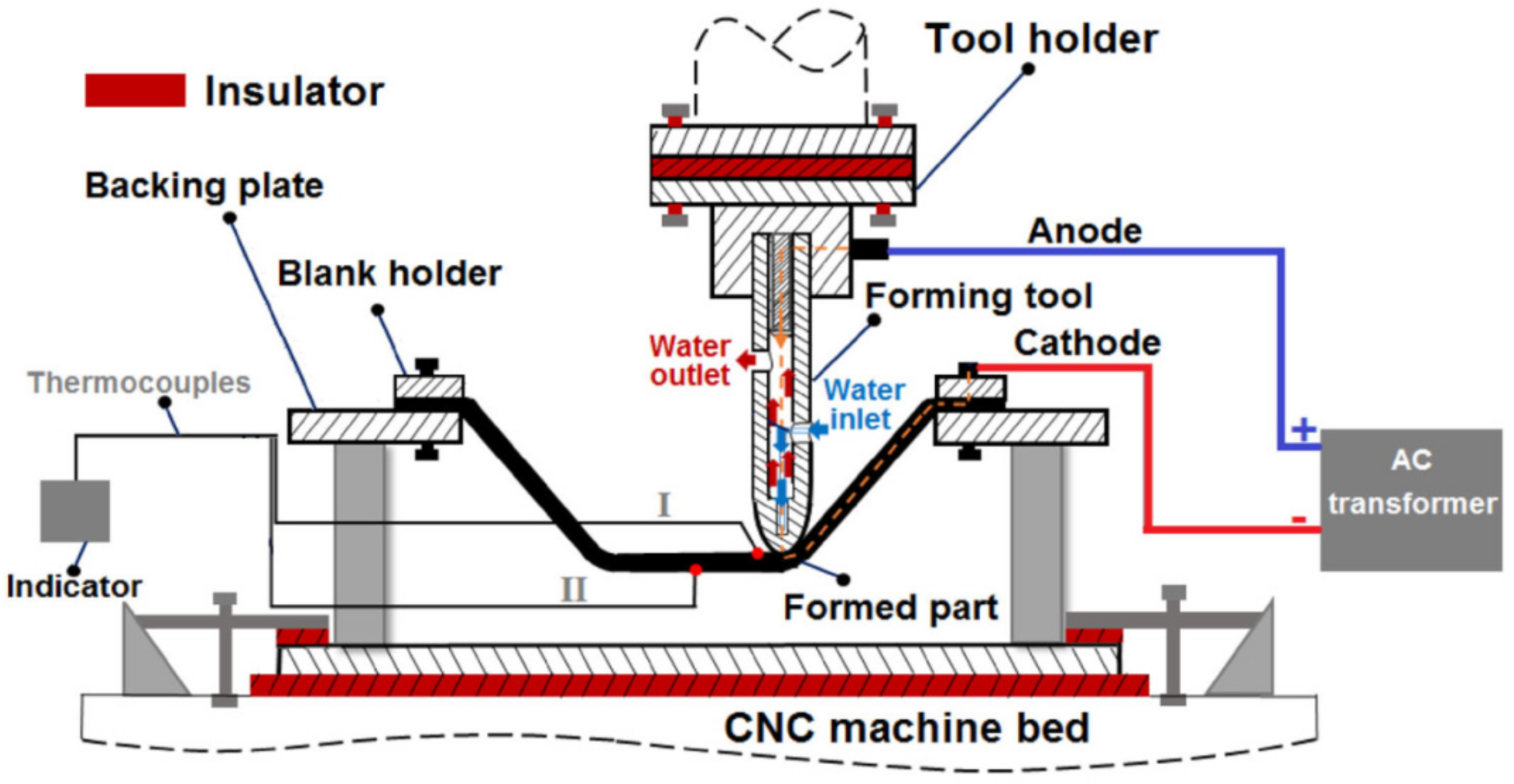
5. Thermally-Assisted Incremental Sheet Forming
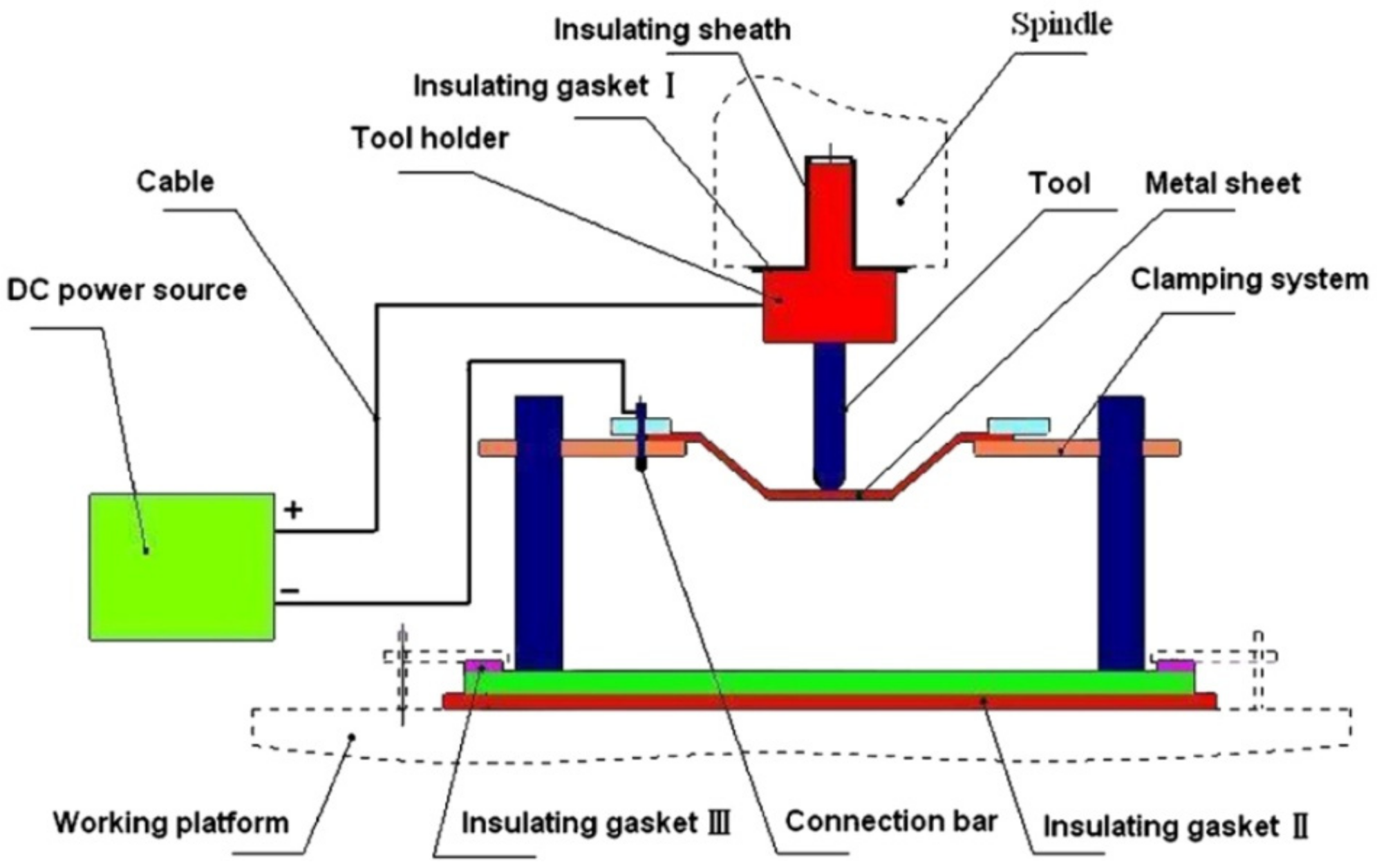
5.1. Electric Heating
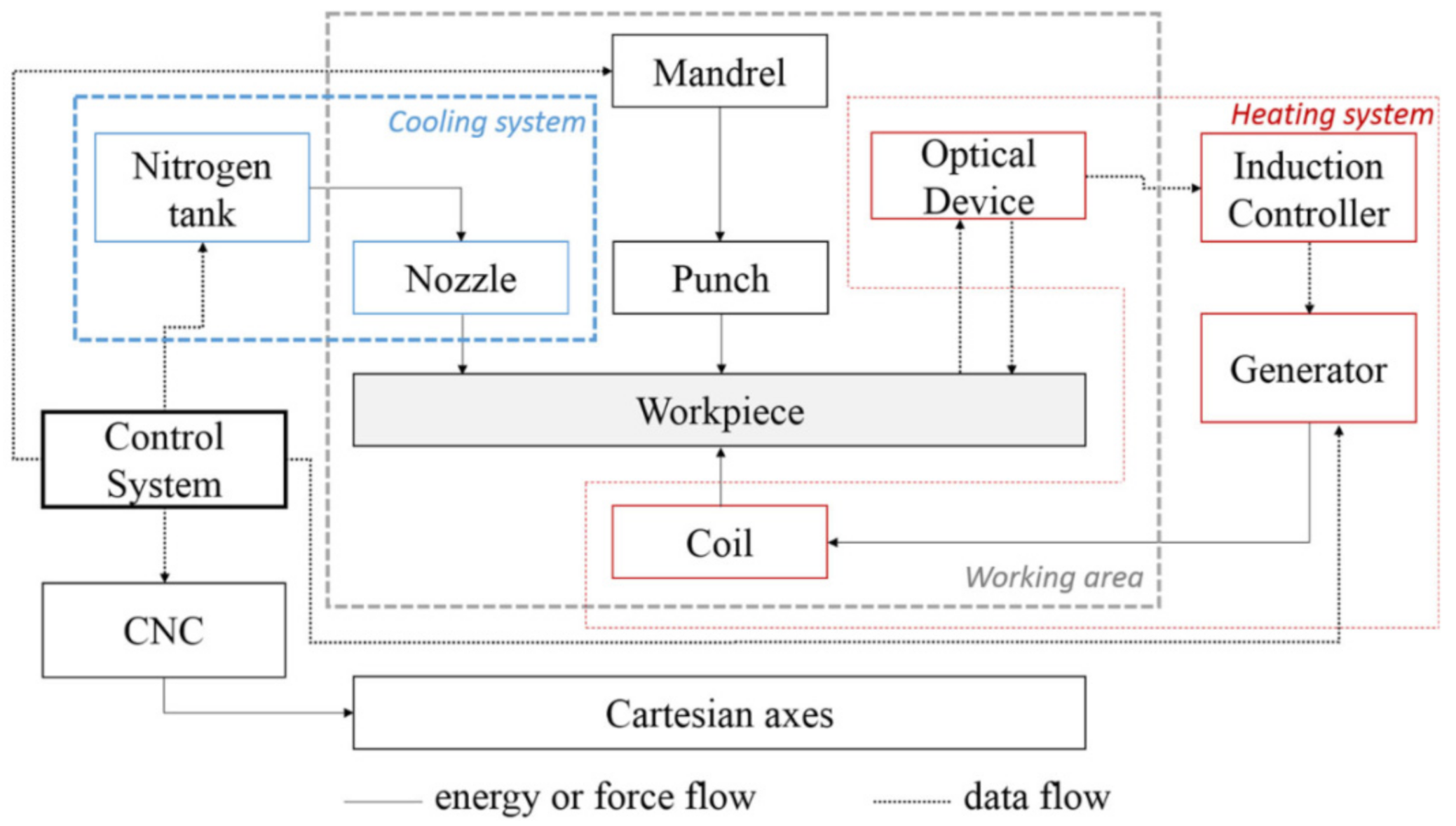
5.2. Induction Heating
5.3. Laser Heating
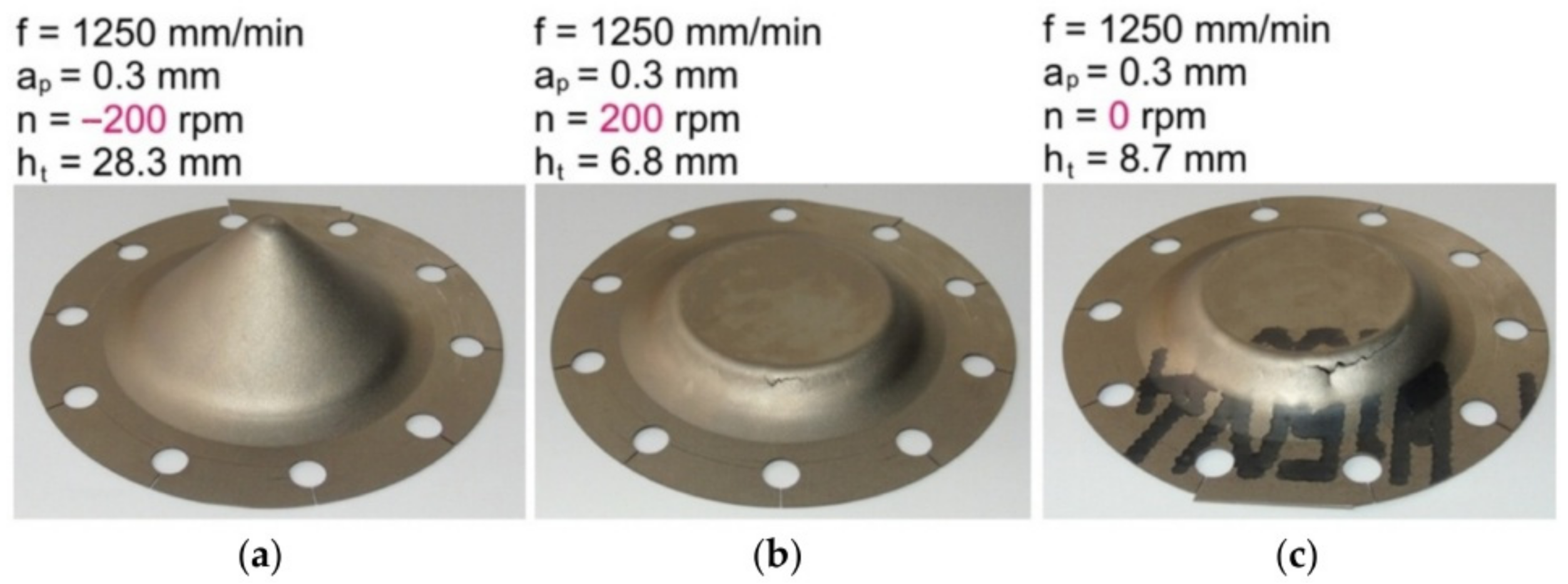
5.4. Friction Heating
5.5. Combined Electrical and Friction Heating
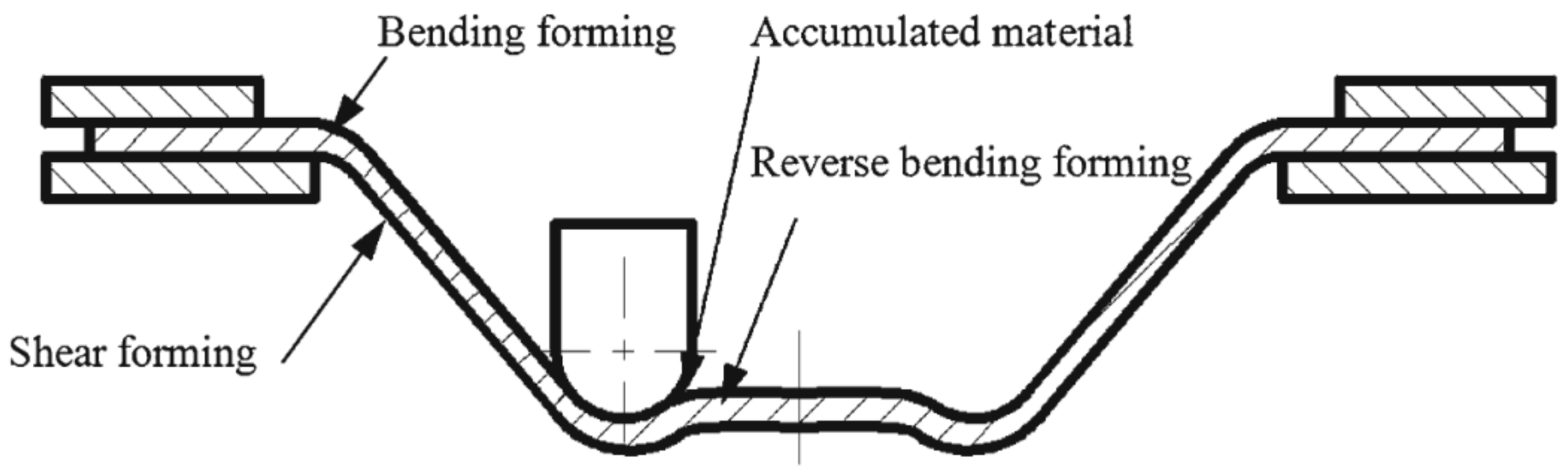
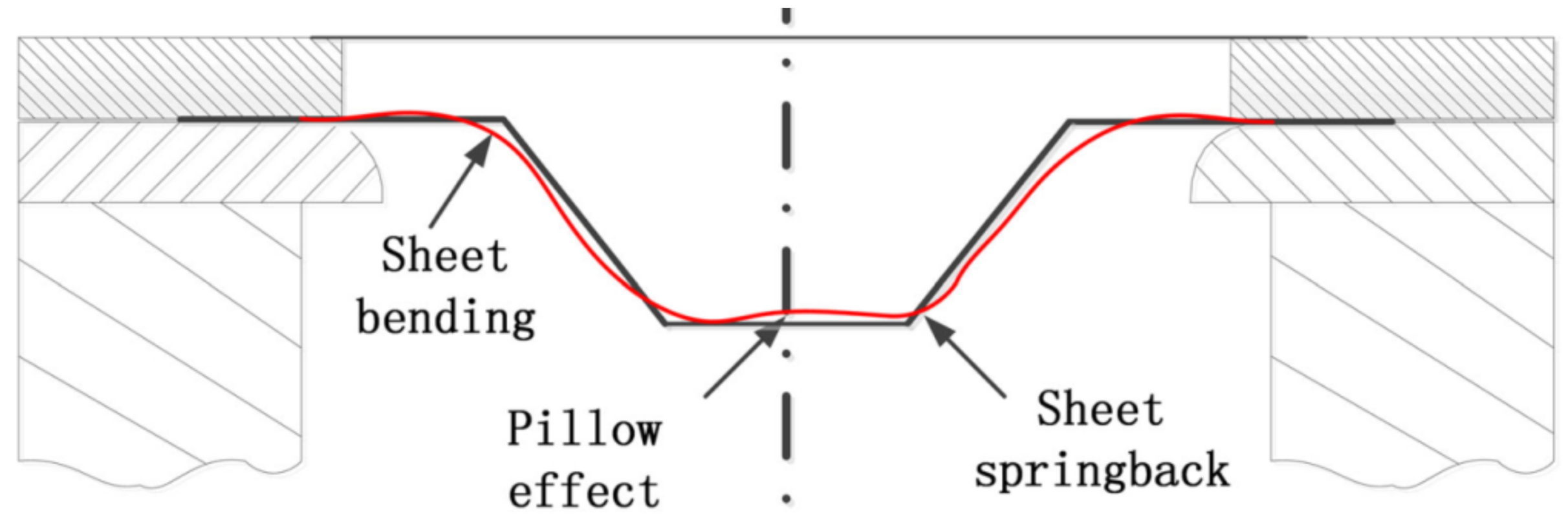
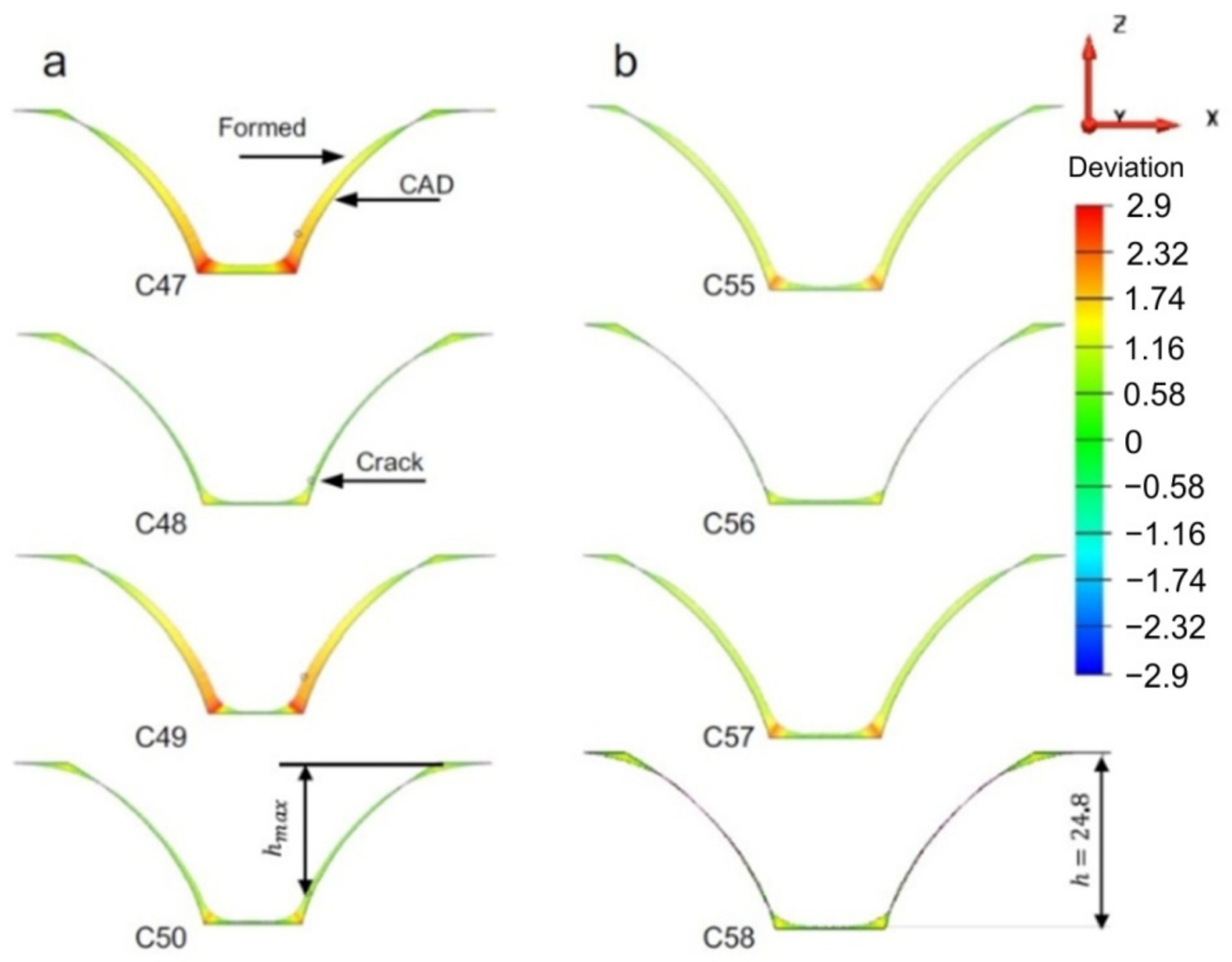
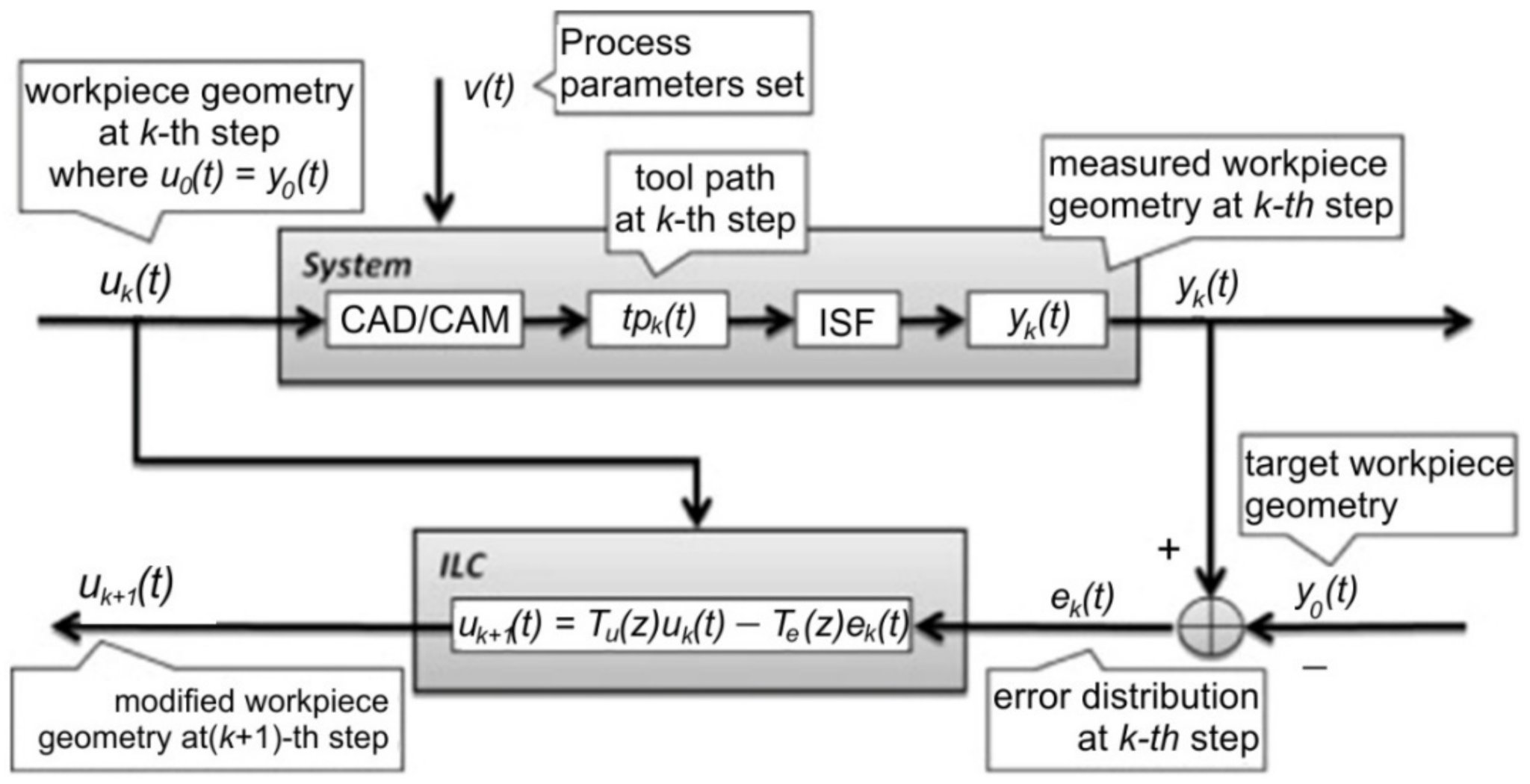
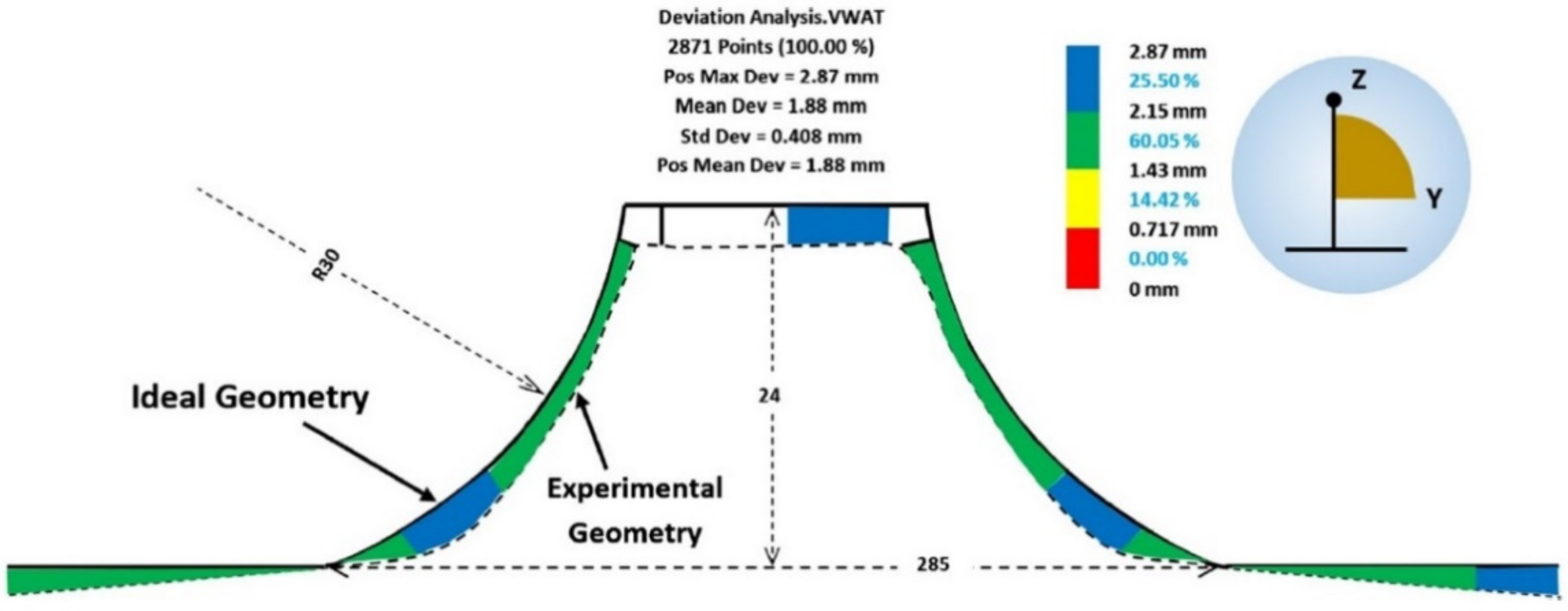
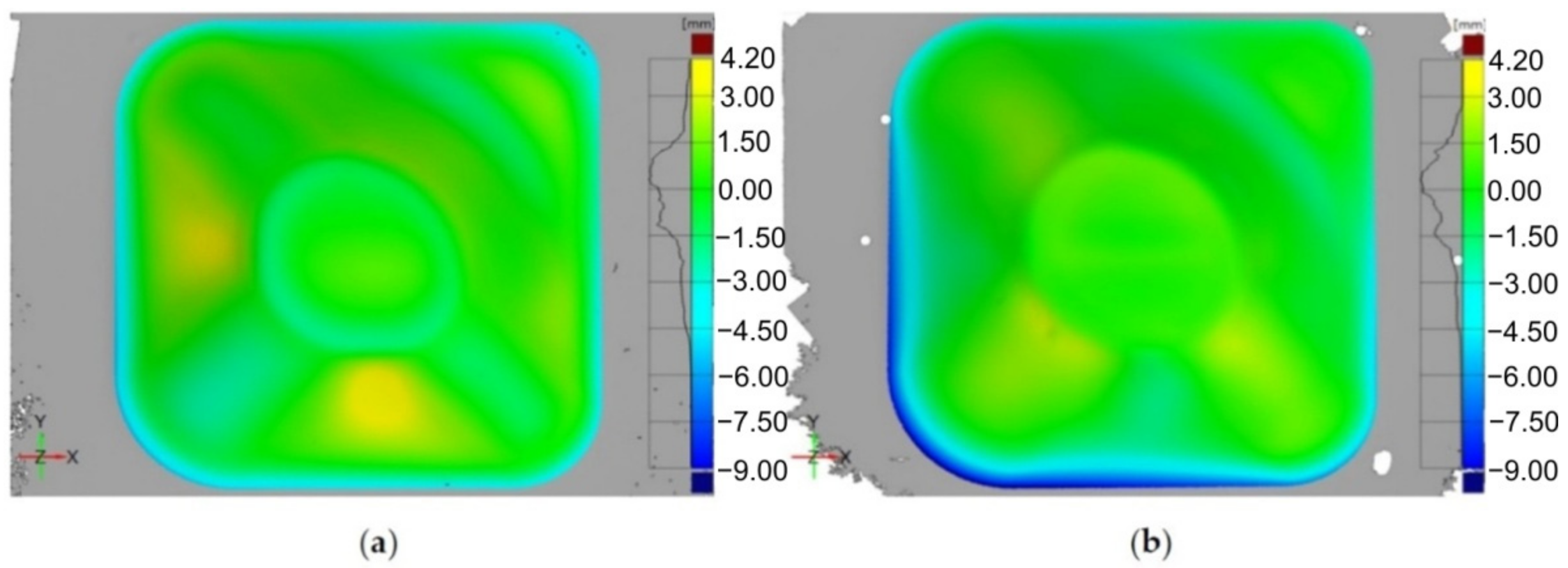
6. Accuracy, Springback Reduction and Toolpath Optimization
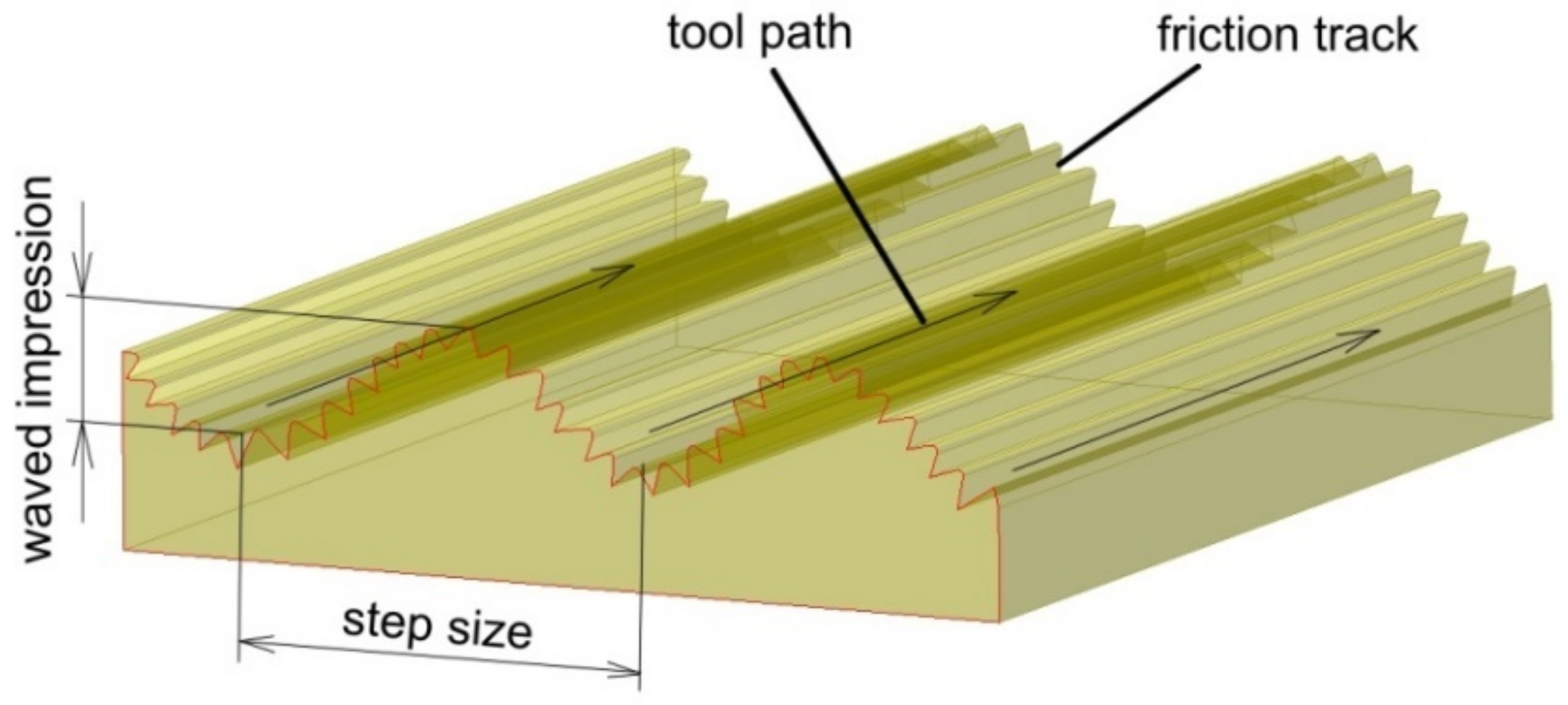
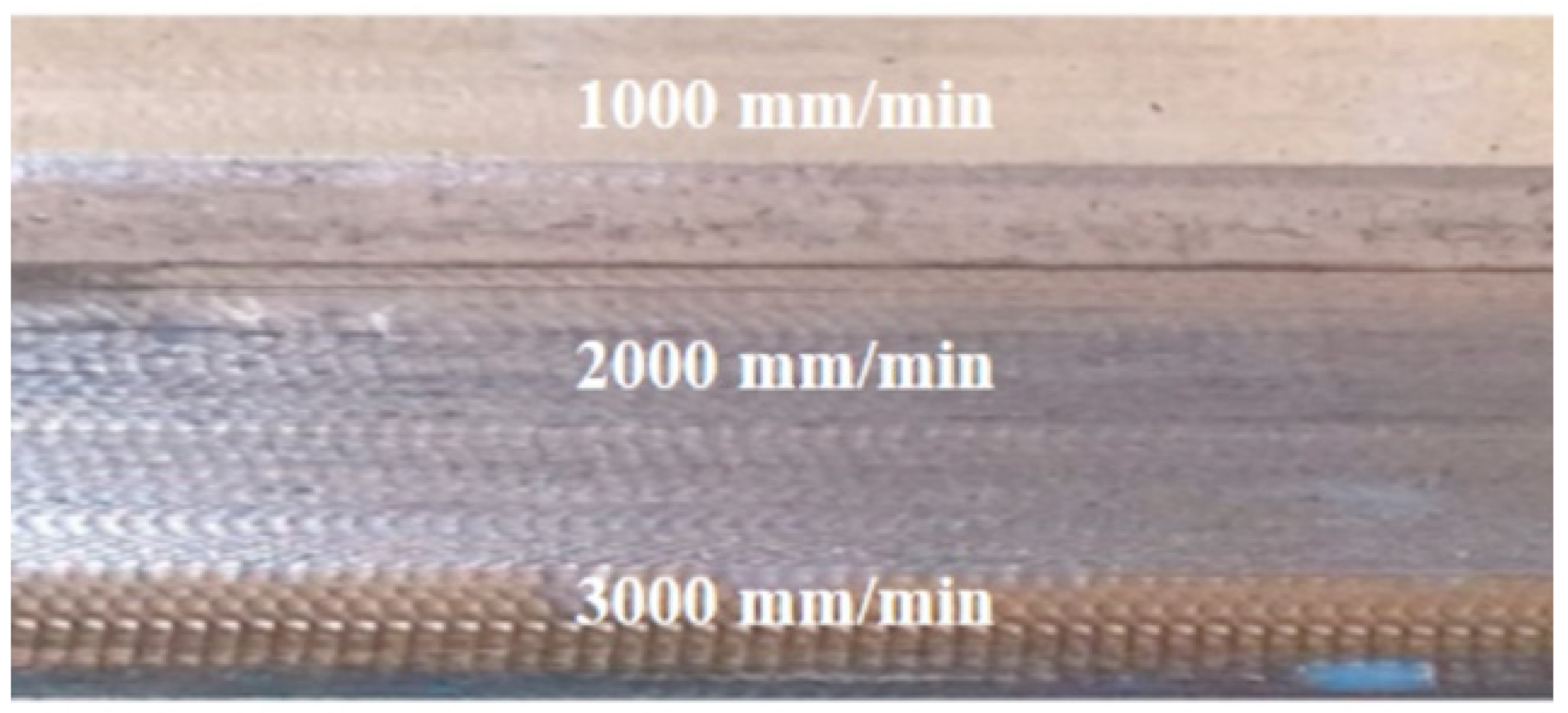
7. Surface Quality
7.1. Forming Strategy
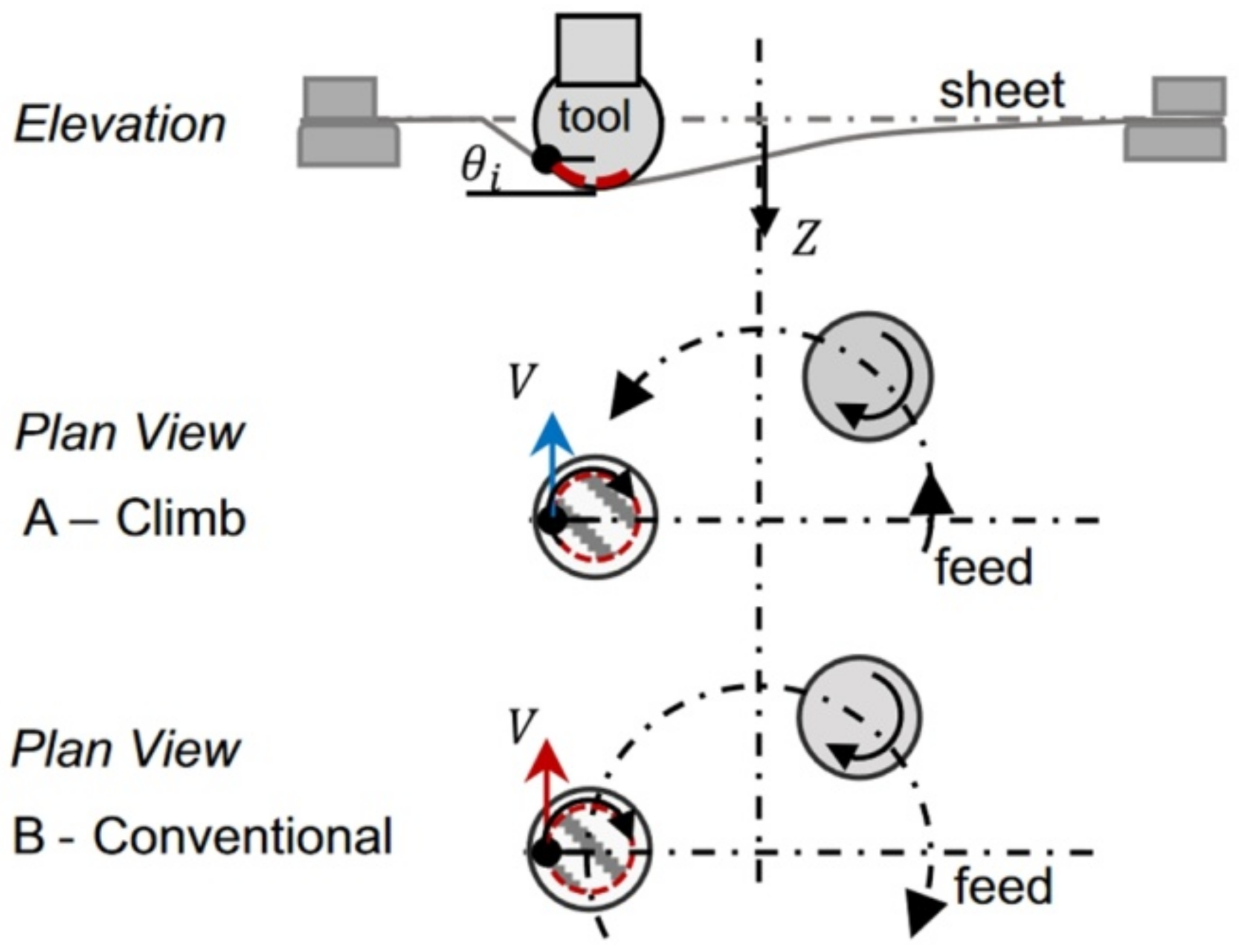
7.2. Friction Conditions
8. Numerical Modeling
9. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Bedinger, G.M. Titanium—Mining Engineering; USGS Publications Warehouse: Washington, DC, USA, 2013. [Google Scholar]
- Veiga, C.; Davim, J.P.; Loureiro, A. Review on machinability of titanium alloys: The process perspective. Rev. Adv. Mater. Sci. 2013, 34, 148–164. [Google Scholar]
- Boyer, R.R.; Briggs, R.D. The use of β titanium alloys in the aerospace industry. J. Mater. Eng. Perform. 2005, 14, 681–685. [Google Scholar] [CrossRef]
- Wang, Z.; Liu, L.; Zhang, L.; Sheng, J.; Wu, D.; Yuan, M. Effect of Heat Treatment on the Microstructure and Mechanical Properties of High-Strength Ti-6Al-4V-5Fe Alloy. Mater. Trans. 2019, 60, 269–276. [Google Scholar] [CrossRef] [Green Version]
- Brice, D.A.; Samimi, P.; Ghamarian, I.; Kiu, Y.; Brice, R.M.; Reidy, R.F.; Cotton, J.D.; Kaufman, M.J.; Collins, P.C. Oxidation behavior and microstructural decomposition of Ti-6Al-4V and Ti-6Al-4V-1B sheet. Corros. Sci. 2016, 112, 338–346. [Google Scholar] [CrossRef] [Green Version]
- Ma, K.; Zhang, R.; Sun, J.; Liu, C. Oxidation Mechanism of Biomedical Titanium Alloy Surface and Experiment. Int. J. Corros. 2020, 2020, 1678615. [Google Scholar] [CrossRef]
- Bocchetta, P.; Chen, L.-Y.; Tardelli, J.D.C.; Reis, A.C.D.; Almeraya-Calderón, F.; Leo, P. Passive Layers and Corrosion Resistance of Biomedical Ti-6Al-4V and β-Ti Alloys. Coatings 2021, 11, 487. [Google Scholar] [CrossRef]
- Aliofkhazrael, M.; Ali, N.; Chipara, M.; Laidani, N.B.; De Hosson, J.T.M. Handbook of Modern Coating Technologies. Fabrication Methods and Functional Properties; Elsevier: Cambridge, MA, USA, 2021. [Google Scholar]
- Wang, K.; Kopec, M.; Chang, S.; Qu, B.; Liu, J.; Politis, D.J.; Wang, L.; Liu, G. Enhanced formability and forming efficiency for two-phase titanium alloys by Fast light Alloys Stamping Technology (FAST). Mater. Des. 2020, 194, 108948. [Google Scholar] [CrossRef]
- Elias, C.N.; Meyers, M.A.; Valiev, R.Z.; Monteiro, S.N. Ultrafine grained titanium for biomedical applications: An overview of performance. J. Mater. Res. Technol. 2013, 2, 340–350. [Google Scholar] [CrossRef] [Green Version]
- Shahmir, H.; Langdon, T. An evaluation of the hexagonal close-packed to face-centered cubic phase transformation in a Ti-6Al-4V alloy during high-pressure torsion. Mater. Sci. Eng. A 2017, 704, 212–217. [Google Scholar] [CrossRef] [Green Version]
- Kolli, R.P.; Devaraj, A. A Review of Metastable Beta Titanium Alloys. Metals 2018, 8, 506. [Google Scholar] [CrossRef] [Green Version]
- Wood, J.R.; Russo, P.A.; Welter, M.F.; Crist, E.M. Thermomechanical processing and heat treatment of Ti–6Al–2Sn–2Zr–2Cr–2Mo–Si for structural application. Mater. Sci. Eng. A 1998, 243, 109–118. [Google Scholar] [CrossRef]
- Gloria, A.; Montanari, R.; Richetta, M.; Varone, A. Alloys for Aeronautic Applications: State of the Art and Perspectives. Metals 2019, 9, 662. [Google Scholar] [CrossRef] [Green Version]
- Oshida, Y. Materials Classification. In Bioscience and Bioengineering of Titanium Materials, 2nd ed.; Yoshida, Y., Ed.; Elsevier: Amsterdam, The Netherlands, 2013; pp. 9–34. [Google Scholar]
- Huang, S.; Zhao, Q.; Wu, C.; Lin, C.; Zhao, Y.; Jia, W.; Mao, C. Effects of β-stabilizer elements on microstructure formation and mechanical properties of titanium alloys. J. Alloys Compd. 2021, 867, 160085. [Google Scholar] [CrossRef]
- Bieler, T.R.; Trevino, R.M.; Zeng, L. Alloys: Titanium. Encyclopedia of Condensed Matter Physics; Elsevier: Amsterdam, The Netherlands, 2005; pp. 65–76. [Google Scholar]
- Sbayti, M.; Ghiotti, A.; Bahloul, R.; Belhadjsalah, H.; Bruschi, S. Finite Element Analysis of hot Single Point Incremental forming of hip prostheses. Matec. Web Conf. 2016, 80, 14006. [Google Scholar] [CrossRef] [Green Version]
- Inagaki, I.; Takechi, T.; Shirai, Y.; Ariyasu, N. Application and Features of Titanium for the Aerospace Industry. Nippon Steel Sumimoto Met. Tech. Rep. 2014, 106, 22–27. [Google Scholar]
- Kim, Y.W. Ordered intermetallic alloys: Part III: Gamma titanium aluminides. J. Miner. Met. Mater. Soc. 1994, 46, 30–39. [Google Scholar] [CrossRef]
- Liu, S.; Shin, C. Additive manufacturing of Ti6Al4V alloy: A review. Mater. Des. 2019, 164, 107552. [Google Scholar] [CrossRef]
- Huang, R.; Riddle, M.; Graziano, D.; Warren, J.; Das, S.; Nimbalkar, S.; Cresko, J.; Masanet, E. Energy and emissions saving potential of additive manufacturing: The case of lightweight aircraft components. J. Clean. Prod. 2016, 135, 1559–1570. [Google Scholar] [CrossRef] [Green Version]
- Sousa, R. Incremental Sheet Forming Technologies. Available online: https://doi.org/10.1016/B978-0-12-803581-8.04055-8 (accessed on 16 October 2021).
- Advantages & Disadvantages of Casting Process. Available online: https://bansengtrade.com/news/advantages-disadvantages-of-casting-process.html (accessed on 16 October 2021).
- Kumar, A.R.; Roy, S.; Joshi, K.K.; Sahoo, A.K.; Das, R.K. Machining of Ti-6Al-4V ELI alloy: A brief review. IOP Conf. Ser. Mater. Sci. Eng. 2018, 390, 012112. [Google Scholar]
- Oldenberger, E.L.; Oldenburg, M.; Thilderkvist, P.; Stoehr, T.; Lechler, J.; Merklein, M. Tool development based on modelling and simulation of hot sheet metal forming of Ti-6Al-4V titanium alloy. J. Mater. Process. Technol. 2011, 211, 1324–1335. [Google Scholar] [CrossRef]
- Abdelkader, W.B.; Bahloul, R.; Arfa, H. Numerical Investigation of the Influence of some Parameters in SPIF Process on the Forming Forces and Thickness Distributions of a Bimetallic Sheet CP-Titanium/Low-carbon Steel Compared to an Individual Layer. Procedia Manuf. 2020, 47, 1319–1327. [Google Scholar] [CrossRef]
- Hagan, E.; Jeswiet, J. A review of conventional and modern single-point sheet metal forming methods. Proc. Inst. Mech. Eng. Part B J. Eng. Manuf. 2003, 217, 213–225. [Google Scholar] [CrossRef]
- Milutinović, M.; Lendjel, R.; Baloš, S.; Zlatanović, D.L.; Sevšek, L.; Pepelnjak, T. Characterisation of geometrical and physical properties of a stainless steel denture framework manufactured by single-point incremental forming. J. Mater. Res. Technol. 2021, 10, 605–623. [Google Scholar] [CrossRef]
- Cristino, V.A.; Magrinho, J.P.; Centeno, G.; Silva, M.B.; Martins, P.A.F. Theory of single point incremental forming of tubes. J. Mater. Process. Technol. 2021, 287, 116659. [Google Scholar] [CrossRef]
- Najm, S.M.; Paniti, I. Experimental Investigation on the Single Point Incremental Forming of AlMn1Mg1 Foils using Flat End Tools. IOP Conf. Ser. Mater. Sci. Eng. 2018, 448, 012032. [Google Scholar] [CrossRef]
- Sbayti, M.; Bahloul, R.; Belhadjsalah, H.; Zemzemi, F. Optimization techniques applied to single point incremental forming process for biomedical application. Int. J. Adv. Manuf. Technol. 2018, 95, 1789–1804. [Google Scholar] [CrossRef]
- Trzepieciński, T.; Oleksik, V.; Pepelnjak, T.; Najm, S.M.; Paniti, I.; Maji, K. Emerging Trends in Single Point Incremental Sheet Forming of Lightweight Metals. Metals 2021, 11, 1188. [Google Scholar] [CrossRef]
- Trzepieciński, T. Recent developments and trends in sheet metal forming. Metals 2020, 10, 779. [Google Scholar] [CrossRef]
- Preferred Reporting Items for Systematic Reviews and Meta-Analyses. Available online: http://prisma-statement.org/ (accessed on 16 August 2021).
- Schreiber, R.G.; Schaeffer, L. Manufacture of absorber fins for solar collector using incremental sheet forming. J. Mater. Res. Technol. 2019, 8, 1132–1140. [Google Scholar] [CrossRef]
- Bastos, R.N.P.; de Sousa, R.J.A.; Ferreira, J.A.F. Enhancing time efficiency on single point incremental forming processes. Int. J. Mater. Form. 2016, 9, 653–662. [Google Scholar] [CrossRef]
- Wei, H.; Hussain, G.; Shi, X.; Isidore, B.B.L.; Alkahtani, M.; Abidi, M.H. Formability of Materials with Small Tools in Incremental Forming. Chin. J. Mech. Eng. 2020, 33, 55. [Google Scholar] [CrossRef]
- Tera, M.; Breaz, R.E.; Racz, S.G.; Girjob, C.E. Processing strategies for single point incremental forming—A CAM approach. Int. J. Adv. Manuf. Technol. 2019, 102, 1761–1777. [Google Scholar] [CrossRef]
- Najm, S.M.; Paniti, I. Artificial neural network for modeling and investigating the effects of forming tool characteristics on the accuracy and formability of thin aluminum alloy blanks when using SPIF. Int. J. Adv. Manuf. Technol. 2021, 114, 2591–2615. [Google Scholar] [CrossRef]
- Saotome, Y.; Okamoto, T. An in-situ incremental microforming system for three-dimensional shell structures of foil materials. J. Mater. Process. Tech. 2001, 113, 636–640. [Google Scholar] [CrossRef]
- Sekine, T.; Obikawa, T. Micro incremental forming characteristics of stainless foil. Key Eng. Mater. 2010, 447–448, 346–350. [Google Scholar] [CrossRef]
- Hapsari, G.; Hmida, R.B.; Richard, F.; Thibaud, S.; Malécot, P. A procedure for ductile damage parameters identification by micro incremental sheet forming. Procedia Eng. 2017, 183, 125–130. [Google Scholar] [CrossRef]
- Cédric, B.; Pierrick, M.; Sébastien, T. Shape Accuracy Improvement Obtained by μ-SPIF by Tool Path Compensation. Procedia Manuf. 2020, 47, 1399–1402. [Google Scholar] [CrossRef]
- Urtekin, L.; Bozkurt, F.; Çanli, M. Analysis of biomedical titanium implant green parts by X-ray tomography. J. Mater. Res. Technol. 2021, 14, 277–286. [Google Scholar] [CrossRef]
- Kurup, A.; Dhatrak, P.; Khasnis, N. Surface modification techniques of titanium and titanium alloys for biomedical dental applications: A review. Mater. Today Proc. 2021, 39, 84–90. [Google Scholar] [CrossRef]
- Jackson, M.J.; Kopac, J.; Balazic, M.; Bombac, D.; Brojan, M.; Kosel, F. Titanium and titanium alloy applications in medicine. In Surface Engineered Surgical Tools and Medical Devices; Jackson, M.J., Ahmed, W., Eds.; Springer: Boston, MA, USA, 2007; pp. 533–576. [Google Scholar]
- Yuan, Z.; He, Y.; Lin, C.; Liu, P.; Cai, K. Antibacterial surface design of biomedical titanium materials for orthopedic applications. J. Mater. Sci. Technol. 2021, 78, 51–67. [Google Scholar] [CrossRef]
- Balasubramanian, R.; Nagumothu, R.; Parfenov, E.; Valiev, R. Development of nanostructured titanium implants for biomedical implants—A short review. Mater. Today Proc. 2021, 46, 1195–1200. [Google Scholar] [CrossRef]
- Hwang, Y.-J.; Choi, Y.-S.; Hwang, Y.-H.; Cho, H.-W.; Lee, D.-G. Biocompatibility and Biological Corrosion Resistance of Ti–39Nb–6Zr+0.45Al Implant Alloy. J. Funct. Biomater. 2021, 12, 2. [Google Scholar] [CrossRef]
- Chen, L.-Y.; Cui, Y.-W.; Zhang, L.-C. Recent Development in Beta Titanium Alloys for Biomedical Applications. Metals 2020, 10, 1139. [Google Scholar] [CrossRef]
- Wang, G.; Li, J.; Lv, K.; Zhang, W.; Ding, X.; Yang, G.; Liu, X.; Jiang, X. Surface thermal oxidation on titanium implants to enhance osteogenic activity and in vivo osseointegration. Sci. Rep. 2016, 6, 31769. [Google Scholar] [CrossRef] [Green Version]
- Eremin, A.; Panin, S.; Byakov, A.; Sharkeev, Y. Mechanical behavior of course-grain and ultrafine-grain titanium for biomedical application. Procedia Struct. Integr. 2019, 23, 233–238. [Google Scholar] [CrossRef]
- Kim, J.; Lee, H.; Jang, T.-S.; Kim, D.; Yoon, C.-B.; Han, G.; Kim, H.-E.; Jung, H.-D. Characterization of Titanium Surface Modification Strategies for Osseointegration Enhancement. Metals 2021, 11, 618. [Google Scholar] [CrossRef]
- Gonsior, M.; Borowski, T.; Czarnowska, E.; Sanak, M.; Kustosz, R.; Ossowski, M.; Wierzchoń, T. Thrombogenicity of Ti(N,C,O) diffusive coating layers developer on titanium alloy as the blood contact surface. Eur. Cells Mater. 2010, 19, 12–17. [Google Scholar]
- Cai, D.; Zhao, X.; Yang, L.; Wang, R.; Qin, G.; Chen, D.F.; Zhang, E. A novel biomedical titanium alloy with high antibacterial property and low elastic modulus. J. Mater. Sci. Technol. 2021, 81, 13–25. [Google Scholar] [CrossRef]
- Castelan, J.; Schaeffer, L.; Daleffe, A.; Fritzen, D.; Salvaro, V.; da Silva, F.P. Manufacture of custom-made cranial implants from DICOM®images using 3D printing, CAD/CAM technology and incremental sheet forming. Eng. Biomed. 2014, 30, 265–273. [Google Scholar] [CrossRef] [Green Version]
- Petrovskaya, T.S.; Toropkov, N.E.; Mironov, E.G.; Azarmi, F. 3D printed biocompatible polylactide-hydroxyapatite based material for bone implants. Mater. Manuf. Process. 2018, 33, 1899–1904. [Google Scholar] [CrossRef]
- Bayirli, A.; Ide, S.; Orujalipour, I.; Demir, O.; Dursun, M.; Ates, H. Nanoscale structural investigation on Ti-6Al-4V implants produced by using selective laser melting technique: The importance of production angle. J. Mater. Res. Technol. 2019, 8, 2796–2801. [Google Scholar] [CrossRef]
- Berretta, S.; Evans, K.; Ghita, O. Additive manufacture of PEEK cranial implants: Manufacturing considerations versus accuracy and mechanical performance. Mater. Des. 2018, 139, 141–152. [Google Scholar] [CrossRef]
- Kim, Y.W. Surface modification of Ti dental implants by grit-blasting and micro-arc oxidation. Mater. Manuf. Process. 2010, 25, 307–310. [Google Scholar] [CrossRef]
- Correa, D.R.N.; Rocha, L.A.; Donato, T.A.G.; Sousa, K.S.J.; Grandini, C.R.; Afonso, C.R.M.; Doi, H.; Tsutsumi, Y.; Hanawa, T. On the mechanical biocompatibility of Ti-15Zr-based alloys for potential use as load-bearing implants. J. Mater. Res. Technol. 2020, 9, 1241–1250. [Google Scholar] [CrossRef]
- Wyte, M.; Nootens, S. Cast/forge a new cost effective approach to the manufacture of medical implants using cobalt–28 chromium–6 molybdenum alloy. In Proceedings of the Materials and Processes for Medical Devices Conference, Anaheim, CA, USA, 8–10 September 2003; ASM International: Novelty, OH, USA; pp. 320–323. [Google Scholar]
- Emmens, W.C.; Sebastiani, G.; van den Boogaard, A.H. The technology of incremental sheet forming—A brief review of the history. J. Mater. Process. Technol. 2010, 210, 981–997. [Google Scholar] [CrossRef] [Green Version]
- Jeswiet, J.; Micari, F.; Hirt, G.; Bramley, A.; Duflou, J.; Allwood, J. Asymmetric single point incremental forming of sheet metal. Ann. CIRP Manuf. Technol. 2005, 54, 623–649. [Google Scholar] [CrossRef]
- Duflou, J.R.; Behera, A.K.; Vanhove, H.; Bertol, L.S. Manufacture of accurate titanium cranio-facial implants with high forming angle using single point incremental forming. Key Eng. Mater. 2013, 549, 223–230. [Google Scholar] [CrossRef]
- Göttmann, A.; Korinth, M.; Schäfer, V.; Araghi, B.T.; Bambach, M.; Hirt, G. Manufacturing of individualized cranial implants usingtwo point incremental sheet metal forming. In Future Trends in Production Engineering; Schuh, G., Neugebauer, R., Uhlmann, E., Eds.; Springer: Berlin/Heidelberg, Germany, 2013; pp. 287–295. [Google Scholar]
- Lu, B.; Ou, H.; Shi, S.Q.; Long, H.; Chen, J. Titanium based cranial reconstruction using incremental sheet forming. Int. J. Mater. Form. 2016, 9, 361–370. [Google Scholar] [CrossRef] [Green Version]
- Lu, B.; Xu, D.K.; Liu, R.Z.; Ou, H.; Long, H.; Chen, J. Cranial reconstruction using double side incremental forming. Key Eng. Mater. 2015, 639, 535–542. [Google Scholar] [CrossRef] [Green Version]
- Eksteen, P.D.; Van der Merwe, A.F. Incremental sheet forming (ISF) in the manufacturing of titanium based plate implants in thebio-medical sector. In Proceedings of the International Conference on Computers & Industrial Engineering (CIE 42), Cape Town, South Africa, 16–18 July 2012; pp. 569–575. [Google Scholar]
- Araújo, R.; Teixeira, P.; Montanari, L.; Reis, A.; Silva, M.B.; Martins, P.A. Single point incremental forming of a facial implant. Prosthet. Orthot. Int. 2013, 38, 369–378. [Google Scholar] [CrossRef] [PubMed]
- Ambrogio, G.; De Napoli, L.; Filice, L.; Gagliardi, F.; Muzzupappa, M. Application of incremental forming process for high customized medical product manufacturing. J. Mater. Process. Technol. 2005, 162–163, 156–162. [Google Scholar] [CrossRef]
- Han, F.; Mo, J.H.; Wang, P.; Deng, Y.Z. A digital manufacture technology for skull prosthesis using incremental sheet forming method. Adv. Mater. Res. 2010, 102–104, 348–352. [Google Scholar] [CrossRef]
- Vanhove, H.; Carette, Y.; Vancleef, S.; Duflou, J.R. Production of thin shell clavicle implants through single point incremental forming. Procedia Eng. 2017, 183, 174–179. [Google Scholar] [CrossRef]
- Araújo, R.; Teixeira, P.; Silva, M.B.; Reis, A.; Martins, P.A.F. Single Point Incremental Forming of a Medical Implant. Key Eng. Mater. 2013, 544–557, 1388–1393. [Google Scholar] [CrossRef]
- Ambrogio, G.; Sgambitterra, E.; De Napoli, L.; Gagliardi, F.; Fragomeni, G.; Piccininni, A.; Gugleilmi, P.; Palumbo, G.; Sorgente, D.; La Barbera, L.; et al. Performances analysis of Titanium prostheses manufactured by Superplastic Forming and Incremental Forming. Procedia Eng. 2017, 183, 168–173. [Google Scholar] [CrossRef]
- Honarpisheh, M.; Abdolhoseini, M.J.; Amini, S. Experimental and numerical investigation of the hot incremental forming of Ti-6Al-4V sheet using electrical current. Int. J. Adv. Manuf. Technol. 2016, 83, 2027–2037. [Google Scholar] [CrossRef]
- Mohammadi, A.; Vanhove, H.; Van Bael, A.; Duflou, J.R. Towards accuracy improvement in single point incremental forming of shallow parts formed under laser assisted conditions. Int. J. Mater. Form. 2016, 9, 339–351. [Google Scholar] [CrossRef]
- Saidi, B.; Giraud Moreau, L.; Mhemed, S.; Cheroaut, A.; Adragna, P.A.; Nasri, R. Hot incremental forming of titanium human skull prosthesis by using cartridge heaters: A reverse engineering approach. Int. J. Adv. Manuf. Technol. 2019, 101, 873–880. [Google Scholar] [CrossRef]
- Palumbo, G.; Piccininni, A.; Ambrogio, G.; Sgambitterra, E. Design of custom cranial prostheses combining manufacturing and drop test finite element simulations. Int. J. Adv. Manuf. Technol. 2020, 111, 1627–1641. [Google Scholar] [CrossRef]
- Oleksik, V.; Pascu, A.; Deac, C.; Fleacă, R.; Bologa, O.; Racz, G. Experimental study on the surface quality of the medical implants obtained by single point incremental forming. Int. J. Mater. Form. 2010, 3, 935–938. [Google Scholar] [CrossRef]
- Donachie, M.J. Titanium: A Technical Guide; ASM International: Materials Park, OH, USA, 2000. [Google Scholar]
- Fan, G.; Sun, F.; Meng, X.; Gao, L.; Tong, G. Electric Hot Incremental Forming of Ti-6Al-4V Titanium Sheet. Int. J. Adv. Manuf. Technol. 2010, 49, 941–947. [Google Scholar] [CrossRef]
- Liu, Z. Heat-Assisted Incremental Sheet Forming: A State-of-the-Art Review. Int. J. Adv. Manuf. Technol. 2018, 98, 2987–3003. [Google Scholar] [CrossRef]
- Sbayti, M.; Bahloul, R.; Belhadjsalah, H. Simulation of the local heating effect on incremental sheet forming process. In Proceedings of the Advances in Mechanical Engineering, Materials and Mechanics, Hammamet, Tunisia, 16–18 December 2019; Kharrat, M., Baccar, M., Dammak, F., Eds.; Springer International Publishing: Cham, Switzerland, 2021; pp. 144–151. [Google Scholar]
- Ambrogio, G.; Gagliardi, F.; Chamanfar, A.; Misiolek, W.Z.; Filice, L. Induction Heating and Cryogenic Cooling in Single Point Incremental Forming of Ti-6Al-4V: Process Setup and Evolution of Microstructure and Mechanical Properties. Int. J. Adv. Manuf. Technol. 2017, 91, 803–812. [Google Scholar] [CrossRef]
- Göttmann, A.; Diettrich, J.; Bergweiler, G.; Bambach, M.; Hirt, G.; Loosen, P.; Poprawe, R. Laser-Assisted Asymmetric Incremental Sheet Forming of Titanium Sheet Metal Parts. Prod. Eng. Res. Dev. 2011, 5, 263–271. [Google Scholar] [CrossRef]
- Göttmann, A.; Bailly, D.; Bergweiler, G.; Bambach, M.; Stollenwerk, J.; Hirt, G.; Loosen, P. A Novel Approach for Temperature Control in ISF Supported by Laser and Resistance Heating. Int. J. Adv. Manuf. Technol. 2013, 67, 2195–2205. [Google Scholar] [CrossRef]
- Mosecker, L.; Göttmann, A.; Saeed-Akbari, A.; Bleck, W.; Bambach, M.; Hirt, G. Deformation Mechanisms of Ti6Al4V Sheet Material during the Incremental Sheet Forming with Laser Heating. Key Eng. Mater. 2013, 549, 372–380. [Google Scholar] [CrossRef]
- Ji, Y.H.; Park, J.J. Formability of magnesium AZ31 sheet in the incremental forming at warm temperature. J. Mater. Proc. Technol. 2008, 201, 354–358. [Google Scholar] [CrossRef]
- Gagliardi, F.; Ambrogio, G.; Filice, L. Incremental Forming with Local Induction Heating on Materials with Magnetic and Non-Magnetic Properties. Procedia Eng. 2017, 183, 143–148. [Google Scholar]
- Fan, G.; Gao, L.; Hussain, G.; Wu, Z. Electric hot incremental forming: A novel technique. Int. J. Mach. Tools Manuf. 2008, 48, 1688–1692. [Google Scholar] [CrossRef]
- Fan, G.; Gao, L. Numerical Simulation and Experimental Investigation to Improve the Dimensional Accuracy in Electric Hot Incremental Forming of Ti-6Al-4V Titanium Sheet. Int. J. Adv. Manuf. Technol. 2014, 72, 1133–1141. [Google Scholar] [CrossRef]
- Ambrogio, G.; Gagliardi, F.; Bruschi, S.; Filice, L. On the high-speed Single Point Incremental Forming of titanium alloys. Cirp. Ann.-Manuf. Technol. 2013, 62, 243–246. [Google Scholar] [CrossRef]
- Grün, P.A.; Uheida, E.H.; Lachmann, L.; Dimitrov, D.; Oosthuizen, G.A. Formability of Titanium Alloy Sheets by Friction Stir Incremental Forming. Int. J. Adv. Manuf. Technol. 2018, 99, 1993–2003. [Google Scholar] [CrossRef] [Green Version]
- Uheida, E.H.; Oosthuizen, G.A.; Dimitrov, D.M.; Bezuidenhout, M.B.; Hugo, P.A. Effects of the relative tool rotation direction on formability during the incremental forming of titanium sheets. Int. J. Adv. Manuf. Technol. 2018, 96, 3311–3319. [Google Scholar] [CrossRef] [Green Version]
- Ao, D.; Chu, X.; Gao, J.; Yang, Y.; Lin, S. Experimental Investigation on the Deformation Behaviors of Ti-6Al-4V Sheet in Electropulsing-Assisted Incremental Forming. Int. J. Adv. Manuf. Technol. 2019, 104, 4243–4254. [Google Scholar] [CrossRef]
- Ao, D.; Gao, J.; Chu, X.; Lin, S.; Lin, J. Formability and Deformation Mechanism of Ti-6Al-4V Sheet under Electropulsing Assisted Incremental Forming. Int. J. Solids Struct. 2020, 202, 357–367. [Google Scholar] [CrossRef]
- Asgar, J.; Lingam, R.; Reddy, V.N. Tool Path Influence on Electric Pulse Aided Deformation during Incremental Sheet Metal Forming. AIP Conf. Proc. 2013, 1567, 840–843. [Google Scholar]
- Fan, G.; Gao, L. Mechanical Property of Ti-6Al-4V Sheet in One-Sided Electric Hot Incremental Forming. Int. J. Adv. Manuf. Technol. 2014, 72, 989–994. [Google Scholar] [CrossRef]
- Liu, R.; Lu, B.; Xu, D.; Chen, J.; Chen, F.; Ou, H.; Long, H. Development of Novel Tools for Electricity-Assisted Incremental Sheet Forming of Titanium Alloy. Int. J. Adv. Manuf. Technol. 2016, 85, 1137–1144. [Google Scholar] [CrossRef]
- Najafabady, S.A.; Ghaei, A. An experimental study on dimensional accuracy, surface quality, and hardness of Ti-6Al-4 V titanium alloy sheet in hot incremental forming. Int. J. Adv. Manuf. Technol. 2016, 87, 3579–3588. [Google Scholar] [CrossRef]
- Xu, D.K.; Lu, B.; Cao, T.T.; Zhang, H.; Chen, J.; Long, H.; Cao, J. Enhancement of Process Capabilities in Electrically-Assisted Double Sided Incremental Forming. Mater. Des. 2016, 92, 268–280. [Google Scholar] [CrossRef]
- Ortiz, M.; Penalva, M.; Puerto, M.J.; Homola, P.; Kafka, V. Hot Single Point Incremental Forming of Ti-6Al-4V Alloy. Key Eng. Mater. 2014, 611–612, 1079–1087. [Google Scholar] [CrossRef]
- Ortiz, M.; Penalva, M.; Iriondo, E.; de Lacalle, L.N.L. Accuracy and Surface Quality Improvements in the Manufacturing of Ti-6Al-4V Parts Using Hot Single Point Incremental Forming. Metals 2019, 9, 697. [Google Scholar] [CrossRef] [Green Version]
- Khazaali, H.; Fereshteh-Saniee, F. Application of the Taguchi Method for Efficient Studying of Elevated-Temperature Incremental Forming of a Titanium Alloy. J. Braz. Soc. Mech. Sci. Eng. 2018, 40, 43. [Google Scholar] [CrossRef]
- Khazaali, H.; Fereshteh-Saniee, F. Process Parameter Enhancement for Incremental Forming of Titanium Ti-6Al-4V Truncated Cone with Varying Wall Angle at Elevated Temperatures. Int. J. Precis. Eng. Manuf. 2019, 20, 769–776. [Google Scholar] [CrossRef]
- Mohanraj, R.; Elangovan, S. Thermal Modeling and Experimental Investigation on the Influences of the Process Parameters on Warm Incremental Sheet Metal Forming of Titanium Grade 2 Using Electric Heating Technique. Int. J. Adv. Manuf. Technol. 2020, 110, 255–274. [Google Scholar] [CrossRef]
- Palumbo, G.; Brandizzi, M. Experimental Investigations on the Single Point Incremental Forming of a Titanium Alloy Component Combining Static Heating with High Tool Rotation Speed. Mater. Des. 2012, 40, 43–51. [Google Scholar] [CrossRef]
- Khazaali, H.; Fereshteh-Saniee, F. A Comprehensive Experimental Investigation on the Influences of the Process Variables on Warm Incremental Forming of Ti-6Al-4V Titanium Alloy Using a Simple Technique. Int. J. Adv. Manuf. Technol. 2016, 87, 2911–2923. [Google Scholar] [CrossRef]
- Palumbo, G.; Brandizzi, M.; Cervelli, G.; Fracchiolla, M. Investigations about the Single Point Incremental Forming of anisotropic Titanium alloy sheets. Adv. Mater. Res. 2011, 164–264, 188–193. [Google Scholar] [CrossRef]
- Sbayti, M.; Bahloul, R.; Belhadjsalah, H. Efficiency of optimization algorithms on the adjustment of process parameters for geometric accuracy enhancement of denture plate in single point incremental sheet forming. Neural. Comput. Appl. 2020, 32, 8829–8846. [Google Scholar] [CrossRef]
- Micari, F.; Ambrogio, G.; Filice, L. Shape and dimensional accuracy in single point incremental forming: State of the art and future trends. J. Mater. Process. Technol. 2007, 191, 390–395. [Google Scholar] [CrossRef]
- Hussain, G.; Gao, L.; Hayat, N.; Cui, Z.; Pang, Y.C.; Dar, N.U. Tool and lubrication for negative incremental forming of a commercially pure titanium sheet. J. Mater. Process. Technol. 2008, 203, 193–201. [Google Scholar] [CrossRef]
- Valoppi, B.; Egea, A.J.S.; Zhang, Z.X.; Rojas, H.A.G.; Ghiotti, A.; Bruschi, S.; Cao, J. A hybrid mixed double-sided incremental forming method for forming Ti6Al4V alloy. CIRP Ann.-Manuf. Technol. 2016, 65, 309–312. [Google Scholar] [CrossRef] [Green Version]
- Saidi, B.; Moreau, L.G.; Cherouat, A.; Nasri, R. Experimental and numerical study on warm single-point incremental sheet forming (WSPIF) of titanium alloy Ti-6Al-4V, using cartridge heaters. J. Braz. Soc. Mech. Sci. 2020, 42, 534. [Google Scholar] [CrossRef]
- Behera, A.K.; Ou, H. Effect of stress relieving heat treatment on surface topography and dimensional accuracy of incrementally formed grade 1 titanium sheet parts. Int. J. Adv. Manuf. Technol. 2016, 87, 3233–3248. [Google Scholar] [CrossRef] [Green Version]
- Racz, S.G.; Breaz, R.E.; Tera, M.; Girjob, C.; Biris, C.; Chicea, A.L.; Bologa, O. Incremental Forming of Titanium Ti6Al4V Alloy for Cranioplasty Plates-Decision-Making Process and Technological Approaches. Metals 2018, 8, 626. [Google Scholar] [CrossRef] [Green Version]
- Behera, A.K.; Lu, B.; Ou, H. Characterization of shape and dimensional accuracy of incrementally formed titanium sheet parts with intermediate curvatures between two feature types. Int. J. Adv. Manuf. Technol. 2016, 83, 1099–1111. [Google Scholar] [CrossRef] [Green Version]
- Fiorentino, A.; Feriti, G.C.; Ceretti, E.; Giardini, C. Capability of iterative learning control and influence of the material properties on the improvement of the geometrical accuracy in incremental sheet forming process. Int. J. Mater. Form. 2018, 11, 125–134. [Google Scholar] [CrossRef]
- Li, F.Q.; Mo, J.H. Springback analysis of TC4 titanium alloy complex part with double curvature under single point incremental forming process. Adv. Mater. Res. 2012, 468–471, 1094–1098. [Google Scholar] [CrossRef]
- Grimm, T.J.; Roth, J.T.; Ragai, I. Electrically assisted global springback elimination in AMS-T-9046 titanium after single point incremental forming. In Proceedings of the ASME 2016 11th International Manufacturing Science and Engineering Conference, Backsburg, VA, USA, 27 June–1 July 2016; pp. 1–12. [Google Scholar]
- Li, X.; Han, K.; Song, X.; Wang, H.; Li, D.; Li, Y.; Li, Q. Experimental and numerical investigation on surface quality for two-point incremental sheet forming with interpolator. Chin. J. Aeronaut. 2020, 33, 2794–2806. [Google Scholar] [CrossRef]
- Liu, Z.; Liu, S.; Li, Y.; Meehan, P.A. Modeling and optimization of surface roughness in incremental sheet forming using a multi-objective function. Mater. Manuf. Process. 2014, 29, 808–818. [Google Scholar] [CrossRef]
- Radu, C.; Cristea, I.; Herghelegiu, E.; Tabacu, S. Improving the accuracy of parts manufactured by single point incremental forming. Appl. Mech. Mater. 2013, 332, 443–448. [Google Scholar] [CrossRef]
- Bahloul, R.; Arfa, H.; Belhajsalah, H. Application of response surface analysis and genetic algorithm for the optimization of single point incremental forming process. Key Eng. Mater. 2013, 554–557, 1265–1272. [Google Scholar] [CrossRef]
- Yao, Z.; Li, Y.; Yang, M.; Yuan, Q.; Shi, P. Parameter optimization for deformation energy and forming quality in single point incremental forming process using response surface methodology. Adv. Mech. Eng. 2017, 9, 1–15. [Google Scholar] [CrossRef]
- Behera, A.; Lauwers, B.; Duflou, J. Tool path generation for single point incremental forming using intelligent sequencing and multi-step mesh morphing techniques. Int. J. Mater. Form. 2015, 8, 517–532. [Google Scholar] [CrossRef]
- Fu, Z.; Mo, J.; Han, F.; Gong, P. Tool path correction algorithm for single-point incremental forming of sheet metal. Int. J. Adv. Manuf. Technol. 2013, 64, 1239–1248. [Google Scholar] [CrossRef]
- Veeraajay, C. Parameter Optimization in Incremental Forming of Titanium Alloy Material. Trans. Indian Inst. Met. 2020, 73, 2403–2413. [Google Scholar]
- Szpunar, M.; Ostrowski, R.; Trzepieciński, T.; Kaščák, L. Central Composite Design Optimisation in Single Point Incremental Forming of Truncated Cones from Commercially Pure Titanium Grade 2 Sheet Metals. Metals 2021, 14, 3634. [Google Scholar]
- Peter, I.; Fracchia, E.; Canale, I.; Maiorano, R. Incremental sheet forming for prototyping automotive modules. Procedia Manuf. 2019, 32, 50–58. [Google Scholar] [CrossRef]
- Mohanraj, R.; Elangovan, S. Incremental sheet metal forming of Ti-6Al-4V alloy for aerospace application. Trans. Can. Soc. Mech. Eng. 2020, 44, 56–64. [Google Scholar] [CrossRef]
- Kumar, D.; Deepak, K.B.; Muzakkir, S.M.; Wani, M.F.; Lijesh, K.P. Enhancing tribological performance of Ti-6Al-4V by sliding process. Tribol. Mater. Surf. Interf. 2018, 12, 137–143. [Google Scholar] [CrossRef]
- Sreesha, R.B.; Kumar, D.; Chandraker, S.; Agrawal, A. Room temperature sliding wear behavior of Ti6Al4V: A review. AIP Conf. Proc. 2021, 2341, 040041. [Google Scholar]
- Cheng, Z.; Li, Y.; Xu, C.; Liu, Y.; Ghafoor, S.; Li, F. Incremental sheet forming towards biomedical implants: A review. J. Mater. Res. Technol. 2020, 9, 7225–7251. [Google Scholar] [CrossRef]
- Kaur, S.; Ghadirinejad, K.; Oskouei, R.H. An Overview on the Tribological Performance of Titanium Alloys with Surface Modifications for Biomedical Applications. Lubricants 2019, 7, 65. [Google Scholar] [CrossRef] [Green Version]
- Ma, J.; Yang, H.; Li, H.; Wang, D.; Li, G.J. Tribological behaviors between commercial pure titanium sheet and tools in warm forming. Trans. Nonferrous Met. Soc. China 2015, 25, 2924–2931. [Google Scholar] [CrossRef]
- Jozwik, J. Evaluation of Tribological Properties and Condition of Ti6Al4V Titanium Alloy Surface. Teh. Vjesn. Tech. Gaz. 2018, 25, 170–175. [Google Scholar]
- Fridrici, V.; Fouvry, S.; Kapsa, P. Fretting Wear Behavior of a Titanium Alloy; Springer: Dordrecht, The Netherlands, 2001; pp. 413–421. [Google Scholar]
- Pakhamov, M.A.; Gorlov, D.; Stolyarov, V. Features of wear and friction in titanium. IOP Conf. Ser. Mater. Sci. Eng. 2020, 996, 012017. [Google Scholar] [CrossRef]
- Zhang, H.; Liu, X.; Liu, H.; Wang, Y.; Tang, B. Improvement of the fretting damage resistance of Ti-811 alloy by CuNi multilayer film. Tribol. Int. 2011, 44, 1488–1494. [Google Scholar] [CrossRef]
- Trzepieciński, T.; Lemu, H.G. Recent developments and trends in the friction testing for conventional sheet metal forming and incremental sheet forming. Metals 2020, 10, 47. [Google Scholar] [CrossRef] [Green Version]
- Trzepieciński, T.; Szpunar, M.; Kaščák, L. Modeling of friction phenomena of Ti-6Al-4V sheets based on backward elimination regression and multi-layer artificial neural networks. Materials 2021, 14, 2570. [Google Scholar] [CrossRef]
- Ajmal, M.; Ali, S.A.; Tahir, H.; Shah, M.R.; Saad, M. Synthesis of Emulsion by Using Vegetable Oil Modified by Titanium Dioxide (TiO2) Nanoparticles: A Peculiar Source for Synthesis of Bio-Based Lubricant and Novel Approach to Enhance the Efficiency of Emulsion. Eur. J. Lipid Sci. Technol. 2021, 123, 2000280. [Google Scholar] [CrossRef]
- Pang, H.; Ngalle, G. Formulation of SiO2/oil nanolubricant for metal forming using hydrodynamic cavitation. Proc. Inst. Mech. Eng. Part B J. Eng. Manuf. 2020, 234, 1549–1558. [Google Scholar] [CrossRef]
- Chen, Y.; Renner, P.; Liang, H. Dispersion of Nanoparticles in Lubricating Oil: A Critical Review. Lubricants 2019, 7, 7. [Google Scholar] [CrossRef] [Green Version]
- Fellah, M.; Assala, O.; Labaïz, M.; Dekhil, L.; Iost, A. Friction and Wear Behavior of Ti-6Al-7Nb Biomaterial Alloy. J. Biomater. Nanotech. 2013, 4, 374–384. [Google Scholar] [CrossRef] [Green Version]
- Niu, Q.L.; Zheng, X.H.; Ming, W.W.; Chen, M. Friction and Wear Performance of Titanium Alloys against Tungsten Carbide under Dry Sliding and Water Lubrication. Tribol. Trans. 2013, 56, 101–108. [Google Scholar] [CrossRef]
- Vahdani, M.; Mirnia, M.J.; Gorji, H.; Bakhshi-Jooybari, M. Experimental Investigation of Formability and Surface Finish into Resistance Single-Point Incremental Forming of Ti-6Al-4V Titanium Alloy Using Taguchi Design. Trans. Indian Inst. Met. 2019, 72, 1031–1041. [Google Scholar] [CrossRef]
- Gagliardi, F.; Ambrogio, G.; Filice, L. Formability of lightweight alloys by hot incremental sheet forming. Mater. Des. 2012, 34, 501–508. [Google Scholar]
- Hussain, G. Microstructure and mechanical and tribological properties of a lubricant coating for incremental forming of a Ti sheet. Trans. Mech. Eng. 2014, 38, 423–429. [Google Scholar]
- Sornsuwit, N.; Sittisakuljaroen, S.; Sangsai, N.; Suwankan, P. Effect of heat treatment on single point incremental forming for titanium grade 2 sheet. In Proceedings of the 2018 Third International Conference on Engineering Science and Innovative Technology (ESIT), North Bangkok, Thailand, 19–22 April 2018; p. 4. [Google Scholar]
- Hussain, G.; Gao, L.; Zhang, Z.Y. Formability evaluation of a pure titanium sheet in the cold incremental forming process. Int. J. Adv. Manuf. Technol. 2008, 37, 920–926. [Google Scholar] [CrossRef]
- Li, Y.; Chen, X.; Liu, Z.; Sun, J.; Li, F.; Li, J.; Zhao, G. A review on the recent development of incremental sheet-forming process. Int. J. Adv. Manuf. Technol. 2017, 92, 2439–2462. [Google Scholar] [CrossRef]
- Sbayti, M.; Bahloul, R.; Belhadjsalah, H. Numerical Modeling of Hot Incremental Forming Process for Biomedical Application. In Design and Modeling of Mechanical Systems—III. CMSM 2017. Lecture Notes in Mechanical Engineering; Haddar, M., Chaari, F., Benamara, A., Chouchane, M., Karra, C., Aifaoui, N., Eds.; Springer: Cham, Switzerland, 2018; pp. 881–891. [Google Scholar]
- Naranjo, J.; Miguel, V.; Martínez, A.; Coello, J.; Manjabacas, M.C.; Valera, J. Influence of temperature on alloy Ti6Al4V formability during the warm SPIF process. Procedia Eng. 2017, 207, 866–871. [Google Scholar] [CrossRef]
- Naranjoa, J.; Miguela, V.; Martínez-Martínez, A.; Gómez-Lópeza, L.M.; Manjabacas, M.C.; Coello, J. Analysis and simulation of Single Point Incremental Forming by ANSYS®. Procedia Eng. 2015, 132, 1104–1111. [Google Scholar] [CrossRef] [Green Version]
- Abdelkefi, A.; Guinesa, D.; Léotoinga, L.; Thuillier, S. Incremental forming of Titanium T40 sheet: Experimental and numerical investigations. In Proceedings of the 24ème Congrès Français de Mécanique, Brest, France, 26–30 August 2019; pp. 1–7. [Google Scholar]
- Piccininni, A.; Gagliardi, F.; Guglielmi, P.; De Napoli, L.; Ambrogio, G.; Sorgente, D.; Palumbo, G. Biomedical Titanium alloy prostheses manufacturing by means of Superplastic and Incremental Forming processes. Matec. Web Conf. 2016, 80, 15007. [Google Scholar] [CrossRef] [Green Version]
- Naranjo, J.; Miguel, V.; Martínez, A.; Coello, J.; Manjabacas, M.C. Analysis of material behavior models for the Ti6Al4V alloy to simulate the Single Point Incremental Forming process. Procedia Eng. 2017, 13, 307–314. [Google Scholar] [CrossRef]
- Nguyen, D.T.; Park, J.G.; Lee, H.J.; Kim, Y.S. Finite element method study of incremental sheet forming for complex shape and its improvement. Proc. Inst. Mech. Eng. Part B J. Eng. Manuf. 2010, 224, 913–924. [Google Scholar] [CrossRef]
- Al-Ghamdi, K.A.; Hussain, G. The pillowing tendency of materials in single-point incremental forming: Experimental and finite element analyses. Proc. Inst. Mech. Eng. Part B J. Eng. Manuf. 2015, 229, 744–753. [Google Scholar] [CrossRef]
- Manco, L.; Filice, L.; Ambrogio, G. Analysis of the thickness distribution varying tool trajectory in single-point incremental forming. Proc. Inst. Mech. Eng. Part B J. Eng. Manuf. 2011, 225, 348–356. [Google Scholar] [CrossRef]
- Henrard, C.; Bouffioux, C.; Eyckens, P.; Sol, H.; Duflou, J.R.; van Houtte, P.; van Bael, A.; Duchêne, L.; Habraken, A.M. Forming forces in single point incremental forming: Prediction by finite element simulations, validation and sensitivity. Comput. Mech. 2011, 47, 573–590. [Google Scholar] [CrossRef]









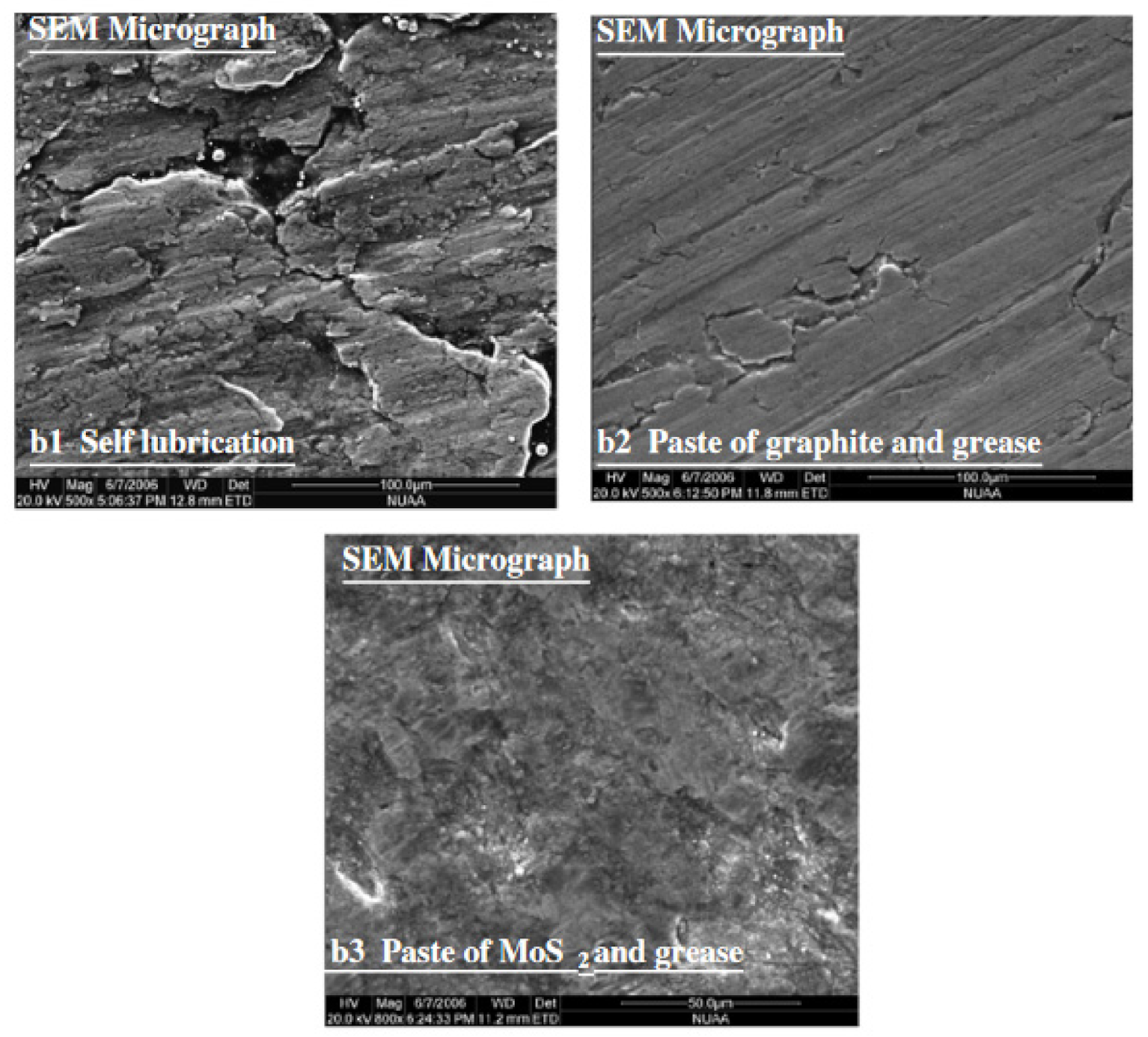
| Lubricant | Forming Conditions | Material | Shape of Drawpiece | Reference |
|---|---|---|---|---|
| OKS 280 lubricant | elevated temperature | Ti-6Al-4V | car door shell | [109] |
| MOS2 | elevated temperature | TiAl2Mn1.5 | tetragonal pyramid | [92] |
| MoS2 | elevated temperature | Ti-6Al-4V | conical frustum/tetragonal pyramid | [83] |
| graphite | elevated temperature | Ti-6Al-4V | conical frustum/tetragonal pyramid | [83] |
| boron nitride spray | elevated temperature | Ti-6Al-4V | conical frustum/tetragonal pyramid | [83] |
| MoS2 | elevated temperature | Ti-6Al-4V | conical frustum | [77] |
| MoS2, graphite powder, graphite-based and copper-based anti-seize lubricants | elevated temperature | Ti-6Al-4V | conical frustum | [150] |
| MoS2 | elevated temperature | Ti-6Al-4V | tetragonal pyramid | [100] |
| MoS2 | elevated temperature | Ti-6Al-4V | conical frustum | [86,93,102] |
| ROCOL copper-based anti-seize compound | elevated temperature | Ti-6Al-4V | conical frustum | [101] |
| boron nitride | elevated temperature | Ti-6Al-4V | tetragonal pyramid | [104,105] |
| OKS 280 lubricant | elevated temperature | Ti-6Al-4V | flat components with grooves | [106] |
| OKS 280 lubricant | elevated temperature | Ti-6Al-4V | truncated cone | [107] |
| MoS2 | elevated temperature | Ti-6Al-4V | truncated cone | [151] |
| carbon-based, dry-film lubricant (Berulit 935) | elevated temperature | Ti-6Al-4V | asymmetric component | [87] |
| dry graphite | elevated temperature | Ti-6Al-4V | flat component with longitudinal pockets | [89] |
| MoS2 | elevated temperature | Grade 2 Ti-6Al-4V | conical frustum | [94,95,96] |
| Rocol RTD compound | cold-forming conditions | Grade 1 | cranial plate | [68] |
| MoS2 | cold-forming conditions | pure titanium (grade not specified) | conical frustum | [152] |
| chlorine-containing forming oil | cold-forming conditions | Grade 1 | denture plate | [112] |
| Nuto 46 hydraulic oil | cold-forming conditions | Grade 2 | clavicle implant | [74] |
| ceramic grease WEICON ASW 040P | cold-forming conditions | Ti Grade 2 | facial implant | [71] |
| MoS2 | elevated temperature | Ti Grade 2 | conical frustum | [153] |
| MoS2 | cold-forming conditions | Pure titanium (grade not specified) | conical frustum | [154] |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Oleksik, V.; Trzepieciński, T.; Szpunar, M.; Chodoła, Ł.; Ficek, D.; Szczęsny, I. Single-Point Incremental Forming of Titanium and Titanium Alloy Sheets. Materials 2021, 14, 6372. https://doi.org/10.3390/ma14216372
Oleksik V, Trzepieciński T, Szpunar M, Chodoła Ł, Ficek D, Szczęsny I. Single-Point Incremental Forming of Titanium and Titanium Alloy Sheets. Materials. 2021; 14(21):6372. https://doi.org/10.3390/ma14216372
Chicago/Turabian StyleOleksik, Valentin, Tomasz Trzepieciński, Marcin Szpunar, Łukasz Chodoła, Daniel Ficek, and Ireneusz Szczęsny. 2021. "Single-Point Incremental Forming of Titanium and Titanium Alloy Sheets" Materials 14, no. 21: 6372. https://doi.org/10.3390/ma14216372
APA StyleOleksik, V., Trzepieciński, T., Szpunar, M., Chodoła, Ł., Ficek, D., & Szczęsny, I. (2021). Single-Point Incremental Forming of Titanium and Titanium Alloy Sheets. Materials, 14(21), 6372. https://doi.org/10.3390/ma14216372








