Optimization Method of Tool Parameters and Cutting Parameters Considering Dynamic Change of Performance Indicators
Abstract
:1. Introduction
2. Establishment and Verification of Finite Element Simulation of Milling Process
2.1. Establishment of Finite Element Simulation Model for Milling Process
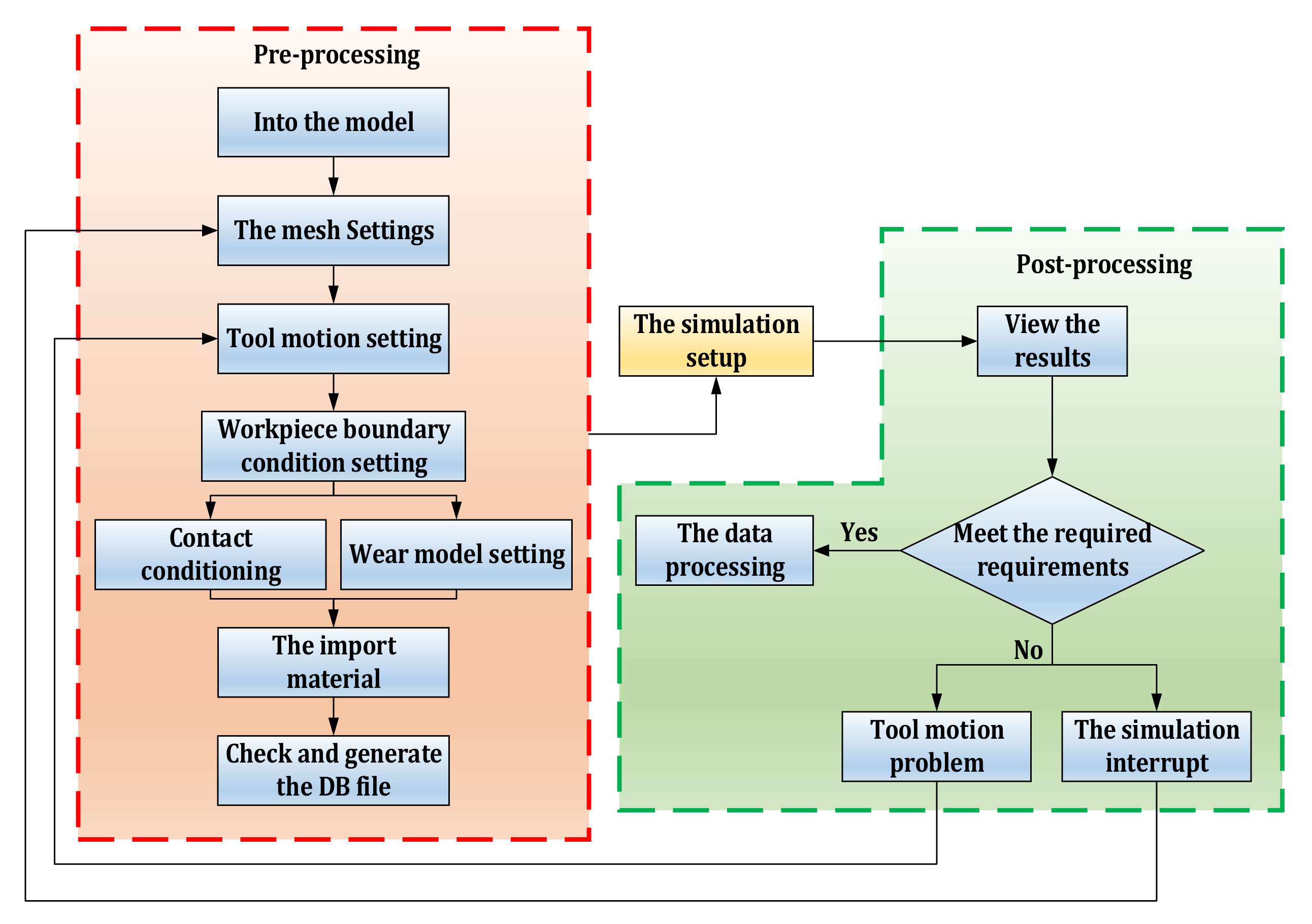
2.1.1. Finite Element Simulation Model Establishment Process
2.1.2. Material Constitutive Model
2.1.3. Material Parameters
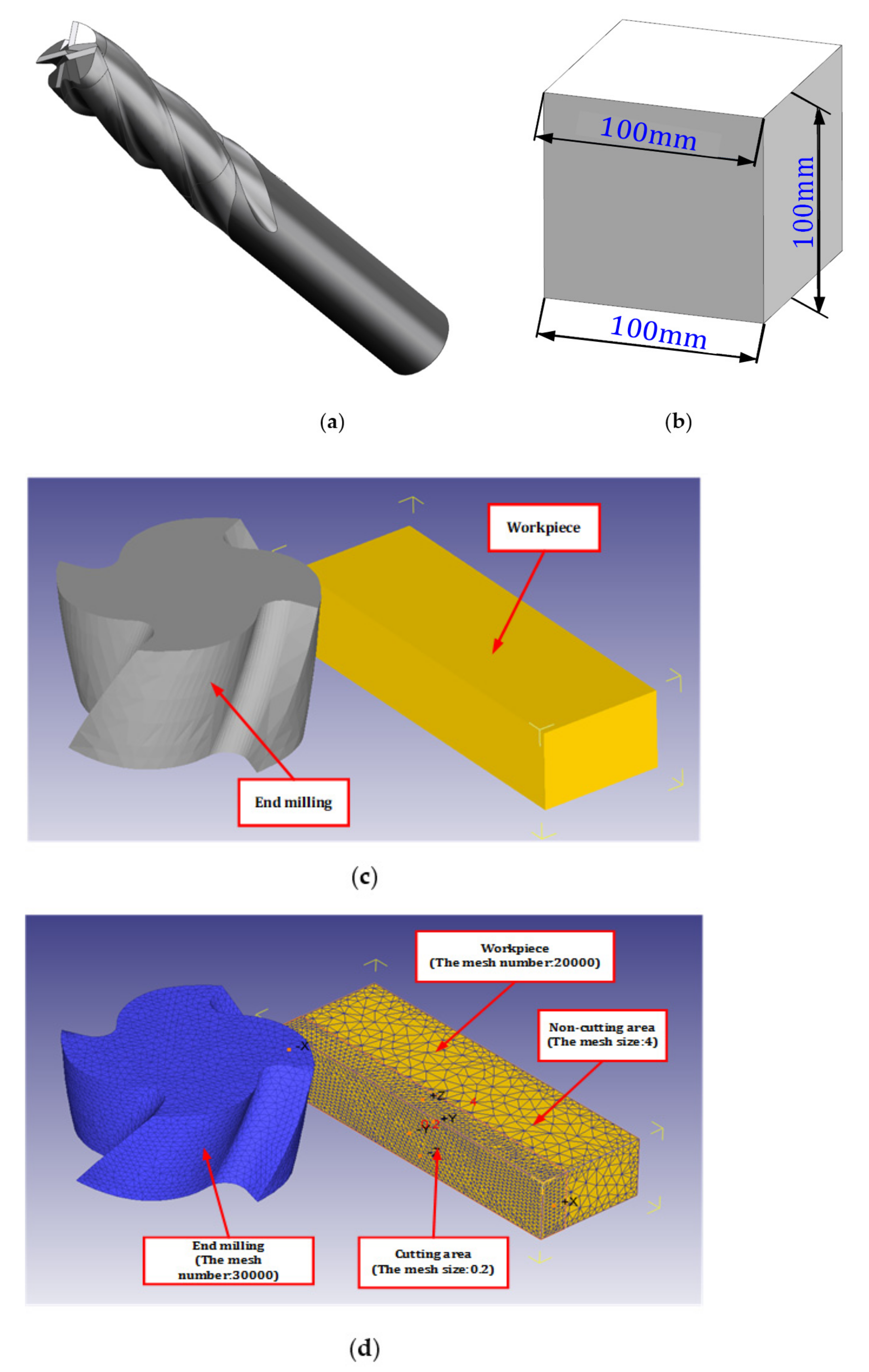
2.1.4. D Model Establishment, Import and Grid Division
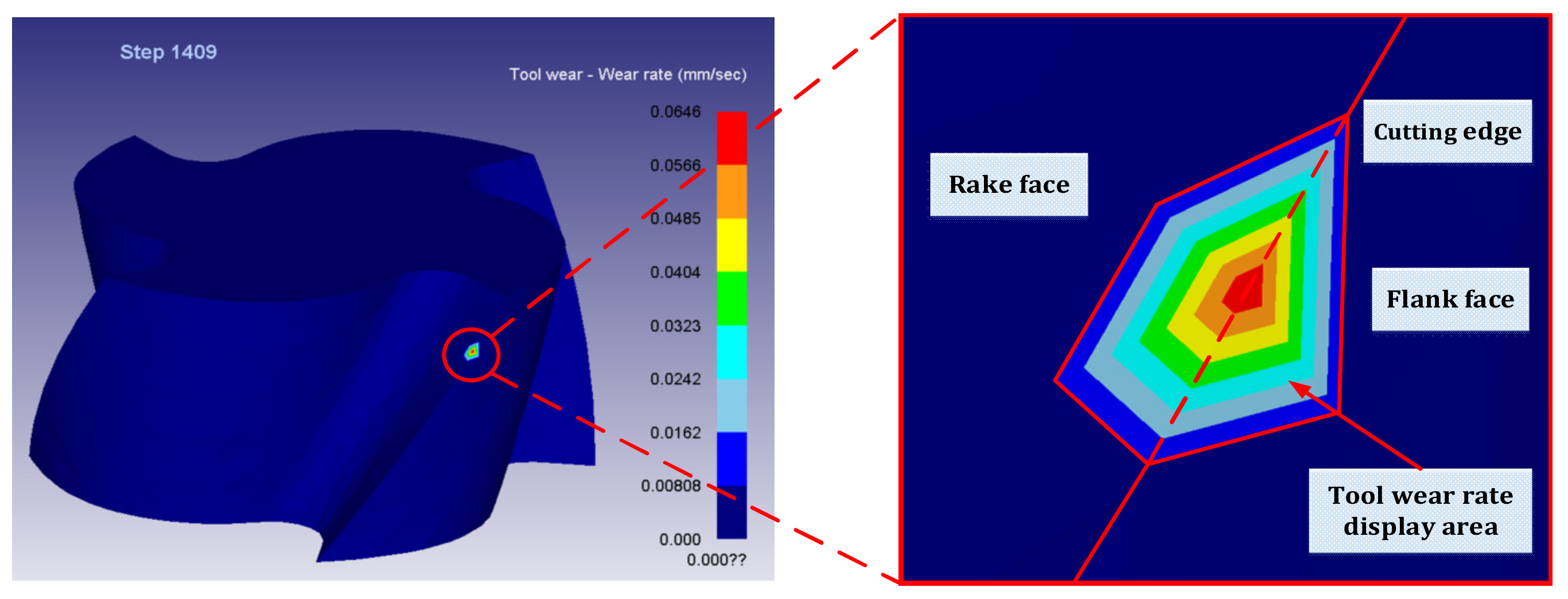
2.1.5. Setting of Tool Wear Model in Finite Element Simulation Software
2.2. Simulation Parameter Selection and Performance Indicator Setting
2.2.1. Simulation Parameter Selection
2.2.2. Setting Performance Indicators
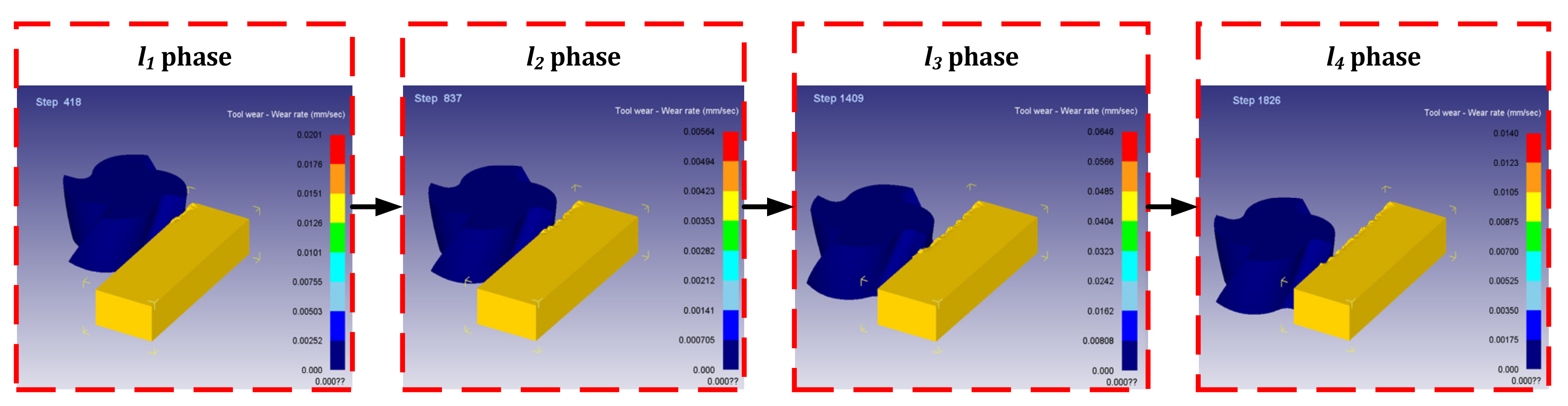
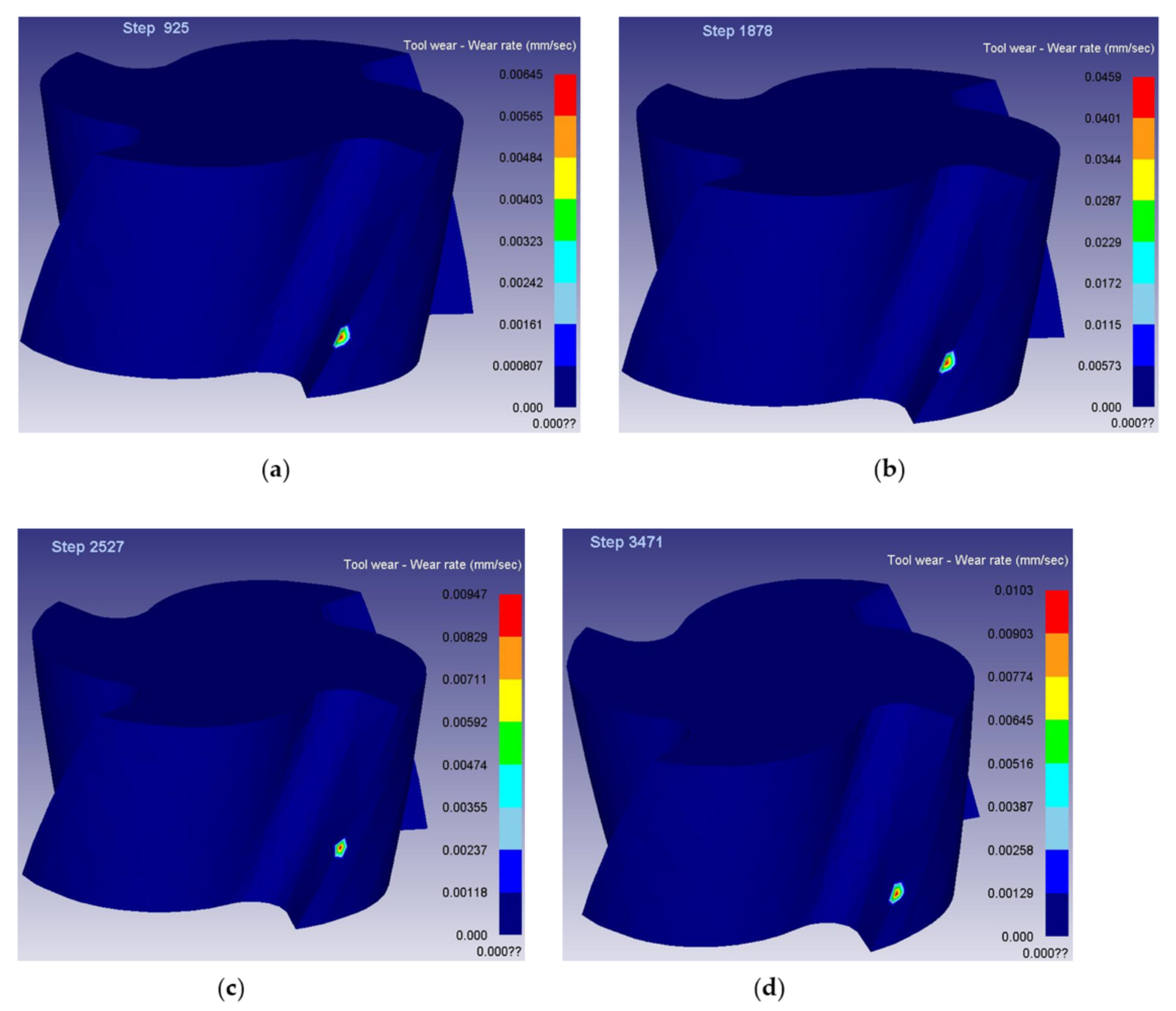
2.3. Finite Element Simulation Results
3. Dynamic Evaluation Method Based on Gain Horizontal Excitation
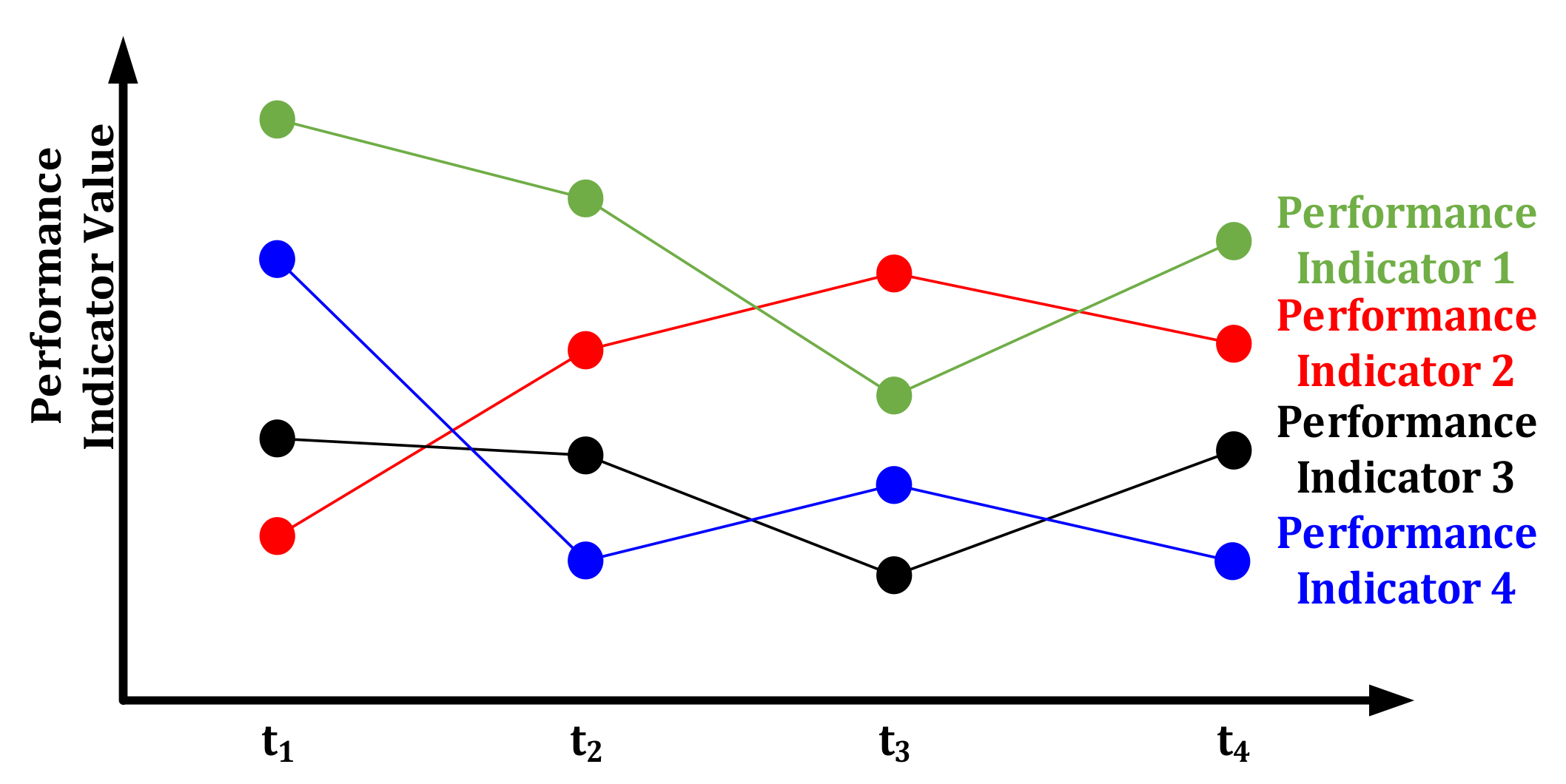
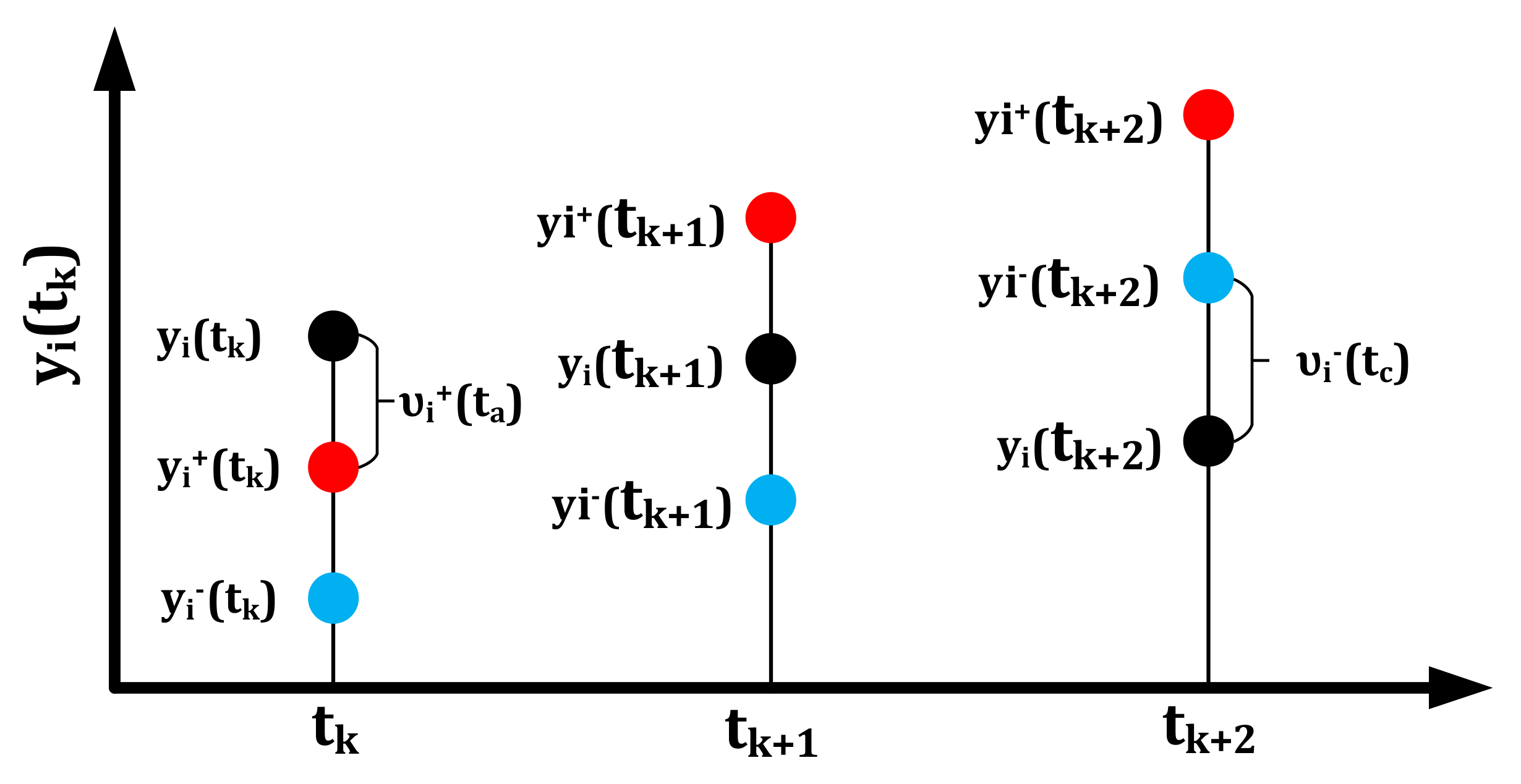
3.1. Dynamic Evaluation Method Based on Gain Level Excitation
3.2. Comprehensive Evaluation of Each Stage Based on Grey-Fuzzy Analytic Hierarchy Process
3.3. Parameter Level Optimization Based on Dynamic Evaluation Method
3.4. Comprehensive Evaluation of Parameter Level in Each Stage
3.5. Dynamic Evaluation of Parameter Level
3.6. Comparison between Parameter Combinations
4. Validation of the Finite Element Model
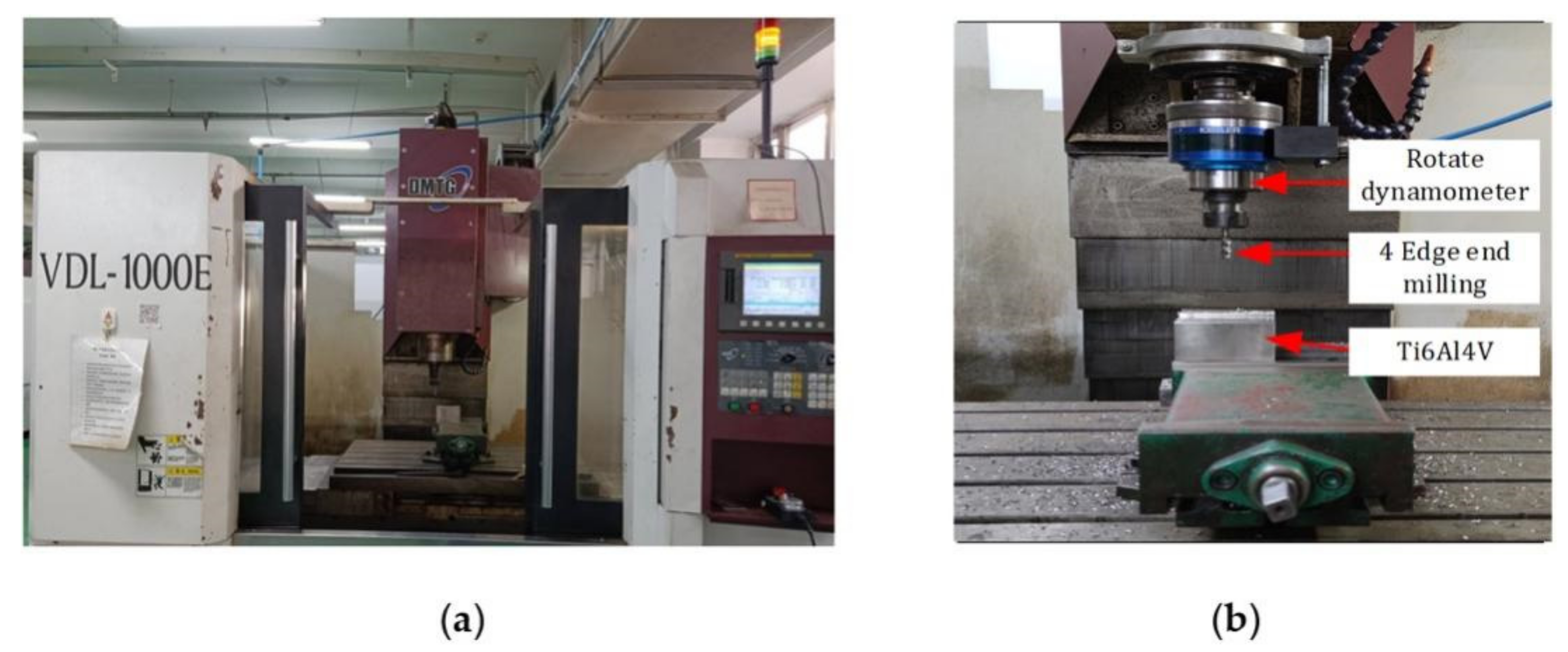
4.1. Setting of Experimental Parameters
4.2. The Experimental Device
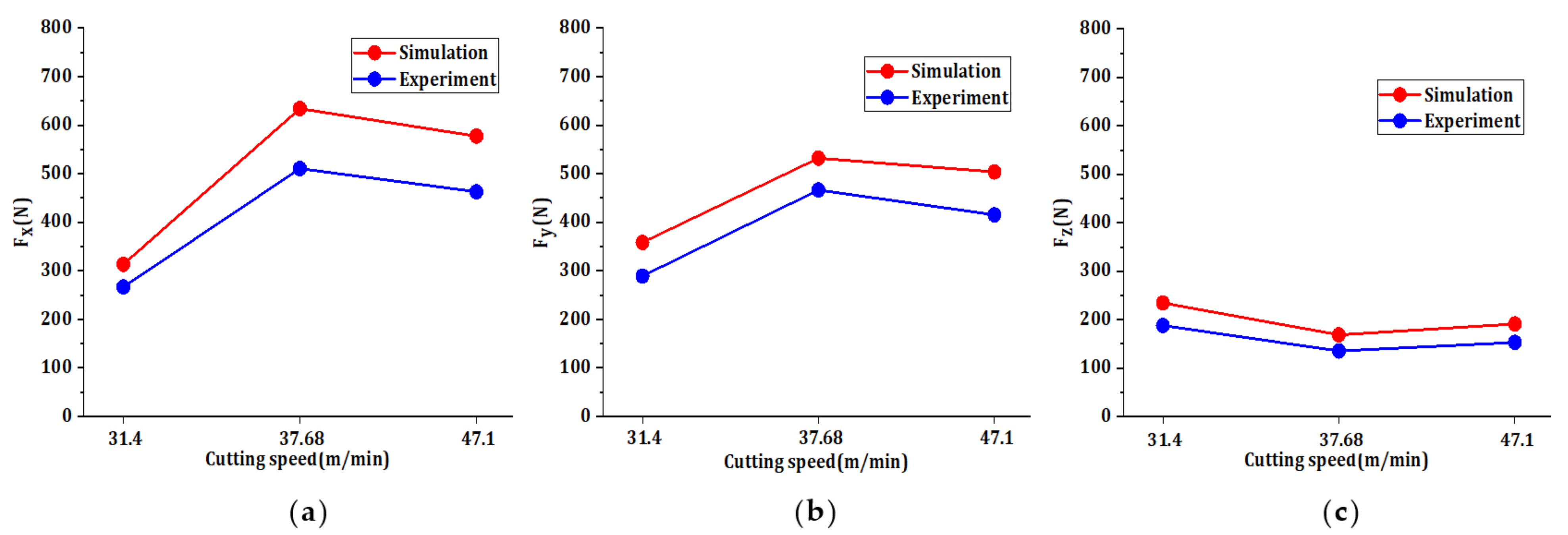
4.3. Reliability Verification of Simulation Model
5. Conclusions and Prospects
- In this paper, the dynamic evaluation method based on gain horizontal excitation was used to optimize the tool parameters and cutting parameters in the process of milling titanium alloy with milling cutter side, and the optimal matching combination of tool parameters and cutting parameters on the tool wear rate and material removal rate was obtained.
- When the rake angle is 8°, the cutting speed is 37.68 m/min, and the cutting width is 0.2 mm, the machining effect of the clearance angle is 9°, the helix angle is 30°, the feed per tooth is 0.15 mm/z, and the cutting depth is 2.5 mm achieves the best, which can simultaneously meet the requirements of long tool life and high machining efficiency. In addition, the reliability of simulation model is verified, and the optimization results are also reliable.
- The comparison between the optimized parameters by finite element method and the parameter combination in Table 6 shows that the optimized parameter combination has higher comprehensive performance.
- In this paper, the performance indicator value is obtained by simulation, but there is some error between simulation value and experimental value. Therefore, in the future, under the condition of sufficient time and funding, the required numerical value of tool wear rate and material removal rate will be obtained through experiments to make the optimization results more accurate.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| Notation | |
| CNC | Computerized Numerical Control |
| PVD | Physical Vapor Deposition |
| Symbol | |
| σ | The equivalent flow stress |
| ε | The equivalent plastic strain rate |
| ε0 | The reference plastic strain rate |
| T | The absolute temperature |
| Tr | The ambient temperature |
| Tm | The melting temperature |
| A | the yield strength |
| B | The hardening modulus |
| C | The strain rate sensitivity coefficient |
| m | The heat softening coefficient |
| n | The strain hardening index |
| V | The material removal rate |
| vf | The feed speed |
| d | The cutter diameter |
| ap | The cutting depth |
| ae | The cutting width |
| fz | The feed per tooth |
| fz | The feed per tooth |
| z | The number of teeth |
| WAdhesion wear | The adhesion wear |
| σn | The positive pressure |
| vc | The chip slip speed |
| T | The celsius |
| Aw | The wear characteristic constant |
| Bw | The wear characteristic constant |
| lk | The kth phase |
| tk | The period k |
| n | The number of evaluated objects |
| si | The ith object to be evaluated |
| m | The number of performance indicators |
| T | The number of time periods |
| xj | The jth performance indicator |
| xij(tk) | The value of the ith evaluated object about the jth indicator at the time tk |
| Bk | The static evaluation matrix of the kth period |
| yi(tk) | The static evaluation value of the ith evaluated object in the kth period |
| Y | The static comprehensive evaluation matrix |
| ηmax | The mean maximum gain |
| ηmin | The mean minimum gain |
| The average gain | |
| η+ | The optimal gain level |
| η− | The inferior gain level |
| yi+(tk) | The optimal excitation point of the ith evaluated object at time tk |
| yi−(tk) | The inferior excitation point of the ith evaluated object at time tk |
| υi+(tk) | The optimal excitation quantity obtained by the ith evaluated object at time tk |
| υi−(tk) | The inferior excitation obtained by the ith evaluated object at time tk |
| zi(tk) | The dynamic comprehensive evaluation value of the ith evaluated object at tk moment |
| h+ | The optimal excitation factor |
| h− | The inferior excitation factor |
| r | The proportional relationship between the total amount of the optimal excitation quantity and the total amount of bad incentives |
| τk | The time factor |
| zi | The total dynamic comprehensive evaluation value of the ith evaluated object |
| Ujh | The weight ratio of the jth performance indicator to the hth performance indicator |
References
- Wang, B.; Liu, Z.; Cai, Y.; Luo, X.; Ma, H.; Song, Q.; Xiong, Z. Advancements in material removal mechanism and surface integrity of high speed metal cutting: A review. Int. J. Mach. Tools Manuf. 2021, 166, 103744. [Google Scholar] [CrossRef]
- Zhang, X.; Ma, J.W.; Jia, Z.Y.; Song, D.N. Machining parameter optimisation for aviation aluminium-alloy thin-walled parts in high-speed milling. Int. J. Mach. Mach. Mater. 2018, 20, 180–192. [Google Scholar] [CrossRef]
- Kubilay, A.; Mohd, D.; Ahmet, H.; Mozammel, M.; Munish, G.; Turnad, G.; Hassan, I. Investigations on surface roughness and tool wear characteristics in micro-turning of Ti-6Al-4V Alloy. Materials 2020, 13, 2998. [Google Scholar]
- Ghosh, T.; Wang, Y.; Martinsen, K.; Wang, K. A surrogate-assisted optimization approach for multi-response end milling of aluminum alloy AA3105. Int. J. Adv. Manuf. Technol. 2020, 111, 2419–2439. [Google Scholar] [CrossRef]
- Juan, C.O.P.; Sepideh, A.; Juan, P.C.R.; Juan, P.C.R. Cutting parameter optimization of Al-6063-O using numerical simulations and particle swarm optimization. Int. J. Adv. Manuf. Technol. 2020, 111, 2507–2532. [Google Scholar]
- Zhang, N.; Wu, D.W.; Jiang, G.J. Optimization of Machining Parameters in blisk processing based on tool reliability. In IOP Conference Series: Materials Science and Engineering; IOP: Bristol, UK, 2021. [Google Scholar]
- Mohammed, Y.E.K.; Shunmugesh, K. Multi-objective optimization of milling process parameters in glass fibre reinforced polymer via grey relational analysis and desirability function. Mater. Today. Proc. 2019, 11, 1015–1023. [Google Scholar]
- Fang, Y.; Zhao, L.; Lou, P.; Yan, J. Cutting parameter optimization method in multi-pass milling based on improved adaptive PSO and SA. J. Phys. Conf. Ser. 2021, 1848, 012116. [Google Scholar] [CrossRef]
- Vimal, P.; Partha, P.D. Parametric optimization of CNC turning on glass-fibre-reinforced plastic (GFRP) pipes: A grey-fuzzy logic approach. In IOP Conference Series: Materials Science and Engineering; IOP: Bristol, UK, 2018. [Google Scholar]
- Viswanathan, R.; Ramesh, S.; Maniraj, S.; Subburam, V. Measurement and multi-response optimization of turning parameters for magnesium alloy using hybrid combination of Taguchi-GRA-PCA technique. Measurement 2020, 159, 107800. [Google Scholar] [CrossRef]
- Suresh, P.; Marimuthu, K.; Ranganathan, S.; Rajmohan, T. Optimization of machining parameters in turning of Al-SiC-Gr hybrid metal matrix composites using grey-fuzzy algorithm. Trans. Nonferrous Met. Soc. China 2014, 24, 2805–2814. [Google Scholar] [CrossRef]
- Gnanavelbabu, A.; Arunachalam, V.; Surendran, K.T.S.; Rajkumar, K. Optimization of machining parameters in CNC turning of AA6061-B4C-CNT hybrid composites using Grey-fuzzy method. In IOP Conference Series: Materials Science and Engineering; IOP: Bristol, UK, 2020. [Google Scholar]
- Swastik, P.; Kalipada, M. Optimization of machining parameter characteristics during turning of Ti-6Al-4V using desirability function analysis. Mater. Today Proc. 2018, 5, 25740–25749. [Google Scholar]
- Yameogo, D.; Haddag, B.; Makich, H.; Nouari, M. Prediction of the cutting forces and chip morphology when machining the Ti6Al4V alloy using a microstructural coupled model. Procedia CIRP 2017, 58, 335–340. [Google Scholar] [CrossRef]
- Yang, S.; Han, P.; Liu, X.; Tong, X. Accurate preparation of mesoscopic geometric characteristics of ball end milling cutter and optimization of cutting performance. Proc. Inst. Mech. Eng. Part B J. Eng. Manuf. 2021. [Google Scholar] [CrossRef]
- Zhang, Z.; Wang, Z.; Wang, W.; Jiang, R.; Xiong, Y.-F. Investigation on surface quality of high-speed cutting titanium alloy Ti6Al4V based on Split-Hopkinson pressure bar. Proc. Inst. Mech. Eng. Part B J. Eng. Manuf. 2020, 234, 1293–1301. [Google Scholar] [CrossRef]
- Charalampous, P. Prediction of cutting forces in milling using machine learning algorithms and finite element analysis. J. Mater. Eng. Perform. 2021, 30, 2002–2013. [Google Scholar] [CrossRef]
- Alajmi, M.; Almeshal, A. Modeling of Cutting Force in the Turning of AISI 4340 Using Gaussian Process Regression Algorithm. Appl. Sci. 2021, 11, 4055. [Google Scholar] [CrossRef]
- Ma, Z.F.; Guo, Y.J.; Zhang, F.M.; Pan, Y.H. Method dynamic comprehensive evaluation based on gain level inspiriting. J. Syst. Eng. 2009, 24, 223–247. [Google Scholar]
- Peng, B.; Bergs, T.; Schraknepper, D.; Klocke, F.; Döbbeler, B. A hybrid approach using machine learning to predict the cutting forces under consideration of the tool wear. Procedia CIRP 2019, 82, 302–307. [Google Scholar]
- Kali, P.V.N.; Anupama, F.K.; Venkata, S.M.Y. Modeling and simulation of Aluminum 1100 alloy in an extrusion process. Mater. Today Proc. 2020, 23, 518–522. [Google Scholar]
- Liu, M.; Ji, Z.; Fan, R.; Wang, X. Finite Element Analysis of Extrusion Process for Magnesium Alloy Internal Threads with Electromagnetic Induction-Assisted Heating and Thread Performance Research. Materials 2020, 13, 2170. [Google Scholar]
- Premnarayan, V.; Ajay, S. 3D Finite Element Analysis of milling process for non-ferrous metal using deform-3D. Mater. Today Proc. 2020, 26, 525–528. [Google Scholar]
- Abdulkadir, Y.; Abdullah, K.; Selçuk, Y. Finite element simulation of drilling operation and theoretical analysis of drill stresses with the deform-3D. Simul. Model. Pract. Theory 2020, 104, 102153. [Google Scholar]
- Li, A.; Zhao, J.; Pei, Z.; Zhu, N. Simulation-based solid carbide end mill design and geometry optimization. Int. J. Adv. Manuf. Technol. 2014, 71, 1889–1900. [Google Scholar] [CrossRef]
- Jia, Y.; Quan, C.H.; Guo, J.M.; Wang, M.; Zhang, Q.J. Finite Element Analysis on Processing of PCD End-Mill Milling Copper. Mater. Sci. Forum 2020, 993, 421–426. [Google Scholar] [CrossRef]
- Fu, J.; Wang, F.L.; Yang, X.J.; Zhu, K.Y. Simulation and experimental study of titanium alloy cutting process considering material damage evolution. Mech. Sci. Technol. 2014, 33, 1245–1250. [Google Scholar]
- Yue, C.X.; Liu, X.; He, G.H.; Li, L.X. Finite element simulation analysis of milling process of thin-walled titanium alloy parts. Aeronaut. Manuf. Technol. 2019, 62, 60–66. [Google Scholar]
- Feng, Y.X.; Hsu, F.C.; Lu, Y.T.; Lin, Y.F.; Lin, C.T.; Lin, C.F.; Lu, Y.C.; Liang, S.Y. Tool wear rate prediction in ultrasonic vibration-assisted milling. Mach. Sci. Technol. 2020, 24, 758–780. [Google Scholar] [CrossRef]
- Liang, X.Y.; Mi, G.B.; He, L.J.; Li, P.J. Numerical Simulation of Combustion Resistant Titanium Alloy Wear Behavior at High Temperature Fields. Mater. Sci. Forum 2018, 913, 168–175. [Google Scholar] [CrossRef]
- Sun, Y.J. Modeling of Machining Parameters Modeling of Milling Titanium Alloy and Prediction of Tool Wear State. Ph.D. Thesis, Shandong University, Shandong, China, April 2014. [Google Scholar]
- Usui, E.; Shirakashi, T.; Kitagawa, T. Analytical prediction of cutting tool wear. Int. J. Wear 1984, 100, 129–151. [Google Scholar] [CrossRef]
- Takey, H.; Murata, R. Basic investigation of tool wear. J. Eng. Ind. 1963, 85, 33–37. [Google Scholar]
- Kloche, F.; Raedt, H.W.; Hopper, S. 2D-FEM simulation of orthogonal high speed cutting process. Mach. Sci. Technol. 2001, 5, 323–340. [Google Scholar] [CrossRef]
- Ahilan, C.; Kumanan, S.; Sivakumaran, N. Multi-objective optimisation of CNC turning process using grey based fuzzy logic. Int. J. Mach. Mach. Mater. 2009, 5, 434. [Google Scholar] [CrossRef]
- Du, D.; Pang, Q.H.; Wu, Y. Modern Comprehensive Evaluation Method and Case Selection, 3rd ed.; Tsinghua University Press: Beijing, China, 2015; pp. 201–206. [Google Scholar]
- Biswajit, D.; Roy, S.; Rai, R.N.; Saha, S.C. Application of grey fuzzy logic for the optimization of CNC milling parameters for Al-4.5%Cu-TiC MMCs with multi-performance characteristics. Eng. Sci. Technol. Int. J. 2016, 19, 857–865. [Google Scholar]
- Laakso, S.; Peltokorpi, J.; Ratava, J.; Lohtander, M.; Varis, J. Graph-based analysis of metal cutting parameters. In Advances in Sustainable and Competitive Manufacturing Systems Lecture Notes in Mechanical Engineering; Springer: Singapore, 2013; pp. 627–636. [Google Scholar]


| A (MPa) | B (MPa) | C | m | n | (s−1) | Tm (°C) | Tr (°C) |
|---|---|---|---|---|---|---|---|
| 875 | 793 | 0.01 | 0.71 | 0.386 | 1 | 1560 | 20 |
| Material Parameter | YG6 | Ti6Al4V |
|---|---|---|
| Density (g/cm3) | 14.85 | 4.44 |
| Young’s modulus (GPa) | 640 | 112 |
| Poission’s Ratio | 0.22 | 0.34 |
| Expansion (/°C) | 4.7 × 10−6 | 9.4 × 10−6 |
| Conductivity (W/m·K) | 79.6 | 6.8 |
| Specific heat (J/(kg·°C)) | 176 | 565 |
| Num. | Parameter | Value |
|---|---|---|
| 1 | The blade number | 4 |
| 2 | The cutter diameter | 10 (mm) |
| 3 | The rake angle | 8 (°) |
| 4 | The width of rake face | 1.0 (mm) |
| 5 | The first clearance angle | 12 (°) |
| 6 | The second clearance angle | 23 (°) |
| 7 | The width of the first flank face | 0.7 (mm) |
| 8 | The width of the second flank face | 0.8 (mm) |
| 9 | The helix angle | 35 (°) |
| 10 | The core diameter | 6.2 (mm) |
| Rake Angle (°) | Cutting Speed (m/min) | Cutting Width (mm) |
|---|---|---|
| 8 | 37.68 | 0.2 |
| Clearance Angle (°) | Helix Angle (°) | Feed Per Tooth (mm/z) | Cutting Depth (mm) | |
|---|---|---|---|---|
| 1 | 8.00 | 30.00 | 0.05 | 1.00 |
| 2 | 9.00 | 32.00 | 0.10 | 1.50 |
| 3 | 10.00 | 33.00 | 0.15 | 2.00 |
| 4 | 11.00 | 34.00 | 0.20 | 2.50 |
| 5 | 12.00 | 35.00 | 0.25 | 3.00 |
| Clearance Angle (°) | Helix Angle (°) | Feed Per Tooth (mm/z) | Cutting Depth (mm) | |
|---|---|---|---|---|
| 1 | 9.00 | 32.00 | 0.20 | 1.50 |
| 2 | 12.00 | 30.00 | 0.10 | 1.50 |
| 3 | 11.00 | 30.00 | 0.15 | 2.00 |
| 4 | 8.00 | 32.00 | 0.25 | 2.00 |
| 5 | 10.00 | 30.00 | 0.20 | 2.50 |
| 6 | 9.00 | 33.00 | 0.15 | 2.50 |
| 7 | 11.00 | 32.00 | 0.10 | 3.00 |
| 8 | 12.00 | 32.00 | 0.05 | 2.50 |
| 9 | 12.00 | 34.00 | 0.20 | 2.00 |
| 10 | 11.00 | 34.00 | 0.25 | 2.50 |
| 11 | 10.00 | 34.00 | 0.05 | 3.00 |
| 12 | 9.00 | 34.00 | 0.10 | 1.00 |
| 13 | 9.00 | 30.00 | 0.25 | 3.00 |
| 14 | 11.00 | 33.00 | 0.05 | 1.50 |
| 15 | 8.00 | 33.00 | 0.20 | 3.00 |
| 16 | 8.00 | 30.00 | 0.05 | 1.00 |
| 17 | 10.00 | 32.00 | 0.15 | 1.00 |
| 18 | 12.00 | 35.00 | 0.15 | 3.00 |
| 19 | 8.00 | 34.00 | 0.15 | 1.50 |
| 20 | 10.00 | 35.00 | 0.25 | 1.50 |
| 21 | 9.00 | 35.00 | 0.05 | 2.00 |
| 22 | 11.00 | 35.00 | 0.20 | 1.00 |
| 23 | 12.00 | 33.00 | 0.25 | 1.00 |
| 24 | 8.00 | 35.00 | 0.10 | 2.50 |
| 25 | 10.00 | 33.00 | 0.10 | 2.00 |
| The First Stage (l1) | The Second Stage (l2) | The Third Stage (l3) | The Fourth Stage (l4) | |||||
|---|---|---|---|---|---|---|---|---|
| Wear Rate (mm/s) | V (mm3/s) | Wear Rate (mm/s) | V (mm3/s) | Wear Rate (mm/s) | V (mm3/s) | Wear Rate (mm/s) | V (mm3/s) | |
| 1 | 0.00934 | 4.8 | 0.00547 | 4.8 | 0.00156 | 4.8 | 0.02260 | 4.8 |
| 2 | 0.07030 | 2.4 | 0.04810 | 2.4 | 0.06770 | 2.4 | 0.13100 | 2.4 |
| 3 | 0.01680 | 4.8 | 0.01240 | 4.8 | 0.00945 | 4.8 | 0.02470 | 4.8 |
| 4 | 0.00623 | 8.0 | 0.01920 | 8.0 | 0.01110 | 8.0 | 0.13000 | 8.0 |
| 5 | 0.03900 | 8.0 | 0.14100 | 8.0 | 0.03130 | 8.0 | 0.02760 | 8.0 |
| 6 | 0.00718 | 6.0 | 0.03550 | 6.0 | 0.00378 | 6.0 | 0.01050 | 6.0 |
| 7 | 0.00168 | 4.8 | 0.00718 | 4.8 | 0.11400 | 4.8 | 0.01590 | 4.8 |
| 8 | 0.02320 | 2.0 | 0.01390 | 2.0 | 0.01760 | 2.0 | 0.01180 | 2.0 |
| 9 | 0.00508 | 6.4 | 0.03820 | 6.4 | 0.04800 | 6.4 | 0.10500 | 6.4 |
| 10 | 0.01030 | 10.0 | 0.02150 | 10.0 | 0.00221 | 10.0 | 0.00834 | 10.0 |
| 11 | 0.00338 | 2.4 | 0.22500 | 2.4 | 0.00802 | 2.4 | 0.05170 | 2.4 |
| 12 | 0.06360 | 1.6 | 0.11400 | 1.6 | 0.04850 | 1.6 | 0.05840 | 1.6 |
| 13 | 0.00747 | 12.0 | 0.05300 | 12.0 | 0.09910 | 12.0 | 0.06970 | 12.0 |
| 14 | 0.01660 | 1.2 | 0.04650 | 1.2 | 0.01180 | 1.2 | 0.07540 | 1.2 |
| 15 | 0.05810 | 9.6 | 0.02610 | 9.6 | 0.05140 | 9.6 | 0.01940 | 9.6 |
| 16 | 0.01460 | 0.8 | 0.03630 | 0.8 | 0.07520 | 0.8 | 0.04940 | 0.8 |
| 17 | 0.00944 | 2.4 | 0.03190 | 2.4 | 0.07750 | 2.4 | 0.00726 | 2.4 |
| 18 | 0.02010 | 6.4 | 0.00564 | 6.4 | 0.06460 | 6.4 | 0.01400 | 6.4 |
| 19 | 0.00544 | 3.2 | 0.00826 | 3.2 | 0.00757 | 3.2 | 0.01910 | 3.2 |
| 20 | 0.03630 | 6.0 | 0.09990 | 6.0 | 0.03680 | 6.0 | 0.03020 | 6.0 |
| 21 | 0.01550 | 1.6 | 0.00282 | 1.6 | 0.01850 | 1.6 | 0.00993 | 1.6 |
| 22 | 0.01930 | 3.2 | 0.01340 | 3.2 | 0.02070 | 3.2 | 0.01630 | 3.2 |
| 23 | 0.01380 | 4.0 | 0.03740 | 4.0 | 0.01010 | 4.0 | 0.00943 | 4.0 |
| 24 | 0.04980 | 4.0 | 0.01570 | 4.0 | 0.02950 | 4.0 | 0.03220 | 4.0 |
| 25 | 0.01970 | 3.2 | 0.01060 | 3.2 | 0.04600 | 3.2 | 0.02340 | 3.2 |
| t1 | t2 | … | tT | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| x1 | x2 | … | xm | x1 | x2 | … | xm | … | x1 | x2 | … | xm | |
| s1 | x11(t1) | x12(t1) | … | x1m(t1) | x11(t2) | x12(t2) | … | x1m(t2) | … | x11(tT) | x12(tT) | … | x1m(tT) |
| s2 | x21(t1) | x22(t1) | … | x2m(t1) | x21(t2) | x22(t2) | … | x2m(t2) | … | x21(tT) | x22(tT) | … | x2m(tT) |
| … | … | … | … | … | … | … | … | … | … | … | … | … | … |
| sn | xn1(t1) | xn2(t1) | … | xnm(t1) | xn1(T2) | xn2(t2) | … | xnm(t2) | … | xn1(tT) | xn2(tT) | … | xnm(tT) |
| xj/xh | x1/x2 | x2/x2 |
|---|---|---|
| Ujh | 1.5 | 1.0 |
| The First Stage (l1) | The Second Stage (l2) | The Third Stage (l3) | The Fourth Stage (l4) | |||||
|---|---|---|---|---|---|---|---|---|
| Wear Rate (mm/s) | V (mm3/s) | Wear Rate (mm/s) | V (mm3/s) | Wear Rate (mm/s) | V (mm3/s) | Wear Rate (mm/s) | V (mm3/s) | |
| 1 | 0.02683 | 5.12 | 0.02111 | 5.12 | 0.03495 | 5.12 | 0.05002 | 5.12 |
| 2 | 0.02062 | 5.20 | 0.04216 | 5.20 | 0.03429 | 5.20 | 0.03427 | 5.20 |
| 3 | 0.09176 | 4.40 | 0.10168 | 4.40 | 0.03992 | 4.40 | 0.02803 | 4.40 |
| 4 | 0.01150 | 5.04 | 0.01728 | 5.04 | 0.04477 | 5.04 | 0.01450 | 5.04 |
| 5 | 0.02650 | 4.24 | 0.02865 | 4.24 | 0.04160 | 4.24 | 0.26369 | 4.24 |
| l1 | l2 | l3 | l4 | ||||
|---|---|---|---|---|---|---|---|
| yi(l1) | yi+(l2) | yi−(l2) | yi+(l3) | yi−(l3) | yi+(l4) | yi−(l4) | |
| 1 | 0.77701 | 0.76937 | 0.74242 | 0.88793 | 0.86098 | 0.86810 | 0.84115 |
| 2 | 0.88889 | 0.88125 | 0.85430 | 0.77800 | 0.75105 | 0.99236 | 0.96541 |
| 3 | 0.75000 | 0.74236 | 0.71541 | 0.74236 | 0.71541 | 0.43160 | 0.40465 |
| 4 | 0.90000 | 0.89236 | 0.86541 | 0.89236 | 0.86541 | 0.49236 | 0.46541 |
| 5 | 0.57008 | 0.56244 | 0.53549 | 0.60416 | 0.57721 | 0.37621 | 0.34926 |
| l1 | l2 | l3 | l4 | |||||
|---|---|---|---|---|---|---|---|---|
| υi+(l1) | υi−(l1) | υi+(l2) | υi−(l2) | υi+(l3) | υi−(l3) | υi+(l4) | υi−(l4) | |
| 1 | 0 | 0 | 0.12640 | 0 | 0 | 0 | 0 | 0.03139 |
| 2 | 0 | 0 | 0 | 0.06866 | 0.22800 | 0 | 0 | 0.04758 |
| 3 | 0 | 0 | 0.00764 | 0 | 0 | 0.27617 | 0.25963 | 0 |
| 4 | 0 | 0 | 0.00764 | 0 | 0 | 0.36541 | 0.40764 | 0 |
| 5 | 0 | 0 | 0.04936 | 0 | 0 | 0.19336 | 0 | 0.01593 |
| z(l1) | z(l2) | z(l3) | z(l4) | z | |
|---|---|---|---|---|---|
| 1 | 0.77701 | 0.95611 | 0.87574 | 0.79340 | 3.40295 |
| 2 | 0.88889 | 0.74986 | 1.10920 | 0.89304 | 3.65099 |
| 3 | 0.75000 | 0.75366 | 0.29534 | 0.70366 | 2.50266 |
| 4 | 0.9000 | 0.90366 | 0.30960 | 1.09524 | 3.20850 |
| 5 | 0.57008 | 0.63544 | 0.28310 | 0.32503 | 1.81365 |
| zHelix angle | zFeed per tooth | zCutting depth | |
|---|---|---|---|
| 1 | 2.44285 | 1.99773 | 1.75018 |
| 2 | 2.20450 | 1.62204 | 1.77147 |
| 3 | 2.31967 | 3.05105 | 2.48153 |
| 4 | 2.04067 | 2.39639 | 2.93874 |
| 5 | 2.06289 | 2.95389 | 2.67173 |
| Wear Rate (mm/s) | V (mm3/s) | |||
|---|---|---|---|---|
| l1 | l2 | l3 | l4 | |
| 0.00645 | 0.0459 | 0.00947 | 0.0103 | 6.0 |
| Cutting Speed (m/min) | Feed Speed (mm/min) | Cutting Depth (mm) | Cutting Width (mm) |
|---|---|---|---|
| 31.40 | 400 | 3 | 0.8 |
| 37.68 | 400 | 3 | 0.8 |
| 47.10 | 400 | 3 | 0.8 |
| Cutting Speed (m/min) | Simulation (N) | Experiment (N) | |
|---|---|---|---|
| Fx | 31.40 | 313.613 | 266.791 |
| 37.68 | 634.243 | 510.750 | |
| 47.10 | 577.552 | 462.902 | |
| Fy | 31.40 | 358.606 | 289.408 |
| 37.68 | 532.094 | 466.600 | |
| 47.10 | 503.908 | 415.483 | |
| Fz | 31.40 | 234.752 | 188.069 |
| 37.68 | 168.884 | 135.653 | |
| 47.10 | 191.216 | 153.299 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yue, D.; Zhang, A.; Yue, C.; Liu, X.; Li, M.; Hu, D. Optimization Method of Tool Parameters and Cutting Parameters Considering Dynamic Change of Performance Indicators. Materials 2021, 14, 6181. https://doi.org/10.3390/ma14206181
Yue D, Zhang A, Yue C, Liu X, Li M, Hu D. Optimization Method of Tool Parameters and Cutting Parameters Considering Dynamic Change of Performance Indicators. Materials. 2021; 14(20):6181. https://doi.org/10.3390/ma14206181
Chicago/Turabian StyleYue, Daxun, Anshan Zhang, Caixu Yue, Xianli Liu, Mingxing Li, and Desheng Hu. 2021. "Optimization Method of Tool Parameters and Cutting Parameters Considering Dynamic Change of Performance Indicators" Materials 14, no. 20: 6181. https://doi.org/10.3390/ma14206181
APA StyleYue, D., Zhang, A., Yue, C., Liu, X., Li, M., & Hu, D. (2021). Optimization Method of Tool Parameters and Cutting Parameters Considering Dynamic Change of Performance Indicators. Materials, 14(20), 6181. https://doi.org/10.3390/ma14206181








