Structure and Strength of Artificial Soils Containing Monomineral Clay Fractions
Abstract
:1. Introduction
2. Materials and Methods
2.1. Substrates
- goethite 71063-100G (Sigma-Aldrich, St Louis, MO, USA);
- kaolinite containing <5% illite and ~10% quartz,
- illite containing ~10% kaolinite and ~5% quartz,
- montmorillonite K10 (Sigma Aldrich Chemie GmbH, Steinheim, Germany);
- zeolite coming from a clinoptilolitic tuff deposit in Sokirnitsa, Ukraine [59] containing clinoptilolite as a dominant phase, ~10% stilbite and ~10% thomsonite.
Substrates Characteristics
2.2. Preparation of Artificial Soil Aggregates
The Aggregates Characteristics
3. Results and Discussion
3.1. Properties of the Substrates
3.2. Mechanical Properties of the Aggregates
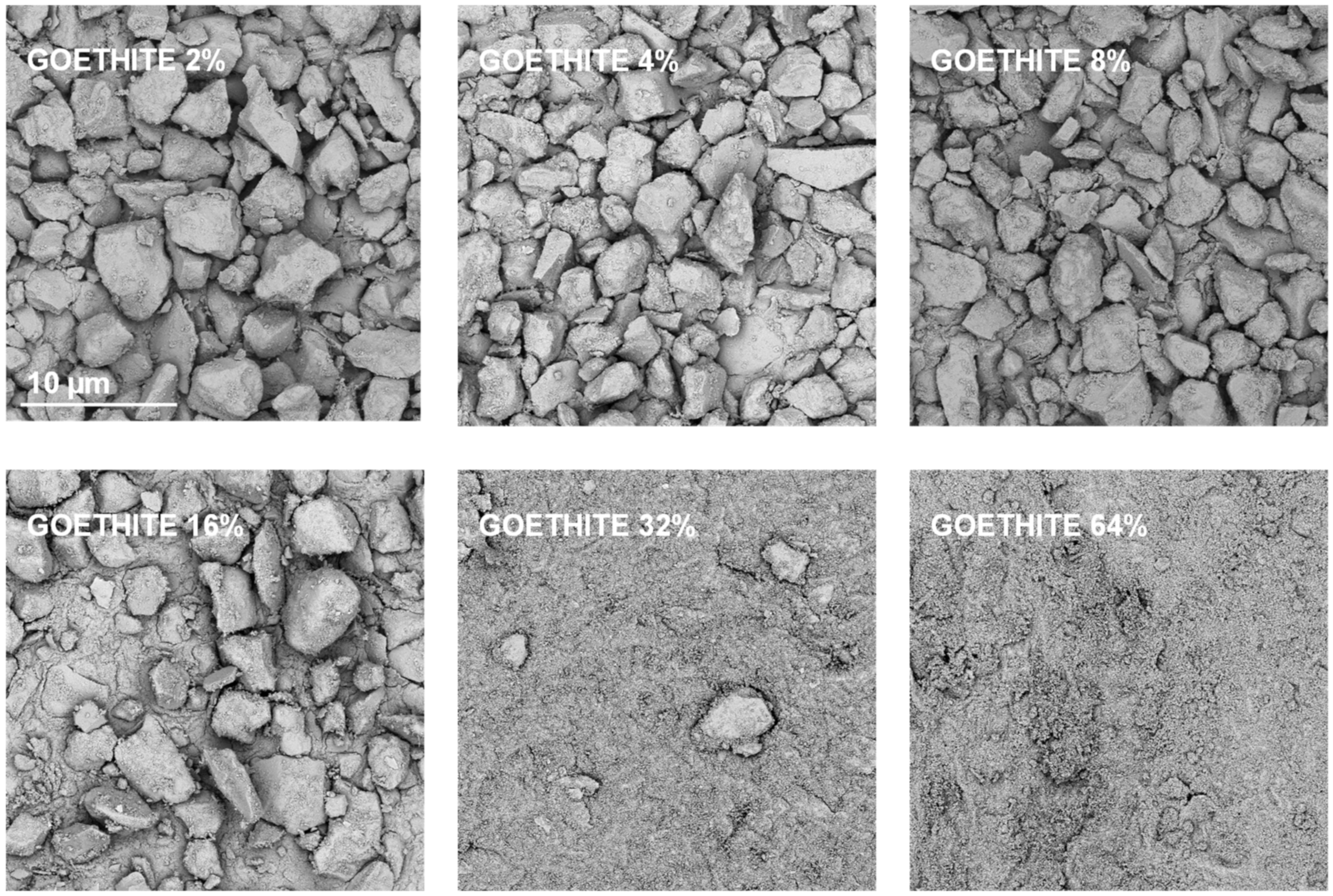
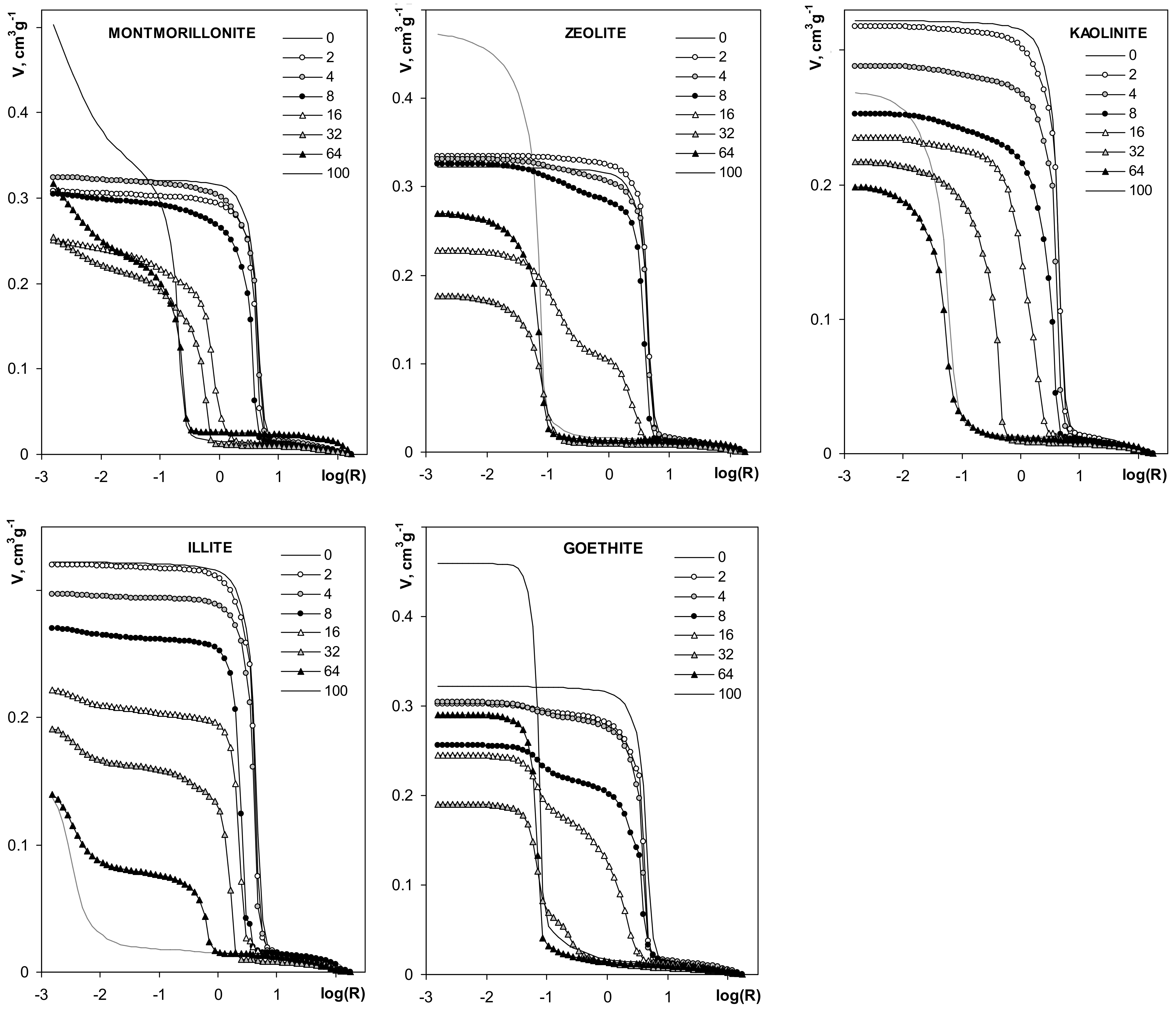
3.3. Structural Properties of the Aggregates
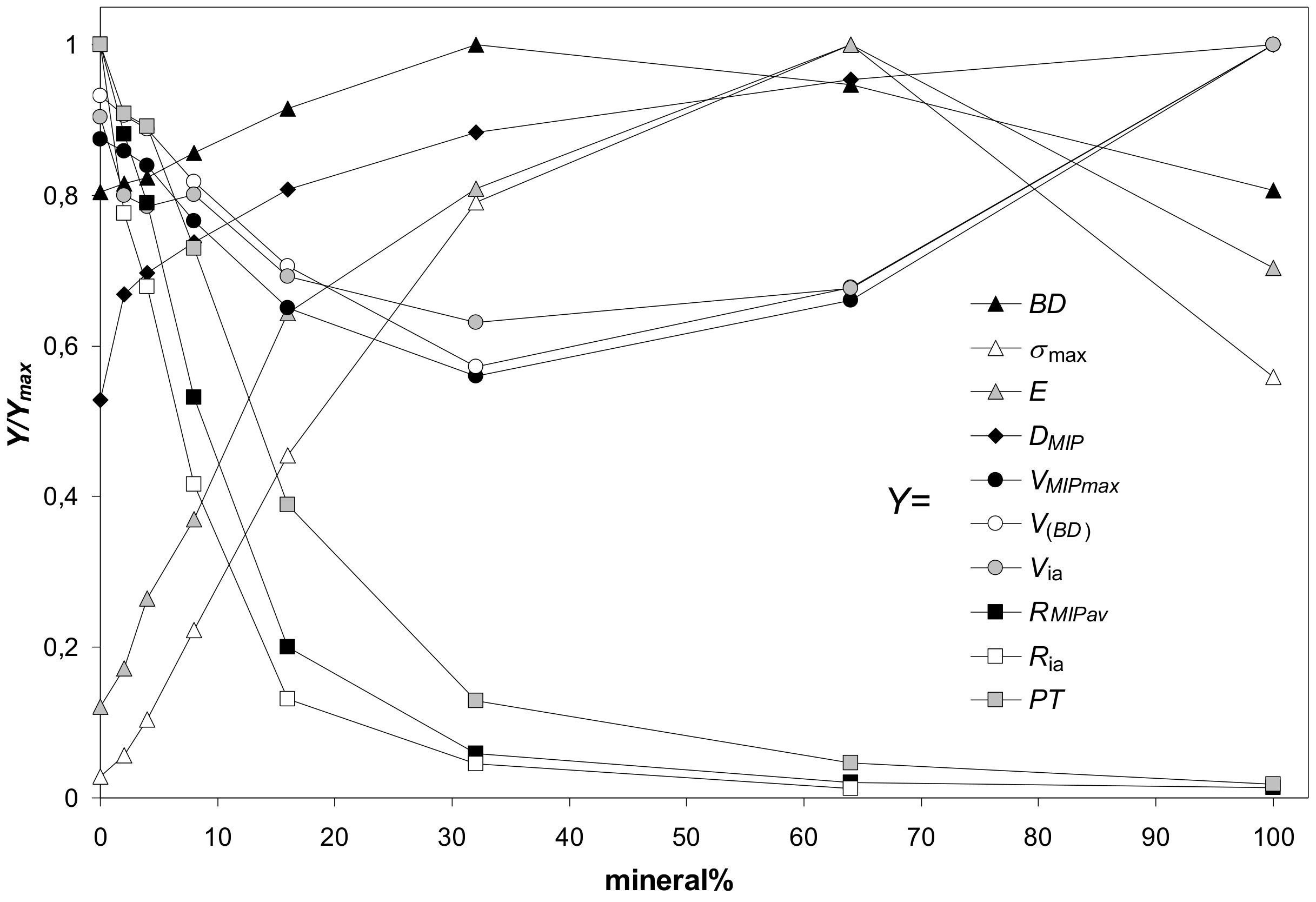
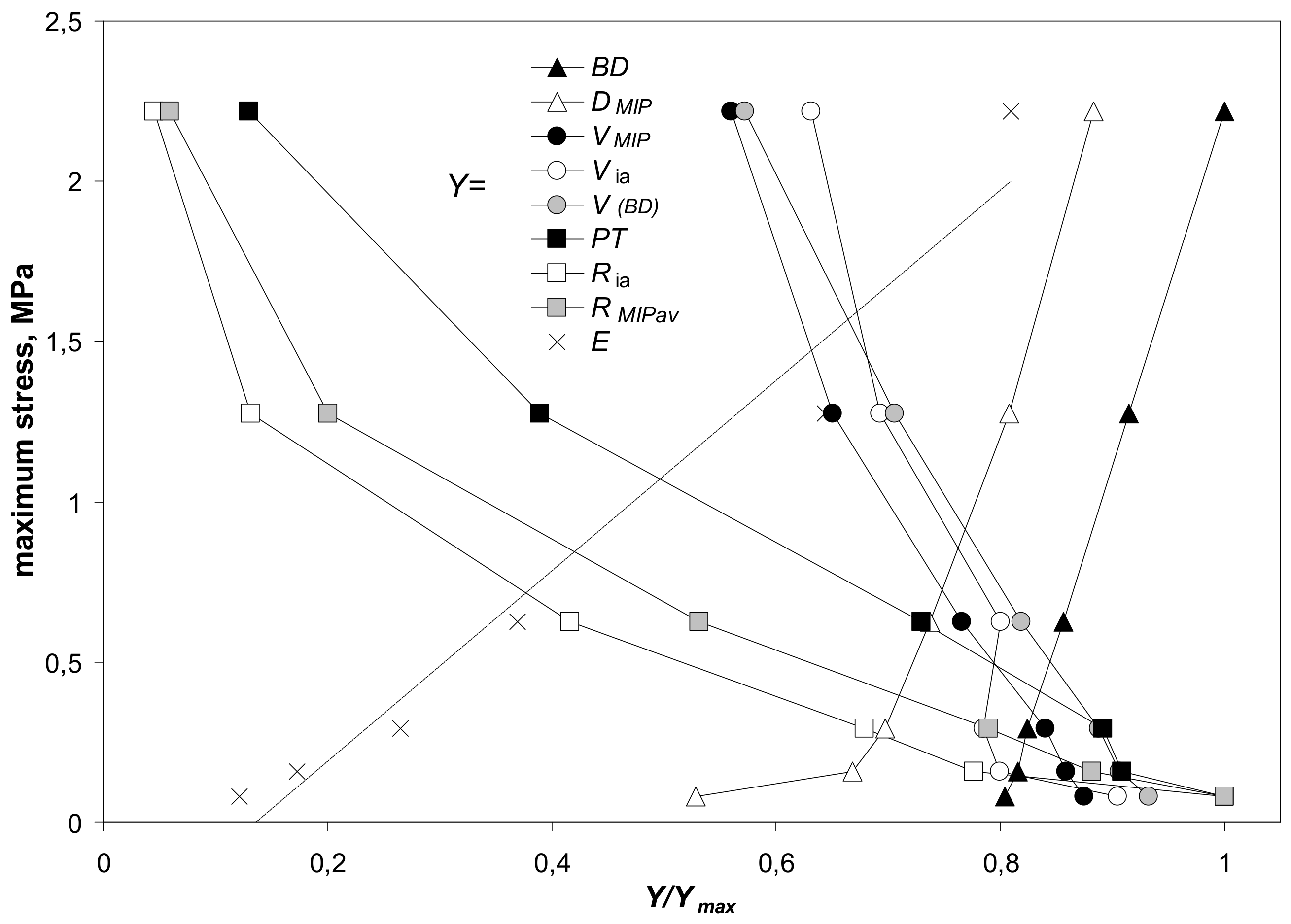
3.4. Relations between Mechanical and Structural Properties of the Aggregates
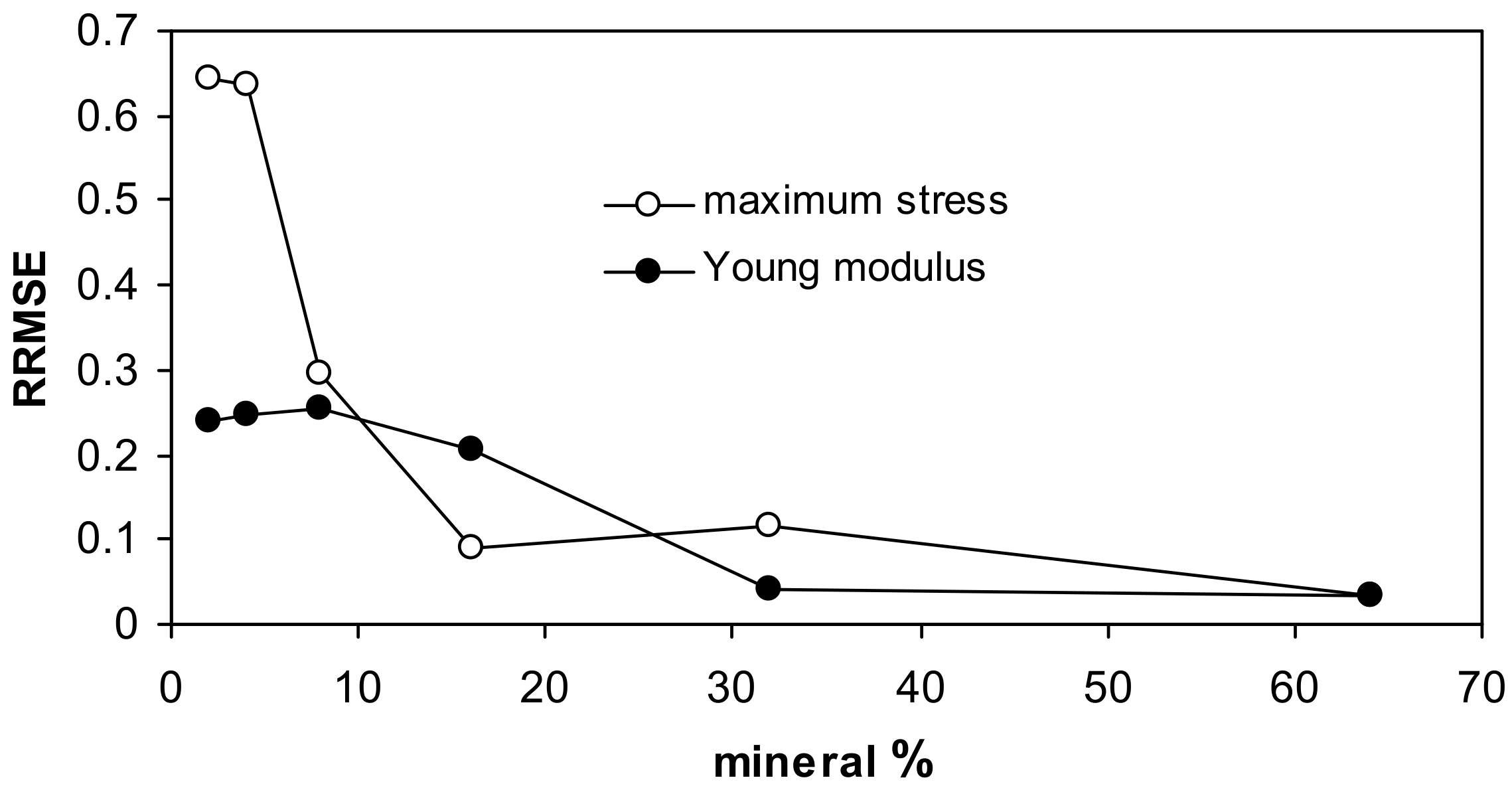
3.5. Relations between Mechanical Properties of the Aggregates and Properties of the Mineral Particles
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Szatanik-Kloc, A.; Horn, R.; Lipiec, J.; Siczek, A.; Boguta, P. Initial growth and root surface properties of dicotyledonous plants in structurally intact field soil and compacted headland soil. Soil Tillage Res. 2019, 195, 104387. [Google Scholar] [CrossRef]
- Vervoort, R.; Radcliffe, D.E.; West, L.T. Soil structure development and preferential solute flow. Water Resour. Res. 1999, 35, 913–928. [Google Scholar] [CrossRef]
- Muukkonen, P.; Hartikainen, H.; Alakukku, L. Effect of soil structure disturbance on erosion and phosphorus losses from Finnish clay soil. Soil Tillage Res. 2009, 103, 84–91. [Google Scholar] [CrossRef]
- Sharma, L.; Singh, R.; Ahmad, M.; Umrao, R.; Singh, T. Experimental Evaluation of Geomechanical Behaviour of Bentonite-Sand Mixture for Nuclear Waste Disposal. Procedia Eng. 2017, 191, 386–393. [Google Scholar] [CrossRef]
- Wasil, M. Effect of Bentonite Addition on the Properties of Fly Ash as a Material for Landfill Sealing Layers. Appl. Sci. 2020, 10, 1488. [Google Scholar] [CrossRef] [Green Version]
- Rout, S.; Singh, S.P. Characterization of pond ash-bentonite mixes as landfill liner material. Waste Manag. Res. 2020, 38, 1420–1428. [Google Scholar] [CrossRef] [PubMed]
- Narloch, P.; Woyciechowski, P.; Kotowski, J.; Gawriuczenkow, I.; Wójcik, E. The Effect of Soil Mineral Composition on the Compressive Strength of Cement Stabilized Rammed Earth. Materials 2020, 13, 324. [Google Scholar] [CrossRef] [Green Version]
- Harabinova, S.; Panulinova, E.; Kormaníkova, E.; Kotrasova, K. Importance of soil shear strength parameters for optimal design of the building foundation. Theory Build. Pr. 2019, 1, 5–11. [Google Scholar] [CrossRef]
- Moayed, R.Z.; Izadi, E.; Heidari, S. Stabilization of saline silty sand using lime and micro silica. J. Central South Univ. 2012, 19, 3006–3011. [Google Scholar] [CrossRef]
- Ural, N. The Importance of Clay in Geotechnical Engineering. In Current Topics in the Utilization of Clay in Industrial and Medical Applications; Zoveidavianpoor, M., Ed.; IntechOpen: London, UK, 2018. [Google Scholar]
- Whalley, W.R.; Watts, C.W.; Gregory, A.S.; Mooney, S.J.; Clark, L.J.; Whitmore, A.P. The effect of soil strength on the yield of wheat. Plant Soil 2008, 306, 237–247. [Google Scholar] [CrossRef]
- Vanorio, T.; Prasad, M.; Nur, A. Elastic properties of dry clay mineral aggregates, suspensions and sandstones. Geophys. J. Int. 2003, 155, 319–326. [Google Scholar] [CrossRef] [Green Version]
- Amézketa, E. Soil Aggregate Stability: A Review. J. Sustain. Agric. 1999, 14, 83–151. [Google Scholar] [CrossRef]
- Bronick, C.; Lal, R. Soil structure and management: A review. Geoderma 2005, 124, 3–22. [Google Scholar] [CrossRef]
- Huat, B.B.K.; Asadi, A.; Kazemian, S. Experimental investigation on geomechanical properties of tropical organic soils and peat. Am. J. Eng. Appl. Sci. 2009, 2, 184–188. [Google Scholar]
- Kolay, P.K.; Aminur, M.R.; Taib, S.N.L.; Zain, M.I.S.M. Correlation between Different Physical and Engineering Properties of Tropical Peat Soils from Sarawak. In Proceedings of the GeoShanghai International Conference, Shanghai, China, 3–5 June 2010; pp. 56–61. [Google Scholar]
- Saride, S.; Chikyala, S.R.; Puppala, A.J.; Harris, P.J. Effects of Organics on Stabilized Expansive Subgrade Soils. J. Geotech. Geoenviron. 2010, 155–164. [Google Scholar] [CrossRef]
- Vasudevan, T.V.S.; Jaya, V. Influence of Organic Content on Fly Ash Stabilization of Clay. In Ground Improvement Techniques and Geosynthetics; Springer: Singapore, 2018; Volume 14, pp. 95–103. [Google Scholar] [CrossRef]
- Jozefaciuk, G.; Czachor, H. Impact of organic matter, iron oxides, alumina, silica and drying on mechanical and water stability of artificial soil aggregates. Assessment of new method to study water stability. Geoderma 2014, 221, 1–10. [Google Scholar] [CrossRef]
- Six, J.; Feller, C.; Denef, K.; Ogle, S.M.; Sá, J.C.D.M.; Albrecht, A. Soil organic matter, biota and aggregation in temperate and tropical soils — Effects of no-tillage. Agronomie 2002, 22, 755–775. [Google Scholar] [CrossRef] [Green Version]
- Igwe, C.; Akamigbo, F.; Mbagwu, J. Chemical and mineralogical properties of soils in southeastern Nigeria in relation to aggregate stability. Geoderma 1999, 92, 111–123. [Google Scholar] [CrossRef]
- Kramarenko, V.; Nikitenkov, A.; Molokov, V.Y.; Matveenko, I.A.; Shramok, A.V. The Relationship of Physical Property Indicators and Clay Soil Structural Strength of Tomsk Oblast Territory. IOP Conf. Series: Earth Environ. Sci. 2015, 27, 012030. [Google Scholar] [CrossRef]
- Norsyahariati, N.D.N.; Hui, K.R.; Juliana, A.G.A. The Effect of Soil Particle Arrangement on Shear Strength Behavior of Silty Sand. MATEC Web Conf. 2016, 47, 3022. [Google Scholar] [CrossRef] [Green Version]
- Tiwari, B.; Ajmera, B. Consolidation and swelling behavior of major clay minerals and their mixtures. Appl. Clay Sci. 2011, 54, 264–273. [Google Scholar] [CrossRef]
- Ye, L.; Jin, Y.F.; Zhu, Q.Y.; Sun, P.P. Influence of mineral constituents on one-dimensional compression behaviour of clayey soils. Geotech. Eng. J. SEAGS AGSSEA 2015, 46. [Google Scholar]
- Osmanlioglu, A.E. Evaluation on mechanical stabilities of clay-sand mixtures used as engineered barrier for radioactive waste disposal. World Acad. Sci. Eng. Technol. Int. J. Environ. Chem. Ecol. Geol. Geophys. Eng. 2016, 10, 632–635. [Google Scholar]
- Charkley, F.N.; Zhang, K.; Mei, G. Shear Strength of Compacted Clays as Affected by Mineral Content and Wet-Dry Cycles. Adv. Civ. Eng. 2019, 2019, 1–8. [Google Scholar] [CrossRef] [Green Version]
- Onyelowe, K. Kaolin soil and its stabilization potentials as nanostructured cementitious admixture for geotechnics purposes. Int. J. Pavement Res. Technol. 2018, 11, 717–724. [Google Scholar] [CrossRef]
- Rajabi, A.M.; Ardakani, S.B. Effects of Natural-Zeolite Additive on Mechanical and Physicochemical Properties of Clayey Soils. J. Mater. Civ. Eng. 2020, 32, 04020306. [Google Scholar] [CrossRef]
- Firoozi, A.A.; Taha, M.R.; Firoozi, A.A.; Tanveer, A. Assessment of heat treatment on clays mixed with silica sand. Aust. J. Basic Appl. Sci. 2014, 8, 310–314. [Google Scholar]
- Carraro, J.A.H.; Prezzi, M.; Salgado, R. Shear Strength and Stiffness of Sands Containing Plastic or Nonplastic Fines. J. Geotech. Geoenviron. Eng. 2009, 135, 1167–1178. [Google Scholar] [CrossRef]
- Salgado, R.; Bandini, P.; Karim, A. Shear Strength and Stiffness of Silty Sand. J. Geotech. Geoenviron. Eng. 2000, 126, 451–462. [Google Scholar] [CrossRef]
- Supandi, S.; Zakaria, Z.; Sukiyah, E.; Sudradjat, A. The Influence of Kaolinite — Illite toward mechanical properties of Claystone. Open Geosci. 2019, 11, 440–446. [Google Scholar] [CrossRef] [Green Version]
- Tembe, S.; Lockner, D.A.; Wong, T.-F. Effect of clay content and mineralogy on frictional sliding behavior of simulated gouges: Binary and ternary mixtures of quartz, illite, and montmorillonite. J. Geophys. Res. Space Phys. 2010, 115, 03416. [Google Scholar] [CrossRef]
- Naeini, S.A.; Gholampoor, N.; Jahanfar, M.A. Effect of leachate’s components on undrained shear strength of clay-bentonite liners. Eur. J. Environ. Civ. Eng. 2017, 23, 395–408. [Google Scholar] [CrossRef]
- Kawajiri, S.; Kawaguchi, T.; Yamasaki, S.; Nakamura, D.; Yamashita, S.; Shibuya, S. Strength characteristics of compacted soil with particular reference to soil structure and anisotropy. Int. J. GEOMATE 2017, 13, 178–185. [Google Scholar] [CrossRef]
- Horn, R.; Fleige, H. Soil Structure and Mechanical Strength. Encyclopedia of Agrophysics. In Encyclopedia of Earth Sciences Series; Gliński, J., Horabik, J., Lipiec, J., Eds.; Springer: Dordrecht, The Netherlands, 2011; pp. 777–780. [Google Scholar]
- Ajayi, A.E.; Junior, M.D.S.D.; Curi, N.; Junior, C.F.A.; Aladenola, O.O.; Souza, T.T.T.; Junior, A.V.I. Comparison of estimation methods of soil strength in five soils. Revista Brasileira de Ciência do Solo 2009, 33, 487–495. [Google Scholar] [CrossRef] [Green Version]
- Brown, E.T. Rock Characterization, Testing and Monitoring, ISRM Suggested Methods; Pergamon Press: Oxford, UK, 1981. [Google Scholar]
- Agustawijaya, D.S. The uniaxial compressive strength of soft rock. Civ. Eng. Dimens. 2007, 9, 9–14. [Google Scholar]
- Bell, F.G.; Culshaw, M.G. Petrographic and engineering properties of sandstones from the Sneinton Formation, Nottinghamshire, England. Q. J. Eng. Geol. Hydrogeol. 1998, 31, 5–19. [Google Scholar] [CrossRef]
- Güneyli, H.; Rüşen, T. Effect of length-to-diameter ratio on the unconfined compressive strength of cohesive soil specimens. Bull. Int. Assoc. Eng. Geol. 2015, 75, 793–806. [Google Scholar] [CrossRef]
- Hawkins, A.B.; McConnell, B.J. Sensitivity of sandstone strength and deformability to changes in moisture content. Q. J. Eng. Geol. Hydrogeol. 1992, 25, 115–130. [Google Scholar] [CrossRef]
- Latifi, N.; Vahedifard, F.; Siddiqua, S.; Horpibulsuk, S. Solidification–Stabilization of Heavy Metal–Contaminated Clays Using Gypsum: Multiscale Assessment. Int. J. Geomech. 2018, 18, 04018150. [Google Scholar] [CrossRef]
- Dowdy, R.H.; Larson, W.E. Tensile Strength of Montmorillonite as a Function of Saturating Cation and Water Content. Soil Sci. Soc. Am. J. 1971, 35, 1010–1014. [Google Scholar] [CrossRef]
- Tetsuka, H.; Katayama, I.; Sakuma, H.; Tamura, K. Effects of humidity and interlayer cations on the frictional strength of montmorillonite. Earth Planets Space 2018, 70, 56. [Google Scholar] [CrossRef] [Green Version]
- Brunhoeber, O.M.; Arakkal, D.; Ji, R.; Miletić, M.; Beckingham, L.E. Impact of mineral composition and distribution on the mechanical properties of porous media. E3S Web Conf. 2020, 205, 02006. [Google Scholar] [CrossRef]
- Horabik, J.; Jozefaciuk, G. Structure and strength of kaolinite–soil silt aggregates: Measurements and modeling. Geoderma 2021, 382, 114687. [Google Scholar] [CrossRef]
- Munkholm, L.J.; Heck, R.J.; Deen, B.; Zidar, T. Relationship between soil aggregate strength, shape and porosity for soils under different long-term management. Geoderma 2016, 268, 52–59. [Google Scholar] [CrossRef]
- Yusof, N.; Zabidi, H. Correlation of Mineralogical and Textural Characteristics with Engineering Properties of Granitic Rock from Hulu Langat, Selangor. Procedia Chem. 2016, 19, 975–980. [Google Scholar] [CrossRef]
- Wang, Y.-H.; Siu, W.-K. Structure characteristics and mechanical properties of kaolinite soils. II. Effects of structure on mechanical properties. Can. Geotech. J. 2006, 43, 601–617. [Google Scholar] [CrossRef]
- Wei, S.; Tan, W.; Zhao, W.; Yu, Y.; Liu, F.; Koopal, L.K. Microstructure, Interaction Mechanisms, and Stability of Binary Systems Containing Goethite and Kaolinite. Soil Sci. Soc. Am. J. 2012, 76, 389–398. [Google Scholar] [CrossRef]
- Mair, K.; Frye, K.M.; Marone, C. Influence of grain characteristics on the friction of granular shear zones. J. Geophys. Res. Space Phys. 2002, 107, ECV-4. [Google Scholar] [CrossRef]
- Cho, G.-C.; Dodds, J.; Santamarina, J.C. Particle Shape Effects on Packing Density, Stiffness, and Strength: Natural and Crushed Sands. J. Geotech. Geoenviron. Eng. 2006, 132, 591–602. [Google Scholar] [CrossRef] [Green Version]
- Mesri, G.; Vardhanabhuti, B. Compression of granular materials. Can. Geotech. J. 2009, 46, 369–392. [Google Scholar] [CrossRef]
- Miller, N.A.; Henderson, J.J. Correlating Particle Shape Parameters to Bulk Properties and Load Stress at Two Water Contents. Agron. J. 2011, 103, 1514–1523. [Google Scholar] [CrossRef]
- Li, Y. Effects of particle shape and size distribution on the shear strength behavior of composite soils. Bull. Int. Assoc. Eng. Geol. 2013, 72, 371–381. [Google Scholar] [CrossRef]
- Lipiec, J.; Turski, M.; Hajnos, M.; Świeboda, R. Pore structure, stability and water repellency of earthworm casts and natural aggregates in loess soil. Geoderma 2015, 243, 124–129. [Google Scholar] [CrossRef]
- Vasylij, I.; Gomonaj, V.I.; Golub, N.P.; Szekeresh, K.Y.; Leboda, R.; Skubiszewska-Zieba, J. Usability of Transcarpathian clinoptilolite for HgII, Cr III i NiII ions adsorption from water solutions. Ochr. Sr. 1998, 4, 3–6. [Google Scholar]
- Ryżak, M.; Bieganowski, A. Methodological aspects of determining soil particle-size distribution using the laser diffraction method. J. Plant Nutr. Soil Sci. 2011, 174, 624–633. [Google Scholar] [CrossRef]
- Wang, W.; Cong, J.; Deng, J.; Weng, X.; Lin, Y.; Huang, Y.; Peng, T. Developing Effective Separation of Feldspar and Quartz While Recycling Tailwater by HF Pretreatment. Minerals 2018, 8, 149. [Google Scholar] [CrossRef] [Green Version]
- Brunauer, S.; Emmett, P.H.; Teller, E. Adsorption of Gases in Multimolecular Layers. J. Am. Chem. Soc. 1938, 60, 309–319. [Google Scholar] [CrossRef]
- Avnir, D.; Farin, D.; Pfeifer, P. Surface geometric irregularity of particulate materials: The fractal approach. J. Colloid Interface Sci. 1985, 103, 112–123. [Google Scholar] [CrossRef]
- Washburn, E.W. The Dynamics of Capillary Flow. Phys. Rev. 1921, 17, 273–283. [Google Scholar] [CrossRef]
- Sridharan, A.; Venkatappa Rao, G. Pore size distribution of soils from mercury intrusion porosimetry data. Soil Sci. Soc. Am. Proc. 1972, 36, 980–981. [Google Scholar] [CrossRef]
- Bartoli, F.; Bird, N.R.A.; Gomendy, V.; Vivier, H.; Niquet, S. The relation between silty soil structures and their mercury porosimetry curve counterparts: Fractals and percolation. Eur. J. Soil Sci. 1999, 50, 9–22. [Google Scholar] [CrossRef]
- Jozefaciuk, G. Effect of the size of aggregates on pore characteristics of minerals measured by mercury intrusion and water-vapor desorption techniques. Clays Clay Miner. 2009, 57, 586–601. [Google Scholar] [CrossRef]
- Pachepsky, Y.A.; Polubesova, T.A.; Hajnos, M.; Sokolowska, Z.; Józefaciuk, G. Fractal Parameters of Pore Surface Area as Influenced by Simulated Soil Degradation. Soil Sci. Soc. Am. J. 1995, 59, 68–75. [Google Scholar] [CrossRef]
- Yokoya, N.; Yamamoto, K.; Funakubo, N. Fractal-based analysis and interpolation of 3D natural surface shapes and their application to terrain modeling. Comput. Vision Graph. Image Process. 1989, 46, 284–302. [Google Scholar] [CrossRef]
- Vdović, N.; Jurina, I.; Škapin, S.D.; Sondi, I. The surface properties of clay minerals modified by intensive dry milling—revisited. Appl. Clay Sci. 2010, 48, 575–580. [Google Scholar] [CrossRef] [Green Version]
- Yates, D.J.C. Studies on the surface area of zeolites, as determined by physical adsorption and X-ray crystallography. Can. J. Chem. 1968, 46, 1695–1701. [Google Scholar] [CrossRef]
- Franus, W.; Jozefaciuk, G.; Bandura, L.; Franus, M. Use of Spent Zeolite Sorbents for the Preparation of Lightweight Aggregates Differing in Microstructure. Minerals 2017, 7, 25. [Google Scholar] [CrossRef] [Green Version]
- Jozefaciuk, G.; Bowanko, G. Effect of acid and alkali treatments on surface areas and adsorption energies of selected minerals. Clays Clay Miner. 2002, 50, 771–783. [Google Scholar] [CrossRef]
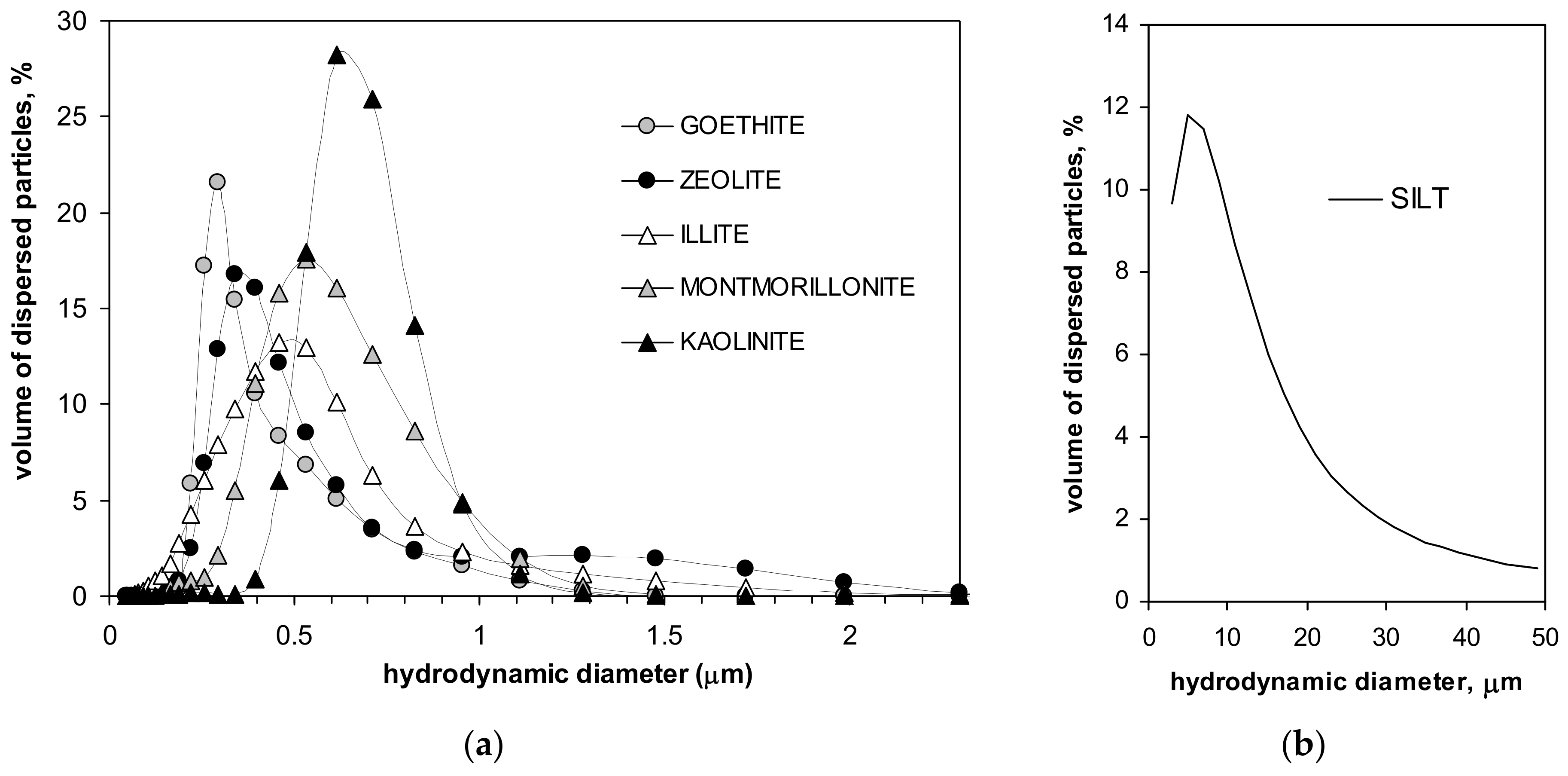
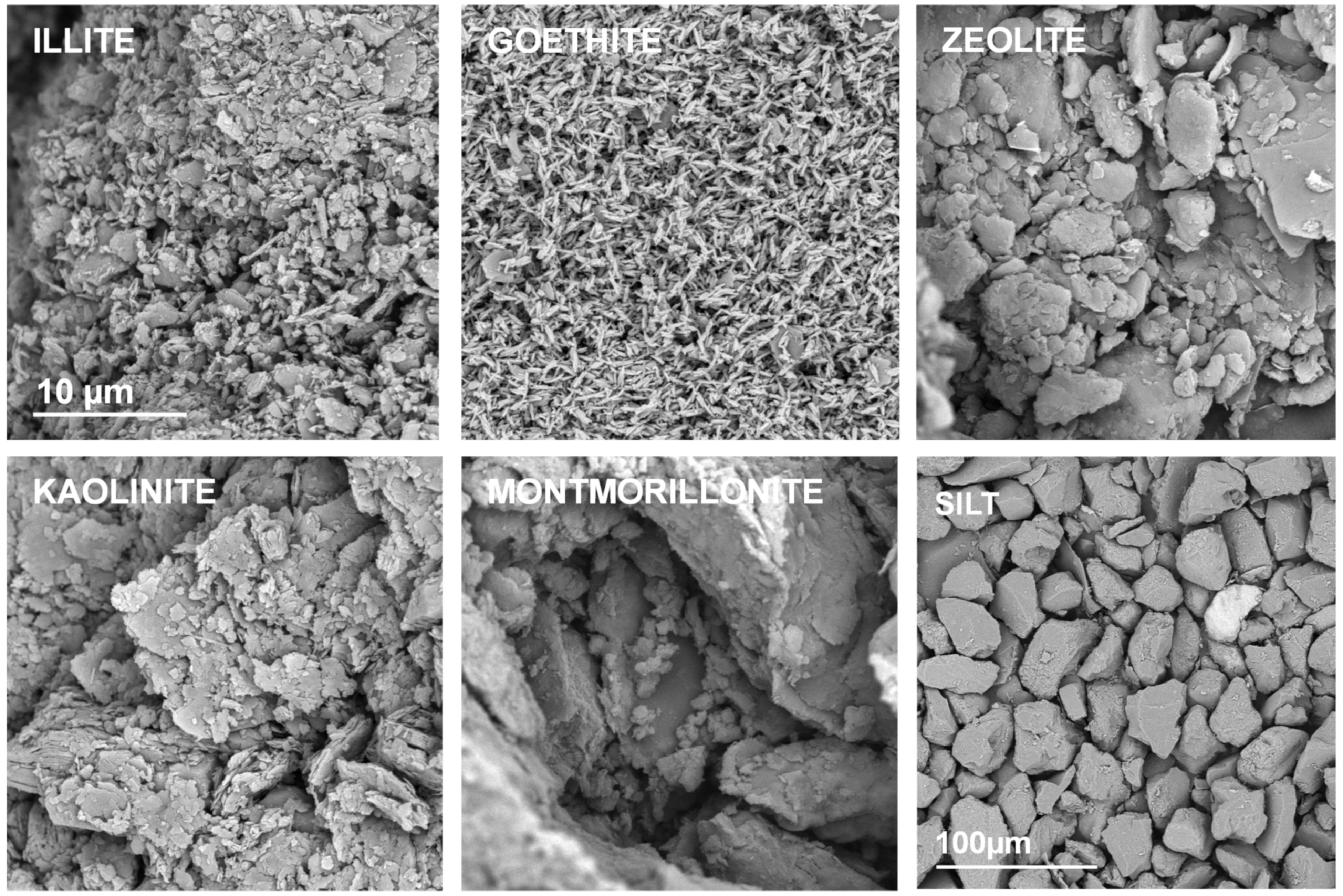
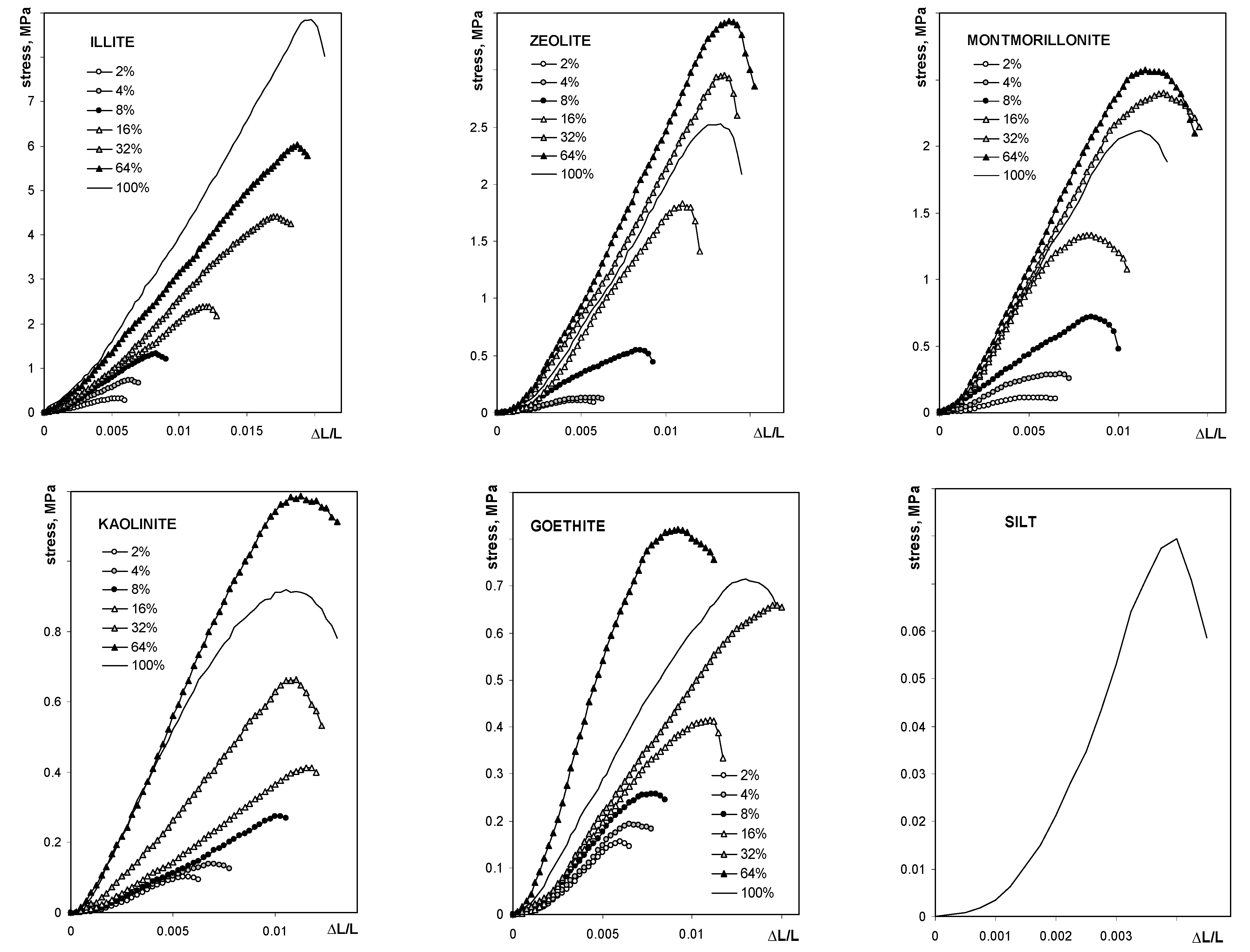
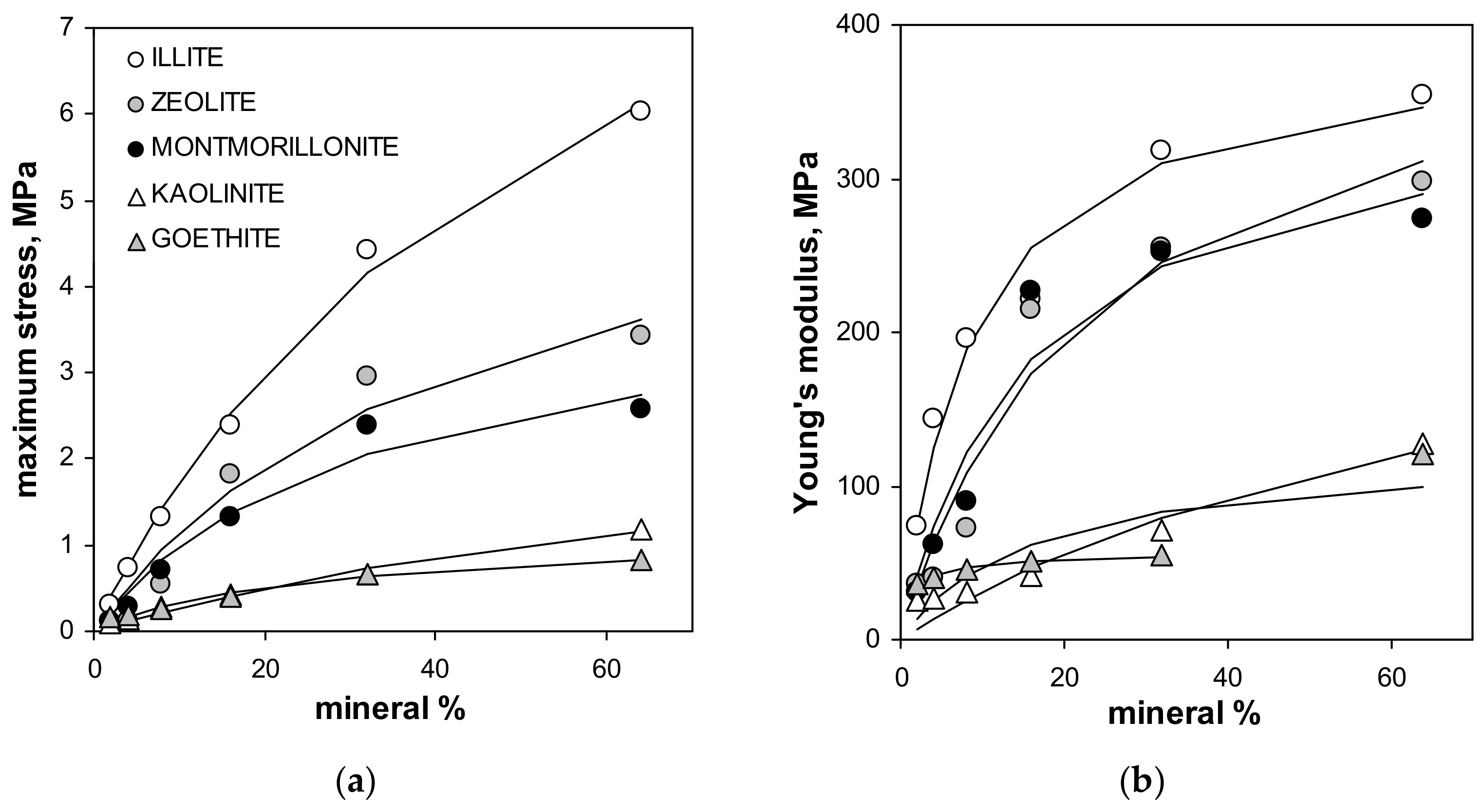



| Silt | Kaolinite | Montmorillonite | Zeolite | Illite | Goethite | Max SD% | Min SD% | ||
|---|---|---|---|---|---|---|---|---|---|
| SN2 | m2·g−1 | 1.7 | 14.4 | 225.2 | 26.1 | 145.5 | 12.5 | 5.9 S | 0.62 G |
| dparticle | µm | 15.0 | 0.66 | 0.58 | 0.51 | 0.49 | 0.39 | 44.4 K | 2.56 G |
| ζ | mV | −45 | −35.4 | −51.5 | −45.5 | −23.2 | 12.1 | 10.7 G | 1.35 M |
| VporeN2 | mm3·g−1 | 0.7 | 6.6 | 119 | 16.5 | 52.5 | 40.5 | 4.9 I | 0.18 M |
| dporeN2 | nm | 5.79 | 5.94 | 6.22 | 2.61 | 6.24 | 4.98 | 5.1 Z | 0.39 M |
| SPD | g cm−3 | 2.70 | 2.62 | 2.52 | 2.33 | 2.74 | 4.11 | 0.18 G | 0.03 S |
| DfracN2 | - | 2.25 | 2.55 | 2.58 | 2.62 | 2.70 | 2.63 | 1.6 S | 0.7 K |
| P | % | 0.2 | 1.7 | 23.1 | 3.7 | 12.6 | 14.3 | - | - |
| Q | % | 0.04 | 0.9 | 1.07 | 0.51 | 1.27 | 1.28 | - | - |
| Data | Constant * | Kaolinite | Monmorillonite | Zeolite | Illite | Goethite |
|---|---|---|---|---|---|---|
| σmax | Cσ, MPa | 3.0 | 4.1 | 6.2 | 11.6 | 1.1 |
| kσ | 0.010 | 0.031 | 0.022 | 0.017 | 0.042 | |
| E | CE, MPa | 272 | 362 | 424 | 393 | 55 |
| kE | 0.013 | 0.064 | 0.043 | 0.116 | 0.771 |
| Material | MIN % | σmax MPa | E MPa | BD gcm−3 | VMIP,max cm3g−1 | V(BD) cm3g−1 | Via cm3g−1 | RMIPav µm | DfracMIP – | PT µm | Ria µm |
|---|---|---|---|---|---|---|---|---|---|---|---|
| Silt | 0.08 | 28.5 | 1.377 | 0.321 | 0.356 | 0.213 | 4.380 | 2.24 | 4.772 | 3.64 | |
| Kaolinite | 2 | 0.10 | 26 | 1.389 | 0.317 | 0.350 | 0.167 | 3.956 | 3.07 | 4.336 | 2.79 |
| 4 | 0.14 | 26.6 | 1.425 | 0.288 | 0.331 | 0.146 | 3.303 | 3.22 | 4.336 | 2.50 | |
| 8 | 0.27 | 30.7 | 1.465 | 0.252 | 0.312 | 0.207 | 2.252 | 3.51 | 3.633 | 1.59 | |
| 16 | 0.41 | 41.7 | 1.521 | 0.251 | 0.286 | 0.196 | 1.004 | 3.99 | 1.966 | 0.73 | |
| 32 | 0.66 | 70.9 | 1.700 | 0.217 | 0.215 | 0.175 | 0.248 | 3.33 | 0.444 | 0.18 | |
| 64 | 1.19 | 127 | 1.676 | 0.198 | 0.219 | 0.119 | 0.065 | 4.54 | 0.054 | 0.03 | |
| 100 | 0.92 | 110.6 | 1.533 | 0.268 | 0.271 | 0.139 | 0.061 | 4.68 | 0.054 | 0.03 | |
| Illite | 2 | 0.32 | 73.7 | 1.406 | 0.320 | 0.341 | 0.185 | 4.036 | 2.87 | 4.336 | 2.97 |
| 4 | 0.72 | 143 | 1.410 | 0.297 | 0.339 | 0.206 | 3.888 | 3.15 | 4.336 | 3.10 | |
| 8 | 1.33 | 195.7 | 1.487 | 0.270 | 0.303 | 0.114 | 2.280 | 3.41 | 2.176 | 1.22 | |
| 16 | 2.39 | 221.5 | 1.578 | 0.222 | 0.265 | 0.116 | 1.581 | 3.71 | 2.176 | 0.76 | |
| 32 | 4.42 | 318 | 1.715 | 0.191 | 0.215 | 0.160 | 0.594 | 4.04 | 1.777 | 0.43 | |
| 64 | 6.03 | 354 | 1.881 | 0.140 | 0.165 | 0.108 | 0.095 | 4.20 | 0.657 | 0.03 | |
| 100 | 8.86 | 510 | 1.860 | 0.138 | 0.173 | 0.056 | 0.013 | 4.51 | 0.003 | 0.00 | |
| Montmorillonite | 2 | 0.11 | 31.1 | 1.389 | 0.307 | 0.349 | 0.209 | 3.756 | 2.55 | 4.336 | 2.97 |
| 4 | 0.29 | 61.4 | 1.381 | 0.325 | 0.353 | 0.189 | 3.567 | 2.55 | 4.336 | 2.41 | |
| 8 | 0.72 | 90.2 | 1.434 | 0.304 | 0.325 | 0.205 | 2.449 | 2.37 | 3.633 | 1.70 | |
| 16 | 1.33 | 226.4 | 1.545 | 0.251 | 0.273 | 0.151 | 0.521 | 2.38 | 0.801 | 0.26 | |
| 32 | 2.40 | 251.7 | 1.536 | 0.254 | 0.272 | 0.162 | 0.219 | 3.38 | 0.540 | 0.10 | |
| 64 | 2.57 | 273.9 | 1.374 | 0.318 | 0.341 | 0.245 | 0.111 | 3.42 | 0.249 | 0.05 | |
| 100 | 2.11 | 236.2 | 1.016 | 0.503 | 0.512 | 0.362 | 0.066 | 3.43 | 0.205 | 0.05 | |
| Zeolite | 2 | 0.11 | 36.1 | 1.377 | 0.332 | 0.355 | 0.178 | 4.241 | 2.56 | 4.336 | 3.18 |
| 4 | 0.13 | 40.1 | 1.385 | 0.331 | 0.349 | 0.206 | 3.531 | 2.86 | 4.336 | 2.55 | |
| 8 | 0.55 | 72.3 | 1.431 | 0.325 | 0.324 | 0.265 | 2.608 | 3.16 | 4.336 | 2.16 | |
| 16 | 1.83 | 214.7 | 1.563 | 0.228 | 0.260 | 0.168 | 0.575 | 4.14 | 2.176 | 0.27 | |
| 32 | 2.96 | 255 | 1.726 | 0.177 | 0.190 | 0.097 | 0.089 | 4.16 | 0.079 | 0.05 | |
| 64 | 3.43 | 298.3 | 1.465 | 0.270 | 0.275 | 0.177 | 0.083 | 4.18 | 0.079 | 0.05 | |
| 100 | 2.53 | 247.9 | 1.096 | 0.472 | 0.483 | 0.361 | 0.070 | 4.66 | 0.079 | 0.05 | |
| Goethite | 2 | 0.15 | 35.6 | 1.424 | 0.301 | 0.335 | 0.204 | 3.324 | 3.09 | 4.336 | 2.24 |
| 4 | 0.19 | 40 | 1.457 | 0.303 | 0.321 | 0.179 | 3.006 | 2.97 | 3.939 | 1.81 | |
| 8 | 0.26 | 45.3 | 1.519 | 0.255 | 0.298 | 0.153 | 2.054 | 3.16 | 3.633 | 0.92 | |
| 16 | 0.41 | 51.6 | 1.629 | 0.244 | 0.264 | 0.186 | 0.705 | 2.87 | 2.176 | 0.37 | |
| 32 | 0.66 | 54.7 | 1.888 | 0.189 | 0.200 | 0.150 | 0.138 | 3.78 | 0.249 | 0.08 | |
| 64 | 0.82 | 121 | 1.717 | 0.289 | 0.293 | 0.148 | 0.093 | 3.84 | 0.071 | 0.06 | |
| 100 | 0.72 | 66.6 | 1.403 | 0.456 | 0.469 | 0.261 | 0.097 | 3.87 | 0.079 | 0.06 | |
| Max SD% | - | 51.3 | 33.3 | 0.7 | 5.3 | - | 13.3 | 6.6 | 5.4 | 10.0 | 5.1 |
| - | G4 | G4 | S | G4 | - | G2 | Z2 | S | G2 | S | |
| Min SD% | - | 12.1 | 3.4 | 0.1 | 2.1 | - | 4.6 | 2.9 | 2.0 | 4.3 | 1.8 |
| - | I100 | M64 | I100 | I100 | - | Z64 | M64 | Z64 | M64 | I64 |
| Parameter | SN2 | dparticle | ζ | VporeN2 | dporeN2 | DfracN2 | P | Q |
|---|---|---|---|---|---|---|---|---|
| Cσ | - | −0.7 | - | - | - | +0.99 | - | - |
| kσ | 0.53 | - | −0.51 | +0.69 | - | - | +0.64 | - |
| CE | - | −0.89 | - | - | - | - | - | - |
| kE | - | −0.59 | - | - | - | +0.80 | - | - |
| σmax | - | −0.55 | +0.56 | - | - | +0.93 | - | - |
| E | - | −0.69 | - | - | - | +0.95 | - | - |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jozefaciuk, G.; Skic, K.; Adamczuk, A.; Boguta, P.; Lamorski, K. Structure and Strength of Artificial Soils Containing Monomineral Clay Fractions. Materials 2021, 14, 4688. https://doi.org/10.3390/ma14164688
Jozefaciuk G, Skic K, Adamczuk A, Boguta P, Lamorski K. Structure and Strength of Artificial Soils Containing Monomineral Clay Fractions. Materials. 2021; 14(16):4688. https://doi.org/10.3390/ma14164688
Chicago/Turabian StyleJozefaciuk, Grzegorz, Kamil Skic, Agnieszka Adamczuk, Patrycja Boguta, and Krzysztof Lamorski. 2021. "Structure and Strength of Artificial Soils Containing Monomineral Clay Fractions" Materials 14, no. 16: 4688. https://doi.org/10.3390/ma14164688
APA StyleJozefaciuk, G., Skic, K., Adamczuk, A., Boguta, P., & Lamorski, K. (2021). Structure and Strength of Artificial Soils Containing Monomineral Clay Fractions. Materials, 14(16), 4688. https://doi.org/10.3390/ma14164688







