Nonlocality-Enabled Magnetic Free Optical Isolation in Hyperbolic Metamaterials
Abstract
1. Introduction
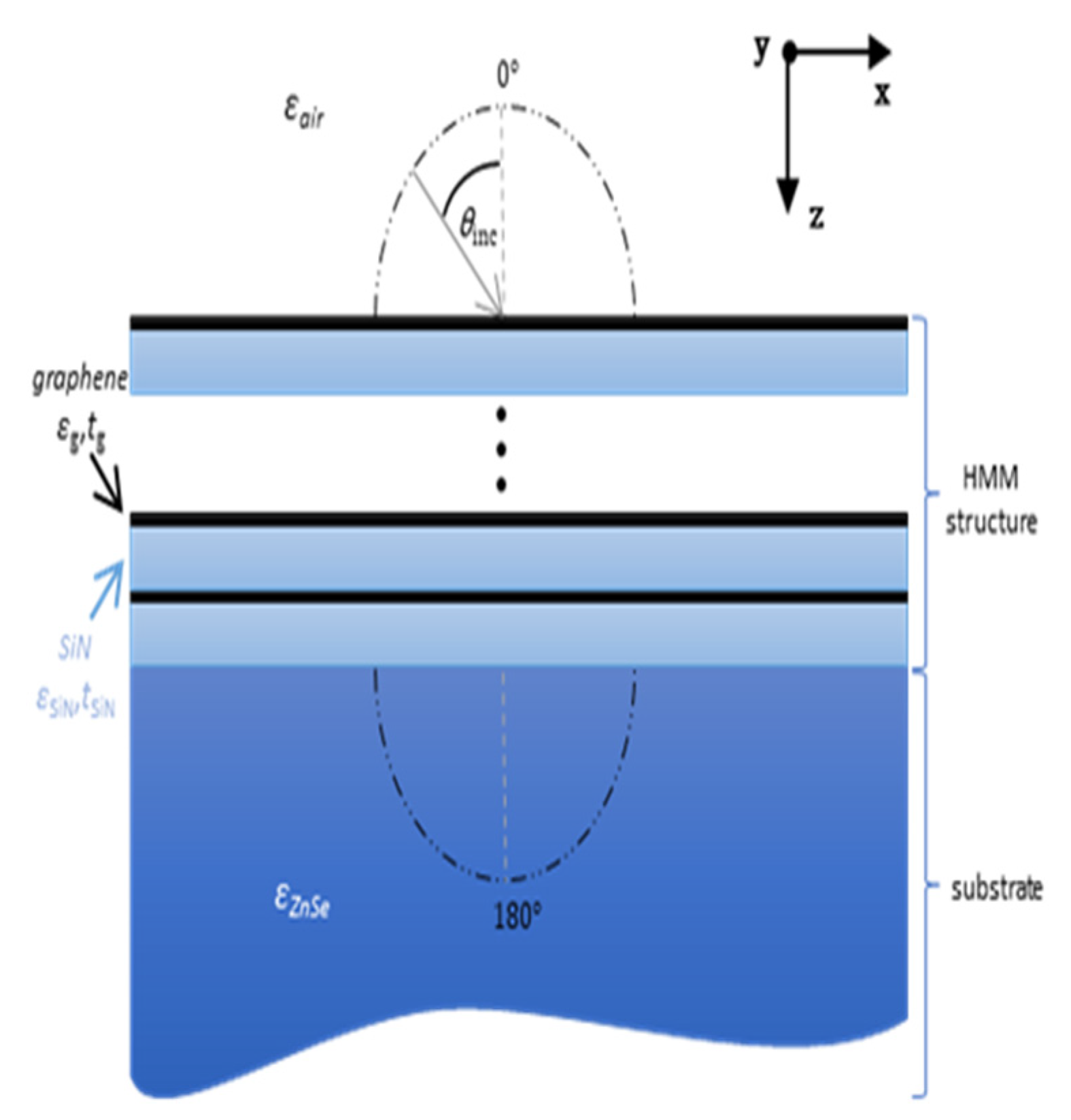
2. Theory
2.1. Effective Medium Theory
2.2. Transfer Matrix Method
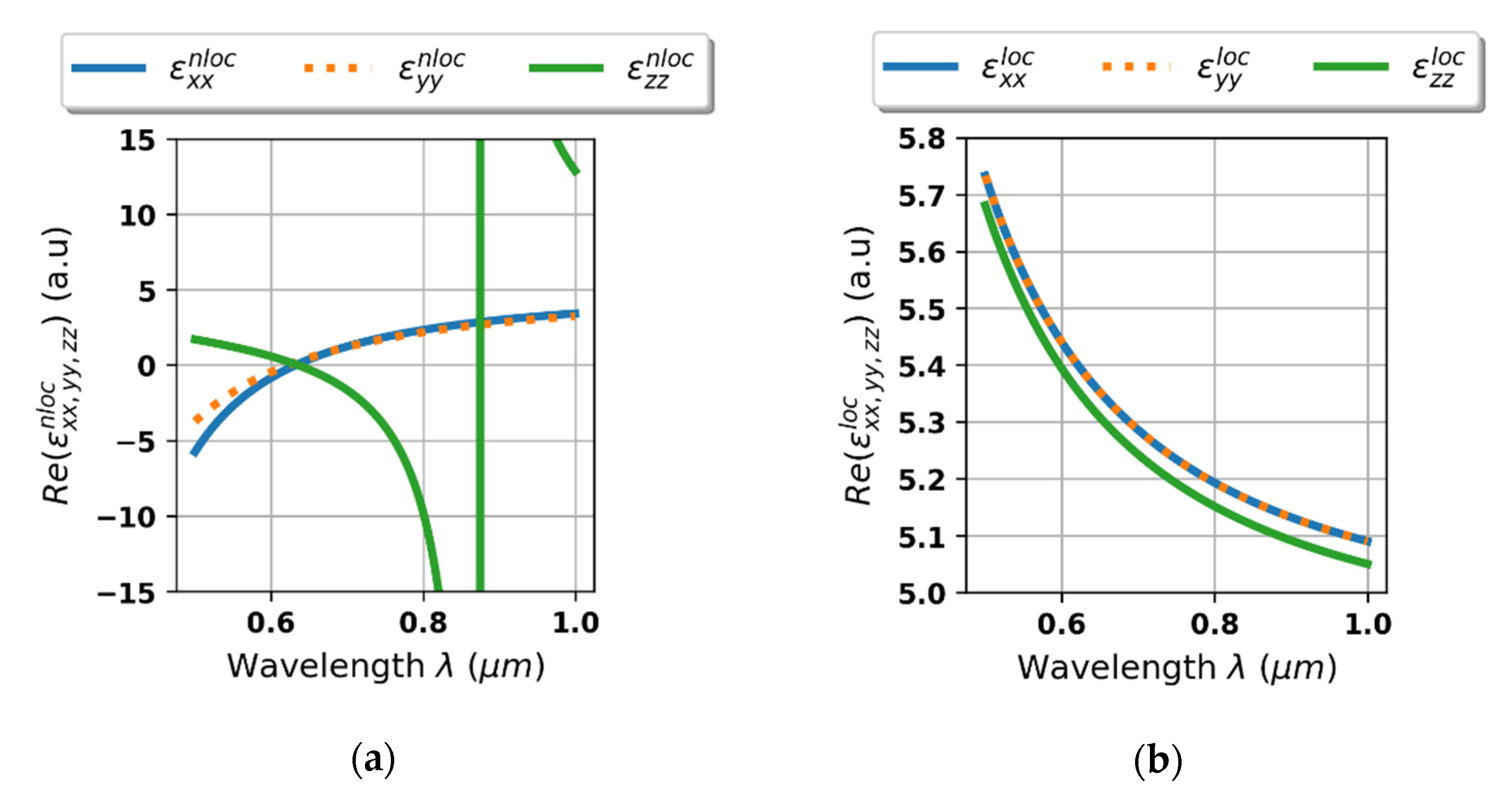
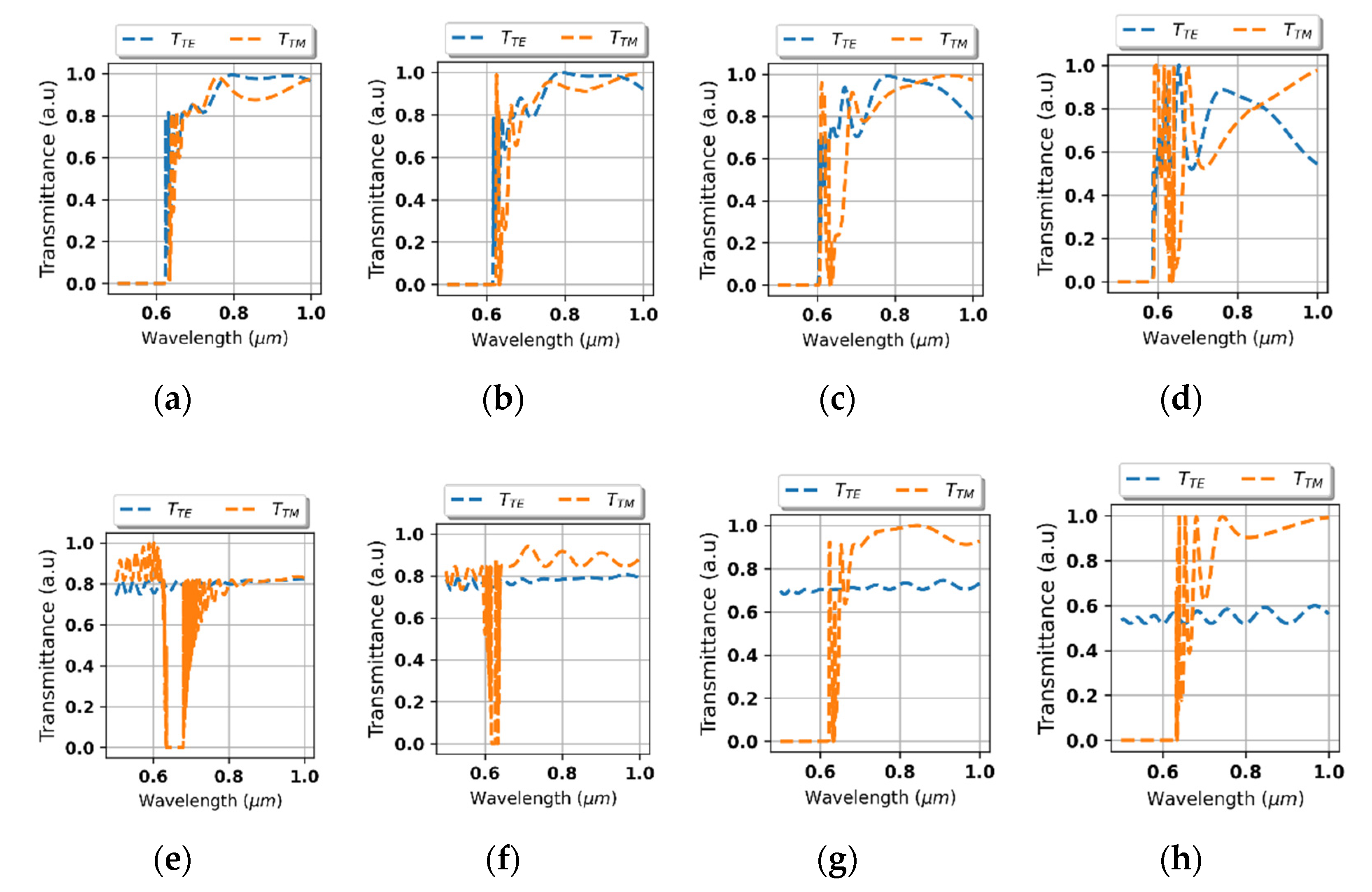
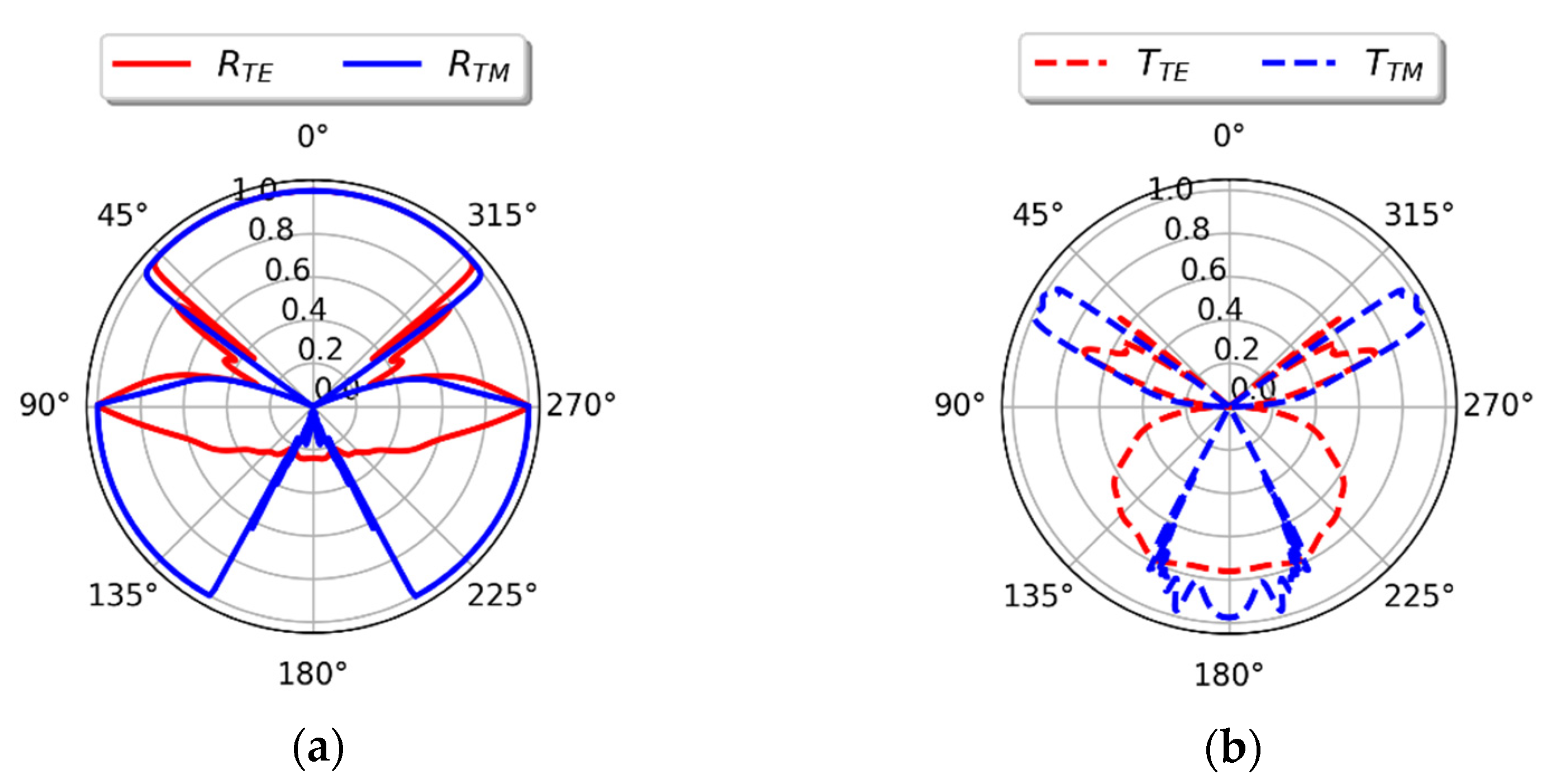
3. Results
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Jalas, D.; Petrov, A.; Eich, M.; Freude, W.; Fan, S.; Yu, Z.; Baets, R.; Popović, M.; Melloni, A.; Joannopoulos, J.D.; et al. What Is—And What Is Not—An Optical Isolator. Nat. Photonics 2013, 7, 579–582. [Google Scholar] [CrossRef]
- Aplet, L.J.; Carson, J.W. A Faraday Effect Optical Isolator. Appl. Opt. 1964, 3, 544. [Google Scholar] [CrossRef]
- Doerr, C.R.; Dupuis, N.; Zhang, L. Optical Isolator Using Two Tandem Phase Modulators. Opt. Lett. 2011, 36, 4293. [Google Scholar] [CrossRef]
- Sobu, Y.; Shoji, Y.; Sakurai, K.; Mizumoto, T. GaInAsP/InP MZI Waveguide Optical Isolator Integrated with Spot Size Converter. Opt. Express 2013, 21, 15373. [Google Scholar] [CrossRef]
- Binandeh Dehaghani, N.; Alizadeh, A.H. Design, Simulation and Optimization of a Nonreciprocal Guided-Wave Optical Isolator Based on Mach-Zehnder Interferometer. J. Phys. Commun. 2019, 3, 125006. [Google Scholar] [CrossRef]
- Yokoi, H.; Mizumoto, T.; Shinjo, N.; Futakuchi, N.; Nakano, Y. Demonstration of an Optical Isolator with a Semiconductor Guiding Layer That Was Obtained by Use of a Nonreciprocal Phase Shift. Appl. Opt. 2000, 39, 6158. [Google Scholar] [CrossRef]
- Zaets, W.; Ando, K. Optical Waveguide Isolator Based on Nonreciprocal Loss/Gain of Amplifier Covered by Ferromagnetic Layer. IEEE Photonics Technol. Lett. 1999, 11, 1012–1014. [Google Scholar] [CrossRef]
- Shoji, Y.; Mizumoto, T.; Yokoi, H.; Hsieh, I.-W.; Osgood, R.M. Magneto-Optical Isolator with Silicon Waveguides Fabricated by Direct Bonding. Appl. Phys. Lett. 2008, 92, 071117. [Google Scholar] [CrossRef]
- Fujita, J.; Levy, M.; Osgood, R.M.; Wilkens, L.; Dötsch, H. Waveguide Optical Isolator Based on Mach–Zehnder Interferometer. Appl. Phys. Lett. 2000, 76, 2158–2160. [Google Scholar] [CrossRef]
- Zhang, Y.; Du, Q.; Wang, C.; Fakhrul, T.; Liu, S.; Deng, L.; Huang, D.; Pintus, P.; Bowers, J.; Ross, C.A.; et al. Monolithic Integration of Broadband Optical Isolators for Polarization-Diverse Silicon Photonics. Optica 2019, 6, 473. [Google Scholar] [CrossRef]
- Shiju, E.; Bharat, M.; Siji Narendran, N.K.; Narayana Rao, D.; Chandrasekharan, K. Optical Diode Activity in an Axially Asymmetric Nonlinear Medium Incorporated with Phenothiazine and Silver Nanoparticles. Opt. Mater. 2020, 99, 109557. [Google Scholar] [CrossRef]
- Gallo, K.; Assanto, G.; Parameswaran, K.R.; Fejer, M.M. All-Optical Diode in a Periodically Poled Lithium Niobate Waveguide. Appl. Phys. Lett. 2001, 79, 314–316. [Google Scholar] [CrossRef]
- Rangelov, A.; Longhi, S. Nonlinear Adiabatic Optical Isolator. Appl. Opt. 2017, 56, 2991. [Google Scholar] [CrossRef]
- Hua, S.; Wen, J.; Jiang, X.; Hua, Q.; Jiang, L.; Xiao, M. Demonstration of a Chip-Based Optical Isolator with Parametric Amplification. Nat. Commun. 2016, 7, 13657. [Google Scholar] [CrossRef] [PubMed]
- Zhou, X.; Wang, Y.; Leykam, D.; Chong, Y.D. Optical Isolation with Nonlinear Topological Photonics. New J. Phys. 2017, 19, 095002. [Google Scholar] [CrossRef]
- Kato, H.; Inoue, M. Reflection-Mode Operation of One-Dimensional Magnetophotonic Crystals for Use in Film-Based Magneto-Optical Isolator Devices. J. Appl. Phys. 2002, 91, 7017. [Google Scholar] [CrossRef]
- Fang, K.; Yu, Z.; Liu, V.; Fan, S. Ultracompact Nonreciprocal Optical Isolator Based on Guided Resonance in a Magneto-Optical Photonic Crystal Slab. Opt. Lett. 2011, 36, 4254. [Google Scholar] [CrossRef] [PubMed]
- Xia, C.-C.; Yan, X.-B.; Tian, X.-D.; Gao, F. Ideal Optical Isolator with a Two-Cavity Optomechanical System. Opt. Commun. 2019, 451, 197–201. [Google Scholar] [CrossRef]
- Zhou, X.; Chong, Y.D. PT Symmetry Breaking and Nonlinear Optical Isolation in Coupled Microcavities. Opt. Express 2016, 24, 6916. [Google Scholar] [CrossRef]
- Chang, L.; Jiang, X.; Hua, S.; Yang, C.; Wen, J.; Jiang, L.; Li, G.; Wang, G.; Xiao, M. Parity–Time Symmetry and Variable Optical Isolation in Active–Passive-Coupled Microresonators. Nat. Photonics 2014, 8, 524–529. [Google Scholar] [CrossRef]
- Shitrit, N.; Kim, J.; Barth, D.S.; Ramezani, H.; Wang, Y.; Zhang, X. Asymmetric Free-Space Light Transport at Nonlinear Metasurfaces. Phys. Rev. Lett. 2018, 121, 046101. [Google Scholar] [CrossRef]
- Chen, S.; Fan, F.; Wang, X.; Wu, P.; Zhang, H.; Chang, S. Terahertz Isolator Based on Nonreciprocal Magneto-Metasurface. Opt. Express 2015, 23, 1015. [Google Scholar] [CrossRef]
- Shen, B.; Polson, R.; Menon, R. Broadband Asymmetric Light Transmission via All-Dielectric Digital Metasurfaces. Opt. Express 2015, 23, 20961. [Google Scholar] [CrossRef]
- Davoyan, A.R.; Mahmoud, A.M.; Engheta, N. Optical Isolation with Epsilon-near-Zero Metamaterials. Opt. Express 2013, 21, 3279. [Google Scholar] [CrossRef]
- Cao, H.; Yang, Z.; Zhao, M.; Wu, L.; Zhang, P. Broadband Optical Isolator Based on Helical Metamaterials. J. Opt. Soc. Am. A 2015, 32, 778. [Google Scholar] [CrossRef]
- Leviyev, A.; Stein, B.; Christofi, A.; Galfsky, T.; Krishnamoorthy, H.; Kuskovsky, I.L.; Menon, V.; Khanikaev, A.B. Nonreciprocity and One-Way Topological Transitions in Hyperbolic Metamaterials. APL Photonics 2017, 2, 076103. [Google Scholar] [CrossRef]
- Xu, T.; Lezec, H.J. Visible-Frequency Asymmetric Transmission Devices Incorporating a Hyperbolic Metamaterial. Nat. Commun. 2014, 5, 4141. [Google Scholar] [CrossRef] [PubMed]
- Janaszek, B.; Szczepański, P. Effect of Nonlocality in Spatially Uniform Anisotropic Metamaterials. Opt. Express 2020, 28, 15447. [Google Scholar] [CrossRef]
- Janaszek, B.; Kieliszczyk, M.; Tyszka-Zawadzka, A.; Szczepański, P. Multiresonance Response in Hyperbolic Metamaterials. Appl. Opt. 2018, 57, 2135. [Google Scholar] [CrossRef]
- Agranovich, V.M.; Ginzburg, V. Crystal Optics with Spatial Dispersion, and Excitons, 2nd ed.; Springer: Berlin/Heidelberg, Germany; New York, NY, USA, 1984; pp. 4, 9 and 27. ISBN 978-3-662-02406-5. [Google Scholar]
- Maier, S.A. Plasmonics: Fundamentals and Applications; Springer: New York, NY, USA, 2007; ISBN 978-0-387-33150-8. [Google Scholar]
- Chern, R.-L. Spatial Dispersion and Nonlocal Effective Permittivity for Periodic Layered Metamaterials. Opt. Express 2013, 21, 16514. [Google Scholar] [CrossRef]
- Janaszek, B.; Kieliszczyk, M.; Tyszka-Zawadzka, A.; Szczepański, P. Influence of Nonlocality on Transmittance and Reflectance of Hyperbolic Metamaterials. Crystals 2020, 10, 577. [Google Scholar] [CrossRef]
- Hanson, G.W. Dyadic Green’s Functions for an Anisotropic, Non-Local Model of Biased Graphene. IEEE Trans. Antennas Propag. 2008, 56, 747–757. [Google Scholar] [CrossRef]
- Luke, K.; Okawachi, Y.; Lamont, M.R.E.; Gaeta, A.L.; Lipson, M. Broadband Mid-Infrared Frequency Comb Generation in a Si_3N_4 Microresonator. Opt. Lett. 2015, 40, 4823. [Google Scholar] [CrossRef]
- Elser, J.; Podolskiy, V.A.; Salakhutdinov, I.; Avrutsky, I. Nonlocal Effects in Effective-Medium Response of Nanolayered Metamaterials. Appl. Phys. Lett. 2007, 90, 191109. [Google Scholar] [CrossRef]
- Amotchkina, T.; Trubetskov, M.; Hahner, D.; Pervak, V. Characterization of E-Beam Evaporated Ge, YbF 3, ZnS, and LaF 3 Thin Films for Laser-Oriented Coatings. Appl. Opt. 2020, 59, A40. [Google Scholar] [CrossRef] [PubMed]
- Katsidis, C.C.; Siapkas, D.I. General Transfer-Matrix Method for Optical Multilayer Systems with Coherent, Partially Coherent, and Incoherent Interference. Appl. Opt. 2002, 41, 3978. [Google Scholar] [CrossRef] [PubMed]
- Kieliszczyk, M.; Janaszek, B.; Tyszka-Zawadzka, A.; Szczepański, P. Tunable Spectral and Spatial Filters for the Mid-Infrared Based on Hyperbolic Metamaterials. Appl. Opt. 2018, 57, 1182. [Google Scholar] [CrossRef]





Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Janaszek, B.; Kieliszczyk, M.; Szczepański, P. Nonlocality-Enabled Magnetic Free Optical Isolation in Hyperbolic Metamaterials. Materials 2021, 14, 2865. https://doi.org/10.3390/ma14112865
Janaszek B, Kieliszczyk M, Szczepański P. Nonlocality-Enabled Magnetic Free Optical Isolation in Hyperbolic Metamaterials. Materials. 2021; 14(11):2865. https://doi.org/10.3390/ma14112865
Chicago/Turabian StyleJanaszek, Bartosz, Marcin Kieliszczyk, and Paweł Szczepański. 2021. "Nonlocality-Enabled Magnetic Free Optical Isolation in Hyperbolic Metamaterials" Materials 14, no. 11: 2865. https://doi.org/10.3390/ma14112865
APA StyleJanaszek, B., Kieliszczyk, M., & Szczepański, P. (2021). Nonlocality-Enabled Magnetic Free Optical Isolation in Hyperbolic Metamaterials. Materials, 14(11), 2865. https://doi.org/10.3390/ma14112865





